The Long-Delayed Response of a Cyclonic Ocean Eddy to the Passage of Typhoons Hinnamnor and Muifa
Abstract
1. Introduction
2. Materials and Methods
2.1. Methods
2.2. Data
3. Results
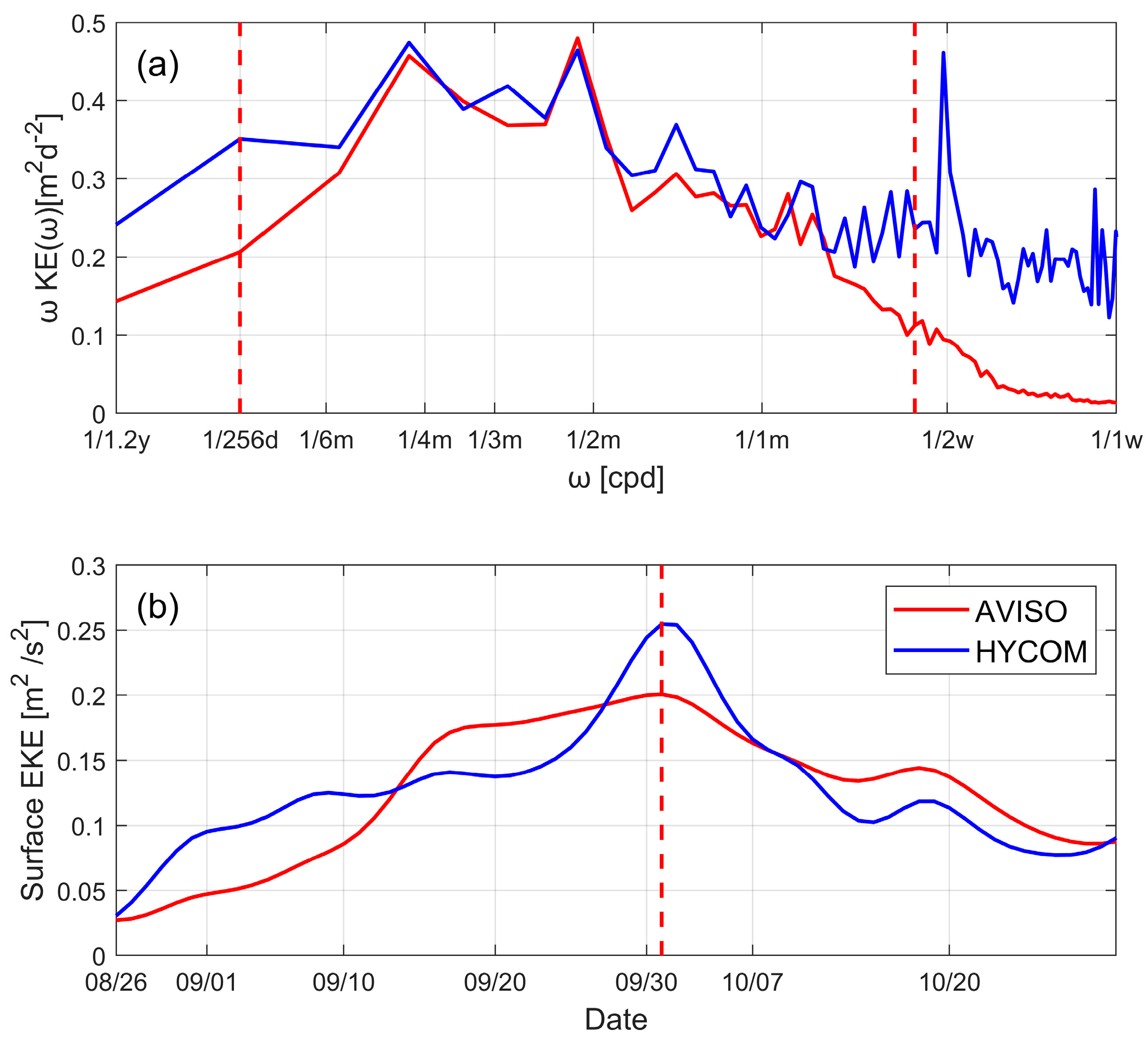
3.1. MS-EVA Setup and Multiscale Window Reconstruction
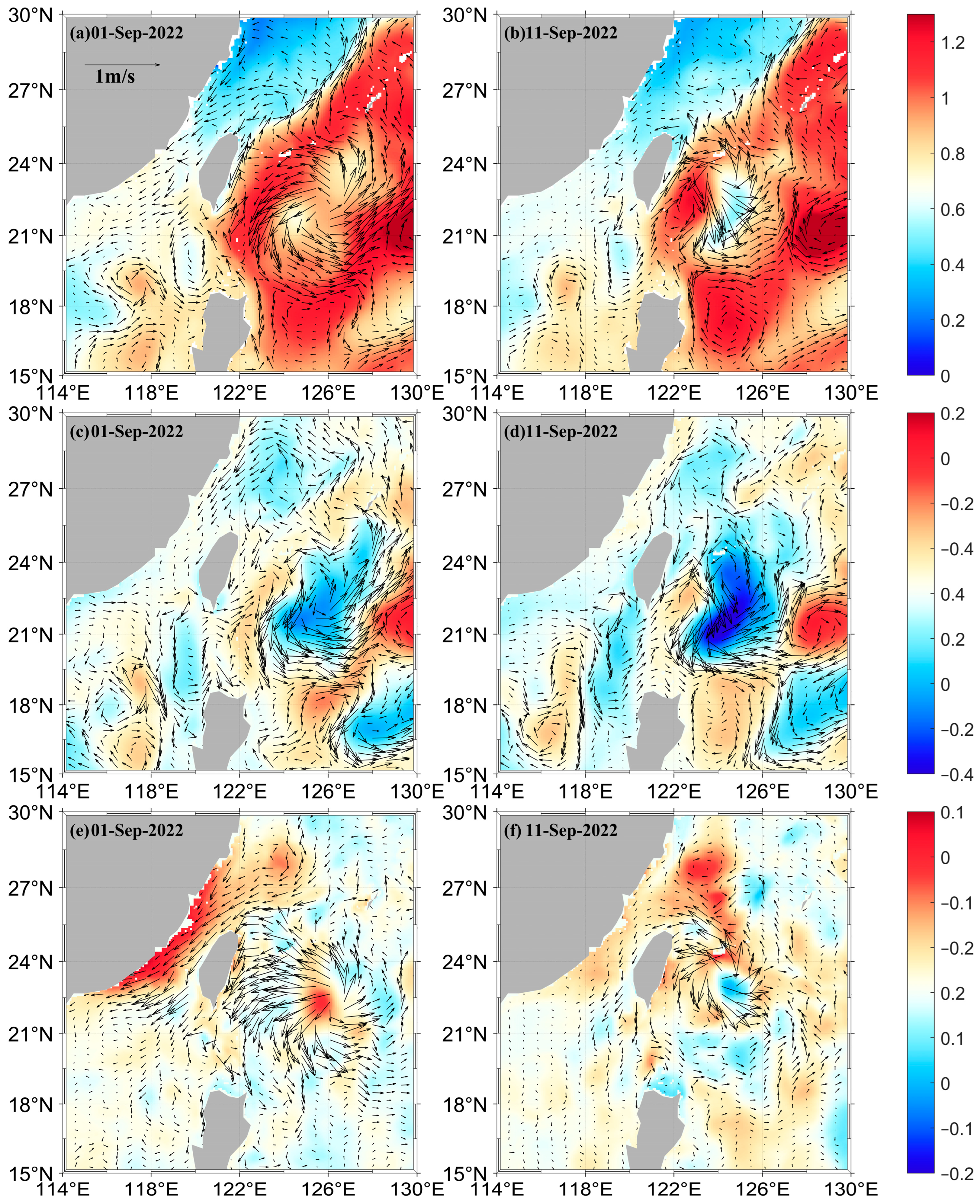
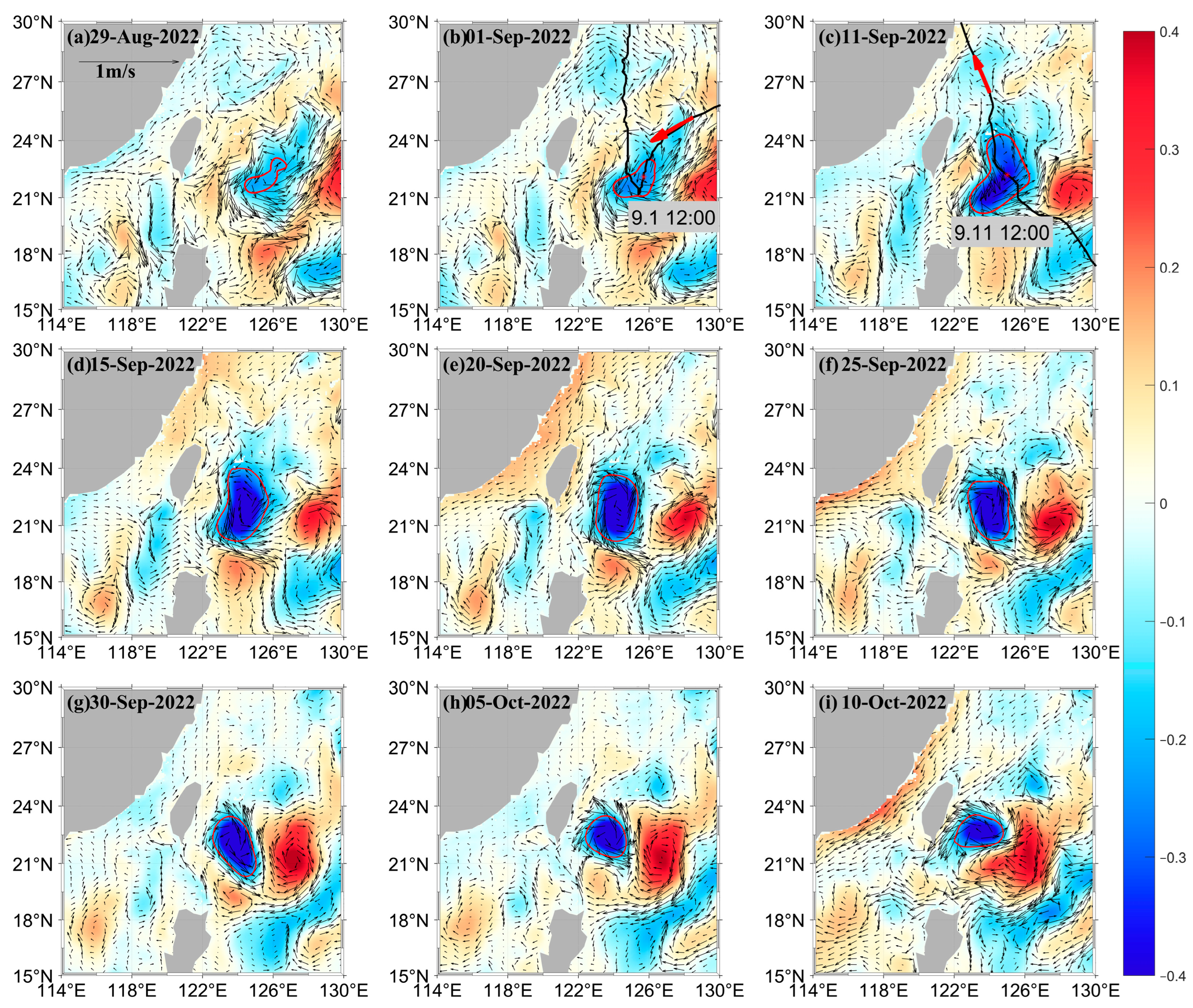
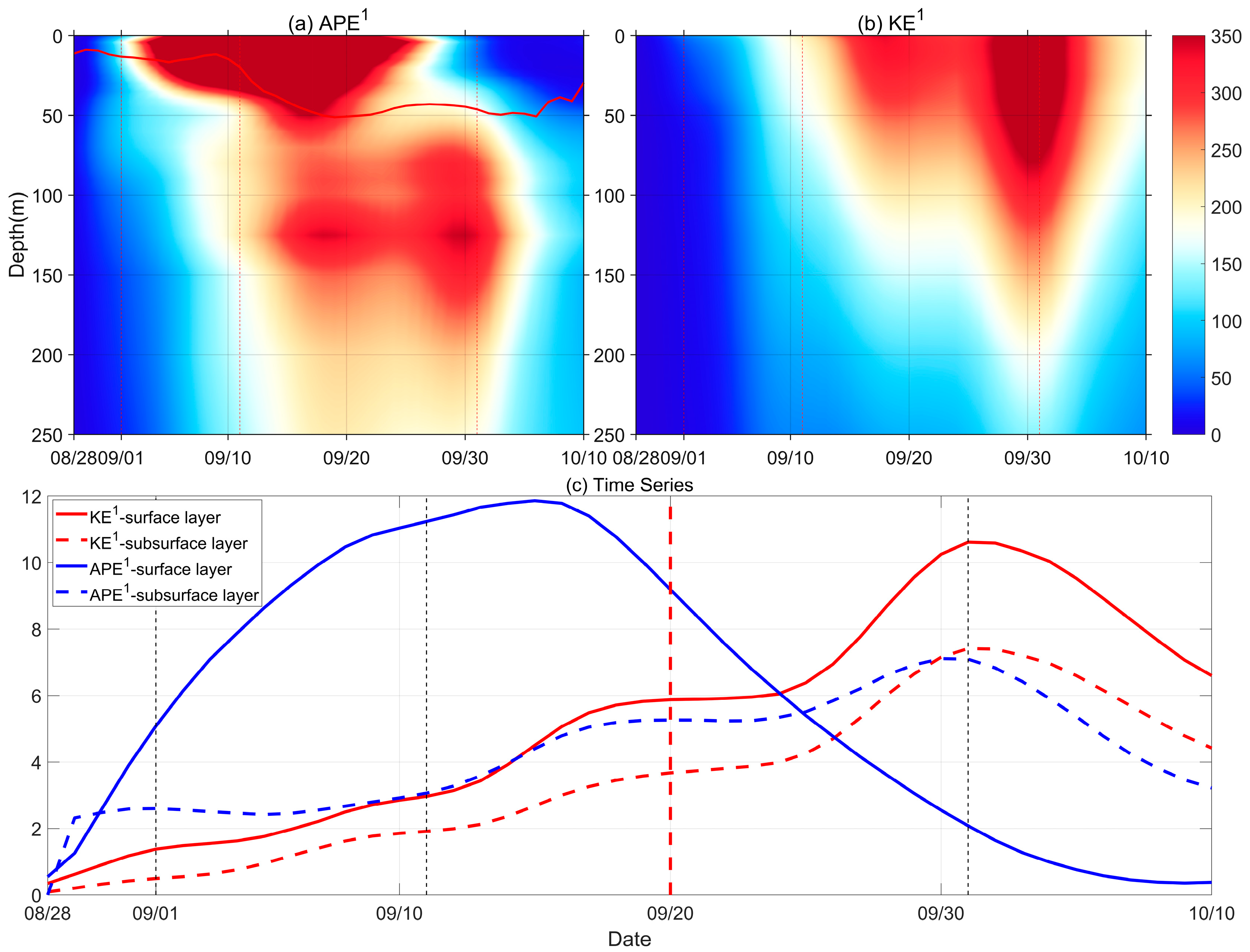
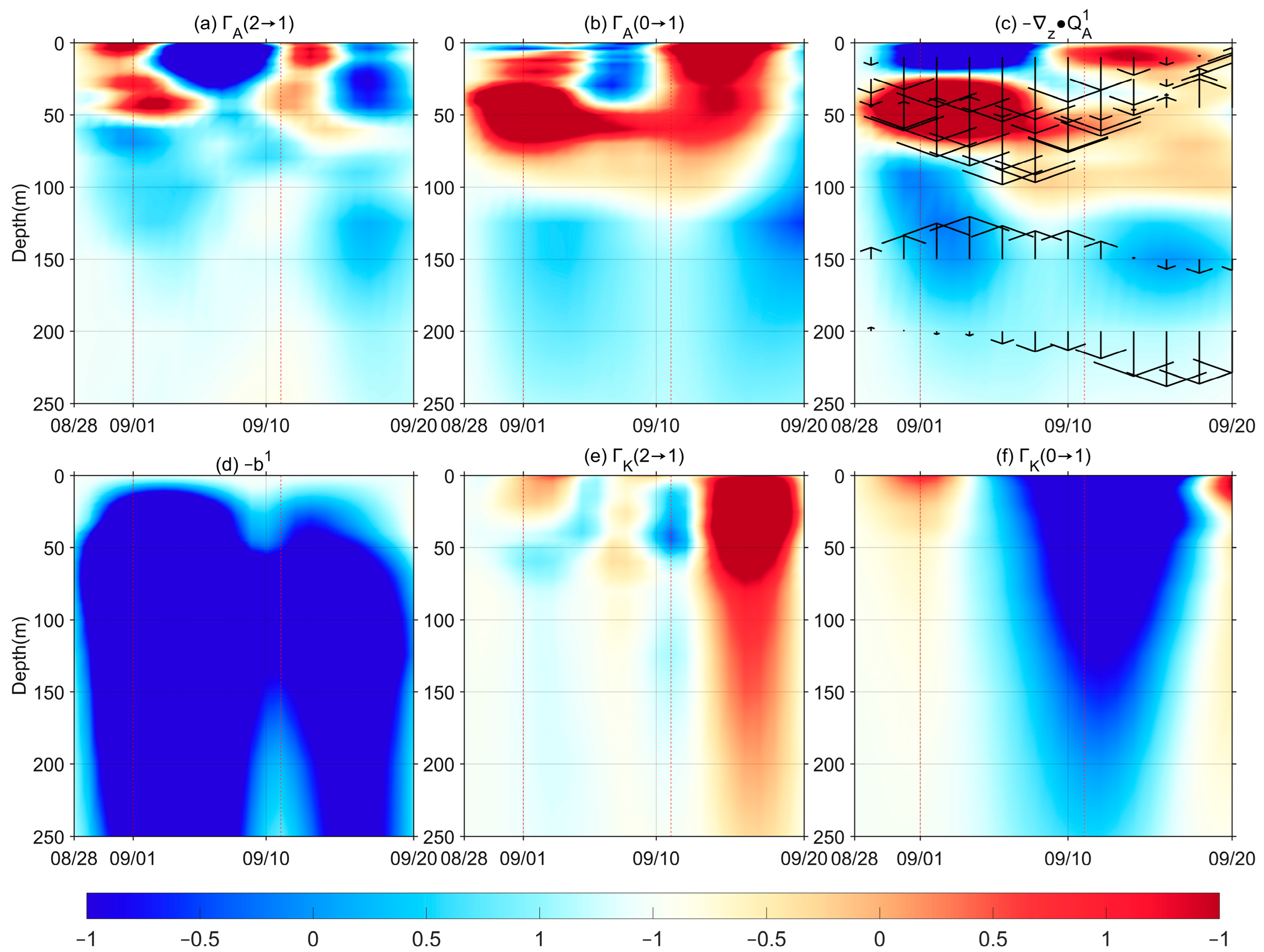
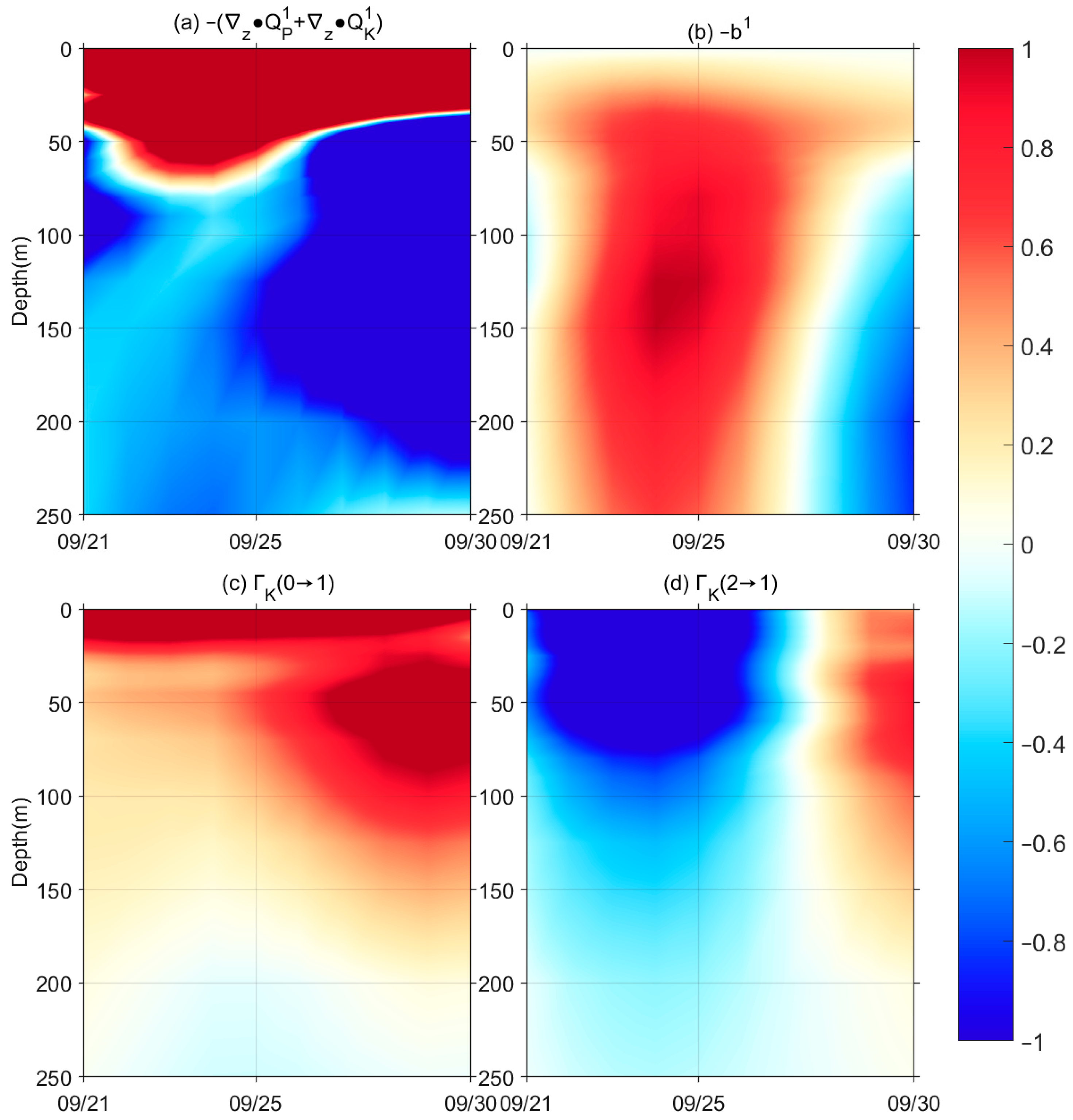
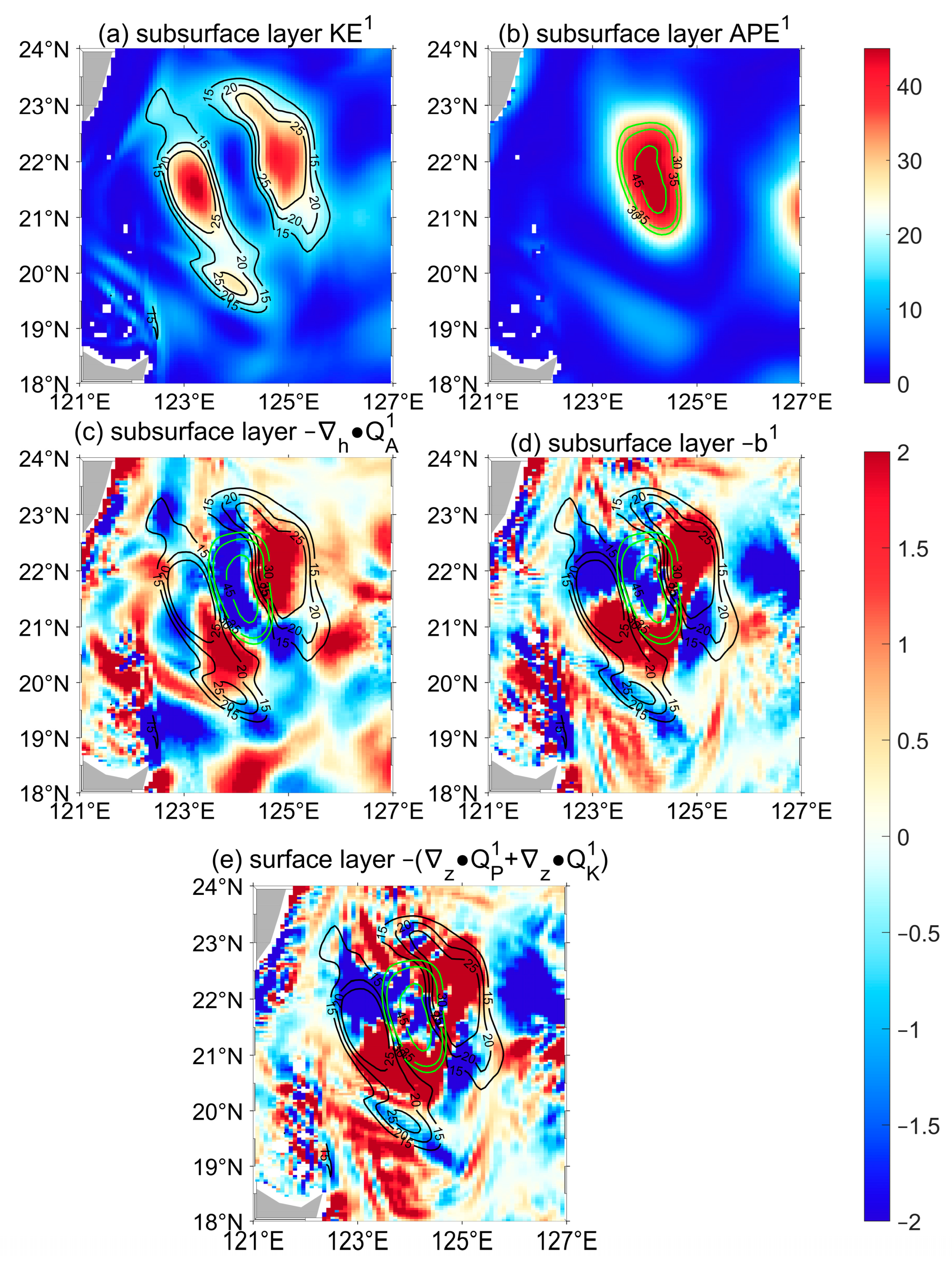
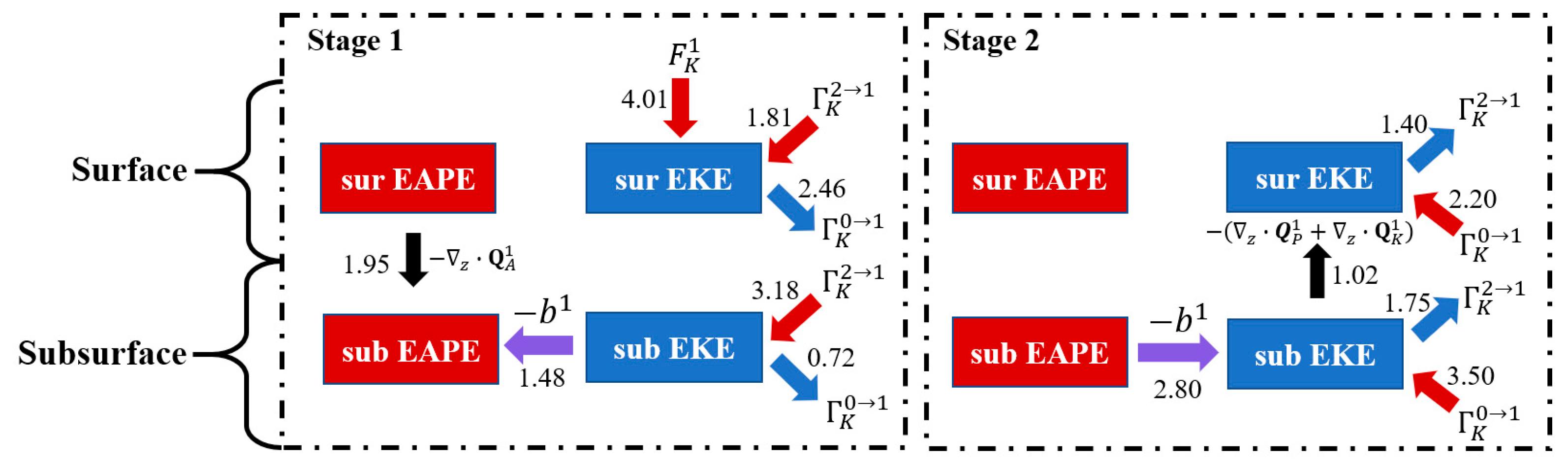
3.2. The Dynamics Study of the COE Evolution
4. Discussion and Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Emanuel, K.A. An Air-Sea Interaction Theory for Tropical Cyclones. Part I: Steady-State Maintenance. J. Atmos. Sci. 1986, 43, 585–605. [Google Scholar] [CrossRef]
- Price, J.F. Upper Ocean Response to a Hurricane. J. Phys. Oceanogr. 1981, 11, 153–175. [Google Scholar] [CrossRef]
- Vincent, E.M.; Lengaigne, M.; Madec, G.; Vialard, J.; Samson, G.; Jourdain, N.C.; Menkes, C.E.; Jullien, S. Processes Setting the Characteristics of Sea Surface Cooling Induced by Tropical Cyclones. J. Geophys. Res. Ocean. 2012, 117, C02020. [Google Scholar] [CrossRef]
- Potter, H.; Drennan, W.M.; Graber, H.C. Upper Ocean Cooling and Air-sea Fluxes under Typhoons: A Case Study. J. Geophys. Res. 2017, 122, 7237–7252. [Google Scholar] [CrossRef]
- Pei, Y.; Zhang, R.-H.; Chen, D. Roles of Different Physical Processes in Upper Ocean Responses to Typhoon Rammasun (2008)-Induced Wind Forcing. Sci. China Earth Sci. 2019, 62, 684–692. [Google Scholar] [CrossRef]
- Jacob, S.D.; Shay, L.K.; Mariano, A.J.; Black, P.G. The 3D Oceanic Mixed Layer Response to Hurricane Gilbert. J. Phys. Oceanogr. 2000, 30, 1407–1429. [Google Scholar] [CrossRef]
- Shay, L.; Goñi, G.; Black, P. Effects of a Warm Oceanic Feature on Hurricane Opal. Mon. Weather Rev. 2000, 128, 1366–1383. [Google Scholar] [CrossRef]
- Zhang, Z.; Wang, W.; Qiu, B. Oceanic Mass Transport by Mesoscale Eddies. Science 2014, 345, 322–324. [Google Scholar] [CrossRef]
- Zhang, H.; Wu, R.; Chen, D.; Liu, X.; He, H.; Tang, Y.; Ke, D.; Shen, Z.; Li, J.; Xie, J.; et al. Net Modulation of Upper Ocean Thermal Structure by Typhoon Kalmaegi (2014). J. Geophys. Res. Ocean. 2018, 123, 7154–7171. [Google Scholar] [CrossRef]
- Yang, Y.J.; Sun, L.; Duan, A.M.; Li, Y.B.; Fu, Y.F.; Yan, Y.F.; Wang, Z.Q.; Xian, T. Impacts of the Binary Typhoons on Upper Ocean Environments in November 2007. J. Appl. Remote Sens. 2012, 6, 3583. [Google Scholar] [CrossRef]
- Sun, L.; Li, Y.X.; Yang, Y.J.; Wu, Q.; Chen, X.T.; Li, Q.Y.; Li, Y.B.; Xian, T. Effects of Super Typhoons on Cyclonic Ocean Eddies in the Western North Pacific: A Satellite Data-based Evaluation between 2000 and 2008. J. Geophys. Res. Ocean. 2014, 119, 5585–5598. [Google Scholar] [CrossRef]
- Shang, X.D.; Zhu, H.B.; Chen, G.Y.; Xu, C.; Yang, Q. Research on Cold Core Eddy Change and Phytoplankton Bloom Induced by Typhoons: Case Studies in the South China Sea. Adv. Meteorol. 2015, 2015, 340432. [Google Scholar] [CrossRef]
- Gordon, A.L.; Shroyer, E.; Murty, V.S.N. An Intrathermocline Eddy and A Tropical Cyclone in the Bay of Bengal. Sci. Rep. 2017, 7, 46218. [Google Scholar] [CrossRef] [PubMed]
- Huang, X.; Wang, G. Response of a Mesoscale Dipole Eddy to the Passage of a Tropical Cyclone: A Case Study Using Satellite Observations and Numerical Modeling. Remote Sens. 2022, 14, 2865. [Google Scholar] [CrossRef]
- Lu, Z.; Wang, G.; Shang, X. Response of a Preexisting Cyclonic Ocean Eddy to a Typhoon. J. Phys. Oceanogr. 2016, 46, 2403–2410. [Google Scholar] [CrossRef]
- Lee, D.K.; Niiler, P.P. The Inertial Chimney: The Near-inertial Energy Drainage from the Ocean Surface to the Deep Layer. Geophys. Res. Ocean. 1998, 103, 7579–7591. [Google Scholar] [CrossRef]
- Ferrari, R.; Wunsch, C. Ocean Circulation Kinetic Energy: Reservoirs, Sources, and Sinks. Annu. Rev. Fluid Mech. 2009, 41, 253–282. [Google Scholar] [CrossRef]
- Polzin, K.L. Mesoscale Eddy–Internal Wave Coupling. Part II: Energetics and Results from PolyMode. J. Phys. Oceanogr. 2010, 40, 789–801. [Google Scholar] [CrossRef]
- Zhang, H.; Chen, D.; Zhou, L.; Liu, X.; Ding, T.; Zhou, B. Upper Ocean Response to Typhoon Kalmaegi (2014). J. Geophys. Res. Ocean. 2016, 121, 6520–6535. [Google Scholar] [CrossRef]
- Lu, Z.; Wang, G.; Shang, X. Strength and Spatial Structure of the Perturbation Induced by a Tropical Cyclone to the Underlying Eddies. J. Geophys. Res. Ocean. 2020, 125, e2020JC016097. [Google Scholar] [CrossRef]
- Zhai, X.; Marshall, D.P. Vertical Eddy Energy Fluxes in the North Atlantic Subtropical and Subpolar Gyres. J. Phys. Oceanogr. 2013, 43, 95–103. [Google Scholar] [CrossRef]
- Yang, G.; Wang, F.; Li, Y.; Lin, P. Mesoscale Eddies in the Northwestern Subtropical Pacific Ocean: Statistical Characteristics and Three-dimensional Structures. J. Geophys. Res. Ocean. 2013, 118, 1906–1925. [Google Scholar] [CrossRef]
- Sun, L.; Yang, Y.J.; Xian, T.; Wang, Y.; Fu, Y.F. Ocean Responses to Typhoon Namtheun Explored with Argo Floats and Multiplatform Satellites. Atmos.-Ocean. 2012, 50, 15–26. [Google Scholar] [CrossRef]
- Liang, X.S.; Anderson, D.G.M. Multiscale Window Transform. Multiscale Model. Simul. 2007, 6, 437–467. [Google Scholar] [CrossRef]
- Liang, X.S. Canonical Transfer and Multiscale Energetics for Primitive and Quasigeostrophic Atmospheres. J. Atmos. Sci. 2016, 73, 4439–4468. [Google Scholar] [CrossRef]
- Yang, Y.; Weisberg, R.H.; Liu, Y.; Liang, X.S. Instabilities and Multiscale Interactions Underlying the Loop Current Eddy Shedding in the Gulf of Mexico. J. Phys. Oceanogr. 2020, 50, 1289–1317. [Google Scholar] [CrossRef]
- Xu, F.; Liang, X.S. The Synchronization between the Zonal Jet Stream and Temperature Anomalies Leads to an Extremely Freezing North America in January 2019. Geophys. Res. Lett. 2020, 47, e2020GL089689. [Google Scholar] [CrossRef]
- Yang, Y.; Liang, X.S.; He, W.B. On the Formation and Maintenance of the Interannual Variability of the North Atlantic Oscillation. J. Atmos. Sci. 2024, 81, 177–208. [Google Scholar] [CrossRef]
- Ma, J.; Liang, X.S.; Chen, D. The Role of Multiscale Interaction in the Maintenance and Propagation of MJO in Boreal Winter. J. Clim. 2023, 36, 7827–7846. [Google Scholar] [CrossRef]
- Maslo, A.; Azevedo Correia de Souza, J.M.; Pardo, J.S. Energetics of the Deep Gulf of Mexico. J. Phys. Oceanogr. 2020, 50, 1655–1675. [Google Scholar] [CrossRef]
- Mak, M.; Cai, M. Local barotropic instability. J. Atmos. Sci. 1988, 46, 3289–3311. [Google Scholar] [CrossRef]
- Holmes, R.M.; Thomas, L.N. Modulation of tropical instability wave intensity by equatorial Kelvin waves. J. Phys. Oceanogr. 2016, 46, 2623–2643. [Google Scholar] [CrossRef]
- Dewar, W.K.; Bane, J.M. Gulf stream dynamics. Pad II: Eddy Energetics at 73°W. J. Phys. Oceanogr. 1989, 19, 1574–1587. [Google Scholar] [CrossRef]
- Chapman, C.C.; McC Hogg, A.; Kiss, E.; Rintoul, S.R. The dynamics of southern ocean storm tracks. J. Phys. Oceanogr. 2015, 45, 884–903. [Google Scholar] [CrossRef]
- Yang, H.; Wu, L.; Chang, P.; Qiu, B.; Jing, Z.; Zhang, Q.; Chen, Z. Mesoscale energy balance and air–sea interaction in the Kuroshio Extension: Low-frequency versus high-frequency variability. J. Phys. Oceanogr. 2021, 51, 895–910. [Google Scholar] [CrossRef]
- Ying, M.; Zhang, W.; Yu, H.; Lu, X.; Feng, J.; Fan, Y.; Zhu, Y.; Chen, D. An Overview of the China Meteorological Administration Tropical Cyclone Database. J. Atmos. Ocean. Technol. 2014, 31, 287–301. [Google Scholar] [CrossRef]
- Lu, X.; Yu, H.; Ying, M.; Zhao, B.; Zhang, S.; Lin, L.; Bai, L.; Wan, R. Western North Pacific Tropical Cyclone Database Created by the China Meteorological Administration. Adv. Atmos. Sci. 2021, 38, 690–699. [Google Scholar] [CrossRef]
- Chaigneau, A.; Eldin, G.; Dewitte, B. Eddy Activity in the Four Major Upwelling Systems from Satellite Altimetry (1992–2007). Prog. Oceanogr. 2009, 83, 117–123. [Google Scholar] [CrossRef]
- Yang, Y.; Lia, X.S. New Perspectives on the Generation and Maintenance of the Kuroshio Large Meander. J. Phys. Oceanogr. 2019, 49, 2095–2113. [Google Scholar] [CrossRef]
- Zhao, Y.-B.; Liang, X.S.; Gan, J. Nonlinear multiscale interactions and internal dynamics underlying a typical eddy-shedding event at Luzon Strait. J. Geophys. Res. Ocean. 2016, 121, 8208–8229. [Google Scholar] [CrossRef]
- Carton, X. Hydrodynamical Modeling of Oceanic Vortices. Surv. Geophys. 2001, 22, 179–263. [Google Scholar] [CrossRef]
- Sun, J.; Oey, L.Y.; Chang, R.; Xu, F.; Huang, S.M. Ocean Response to Typhoon Nuri (2008) in Western Pacific and South China Sea. Ocean. Dyn. 2015, 65, 735–749. [Google Scholar] [CrossRef]
- Tseng, Y.H.; Jan, S.; Dietrich, D.E.; Lin, I.I.; Chang, Y.T.; Tang, T.Y. Modeled Oceanic Response and Sea Surface Cooling to Typhoon Kai-Tak. Terr. Atmos. Ocean. Sci. 2010, 21, 85–98. [Google Scholar] [CrossRef]
- Kuo, Y.C.; Chern, C.S.; Wang, J.; Tsai, Y.L. Numerical Study of Upper Ocean Response to a Typhoon Moving Zonally across the Luzon Strait. Ocean. Dyn. 2011, 61, 1783–1795. [Google Scholar] [CrossRef]
- Sriver, R.; Huber, M. Observational evidence for an ocean heat pump induced by tropical cyclones. Nature 2007, 447, 577–580. [Google Scholar] [CrossRef]
- Wang, H.; Li, J.; Song, J.; Leng, H.; Wang, H.; Zhang, Z.; Zhang, H.; Zheng, M.; Yang, X.; Wang, C. The abnormal track of super typhoon Hinnamnor (2022) and its interaction with the upper ocean. Deep Sea Res. Part I Oceanogr. Res. Pap. 2023, 201, 104160. [Google Scholar] [CrossRef]
| Term | Mathematical Expression | Physical Interpretation |
|---|---|---|
| Tendency of KE on scale window | ||
| Tendency of APE on scale window | ||
| KE flux on scale window | ||
| Pressure flux on scale window | ||
| APE flux on scale window | ||
| Buoyancy (KE-to-APE) conversion on scale window | ||
| Canonical transfer of KE to scale window | ||
| Canonical transfer of APE to scale window | ||
| , | Residues |
| Surface | 0.59 | 2.2 | −1.4 | 11.3 |
| Subsurface | 2.8 | 3.5 | −1.75 | −12.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, J.; Rong, Y. The Long-Delayed Response of a Cyclonic Ocean Eddy to the Passage of Typhoons Hinnamnor and Muifa. Atmosphere 2025, 16, 601. https://doi.org/10.3390/atmos16050601
Wang J, Rong Y. The Long-Delayed Response of a Cyclonic Ocean Eddy to the Passage of Typhoons Hinnamnor and Muifa. Atmosphere. 2025; 16(5):601. https://doi.org/10.3390/atmos16050601
Chicago/Turabian StyleWang, Jiaqi, and Yineng Rong. 2025. "The Long-Delayed Response of a Cyclonic Ocean Eddy to the Passage of Typhoons Hinnamnor and Muifa" Atmosphere 16, no. 5: 601. https://doi.org/10.3390/atmos16050601
APA StyleWang, J., & Rong, Y. (2025). The Long-Delayed Response of a Cyclonic Ocean Eddy to the Passage of Typhoons Hinnamnor and Muifa. Atmosphere, 16(5), 601. https://doi.org/10.3390/atmos16050601






