Frictional and Particle Emission Behavior of Different Brake Disk Concepts Correlated with Optical Pin Surface Characterization
Abstract
1. Introduction
2. Materials and Methods
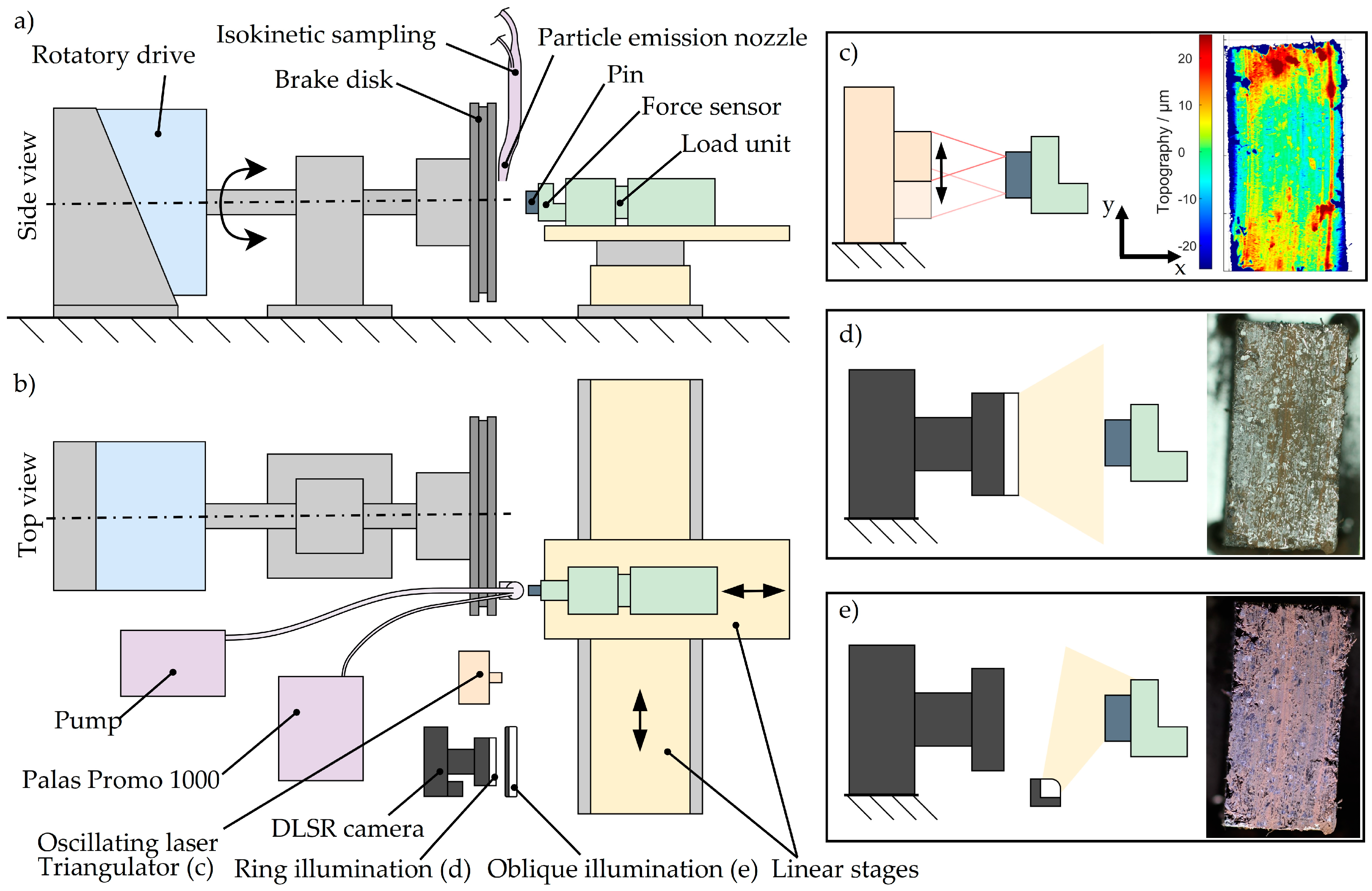
2.1. Measurement Setup
2.2. Friction Materials and Braking Conditions
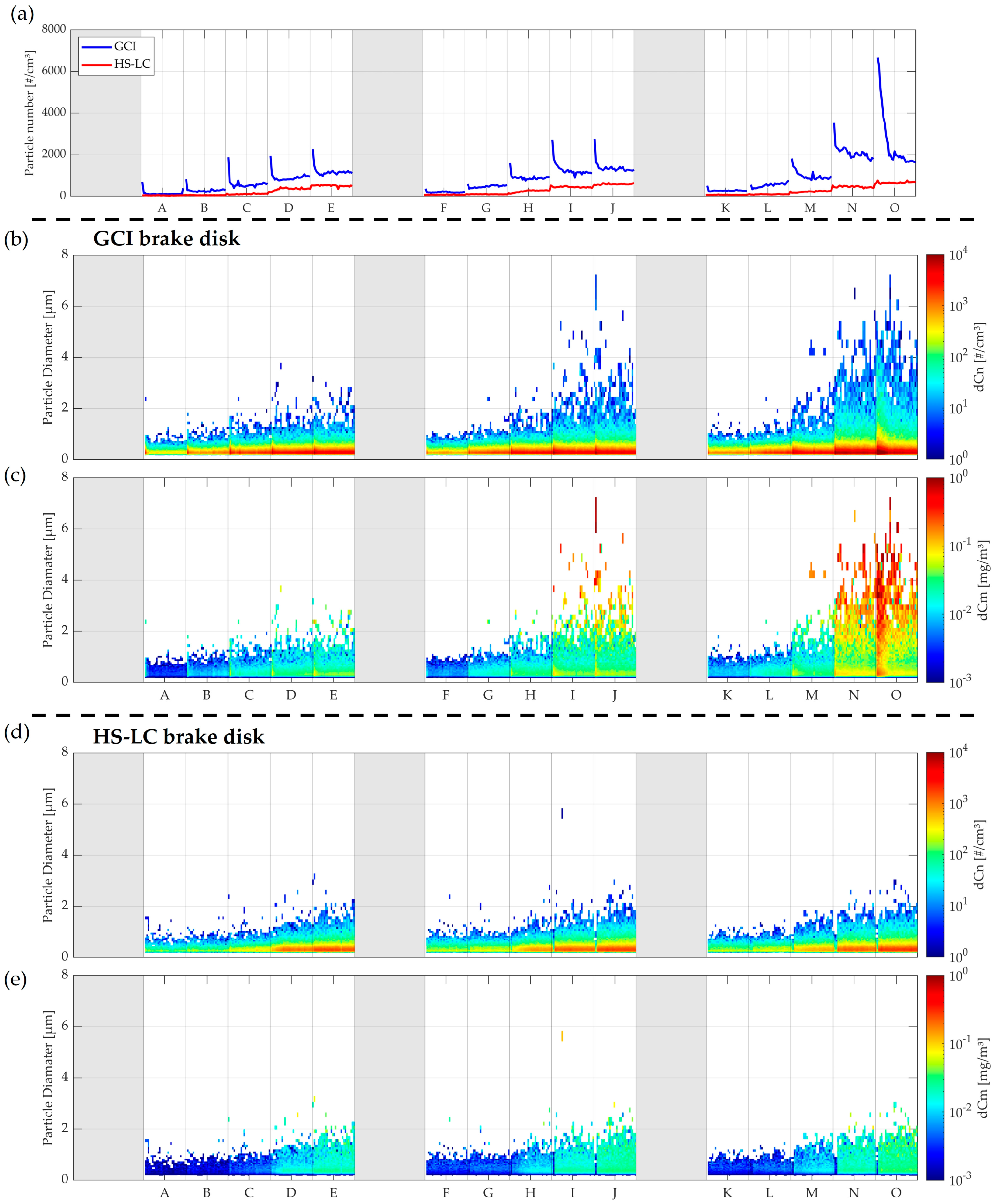
3. Friction Test Results and Surface Imaging
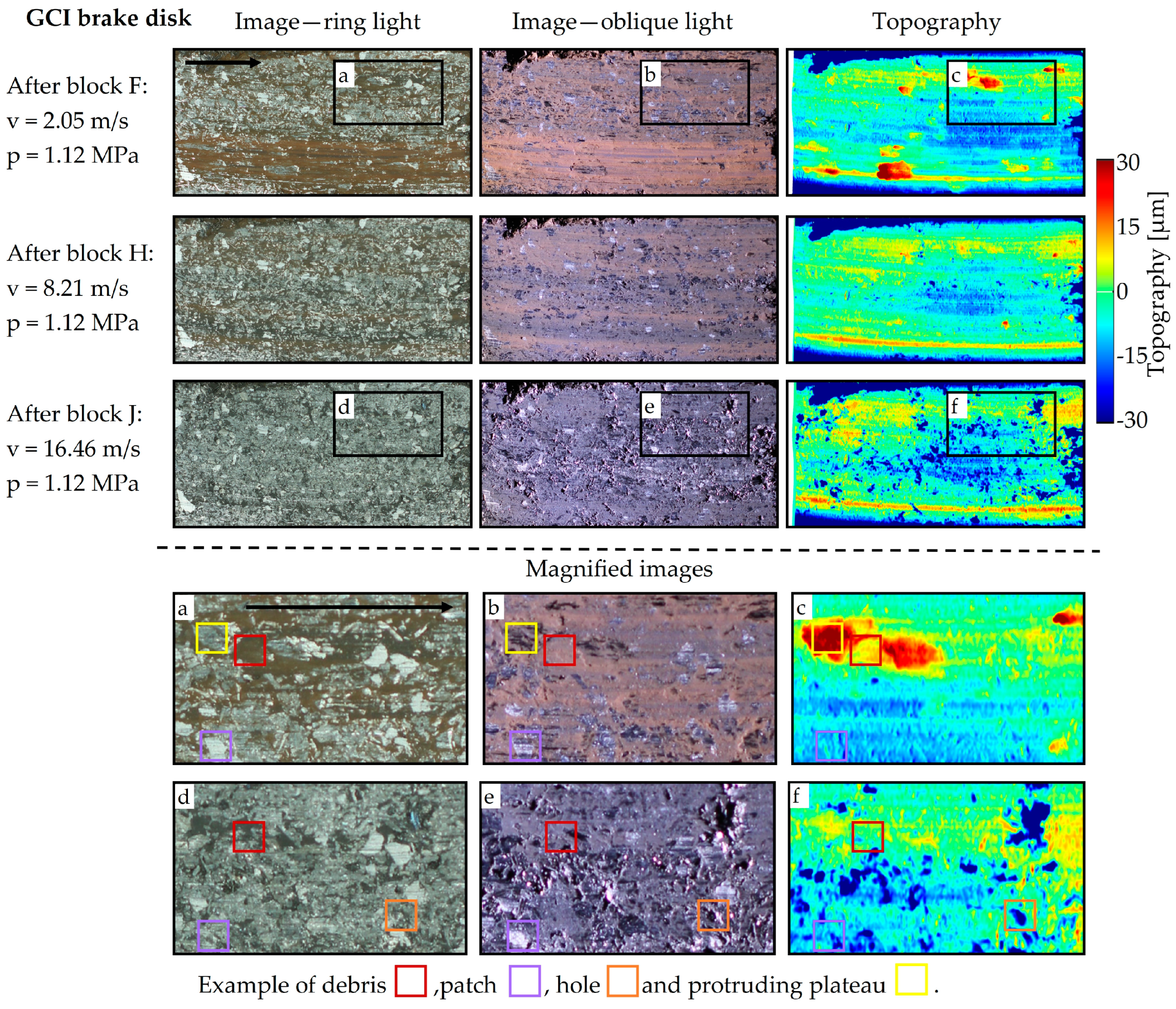
- Images with ring illumination have areas with brown, light gray, dark gray and white colors. The brown area increases in the initial block (F) before disappearing completely in block (I); conversely the light and dark gray areas enlarge.
- Images with oblique illumination display areas with red/brown, light gray, dark gray, white and black color. The red/brown areas match the brown areas, and even cover some gray and white areas of the ring illumination. The white areas in the ring illumination display under the oblique illumination red/brown, gray and white colors. Black areas are present near the pin border and also start to appear in the central region during block (H) after the removal of the red/brown areas.
- The topographic data show protruding patches. In the initial block (F), these patches seem to grow, before being removed completely in the next two blocks (G–H). Holes starts to appear as the patches are removed, showing a maximum at the highest velocity in this segment (J). The holes spatially match the black areas with the oblique illumination. The patches do not show clear color matching with either ring or oblique illumination images.
- Images with ring illumination show areas with yellow/gray, light gray, dark gray, and white colors. In general, there are only minor changes that are more visible comparing the initial and last blocks (F vs. J).
- Images with oblique illumination show areas with yellow/brown, light gray, dark gray, white and black colors. The yellow/brown areas are more uniformly distributed in between the patches after block (F), while after block (J) the only brown areas are in the upper and bottom regions.
- The topographic data show only small protruding patches. Holes are uniformly distributed in all blocks and do not show significant change with increased sliding velocity.
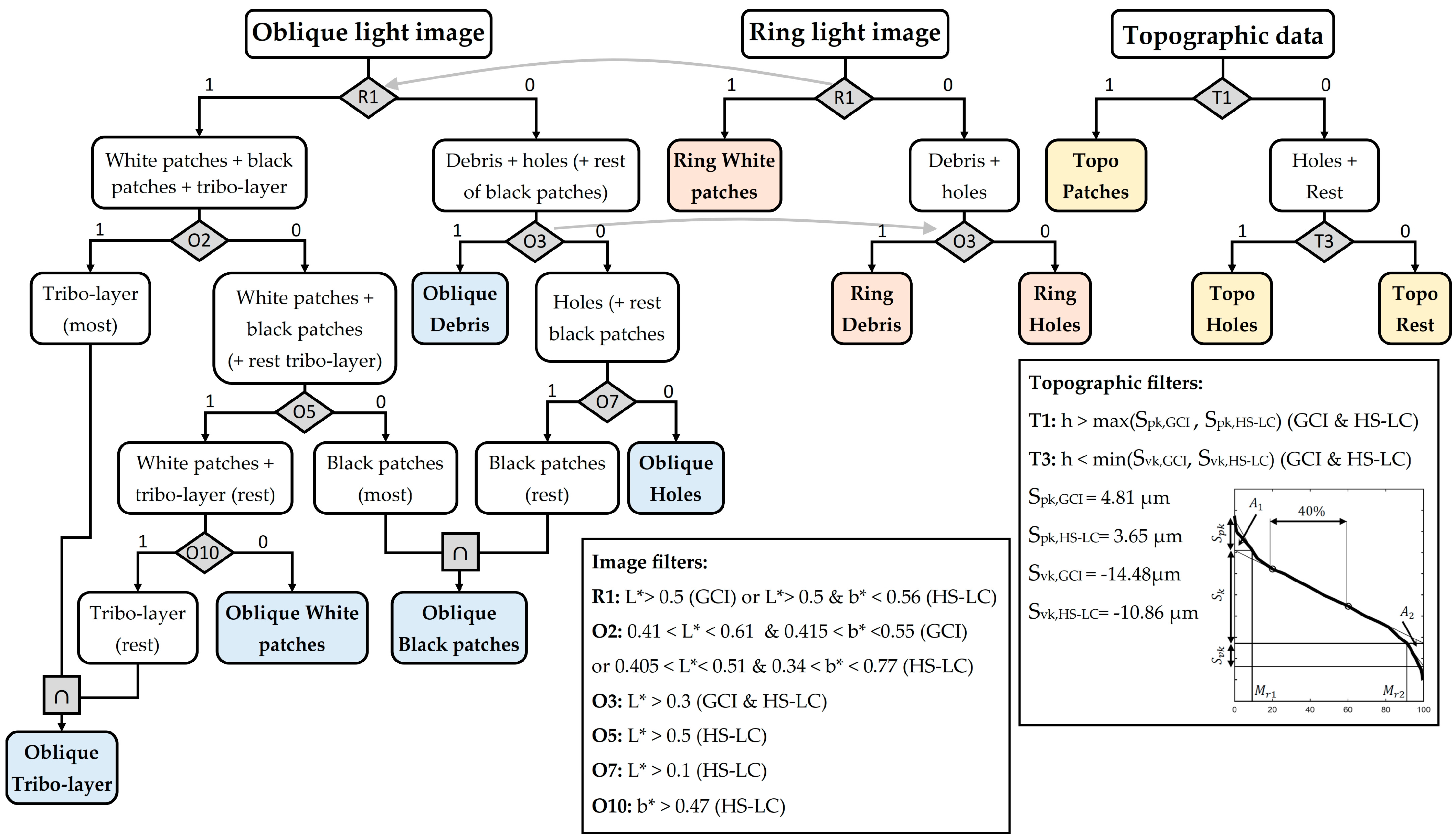
4. Image Segmentation and Correlation Analysis
4.1. Registration of Images and Topographic Data
- Image-to-image: color images are transformed to gray-scale images. Using speeded up robust features (SURF), the transformation matrix is determined. Only translation and rotation are considered for registration, and all images are registered with reference to the first image.
- Topographic level correction: a 3D point cloud is formed using the topographic data and the sample point locations in the x– and y–directions. The outliers, e.g., points on the pin holster, as well as the holes and patches, are ignored to ease the fitting of a plane. The slopes α (y–z) and β (x–z) of the fitted plane are used to correct the tilt of the point cloud. This step is repeated five times, while harshness of the threshold for holes and patches is increased. The transformation parameters are stored cumulatively in a final transformation matrix that is applied to the initial point cloud (without data removal). The transformed point cloud is interpolated with a new 2D mesh (x–y) and stored as a 2D matrix.
- Topography-to-topography: The topographic data are binned to generate a gray-scale image (topographic image), where the range of −20 µm to 20 µm is represented in 0–255 intensity counts. For the registration, an algorithm was developed that uses the cross-correlation values of a sliding interrogation window to generate a displacement vector field. This method is capable of accounting for various geometric transformations, such as rotation, translation, shear and scaling, while being robust to intensity changes of a matching structure (e.g., height difference of the same patch). All topographic images are registered with respect to the first topographic image.
- Topography-to-image registration is performed with the cross-correlation algorithm. The displacement field of an initial registration of the first image and the first topographic image is used for all subsequent topographic images. Remaining disparities are corrected by individual registration. The final displacement matrix is applied to the topographic data to maintain the full dynamic range in depth scale.
4.2. Segmentation Scheme
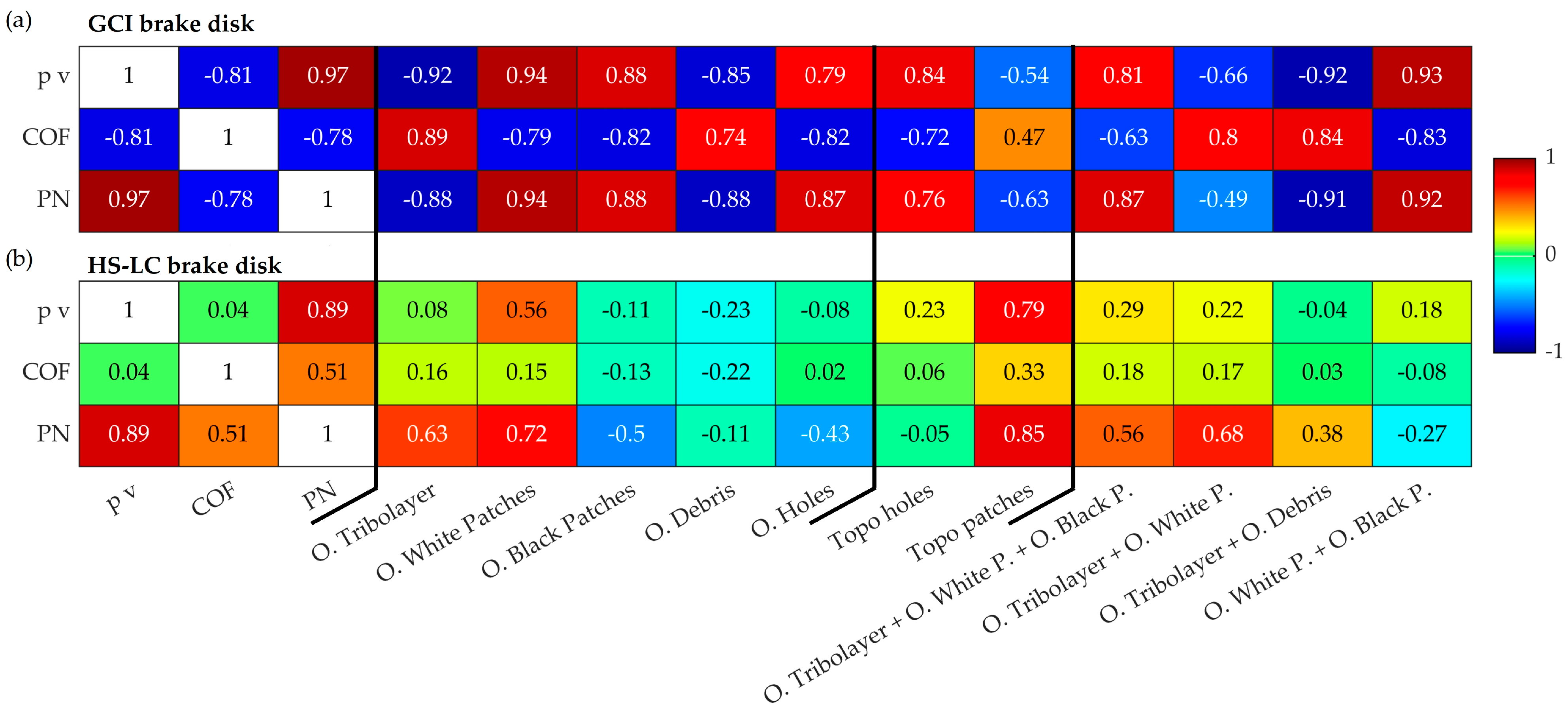
4.3. Segmentation Ratios over Brake Parameters
- COF: shows a steady decline as pv increases, although at the harshest braking block (24 MPa m/s) increases slightly.
- PN: increases steadily, even though between 7.5 MPa m/s and 15 MPa m/s the slope is less steep. At a pv of 24 MPa m/s a large variation can be observed.
- Oblique debris + oblique tribolayer: both behave similarly yet the debris layer is 20% higher. Up to a pv of 5 MPa m/s, both stay constant before declining by 20% and reaching a stable level after 17 MPa m/s.
- Oblique white patches and oblique black patches: show similar behavior, although the black patches expand over 10% more surface. Between 5 MPa m/s and 17 MPa m/s, both classes increase in coverage area. At 24 MPa m/s, the black patches seem to decrease slightly.
- Oblique holes: increase slowly up to 5 MPa m/s, then it increases with a steeper angle.
- Topo holes: show a similar behavior to oblique holes, but cover 5% more area.
- Topo patches: decrease steadily, reaching a stable level around 7%. At 24 MPa m/s it decreases further.
- COF: shows unstable behavior. Up to 5 MPa m/s, an increase with low spreading can be seen. Afterwards, the increase continues shortly, before a steady decline with larger spreading is established.
- PN: shows a stable, steady increase.
- Oblique debris + oblique tribolayer: behave similarly and maintain a constant level around 30%
- Oblique white patches: show a low coverage that slowly increases.
- Oblique holes + oblique black patches: behave similarly, although the black patches have an offset of 5%. Both decline steadily before reaching 7.5 MPa m/s, from which they increase slowly.
- Topo holes: vary slightly, maintaining a level around 8%.
- Topo patches: increase slightly, but steadily, reaching a maximum of 8%.
5. Discussion
- Moderate wear: exhibits a high COF with low PN emission. Secondary, protruding patches (topo patches) are present. Most of the surface is covered with oxidated debris (oblique debris and oblique tribolayer). The wear is also dominated by tribo-oxidative processes, in which an oxide layer is formed between the friction materials, thereby lubricating and protecting them [57,58].
- Transitional region: COF declines while PN emission increases steadily. The secondary patches are destroyed progressively (topo patches). Simultaneously, debris is removed from the surface, exposing more patches and holes. This has been observed in [42,56]. The remaining debris is barely oxidated and accumulated between the white and black patches. Some patches are removed, leaving cavities (topo holes). Three-body abrasion, defined as the material wear caused by the penetration of a harder particles (from the removed patches) between the two contacting surfaces in the softer surface [57], is present. This phenomenon might explain the increase of oblique black patches, as observed by [34] using a light microscope.
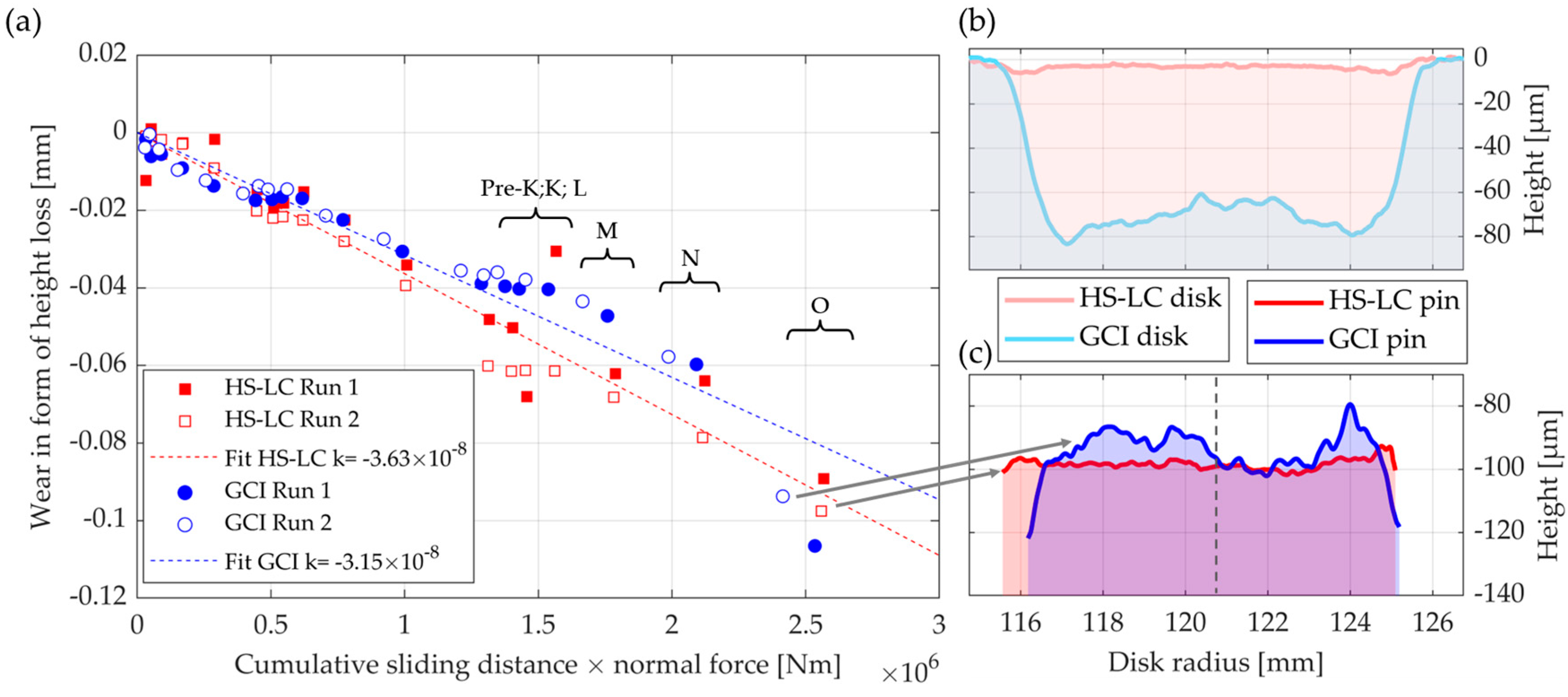
- Severe wear: COF stagnates at a lower level than at moderate wear. The further increase in number of cavities (topo holes) means that aggressive three-body abrasion is predominant. The unstable behavior of COF is observed in the harshest conditions, see block O in Figure 2b. The emission of larger particles is evident in Figure 3c,e. Furthermore, the wear increases non-linearly, as depicted in Figure 4a.
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| PM | Particulate Matter |
| NAO | Non-asbestos organic |
| LM | Low-metallic |
| GCI | Gray cast iron |
| HS-LC | High-speed laser cladding |
| COF | Coefficient of friction |
| CA | Cellular automaton |
| FEA | Finite element analysis |
| CFD | Computational fluid dynamics |
| LIM | Light interference microscopy |
| OM | Optical microscopy |
| SIM | Scanning ion microscopy |
| FIB | Focused ion beam |
| SEM | Scanning electron microscopy |
| TEM | Transmission electron microscopy |
| PN | Particle number |
| AUT | Automated universal tribotester |
| RPM | Revolutions per minute |
| WLTP | Worldwide harmonized light vehicles test procedure |
| SURF | Speeded up robust features |
| CIE | Commission internationale de l’éclairage |
References
- Grigoratos, T.; Martini, G. Brake wear particle emissions: A review. Environ. Sci. Pollut. Res. Int. 2015, 22, 2491–2504. [Google Scholar] [CrossRef] [PubMed]
- COM. 586–Proposal for a Regulation on Type-Approval of Motor Vehicles and Engines and of Systems, Components and Separate Technical Units Intended for Such Vehicles, with Respect to Their Emissions and Battery Durability (Euro7). Directorate General for Internal Market, Industry, Entrepreneurship and SMEs. Available online: https://single-market-economy.ec.europa.eu/sectors/automotive-industry/environmental-protection/emissions-automotive-sector_en (accessed on 28 February 2025).
- Neudeck, D. Low emission brakes–can the friction brake still be saved? In 9th International Munich Chassis Symposium 2018; Pfeffer, P., Ed.; Proceedings; Springer Fachmedien: Wiesbaden, Germany, 2019; pp. 675–687. [Google Scholar]
- Hascoët, M.; Adamczak, L. At source brake dust collection system. Results Eng. 2020, 5, 100083. [Google Scholar] [CrossRef]
- Storch, L.; Hamatschek, C.; Hesse, D.; Feist, F.; Bachmann, T.; Eichler, P.; Grigoratos, T. Comprehensive Analysis of Current Primary Measures to Mitigate Brake Wear Particle Emissions from Light-Duty Vehicles. Atmosphere 2023, 14, 712. [Google Scholar] [CrossRef]
- Piscitello, A.; Bianco, C.; Casasso, A.; Sethi, R. Non-exhaust traffic emissions: Sources, characterization, and mitigation measures. Sci. Total Environ. 2021, 766, 144440. [Google Scholar] [CrossRef] [PubMed]
- Giechaskiel, B.; Grigoratos, T.; Dilara, P.; Karageorgiou, T.; Ntziachristos, L.; Samaras, Z. Light-Duty Vehicle Brake Emission Factors. Atmosphere 2024, 15, 97. [Google Scholar] [CrossRef]
- Breuer, B.; Bill, K.H. Bremsenhandbuch; Springer Fachmedien: Wiesbaden, Germany, 2017. [Google Scholar]
- Aranke, O.; Algenaid, W.; Awe, S.; Joshi, S. Coatings for Automotive Gray Cast Iron Brake Discs: A Review. Coatings 2019, 9, 552. [Google Scholar] [CrossRef]
- Hamatschek, C.; Augsburg, K.; Schobel, D.; Gramstat, S.; Stich, A.; Gulden, F.; Hesse, D. Comparative Study on the Friction Behaviour and the Particle Formation Process between a Laser Cladded Brake Disc and a Conventional Grey Cast Iron Disc. Metals 2023, 13, 300. [Google Scholar] [CrossRef]
- Candeo, S.; Nogueira, A.P.; Gialanella, S.; Straffelini, S. Wear-emission correlation in brake materials. Wear 2025, 562–563, 205650. [Google Scholar] [CrossRef]
- Federici, M.; Menapace, C.; Mancini, A.; Straffelini, G.; Gialanella, S. Pin-on-disc study of dry sliding behavior of Co-free HVOF-coated disc tested against different friction materials. Friction 2021, 9, 1242–1258. [Google Scholar] [CrossRef]
- Manoj, A.; Saurabh, A.; Narala, S.K.R.; Saravanan, P.; Natu, H.P.; Verma, P.C. Surface modification of grey cast iron by laser cladding for automotive brake disc application. Wear 2023, 532–533, 205099. [Google Scholar] [CrossRef]
- Lyu, Y.; Varriale, F.; Malmborg, V.; Ek, M.; Pagels, J.; Wahlström, J. Tribology and airborne particle emissions from grey cast iron and WC reinforced laser cladded brake discs. Wear 2024, 556–557, 205512. [Google Scholar] [CrossRef]
- Hesse, D.; Hamatschek, C.; Augsburg, K.; Weigelt, T.; Prahst, A.; Gramstat, S. Testing of Alternative Disc Brakes and Friction Materials Regarding Brake Wear Particle Emissions and Temperature Behavior. Atmosphere 2021, 12, 436. [Google Scholar] [CrossRef]
- Manoj, A.; Verma, P.C.; Thangaraju, S.; Narala, S.K.R.; Saravanan, P. High-temperature dry sliding tribological behavior of Fe-based laser cladded grey cast iron for automotive brake disc application. Wear 2025, 205819. [Google Scholar] [CrossRef]
- Athanassiou, N.; Olofsson, U.; Wahlström, J.; Dizdar, S. Simulation of thermal and mechanical performance of laser cladded disc brake rotors. Proc. Inst. Mech. Eng. Part J J. Eng. Tribol. 2022, 236, 3–14. [Google Scholar] [CrossRef]
- Godet, M. The third-body approach: A mechanical view of wear. Wear 1984, 100, 437–452. [Google Scholar] [CrossRef]
- Eriksson, M.; Bergman, F.; Jacobson, S. Surface characterisation of brake pads after running under silent and squealing conditions. Wear 1999, 232, 163–167. [Google Scholar] [CrossRef]
- Eriksson, M.; Jacobson, S. Tribological surfaces of organic brake pads. Tribol. Int. 2000, 33, 817–827. [Google Scholar] [CrossRef]
- Eriksson, M.; Bergman, F.; Jacobson, S. On the nature of tribological contact in automotive brakes. Wear 2002, 252, 26–36. [Google Scholar] [CrossRef]
- Eriksson, M.; Lord, J.; Jacobson, S. Wear and contact conditions of brake pads: Dynamical in situ studies of pad on glass. Wear 2001, 249, 272–278. [Google Scholar] [CrossRef]
- Ostermeyer, G.P. Friction and wear of brake systems. Forsch. Im Ingenieurwesen 2001, 66, 267–272. [Google Scholar] [CrossRef]
- Ostermeyer, G.P.; Müller, M. Dynamic interaction of friction and surface topography in brake systems. Tribol. Int. 2006, 39, 370–380. [Google Scholar] [CrossRef]
- Müller, M.; Ostermeyer, G.P. A Cellular Automaton model to describe the three-dimensional friction and wear mechanism of brake systems. Wear 2007, 263, 1175–1188. [Google Scholar] [CrossRef]
- Wahlström, J.; Söderberg, A.; Olofsson, U. A Cellular Automaton Approach to Numerically Simulate the Contact Situation in Disc Brakes. Tribol. Lett. 2011, 42, 253–262. [Google Scholar] [CrossRef]
- Wahlström, J. Towards a cellular automaton to simulate friction, wear, and particle emission of disc brakes. Wear 2014, 313, 75–82. [Google Scholar] [CrossRef]
- Wahlström, J. A comparison of measured and simulated friction, wear, and particle emission of disc brakes. Tribol. Int. 2015, 92, 503–511. [Google Scholar] [CrossRef]
- Ostermeyer, G.-P.; Merlis, J.H. Modeling the Friction Boundary Layer of an Entire Brake Pad with an Abstract Cellular Automaton. Lubricants 2018, 6, 44. [Google Scholar] [CrossRef]
- Wahlström, J.; Söderberg, A.; Olander, L.; Jansson, A.; Olofsson, U. A pin-on-disc simulation of airborne wear particles from disc brakes. Wear 2010, 268, 763–769. [Google Scholar] [CrossRef]
- Riva, G.; Valota, G.; Perricone, G.; Wahlström, J. An FEA approach to simulate disc brake wear and airborne particle emissions. Tribol. Int. 2019, 138, 90–98. [Google Scholar] [CrossRef]
- Riva, G.; Perricone, G.; Wahlström, J. A Multi-Scale Simulation Approach to Investigate Local Contact Temperatures for Commercial Cu-Full and Cu-Free Brake Pads. Lubricants 2019, 7, 80. [Google Scholar] [CrossRef]
- Bettge, D.; Starcevic, J. Quantitative description of wear surfaces of disc brakes using interference microscopy. Wear 2001, 248, 121–127. [Google Scholar] [CrossRef]
- Limmer, F.; Paulus, A.; Barton, D.; Brooks, P.; Neville, A.; Kosarieh, S. A comparison of methods for characterizing brake pad surfaces. In Proceedings of the Eurobrake 2020 Conference, Barcelona, Spain, 2–4 June 2020. [Google Scholar]
- Österle, W.; Bettge, D. Vergleich von Methoden zur Charakterisierung von Bremsbelag-Oberflächen/A Comparison of Methods for Characterizing Brake Lining Surfaces. Pract. Metallogr. 2004, 41, 494–505. [Google Scholar] [CrossRef]
- Österle, W.; Urban, I. Friction layers and friction films on PMC brake pads. Wear 2004, 257, 215–226. [Google Scholar] [CrossRef]
- Neis, P.D.; Ferreira, N.F.; Sukumaran, J.; de Baets, P.; Ando, M.; Matozo, L.T.; Masotti, D. Characterization of surface morphology and its correlation with friction performance of brake pads. SCAD 2015, 6, 6. [Google Scholar] [CrossRef]
- Barros, L.Y.; Neis, P.D.; Ferreira, N.F.; Pavlak, R.P.; Masotti, D.; Matozo, L.T.; Sukumaran, J.; de Baets, P.; Andó, M. Morphological analysis of pad–disc system during braking operations. Wear 2016, 352–353, 112–121. [Google Scholar] [CrossRef]
- Neis, P.D.; Ferreira, N.F.; Fekete, G.; Matozo, L.T.; Masotti, D. Towards a better understanding of the structures existing on the surface of brake pads. Tribol. Int. 2017, 105, 135–147. [Google Scholar] [CrossRef]
- Poletto, J.C.; Barros, L.Y.; Neis, P.D.; Ferreira, N.F. Analysis of the error in the estimation of the morphology of contact plateaus existing on the surface of brake pads. Tribol. Int. 2018, 126, 297–306. [Google Scholar] [CrossRef]
- Barros, L.Y.; Poletto, J.C.; Buneder, D.; Neis, P.D.; Ferreira, N.F.; Pavlak, R.P.; Matozo, L.T. Effect of pressure in the transition between moderate and severe wear regimes in brake friction materials. Wear 2019, 438–439, 203112. [Google Scholar] [CrossRef]
- Barros, L.Y.; Poletto, J.C.; Gehlen, G.S.; Lasch, G.; Neis, P.D.; Ramalho, A.; Ferreira, N.F. Transition in wear regime during braking applications: An analysis of the debris and surfaces of the brake pad and disc. Tribol. Int. 2023, 189, 108968. [Google Scholar] [CrossRef]
- Limmer, F.; Brooks, P.C.; Gilkeson, C.; Kosarieh, S.; Barton, D.C. Tribo-oxidation of a brake friction couple under varying sliding conditions. Tribol. Int. 2023, 185, 108536. [Google Scholar] [CrossRef]
- Österle, W.; Urban, I. Third body formation on brake pads and rotors. Tribol. Int. 2006, 39, 401–408. [Google Scholar] [CrossRef]
- Bettge, D.; Starcevic, J. Topographic properties of the contact zones of wear surfaces in disc brakes. Wear 2003, 254, 195–202. [Google Scholar] [CrossRef]
- Otsu, N. A Threshold Selection Method from Gray-Level Histograms. IEEE Trans. Syst. Man Cybern. 1979, 9, 62–66. [Google Scholar] [CrossRef]
- Ostermeyer, G.P.; Schramm, T.; Raczek, S.; Bubser, F.; Perzborn, N. The automated universal tribotester. In Proceedings of the EuroBrake 2015 Conference, EB2015-STQ-016, Dresden, Germany, 4–6 May 2015. [Google Scholar]
- Perzborn, N.; Agudelo, C.; Ostermeyer, G.P. On Similarities and Differences of Measurements on Inertia Dynamometer and Scale Testing Tribometer for Friction Coefficient Evaluation. SAE Int. J. Mater. Manuf. 2015, 8, 104–117. Available online: www.jstor.org/stable/26268697 (accessed on 12 March 2025). [CrossRef]
- Raczek, S.; Ostermeyer, G.-P. Development and Implementation of Constant Friction Power Control in a Reduced Scale Brake Dynamometer for Investigations of Automotive Brake Systems. J. Mech. Eng. Autom. 2016, 6, 282–287. [Google Scholar] [CrossRef][Green Version]
- Schramm, T. Automatisierte Topografische Vermessung von Hochlastkontakten in Einem Tribometer. Ph.D. Thesis, TU Braunschweig, Braunschweig, Germany, 2019. [Google Scholar]
- Schramm, T.; Ostermeyer, G.-P. Automated Brake Pad Surface Topography Measurement Using the AUT. In Proceedings of the EuroBrake 2016 Conference, EB2016-SVM-023, Milan, Italy, 13–15 June 2016. [Google Scholar]
- Vogel, A.; Ostermeyer, G.-P. Adaptronic Actuator to Minimize the Pins Misalignment on Pin-on-Disc Testers; SAE Technical Paper Series; SAE International: Warrendale, PA, USA, 2018. [Google Scholar]
- Busin, L.; Vandenbroucke, N.; Macaire, L. Color Spaces and Image Segmentation; Advances in Imaging and Electron Physics; Elsevier: Amsterdam, The Netherlands, 2009; pp. 65–168. [Google Scholar]
- Commission Internationale de l’Éclairage. Colorimetry; Technical report CIE 15 2004; CIE: Vienna, Austria, 2004. [Google Scholar]
- DIN EN ISO 13565-2:1998-04; Geometrische Produktspezifikationen (GPS)_-Oberflächenbeschaffenheit: Tastschnittverfahren_-Oberflächen mit Plateauartigen Funktionsrelevanten Eigenschaften_-Teil_2: Beschreibung der Höhe Mittels Linearer Darstellung der Materialanteilkurve (ISO_13565-2:1996); Deutsche Fassung EN_ISO_13565-2:1997. Beuth Verlag GmbH: Berlin, Germany, 1998. [CrossRef]
- Candeo, S.; Leonardi, M.; Gialanella, S.; Straffelini, S. Influence of contact pressure and velocity on the brake behaviour and particulate matter emissions. Wear 2023, 514–515, 204579. [Google Scholar] [CrossRef]
- Straffelini, G. Friction and Wear; Springer International Publishing: Cham, Switzerland, 2015. [Google Scholar]
- Stott, F.H. The role of oxidation in the wear of alloys. Tribol. Int. 1998, 31, 61–71. [Google Scholar] [CrossRef]
- Barros, L.Y.; Poletto, J.C.; Buneder, D.; Flores, R.; Gehlen, G.; Neis, P.D.; Ferreira, N.F.; Matozo, L.T. An experimental study of the transition in the wear regime of brake friction materials. Polym. Compos. 2021, 42, 6310–6321. [Google Scholar] [CrossRef]
- Oesterle, W.; Dmitriev, A.I. Some Considerations on the Role of Third Bodies during Automotive Braking. SAE Int. J. Passeng. Cars-Mech. Syst. 2014, 7, 1287–1294. [Google Scholar] [CrossRef]
- Dizdar, S.; Lyu, Y.; Lampa, C.; Olofsson, U. Grey Cast Iron Brake Discs Laser Cladded with Nickel-Tungsten Carbide—Friction, Wear and Airborne Wear Particle Emission. Atmosphere 2020, 11, 621. [Google Scholar] [CrossRef]
- Candeo, S.; Varriale, F.; Nogueira, A.P.; Gialanella, S.; Straffelini, G. Performance of a cast-iron and WC Co-free iron-based coated disc under mild and severe brake conditions. Wear 2024, 548–549, 205369. [Google Scholar] [CrossRef]
- Stender, M.; Tiedemann, M.; Spieler, D.; Schoepflin, D.; Hoffmann, N.; Oberst, S. Deep learning for brake squeal: Brake noise detection, characterization and prediction. Mech. Syst. Signal Process. 2021, 149, 107181. [Google Scholar] [CrossRef]
- Caradec, Q.; Thévenot, M.; Durain, S.; Brunel, J.-F.; Dufrénoy, P.; Stender, M. Investigation of the Conditions of Brake Squeal Occurrence Using Machine Learning Methods. 2024. Available online: https://ssrn.com/abstract=5002168 (accessed on 26 March 2025).
- Steffan, J.J.; Jebadurai, I.J.; Asirvatham, L.G.; Manova, S.; Larkins, J.P. Prediction of Brake Pad Wear Using Various Machine Learning Algorithms. In Recent Trends in Design, Materials and Manufacturing; Singh, M.K., Gautam, R.K., Eds.; Lecture Notes in Mechanical Engineering; Springer Nature: Singapore, 2022; pp. 529–543. [Google Scholar]
- Sieberg, P.M.; Kurtulan, D.; Hanke, S. Wear Mechanism Classification Using Artificial Intelligence. Materials 2022, 15, 2358. [Google Scholar] [CrossRef] [PubMed]




| Section | Nominal Force [N] | Nominal Pressure 1 [MPa] | Sliding Velocity 2 [m/s] | Duration [s] | Number of Applications [-] |
|---|---|---|---|---|---|
| Bedding | 335 | 1.68 | 7.77 (GCI)/11.66 (HS-LC) | 10 | 200 |
| Segment 1 running-in | 112 | 0.56 | 1.94 | 3 | 50 |
| Segment 1 (blocks A-E) | 112 | 0.56 | 1.94, 3.98, 7.77, 11.66, 15.55 | 3 | 30 per velocity |
| Segment 2 running-in | 223 | 1.12 | 1.94 | 3 | 50 |
| Segment 2 (blocks F-J) | 223 | 1.12 | 1.94, 3.98, 7.77, 11.66, 15.55 | 3 | 30 per velocity |
| Segment 3 running-in | 335 | 1.68 | 1.94 | 3 | 50 |
| Segment 3 (blocks K-O) | 335 | 1.68 | 1.94, 3.98, 7.77, 11.66, 15.55 | 3 | 30 per velocity |
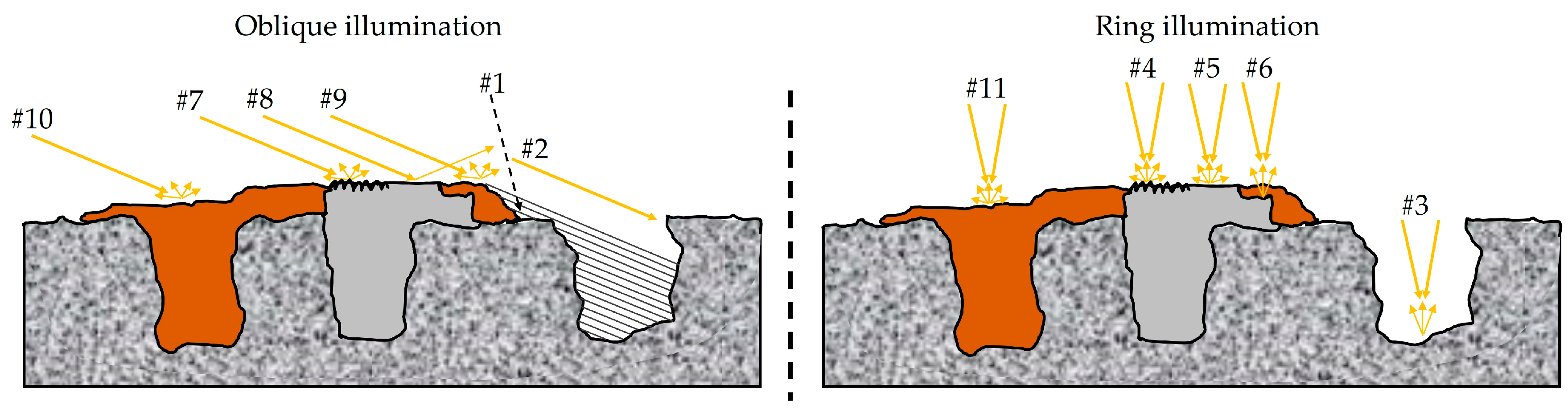
| Topographic Classes | Ring Light Classes 1 | Oblique Light Classes | Observed Color/Features | Example in Zoom-Images | Possible Match to Theory |
|---|---|---|---|---|---|
| - | White patches | White patches | White, bright areas |  | Primary patches |
| - | Black patches | Black areas that show white under ring light |  | Primary patches | |
| - | Tribolayer | Brown/light gray areas that show white under ring light |  | Secondary patches | |
| - | Debris | Debris | Brown areas |  | Secondary patches |
| - | Holes | Holes | Black/dark grey areas |  | Lowlands |
| Patches | - | - | Protruding from surface |  | Primary and secondary patches |
| Holes | - | - | Cavities on the surface |  | Lowlands |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Londono Alfaro, J.C.; Brandt, S.; Fang, C.; Hesse, D.; Gericke, T.; Schiefer, F.; Schilde, C.; Kaiser, S.A. Frictional and Particle Emission Behavior of Different Brake Disk Concepts Correlated with Optical Pin Surface Characterization. Atmosphere 2025, 16, 563. https://doi.org/10.3390/atmos16050563
Londono Alfaro JC, Brandt S, Fang C, Hesse D, Gericke T, Schiefer F, Schilde C, Kaiser SA. Frictional and Particle Emission Behavior of Different Brake Disk Concepts Correlated with Optical Pin Surface Characterization. Atmosphere. 2025; 16(5):563. https://doi.org/10.3390/atmos16050563
Chicago/Turabian StyleLondono Alfaro, Juan C., Sven Brandt, Chengyuan Fang, David Hesse, Timo Gericke, Frank Schiefer, Carsten Schilde, and Sebastian A. Kaiser. 2025. "Frictional and Particle Emission Behavior of Different Brake Disk Concepts Correlated with Optical Pin Surface Characterization" Atmosphere 16, no. 5: 563. https://doi.org/10.3390/atmos16050563
APA StyleLondono Alfaro, J. C., Brandt, S., Fang, C., Hesse, D., Gericke, T., Schiefer, F., Schilde, C., & Kaiser, S. A. (2025). Frictional and Particle Emission Behavior of Different Brake Disk Concepts Correlated with Optical Pin Surface Characterization. Atmosphere, 16(5), 563. https://doi.org/10.3390/atmos16050563









