Regionalization of the Hargreaves-Samani Coefficients to Estimate Reference Evapotranspiration in High-Altitude Areas
Abstract
1. Introduction
2. Materials and Methods
2.1. Study Area
2.2. Climatic and Terrain Data
2.3. Penman–Monteith Method
2.4. Hargreaves-Samani Method
2.5. Statistical Metrics of Performance
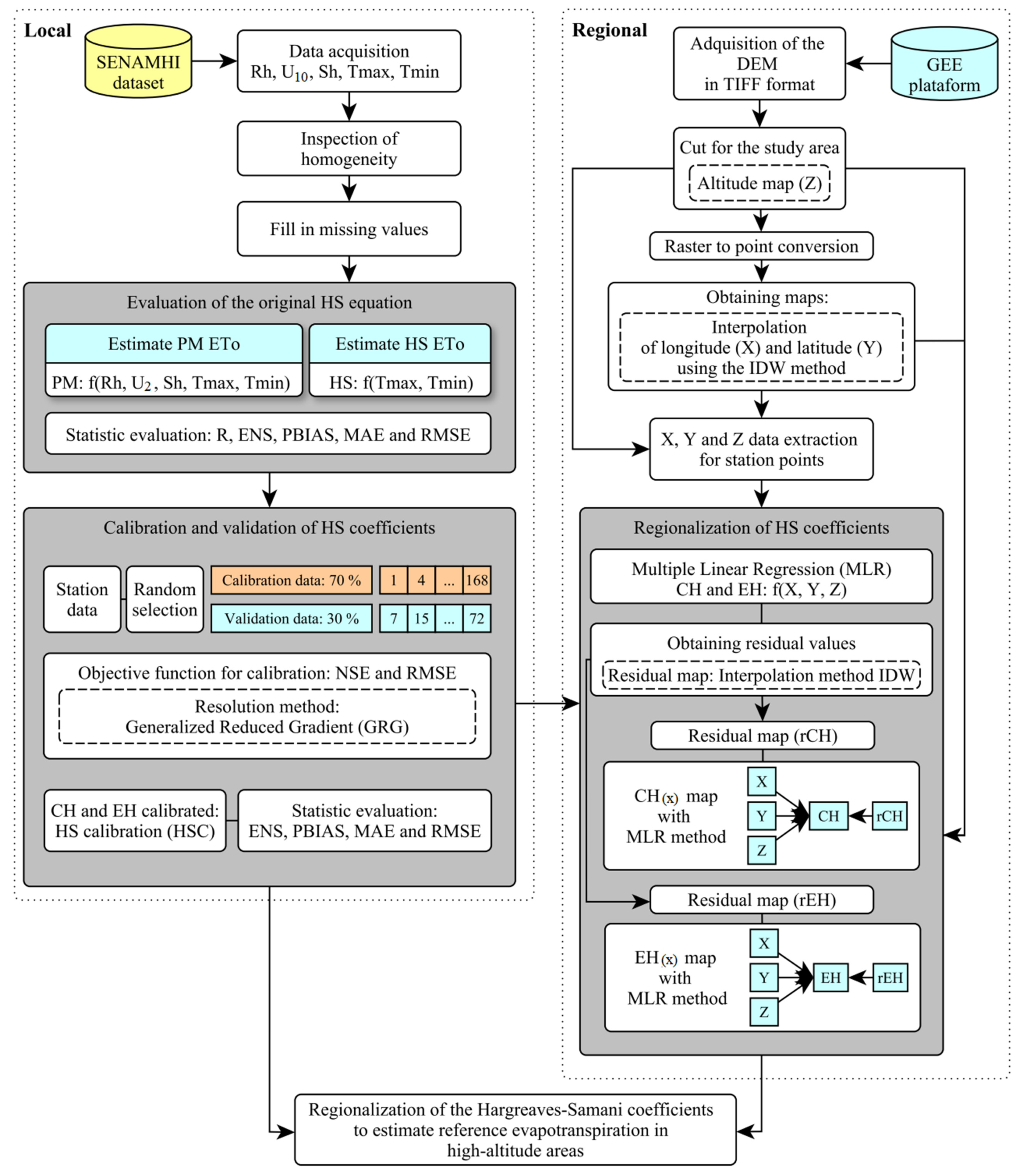
2.6. Calibration and Validation of the Coefficients of the HS Equation
- Simultaneous calibration of the empirical Hargreaves coefficient (CH) and empirical Hargreaves exponent (EH), keeping the empirical temperature Hargreaves (CT = 17.8) constant.
2.7. Regionalization of the Coefficients of the HS Equation
3. Results and Discussion
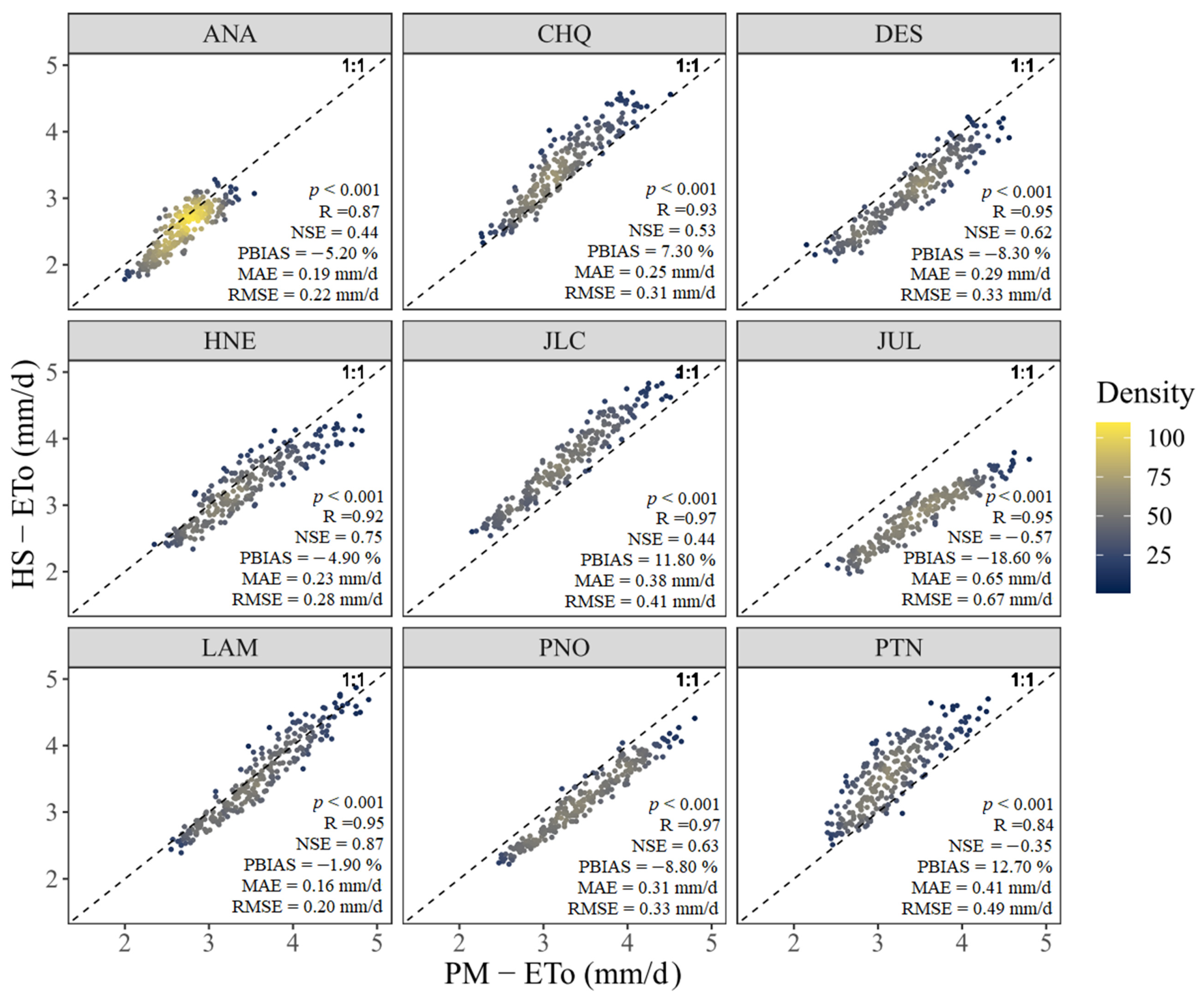
3.1. Evaluation of the Original HS Equation
3.2. Calibration and Validation of the HS Coefficients
Calibration and Validation of the CH and EH Coefficient
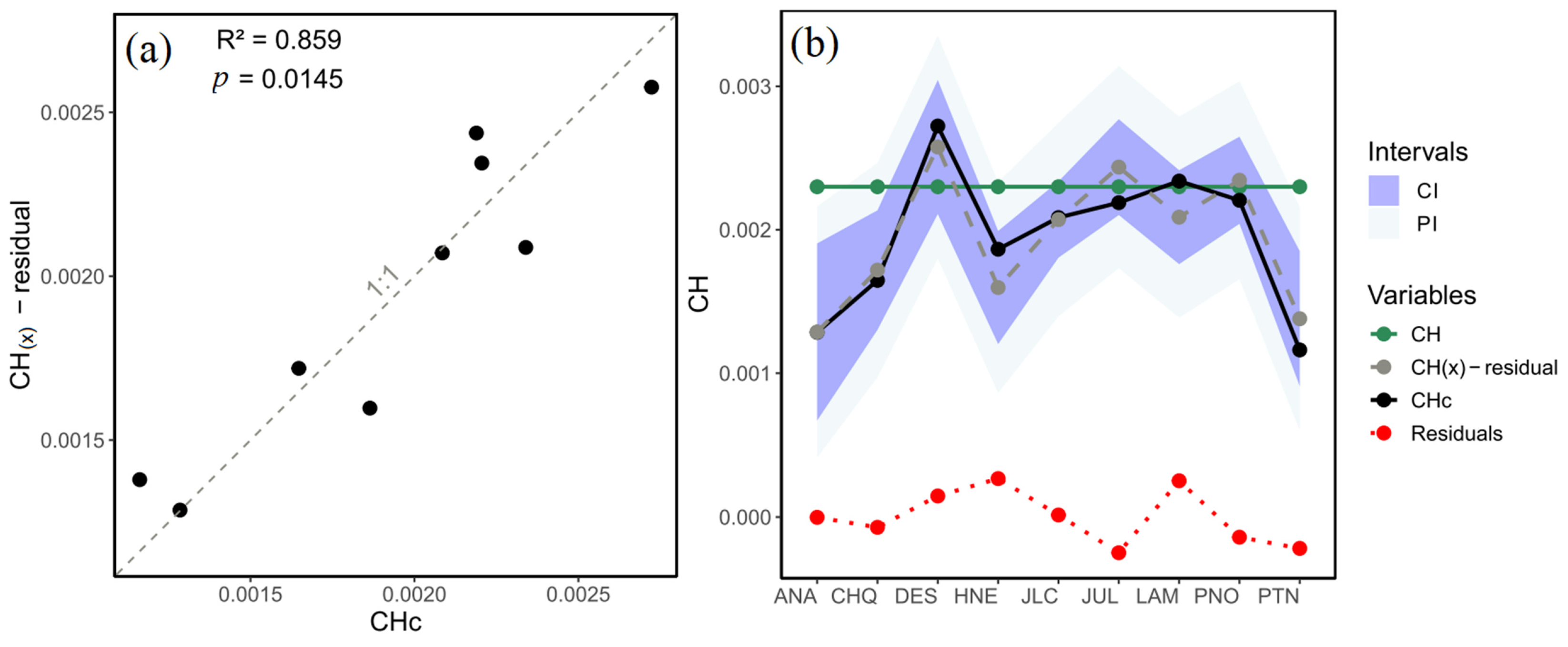
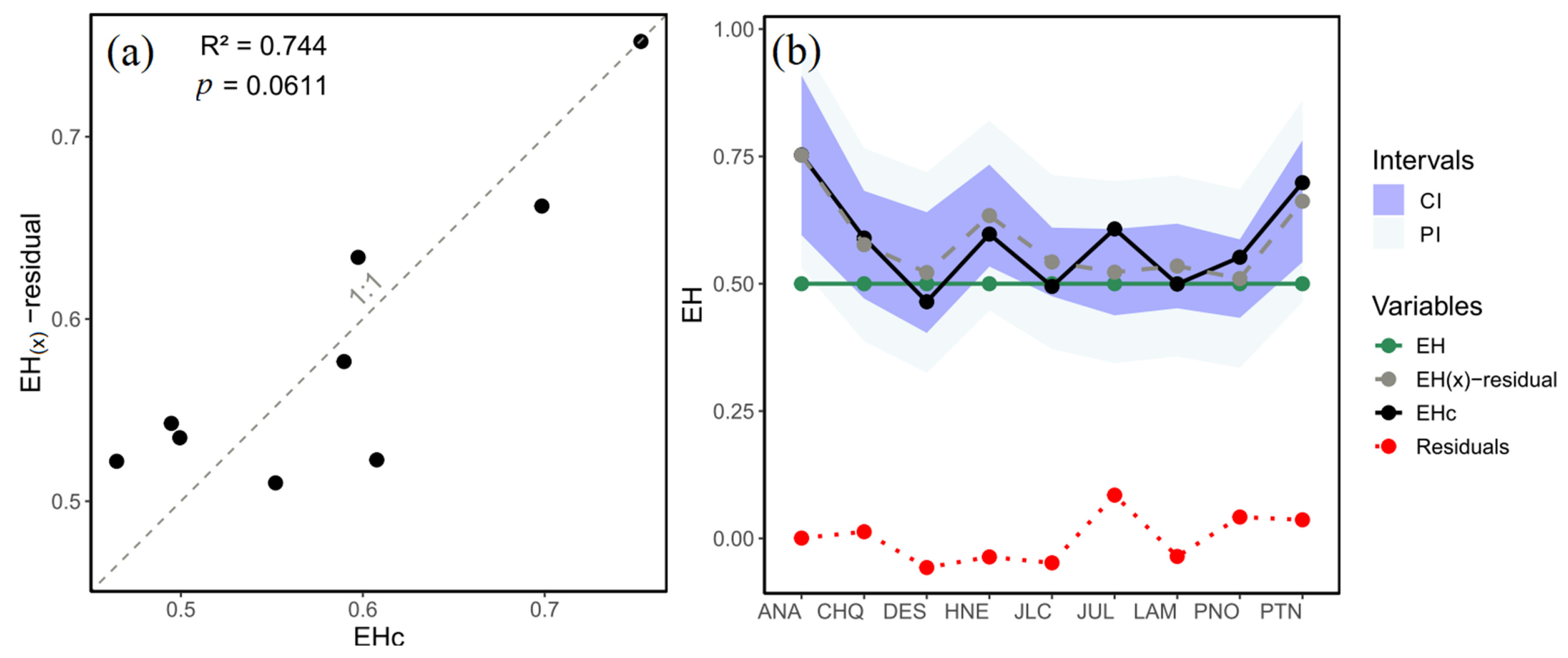
3.3. Regionalization of the HS Coefficients
Regionalization of the CHC and EHC Coefficients
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Hadria, R.; Benabdelouhab, T.; Lionboui, H.; Salhi, A. Comparative assessment of different reference evapotranspiration models towards a fit calibration for arid and semi-arid areas. J. Arid. Environ. 2021, 184, 104318. [Google Scholar] [CrossRef]
- Martınez-Cob, A.; Tejero-Juste, M. A wind-based qualitative calibration of the Hargreaves ET0 estimation equation in semiarid regions. Agric. Water Manag. 2004, 64, 251–264. [Google Scholar] [CrossRef]
- Berti, A.; Tardivo, G.; Chiaudani, A.; Rech, F.; Borin, M. Assessing reference evapotranspiration by the Hargreaves method in north-eastern Italy. Agric. Water Manag. 2014, 140, 20–25. [Google Scholar] [CrossRef]
- Droogers, P.; Allen, R.G. Estimating reference evapotranspiration under inaccurate data conditions. Irrig. Drain. Syst. 2002, 16, 33–45. [Google Scholar] [CrossRef]
- Chipana, R.; Yujra, R.; Paredes, P.; Pereira, L.S. Determinación de la evapotranspiración de referencia con datos limitados en zonas de altura del Altiplano Boliviano aplicando la metodología de la FAO y la ecuación de Hargreaves-Samani. In I Congreso Boliviano de Riego y Drenaje; Facultad de Agronomía: La Paz, Bolivia, 2010; pp. 12–14. [Google Scholar]
- Todorovic, M.; Karic, B.; Pereira, L.S. Reference Evapotranspiration estimate with limited weather data across a range of Mediterranean climates. J. Hydrol. 2013, 481, 166–176. [Google Scholar] [CrossRef]
- Raziei, T.; Pereira, L.S. Estimation of ETo with Hargreaves–Samani and FAO-PM temperature methods for a wide range of climates in Iran. Agric. Water Manag. 2013, 121, 1–18. [Google Scholar] [CrossRef]
- Trajkovic, S. Hargreaves versus Penman-Monteith under Humid Conditions. J. Irrig. Drain. Eng. 2007, 133, 38–42. [Google Scholar] [CrossRef]
- Muhammad, M.K.I.; Nashwan, M.S.; Shahid, S.; Ismail, T.B.; Song, Y.H.; Chung, E.S. Evaluation of empirical reference evapotranspiration models using compromise programming: A case study of Peninsular Malaysia. Sustainability 2019, 11, 4267. [Google Scholar] [CrossRef]
- Adamala, S.; Raghuwanshi, N.S.; Mishra, A.; Tiwari, M.K. Evapotranspiration modeling using second-order neural networks. J. Hydrol. Eng. 2014, 19, 1131–1140. [Google Scholar] [CrossRef]
- Srivastava, A.; Sahoo, B.; Raghuwanshi, N.S.; Singh, R. Evaluation of Variable-Infiltration Capacity Model and MODIS-Terra Satellite-Derived Grid-Scale Evapotranspiration Estimates in a River Basin with Tropical Monsoon-Type Climatology. J. Irrig. Drain. Eng. 2017, 143, 4017028. [Google Scholar]
- Kumari, N.; Srivastava, A. An Approach for Estimation of Evapotranspiration by Standardizing Parsimonious Method. Agric. Res. 2020, 9, 301–309. [Google Scholar]
- Hamed, M.M.; Khan, N.; Muhammad, M.K.I.; Shahid, S. Ranking of Empirical Evapotranspiration Models in Different Climate Zones of Pakistan. Land 2022, 11, 2168. [Google Scholar] [CrossRef]
- Pereira, L.S.; Allen, R.G.; Smith, M.; Raes, D. Crop evapotranspiration estimation with FAO56: Past and future. Agric. Water Manag. 2015, 147, 4–20. [Google Scholar]
- Allen, R.G.; Pereira, L.S.; Raes, D.; Smith, M. Crop Evapotranspiration-Guidelines for Computing Crop Water Requirements-FAO Irrigation and Drainage Paper 56; FAO: Rome, Italy, 1998; Volume 300; p. D05109. [Google Scholar]
- Zhu, X.; Luo, T.; Luo, Y.; Yang, Y.; Guo, L.; Luo, H.; Fang, C.; Cui, Y. Calibration and validation of the Hargreaves-Samani model for reference evapotranspiration estimation in China. Irrig. Drain. 2019, 68, 822–836. [Google Scholar]
- Almorox, J.; Senatore, A.; Quej, V.H.; Mendicino, G. Worldwide assessment of the Penman–Monteith temperature approach for the estimation of monthly reference evapotranspiration. Theor. Appl. Climatol. 2018, 131, 693–703. [Google Scholar]
- Hargreaves, G.H.; Samani, Z.A. Reference Crop Evapotranspiration from Temperature. Appl. Eng. Agric. 1985, 1, 96–99. [Google Scholar]
- Cobaner, M.; Citakoğlu, H.; Haktanir, T.; Kisi, O. Modifying Hargreaves–Samani equation with meteorological variables for estimation of reference evapotranspiration in Turkey. Hydrol. Res. 2017, 48, 480–497. [Google Scholar]
- Bogawski, P.; Bednorz, E. Comparison and validation of selected evapotranspiration models for conditions in Poland (Central Europe). Water Resour. Manag. 2014, 28, 5021–5038. [Google Scholar]
- Čadro, S.; Uzunović, M.; Žurovec, J.; Žurovec, O. Validation and calibration of various reference evapotranspiration alternative methods under the climate conditions of Bosnia and Herzegovina. Int. Soil Water Conserv. Res. 2017, 5, 309–324. [Google Scholar]
- Awal, R.; Habibi, H.; Fares, A.; Deb, S. Estimating reference crop evapotranspiration under limited climate data in West Texas. J. Hydrol. Reg. Stud. 2020, 28, 100677. [Google Scholar]
- Xu, C.Y.; Singh, V.P. Evaluation and generalization of temperature-based methods for calculating evaporation. Hydrol. Process. 2001, 15, 305–319. [Google Scholar] [CrossRef]
- Vanderlinden, K.; Giraldez, J.V.; Van Meirvenne, M. Assessing reference evapotranspiration by the Hargreaves method in southern Spain. J. Irrig. Drain. Eng. 2004, 130, 184–191. [Google Scholar] [CrossRef]
- Sepaskhah, A.R.; Razzaghi, F. Evaluation of the adjusted Thornthwaite and Hargreaves-Samani methods for estimation of daily evapotranspiration in a semi-arid region of Iran. Arch. Agron. Soil Sci. 2009, 55, 51–66. [Google Scholar] [CrossRef]
- Tabari, H.; Talaee, P.H. Local calibration of the Hargreaves and Priestley-Taylor equations for estimating reference evapotranspiration in arid and cold climates of Iran based on the Penman-Monteith model. J. Hydrol. Eng. 2011, 16, 837. [Google Scholar] [CrossRef]
- Ferreira, L.B.; Cunha, F.F.D.; Duarte, A.B.; Sediyama, G.C.; Cecon, P.R. Calibration methods for the Hargreaves-Samani equation. Ciência Agrotecnol. 2018, 42, 104–114. [Google Scholar] [CrossRef]
- Subburayan, S.; Murugappan, A.; Mohan, S. Modified Hargreaves equation for estimation of ETo in a hot and humid location in Tamilnadu State, India. Int. J. Eng. Sci. Technol. 2011, 3, 592–600. [Google Scholar]
- Kelso-Bucio, H.A.; Bâ, K.M.; Sánchez-Morales, S.; Reyes-López, D. Calibración del exponente de la ecuación Hargreaves-ETo en los estados de Chiapas, Oaxaca, Puebla y Veracruz, México. Agrociencia 2012, 46, 221–229. [Google Scholar]
- Almorox, J.; Elisei, V.; Aguirre, M.E.; Commegna, M. Calibración del modelo de Hargreaves para la estimación de la evapotranspiración de referencia en Coronel Dorrego, Argentina. Rev. Fac. Cienc. Agrar. Univ. Nac. Cuyo 2012, 44, 101–109. [Google Scholar]
- Patel, J.; Patel, H.; Bhatt, C. Modified Hargreaves equation for accurate estimation of evapotranspiration of diverse climate locations in India. Proc. Natl. Acad. Sci. India Sect. B Biol. Sci. 2014, 85, 161–166. [Google Scholar] [CrossRef]
- Mohawesh, O.E.; Talozi, S.A. Comparison of Hargreaves and FAO56 equations for estimating monthly evapotranspiration for semi-arid and arid environments. Arch. Agron. Soil Sci. 2012, 58, 321–334. [Google Scholar] [CrossRef]
- Pandey, V.; Pandey, P.K.; Mahanta, A.P. Calibration and performance verification of Hargreaves Samani equation in a humid region. Irrig. Drain. 2014, 63, 659–667. [Google Scholar]
- Dorji, U.; Olesen, J.E.; Seidenkrantz, M.S. Water balance in the complex mountainous terrain of Bhutan and linkages to land use. J. Hydrol. Reg. Stud. 2016, 7, 55–68. [Google Scholar]
- Pandey, P.K.; Pandey, V. Parametric calibration of Hargreaves–Samani (HS) reference evapotranspiration equation with different coefficient combinations under the humid environment. HydroResearch 2023, 6, 147–155. [Google Scholar]
- Gavilán, P.; Lorite, I.J.; Tornero, S.; Berengena, J. Regional calibration of Hargreaves equation for estimating reference et in a semiarid environment. Agric. Water Manag. 2006, 81, 257–281. [Google Scholar]
- Garcia, M.; Raes, D.; Allen, R.; Herbas, C. Dynamics of reference evapotranspiration in the Bolivian highlands (Altiplano). Agric. For. Meteorol. 2004, 125, 67–82. [Google Scholar] [CrossRef]
- Servicio Nacional de Meteorología e Hidrología del Perú (SENAMHI). Climas del Perú—Mapa de Clasificación Climática Nacional; Dirección de Meteorología y Evaluación Ambiental Atmosférica: Lima, Peru, 2020. [Google Scholar]
- Huerta, A.; Lavado, W. ATLAS Zonas Áridas Del Perú: Una Evaluación Presente Y Futura; SENAMHI: Lima, Peru, 2021. [Google Scholar]
- Lujano, A.; Sanchez-Delgado, M.; Lujano, E. Improvement of Hargreaves–Samani Reference Evapotranspiration Estimates in the Peruvian Altiplano. Water 2023, 15, 1410. [Google Scholar] [CrossRef]
- Vera, L.; Villegas, E.; Oria, C.; Arboleda, F. Control de Calidad de Datos de Estaciones Meteorológicas e Hidrológicas Automáticas en El Centro de Procesamiento de Datos Del SENAMHI; Servicio Nacional de Meteorología e Hidrología del Perú (SENAMHI): Lima, Peru, 2021. [Google Scholar]
- Chiew, F.H.; Siriwardena, L. Trend/Change Detection Software. USER GUIDE; CRC for Catchment Hydrology: Clayton, Australia, 2005. [Google Scholar]
- Van Buuren, S.; Groothuis-Oudshoorn, K. Mice: Multivariate imputation by chained equations in R. J. Stat. Softw. 2011, 45, 1–67. [Google Scholar]
- Woldesenbet, T.A.; Elagib, N.A.; Ribbe, L.; Heinrich, J. Gap filling and homogenization of climatological datasets in the headwater region of the Upper Blue Nile Basin, Ethiopia. Int. J. Climatol. 2017, 37, 2122–2140. [Google Scholar]
- Tomas-Burguera, M.; Vicente-Serrano, S.M.; Beguería, S.; Reig, F.; Latorre, B. Reference crop evapotranspiration database in Spain (1961–2014). Earth Syst. Sci. Data. 2019, 11, 1917–1930. [Google Scholar]
- Jarvis, A.; Reuter, H.I.; Nelson, A.; Guevara, E. Hole-Filled SRTM for the Globe Version 4. Available from the CGIAR-CSI SRTM 90m Database. 2008. Available online: http://srtm.csi.cgiar.org (accessed on 15 July 2022).
- Almorox, J.; Grieser, J. Calibration of the Hargreaves–Samani method for the calculation of reference evapotranspiration in different Köppen climate classes. Hydrol. Res. 2016, 47, 521–531. [Google Scholar]
- Ye, J.; Guo, A.; Sun, G. Statistical analysis of reference evapotranspiration on the Tibetan Plateau. J. Irrig. Drain. Eng. 2009, 135, 134–140. [Google Scholar]
- Legates, D.R.; McCabe, G.J. Evaluating the use of ‘goodness-of-fifit’ measures in hydrologic and hydroclimatic model validation. Water Resour. Res. 1999, 35, 233–241. [Google Scholar]
- Nash, J.E.; Sutcliffe, J.V. River flow forecasting through conceptual models part I—A discussion of principles. J. Hydrol. 1970, 10, 282–290. [Google Scholar]
- Moriasi, D.; Arnold, J.; Van Liew, M.; Bingner, R.; Harmel, R.; Veith, T. Model evaluation guidelines for systematic quantifification of accuracy in watershed simulations. Trans. ASABE 2007, 50, 885–900. [Google Scholar]
- Magaña-Hernández, F.; Bá, K.M.; Guerra-Cobián, V.H. Estimación del hidrograma de crecientes con modelación determinística y precipitación derivada de radar. Agrociencia 2013, 47, 739–752. [Google Scholar]
- Vu, T.T.; Li, L.; Jun, K.S. Evaluation of multi-satellite precipitation products for streamflow simulations: A case study for the Han River Basin in the Korean Peninsula, East Asia. Water 2018, 10, 642. [Google Scholar] [CrossRef]
- Moriasi, D.N.; Gitau, M.W.; Pai, N.; Daggupati, P. Hydrologic and water quality models: Performance measures and evaluation criteria. Trans. ASABE 2015, 58, 1763–1785. [Google Scholar]
- Willmott, C.J.; Matsuura, K. Advantages of the mean absolute error (MAE) over the root mean square error (RMSE) in assessing average model performance. Clim. Res. 2005, 30, 79–82. [Google Scholar] [CrossRef]
- Shiri, J.; Sadraddini, A.A.; Nazemi, A.H.; Marti, P.; Fard, A.F.; Kisi, O.; Landeras, G. Independent testing for assessing the calibration of the Hargreaves–Samani equation: New heuristic alternatives for Iran. Comput. Electron. Agric. 2015, 117, 70–80. [Google Scholar]
- Feng, Y.; Jia, Y.; Cui, N.; Zhao, L.; Li, C.; Gong, D. Calibration of Hargreaves model for reference evapotranspiration estimation in Sichuan basin of southwest China. Agric. Water Manag. 2017, 181, 1–9. [Google Scholar] [CrossRef]
- Lima, J.C.D.; Arraes, F.D.D.; Oliveira, J.B.D.; Nascimento, F.A.L.D.; Macêdo, K.G.D. Parametrização da equação de Hargreaves e Samani para estimativa da evapotranspiração de referência no Estado do Ceará, Brasil. Rev. Ciência Agron. 2016, 47, 447–454. [Google Scholar]
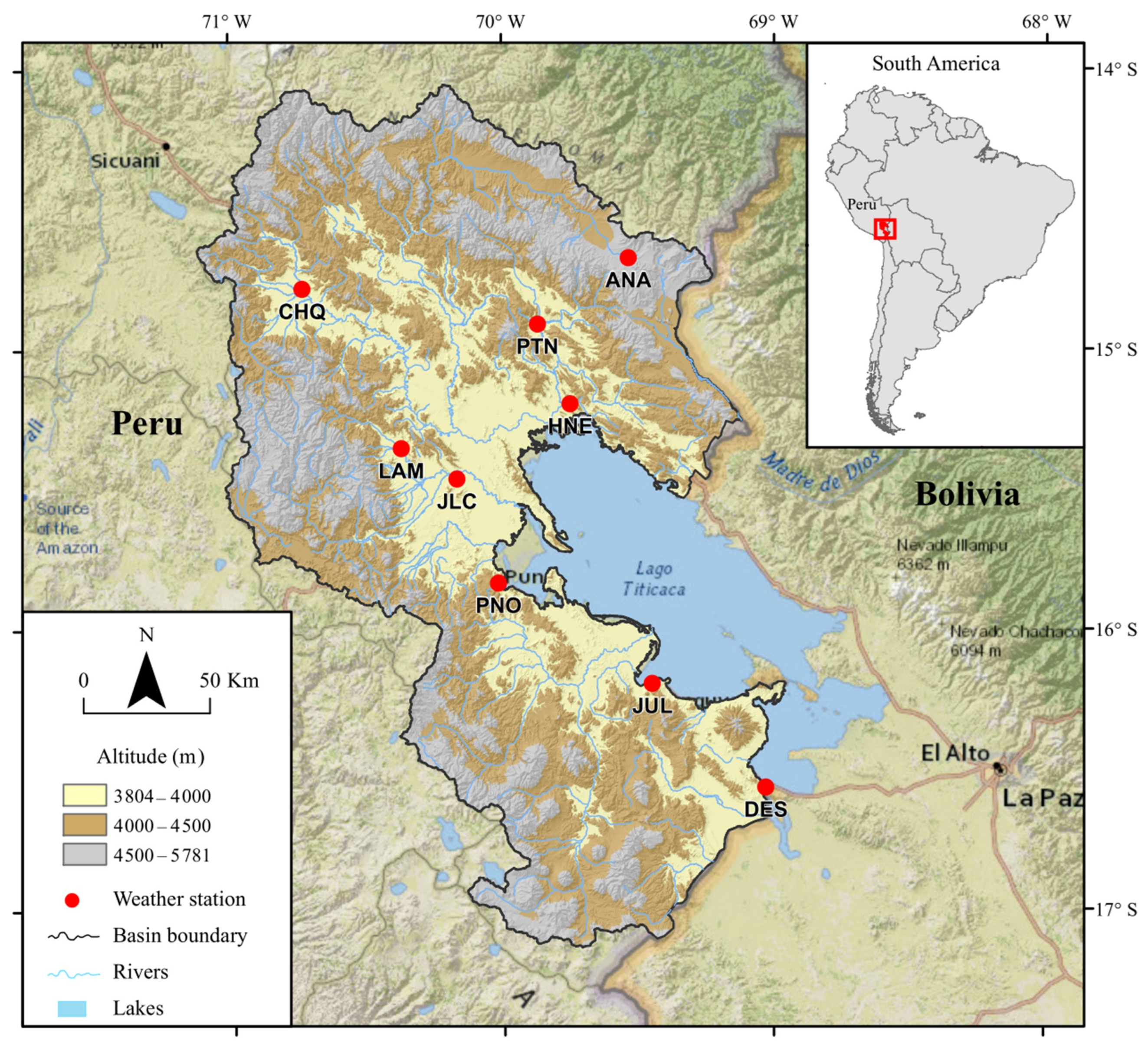

| Station | Lat. | Lon. | Alt. | Rh | U2 | Sh | ETo | P | AI | CC | ||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Ananea (ANA) | −14.676 | −69.534 | 4660 | 10.5 | −1.9 | 80.6 | 2.0 | 6.3 | 2.7 | 658.4 | 0.67 | Humid subhumid |
| Chuquibambilla (CHQ) | −14.788 | −70.728 | 3918 | 16.3 | −2.2 | 61.3 | 2.1 | 7.2 | 3.2 | 787.0 | 0.67 | Humid subhumid |
| Desaguadero (DES) | −16.563 | −69.037 | 3833 | 15.2 | 1.7 | 65.7 | 5.5 | 7.4 | 3.4 | 736.9 | 0.60 | Dry subhumid |
| Huancané (HNE) | −15.207 | −69.758 | 3840 | 15.7 | 0.3 | 58.9 | 2.9 | 7.7 | 3.4 | 650.5 | 0.52 | Dry subhumid |
| Juliaca (JLC) | −15.444 | −70.208 | 3838 | 17.8 | −0.5 | 75.0 | 1.5 | 7.8 | 3.2 | 624.9 | 0.53 | Dry subhumid |
| Juli (JUL) | −16.204 | −69.460 | 3830 | 14.1 | 3.0 | 58.3 | 2.4 | 8.4 | 3.5 | 948.3 | 0.78 | Humid subhumid |
| Lampa (LAM) | −15.361 | −70.374 | 3866 | 17.1 | −0.3 | 55.0 | 2.4 | 8.1 | 3.6 | 757.1 | 0.60 | Dry subhumid |
| Puno (PNO) | −15.826 | −70.012 | 3812 | 16.3 | 3.5 | 61.1 | 1.8 | 8.1 | 3.5 | 750.6 | 0.59 | Dry subhumid |
| Putina (PTN) | −14.921 | −69.876 | 3861 | 17.3 | 0.1 | 70.1 | 2.6 | 6.9 | 3.1 | 643.9 | 0.56 | Dry subhumid |
| Station | Original HS Equation (CH = 0.0023 y EH = 0.5) | Calibration HS Equation (Constant CT = 17.8) | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Calibrated | Calibration | Validation | ||||||||||||
| NSE | PBIAS | MAE | RMSE | CH | EH | NSE | PBIAS | MAE | RMSE | NSE | PBIAS | MAE | RMSE | |
| ANA | 0.44 | −5.20 | 0.19 | 0.22 | 0.0013 | 0.753 | 0.70 | −0.43 | 0.01 | 0.16 | 0.67 | 1.00 | 0.03 | 0.18 |
| CHQ | 0.53 | 7.30 | 0.25 | 0.31 | 0.0016 | 0.590 | 0.85 | −0.43 | 0.01 | 0.17 | 0.86 | −0.27 | 0.01 | 0.17 |
| DES | 0.62 | −8.30 | 0.29 | 0.33 | 0.0027 | 0.465 | 0.87 | −0.52 | 0.02 | 0.20 | 0.88 | −0.55 | 0.02 | 0.18 |
| HNE | 0.75 | −4.90 | 0.23 | 0.28 | 0.0019 | 0.597 | 0.86 | 0.04 | 0.00 | 0.20 | 0.86 | 1.37 | 0.05 | 0.21 |
| JLC | 0.44 | 11.80 | 0.38 | 0.41 | 0.0021 | 0.495 | 0.94 | −0.01 | 0.00 | 0.14 | 0.93 | 0.42 | 0.01 | 0.15 |
| JUL | −0.57 | −18.60 | 0.65 | 0.67 | 0.0022 | 0.608 | 0.92 | 0.02 | 0.00 | 0.15 | 0.92 | 0.55 | 0.02 | 0.15 |
| LAM | 0.87 | −1.90 | 0.16 | 0.20 | 0.0023 | 0.499 | 0.87 | −0.44 | 0.02 | 0.19 | 0.89 | 0.53 | 0.02 | 0.18 |
| PNO | 0.63 | −8.80 | 0.31 | 0.33 | 0.0022 | 0.552 | 0.94 | −0.19 | 0.01 | 0.13 | 0.94 | 0.20 | 0.01 | 0.13 |
| PTN | −0.35 | 12.70 | 0.41 | 0.49 | 0.0012 | 0.698 | 0.75 | −0.29 | 0.01 | 0.21 | 0.77 | −0.20 | 0.01 | 0.20 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lujano, A.; Sanchez-Delgado, M.; Montalvo-Arquiñigo, N.; Vasquez-Villanueva, A.; Mejia-Marcacuzco, A.; Lujano, E. Regionalization of the Hargreaves-Samani Coefficients to Estimate Reference Evapotranspiration in High-Altitude Areas. Atmosphere 2025, 16, 408. https://doi.org/10.3390/atmos16040408
Lujano A, Sanchez-Delgado M, Montalvo-Arquiñigo N, Vasquez-Villanueva A, Mejia-Marcacuzco A, Lujano E. Regionalization of the Hargreaves-Samani Coefficients to Estimate Reference Evapotranspiration in High-Altitude Areas. Atmosphere. 2025; 16(4):408. https://doi.org/10.3390/atmos16040408
Chicago/Turabian StyleLujano, Apolinario, Miguel Sanchez-Delgado, Nestor Montalvo-Arquiñigo, Absalon Vasquez-Villanueva, Abel Mejia-Marcacuzco, and Efrain Lujano. 2025. "Regionalization of the Hargreaves-Samani Coefficients to Estimate Reference Evapotranspiration in High-Altitude Areas" Atmosphere 16, no. 4: 408. https://doi.org/10.3390/atmos16040408
APA StyleLujano, A., Sanchez-Delgado, M., Montalvo-Arquiñigo, N., Vasquez-Villanueva, A., Mejia-Marcacuzco, A., & Lujano, E. (2025). Regionalization of the Hargreaves-Samani Coefficients to Estimate Reference Evapotranspiration in High-Altitude Areas. Atmosphere, 16(4), 408. https://doi.org/10.3390/atmos16040408








