An Analysis of Meteorological Anomalies in Kamchatka in Connection with the Seismic Process
Abstract
1. Introduction
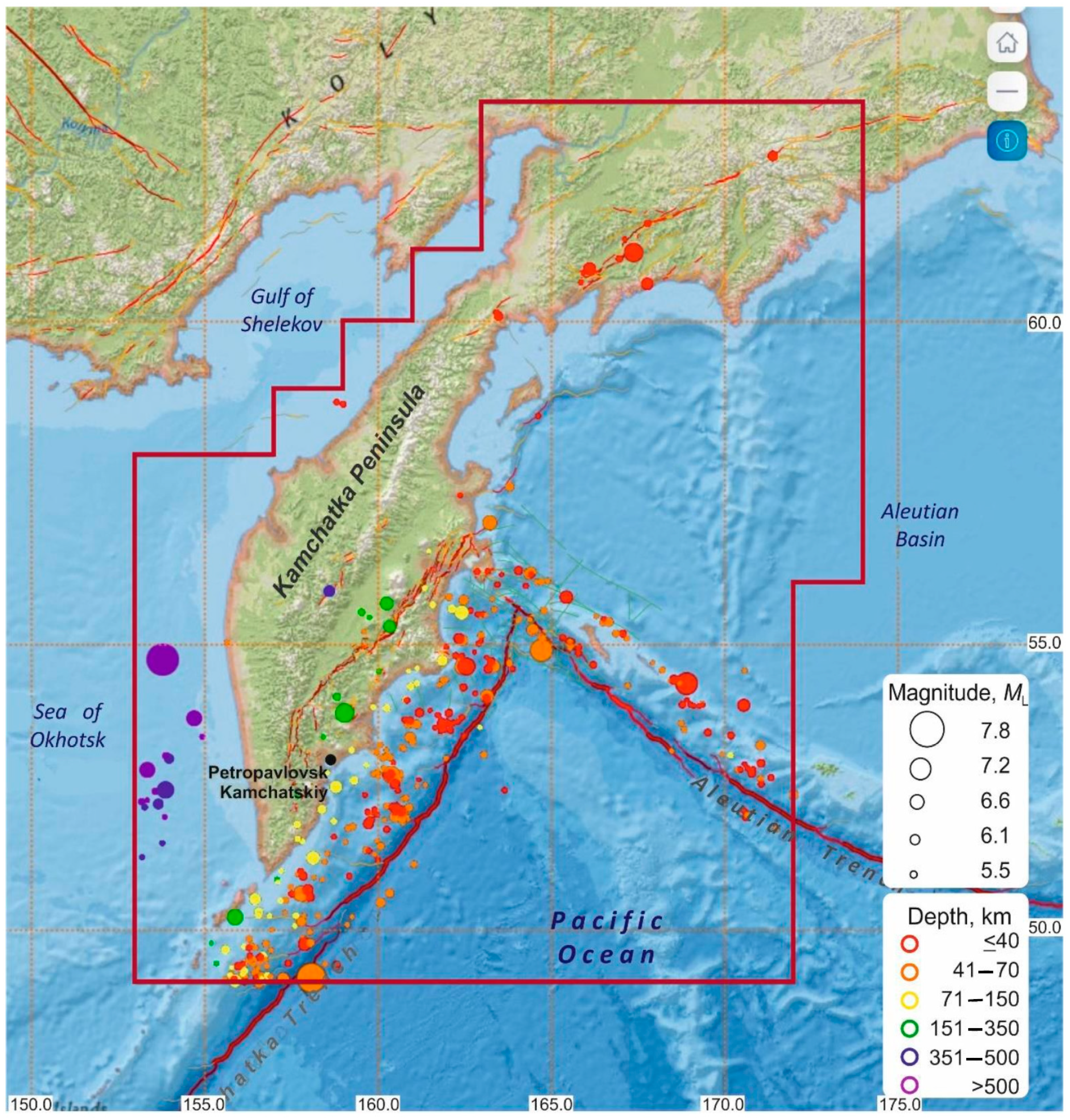
2. Initial Data
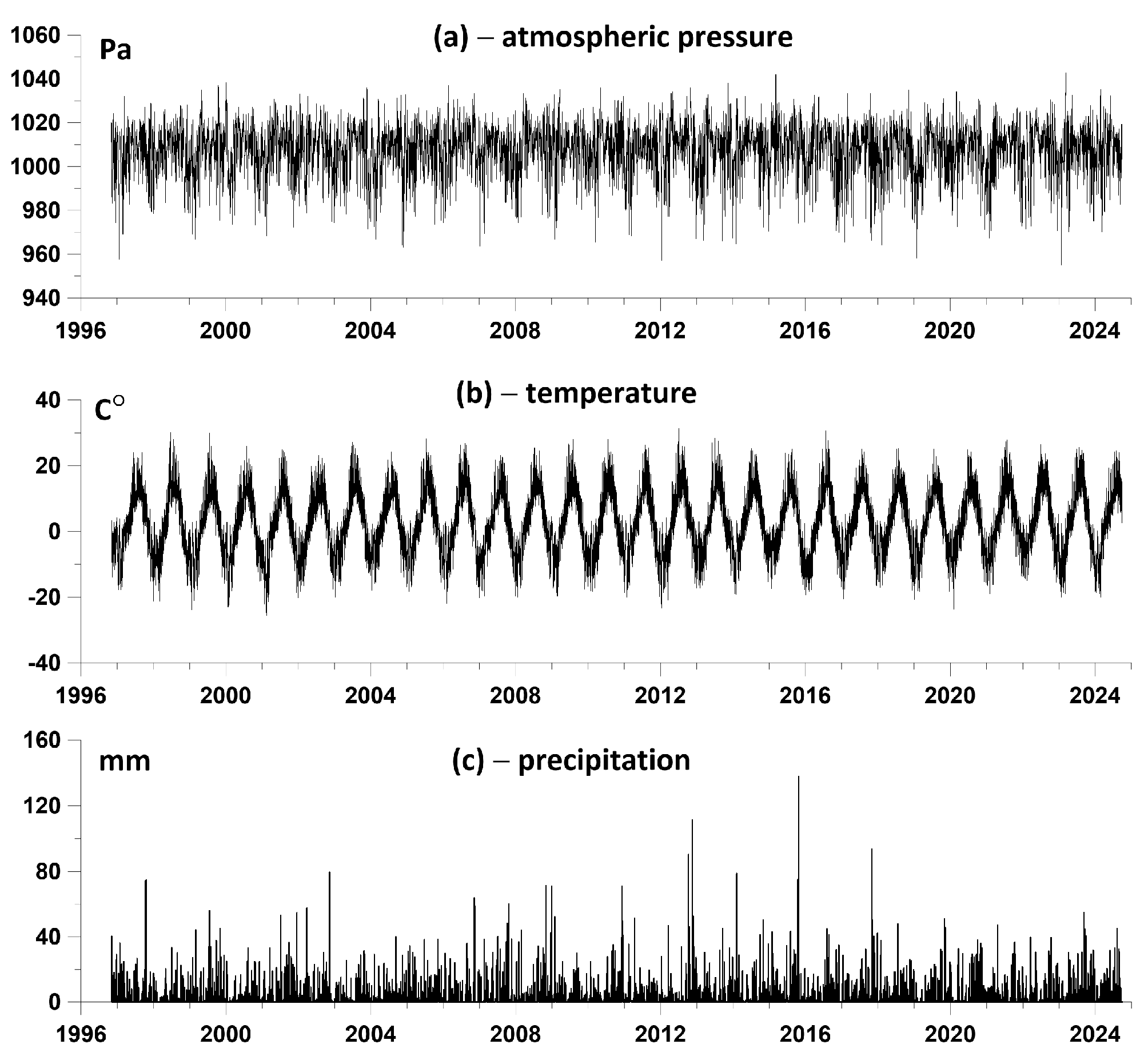
2.1. Meteorological Data
2.2. Earthquake Data
3. Research Method
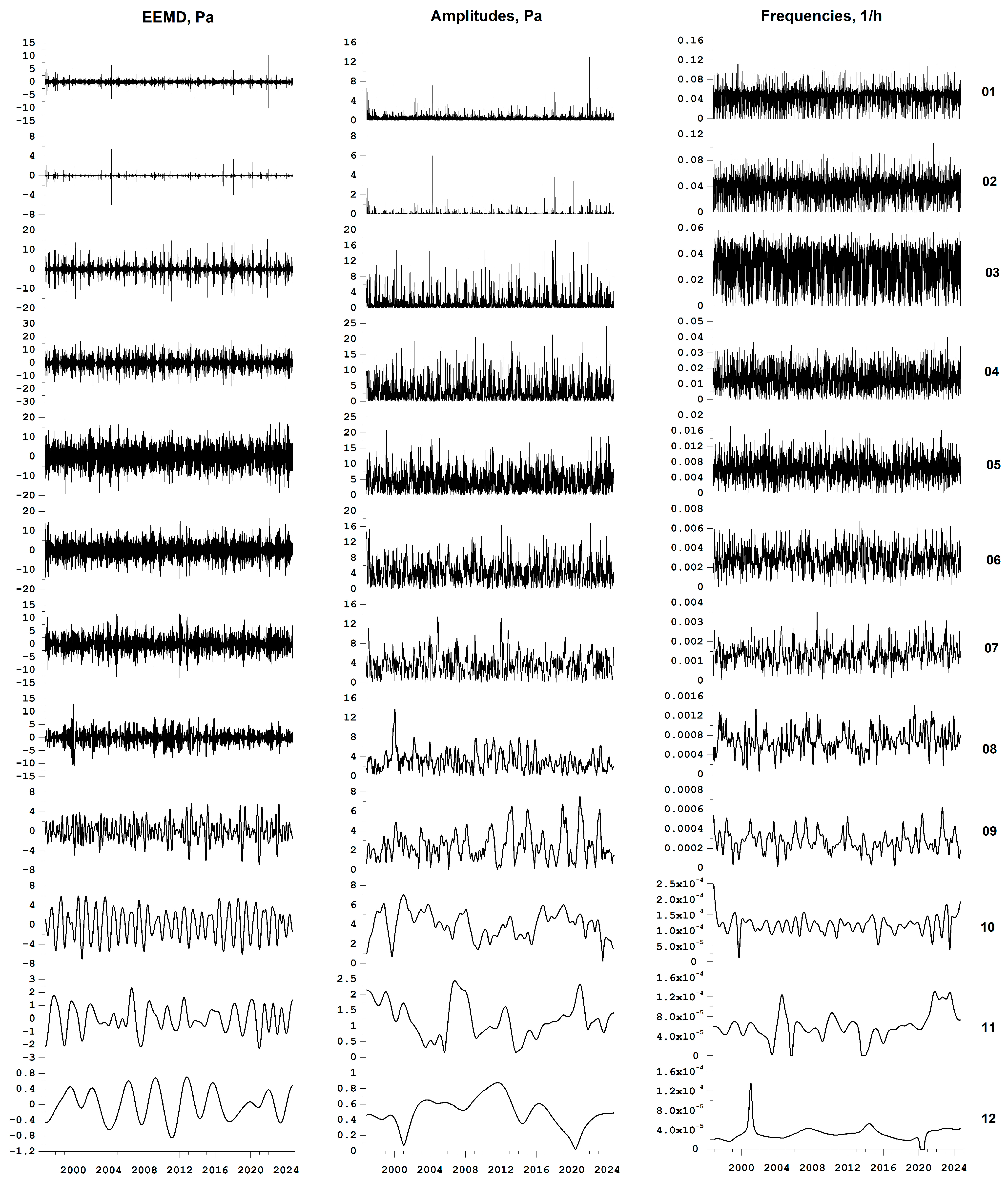
3.1. Empirical Mode Decomposition
3.2. Ensemble Empirical Mode Decomposition
- A white noise realization is added to the original data.
- The white noise-added data are decomposed into empirical modes.
- Steps 1 and 2 are repeated a large number of times for independent white noise realizations.
- All white-noise added empirical modes are averaged for each IMF level.
3.3. Hilbert Transform
3.4. Influence Matrix
- (1)
- A sequence of time moments corresponding to the largest local maxima of the envelope amplitudes at some IMF levels of the EEMD decomposition;
- (2)
- The sequence of times of seismic events with a magnitude not less than a given value (in our case, with a magnitude of ML ≥ 5.5).
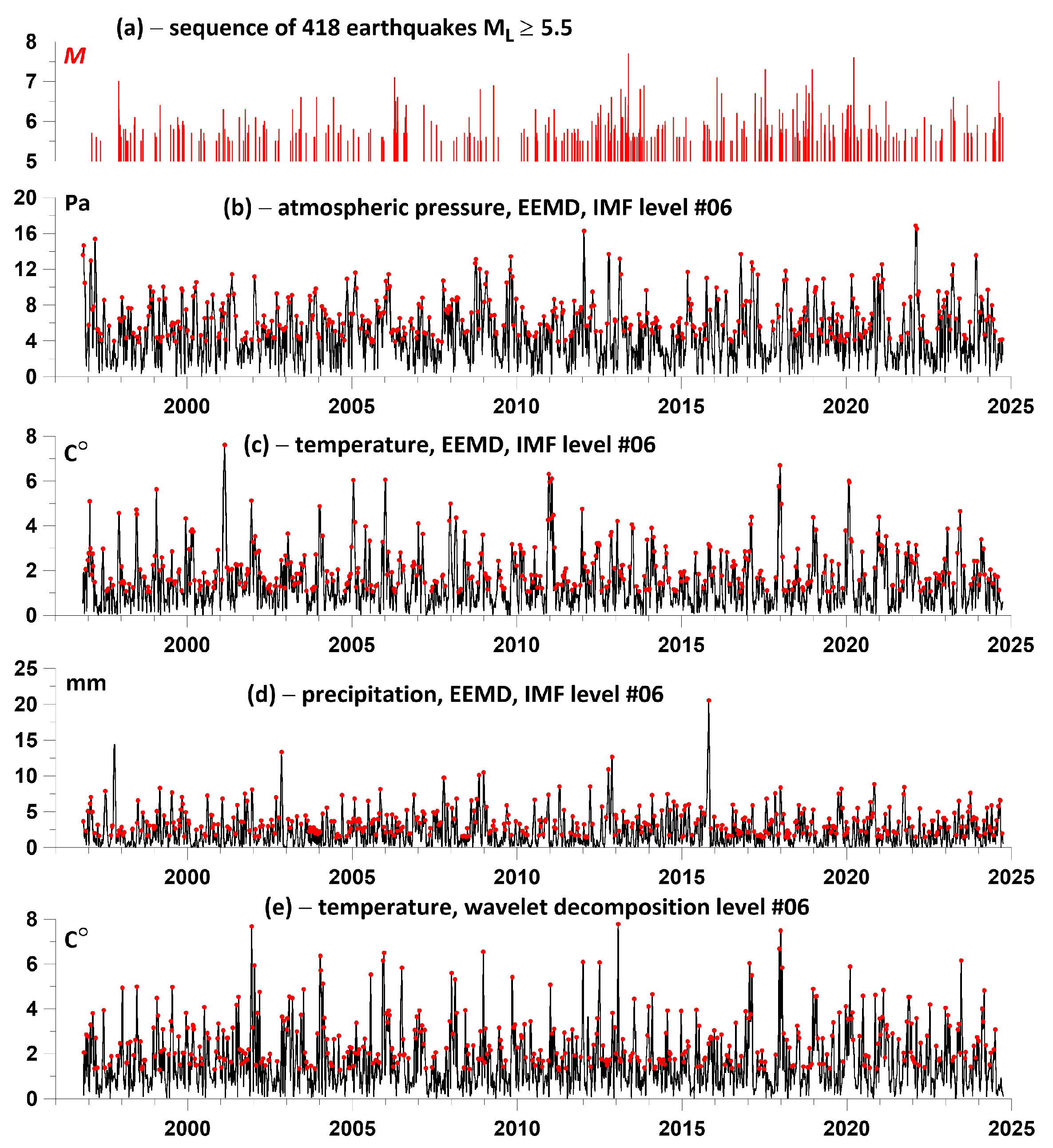
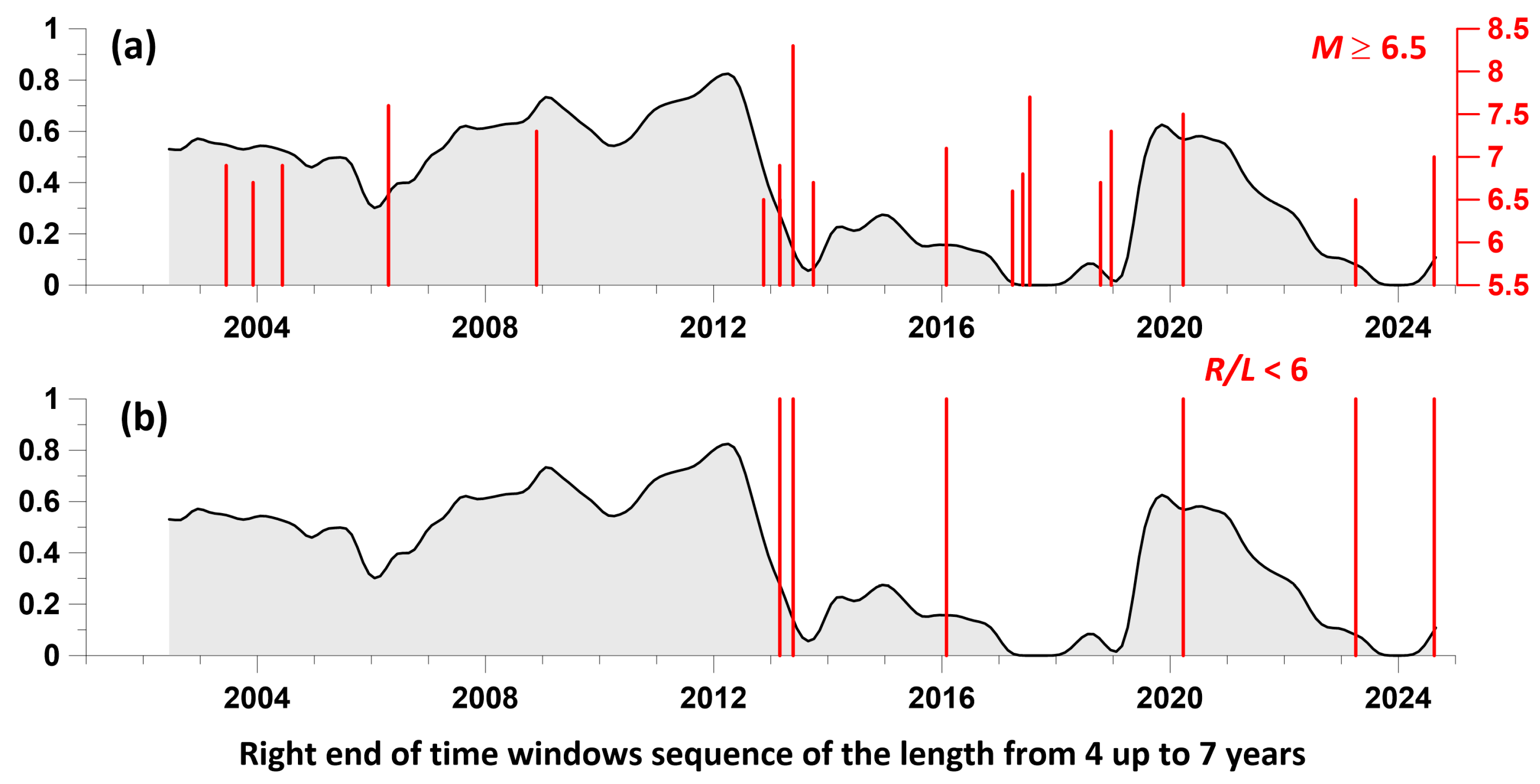
3.5. Relationship Between Local Maxima of the Instantaneous Amplitudes of Meteorological Parameters and Seismic Events
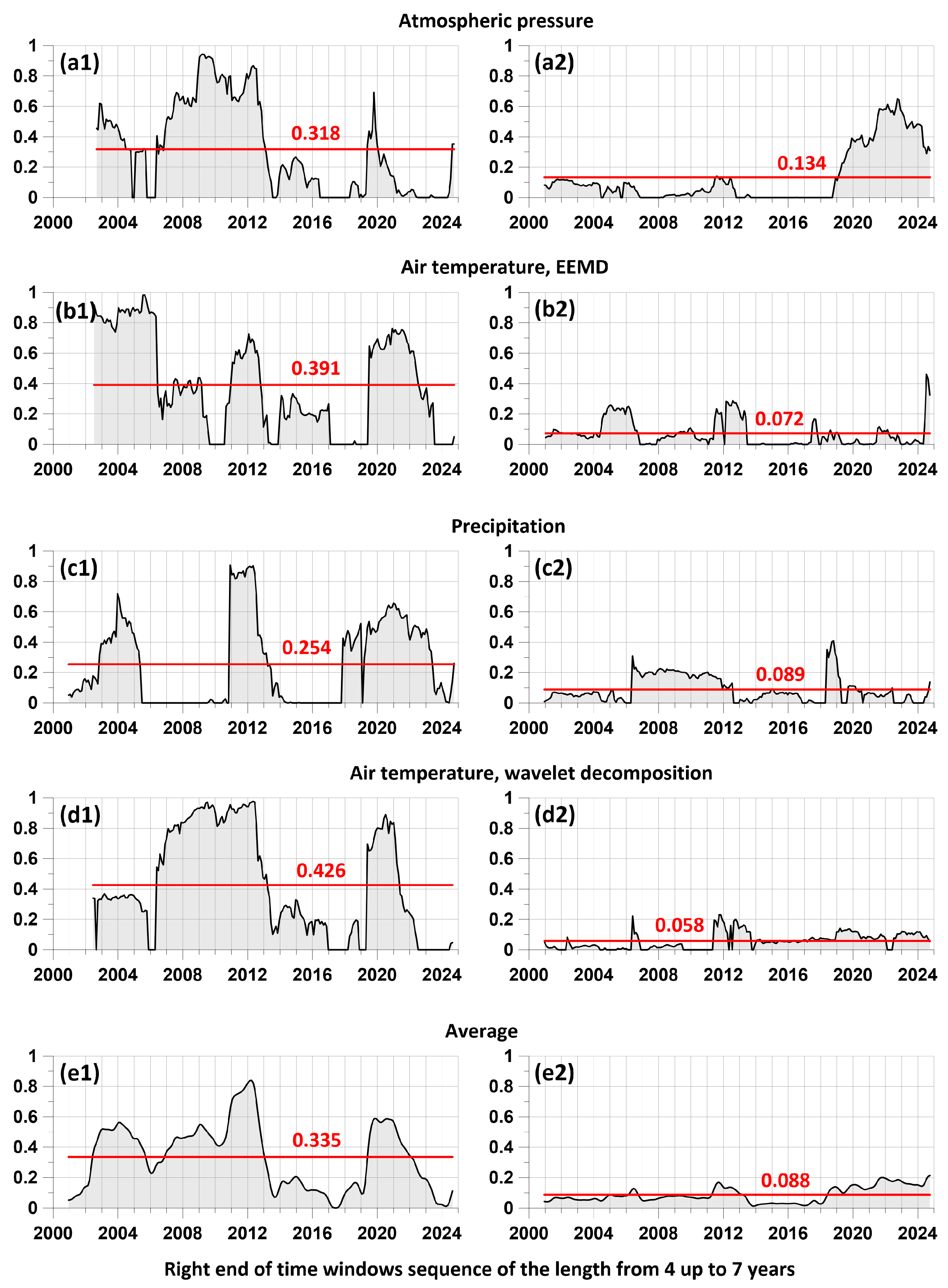
4. Average Values of the Components of the Influence Matrices
5. Discussion
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Dunajecka, M.A.; Pulinets, S.A. Atmospheric and thermal anomalies observed around the time of strong earthquakes in Mexico. Atmosfera 2005, 18, 235–247. [Google Scholar]
- Genzano, N.; Filizzaola, C.; Hattori, K.; Pergola, N.; Tramutoli, V. Statistical correlation analysis between thermal infrared anomalies observed from MTSATs and large earthquakes occurred in Japan (2005–2015). J. Geophys. Res. Solid Earth 2021, 126, e2020JB020108. [Google Scholar] [CrossRef]
- Hayakawa, M.; Izutsu, J.; Schekotov, A.; Yang, S.-S.; Solovieva, M.; Budilova, E. Lithosphere-atmosphere-ionosphere coupling effects based on multiparameter precursor observations for February–March 2021 earthquakes (M~7) in the offshore of Tohoku area of Japan. Geosciences 2021, 11, 481. [Google Scholar] [CrossRef]
- Pulinets, S.A.; Boyarchuk, K.A. Ionospheric Precursors of Earthquakes; Springer: Berlin/Heidelberg, Germany, 2004; p. 288. [Google Scholar]
- Ouzounov, D.; Pulinets, S.; Kafatos, M.C.; Taylor, P. Thermal Radiation Anomalies Associated with Major Earthquakes. In Pre-Earthquakes Processes: A Multidisciplinary Approach to Earthquake Prediction Studies, AGU Geophysical Monograph Series; John Wiley & Sons Inc.: New York, NY, USA, 2018; Volume 234, pp. 259–274. [Google Scholar] [CrossRef]
- Pulinets, S.; Ouzounov, D. Lithosphere–Atmosphere–Ionosphere Coupling (LAIC) model—An unified concept for earthquake precursors validation. J. Asian Earth Sci. 2011, 41, 371–382. [Google Scholar] [CrossRef]
- Ouzounov, D.; Pulinets, S.; Kafatos, M.C.; Taylor, P. (Eds.) Pre-Earthquakes Processes: A Multidisciplinary Approach to Earthquake Prediction Studies, AGU Geophysical Monograph Series; John Wiley & Sons Inc.: New York, NY, USA, 2018; Volume 234. [Google Scholar] [CrossRef]
- Shitov, A.V.; Pulinets, S.A.; Budnikov, P.A. Effect of Earthquake Preparation on Changes in Meteorological Characteristics (Based on the Example of the 2003 Chuya Earthquake). Geomagn. Aeron. 2023, 63, 395–408. [Google Scholar] [CrossRef]
- Cicerone, R.D.; Ebel, J.E.; Beitton, J.A. Systematic compilation of earthquake precursors. Tectonophysics 2009, 476, 371–396. [Google Scholar] [CrossRef]
- Pulinets, S.A.; Ouzounov, D.P.; Karelin, A.V.; Davidenko, D.V. Physical bases of the generation of short-term earthquake precursors: A complex model of ionization-induced geophysical processes in the lithosphere-atmosphere-ionosphere-magnetosphere system. Geomagn. Aeron. 2015, 55, 521–538. [Google Scholar] [CrossRef]
- Kuo, C.L.; Lee, L.C.; Huba, J.D. An Improved Coupling Model for the Lithosphere-Atmosphere-Ionosphere System. J. Geophys. Res. Space Phys. 2014, 119, 3189–3205. [Google Scholar] [CrossRef]
- Lukianova, R.; Daurbayeva, G.; Siylkanova, A. Ionospheric and Meteorological Anomalies Associated with the Earthquake in Central Asia on 22 January 2024. Remote Sens. 2024, 16, 3112. [Google Scholar] [CrossRef]
- Lukianova, R.; Christiansen, F. Modeling of the global distribution of ionospheric electric fields based on realistic maps of field-aligned currents. J. Geophys. Res. 2006, 111, A03213. [Google Scholar] [CrossRef]
- Schekotov, A.; Borovleva, K.; Pilipenko, V.; Chebrov, D.; Hayakawa, M. Meteorological Response of Kamchatka Seismicity. In Springer Proceedings in Earth and Environmental Sciences, Proceedings of the Problems of Geocosmos—2022 ICS 2022, St. Petersburg, Russia, 3–7 October 2022; Kosterov, A., Lyskova, E., Mironova, I., Apatenkov, S., Baranov, S., Eds.; Springer: Cham, Switzerland, 2023. [Google Scholar] [CrossRef]
- Uyeda, S.; Nagao, T.; Hattori, K.; Hayakawa, M.; Miyaki, K.; Molchanov, O.; Gladychev, V.; Baransky, L.; Chtchekotov, A.; Fedorov, E.; et al. Geophysical Observatory in Kamchatka region for monitoring of phenomena connected with seismic activity. Nat. Hazards Earth Syst. Sci. 2001, 1, 3–7. [Google Scholar] [CrossRef]
- Konovalova, A.A.; Saltykov, V.A. Seismic Precursors of Large (M 6.0) Earthquakes in the Junction Zone between the Kuril-Kamchatka and Aleutian Island Arcs. J. Volcanol. Seismol. 2023, 17, 60–77. [Google Scholar] [CrossRef]
- Gavrilov, V.A.; Panteleev, I.A.; Deshcherevskii, A.V.; Lander, A.V.; Morozova, Y.V.; Buss, Y.Y.; Vlasov, Y.A. Stress-Strain State Monitoring of the Geological Medium Based on The Multi-instrumental Measurements in Boreholes: Experience of Research at the Petropavlovsk-Kamchatskii Geodynamic Testing Site (Kamchatka, Russia). Pure Appl. Geophys. 2020, 177, 397–419. [Google Scholar] [CrossRef]
- Kopylova, G.; Boldina, S. Hydrogeological Earthquake Precursors: A Case Study from the Kamchatka Peninsula. Front. Earth Sci. 2020, 8, 576017. [Google Scholar] [CrossRef]
- Kopylova, G.N.; Serafimova, Y.K.; Lyubushin, A.A. Meteorological Anomalies and Strong Earthquakes: A Case Study of the Petropavlovsk-Kamchatsky Region, Kamchatka Peninsula. Izv. Phys. Solid Earth 2024, 60, 494–507. [Google Scholar] [CrossRef]
- Kopylova, G.N.; Ivanov, V.Y.; Kasimova, V.A. The implementation of information system elements for interpreting integrated geophysical observations in Kamchatka. Russ. J. Earth Sci. 2009, 11, ES1006. [Google Scholar] [CrossRef]
- Chebrova, A.Y.; Chemarev, A.S.; Matveenko, E.A.; Chebrov, D.V. Seismological data information system in Kamchatka branch of GS RAS: Organization principles, main elements and key functions. Geophys. Res. 2020, 21, 66–91. [Google Scholar] [CrossRef]
- Chubarova, O.S.; Gusev, A.A.; Chebrov, V.N. The ground motion excited by the Olyutorskii earthquake of April 20, 2006 and by its aftershocks based on digital recordings. J. Volcanol. Seismol. 2010, 4, 126–138. [Google Scholar] [CrossRef]
- Zelenin, E.; Bachmanov, D.; Garipova, S.; Trifonov, V.; Kozhurin, A. The Active Faults of Eurasia Database (AFEAD): The ontology and design behind the continental-scale dataset. Earth Syst. Sci. Data 2022, 14, 4489–4503. [Google Scholar] [CrossRef]
- Zavyalov, A.D.; Zotov, O.D. A new way to determine the characteristic size of the source zone. J. Volcanol. Seismol. 2021, 15, 19–25. [Google Scholar] [CrossRef]
- Huang, N.E.; Shen, Z.; Long, S.R.; Wu, V.C.; Shih, H.H.; Zheng, Q.; Yen, N.C.; Tung, C.C.; Liv, H.H. The empirical mode decomposition and the Hilbert spectrum for nonlinear and non-stationary time series analysis. Proc. Roy. Soc. Lond. Ser. A 1998, 454, 903–995. [Google Scholar] [CrossRef]
- Huang, N.E.; Wu, Z. A review on Hilbert-Huang transform: Method and its applications to geophysical studies. Rev. Geophys. 2008, 46, RG2006. [Google Scholar] [CrossRef]
- Bendat, J.S.; Piersol, A.G. Random Data. Analysis and Measurement Procedures, 4th ed.; Wiley & Sons: Hoboken, NJ, USA, 2010. [Google Scholar]
- Mallat, S.A. Wavelet Tour of Signal Processing, 2nd ed.; Academic Press: Cambridge, MA, USA, 1999. [Google Scholar]
- Huang, N.E.; Wu, M.; Qu, W.; Long, S.R.; Shen, S.S.P. Applications of Hilbert-Huang transform to non-stationary financial time series analysis. Appl. Stoch. Models Bus. Ind. 2003, 19, 245–268. [Google Scholar] [CrossRef]
- Huang, Y.; Schmitt, F.G.; Lu, Z.; Liu, Y. Analysis of daily river flow fluctuations using empirical mode decomposition and arbitrary order Hilbert spectral analysis. J. Hydrol. 2009, 373, 103–111. [Google Scholar] [CrossRef]
- Li, H.; Kwong, S.; Yang, L.; Huang, D.; Xiao, D. Hilbert-Huang Transform for Analysis of Heart Rate Variability in Cardiac Health. IEEE/ACM Trans. Comput. Biol. Bioinform. 2011, 8, 1557–1567. [Google Scholar] [CrossRef]
- Wei, H.-C.; Xiao, M.-X.; Chen, H.-Y.; Li, Y.-Q.; Wu, H.-T.; Sun, C.-K. Instantaneous frequency from Hilbert-Huang transformation of digital volume pulse as indicator of diabetes and arterial stiffness in upper-middle-aged subjects. Sci. Rep. 2018, 8, 15771. [Google Scholar] [CrossRef]
- Li, W.; Guo, J. Extraction of periodic signals in Global Navigation Satellite System (GNSS) vertical coordinate time series using the adaptive ensemble empirical modal decomposition method. Nonlin. Process. Geophys. 2024, 31, 99–113. [Google Scholar] [CrossRef]
- Pan, Y.; Shen, W.-B.; Ding, H.; Hwang, C.; Li, J.; Zhang, T. The Quasi-Biennial Vertical Oscillations at Global GPS Stations: Identification by Ensemble Empirical Mode Decomposition. Sensors 2015, 15, 26096–26114. [Google Scholar] [CrossRef]
- Pan, Z. Tornado Occurrence in the United States as Modulated by Multidecadal Oceanic Oscillations Using Empirical Model Decomposition. Atmosphere 2024, 15, 1257. [Google Scholar] [CrossRef]
- Lyubushin, A.; Rodionov, E. Prognostic Properties of Instantaneous Amplitudes Maxima of Earth Surface Tremor. Entropy 2024, 26, 710. [Google Scholar] [CrossRef]
- Lyubushin, A. Investigation of the Global Seismic Noise Properties in Connection to Strong Earthquakes. Front. Earth Sci. 2022, 10, 905663. [Google Scholar] [CrossRef]
- Lyubushin, A.; Rodionov, E. Wavelet-based correlations of the global magnetic field in connection to strongest earthquakes. Adv. Space Res. 2024, 74, 3496–3510. [Google Scholar] [CrossRef]
- Cox, D.R.; Lewis, P.A.W. The Statistical Analysis of Series of Events; Methuen: London, UK, 1966. [Google Scholar]
- Daley, D.J.; Vere-Jones, D. An Introduction to the Theory of Point Processes, Volume I: Elementary Theory and Methods, 2nd ed.; Springer: New York, NY, USA, 2003. [Google Scholar]
- Hawkes, A.G. Spectra of some self-exciting and mutually exciting point processes. Biometrika 1971, 58, 83–90. [Google Scholar] [CrossRef]
- Laub, P.J.; Lee, Y.; Taimre, T. The Elements of Hawkes Processes; Springer International Publishing: Cham, Switzerland, 2021. [Google Scholar] [CrossRef]



| Date dd.mm.yyyy | Time hh:mm:ss | Latitude, N° | Longitude, E° | Depth, km | ML | R, km | MW NEIC | L, km | R/L |
|---|---|---|---|---|---|---|---|---|---|
| 16.06.2003 | 22:08:02 | 55.30 | 160.34 | 190 | 6.6 | 331 | 6.9 | 50 | 6.7 |
| 05.12.2003 | 21:26:14 | 55.78 | 165.43 | 29 | 6.7 | 533 | 6.7 | 41 | 13.1 |
| 10.06.2004 | 15:19:55 | 55.68 | 160.25 | 208 | 6.7 | 370 | 6.9 | 50 | 7.4 |
| 20.04.2006 | 23:24:28 | 60.98 | 167.37 | 1 | 7.1 | 1019 | 7.6 | 100 | 10.2 |
| 24.11.2008 | 9:02:52 | 53.77 | 154.69 | 564 | 6.8 | 623 | 7.3 | 74 | 8.4 |
| 16.11.2012 | 18:12:39 | 49.06 | 155.87 | 78 | 6.7 | 486 | 6.5 | 33 | 14.5 |
| 28.02.2013 | 1:05:48 | 50.67 | 157.77 | 61 | 6.8 | 271 | 6.9 | 50 | 5.4 |
| 01.03.2013 | 13:20:49 | 50.64 | 157.90 | 62 | 6.8 | 272 | 6.5 | 33 | 8.1 |
| 24.05.2013 | 5:44:47 | 54.75 | 153.79 | 630 | 7.8 | 726 | 8.3 | 199 | 3.6 |
| 01.10.2013 | 3:38:19 | 52.88 | 153.34 | 608 | 6.8 | 700 | 6.7 | 41 | 17.1 |
| 30.01.2016 | 3:25:08 | 53.85 | 159.04 | 178 | 7.1 | 200 | 7.2 | 67 | 3.0 |
| 29.03.2017 | 4:09:22 | 56.97 | 163.22 | 43 | 6.8 | 521 | 6.6 | 37 | 14.1 |
| 02.06.2017 | 22:24:47 | 53.99 | 170.55 | 32 | 6.6 | 795 | 6.8 | 45 | 17.6 |
| 17.07.2017 | 23:34:08 | 54.35 | 168.90 | 7 | 7.3 | 692 | 7.7 | 110 | 6.3 |
| 13.10.2018 | 11:10:20 | 52.53 | 153.87 | 499 | 7.0 | 592 | 6.7 | 41 | 14.5 |
| 20.12.2018 | 17:01:54 | 54.91 | 164.71 | 54 | 7.3 | 448 | 7.3 | 74 | 6.1 |
| 25.03.2020 | 2:49:20 | 49.11 | 158.08 | 48 | 7.7 | 438 | 7.5 | 90 | 4.9 |
| 03.04.2023 | 3:06:56 | 52.58 | 158.78 | 105 | 6.6 | 120 | 6.5 | 33 | 3.6 |
| 17.08.2024 | 19:10:25 | 52.79 | 160.38 | 43 | 7.0 | 126 | 7.0 | 55 | 2.3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Lyubushin, A.; Kopylova, G.; Rodionov, E.; Serafimova, Y. An Analysis of Meteorological Anomalies in Kamchatka in Connection with the Seismic Process. Atmosphere 2025, 16, 78. https://doi.org/10.3390/atmos16010078
Lyubushin A, Kopylova G, Rodionov E, Serafimova Y. An Analysis of Meteorological Anomalies in Kamchatka in Connection with the Seismic Process. Atmosphere. 2025; 16(1):78. https://doi.org/10.3390/atmos16010078
Chicago/Turabian StyleLyubushin, Alexey, Galina Kopylova, Eugeny Rodionov, and Yulia Serafimova. 2025. "An Analysis of Meteorological Anomalies in Kamchatka in Connection with the Seismic Process" Atmosphere 16, no. 1: 78. https://doi.org/10.3390/atmos16010078
APA StyleLyubushin, A., Kopylova, G., Rodionov, E., & Serafimova, Y. (2025). An Analysis of Meteorological Anomalies in Kamchatka in Connection with the Seismic Process. Atmosphere, 16(1), 78. https://doi.org/10.3390/atmos16010078







