Impact of Polar Vortex Modes on Winter Weather Patterns in the Northern Hemisphere
Abstract
1. Introduction
2. Data and Methods
2.1. ERA5 Reanalysis
2.2. Classification of Winters in M22
2.3. Calculating the NAM Indices
2.4. Statistical Significance Assessment: Wilcoxon Signed-Rank Test
2.5. Ural and Aleutian Blocking Regions
2.6. The Eliassen–Palm Flux
3. Results
3.1. Northern Annular Mode Indices: Stratosphere–Troposphere Coupling
3.2. MSLP Anomalies and Regional Blocking
3.2.1. Monthly MSLP Anomalies for Stratospheric Modes
3.2.2. Lead–Lag Correlations between Stratospheric Modes and Regional Blocking
3.3. Eliassen–Palm Flux Analysis of Planetary Wave Dynamics
4. Discussion
5. Conclusions
- As precursors, the January and Double modes present Ural blocking and Aleutian trough events in December and November, respectively, associated with an upward–equatorward wave-1 propagation, while the February and Dynamical modes display Ural trough and Aleutian blocking events during this period, associated with a downward–poleward wave-1 propagation. Specifically, the Radiative mode exhibits a significant Aleutian blocking event as a precursor in November and December.
- In the pre-warming phases, the January and Double modes display upward–equatorward wave-1 and downward–poleward wave-2 propagations, while the February and Dynamical modes show upward–equatorward wave-1 and wave-2 propagations.
- During warming, the January and Double modes exhibit downward and poleward wave-1 and wave-2 propagations, associated with negative AO-like responses, while the February mode displays downward–poleward wave-1 and an upward–equatorward wave-2 propagations without generating particular tropospheric responses.
- In the recovery phase, the February mode displays a downward–poleward propagation of both wave-1 and wave-2, which is associated with a slight surface impact outside the Ural and Aleutian regions.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
- January single-warming mode: 1950/1951, 1952/1953, 1954/1955, 1959/1960, 1967/1968, 1969/1970, 1970/1971, 1976/1977, 1984/1985, 1997/1998, 2001/2002, 2002/2003, 2003/2004, 2005/2006, 2011/2012, 2012/2013, 2018/2019
- February single-warming mode: 1956/1957, 1957/1958, 1962/1963, 1972/1973, 1978/ 1979, 1980/1981, 1982/1983, 1986/1987, 1988/1989, 1989/1990, 1990/1991, 1994/1995, 2007/2008, 2008/2009, 2009/2010, 2016/2017, 2017/2018
- Double warmings mode: 1951/1952, 1965/1966, 1968/1969, 1979/1980, 1987/1988, 1998/1999, 2000/2001
- Dynamical final warming mode: 1955/1956, 1958/1959, 1960/1961, 1963/1964, 1973/ 1974, 1974/1975, 1975/1976, 1985/1986, 1992/1993, 1995/1996, 1999/2000, 2010/2011, 2013/2014, 2014/2015, 2015/2016
- Radiative final warming mode: 1961/1962, 1964/1965, 1966/1967, 1996/1997, 2019/ 2020
- Unclassified winters: 1953/1954, 1971/1972, 1977/1978, 1981/1982, 1983/1984, 1991/ 1992, 1993/1994, 2004/2005, 2006/2007
References
- Sigmond, M.; Scinocca, J.; Kharin, V.; Shepherd, T. Enhanced seasonal forecast skill following stratospheric sudden warmings. Nat. Geosci. 2013, 6, 98–102. [Google Scholar] [CrossRef]
- Domeisen, D.I.; Butler, A.H.; Charlton-Perez, A.J.; Ayarzagüena, B.; Baldwin, M.P.; Dunn-Sigouin, E.; Furtado, J.C.; Garfinkel, C.I.; Hitchcock, P.; Karpechko, A.Y.; et al. The Role of the Stratosphere in Subseasonal to Seasonal Prediction: 1. Predictability of the Stratosphere. J. Geophys. Res. Atmos. 2020, 125, e2019JD030920. [Google Scholar] [CrossRef]
- Waugh, D.W.; Sobel, A.H.; Polvani, L.M. What Is the Polar Vortex and How Does It Influence Weather? Bull. Am. Meteorol. Soc. 2017, 98, 37–44. [Google Scholar] [CrossRef]
- Matsuno, T. A Dynamical Model of the Stratospheric Sudden Warming. J. Atmos. Sci. 1971, 28, 1479–1494. [Google Scholar] [CrossRef]
- Butler, A.H.; Sjoberg, J.P.; Seidel, D.J.; Rosenlof, K.H. A sudden stratospheric warming compendium. Earth Syst. Sci. Data 2017, 9, 63–76. [Google Scholar] [CrossRef]
- Baldwin, M.P.; Dunkerton, T.J. Stratospheric Harbingers of Anomalous Weather Regimes. Science 2001, 294, 581–584. [Google Scholar] [CrossRef]
- Baldwin, M.P.; Thompson, D.W. A critical comparison of stratosphere–troposphere coupling indices. Q. J. R. Meteorol. Soc. 2009, 135, 1661–1672. [Google Scholar] [CrossRef]
- Thompson, D.W.J.; Wallace, J.M. Regional Climate Impacts of the Northern Hemisphere Annular Mode. Science 2001, 293, 85–89. [Google Scholar] [CrossRef]
- Butler, A.H.; Domeisen, D.I.V. The wave geometry of final stratospheric warming events. Weather Clim. Dyn. 2021, 2, 453–474. [Google Scholar] [CrossRef]
- Butler, A.H.; Seidel, D.J.; Hardiman, S.C.; Butchart, N.; Birner, T.; Match, A. Defining Sudden Stratospheric Warmings. Bull. Am. Meteorol. Soc. 2015, 96, 1913–1928. [Google Scholar] [CrossRef]
- Butler, A.H.; Gerber, E.P. Optimizing the Definition of a Sudden Stratospheric Warming. J. Clim. 2018, 31, 2337–2344. [Google Scholar] [CrossRef]
- Charlton, A.J.; Polvani, L.M. A New Look at Stratospheric Sudden Warmings. Part I: Climatology and Modeling Benchmarks. J. Clim. 2007, 20, 449–469. [Google Scholar] [CrossRef]
- Cohen, J.; Jones, J. Tropospheric Precursors and Stratospheric Warmings. J. Clim. 2011, 24, 6562–6572. [Google Scholar] [CrossRef]
- Seviour, W.J.M.; Mitchell, D.M.; Gray, L.J. A practical method to identify displaced and split stratospheric polar vortex events. Geophys. Res. Lett. 2013, 40, 5268–5273. [Google Scholar] [CrossRef]
- Mitchell, D.M.; Gray, L.J.; Anstey, J.; Baldwin, M.P.; Charlton-Perez, A.J. The Influence of Stratospheric Vortex Displacements and Splits on Surface Climate. J. Clim. 2013, 26, 2668–2682. [Google Scholar] [CrossRef]
- Nakagawa, K.I.; Yamazaki, K. What kind of stratospheric sudden warming propagates to the troposphere? Geophys. Res. Lett. 2006, 33, L04801. [Google Scholar] [CrossRef]
- Lehtonen, I.; Karpechko, A.Y. Observed and modeled tropospheric cold anomalies associated with sudden stratospheric warmings. J. Geophys. Res. Atmos. 2016, 121, 1591–1610. [Google Scholar] [CrossRef]
- Maycock, A.C.; Hitchcock, P. Do split and displacement sudden stratospheric warmings have different annular mode signatures? Geophys. Res. Lett. 2015, 42, 10,943–10,951. [Google Scholar] [CrossRef]
- Ayarzagüena, B.; Langematz, U.; Serrano, E. Tropospheric forcing of the stratosphere: A comparative study of the two different major stratospheric warmings in 2009 and 2010. J. Geophys. Res. Atmos. 2011, 116, D18114. [Google Scholar] [CrossRef]
- Kodera, K.; Funatsu, B.M.; Claud, C.; Eguchi, N. The role of convective overshooting clouds in tropical stratosphere–troposphere dynamical coupling. Atmos. Chem. Phys. 2015, 15, 6767–6774. [Google Scholar] [CrossRef]
- Bancalá, S.; Krüger, K.; Giorgetta, M. The preconditioning of major sudden stratospheric warmings. J. Geophys. Res. Atmos. 2012, 117, D04101. [Google Scholar] [CrossRef]
- Barriopedro, D.; Calvo, N. On the Relationship between ENSO, Stratospheric Sudden Warmings, and Blocking. J. Clim. 2014, 27, 4704–4720. [Google Scholar] [CrossRef]
- Martius, O.; Polvani, L.M.; Davies, H.C. Blocking precursors to stratospheric sudden warming events. Geophys. Res. Lett. 2009, 36, L14806. [Google Scholar] [CrossRef]
- Peings, Y. Ural Blocking as a Driver of Early-Winter Stratospheric Warmings. Geophys. Res. Lett. 2019, 46, 5460–5468. [Google Scholar] [CrossRef]
- Kohler, R.H.; Jaiser, R.; Handorf, D. How do different pathways connect the stratospheric polar vortex to its tropospheric precursors? Weather Clim. Dyn. 2023, 4, 1071–1086. [Google Scholar] [CrossRef]
- Kodera, K.; Mukougawa, H.; Maury, P.; Ueda, M.; Claud, C. Absorbing and reflecting sudden stratospheric warming events and their relationship with tropospheric circulation. J. Geophys. Res. Atmos. 2016, 121, 80–94. [Google Scholar] [CrossRef]
- Runde, T.; Dameris, M.; Garny, H.; Kinnison, D.E. Classification of stratospheric extreme events according to their downward propagation to the troposphere. Geophys. Res. Lett. 2016, 43, 6665–6672. [Google Scholar] [CrossRef]
- Karpechko, A.Y.; Hitchcock, P.; Peters, D.H.W.; Schneidereit, A. Predictability of downward propagation of major sudden stratospheric warmings. Q. J. R. Meteorol. Soc. 2017, 143, 1459–1470. [Google Scholar] [CrossRef]
- Domeisen, D.I. Estimating the Frequency of Sudden Stratospheric Warming Events From Surface Observations of the North Atlantic Oscillation. J. Geophys. Res. Atmos. 2019, 124, 3180–3194. [Google Scholar] [CrossRef]
- Afargan-Gerstman, H.; Domeisen, D.I.V. Pacific Modulation of the North Atlantic Storm Track Response to Sudden Stratospheric Warming Events. Geophys. Res. Lett. 2020, 47, e2019GL085007. [Google Scholar] [CrossRef]
- Black, R.X.; McDaniel, B.A. Diagnostic Case Studies of the Northern Annular Mode. J. Clim. 2004, 17, 3990–4004. [Google Scholar] [CrossRef]
- Hitchcock, P.; Shepherd, T.G.; Manney, G.L. Statistical Characterization of Arctic Polar-Night Jet Oscillation Events. J. Clim. 2013, 26, 2096–2116. [Google Scholar] [CrossRef]
- Waugh, D.W.; Rong, P.P. Interannual variability in the decay of lower stratospheric Arctic vortices. J. Meteorol. Soc. Jpn. Ser. II 2002, 80, 997–1012. [Google Scholar] [CrossRef]
- Hauchecorne, A.; Claud, C.; Keckhut, P.; Mariaccia, A. Stratospheric Final Warmings fall into two categories with different evolution over the course of the year. Commun. Earth Environ. 2022, 3, 4. [Google Scholar] [CrossRef]
- Vargin, P.; Luk’yanov, A.; Kiryushov, B. Dynamic Processes in the Arctic Stratosphere in the Winter of 2018/2019. Russ. Meteorol. Hydrol. 2020, 45, 387–397. [Google Scholar] [CrossRef]
- Kelleher, M.E.; Ayarzagüena, B.; Screen, J.A. Interseasonal Connections between the Timing of the Stratospheric Final Warming and Arctic Sea Ice. J. Clim. 2020, 33, 3079–3092. [Google Scholar] [CrossRef]
- Mariaccia, A.; Keckhut, P.; Hauchecorne, A. Classification of Stratosphere Winter Evolutions Into Four Different Scenarios in the Northern Hemisphere. J. Geophys. Res. Atmos. 2022, 127, e2022JD036662. [Google Scholar] [CrossRef]
- Maury, P.; Claud, C.; Manzini, E.; Hauchecorne, A.; Keckhut, P. Characteristics of stratospheric warming events during Northern winter. J. Geophys. Res. Atmos. 2016, 121, 5368–5380. [Google Scholar] [CrossRef]
- Monnin, E.; Kretschmer, M.; Polichtchouk, I. The role of the timing of sudden stratospheric warmings for precipitation and temperature anomalies in Europe. Int. J. Climatol. 2022, 42, 3448–3462. [Google Scholar] [CrossRef]
- Díaz-Durán, A.; Serrano, E.; Ayarzagüena, B.; Abalos, M.; de la Cámara, A. Intra-seasonal variability of extreme boreal stratospheric polar vortex events and their precursors. Clim. Dyn. 2017, 49, 3473–3491. [Google Scholar] [CrossRef]
- Hersbach, H.; Bell, B.; Berrisford, P.; Hirahara, S.; Horányi, A.; Muñoz-Sabater, J.; Nicolas, J.; Peubey, C.; Radu, R.; Schepers, D.; et al. The ERA5 global reanalysis. Q. J. R. Meteorol. Soc. 2020, 146, 1999–2049. [Google Scholar] [CrossRef]
- Bell, B.; Hersbach, H.; Simmons, A.; Berrisford, P.; Dahlgren, P.; Horányi, A.; Muñoz-Sabater, J.; Nicolas, J.; Radu, R.; Schepers, D.; et al. The ERA5 global reanalysis: Preliminary extension to 1950. Q. J. R. Meteorol. Soc. 2021, 147, 4186–4227. [Google Scholar] [CrossRef]
- Marlton, G.; Charlton-Perez, A.; Harrison, G.; Polichtchouk, I.; Hauchecorne, A.; Keckhut, P.; Wing, R.; Leblanc, T.; Steinbrecht, W. Using a network of temperature lidars to identify temperature biases in the upper stratosphere in ECMWF reanalyses. Atmos. Chem. Phys. 2021, 21, 6079–6092. [Google Scholar] [CrossRef]
- Mariaccia, A.; Keckhut, P.; Hauchecorne, A.; Claud, C.; Le Pichon, A.; Meftah, M.; Khaykin, S. Assessment of ERA-5 Temperature Variability in the Middle Atmosphere Using Rayleigh LiDAR Measurements between 2005 and 2020. Atmosphere 2022, 13, 242. [Google Scholar] [CrossRef]
- Wilcoxon, F. Individual Comparisons by Ranking Methods. Biom. Bull. 1945, 1, 80–83. [Google Scholar] [CrossRef]
- Garfinkel, C.I.; Hartmann, D.L.; Sassi, F. Tropospheric Precursors of Anomalous Northern Hemisphere Stratospheric Polar Vortices. J. Clim. 2010, 23, 3282–3299. [Google Scholar] [CrossRef]
- Eliassen, A.; Palm, E. On the transfer of energy in stationary mountain waves. Geofys. Publ. 1961, 22, 1–23. [Google Scholar]
- Kuroda, Y.; Kodera, K. Role of planetary waves in the stratosphere-troposphere coupled variability in the northern hemisphere winter. Geophys. Res. Lett. 1999, 26, 2375–2378. [Google Scholar] [CrossRef]
- Jucker, M. Scaling of Eliassen-Palm flux vectors. Atmos. Sci. Lett. 2021, 22, e1020. [Google Scholar] [CrossRef]
- Andrews, D.G.; Mahlman, J.D.; Sinclair, R.W. Eliassen-Palm Diagnostics of Wave-Mean Flow Interaction in the GFDL “SKYHI” General Circulation Model. J. Atmos. Sci. 1983, 40, 2768–2784. [Google Scholar] [CrossRef]
- Seidel, D.J.; Randel, W.J. Variability and trends in the global tropopause estimated from radiosonde data. J. Geophys. Res. Atmos. 2006, 111, D21101. [Google Scholar] [CrossRef]
- Esler, J.G.; Scott, R.K. Excitation of Transient Rossby Waves on the Stratospheric Polar Vortex and the Barotropic Sudden Warming. J. Atmos. Sci. 2005, 62, 3661–3682. [Google Scholar] [CrossRef]
- Huang, J.; Tian, W.; Zhang, J.; Huang, Q.; Tian, H.; Luo, J. The connection between extreme stratospheric polar vortex events and tropospheric blockings. Q. J. R. Meteorol. Soc. 2017, 143, 1148–1164. [Google Scholar] [CrossRef]
- Hersbach, H.; Bell, B.; Berrisford, P.; Biavati, G.; Horányi, A.; Muñoz Sabater, J.; Nicolas, J.; Peubey, C.; Radu, R.; Rozum, I.; et al. ERA5 Hourly Data on Single Levels from 1940 to Present [Dataset]. Copernicus Climate Change Service (C3S) Climate Data Store (CDS). 2023. Available online: https://cds.climate.copernicus.eu/cdsapp#!/dataset/10.24381/cds.adbb2d47?tab=overview (accessed on 1 February 2023).
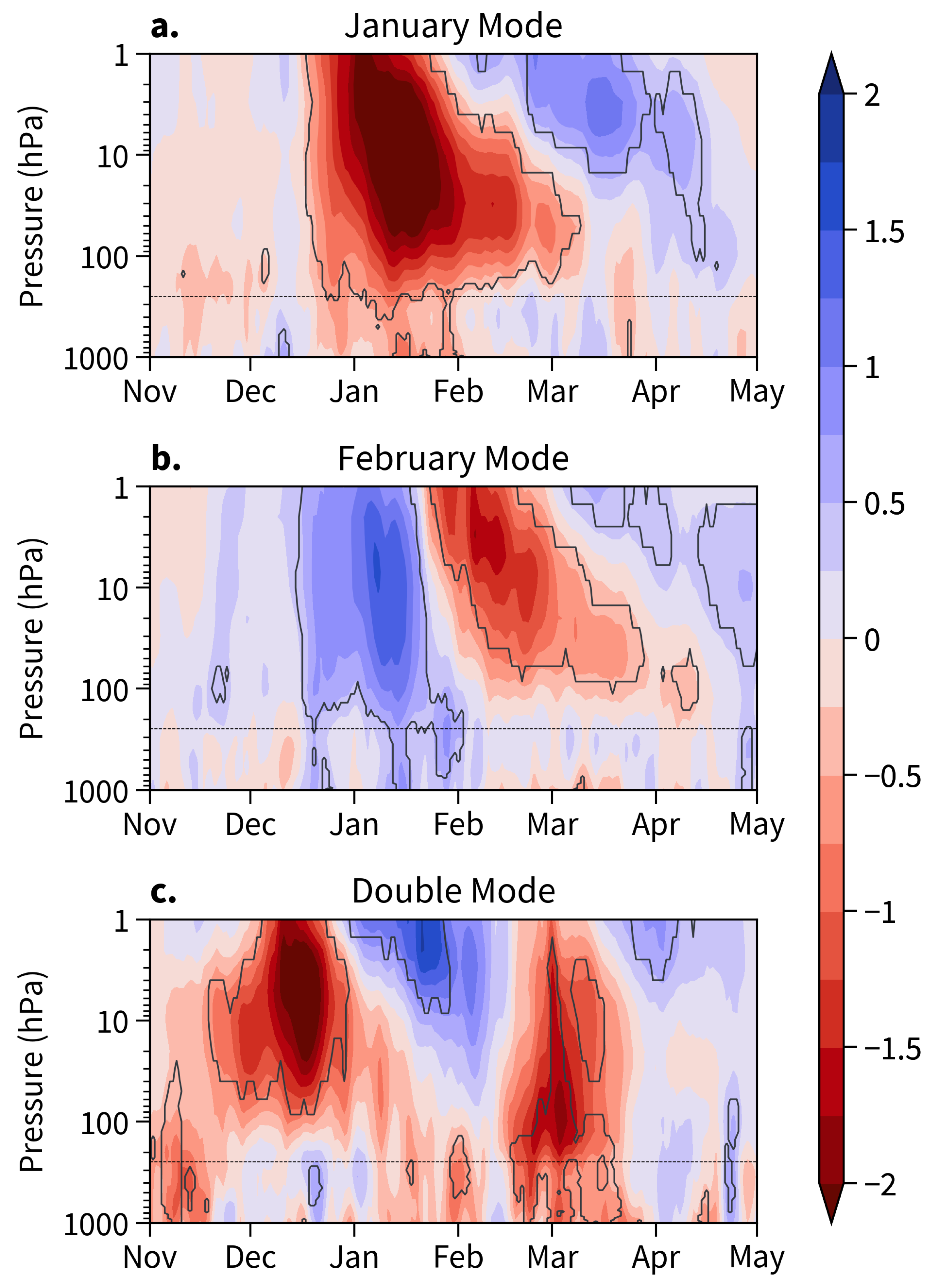

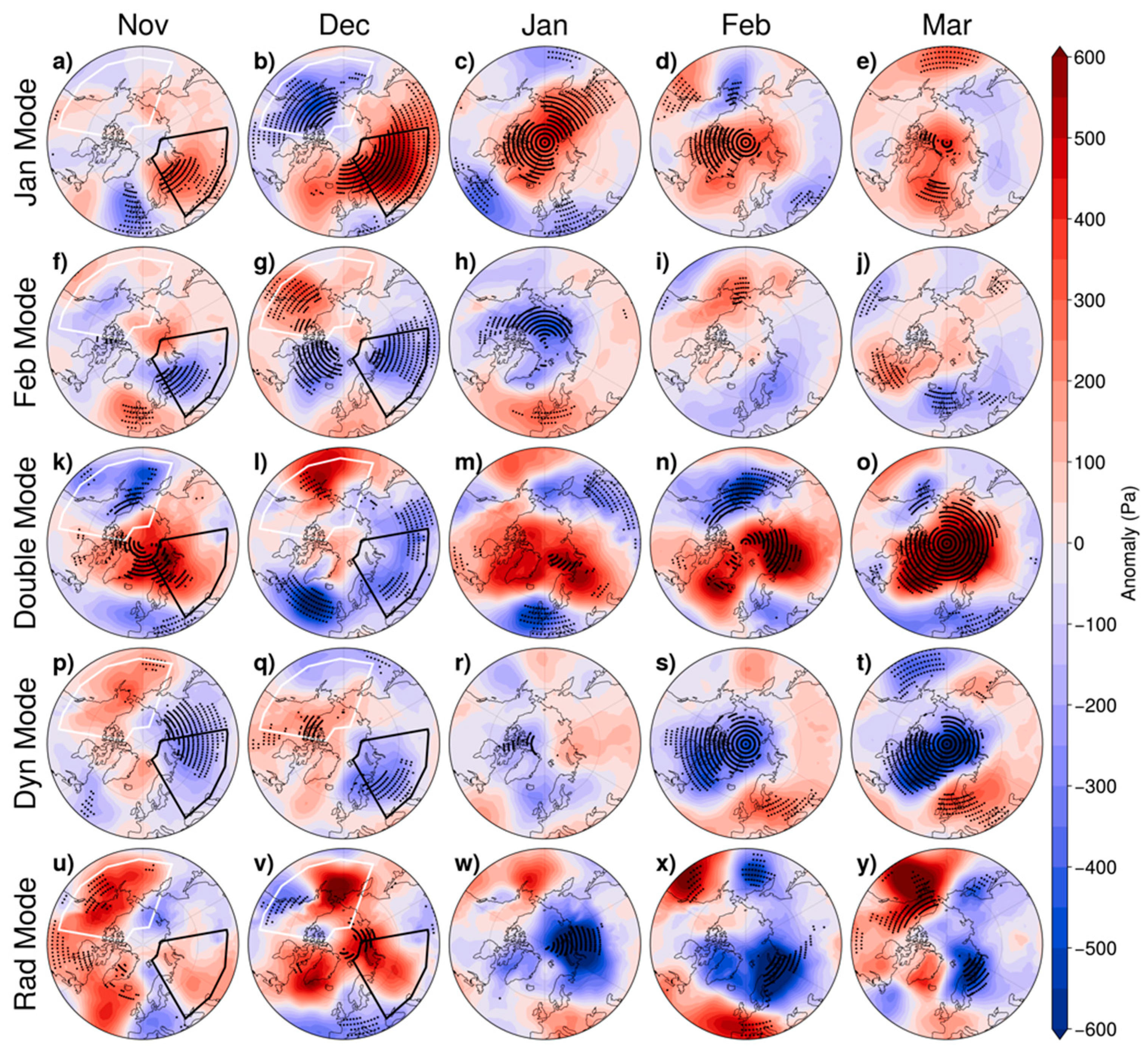




| MSLP Anomaly (Pa) | Jan Mode | Feb Mode | Double Mode | Dynamical Mode | Radiative Mode | |
|---|---|---|---|---|---|---|
| Ural blocking region | November | 159 | −87 | 311 | −166 | 76 |
| December | 395 | −180 | −186 | −184 | 233 | |
| Aleutian blocking region | November | 10 | −24 | −89 | 100 | 167 |
| December | −191 | 119 | 101 | 77 | 135 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mariaccia, A.; Keckhut, P.; Hauchecorne, A. Impact of Polar Vortex Modes on Winter Weather Patterns in the Northern Hemisphere. Atmosphere 2024, 15, 1062. https://doi.org/10.3390/atmos15091062
Mariaccia A, Keckhut P, Hauchecorne A. Impact of Polar Vortex Modes on Winter Weather Patterns in the Northern Hemisphere. Atmosphere. 2024; 15(9):1062. https://doi.org/10.3390/atmos15091062
Chicago/Turabian StyleMariaccia, Alexis, Philippe Keckhut, and Alain Hauchecorne. 2024. "Impact of Polar Vortex Modes on Winter Weather Patterns in the Northern Hemisphere" Atmosphere 15, no. 9: 1062. https://doi.org/10.3390/atmos15091062
APA StyleMariaccia, A., Keckhut, P., & Hauchecorne, A. (2024). Impact of Polar Vortex Modes on Winter Weather Patterns in the Northern Hemisphere. Atmosphere, 15(9), 1062. https://doi.org/10.3390/atmos15091062








