Interdecadal Variation in Rossby Wave Source over the Tibetan Plateau and Its Impact on the East Asia Circulation Pattern during Boreal Summer
Abstract
1. Introduction
2. Data and Methods
3. Results
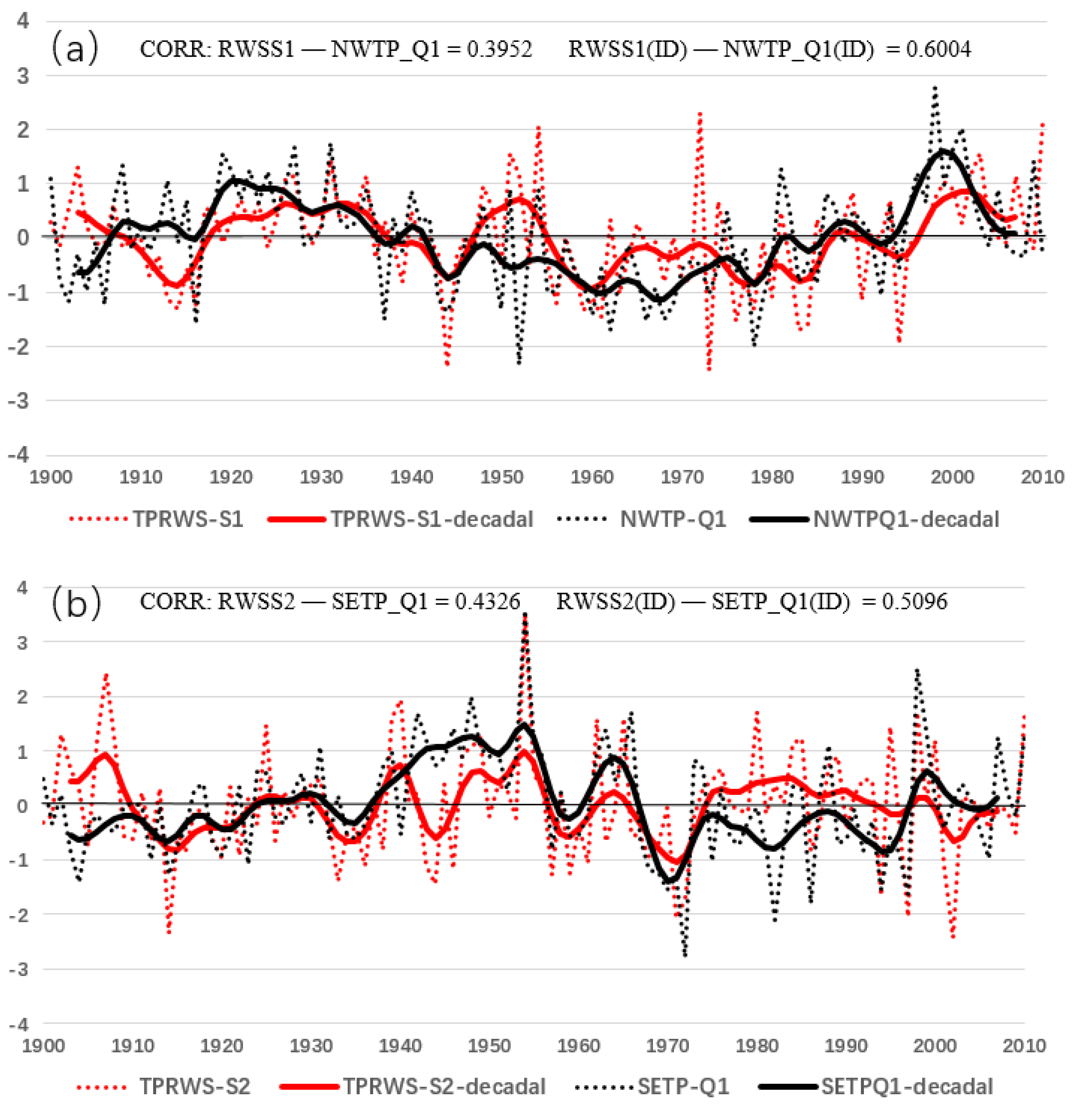
3.1. Interdecadal Variation in Rossby Wave Source over the Tibetan Plateau
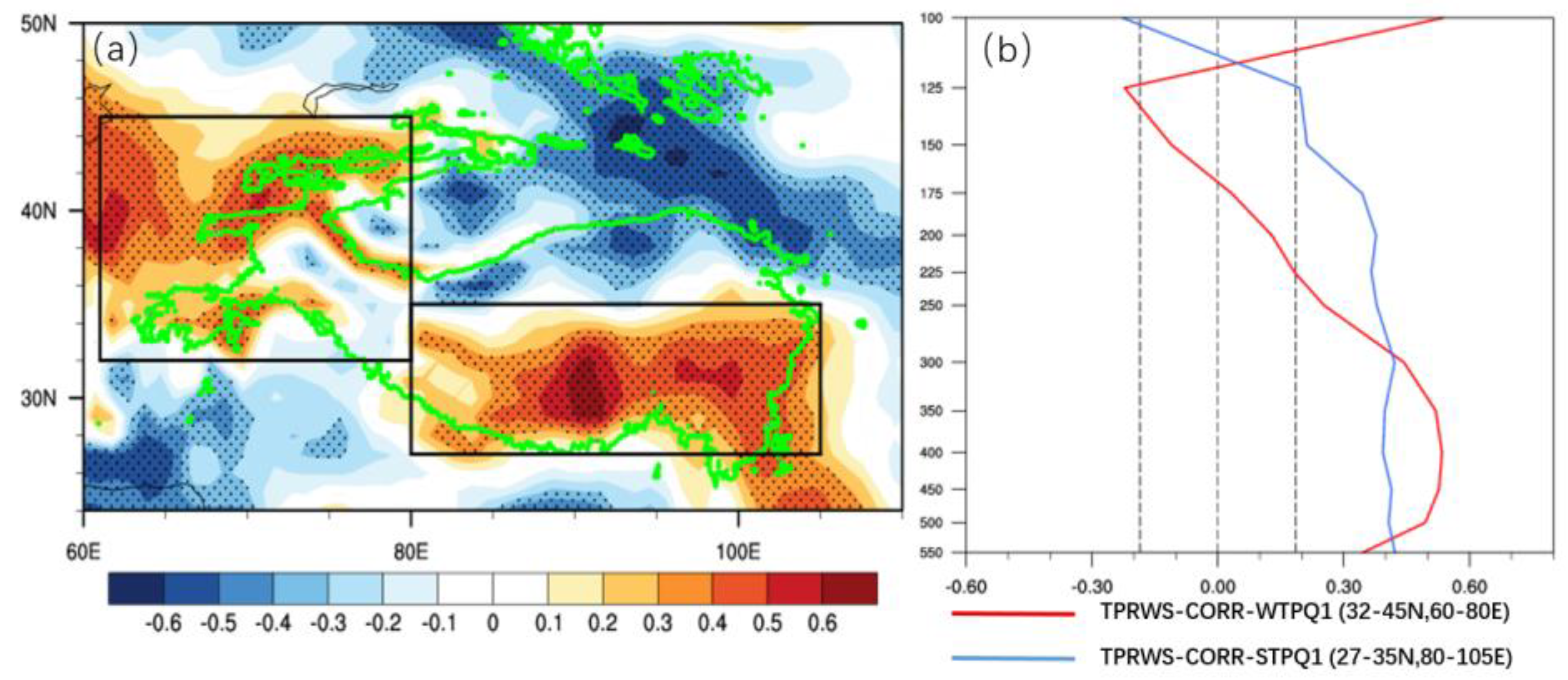
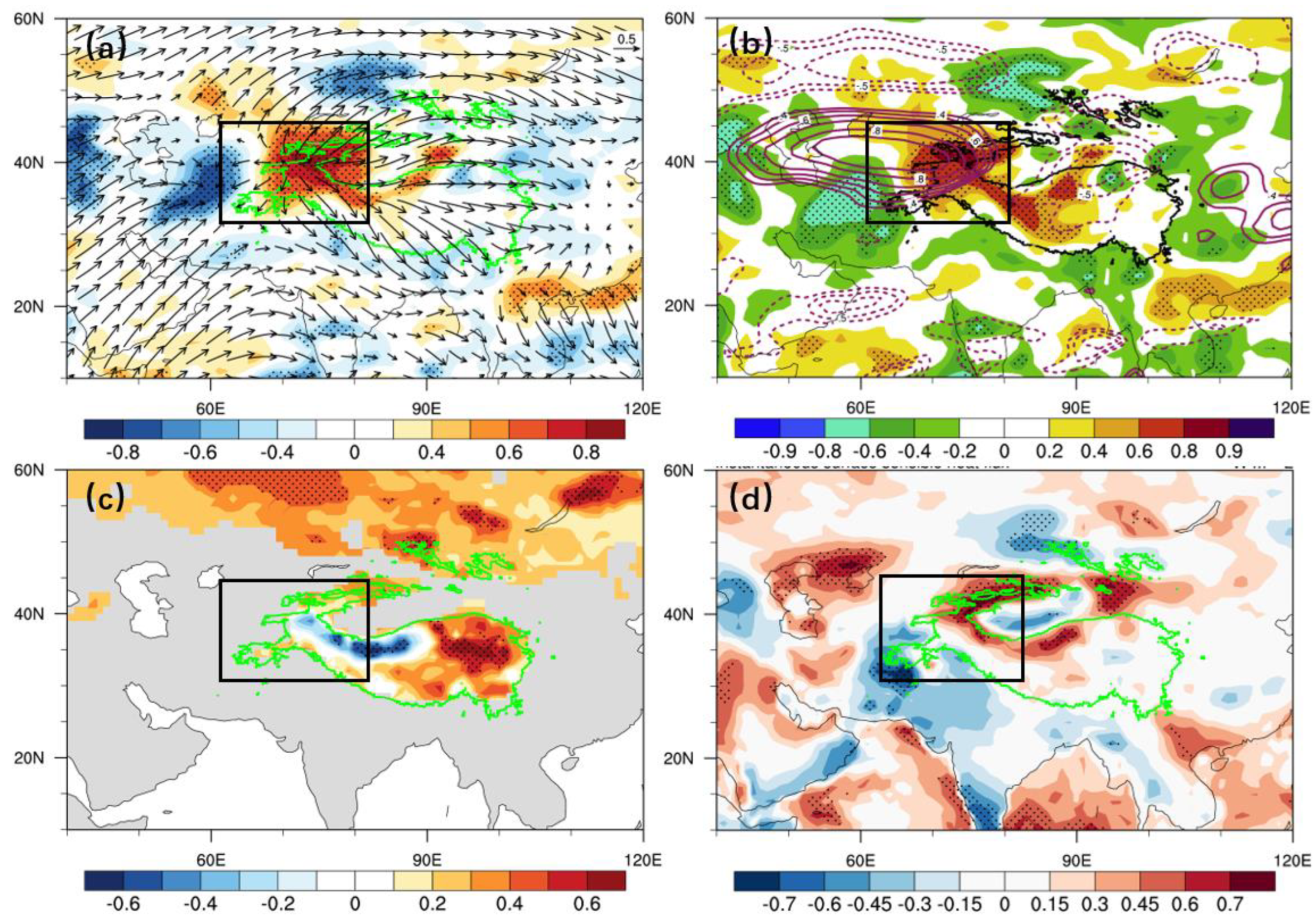
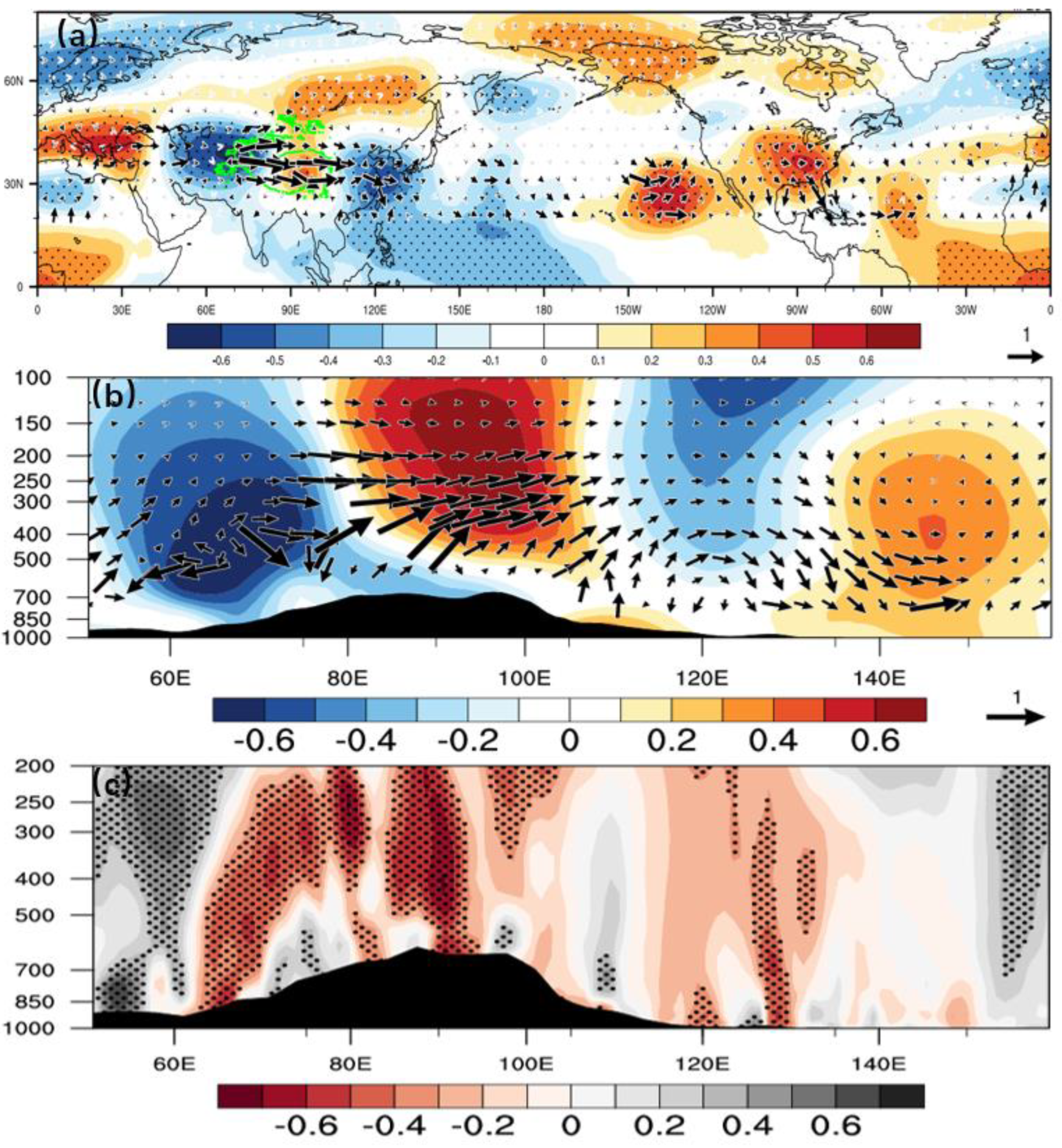
3.2. The Mechanisms Contributing to Interdecadal Variations in the TP-RWS
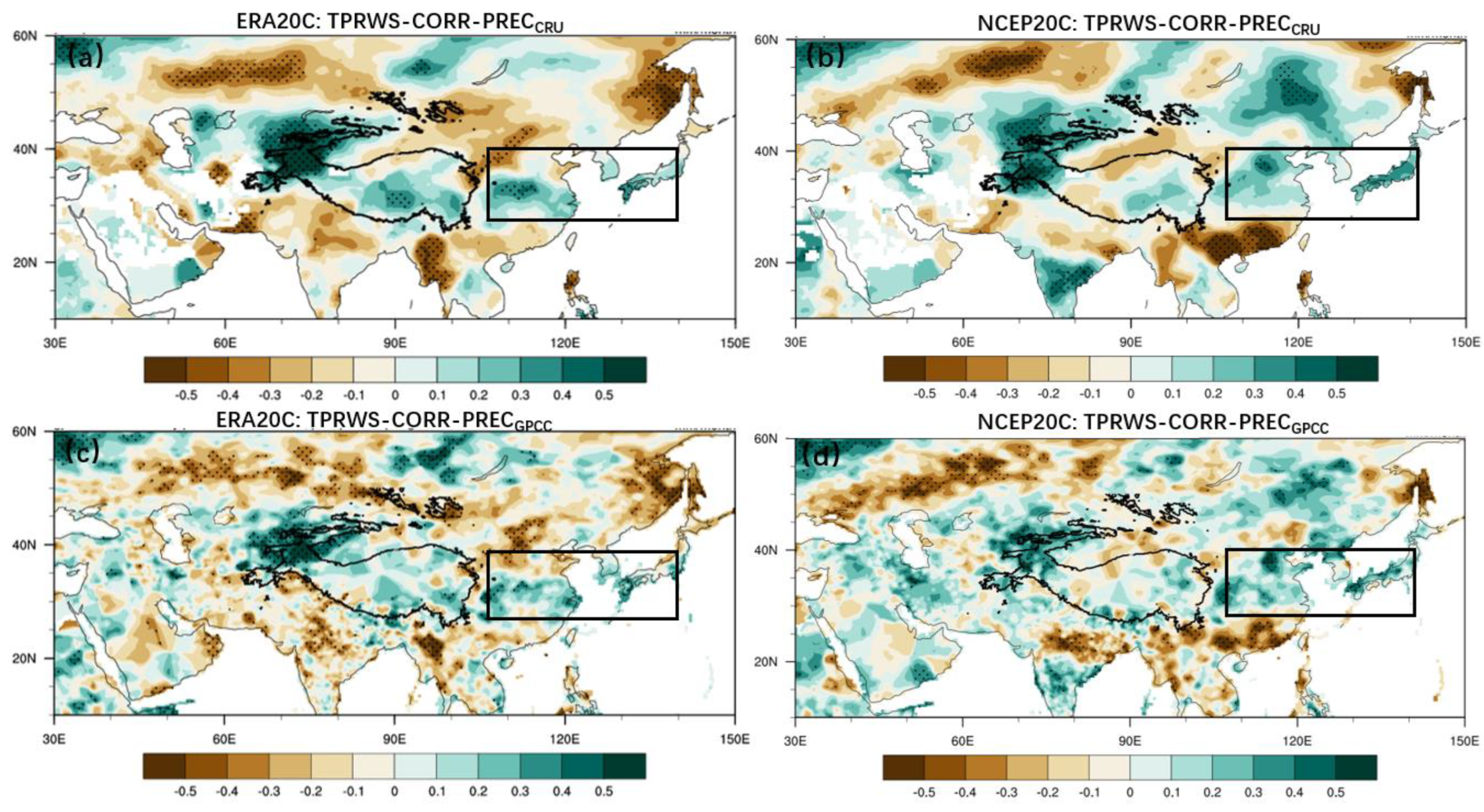
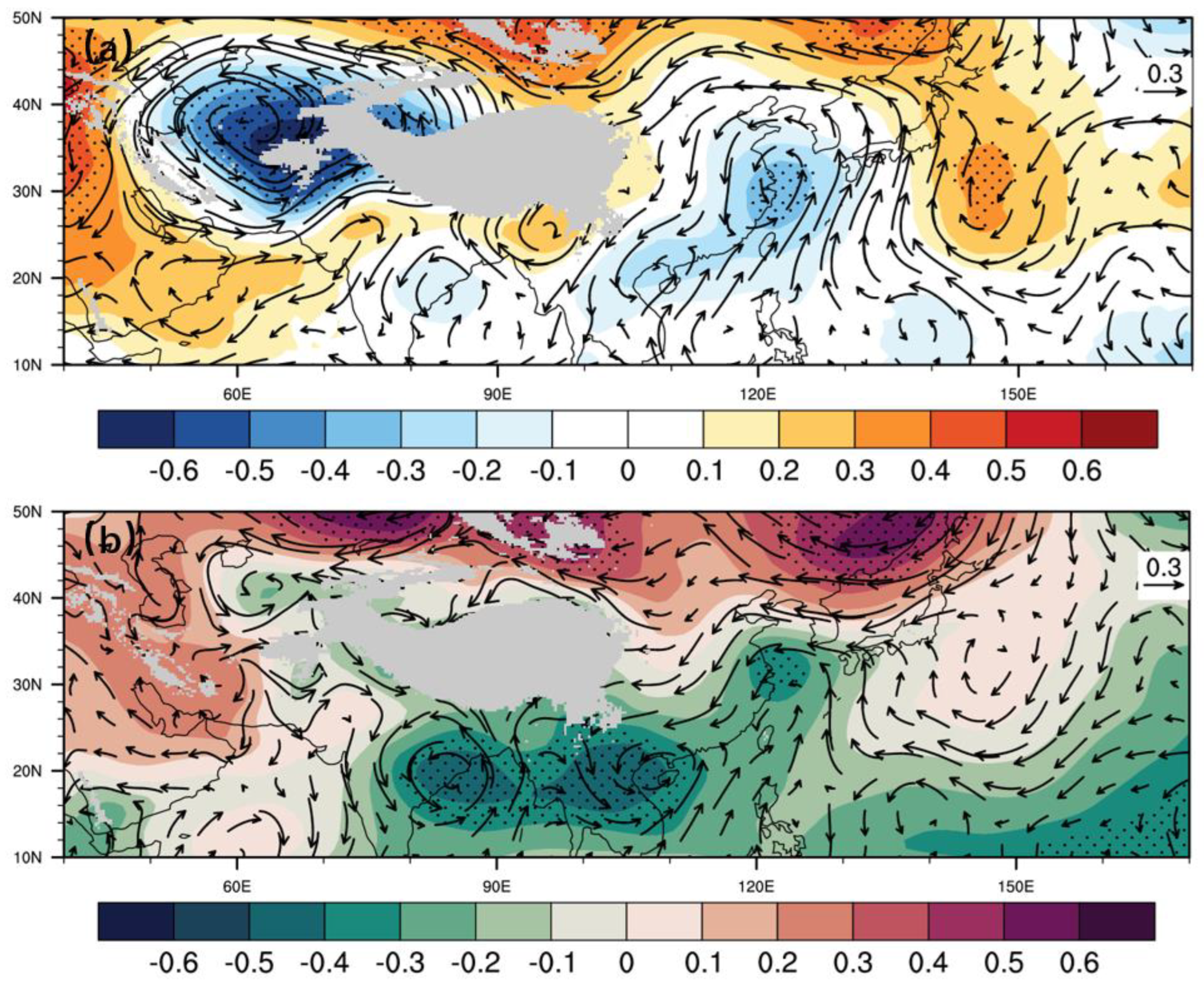
3.3. Impacts of the Interdecadal TP-RWS on East Asia Circulation Pattern
4. Conclusions
- (1)
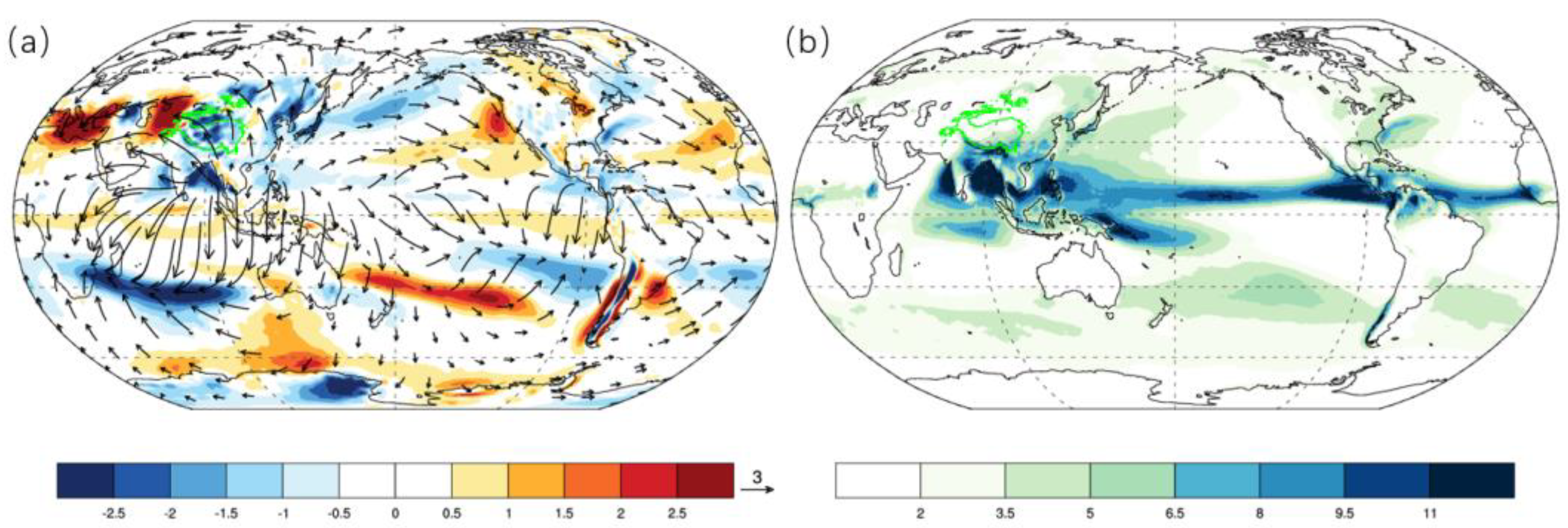
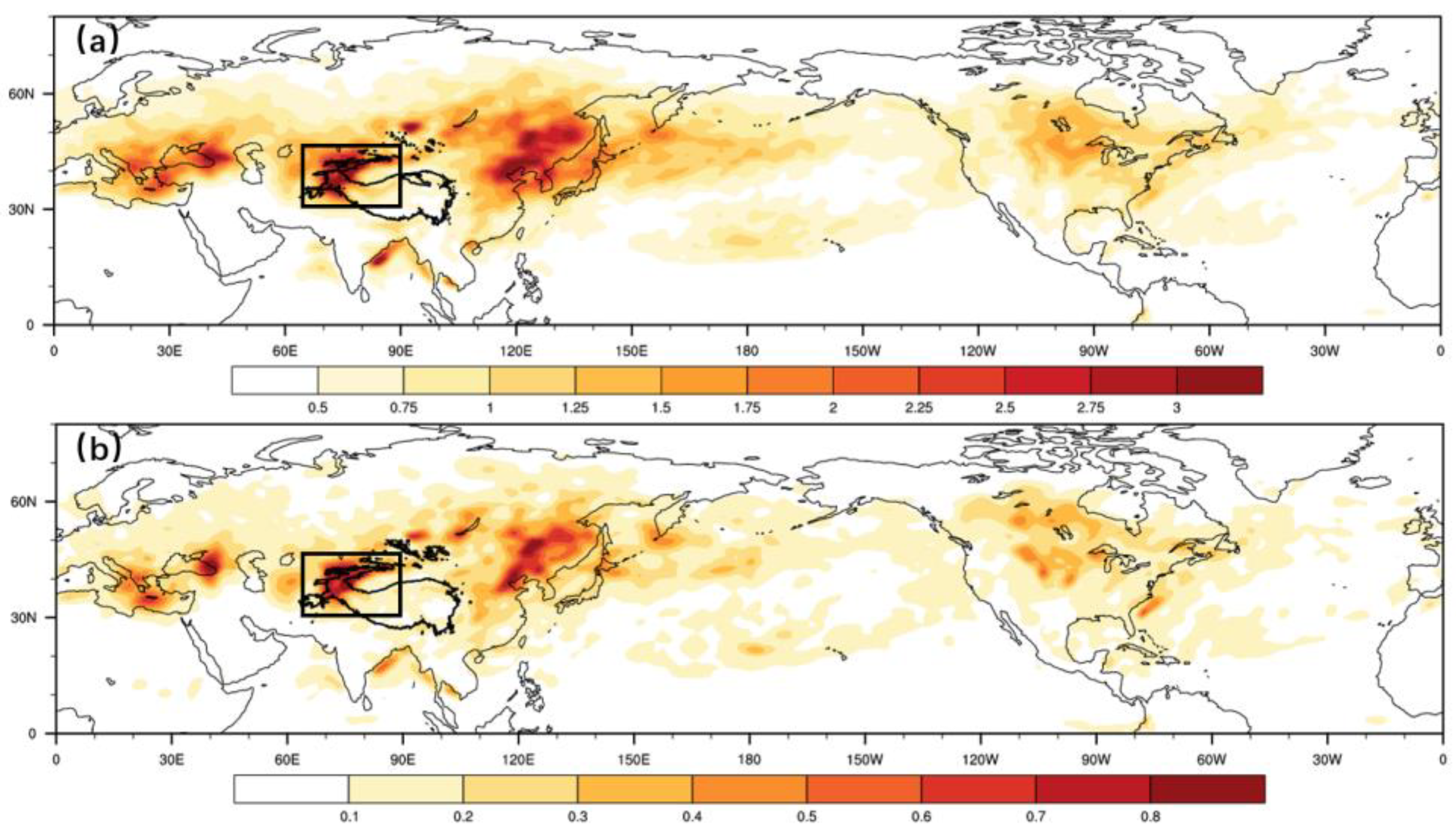
- There is a strong negative RWS over the TP during boreal summer. In the recent 110 years, the RWS variability has shown great regional differences in the NH midlatitudes. The regions with large interannual and interdecadal variability occur over the northwestern TP, the northeast of China to the Korean Peninsula, and areas near the Mediterranean Sea along the westerly jet, among which the variability over the TP is the largest.
- (2)
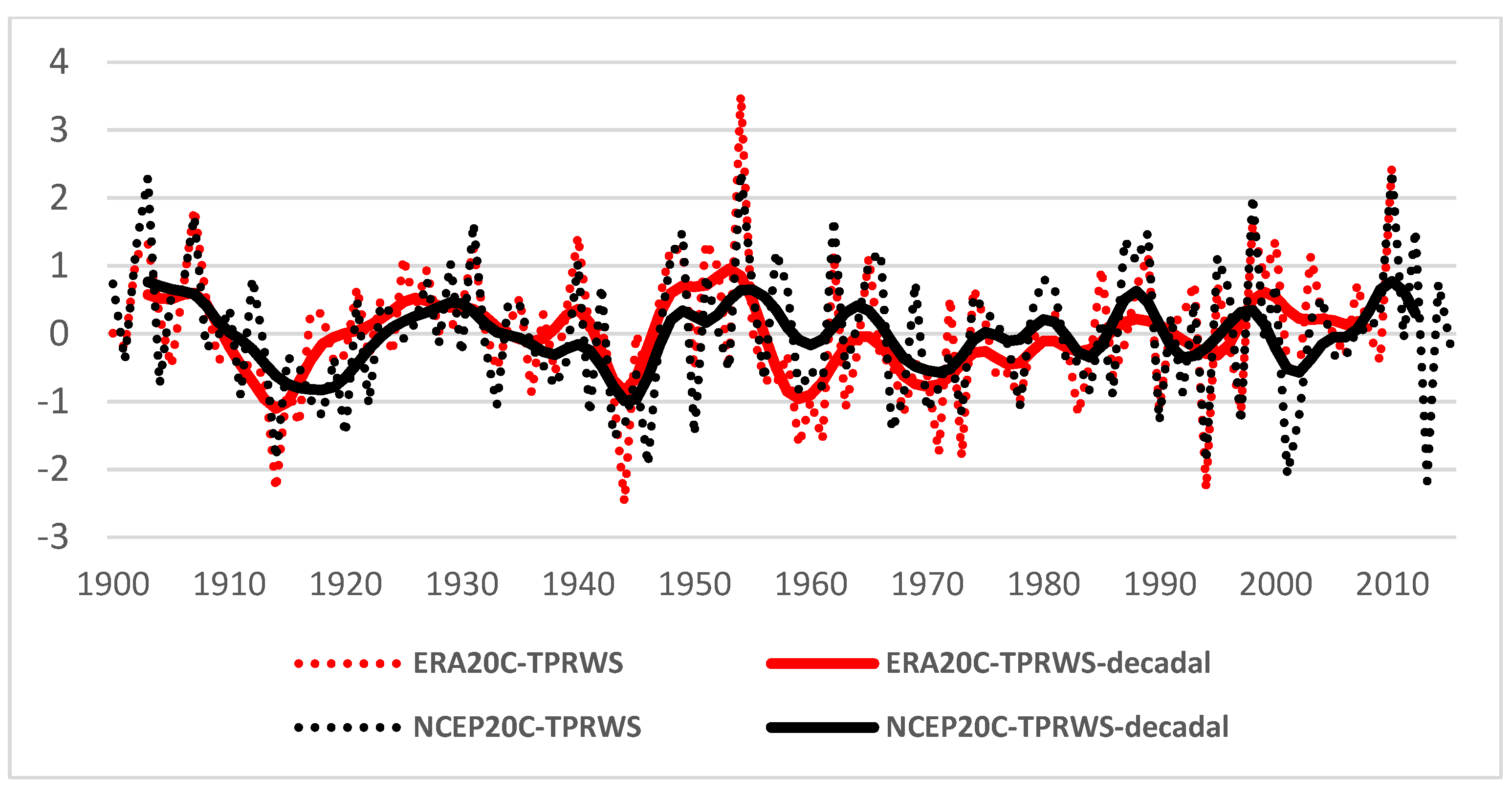
- The TP-RWS during 1900 to 2010 is characterized by multidecadal variations. From the perspective of intensity changes, it showed continuous strong periods during the early 20th century, the 1920s to 1940s, the 1950s to 1970s, and after 2000. Correspondingly, the temporal series turned into weak periods during the 1910s to 1920s, 1940s to 1950s, and from 1970s to the end of the 20th century. When the interdecadal TP-RWS intensifies, it is accompanied by strong divergence flow at the upper troposphere.
- (3)
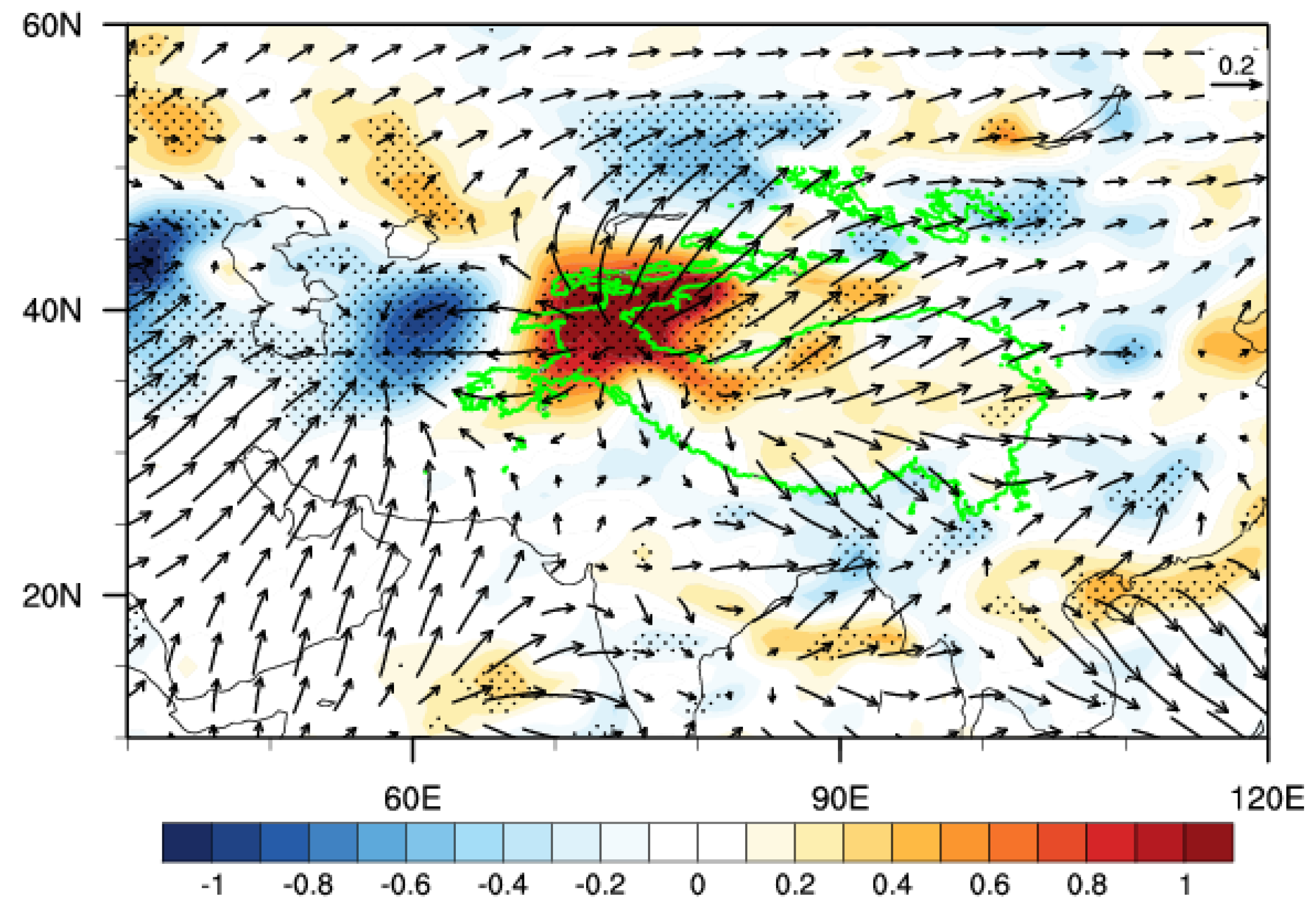
- The two sub-items TPRWS-S1 and TPRWS-S2, which constitute the TPRWS have independent interdecadal variations. Meanwhile, similar interdecadal temporal variations exist between TPRWS-S1 and northwestern TP-Q1 and between TPRWS-S2 and southeastern TP-Q1. The interdecadal variations in snow cover over the TP cause the divergence and absolute vorticity anomalies by affecting atmospheric heating from TP surface to the middle troposphere, and then contribute to the TP-RWS. On the other hand, due to latent heat release of deep convection in southeastern TP, the TP-RWS can be formed through absolute vorticity advection caused by large-scale divergent flow in the middle and upper troposphere. Therefore, although the TP-RWS manifests as a whole, the causes of its interdecadal variations are not the same in northwestern and southeastern TP.
- (4)
- The interdecadal TP-RWS has a great climate effect on the atmospheric circulation and precipitation pattern in Huang-huai River Basin, South Korea, and Japan Island. The enhanced TP-RWS can excite an anomalous wave active flux originating from the northwestern TP and propagating eastward along the westerly jet to East Asia and the Northwest Pacific. The TP-RWS teleconnection exhibits an alternatively positive and negative pattern of geopotential height anomalies from the northwestern TP to the North Pacific, which has an equivalent barotropic structure at vertical profile. Under the guidance of the anomalous cyclonic circulation in East Asia, the prevailing southerly and easterly winds over the West Pacific and Huang-huai River Basin are conducive to the water vapor convergence and precipitation anomaly.
5. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Rossby, C.G. Relation between variations in the intensity of the zonal circulation of the atmosphere and the displacements of the semi-permanent centers of action. J. Mar. Res. 1939, 2, 38–55. [Google Scholar] [CrossRef]
- Shimizu, M.H.; Cavalcanti, I. Variability patterns of Rossby wave source. Clim. Dyn. 2011, 37, 441–454. [Google Scholar] [CrossRef]
- Hoskins, B.J.; Karoly, D.J. The steady linear response of a spherical atmosphere to thermal and orographical forcing. J. Atmos. Sci. 1981, 38, 1179–1196. [Google Scholar] [CrossRef]
- Sardeshmukh, P.D.; Hoskins, B.J. The generation of global rotational flow by steady idealized tropical divergence. J. Atmos. Sci. 1988, 45, 1228–1251. [Google Scholar] [CrossRef]
- Geng, Q.; Chen, S. Vorticity Source by Stationary Divergent Wind and Energy Propagation of Stationary Waves. Chin. J. Atmos. Sci. 1996, 20, 298–305. [Google Scholar]
- James, I.N. Introduction to Circulating Atmospheres; Cambridge University Press: Cambridge, UK, 1994. [Google Scholar]
- Ye, D.Z.; Zhu, B.Z. Some Basic Problems of Atmospheric Circulation; Science Press: Beijing, China, 1958. [Google Scholar]
- Sardeshmukh, P.D.; Hoskins, B.J. Vorticity balances in the tropics during the 1982-83 El Niño-Southern Oscillation event. Quart. J. Roy. Meteor. Soc. 1985, 111, 261–278. [Google Scholar] [CrossRef]
- Lu, R.Y.; Baek-Jo, K.I.M. The Climatological Rossby Wave Source over the STCZs in the Summer Northern Hemisphere. J. Meteorol. Soc. Japan. Ser. II 2004, 82, 2, 657–669. [Google Scholar] [CrossRef]
- Plumb, R.A. On The three-dimensional propagation of stationary waves. J. Atmos. Sci 1985, 42, 217–229. [Google Scholar] [CrossRef]
- Yeh, T.C. On energy dispersion in the atmosphere. J. Atmos. Sci. 2010, 6, 1–16. [Google Scholar] [CrossRef]
- Tao, S.Y.; Wei, J.; Liang, F.; Zhang, X.L. Analysis of High Impact Weather Induced by the Downstream Effect of Rossby Waves. Meteor Mon. 2010, 36, 81–93. [Google Scholar]
- Xu, J.P.; Wang, W.; Cai, X.J.; Xu, Z.L.; Xu, J.X. A comparison of the Rossby wave activities and circulation features of the drought in winter-spring of 2011 and in summer of 2013 over mid-lower reaches of the Yangtze river basin. J. Trop. Meteorol. 2017, 33, 992–999. [Google Scholar]
- Wang, X.W.; Li, Y.; Zhou, B. An Applied Research About Spread Effects of Rossby Wave in Medium Range Forecast on Heavy Rain During Meiyu Flood Period. Meteor. Mon. 2012, 38, 1070–1077. [Google Scholar]
- Sun, X.; Ding, Q.; Wang, S.Y.S.; Topál, D.; Li, Q.; Castro, C.; Teng, H.; Luo, R.; Ding, Y. Enhanced jet stream waviness induced by suppressed tropical Pacific convection during boreal summer. Nat. Commun. 2022, 13, 1288. [Google Scholar] [CrossRef] [PubMed]
- Li, M.; Guan, Z.; Mei, S. Interannual and Interdecadal Variations of Summer Rainfall Duration over the Middle and Lower Reaches of the Yangtze River in Association with Anomalous Circulation and Rossby Wave Activities. Chin. J. Atmos. Sci. 2016, 40, 1199–1214. [Google Scholar]
- Yang, N.; Jin, R.; Xiao, T.; Sun, X. Propagation of Rossby Wave Along Asian Subtropical Westerly Jet in Summer and Its Impact on Anomaly of Precipitation in China. Meteor. Mon. 2020, 46, 1–14. [Google Scholar]
- Duan, A.M.; Li, F.; Wang, M.R.; Wu, G. Persistent weakening trend in the spring sensible heat source over the Tibetan Plateau and its impact on the Asian summer monsoon. J. Clim. 2011, 24, 5671–5682. [Google Scholar] [CrossRef]
- Wu, G.X.; Liu, Y.M.; He, B.; Bao, Q.; Duan, A.; Jin, F.F. Thermal controls on the Asian summer monsoon. Sci. Rep. 2012, 2, 404. [Google Scholar] [CrossRef] [PubMed]
- Xu, X.D.; Lu, C.G.; Ding, Y.H.; Shi, X.; Guo, Y.; Zhu, W. What is the relationship between China summer precipitation and the change of apparent heat source over the Tibetan Plateau? Atmos. Sci. Lett. 2013, 14. [Google Scholar] [CrossRef]
- Lu, M.M.; Yang, S.; Li, Z.N.; He, B.; He, S.; Wang, Z. Possible effect of the Tibetan Plateau on the ‘upstream’ climate over West Asia, North Africa, South Europe and the North Atlantic. Clim. Dyn. 2018, 51, 1485–1498. [Google Scholar] [CrossRef]
- Wu, G.X.; He, B.; Liu, Y.M.; Bao, Q.; Ren, R. Location and variation of the summertime upper-troposphere temperature maximum over South Asia. Clim. Dyn. 2015, 45, 2757–2774. [Google Scholar] [CrossRef]
- Sun, R.Z.; Duan, A.M.; Chen, L.L.; Li, Y.; Xie, Z.; Zhao, Y. Interannual variability of the North Pacific mixed layer associated with the spring Tibetan Plateau thermal forcing. J. Clim. 2019, 32, 3109–3130. [Google Scholar] [CrossRef]
- Dong, L.; Xu, X.; Zhao, T.; Ren, H. Linkage between moisture transport over the Yangtze River Basin and a critical area of the Tibetan Plateau during the Meiyu. Clim. Dyn. 2019, 53, 2643–2662. [Google Scholar] [CrossRef]
- Duan, A.; Wang, M.; Lei, Y.; Cui, Y. Trends in summer rainfall over China associated with the Tibetan Plateau sensible heat source during 1980–2008. J. Clim. 2013, 26, 261–275. [Google Scholar] [CrossRef]
- Duan, A.; Wu, G. Role of the Tibetan Plateau thermal forcing in the summer climate patterns over subtropical Asia. Clim. Dyn. 2005, 24, 793–807. [Google Scholar] [CrossRef]
- Liu, Y.; Lu, M.; Yang, H.; Duan, A.; He, B.; Yang, S.; Wu, G. Land–atmosphere–ocean coupling associated with the Tibetan Plateau and its climate impacts. Nat. Sci. Rev. 2020, 7, 534–552. [Google Scholar] [CrossRef] [PubMed]
- Song, L.; Wu, R.; An, L. Diferent sources of 10- to 30-day intraseasonal variations of autumn snow over western and eastern Tibetan Plateau. Geophy. Res. Lett. 2019, 46, 9118–9125. [Google Scholar] [CrossRef]
- Zhao, P.; Zhou, Z.; Liu, J. Variability of Tibetan spring snow and its associations with the hemispheric extratropical circulation and East Asian summer monsoon rainfall: An observational investigation. J. Clim. 2007, 20, 3942–3955. [Google Scholar] [CrossRef]
- Wu, R.; Zhao, P.; Liu, G. Change in the contribution of spring snow cover and remote oceans to summer air temperature anomaly over Northeast China around 1990. J. Geophy. Res. Atmos. 2014, 119, 663–676. [Google Scholar] [CrossRef]
- Si, D.; Ding, Y. Decadal change in the correlation pattern between the Tibetan Plateau winter snow and the East Asian summer precipitation during 1979–2011. J. Clim. 2013, 26, 7622–7634. [Google Scholar] [CrossRef]
- Zhou, X.J.; Zhao, P.; Chen, J.; Chen, L.; Li, W. Impacts of thermodynamic processes over the Tibetan Plateau on the Northern Hemispheric climate. Sci. China Ser. D Earth Sci. 2009, 52, 1679–1693. [Google Scholar] [CrossRef]
- Wu, B.; Lin, J.; Zhou, T. Interdecadal circumglobal teleconnection pattern during boreal summer. Atmos. Sci. Lett. 2016, 17, 446–452. [Google Scholar] [CrossRef]
- Li, Q.Q.; Zhao, M.C.; Yang, S.; Shen, X.; Dong, L.; Liu, Z. A zonally-oriented teleconnection pattern induced by heating of the western Tibetan Plateau in boreal summer. Clim. Dyn. 2021, 57, 2823–2842. [Google Scholar] [CrossRef]
- Poli, P.; Hersbach, H.; Dee, D.P.; Berrisford, P.; Simmons, A.J.; Vitart, F.; Laloyaux, P.; Tan, D.G.; Peubey, C.; Thépaut, J.N.; et al. ERA-20C: An Atmospheric Reanalysis of the Twentieth Century. J. Clim. 2016, 29, 4083–4097. [Google Scholar] [CrossRef]
- Compo, G.P.; Whitaker, J.S.; Sardeshmukh, P.D.; Matsui, N.; Allan, R.J.; Yin, X.; Gleason, B.E.; Vose, R.S.; Rutledge, G.; Bessemoulin, P.; et al. The Twentieth Century Reanalysis Project. Q. J. R. Meteorol. Soc. 2011, 137, 1–28. [Google Scholar] [CrossRef]
- Xu, G.Q.; Zhu, Q.G. Source or Sink Features of Atmospheric Low Frequency Oscillation over the Tibetan Plateau. Trans Atmos Sci. 2002, 3, 358–365. [Google Scholar]
- Yanai, M.; Li, C.F.; Song, Z.S. Seasonal heating of the Tibetan Plateau and its effects on the evolution of the Asian summer monsoon. J. Meteor. Soc. Jpn. 1992, 70, 319–350. [Google Scholar] [CrossRef]
- Takaya, K.; Nakamura, H. A formulation of a wave-activity flux for stationary Rossby waves on a zonally varying basic flow. Geophys. Res. Lett. 1997, 24, 2985–2988. [Google Scholar] [CrossRef]
- Takaya, K.; Nakamura, H. A formulation of a phase-independent wave-activity flux for stationary and migratory quasigeostrophic eddies on a zonally varying basic flow. J. Atmos. Sci. 2001, 58, 608–627. [Google Scholar] [CrossRef]
- Shi, C.H.; Jin, X.; Liu, R.Q. The differences in characteristics and applicability among three types of Rossby wave activity flux in atmospheric dynamics. Trans. Atmos. Sci. 2017, 40, 850–855. [Google Scholar]
- Moon, J.Y.; Ha, K.J. Association between tropical convection and boreal wintertime extratropical circulation in 1982/83 and 1988/89. Adv. Atmos. Sci. 2003, 20, 593–603. [Google Scholar] [CrossRef]
- Sun, X.T.; Ding, Y.H.; Li, Q.Q. Interdecadal Variation of the Atmospheric Heat Source over the Tibetan Plateau and Surrounding Asian Monsoon Region: Impact on the Northern Hemisphere Summer Circulation. J. Meteor. Res. 2021, 35, 1–20. [Google Scholar] [CrossRef]
- Webster., P.J. The Large-Scale Structure of the Tropical Atmosphere, Large-Scale Dynamical Processes in the Atmosphere; Hoskins, B.J., Pearce, E.P., Eds.; Academic Press: London, UK, 1983; pp. 235–273. [Google Scholar]
- Kato, K. Seasonal transition of the lower-level circulation systems around the Baiu front in China in 1979 and its relation to the northern summer monsoon. J. Meteor. Soc. Jpn. 1989, 67, 249–265. [Google Scholar] [CrossRef]
- Ose, T. Seasonal change of Asian summer monsoon circulation and its heat source. J. Meteor. Soc. Jpn. 1998, 76, 1045–1063. [Google Scholar] [CrossRef]
- Yao, T.; Bolch, T.; Chen, D.; Gao, J.; Immerzeel, W.; Piao, S.; Su, F.; Thompson, L.; Wada, Y.; Wang, L.; et al. The imbalance of the Asian water tower. Nat. Rev. Earth Environ. 2022, 3, 618–632. [Google Scholar] [CrossRef]
- Zhang, Z.; Sun, X.; Yang, X.Q. Understanding the interdecadal variability of East Asian summer monsoon precipitation: Joint influence of three oceanic signals. J. Clim. 2018, 31, 5485–5506. [Google Scholar] [CrossRef]
- Cane, M. A moist model monsoon. Nature 2010, 463, 163–164. [Google Scholar] [CrossRef] [PubMed]
- Wu, G.; Duanm, A.; Lium, Y.; Mao, J.; Ren, R.; Bao, Q.; He, B.; Liu, B.; Hu, W. Tibetan Plateau climate dynamics: Recent research progress and outlook. Natl. Sci. Rev. 2014, 2, 100–116. [Google Scholar] [CrossRef]
- Zhao, H.; Moore, G.W.K. On the relationship between Tibetan snow cover, the Tibetan plateau monsoon and the Indian summer monsoon, Geophys. Res. Lett. 2004, 31, L14204. [Google Scholar] [CrossRef]
- Zhang, C.; Guo, Y.; Wen, Z. Interdecadal change in the effect of Tibetan Plateau snow cover on spring precipitation over Eastern China around the early 1990s. Clim Dyn. 2022, 58, 2807–2824. [Google Scholar] [CrossRef]
- Sutton, R.T.; Hodson, D.L.R. Atlantic Ocean forcing of North American and European summer climate. Science 2005, 309, 115–118. [Google Scholar] [CrossRef] [PubMed]
- Knight, J.R.; Folland, C.K.; Scaife, A.A. Climate impacts of the Atlantic multidecadal oscillation. Geophys. Res. Lett. 2006, 33, L17706. [Google Scholar] [CrossRef]
- Wang, Y.; Li, S.; Luo, D. Seasonal response of Asian monsoonal climate to the Atlantic multidecadal oscillation. J. Geophys. Res. 2009, 114, D02112. [Google Scholar] [CrossRef]
- Miao, J.; Jiang, D. Multidecadal variations in the East Asian winter monsoon and their relationship with the Atlantic Multidecadal Oscillation since 1850. J. Clim. 2021, 34, 7525–7539. [Google Scholar] [CrossRef]
- Zhang, P.; Duan, A.; Hu, J. Combined effect of the tropical Indian Ocean and tropical North Atlantic sea surface temperature anomaly on the Tibetan Plateau precipitation anomaly in late summer. J. Clim. 2022, 35, 3899–3918. [Google Scholar] [CrossRef]
- Wang, L.; Yu, J.-Y.; Paek, H. Enhanced biennial variability in the Pacific due to Atlantic capacitor effect. Nat. Commun. 2017, 8, 1–7. [Google Scholar] [CrossRef] [PubMed]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ding, Y.; Sun, X.; Li, Q.; Song, Y. Interdecadal Variation in Rossby Wave Source over the Tibetan Plateau and Its Impact on the East Asia Circulation Pattern during Boreal Summer. Atmosphere 2023, 14, 541. https://doi.org/10.3390/atmos14030541
Ding Y, Sun X, Li Q, Song Y. Interdecadal Variation in Rossby Wave Source over the Tibetan Plateau and Its Impact on the East Asia Circulation Pattern during Boreal Summer. Atmosphere. 2023; 14(3):541. https://doi.org/10.3390/atmos14030541
Chicago/Turabian StyleDing, Yihui, Xiaoting Sun, Qingquan Li, and Yafang Song. 2023. "Interdecadal Variation in Rossby Wave Source over the Tibetan Plateau and Its Impact on the East Asia Circulation Pattern during Boreal Summer" Atmosphere 14, no. 3: 541. https://doi.org/10.3390/atmos14030541
APA StyleDing, Y., Sun, X., Li, Q., & Song, Y. (2023). Interdecadal Variation in Rossby Wave Source over the Tibetan Plateau and Its Impact on the East Asia Circulation Pattern during Boreal Summer. Atmosphere, 14(3), 541. https://doi.org/10.3390/atmos14030541






