Exact Expressions for Lightning Electromagnetic Fields: Application to the Rusck Field-to-Transmission Line Coupling Model
Abstract
:1. Introduction
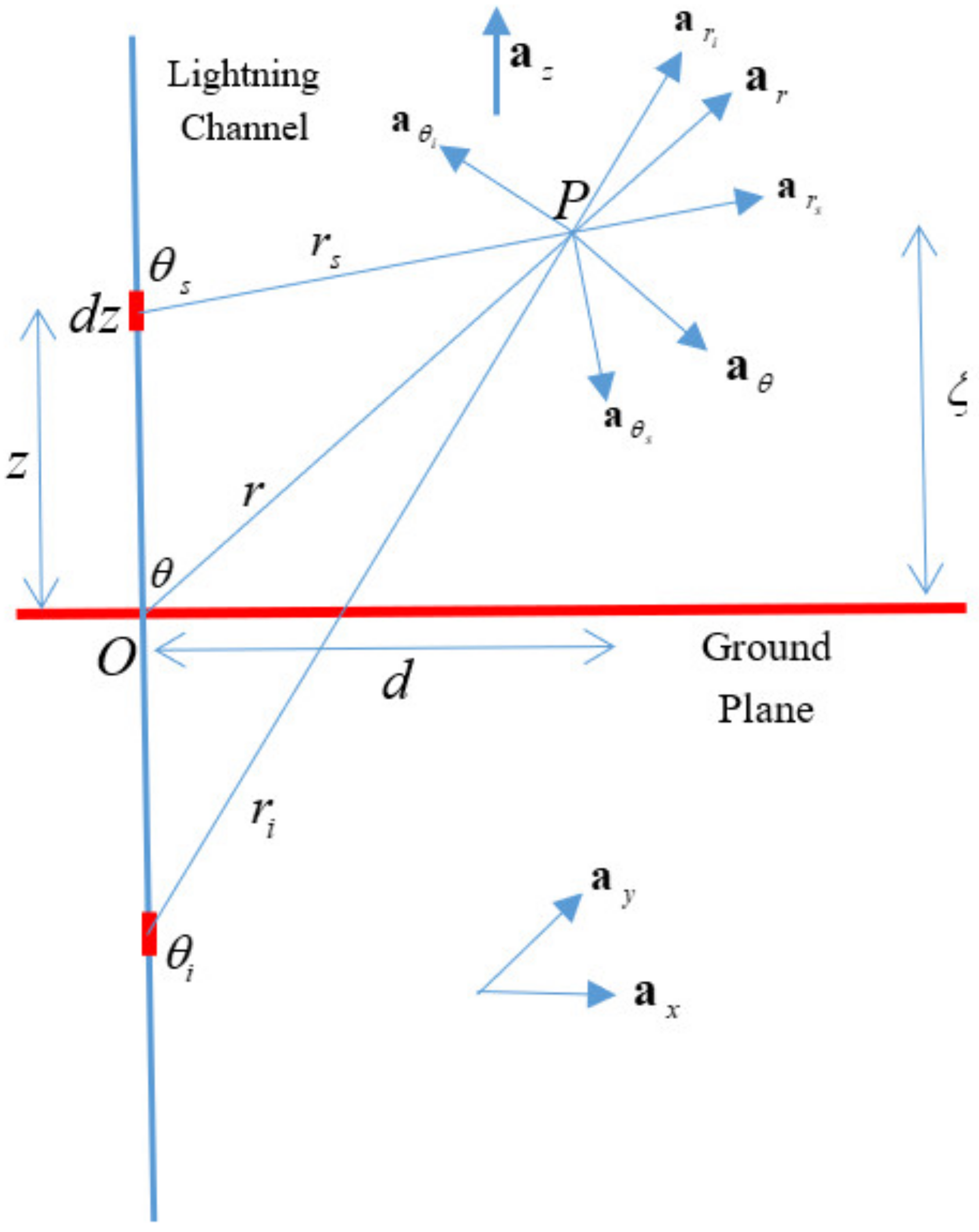
2. Problem Formulation
3. Electric Field of the Return Stroke
3.1. Expressions for the Vertical and Horizontal Electric Fields Based on Rusck’s Formulation
3.2. Exact Expressions for the Electric Field at Any Point in Space
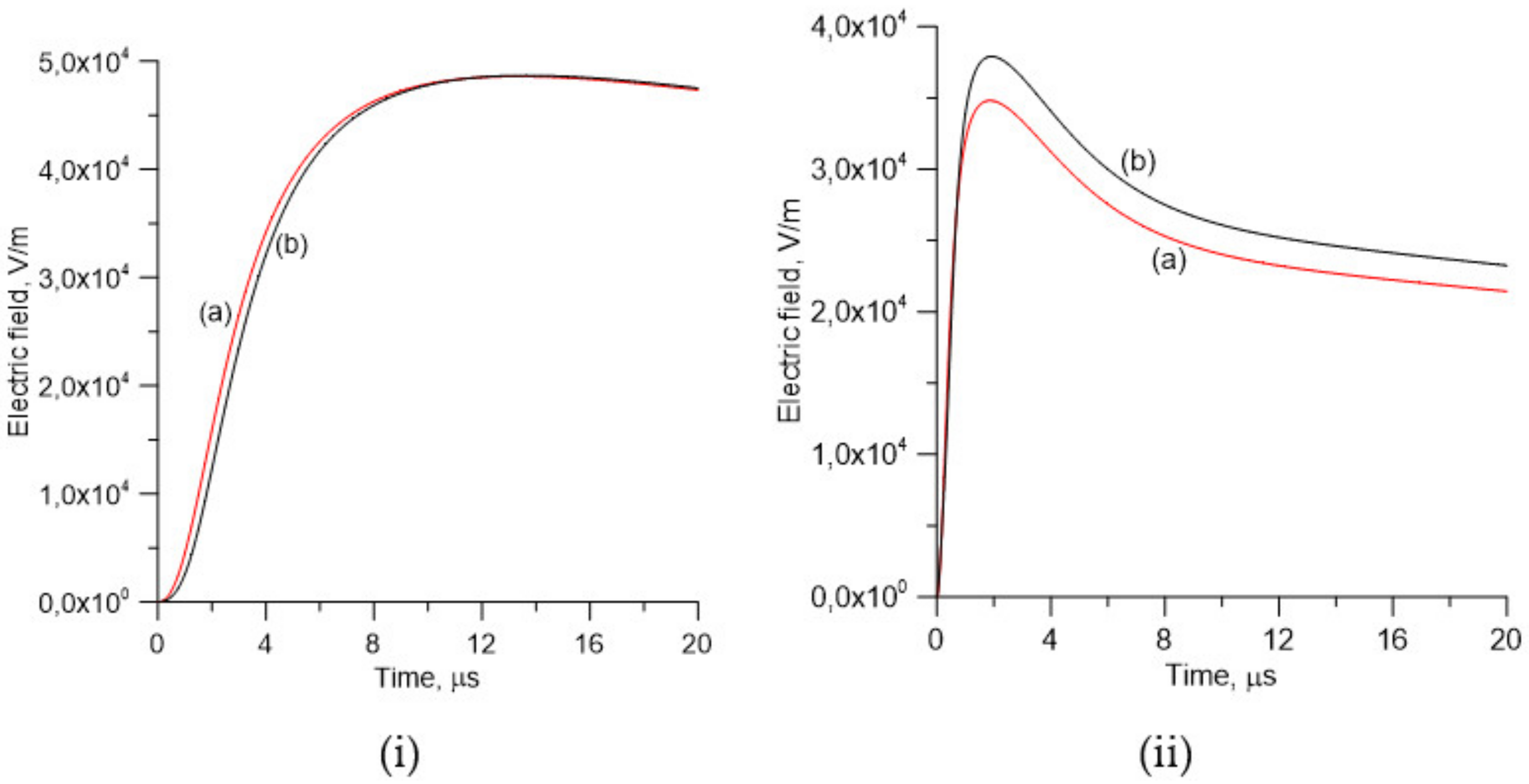
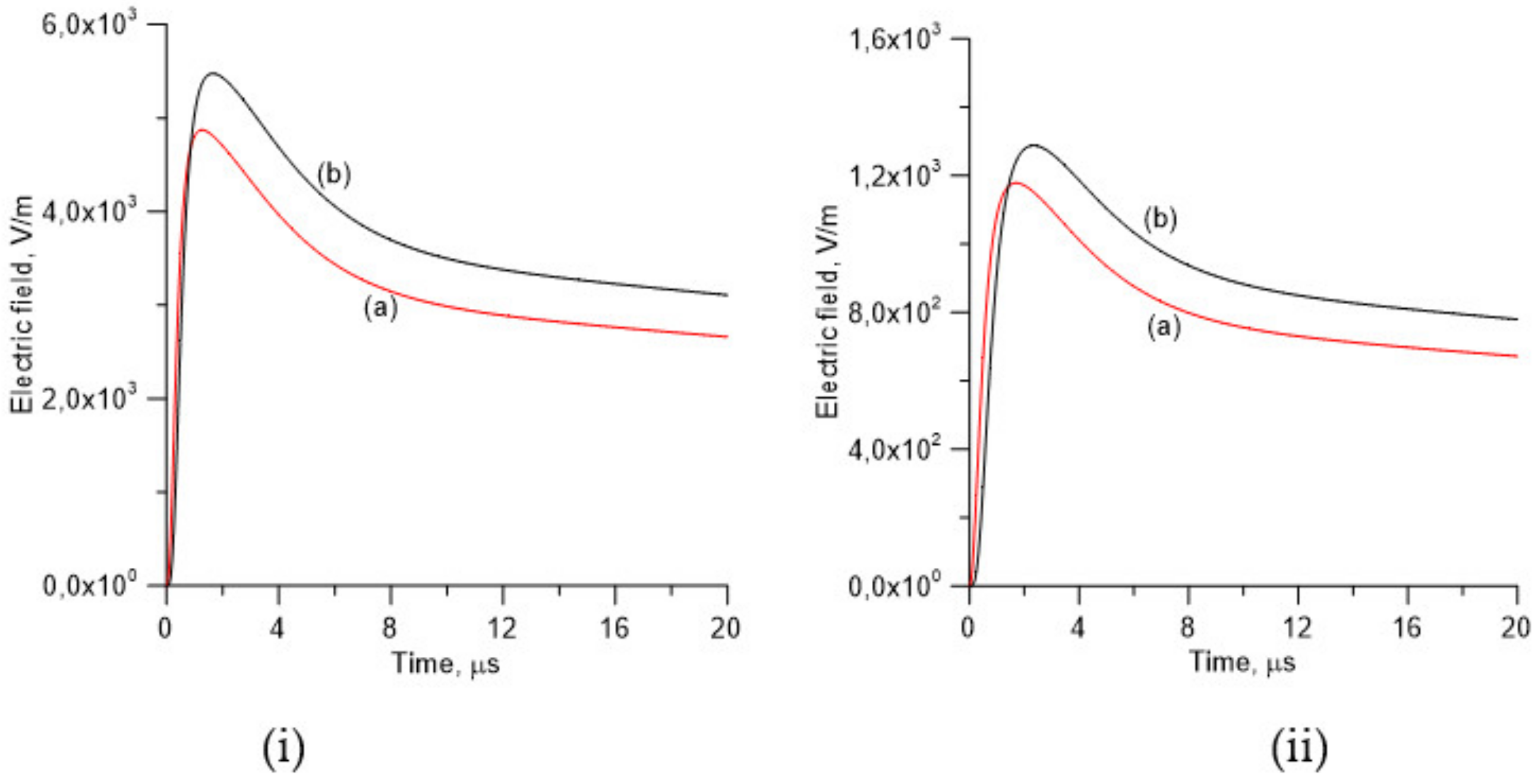
4. Comparison of Rusck’s Expression with the Exact Vertical Electric Field at any Given Point in Space
5. Exact Analytical Expression for the Electric Field of a Step Current Pulse at any Point in Space
Electric Field at Ground Level
6. Exact Analytical Expression for the Electric Field of an Impulse Current Pulse at any Point in Space
7. Discussion
8. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Nucci, C.A.; Rachidi, F.; Rubinstein, M. Interaction of Lightning-Generated Electromagnetic Fields with Overhead and Underground Cables; IET: London, UK, 2012. [Google Scholar]
- Paulino, J.O.S.; Barbosa, C.F.; Lopes, I.J.S.; Boaventura, W.C.; Cardoso, E.N.; Guimarães, M.F. Lightning protection of overhead distribution lines installed on high resistivity soil. Electr. Power Syst. Res. 2022, 209, 107952. [Google Scholar] [CrossRef]
- Paulino, J.O.S.; Barbosa, C.F. Effect of high-resistivity ground on the lightning performance of overhead lines. Electr. Power Syst. Res. 2019, 172, 253–259. [Google Scholar] [CrossRef]
- Paulino, J.O.S.; Barbosa, C.F.; Lopes, I.J.S.; Boaventura, W.C. Assessment and analysis of indirect lightning performance of overhead lines. Electr. Power Syst. Res. 2015, 118, 55–61. [Google Scholar] [CrossRef]
- RUSCK, S. Induced Lightning Over-Voltages on Power-Transmission Lines with Special Reference to the Over-Voltage Protection of Low Voltage Networks; KTH: Stockholm, Sweden, 1958. [Google Scholar]
- Agrawal, A.; Price, H.; Gurbaxani, S. Transient response of multiconductor transmission lines excited by a nonuniform electromagnetic field. In Proceedings of the 1980 Antennas and Propagation Society International Symposium, Quebec, Canada, 2–6 June 1980; Volume 18, pp. 432–435. [Google Scholar]
- Taylor, C.; Satterwhite, R.; Harrison, C. The response of a terminated two-wire transmission line excited by a nonuniform electromagnetic field. IEEE Trans. Antennas Propag. 1965, 13, 987–989. [Google Scholar] [CrossRef]
- Rachidi, F. Formulation of the field-to-transmission line coupling equations in terms of magnetic excitation field. IEEE Trans. Electromagn. Compat. 1993, 35, 404–407. [Google Scholar] [CrossRef]
- Cooray, V.; Rubinstein, M.; Rachidi, F. Field-to-Transmission Line Coupling Models With Special Attention to the Cooray–Rubinstein Approximation. IEEE Trans. Electromagn. Compat. 2020, 63, 484–493. [Google Scholar] [CrossRef]
- Cooray, V. Calculating lightning-induced overvoltages in power lines. A comparison of two coupling models. IEEE Trans. Electromagn. Compat. 1994, 36, 179–182. [Google Scholar] [CrossRef]
- Nucci, C.A.; Rachidi, F. On the contribution of the electromagnetic field components in field-to-transmission line interaction. IEEE Trans. Electromagn. Compat. 1995, 37, 505–508. [Google Scholar] [CrossRef]
- Piantini, A. Extension of the Rusck Model for calculating lightning-induced voltages on overhead lines considering the soil electrical parameters. IEEE Trans. Electromagn. Compat. 2016, 59, 154–162. [Google Scholar] [CrossRef]
- Nucci, C.A.; Rachidi, F.; Ianoz, M.; Mazzetti, C. Comparison of two coupling models for lightning-induced overvoltage calculations. IEEE Trans. Power Deliv. 1995, 10, 330–339. [Google Scholar] [CrossRef]
- Cooray, V. Return stroke models with special attention to engineering applications. In The Lightning Flash, 2nd ed; Cooray, V., Ed.; IET: London, UK, 2014. [Google Scholar]
- Zhang, Q.; Shi, L.; Chen, H.; Qiu, S. Channel charge density behind commonly used return stroke engineering model. Electr. Power Syst. Res. 2022, 210, 108109. [Google Scholar] [CrossRef]
- Brignone, M.; Mestriner, D.; Procopio, R.; Delfino, F. A review on the return stroke engineering models attenuation function: Proposed expressions, validation and identification methods. Electr. Power Syst. Res. 2019, 172, 230–241. [Google Scholar] [CrossRef]
- Huang, L.; Meng, J. Bidirectional Return-Stroke Model for the Calculation of Lightning Electromagnetic Fields. IEEE Trans. Electromagn. Compat. 2020, 62, 2483–2490. [Google Scholar] [CrossRef]
- Uman, M.A.; McLain, D.K. Magnetic field of lightning return stroke. J. Geophys. Res. 1969, 74, 6899–6910. [Google Scholar] [CrossRef]
- Rachidi, F.; Nucci, C.A. On the Master, Uman, Lin, Standler and the modified transmission line lightning return stroke current models. J. Geophys. Res. Atmos. 1990, 95, 20389–20393. [Google Scholar] [CrossRef]
- Nucci, C.A.; Rachidi, F. Interaction of electromagnetic fields with electrical networks generated by lightning. In The Lightning Flash: Physical and Engineering Aspects; IEE Press: London, UK, 2003; Volume 8. [Google Scholar]
- Rakov, V.A.; Dulzon, A.A. A Modified Transmission Line Model for Lightning Return Stroke Field Calculation. In Proceedings of the 9th International Symposium on Electromagnetic Compatibility, Zurich, Switzerland, 12–14 March 1991; pp. 229–235. [Google Scholar]
- Cooray, V.; Orville, R.E. The effects of variation of current amplitude, current risetime, and return stroke velocity along the return stroke channel on the electromagnetic fields generated by return strokes. J. Geophys. Res. Atmospheres 1990, 95, 18617–18630. [Google Scholar] [CrossRef]
- Cooray, V.; Rubinstein, M.; Rachidi, F. Modified Transmission Line Model with a Current Attenuation Function Derived from the Lightning Radiation Field—MTLD Model. Atmosphere 2021, 12, 249. [Google Scholar] [CrossRef]
- Rubinstein, M.; Uman, M.A. Transient electric and magnetic fields associated with establishing a finite electrostatic dipole, revisited. IEEE Trans. Electromagn. Compat. 1991, 33, 312–320. [Google Scholar] [CrossRef]
- Napolitano, F. An analytical formulation of the electromagnetic field generated by lightning return strokes. IEEE Trans. Electromagn. Compat. 2010, 53, 108–113. [Google Scholar] [CrossRef]
- Andreotti, A.; Assante, D.; Mottola, F.; Verolino, L. An exact closed-form solution for lightning-induced overvoltages calculations. IEEE Trans. Power Deliv. 2009, 24, 1328–1343. [Google Scholar] [CrossRef]
- Rachidi, F.; Nucci, C.A.; Ianoz, M.; Mazzetti, C. Influence of a lossy ground on lightning-induced voltages on overhead lines. IEEE Trans. Electromagn. Compat. 1996, 38, 250–264. [Google Scholar] [CrossRef]
- Cooray, V. On the various approximations to calculate lightning return stroke-generated electric and magnetic fields over finitely conducting ground. In Lightning Electromagnetics; IET: London, UK, 2012. [Google Scholar]
- Li, D.; Luque, A.; Rachidi, F.; Rubinstein, M.; Azadifar, M.; Diendorfer, G.; Pichler, H. The propagation effects of lightning electromagnetic fields over mountainous terrain in the earth-Ionosphere waveguide. J. Geophys. Res. Atmos. 2019, 124, 14198–14219. [Google Scholar] [CrossRef] [PubMed]
- Cooray, V.; Rachidi, F.; Rubinstein, M. Formulation of the field-to-transmission line coupling equations in terms of scalar and vector potentials. IEEE Trans. Electromagn. Compat. 2017, 59, 1586–1591. [Google Scholar] [CrossRef]
- Cooray, V.; Scuka, V. Lightning-induced overvoltages in power lines: Validity of various approximations made in overvoltage calculations. IEEE Trans. Electromagn. Compat. 1998, 40, 355–363. [Google Scholar] [CrossRef]
- Rubinstein, M. An approximate formula for the calculation of the horizontal electric field from lightning at close, intermediate, and long range. IEEE Trans. Electromagn. Compat. 1996, 38, 531–535. [Google Scholar] [CrossRef]
- Barbosa, C.F.; Paulino, J.O.S. Effect of Rusck’s Approximation on the Indirect Lightning Performance Assessment of Aerial Power Lines. IEEE Trans. Electromagn. Compat. 2020, 63, 181–188. [Google Scholar] [CrossRef]
- Thottappillil, R.; Rakov, V.A. On different approaches to calculating lightning electric fields. J. Geophys. Res. Atmos. 2001, 106, 14191–14205. [Google Scholar] [CrossRef]
- Cooray, V.; Cooray, G.; Rubinstein, M.; Rachidi, F. Generalized electric field equations of a time-varying current distribution based on the electromagnetic fields of moving and accelerating charges. Atmosphere 2019, 10, 367. [Google Scholar] [CrossRef]
- Cooray, V.; Cooray, G. The electromagnetic fields of an accelerating charge: Applications in lightning return-stroke models. IEEE Trans. Electromagn. Compat. 2010, 52, 944–955. [Google Scholar] [CrossRef]
- Heidler, F. Traveling current source model for LEMP calculation. In Proceedings of the Proceeding 6th International Zurich Symposium on Electromagnetic Compatibility, Zurich, Switzerland, 5–7 March 1985. [Google Scholar]
- Cooray, V.; Cooray, G.; Rubinstein, M.; Rachidi, F. On the Apparent Non-Uniqueness of the Electromagnetic Field Components of Return Strokes Revisited. Atmosphere 2021, 12, 1319. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cooray, V.; Cooray, G.; Rubinstein, M.; Rachidi, F. Exact Expressions for Lightning Electromagnetic Fields: Application to the Rusck Field-to-Transmission Line Coupling Model. Atmosphere 2023, 14, 350. https://doi.org/10.3390/atmos14020350
Cooray V, Cooray G, Rubinstein M, Rachidi F. Exact Expressions for Lightning Electromagnetic Fields: Application to the Rusck Field-to-Transmission Line Coupling Model. Atmosphere. 2023; 14(2):350. https://doi.org/10.3390/atmos14020350
Chicago/Turabian StyleCooray, Vernon, Gerald Cooray, Marcos Rubinstein, and Farhad Rachidi. 2023. "Exact Expressions for Lightning Electromagnetic Fields: Application to the Rusck Field-to-Transmission Line Coupling Model" Atmosphere 14, no. 2: 350. https://doi.org/10.3390/atmos14020350
APA StyleCooray, V., Cooray, G., Rubinstein, M., & Rachidi, F. (2023). Exact Expressions for Lightning Electromagnetic Fields: Application to the Rusck Field-to-Transmission Line Coupling Model. Atmosphere, 14(2), 350. https://doi.org/10.3390/atmos14020350








