Global Per Capita CO₂ Emission Trends

Abstract

In recent years, carbon emissions have become a hot spot issue, and countries have made efforts to control the increasing rate of CO₂ concentration. Prior studies have mainly focused on the national total carbon emissions, but per capita carbon emissions are still poorly known. Here, we used multiple economic development indices to investigate the dynamics of per capita carbon emissions. Additionally, we used the Mann–Kendall test to assess the directions and magnitudes of trends and to investigate abrupt changes in per capita carbon emissions. Our results showed the highest positive growth rate of 0.439 mts/yr in Oman, and the highest negative growth rate of −0.462 mts/yr in the United Arab Emirates. Hurst Index analysis showed that about 86% of countries will keep the current trends of carbon emissions if current mitigation measures remain unchanged. Furthermore, we analyzed the shift in the center of gravity for per capita carbon emissions and used the contribution decomposition method to identify the drivers for the shift, which changed direction in 2004. The main driver behind the westward shift in the gravity center before 2004 was the fact that carbon emissions grew more strongly in the west than in the east before 2004, while the driver for behind the eastward shift in the gravity center after 2004 was a combination of emission reductions in the west and emission increases in the east. Our results highlighted the importance of understanding that the per capita CO₂ emissions are clearly defined within the context of global carbon neutrality, which can help policymakers set more reasonable targets with which to better achieve carbon neutrality goals.

1. Introduction

According to the Intergovernmental Panel on Climate Change (IPCC 2018) reports, human activities play a dominant role in global climate change, especially with respect to the global warming. Excessive human disturbances have resulted in a higher temperature than pre-industrial levels, with warming ranging from 0.8 °C to 1.2 °C (IPCC 2018). If warming continues at the current rate, global warming could reach 1.5 °C between 2030 and 2052 [1]. As an important greenhouse gas, carbon dioxide (CO₂) has significantly accelerated the process of global warming and will remain the major driving factor for inter-annual variations of carbon budget [2]. Meanwhile, human activities have increased global greenhouse gas emissions over the last few decades, leading to an atmospheric CO₂ content that is 30% higher than pre-industrial levels [3,4]. If the current rate of warming is maintained, the remaining carbon emission quota (the amount of carbon dioxide that can be emitted by controlling the temperature rise below 1.5 °C) will be exhausted within 30 years [5]. Consequently, several countries have made efforts to reduce the emission of CO₂ in order to mitigate the process of global warming. However, most studies were mainly focused on the total carbon emissions; the trends in per capita carbon dioxide emissions are still unknown and should be assessed.

The use of fossil fuels and the production of cement are the main sources of carbon dioxide emissions from human activity. In most cities, carbon dioxide emissions from fossil fuels account for more than 80% of the total emissions [6], and the emissions from fossil fuels have increased. Recently, these emissions have reached almost 10 Pg C/yr, which is the same order of magnitude as for the carbon exchange between the atmosphere, land, and ocean [7]. The impact of carbon dioxide generated by fossil fuel combustion on natural carbon fluxes was estimated via satellite observations and other methods [8,9,10,11], but its contribution to natural carbon fluxes is highly uncertain. Ref. [12] put forward a carbon neutralization pathway diagram for the construction industry by studying differences between construction industry carbon emissions in different provinces of China. Economic development also increases carbon dioxide emissions; with each 1% increase in economic development, carbon dioxide emissions increase by approximately 1.05% [13]. A long-term positive correlation between urbanization, energy consumption, and carbon dioxide emissions has been observed, which reflected a two-way causal relationship [14]. However, the specific impact of urbanization on carbon dioxide emissions needs to be further explored [15,16,17]. Carbon dioxide emissions may vary nonlinearly over time, but the C emissions from 17 of the 20 countries with the highest emissions have converged over time [18]. The different levels of emissions per capita for each of these countries reflects their different income levels [19].

To date, research into changing carbon emissions has mainly focused on changes and trends within a single country and/or between different provinces or states [20,21]. Only a few large-scale studies analyzed and compared the carbon emission changes between different countries [22], which may depend on the stage of development [9]. Many studies have looked at the spatial evolution of the center of gravity shift for carbon emissions through gravity models and other methods and have explored the direction of the shift for different regions and the driving factors behind it [23,24,25]. However, these studies have generally focused on a small area. The carbon emission reduction targets that are currently set require different actions to be taken by different countries in order to achieve the same reduction in carbon emissions. The large discrepancy between the cost of carbon emissions reduction in developed and developing countries will lead to the changes in the effectiveness of the implemented actions to reduce carbon emissions [26]. Moreover, population size is one of the most important factors affecting carbon dioxide emissions, and differences in population size mean that the same targets have different implications when applied to countries with different population sizes [27]. Thus, measuring the reduction in carbon emissions from a country only from the total emissions is unreasonable.

To explore trends in global per capita CO₂ emissions and the results in the center of gravity shift, here, using the dataset published by World Bank and multiple methods (i.e., Mann–Kendall (MK) analysis, gravity center shift, and the Hurst Index (see Methods)), we quantified the per capita carbon emissions for most countries and regions in the world to assess the growth distribution of global per capita carbon emissions. We then determined whether and when any change in the carbon emissions per capita had occurred in different countries during the study period. We also estimated the future trend for each country and derived the future trend in per capita CO₂ emissions for each country if no other policy controls were imposed. Finally, we calculated the center of gravity shift trajectory and identified the 20 countries that contributed the most positively and negatively to the center of gravity shift in different years by using the contribution decomposition method for each country’s contribution to the inter-annual center of gravity shift.

The contributions of this study include the following: first, we clarified the trend of changes in per capita carbon emissions in various countries; second, we used the Hurst Index to evaluate future trends and predict the changes in per capita CO₂ emissions in the future if countries do not take other measures; third, we clarified the contribution of each country to the shift of gravity center with respect to per capita CO₂ emission. This can provide a theoretical reference according to which countries can formulate emission reduction policies in the future.

2. Data and Methods

2.1. Data

Data that include almost all economic development indices for most countries and regions for 1992–2018 were obtained from the World Bank (https://databank.worldbank.org/source/world-development-indicators, accessed on 23 February 2023). The World Band database included more than 200 countries and regions in the world, including economic, financial, demographic, environmental, education, and health care, there are 52 categories and a total of 1444 subcategories in the dataset. In the present study, we only considered carbon emissions per capita, which can be considered a sub-indicator under the other economic indicators. It included a total of 266 countries, regions, or communities, but some of which have valid data. Based on the integrity of the data, we selected 190 countries and regions to use in our study.

2.2. Methods

2.2.1. Mann–Kendall (MK) Test

Mann–Kendall (MK) analysis is based on the rank of the data, rather than on the data themselves, and can be used to analyze time series data with an unstable central tendency [28,29]. It treats outliers more appropriately than other methods [30]. The MK test is applied to analyze time series data with a continuous increasing or decreasing trend (monotonic trend). It is a non-parametric test that can be applied to all distributions (i.e., the data need not be normally distributed), provided that the data are not serially correlated. If the data are serially correlated, this will have an impact on the significance level (p-value). Our MK analysis involved the MK trend test and the MK change test. The calculation for the MK trend test is as follows:

$$\begin{array}{c}\mathrm{\beta }=\mathrm{m}\mathrm{e}\mathrm{d}\mathrm{i}\mathrm{a}\mathrm{n}\left(\frac{X_j-X_i}{j-i}\right) \forall 1<i<j<n,\end{array}$$

(1)

where $\mathsf{\beta }$ is the MK trend value. If $\mathsf{\beta }>0$, the data have an increasing trend. If $\mathsf{\beta }<0$, the data have a decreasing trend. The larger the absolute value of $\mathsf{\beta }$, the stronger the trend in the data. The steps to calculate the MK trend test are as follows:

(1)

Construct the rank sequence:

$$\begin{array}{c}{S}_k={\displaystyle \sum _{i=1}^k}{r}_i\left(k=2, 3, \dots n\right),\end{array}$$

(2)

$$\begin{array}{c}{r}_i=\left\{\begin{array}{c}1, x_i>x_j\\ 0, x_i\le x_j\end{array}\right.\left(0<j<i\right),\end{array}$$

(3)

where $S_k$ is the rank sequence after replacing the original data; $k$ is the length of the data sequence used to calculate the rank for a specific year; and $r_i$ is the cumulative number for the $i_{th}$ data point in the sequence of data used for that year.

(2)

Forward statistic, ${UF}_k$:

$$\begin{array}{c}{UF}_k=\frac{S_k-E\left(S_k\right)}{\sqrt{Var\left(S_k\right)}}\left(k=2, 3,\dots ., n\right),\end{array}$$

(4)

where ${UF}_k$ is the standard normal distribution statistic; ${UF}_1=0$; $E\left(S_k\right)$ is the mean of $S_k$; $Var\left(S_k\right)$ is the variance of $S_k$; and $E\left(S_k\right)$ and $Var\left(S_k\right)$ are calculated as follows:

$$\begin{array}{c}E\left(S_k\right)=\frac{n\ast \left(n-1\right)}{4},\end{array}$$

(5)

$$\begin{array}{c}Var\left(S_k\right)=\frac{n\ast \left(n-1\right)\ast \left(2\ast n+5\right)}{72},\end{array}$$

(6)

where $n$ is the total number of data sequences.

(3)

Backward statistic ${UB}_k$

Reverse the original data, repeat Step (1) and Step (2), and reverse the calculation results to obtain the reverse statistic, ${UB}_k$; ${UB}_1=0$.

Where the forward statistic, ${UF}_k$, has a value greater than 0, the data series has an increasing trend, otherwise it has a decreasing trend. For a given significance level (e.g., p < 0.05), any portion of ${UF}_k$ that exceeds the critical value represents a significant increasing or decreasing trend. If there is an intersection between ${UF}_k$ and ${UB}_k$ (i.e., a point in the sequence where both values are the same), and the value here exceeds the critical value, then the intersection marks the time point when a change starts. If ${UF}_k$ shows an increasing (decreasing) trend at this point, then the change is a change to an increasing (decreasing) trend.

2.2.2. Hurst Index

The Hurst Index (H) is used to indicate whether time series data follow a random walk or a biased random walk and is calculated using the rescaled range method (R/S) [31]. It was first used to study inflows and outflows between reservoirs and rivers and has since been used in many fields to study data development laws in fields such as energy consumption [32] and natural disasters [33]. The Hurst Index describes the autocorrelation of stationary time series data over a long time period [34]. To calculate the Hurst Index using the R/S method, conduct the following:

(1)

$X$ is a time series of length $M$. Construct a logarithmic difference sequence with length $N=M-1$:

$$\begin{array}{c}{N}_i=\log \left(\frac{X_{i+1}}{X_i}\right), i=1, 2,\dots , M-1 .\end{array}$$

(7)

(2)

Equate a logarithmic sequence of length $N$ in $A$ subsets, each of length $n=N/A$. Calculate the mean value of each subset ($e_a, a=1, 2,\dots , A)$:

$$\begin{array}{c}{e}_a=\frac{1}{n}{\displaystyle \sum _{t=1}^n}{N}_t, a=1, 2,\dots ,A .\end{array}$$

(8)

(3)

Within each subset, $a$, calculate the cumulative deviation of the first $k$ points ($k=\mathrm{1,2},\dots ,n$), relative to the mean $e_a$ for that subset:

$$\begin{array}{c}{X}_{k,a}={\displaystyle \sum _{i=1}^k}\left(N_{i,a}-e_a\right), k=\mathrm{1,2}, \dots ,n.\end{array}$$

(9)

(4)

Calculate the range of the fluctuations in the logarithmic series within each subset $a$:

$$\begin{array}{c}{R}_a=\max \left(X_{k,a}\right)-\min \left(X_{k,a}\right), 1\le k\le n .\end{array}$$

(10)

(5)

Calculate the standard deviation of the log-return series within each subset $a$:

$$\begin{array}{c}{S}_a=\sqrt{\frac{1}{n}{\displaystyle \sum _{i=1}^k}{\left(N_{i,a}-e_a\right)}^2}, 1\le k\le n .\end{array}$$

(11)

From the definition of the Hurst exponent, we know that it describes the positive relationship between ${\left(R/S\right)}_n$ and $n^H$, ${\left(R/S\right)}_n=C\times n^H$. Take the logarithm of both sides at the same time, $\log \left({\left(R/S\right)}_n\right)=H\times \log \left(n\right)+\log \left(C\right)$. $H$ is the Hurst Index. The following three types of $H$ can determine whether the future trend is continuous or completely random: When $0\le H<0.5$, the trend is anti-sustainable; that is, the trend in the future opposes the past trend. When $0.5<H\le 1$, the trend is continuous; that is, the future trend is consistent with the past trend. When $H=0.5$, the future trend is random.

2.2.3. Gravity Center Shift

The center of gravity was originally a physical concept, referring to a point within a region at which the force received in all directions is balanced. In recent years, the center of gravity model has also been applied to studies of population migration and economic development [35,36]. The direction and extent of the spatial offset of a changing center of gravity position reflect the spatial distribution patterns and evolution characteristics of an element. Analyzing the migration trajectory for the center of gravity is therefore a useful means of analyzing the spatial and temporal patterns of the element. Many studies have also used this method to assess the transfer of carbon emissions [27,37]:

$$\begin{array}{c}{X}_t={\displaystyle \sum _{i=1}^n}\left(C_{ti}\ast X_{ti}\right)/{\displaystyle \sum _{i=1}^n}{C}_i,\end{array}$$

(12)

$$\begin{array}{c}{Y}_t={\displaystyle \sum _{i=1}^n}\left(C_{ti}\ast Y_{ti}\right)/{\displaystyle \sum _{i=1}^n}{C}_i,\end{array}$$

(13)

where $X_t$ and $Y_t$ represent the x and y coordinates of the gravity center for global per capita carbon emissions in year $t$; $C_{ti}$ are the per capita carbon emissions from the $i_{th}$ country in year $t$; and $X_{ti}$ and $Y_{ti}$ are the x and y coordinates for the $i_{th}$ country.

2.2.4. Contribution Decomposition Method (CDM)

The contribution decomposition method (CDM) is a technique for assessing the contribution of specific regions to the movement of the center of gravity [38]. If there are $n$ small regions within a large region, the contribution of region $i$ to the center of gravity, ${MC}_{xi}$, is calculated by the following:

$$\begin{array}{c}{MC}_{xi}=∆X\left(n\right)-∆X\left(n/i\right),\end{array}$$

(14)

where $∆X\left(n\right)$ is the longitudinal displacement of the center of gravity when region $i$ is included in the assessment, and $∆X\left(n/i\right)$ is the longitudinal displacement when region $i$ is excluded from the assessment. The contribution of region $i$ to the latitudinal displacement, ${MC}_{yi}$, is calculated as follows:

$$\begin{array}{c}{MC}_{yi}=∆Y\left(n\right)-∆Y\left(n/i\right),\end{array}$$

(15)

where $∆Y\left(n\right)$ is the latitudinal displacement of the center of gravity when region $i$ is included in the assessment, and $∆Y\left(n/i\right)$ is the latitudinal displacement when region $i$ is excluded.

To reduce the influence of different geographical locations and other regions on the shift of the center of gravity when region $i$ is excluded, we replace region $i$ with the carbon emissions per capita from region $i$ in the last year.

3. Results

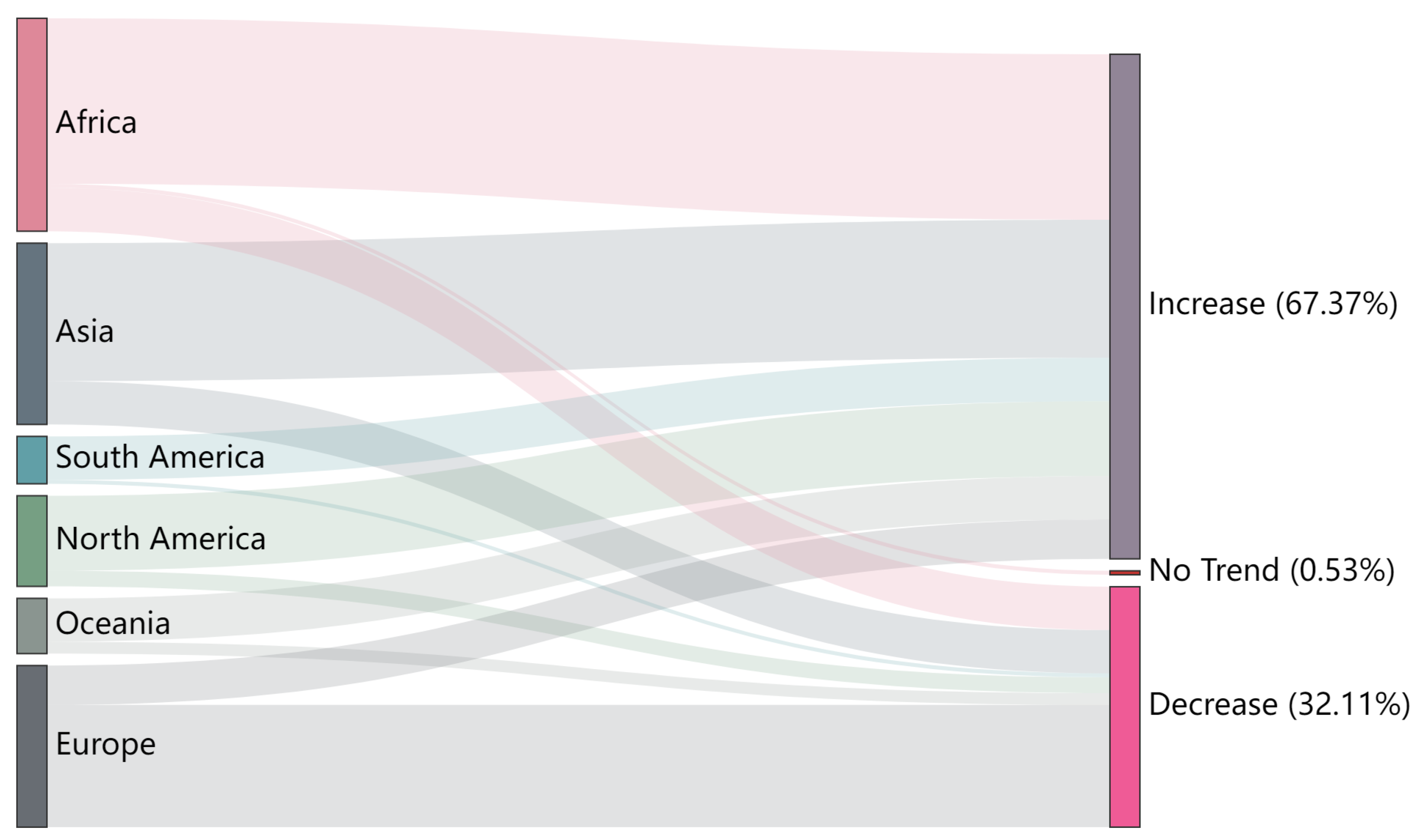
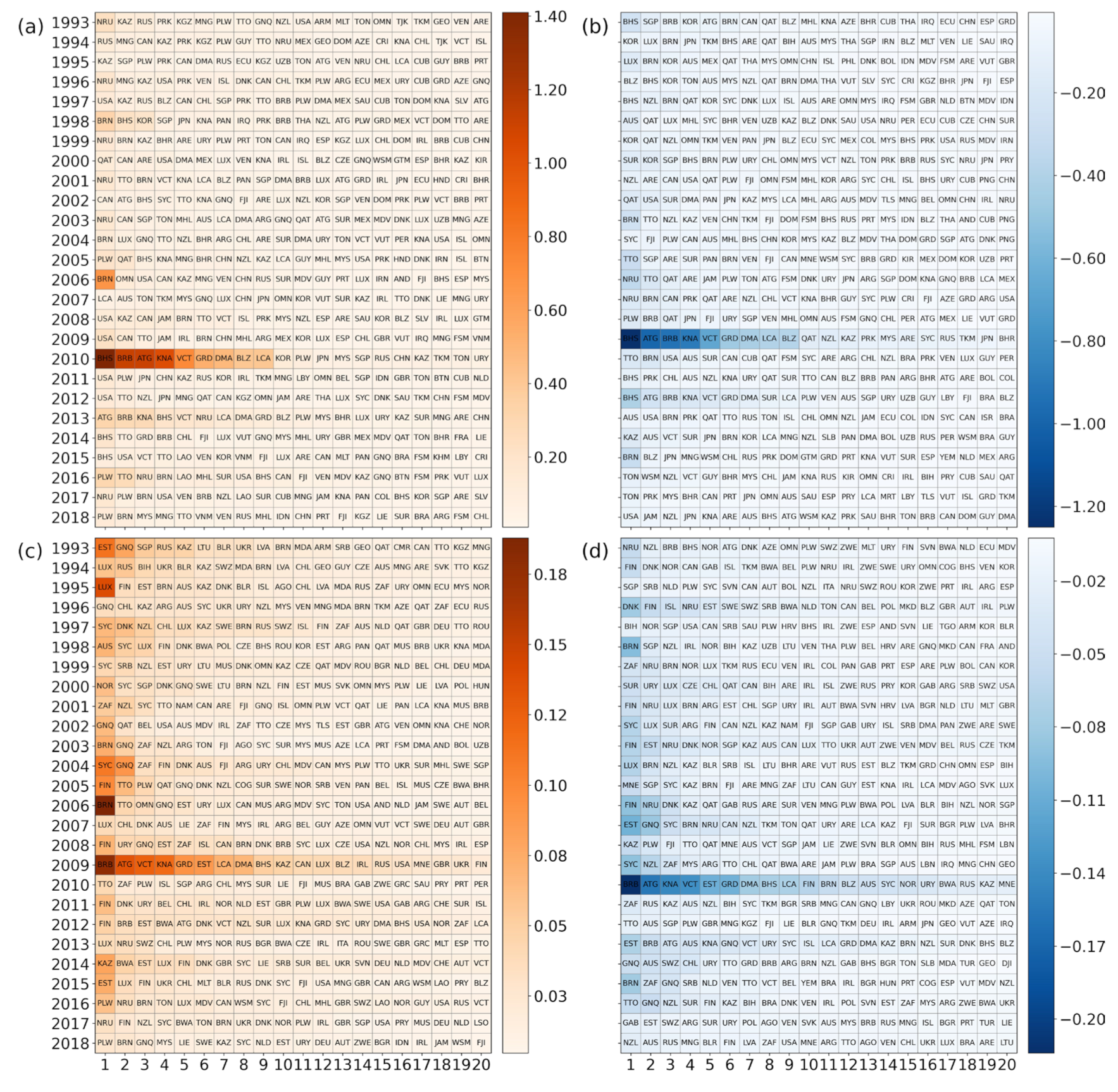
3.1. MK Trend Test

The carbon emissions per capita from 190 countries were included in our study. The per capita emissions from 128 of these countries exhibited a positive growth rate, constituting 67.37% of the total per capita emissions. Conversely, the per capita emissions from 61 countries displayed a negative growth rate, contributing to 32.11% of the total per capita emissions. Notably, only one country, South Sudan, experienced a zero growth rate in emissions. Figure 1 shows the trends for the per capita emissions from each continent.

Figure 1 shows that the net trend for per capita carbon emissions for all continents is positive growth. 91.67% of countries in South America and 82.6% of countries in North America had positive growth trends. Countries with a positive growth trend accounted for 78.57%, 77.78%, and 76.08% of the per capita emissions from Oceania, Africa, and Asia, respectively. Europe had the smallest increase in per capita carbon emissions of all the continents, and countries with a positive growth trend accounted for 24.39% of the total per capita emissions from Europe.

Figure 2 shows the density distributions for the growth rate of per capita carbon emissions from all continents. It can be seen from the figure that the growth rate for most of Europe is negative, while the peak for other continents corresponds to slight positive growth. The distribution is particularly narrow for South America and Africa, and the peak is slightly higher for South America than for Africa. The density distribution for the growth rate in Asia is the broadest, showing the growth rate here to be more decentralized than for the other continents. Compared with other continents, the frequency of high growth rates in Asia is higher. There is a secondary peak in the distribution for Oceania at around a growth rate of −0.3 mts/yr.

Table 1. Twenty countries with the strongest rates of increase and decrease.

Country Code	Increase Rate	Country Code	Decrease Rate
OMN	0.438733	ARE	−0.46155
GNQ	0.272753	LUX	−0.37608
TKM	0.266631	NRU	−0.28774
SAU	0.261462	DNK	−0.26567
TTO	0.25524	USA	−0.20121
CHN	0.244688	BHR	−0.16523
BIH	0.209024	BEL	−0.16132
KOR	0.185939	GBR	−0.15022
MNE	0.176898	FIN	−0.14466
IRN	0.176237	PRK	−0.13669
MYS	0.175769	SWE	−0.13565
KAZ	0.148168	UKR	−0.12939
BRN	0.123302	SGP	−0.12824
MNG	0.120517	CZE	−0.12356
SYC	0.120106	AZE	−0.12325
MDV	0.110251	LIE	−0.12103
CHL	0.093517	SVK	−0.10171
TUR	0.08693	DEU	−0.08428
ZAF	0.086919	GAB	−0.08306
MUS	0.084493	ISL	−0.07974

lists the countries with the twenty highest positive and negative growth rates. Twelve of the strongest twenty countries for growth are in Asia, accounting for 60% of the top twenty growth countries. The area around 30° N is the most concentrated area of countries with high growth rates. This area includes four countries in Africa; two countries in Europe; and one country each in North and South America. Europe has the greatest number of countries with negative growth rates in the top twenty. These twelve European countries account for 60% of all countries in the top-twenty list for strong negative growth, which also includes five countries in Asia and one country each in North America, Africa, and Oceania.

3.2. Future Trends in Per Capita Carbon Emissions

We calculated the Hurst Index for the per capita carbon emissions from every country in the world. We found that 165 countries, accounting for 86.84% of all countries, had a Hurst Index greater than 0.5, while 25 countries, representing 13.16% of all countries, had a Hurst Index less than 0.5. None of the countries exhibited a Hurst Index of 0.5. This indicates that the future per capita carbon emissions from most countries will continue to follow the current trend.

We combined the Hurst Index with the current growth rate to estimate future per capita carbon emissions from each country. We divided the future trends into five types: persistent decrease (increase rate < 0, H > 0.5, decrease expected to continue into the future), persistent increase (increase rate > 0, H > 0.5, increase expected to continue into the future), anti-persistent decrease (increase rate < 0, H < 0.5, current trend in per capita carbon emissions expected to reverse and become an increasing trend in the future), anti-persistent increase (increase rate > 0, H < 0.5, current trend in per capita carbon emissions expected to reverse and become a decreasing trend in the future), and unidentified (H = 0.5, random changes in future trends expected).

As shown in Figure 3, the emissions trends from most countries are likely to persist into the future. A persistent decrease is anticipated for 57 countries, accounting for 30.00% of all countries, and these countries are mainly concentrated in Europe (except Central Europe), areas near 0° in Africa, and the border region between Asia and Europe. There are 37 countries in Europe, 11 countries in Asia, 11 countries in Africa, 4 countries in North America, 3 countries in Oceania, and 1 country in South America for which a persistent decrease is expected. A persistent increase is expected for 108 countries, accounting for 56.84% of all countries, and most of these countries (36) are in Africa. Africa is followed by Asia and North America, where persistent increases are expected for 31 and 15 countries, respectively, and then by North America, Oceana, and Europe, in which a persistent increase is expected for 9, 9, and 8 countries, respectively. An anti-persistent decrease is expected for only five countries, accounting for 2.63% of all countries: four in Europe and one in Africa. An anti-persistent increase is expected for 20 countries, accounting for 10.53% of all countries, and Africa accounts for the greatest number of these (6). Africa is followed by Asia and North America, each of which include four countries for which an anti-persistent increase is expected. Two countries in each of North America, Oceania, and Europe are also associated with an expected anti-persistent increase.

3.3. MK Change Test

Figure 4 shows the years when an MK change occurred for the first time for each country. During 1992 to 2018, 77 out of 190 countries experienced a change-point: Europe accounted for the greatest number of countries (21 (27.27%)), followed by Africa and Asia, which accounted for 18 and 17 countries (23.38% and 22.08%), respectively. North America, Oceania, and South America accounted for 11 (14.29%), 6 (7.80%), and 4 (5.19%) countries that experienced a change-point, respectively. The proportion of countries in Europe where the trend is likely to mutate is 52.5%, the highest proportion for any continent, followed by North America at 47.8% and Oceania at 42.9%. Asia, Africa, and South America have the lowest proportions of mutation countries at 36.9%, 33.9%, and 33.3%, respectively.

The number of change-points was greatest in 1994 and smallest in 1999. From 2009 to 2013, the number of change-points increased year by year, and the distribution of change-points over the remaining years was relatively uniform. Changes to increasing trends were more concentrated before 1998, and changes to decreasing trends became clearer after 2011. The distribution of ascending and descending trend mutations was more random in other periods and the number of changes to increasing trends was 42, slightly higher than the number of changes to decreasing trends, which was 35. In 1994 and 2015, changes occurred in two Asia countries. When changes occurred in Asia in other years, they only occurred in one country and were generally a change to an increasing trend. In Europe, changes occurred in different years in different countries, with a gap between those where the change occurred earliest and those countries where the change followed after a lag. Changes in Europe are mainly concentrated after 2003, and most changes were to decreasing trends. In North America, change-points were mainly concentrated at the beginning and the end of the study period. At the start of the study period, most changes were to increasing trends, while all changes at the end of the study period were towards decreasing trends. South America had the smallest number of countries with change-points (only four). In 1994, two countries experienced change-points. One transitioning to an increasing trend and the other to a decreasing trend. Additionally, in 1997, another country witnessed a change to an increasing trend, and in 2004, another country observed a shift to an increasing trend. There were two countries with change-points in Africa in 1994, 2001, 2011, and 2014. In other years, there was only one country in Africa with a change-point. Before 2002, the number of changes to increasing and to decreasing trends was similar. After 2002, most changes were to increasing trends. The times for the changes in Oceania countries are discrete and all changes are to increasing trends. The timing of change-points is different for the different continents. The changes in America and Oceania mainly occurred near the start of the study period, while most changes in Europe were concentrated in later stages of the study period. There were more slight changes in Africa towards the end of the study period than at the start, and changes in Asia occurred throughout the study period.

3.4. The Gravity Center Shift for Per Capita Carbon Emissions

Figure 5 shows the annual shift of the gravity center for per capita carbon emissions from 1992 to 2018 and shows that there was a longitudinal shift in three stages. From 1992 to 1998, the gravity center moved westward relatively quickly. From 1999 to 2006, the center of gravity fluctuated longitudinally, moving alternately eastward and westward relatively slowly, eventually moving slightly eastward. From 2007 to 2018, the gravity center moved quickly eastwards. Over the whole study period, the gravity center for per capita carbon emissions moved 1.95° eastwards. In the latitude direction, the center consistently moved southward. From 1992 to 1998, the southward movement was relatively fast; from 1999 to 2006, the rate of southward movement was relatively slow and, occasionally, small northwards movements occurred. From 2006 to 2018, the gravity center continued to move steadily to the south, but the speed gradually slowed down, becoming almost stagnant after 2014. At this same time, the speed of eastward movement accelerated significantly. Over the whole study period, the center of gravity for per capita carbon emissions moved to the south by 4.51°. The distance moved in the second stage is not great in terms of longitude or latitude, and there are some fluctuations in the direction during this stage. We therefore simplified our description of the shift in the center of gravity into two stages. From 1992 to 2004, the gravity center for per capita carbon emissions gradually shifted to the southwest, and, after 2004, it gradually shifted to the southeast until 2018. With the exception of 2009 and 2012, there was a clear shift in the gravity center location.

3.5. The Drivers for the Gravity Center Shift

The trend in the shift for the center of gravity for per capita carbon emissions changed in 2004. Before 2004, the center of gravity moved to the southwest; after 2004, it moved to the southeast. Therefore, we describe the shift in longitude in 2004 and earlier as a positive contribution to the west and a negative contribution to the east; after 2004, there is a longitudinal shift to the east and so a positive contribution to the east and a negative contribution to the west. The near-constant southward movement can be described as a positive contribution to the south and a negative contribution to the north. Figure 6 shows the top-twenty positive and negative contributors to the shift in the gravity center for per capita carbon emissions each year from 1993 to 2018.

Figure 6a shows that Palau (PLW) and Canada (CAN) were the most frequent positive contributors to the longitudinal shift before 2004; both contributed positively eight times, followed by Trinidad and Toba Colombia (TTO), Chile(CHL), Kazakhstan (KAZ), Nauru (NRU), North Korea (PRK), and Saint Kitts and Nevis (KNA), each of which contributed positively to the longitudinal shift seven times. Mexico (MEX), Dominica (DMA), Antigua and Barbuda (ATG), United Arab Emirates (ARE), Singapore (SGP), Tonga (TON), Equatorial Guinea (GNQ), Barbados (BRB), and Luxembourg (LUX) all made positive contributions six times before 2004. After 2004, Mongolia (MNG), China (CHN), the United States (USA), and Luxembourg (LUX) were the most frequent positive-contributing countries for the longitudinal shift; all of these countries contributed positively nine times, followed by the Bahamas (BHS), Kazakhstan (KAZ), Malaysia (MYS), and Mongolia (LUX). Trinidad and Tobago (TTO) contributed positively to the longitudinal shift eight times, and Palau (PLW) and South Korea (KOR) each contributed positively to the longitudinal shift seven times. Figure 6b shows that Malaysia (MYS) contributed negatively to the longitudinal shift ten times before 2004, which is more than any other country; Bahamas (BHS), South Korea (KOR), and Qatar (QAT) each contributed negatively nine times, and China (CHN) contributed negatively eight times. After 2004, Qatar (QAT), St. Kitts and Nevis (KNA), North Korea (PRK), New Zealand (NZL), and Australia (AUS) were the most frequent negative-contributing countries for the longitudinal shift, and each of these countries contributed negatively eight times, followed by the United Arab Emirates (ARE), Canada (CAN), Barbados (BRB), Grenada (GRD), Japan (JPN), and Chile (CHL), each of which contributed negatively seven times. Suriname (SUR), Brunei Darussalam (BRN), Antigua and Barbuda (ATG), Guyana (GUY), and Bahrain (BHR) contributed negatively to the longitudinal shift six times.

As shown in Figure 6c, Seychelles (SYC) was the most frequent positive-contributor to shifts in latitude for the gravity center, contributing positively 16 times, followed by Denmark (DNK), Chile (CHL), Estonia (EST), Finland (FIN), and Luxembourg (LUX), each of which contributed positively 14 times. Malaysia (MYS) and Brunei Darussalam (BRN) each contributed positively to the latitudinal shift for the gravity center 12 times. Figure 6d shows that New Zealand (NZL) made the most frequent negative contribution to the latitudinal shift 14 times through the study period, followed by Palau (PLW), Canada (CAN), Ireland (IRL), and Kazakhstan (KAZ), each of which negatively contributed 12 times, and Singapore (SGP), which contributed negatively 11 times.

The three countries that made the greatest cumulative contribution to longitude shifts in the gravity center before 2004 were Nauru (NRU), Trinidad and Tobago (TTO), and Kazakhstan (KAZ), which contributed 0.886°, 0.307°, and 0.299°, respectively to the westward shift in the center of gravity. The three countries that made the greatest cumulative negative contributions before 2004 were Korea (KOR), Qatar (QAT), and Australia (AUS), which, respectively, contributed 0.437°, 0.417°, and 0.394° to the eastward shift of the center of gravity. After 2004, the three countries with the greatest cumulative positive contributions were the United States (USA), Palau (PLW), and China (CHN), which contributed 0.715°, 0.630°, and 0.322°, respectively, to the eastward shift of gravity center. The top three countries with cumulative negative contributions were Australia (AUS), Qatar (QAT), and North Korea (PRK), which contributed 0.361°, 0.325°, and 0.304°, respectively, to the westward shift of the center of gravity.

The three countries with the greatest positive cumulative contributions to the latitude shift for the gravity center were Luxembourg (LUX), Chile (CHL), and Seychelles (SYC), which contributed 0.351°, 0.199°, and 0.198° to the southward shift of the center of gravity, respectively. The three countries with the greatest negative cumulative contribution were Nauru (NRU), Gabon (GAB), and Montenegro (MNE), which contributed 0.213°, 0.093°, and 0.063° to the northward shift of the center of gravity, respectively.

Taking 1992 and 2004 as base points for the shift of the gravity center for per capita carbon emissions, the total contribution of countries to the left of the base point is the total contribution of western countries, and the total contribution of countries to the right of the base point is the total contribution of eastern countries. Before 2004, the total contribution of western countries is a westward shift of 2.33°, and the total contribution of eastern countries is an eastward shift of 1.03°. This indicates that the overall growth in per capita carbon emissions was greater in western countries than in eastern countries before 2004. After 2004, the total contribution of western countries was an eastward shift of 1.92° and that of eastern countries was an eastward shift of 1.81°, indicating that per capita carbon emissions from western countries entered a generally declining stage, while the per capita carbon emissions from eastern countries continued to increase.

4. Discussion

The increasing rate of global per capita CO₂ emissions us still enhanced, with developed countries being the main drivers of the current reduction in per capita CO₂ emissions, and the per capita CO₂ emissions in developing countries showing a greater volatility. The stable trends of per capita CO₂ emissions in large countries have a greater impact on the center of gravity shift of carbon emissions than occasional sharp fluctuations in small countries.

The changes in global per capita carbon emissions show that trends in most places are continuing as increasing trends; these occur in 67% of the total number of countries in our study and are mainly distributed in Asia and Africa. The countries with decreasing per capita carbon emissions are mainly in Europe, which accounts for more than half of all countries with reduced carbon emissions. Although many countries have started to show a decreasing trend of per capita carbon emissions, many of these trends do not pass the significance test, indicating that the decrease in carbon emissions is still somewhat random and has not yet formed a significant trend. Most of the top-20 countries with increasing trends are located near 30° S or 30° N, and these countries are most highly concentrated around 30° N in Asia. In North America, there is a general increasing trend in per capita carbon emissions; however, there have been large emission reductions in the major countries, and these have significantly offset the increase in carbon emissions from the smaller countries in North America. In regions with higher economic development, abrupt changes in carbon emissions occur more regularly. Near the start of our study period, the abrupt changes are mainly changes towards increasing trends. When development reaches a certain stage, gradual implementation of emission-reduction policies will lead to changes towards decreasing trends. In regions with lower economic development, changes in carbon emissions are more unstable and more susceptible to the impact of factors such as war and environmental change. This is clearest in Africa and Central Asia. The shift of the center of gravity for per capita carbon emission is strongly influenced by countries with large per capita carbon emission levels and many change-points. Before and after 2004, many countries appeared many times as drivers for shifts in the gravity center. However, due to the small magnitude of the changes, they did not make large contributions overall. Large carbon-emitting countries, such as China and the United States, do not appear often during the study period as leading positive drivers for shifts in the gravity center; the impact of changes in these countries is greater because of the high volume of emissions that they produce. These countries, therefore, have a greater responsibility for reducing carbon emissions. Most European countries are developed countries that have entered a post-industrial development period. Industrial emissions are one of the main factors affecting carbon emissions, and the impact of industrial development in these countries is no longer growing; indeed, industrial transformation here has resulted in the reduction of carbon emissions. Europe has become the main driving force for reducing carbon emissions per capita. The number of developed countries on other continents is smaller, and most countries are still developing countries. The rapid development of industry and manufacturing has resulted in considerable increases in carbon dioxide emissions from these countries, which has led to an increase in per capita carbon dioxide emissions, especially in China and India.

Most previous studies have calculated total carbon dioxide emissions, whereas in this paper, we have focused more on analyzing per capita carbon emissions. Compared with total carbon dioxide emissions, the shift in the center of gravity for per capita carbon dioxide emissions has been a move from northwest to southeast. The shift in the center of gravity for per capita carbon dioxide emissions has shown this trend since 2004, but it was different prior to this, and it shifted significantly in 2009 [39]. As can be seen from Figure 6, the per capita carbon emissions from the Bahamas (BHS), Barbados (BRB), Antigua and Barbuda (ATG), Saint Kitts and Nevis (KNA), Saint Vincent and the Grenadines (VCT), Grenada (GRD), Dominica (DMA), Belize (BLZ), Saint Lucia (LCA), and other countries increased sharply in 2009 and recovered in 2010. All of these countries are located near 0° in the Americas and their location is closer to the southwest than the previous year’s per capita carbon emissions center of gravity. Increases in these countries led directly to the southwest shift of the gravity center for per capita carbon emissions.

In this work, we have researched the spatial distribution of the global per capita carbon emission growth rate and the shift in the center of gravity for per capita carbon emissions; however, there are some shortcomings in our research process. For example, due to the availability of data, the effects of many other factors on carbon emissions were not considered in this study. This can be discussed in detail when more refined data are available. To calculate the shift in the center of gravity, the geometric center was used as the location of a country, and the center of gravity for carbon emissions was calculated based on this. Heterogeneous development within a country makes it difficult to determine the center of gravity for carbon emissions within the country and simply assuming the geometric center to be the gravity center is not representative. While a necessary assumption for this analysis, we hope to find a more appropriate method to apply in calculations in future work.

5. Conclusions

The overall growth rate of carbon emissions from Europe is negative, indicating that per capita carbon emissions from Europe are declining year by year and have begun to enter the stage of carbon dioxide emission reduction, while positive trends dominate for emissions from other continents. In North America, the growth rate of per capita carbon emissions is negative for the United States, Canada, and other major countries, but is positive for other countries in the continent. The small populations of these other countries means that the overall growth in carbon emissions is not particularly large. The growth rate for per capita carbon emissions in most countries in Asia, Africa, and South America is positive. With a large population base, this reflects a large increase in overall carbon emissions, especially in China and India. The top-20 countries with increasing trends for per capita carbon emissions are clustered in Asia, making Asia the continent with the largest and clearest increase in carbon emissions. In the future, the current trend in carbon emissions with persist for most countries in the world, and our results show that it is only anticipated to change for a few countries.

Of the 190 countries in our study, only 77 experienced change-points in our study period. The proportions of countries that experienced change-points within each continent were as follows: 52.5% in Europe, 47.8% in North America, 42.8% in Oceania, 36.9% in Asia, 33.9% in Africa, and 33.3% in South America. We use the proportion—rather than the absolute number,—countries with change-points to better-reflect the overall economic development of each continent. The absolute number of changes towards increasing and decreasing trends in each continent bears little relationship to the overall economic development of each continent.

The shift in the center of gravity for per capita carbon emissions changed around 2004, and the driving factors for the longitudinal shift were different before and after this year. Before 2004, the growth of per capita carbon emissions was greater in western countries than in eastern countries. As a result, the center of gravity moved westward. After 2004, per capita carbon emissions from western countries decreased and per capita carbon emissions from eastern countries continued to increase, which led to an eastward shift in the center of gravity.