1. Introduction
The mesosphere is the region between the stratopause and the thermosphere. As the link between the upper and lower atmospheres, the mesosphere plays a connecting role in the coupling mechanism of the various circles in the sun–terrestrial space and has become an important object in space and atmospheric physics research. As a critical parameter of atmospheric dynamics, the wind directly affects the occurrence and evolution of various physical processes in the mesosphere, and also affects the transport of momentum, energy, and components between the lower and upper atmospheres [
1]. In addition, as the pass-through and residence area of various orbiting spacecraft and hypersonic vehicles, the wind of the mesosphere directly influences the efficiency of flight control and space launching [
2]. Therefore, mesosphere atmospheric wind field observation is of great value to space and atmospheric physics research and related aerospace applications.
At present, atmospheric wind measurement mainly relies on the meteor radar [
3], the Doppler LIDAR [
4], and the airglow interference technique [
5]. Among these, the airglow interference technique has been widely used as the space-based payload by which to obtain the global-scale atmospheric wind field due to its good aerospace adaptability [
6]. The interferometer for atmospheric wind measurement mainly includes three types of structures: the Fabry–Pérot Interferometer (FPI) [
7], the Michelson Interferometer (MI) [
8], and the Doppler Asymmetric Spatial Heterodyne (DASH) [
9]. The FPI technique was applied to the High-Resolution Doppler Imager (HRDI) [
10] of the Upper Atmosphere Research Satellite (UARS) and the TIMED Doppler Interferometer (TIDI) [
11] of the Thermosphere–Ionosphere–Mesosphere Energetics and Dynamics (TIMED) satellite. The HRDI observed the emission and absorption line of O
2 molecules to measure the vector wind from the stratosphere (10 to 40 km) to the mesosphere and lower thermosphere (50 to 120 km). The TIDI measured the wind of the mesosphere and lower thermosphere–ionosphere (MLTI) from an altitude of 60 to 300 km using O
2 molecule and O atom emission lines. The Wind Imaging Interferometer (WINDII) [
12], a wide-angle Michelson interferometer, on the UARS measured the wind of the mesosphere and thermosphere using the red line (630.0 nm) and green line (557.7 nm) of the O atom. Based on the satisfying performance of the WINDII, the Stratospheric Wind Interferometer for Transport studies (SWIFT) [
13] with the MI technique was proposed to measure the wind from 24 km to 60 km with the ozone emission line at 1133.4335 cm
−1. The SWIFT was not able to completely accomplish this due to the difficulty of single emission line isolating components, and it transformed its instrument structure to that of DASH [
14]. The Michelson Interferometer for Global High-Resolution Thermospheric Imaging (MIGHTI) [
15] of the Ionospheric Connection Explorer satellite (ICON) [
16] is based on the DASH technique and focuses on the wind of the thermosphere at an altitude of 80–300 km.
As a new wind interferometer structure proposed in recent years, DASH implements the equal thickness interference, producing the parallel interference fringe [
17]. Compared with the iso-inclined interference of FPI and MI, DASH requires less precision for optics components due to differences in interference types [
18]. More importantly, the input spectrum and interferogram of DASH form a Fourier transform relationship, so DASH does not require the extremely narrow filter to isolate the target emission line [
19]. Additionally, DASH with appropriate spectral resolution can observe multiple emission lines simultaneously by Fourier spectral transform. MIMI [
20] and WAMI [
21] can also achieve multiple-lines observation simultaneously by the wavelength modulation of the FP filter. The different angles of incidence have different transmission functions, making different areas of the detector receive different emission lines’ radiation. While compared with DASH, this structure cannot make each line cover the whole altitude range and also loses the amount of valid data. Therefore, DASH is more adaptable for observation targets with multiple emission line structures [
22]. The above characteristics significantly increase the efficiency and applicability of DASH, making DASH suitable for the atmospheric wind measurement, especially for the multiple lines structure target.
For the mesosphere wind measurement, various target airglows have differentiated altitude coverages and application performances. Based on the ozone emission line at 1133.4335 cm
−1, the wind at the bottom of the mesosphere can be observed with high instrument costs [
23]. The green line (557.7 nm) of the O atom can only achieve wind at the mesopause at about 80 km above [
24]. O
2(a
1△
g) dayglow near 1.27 µm is suitable for mesosphere wind measurement with an altitude ranging from 30 km to 90 km [
25], whereas self-absorption and SWIR band interferometer development are still significant obstacles [
26]. The emission line of O
2(0-1) vibrational bands is of bright radiance and good transmissions within the mesosphere’s altitude range [
27]. Therefore, the emission line of the O
2(0-1) band could be a proven path for mesosphere wind measurement.
For the wind measurements in the middle atmosphere, the available observation sources, including those described above, are all characterized with narrow spacing multi-emission-lines structures [
14,
21], which impose higher instrument specifications, process difficulties, and development costs based on the traditional wind measurement scheme, which uses extremely narrow filter to achieve the single emission line observation. In order to expand the feasibility of passive interference technology in the field of middle-atmosphere wind measurement, reducing the instrument development process and cost and improving the measurement precision, it is important to explore how to achieve a high performance simultaneous multi-emission line measurement scheme.
This study is based on the DASH technique, due to its sampling characteristics satisfying the Fourier transform relation, which is able to obtain the input spectrum by interferogram recovery to achieve effective multiple emission lines wind measurement with low instrumental cost. As the adoption of a multiple-emission-line wind measurement scheme, the existing method cannot satisfy the demands of the new scheme’s observation data retrieval. It is also significant to construct a space-based wind retrieval strategy for multi-lines observation based on the DASH concept.
In this paper, we propose a space-based technical solution for the measurement of the atmospheric wind by employing a DASH interferometer to observe the four emission lines of O
2(0-1) vibrational bands at about 866.5 nm, which is named the Mesosphere Wind Image Interferometer (MWII). According to the DASH concept, the MWII could synchronously observe the multiple emission lines in the target band. Based on basic principles of DASH, the multiple-emission-line retrieval strategy is proposed to separately obtain the wind speed information of each line. Lastly, the joint wind decision method is proposed to achieve higher wind retrieval precision through the multiple lines wind retrieval result.
Section 2 describes the basic principles and observation mode of space-based DASH for wind measurement. The radiation and transmission characteristics of the target dayglow and background radiation are described in
Section 3.
Section 4 describes the forward model and noise model of the MWII, and then the expected observation interferogram is also described and analyzed. The multiple-emission line retrieval strategy and the wind measurement error performance are expressed and discussed exhaustively in
Section 5. The analysis of the simulation result and the comparison with a similar study is shown in
Section 6.
Section 7 outlines the conclusions of the full paper.
2. Measurement and Instrument Concept
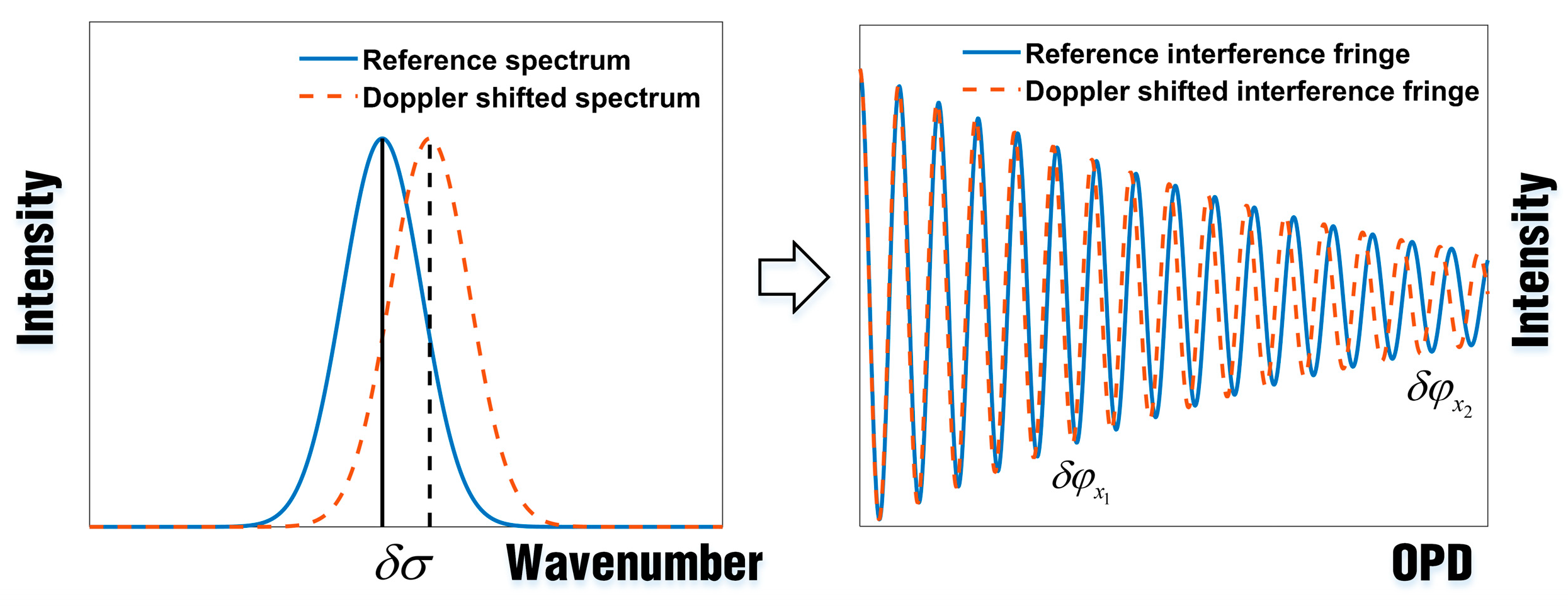
The passive interferometer observes the Doppler frequency shift of the airglow, which is caused by the atmospheric motion to achieve the wind measurement. For the interferometer, a shift of the airglow emission line would result in a change in the interferogram frequency.
Figure 1 gives the schematic diagram of the Doppler shift of the emission line and the interferogram frequency change.
According to the interferogram frequency measurement, the Doppler shift can be calculated and the wind speed can be obtained. However, the wavelength shift of the target emission line caused by the wind speed being very small, at the level of about tens of femtometers. Therefore, the interferogram frequency change is also very small under the small optical path difference (OPD). Additionally, with the increase in the OPD, the frequency changes predominantly appear as phase shifts, which can be much more effectively measured. The relationship among the wind speed, the Doppler frequency shift, and the phase shift is described as follows in Equations (1) and (2):
where
is the atmospheric wind speed,
is the wavenumber shift caused by the atmospheric motion,
is the nominal wavenumber of the emission line,
is the phase shift of the interferogram,
is the OPD corresponding to the interferogram, and
is light speed in a vacuum.
In order to achieve high wind measurement sensitivity, various structures of wind interferometers, including DASH, collect interference signals under a large OPD [
28]. DASH is essentially a kind of Spatial Heterodyne Spectroscopy (SHS), which achieves an asymmetric structure by introducing an additional optical path offset in one arm of the interferometer [
29].
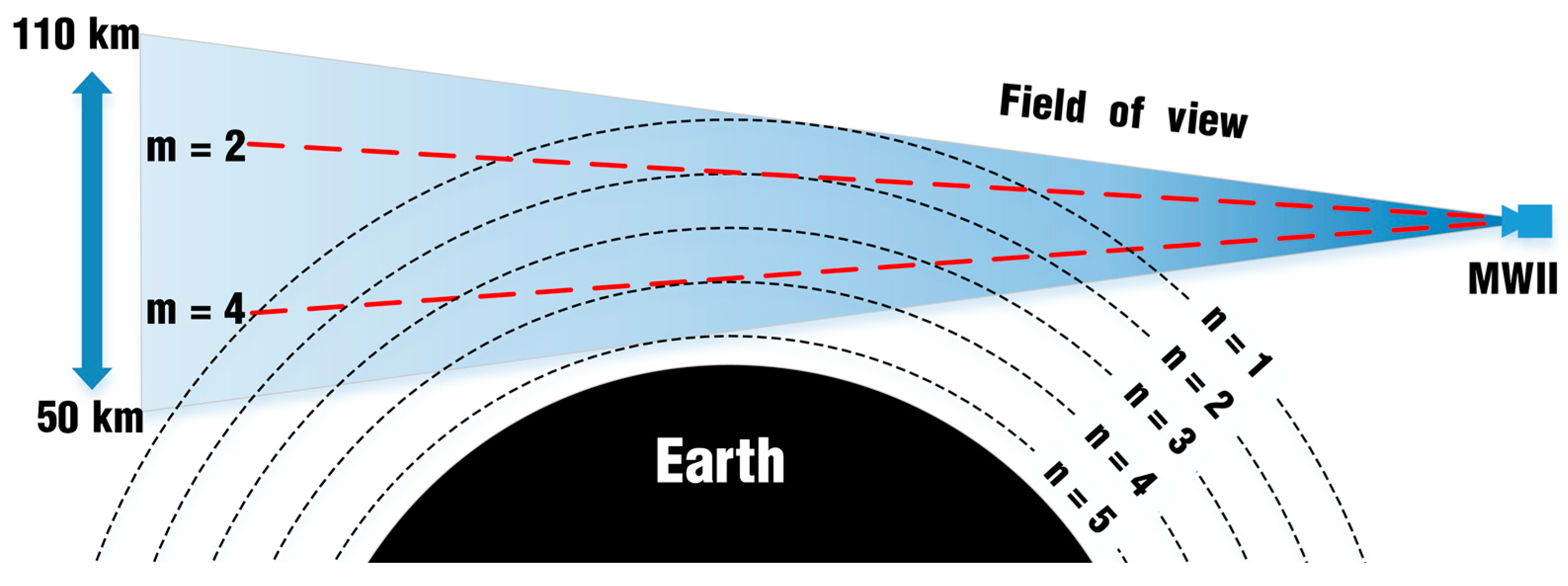
MWII, based on DASH, utilizes a limb-viewing mode to observe the atmospheric radiation signals from different altitudes for the wind profile measurement. Based on an orbit altitude of 500 km, the field of view is precisely designed to ensure an altitude coverage from 50 km to 110 km. The limb-viewing observation signal is the integral of airglow radiation from different altitude. The integral airglow radiation from different tangent altitude enters the MWII with different angles, causing the interference signals of different altitude to be acquired separately. Based on the retrieval method, the wind information of the tangent area could be peeled off from the integral signal to achieve the wind profile measurement. The limb-view observation geometry is shown in
Figure 2.
The passive wind measurement interferometer can only obtain the line-of-sight (LOS) wind speed by a single observation from one direction. In order to achieve the vector wind field measurement, it is necessary to observe from at least two different directions, preferably orthogonal. Therefore, the MWII has two nearly orthogonal fields of view (FOV1 and FOV2) to obtain the vector wind field. After FOV1 observes the mesosphere of a specific area, through the movement of the satellite, FOV2 makes a second observation of the same area from a direction orthogonal to the first observation after about 10 min. The orthogonality of the two observations is guaranteed by the observation geometry design without the need for satellite attitude adjustment.
5. Retrieval Strategy and Error Verification
For the wind retrieval of the space-based DASH, there are two key aspects to be considered: retrieval from the LOS integral signal to target the tangent altitude signal and retrieval from the interference fringe to wind speed.
The existing retrieval strategy puts the decomposition of the LOS integral signal ahead of the interference fringe retrieval [
35]. As Equation (3) shows, the LOS integral radiance can be expressed as the weighted sum of the VER from the top layer to the tangent layer with the atmospheric optical length as the weight coefficient, and the interferogram can also be expressed in the same form. The existing retrieval strategy first carries out LOS integral signal decomposition in the interference domain, peeling off the influence of the upper atmospheric interference fringes on the lower interference fringes layer by layer, from top to bottom, according to the radiance intensity and atmospheric optical length. Then, the interferogram of each layer can be obtained, which only includes the tangent area’s atmospheric signal. According to the retrieval of the decomposed interferogram, the wind profile can be obtained.
However, the existing retrieval strategy cannot be directly migrated to the multiple-line retrieval strategy. The existing strategy is oriented to single-line observation scenarios. For a multiple-line input, the radiance variation with altitude of each spectral line tends to be different, resulting in different weight coefficients for each line in their integrated signals. Therefore, it is impossible to decompose the integral signal in the interference domain by using a uniform weight coefficient.
5.1. Wind Retrieval Strategy for Multiple Emission Lines
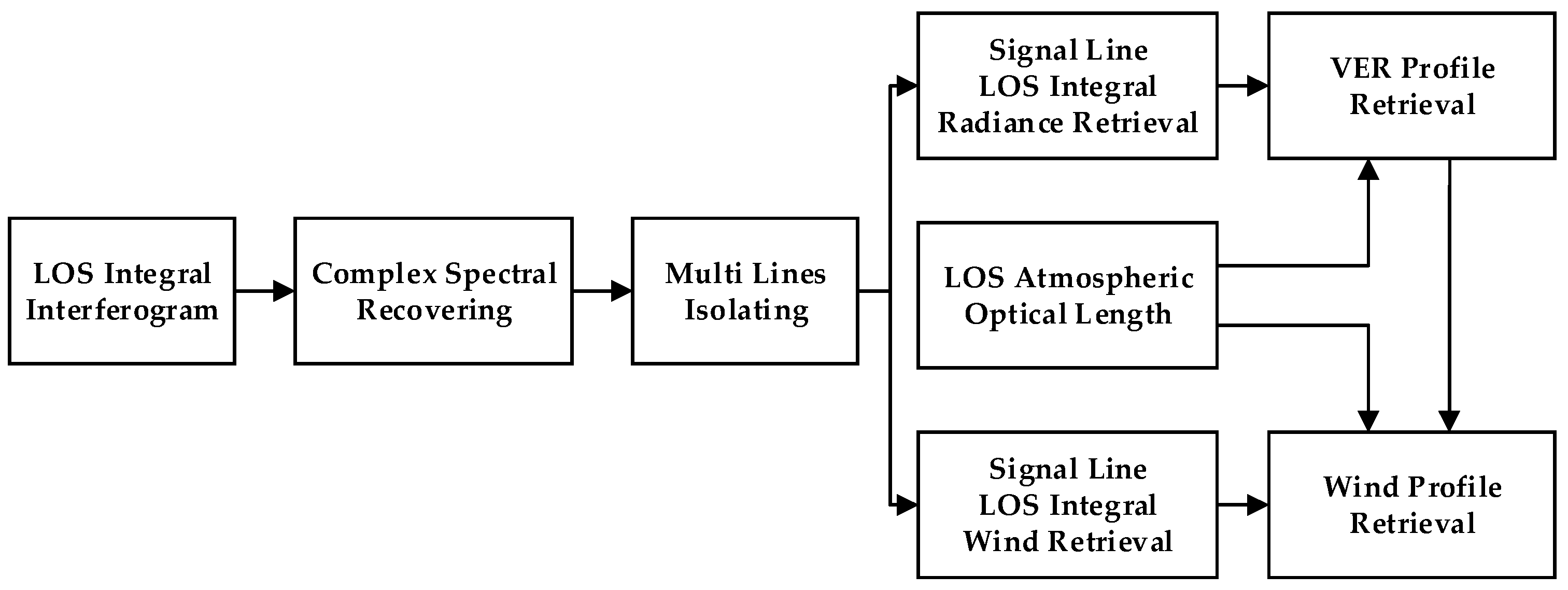
This paper proposes the multiple-emission-line retrieval strategy based on the interference fringe retrieval priority, making each line have an independent weight coefficient, respectively. Thus, each emission line can be independently retrieved to obtain the wind. The specific process is shown in the following
Figure 11.
The proposed strategy first recovers the integral interferogram and obtains the inversion complex spectrum. Each spectral line is extracted by the corresponding window function, and then its integrated radiance and wind speed are inverted, respectively. Lastly, combined with the atmospheric optical length, the VER profile and the wind speed profile are sequentially retrieved.
The interferogram of multiple emission lines can be written in the form of emission-line superposition, as shown in Equation (9).
where
is the intensity term of the line
j;
is the interference efficiency term;
is the interference frequency term;
is the additive phase; and
is the phase shift resulting from the Doppler shift of each line.
Based on the discrete Fourier transform, the complex spectrum can be recovered from the interferogram [
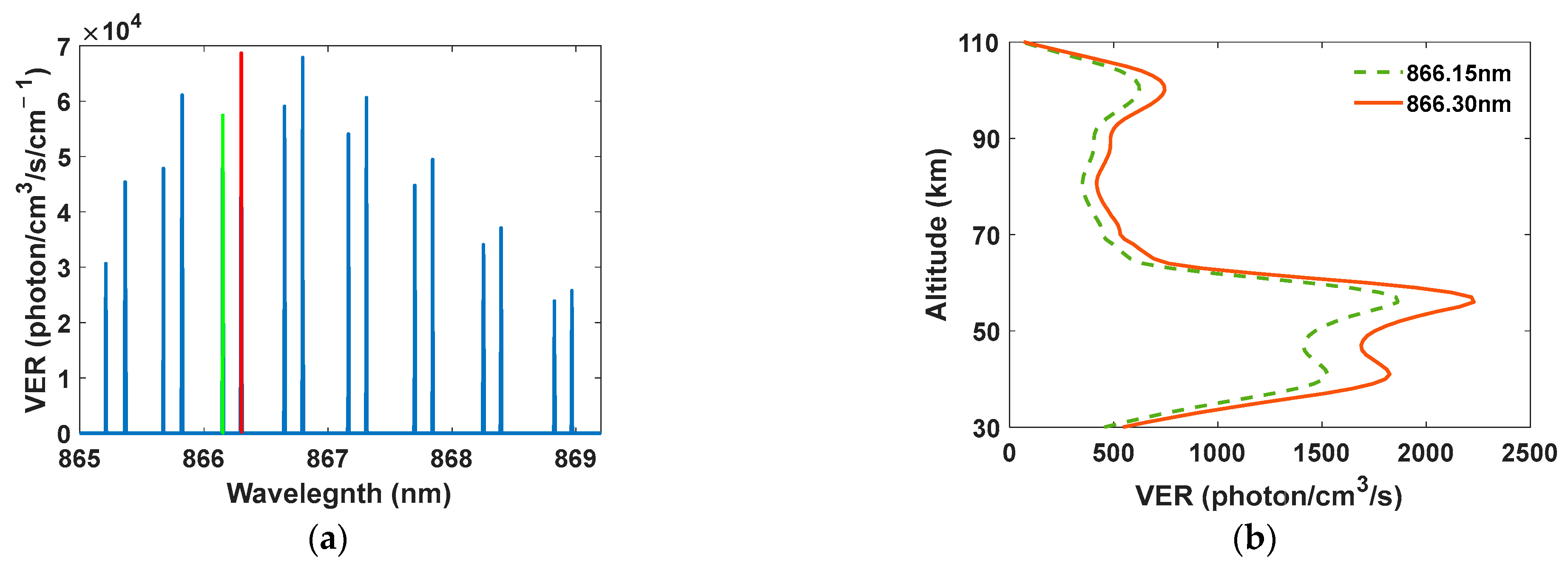
9]. The modulus of the complex spectrum is shown in
Figure 12.
The window function is applied to isolate each line, and then the relative LOS integral intensity can be obtained from the recovered spectrum. According to relative LOS integral intensity corresponding to each altitude, the LOS integral radiance can be determined with the support of pre-acquired radiometric calibration parameters.
In order to retrieve the LOS integral wind, the inverse discrete Fourier transform is applied to the isolated complex spectrum of each line, thereby achieving the complex interferogram, as shown in Equation (10).
The interferogram phase, , can be obtained by calculating the arctangent of the ratio of the imaginary part and the real part at each sampling position of the complex interferogram. By the subtraction of which is called the reference phase, , caused by the Doppler shift of the wind, can be achieved. According to Equations (1) and (2), the LOS integral wind can be calculated.
Based on the uniform spherical symmetry model, the VER profile can be retrieved from the top layer to the bottom layer, as follows in Equation (11), where
is the radiance of line
j at layer
m.
According to the phase characteristics of DASH, the LOS integral wind could be considered as the weighted sum of the wind from the top layer to the tangent layer with the VER and atmospheric optical length as the weight coefficient. Therefore, the wind profile can be expressed as in Equation (12):
where
is the LOS wind speed at layer
m, and
is the LOS integral wind speed at layer
m.
In order to evaluate the error of the MWII with the proposed retrieval strategy, the simulation was carried out based on the raw interferogram and the noise level shown in
Section 4. Under the constraints of the same noise model, the simulation was performed 10,000 times using the Monte Carlo method to evaluate the accuracy and precision of the full link.
Figure 13 gives the results of the accuracy verification.
Figure 13a is the input LOS wind profile from the horizontal wind model (HWM).
Figure 13b is the wind retrieval accuracy of the four emission lines. The accuracy data were derived from the comparison of the average of multiple measurements with the input wind speed. The altitude average accuracy values of the four emission lines were 1.20 m/s, 1.01 m/s, 1.18 m/s, and 1.02 m/s, respectively. The accuracy of the wind speed measurement at altitudes around 80 km was the worst, at −4 m/s.
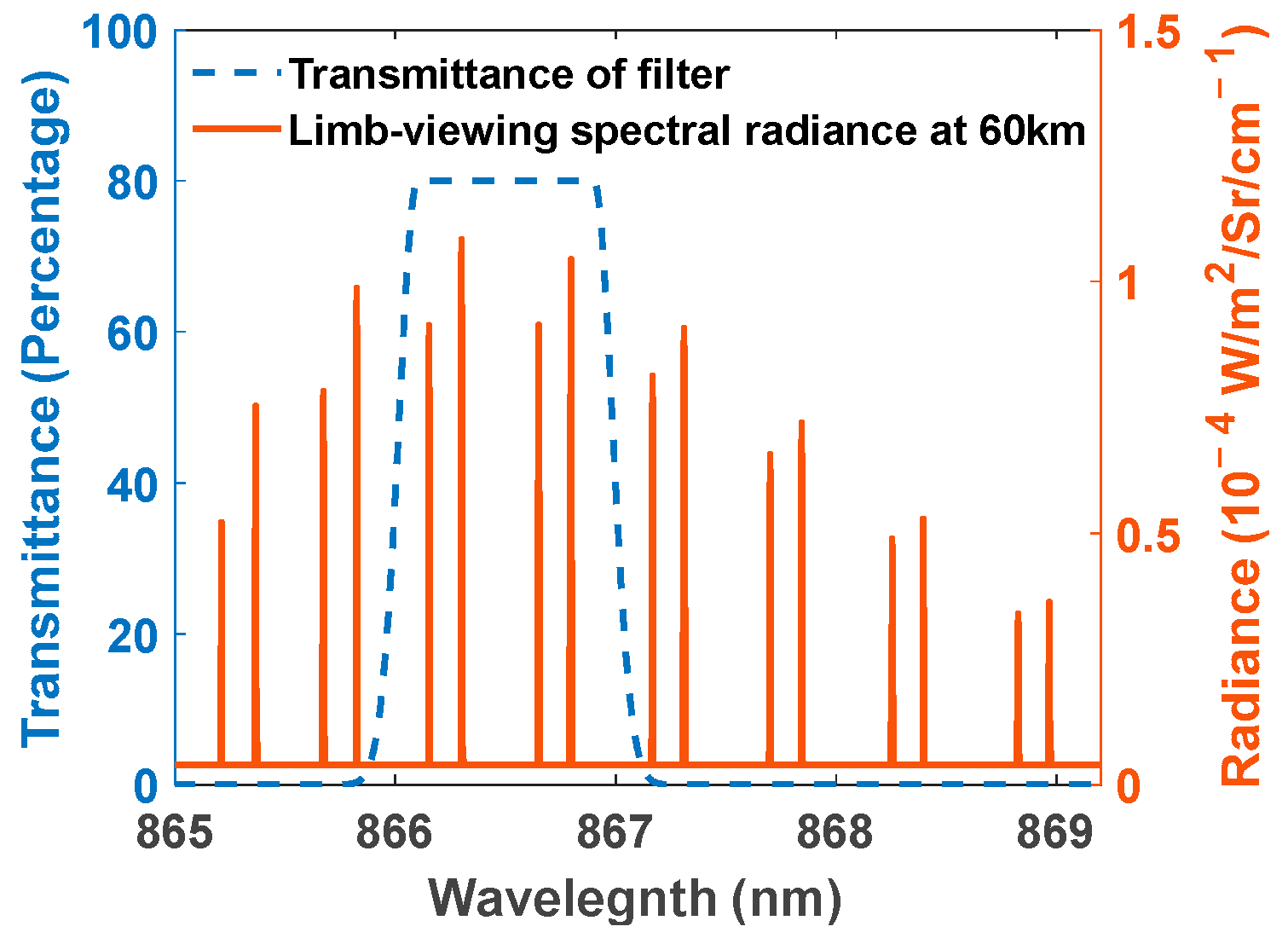
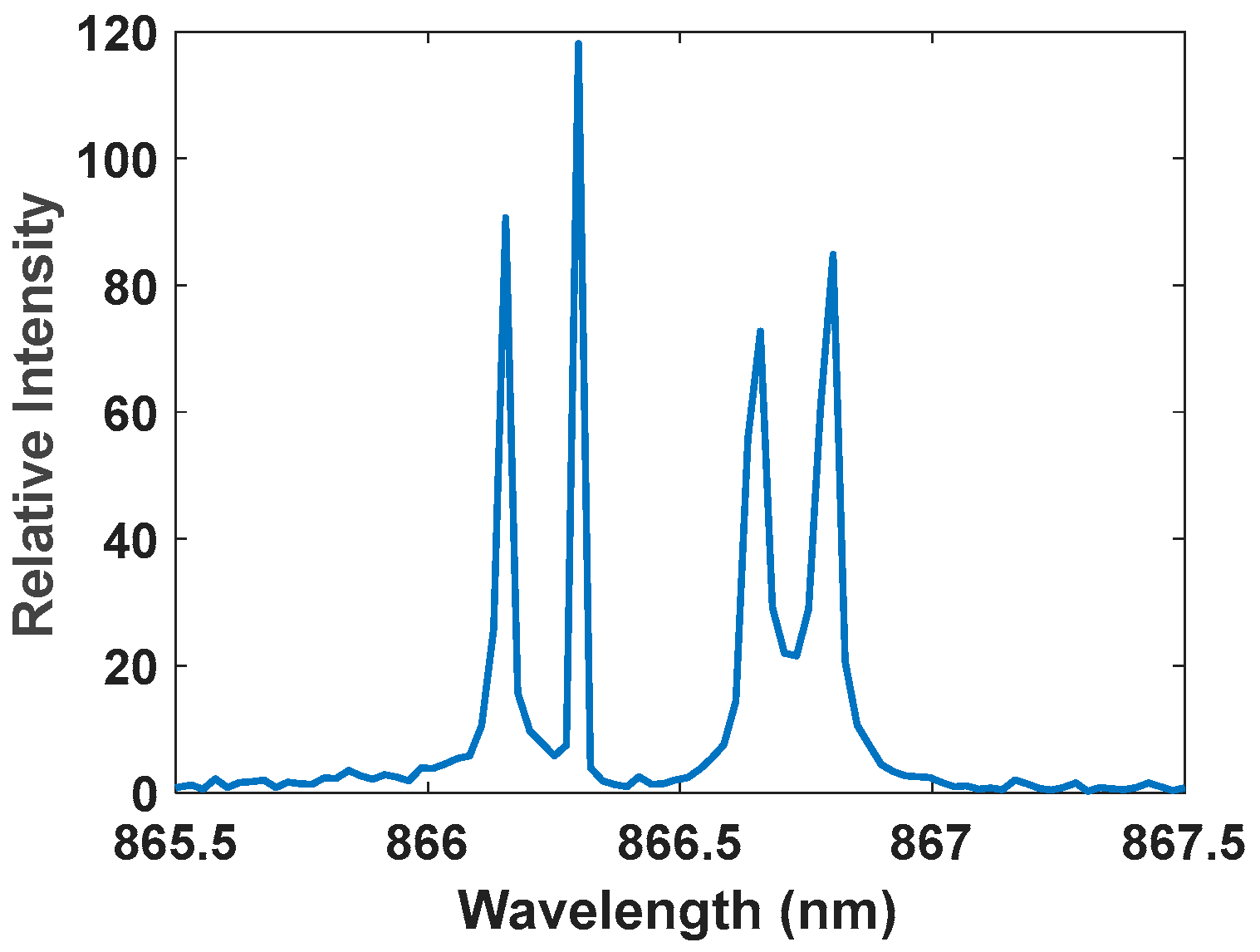
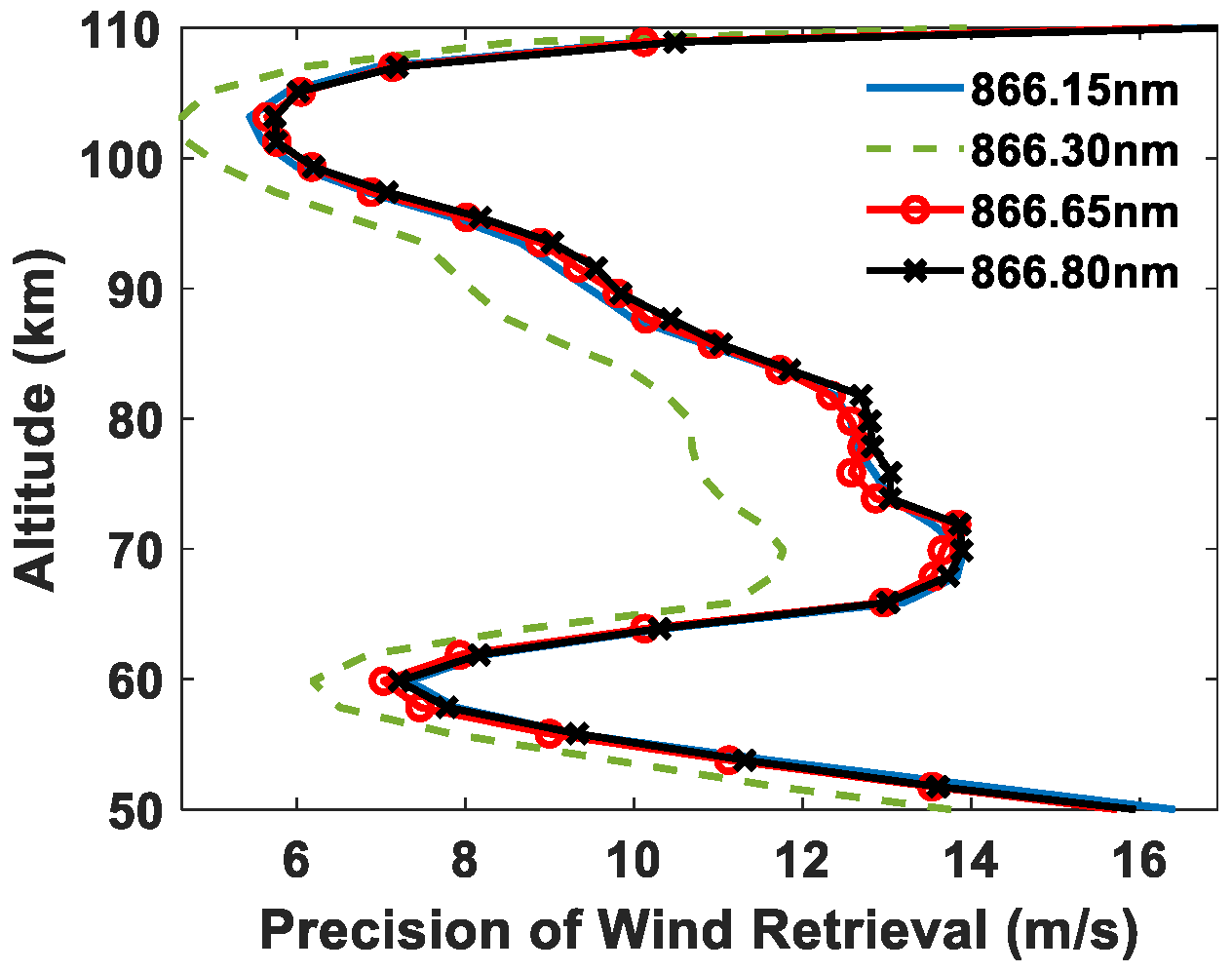
Figure 14 gives the precision of the four emission lines. The line at 866.30 nm had better precision than the other three due to its better relative radiance and isolation, as shown in
Figure 12. Limited by the radiance of the observation target and background radiation interference, no emission line achieved an acceptable precision individually, which generally needs to be at least 10 m/s.
5.2. Multiple-Emission-Line Joint Wind Decision Method
For the multiple-emission-line observation, each emission line measures the wind speed of the same area of the atmosphere. Therefore, the true values of the measurement results are the same. However, the radiance profile of each emission line is different, so the emission lines are under different noise levels, resulting in every emission line having different wind measurement precisions. Based on the white noise characteristics, the wind measurement results of each emission line should statistically conform to a normal distribution with the same mean but different variances. Based on the precision estimation of each emission line, a joint decision-making model based on the minimum error expectation principle is constructed as shown in the following Equation (13), which can effectively improve the final wind speed measurement precision.
where
is the joint wind measurement result of layer
m;
N is the total number of the involved lines;
is the wind measurement result of the
jth emission line at layer
m; and
is the wind measurement precision of the kth emission line. For a specific single measurement, the precision of the emission line can be estimated based on its recovering spectral intensity and the noise model described in
Section 4.2.2 and
Section 5.1. The joint decision-making model is based on the minimum variance combinatorial theory, making the joint’s result better than any individual line’s result, and the precision of the joint wind measurement is shown in the following Equation (14).
where
is the precision of joint wind measurement result of layer
m.
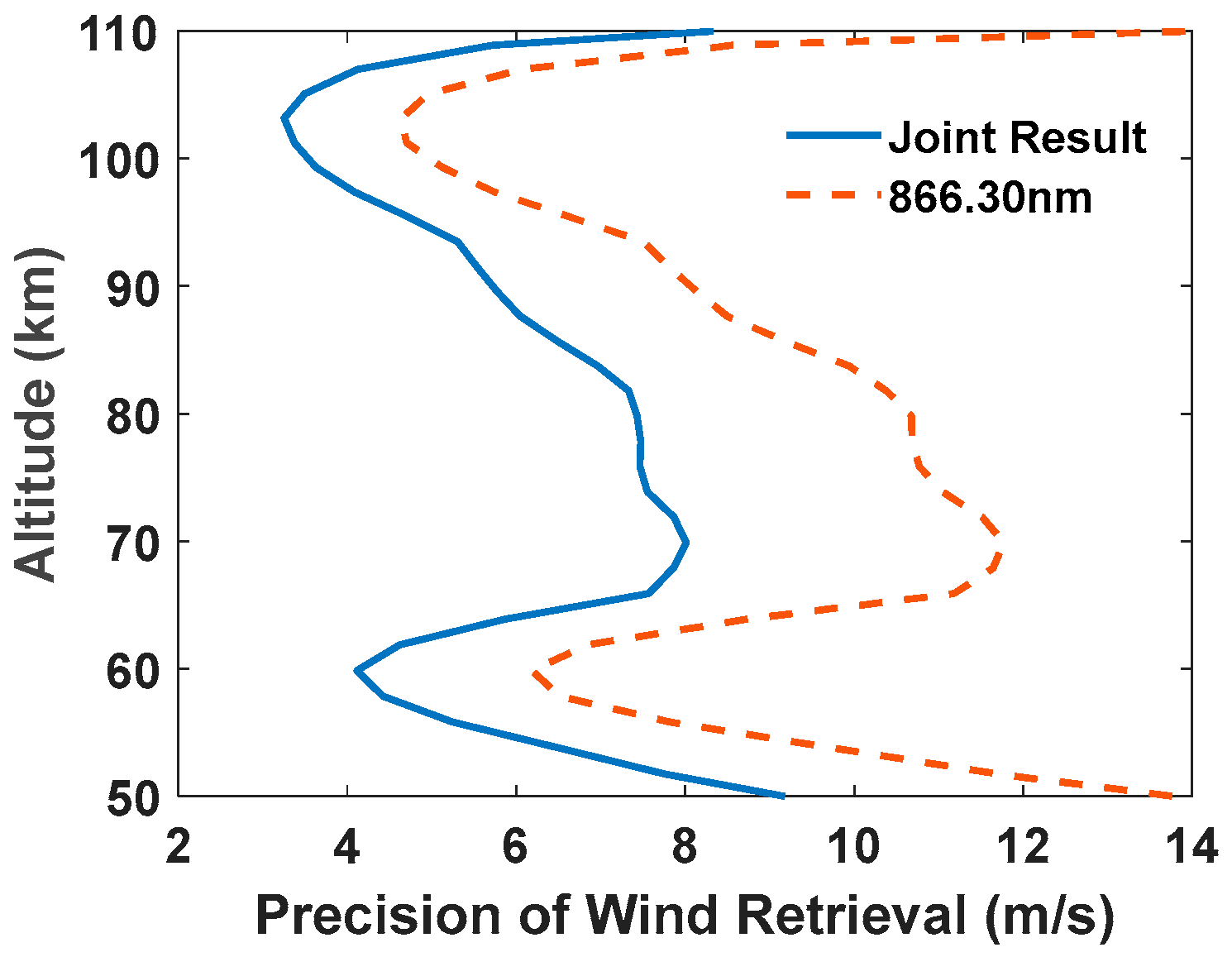
Figure 15 gives the joint wind measurement precision and a comparison with the individual highest precision of the four emission lines. The joint method was found to significantly improve the wind measurement precision performance. The wind measurement precision of altitude average is improved from 8.67 m/s to 5.97 m/s, which is more than 30%. The highest precision value was less than 4 m/s at about 105 km. For the altitude from 50 to 110 km, the precision value was in the range of 3–9 m/s, proving the efficiency of the joint wind measurement method.
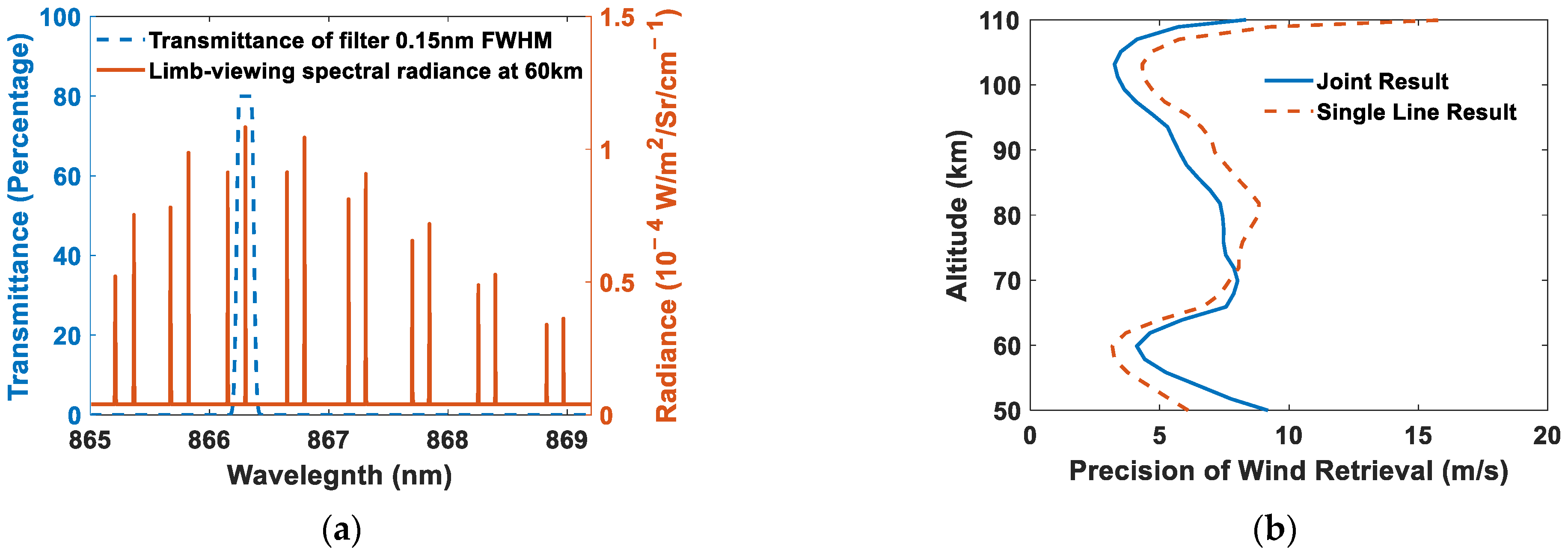
In order to further verify the precision performance of the joint method, a single-emission-line wind measurement simulation was conducted based on the forward model and retrieval strategy of this paper. In the additional simulation, the FWHM of the filter reduced from 1 nm to 0.15 nm, optically isolating the brightest emission line in the band shown in
Figure 16a. The performance comparison of the proposed joint method and the single-line isolating scheme is shown in
Figure 16b. Overall, the precision of the two methods was found to be comparable.
6. Discussion
Based on the full-link numerical simulation, the accuracy and precision performance of MWII are shown in
Figure 13,
Figure 14,
Figure 15 and
Figure 16.
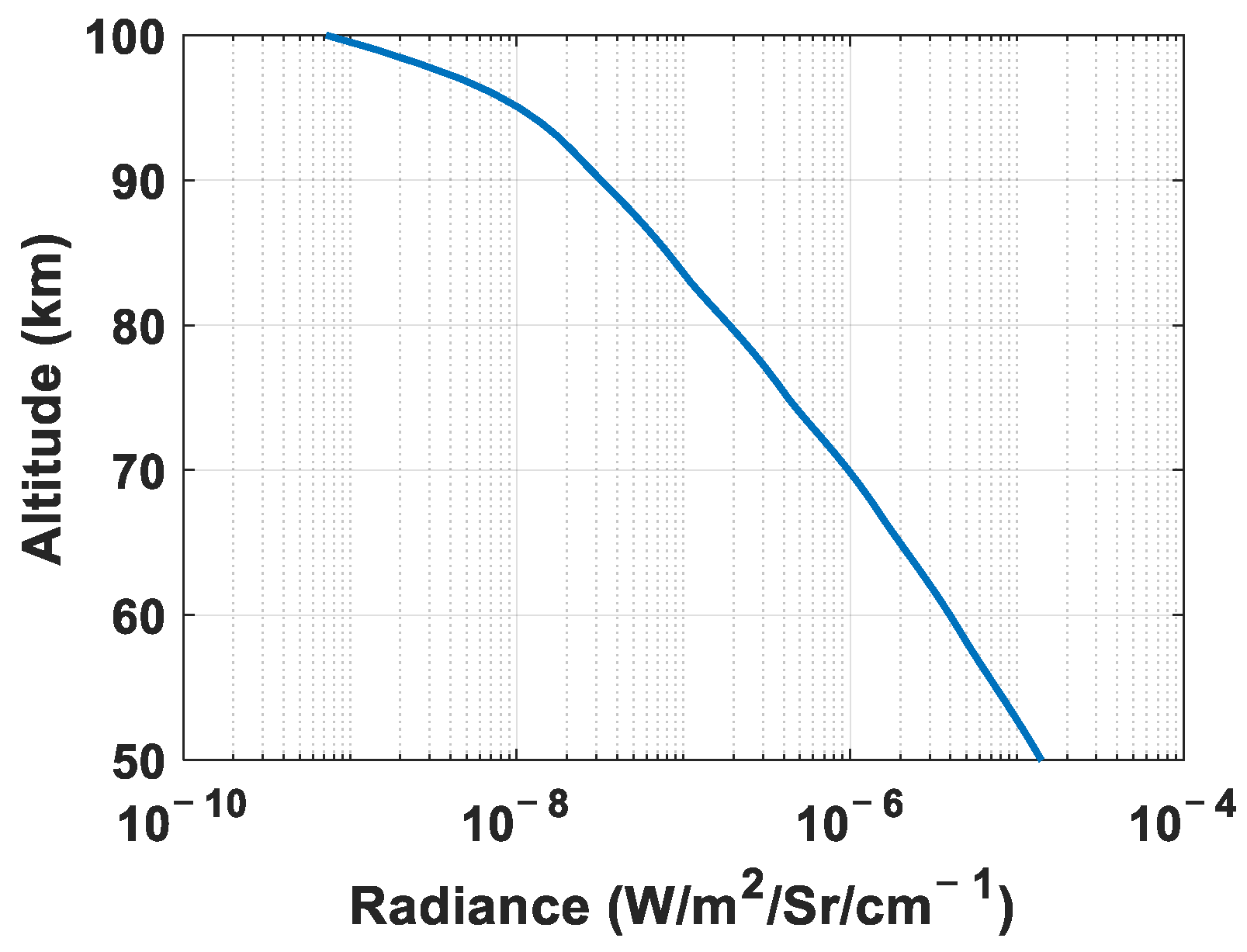
Figure 13 shows the accuracy profile. This deviation is mainly attributed to the observation geometric model’s error caused by pixel binning in the altitude direction. The input radiance with wind information is generated with an altitude resolution of 0.125 km, which corresponds to the pixels’ altitude resolution, whereas, in the retrieval, the 16 pixels in the altitude direction are binned as one data point in order to achieve better precision performance. Therefore, the system deviation of the atmospheric optical length, VER and wind was brought into the retrieval due to the average effect. The more rapidly changing the wind, the worse the wind accuracy of retrieval performance, resulting in the worst accuracy performance appearing in the altitude range of 80–90 km. By reducing the number of pixel binning, the average effect can be significantly lower, improving the accuracy performance. Meanwhile, with the less binned pixels, the SNR of the retrieval data also be reduced, resulting in worse performance in precision. Therefore, the accuracy and the precision need to be trade-offs and compromises with each other.
The precision performance of MWII is shown in
Figure 14 and
Figure 15. The precision is mainly influenced by the radiance of the observation target and background radiation. At relatively high altitudes, the dark radiance of the observation target limits the wind measurement precision, whereas at relatively lower altitudes, the background radiation is the core reason for the decrease in precision according to lower the contrast of interferogram. In addition, the peeling off retrieval used in this study also further reduces the precision at lower altitudes. The altitude range of 65–85 km appears to be a lower precision when compared with those at 60 km and 100 km, which is because of the lower radiance, shown in the
Figure 3b.
Figure 16 gives the precision comparison with traditional single-line observation with the proposed multiple-emission-line observation. The proposed method performed better at relatively higher altitudes due to its better radiation utilization capability for dark inputs. The traditional single-line scheme achieved higher precision at lower altitudes due to the stronger background radiation suppression capability. Considering the difficulty and costs of an extremely narrow filter, the proposed multiple-emission-line scheme has obvious advantages for applicability.
In this study, the MWII performed good altitude coverage and measurement precision in the mesosphere wind measurement. There are some other mesosphere wind measurement schemes which can carry out further comparative studies with MWII.
The TIDI is a well-known wind measurement payload. TIDI also utilizes the O
2(0-1) band with the line at 867.13 nm for the mesosphere wind measurement, and the average precision is reported as 7.8 m/s [
27]. The MWII has better precision performance than TIDI as the multiple-line joint measurement capability and has lower hardware requirements in the filter. The WAMI is also a wind measurement targeting the mesosphere. WAMI uses two sets of lines within the O
2(a
1△
g) band to measure the wind at altitudes of 60–100 km and 45–75 km, respectively, and the precision is reported as 3–5 m/s [
21]. WAMI preforms better precision than MWII as the much brighter observation target. In general, the emission line in O
2(a
1△
g) band is an order of magnitude brighter than that in O
2(0-1) band. In terms of instrument cost, MWII achieves similar altitude coverage to WAMI with only one set of lines. In addition, WAMI involves two etalons with the bandwidth of 0.1 nm and 0.13 nm to isolate the line sets. Our research group has also explored the mesosphere wind measurement based on the single emission line of the O
2(a
1△
g) band. The emission line the O
19P
18 (1286.7 nm) of O
2(a1Δg) band is involved for the altitude range of 30–80 km wind measurement with the precision of 2–10 m/s [
26]. When compared with MWII, the scheme with O
2(a1Δg) band has better precision in altitude range of 50 to 70 km but worse from 80 to 110 km. Additionally, the fewer development difficulties and lower costs of the NIR band interferometer make MWII more acceptable than the SWIR band instrument.
According to the comparison with other mesosphere wind measurement schemes, MWII preforms better altitude coverage with acceptable measurement precision. In addition, due to the innovative multiple-emission-line measurement strategy, the difficulty and cost of instrument development have been effectively controlled, which makes MWII competitive in the field of mesospheric wind measurement.
7. Conclusions
A new mesosphere wind measurement scheme is proposed in the article, called MWII. The MWII carries out mesosphere wind measurements using the DASH technique. Based on the advantage of DASH being able to achieve multi-line simultaneous observation, four emission lines of the O2(0-1) band were selected as the target airglow in the MWII. In order to achieve the multiple-emission-line wind retrieval, we propose a retrieval strategy with the idea of interference fringe retrieval priority, allowing for each line to have the independent weight coefficient, respectively, based on the recovered spectrum, and achieving respective wind retrieval for each emission line. Based on the multiple-line wind retrieval results with different precision levels, a joint wind decision model with minimum error expectation was constructed to achieve higher precision wind measurement. The numerical simulation, including airglow and background calculation, the instrument forward model, and the proposed wind retrieval method, was performed to verify the performance of the MWII.
The simulation results indicated that the multi-line retrieval strategy can obtain the wind speed correctly with an accuracy of about 1 m/s. Moreover, the joint wind decision model can significantly improve the precision performance when compared with any other single-line result, achieving a wind measurement precision of 3–9 m/s at the altitude range of 50–110 km. The precision results indicate that the MWII can both cover the whole altitude of the mesosphere and also provide wind measurement capability in the lower thermosphere. According to the performance comparison with the scheme with an extremely narrow filter, the MWII is proven to have comparable observational performance but with lower instrumental costs.
The multiple-emission-line measurement strategy used in MWII can not only apply to the O2(0-1) band, but also can be widely extended to various types of observation targets with narrow-spacing multiple-emission line structure. On the one hand, this strategy can reduce the difficulty of instrument development and improve the measurement precision. On the other hand, the range of selectable emission lines can also be widened, as the many narrow-spacing observation targets can become available based on the proposed measurement strategy.