A GIS-Based Methodology to Combine Rain Gauge and Radar Rainfall Estimates of Precipitation Using the Conditional Merging Technique for High-Resolution Quantitative Precipitation Forecasts in Țibleș and Rodnei Mountains

Abstract

Rain gauges provide accurate rainfall amount data; however, the interpolation of their data is difficult, especially because of the high spatial and temporal variability. On the other hand, a high-resolution type of information is highly required in hydrological modeling for discharge calculations in small catchments. This problem is partially solved by meteorological radars, which provide precipitation data with high spatial and temporal distributions over large areas. The purpose of this study is to validate a conditional merging technique (CMT) for 15 rainfall events that occurred on the southern slope of the Tibleș and Rodnei Mountains (Northern Romania). A Geographic Information System (GIS) methodology, based on three interpolation techniques—simple kriging, ordinary kriging, and cokriging—were utilized to derive continuous precipitation fields based on discrete rain gauge precipitation data and to derive interpolated radar data at rain gauge locations, and spatial analysis tools were developed to extract and analyze the optimal information content from both radar data and measurements. The dataset contains rainfall events that occurred in the period of 2015–2018, having 24 h temporal resolution. The model performance accuracy was carried out by using three validation metrics: mean bias error (MBE), mean absolute error (MAE), and root mean square error (RMSE). The validation stage showed that our model, based on conditional merging technique, performed very well in 11 out of 15 rainfall events (approximate 78%), with an MAE under 0.4 mm and RMSE under 0.7 mm.

1. Introduction

To forecast flash flood events, hydrologists can use basic tools (such as rainfall-discharge curves) or sophisticated tools (such as complex physical and hydrological models). The task is to convert atmospheric and soil conditions into maximum discharge, accumulated volumes, and arrival times. All these tools have uncertainties related to their input data and parameter settings. Rainfall-runoff models are sensitive to rainfall quantities and their spatial distribution throughout the catchment; thus, errors in precipitation estimates have a significant impact on predictability and event reconstruction [1].

In general, the anticipation interval for the phenomenon that is embedded with convective systems, which generate flash floods, is in the range of tens of minutes to 2–3 h. Furthermore, landforms can modify the trajectory of convective systems. This can reduce the rainfall forecasting accuracy, even in the case of forecasts performed several minutes before the events.

The spatial coverage of the hydrometric and rain gauge networks in Romania showed a slight increase over the years. According to Vladimirescu [2], the hydrometric network in Romania included 765 hydrometric stations in 1978; thus, the average density was one point per 325 km². At the national level, for technical and operational reasons, most of the rain gauges of the “Romanian Waters” National Administration are located at hydrometric stations, hydrometeorological stations, and river estuaries. This has led to the deficiency of rain gauges in the upper part of the river basins (especially those of small and medium size). In the Someș-Tisa Water Branch (STWB), the hydrometeorological data monitoring network consists of 101 hydrometric stations, of which 79 have an automatic transmission system. Additionally, 59 rain gauges are located over the basin for precipitation measurements. The average density of hydrometric stations at the STWB level is 1 station per 221 km², and the density of the rain gauges is 1 station per 140 km², which is insufficient to properly capture the spatial variation of precipitation over the space.

The interpolation of these pointwise measurements to determine the amount of precipitation falling in the entire river basin can be elaborated only in the case of basins that cover substantially large areas. In the case of basins with areas less than 20 km², these interpolations can lead to high errors that amplify the errors of the small-scale convective systems forecast [3]. Considering this important aspect, obtaining a realistic spatial distribution of precipitation using interpolation data from the existing low-density rain gauge network is difficult [4]. The smaller the river basin and the higher the rain intensity, the more difficult it is to calculate the basin response and concentration time, which are basic parameters in hydrological modeling [5,6].

The low density of rain gauges, their lack in the upper part of torrential river basins, and the uncertainty in the rainfall data processing are considered the main sources of errors in the simulated discharges [3,6].

The main objective of the study consists of the adaptation and validation of a GIS-based approach to derive high spatial resolution precipitation data combining radar images and in situ measurements. These products are highly required in flash flood forecasting models.

It is necessary to enhance the input data quality in hydrological models to increase the accuracy of the simulated discharges. Using meteorological radars, high-resolution precipitation data can be obtained [1]. Weather radar has a special technique of sampling precipitating clouds at high spatial and temporal resolutions, exceeding the resolution of rain gauges or satellites. The main disadvantage of the meteorological radar is that the rainfall values are derived using empirical formulas, which can lead to multiple random errors. Furthermore, weather radar measurements are often affected by atmospheric conditions, attenuation, the earth’s curvature, beam blocking, uneven radar beam filling, clutter contamination, regional climate variability, and other factors [7].

By combining the radar and rain gauge precipitation data, a high-resolution product with smaller errors, compared to those obtained from the individual interpolation of rain gauges or radar data, can be derived [8].

Several studies have presented different techniques for accomplishing this data merging task for rainfall map production [8,9,10,11,12,13,14,15,16,17].

Moreover, most GIS software packages integrate a series of interpolation functions based on the distance between stations. However, factors affecting the spatial distribution of the targeted climatic elements are not considered. Common interpolation methods include Thiessen, inverse distance weighting (IDW), spline, and natural neighbor. In these methods, a problem of spatial autocorrelation among climatic parameters exists [18].

According to Jin Li and Heap [19], more than 40 spatial interpolation methods have been developed and applied worldwide. After performing 51 comparative studies using 62 methods and sub-methods, their results show that the alteration within the data is the main factor that has an enormous impact on the performance of the spatial interpolators. The alteration involves the modification of the precipitation values at the control rain gauge points by using an inadequate interpolation method for the entire dataset.

Kriging, especially ordinary kriging, has become a widely used tool in the analysis of spatial data [20]. Stisen and Tumbo [21] applied a regression interpolation to combine precipitation data from rain gauge records with the spatial pattern information obtained from satellite precipitation products in the Great Ruaha River basin (Tanzania).

In recent decades, cokriging techniques for merging rain gauge measured data with radar quantitative precipitation estimates (QPE) have become increasingly common [5,22,23].

The conditional merging technique (CMT) proposed by Pegram [20] was developed by Uwe Ehret in 2002, in his PhD thesis. He developed a short-term flood warning model for Goldersback (catchment size 75 km²). Based on this CMT, several studies have been conducted worldwide. Pettazi and Salsón [4] applied it in a summer storm situation in Galicia (NW Spain), when the most populated city, Vigo, was flooded (SW Galicia). Several case studies have also been conducted in the Eastern Asian region [24,25].

Moreover, studies on monitoring heavy precipitation amounts and their impact on small river basins have been conducted in Romania. Investigating three extreme flash flood events in catchments between 36 and 167 km² located in the Carpathian range (Romania) for the period of 2005–2007, Zoccatelli et al. [26], demonstrated that the weak representation of the spatial rainfall variability resulted in high errors in simulations. Ștreang et al. [3] carried out a GIS project in small- and medium-sized rivers basins (right bank tributaries of the river Crişul Repede with an area between 16 and 175 km²) using Weather Surveillance Radar products, designed in 1998, which use the Doppler effect to locate precipitation, calculate its motion, and estimate its type (WSR-98D). They determined the average amounts of precipitation estimated on small river basins, which are susceptible to floods. In a recent study in the Bistra River basin, Haidu and Strapazan [27] noted the importance of high-resolution precipitation data in modeling flash flood events in small catchments.

The lack of databases representing the quantities of precipitation that generate maximum runoff with a direct impact on the human component and generate direct risks requires the identification of alternative, innovative solutions for identifying precipitation nuclei and predicting the associated risk in order to reduce it. The development of a methodology for extracting and spatializing quantitative values of precipitation based on radar information is absolutely necessary in the context of the indirect acquisition of input databases in complex spatial analysis models developed to reduce the impact and reduce risks associated with extreme weather events.

As far as we know, the present study is the first attempt in Romania to combine radar and rain gauge precipitation data by applying the CMT to obtain a high-resolution map product for flash flood forecasting and modeling, using data covering four convective seasons (2015–2018).

2. Study Area

The study area is located in the northern part of Romania, within the Someș-Tisa River basin, in Bistrița-Năsăud County, and is highly affected by flash floods, being facilitated by the perpendicular orientation of the drainage basins to the dominant circulation of the air masses, respectively, the big altitude differences. Extending on the southern slope of the Țibleș and Rodna Mountains toward the Somesul Mare riverbed, the study site includes the Țibleș (99 km²), the Sălăuța (414 km²), and the Runc (49 km²) watersheds. Additionally, the Agrieș, Mocod, Rebrisoarva, Parva, and Săcel rain gauges were also included from the nearest neighborhood. The study area is located within the range of the Bobohalma weather radar, positioned northwards (Figure 1).

The Țibleș Mountains (1840 m) are the source of the headwaters of Țibleș and Sălăuța Rivers, while the Runc River originates from the Năsăud Hills, located in the southern side of the mountains. The Sălăuța River collects its waters not only from the eastern and southeastern sides of the Țibleș Mountains, but also from the western slopes of the Rodna Mountains, draining also the Năsăud Hills towards the south. The Țibleș River collects its waters draining along the southern slopes of the Țibleș Mountains and the Năsăud Hills. All three rivers are right bank tributaries of the Someșul Mare River [28]. These catchments are subject to heavy rainfall and extreme precipitation, leading to flash flooding events as they drain the mountainous and hilly terrain.

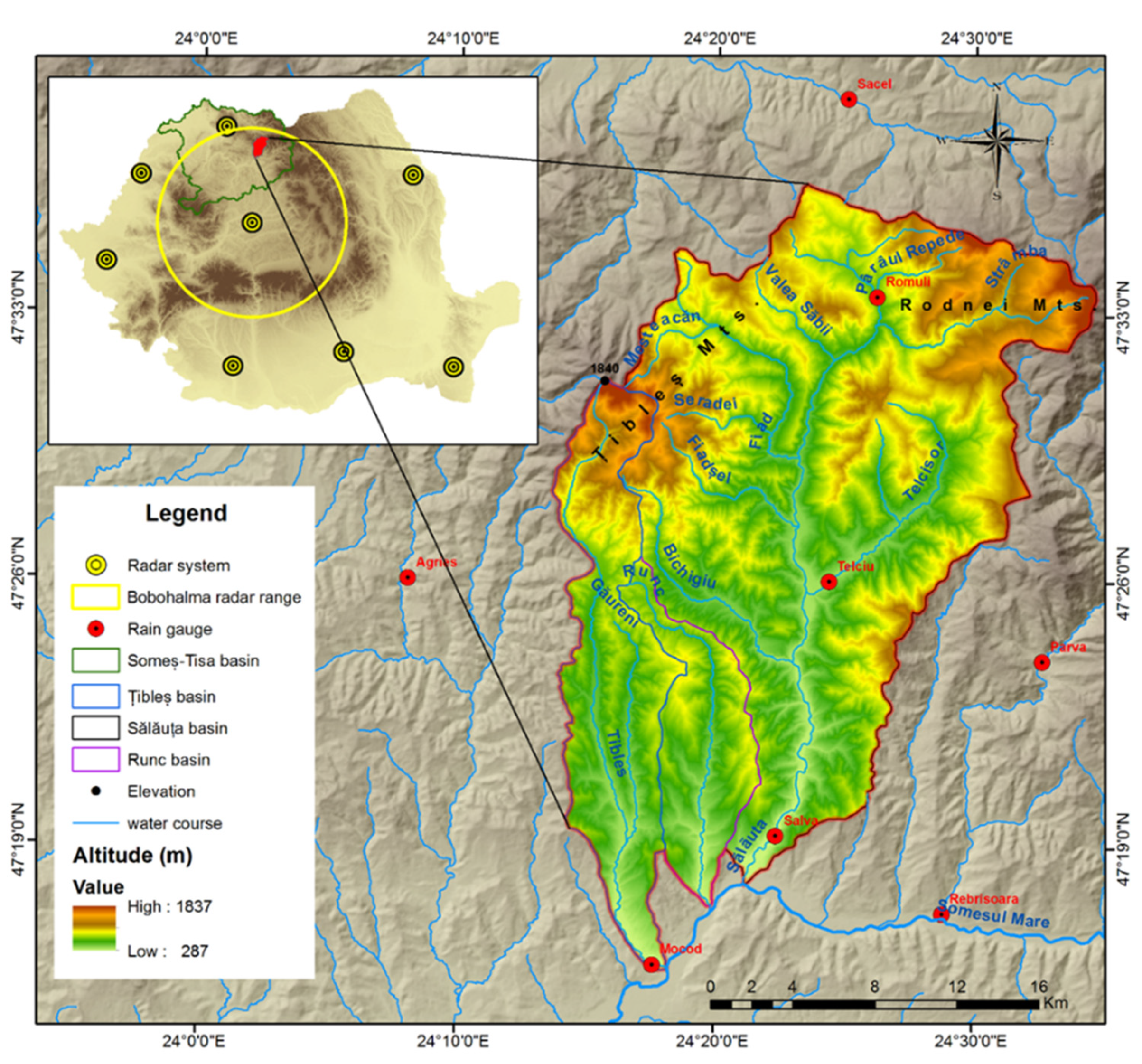
3. Methodology and Database

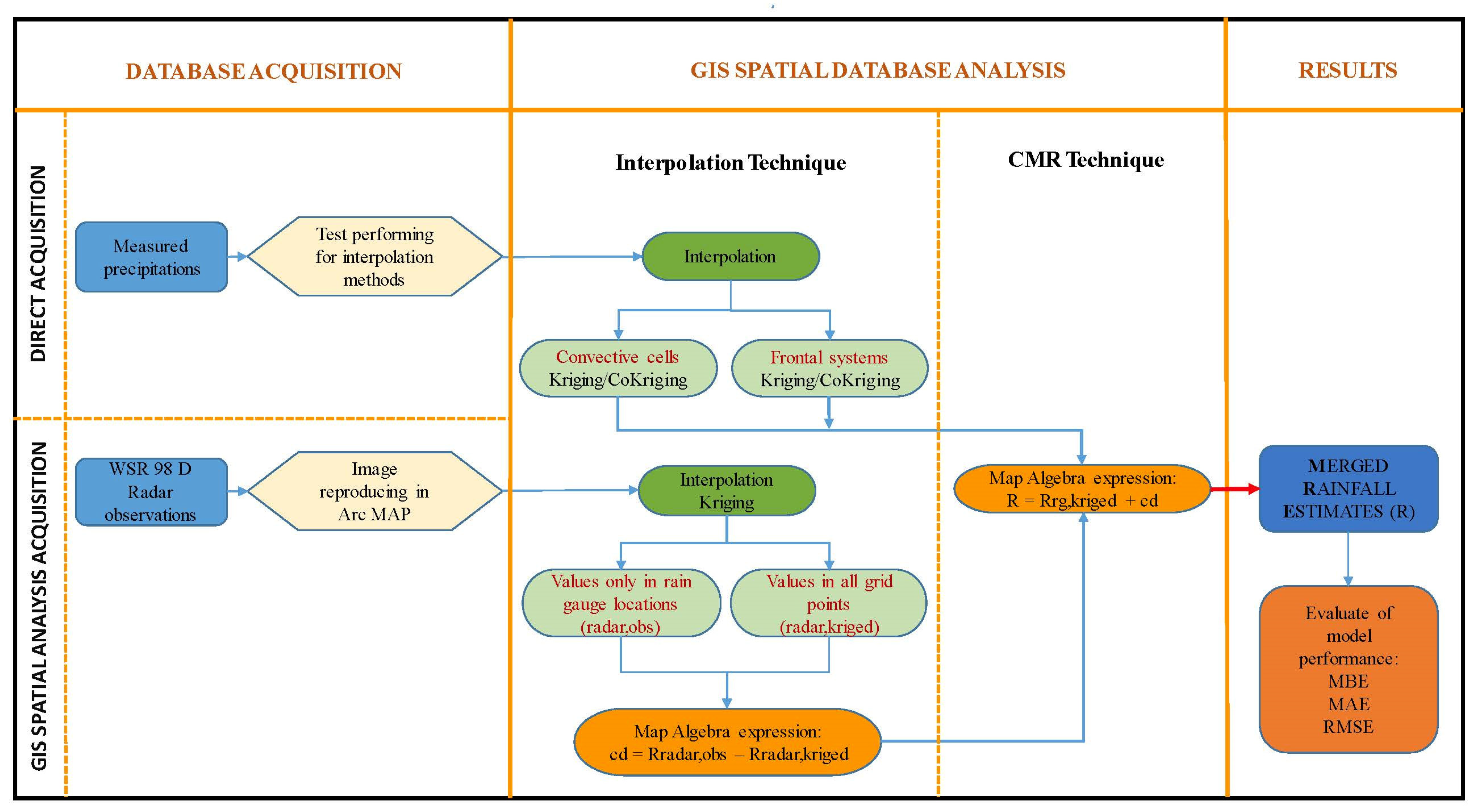
Geographic Information Systems (GIS) provide a platform where the measurements from both radar and rain gauges can be displayed in the same spatial framework, allowing the comparison, analysis, and mathematical correction of the precipitation estimate [29]. In order to combine radar and rain gauge rainfall data using the CMT method in our study area, the collected data, presented below, were processed through many methodological steps. The overall methodological workflow is graphically represented in Figure 2.

From a methodological point of view, the research approach is based on two main stages that define a model for the integrated analysis of singular components in order to obtain the results materialized in databases useful in the flash flood modeling process. The proposed methodology involves the acquisition of databases that substantiate the model of spatial analysis through two distinct and different techniques: direct acquisition (24 h precipitation measured at rain gauges) and indirect acquisition based on spatial analysis supported by the integration of remote sensing images (24 h radar rainfall intensity).

The indirect acquisition of the databases within the proposed model is materialized in a submodel of spatial analysis that is validated through the databases acquired directly. Thus, the stage of the acquisition of the database has a double role within the general model, that of ensuring the input of spatial databases and of validating the spatial analysis submodel.

To validate the CMT in the study area, 15 rainfall events were selected and analyzed. The events occurred in the period of 2015–2018, from the second half of May until mid-September (

Table 1. Rainfall events between 2015–2018.

No.	Data Events	Precipitation Warnings at Mocod Start/End Time	Duration hh: mm	Amount l/mp	Rain Gauges 24 h Precipitation Amounts							Rain Type *
					Mocod	Salva	Telciu	Romuli	Rebrisoara	Parva	Agries
1	6 June 2018	14:15–15:30	01:15	27.7	27.7	3.5	3.9	0	0.0	0	0	C.c.
2	3 June 2018				15.5	24.0	9.6	5.2	10.2	14.4	10.5	F.s.
3	13 June 2018				14.0	26.3	10.3	5.8	23.7	9.1	39.7	F.s.
4	23 June 2018				10.0	12.9	14.4	32.6	10.5	17.9	21.3	F.s.
5	17 August 2018				14.7	21.2	8.7	20.4	12.3	23.5	5.2	F.s.
6	16 May 2018				16.0	12.0	13.8	21.8	14.4	13.1	9.9	F.s.
7	17 May 2018				12.1	24.5	21.0	22.6	18.5	17.8	13.1	F.s.
8	14 May 2017				51.0	25.2	19.1	4.9	31.3	11.8	17.7	F.s.
9	25 May 2017				16.0	6.0	7.3	10.6	8.9	11.0	10.6	F.s.
10	26 May 2017				23.0	21.0	19.6	18.6	27.7	25.2	8.1	F.s.
11	3 September 2017	16:45–18:45	02:00	22.5	25.0	17.9	20.6	30.7	23.5	27.8	30.9	C.c.
12	21 June 2017	15:00–16:45	01:45	29.0	29.0	15.5	15.3	2.8	22.5	7.5	12.3	C.c.
13	15 June 2016	15:40–18:40	03:00	38.0	39.6	14.0	30.7	4.5	9.8	14.6	7.7	C.c.
14	26 July 2016	17:00–19:00	02:00	38.5	41.0	9.0	0.9	11.0	68.2	4.2	0.7	C.c.
15	5 September 2015	08:00–18:00	10:00	32.0	50.0	34.0	28.3	28.2	45.3	37.8	21.6	C.c.

* C.c.—Convective cells; F.s.—Frontal systems.

).

The analyzed rainfall events had two types of origins: convective cells and frontal systems. In the study, there were selected convective cells and frontal system rainfall events based on quantitative criteria when the precipitation thresholds were exceeded at least at one rain gauge, or water amounts were recorded at all rain gauge points and ones triggering flash floods. The precipitation warnings, respectively, the 24 rainfall amount datum were obtained from the Somes Tisa Water Branch dispatcher service database. The most significant rainfall events from 2006 to 2018 were identified and selected in terms of quantities, correlated with flash floods at Romuli, Telciu, Salva, and Mocod hydrometric stations, located at the endpoint of the study area. The selection of events was also determined by the availability of radar data for the identified events.

Warning thresholds for precipitation affecting hydrological cycles are determined by the Romanian National Hydrological Service as the following:

• Attention threshold: 15 mm/m² in a maxim of 3 h;
• Alert threshold: 25 mm/m² in a maxim of 6 h;
• Danger threshold: 25 mm/m² in a maxim of 1 h determining sudden increases in water level and overland water runoff.

Six of them had a torrential character that exceeded the precipitation warning thresholds. Convective cells develop in unstable air masses, where high temperatures at the surface generate intense upward air currents. These are often accompanied by storms and occur predominantly in the afternoon. Convective rainfall is of high intensity but usually has a short duration and limited spatial extension. According to Cazacioc [30], 70 of the largest maximum daily precipitation amounts reported from 94 Romanian stations between 1961–1996 were summer storms. A characteristic attribute of this type of rainfall is its extreme spatial variability [9].

Nine rainfall events were generated by frontal systems. Compared to convective rainfall, their spatial extension and duration were significantly higher (continuous precipitation).

Two main datasets were used in this study: 24 h precipitation measurements from eight rain gauges from the “Romanian Waters” National Administration, Someș-Tisa Water Branch network (

Table 2. Rain gauge characteristics.

Characteristics	Rain Gauges
	Agrieș	Mocod	Salva	Telciu	Romuli	Săcel	Parva	Rebrișoara
Elevation (m)	498	296	318	392	611	517	520	339
Distance from Bobohalma (km)	119.5	100.7	107.5	120.1	134.2	143.6	118	105

), and 24 h rainfall intensity observations from the National Meteorological Administration WSR98-RDBB Bobohalma radar. The radar system is located in Mureș County at an altitude of 523 m, having a geographical position with a Lat of 46.360 deg. and Long. of 24.255°, with a range of 230 km and a resolution of 4 km² that operates in the S-band.

Given that the meteorological radars dataset provides continuous spatial coverage and captures the rainfall variability with less uncertainty than simple point data, it has become a reliable source of input data for hydrologic models.

In order to identify the radar that provided the most realistic images of the rainfall events that occurred in 2015–2018, we compared the estimated precipitation intensities (in the case of convective rains), respectively, the 24 h precipitation estimates (in the case of frontal systems) between the radar from Oradea (located west of the study area) and the one from Bobohalma (located in the south of the study area). The measured precipitation amounts at the rain gauges confirmed the high accuracy of the images provided by the Bobohalma radar. The distances from the two radars are approximately equal to the study area, but the radar from Oradea is shaded by the Apuseni Mountains (1849 m). In the case of the radar from Bobohalma, there are no orographic obstacles [31].

In order to provide reliable information and to be able to integrate into the Romanian National Meteorological System, the Bobohalma radar range was set to 230 km. Given that the farthest rain gauge (Sacel) is located only 134.2 km from the Bobohalma radar, and the entire study area represents a homogeneous area in terms of relief, with parallel valleys drained to the south in the direction of the radar, the range does not affect the precision of the radar products. Doppler radars have the best accuracy in estimating precipitation between 50–150 km from radar.

The territory of Romania is currently monitored by eight Doppler weather radars that provide the location, spatial extent, and intensity of atmospheric precipitation. The WSR-98D radars estimate the rainfall rate using the NEXRAD Z-R (reflectivity-rainfall rate) relation: Z = 300 R1.4. To completely sample the atmosphere, they employ scanning strategies or volume coverage patterns (VCP). A VCP is a series of 360-degree sweeps of the antenna at pre-determined elevation angles completed in a specified period of time [32]. The available information is defined by high spatial and temporal resolutions (4 km² and 6 min, respectively). The radar products of the National Integrated Meteorological System (SIMIN) are transmitted from the radar location to the corresponding regional forecast center and to the NMA headquarters.

The spatial analysis stage focuses on the interpolation technique as the main method of spatializing the information provided by the input databases, which refer to the pointwise precipitation acquired, and those acquired by analyzing radar images. Interpolation of values is an essential condition in finalizing the spatial analysis given that the CMT is implemented in areas with territorial continuity. The interpolation of discrete precipitation values was performed using two statistical methods based on kriging (to better capture the variation of interpolated values in space without being constrained by variation) and cokriging, because in the process of spatial analysis, the altitudes were used as a basis for precipitation variation.

The kriging family includes several interpolation methods, of which three were used in our study: simple, ordinary kriging, and cokriging. These methods are based on the autocorrelation concept [33], according to which the values of a variable are more similar in close locations and the degree of similarity decreases with point distance. The semivariogram function, which models this similarity, is the basis for deriving point weights which are further used to estimate values in unsampled locations. Unlike simple and ordinary kriging, the cokriging method uses co-variables in addition to derive the spatial model. In our study, we used elevation as a covariate in cases where precipitation was correlated to this terrain parameter.

Since the spatial distribution of precipitation data associated to a rainfall event is quite localized, the use of other interpolation methods, such as regression and regression-kriging, is not necessary. However, to account for elevation dependence which, in some cases, may be important, we also tested the cokriging method with elevation as a covariable, as mentioned before.

To accomplish this task, we applied the conditional merging technique developed by Ehret [9]. Accordingly, radar information can be used to correct the interpolation of the rain gauges. The result is an estimated merged rainfall field, which preserves the radar spatial structure being conditioned at the same time using rain gauge data [5]. In addition, the advancement in the merged product is equally applicable to stratiform and non-correlated meteorological conditions, removing the requirement to use different merging schemes for different type of rainfall events.

The main concept of the merging process follows the steps below.

• The precipitation field was observed at the rain gauge points (Rrg, obs).
• The precipitation field was observed by radar on a regular, volume-integrated grid (Rradar, obs).
• Cokriging used rain gauge observations to obtain the best linear unbiased estimate of precipitation, correlated with the radar grid points (Rrg, kriged, where R is precipitation and rg is rain gauge).
• Ordinary kriging is used for radar pixel values, exclusively those from the rain gauge point locations to estimate the interpolated radar rainfall at each grid point (Rradar, kriged).
• At each grid point, the difference cd of the observed and interpolated radar values was calculated using a suitable method. At the rain gauge locations, the cd is always equal to zero.
• The field of differences (cd) was added to the rain fields from rain gauge interpolation.
• A merged rainfall field was obtained that maintained the mean-field of the rain gauge interpolation while preserving the mean-field deviations and spatial structure of the radar data.

The deviation cd between the observed and interpolated radar field was calculated as a simple difference according to Equation (1) and the merged field according to (2). Some underestimation occurred and negative merged values had to be excluded, setting a lower limit of zero [9]. This problem was solved by using the “Map Algebra expression” from the ArcMap Raster Calculator.

cd = Rradar,obs − Rradar,kriged

(1)

R* = Rrg,kriged + cd

(2)

where cd [mm/h] is the difference between the radar pixel and interpolated radar value; Rradar, obs [mm/h] is the observed radar pixel value; Rradar is the kriged [mm/h] interpolated radar value; Rrg, kriged [mm/h] is the interpolated rain gauge value; R* [mm/h] is the merged rainfall estimate (MRE).

The validation step was performed based on statistical analysis using three validation metrics: mean bias error (MBE), mean absolute error (MAE), and root mean square error (RMSE). We did not compare the performance of the final rainfall model with the rain gauge and radar models because it would require an independent validation sample. Therefore, we used three metrics to assess the capacity of the final model to estimate the spatial distribution of the different rainfall events.

The validation metrics are computed as follows:

$$\mathrm{MBE}=\frac{1}{\mathrm{N}}{\displaystyle \sum }_{\mathrm{i}=1}^{\mathrm{N}}\left({\mathrm{Y}}_{\mathrm{i}}-{\hat{\mathrm{Y}}}_{\mathrm{i}}\right)$$

(3)

$$\mathrm{MAE}=\frac{1}{\mathrm{N}}{\displaystyle \sum }_{\mathrm{i}=1}^{\mathrm{N}}\left|{\mathrm{Y}}_{\mathrm{i}}-{\hat{\mathrm{Y}}}_{\mathrm{i}}\right|$$

(4)

$$\mathrm{RMSE}=\frac{1}{\mathrm{N}}{\displaystyle \sum }_{\mathrm{i}=1}^{\mathrm{N}}{\left({\mathrm{Y}}_{\mathrm{i}}-{\hat{\mathrm{Y}}}_{\mathrm{i}}\right)}^2$$

(5)

where Y_i and ${\hat{\mathrm{Y}}}_{\mathrm{i}}$ are the observed and predicted rainfall values, respectively, and N is the number of observations (number of rain gauges in our case).

The mean bias error (MBE) is used to assess the overall bias of a model, where the positive and negative errors cancel each other out, leading to a small bias. Positive values indicate dominance of positive error, meaning that the model underestimates the point values, whereas a negative mean bias indicates an overestimation of point values by the model.

The mean absolute error (MAE) is the average of the point errors regardless of their sign. The closer it is to zero, the better the model.

The root mean square error (RMSE) was similar to MAE. However, by squaring the differences between the observed and predicted values, the RMSE assigns higher weights to larger errors. RMSE will have significantly higher values when the MAE presents large errors.

4. Results

The main database that substantiated the proposed GIS spatial analysis models is represented by radar images obtained in analog format, images that required detailed processing of the information presented, in order to obtain a database structure with high accuracy for inclusion within the proposed research methodology.

Obtaining databases in raster format representing the averages of the observed precipitation is outlined in the initial results with a major impact within the proposed models, results obtained based on the spatial analysis of the vector structures, and the raster–vector overlay.

The Bobohalma radar operating software was released in 1998. In the case of this old software, there is no possibility to export files in raster format, ones compatible with ArcGIS applications. In this way, in processing radar data, we had to export jpg images and reproduce them in a GIS environment.

To exclude the redundancy elements of the radar map (distance circles, names of localities, and administrative contours), the vectorization of the radar images was manually processed. This working procedure requires the following operations.

• The 24 h precipitation data from the radar images of Bobohalma were extracted and provided in jpg format by the Regional Meteorological Center “Transylvania North” (CMRTN).
• The radar images were imported in ArcMap, version 10.7.1 (Esri, Redlands, CA, USA). with selected watercourses and road networks.
• Georeferencing the images considering well-highlighted reference points on the images, the assignment of the stereo’s 70 coordinates to these points was obtained. As a result, all the images have the same geographical coordinates and overlap perfectly, allowing further operations.
• Vectorization of a polygonal-type grid file (mask), according to the resolution (4 km²) of a georeferenced radar image including 332 polygons (Figure 3).

  Figure 3. Left—24 h rain intensity radar image. Right—radar image reproduced in ArcMap, an event from 13 June 2018.

  Figure 3. Left—24 h rain intensity radar image. Right—radar image reproduced in ArcMap, an event from 13 June 2018.
• Overlap the created grid over each radar image and assign the precipitation values to each polygon (Figure 3).
• Creating attribute tables for the analyzed rainfall events.

The spatial representation of climatic variables is a problem because of the small number of control points (weather stations and rain gauges) and the complexity of the factors that influence the spatial distribution of these elements. Spatial interpolation involves finding a function (relative to the x and y coordinates) that is representative of the entire study area, starting from point data of known x and y coordinates. To establish the link between the amount of precipitation and an independent parameter (for example, altitude), we used a linear regression method [18].

To choose the most suitable interpolation methods for processing the datasets used in this study, numerous tests were performed by comparing the results from different spatial interpolation methods. Thus, for the interpolation of daily precipitation, we opted for the kriging methods. We used the simple, ordinary, and cokriging techniques among the several variants of the existing kriging methods in this study. The selection of the methods was based on the root mean square error, smallest nugget, and largest partial sill (

Table 3. Interpolation tests results.

Rainfall Event Date	Rainfall type	Method *	Nugget	Partial Sill **	RMSE	Standardized RMSE
16 May 2018	F.s.	Cokriging	0	2.522	6.820	2.713
16 May 2018		Ordinary kriging	0	24.655	5.885	1.393
25 May 2017	F.s.	Cokriging	0	6.023	3.905	1.835
25 May 2017		Ordinary kriging	0	14.044	3.633	1.213
3 September 2017	C.c.	Ordinary kriging	0	1.611	4.387	0.919
3 September 2017		Ordinary kriging	1.695	35.384	4.119	0.859
3 September 2017		Cokriging	0	205.993	3.360	1.045
5 September 2015	C.c.	Simple kriging	0	2.672	9.021	0.984
5 September 2015		Ordinary kriging	0	471.775	9.146	0.988
14 May 2017	F.s.	Simple kriging	0	3.152	9.763	0.659
14 May 2017		Ordinary kriging	0	586.905	10.965	0.893
21 June 2017	C.c.	Simple kriging	0	2.662	6.823	0.971
21 June 2017		Ordinary kriging	0	318.457	6.885	0.980
21 June 2017		Cokriging	0	−611.112	6.366	0.802
15 June 2016	C.c.	Simple kriging	0	2.511	14.094	1.114
15 June 2016		Ordinary kriging	0	187.830	13.359	0.987
26 July 2016	C.c.	Cokriging	0	−422.270	25.419	1.419
26 July 2016		Simple kriging	0.500	2.363	22.613	0.707
3 June 2018	F.s.	Cokriging	0	−316.768	10.405	2.627
3 June 2018		Ordinary kriging	18.700	74.480	5.853	0.883
13 June 2018	F.s.	Cokriging	0	−325.896	15.209	1.666
13 June 2018		Ordinary kriging	0	230.470	12.241	0.996
6 June 2018	C.c.	Cokriging	0	−174.370	11.508	1.864
6 June 2018		Simple kriging	1.142	0.000	8.802	0.775
26 May 2017	F.s.	Cokriging	0	−82.306	4.018	0.762
26 May 2017		Ordinary kriging	0.070	72.116	4.889	1.727
17 May 2018	F.s.	Cokriging	0	−35.704	7.770	2.102
17 May 2018		Ordinary kriging	0	46.481	6.960	1.213
23 June 2018	F.s.	Cokriging	0	225.209	6.588	1.319
23 June 2018		Ordinary kriging	0	118.850	8.911	2.186
17 August 2018	F.s.	Cokriging	0	−48.498	11.497	2.602
17 August 2018		Ordinary kriging	0	74.057	9.506	1.306

* The selected method is marked in italics. ** partial sill values represent semivariance values for ordinary kriging, semivariance normal score values for simple kriging, and covariance values for cokriging.

).

Kriging assumes that the values of a spatial variable are auto-correlated over short distances. The existence of spatial autocorrelation can be verified by calculating the semi-variances of the values separated by increasing distances [34]. Using variography, we obtain indications of how similar or different the values of adjacent data points are as a function of their distance from each other. Among the standard semivariograms, the spherical, exponential, and stable ones were used.

In the case of simple kriging, the unknown value is estimated as the weighted average of the values from the neighboring points. This method was applied for one convective cell type precipitation (Cumulonimbus clouds), when large amounts of water fall in small areas within a short time range (1–3 h), and for one frontal system type precipitation.

Ordinary kriging was applied to residuals obtained after the removal of the arithmetic mean [34]. The two methods were also used to interpolate the precipitation values derived from the radar measurements.

Beside the convective cells type of rainfall, cokriging was applied for interpolation of the frontal-type rainfall measured on the ground too, with a relatively uniform spatial distribution over a large area. In this case, the linear correlation between altitude and precipitation can be determined. Thus, the altitude of the rain gauges was used as an auxiliary variable. This integration makes the interpolation procedure more laborious and is necessary to obtain the auxiliary semivariogram and cross-semivariogram.

The conditional merging technique was applied to 15 rainfall events. In this study, the event that occurred on 13 June 2018, is detailed. The 24 h accumulated rainfall estimated by the radar and interpolated from the rain gauge observations are shown in Figure 4a,b. Comparing the two images, the higher spatial resolution of the radar-derived data is obvious. We noticed that the area with higher rainfall values partially covered the rain gauge locations on the western extremity of the area. The radar field showed a more reliable rain distribution. In this case, the rain estimated by the radar was higher than that observed by the rain gauges.

Figure 4c shows the interpolated rain field estimated by the radar at the rain gauge locations. Upon comparing this picture with Figure 4b, the same structure may be observed, but with higher values. The final product obtained by the conditional merging technique is presented in Figure 4d.

The mean bias error was negative in all cases, indicating that the rainfall model tended to overestimate precipitation at rain gauge locations. The lowest bias error (−2.225 mm) was associated with the rainfall event (convective cell) on 16 June 2016, indicating that the precipitation of this event was the most overestimated by the model among the other analyzed rainfall events. In contrast, the bias value closest to zero (−0.022 mm) is associated with the rainfall event on 23 June 2018 (frontal system). Overall, the bias error values are small for most of the rain gauges, and, therefore, they can be considered as negligible (11 out of 15 rain gauges present MBE values between −0.5 and 0 mm; these errors represent less than 1% of the average precipitation values for 9 rain gauges and 1–2% for 3 rain gauges).

In agreement with the MBE value, the mean absolute error (2.225 mm) and root mean square error (4.188 mm) also indicated that the model is less accurate for the rainfall event on 16 June 2016.

Based on the low MAE and RMSE, the model performs best in the case of rainfall events on 3 June 2018 and 26 May 2017 (both have frontal system origins).

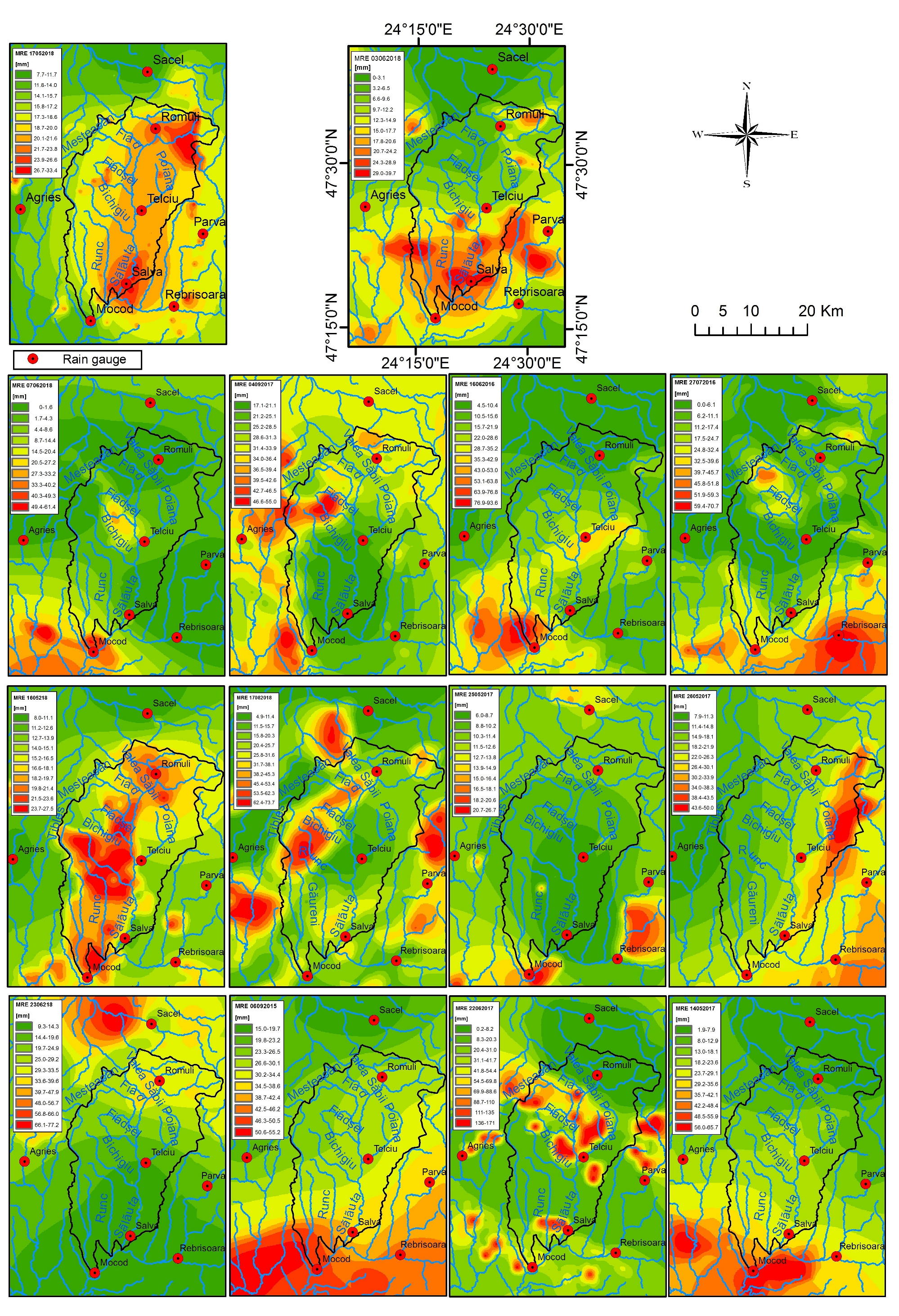
The final high-resolution merged rainfall estimate map obtained for the 15 studied rainfall events offers a real spatial distribution of precipitation over an analyzed catchment, keeping the radar spatial structure while being conditioned at the same time using rain gauge data (Figure 5). It can be observed that the highest amounts of precipitation in the case of some events are discharged in the same location, or close to the rain gauges. Using this final map product, we can improve the quality of the input precipitation data for hydrological models.

Analyzing the merged rainfall estimates’ final product maps, we can emphasize the fact that both in the case of convective and frontal systems rainfall events, the Bobohalma radar detects the areas of precipitations and has a good estimation of the maximum values recorded on the entire studied area.

In the case of frontal systems rainfall events, the weather radar represents difficulties in terms of correctly estimating the precipitation values at the rain gauge points location. Although the radar detects areas with a significant load of precipitation, it tends to slightly overestimate the values compared to the measured quantities (Figure 6).

On the other hand, in the case of convective cells rainfall events, the weather radar tends to slightly underestimate the values compared to the measured precipitation at the rain gauge points location (Figure 7).

Applying the conditional merging technique to combine rain gauge and radar rainfall estimates precipitation over the database covering four convective seasons, the spatial structure of the merged rainfall field shows us that in most of the cases, the maximum precipitation values were not captured by the rain gauges, even if they were located at the edge of the area with maximum precipitation intensities. Furthermore, the simple use of rainfall measured by rain gauges leads to an underestimation of the magnitude of floods in hydrological processes in small river basins.

The results obtained represent a potential advantage by extending the radar–rain gauge merged precipitation over the Runc small-size watershed, unmonitored from a hydrometeorological point of view, and located between the Țibleș and Sălăuța River basins (Figure 1).

Source of Errors/Model Validation

As mentioned in the introduction, the use of weather radar can lead to multiple errors. We analyzed two types of precipitation data and identified several error sources mentioned in the literature [7,19,30,34,35,36,37], which can seriously influence the quality of the results. For example, in the presence of ice cores (mixed precipitation), the radar overestimates the amount of water. On analyzing the event from 22 June 2017, we can see the extent to which ice influences radar precipitation estimates compared to the quantities measured by rain gauges (

Table 4. Radar precipitation estimates influenced by ice cores.

Rain Gauge	22 June 2017			Hail Observations from Radar
	Measured	Estimated	Final	Time	Size (cm)	POSH/POH * (%)
Agries	12.3	57.2	12.7	-	-	-
Mocod	29.0	47.6	30.1	15.51	3.81	80/100
Parva	7.5	15.9	7.5	13.41 13.47	3.18 3.81	70/100 80/100
Rebrisoara	22.5	69.9	26.0	14.36 15.26	3.81 2.54	80/100 60/100
Romuli	2.8	4.5	2.8	-	-	-
Sacel	0.4	1.3	0.4	-	-	-
Salva	15.5	57.2	16.3	15.13 16.21 16.28	4.45 2.54 3.81	90/100 70/100 90/100
Telciu	15.3	34.9	17.4	12.56 13.02 13.09	2.54 3.18 3.81	70/100 70/100 90/100

* POSH—probability severe hail; POH—probability hail.

). The rain was torrential; the amount of 29.0 L/m² measured at the Mocod rain gauge fell in a short time, between 15.00–16.45, associated with hail.

The next problem is related to extreme values. An outlier deviates significantly from the statistical model (with a large residual value) and marks spatial anomalies. Such a “rebel” value can also be an error and must be verified. If it is determined to not be an error, then we must determine the extent to which this value alters our model statistics, mainly in the case of the regression, which cannot reproduce spatial anomalies [32].

The number of points (rain gauges) in the spatialization of meteorological variables (precipitation) could be another source of error. However, when choosing the correct interpolation methods, the number of rain gauges did not induce significant errors.

Data variability is also a dominant factor influencing the performance of spatial interpolators. As the variability increases, the accuracy of all the methods decreases [19]. It was noted that the accuracy of the model was lower in the case of high-intensity local torrential rainfall events, where large variability of the measured values was detected.

Errors resulting from orographic reasons also appear, for example, the positioning of rain gauges in the “shadow” of the radar. Similarly, the radar is not capable of viewing the bottom section of the cumulonimbus clouds.

Further sources of error could be technical problems (related to the Z-R relationship), inaccurate georeferencing of radar images (false precipitation–latitude correlation), or human errors in terms of the uncertainty of observations, data recordings, etc.

Overall, we notice that the model performed very well in 11 out of 15 rainfall events (approximately 78%), with MAE under 0.4 mm and RMSE under 0.7 mm. The model accuracy was lower in the case of three rainfall events (20%), namely those on 7 June 2018, 6 September 2015, and 22 June 2017 (convective cells), and the lowest for the 16 June 2016 event (

Table 5. Validation metrics computed for rainfall events.

Rainfall Event	MBE	MAE	RMSE
3 June 2018	−0.022	0.028	0.051
13 June 2018	−0.102	0.102	0.224
23 June 2018	−0.161	0.171	0.369
17 August 2018	−0.125	0.125	0.180
16 May 2018	−0.252	0.252	0.456
17 May 2018	−0.037	0.047	0.112
25 May 2017	−0.032	0.032	0.072
26 May 2017	−0.025	0.028	0.050
7 June 2018	−0.568	0.568	1.057
4 September 2017	−0.360	0.371	0.614
22 June 2017	−0.986	0.986	1.514
16 June 2016	−2.225	2.225	4.188
27 July 2016	−0.029	0.088	0.124
6 September 2015	−0.539	0.545	1.485
14 May 2017	−0.076	0.083	0.113
Total rainfall events	−0.369	0.377	0.707

).

The validation of the model is highlighted mainly by a large number of cases compared to the total analyzed cases in which the model performed very well and at the same time by the higher validation percentage of about 78%, a percentage that places the model in the top quantum in terms of percentage validation. The presented model is validated and can be applied on surfaces with the same environmental characteristics in the conditions in which the precipitation core has a high precision and spatial accuracy compared to the analysis surface.

5. Conclusions

This study presents a GIS methodology to combine radar and rain gauge rainfall data using the CMT method developed by Ehret [9]. We obtained reliable merged precipitation maps for 15 rainfall events in small river basins located in the northern part of Romania. This type of information is required as input data in event-based, distributed, semi-distributed, and lumped hydrological models. An important outcome of this study was the validation of the CMT method. In conclusion, we noted that the accuracy of the model was lower in the case of high-intensity, local torrential (convective cells) rainfall events, where extreme values and consequently large variability of the measured values were detected, and with high accuracy in the case of evenly distributed rainfall events (frontal systems). Overall, the final model performed well in estimating the spatial distribution of different rainfall events.

Our findings are in accordance with previous similar studies, highlighting that kriging is the preferred method for comparing and combining gauge and radar data [23,30]. By increasing the number of gauge observations in the study area, we can significantly improve the spatial variation of precipitation, and consequently, the effectiveness of radar-based observation of the merged rainfall decreases.

Furthermore, to accelerate the CMT data processing and to minimize the error generating sources, it is necessary to automate the entire time-consuming working procedure, which represents a disadvantage of this methodology. A solution for this could be to create a model builder in ArcMap, and to upgrade the software that processes radar products, offering the possibility to extract datasets compatible with the GIS environment. The advantage of the presented methodology is that it processes radar precipitation data, which are considered the most reliable, real-time, and accessible data source in flash flood warnings activities.

Our study provides a potential advantage for extending radar–rain gauge merged precipitation to those small-size watersheds where gauge observation is limited.