A Polarimetric Radar Operator and Application for Convective Storm Simulation
Abstract
1. Introduction
2. Materials and Methods
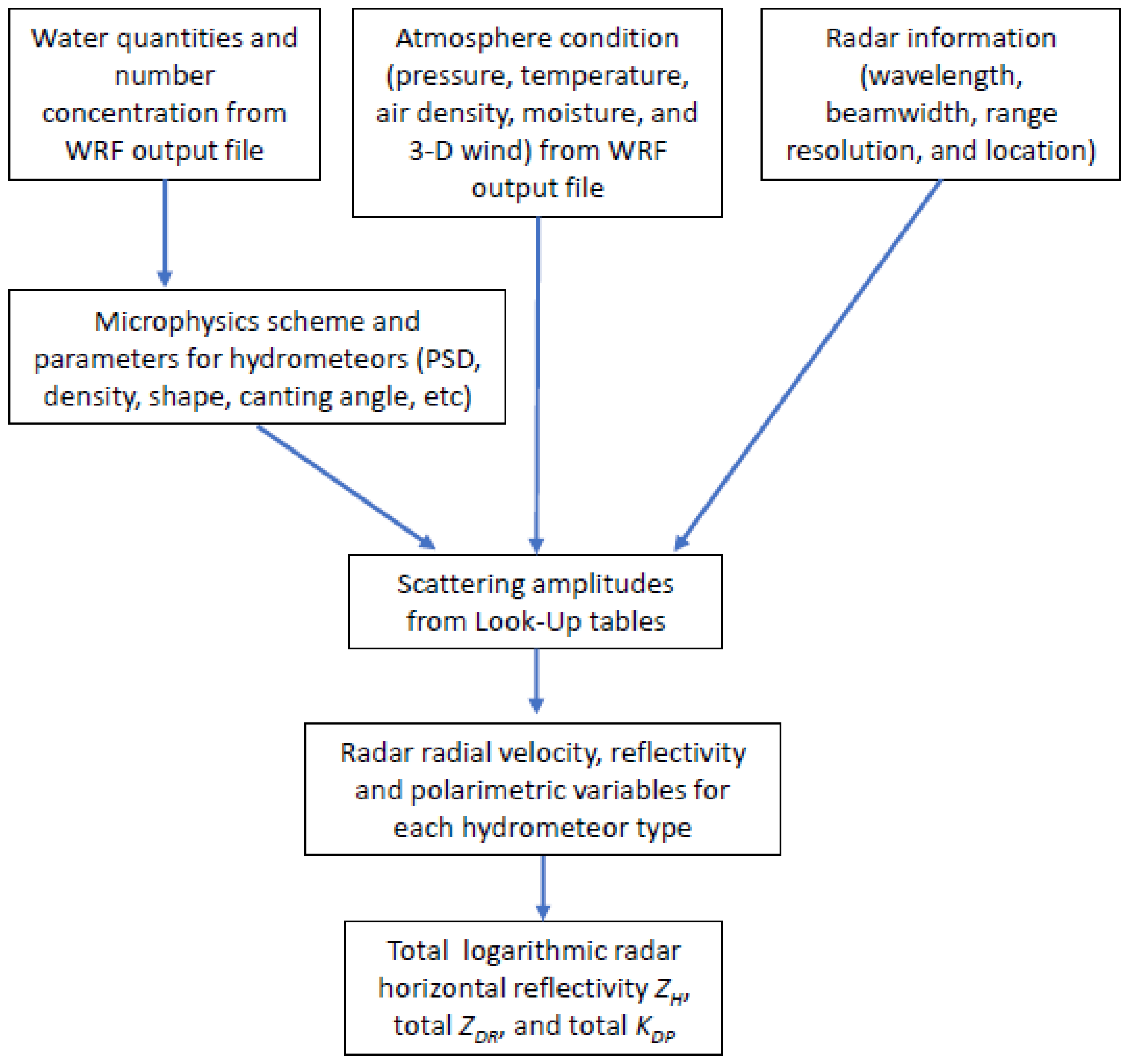
2.1. Polarimetric Radar Forward Operator
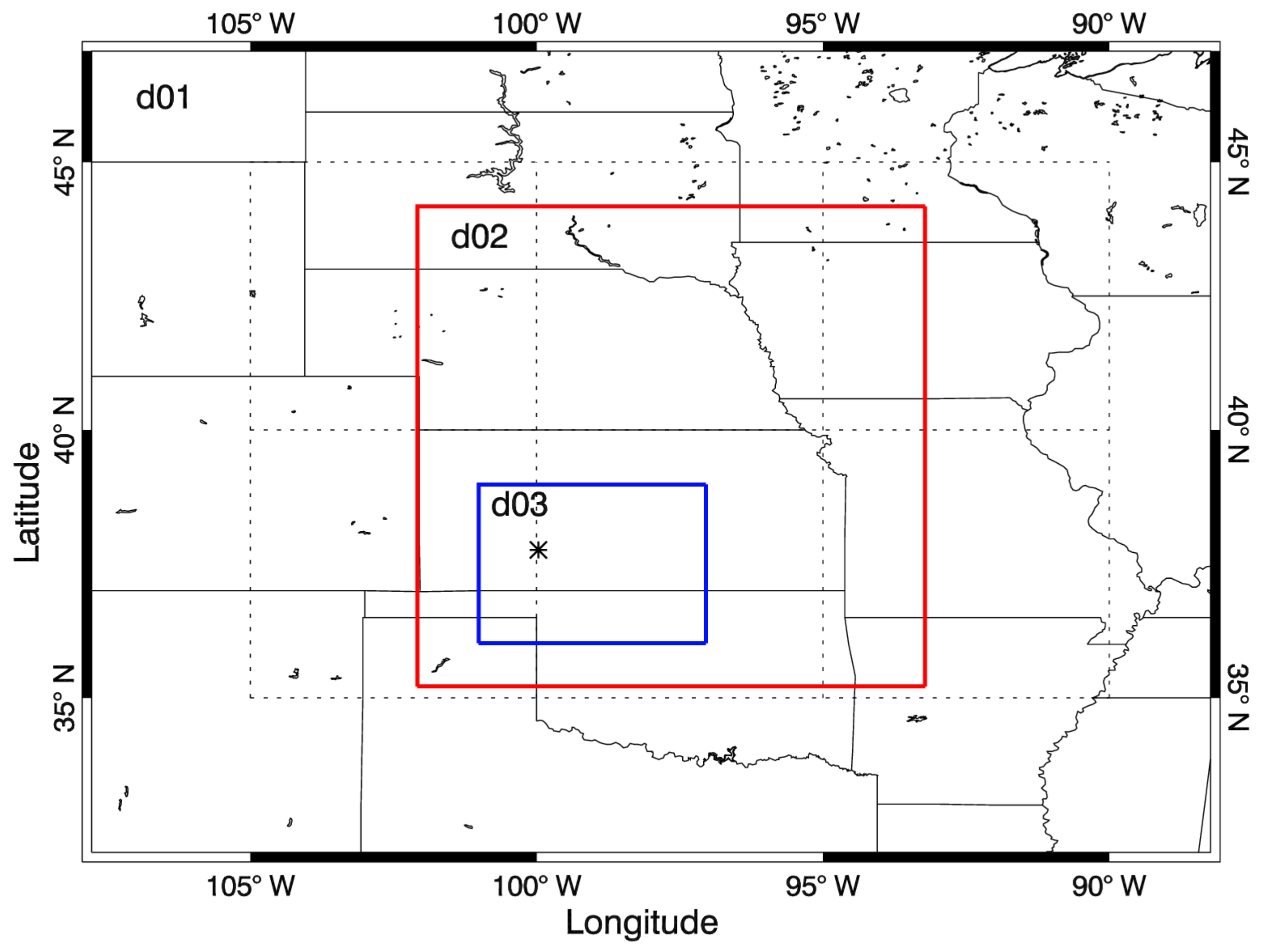
2.2. Numerical Experiments
3. Results
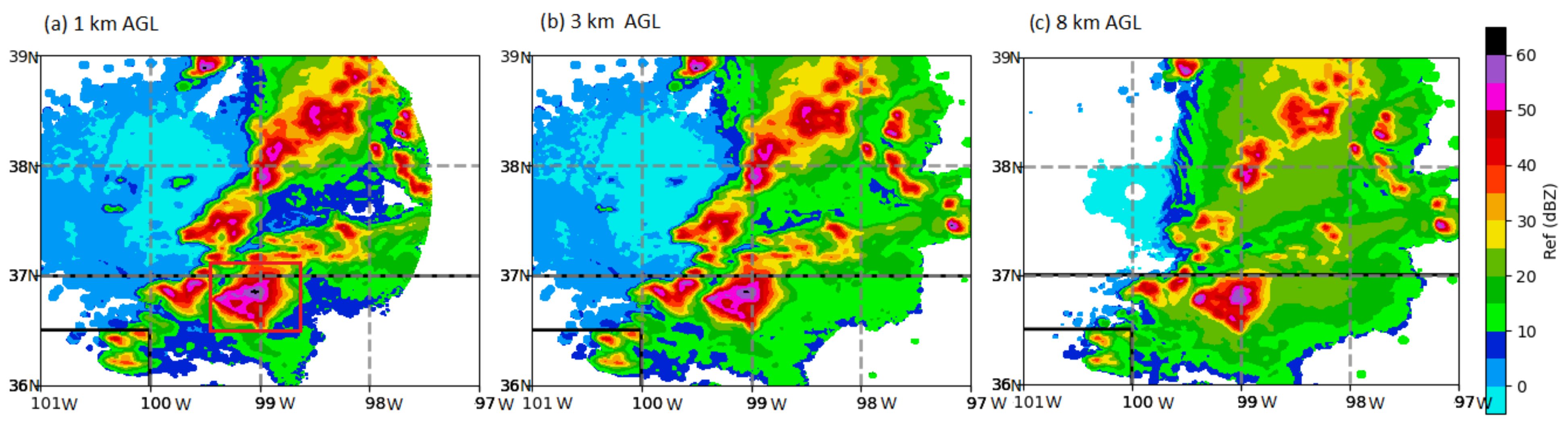
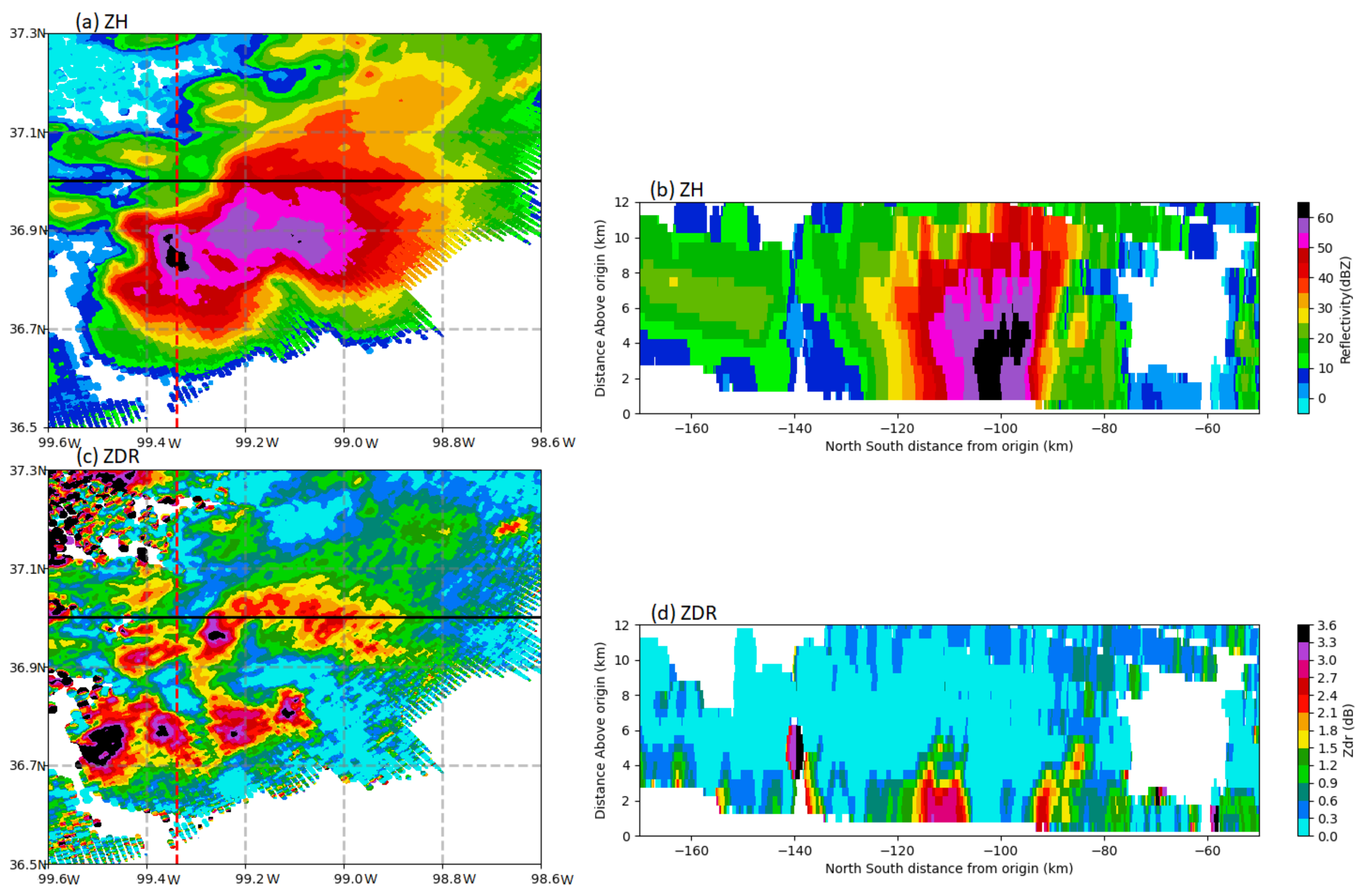
3.1. Case Event and WSR-88D Radar Observation
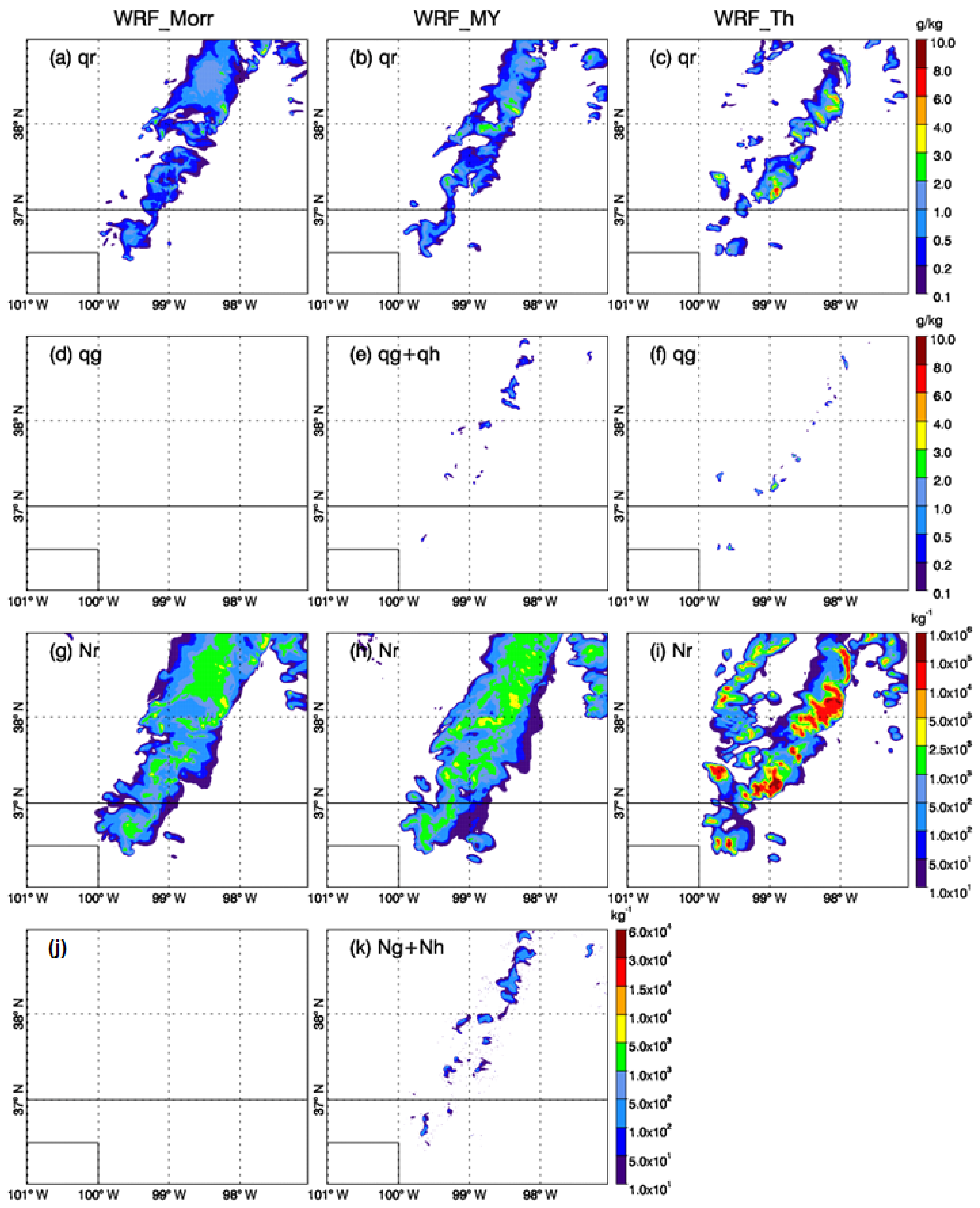
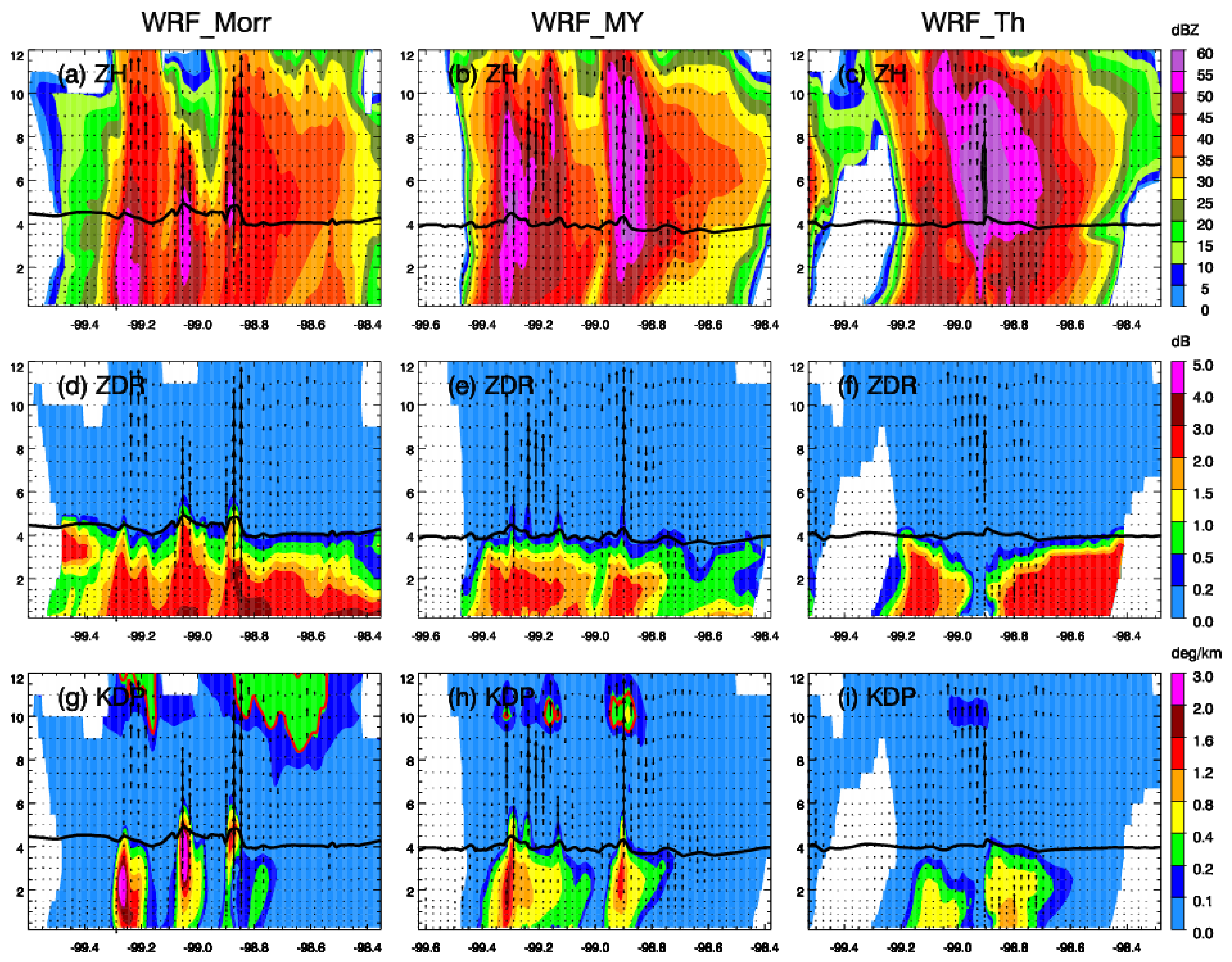
3.2. Radar Operator Result
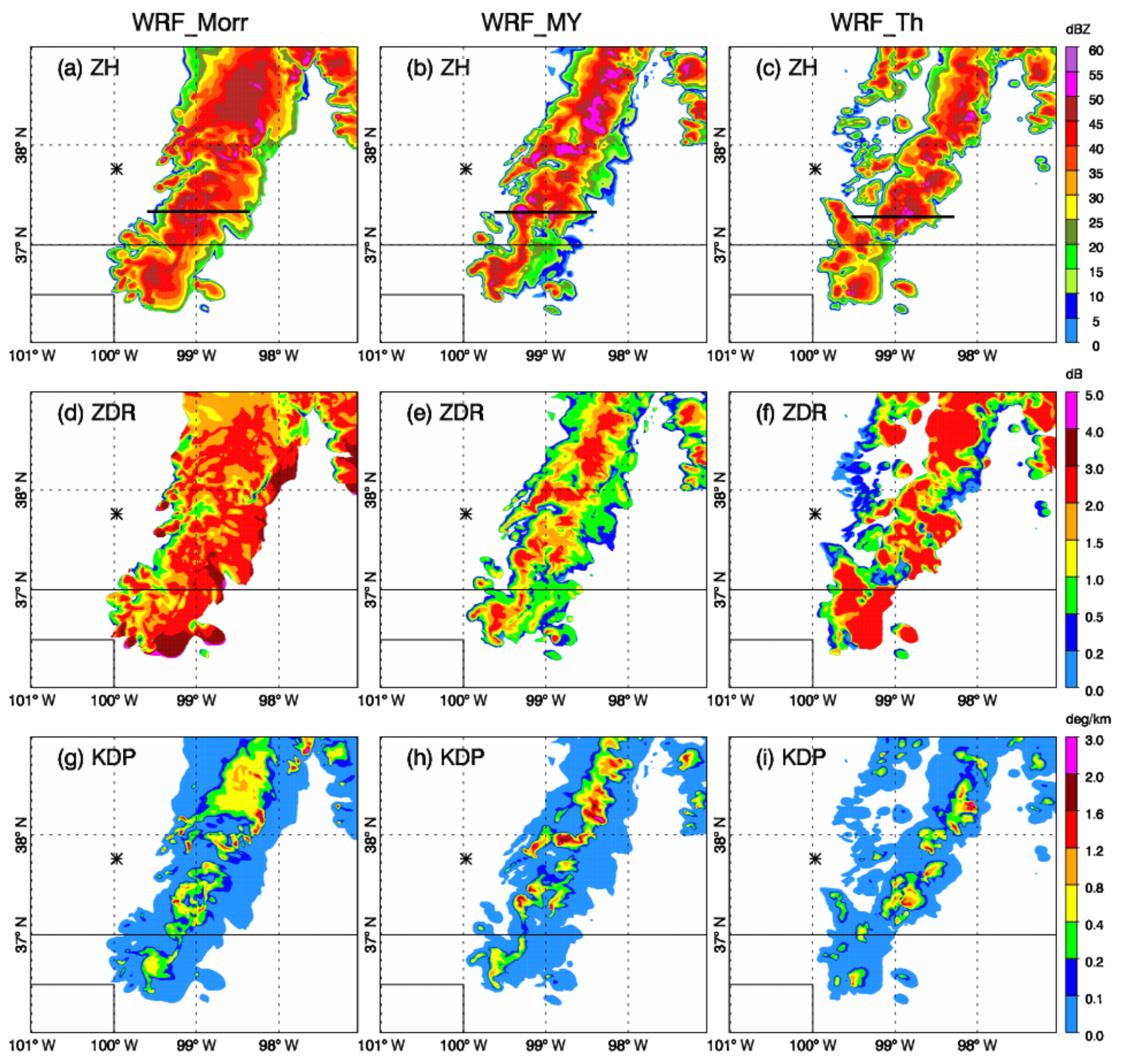
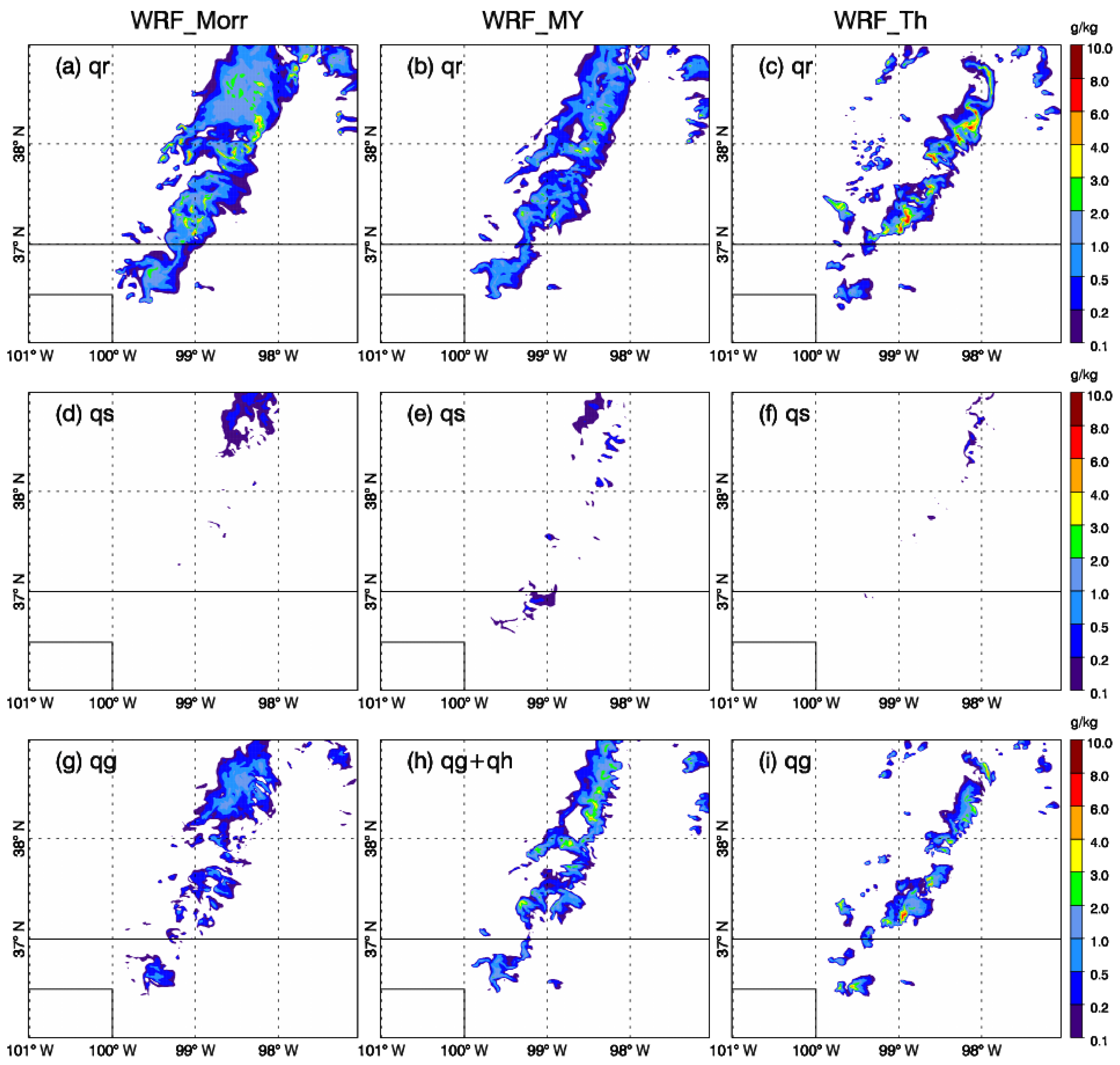
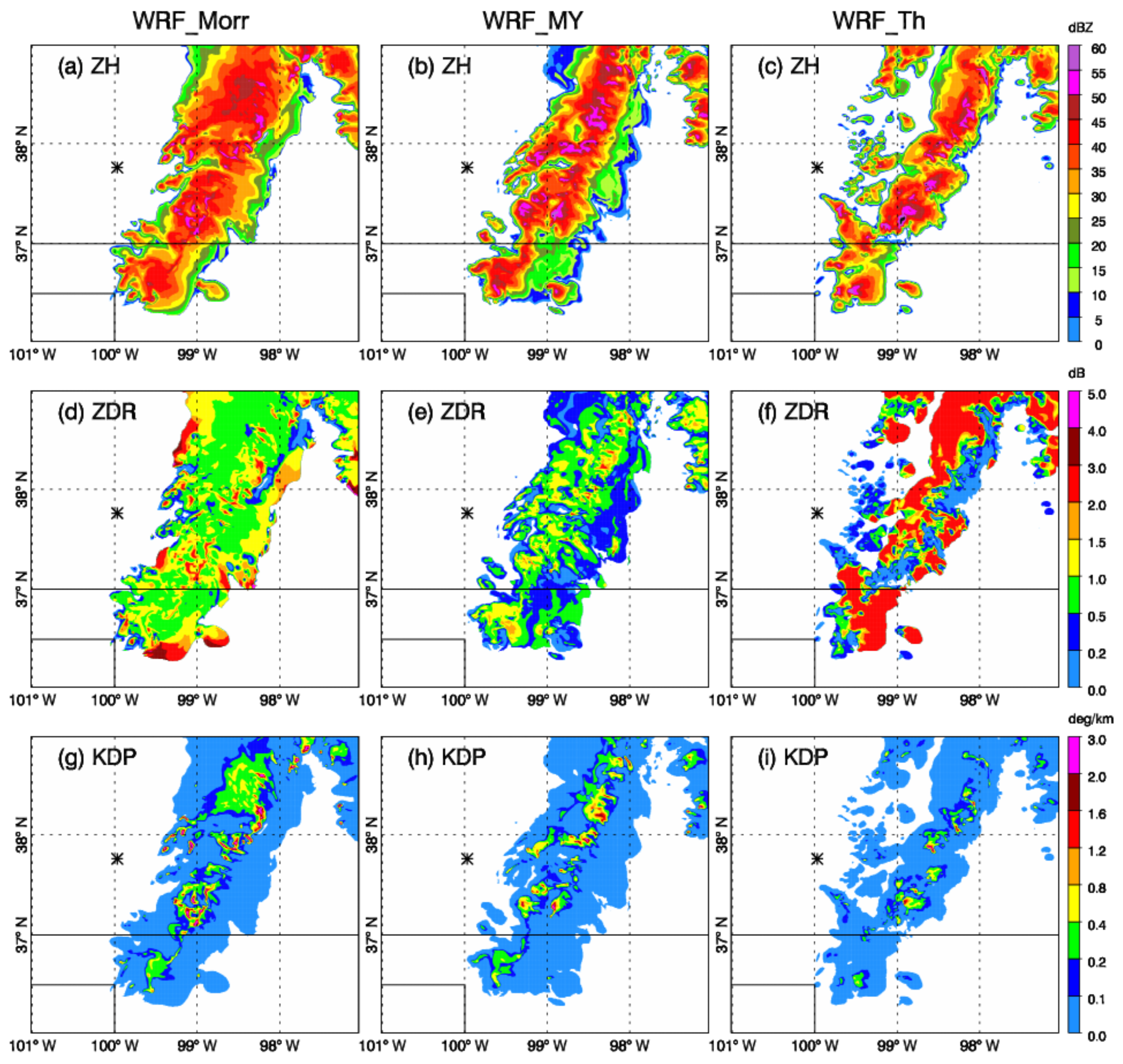
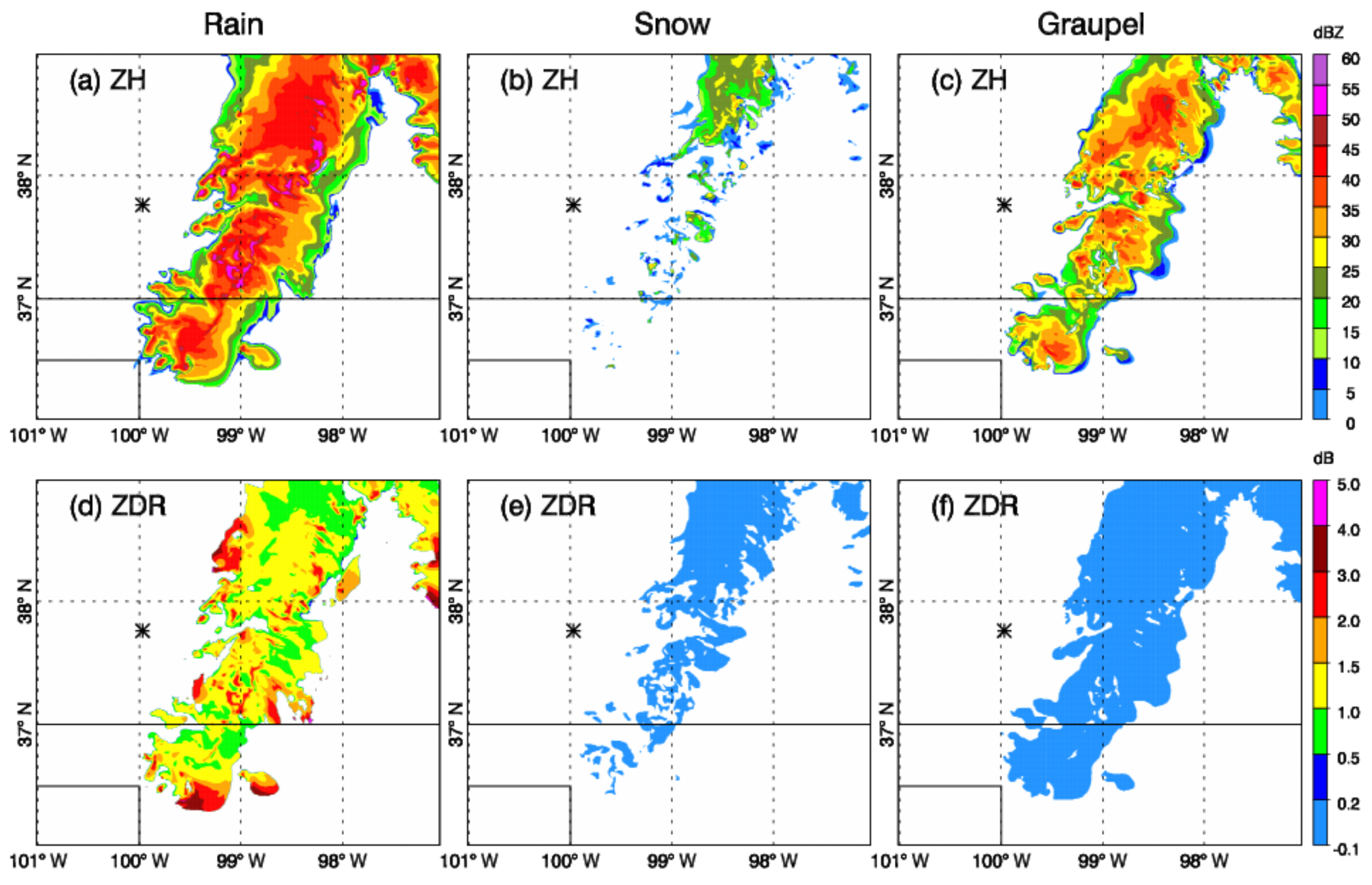
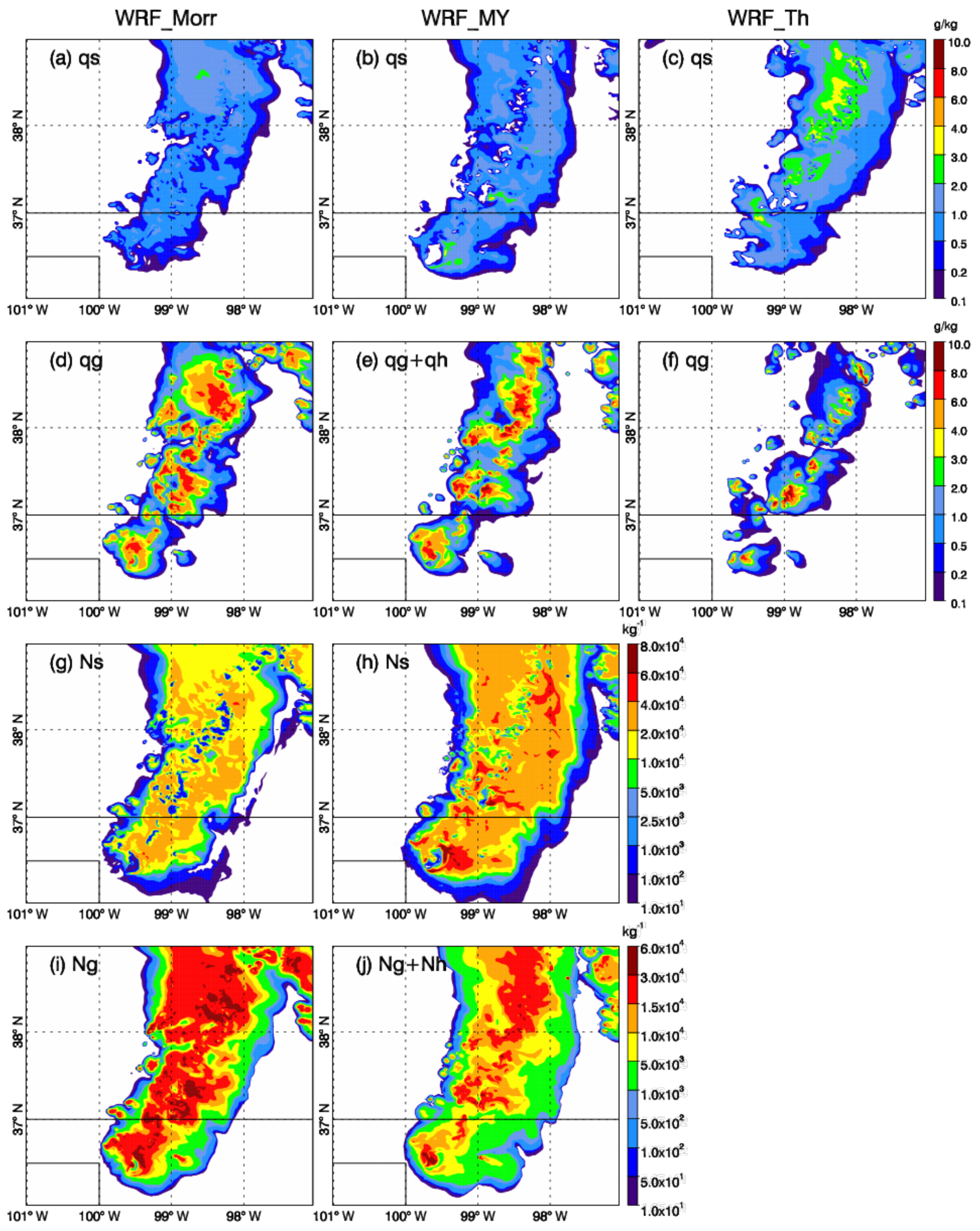
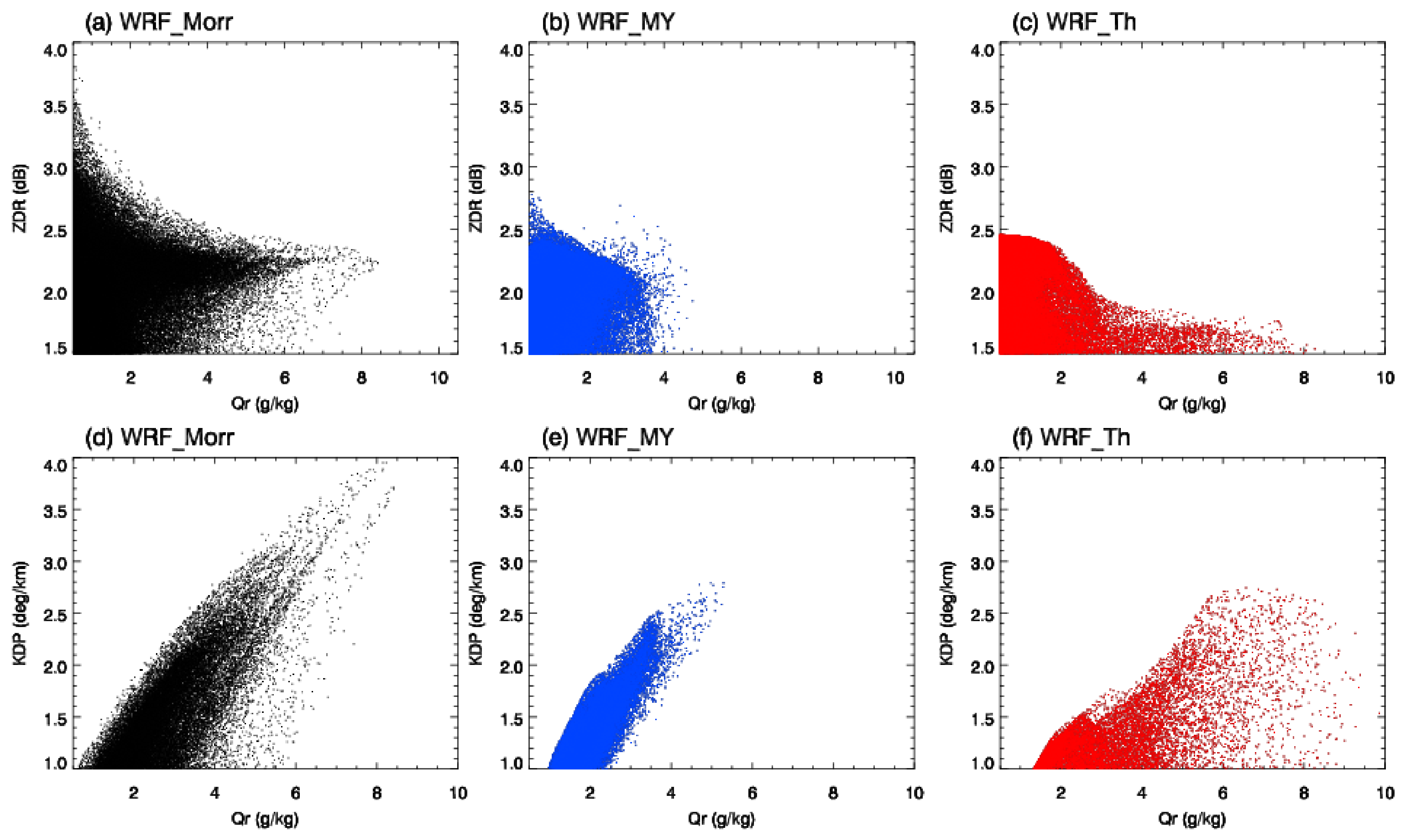
3.3. Observation and Simulated Variables for a Hail Storm
4. Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Rinehart, R.E. Radar for Meteorologists, 4th ed.; Rinehart: Columbia, MO, USA, 2004; p. 482. ISBN 0965800210. [Google Scholar]
- Seliga, T.A.; Bringi, V.N. Potential Use of Radar Differential Reflectivity Measurements at Orthogonal Polarizations for Measuring Precipitation. J. Appl. Meteor. 1976, 15, 69–76. [Google Scholar] [CrossRef]
- Sachidananda, M.; Zrnić, D.S. Rain rate estimates from differential polarization measurements. J. Atmos. Ocean. Technol. 1987, 4, 588–598. [Google Scholar] [CrossRef]
- Straka, J.M.; Zrnić, D.S.; Ryzhkov, A.V. Bulk Hydrometeor Classification and Quantification Using Polarimetric Radar Data: Synthesis of Relations. J. Appl. Meteorol. 2000, 39, 1341–1372. [Google Scholar] [CrossRef]
- Seliga, T.A.; Bringi, V.N.; AI-Khatib, H.H. A preliminary study of comparative measurements of rainfall rate using the differential reflectivity radar technique and a raingage network. J. App. Meteorol. 1981, 20, 1362–1368. [Google Scholar] [CrossRef]
- Seliga, T.A.; Aydin, K.; Direskeneli, H. Disdrometer measurements during an intense rainfall event in central Illinois: Implications for differential reflectivity radar observations. J. Appl. Meteorol. 1986, 25, 835–846. [Google Scholar] [CrossRef][Green Version]
- Ulbrich, C.W.; Atlas, D. Assessment of the contribution of differential polarization to improved rainfall measurements. Radio Sci. 1984, 19, 49–57. [Google Scholar] [CrossRef]
- Ryzhkov, A.; Zrnić, D. Comparison of dual-polarization radar estimators of rain. J. Atmos. Ocean. Technol. 1995, 12, 249–256. [Google Scholar] [CrossRef]
- Hall, M.; Goddard, J.; Cherry, S. Identification of hydrometeors and other targets by dual-polarization radar. Radio Sci. 1984, 19, 132–140. [Google Scholar] [CrossRef]
- Chandrasekar, V.; Bringi, V.; Balakrishnan, N.; Zrnić, D. Error structure of multiparameter radar and surface measurements of rainfall: Part III. Specific differential phase. J. Atmos. Ocean. Technol. 1990, 7, 621–629. [Google Scholar] [CrossRef]
- Zrnić, D.; Ryzhkov, A. Advantages of rain measurements using specific differential phase. J. Atmos. Ocean. Technol. 1996, 13, 454–464. [Google Scholar] [CrossRef]
- Carey, L.; Rutledge, S.; Ahijevych, D.; Keenan, T. Correcting propagation effects in C-band polarimetric radar observations of tropical convection using differential propagation phase. J. Appl. Meteorol. 2000, 39, 1405–1433. [Google Scholar] [CrossRef]
- Zhang, G.; Vivekanandan, J.; Brandes, E. A method for estimating rain rate and drop size distribution from polarimetric radar measurements. IEEE Trans. Geosci. Remote Sens. 2001, 39, 830–841. [Google Scholar] [CrossRef]
- Brandes, E.; Zhang, G.; Vivekanandan, J. Experiments in rainfall estimation with a polarimetric radar in a subtropical environment. J. Appl. Meteorol. 2002, 41, 674–685. [Google Scholar] [CrossRef]
- Vivekanandan, J.; Zhang, G.; Brandes, E. Polarimetric radar estimators based on a constrained gamma drop size distribution model. J. Appl. Meteorol. 2004, 43, 217–230. [Google Scholar] [CrossRef]
- Aydin, K.; Zhao, Y.; Seliga, T. A differential reflectivity radar hail measurement technique: Observations during the Denver hailstorm of 13 June 1984. J. Atmos. Ocean. Technol. 1990, 7, 104–113. [Google Scholar] [CrossRef]
- Aydin, K.; Seliga, T.; Balaji, V. Remote sensing of hail with a dual linear polarization radar. J. Clim. Appl. Meteorol. 1986, 25, 1475–1484. [Google Scholar] [CrossRef]
- Carey, L.; Rutledge, S. Electrical and multiparameter radar observations of a severe hailstorm. J. Geophys. Res. 1998, 103, 13979–14000. [Google Scholar] [CrossRef]
- Hubbert, J.; Bringi, V.; Carey, L. CSU-CHILL polarimetric radar measurements from a severe hail storm in Eastern Colorado. J. Appl. Meteorol. 1998, 37, 749–775. [Google Scholar] [CrossRef]
- Chandrasekar, V.; Kernen, R.; Lim, S.; Moisseev, D. Recent advances in classification of observations from dualpolarization weather radars. Atmos. Res. 2013, 119, 97–111. [Google Scholar] [CrossRef]
- Thompson, E.; Rutledge, S.; Dolan, B.; Chandrasekar, V.; Cheong, B. A dual-polarization radar hydrometeor classification algorithm for winter precipitation. J. Atmos. Ocean. Technol. 2014, 31, 1457–1481. [Google Scholar] [CrossRef]
- Wen, G.; Protat, A.; May, P.; Wang, X.; Moran, W. A cluster-based method for hydrometeor classification using polarimetric variables. Part I: Interpretation and analysis. J. Atmos. Ocean. Technol. 2015, 32, 1320–1340. [Google Scholar] [CrossRef]
- Vivekanandan, J.; Ellis, S.; Oye, R.; Zrnić, D.; Ryzhkov, A.; Straka, J. Cloud microphysics retrieval using S-band dual-polarization radar measurements. Bull. Am. Meteorol. Soc. 1999, 80, 381–388. [Google Scholar] [CrossRef]
- Deierling, W. The Relationship between Total Lightning and Ice Fluxes. Ph.D. Thesis, University of Alabama in Huntsville, Huntsville, AL, USA, 2006. [Google Scholar]
- Kumjian, M.R.; Khain, A.P.; Benmoshe, N.; Ilotoviz, E.; Ryzhkov, A.V.; Phillips, V. The anatomy and physics of ZDR columns: Investigating a polarimetric radar signature with a spectral bin microphysical model. J. Appl. Meteorol. Climatol. 2014, 53, 1820–1843. [Google Scholar] [CrossRef]
- Kuster, C.M.; Schuur, T.J.; Lindley, T.T.; Snyder, J.C. Using ZDR Columns in Forecaster Conceptual Models and Warning Decision-Making. Weather Forecast. 2020, 35, 2507–2522. [Google Scholar] [CrossRef]
- Ryzhkov, A.V.; Kumjian, M.R.; Ganson, S.M.; Khain, A.P. Polarimetric radar characteristics of melting hail. Part I: Theoretical simulations using spectral microphysical modeling. J. Appl. Meteorol. Climatol. 2013, 52, 2849–2870. [Google Scholar] [CrossRef]
- Ryzhkov, A.V.; Kumjian, M.R.; Ganson, S.M.; Khain, A.P. Polarimetric radar characteristics of melting hail. Part II: Practical implication. J. Appl. Meteorol. Climatol. 2013, 52, 2871–2886. [Google Scholar] [CrossRef]
- Ortega, K.L.; Krause, J.M.; Ryzhkov, A.V. Polarimetric radar characteristics of melting hail. Part III: Validation of the algorithm for hail size discrimination. J. Appl. Meteorol. Climatol. 2016, 55, 829–848. [Google Scholar] [CrossRef]
- Balakrishnan, N.; Zrnić, D. Use of polarization to characterize precipitation and discriminate large hail. J. Atmos. Sci. 1990, 47, 1525–1540. [Google Scholar] [CrossRef]
- Holler, H.; Bringi, V.; Hubbert, J.; Hagen, M.; Meischner, P. Life cycle and precipitation formation in a hybridtype hailstorm revealed by polarimetric and Doppler radar measurements. J. Atmos. Sci. 1994, 51, 2500–2522. [Google Scholar] [CrossRef]
- Brandes, E.; Ikeda, K. Freezing-level estimation with polarimetric radar. J. Appl. Meteor. 2004, 43, 1541–1553. [Google Scholar] [CrossRef]
- Bluestein, H.; French, M.; Tanamachi, R.; Frasier, S.; Hardwick, K.; Junyent, F.; Pazmany, A. Close-Range observations of tornadoes in supercells made with a dual-polarization, X-band, mobile Doppler radar. Mon. Weather Rev. 2007, 135, 1522–1543. [Google Scholar] [CrossRef]
- Deierling, W.; Petersen, W.; Latham, J.; Ellis, S.; Christian, H. The relationship between lightning activity and ice fluxes in thunderstorms. J. Geophys. Res. 2008, 113, D15210. [Google Scholar] [CrossRef]
- Payne, C.; Schuur, T.; MacGorman, D.; Biggerstaff, M.; Kuhlman, K.; Rust, W. Polarimetric and electrical characteristics of a lightning ring in a supercell storm. Mon. Weather Rev. 2010, 138, 2405–2425. [Google Scholar] [CrossRef]
- Van Den Broeke, M.S.; Jauernic, S.T. Spatial and temporal characteristics of polarimetric tornadic debris signatures. J. Appl. Meteorol. Climatol. 2014, 53, 2217–2231. [Google Scholar] [CrossRef][Green Version]
- Snyder, J.; Ryzhkov, A. Automated Detection of Polarimetric Tornadic Debris Signatures Using a Hydrometeor Classification Algorithm. J. Appl. Meteorol. Climatol. 2015, 54, 1861–1870. Available online: https://journals.ametsoc.org/view/journals/apme/54/9/jamc-d-15-0138.1.xml (accessed on 15 April 2022). [CrossRef]
- Bluestein, H.B.; French, M.M.; Popstefanija, I.; Bluth, R.T.; Knorr, J.B. A mobile, phased-array doppler radar for the study of severe convective storms: The MWR-05XP. Bull. Am. Meteorol. Soc. 2010, 91, 579–600. [Google Scholar] [CrossRef]
- Bluestein, H.B.; Thiem, K.J.; Snyder, J.C.; Houser, J.B. Tornadogenesis and early Tornado evolution in the El Reno, Oklahoma, supercell on 31 May 2013. Mon. Weather. Rev. 2019, 147, 2045–2066. [Google Scholar] [CrossRef]
- Pazmany, A.L.; Mead, J.B.; Bluestein, H.B.; Snyder, J.C.; Houser, J.B. A mobile rapid-scanning X-band polarimetric (RaXPol) Doppler radar system. J. Atmos. Ocean. Technol. 2013, 30, 1398–1413. [Google Scholar] [CrossRef]
- French, M.M.; Bluestein, H.B.; PopStefanija, I.; Baldi, C.A.; Bluth, R.T. Reexamining the vertical development of tornadic vortex signatures in supercells. Mon. Weather Rev. 2013, 141, 4576–4601. [Google Scholar] [CrossRef]
- Griffin, C.B.; Bodine, D.J.; Kurdzo, J.M.; Mahre, A.; Palmer, R.D. High-temporal resolution observations of the 27 May 2015 Canadian, Texas, Tornado using the Atmospheric Imaging Radar. Mon. Weather Rev. 2019, 147, 873–891. [Google Scholar] [CrossRef]
- Houser, J.L.; Bluestein, H.B.; Snyder, J.C. Rapid-scan, polarimetric, doppler radar observations of tornadogenesis and tornado dissipation in a tornadic supercell: The “El Reno, Oklahoma” storm of 24 May 2011. Mon. Weather Rev. 2015, 143, 2685–2710. [Google Scholar] [CrossRef]
- Kosiba, K.A.; Wurman, J. The three-dimensional structure and evolution of a tornado boundary layer. Weather Forecast. 2013, 28, 1552–1561. [Google Scholar] [CrossRef]
- Kuster, C.M.; Snyder, J.C.; Schuur, T.J.; Lindley, T.T.; Heinselman, P.L.; Furtado, J.C.; Brogden, J.W.; Toomey, R. Rapid-update radar observations of ZDR column depth and its use in the warning decision process. Weather Forecast. 2019, 34, 1173–1188. [Google Scholar] [CrossRef]
- Kurdzo, J.M.; Nai, F.; Bodine, D.J.; Bonin, T.A.; Isom, B.; Palmer, R.D.; Cheong, B.L.; Lujan, J.; Mahre, A.; Byrd, A. Observations of severe local storms and tornadoes with the Atmospheric Imaging Radar. Bull. Amer. Meteorol. Soc. 2017, 98, 915–935. [Google Scholar] [CrossRef]
- Snyder, J.C.; Bluestein, H.B. Some considerations for the use of high-resolution mobile radar data in tornado intensity determination. Weather Forecast. 2014, 29, 799–827. [Google Scholar] [CrossRef]
- Witt, A.; Burgess, D.W.; Seimon, A.; Allen, J.T.; Snyder, J.C.; Bluestein, H.B. Rapid-scan radar observations of an Oklahoma tornadic hailstorm producing giant hail. Weather Forecast. 2018, 33, 1263–1282. [Google Scholar] [CrossRef]
- Wurman, J.; Kosiba, K.; Robinson, P.; Marshall, T. The role of multiple-vortex tornado structure in causing storm researcher fatalities. Bull. Amer. Meteorol. Soc. 2014, 95, 31–45. [Google Scholar] [CrossRef]
- Ryzhkov, A.V.; Snyder, J.; Carlin, J.T.; Khain, A.; Pinsky, M. What polarimetric weather radars offer to cloud modelers: Forward radar operators and microphysical/thermodynamic retrievals. Atmosphere 2020, 11, 362. [Google Scholar] [CrossRef]
- Pfeifer, M.; Craig, G.; Hagen, M.; Keil, C. A polarimetric radar forward operator for model evaluation. J. Appl. Meteor. Climatol. 2008, 47, 3202–3220. [Google Scholar] [CrossRef]
- Ryzhkov, A.; Pinsky, M.; Pokrovsky, A.; Khain, A. Polarimetric Radar Observation Operator for a Cloud Model with Spectral Microphysics. J. Appl. Meteor. Climatol. 2011, 50, 873–894. [Google Scholar] [CrossRef]
- Jung, Y.; Xue, M.; Zhang, G.; Straka, J. Assimilation of simulated polarimetric radar data for a convective storm using the ensemble Kalman filter. Part I: Observation operators for reflectivity and polarimetric variables. Mon. Wea. Rev. 2008, 136, 2228–2245. [Google Scholar] [CrossRef]
- Pincus, R.; Platnick, S.; Ackerman, S.A.; Hemler, R.S.; Hofmann, R.J.P. Reconciling Simulated and Observed Views of Clouds: MODIS, ISCCP, and the Limits of Instrument Simulators. J. Clim. 2012, 25, 4699–4720. [Google Scholar] [CrossRef]
- Botygina, N.N.; Kovadlo, P.G.; Kopylov, E.A.; Lukin, V.P.; Tuev, M.V.; Shikhovtsev, A.Y. Estimation of the astronomical seeing at the large solar vacuum telescope site from optical and meteorological measurements. Atmos. Ocean. Opt. 2014, 27, 142–146. [Google Scholar] [CrossRef]
- Kovadlo, P.G.; Shikhovtsev, A.Y.U.; Kopylov, E.A.; Kiselev, A.V.; Russkikh, I.V. Study of the Optical Atmospheric Distortions using Wavefront Sensor Data. Russ. Phys. J. 2021, 63, 1952–1958. [Google Scholar] [CrossRef]
- Avila, R.; Carrasco, E.; Ibañez, F.; Vernin, J.; Prieur, J.-L.; Cruz, D.X. Generalized SCIDAR Measurements at San Pedro Mártir. II. Wind Profile Statistics. Publ. Astron. Soc. Pac. 2006, 118, 503–515. [Google Scholar] [CrossRef]
- Voyez, J.; Robert, C.; Conan, J.-M.; Mugnier, L.M.; Samain, E.; Ziad, A. First on-sky results of the CO-SLIDAR Cn2 profiler. Opt. Express 2014, 22, 10948–10967. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Snyder, J.C.; Ryzhkov, A.V.; Kumjian, M.R.; Khain, A.P.; Picca, J. A ZDR column detection algorithm to examine convective storm updrafts. Weather Forecast. 2015, 30, 1819–1844. [Google Scholar] [CrossRef]
- Ilotoviz, E.; Khain, A.; Ryzhkov, A.V.; Snyder, J.C. Relationship between aerosols, hail microphysics, and ZDR columns. J. Atmos. Sci. 2018, 75, 1755–1781. [Google Scholar] [CrossRef]
- Shpund, J.; Khain, A.; Lynn, B.; Fan, J.; Han, B.; Ryzhkov, A.; Snyder, J.; Dudhia, J.; Gill, D. Simulating a mesoscale convective system using WRF with a new spectral bin microphysics: 1: Hail vs graupel. J. Geophys. Res. Atmos. 2019, 124, 14072–14101. [Google Scholar] [CrossRef]
- Jung, Y.; Xue, M.; Zhang, G.; Straka, J. Simulations of polarimetric radar signatures of a supercell storm using a two-moment bulk microphysics scheme. J. Appl. Meteor. Climatol. 2010, 49, 146–163. [Google Scholar] [CrossRef]
- Jung, Y.; Xue, M.; Zhang, G.; Straka, J.M. Assimilation of Simulated Polarimetric Radar Data for a Convective Storm Using the Ensemble Kalman Filter. Part II: Impact of Polarimetric Data on Storm Analysis. Mon. Weather Rev. 2008, 136, 2246–2260. Available online: https://journals.ametsoc.org/view/journals/mwre/136/6/2007mwr2288.1.xml (accessed on 15 April 2022). [CrossRef]
- Snook, N.; Xue, M.; Jung, Y. Analysis of a Tornadic Mesoscale Convective Vortex Based on Ensemble Kalman Filter Assimilation of CASA X-Band and WSR-88D Radar Data. Mon. Weather Rev. 2011, 139, 3446–3468. [Google Scholar] [CrossRef]
- Jung, Y.; Xue, M.; Tong, M. Ensemble Kalman Filter Analyses of the 29–30 May 2004 Oklahoma Tornadic Thunderstorm Using One- and Two-Moment Bulk Microphysics Schemes, with Verification against Polarimetric Radar Data. Mon. Weather Rev. 2012, 140, 1457–1475. Available online: https://journals.ametsoc.org/view/journals/mwre/140/5/mwr-d-11-00032.1.xml (accessed on 15 April 2022). [CrossRef]
- Putnam, B.; Xue, M.; Jung, Y.; Snook, N.; Zhang, G. Ensemble Kalman Filter Assimilation of Polarimetric Radar Observations for the 20 May 2013 Oklahoma Tornadic Supercell Case. Mon. Weather Rev. 2019, 147, 2511–2533. Available online: https://journals.ametsoc.org/view/journals/mwre/147/7/mwr-d-18-0251.1.xml (accessed on 15 April 2022). [CrossRef]
- Snyder, J.; Bluestein, H.; Dawson, D., II; Jung, Y. Simulations of Polarimetric, X-Band Radar Signatures in Supercells. Part I: Description of Experiment and Simulated ρhv Rings. J. Appl. Meteor. Climatol. 2017, 56, 1977–1999. [Google Scholar] [CrossRef]
- Snyder, J.; Bluestein, H.; Dawson, D., II; Jung, Y. Simulations of Polarimetric, X-Band Radar Signatures in Supercells. Part II: ZDR Columns and Rings and KDP Columns. J. Appl. Meteor. Climatol. 2017, 56, 2001–2026. [Google Scholar] [CrossRef]
- Oue, M.; Tatarevic, A.; Kollias, P.; Wang, D.; Yu, K.; Vogelmann, A. The Cloud-resolving model Radar SIMulator (CR-SIM) Version 3.3: Descriptin and applications of a virtual observatory. Geosci. Model Dev. 2020, 13, 1975–1998. [Google Scholar] [CrossRef]
- Skamarock, W.; Klemp, J.; Dudhia, J.; Gill, D.; Barker, D.; Duda, M.; Huang, X.-Y.; Wang, W.; Powers, J.A. Description of the Advanced Research WRF Version 3; NCAR Tech. Note, NCAR/TN-4751STR; University Corporation for Atmospheric Research: Boulder, CO, USA, 2008; p. 113. [Google Scholar] [CrossRef]
- Davis, C.; Wang, W.; Chen, S.S.; Chen, Y.; Corbosiero, K.; DeMaria, M.; Dudhia, J.; Holland, G.; Klemp, J.; Michalakes, J.; et al. Prediction of Landfalling Hurricanes with the Advanced Hurricane WRF Model. Mon. Weather Rev. 2008, 136, 1990–2005. [Google Scholar] [CrossRef]
- Karan, H.; Fitzpatrick, P.; Hill, C.; Li, Y.; Xiao, Q.; Lim, E. The Formation of Multiple Squall Lines and the Impacts of WSR-88D Radial Winds in a WRF Simulation. Weather Forecast. 2010, 25, 242–262. [Google Scholar] [CrossRef][Green Version]
- Gray, K.; Frame, J. Investigating the Transition from Elevated Multicellular Convection to Surface-Based upercells during the Tornado Outbreak of 24 August 2016 Using a WRF Model Simulation. Weather Forecast. 2019, 34, 1051–1079. [Google Scholar] [CrossRef]
- Zhang, F.; Li, M.; Ross, A.; Lee, S.; Zhang, D. Sensitivity Analysis of Hurricane Arthur (2014) Storm Surge Forecasts to WRF Physics Parameterizations and Model Configurations. Weather Forecast. 2017, 32, 1745–1764. [Google Scholar] [CrossRef]
- Bao, J.-W.; Michelson, S.; Grell, E. Microphysical Process Comparison of Three Microphysics Parameterization Schemes in the WRF Model for an Idealized Squall-Line Case Study. Mon. Weather Rev. 2019, 147, 3093–3120. [Google Scholar] [CrossRef]
- McCaul, E., Jr.; Priftis, G.; Case, J.; Chronis, T.; Gatlin, P.; Goodman, S.; Kong, F. Sensitivities of the WRF Lightning Forecasting Algorithm to Parameterized Microphysics and Boundary Layer Schemes. Weather Forecast. 2020, 35, 1545–1560. [Google Scholar] [CrossRef]
- Sunny Lim, K.; Chang, E.; Sun, R.; Kim, K.; Tapiador, F.J.; Lee, G. Evaluation of Simulated Winter Precipitation Using WRF-ARW during the ICE-POP 2018 Field Campaign. Weather Forecast. 2020, 35, 2199–2213. [Google Scholar] [CrossRef]
- Morrison, H.; Thompson, G.; Tatarskii, V. Impact of cloud micrpohysics on the development of trailing stratiform precipitation in a simulated squall line: Comparison of one- and two-moment schemes. Mon. Weather Rev. 2009, 137, 991–1007. [Google Scholar] [CrossRef]
- Milbrandt, J.; Yau, M. A multimoment bulk microphysics parameterization. Part I: Analysis of the role of the spectral shape parameter. J. Atmos. Sci. 2005, 62, 3051–3064. [Google Scholar] [CrossRef]
- Milbrandt, J.; Yau, M. A multimoment bulk microphysics parameterization. Part II: A proposed three–moment closure and scheme description. J. Atmos. Sci. 2005, 62, 3065–3081. [Google Scholar] [CrossRef]
- Thompson, G.; Field, P.R.; Rasmussen, R.M.; Hall, W.D. Explicit forecasts of winter precipitation using an improved bulk microphysics scheme. Part II: Implementation of a new snow parameterization. Mon. Weather Rev. 2008, 136, 5095–5155. [Google Scholar] [CrossRef]
- Mishchenko, M.I. Calculation of the amplitude matrix for a nonspherical particle in a fixed orientation. Appl. Opt. 2000, 39, 1026–1031. [Google Scholar] [CrossRef]
- Mlawer, E.J.; Taubman, S.J.; Brown, P.D.; Iacono, M.J.; Clough, S.A. Radiative transfer for inhomogeneous atmosphere: RRTM, a validated correlated-k model for the longwave. J. Geophys. Res. 1997, 102, 16663–16682. [Google Scholar] [CrossRef]
- Dudhia, J. Numerical study of convection observed during the winter monsoon experiment using a mesoscale two-dimensional model. J. Atmos. Sci. 1989, 46, 3077–3107. [Google Scholar] [CrossRef]
- Janjic, Z.I. The step-mountain eta coordinate model: Further developments of the convection, viscous sublayer and turbulence closure schemes. Mon. Weather Rev. 1994, 122, 927–945. [Google Scholar] [CrossRef]
- Chen, F.; Dudhia, J. Coupling an advanced land surface–hydrology model with the Penn State–NCAR MM5 modeling system. Part I: Model implementation and sensitivity. Mon. Weather Rev. 2001, 129, 569–585. [Google Scholar] [CrossRef]
- Helmus, J.J.; Collis, S.M. The Python ARM Radar Toolkit (Py-ART), a Library for Working with Weather Radar Data in the Python Programming Language. J. Open Res. Softw. 2016, 4, e25. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, X.; Mecikalski, J.R.; Otkin, J.A.; Henderson, D.S.; Srikishen, J. A Polarimetric Radar Operator and Application for Convective Storm Simulation. Atmosphere 2022, 13, 645. https://doi.org/10.3390/atmos13050645
Li X, Mecikalski JR, Otkin JA, Henderson DS, Srikishen J. A Polarimetric Radar Operator and Application for Convective Storm Simulation. Atmosphere. 2022; 13(5):645. https://doi.org/10.3390/atmos13050645
Chicago/Turabian StyleLi, Xuanli, John R. Mecikalski, Jason A. Otkin, David S. Henderson, and Jayanthi Srikishen. 2022. "A Polarimetric Radar Operator and Application for Convective Storm Simulation" Atmosphere 13, no. 5: 645. https://doi.org/10.3390/atmos13050645
APA StyleLi, X., Mecikalski, J. R., Otkin, J. A., Henderson, D. S., & Srikishen, J. (2022). A Polarimetric Radar Operator and Application for Convective Storm Simulation. Atmosphere, 13(5), 645. https://doi.org/10.3390/atmos13050645





