The Zonal Wind Intraseasonal Oscillation in the Exit Region of the East Asian Subtropical Westerly Jet in Winter and Its Thermodynamic Mechanism
Abstract
1. Introduction
2. Data and Methods
2.1. Data
2.2. Methods
3. Results
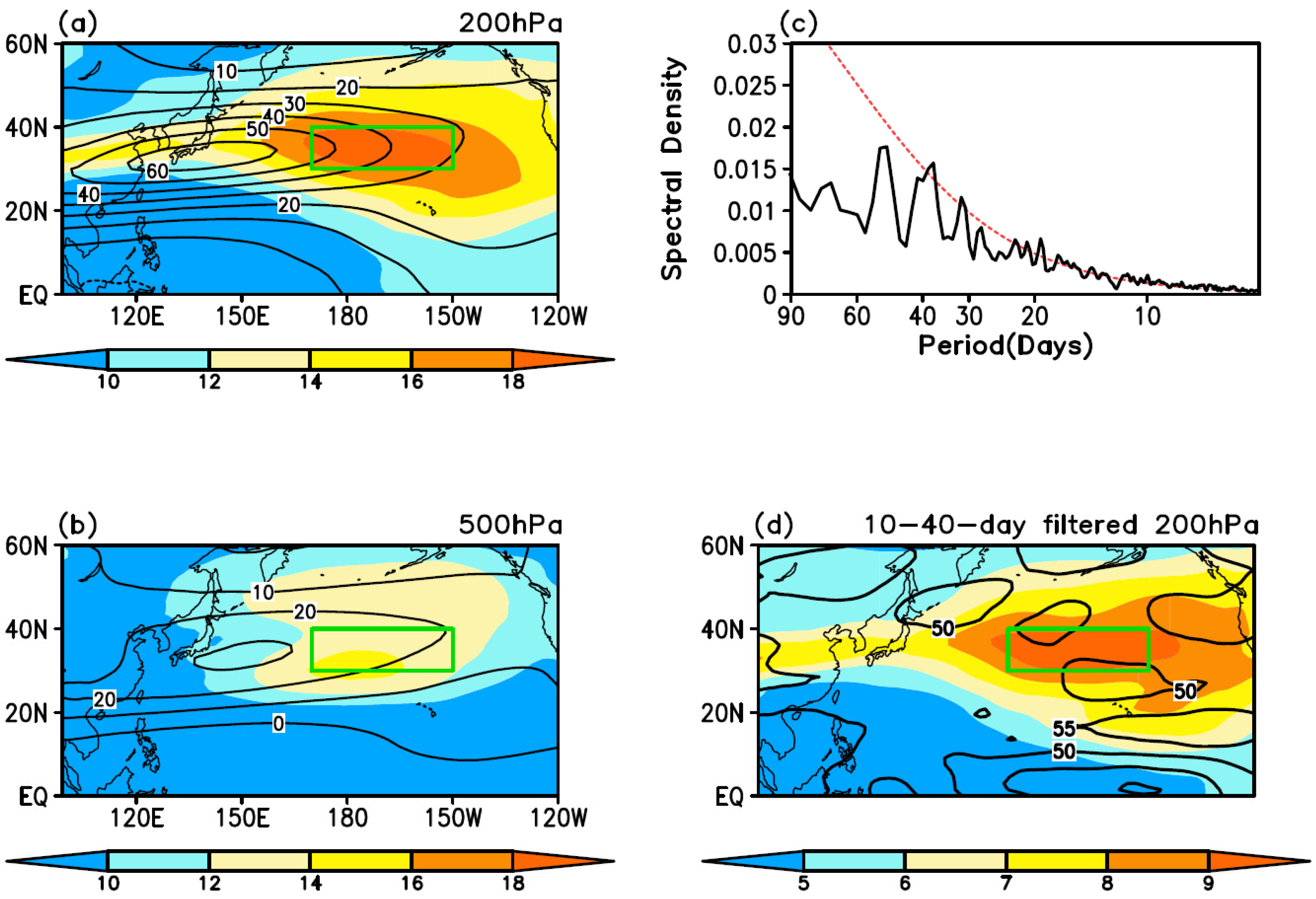
3.1. The Characteristics of the Zonal Wind ISO at the Jet Exit
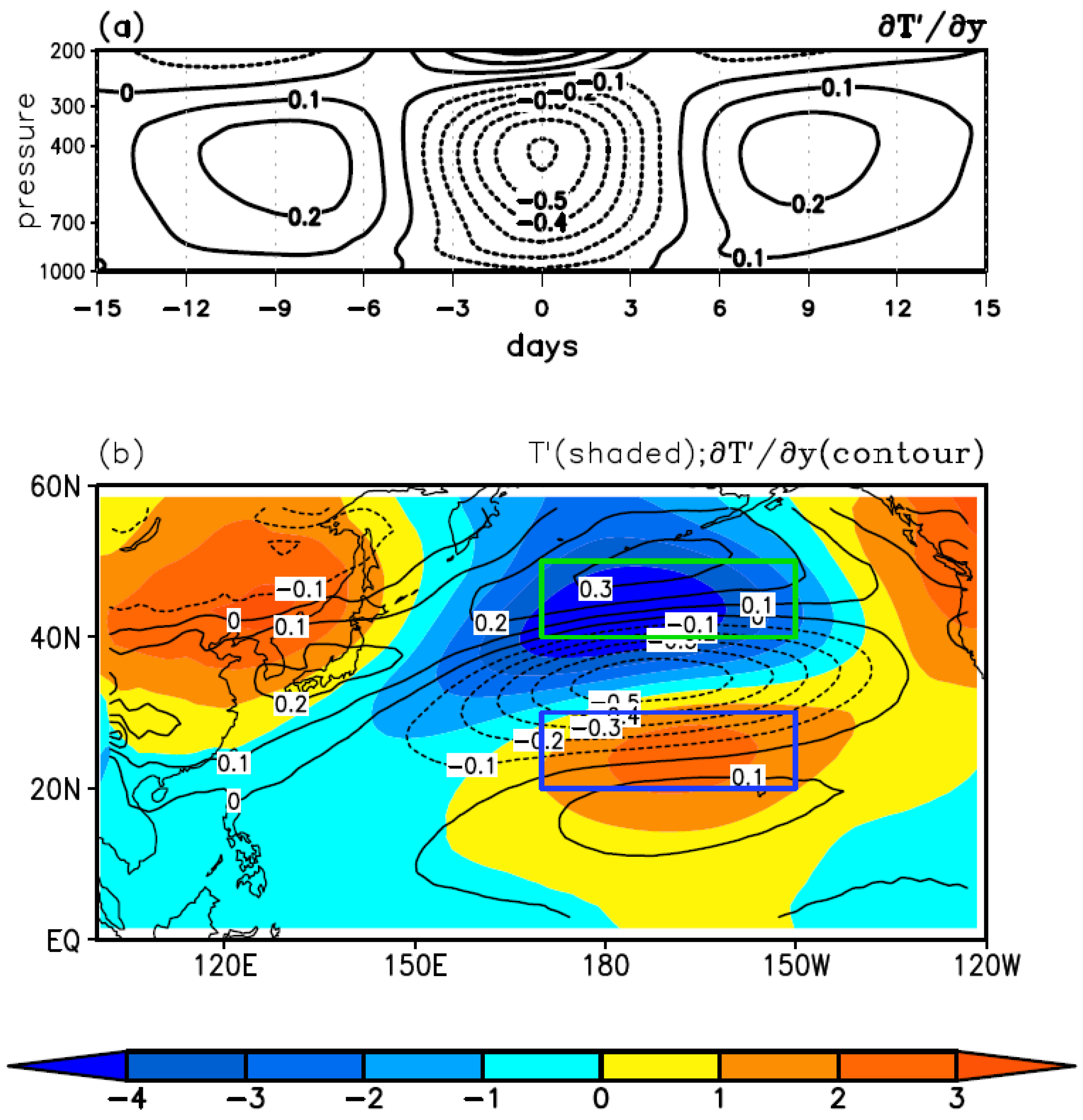
3.2. Zonal Wind ISO and Intraseasonal Temperature Variation
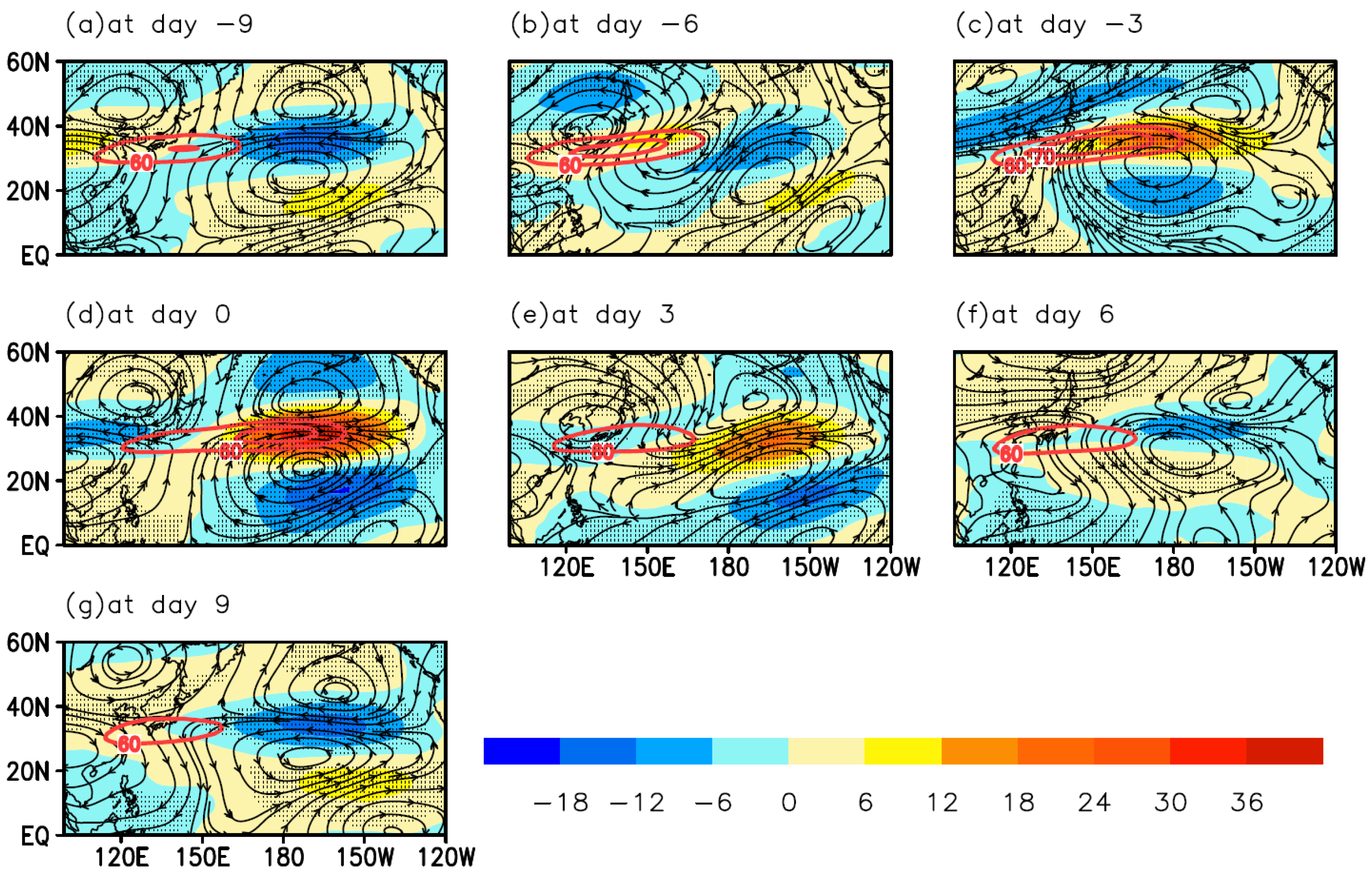
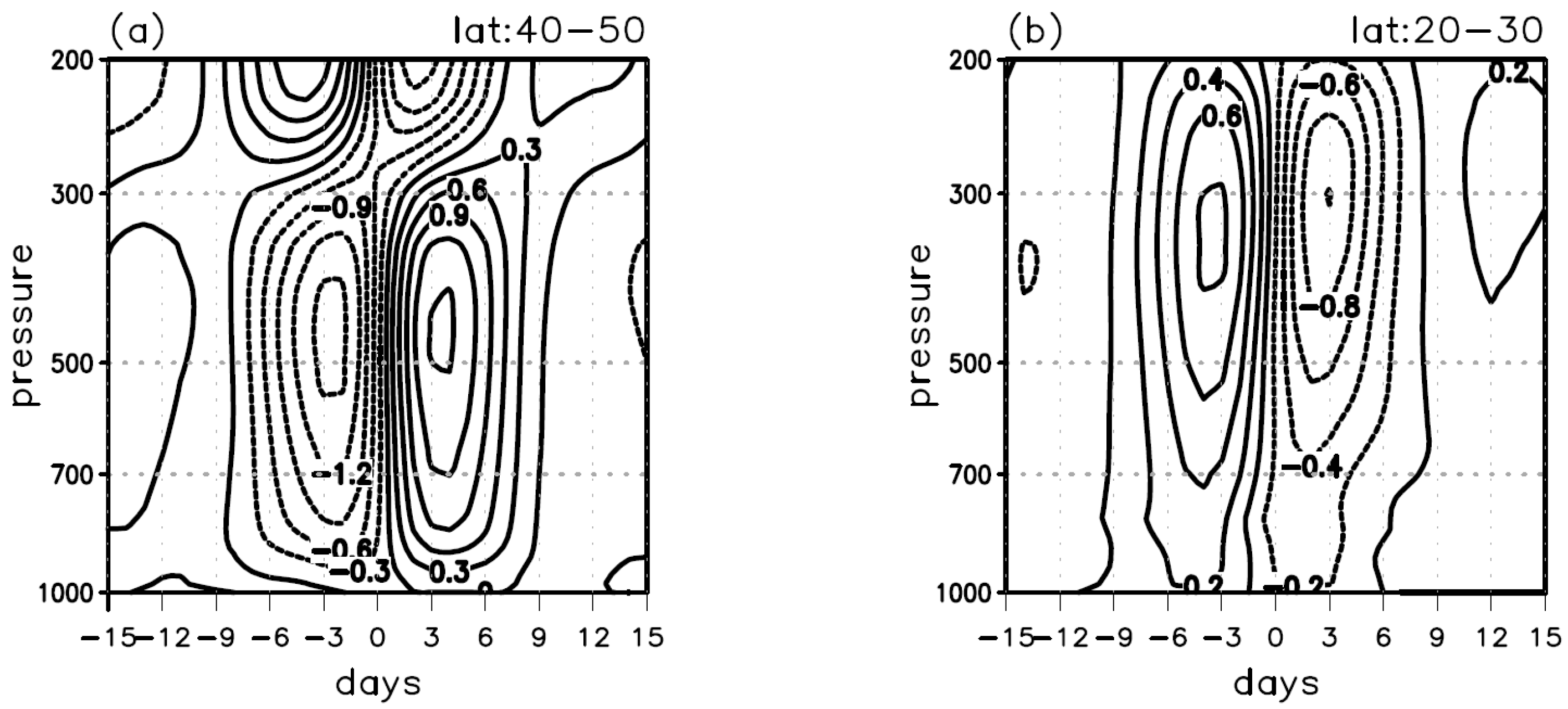
3.3. The Intraseasonal Meridional Circulation near the Jet Exit
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Madden, R.; Julian, P. Detection of a 40–50 day oscillation in the zonal wind in the tropical Pacific. J. Atmos. Sci. 1971, 28, 702–708. [Google Scholar] [CrossRef]
- Madden, R.; Julian, P. Description of global scale circulation cells in the tropics with 40–50 day period. J. Atmos. Sci. 1972, 29, 1109–1123. [Google Scholar] [CrossRef]
- Li, C.; Wu, P. An observational study of the 30–50 day atmospheric oscillations. Part I: Structure and propagation. Adv. Atmos. Sci. 1990, 7, 294–304. [Google Scholar] [CrossRef]
- Jiang, X.; Li, T.; Wang, B. Structures and Mechanisms of the Northward Propagating Boreal Summer Intraseasonal Oscillation. J. Clim. 2004, 17, 1022–1039. [Google Scholar] [CrossRef]
- Hsu, P.; Li, T.; Murakami, H. Moisture asymmetry and MJO eastward propagation in an aqua-planet general circulation model. J. Clim. 2014, 27, 8747–8760. [Google Scholar] [CrossRef]
- Feng, J.; Li, T.; Zhu, W. Propagating and Non-Propagating MJO Events over Maritime Continent. J. Clim. 2015, 28, 8430–8449. [Google Scholar] [CrossRef]
- Kuang, X.; Zhang, Y. Seasonal Variation of the East Asian Subtropical Westerly Jet and Its Association with the Heating Field over East Asia. Adv. Atmos. Sci. 2005, 22, 831–840. [Google Scholar]
- Jhun, J.; Lee, E. A new East Asian winter monsoon index and associated characteristics of the winter monsoon. J. Clim. 2004, 17, 711–726. [Google Scholar] [CrossRef]
- Ren, X.; Yang, X.; Zhou, T.; Fang, J. Diagnostic Comparison of Wintertime East Asian Subtropical Jet and Polar-front Jet: Large-scale Characteristics and Transient Eddy Activities. Acta Meteorol. Sin. 2011, 25, 21–23. [Google Scholar] [CrossRef]
- Wang, N.; Zhang, Y. Evolution of Eurasian teleconnection pattern and its relationship to climate anomalies in china. Clim. Dyn. 2014, 44, 1017–1028. [Google Scholar] [CrossRef]
- Kuang, X.; Zhang, Y.; Huang, Y.; Huang, D. Spatial differences in seasonal variation of the upper-tropospheric jet stream in the Northern Hemisphere and its thermal dynamic mechanism. Theor. Appl. Climatol. 2014, 17, 103–112. [Google Scholar] [CrossRef]
- Charney, J.; Eliassen, A. A numerical method for prediction the perturbation of the middle latitude westerlies. Tellus 1949, 1, 38–55. [Google Scholar] [CrossRef]
- Shi, Z.; Liu, X.; Liu, Y.; Sha, Y.; Xu, T. Impact of mongolian plateau versus tibetan plateau on the westerly jet over north pacific ocean. Clim. Dyn. 2015, 44, 3067–3076. [Google Scholar] [CrossRef]
- Krishnamurti, T. The subtropical jet stream of winter. J. Atmos. Sci. 1961, 18, 172–191. [Google Scholar] [CrossRef]
- Yang, S.; Webster, P. The effect of summer tropical heating on the location and intensity of the extratropical westerly jet streams. J. Geophys. Res. Atmos. 1990, 95, 18705–18721. [Google Scholar] [CrossRef]
- Bjerknes, J. A possible response of the atmospheric Hadley circulation to equatorial anomalies of ocean temperature. Tellus 1966, 18, 820–829. [Google Scholar] [CrossRef]
- Kuo, H. The motion of atmospheric vortices and the general circulation. J. Atmos. Sci. 1950, 7, 247–258. [Google Scholar] [CrossRef][Green Version]
- Ren, X.; Yang, X.; Chu, C. Seasonal variations of the synoptic-scale transient eddy activity and polar front jet over East Asia. J. Clim. 2010, 23, 3222–3233. [Google Scholar] [CrossRef]
- Xiang, Y.; Yang, X. The effect of transient eddy on interannual meridional displacement of summer East Asian Subtropical Jet. Adv. Atmos. Sci. 2012, 29, 484–492. [Google Scholar] [CrossRef]
- Lee, S.; Kim, H. The dynamical relationship between subtropical and eddy-driven jets. J. Atmos. Sci. 2003, 60, 1490–1530. [Google Scholar] [CrossRef]
- Lu, R.; Lin, Z. Role of subtropical precipitation anomalies in maintaining the summertime meridional teleconnection over the Western North Pacific and East Asia. J. Clim. 2009, 22, 2058–2072. [Google Scholar] [CrossRef]
- Guo, Y.; Wen, Z.; Wu, R.; Lu, R.; Chen, Z. Impact of tropical pacific precipitation anomaly on the East Asian upper-tropospheric westerly jet during the boreal winter. J. Clim. 2015, 28, 6457–6474. [Google Scholar] [CrossRef]
- Xue, D.; Zhang, Y. Concurrent variations in the location and intensity of the asian winter jet streams and the possible mechanism. Clim. Dyn. 2017, 49, 37–52. [Google Scholar] [CrossRef]
- Yu, X.; Zhang, L.; Zhou, T. The Asian Subtropical Westerly Jet Stream in CRA-40, ERA5, and CFSR Reanalysis Data: Comparative Assessment. J. Meteorol. Res. 2021, 35, 46–63. [Google Scholar] [CrossRef]
- Jin, R.; Li, W.; Zhang, B.; Yan, C. A study of the relationship between East Asia subtropical westerly jet and abnormal Meiyu in the middle-lower reaches of the Yangtze river. Chin. J. Atmos. Sci. 2012, 36, 722–732. [Google Scholar]
- Yao, C.; Huang, Q.; Zhu, B.; Liu, F. The 10–30-day oscillation of winter zonal wind in the entrance region of the East Asian subtropical jet and its relationship with precipitation in southern China. Dyn. Atmos. Ocean. 2018, 82, 76–88. [Google Scholar] [CrossRef]
- Han, R.; Li, W.; Dong, M. Temporal and Spatial Characteristics of Intraseasonal Oscillations in the Meridional Wind Field over the Subtropical Northern Pacific. Acta Meteorol. Sin. 2010, 24, 276–286. [Google Scholar]
- Barroso, J.; Zuritagotor, P. Intraseasonal Variability of the Zonal-Mean Extratropical Tropopause: The Role of Changes in Polar Vortex Strength and Upper-Troposphere Wave Breaking. J. Atmos. Sci. 2016, 73, 1383–1399. [Google Scholar] [CrossRef]
- Yao, S.; Huang, Q.; Li, T.; Zhang, C. The intraseasonal oscillations of precipitation and circulations from January to March in 2010 in East Asia. Meteorol. Atmos. Phys. 2014, 123, 67–79. [Google Scholar] [CrossRef]
- Yao, S.; Gong, K.; Zhao, C. Intraseasonal oscillation of the winter geopotential height in the middle latitude of the Northern Hemisphere. J. Meteorol. Sci. 2016, 36, 622–628. [Google Scholar]
- Dee, D.; Uppala, S.; Simmons, A.; Berrisford, P.; Poli, P.; Kobayashi, S.; Andrae, U.; Balmaseda, M.; Balsamo, G.; Bauer, P.; et al. The ERA-Interim reanalysis: Configuration and performance of the data assimilation system. Q. J. R. Meteorol. Soc. 2011, 137, 553–597. [Google Scholar] [CrossRef]
- Holton, J. An Introduction to Dynamic Meteorology; Academic Press: London, UK, 1992. [Google Scholar]
- Hsu, P.; Li, T. Interactions between boreal summer Intraseasonal oscillations and synoptic-scale disturbances over the western North Pacific. Part II: Apparent heat and moisture sources and eddy momentum transport. J. Clim. 2011, 24, 942–961. [Google Scholar] [CrossRef]


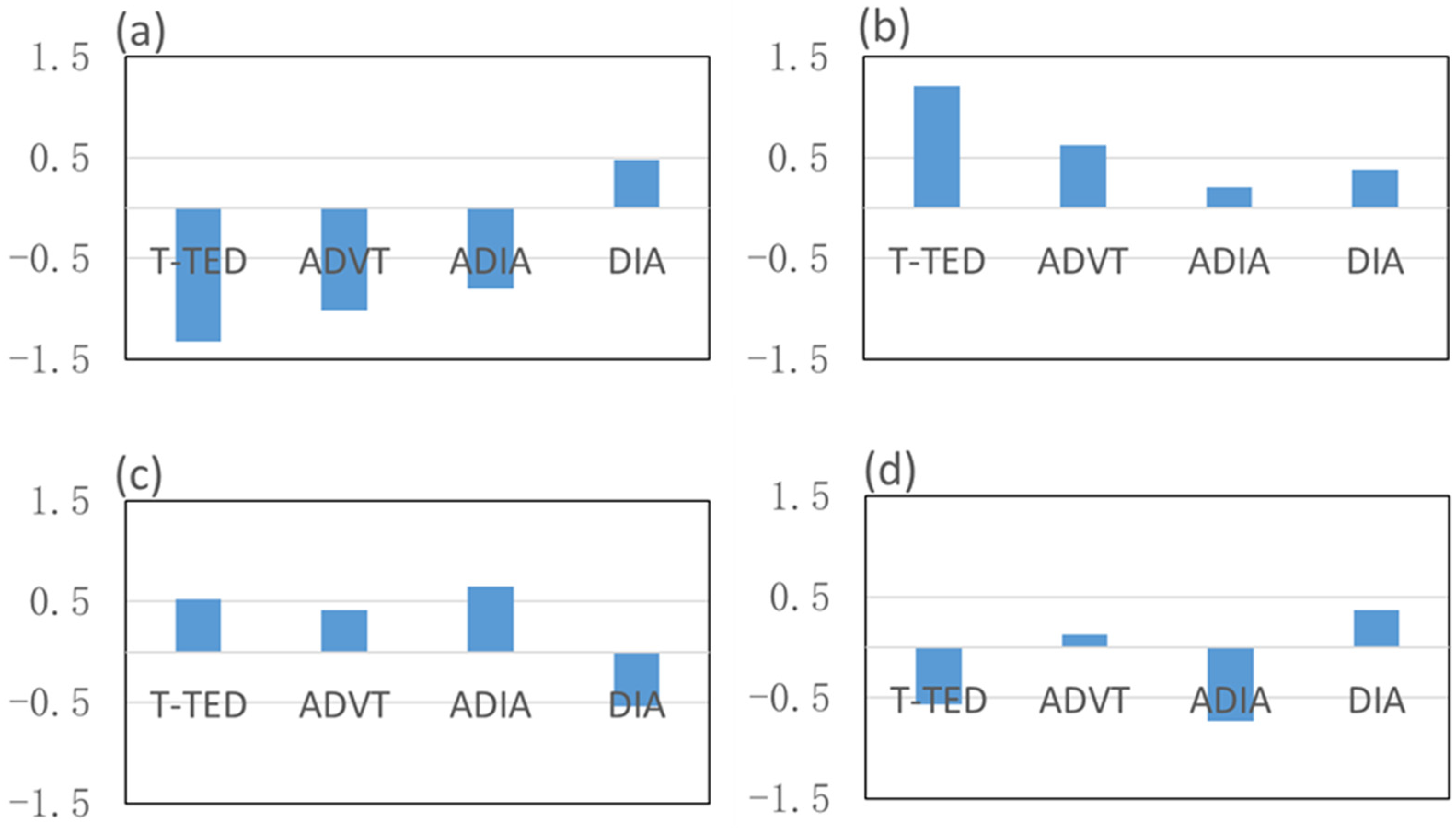

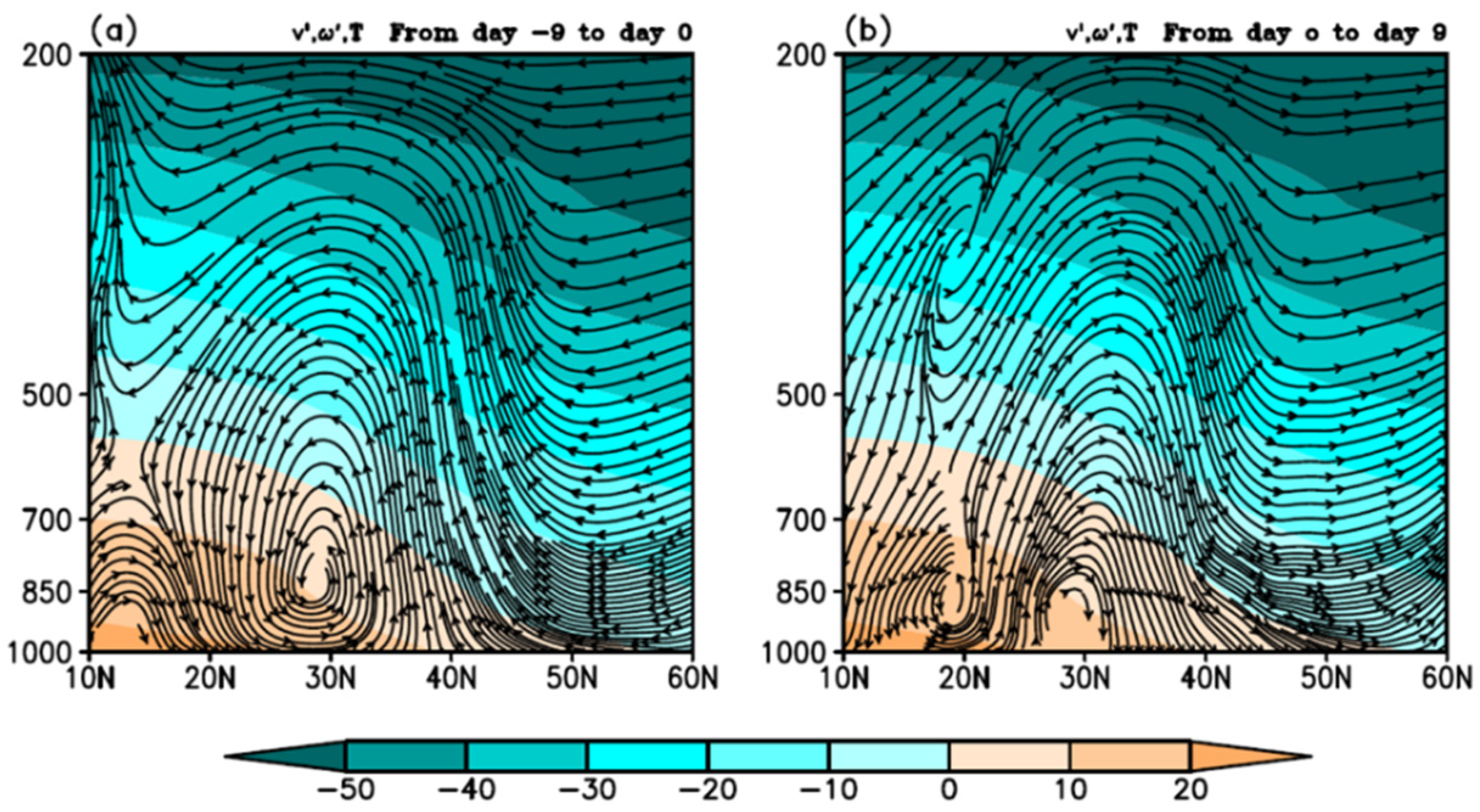
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yao, S.; Liu, Y. The Zonal Wind Intraseasonal Oscillation in the Exit Region of the East Asian Subtropical Westerly Jet in Winter and Its Thermodynamic Mechanism. Atmosphere 2022, 13, 395. https://doi.org/10.3390/atmos13030395
Yao S, Liu Y. The Zonal Wind Intraseasonal Oscillation in the Exit Region of the East Asian Subtropical Westerly Jet in Winter and Its Thermodynamic Mechanism. Atmosphere. 2022; 13(3):395. https://doi.org/10.3390/atmos13030395
Chicago/Turabian StyleYao, Suxiang, and Yishan Liu. 2022. "The Zonal Wind Intraseasonal Oscillation in the Exit Region of the East Asian Subtropical Westerly Jet in Winter and Its Thermodynamic Mechanism" Atmosphere 13, no. 3: 395. https://doi.org/10.3390/atmos13030395
APA StyleYao, S., & Liu, Y. (2022). The Zonal Wind Intraseasonal Oscillation in the Exit Region of the East Asian Subtropical Westerly Jet in Winter and Its Thermodynamic Mechanism. Atmosphere, 13(3), 395. https://doi.org/10.3390/atmos13030395





