A Possible Reconciliation between Eddy Covariance Fluxes and Surface Energy Balance Closure
Abstract
1. Introduction
2. Material and Methods
EC Fluxes
3. Results
3.1. Additional Terms in the SEB Equation
3.1.1. Additional Term Related to the Latent Heat Flux
3.1.2. Additional Term Related to the Sensible Heat Flux
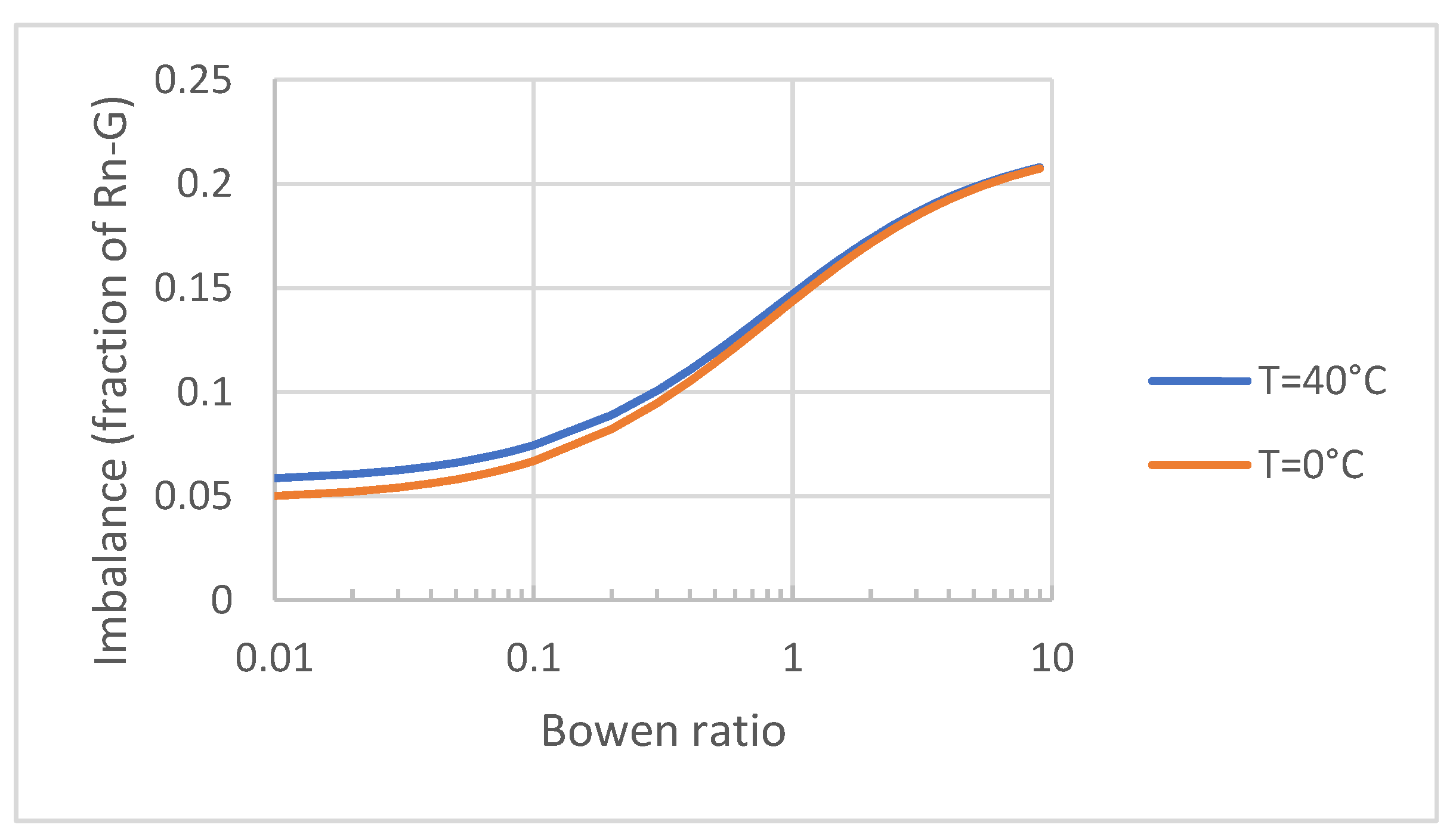
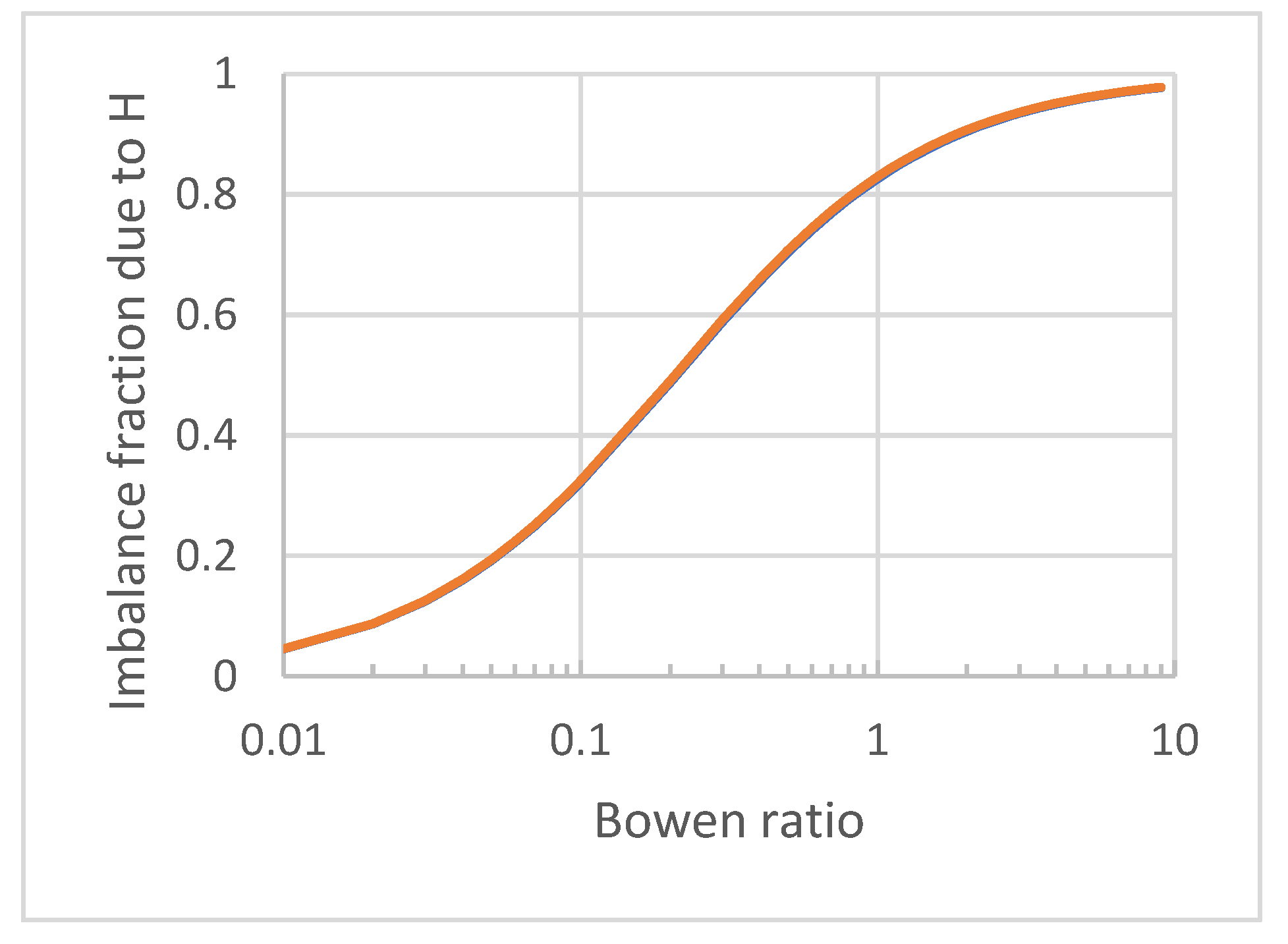
3.2. The Modified SEB Equation
3.3. Application to Observation Datasets
4. Discussion and Conclusions
Funding
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Cuxart, J.; Conangla, L.; Jiménez, M.A. Evaluation of the surface energy budget equation with experimental data and the ECMWF model in the Ebro Valley. J. Geophys. Res. Atmos. 2015, 120, 1008–1022. [Google Scholar] [CrossRef]
- Mauder, M.; Foken, T.; Cuxart, J. Surface-energy-balance closure over land: A review. Bound.-Layer Meteorol. 2020, 177, 395–426. [Google Scholar] [CrossRef]
- Leuning, R.; Denmead, O.T.; Lang, A.R.G.; Ohtaki, E. Effects of heat and water vapor transport on eddy covariance measurement of CO2 fluxes. Bound.-Layer Meteorol 1982, 23, 209–222. [Google Scholar] [CrossRef]
- Desjardins, R.L. Carbon dioxide budget of maize. Agric. For. Meteorol. 1985, 36, 29–41. [Google Scholar] [CrossRef]
- Kanemasu, E.T.; Verma, S.B.; Smith, E.A.; Fritschen, L.J.; Wesely, M.; Field, R.T.; Kustas, W.P.; Weaver, H.; Stewart, J.B.; Gurney, R.; et al. Surface flux measurements in FIFE: An overview. J. Geophys. Res. 1992, 97, 18547–18555. [Google Scholar] [CrossRef]
- Panin, G.N.; Raabe, A.; Tetzlaff, G. Inhomogeneity of the land surface and problems in parametrization of surface fluxes in natural conditions. Theor. Appl. Climatol. 1998, 60, 163–178. [Google Scholar] [CrossRef]
- Barr, A.; King, K.M.; Gillespie, T.J.; Hartog, G.D.; Neumann, H.H. A comparison of Bowen ratio and eddy correlation sensible and latent heat flux measurements above deciduous forest. Bound.-Layer Meteorol. 1994, 71, 21–41. [Google Scholar] [CrossRef]
- Jacobs, A.F.G.; Heusinkveld, B.G.; Holtslag, A.A.M. Towards closing the surface energy budget of a mid-latitude grassland. Bound.-Layer Meteorol. 2008, 126, 125–136. [Google Scholar] [CrossRef]
- Leuning, R.; van Gorsel, E.; Massman, W.J.; Isaac, P.R. Reflections on the surface energy imbalance problem. Agric. For. Meteorol. 2012, 156, 65–74. [Google Scholar] [CrossRef]
- Foken, T. The energy balance closure problem: An overview. Ecol. Appl. 2008, 18, 1351–1367. [Google Scholar] [CrossRef]
- Foken, T.; Aubinet, M.; Finnigan, J.J.; Leclerc, M.Y.; Mauder, M.; Paw, U.K. Results of a panel discussion about the energy balance closure correction for trace gases. Bull. Am. Meteorol. Soc. 2011, 92, ES13–ES18. [Google Scholar] [CrossRef]
- Oncley, S.P.; Foken, T.; Vogt, R.; Kohsiek, W.; DeBruin, H.A.; Bernhofer, C.; Christen, A.; Gorsel, E.V.; Grantz, D.; Feigenwinter, C.; et al. The energy balance experiment EBEX-2000. Part I: Overview and energy balance. Bound.-Layer Meteorol. 2007, 123, 1–28. [Google Scholar] [CrossRef]
- Wilson, K.; Goldstein, A.; Falge, E.; Aubinet, M.; Baldocchi, D.; Berbigier, P.; Bernhofer, C.; Ceulemans, R.; Dolman, H.; Field, C.; et al. Energy balance closure at FLUXNET sites. Agric. For. Meteorol. 2002, 113, 223–243. [Google Scholar] [CrossRef]
- Franssen, H.H.; Stöckli, R.; Lehner, I.; Rotenberg, E.; Seneviratne, S.I. Energy balance closure of eddy-covariance data: A multisite analysis for European FLUXNET stations. Agric. For. Meteorol. 2010, 150, 1553–1567. [Google Scholar] [CrossRef]
- Stoy, P.C.; Mauder, M.; Foken, T.; Marcolla, B.; Boegh, E.; Ibrom, A.; Arain, M.A.; Arneth, A.; Aurela, M.; Bernhofer, C.; et al. A data-driven analysis of energy balance closure across FLUXNET research sites: The role of landscape-scale heterogeneity. Agric. For. Meteorol. 2013, 171–172, 137–152. [Google Scholar] [CrossRef]
- Gao, Z.; Liu, H.; Chen, X.; Huang, M.; Missik, J.E.C.; Yao, J.; Arntzen, E.; Mcfarland, D.P. Enlarged nonclosure of surface energy balance with increasing atmospheric instabilities linked to changes in coherent structures. J. Geophys. Res. Atmos. 2020, 125, e2020JD032889. [Google Scholar] [CrossRef]
- Li, P.; Wang, Z.-H. A nonequilibrium thermodynamic approach for surface energy balance closure. Geophys. Res. Lett. 2020, 47, e2019GL085835. [Google Scholar] [CrossRef]
- Liu, H.; Gao, Z.; Katul, G.G. Non-closure of surface energy balance linked to asymmetric turbulent transport of scalars by large eddies. J. Geophys. Res. Atmos. 2021, 126, e2020JD034474. [Google Scholar] [CrossRef]
- Sun, J.; Massman, W.J.; Banta, R.M.; Burns, S.P. Revisiting the surface energy imbalance. J. Geophys. Res. Atmos. 2021, 126, e2020JD034219. [Google Scholar] [CrossRef]
- Webb, E.K.; Pearman, G.I.; Leuning, R. Correction of the flux measurements for density effects due to heat and water vapour transfer. Q. J. R Meteorol. Soc. 1980, 106, 85–100. [Google Scholar] [CrossRef]
- Fuehrer, P.L.; Friehe, C.A. Flux Corrections Revisited. Bound.-Layer Meteorol. 2002, 102, 15–457. [Google Scholar] [CrossRef]
- Wyngaard, J.C.; Coté, O. The budgets of turbulent kinetic energy and temperature variance in the atmospheric surface layer. J. Atmos. Sci. 1971, 28, 190–201. [Google Scholar] [CrossRef]
- Charuchittipan, D.; Babel, W.; Mauder, M.; Leps, J.P.; Foken, T. Extension of the averaging time in eddy-covariance measurements and its effect on the energy balance closure. Bound.-Layer Meteorol. 2014, 152, 303–327. [Google Scholar] [CrossRef]
- Twine, T.E.; Kustas, W.P.; Norman, J.M.; Cook, D.R.; Houser, P.; Meyers, T.P.; Prueger, J.H.; Starks, P.J.; Wesely, M.L. Correcting eddy-covariance flux underestimates over a grassland. Agric. For. Meteorol. 2000, 103, 279–300. [Google Scholar] [CrossRef]
- Eder, F.; De Roo, F.; Kohnert, K.; Desjardins, R.L.; Schmid, H.P.; Mauder, M. Evaluation of two energy balance closure parametrizations. Bound.-Layer Meteorol. 2014, 151, 195–219. [Google Scholar] [CrossRef]
- Anthoni, P.M.; Knohl, A.; Rebmann, C.; Freibauer, A.; Mund, M.; Ziegler, W.; Kolle, O.; Schulze, E.-D. Forest and agricultural land-use-dependent CO2 exchange in Thuringia, Germany. Glob. Chang. Biol. 2004, 10, 2005–2019. [Google Scholar] [CrossRef]
- Prescher, A.-K.; Grünwald, T.; Bernhofer, C. Land use regulates carbon budgets in eastern Germany: From NEE to NBP. Agric. For. Meteorol. 2010, 150, 1016–1025. [Google Scholar] [CrossRef]
- Ma, S.; Baldocchi, D.D.; Xu, L.; Hehn, T. Inter-annual variability in carbon dioxide exchange of an oak/grass savanna and open grassland in California. Agric. For. Meteorol. 2007, 147, 157–171. [Google Scholar] [CrossRef]
- Scott, R.L.; Hamerlynck, E.P.; Jenerette, G.D.; Moran, M.S.; Barron-Gafford, G.A. Carbon dioxide exchange in a semidesert grassland through drought-induced vegetation change. J. Geophys. Res. 2010, 115, G03026. [Google Scholar] [CrossRef]
- Desai, A.R.; Richardson, A.D.; Moffat, A.M.; Kattge, J.; Hollinger, D.Y.; Barr, A.G.; Falge, E.; Noormets, A.; Papale, D.; Reichstein, M.; et al. Cross-site evaluation of eddy covariance GPP and RE decomposition techniques. Agric. For. Meteorol. 2008, 148, 821–838. [Google Scholar] [CrossRef]
- Fischer, M.L.; Billesbach, D.P.; Berry, J.A.; Riley, W.J.; Torn, M.S. Spatiotemporal variations in growing season exchanges of CO2, H2O, and sensible heat in agricultural fields of the Southern Great Plains. Earth Interact. 2007, 11, 1–21. [Google Scholar] [CrossRef]
- Hamerlynck, E.P.; Scott, R.L.; Sánchez-Cañete, E.P.; Barron-Gafford, G.A. Nocturnal soil CO2 uptake and its relationship to subsurface soil and ecosystem carbon fluxes in a Chihuahuan Desert shrubland. J. Geophys. Res. Biogeosci. 2013, 118, 1593–1603. [Google Scholar] [CrossRef]
- Scott, R.L.; Biederman, J.A.; Hamerlynck, E.P.; Barron-Gafford, G.A. The carbon balance pivot point of southwestern U.S. semiarid ecosystems: Insights from the 21st century drought. J. Geophys. Res. Biogeosci. 2015, 120, 2612–2624. [Google Scholar] [CrossRef]
- Allison, V.J.; Miller, R.M.; Jastrow, J.D.; Matamala, R.; Zak, D.R. Changes in soil microbial community structure in a tallgrass prairie chronosequence. Soil Sci. Soc. Am. J. 2005, 69, 1412–1421. [Google Scholar] [CrossRef]
- Scott, R.L.; Jenerette, G.D.; Potts, D.L.; Huxman, T.E. Effects of seasonal drought on net carbon dioxide exchange from a woody-plant-encroached semiarid grassland. J. Geophys. Res. Biogeosci. 2009, 114, G04004. [Google Scholar] [CrossRef]
- Marcolla, B.; Cescatti, A.; Manca, G.; Zorer, R.; Cavagna, M.; Fiora, A.; Gianelle, D.; Rodeghiero, M.; Sottocornola, M.; Zampedri, R. Climatic controls and ecosystem responses drive the inter-annual variability of the net ecosystem exchange of an alpine meadow. Agric. For. Meteorol. 2011, 151, 1233–1243. [Google Scholar] [CrossRef]
- Reichstein, M.; Tenhunen, J.D.; Roupsard, O.; Ourcival, J.-M.; Rambal, S.; Miglietta, F.; Peressotti, A.; Pecchiari, M.; Tirone, G.; Valentini, R. Severe drought effects on ecosystem CO2 and H2O fluxes at three Mediterranean evergreen sites: Revision of current hypotheses? Glob. Chang. Biol. 2002, 8, 999–1017. [Google Scholar] [CrossRef]
- Galvagno, M.; Wohlfahrt, G.; Cremonese, E.; Rossini, M.; Colombo, R.; Filippa, G.; Julitta, T.; Manca, G.; Siniscalco, C.; Morra di Cella, U.; et al. Phenology and carbon dioxide source/sink strength of a subalpine grassland in response to an exceptionally short snow season. Environ. Res. Lett. 2013, 8, 025008. [Google Scholar] [CrossRef]
- Loubet, B.; Laville, P.; Lehuger, S.; Larmanou, E.; Fléchard, C.; Mascher, N.; Génermont, S.; Roche, R.; Ferrara, R.M.; Stella, P.; et al. Carbon, nitrogen and Greenhouse gases budgets over a four years crop rotation in northern France. Plant Soil 2011, 343, 109–137. [Google Scholar] [CrossRef]
- Imer, D.; Merbold, L.; Eugster, W.; Buchmann, N. Temporal and spatial variations of soil CO2, CH4 and N2O fluxes at three differently managed grasslands. Biogeosciences 2013, 10, 5931–5945. [Google Scholar] [CrossRef]
- Merbold, L.; Eugster, W.; Stieger, J.; Zahniser, M.; Nelson, D.; Buchmann, N. Greenhouse gas budget (CO2, CH4 and N2O) of intensively managed grassland following restoration. Glob. Chang. Biol. 2014, 20, 1913–1928. [Google Scholar] [CrossRef] [PubMed]
- Wohlfahrt, G.; Hammerle, A.; Haslwanter, A.; Bahn, M.; Tappeiner, U.; Cernusca, A. Seasonal and inter-annual variability of the net ecosystem CO2 exchange of a temperate mountain grassland: Effects of weather and management. J. Geophys. Res. 2008, 113, D08110. [Google Scholar]
- Moureaux, C.; Debacq, A.; Bodson, B.; Heinesch, B.; Aubinet, M. Annual net ecosystem carbon exchange by a sugar beet crop. Agric. For. Meteorol. 2006, 139, 25–39. [Google Scholar] [CrossRef]
- Serrano-Ortiz, P.; Domingo, F.; Cazorla, A.; Were, A.; Cuezva, S.; Villagarcía, L.; Alados-Arboledas, L.; Kowalski, A.S. Interannual CO2 exchange of a sparse Mediterranean shrubland on a carbonaceous substrate. J. Geophys. Res. 2009, 114, G04015. [Google Scholar]
- Dušek, J.; Čížková, H.; Stellner, S.; Czerný, R.; Květ, J. Fluctuating water table affects gross ecosystem production and gross radiation use efficiency in a sedge-grass marsh. Hydrobiologia 2012, 692, 57–66. [Google Scholar] [CrossRef]
- van der Molen, M.K.; van Huissteden, J.; Parmentier, F.J.W.; Petrescu, A.M.R.; Dolman, A.J.; Maximov, T.C.; Kononov, A.V.; Karsanaev, S.V.; Suzdalov, D.A. The growing season greenhouse gas balance of a continental tundra site in the Indigirka lowlands, NE Siberia. Biogeosciences 2007, 4, 985–1003. [Google Scholar] [CrossRef]
- Jacobs, C.M.J.; Jacobs, A.F.G.; Bosveld, F.C.; Hendriks, D.M.D.; Hensen, A.; Kroon, P.S.; Moors, E.J.; Nol, L.; Schrier-Uijl, A.; Veenendaal, E.M. Variability of annual CO2 exchange from Dutch grasslands. Biogeosciences 2007, 4, 803–816. [Google Scholar] [CrossRef]
- Beringer, J.; Hutley, L.B.; Tapper, N.J.; Cernusak, L.A. Savanna fires and their impact on net ecosystem productivity in North Australia. Glob. Chang. Biol. 2007, 13, 990–1004. [Google Scholar] [CrossRef]
| Site ID | Country | Latitude (°N) | Longitude (°E) | Land Use | Time Period of Observations (yr) | Number of 30-min Samples | Description Reference | Dataset Reference |
|---|---|---|---|---|---|---|---|---|
| DE-Geb | Germany | 51.099 | 10.9146 | Cropland | 13.95 | 79,598 | Anthoni et al., 2004 [26] | https://doi.org/10.18140/FLX/1440146 |
| DE-Gri | Germany | 50.9500 | 13.5126 | Grassland | 11.00 | 60,439 | Prescher et al., 2010 [27] | https://doi.org/10.18140/FLX/1440147 |
| DE-Cli | Germany | 50.8931 | 13.5224 | Cropland | 10.75 | 48,470 | Prescher et al., 2010 [27] | https://doi.org/10.18140/FLX/1440149 |
| US-Var | USA | 38.4133 | −120.9508 | Grassland and Wetland | 14.19 | 88,913 | Ma et al., 2007 [28] | https://doi.org/10.18140/FLX/1440094 |
| US-Wkg | USA | 31.7365 | −109.9419 | Grassland | 10.65 | 75,903 | Scott et al., 2010 [29] | https://doi.org/10.18140/FLX/1440096 |
| US-Los | USA | 46.0827 | −89.9792 | Wetland | 10.31 | 48,447 | Desai et al., 2008 [30] | https://doi.org/10.18140/FLX/1440076 |
| US-ARM | USA | 36.6058 | −97.4888 | Cropland | 10.00 | 64,796 | Fischer et al., 2007 [31] | https://doi.org/10.18140/FLX/1440066 |
| US-Whs | USA | 31.7438 | −110.0522 | Shrubland | 7.51 | 55,470 | Hamerlynck et al., 2013 [32] | https://doi.org/10.18140/FLX/1440097 |
| US-SRG | USA | 31.7894 | −110.8277 | Grassland | 6.72 | 45,240 | Scott et al., 2015 [33] | https://doi.org/10.18140/FLX/1440114 |
| US-IB2 | USA | 41.8406 | −88.2410 | Grassland | 7.23 | 47,351 | Allison et al., 2005 [34] | https://doi.org/10.18140/FLX/1440072 |
| US-SRM | USA | 31.8214 | −110.8661 | Woody Savanna | 10.99 | 78,193 | Scott et al., 2009 [35] | https://doi.org/10.18140/FLX/1440090 |
| IT-MBo | Italy | 46.0147 | 11.0458 | Grassland | 10.89 | 49,862 | Marcolla et al., 2011 [36] | https://doi.org/10.18140/FLX/1440170 |
| IT-NOe | Italy | 40.6062 | 8.1517 | Shrubland | 10.74 | 62,614 | Reichstein et al., 2002 [37] | https://doi.org/10.18140/FLX/1440171 |
| IT-Tor | Italy | 45.8444 | 7.5781 | Grassland | 6.49 | 27,867 | Galvagno et al., 2013 [38] | https://doi.org/10.18140/FLX/1440237 |
| FR-Gri | France | 48.8442 | 1.9519 | Cropland | 10.36 | 55,252 | Loubet et al., 2011 [39] | https://doi.org/10.18140/FLX/1440162 |
| CH-Fru | Switzerland | 47.1158 | 8.5378 | Grassland | 6.97 | 40,118 | Imer et al., 2013 [40] | https://doi.org/10.18140/FLX/1440133 |
| CH-Cha | Switzerland | 47.2102 | 8.4104 | Grassland | 7.00 | 43,892 | Merbold et al., 2014 [41] | https://doi.org/10.18140/FLX/1440131 |
| AT-Neu | Austria | 47.1167 | 11.3175 | Grassland | 10.93 | 46,588 | Wohlfahrt et al., 2008 [42] | https://doi.org/10.18140/FLX/1440121 |
| BE-Lon | Belgium | 50.5516 | 4.7462 | Cropland | 10.75 | 49,670 | Moureaux et al., 2006 [43] | https://doi.org/10.18140/FLX/1440129 |
| ES-LJu | Spain | 36.9266 | −2.7521 | Shrubland | 9.67 | 65,507 | Serrano-Ortiz et al., 2009 [44] | https://doi.org/10.18140/FLX/1440157 |
| CZ-WET | Czechia | 49.0247 | 14.7704 | Wetland | 8.68 | 49,302 | Dušek et al., 2012 [45] | https://doi.org/10.18140/FLX/1440145 |
| Ru-Cok | Russia | 70.8291 | 147.4943 | Shrubland | 9.68 | 31,821 | van der Molen et al., 2007 [46] | https://doi.org/10.18140/FLX/1440182 |
| NL-HoR | The Netherlands | 52.2403 | 5.0713 | Grassland | 7.52 | 23,609 | Jacobs et al., 2007 [47] | https://doi.org/10.18140/FLX/1440177 |
| AU-HoW | Australia | −12.4943 | 131.1523 | Woody Savanna | 11.33 | 47,279 | Beringer et al., 2007 [48] | https://doi.org/10.18140/FLX/1440125 |
| Site ID | Slope a (BC) Rn - G = a(H + LvE) + b | Slope ac (AC) Rn − G = ac(H + LvE + Δ(H) + Δ(LvE)) + bc | R2 | BC Slope (Geometric Mean) | AC Slope (Geometric Mean) |
|---|---|---|---|---|---|
| DE-Geb | 0.831 | 0.958 | 0.84 | 0.901 | 1.043 |
| DE-Gri | 0.616 | 0.692 | 0.90 | 0.651 | 0.731 |
| DE-Cli | 0.669 | 0.777 | 0.82 | 0.741 | 0.860 |
| US-Var | 0.656 | 0.807 | 0.83 | 0.715 | 0.887 |
| US-Wkg | 0.743 | 0.916 | 0.81 | 0.823 | 1.017 |
| US-Los | 0.653 | 0.766 | 0.82 | 0.725 | 0.845 |
| US-ARM | 0.753 | 0.893 | 0.84 | 0.817 | 0.975 |
| US-Whs | 0.812 | 1.008 | 0.86 | 0.876 | 1.090 |
| US-SRG | 0.778 | 0.943 | 0.86 | 0.834 | 1.017 |
| US-IB2 | 0.782 | 0.885 | 0.90 | 0.822 | 0.934 |
| US-SRM | 0.793 | 0.982 | 0.87 | 0.848 | 1.053 |
| IT-MBo | 0.811 | 0.921 | 0.84 | 0.888 | 1.007 |
| IT-NOe | 0.935 | 1.172 | 0.82 | 1.030 | 1.290 |
| IT-Tor | 0.857 | 0.981 | 0.88 | 0.912 | 1.044 |
| FR-Gri | 0.695 | 0.808 | 0.82 | 0.765 | 0.889 |
| CH-Fru | 0.795 | 0.894 | 0.86 | 0.861 | 0.965 |
| CH-Cha | 0.780 | 0.866 | 0.84 | 0.854 | 0.943 |
| AT-Neu | 0.753 | 0.838 | 0.80 | 0.844 | 0.938 |
| BE-Lon | 0.755 | 0.861 | 0.75 | 0.877 | 0.992 |
| ES-LJu | 0.605 | 0.755 | 0.80 | 0.676 | 0.843 |
| CZ-WET | 0.728 | 0.838 | 0.87 | 0.779 | 0.898 |
| Ru-Cok | 0.641 | 0.758 | 0.73 | 0.748 | 0.887 |
| NL-HoR | 0.576 | 0.649 | 0.49 | 0.831 | 0.926 |
| AU-HoW | 0.818 | 0.943 | 0.71 | 0.977 | 1.122 |
| Averages | 0.743 | 0.871 | 0.81 | 0.825 | 0.967 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Durand, P. A Possible Reconciliation between Eddy Covariance Fluxes and Surface Energy Balance Closure. Atmosphere 2022, 13, 1965. https://doi.org/10.3390/atmos13121965
Durand P. A Possible Reconciliation between Eddy Covariance Fluxes and Surface Energy Balance Closure. Atmosphere. 2022; 13(12):1965. https://doi.org/10.3390/atmos13121965
Chicago/Turabian StyleDurand, Pierre. 2022. "A Possible Reconciliation between Eddy Covariance Fluxes and Surface Energy Balance Closure" Atmosphere 13, no. 12: 1965. https://doi.org/10.3390/atmos13121965
APA StyleDurand, P. (2022). A Possible Reconciliation between Eddy Covariance Fluxes and Surface Energy Balance Closure. Atmosphere, 13(12), 1965. https://doi.org/10.3390/atmos13121965





