Accurate Stall Prediction for Thick Airfoil by Delayed Detached-Eddy Simulations
Abstract
1. Introduction
2. Numerical Simulation
2.1. Numerical Models
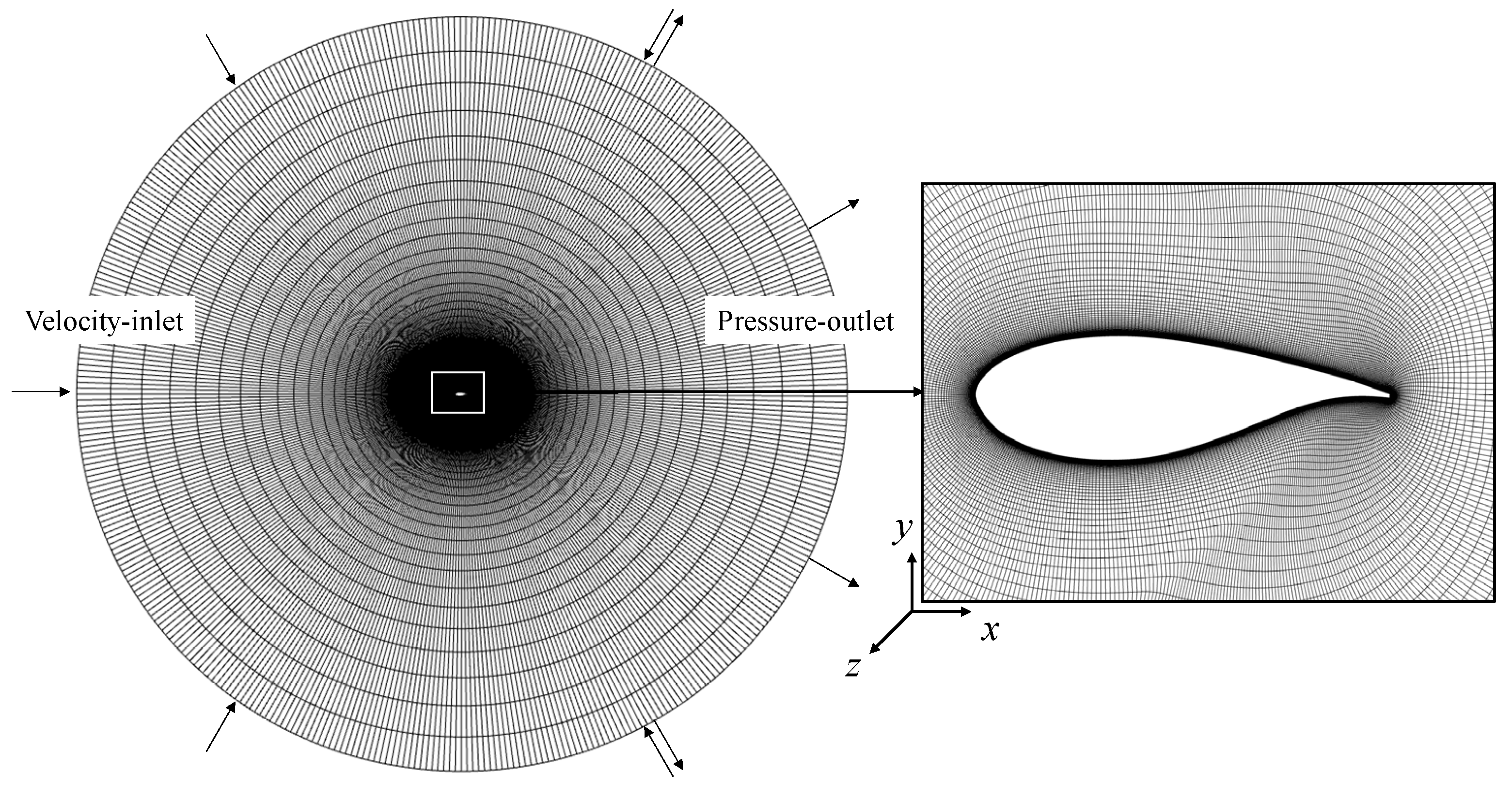
2.2. Mesh and Boundary Conditions
3. Results and Discussion
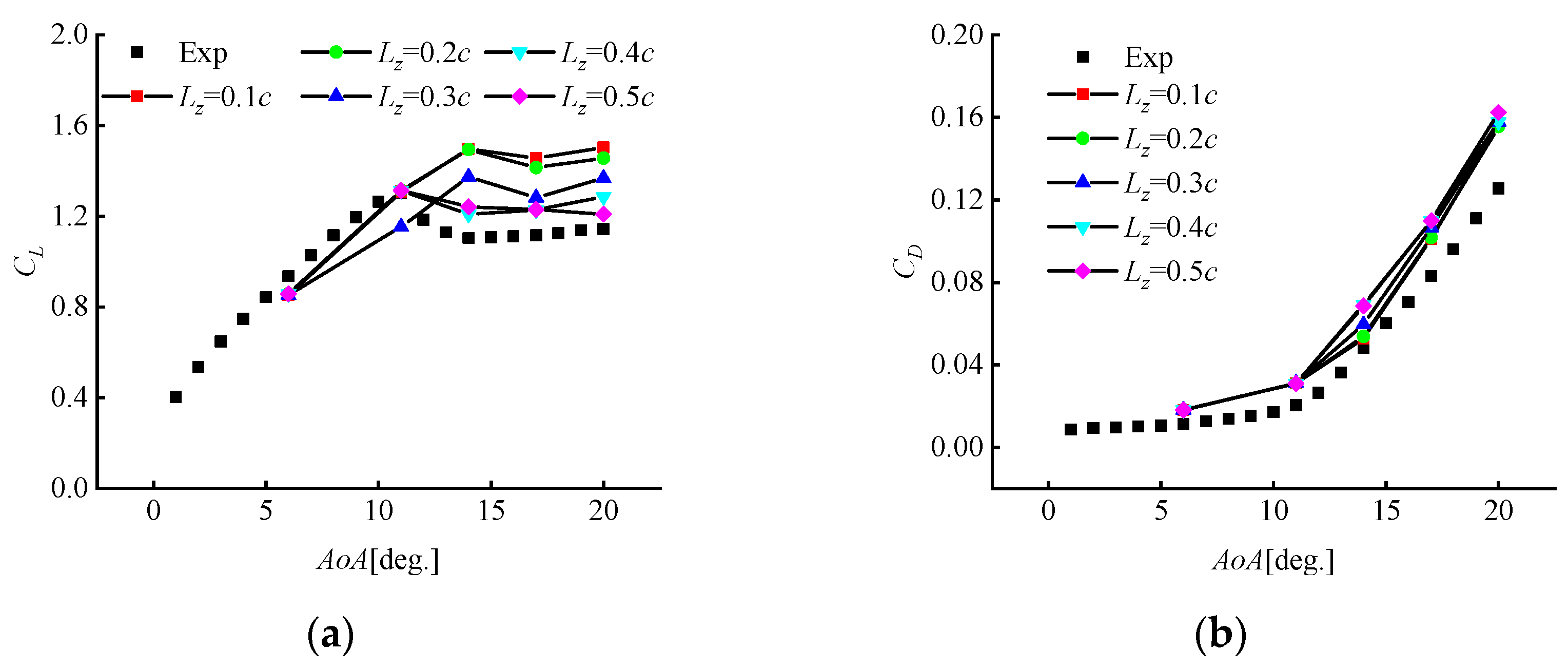
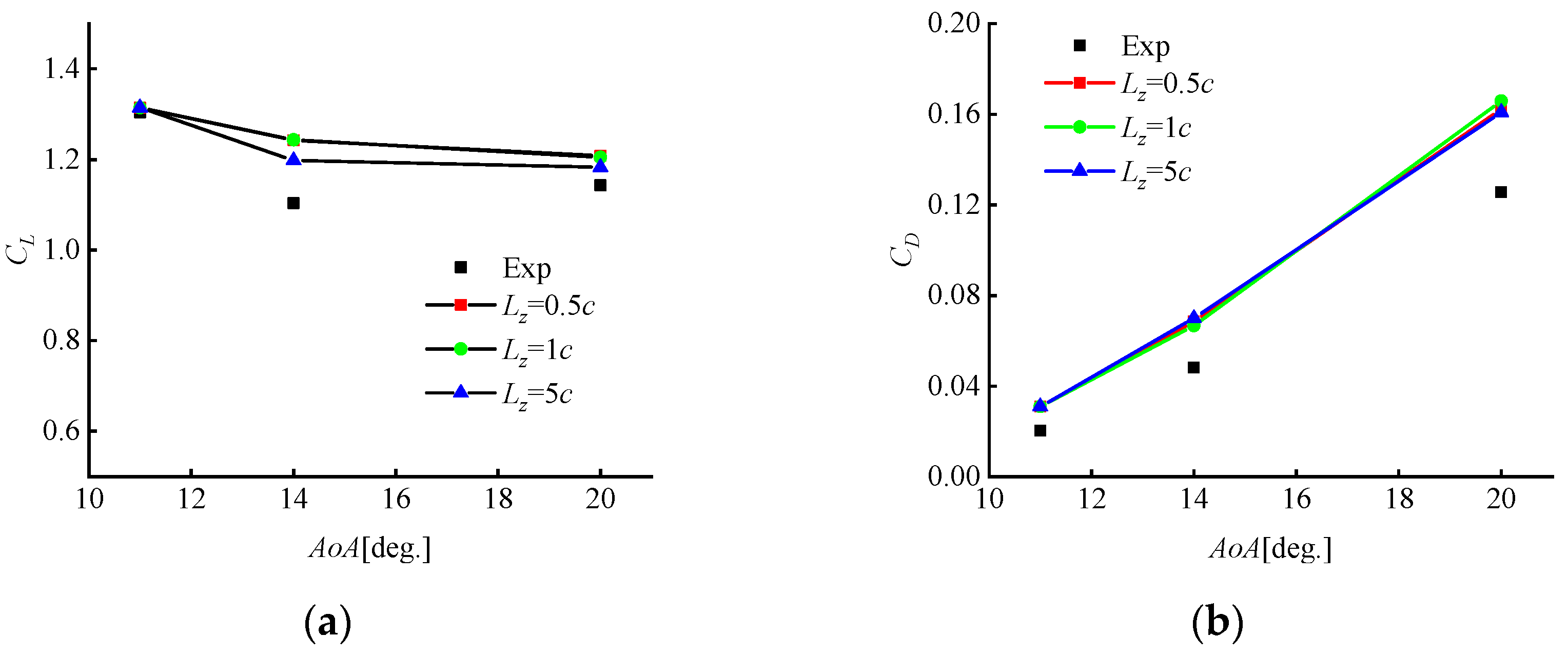
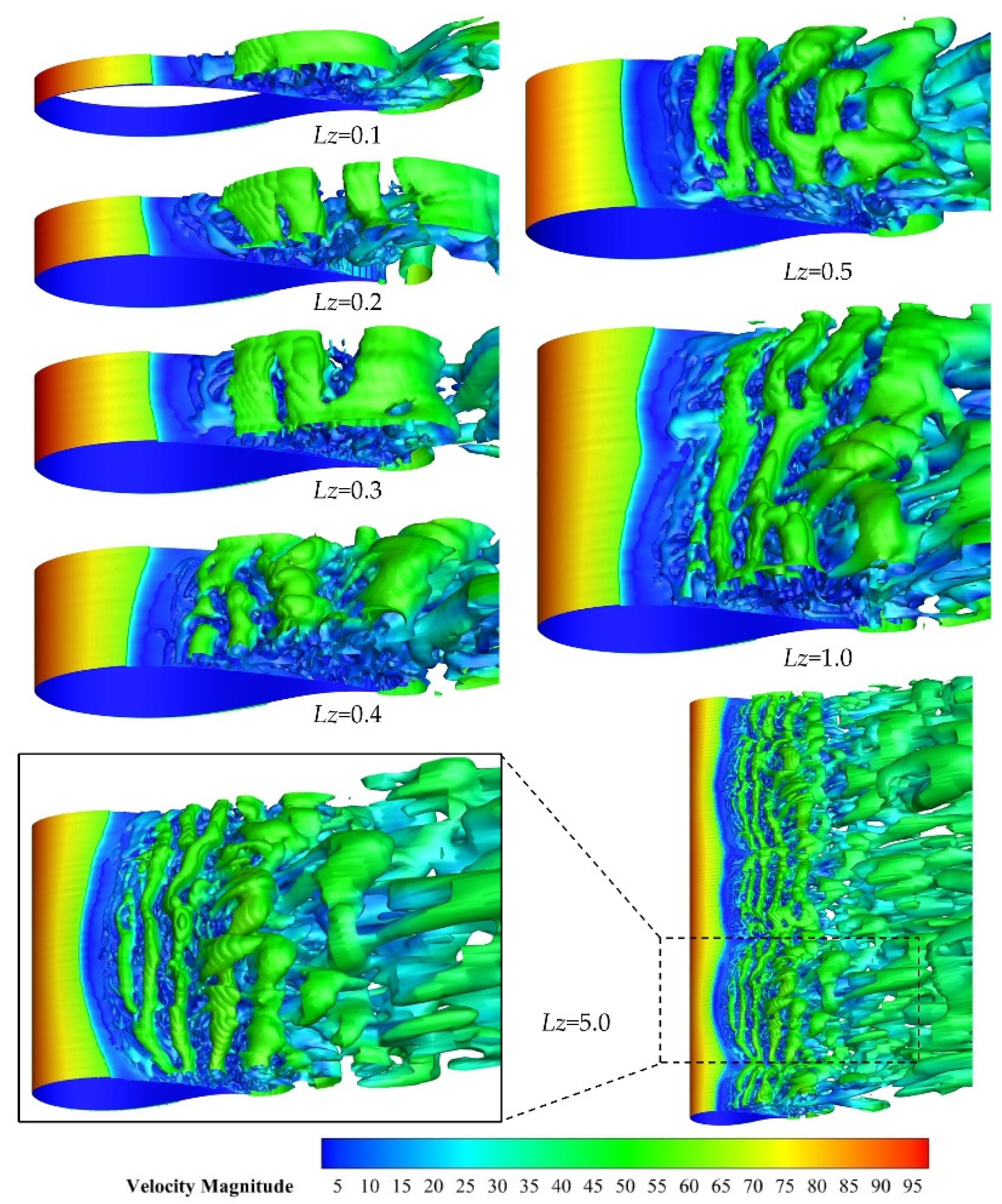
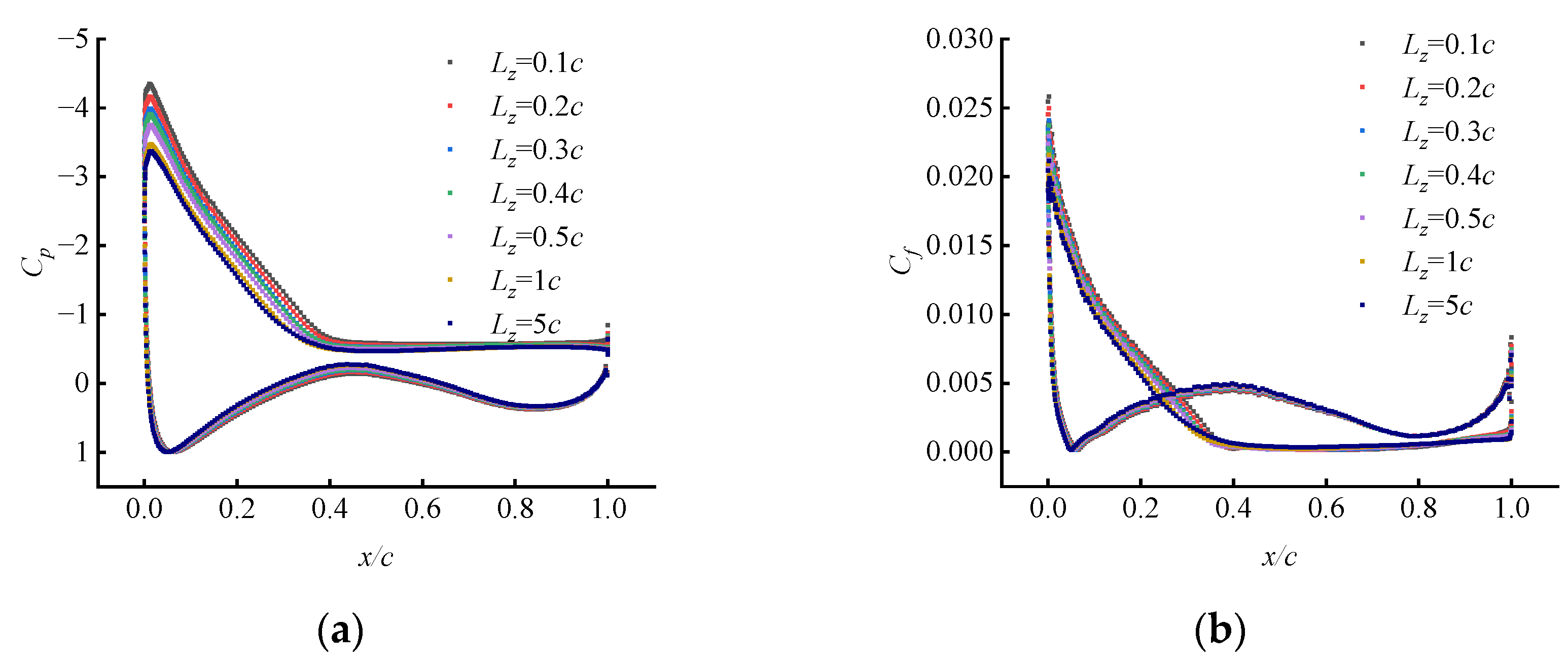
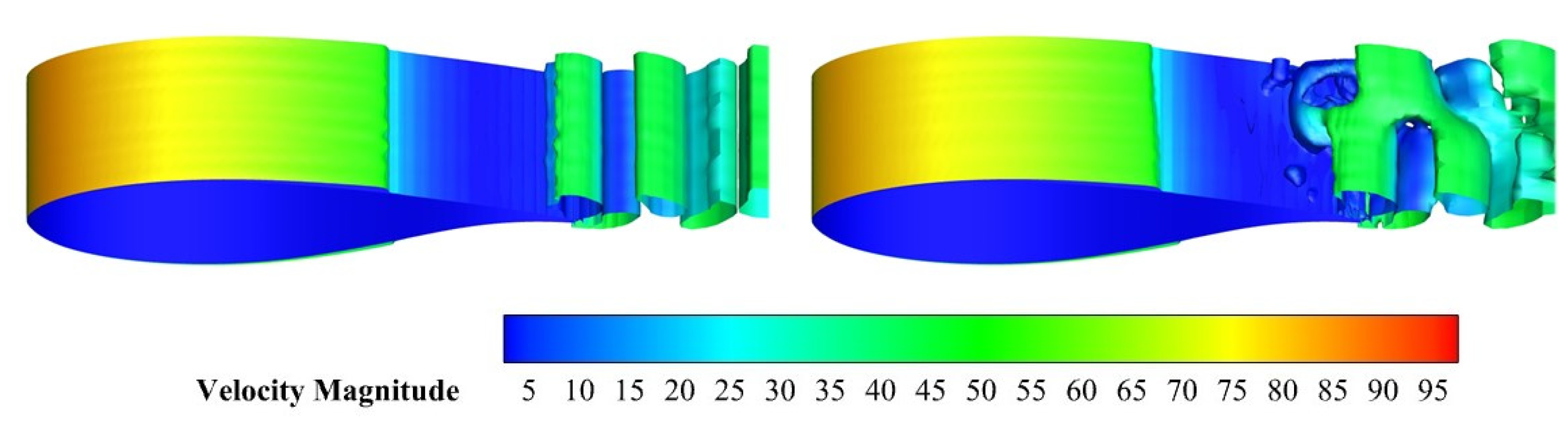
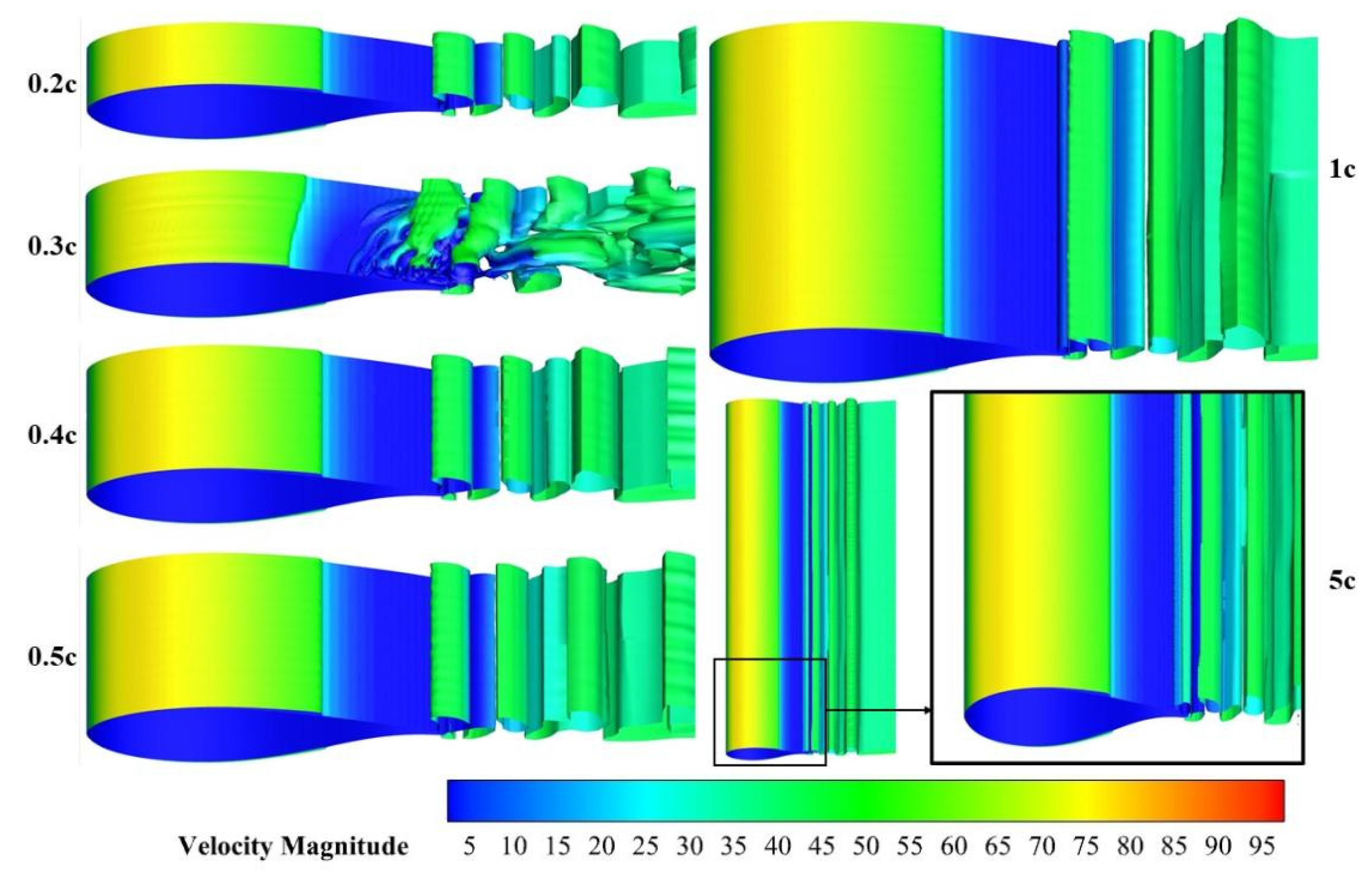
3.1. Spanwise Length Analysis
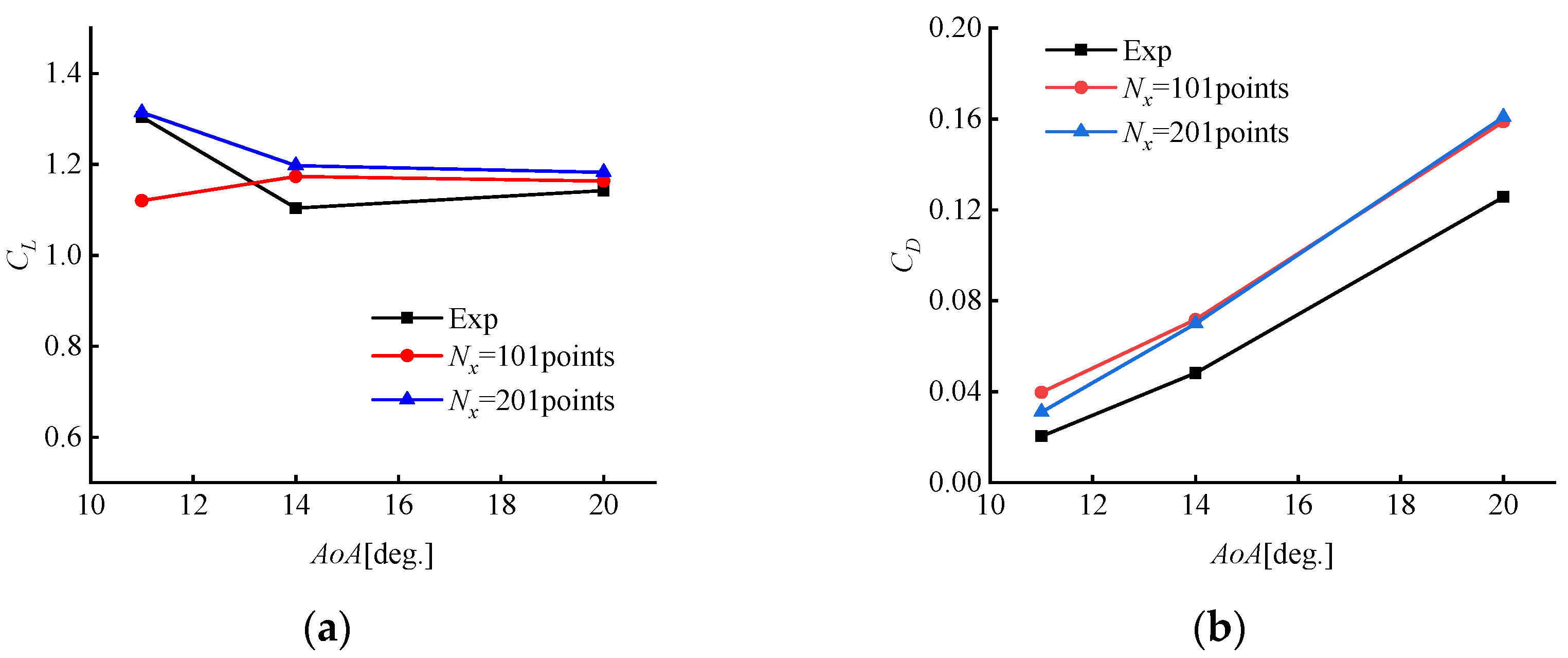
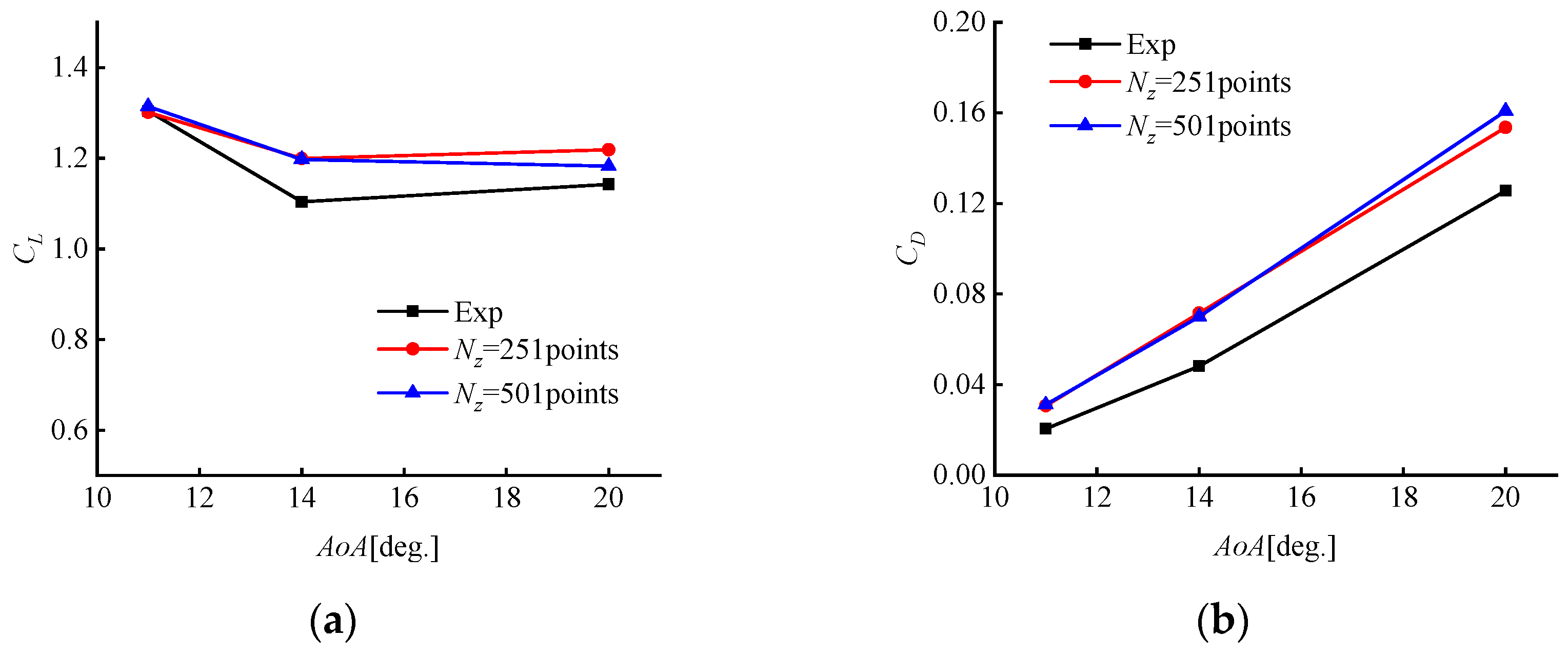
3.2. The Grid Sensitivity
3.3. The Accurate Prediction of Stall-Starting AOA
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Sun, Z.; Zhu, W.J.; Shen, W.Z.; Zhong, W.; Cao, J.; Tao, Q. Aerodynamic Analysis of Coning Effects on the DTU 10 MW Wind Turbine Rotor. Energies 2020, 13, 5753–5771. [Google Scholar] [CrossRef]
- Loth, E.; Steele, A.; Ichter, B.; Selig, M.; Moriarty, P. Segmented Ultralight Pre-Aligned Rotor for Extreme-Scale Wind Turbines. In Proceedings of the 50th AIAA Aerospace Sciences Meeting including the New Horizons Forum and Aerospace Exposition, American Institute of Aeronautics and Astronautics, Nashville, TN, USA, 9 January 2012. [Google Scholar]
- Barnes, R.H.; Morozov, E.V. Structural Optimisation of Composite Wind Turbine Blade Structures with Variations of Internal Geometry Configuration. Compos. Struct. 2016, 152, 158–167. [Google Scholar] [CrossRef]
- Chen, J.; Wang, Q.; Shen, W.Z.; Pang, X.; Li, S.; Guo, X. Structural Optimization Study of Composite Wind Turbine Blade. Mater. Des. 2013, 46, 247–255. [Google Scholar] [CrossRef]
- Sun, Z.; Sessarego, M.; Chen, J.; Shen, W.Z. Design of the OffWindChina 5 MW Wind Turbine Rotor. Energies 2017, 10, 777–796. [Google Scholar] [CrossRef]
- Hrgovan, I.; Shen, W.Z.; Zhu, W.J.; Madsen, J.; Hansen, R. Design and Experimental Validation of Thick Airfoils for Large Wind Turbines. In Renewable Energy in the Service of Mankind Vol I: Selected Topics from the World Renewable Energy Congress WREC 2014; Sayigh, A., Ed.; Springer International Publishing: Cham, Switzerland, 2015; pp. 855–863. [Google Scholar]
- Zahle, F.; Bak, C.; Sørensen, N.N.; Vronsky, T.; Gaudern, N. Design of the LRP Airfoil Series Using 2D CFD. J. Phys. Conf. Ser. 2014, 524, 12020. [Google Scholar] [CrossRef]
- Drela, M. XFOIL: An Analysis and Design System for Low Reynolds Number Airfoils. In Proceedings of the Low Reynolds Number Aerodynamics; Mueller, T.J., Ed.; Springer: Berlin/Heidelberg, Germany, 1989; pp. 1–12. [Google Scholar]
- Szydlowski, J.; Costes, M. Simulation of Flow Around a Static and Oscillating in Pitch NACA 0015 Airfoil Using URANS and DES. In Proceedings of the Volume 2, Parts A and B; ASMEDC: Charlotte, NC, USA, 2004; pp. 891–908. [Google Scholar]
- Richez, F.; Mary, I.; Gleize, V.; Basdevant, C. Near Stall Simulation of the Flow around an Airfoil Using Zonal RANS/LES Coupling Method. Comput Fluids 2008, 37, 857–866. [Google Scholar] [CrossRef]
- Richez, F.; Mary, I.; Gleize, V.; Basdevant, C. Zonal RANS/LES Coupling Simulation of a Transitional and Separated Flow around an Airfoil near Stall. Theor. Comput. Fluid Dyn. 2008, 22, 305–315. [Google Scholar] [CrossRef]
- Sandham, N.D. Transitional Separation Bubbles and Unsteady Aspects of Aerofoil Stall. Aeronaut. J. 2008, 112, 395–404. [Google Scholar] [CrossRef]
- Almutairi, J.; Jones, L.; Sandham, N. Intermittent Bursting of a Laminar Separation Bubble on an Airfoil. AIAA J. 2010, 48, 414–426. [Google Scholar] [CrossRef]
- Fukumoto, H.; Aono, H.; Nonomura, T.; Oyama, A.; Fujii, K. Significance of Computational Spanwise Domain Length on LES for the Flowfield with Large Vortex Structure; American Institute of Aeronautics and Astronautics: Nashville, TN, USA, 4 January 2016; Volume 7. [Google Scholar]
- Manni, L.; Nishino, T.; Delafin, P.-L. Numerical Study of Airfoil Stall Cells Using a Very Wide Computational Domain. Comput Fluids 2016, 140, 260–269. [Google Scholar] [CrossRef]
- Sharma, A.; Visbal, M. Numerical Investigation of the Effect of Airfoil Thickness on Onset of Dynamic Stall. J. Fluid Mech. 2017, 870, 870–900. [Google Scholar] [CrossRef]
- Asada, K.; Kawai, S. Large-Eddy Simulation of Airfoil Flow near Stall Condition at Reynolds Number 2.1 × 106. Phys. Fluids 2018, 30, 85103. [Google Scholar] [CrossRef]
- Yalçın, Ö.; Cengiz, K.; Özyörük, Y. High-Order Detached Eddy Simulation of Unsteady Flow around NREL S826 Airfoil. J. Wind Eng. Ind. Aerodyn. 2018, 179, 125–134. [Google Scholar] [CrossRef]
- Cui, W.; Xiao, Z.; Yuan, X. Simulations of Transition and Separation Past a Wind-Turbine Airfoil near Stall. Energy 2020, 205, 118003. [Google Scholar] [CrossRef]
- Tamaki, Y.; Fukushima, Y.; Kuya, Y.; Kawai, S. Physics and Modeling of Trailing-Edge Stall Phenomena for Wall-Modeled Large-Eddy Simulation. Phys. Rev. Fluid 2020, 5, 74602. [Google Scholar] [CrossRef]
- Gleize, V.; Costes, M.; Mary, I. Numerical Simulation of NACA4412 Airfoil in Pre-Stall Conditions. International Journal of Numer. Methods Heat Fluid Flow 2021, 32, 1375–1397. [Google Scholar] [CrossRef]
- Menter, F.; Kuntz, M.; Langtry, R. Ten Years of Industrial Experience with the SST Turbulence Model. Turbul. Heat Mass Transf. 2003, 4, 625–632. [Google Scholar]
- Menter, F.R. Two-Equation Eddy-Viscosity Turbulence Models for Engineering Applications. AIAA J. 1994, 32, 1598–1605. [Google Scholar] [CrossRef]
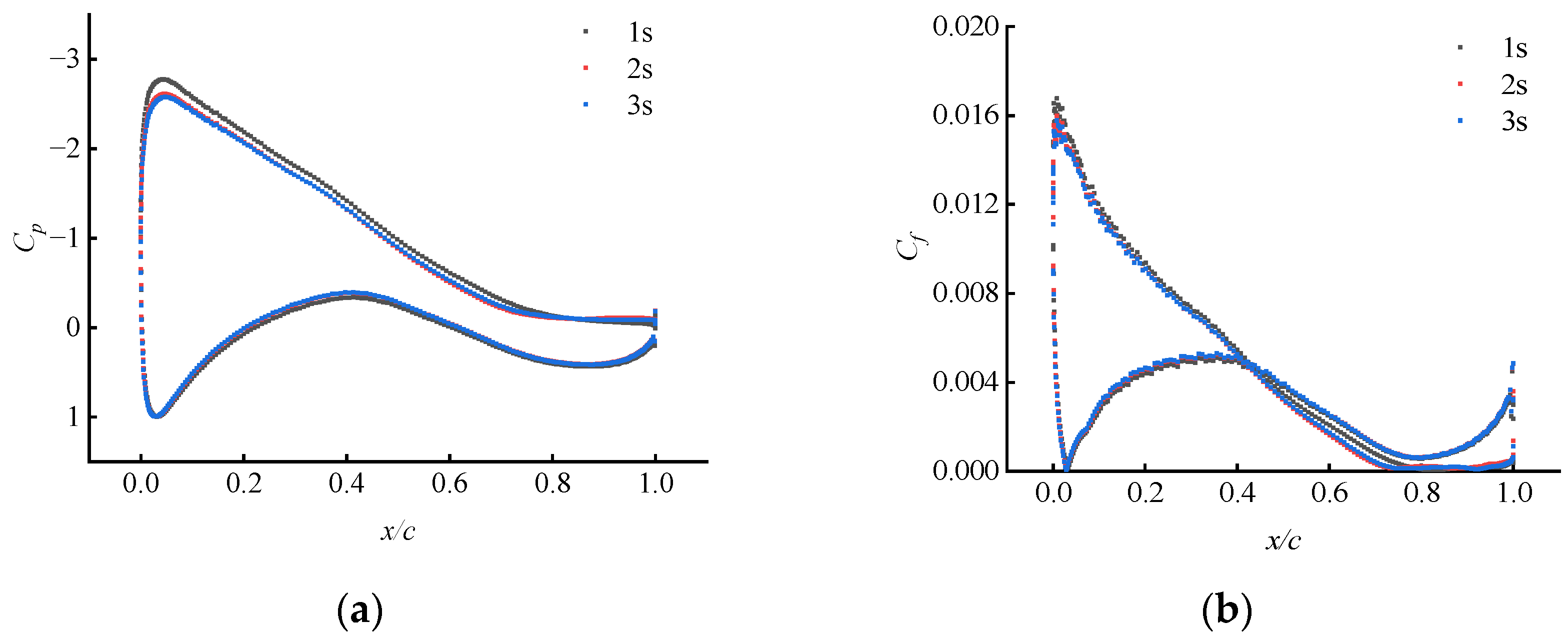
| Lz/c | Nx | Ny | Nz | ∆x+ | ∆y+ | ∆z+ |
|---|---|---|---|---|---|---|
| 0.1 | 201 | 134 | 11 | ~600 | 1 | 1200 |
| 0.2 | 201 | 134 | 21 | ~600 | 1 | 1200 |
| 0.3 | 201 | 134 | 31 | ~600 | 1 | 1200 |
| 0.4 | 201 | 134 | 41 | ~600 | 1 | 1200 |
| 0.5 | 201 | 134 | 51 | ~600 | 1 | 1200 |
| 1 | 201 | 134 | 101 | ~600 | 1 | 1200 |
| 5 | 201 | 134 | 501 | ~600 | 1 | 1200 |
| 5 | 201 | 134 | 251 | ~600 | 1 | 2400 |
| 5 | 101 | 134 | 501 | ~1200 | 1 | 1200 |
| ∆CL | 6° | 11° | 14° | 17° | 20° |
|---|---|---|---|---|---|
| Lz = 0.1c | −8.92% | 0.35% | 35.69% | 30.38% | 31.53% |
| Lz = 0.2c | −8.28% | 0.75% | 35.39% | 26.75% | 27.47% |
| Lz = 0.3c | −8.97% | −11.57% | 24.45% | 14.79% | 19.75% |
| Lz = 0.4c | −8.28% | 0.75% | 9.54% | 9.99% | 12.51% |
| Lz = 0.5c | −8.28% | 0.74% | 12.56% | 10.19% | 5.73% |
| ∆ CD | 6° | 11° | 14° | 17° | 20° |
|---|---|---|---|---|---|
| Lz = 0.1c | 59.54% | 51.22% | 9.69% | 21.70% | 25.08% |
| Lz = 0.2c | 59.91% | 51.59% | 11.58% | 22.40% | 23.82% |
| Lz = 0.3c | 60.04% | 52.29% | 23.74% | 28.24% | 25.67% |
| Lz = 0.4c | 59.91% | 51.60% | 43.57% | 32.02% | 25.79% |
| Lz = 0.5c | 59.91% | 51.58% | 42.24% | 32.29% | 29.22% |
| 11° | 14° | 20° | ||
|---|---|---|---|---|
| Lz = 0.5c | 0.74% | 12.56% | 5.73% | |
| ∆CL | Lz = 1c | 0.75% | 12.64% | 5.42% |
| Lz = 5c | 0.76% | 8.54% | 3.50% | |
| Lz = 0.5c | 51.58% | 42.24% | 29.22% | |
| ∆CD | Lz = 1c | 51.60% | 38.47% | 32.04% |
| Lz = 5c | 51.61% | 45.19% | 28.07% |
| 11° | 14° | 20° | ||
|---|---|---|---|---|
| Nx = 201, Nz = 501 | 0.76% | 8.54% | 3.50% | |
| ∆CL | Nx = 101, Nz = 501 | −14.12% | 6.33% | 1.81% |
| Nx = 201, Nz = 251 | −0.23% | 8.70% | 6.68% | |
| Nx = 201, Nz = 501 | 51.61% | 45.19% | 28.07% | |
| ∆CD | Nx = 101, Nz = 501 | 93.66% | 48.84% | 26.40% |
| Nx = 201, Nz = 251 | 49.46% | 48.34% | 22.25% |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sun, Z.; Shi, R.; Zhu, W.; Li, X.; Yang, J. Accurate Stall Prediction for Thick Airfoil by Delayed Detached-Eddy Simulations. Atmosphere 2022, 13, 1804. https://doi.org/10.3390/atmos13111804
Sun Z, Shi R, Zhu W, Li X, Yang J. Accurate Stall Prediction for Thick Airfoil by Delayed Detached-Eddy Simulations. Atmosphere. 2022; 13(11):1804. https://doi.org/10.3390/atmos13111804
Chicago/Turabian StyleSun, Zhenye, Rongkun Shi, Weijun Zhu, Xiaochuan Li, and Junwei Yang. 2022. "Accurate Stall Prediction for Thick Airfoil by Delayed Detached-Eddy Simulations" Atmosphere 13, no. 11: 1804. https://doi.org/10.3390/atmos13111804
APA StyleSun, Z., Shi, R., Zhu, W., Li, X., & Yang, J. (2022). Accurate Stall Prediction for Thick Airfoil by Delayed Detached-Eddy Simulations. Atmosphere, 13(11), 1804. https://doi.org/10.3390/atmos13111804











