The Sun and Space Weather
Abstract
1. Introduction
2. Basic Properties of CMEs
2.1. Morphological Properties
2.2. Physical Properties
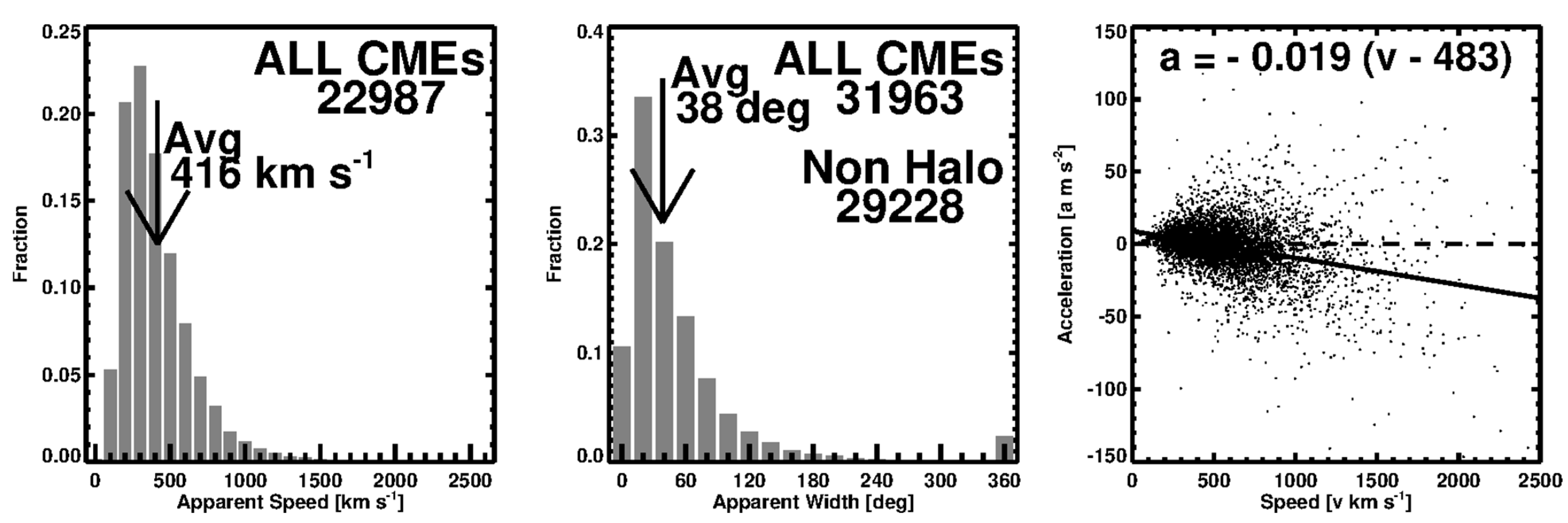
2.3. Kinematic Properties
2.4. CME Mass and Kinetic Energy
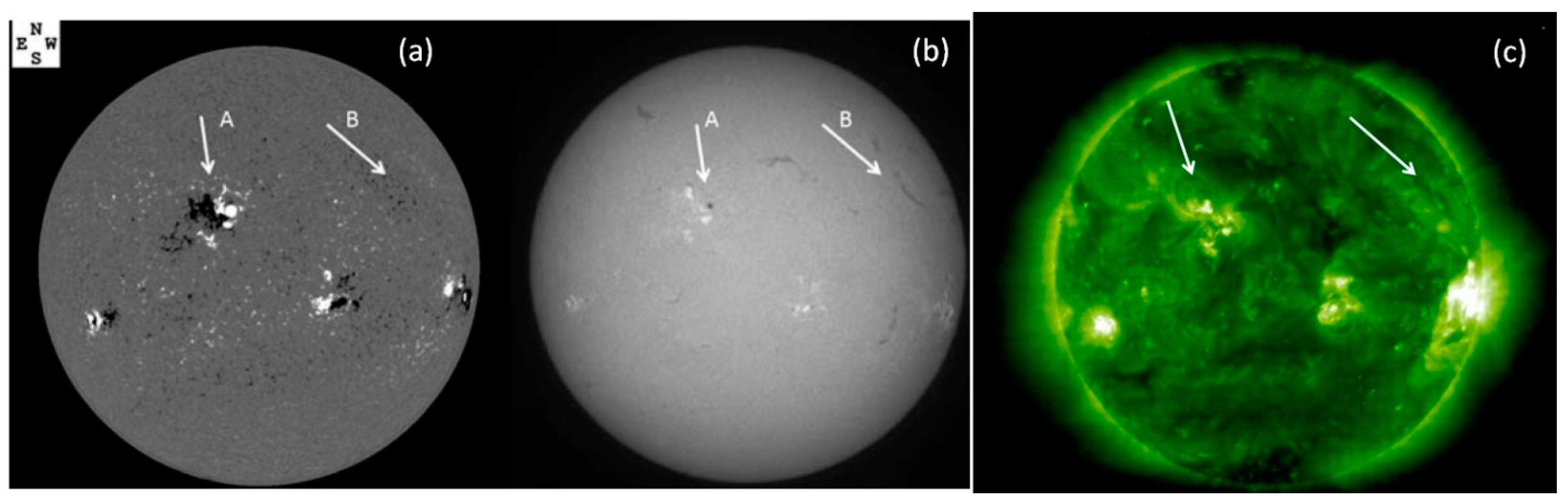
3. CME Source Regions, Flares, and Filaments
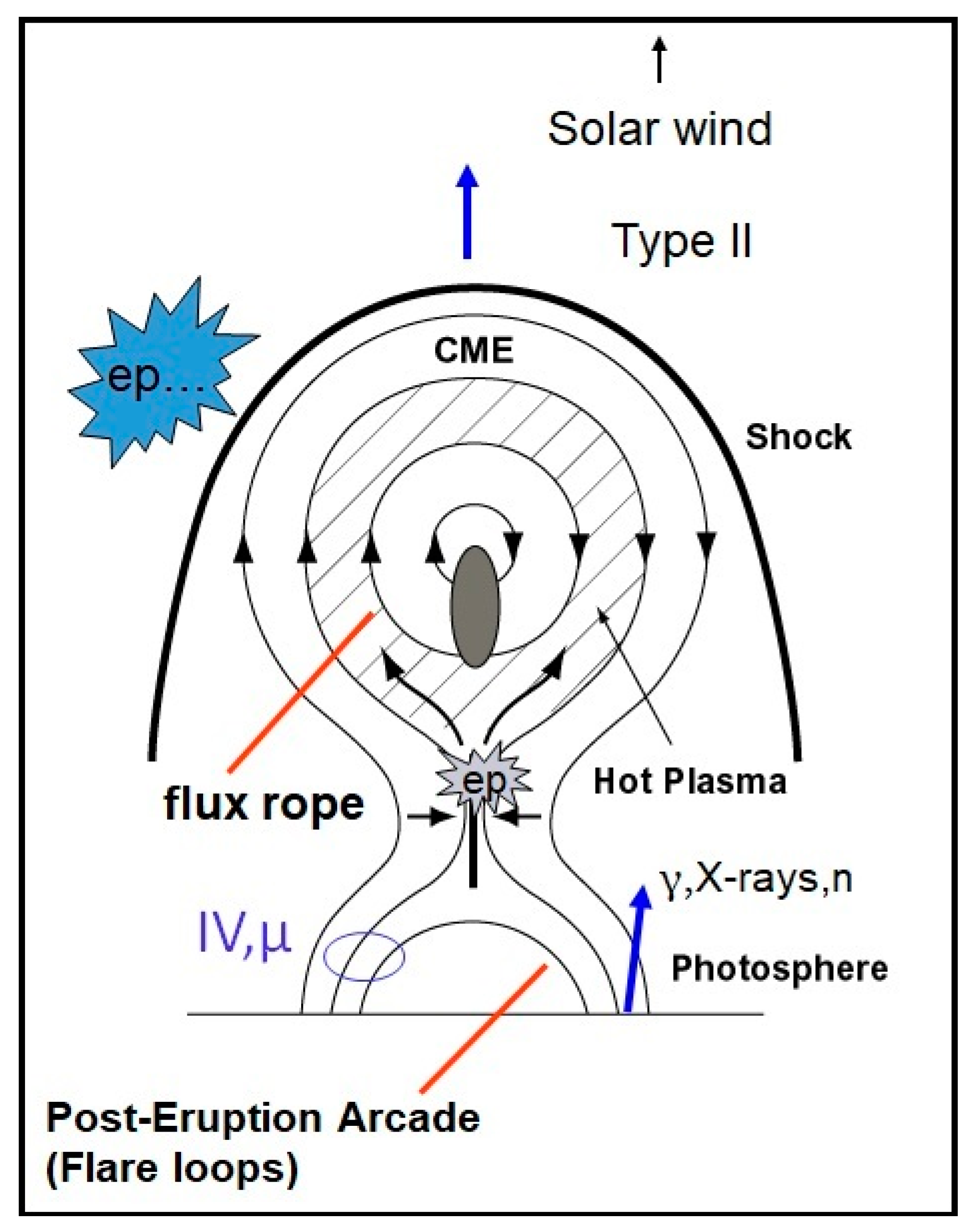
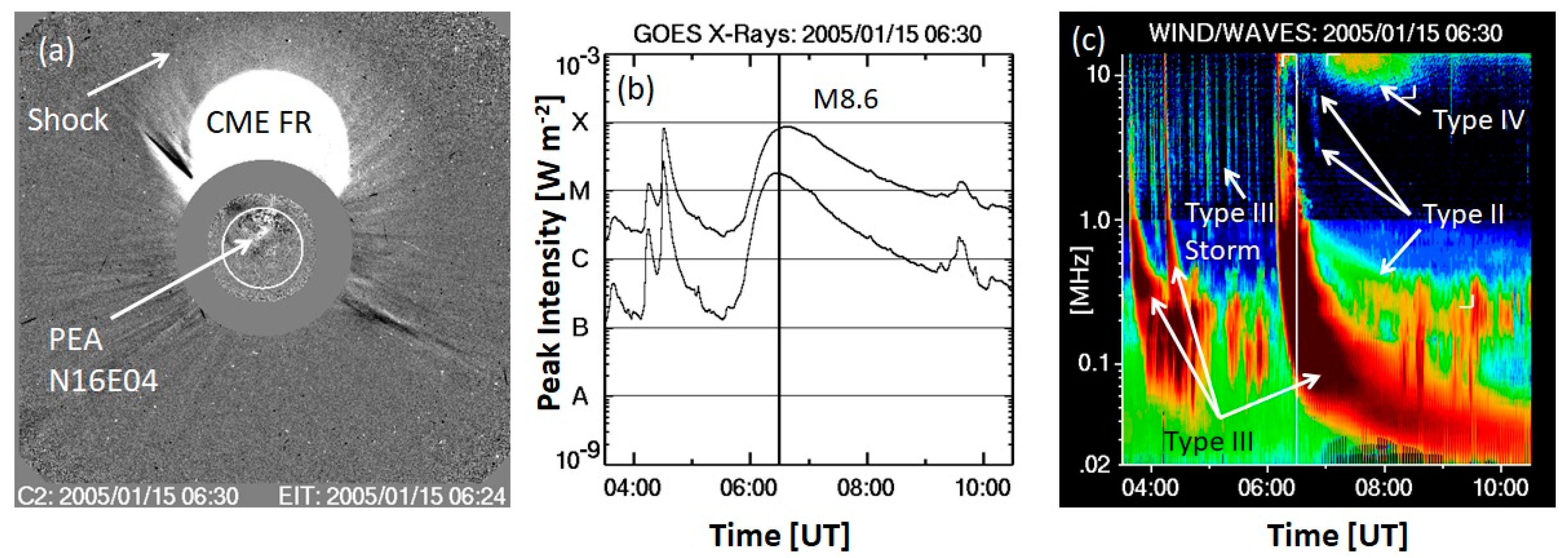
4. CMEs and Radio Bursts
5. Solar Connection to Geomagnetic Storms
5.1. CMEs and Geomagnetic Storms
5.2. Coronal Holes and Geomagnetic Storms
6. Solar Eruptions and SEPs
7. Space Weather Events and Spacecraft Anomalies
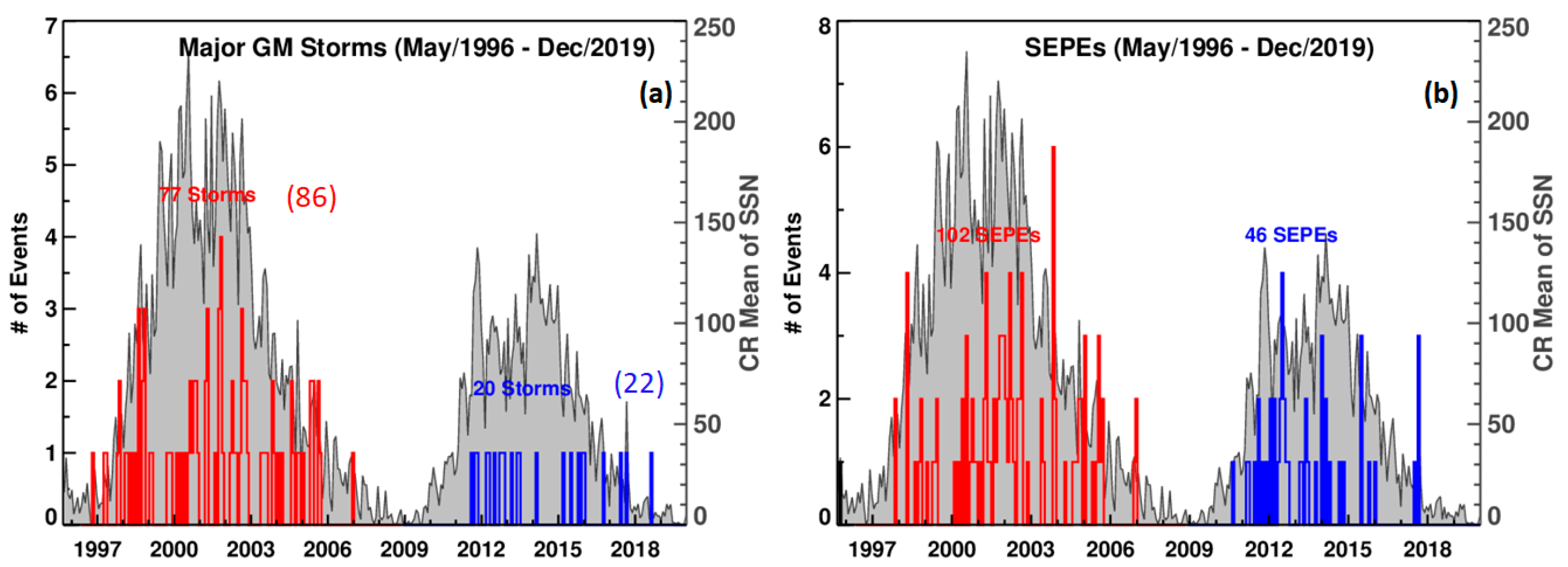
8. Solar Cycle Variation of Space Weather Events
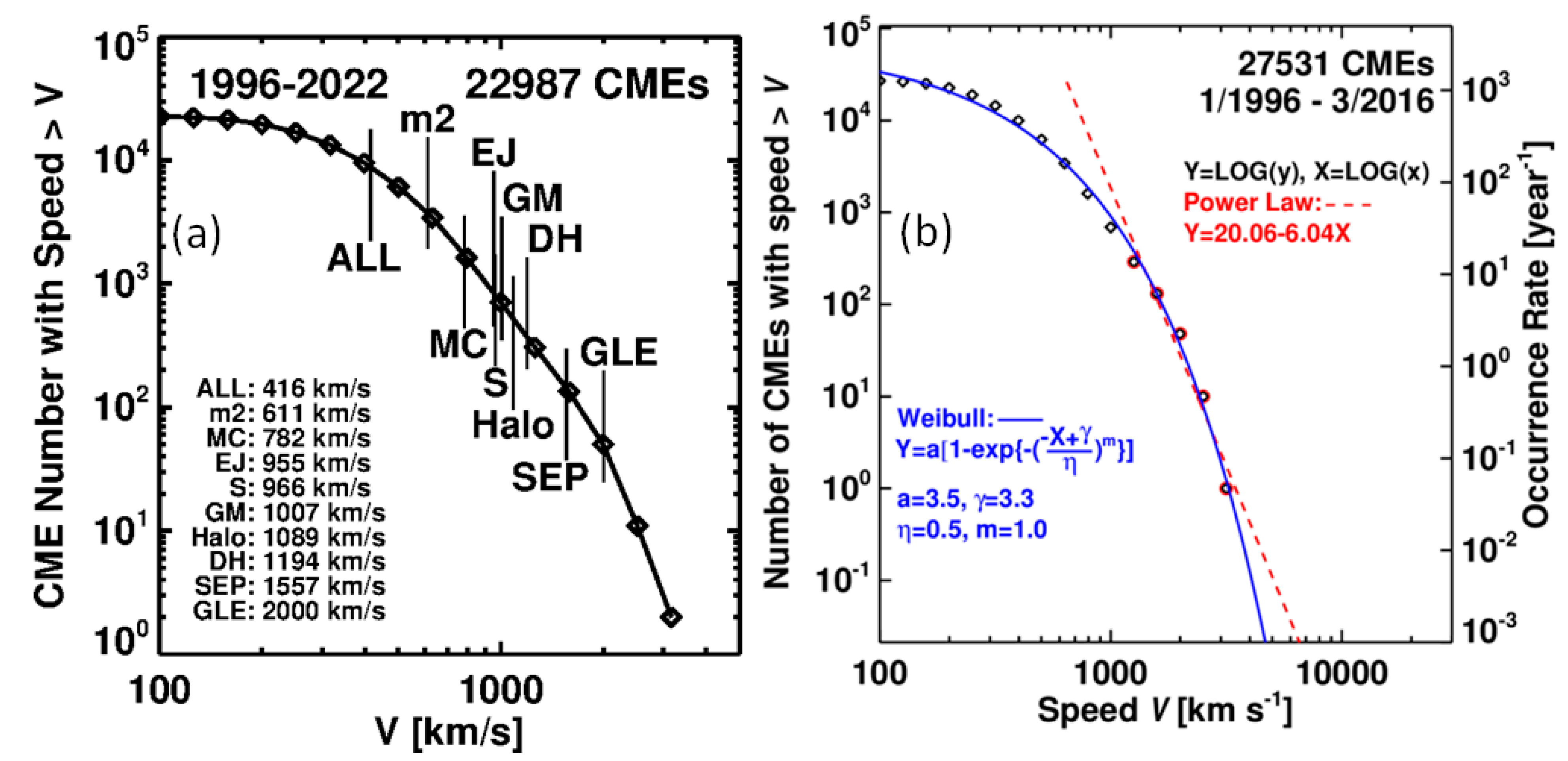
8.1. CME Rate–Sunspot Number Relationship
8.2. Solar Cycle Dependence of Space Weather Consequences
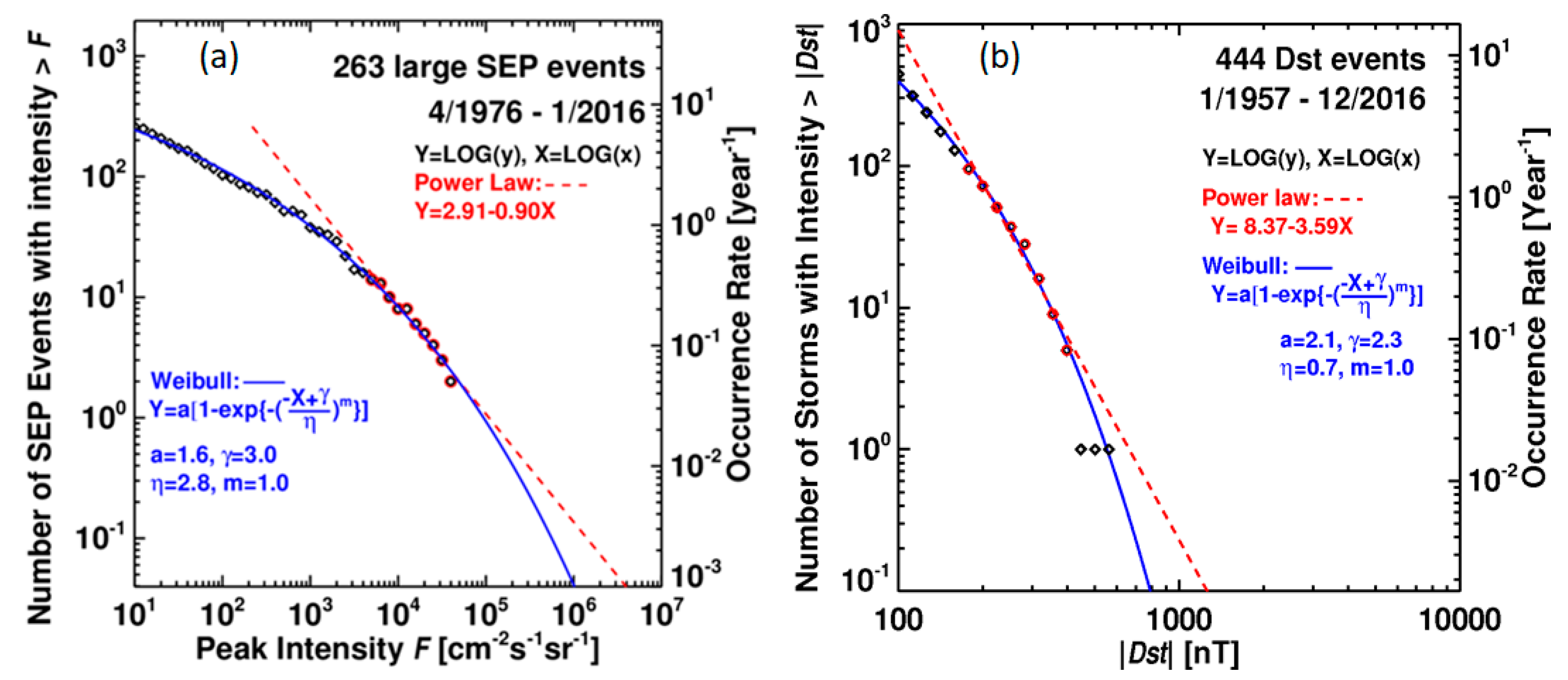
9. Extreme Space Weather Events
10. Concluding Remarks
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Schrijver, C.J.; Kauristie, K.; Aylward, A.D.; Denardini, C.M.; Gibson, S.E.; Glover, A.; Gopalswamy, N.; Grande, M.; Hapgood, M.; Heynderickx, D.; et al. Understanding space weather to shield society: A global road map for 2015–2025 commissioned by COSPAR and ILWS. Adv. Space Res. 2015, 55, 2745–2807. [Google Scholar] [CrossRef]
- Gopalswamy, N. History and development of coronal mass ejections as a key player in solar terrestrial relationship. Geosci. Lett. 2016, 3, 8–26. [Google Scholar] [CrossRef]
- Manchester, W.; Kilpua, E.K.J.; Liu, Y.D.; Lugaz, N.; Riley, P.; Török, T.; Vršnak, B. The Physical Processes of CME/ICME Evolution. Space Sci. Rev. 2017, 212, 1159–1219. [Google Scholar] [CrossRef]
- Georgieva, K.; Shiokawa, K. Variability of the Sun and Its Terrestrial Impacts. J. Atmos. Sol.-Terr. Phys. 2018, 180, 1–2. [Google Scholar] [CrossRef]
- Zhang, J.; Temmer, M.; Gopalswamy, N.; Malandraki, O.; Nitta, N.V.; Patsourakos, S.; Shen, F.; Vršnak, B.; Wang, Y.; Webb, D.; et al. Earth-affecting solar transients: A review of progresses in solar cycle 24. Prog. Earth Planet. Sci. 2021, 8, 56. [Google Scholar] [CrossRef]
- Temmer, M. Space weather: The solar perspective. Living Rev. Sol. Phys. 2021, 18, 4. [Google Scholar] [CrossRef]
- Shen, F.; Shen, C.; Xu, M.; Liu, Y.; Feng, X.; Wang, Y. Propagation characteristics of coronal mass ejections (CMEs) in the corona and interplanetary space. Rev. Mod. Plasma Phys. 2022, 6, 8. [Google Scholar] [CrossRef]
- Webb, D.F.; Howard, T.A. Coronal Mass Ejections: Observations. Living Rev. Sol. Phys. 2022, 9, 3. [Google Scholar] [CrossRef]
- Fisher, R.; Garcia, C.J.; Seagraves, P. On the coronal transient-eruptive prominence of 1980 August 5. Astrophys. J. 1981, 246, 161–164. [Google Scholar] [CrossRef]
- Hundhausen, A.J. Sizes and locations of coronal mass ejections: SMM observations from 1980 and 1984–1989. J. Geophys. Res. 1993, 98, 13177–13200. [Google Scholar] [CrossRef]
- Sheeley, N.R., Jr.; Hakala, W.N.; Wang, Y.-M. Detection of coronal mass ejection associated shock waves in the outer corona. J. Geophys. Res. 2000, 105, 5081–5092. [Google Scholar] [CrossRef]
- Vourlidas, A.; Wu, S.T.; Wang, A.H.; Subramanian, P.; Howard, R.A. Direct Detection of a Coronal Mass Ejection-Associated Shock in Large Angle and Spectrometric Coronagraph Experiment White-Light Images. Astrophys. J. 2003, 598, 1392–1402. [Google Scholar] [CrossRef]
- Gopalswamy, N. Coronal mass ejections and space weather. In Climate and Weather of the Sun-Earth System (CAWSES): Selected Papers from the 2007 Kyoto Symposium; Tsuda, T., Fujii, R., Shibata, K., Geller, M.A., Eds.; Terra Pub: Tokyo, Japan, 2009; pp. 77–120. [Google Scholar]
- Gopalswamy, N.; Thompson, W.; Davila, J.; Kaiser, M.L.; Yashiro, S.; Mäkelä, P.; Michalek, G.; Bougeret, J.-L.; Howard, R.A. Relation between Type II Bursts and CMEs Inferred from STEREO Observations. Sol. Phys. 2009, 259, 227–254. [Google Scholar] [CrossRef]
- Ontiveros, V.; Vourlidas, A. Quantitative Measurements of Coronal Mass Ejection-Driven Shocks from LASCO Observations. Astrophys. J. 2009, 693, 267–275. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Yashiro, S. The strength and radial profile of the coronal magnetic field from the standoff distance of a coronal mass ejection-driven shock. Astrophys. J. Lett. 2011, 736, L17. [Google Scholar] [CrossRef]
- Gopalswamy, N. Corona Mass Ejections: A Summary of Recent Results. In Proceedings of the 20th National Solar Physics Meeting, Papradno, Slovakia, 31 May–4 June 2010; pp. 108–130. [Google Scholar]
- Wang, C.B.; Chao, J.K.; Lin, C. Influence of the solar wind dynamic pressure on the decay and injection of the ring current. J. Geophys. Res. Earth Surf. 2003, 108. [Google Scholar] [CrossRef]
- Xie, H.; Gopalswamy, N.; Cyr, O.C.S.; Yashiro, S. Effects of solar wind dynamic pressure and preconditioning on large geomagnetic storms. Geophys. Res. Lett. 2008, 35. [Google Scholar] [CrossRef]
- Li, D.; Yao, S. Stronger Southward Magnetic Field and Geoeffectiveness of ICMEs Containing Prominence Materials Measured from 1998 to 2011. Astrophys. J. Lett. 2020, 891, 79. [Google Scholar] [CrossRef]
- Reames, D.V. Particle acceleration at the Sun and in the heliosphere. Space Sci. Rev. 1999, 90, 413–491. [Google Scholar] [CrossRef]
- Cliver, E.W.; Kahler, S.W.; Kazachenko, M.; Shimojo, M. The Disappearing Solar Filament of 2013 September 29 and Its Large Associated Proton Event: Implications for Particle Acceleration at the Sun. Astrophys. J. Lett. 2019, 877, 11. [Google Scholar] [CrossRef]
- Schmahl, E.; Hildner, E. Coronal mass-ejections-kinematics of the 19 December 1973 event. Sol. Phys. 1977, 55, 473–490. [Google Scholar] [CrossRef]
- Hanaoka, Y.; Shinkawa, T. Heating of Erupting Prominences Observed at 17 GHz. Astrophys. J. Lett. 1999, 510, 466–473. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Yashiro, S. Obscuration of Flare Emission by an Eruptive Prominence. Publ. Astron. Soc. Jpn. 2013, 65, 11–18. [Google Scholar] [CrossRef]
- Burlaga, L.; Fitzenreiter, R.; Lepping, R.; Ogilvie, K.; Szabo, A.; Lazarus, A.; Steinberg, J.; Gloeckler, G.; Howard, R.A.; Michels, D.; et al. A magnetic cloud containing prominence material: January 1997. J. Geophys. Res. Earth Surf. 1998, 103, 277–285. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Hanaoka, Y.; Kosugi, T.; Lepping, R.P.; Steinberg, J.T.; Plunkett, S.; Howard, R.A.; Thompson, B.J.; Gurman, J.; Ho, G.; et al. On the relationship between coronal mass ejections and magnetic clouds. Geophys. Res. Lett. 1998, 25, 2485–2488. [Google Scholar] [CrossRef]
- Reinard, A.A. Analysis of Interplanetary Coronal Mass Ejection Parameters as a Function of Energetics, Source Location, and Magnetic Structure. Astrophys. J. Lett. 2008, 682, 1289–1305. [Google Scholar] [CrossRef]
- Lepri, S.T.; Zurbuchen, T.H. Direct observational evidence of filament material within interplanetary coronal mass ejections. Astrophys. J. Lett. 2010, 723, L22–L27. [Google Scholar] [CrossRef]
- Gilbert, J.A.; Lepri, S.T.; Landi, E.; Zurbuchen, T.H. First measurements of the complete heavy-ion charge state distributions of c, o, and fe associated with interplanetary coronal mass ejections. Astrophys. J. Lett. 2012, 751, 20. [Google Scholar] [CrossRef]
- Gruesbeck, J.; Lepri, S.T.; Zurbuchen, T.H. Two-plasma model for low charge state interplanetary coronal mass ejection observations. Astrophys. J. Lett. 2012, 760, 141. [Google Scholar] [CrossRef]
- Sharma, R.; Srivastava, N. Presence of solar filament plasma detected in interplanetary coronal mass ejections by in situ spacecraft. J. Space Weather Space Clim. 2012, 2, A10. [Google Scholar] [CrossRef]
- Sharma, R.; Srivastava, N.; Chakrabarty, D.; Möstl, C.; Hu, Q. Interplanetary and geomagnetic consequences of 5 January 2005 CMEs associated with eruptive filaments. J. Geophys. Res. Space Phys. 2013, 118, 3954–3967. [Google Scholar] [CrossRef]
- Gopalswamy, N. The Dynamics of Eruptive Prominences. In Solar Prominences, Astrophysics and Space Science Library; Vial, J.-C., Engvold, O., Eds.; Springer International Publishing: Cham, Switzerland, 2015; Volume 415, pp. 381–409. [Google Scholar]
- Mishra, W.; Srivastava, N. Heliospheric tracking of enhanced density structures of the 6 October 2010 CME. J. Space Weather Space Clim. 2015, 5, A20. [Google Scholar] [CrossRef]
- Wang, J.; Feng, H.Q.; Zhao, G. Cold prominence materials detected within magnetic clouds during 1998–2007. Astron. Astrophys. 2018, 616, A41. [Google Scholar] [CrossRef]
- Kozyra, J.U.; Manchester, W.B.; Escoubet, C.P.; Lepri, S.T.; Liemohn, M.W.; Gonzalez, W.D.; Thomsen, M.W.; Tsurutani, B.T. Earth’s collision with a solar filament on 21 January 2005: Overview. J. Geophys. Res. Space Phys. 2013, 118, 5967–5978. [Google Scholar] [CrossRef]
- Manchester, W.B.; Kozyra, J.U.; Lepri, S.T.; Lavraud, B. Simulation of magnetic cloud erosion during propagation. J. Geophys. Res. Space Phys. 2014, 119, 5449–5464. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Mäkelä, P.; Akiyama, S.; Xie, H.; Yashiro, S.; Reinard, A.A. The Solar Connection of Enhanced Heavy Ion Charge States in the Interplanetary Medium: Implications for the Flux-Rope Structure of CMEs. Sol. Phys. 2013, 284, 17–46. [Google Scholar] [CrossRef]
- Yashiro, S.; Gopalswamy, N.; Mäkelä, P.; Akiyama, S. Post-Eruption Arcades and Interplanetary Coronal Mass Ejections. Sol. Phys. 2013, 284, 5–15. [Google Scholar] [CrossRef]
- Marubashi, K.; Akiyama, S.; Yashiro, S.; Gopalswamy, N.; Cho, K.-S.; Park, Y.-D. Geometrical Relationship between Interplanetary Flux Ropes and Their Solar Sources. Sol. Phys. 2015, 290, 1371–1397. [Google Scholar] [CrossRef]
- Yurchyshyn, V.; Yashiro, S.; Abramenko, V.; Wang, H.; Gopalswamy, N. Statistical Distributions of Speeds of Coronal Mass Ejections. Astrophys. J. Lett. 2005, 619, 599–603. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Yashiro, S.; Michalek, G.; Xie, H.; Mäkelä, P.; Vourlidas, A.; Howard, R. A Catalog of Halo Coronal Mass Ejections from SOHO. Sun Geosph. 2010, 5, 7–16. [Google Scholar]
- Wood, B.E.; Karovska, M.; Chen, J.; Brueckner, G.E.; Cook, J.W.; Howard, R.A. Comparison of Two Coronal Mass Ejections Observed by EIT and LASCO with a Model of an Erupting Magnetic Flux Rope. Astrophys. J. Lett. 1999, 512, 484–495. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Yashiro, S.; Kaiser, M.L.; Thompson, B.J.; Plunkett, S. Multi-wavelength Signatures of Coronal Mass Ejection. Proc. Nobeyama Symp. 1999, 479, 207–210. [Google Scholar]
- Gopalswamy, N.; Thompson, B. Early life of coronal mass ejections. J. Atmos. Sol.-Terr. Phys. 2000, 62, 1457–1469. [Google Scholar] [CrossRef]
- Zhang, J.; Dere, K.P.; Howard, R.A.; Kundu, M.R.; White, S.M. On the Temporal Relationship between Coronal Mass Ejections and Flares. Astrophys. J. Lett. 2001, 559, 452–462. [Google Scholar] [CrossRef]
- Zhang, J.; Dere, K.P. A Statistical Study of Main and Residual Accelerations of Coronal Mass Ejections. Astrophys. J. Lett. 2006, 649, 1100–1109. [Google Scholar] [CrossRef]
- Howard, R.A.; Sheeley, N.R., Jr.; Michels, D.J.; Koomen, M.J. Coronal mass ejections–1979-1981. J. Geophys. Res. 1985, 90, 8173. [Google Scholar] [CrossRef]
- Bein, B.M.; Berkebile-Stoiser, S.; Veronig, A.; Temmer, M.; Muhr, N.; Kienreich, I.W.; Utz, D.; Vrsnak, B. Impulsive acceleration of coronal mass ejections. I. statistics and coronal mass ejection source region characteristics. Astrophys. J. Lett. 2011, 738, 191. [Google Scholar] [CrossRef]
- Vourlidas, A.; Buzasi, D.; Howard, R.A.; Esfandiari, E. Mass and Energy Properties of LASCO CMEs in Solar Variability: From Core to Outer Frontiers (ESA SP-506); Wilson, A.A., Ed.; ESA Publications Division: Noordwijk, The Netherlands, 2002; p. 91. [Google Scholar]
- Gopalswamy, N. A Global Picture of CMEs in the Inner Heliosphere. In The Sun and the Heliosphere as an Integrated System; Poletto, G., Suess, S.T., Eds.; Kluwer Academic Publishers: Dordrecht, The Netherlands, 2004; Volume 201. [Google Scholar]
- Hundhausen, A.J.; Sawyer, C.B.; House, L.; Illing, R.M.E.; Wagner, W.J. Coronal mass ejections observed during the Solar Maximum Mission: Latitude distribution and rate of occurrence. J. Geophys. Res. Earth Surf. 1984, 89, 2639–2646. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Dal Lago, A.; Yashiro, S.; Akiyama, S. The Expansion and Radial Speeds of Coronal Mass Ejections. Cent. Eur. Astrophys. Bull. 2009, 33, 115–124. [Google Scholar]
- Gopalswamy, N.; Akiyama, S.; Yashiro, S.; Xie, H.; Mäkelä, P.; Michalek, G. Anomalous expansion of coronal mass ejections during solar cycle 24 and its space weather implications. Geophys. Res. Lett. 2014, 41, 2673–2680. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Aguilar-Rodriguez, E.; Yashiro, S.; Nunes, S.; Kaiser, M.L.; Howard, R.A. Type II radio bursts and energetic solar eruptions. J. Geophys. Res. 2005, 110, 12. [Google Scholar] [CrossRef]
- Michalek, G.; Gopalswamy, N.; Yashiro, S. Study of the Mass-loss Rate from the Sun. Astrophys. J. Lett. 2022, 930, 74. [Google Scholar] [CrossRef]
- Webb, D.F.; Howard, R.A. The solar cycle variation of coronal mass ejections and the solar wind mass flux. J. Geophys. Res. Earth Surf. 1994, 99, 4201–4220. [Google Scholar] [CrossRef]
- Mishra, W.; Srivastava, N.; Wang, Y.; Mirtoshev, Z.; Zhang, J.; Liu, R. Mass loss via solar wind and coronal mass ejections during solar cycles 23 and 24. Mon. Not. R. Astron. Soc. 2019, 486, 4671–4685. [Google Scholar] [CrossRef]
- Forbes, T.G. A review on the genesis of coronal mass ejections. J. Geophys. Res. Earth Surf. 2000, 105, 23153–23166. [Google Scholar] [CrossRef]
- Akiyama, S.; Yashiro, S.; Gopalswamy, N. The CME-productivity associated with flares from two active regions. Adv. Space Res. 2007, 39, 1467–1470. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, J. A Comparative Study between Eruptive X-Class Flares Associated with Coronal Mass Ejections and Confined X-Class Flares. Astrophys. J. Lett. 2007, 665, 1428–1438. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Akiyama, S.; Yashiro, S. Major solar flares without coronal mass ejections. IAU Symp. 2009, 257, 283–286. [Google Scholar] [CrossRef]
- Wild, J. Outbursts of radio noise from the sun. Vistas Astron. 1955, 1, 573–584. [Google Scholar] [CrossRef]
- Aubier, M.G. Transition between type I and type III bursts in closed or open magnetic field lines. In Radio Physics of the Sun, Proceedings of the Symposium, College Park, MD, USA, 7–10 August 1979, (A80-53501 24-92); Reidel Publishing Co.: Dordrecht, The Netherlands, 1980; pp. 363–368. [Google Scholar]
- Morioka, A.; Miyoshi, Y.; Masuda, S.; Tsuchiya, F.; Misawa, H.; Matsumoto, H.; Hashimoto, K.; Oya, H. Micro-Type III Radio Bursts. Astrophys. J. 2007, 657, 567–576. [Google Scholar] [CrossRef]
- Del Zanna, G.; Aulanier, G.; Klein, K.-L.; Török, T. A single picture for solar coronal outflows and radio noise storms. Astron. Astrophys. 2011, 526, 137–148. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Mäkelä, P.; Yashiro, S.; Akiyama, S.; Xie, H. The Relation between Type III Radio Storms and CIR Energetic Particles. In Proceedings of the 3rd URSI AT-AP-RASC, Gran Canaria, Spain, 29 May–3 June 2022. [Google Scholar]
- Nelson, G.J.; Melrose, D.B. Type II bursts. In Solar Radiophysics: Studies of Emission from the Sun at Metre Wavelengths (A87-13851 03-92); Cambridge University Press: Cambridge, MA, USA, 1985; pp. 333–359. [Google Scholar]
- Reid, H.A.S.; Ratcliffe, H. A review of solar type III radio bursts. Res. Astron. Astrophys. 2014, 14, 773–804. [Google Scholar] [CrossRef]
- Pick, M. Observations of radio continua and terminology. Sol. Phys. 1986, 104, 19–32. [Google Scholar] [CrossRef]
- Ginzburg, V.L.; Zhelezniakov, V.V. On the Possible Mechanisms of Sporadic Solar Radio Emission (Radiation in an Iso-tropic Plasma). Soviet Astron. 1958, 2, 653. [Google Scholar]
- Gopalswamy, N. Coronal Mass Ejections and Solar Radio Emissions. In Planetary Radio Emissions VII, Proceedings of the 7th International Workshop held at Graz, Austria, 15–17 September 2010; Rucker, H.O., Kurth, W.S., Louarn, P., Fischer, G., Eds.; Austrian Academy of Sciences Press: Vienna, Austria, 2011; pp. 325–342. [Google Scholar]
- Gopalswamy, N. Low-Frequency Radio Bursts and Space Weather. In Proceedings of the URSI Asia-Pacific Radio Science Conference, Seoul, Korea, 21–25 August 2016. [Google Scholar]
- Gopalswamy, N.; Mäkelä, P.; Yashiro, S. A Catalog of Type II radio bursts observed by Wind/WAVES and their Statistical Properties. Sun Geosph. 2019, 14, 111–121. [Google Scholar]
- Cremades, H.; Iglesias, F.A.; Cyr, O.C.S.; Xie, H.; Kaiser, M.L.; Gopalswamy, N. Low-Frequency Type-II Radio Detections and Coronagraph Data Employed to Describe and Forecast the Propagation of 71 CMEs/Shocks. Sol. Phys. 2015, 290, 2455–2478. [Google Scholar] [CrossRef]
- Cerruti, A.P.; Kintner, P.M.; Gary, D.E.; Lanzerotti, L.J.; de Paula, E.R.; Vo, H.B. Observed solar radio burst effects on GPS/Wide Area Augmentation System carrier-to-noise ratio. Space Weather 2006, 4, S10006. [Google Scholar] [CrossRef]
- Cliver, E.W.; Schrijver, C.J.; Shibata, K.; Usoskin, I.G. Extreme solar events. Living Rev. Sol. Phys. 2022, 19, 2. [Google Scholar] [CrossRef]
- Raulin, J.-P.; Bertoni, F.C.P.; Gavilán, H.R.; Guevara-Day, W.; Rodriguez, R.; Fernandez, G.; Correia, E.; Kaufmann, P.; Pacini, A.; Stekel, T.R.C.; et al. Solar flare detection sensitivity using the South America VLF Network (SAVNET). J. Geophys. Res. Earth Surf. 2010, 115. [Google Scholar] [CrossRef]
- Curto, J.J. Geomagnetic solar flare effects: A review. J. Space Weather Space Clim. 2020, 10, 27. [Google Scholar] [CrossRef]
- Sastri, J.H.; Murthy, B.S. On the relationships between sfe (crochet) and solar X-ray and microwave bursts. Sol. Phys. 1975, 41, 477–485. [Google Scholar] [CrossRef]
- Dungey, J.W. The steady state of the Chapman-Ferraro problem in two dimensions. J. Geophys. Res. Earth Surf. 1961, 66, 1043–1047. [Google Scholar] [CrossRef]
- Fairfield, D.H.; Cahill, L.J. Transition region magnetic field and polar magnetic disturbances. J. Geophys. Res. Earth Surf. 1966, 71, 155–169. [Google Scholar] [CrossRef]
- Nose, M.; Iyemori, T.; Sugiura, M.; Kamei, T. World Data Center for Geomagnetism, Kyoto Geomagnetic Dst Index. 2015. Available online: https://doi.org/10.17593/14515-74000 (accessed on 1 October 2022).
- Murayama, T. Coupling Function between Solar Wind Parameters and Geomagnetic Indices (Paper 2R0436). Rev. Geophys. Space Phys. 1982, 20, 623. [Google Scholar] [CrossRef]
- Gonzalez, W.D.; Tsurutani, B.T.; Gonzalez, A.L.C.; Smith, E.J.; Tang, F.; Akasofu, S.-I. Solar wind-magnetosphere coupling during intense magnetic storms (1978–1979). J. Geophys. Res. Earth Surf. 1989, 94, 8835–8851. [Google Scholar] [CrossRef]
- Wang, Y.; Shen, C.L.; Wang, S.; Ye, P.Z. An empirical formula relating the geomagnetic storm’s intensity to the inter-planetary parameters: −VBz and δt. Geophys. Res. Lett. 2003, 30, 2039. [Google Scholar] [CrossRef]
- Weigel, R.S. Solar wind density influence on geomagnetic storm intensity. J. Geophys. Res. Earth Surf. 2010, 115. [Google Scholar] [CrossRef]
- Cheng, L.-B.; Zhao, G.-M.; Le, M.-X. Sun-Earth connection event of super geomagnetic storm on 2001 March 31: The importance of solar wind density. Res. Astron Astrophys. 2020, 20, 36. [Google Scholar] [CrossRef]
- Zhao, M.X.; Le, G.M.; Li, Q.; Liu, G.A.; Mao, T. Dependence of Great Geomagnetic Storm (ΔSYM-H≤−200 nT) on Associated Solar Wind Parameters. Sol. Phys. 2021, 296, 66. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Yashiro, S.; Akiyama, S.; Xie, H.; Mäkelä, P.; Fok, M.-C.; Ferradas, C.P. What Is Unusual About the Third Largest Geomagnetic Storm of Solar Cycle 24? J. Geophys. Res. 2022, 127, e30404. [Google Scholar] [CrossRef]
- Wilson, R.M. Geomagnetic response to magnetic clouds. Planet. Space Sci. 1987, 35, 329–335. [Google Scholar] [CrossRef]
- Zhang, G.; Burlaga, L.F. Magnetic clouds, geomagnetic disturbances, and cosmic ray decreases. J. Geophys. Res. 1988, 93, 2511–2518. [Google Scholar] [CrossRef]
- Gonzalez, W.D.; Tsurutani, B.T. Criteria of interplanetary parameters causing intense magnetic storms (Dst <−100 nT). Planet. Space Sci. 1987, 35, 1101–1109. [Google Scholar] [CrossRef]
- Gosling, J.T.; McComas, D.J. Field line draping about fast coronal mass ejecta: A source of strong out-of-the-ecliptic in-terplanetary magnetic fields. Geophys. Res. Lett. 1987, 14, 355–358. [Google Scholar] [CrossRef]
- Tsurutani, B.T.; Gonzalez, W.D.; Tang, F.; Akasofu, S.I.; Smith, E.J. Origin of interplanetary southward magnetic fields responsible for major magnetic storms near solar maximum (1978–1979). J. Geophys. Res. Earth Surf. 1988, 93, 8519–8531. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Xie, H.; Mäkelä, P.; Akiyama, S.; Yashiro, S.; Kaiser, M.L.; Howard, R.A.; Bougeret, J.-L. Interplanetary Shocks Lacking Type II Radio Bursts. Astrophys. J. 2010, 710, 1111–1126. [Google Scholar] [CrossRef]
- Kilpua, E.; Koskinen, H.E.J.; Pulkkinen, T.I. Coronal mass ejections and their sheath regions in interplanetary space. Living Rev. Sol. Phys. 2017, 14, 1–83. [Google Scholar] [CrossRef]
- Cranmer, S.R. Coronal Holes. Living Rev. Sol. Phys. 2009, 6, 3. [Google Scholar] [CrossRef]
- Nolte, J.T.; Krieger, A.S.; Timothy, A.F.; Gold, R.E.; Roelof, E.C.; Vaiana, G.; Lazarus, A.J.; Sullivan, J.D.; McIntosh, P.S. Coronal holes as sources of solar wind. Sol. Phys. 1976, 46, 303–322. [Google Scholar] [CrossRef]
- Vršnak, B.; Temmer, M.; Veronig, A.M. Coronal Holes and Solar Wind High-Speed Streams: I. Forecasting the Solar Wind Parameters. Sol. Phys. 2007, 240, 315–330. [Google Scholar] [CrossRef]
- Akiyama, S.; Gopalswamy, N.; Yashiro, S.; Mäkelä, P. A Study of Coronal Holes Observed by SoHO/EIT and the No-beyama Radioheliograph. Pub. Astron. Soc. Japan. 2013, 65, 15–25. [Google Scholar] [CrossRef]
- Fujiki, K.; Tokumaru, M.; Hayashi, K.; Satonaka, D.; Hakamada, K. Long-term trend of solar coronal hole distribution from 1975 to 2014. Astrophys. J. Lett. 2016, 827, L41. [Google Scholar] [CrossRef]
- Hofmeister, S.; Utz, D.; Heinemann, S.; Veronig, A.; Temmer, M. The photospheric structure of coronal holes: Magnetic elements. Catalyzing Solar Connections. In Proceedings of the 2018 SDO Science Workshop, Ghent, Belgium, 29 October–2 November 2018; p. 129. [Google Scholar]
- Heinemann, S.G.; Jerčić, V.; Temmer, M.; Hofmeister, S.J.; Dumbović, M.; Vennerstrom, S.; Verbanac, G.; Veronig, A.M. A statistical study of the long-term evolution of coronal hole properties as observed by SDO. Astron. Astrophys. 2020, 638, 68–78. [Google Scholar] [CrossRef]
- Wilcox, J.M.; Ness, N.F. Quasi-stationary corotating structure in the interplanetary medium. J. Geophys. Res. Earth Surf. 1965, 70, 5793–5805. [Google Scholar] [CrossRef]
- Belcher, J.W.; Davis, L., Jr. Large-amplitude Alfvén waves in the interplanetary medium. J. Geophys. Res. 1971, 76, 3534. [Google Scholar] [CrossRef]
- Gosling, J.T.; Hundhausen, A.J.; Pizzo, V.; Asbridge, J.R. Compressions and rarefactions in the solar wind: Vela 3. J. Geophys. Res. Earth Surf. 1972, 77, 5442–5454. [Google Scholar] [CrossRef]
- Smith, E.J.; Wolfe, J.H. Observations of interaction regions and corotating shocks between one and five AU: Pioneers 10 and 11. Geophys. Res. Lett. 1976, 3, 137–140. [Google Scholar] [CrossRef]
- Barnes, C.W.; Simpson, J.A. Evidence for interplanetary acceleration of nucleons in corotating interaction regions. Astrophys. J. Lett. 1976, 210, L91. [Google Scholar] [CrossRef]
- Jian, L.K.; Luhmann, J.G.; Russell, C.T.; Galvin, A.B. Solar Terrestrial Relations Observatory (STEREO) Observations of Stream Interaction Regions in 2007–2016: Relationship with Heliospheric Current Sheets, Solar Cycle Variations, and Dual Observations. Sol. Phys. 2019, 294, 31–57. [Google Scholar] [CrossRef]
- Maghradze, A.D.; Chargeishvili, B.B.; Japaridze, D.R.; Oghrapishvili, N.B. Long-term variation of coronal holes latitudinal distribution. Mon. Not. R. Astron. Soc. 2022, 511, 5217–5224. [Google Scholar] [CrossRef]
- Legrand, J.-P.; Simon, P.A. Solar cycle and geomagnetic activity: A review for geophysicists. Part 1. The contributions to geomagnetic activity of shock waves and of the solar wind. Annales Geophysicae. 1989, 7, 565–593. [Google Scholar]
- Hajra, R.; Sunny, J.V. Corotating Interaction Regions during Solar Cycle 24: A Study on Characteristics and Geoeffectiveness. Sol. Phys. 2022, 297, 30. [Google Scholar] [CrossRef]
- Gopalswamy, N. Solar connections of geoeffective magnetic structures. J. Atmos. Solar-Terr. Phys. 2008, 70, 2078–2100. [Google Scholar] [CrossRef]
- Kamide, Y.; Yokoyama, N.; Gonzalez, W.D.; Tsurutani, B.T.; Daglis, I.; Brekke, A.; Masuda, S. Two-step development of geomagnetic storms. J. Geophys. Res. Earth Surf. 1998, 103, 6917–6921. [Google Scholar] [CrossRef]
- Zhang, J.; Richardson, I.G.; Webb, D.F. Interplanetary origin of multiple-dip geomagnetic storms. J. Geophys. Res. Earth Surf. 2008, 113. [Google Scholar] [CrossRef]
- Bothmer, V.; Schwenn, R. The structure and origin of magnetic clouds in the solar wind. Annales Geophysicae. 1998, 16, 1–24. [Google Scholar] [CrossRef]
- Mulligan, T.; Russell, C.T.; Luhmann, J.G. Solar cycle evolution of the structure of magnetic clouds in the inner helio-sphere. Geophys. Res. Lett. 1998, 25, 2959–2962. [Google Scholar] [CrossRef]
- Echer, E.; Alves, M.; Gonzalez, W. A statistical study of magnetic cloud parameters and geoeffectiveness. J. Atmos. Sol.-Terr. Phys. 2005, 67, 839–852. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Akiyama, S.; Yashiro, S.; Michalek, G.; Lepping, R. Solar sources and geospace consequences of interplanetary magnetic clouds observed during solar cycle 23. J. Atmos. Sol.-Terr. Phys. 2008, 70, 245–253. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Yashiro, S.; Xie, H.; Akiyama, S.; Mäkelä, P. Properties and geoeffectiveness of magnetic clouds during solar cycles 23 and 24. J. Geophys. Res. Space Phys. 2015, 120, 9221–9245. [Google Scholar] [CrossRef]
- Li, Y.; Luhmann, J.G.; Lynch, B.; Kilpua, E.K.J. Cyclic Reversal of Magnetic Cloud Poloidal Field. Sol. Phys. 2011, 270, 331–346. [Google Scholar] [CrossRef]
- Li, Y.; Luhmann, J.G.; Lynch, B.J. Magnetic Clouds: Solar Cycle Dependence, Sources, and Geomagnetic Impacts. Sol. Phys. 2018, 293, 1–19. [Google Scholar] [CrossRef] [PubMed]
- Fenrich, F.R.; Luhmann, J.G. Geomagnetic response to magnetic clouds of different polarity. Geophys. Res. Lett. 1998, 25, 2999–3002. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Yashiro, S.; Liu, Y.; Michalek, G.; Vourlidas, A.; Kaiser, M.L.; Howard, R.A. Coronal mass ejections and other extreme characteristics of the 2003 October-November solar eruptions. J. Geophys. Res. Earth Surf. 2005, 110. [Google Scholar] [CrossRef]
- Yurchyshyn, V.; Wang, H.; Goode, P.R.; Deng, Y. Orientation of the Magnetic Fields in Interplanetary Flux Ropes and Solar Filaments. Astrophys. J. Lett. 2001, 563, 381–388. [Google Scholar] [CrossRef]
- Qiu, J.; Yurchyshyn, V. Magnetic Reconnection Flux and Coronal Mass Ejection Velocity. Astrophys. J. Lett. 2005, 634, L121–L124. [Google Scholar] [CrossRef]
- Qiu, J.; Hu, Q.; Howard, T.A.; Yurchyshyn, V. On the Magnetic Flux Budget in Low-Corona Magnetic Reconnection and Interplanetary Coronal Mass Ejections. Astrophys. J. Lett. 2007, 659, 758–772. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Akiyama, S.; Yashiro, S.; Xie, H. Coronal flux ropes and their interplanetary counterparts. J. Atmos. Sol.-Terr. Phys. 2018, 180, 35–45. [Google Scholar] [CrossRef]
- Gonzalez, W.D.; de Gonzalez, A.L.C.; Dal Lago, A.; Tsurutani, B.T.; Arballo, J.K.; Lakhina, G.K.; Buti, B.; Ho, C.M.; Wu, S.-T. Magnetic cloud field intensities and solar wind velocities. Geophys. Res. Lett. 1998, 25, 963–966. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Yashiro, S.; Akiyama, S. Geoeffectiveness of halo coronal mass ejections. J. Geophys. Res. Earth Surf. 2007, 112. [Google Scholar] [CrossRef]
- Newton, H.W. Solar Flares and Magnetic Storms. Mon. Not. R. Astron. Soc. 1943, 103, 244–257. [Google Scholar] [CrossRef]
- Wang, Y.; Chen, C.; Gui, B.; Shen, C.; Ye, P.; Wang, S. Statistical study of coronal mass ejection source locations: Understanding CMEs viewed in coronagraphs. J. Geophys. Res. 2011, 116, A04104. [Google Scholar] [CrossRef][Green Version]
- Gosling, J.T.; Thomsen, M.F.; Bame, S.J.; Zwickl, R.D. The eastward deflection of fast coronal mass ejecta in interplanetary space. J. Geophys. Res. Earth Surf. 1987, 92, 12399–12406. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Xie, H.; Akiyama, S.; Makela, P.; Yashiro, S.; Michalek, G. The peculiar behavior of halo coronal mass ejections in solar cycle 24. Astrophys. J. Lett. 2015, 804, L23. [Google Scholar] [CrossRef]
- Huttunen, K.E.J.; Koskinen, H.; Pulkkinen, T.; Pulkkinen, A.; Palmroth, M.; Reeves, G.; Singer, H.J. April 2000 magnetic storm: Solar wind driver and magnetospheric response. J. Geophys. Res. Earth Surf. 2002, 107, 1440. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Yashiro, S.; Xie, H.; Akiyama, S.; Mäakelä, P. Large Geomagnetic Storms Associated with Limb Halo Coronal Mass Ejections; Ip, W.-H., Duldig, M., Eds.; World Scientific: Singapore, 2010; pp. 71–82. [Google Scholar]
- Cid, C.; Cremades, H.; Aran, A.; Mandrini, C.; Sanahuja, B.; Schmieder, B.; Menvielle, M.; Rodriguez, L.; Saiz, E.; Cerrato, Y.; et al. Can a halo CME from the limb be geoeffective? J. Geophys. Res. 2012, 117, A11102. [Google Scholar] [CrossRef]
- Zhang, J.; Richardson, I.G.; Webb, D.F.; Gopalswamy, N.; Huttunen, E.; Kasper, J.C.; Nitta, N.V.; Poomvises, W.; Thompson, B.J.; Wu, C.-C.; et al. Solar and interplanetary sources of major geomagnetic storms (Dst ≤ −100 nT) during 1996–2005. J. Geophys. Res. 2007, 112, A10102. [Google Scholar]
- Chi, Y.; Shen, C.; Luo, B.; Wang, Y.; Xu, M. Geoeffectiveness of Stream Interaction Regions From 1995 to 2016. J. Geophys. Res. 2018, 16, 1960–1971. [Google Scholar] [CrossRef]
- Yermolaev, Y.I.; Nikolaeva, N.S.; Lodkina, I.G.; Yermolaev, M.Y. Geoeffectiveness and efficiency of CIR, sheath, and ICME in generation of magnetic storms. J. Geophys. Res. Earth Surf. 2012, 117, A00L07. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Akiyama, S.; Yashiro, S.; Xie, H.; Makela, P.; Michalek, G. The Mild Space Weather in Solar Cycle 24. In Proceedings of the 14th International Ionospheric Effects Symposium on ’Bridging the Gap between Applications and Research Involving Ionospheric and Space Weather Disciplines, Alexandria, VA, USA, 12–14 May 2015. [Google Scholar]
- Gopalswamy, N.; Mäkelä, P.; Yashiro, S.; Akiyama, S.; Xie, H. Solar activity and space weather. J. Physics: Conf. Ser. 2022, 2214, 012021. [Google Scholar] [CrossRef]
- Nakagawa, Y.; Nozawa, S.; Shinbori, A. Relationship between the low-latitude coronal hole area, solar wind velocity, and geomagnetic activity during solar cycles 23 and 24. Earth, Planets Space 2019, 71, 24. [Google Scholar] [CrossRef]
- Grandin, M.; Aikio, A.T.; Kozlovsky, A. Properties and Geoeffectiveness of Solar Wind High-Speed Streams and Stream Interaction Regions During Solar Cycles 23 and 24. J. Geophys. Res. Space Phys. 2019, 124, 3871–3892. [Google Scholar] [CrossRef]
- Forbush, S.E. Three Unusual Cosmic-Ray Increases Possibly Due to Charged Particles from the Sun. Phys. Rev. (Ser. I) 1946, 70, 771–772. [Google Scholar] [CrossRef]
- Payne-Scott, R.; Yabsley, D.E.; Bolton, J.G. Relative Times of Arrival of Bursts of Solar Noise on Different Radio Fre-quencies. Nature 1947, 160, 256–257. [Google Scholar] [CrossRef] [PubMed]
- Wild, J.P.; McCready, L.L. Observations of the Spectrum of High-Intensity Solar Radiation at Metre Wavelengths. I. The Apparatus and Spectral Types of Solar Burst Observed. Aust. J. Scientific Res. A. 1950, 3, 387. [Google Scholar]
- Uchida, Y. On the Exciters of Type II and Type III Solar Radio Bursts. Publ. Astron. Soc. Jpn. 1960, 12, 376. [Google Scholar]
- Lin, R.P. The Emission and Propagation of 40 keV Solar Flare Electrons. I: The Relationship of 40 keV Electron to Energetic Proton and Relativistic Electron Emission by the Sun. Sol. Phys. 1970, 12, 266–303. [Google Scholar] [CrossRef]
- Rao, U.R.; McCracken, K.G.; Bukata, R.P. Cosmic-ray propagation processes: 2. The energetic storm-particle event. J. Geophys. Res. Earth Surf. 1967, 72, 4325–4341. [Google Scholar] [CrossRef]
- Kahler, S.W.; Hildner, E.; Van Hollebeke, M.A.I. Prompt solar proton events and coronal mass ejections. Sol. Phys. 1978, 57, 429–443. [Google Scholar] [CrossRef]
- Cliver, E.W. Flare versus shock acceleration of high energy protons in solar energetic particle events. Astrophys J. 2016, 832, 128. [Google Scholar] [CrossRef]
- Reames, D.V. The Two Sources of Solar Energetic Particles. Space Sci. Rev. 2013, 175, 53–92. [Google Scholar] [CrossRef]
- Desai, M.; Giacalone, J. Large gradual solar energetic particle events. Living Rev. Sol. Phys. 2016, 13, 3–135. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Yashiro, S.; Thakur, N.; Mäkelä, P.; Xie, H.; Akiyama, S. The 2012 July 23 Backside Eruption: An Ex-treme Energetic Particle Event? Astrophys. J. 2016, 833, 216. [Google Scholar] [CrossRef]
- Siddiqi, A.A. Beyond Earth: A Chronicle of Deep Space Exploration, 1958–2016; NASA History Program Office: Washington, DC, USA, 2018. [Google Scholar]
- Zeitlin, C.; Boynton, W.; Mitrofanov, I.; Hassler, D.; Atwell, W.; Cleghorn, T.F.; Cucinotta, F.A.; Dayeh, M.; Desai, M.; Guetersloh, S.B.; et al. Mars Odyssey measurements of galactic cosmic rays and solar particles in Mars orbit, 2002–2008. Space Weather 2010, 8, S00E06. [Google Scholar] [CrossRef]
- Marvin, D.C.; Gorney, D.J. Solar proton events of 1989–Effects on spacecraft solar arrays. J. Spacecr. Rocket. 1991, 28, 713–719. [Google Scholar] [CrossRef]
- Iucci, N.; Levitin, A.E.; Belov, A.V.; Eroshenko, E.A.; Ptitsyna, N.G.; Villoresi, G.; Chizhenkov, G.V.; Dorman, L.I.; Gromova, L.I.; Parisi, M.; et al. Space weather conditions and spacecraft anomalies in different orbits. Space Weather 2005, 3, 01001. [Google Scholar] [CrossRef]
- Jaynes, A.N.; Baker, D.N.; Singer, H.J.; Rodriguez, J.V.; Loto’Aniu, T.M.; Ali, A.F.; Elkington, S.R.; Li, X.; Kanekal, S.G.; Claudepierre, S.G.; et al. Source and seed populations for relativistic electrons: Their roles in radiation belt changes. J. Geophys. Res. Space Phys. 2015, 120, 7240–7254. [Google Scholar] [CrossRef]
- Borovsky, J.E.; Denton, M. Differences between CME-driven storms and CIR-driven storms. J. Geophys. Res. Earth Surf. 2006, 111, A07S08. [Google Scholar] [CrossRef]
- Hands, A.D.P.; Ryden, K.A.; Meredith, N.P.; Glauert, S.A.; Horne, R.B. Radiation Effects on Satellites During Extreme Space Weather Events. Space Weather 2018, 16, 1216–1226. [Google Scholar] [CrossRef]
- Hajra, R.; Echer, E.; Tsurutani, B.T.; Gonzalez, W.D. Solar cycle dependence of High-Intensity Long-Duration Continuous AE Activity (HILDCAA) events, relativistic electron predictors? J. Geophys. Res. Space Phys. 2013, 118, 5626–5638. [Google Scholar] [CrossRef]
- Feynman, J.; Gabriel, S.B. On space weather consequences and predictions. J. Geophys. Res. Earth Surf. 2000, 105, 10543–10564. [Google Scholar] [CrossRef]
- Albert, J.; Ginet, G.P.; Gussenhoven, M.S. CRRES observations of radiation belt protons: 1. Data overview and steady state radial diffusion. J. Geophys. Res. Earth Surf. 1998, 103, 9261–9273. [Google Scholar] [CrossRef]
- Hudson, M.K.; Kress, B.T.; Mazur, J.E.; Perry, K.L.; Slocum, P.L. 3D modeling of shock-induced trapping of solar energetic particles in the Earth’s magnetosphere. J. Atmos. Sol.-Terr. Phys. 2004, 66, 1389–1397. [Google Scholar] [CrossRef]
- Zhang, K.; Li, X.; Zhao, H.; Schiller, Q.; Khoo, L.; Xiang, Z.; Selesnick, R.; Temerin, M.A.; Sauvaud, J.A. Cosmic Ray Albedo Neutron Decay (CRAND) as a Source of Inner Belt Electrons: Energy Spectrum Study. Geophys. Res. Lett. 2019, 46, 544–552. [Google Scholar] [CrossRef]
- Mironova, I.A.; Aplin, K.L.; Arnold, F.; Bazilevskaya, G.A.; Harrison, R.G.; Krivolutsky, A.A.; Nicoll, K.A.; Rozanov, E.V.; Turunen, E.; Usoskin, I.G. Energetic Particle Influence on the Earth’s Atmosphere. Space Sci. Rev. 2015, 194, 1–96. [Google Scholar] [CrossRef]
- Jackman, C.H.; Deland, M.T.; Labow, G.J.; Fleming, E.L.; López-Puertas, M. Satellite Measurements of Middle Atmos-pheric Impacts by Solar Proton Events in Solar Cycle 23. Space Sci. Rev. 2006, 125, 381–391. [Google Scholar] [CrossRef]
- Shea, M.A.; Smart, D.F. Space Weather and the Ground-Level Solar Proton Events of the 23rd Solar Cycle. Space Sci. Rev. 2012, 171, 161–188. [Google Scholar] [CrossRef]
- Boteler, D.; Pirjola, R.; Nevanlinna, H. The effects of geomagnetic disturbances on electrical systems at the Earth’s surface. Adv. Space Res. 1998, 22, 17–27. [Google Scholar] [CrossRef]
- Pulkkinen, A.; Lindahl, S.; Viljanen, A.; Pirjola, R. Geomagnetic storm of 29–31 October 2003: Geomagnetically induced currents and their relation to problems in the Swedish high-voltage power transmission system. Space Weather 2005, 13, S08C03. [Google Scholar] [CrossRef]
- Kappenman, J.G. Storm sudden commencement events and the associated geomagnetically induced current risks to ground-based systems at low-latitude and midlatitude locations. Space Weather 2003, 1, 1016. [Google Scholar] [CrossRef]
- Belakhovsky, V.; Pilipenko, V.; Engebretson, M.; Sakharov, Y.; Selivanov, V. Impulsive disturbances of the geomagnetic field as a cause of induced currents of electric power lines. J. Space Weather Space Clim. 2019, 9, A18. [Google Scholar] [CrossRef]
- Pandya, M.; Bhaskara, V.; Ebihara, Y.; Kanekal, S.G.; Baker, D.N. Variation of Radiation Belt Electron Flux During CME- and CIR-Driven Geomagnetic Storms: Van Allen Probes Observations. J. Geophys. Res. Space Phys. 2019, 124, 6524. [Google Scholar] [CrossRef]
- Pulkkinen, A.; Bernabeu, E.; Eichner, J.; Beggan, C.; Thomson, A.W.P. Generation of 100-year geomagnetically induced current scenarios. Space Weather 2012, 10, S04003. [Google Scholar] [CrossRef]
- Ngwira, C.M.; Pulkkinen, A.; Wilder, F.D.; Crowley, G. Extended study of extreme geoelectric field event scenarios for geomagnetically induced current applications. Space Weather 2013, 11, 121–131. [Google Scholar] [CrossRef]
- Fiori, R.A.D.; Boteler, D.H.; Gillies, D.M. Assessment of GIC risk due to geomagnetic sudden commencements and identification of the current systems responsible. Space Weather 2014, 12, 76–91. [Google Scholar] [CrossRef]
- Marshall, R.A.; Dalzell, M.; Waters, C.L.; Goldthorpe, P.; Smith, A.E. Geomagnetically induced currents in the New Zealand power network. Space Weather 2012, 10, S08003. [Google Scholar] [CrossRef]
- Carter, B.A.; Yizengaw, E.; Pradipta, R.; Halford, A.J.; Norman, R.; Zhang, K. Interplanetary shocks and the resulting geomagnetically induced currents at the equator. Geophys. Res. Lett. 2015, 42, 6554–6559. [Google Scholar] [CrossRef]
- Hathaway, D.H. The Solar Cycle. Living Rev. Sol. Phys. 2015, 12, 4. [Google Scholar]
- Gopalswamy, N.; Lara, A.; Yashiro, S.; Nunes, S.M.; Howard, R.A. Coronal mass ejection activity during solar cycle 23. In Solar Variability as an Input to the Earth’s Environment. In Proceedings of the International Solar Cycle Studies (ISCS) Symposium, Tatranská Lomnica, Slovakia, 23–28 June 2003; Wilson, A., Ed.; ESA Publications Division: Noordwijk, The Netherlands, 2003; pp. 403–414. [Google Scholar]
- Gopalswamy, N.; Akiyama, S.; Yashiro, S.; Mäkelä, P. Coronal Mass Ejections from Sunspot and Non-Sunspot Regions. In Magnetic Coupling between the Interior and Atmosphere of the Sun; Hasan, S.S., Rutten, R.J., Eds.; Astrophysics and Space Science Proc; Springer: Berlin/Heidelberg, Germany, 2010; pp. 289–307. [Google Scholar]
- Gopalswamy, N.; Mäkelä, P.; Yashiro, S.; Akiyama, S. Long-term solar activity studies using microwave imaging ob-servations and prediction for cycle 25. J. Atmos. Sol.-Terr. Phys. 2018, 176, 26–33. [Google Scholar] [CrossRef]
- Gopalswamy, N.; Shimojo, M.; Lu, W.; Yashiro, S.; Shibasaki, K.; Howard, R.A. Prominence Eruptions and Coronal Mass Ejection: A Statistical Study Using Microwave Observations. Astrophys. J. Lett. 2003, 586, 562–578. [Google Scholar] [CrossRef]
- Mewaldt, R.; Li, G.; Hu, J.; Cohen, C. What is Causing the Deficit of High-Energy Solar Particles in Solar Cycle 24? In Proceedings of the 35th International Cosmic Ray Conference, Bexco, Busan, Korea, 10–20 July 2017; Volume 301, p. 111. [Google Scholar]
- Shea, M.A.; Smart, D.F. March 1991 Solar-Terrestrial Phenomena and Related Technological Consequences. In Proceedings of the 23rd International Cosmic Ray Conference, University of Calgary, Alberta, Canada, 19–30 July 1993; Leahy, D.A., Hicks, R.B., Venkatesan, D., Eds.; World Scientific: Singapore, 1993; Volume 3, p. 739. [Google Scholar]
- Miyake, F.; Nagaya, K.; Masuda, K.; Nakamura, T. A signature of cosmic-ray increase in ad 774–775 from tree rings in Japan. Nature 2012, 486, 240–242. [Google Scholar] [CrossRef] [PubMed]
- Gopalswamy, N. Chapter 2–Extreme Solar Eruptions and their Space Weather Consequences. In Extreme Events in Geospace; Buzulukova, N., Ed.; Elsevier: Amsterdam, The Netherlands, 2018; pp. 37–63. [Google Scholar]
- Siscoe, G.; Crooker, N.; Clauer, C. Dst of the Carrington storm of 1859. Adv. Space Res. 2006, 38, 173–179. [Google Scholar] [CrossRef]
- Tsurutani, B.T.; Gonzalez, W.D.; Lakhina, G.; Alex, S. The extreme magnetic storm of 1–2 September 1859. J. Geophys. Res. Earth Surf. 2003, 108. [Google Scholar] [CrossRef]
- Antia, H.M.; Chitre, S.M.; Thompson, M.J. Helioseismic search for magnetic field in the solar interior. J. Astrophys. Astron. 2000, 21, 343–347. [Google Scholar] [CrossRef]
- Daglis, I.A.; Chang, L.C.; Dasso, S.; Gopalswamy, N.; Khabarova, O.V.; Kilpua, E.; Lopez, R.; Marsh, D.; Matthes, K.; Nandy, D.; et al. Predictability of variable solar-terrestrial coupling. Ann. Geophys. 2021, 39, 1013. [Google Scholar] [CrossRef]
- Leka, K.D.; Park, S.-H.; Kusano, K.; Andries, J.; Barnes, G.; Bingham, S.; Bloomfield, D.S.; McCloskey, A.E.; Delouille, V.; Falconer, D.; et al. A Comparison of Flare Forecasting Methods. II. Benchmarks, Metrics, and Performance Results for Operational Solar Flare Forecasting Systems. Astrophys. J. Suppl. Ser. 2019, 243, 36. [Google Scholar] [CrossRef]
- Bain, H.M.; Steenburgh, R.A.; Onsager, T.G.; Stitely, E.M. A Summary of National Oceanic and Atmospheric Admin-istration Space Weather Prediction Center Proton Event Forecast Performance and Skill. Space Weather 2021, 19, e02670. [Google Scholar] [CrossRef]
- Malandraki, O.E.; Crosby, N.B. Solar Energetic Particles and Space Weather: Science and Applications. In Solar Particle Radiation Storms Forecasting and Analysis; Malandraki, O.E., Crosby, N.B., Eds.; Astrophysics and Space Science Library; Springer: Berlin/Heidelberg, Germany, 2018; Volume 444, pp. 1–26. [Google Scholar]
- Aminalragia-Giamini, S.; Raptis, S.; Anastasiadis, A.; Tsigkanos, A.; Sandberg, I.; Papaioannou, A.; Papadimitriou, C.; Jiggens, P.; Aran, A.; Daglis, I.A. Solar Energetic Particle Event occurrence prediction using Solar Flare Soft X-ray measurements and Machine Learning. J. Space Weather Space Clim. 2021, 11, 59. [Google Scholar] [CrossRef]
- Thakur, N.; Gopalswamy, N.; Mäkelä, P.; Akiyama, S.; Yashiro, S.; Xie, H. Two Exceptions in the Large SEP Events of Solar Cycles 23 and 24. Sol. Phys. 2016, 291, 513–530. [Google Scholar] [CrossRef]
- Belov, A.; Shlyk, N.; Abunina, M.; Abunin, A.; Papaioannou, A. Arrival Time Estimates of Earth-Directed CME-Driven Shocks. Universe 2022, 8, 327. [Google Scholar] [CrossRef]
- Moore, R.L.; Sterling, A.C.; Gary, G.A.; Cirtain, J.W.; Falconer, D.A. Observed Aspects of Reconnection in Solar Eruptions. Space Sci. Rev. 2011, 160, 73. [Google Scholar] [CrossRef]
- Kusano, K.; Iju, T.; Bamba, Y.; Inoue, S. A physics-based method that can predict imminent large solar flares. Science 2020, 369, 587–591. [Google Scholar] [CrossRef] [PubMed]
- Toriumi, S. Flux emergence and generation of flare-productive active regions. Adv. Space Res. 2022, 70, 1549–1561. [Google Scholar] [CrossRef]
- Titov, V.S.; Downs, C.; Török, T.; Linker, J.A. A Magnetogram-matching Method for Energizing Magnetic Flux Ropes Toward Eruption. Astrophys. J. Lett. 2022, 936, 121. [Google Scholar] [CrossRef]
- Welsch, B.T. Flux Accretion and Coronal Mass Ejection Dynamics. Sol. Phys. 2018, 293, 113. [Google Scholar] [CrossRef]
- Kliem, B.; Lee, J.; Liu, R.; White, S.M.; Liu, C.; Masuda, S. Nonequilibrium Flux Rope Formation by Confined Flares Preceding a Solar Coronal Mass Ejection. Astrophys. J. Lett. 2021, 909, 91. [Google Scholar] [CrossRef]
- Nindos, A.; Patsourakos, S.; Vourlidas, A.; Cheng, X.; Zhang, J. When do solar erupting hot magnetic flux ropes form? Astron. Astrophys. 2020, 642, A109. [Google Scholar] [CrossRef]
- van Ballegooijen, A.A. Observations and Modeling of a Filament on the Sun. Astrophys. J. 2004, 612, 519. [Google Scholar] [CrossRef]
- Savcheva, A.; Pariat, E.; van Ballegooijen, A.; Aulanier, G.; DeLuca, E. Sigmoidal active region on the sun: Comparison of a magnetohydrodynamical simulation and a nonlinear force-free field model. Astrophys. J. Lett. 2012, 750. [Google Scholar] [CrossRef]
- Papaioannou, A.; Belov, A.; Abunina, M.; Eroshenko, E.; Abunin, A.; Anastasiadis, A.; Patsourakos, S.; Mavromichalaki, H. Interplanetary Coronal Mass Ejections as the Driver of Non-recurrent Forbush Decreases. Astrophys. J. Lett. 2020, 890, 101. [Google Scholar] [CrossRef]
- Janvier, M.; Démoulin, P.; Guo, J.; Dasso, S.; Regnault, F.; Topsi-Moutesidou, S.; Gutierrez, C.; Perri, B. The Two-step Forbush Decrease: A Tale of Two Substructures Modulating Galactic Cosmic Rays within Coronal Mass Ejections. Astrophys. J. Lett. 2021, 922, 216. [Google Scholar] [CrossRef]
- Dumbovic, M.; Vrsnak, B.; Temmer, M.; Heber, B.; Kühl, P. Generic profile of a long-lived corotating interaction region and associated recurrent Forbush decrease. Astron. Astrophys. 2022, 658, A187. [Google Scholar] [CrossRef]
- Fadaaq, M.; Badruddin, B. Modulation of Galactic Cosmic Rays Due to Magnetic Clouds and Associated Structures in the Interplanetary Space: 1996–2018. Astrophysics 2021, 64, 210. [Google Scholar] [CrossRef]






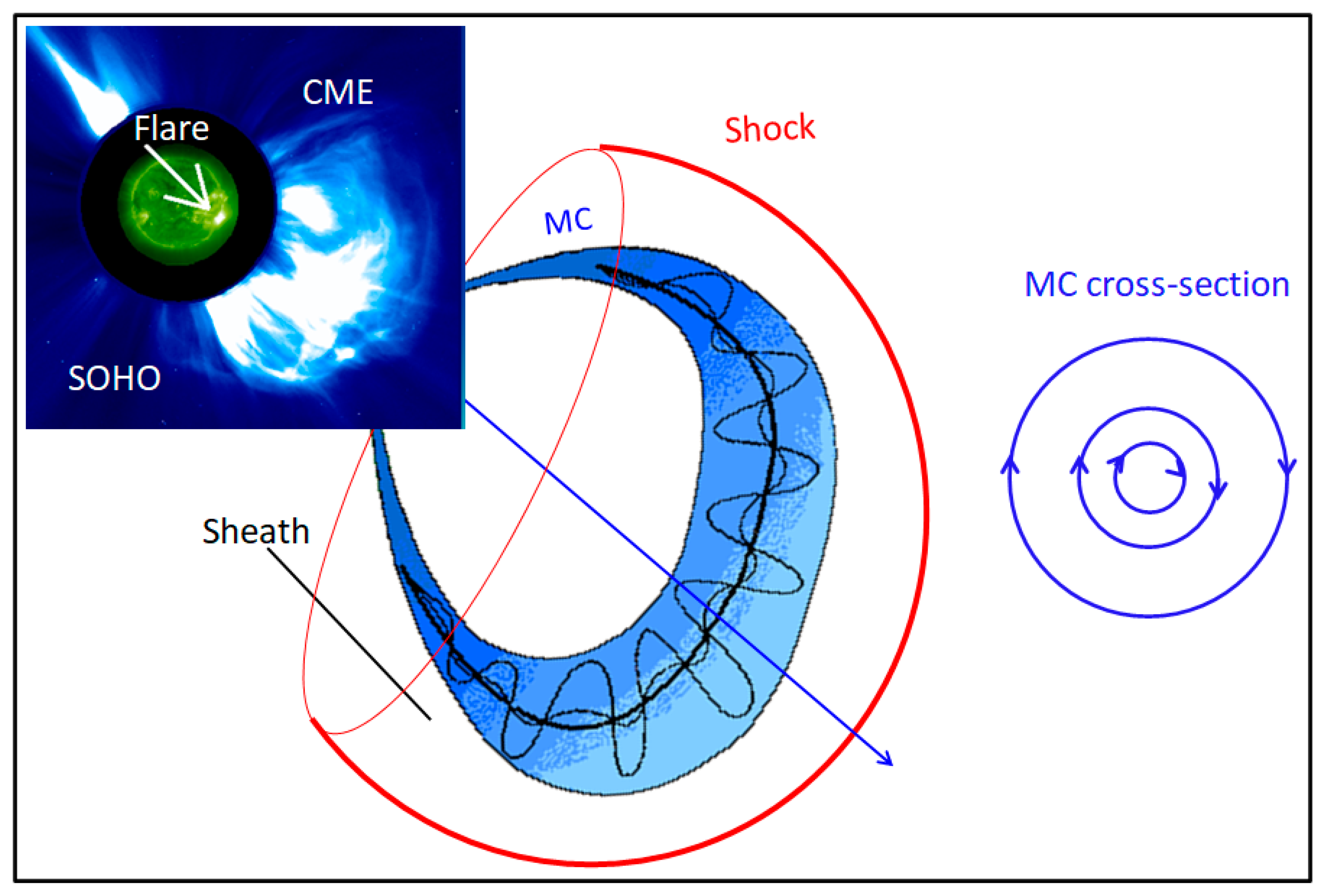
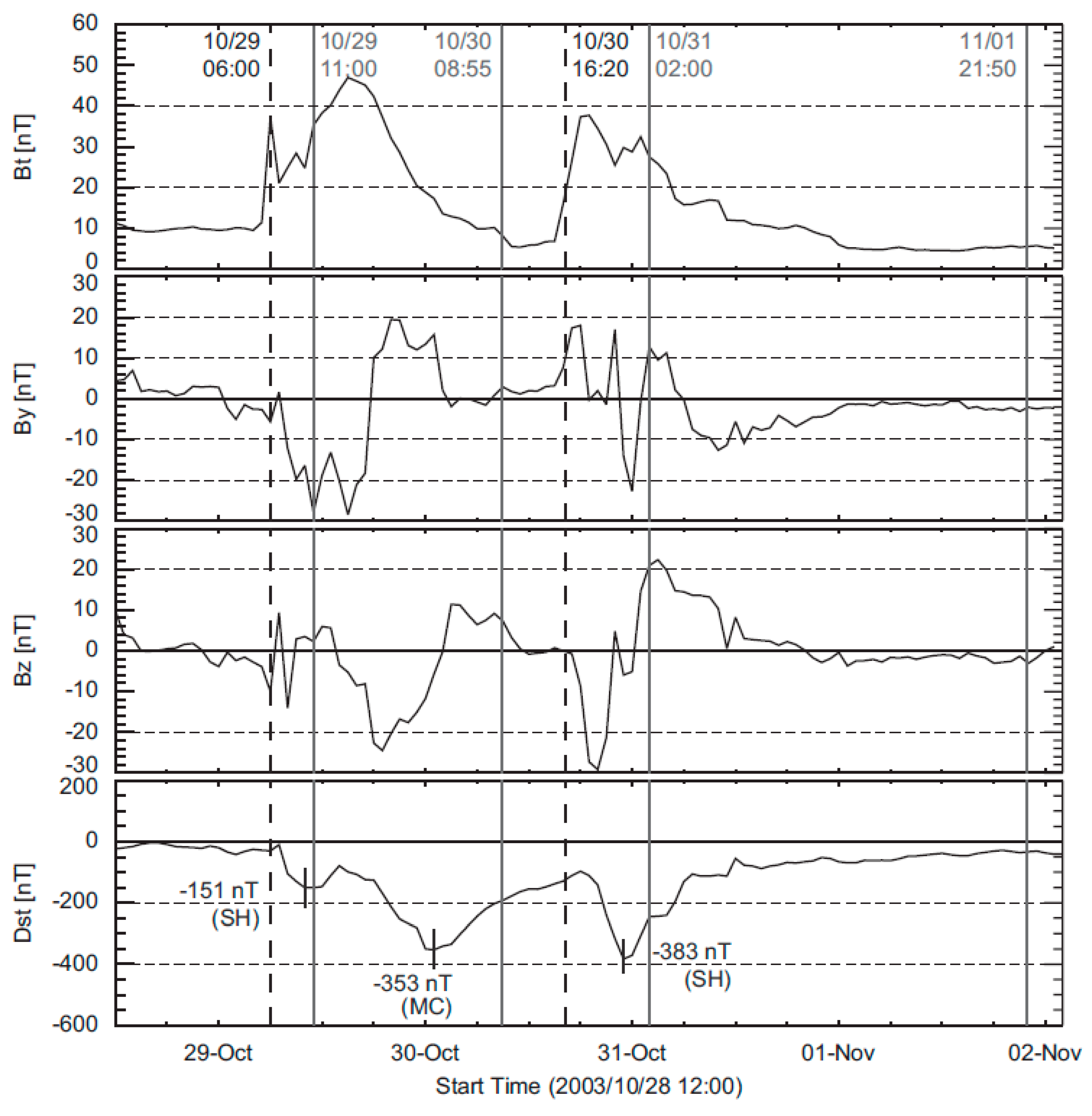
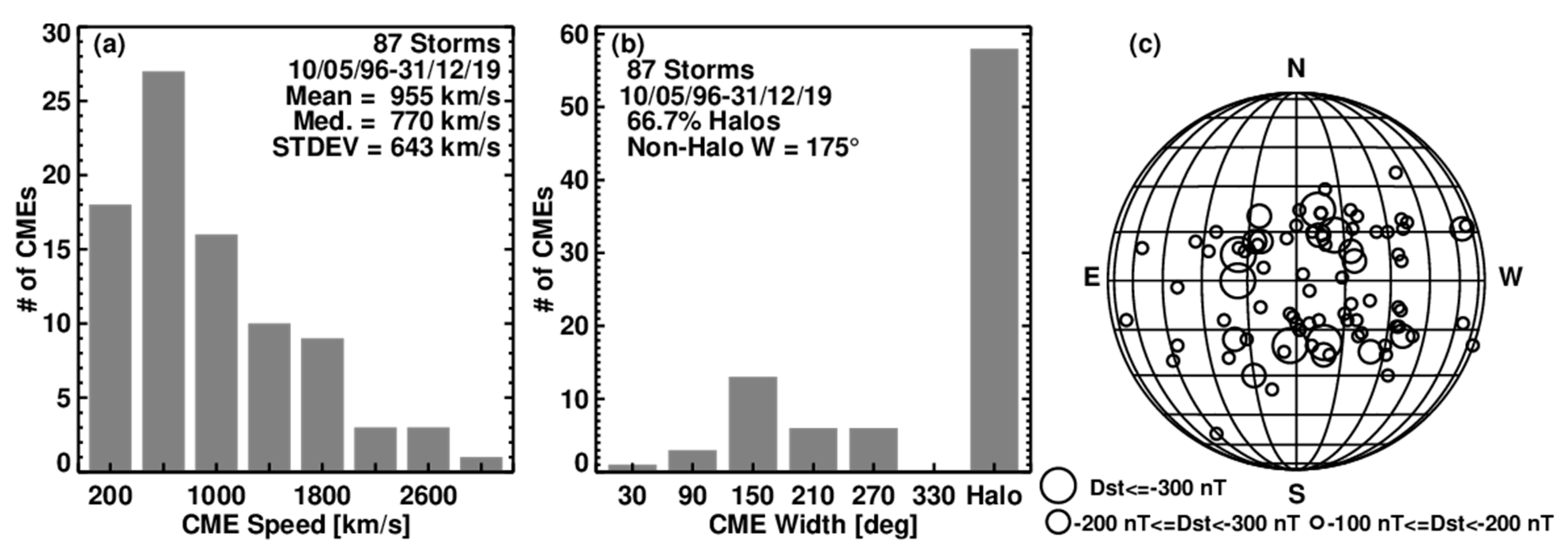

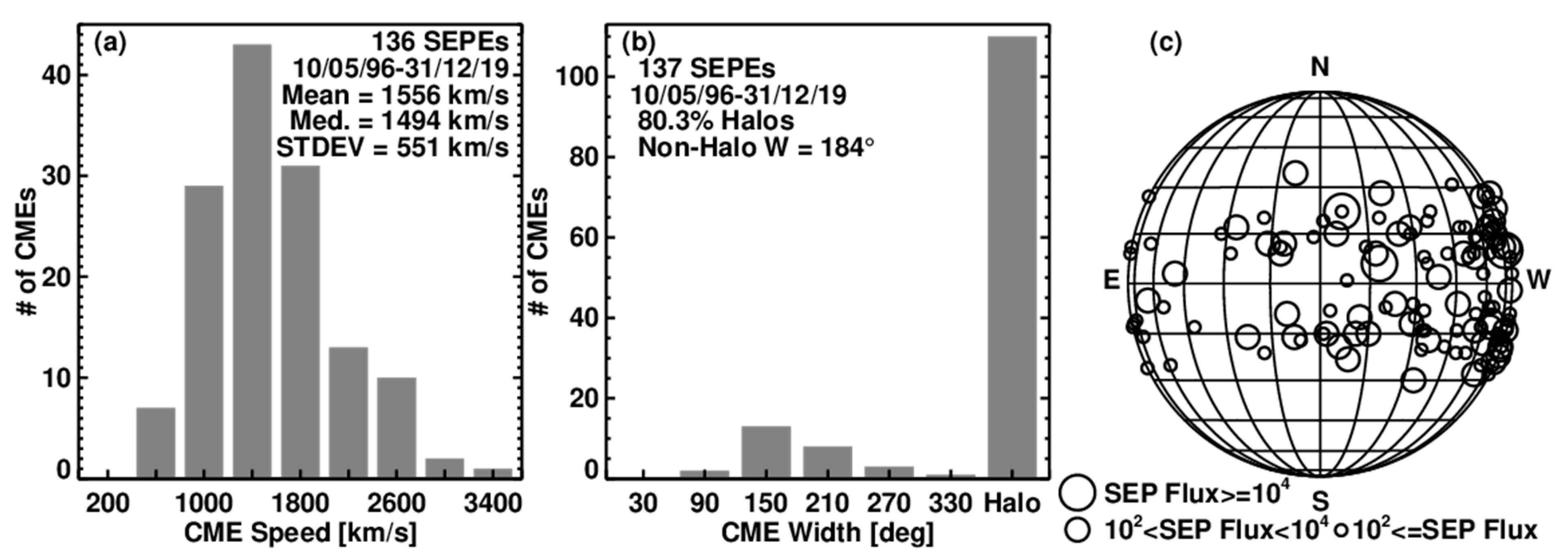
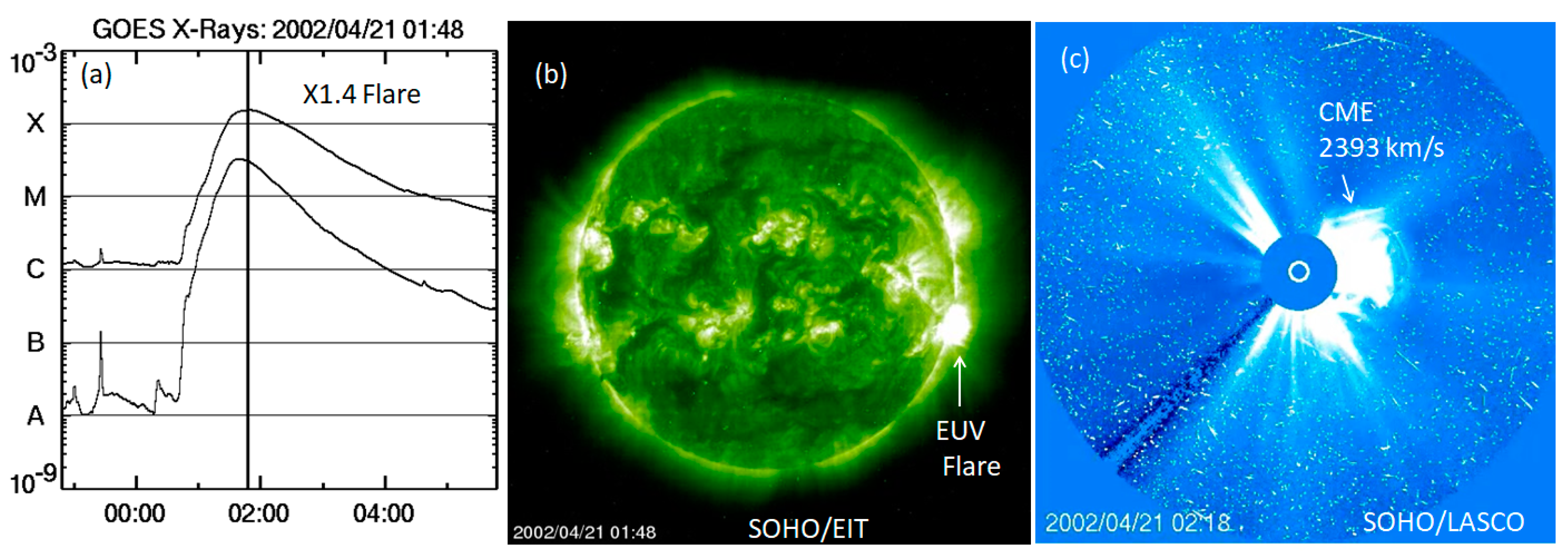
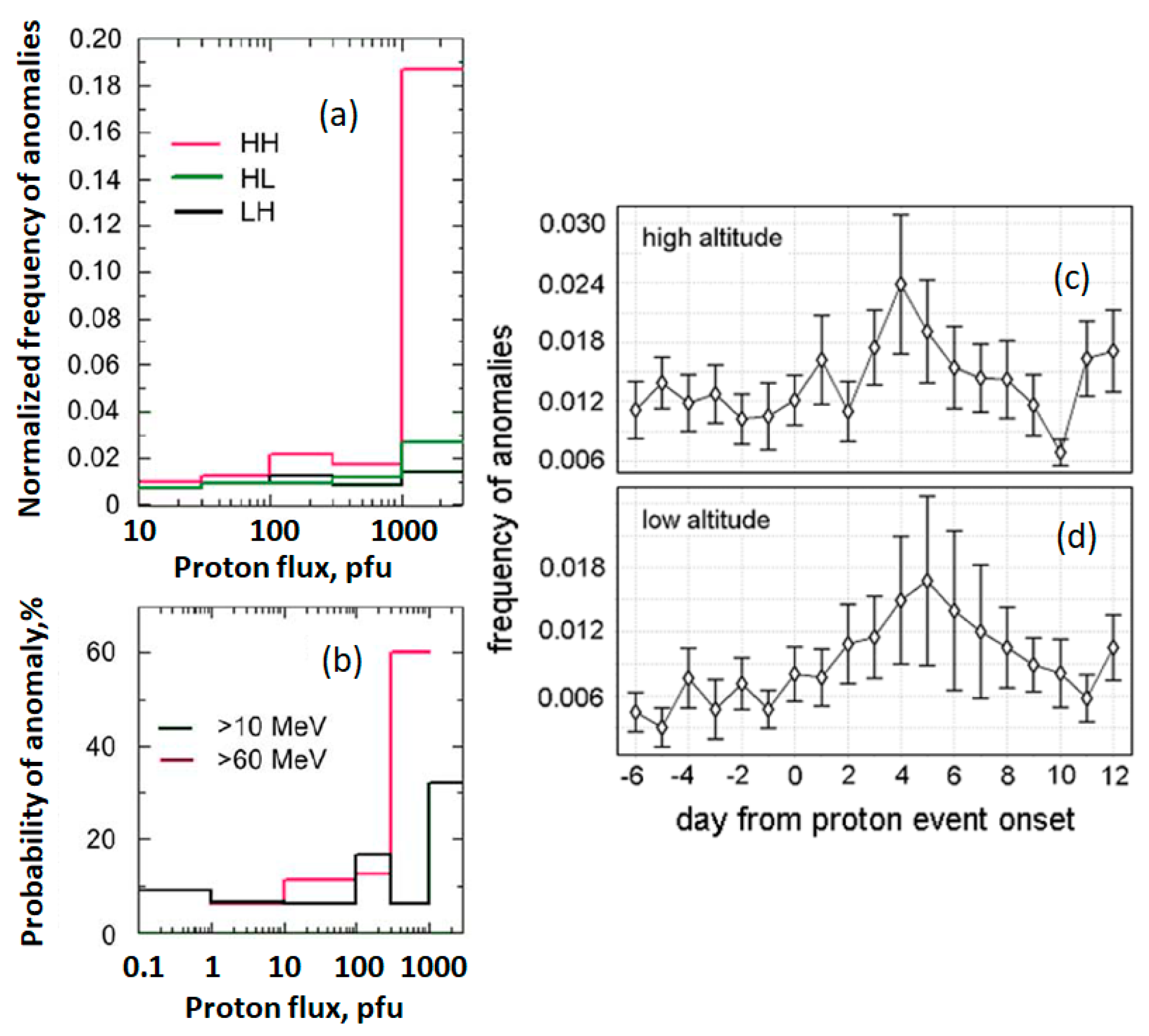
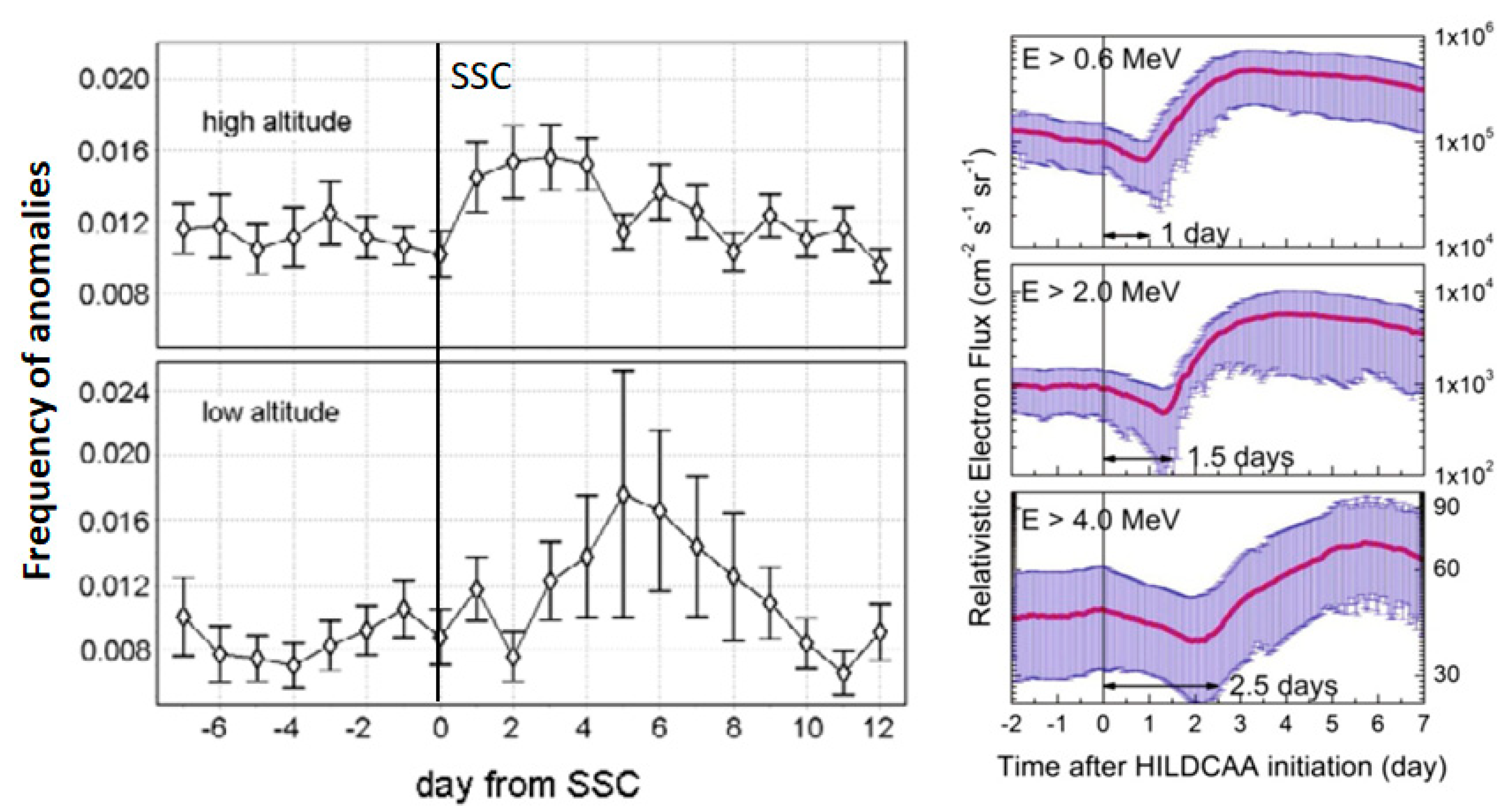
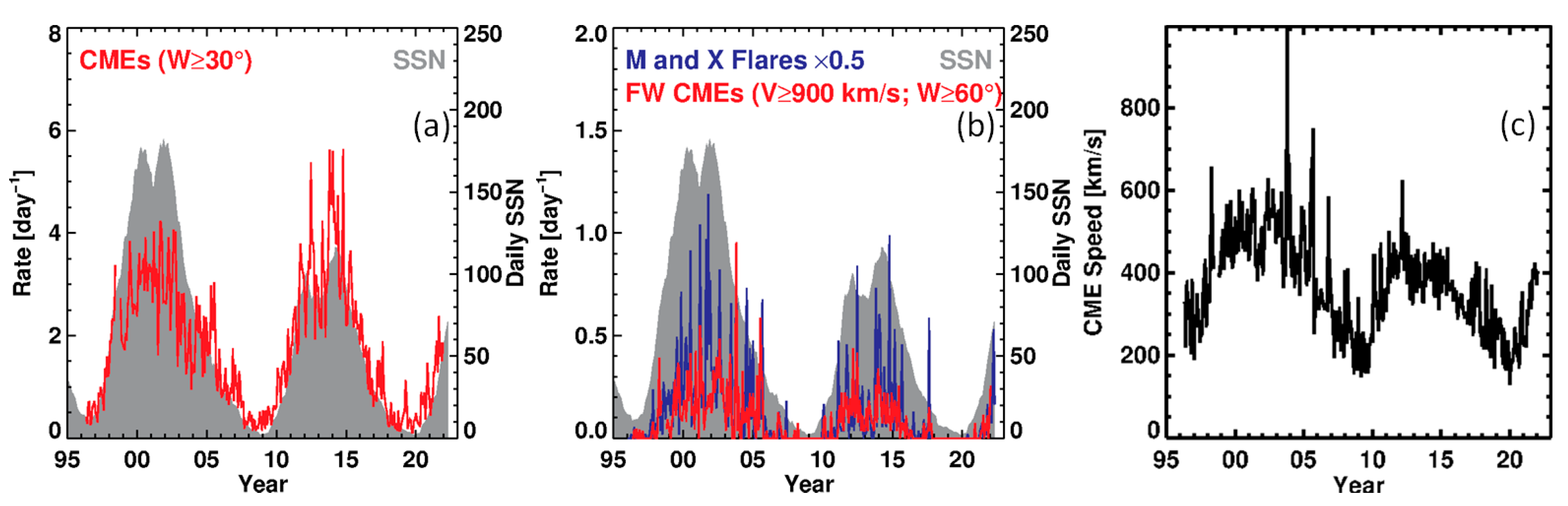



| Parameter | CIR | Solar Wind | Ratio | |
|---|---|---|---|---|
| Range | Mean | |||
| Density [cm−3] | 2.4–81.0 | 29.3 | 6.7 | 2.93 |
| Dynamic pressure [nPa] | 1.4–57.2 | 10.5 | 2.3 | 4.57 |
| Temperature [105 K] | 0.97–26.35 | 4.91 | 1.02 | 4.81 |
| Magnetic field [nT] | 4.6–44.9 | 14.8 | 5.5 | 2.69 |
| Duration [hr] | 2.75–82.10 | 26.47 | ---- | ---- |
| Extent [au] | 0.03–0.98 | 0.31 | ---- | ---- |
| CME Date | Time (UT) | Speed (km/s) | Location | Ip (GOES) | Ip (STB) |
|---|---|---|---|---|---|
| 28 December 2001 | 20:30 | 2216 | S26E90 | 76 | --- |
| 8 January 2002 | 17:54 | 1794 | >NE90 | 28 | --- |
| 27 July 2005 | 04:54 | 1787 | N11E90 | 41 | --- |
| 22 September 2011 | 10:48 | 1905 | N09E89 | 35 | 5000 |
| 21 June 2013 | 03:12 | 1900 | S16E73 | 14 | 100 |
| 25 Febraury 2014 | 01:25 | 2147 | S12E82 | 24 | 300 |
| Particle Type | Energy Range | Effects | Sources |
|---|---|---|---|
| Electrons | 10–100 keV | Spacecraft charging | Trapped particles |
| Electrons | >100 keV | Deep dielectric charging, solar cell damage | Trapped particles |
| Electrons | >1 MeV | Radiation damage (ionization) | Trapped/quasi trapped |
| Protons | 0.1–1 MeV | Surface damage to materials | Trapped particles |
| Protons | 1–10 MeV | Displacement damage in solar cells | Trapped particles, ESP |
| Protons | >10 MeV | Ionization, displacement damage; sensor background | Radiation belt, SEPs, GCRs |
| Protons | >30 MeV | Damage to biological systems | Radiation belt, SEPs, GCRs |
| Protons | >50 MeV | Single event effects | Radiation belt, SEPs, GCRs |
| Ions | >10 MeV/nuc | Single event effects | SEPs, GCRs |
| Protons | >500 MeV | Single event effects, hazard to humans in polar flights and in deep space | SEPs, GCRs |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gopalswamy, N. The Sun and Space Weather. Atmosphere 2022, 13, 1781. https://doi.org/10.3390/atmos13111781
Gopalswamy N. The Sun and Space Weather. Atmosphere. 2022; 13(11):1781. https://doi.org/10.3390/atmos13111781
Chicago/Turabian StyleGopalswamy, Nat. 2022. "The Sun and Space Weather" Atmosphere 13, no. 11: 1781. https://doi.org/10.3390/atmos13111781
APA StyleGopalswamy, N. (2022). The Sun and Space Weather. Atmosphere, 13(11), 1781. https://doi.org/10.3390/atmos13111781






