Equatorial Plasma Bubbles: A Review
Abstract
1. Introduction
2. Observations of EPBs and Associated Irregularities
2.1. In Situ Observations Using Rockets and Satellites
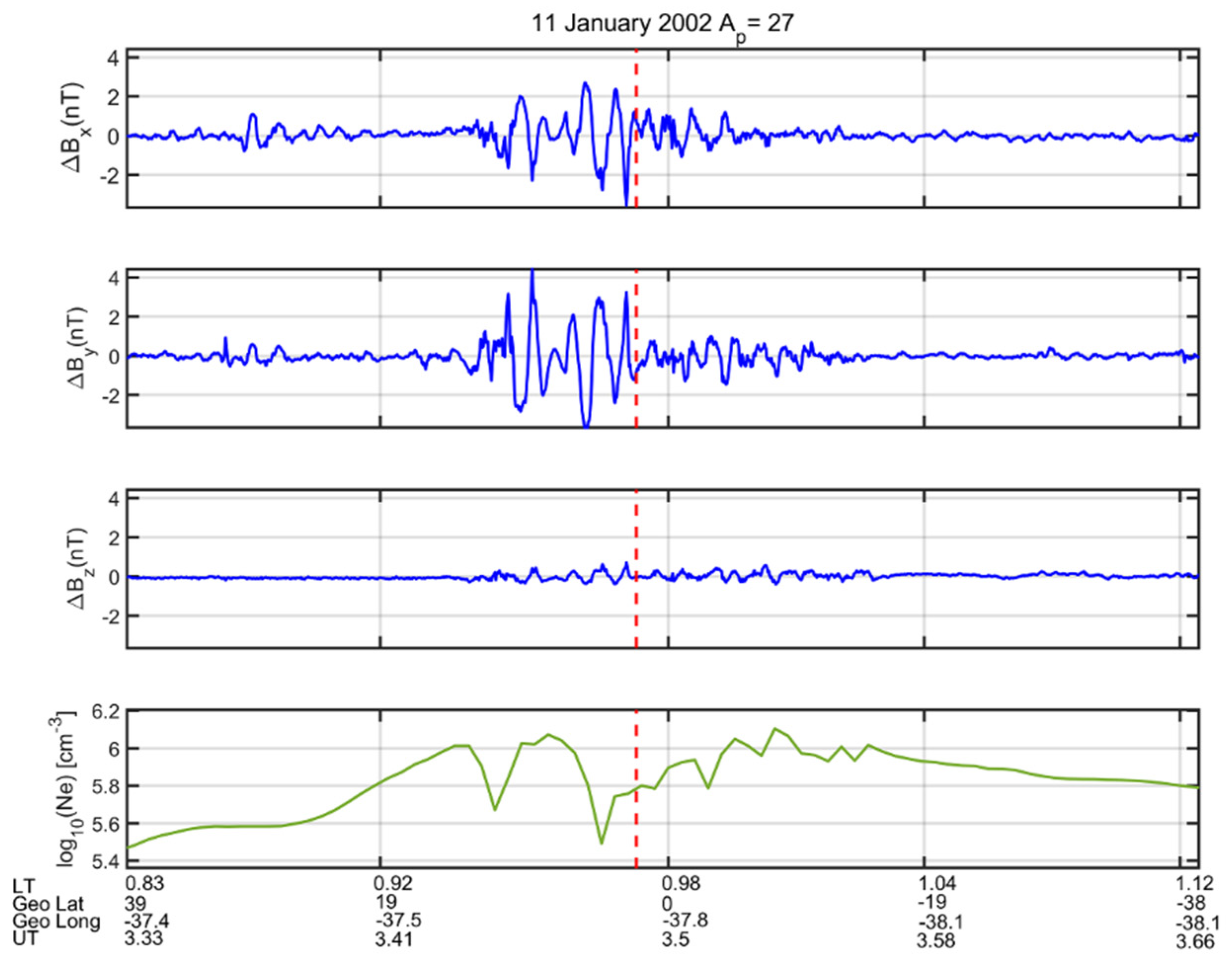
Magnetic Signatures of EPBs
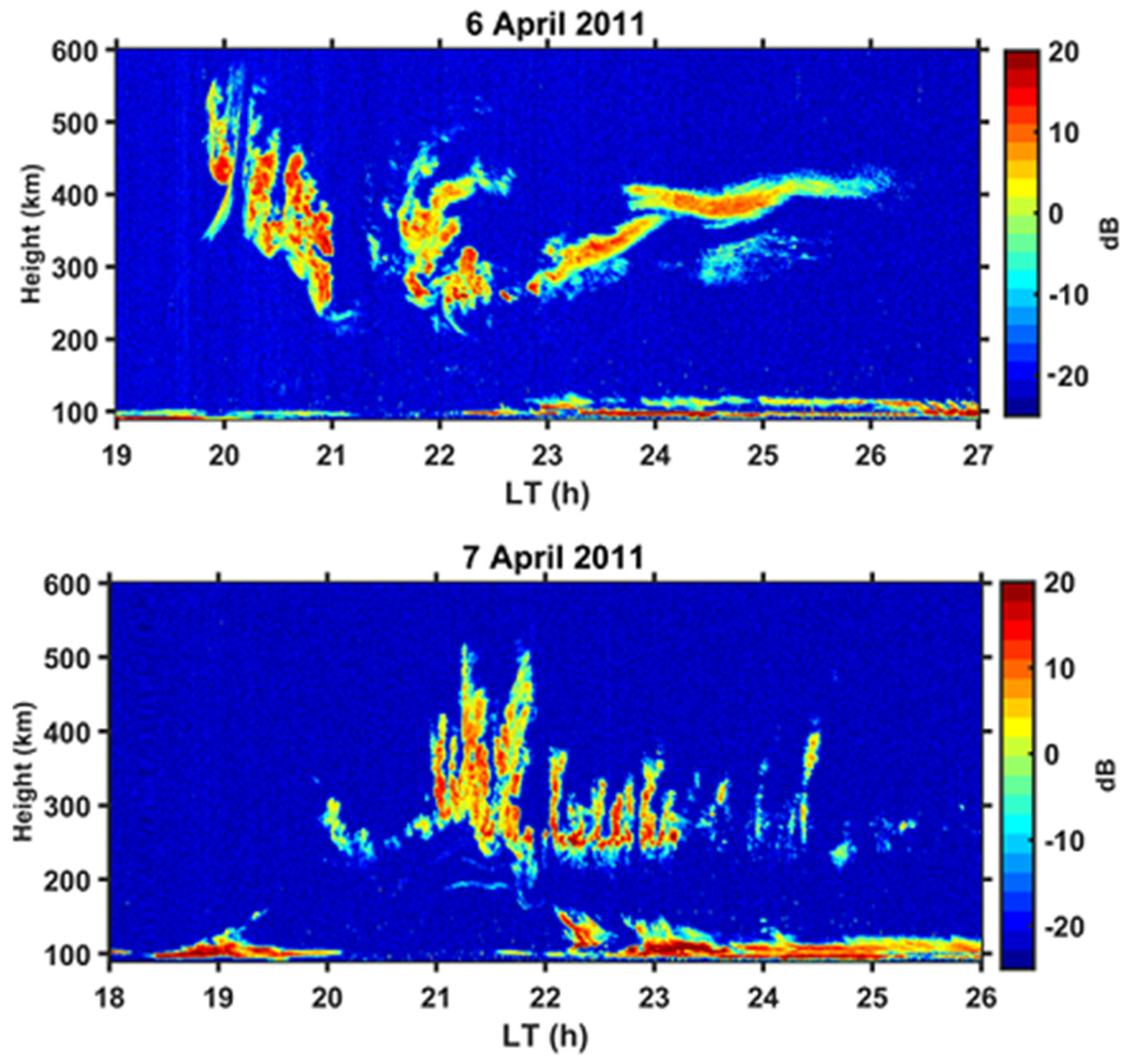
2.2. Radar Observations
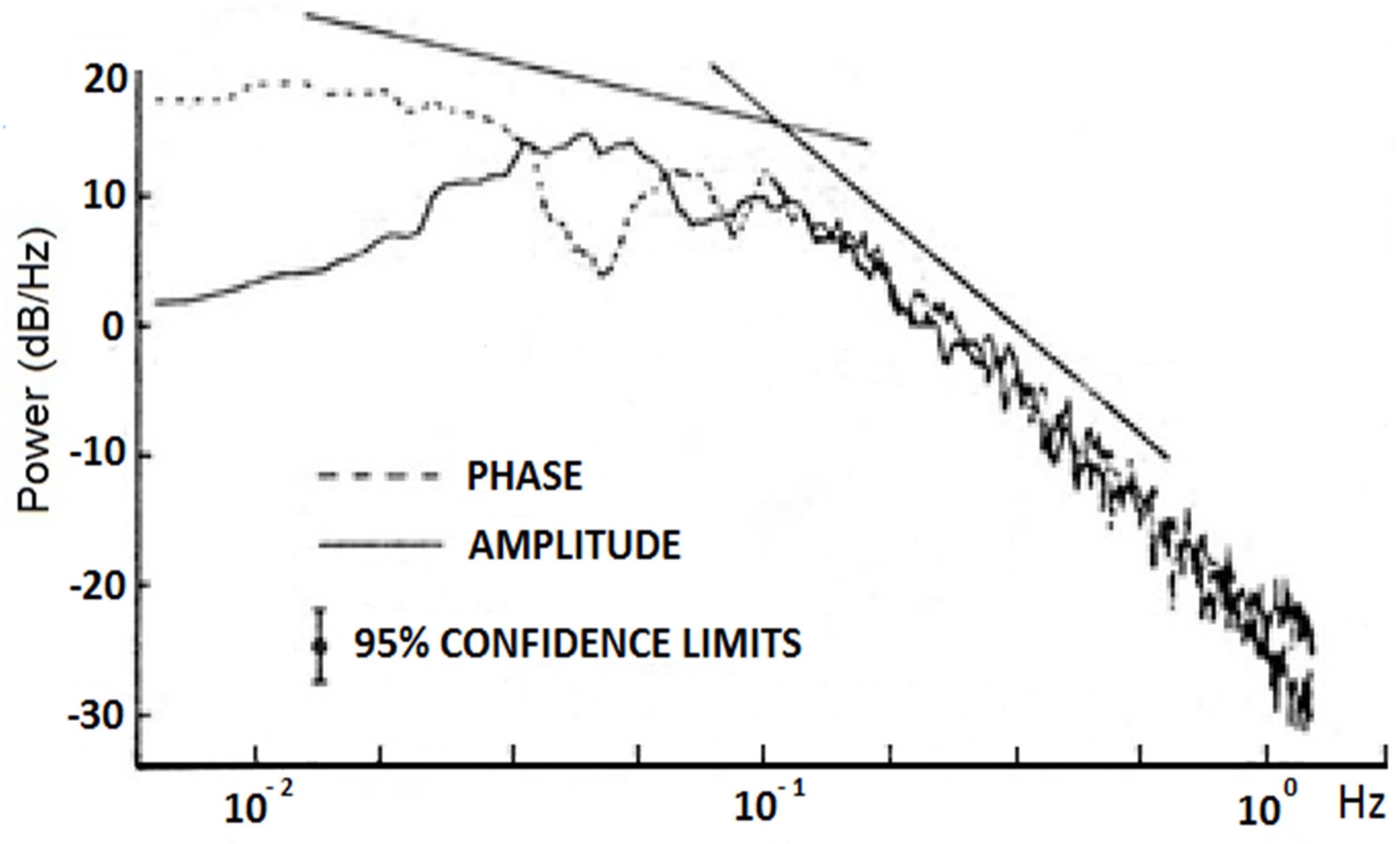
2.3. Ionospheric Scintillation Measurements
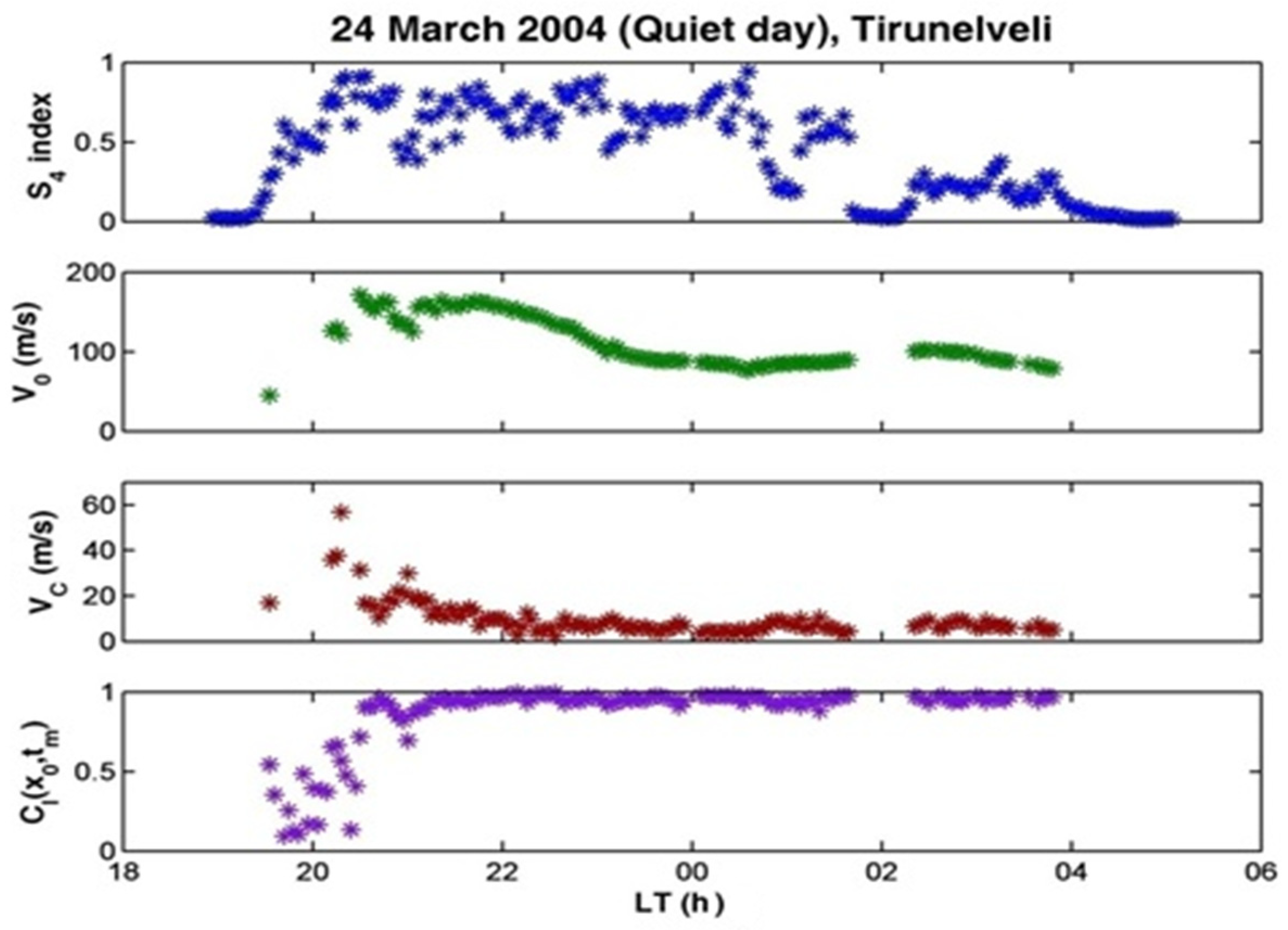
2.3.1. Dependence of Scintillation Parameters on Irregularity Characteristics
3. Development of EPBs and Associated Irregularities: Theoretical Aspects
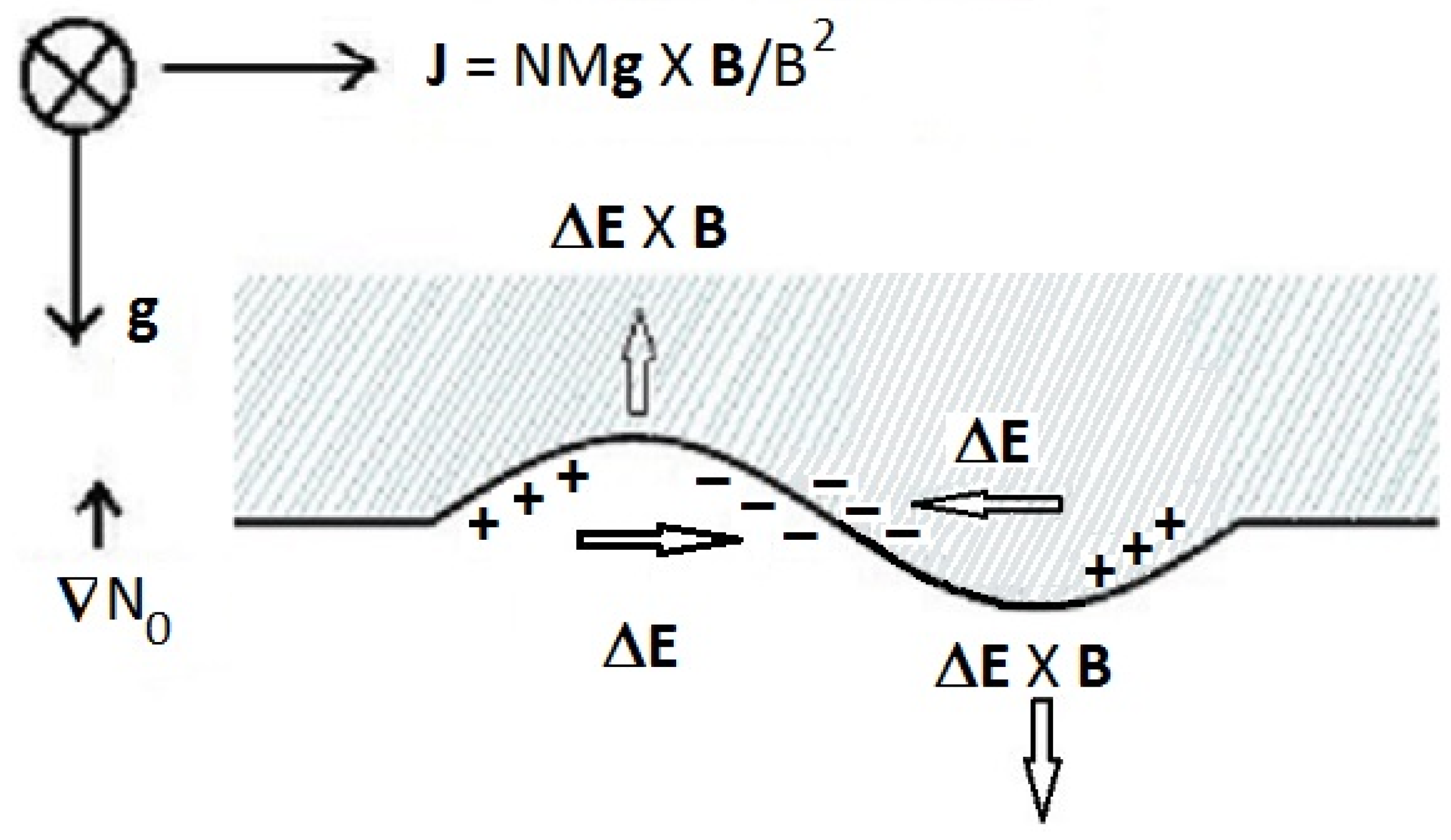
3.1. Electrostatic Rayleigh–Taylor Instability
3.2. Electromagnetic Rayleigh–Taylor Instability
4. Present Scenario
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| AE-E | Atmospheric Explorer E |
| ALTAIR | ARPA Long-range Tracking And Instrumentation Radar |
| CHAMP | Challenging Mini-satellite Payload |
| C/NOFS | Communication/ Navigation Outage Forecasting System |
| CRRES | Combined Release and Radiation Effects Satellite |
| DDEF | Disturbance dynamo electric field |
| DEMETER | Detection of Electromagnetic Emissions from Earthquake Regions |
| DMSP | Defense Meteorological Satellite Program |
| EAR | Equatorial Atmosphere Radar |
| EIA | Equatorial ionization anomaly |
| EPB | Equatorial plasma bubble |
| ESA | European Space Agency |
| ESF | Equatorial spread F |
| FAC | Field aligned current |
| GNSS | Global Navigation Satellite System |
| GOLD | Global-scale Observations of Limb and Disk |
| GRT | Generalized Rayleigh- Taylor |
| HIRB | High resolution bubble |
| ICON | Ionospheric Connection |
| JRO | Jicamarca Radio Observatory |
| LSWS | Large scale wave structure |
| MST | Mesosphere–stratosphere–troposphere |
| PPEF | Promptly penetrated electric field |
| ROCSAT | Republic of China Satellite |
| R–T | Rayleigh–Taylor |
| RTI | Range–time–intensity |
| SAMI3 | Sami3 is Also a Model of the Ionosphere |
| VHF | Very high frequency |
References
- Berkner, L.V.; Wells, H.W. F-region ionospheric investigations at low latitudes. Terr. Magn. Atmos. Elec. 1934, 39, 215–230. [Google Scholar] [CrossRef]
- Booker, H.G.; Wells, H.W. Scattering of radio waves by theF-region of the ionosphere. J. Geophys. Res. 1938, 43, 249–256. [Google Scholar] [CrossRef]
- Booker, H.G. Turbulence in the ionosphere with applications to meteor trails, radio-star scintillation, auroral radar echoes and other phenomena. J. Geophys. Res. 1956, 61, 673–705. [Google Scholar] [CrossRef]
- Koster, J.R.; Wright, R.W. Scintillation, spread F, and transequatorial scatter. J. Geophys. Res. 1960, 65, 2303–2306. [Google Scholar] [CrossRef]
- Aarons, J. Global morphology of ionospheric scintillations. Proc. IEEE 1982, 70, 360–378. [Google Scholar] [CrossRef]
- Basu, S.; Aarons, J.; McClure, J.; LaHoz, C.; Bushby, A.; Woodman, R. Preliminary comparisons of VHF radar maps of F-region irregularities with scintillations in the equatorial region. J. Atmos. Terr. Phys. 1977, 39, 1251–1261. [Google Scholar] [CrossRef]
- Dungey, J.W. Convective diffusion in the equatorial F-region. J. Atmos. Terr. Phys. 1956, 9, 304–310. [Google Scholar] [CrossRef]
- Farley, D.T.; Balsey, B.B.; Woodman, R.F.; McClure, J.P. Equatorial spread F: Implications of VHF radar observations. J. Geophys. Res. 1970, 75, 7199–7216. [Google Scholar] [CrossRef]
- Woodman, R.F.; LaHoz, C. Radar observations of F-region equatorial irregularities. J. Geophys. Res. 1976, 81, 5447–7216. [Google Scholar] [CrossRef]
- Scannapieco, A.J.; Ossakow, S.L. Nonlinear equatorial spread F. Geophys. Res. Lett. 1976, 3, 451–454. [Google Scholar] [CrossRef]
- Hudson, M.K.; Kennel, C.F. The electromagnetic interchange mode in a partly-ionized collisional plasma. J. Plasma Phys. 1975, 14, 121–134. [Google Scholar] [CrossRef]
- Tsunoda, R.T. Magnetic-Field-Aligned Characteristics of Plasma Bubbles in the Nighttime Equatorial Ionosphere. J. Atmos. Terr. Phys. 1980, 42, 743–752. [Google Scholar] [CrossRef]
- Rastogi, R.; Chandra, H.; Janardhan, P.; Reinisch, B.; Bisoi, S.K. Post sunset equatorial spread-F at Kwajalein and interplanetary magnetic field. Adv. Space Res. 2017, 60, 1708–1715. [Google Scholar] [CrossRef]
- Dyson, P.L.; McClure, J.P.; Hanson, W.B. In situ measurements of the spectral characteristics of F region ionospheric irregu-larities. J. Geophys. Res. 1974, 79, 1497. [Google Scholar] [CrossRef]
- Livingston, R.C.; Rino, C.L.; McClure, J.P.; Hanson, W.B. Spectral characteristics of medium-scale equatorial F region irregu-larities. J. Geophys. Res. 1981, 86, 2421. [Google Scholar] [CrossRef]
- Sinha, H.S.S.; Raizada, S.; Mishra, R.N. First simultaneous in situ measurement of electron density and electric field fluctuations during spread F in the Indian zone. Geophys. Res. Lett. 1999, 20, 1669. [Google Scholar] [CrossRef]
- Su, S.-Y.; Yeh, H.C.; Heelis, R.A. ROCSAT 1 ionospheric plasma and electrodynamics instrument observations of equatorial spreadF: An early transitional scale result. J. Geophys. Res. 2001, 106, 29153–29159. [Google Scholar] [CrossRef]
- Shume, E.; Hysell, D. Spectral analysis of plasma drift measurements from the AE-E satellite: Evidence of an inertial subrange in equatorial spread F. J. Atmos. Sol. Terr. Phys. 2003, 66, 57–65. [Google Scholar] [CrossRef]
- Rodrigues, F.S.; Kelley, M.C.; Roddy, P.A.; Hunton, D.E.; Pfaff, R.F.; de la Beaujardière, O.; Bust, G.S. C/NOFS observations of intermediate and transitional scale-size equatorial spread F irregularities. Geophys. Res. Lett. 2009, 36, L00C05. [Google Scholar] [CrossRef]
- Kelley, M.C.; Haerandel, G.; Kappler, H.; Valenzuela, A.; Balsley, B.B.; Carter, D.A.; Ecklund, W.L.; Carlson, C.W.; Häusler, B.; Torbert, R. Evidence for a Rayleigh-Taylor type instability and upwelling of density depleted regions during equatorial spread F. Geophys. Res. Lett. 1976, 3, 448–450. [Google Scholar] [CrossRef]
- Rino, C.L.; Tsunoda, R.T.; Petriceks, J.; Livingston, R.C.; Kelley, M.C.; Baker, K.D. Simultaneous rocket-borne beacon and in situ measurements of equatorial spread F–intermediate wavelength results. J. Geophys. Res. 1981, 86, 2411. [Google Scholar] [CrossRef]
- Tsunoda, R.T.; Livingston, R.C.; McClure, J.P.; Hanson, W.B. Equatorial plasma bubbles–vertically elongated wedges from the bottom-side F layer. J. Geophys. Res. 1982, 87, 9171–9180. [Google Scholar] [CrossRef]
- Burke, W.J.; Gentile, L.C.; Huang, C.Y.; Valladares, C.E.; Su, S.Y. Longitudinal variability of equatorial plasma bubbles observed by DMSP and ROCSAT-1. J. Geophys. Res. 2004, 109, A12301. [Google Scholar] [CrossRef]
- Heelis, R.A.; Stoneback, R.; Earle, G.D.; Haaser, R.A.; Abdu, M.A. Medium-scale equatorial plasma irregularities observed by Coupled Ion-Neutral Dynamics Investigation sensors aboard the Communication Navigation Outage Forecast System in a prolonged solar minimum. J. Geophys. Res. 2010, 115, A10321. [Google Scholar] [CrossRef]
- Huang, C.-S.; de la Beaujardiere, O.; Pfaff, R.F.; Retterer, J.M.; Roddy, P.A.; Hunton, D.E.; Su, Y.-I.; Su, S.-Y.; Rich, F.J. Zonal drift of plasma particles inside equatorial plasma bubbles and its relation to the zonal drift of the bubble structure. J. Geophys. Res. 2010, 115, A07316. [Google Scholar] [CrossRef]
- Aggson, T.L.; Burke, W.J.; Maynard, N.C.; Hanson, W.B.; Anderson, P.C.; Slavin, J.A.; Hoegy, W.R.; Saba, J.L. Equatorial bubbles updrafting at supersonic speeds. J. Geophys. Res. 1992, 97, 8581. [Google Scholar] [CrossRef]
- Koons, H.C.; Roeder, J.L.; Rodriguez, P. Plasma waves observed inside plasma bubbles in the equatorial F region. J. Geophys. Res. 1997, 102, 4577–4583. [Google Scholar] [CrossRef]
- Pottelette, R.; Malingre, M.; Berthelier, J.J.; Seran, E.; Parrot, M. Filamentary Alfvénic structures excited at the edges of equatorial plasma bubbles. Ann. Geophys. 2007, 25, 2159. [Google Scholar] [CrossRef]
- Stolle, C.; Lühr, H.; Rother, M.; Balasis, G. Magnetic signatures of equatorial spread F as observed by the CHAMP satellite. J. Geophys. Res. 2006, 111, A02304. [Google Scholar] [CrossRef]
- Park, J.; Lühr, H.; Stolle, C.; Rother, M.; Min, K.W.; Michaelis, I. The characteristics of field-aligned currents associated with equatorial plasma bubbles, as observed by the CHAMP satellite. Ann. Geophys. 2009, 27, 2685–2697. [Google Scholar] [CrossRef]
- Park, J.; Noja, M.; Stolle, C.; Lühr, H. The ionospheric bubble index deduced from magnetic field and plasma observations on board Swarm. Earth Planets Space 2013, 65, 1333–1344. [Google Scholar] [CrossRef]
- Luo, X.; Xiong, C.; Gu, S.; Lou, Y.; Stolle, C.; Wan, X.; Liu, K.; Song, W. Geomagnetically conjugate observations of equatorial plasma irregularities from Swarm constellation and ground-based GPS stations. J. Geophys. Res. Space Phys. 2019, 124, 3650–3665. [Google Scholar] [CrossRef]
- Jin, Y.; Xiong, C.; Clausan, L.; Spicher, A.; Kotova, D.; Brask, S.; Kervalishvili, G.; Stolle, C.; Miloch, W. Ionospheric plasma irregularities based on in situ measurements from Swarm satellites. J. Geophys. Res. Space Phys. 2020, 124, e2020JA028103. [Google Scholar] [CrossRef]
- Hysell, D.L.; Burcham, J.D. JULIA radar studies of equatorial spread F. J. Geophys. Res. 1998, 103, 29155–29167. [Google Scholar] [CrossRef]
- Patra, A.K.; Tiwari, D.; Sripathi, S.; Rao, P.B.; Sridharan, R.; Devasia, C.V.; Viswanathan, K.S.; Subbarao, K.S.V.; Sekar, R.; Kherani, E.A. Simultaneous radar observations of meter scale F region irregularities at and off the magnetic equator over India. J. Geophys. Res. 2005, 110, A02307. [Google Scholar] [CrossRef]
- Patra, A.K.; Srinivasulu, P.; Chaitanya, P.P.; Rao, M.D.; Jayaraman, A. First results on low latitude E and F region irregularities obtained using the Gadanki Ionospheric Radar Interferometer. J. Geophys. Res. 2014, 119, 10276–10293. [Google Scholar] [CrossRef]
- Fukao, S.; Ozawa, Y.; Yokoyama, T.; Yamamoto, M.; Tsunoda, R.T. First observations of spatial structure of F region 3 m–scale field-aligned irregularities with the Equatorial Atmosphere Radar in Indonesia. J. Geophys. Res. 2004, 109, A02304. [Google Scholar] [CrossRef]
- Yokoyama, T.; Fukao, S.; Yamamoto, M. Relationship of the onset of equatorial F-region irregularities with the sunset terminator observed with the Equatorial Atmosphere Radar. Geophys. Res. Lett. 2004, 31, L24804. [Google Scholar] [CrossRef]
- Yeh, K.C.; Liu, C.H. Radio wave scintillations in the ionosphere. Proc. IEEE 1982, 70, 324–360. [Google Scholar]
- Bhattacharyya, A.; Yeh, K.C.; Franke, S.J. Deducing turbulence parameters from transionospheric scintillation measurements. Space. Sci. Rev. 1992, 61, 335–386. [Google Scholar] [CrossRef]
- Rino, C.L. On the application of phase screen models to the interpretation of ionospheric scintillation data. Radio Sci. 1982, 17, 855–867. [Google Scholar]
- Bhattacharyya, A.; Rastogi, R.G. Phase scintillations due to equatorial F region irregularities with two-component power law spectrum. J. Geophys. Res. 1986, 91, 11359–11364. [Google Scholar] [CrossRef]
- Bhattacharyya, A.; Beach, T.L.; Basu, S.; Kintner, P.M. Nighttime equatorial ionosphere: GPS scintillations and differential carrier phase fluctuations. Radio Sci. 2000, 35, 209–224. [Google Scholar] [CrossRef]
- Vacchione, J.D.; Franke, S.J.; Yeh, K.C. A new analysis technique for estimating zonal irregularity drifts and variability in the equatorial F region using spaced receiver scintillation data. Radio Sci. 1987, 22, 745–756. [Google Scholar] [CrossRef]
- Briggs, B.H. The analysis of spaced sensor records by correlation techniques. In Middle Atmosphere Program, Handbook for MAP; Vincent, R.A., Ed.; ICSU: Paris, France, 1984; Volume 13, pp. 166–186. [Google Scholar]
- Bhattacharyya, A. Challenges of predicting low-latitude ionospheric scintillations. Radio Sci. Bull. 2019, 371, 17–31. [Google Scholar] [CrossRef]
- Bhattacharyya, A.; Franke, S.J.; Yeh, K.C. Characteristic velocity of equatorial F region irregularities determined from spaced receiver scintillation data. J. Geophys. Res. 1989, 94, 11959–11969. [Google Scholar] [CrossRef]
- Bhattacharyya, A.; Basu, S.; Groves, K.M.; Valladares, C.E.; Sheehan, R. Dynamics of equatorial F region irregularities from spaced receiver scintillation observations. Geophys. Res. Lett. 2001, 28, 119–122. [Google Scholar] [CrossRef]
- Bhattacharyya, A.; Kakad, B.; Sripathi, S.; Jeeva, K.; Nair, K.U. Development of intermediate scale structure near the peak of the F region within an equatorial plasma bubble. J. Geophys. Res. Space Phys. 2014, 119, 3066–3076. [Google Scholar] [CrossRef]
- Kelley, M.C.; Makela, J.J.; Ledvina, B.M.; Kintner, P.M. Observations of equatorial spread F from Haleakala, Hawaii. Geophys. Res. Lett. 2002, 29, 2003. [Google Scholar] [CrossRef]
- Otsuka, Y.; Shiokawa, K.; Ogawa, T.; Wilkinson, P. Geomagnetic conjugate observations of equatorial airglow depletions. Geophy. Res. Lett. 2002, 29, 1753. [Google Scholar] [CrossRef]
- Makela, J.J.; Kelley, M.C. Field-aligned 777.4 nm composite airglow images of equatorial plasma depletions. Geophy. Res. Lett. 2003, 30, 1442. [Google Scholar] [CrossRef]
- Fukushima, D.; Shiokawa, K.; Otsuka, Y.; Nishioka, M.; Kubota, M.; Tsugawa, T.; Nagatsuma, T.; Komonjinda, S.; Yatini, C.Y. Geomagnetically conjugate observations of plasma bubbles and thermospheric neutral winds at low latitudes. J. Geophys. Res. Space Phys. 2015, 120, 2222–2231. [Google Scholar] [CrossRef]
- Kelley, M.C. The Earth’s Ionosphere: Plasma Physics and Electrodynamics, 2nd ed.; Academic Press: San Diego, CA, USA, 2009. [Google Scholar]
- Zalazek, S.T.; Ossakow, S.L.; Chaturvedi, P.K. Nonlinear equatorial spread F: The effect of neutral winds and background Pedersen conductivity. J. Geophys. Res. 1982, 87, 151. [Google Scholar]
- Tsunoda, R.T. Control of the seasonal and longitudinal occurrence of equatorial scintillations by the longitudinal gradient in integrated E region Pedersen conductivity. J. Geophys. Res. 1985, 90, 447–456. [Google Scholar] [CrossRef]
- Haerendel, G. Theory of Equatorial Spread F. Max Planck Inst. Phys. Astrophys. 1973. preprint. [Google Scholar]
- Hudson, M.K.; Kennel, C.F. Linear theory of equatorial spread F. J. Geophys. Res. 1975, 80, 4581–4590. [Google Scholar] [CrossRef]
- Sultan, P.J. Linear theory and modelling of the Rayleigh-Taylor instability leading to the occurrence of equatorial spread F. J. Geophys. Res. 1996, 101, 26875–26891. [Google Scholar] [CrossRef]
- Huba, J.D.; Hassam, A.B.; Schwartz, I.B.; Keskinen, M.J. Ionospheric turbulence: Interchange instability and chaotic fluid behavior. Geophys. Res. Lett. 1985, 12, 65. [Google Scholar] [CrossRef]
- Lorenz, E.N. Deterministic nonperiodic flow. J. Atmos. Sci. 1963, 20, 130–141. [Google Scholar] [CrossRef]
- Sekar, R.; Raghavarao, R. Critical role of the equatorial topside F region on the evolutionary characteristics of the plasma bubbles. Geophys. Res. Lett. 1995, 22, 3255–3258. [Google Scholar] [CrossRef]
- Sekar, R.; Kelley, M.C. On the combined effects of vertical shear and zonal electric field patterns on nonlinear equatorial spread F evolution. J. Geophys. Res. 1998, 103, 20735. [Google Scholar] [CrossRef]
- Huba, J.; Joyce, G. Equatorial spread F modelling: Multiple bifurcated structures, secondary instabilities, large density ‘bite-outs’, and supersonic flows. Geophys. Res. Lett. 2007, 34, L07105. [Google Scholar] [CrossRef]
- Keskinen, M.J.; Ossakow, S.L.; Fejer, B.G. Three-dimensional nonlinear evolution of equatorial ionospheric spread F bubbles. Geophys. Res. Lett. 2003, 30, 1855. [Google Scholar] [CrossRef]
- Krall, J.; Huba, J.D.; Ossakow, S.L.; Joyce, G. Why do equatorial ionospheric bubbles stop rising? Geophys. Res. Lett. 2010, 37, L09105. [Google Scholar] [CrossRef]
- Retterer, J.M. Forecasting low-latitude radio scintillation with 3-D ionospheric plume models: 1. Plume model. J. Geophys. Res. 2010, 115, A03306. [Google Scholar] [CrossRef]
- Aveiro, H.C.; Hysell, D.L. Three-dimensional numerical simulation of equatorial F region plasma irregularities with bottomside shear flow. J. Geophys. Res. 2010, 115, A11321. [Google Scholar] [CrossRef]
- Yokoyama, T.; Shinagawa, H.; Jin, H. Nonlinear growth, bifurcation, and pinching of equatorial plasma bubble simulated by three-dimensional high-resolution bubble model. J. Geophys. Res. Space Phys. 2014, 119, 10474–10482. [Google Scholar] [CrossRef]
- Bhattacharyya, A.; Burke, W.J. A transmission line analogy for the development of equatorial ionospheric bubbles. J. Geophys. Res. 2000, 105, 24941–24950. [Google Scholar] [CrossRef]
- Basu, B. Characteristics of electromagnetic Rayleigh–Taylor modes in nighttime equatorial plasma. J. Geophys. Res. 2005, 110, A02303. [Google Scholar] [CrossRef]
- Keskinen, M.J.; Ossakow, S.L.; Basu, S.; Sultan, P.J. Magnetic-flux-tube-integrated evolution of equatorial ionospheric plasma bubbles. J. Geophys. Res. 1998, 103, 3957–3967. [Google Scholar] [CrossRef]
- Bhattacharyya, A. Role of E region conductivity in the development of equatorial ionospheric plasma bubbles. Geophys. Res. Lett. 2004, 31, L06806. [Google Scholar] [CrossRef]
- Fejer, B.G.; Scherliess, L.; de Paula, E.R. Effects of the vertical plasma drift velocity on the generation and evolution of equatorial spread F. J. Geophys. Res. 1999, 104, 19859–19869. [Google Scholar] [CrossRef]
- Dao, E.; Seyler, C.E.; Kelley, M.C. Three-dimensional modelling of the electromagnetic characteristics of equatorial plasma depletions. J. Geophys. Res. Space Phys. 2013, 118, 3505–3514. [Google Scholar] [CrossRef]
- Rodriguez-Zuluaga, J.; Stolle, C.; Hysell, D.; Knudsen, D.J. Topside equatorial spread F-related field-aligned Poynting flux: Observations and simulations. Earth Planets Space 2022, 74, 119. [Google Scholar] [CrossRef]
- Thampi, S.V.; Yamamoto, M.; Tsunoda, R.T.; Otsuka, Y.; Tsugawa, T.; Uemoto, J.; Ishii, M. First observations of large-scale wave structure and equatorial spread F using CERTO radio beacon on the C/NOFS satellite. Geophys. Res. Lett. 2009, 36, L18111. [Google Scholar] [CrossRef]
- Fejer, B.G.; Maute, A. Equatorial Ionospheric Electrodynamics. In Space Physics and Aeronomy Collection: Ionosphere Dynamics and Applications, Geophysical Monograph 260, 1st ed.; Huang, C., Lu, G., Eds.; John and Wiley and Sons: Hoboken, NJ, USA, 2021; Volume 3, pp. 161–183. [Google Scholar]
- Blanc, M.; Richmond, A.D. The ionospheric disturbance dynamo. J. Geophys. Res. 1980, 85, 1669–1686. [Google Scholar] [CrossRef]
- Maruyama, N.; Richmond, A.D.; Fuller-Rowell, T.J.; Codrescu, M.V.; Sazykin, S.; Toffoletto, F.R.; Spiro, R.W.; Millward, G.H. Interaction between direct penetration and disturbance dynamo electric fields in the storm-time equatorial ionosphere. Geophys. Res. Lett. 2005, 32, L17105. [Google Scholar] [CrossRef]
- Bhattacharyya, A.; Basu, S.; Groves, K.M.; Valladares, C.E.; Sheehan, R. Effect of magnetic activity on the dynamics of equatorial F region irregularities. J. Geophys. Res. 2002, 107, 1489. [Google Scholar] [CrossRef]
- Groves, K.; Basu, S.; Weber, E.J.; Smitham, M.; Kuenzler, H.; Valladares, C.E.; Sheehan, R.; Mackenzie, E.; Secan, J.A.; Ning, P.; et al. Equatorial scintillation and systems support. Radio Sci. 1997, 32, 2047–2064. [Google Scholar] [CrossRef]
- Bhattacharyya, A.; Groves, K.M.; Basu, S.; Kuenzler, H.; Valladares, C.E.; Sheehan, R. L-band scintillation activity and space-time structure of low-latitude UHF scintillations. Radio Sci. 2003, 38, 1004. [Google Scholar] [CrossRef]
- Bhattacharyya, A.; Kakad, B.; Gurram, P.; Sripathi, S.; Sunda, S. Development of intermediate-scale structure at different altitudes within an equatorial plasma bubble: Implications for L-band scintillations. J. Geophys. Res. Space Phys. 2017, 122, 1015–1030. [Google Scholar] [CrossRef]
- Retterer, J.M. Forecasting low-latitude radio scintillation with 3-D ionospheric plume models: 2. Scintillation calculation. J. Geophys. Res. 2010, 115, A03307. [Google Scholar] [CrossRef]
- Ossakow, S.L.; Chaturvedi, P.K. Morphological studies of rising equatorial spread F bubbles. J. Geophys. Res. 1978, 83, 2085–2090. [Google Scholar] [CrossRef]
- Bhattacharyya, A.; Fedrizzi, M.; Fuller-Rowell, T.J.; Gurram, P.; Kakad, B.; Sripathi, S.; Sunda, S. Effect of magnetic storm related thermospheric changes on the evolution of equatorial plasma bubbles. J. Geophys. Res. Space Phys. 2019, 124, 2256–2270. [Google Scholar] [CrossRef]
- Immel, T.; Harding, B.J.; Heelis, R.A.; Maute, A.; Forbes, J.M.; England, S.L.; Mende, S.B.; Englert, C.R.; Stoneback, R.A.; Marr, K.; et al. Regulation of ionospheric plasma velocities by thermospheric winds. Nat. Geosci. 2021, 14, 893–898. [Google Scholar] [CrossRef]
- Loi, S.T.; Murphy, T.; Cairns, I.H.; Menk, F.W.; Waters, C.L.; Erickson, P.J.; Trott, C.M.; Hurley-Walker, N.; Morgan, J.; Lenc, E.; et al. Real-time imaging of density ducts between the plasmasphere and ionosphere. Geophys. Res. Lett. 2015, 42, 3707–3714. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bhattacharyya, A. Equatorial Plasma Bubbles: A Review. Atmosphere 2022, 13, 1637. https://doi.org/10.3390/atmos13101637
Bhattacharyya A. Equatorial Plasma Bubbles: A Review. Atmosphere. 2022; 13(10):1637. https://doi.org/10.3390/atmos13101637
Chicago/Turabian StyleBhattacharyya, Archana. 2022. "Equatorial Plasma Bubbles: A Review" Atmosphere 13, no. 10: 1637. https://doi.org/10.3390/atmos13101637
APA StyleBhattacharyya, A. (2022). Equatorial Plasma Bubbles: A Review. Atmosphere, 13(10), 1637. https://doi.org/10.3390/atmos13101637




