Propagation of a Meteotsunami from the Yellow Sea to the Korea Strait in April 2019
Abstract
1. Introduction
2. Materials and Methods
2.1. Observational Data
2.2. Numerical Model Simulation
3. Results
3.1. Atmospheric and Ocean Observation
3.2. Numerical Simulations
4. Discussion
4.1. Proudman and Greenspan Resonances
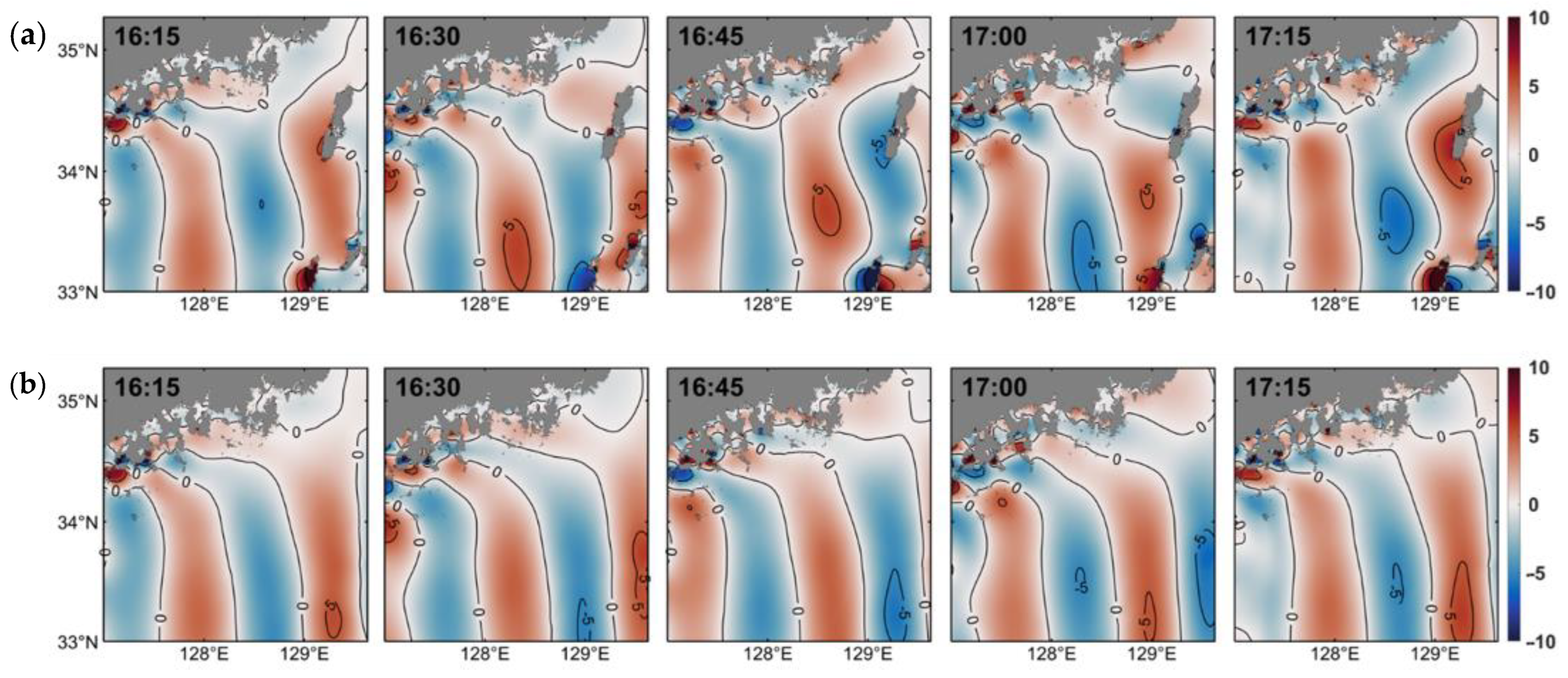
4.2. Refraction and Reflection by Offshore Islands
4.3. Local Topographic Effects
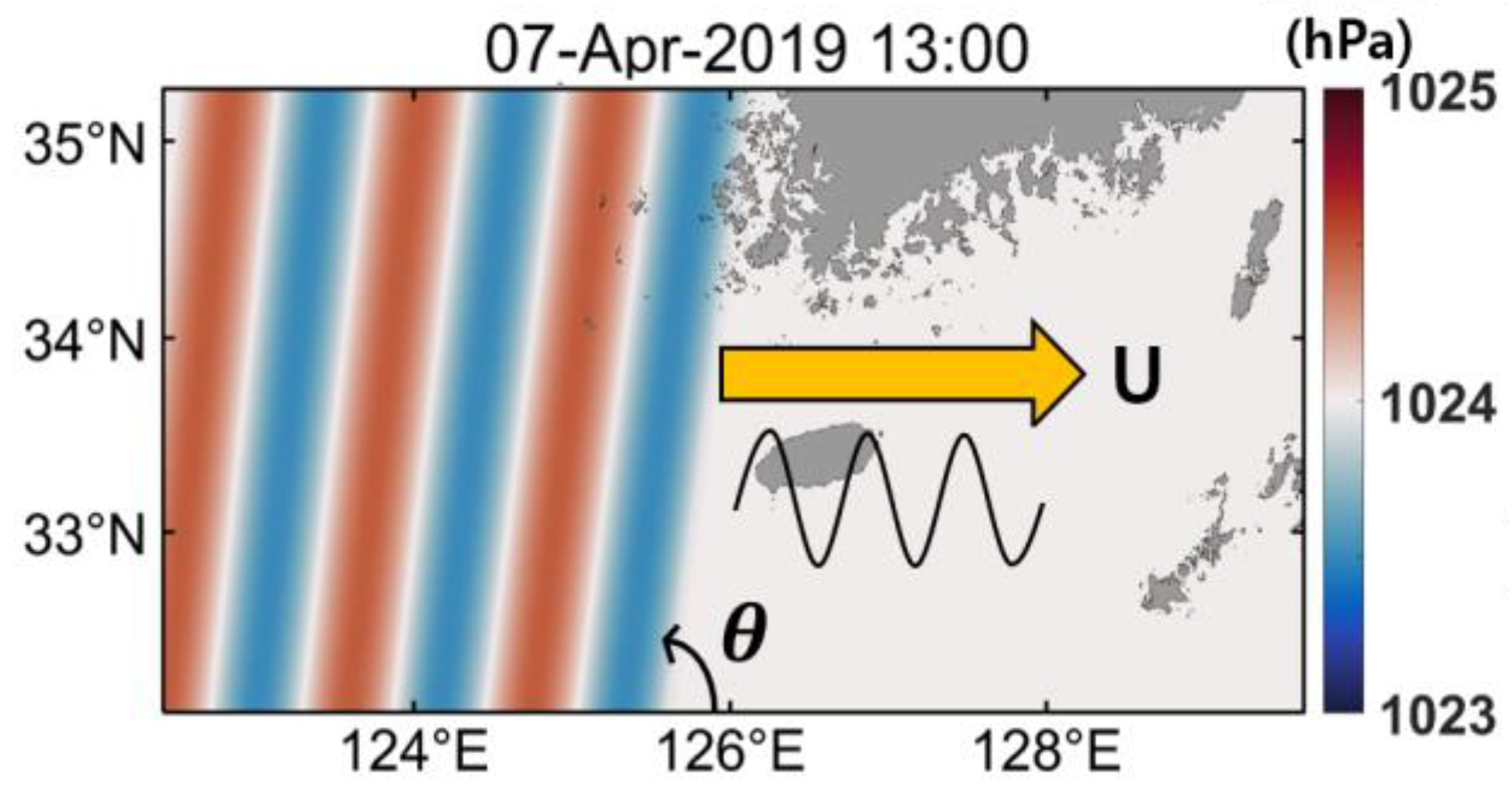
4.4. Effects of Speed and Angle of the Atmospheric Pressure Disturbances
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Monserrat, S.; Vilibić, I.; Rabinovich, A.B. Meteotsunamis: Atmospherically induced destructive ocean waves in the tsunami frequency band. Nat. Hazards Earth Syst. Sci. 2006, 6, 1035–1051. [Google Scholar] [CrossRef]
- Rabinovich, A.B. Twenty-Seven Years of Progress in the Science of Meteorological Tsunamis Following the 1992 Daytona Beach Event. Pure Appl. Geophys. 2020, 177, 1193–1230. [Google Scholar] [CrossRef]
- Proudman, J. The effects on the sea of changes in atmospheric pressure. Geophys. Suppl. Mon. Not. R. Astron. Soc. 1929, 2, 197–209. [Google Scholar] [CrossRef]
- Greenspan, H.P. The generation of edge waves by moving pressure distributions. J. Fluid Mech. 1956, 1, 574–592. [Google Scholar] [CrossRef]
- Pattiaratchi, C.B.; Wijeratne, E.M.S. Are meteotsunamis an underrated hazard? Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2015, 20140377. [Google Scholar] [CrossRef] [PubMed]
- Renault, L.; Vizoso, G.; Jansá, A.; Wilkin, J.; Tintoré, J. Toward the predictability of meteotsunamis in the Balearic Sea using regional nested atmosphere and ocean models. Geophys. Res. Lett. 2011, 38, L10601. [Google Scholar] [CrossRef]
- Vilibic, I. Numerical simulations of the Proudman resonance. Cont. Shelf. Res. 2008, 28, 574–581. [Google Scholar] [CrossRef]
- Vilibić, I.; Šepić, J.; Rabinovich, A.B.; Monserrat, S. Modern Approaches in Meteotsunami Research and Early Warning. Front. Mar. Sci. 2016, 3, 57. [Google Scholar] [CrossRef]
- Bailey, K.; DiVeglio, C.; Welty, A. An Examination of the June 2013 East Coast Meteotsunami Captured by NOAA Observing Systems. NOAA Technical Report; 2014; pp. 1–42. Available online: https://repository.library.noaa.gov/view/noaa/14435 (accessed on 20 August 2021).
- Šepić, J.; Vilibić, I.; Rabinovich, A.B.; Monserrat, S. Widespread tsunami-like waves of 23-27 June in the Mediterranean and Black Seas generated by high-altitude atmospheric forcing. Sci. Rep. 2015, 5, 11682. [Google Scholar] [CrossRef]
- Raichlen, F. Harbor resonance. In Estuary and Coastline Hydrodynamics, 1st ed.; Eagleson, P.S., Ippen, A.T., Eds.; McGraw Hill Book Co.: New York, NY, USA, 1966; pp. 281–340. [Google Scholar]
- Mei, C.C. The Applied Dynamics of Ocean Surface Waves, 2nd ed.; World Scientific: London, UK, 1992; p. 740. [Google Scholar]
- Wilson, B. Seiches. Adv. Hydrosci. 1972, 8, 1–94. [Google Scholar] [CrossRef]
- Miles, J.W. Harbor seiching. Ann. Rev. Fluid Mech. 1974, 6, 17–36. [Google Scholar] [CrossRef]
- Choi, B.-J.; Hwang, C.; Lee, S.-H. Meteotsunami-tide interactions and high-frequency sea level oscillations in the eastern Yellow Sea. J. Geophys. Res. Ocean. 2014, 119, 6725–6742. [Google Scholar] [CrossRef]
- Ozsoy, O.; Haigh, I.D.; Wadey, M.P.; Nicholls, R.J.; Wells, N.C. High-frequency sea level variations and implications for coastal flooding: A case study of the Solent, UK. Cont. Shelf Res. 2016, 122, 1–13. [Google Scholar] [CrossRef]
- Vilibić, I.; Rabinovich, A.B.; Anderson, E.J. Special issue on the global perspective on meteotsunami science: Editorial. Nat. Hazards 2021, 106, 1087–1104. [Google Scholar] [CrossRef]
- Hibiya, T.; Kajiura, K. Origin of the Abiki phenomenon (a kind of seiche) in Nagasaki Bay. J. Oceanogr. Soc. Jpn. 1982, 38, 172–182. [Google Scholar] [CrossRef]
- Tanaka, K. Atmospheric pressure-wave bands around a cold front resulted in a meteotsunami in the East China Sea in February 2009. Nat. Hazards Earth Syst. Sci. 2010, 10, 2599–2610. [Google Scholar] [CrossRef][Green Version]
- Heo, K.-Y.; Yoon, J.-S.; Bae, J.-S.; Ha, T. Numerical Modeling of Meteotsunami-Tide Interaction in the Eastern Yellow Sea. Atmosphere 2019, 10, 369. [Google Scholar] [CrossRef]
- Kim, M.-S.; Eom, H.; You, S.H.; Woo, S.-B. Real-time pressure disturbance monitoring system in the Yellow Sea: Pilot test during the period of March to April 2018. Nat. Hazards 2021, 106, 1703–1728. [Google Scholar] [CrossRef]
- Park, S.J.; Choi, B.-J.; Sim, H.S.; Byun, D.-S. Arrival of Long Ocean Waves and Hourly Sea Level Oscillations in Masan Bay, Korea on 19–22 March 2014. J. Coast. Res. 2020, 95, 1510–1514. [Google Scholar] [CrossRef]
- Haidvogel, D.B.; Arango, H.G.; Hedstrom, K. Model evaluation experiments in the North Atlantic Basin: Simulations in nonlinear terrain-following coordinates. Dynam. Atmos. Oceans 2000, 32, 239–281. [Google Scholar] [CrossRef]
- Shchepetkin, A.F.; McWilliams, J.C. The regional oceanic modeling system (ROMS): A split-explicit, free-surface, topography-following-coordinate oceanic model. Ocean Model. 2005, 9, 347–404. [Google Scholar] [CrossRef]
- Seo, S.-N. Digital 30sec gridded bathymetric data of Korea marginal seas—KorBathy30s. J. Korean Soc. Coast. Ocean. Eng. 2008, 20, 110–120. (In Korean) [Google Scholar]
- Chapman, D.C. Numerical treatment of cross-shelf open boundaries in a barotropic coastal ocean model. J. Phys. Oceanogr. 1985, 15, 1060–1075. [Google Scholar] [CrossRef]
- Flather, R.A. A tidal model of the northwest European continental shelf. Mem. Soc. R. Sci. Liege. 1976, 6, 141–164. [Google Scholar]
- Bechle, A.J.; Wu, C.H. The Lake Michigan meteotsunamis of 1954 revisited. Nat. Hazards 2014, 74, 155–177. [Google Scholar] [CrossRef]
- Williams, D.A.; Horsburgh, K.J.; Schultz, D.M.; Hughes, C.W. Examination of Generation Mechanisms for an English Channel Meteotsunami: Combining Observations and Modeling. J. Phys. Oceanogr. 2019, 49, 103–120. [Google Scholar] [CrossRef]
- Donn, W.L.; Ewing, M. Stokes’ edge waves in Lake Michigan. Science 1956, 124, 1238–1242. [Google Scholar] [CrossRef]
- Ursell, F. Edge waves on a sloping beach. Proc. R. Soc. Lond. Ser. A Math. Phys. Sci. 1952, 214, 79–98. [Google Scholar] [CrossRef]










| Station | Arrival Times (Hour:Minute) | Δt (Minutes) | ΔP (hPa) |
|---|---|---|---|
| CJ | 13:49 | 59 | 3.9 |
| SGP | 14:29 | 34 | 2.6 |
| GH | 14:15 | 17 | 2.1 |
| GM | 14:50 | 17 | 2.0 |
| YS | 14:33 | 12 | 2.1 |
| GJ | 15:58 | 29 | 1.9 |
| MS | 15:46 | 26 | 1.5 |
| GD | 16:21 | 33 | 2.5 |
| SSB | 17:35 | 71 | 2.7 |
| HKT | 18:15 | 66 | 2.7 |
| Station | Arrival Time (Hour:Minute) | Maximum Wave Height (cm) |
|---|---|---|
| CJ | 13:59 | 38.1 |
| SGP | 14:14 | 26.6 |
| GH | 15:07 | 42.5 |
| GM | 14:56 | 16.3 |
| YS | 15:48 | 29.7 |
| GJ | 16:47 | 30.1 |
| MS | 17:48 | 91.9 |
| GD | 17:05 | 30.1 |
| SSB | 17:18 | 38.6 |
| HKT | 20:08 | 39.0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kwon, K.; Choi, B.-J.; Myoung, S.-G.; Sim, H.-S. Propagation of a Meteotsunami from the Yellow Sea to the Korea Strait in April 2019. Atmosphere 2021, 12, 1083. https://doi.org/10.3390/atmos12081083
Kwon K, Choi B-J, Myoung S-G, Sim H-S. Propagation of a Meteotsunami from the Yellow Sea to the Korea Strait in April 2019. Atmosphere. 2021; 12(8):1083. https://doi.org/10.3390/atmos12081083
Chicago/Turabian StyleKwon, Kyungman, Byoung-Ju Choi, Sung-Gwan Myoung, and Han-Seul Sim. 2021. "Propagation of a Meteotsunami from the Yellow Sea to the Korea Strait in April 2019" Atmosphere 12, no. 8: 1083. https://doi.org/10.3390/atmos12081083
APA StyleKwon, K., Choi, B.-J., Myoung, S.-G., & Sim, H.-S. (2021). Propagation of a Meteotsunami from the Yellow Sea to the Korea Strait in April 2019. Atmosphere, 12(8), 1083. https://doi.org/10.3390/atmos12081083






