Mean Radiant Temperature Measurements through Small Black Globes under Forced Convection Conditions
Abstract
1. Introduction
2. Materials and Methods
2.1. Theoretical Background
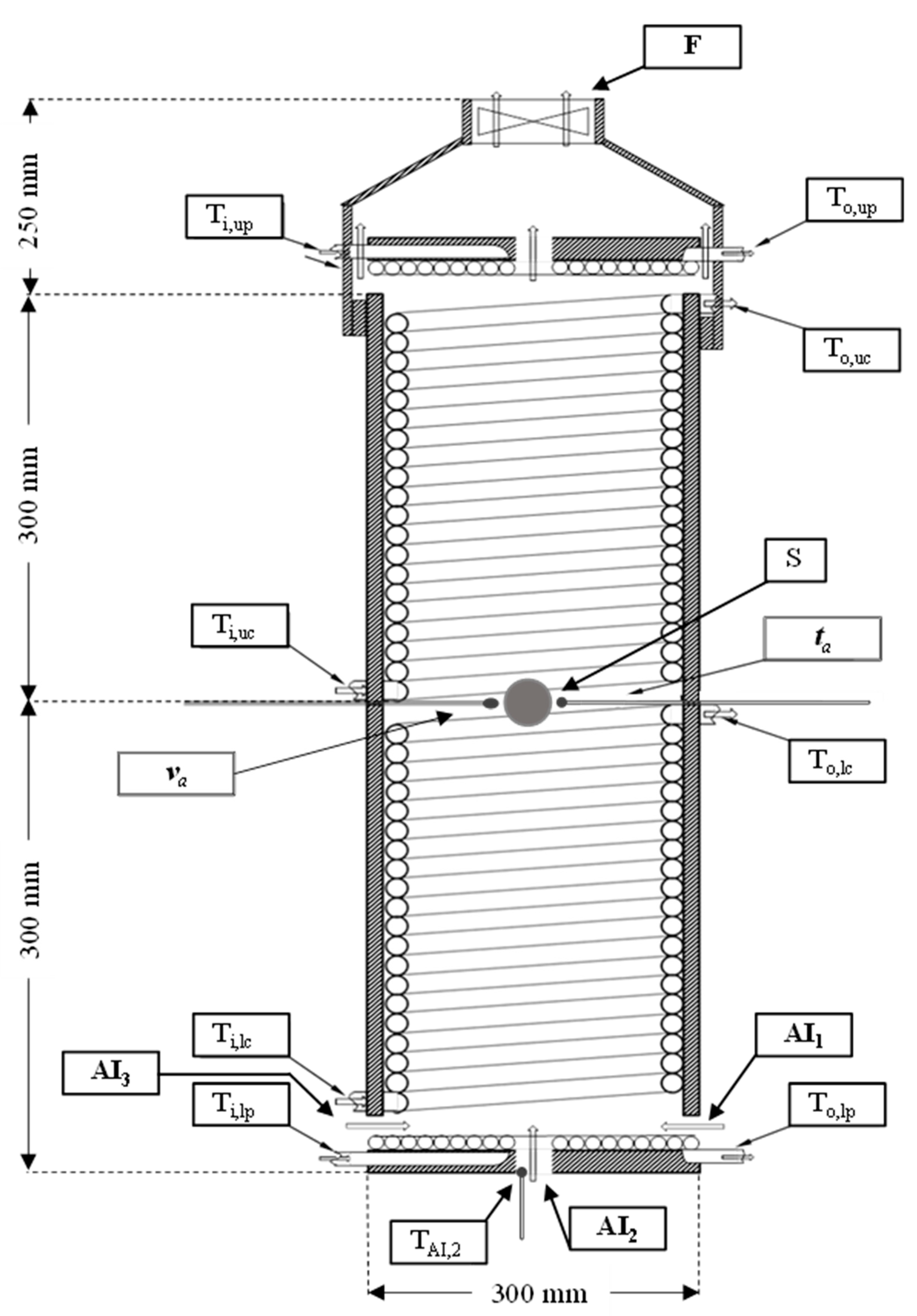
2.2. The Experimental Apparatus
- Two plates made by black painted spiral-wound copper tubes.
- Two stacked cylinders made by black painted solenoid-wound copper tubes.
- One hood provided with an air extraction system as shown in Figure 1.
2.3. The Measurement Protocol
- (a)
- Preparation
- Installation of the globe at the center of the enclosure as depicted in Figure 1.
- Setting thermostatic baths at a fixed temperature (in the range from 20 to 60 °C) to obtain the desired internal surface temperature.
- Setting fan at a fixed voltage (in the range from +3 to +13 V) to obtain a fixed value of the air velocity.
- (b)
- Measurement
- Reaching of steady state conditions for heat transfer fluid, air temperature and globe temperature. The mean value of the enclosure surface temperature ts has been used as a reference value for the mean radiant temperature of each single run. It was calculated from the mean heat transfer fluid temperatures recorded at the inlet and the outlet of the 4 main components of the apparatus. These values were finally averaged accounting for the angle factor between the globe and each component of the enclosure (the two plates and the two staked cylinders).
- Sampling duration of 15 min with a sampling rate of 1 min.
- Starting of a new single run as summarized above.
3. Results and Discussion
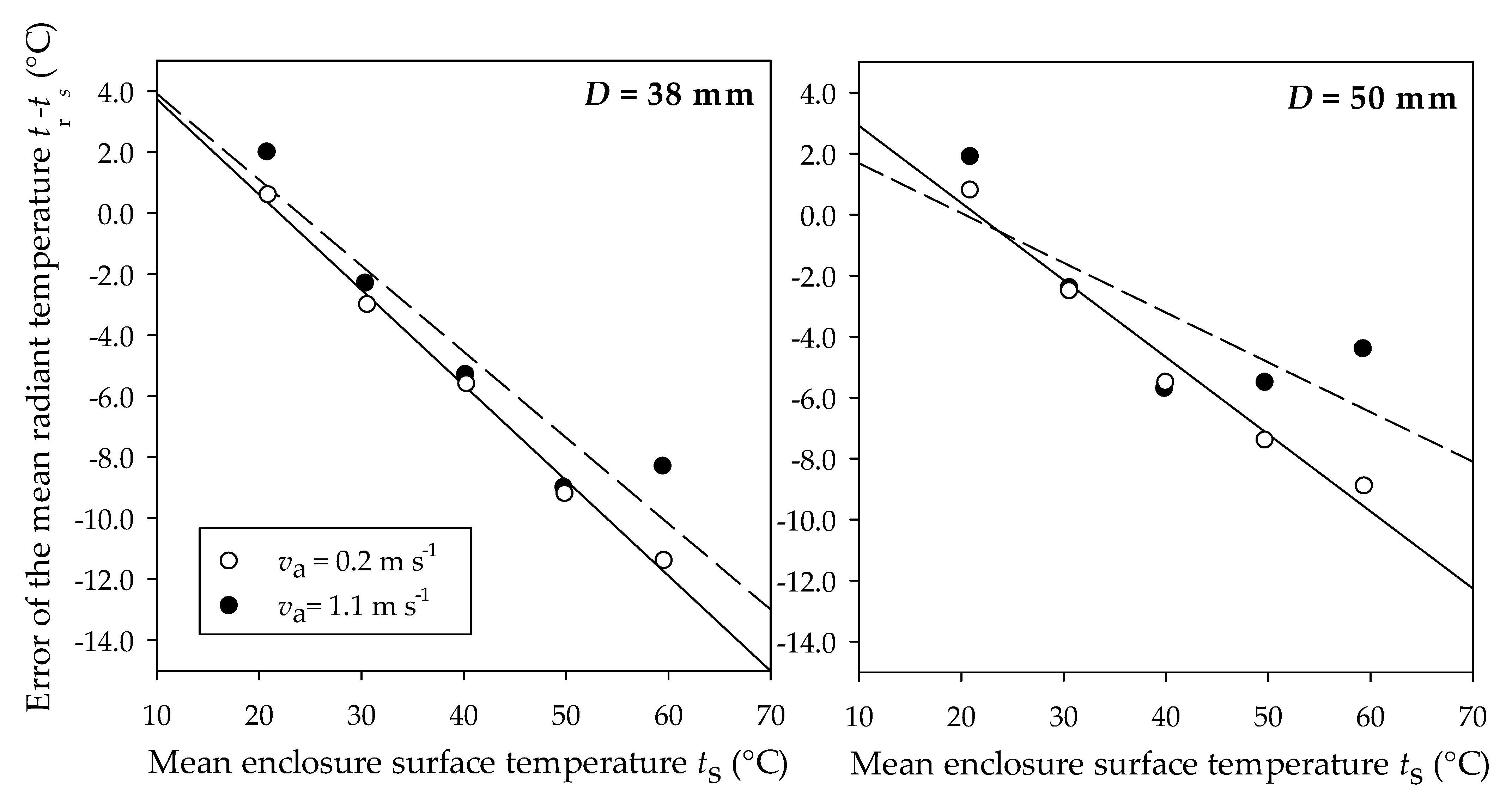
3.1. Mean Radiant Temperature Measurements
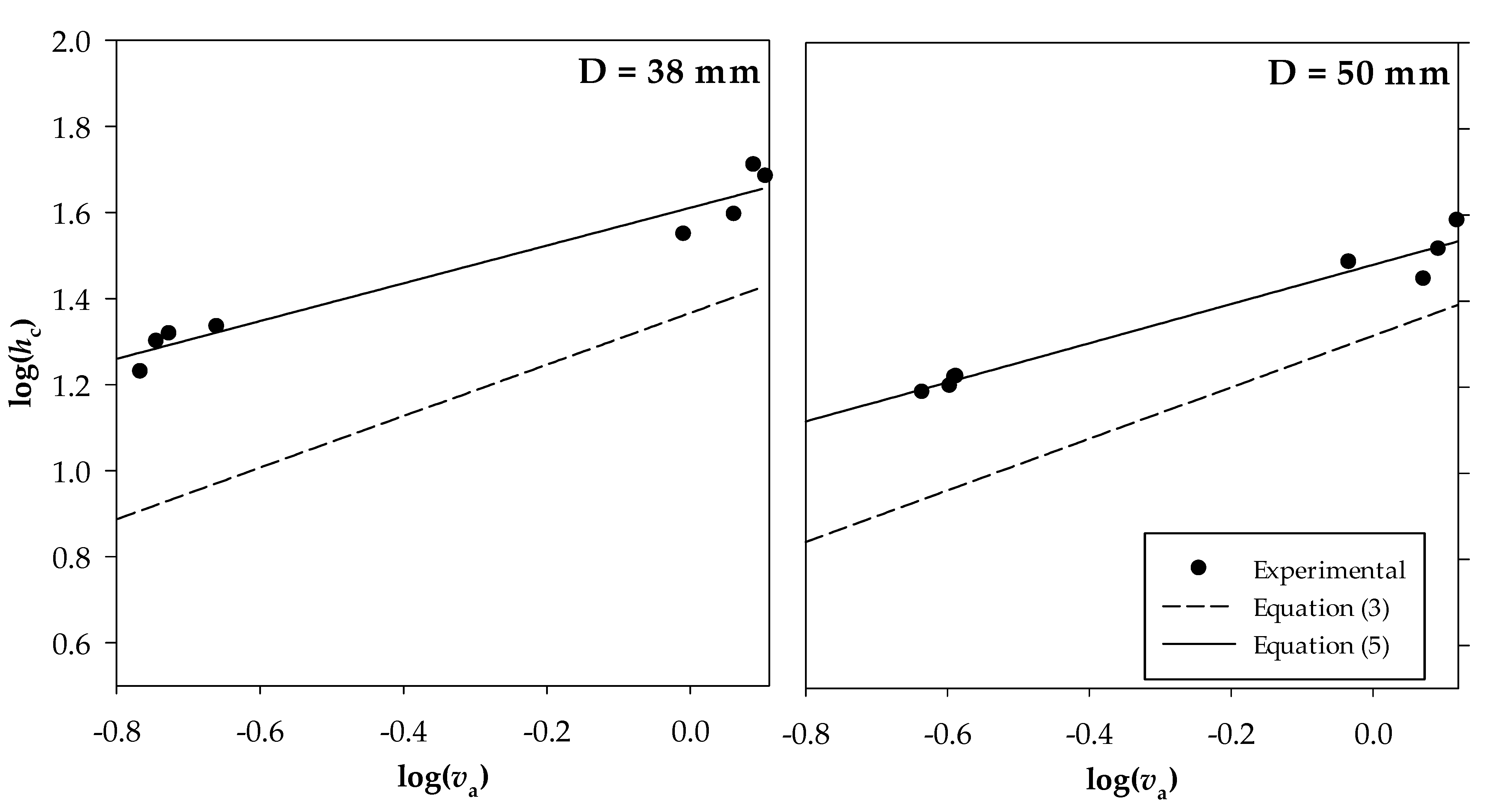
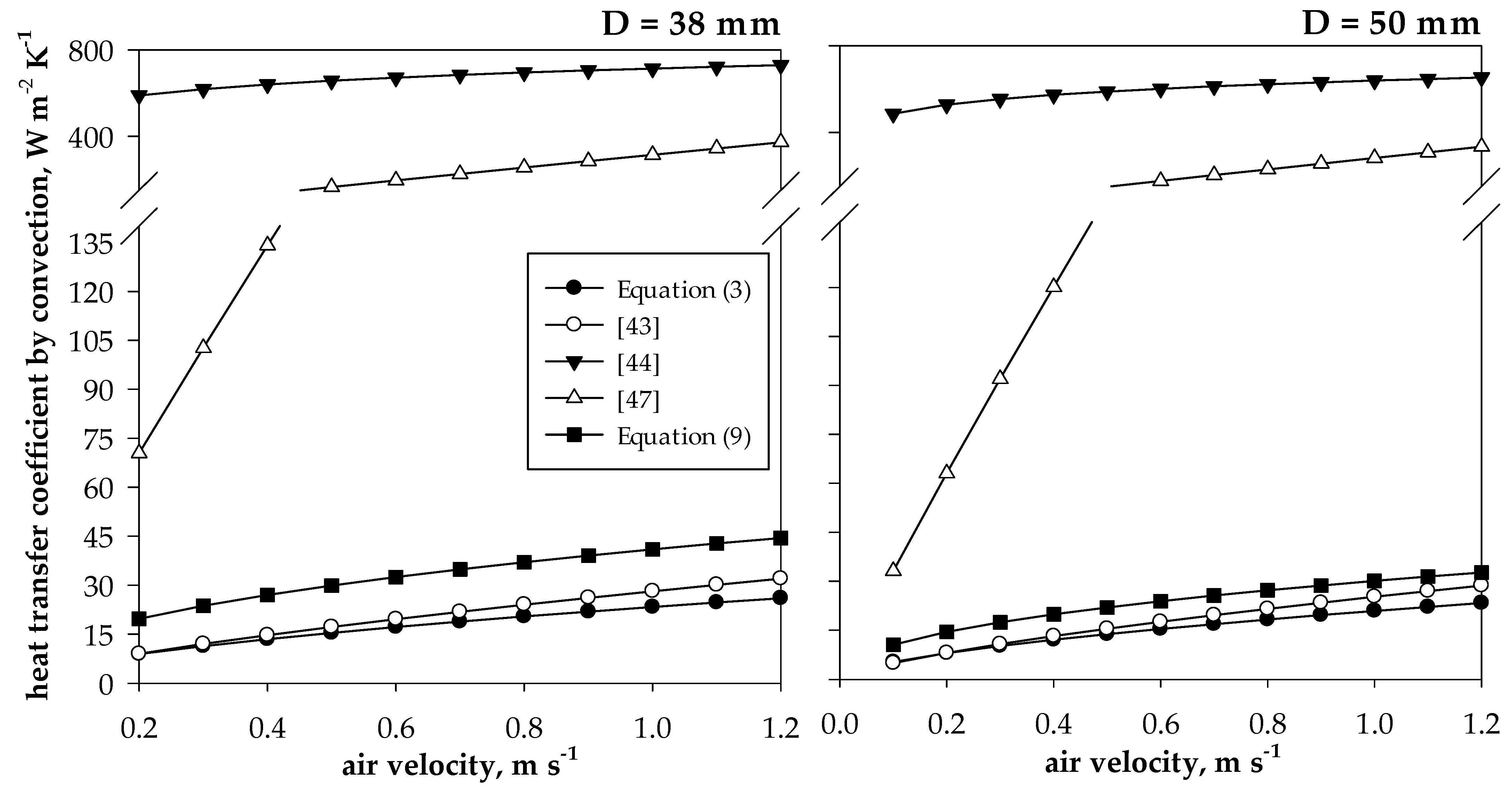
3.2. Analysis of the Heat Transfer by Convection on the Globes
- through one simple empirical power law:hc,g = a0 (va)m
- through two power laws, formulated consistently to the Nusselt number’s definition as a function of the diameter of the globe D:hc,g = Nu kf/DNu = a Rem
3.3. Impact of Measurement Errors on the Assessment of Thermal Environments
- Thermal Comfortta = 22.8 °C; tr = ts = 30.0 °C; va = 0.2 m s−1; RH = 50%
- Heat stressta = 28.6 °C; tr = ts = 50.0 °C; va = 0.2 m s−1; RH = 50%
4. Conclusions
- Firstly, in the presence of high-radiative loads, small globes exhibit a general trend of underestimation of the mean radiant temperature calculated according to the ISO 7726 model. This phenomenon is affected by the temperature of the enclosure (generally, the warmer the enclosure, the greater the underestimation) and the air velocity (at higher air velocity, the errors are reduced by 3–4 °C).
- Moreover, larger errors have been observed for a 38 mm globe (i.e., table tennis ball) leading to mean radiant temperature values even 11.4 °C lower than the enclosure temperature. On the other hand, the 50 mm globe showed lower errors (i.e., up to 8.9 °C).
- The main effect can be ascribed to the underestimation of the heat transfer convective coefficients calculated according to the ISO 7726 Standard. To this aim, the authors proposed new experimental correlations leading to an accuracy within 1.0 ÷ 1.5 °C for the mean radiant temperature.
- The measurement errors exhibited by small globes lead to critical issues in the objective assessment of thermal comfort and heat stress when ISO 7726 algorithms are used. On the contrary, the proposed experimental correlations result in a more reliable assessment of microclimatic conditions.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Symbols
| a | Constant in Equation (7) |
| a0 | Constant in Equation (5) |
| a’0 | Constant in Equation (8) |
| a’’0 | Constant in Equation (9) |
| D | Globe diameter, mm |
| DLE | Duration of limit exposure, min |
| Dlimloss,95 | Maximum allowable exposure time for water loss, 95% of the working population, min |
| Dlim,tre | Maximum allowable exposure time for heat storage, min |
| hc,g | Convective heat transfer coefficient of the globe W m−2 K−1 |
| Icl | Basic clothing insulation, m2 K W−1 or clo |
| kf | Air thermal conductivity, W m−1 K−1 |
| m | Coefficient in in Equations (5), (8) and (9), 1 |
| n | Coefficient in Equation (9), 1 |
| Nu | Nusselt’s number, 1 |
| PMV | Predicted mean vote |
| PHS | Predicted heat strain |
| Re | Reynolds’ number, 1 |
| RH | Relative humidity, % |
| ta | Air temperature, °C |
| tg | Globe temperature, °C |
| ts | Mean surface temperature of the enclosure, °C |
| tr | Mean radiant temperature, °C |
| va | Air velocity, m s−1 |
| WBGT | Wet bulb globe temperature, °C |
| ε | Globe emissivity, 1 |
| ν | Cinematic viscosity or air, m2 s−1 |
| σ | Stefan–Boltzmann constant, W m−2 K−4 |
References
- Simberloff, D. The Balance of Nature—Evolution of a Panchreston. PLoS Biol. 2014, 12, e1001963. [Google Scholar] [CrossRef] [PubMed]
- United Nations Framework Convention on Climate Change (UNFCCC). The Paris Agreement. 2016. Available online: https://unfccc.int/sites/default/files/resource/parisagreement_publication.pdf (accessed on 11 May 2021).
- Patz, J.A.; Campbell-Lendrum, D.; Holloway, T.; Foley, J.A. Impact of regional climate change on human health. Nature 2005, 438, 310–317. [Google Scholar] [CrossRef]
- Watts, N.; Amann, M.; Arnell, N.; Ayeb-Karlsson, S.; Belesova, K.; Boykoff, M.; Byass, P.; Cai, W.; Campbell-Lendrum, D.; Capstick, S.; et al. The 2019 report of The Lancet Countdown on health and climate change: Ensuring that the health of a child born today is not defined by a changing climate. Lancet 2019, 394, 1836–1878. [Google Scholar] [CrossRef]
- Global Alliance for Buildings and Construction; International Energy Agency; United Nations Environment Programme. 2019 Global Status Report for Buildings and Construction: Towards a Zero-Emission, Efficient and Resilient Buildings, and Construction Sector; Global Alliance for Buildings and Construction: Paris, France, 2019. [Google Scholar]
- European Parliament. Council Directive of 16 December 2002 on the energy performance of buildings (2002/91/EC). Off. J. Eur. Communities 2003, 46, 65–71. [Google Scholar]
- European Parliament. Directive 2018/844/EU of The European Parliament and of the Council of 30 May 2018 amending Directive 2010/31/EU on the energy performance of buildings and Directive 2012/27/EU on energy efficiency. Off. J. Eur. Communities 2018, 61, 75–91. [Google Scholar]
- European Committee for Standardization. EN 15251. Indoor Environmental Input Parameters for Design and Assessment of Energy Performance of Buildings Addressing Indoor Air Quality, Thermal Environment, Lighting and Acoustics; European Committee for Standardization: Brussels, Belgium, 2007. [Google Scholar]
- European Committee for Standardization. EN 16798-1. Energy Performance of Buildings—Ventilation for Buildings—Part 1: Indoor Environmental Input Parameters for Design and Assessment of Energy Performance of Buildings Addressing Indoor Air Quality, Thermal Environment, Lighting and Acoustics—Module M1-6; European Committee for Standardization: Brussels, Belgium, 2019. [Google Scholar]
- European Committee for Standardization. EN 16798-2. Energy Performance of Buildings—Ventilation for Buildings—Part 2: Interpretation of the Requirements in EN 16798—Indoor Environmental Input Parameters for Design and Assessment of Energy Performance of Buildings Addressing Indoor Air Quality, Thermal Environment, Lighting and Acoustics (Module M1-6); European Committee for Standardization: Brussels, Belgium, 2019. [Google Scholar]
- International Standardization Organization. ISO 17772-1. Energy Performance of Buildings—Indoor Environmental Quality—Part 1: Indoor Environmental Input Parameters for the Design and Assessment of Energy Performance of Buildings; International Standardization Organization: Geneva, Switzerland, 2017. [Google Scholar]
- Attaianese, E.; Duca, G. The human component of sustainability: A study for assessing human performances of energy efficient construction blocks. Work 2012, 41, 2141–2146. [Google Scholar] [CrossRef]
- Andersson, J.; Boerstra, A.; Clements-Croome, D.; Fitzner, K.; Hanssen, S.O. Indoor Climates and Productivity in Offices—REHVA Guidebook No. 6; REHVA: Brussels, Belgium, 2006. [Google Scholar]
- Mahmoud, S.; Zayed, T.; Fahmy, M. Development of sustainability assessment tool for existing buildings. Sustain. Cities Soc. 2019, 44, 99–119. [Google Scholar] [CrossRef]
- Olesen, B.W. The philosophy behind EN 15251: Indoor environmental criteria for design and calculation of energy performance of buildings. Energ. Build. 2007, 39, 740–749. [Google Scholar] [CrossRef]
- d’Ambrosio Alfano, F.R.; Olesen, B.W.; Palella, B.I.; Riccio, G. Thermal comfort: Design and assessment of energy saving. Energ. Build. 2014, 81, 326–336. [Google Scholar] [CrossRef]
- ASHRAE. Thermal comfort. In ASHRAE Fundamentals; American Society of Heating, Refrigerating and Air Conditioning Engineers: Atlanta, GA, USA, 2017. [Google Scholar]
- Fanger, P.O. Thermal Comfort; Danish Technical Press: Copenhagen, Denmark, 1970. [Google Scholar]
- Parsons, K.C. Human Thermal Environments: The Effects of Hot, Moderate, and Cold Environments on Human Health, Comfort and Performance, 3rd ed.; Taylor and Francis: London, UK, 2014. [Google Scholar]
- ISO. ISO 7726. Ergonomics of the Thermal Environment—Instruments for Measuring Physical Quantities; International Standardization Organization: Geneva, Switzerland, 1998. [Google Scholar]
- d’Ambrosio Alfano, F.R.; Dell’Isola, M.; Palella, B.I.; Riccio, G.; Russi, A. On the measurement of the mean radiant temperature and its influence on the indoor thermal environment assessment. Build. Environ. 2013, 63, 79–88. [Google Scholar] [CrossRef]
- Vernon, H.M. The measurement of radiant heat in relation to human comfort. J. Ind. Hyg. 1932, 14, 95–111. [Google Scholar]
- ISO. ISO 7243. Ergonomics of the Thermal Environment—Assessment of Heat Stress using the WBGT (Wet Bulb Globe Temperature) Index; International Standardization Organization: Geneva, Switzerland, 2017. [Google Scholar]
- ACGIH. Threshold Limit Values for Chemical Substances and Physical Agents and Biological Exposures Indices; American Conference of Governmental Industrial Hygienists: Cincinnati, OH, USA, 2019. [Google Scholar]
- d’Ambrosio Alfano, F.R.; Dell’Isola, M.; Ficco, G.; Palella, B.I.; Riccio, G. On the Measurement of the Mean Radiant Temperature by means of Globes: An Experimental Investigation under Black Enclosure Conditions. Build. Environ. 2021, 193, 107655. [Google Scholar] [CrossRef]
- ISO. ISO 7730. Ergonomics of the Thermal Environment—Analytical Determination and Interpretation of Thermal Comfort using Calculation of the PMV and PPD Indices and Local Thermal Comfort; International Standardization Organization: Geneva, Switzerland, 2005. [Google Scholar]
- ANSI. ASHRAE 55. Thermal Environmental Conditions for Human Occupancy; American Society of Heating, Refrigerating, and Air-Conditioning Engineers, Inc.: Atlanta, GA, USA, 2021. [Google Scholar]
- Oliveira, A.V.M.; Raimundo, A.M.; Gaspar, A.R.; Quintela, D.A. Globe Temperature and Its Measurement: Requirements and Limitations. Ann. Work Expo. Health 2019, 63, 743–758. [Google Scholar] [CrossRef] [PubMed]
- Guo, H.; Aviv, D.; Loyola, M.; Teitelbaum, E.; Houchois, N.; Meggers, F. On the understanding of the mean radiant temperature within both the indoor and outdoor environment, a critical review. Renew. Sust. Energ. Rev. 2020, 117, 109207. [Google Scholar] [CrossRef]
- Guo, H.; Ferrara, M.; Coleman, J.; Loyola, M.; Meggers, F. Simulation and measurement of air temperatures and mean radiant temperatures in a radiantly heated indoor space. Energy 2020, 193, 116369. [Google Scholar] [CrossRef]
- Humphreys, M.A. The optimum diameter for a globe thermometer for use indoors. Ann. Occup. Hyg. 1977, 20, 135–140. [Google Scholar]
- Fountain, M. Instrumentation for Thermal Comfort Measurements: The Globe Thermometer; UC Berkeley—Center for the Built Environment: Berkeley, CA, USA, 1987; Available online: https://escholarship.org/uc/item/1qx8c7sm (accessed on 9 May 2021).
- Fontana, L. Experimental study on the globe thermometer behaviour in conditions of asymmetry of the radiant temperature. Appl. Therm. Eng. 2010, 30, 732–740. [Google Scholar] [CrossRef]
- Wang, S.; Li, Y. Suitability of acrylic and copper globe thermometers for diurnal outdoor settings. Build. Environ. 2015, 89, 279–294. [Google Scholar] [CrossRef]
- de Sampaio, P.C.; Terezo, R.F.; Júnior, I.V.; da Silva, L.M.; Borges, L.K. Hysteresis and Thermal Inertia of Spheres of Alternative Materials for Black Globe Thermometers. Eng. Agríc. 2019, 39, 567–572. [Google Scholar] [CrossRef]
- Obando Vega, F.A.; Montoya Rìos, A.P.; Osorio Saraz, J.A.; Vargas Quiroz, L.G.; Damasceno, F.A. Assessment of black globe thermometers employing various sensors and alternative materials. Agr. For. Meteorol. 2020, 284, 107891. [Google Scholar] [CrossRef]
- McIntyre, D.A. Indoor Climate; Applied Science Publisher Ltd.: London, UK, 1980. [Google Scholar]
- Quintela, D.A.; Oliveira, A.V.M.; Cardoso, D. Assessment of the performance of globe thermometers under different environmental conditions. In Occupational Safety and Hygiene II; Arezes, P.M., Baptista, J.S., Barroso, M.P., Carneiro, P., Cordeiro, P., Costa, N., Melo, R.B., et al., Eds.; CRC Press: London, UK, 2014; pp. 523–528. [Google Scholar]
- Halawa, E.E.H. Operative Temperature Measurement and Control. Master’s Thesis, School of Manufacturing and Mechanical Engineering, University of South Australia, Adelaide, Australia, 1994. [Google Scholar]
- Bernard, T.E.; Barrow, A. Empirical Approach to Outdoor WBGT from Meteorological Data and Performance of Two Different Instrument Designs. Ind. Health 2013, 51, 79–85. [Google Scholar] [CrossRef] [PubMed]
- d’Ambrosio Alfano, F.R.; Malchaire, J.; Palella, B.I.; Riccio, G. The WBGT index revisited after 60 years of use. Ann. Occup. Hyg. 2014, 58, 955–970. [Google Scholar] [CrossRef]
- Vargas-Salgado, C.; Chiñas-Palacios, C.; Aguila-Leónc, J.; Alfonso-Solar, D. Measurement of the black globe temperature to estimate the MRT and WBGT indices using a smaller diameter globe than a standardized one: Experimental analysis. In Proceedings of the 5th CARPE Conference, Valencia, Spain, 23–25 October 2019; pp. 201–207. [Google Scholar] [CrossRef]
- Thorsson, S.; Lindberg, F.; Eliasson, I.; Holmer, B. Different methods for estimating the mean radiant temperature in an outdoor urban setting. Int. J. Climatol. 2007, 27, 1983–1993. [Google Scholar] [CrossRef]
- Tan, N.H.; Wong, S.; Jusuf, S.K. Outdoor mean radiant temperature estimation in the tropical urban environment. Build. Environ. 2013, 64, 118–129. [Google Scholar] [CrossRef]
- Teitelbaum, E.; Rysanek, A.; Pantelic, J.; Aviv, D.; Obelz, S.; Buff, A.; Luo, Y.; Sheppard, D.; Meggers, F. Revisiting radiant cooling: Condensation-free heat rejection using infrared-transparent enclosures of chilled panels. Archit. Sci. Rev. 2019, 62, 152–159. [Google Scholar] [CrossRef]
- Guo, H.; Teitelbaum, E.; Houchois, N.; Bozlar, M.; Meggers, F. Revisiting the use of globe thermometers to estimate radiant temperature in studies of heating and ventilation. Energ. Build. 2018, 180, 83–94. [Google Scholar] [CrossRef]
- Manavvi, S.; Rajasekar, E. Estimating outdoor mean radiant temperature in a humid subtropical climate. Build. Environ. 2020, 171, 106658. [Google Scholar] [CrossRef]
- Höppe, P. A new procedure to determine the mean radiant temperature outdoors. Wetter unt Leben 1992, 44, 147–151. [Google Scholar]
- Kàntor, N.; Unger, J. The most problematic variable in the course of human biometeorological comfort assessment—The mean radiant temperature. Cent. Eur. J. Geosci. 2011, 3, 90–100. [Google Scholar] [CrossRef]
- Lin, T.-P.; Matzarakis, A. Estimation of outdoor mean radiant temperature by field experiment and modelling for human biometeorology use. In Proceedings of the 11th Annual Meeting of the European Meteorological Society, Berlin, Germany, 19–22 September 2011. [Google Scholar]
- Chen, Y.G.; Lin, T.P.; Matzarakis, A. Comparison of mean radiant temperature from field experiment and modelling: A case study in Freiburg, Germany. Theor. Appl. Climatol. 2014, 118, 535–551. [Google Scholar] [CrossRef]
- Teitelbaum, E.; Chen, K.W.; Meggers, F.; Guo, H.; Houchois, N.; Pantelic, J.; Rysanek, A. Globe thermometer free convection error potentials. Sci. Rep. 2020, 10, 2652. [Google Scholar] [CrossRef] [PubMed]
- ISO. ISO 7933. Ergonomics of the Thermal Environment—Analytical Determination and Interpretation of Heat Stress using Calculation of the Predicted Heat Strain; International Organization for Standardization: Geneva, Switzerland, 2004. [Google Scholar]
- Höppe, P. The physiological equivalent temperature—A universal index for the biometeorological assessment of the thermal environment. Int. J. Biometeorol. 1999, 43, 71–75. [Google Scholar] [CrossRef] [PubMed]
- Matzarakis, A.; Mayer, H.; Iziomon, M.G. Applications of a universal thermal index: Physiological equivalent temperature. Int. J. Biometeorol. 1999, 43, 76–84. [Google Scholar] [CrossRef]
- Steiger, H.; Matzarakis, A. Accuracy of Mean Radiant Temperature Derived from Active and Passive Radiometry. Atmosphere 2020, 11, 805. [Google Scholar] [CrossRef]
- Jendritzky, G.; de Dear, R.; Havenith, G. UTCI-Why another thermal index? Int. J. Biometeorol. 2012, 56, 421–428. [Google Scholar] [CrossRef] [PubMed]
- Havenith, G.; Fiala, D. Thermal Indices and Thermophysiological Modeling for Heat Stress. Compr. Physiol. 2016, 6, 255–302. [Google Scholar] [CrossRef]
- Fiala, D.; Lomas, K.J.; Stohrer, M. A computer model of human thermoregulation for a wide range of environmental conditions: The passive system. J. Appl. Physiol. 1999, 87, 1957–1972. [Google Scholar] [CrossRef]
- Kobayashi, Y.; Tanabe, S. Development of JOS-2 human thermoregulation model with detailed vascular system. Build. Environ. 2013, 66, 1–10. [Google Scholar] [CrossRef]
- Houchois, N.; Teitelbaum, E.; Chen, K.W.; Rucewicz, S.; Meggers, F. The SMART sensor: Fully characterizing radiant heat transfer in the built environment. J. Phys. Conf. Ser. 2019, 1343, 012073. [Google Scholar] [CrossRef]
- Park, H.; Rhee, S. IoT-Based Smart Building Environment Service for Occupants’ Thermal Comfort. J. Sens. 2018, 1757409, 1757409. [Google Scholar] [CrossRef]
- Izhar, U.H.; Wang, X.; Xu, W.; Tavakkoli, H.; Yuen, Z.; Shan, X.; Lee, Y.H. Integrated Predicted Mean Vote Sensing System Using MEMS Multi-Sensors for Smart HVAC Systems. IEEE Sens. J. 2021, 21, 8400–8410. [Google Scholar] [CrossRef]
- Tomat, V.; Ramallo-González, A.P.; Skarmeta Gómez, A.F. A Comprehensive Survey about Thermal Comfort under the IoT Paradigm: Is Crowdsensing the New Horizon? Sensors 2020, 20, 4647. [Google Scholar] [CrossRef]
- Kreith, F.; Mangli, R.M.; Bohn, M.S. Principles of Heat Transfer; Cengage Learning Inc.: Stamford, CT, USA, 2011. [Google Scholar]
- SigmaPlot. Systat Software; SigmaPlot: San Jose, CA, USA, 2012. [Google Scholar]
- ISO. ISO 8996. Ergonomics of the Thermal Environment—Determination of Metabolic Rate; International Standardization Organization: Geneva, Switzerland, 2004. [Google Scholar]
- ISO. ISO 9920. Ergonomics of the Thermal Environment—Estimation of Thermal Insulation and Water Vapour Resistance of a Clothing Ensemble; International Standardization Organization: Geneva, Switzerland, 2007. [Google Scholar]
- d’Ambrosio Alfano, F.R.; Palella, B.I.; Riccio, G. Notes on the implementation of the IREQ model for the assessment of extreme cold environments. Ergonomics 2013, 56, 707–724. [Google Scholar] [CrossRef] [PubMed]
- d’Ambrosio Alfano, F.R.; Palella, B.I.; Riccio, G.; Toftum, J. Fifty years of Fanger’s equation: Is there anything to discover yet? Int. J. Ind. Ergonom. 2018, 66, 157–160. [Google Scholar] [CrossRef]
- d’Ambrosio Alfano, F.R.; Olesen, B.W.; Palella, B.I.; Pepe, D.; Riccio, G. Fifty years of PMV model: Reliability, implementation, and design of software for its calculation. Atmosphere 2020, 11, 49. [Google Scholar] [CrossRef]
- d’Ambrosio Alfano, F.R.; Palella, B.I.; Riccio, G. The Role of Measurement Accuracy on the Thermal Environment Assessment by means of PMV Index. Build. Environ. 2011, 46, 1361–1369. [Google Scholar] [CrossRef]
- d’Ambrosio Alfano, F.R.; Palella, B.I.; Riccio, G. The role of Measurement Accuracy on the Heat Stress Assessment according to ISO 7933: 2004. WIT Trans. Biomed. Health 2007, 11, 115–124. [Google Scholar] [CrossRef]


| Description | Globe A | Globe B |
|---|---|---|
| Globe thickness | 1.5 mm | 1.5 mm |
| Globe diameter | 38 mm | 50 mm |
| Temperature sensor type | Pt-100 | Pt-100 |
| Set Point Temperature of the Baths (°C) | Vertical Air Temperature Gradient in the Enclosure (°C cm−1) | Vertical Air Temperature Gradient in the Globe (°C) | ||
|---|---|---|---|---|
| D = 38 mm | D = 50 mm | D = 38 mm | D = 50 mm | |
| va = 0.2 m s−1 | ||||
| 20 | 0.00 | 0.01 | 0.0 | 0.1 |
| 30 | 0.08 | 0.06 | 0.2 | 0.2 |
| 40 | 0.15 | 0.18 | 0.5 | 0.6 |
| 50 | 0.23 | 0.24 | 0.7 | 0.8 |
| 60 | 0.29 | 0.37 | 0.9 | 1.1 |
| va = 1.1 m s−1 | ||||
| 20 | 0.00 | 0.01 | 0.0 | 0.0 |
| 30 | 0.06 | 0.05 | 0.2 | 0.2 |
| 40 | 0.12 | 0.17 | 0.4 | 0.5 |
| 50 | 0.20 | 0.22 | 0.7 | 0.7 |
| 60 | 0.24 | 0.30 | 0.8 | 1.0 |
| Set Point Temperature of the Baths (°C) | D = 38 mm | D = 50 mm | ||
|---|---|---|---|---|
| va = 0.2 m s−1 | va = 1.1 m s−1 | va = 0.2 m s−1 | va = 1.1 m s−1 | |
| 20 | 18.7 | 18.5 | 18.8 | 18.6 |
| 30 | 22.8 | 22.6 | 22.6 | 22.4 |
| 40 | 27.4 | 26.2 | 21.4 | 20.8 |
| 50 | 28.6 | 27.9 | 25.5 | 24.9 |
| 60 | 31.6 | 29.9 | 29.4 | 26.6 |
| ts (°C) | D = 38 mm | D = 50 mm | ||
|---|---|---|---|---|
| va = 0.2 m s−1 | va = 1.1 m s−1 | va = 0.2 m s−1 | va = 1.1 m s−1 | |
| 20 | 0.6 | 2.0 | 0.8 | 1.9 |
| 30 | −3.0 | −2.3 | −2.5 | −2.4 |
| 40 | −5.6 | −5.3 | −5.5 | −5.7 |
| 50 | −9.2 | −9 | −7.4 | −5.5 |
| 60 | −11.4 | −8.3 | −8.9 | −4.4 |
| MEAN | −5.7 | −4.6 | −4.7 | −3.2 |
| Equation | a0 | a’0 | a’’0 | m | n | R2 | Data Set |
|---|---|---|---|---|---|---|---|
| (5) | 40.8 | - | - | 0.439 | - | 0.950 | D = 38 mm |
| (5) | 30.5 | - | - | 0.455 | - | 0.962 | D = 50 mm |
| (8) | - | 5.78 | - | 0.431 | - | 0.906 | D = 38 mm and D = 50 mm |
| (9) | - | - | 1.05 | 0.455 | 1.12 | 0.941 | D = 38 mm and D = 50 mm |
| a’’0 | m | n | Ref. | Data Set/Experimental Conditions |
|---|---|---|---|---|
| 6.3 | 0.6 | 0.4 | [20] | No restrictions |
| 7.6 | 0.71 | 0.4 | [43] | D = 38 mm; grey globe; va = 0.1 ÷ 4.0 m s−1 incoming short-wave radiation 100 ÷ 850 W m−2 (clear summer/autumn days in Göteborg) |
| 194 | 0.119 | 0.4 | [44] | D = 40 mm; grey globe; va = 0.1 ÷ 4.0 m s−1incoming short-wave radiation up to 1300 W/m2 (February–April and August–September in Singapore) |
| 85 | 0.93 | 0.4 | [47] | Small globe placed on a portable data logger; va = 0.1 ÷ 4.0 m s−1 incoming short-wave radiation up to 950 W m−2 (March, May, and December in Singapore) |
| ts (°C) | Equation (5) | Equation (8) | Equation (9) | [43] | ||||
|---|---|---|---|---|---|---|---|---|
| 0.2 m s−1 | 1.1 m s−1 | 0.2 m s−1 | 1.1 m s−1 | 0.2 m s−1 | 1.1 m s−1 | 0.2 m s−1 | 1.1 m s−1 | |
| D = 38 mm | ||||||||
| 20 | 2.7 | 4.3 | 2.4 | 3.8 | 2.6 | 4.4 | 0.7 | 2.9 |
| 30 | 0.6 | 0.9 | 0.1 | 0.3 | 0.7 | 1.2 | −3 | −1.4 |
| 40 | −0.4 | −0.7 | −1.1 | −1.7 | −0.6 | −0.6 | −5.6 | −3.7 |
| 50 | −2.4 | −2.3 | −2 | −3.8 | −1.2 | −2.2 | −9.2 | −6.2 |
| 60 | −0.6 | 2.2 | −2.1 | 0 | −1 | 2.3 | −11.3 | −4.9 |
| MEAN | −0.1 | 0.9 | −0.5 | −0.3 | 0.1 | 1.0 | −5.7 | −2.7 |
| D = 50 mm | ||||||||
| 20 | 2.1 | 3.2 | 2.3 | 3.3 | 2.1 | 3.1 | 1 | 2.8 |
| 30 | 0.1 | 1.8 | 0.5 | 0 | 0 | −0.4 | −2.4 | −1.6 |
| 40 | 0.3 | −1.6 | 1.3 | −1.2 | 0.1 | −1.9 | −5.2 | −3.2 |
| 50 | −0.3 | 0.2 | 0.9 | 0.9 | −0.5 | 0 | −7 | −2.2 |
| 60 | −0.4 | 3.7 | 1.1 | 4.7 | −0.6 | 3.3 | −8.4 | −0.3 |
| MEAN | 0.4 | 1.5 | 1.2 | 1.5 | 0.2 | 0.8 | −4.4 | −0.9 |
| tr | Globe A D = 38 mm | Globe B D = 50 mm | ||
|---|---|---|---|---|
| PMV | Category | PMV | Category | |
| tr = ts (reference) | −0.33 | II | −0.33 | II |
| Equation (3) | −0.76 | IV | −0.68 | III |
| Equation (5) | −0.24 | II | −0.31 | II |
| Equation (8) | −0.31 | II | −0.25 | II |
| Equation (9) | −0.21 | II | −0.33 | II |
| tr | Globe A D = 38 mm | Globe B D = 50 mm | ||||
|---|---|---|---|---|---|---|
| DLE | Dlim,tre | Dlim,loss95 | DLE | Dlim,tre | Dlim,loss95 | |
| tr = ts (reference) | 297 | >480 | 297 | 297 | >480 | 297 |
| Equation (3) | 388 | 388 | 368 | 368 | ||
| Equation (5) | 318 | 318 | 299 | 299 | ||
| Equation (8) | 315 | 315 | 305 | 305 | ||
| Equation (9) | 307 | 307 | 301 | 301 | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
d’Ambrosio Alfano, F.R.; Ficco, G.; Frattolillo, A.; Palella, B.I.; Riccio, G. Mean Radiant Temperature Measurements through Small Black Globes under Forced Convection Conditions. Atmosphere 2021, 12, 621. https://doi.org/10.3390/atmos12050621
d’Ambrosio Alfano FR, Ficco G, Frattolillo A, Palella BI, Riccio G. Mean Radiant Temperature Measurements through Small Black Globes under Forced Convection Conditions. Atmosphere. 2021; 12(5):621. https://doi.org/10.3390/atmos12050621
Chicago/Turabian Styled’Ambrosio Alfano, Francesca Romana, Giorgio Ficco, Andrea Frattolillo, Boris Igor Palella, and Giuseppe Riccio. 2021. "Mean Radiant Temperature Measurements through Small Black Globes under Forced Convection Conditions" Atmosphere 12, no. 5: 621. https://doi.org/10.3390/atmos12050621
APA Styled’Ambrosio Alfano, F. R., Ficco, G., Frattolillo, A., Palella, B. I., & Riccio, G. (2021). Mean Radiant Temperature Measurements through Small Black Globes under Forced Convection Conditions. Atmosphere, 12(5), 621. https://doi.org/10.3390/atmos12050621










