The Modulation Effect on the ELVEs and Sprite Halos by Concentric Gravity Waves Based on the Electromagnetic Pulse Coupled Model
Abstract
1. Introduction
2. Establishment of Tropospheric Mid-to-High Altitude EMP Model
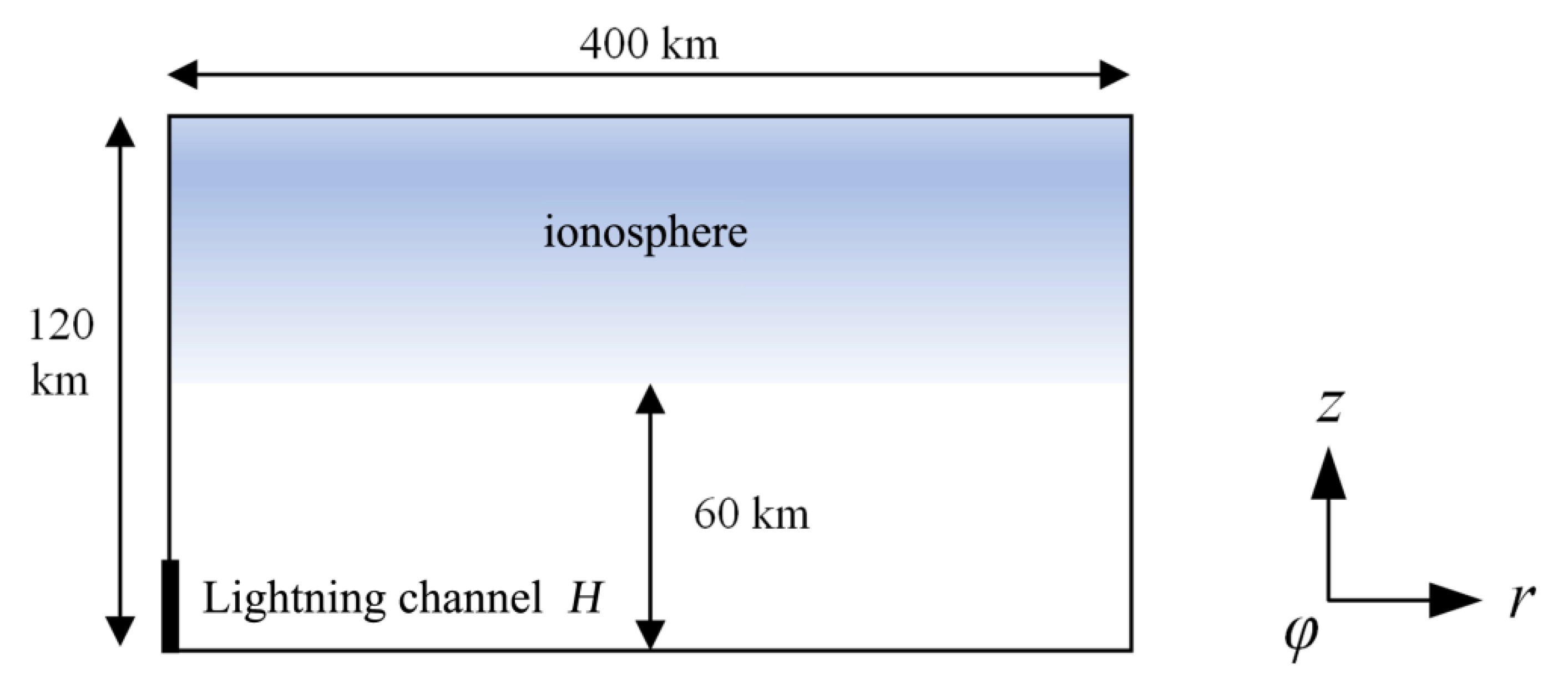
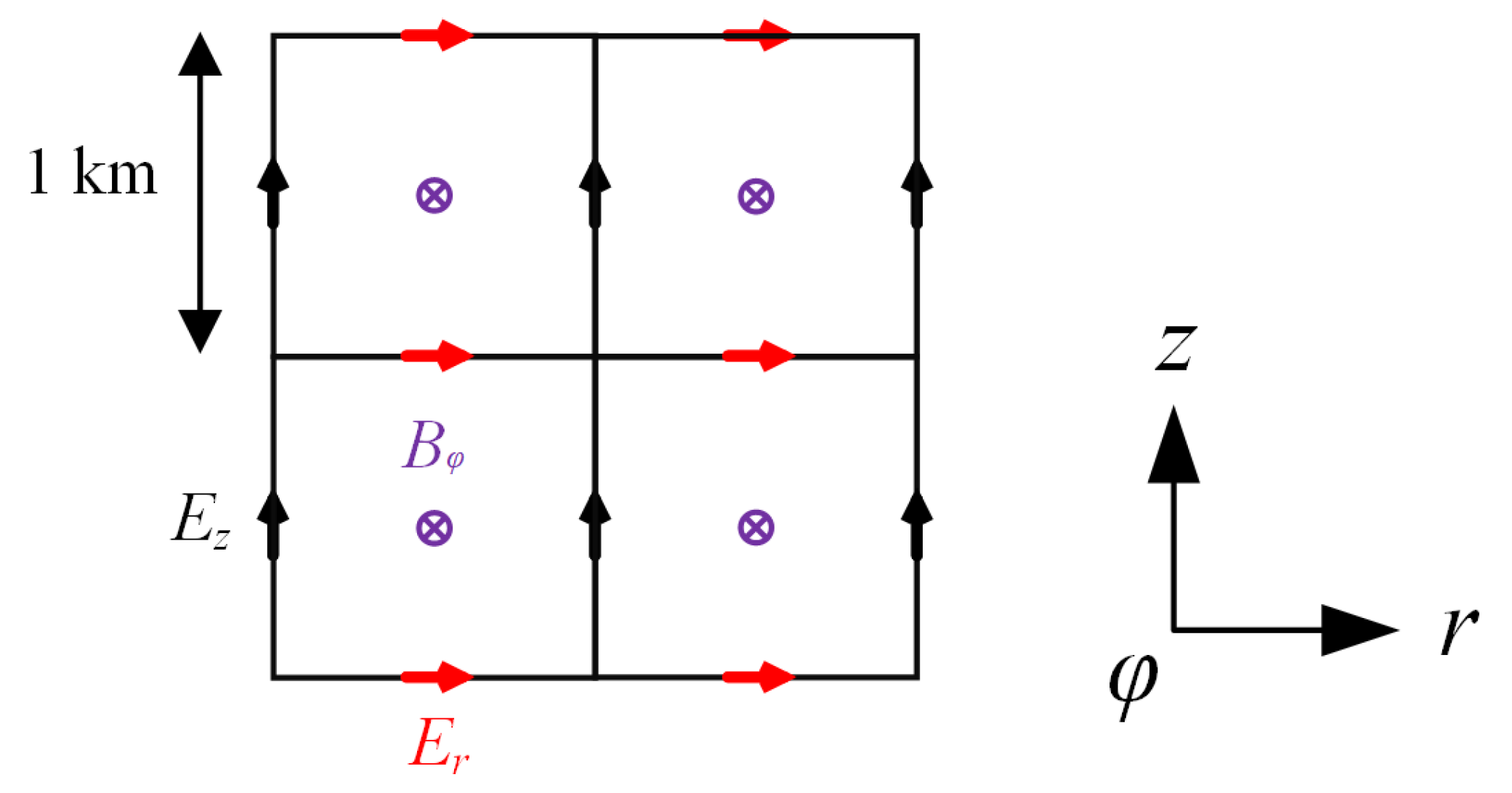
2.1. Introduction of 2D FDTD EMP Model
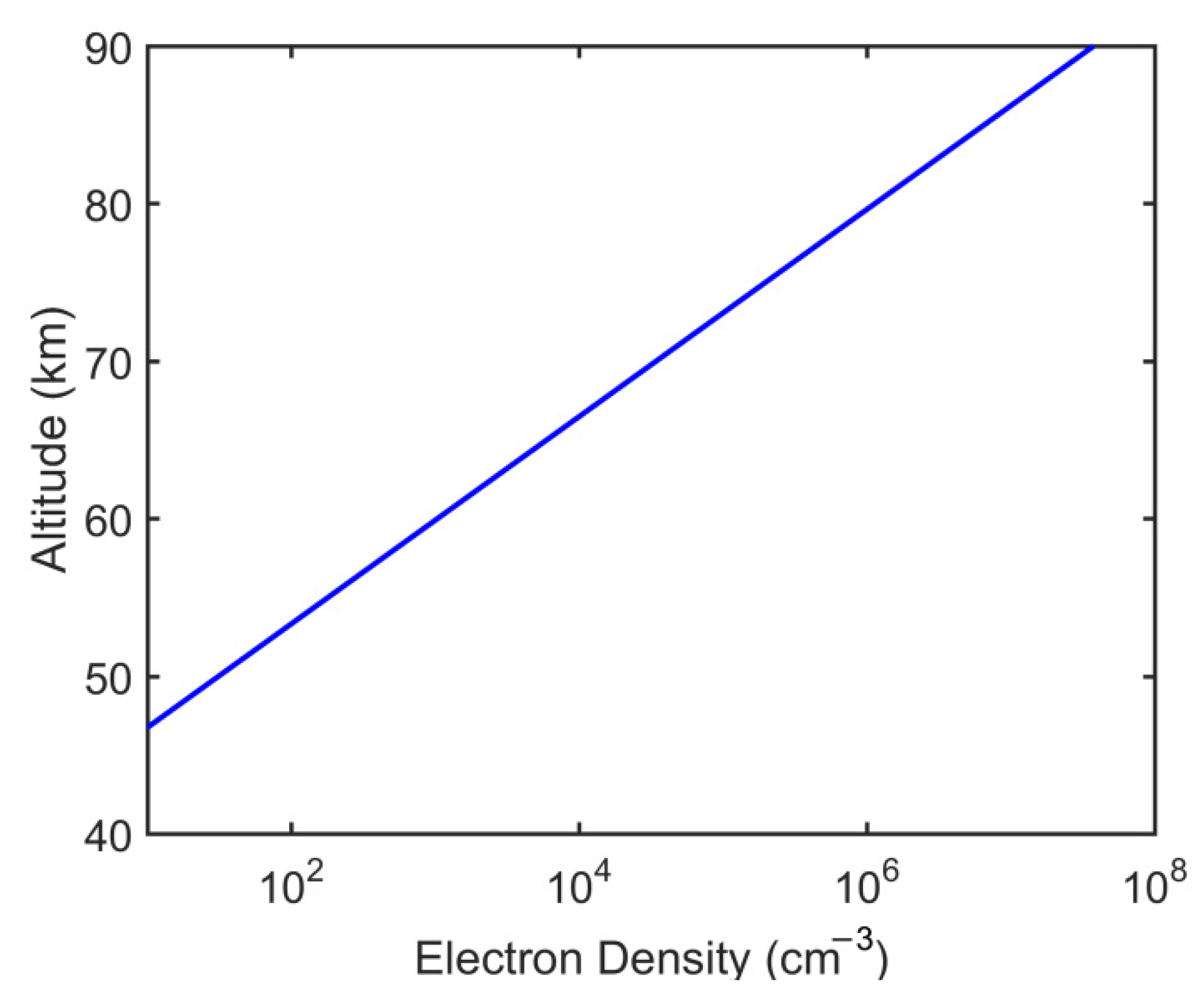
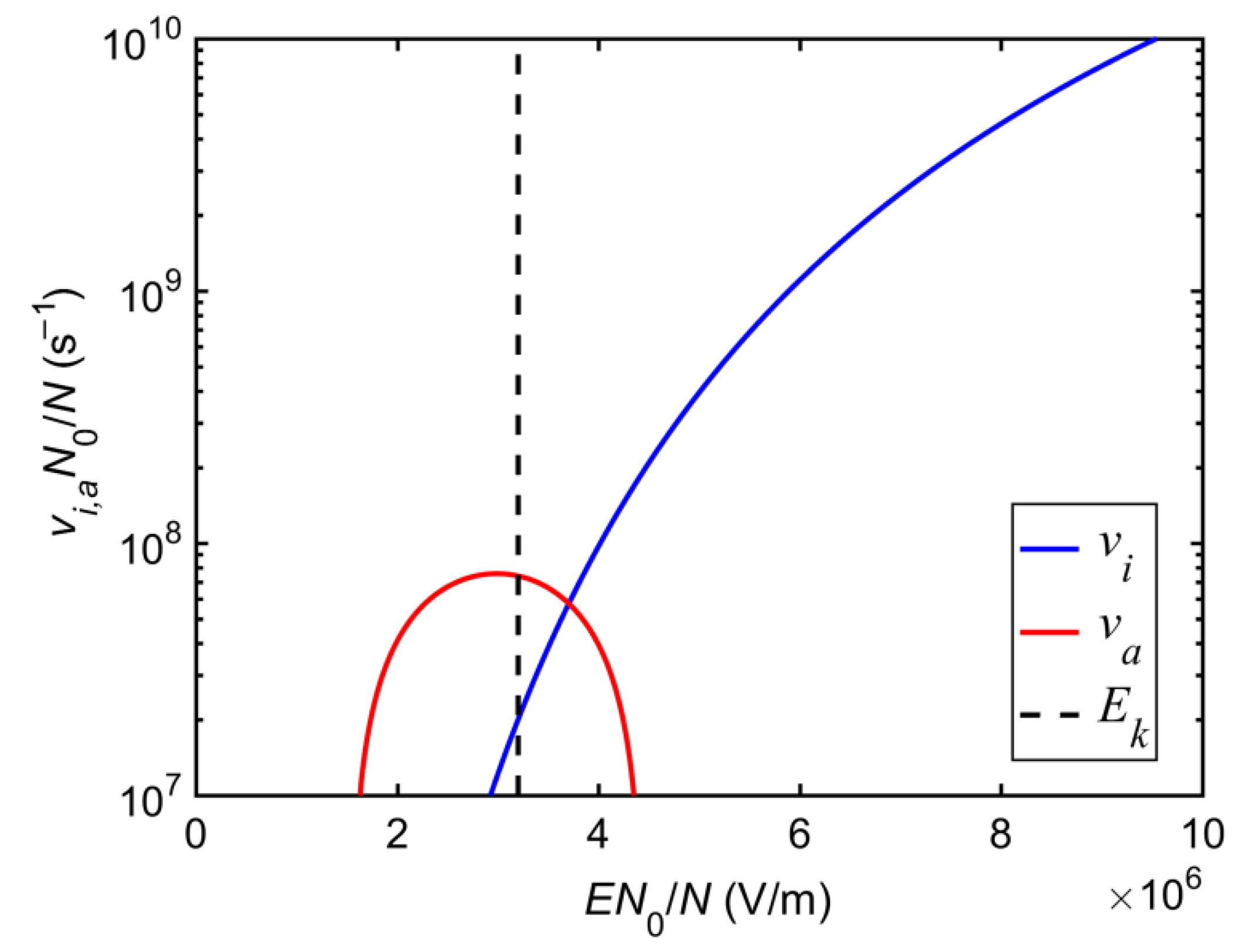
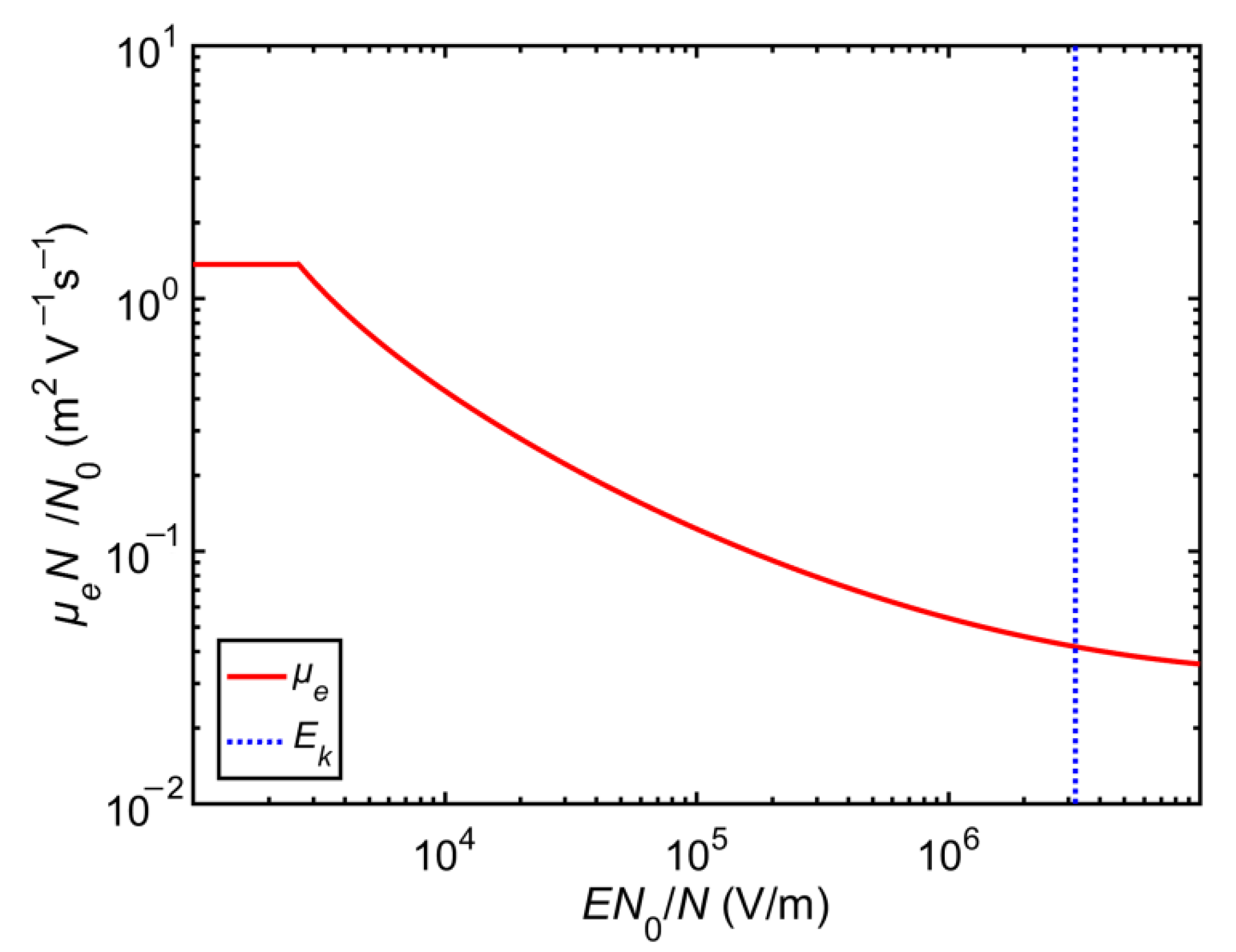
2.2. Calculation of Ionospheric Parameters
2.3. Calculation of Optical Radiation
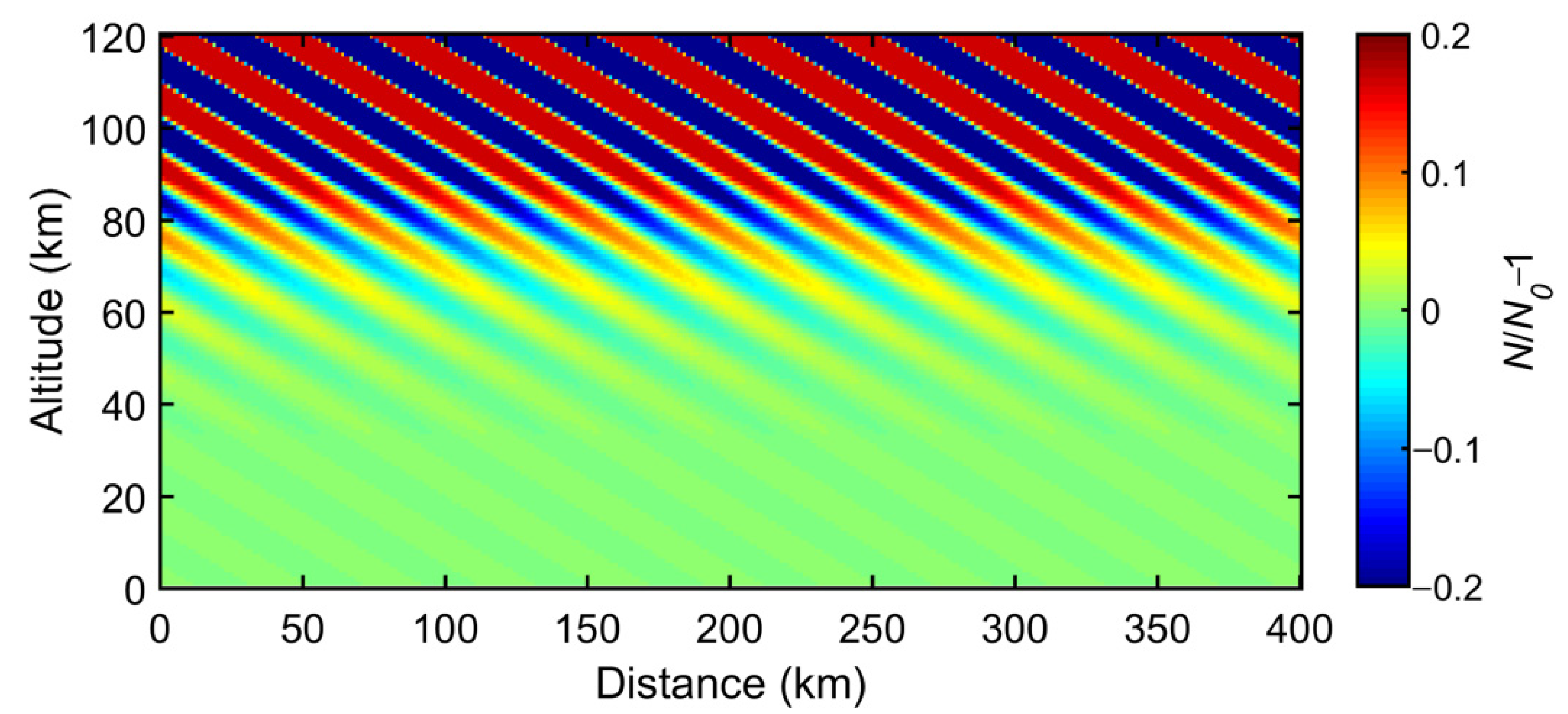
2.4. Parameterization of CGWs
2.5. Algorithm Accuracy Verification
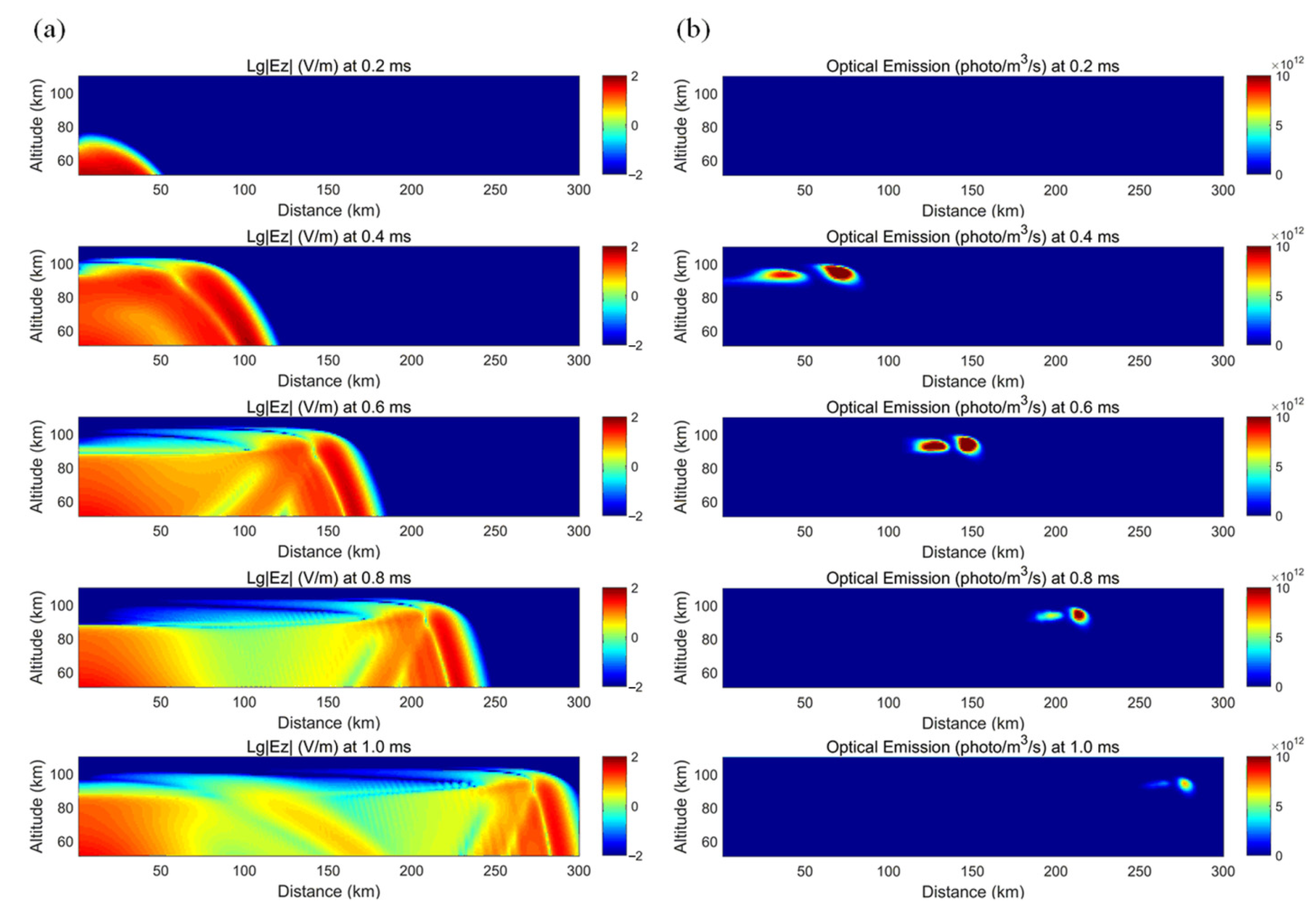
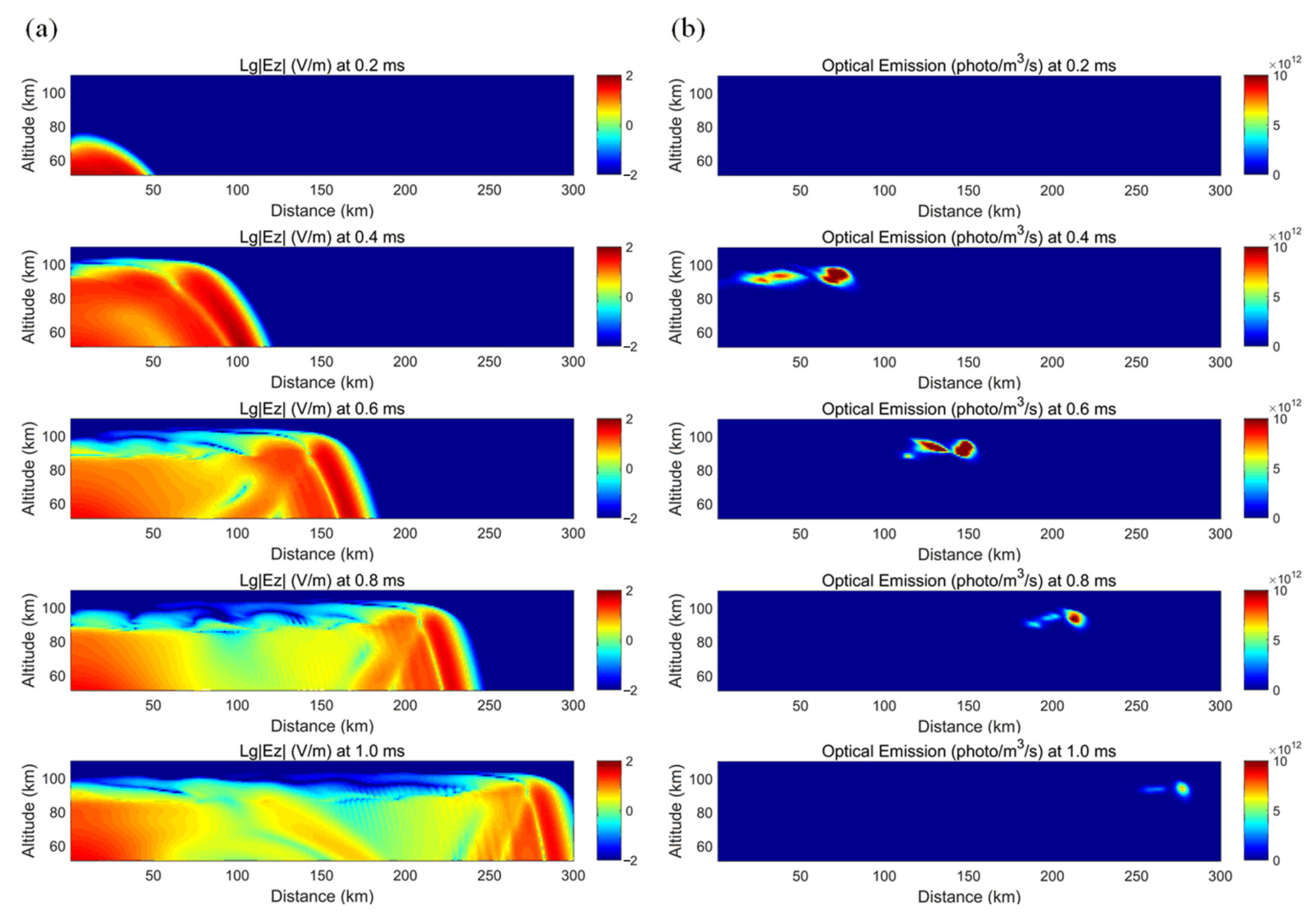
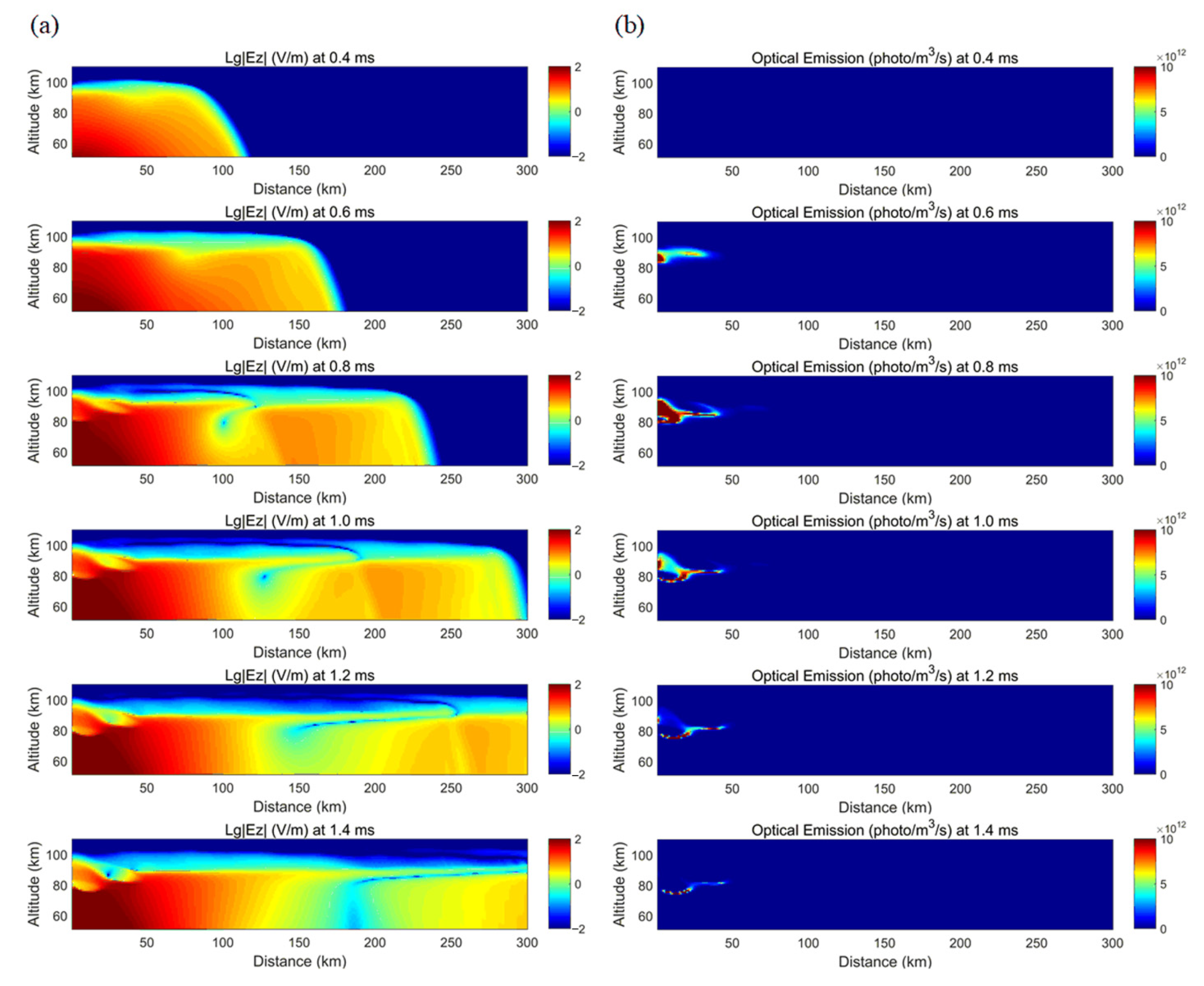
3. Effect of Gravity Wave Disturbance on Mid-to-High Altitude ELVEs
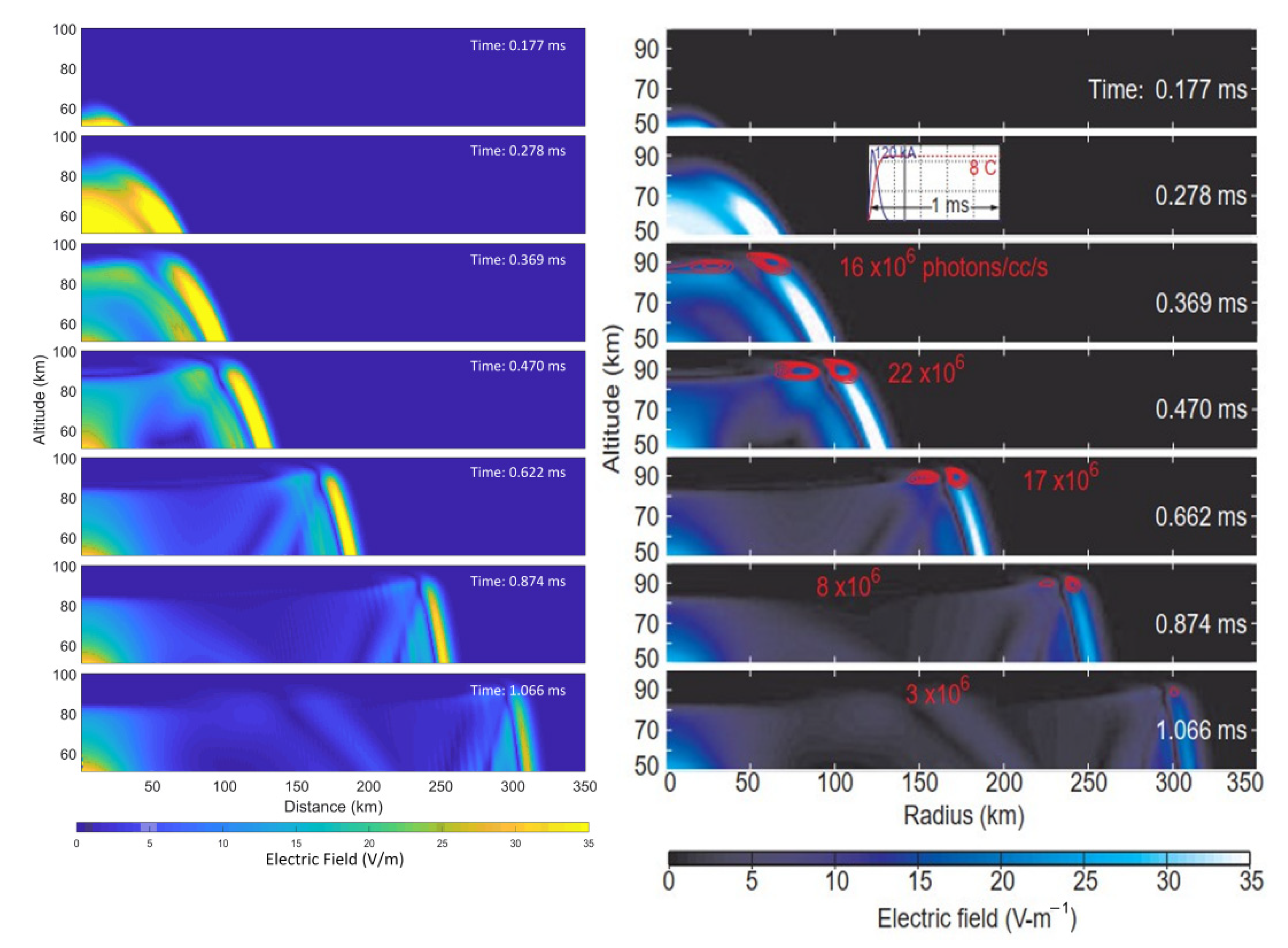
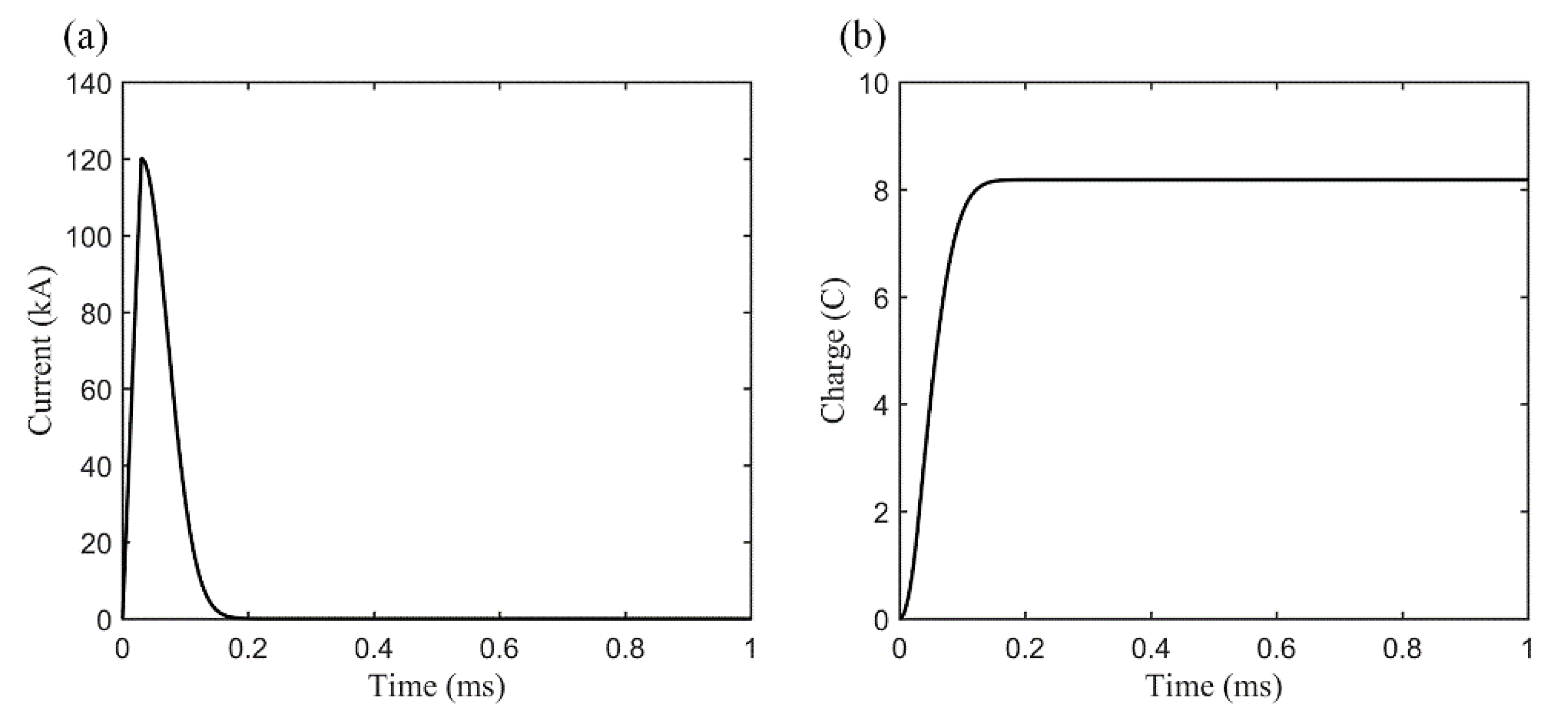
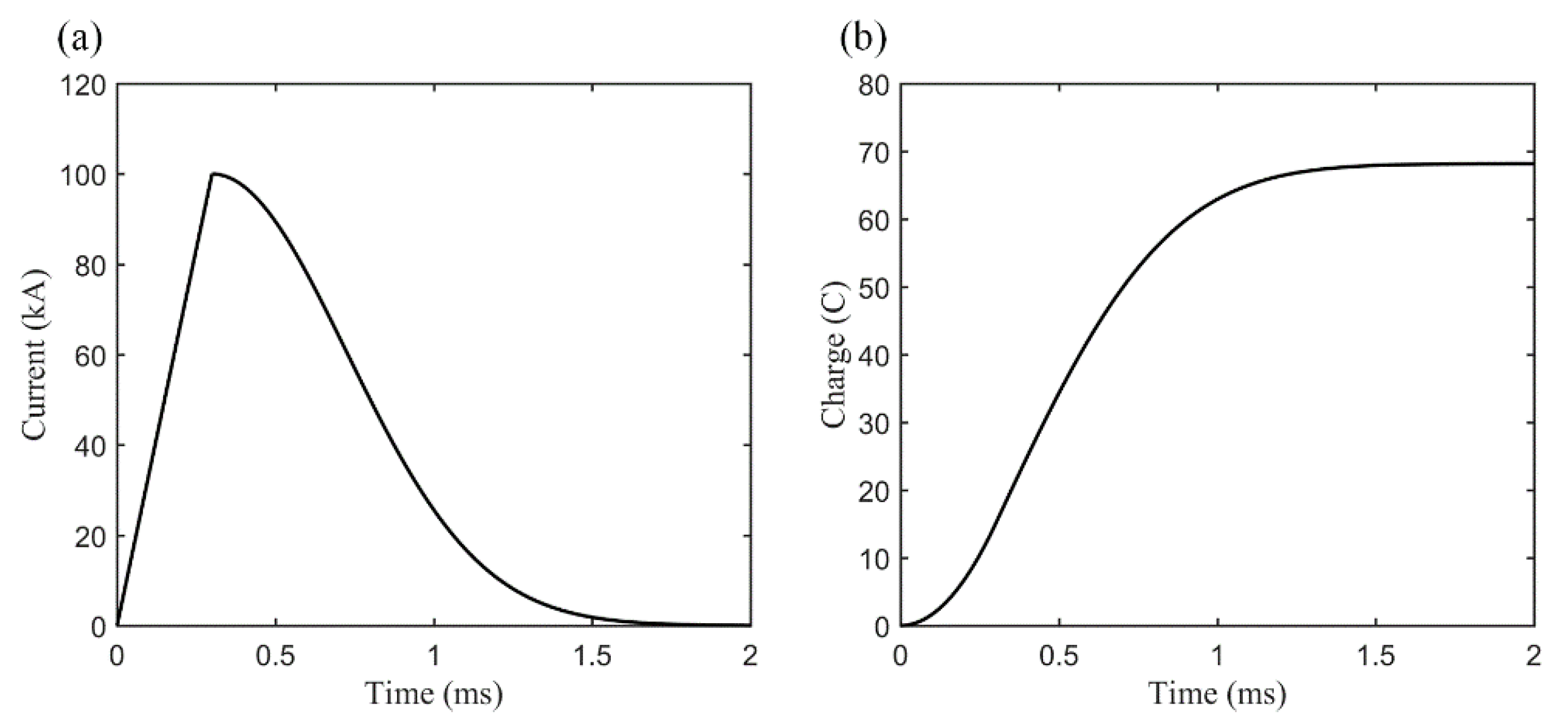
3.1. Selection of Lightning Excitation Source
3.2. Simulation Results
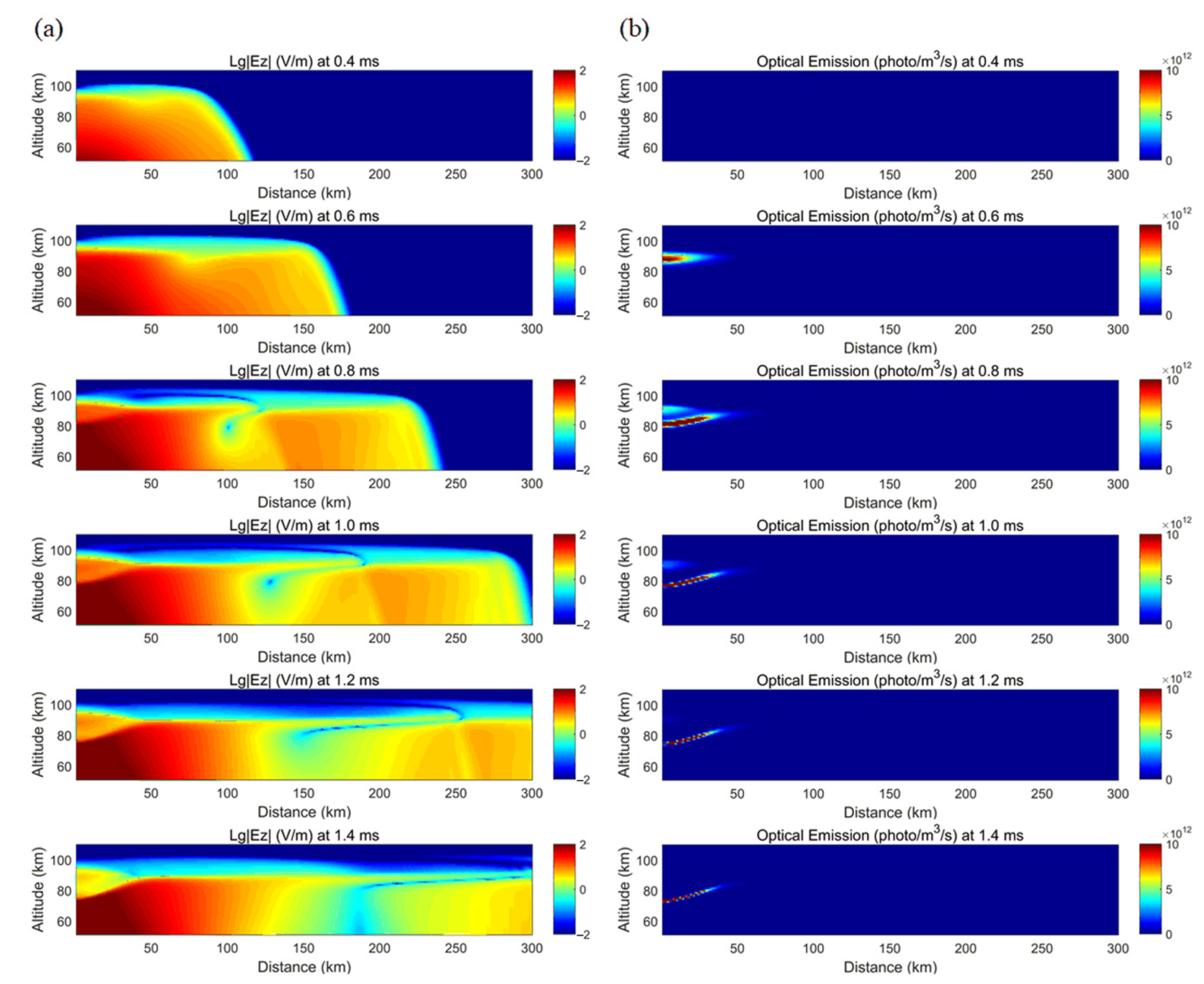
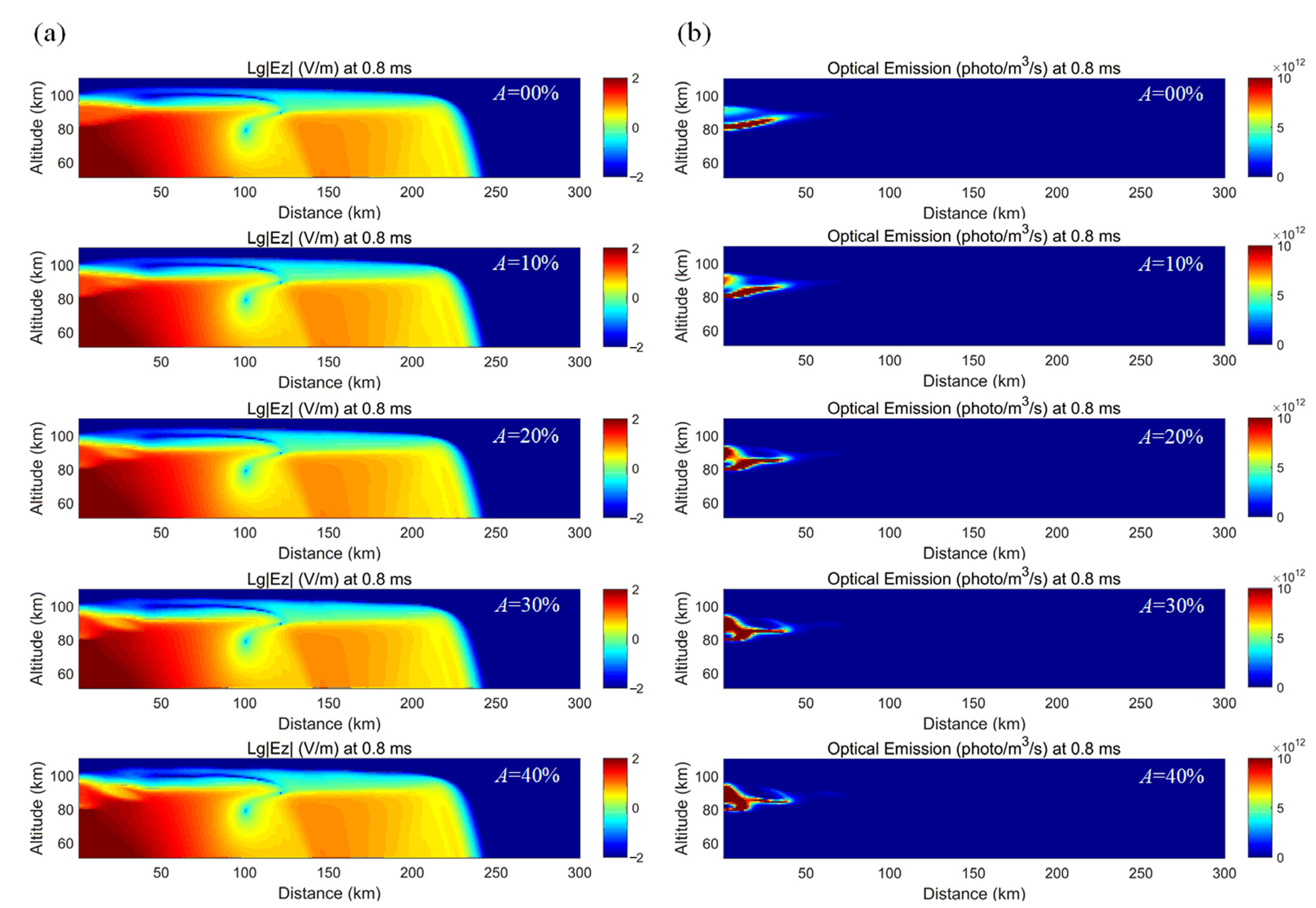
4. Effect of Gravity Wave Disturbance on Mid-to-High Altitude Halos
4.1. Selection of Lightning Excitation Source
4.2. Simulation Results
5. Conclusions and Discussion
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Qie, X.S.; Lv, D.R.; Bian, J.C.; Yang, J. Transient Luminous Events (TLEs) at high altitudes above thunderstorms and their possible effects. Adv. Earth Sci. 2009, 24, 286–296. [Google Scholar] [CrossRef]
- Zhang, J.B.; Zhang, Q.L.; Guo, X.F.; Hou, W.H.; Gao, H.Y. Simulated impacts of atmospheric gravity waves on the initiation and optical emissions of sprite halos in the mesosphere. Sci. China Earth Sci. 2019, 62, 631–642. [Google Scholar] [CrossRef]
- Franz, R.C.; Nemzek, R.J.; Winckler, J.R. Television image of a large upward electric discharge above a thunderstorm system. Science 1990, 249, 48–51. [Google Scholar] [CrossRef]
- Wu, M.L.; Xu, J.Y.; Ma, R.P. The simulation study of spherics and red sprite phenomenon produced by lightning. Acta Phys. Sin. 2006, 55, 5007–5013. [Google Scholar] [CrossRef]
- Rycroft, M.J.; Harrison, R.G.; Nicoll, K.A.; Mareev, E.A. An overview of earth’s global electric circuit and atmospheric conductivity. Space Sci. Rev. 2008, 137, 83–105. [Google Scholar] [CrossRef]
- Yang, J.; Qie, X.S.; Zhang, G.S.; Zhao, Y.; Zhang, T. Red sprites over thunderstorms in the coast of Shandong Province, China. Chin. Sci. Bull. 2008, 53, 482–488. (In Chinese) [Google Scholar] [CrossRef]
- Zhang, Q.L.; Tian, Y.; Lu, G.P. Effects of the nonlinear atmospheric electric parameters at the high altitudes on the propagation of lightning return stroke electromagnetic field. Acta Meteorol. Sin. 2014, 72, 805–814. [Google Scholar] [CrossRef]
- Pasko, V.P.; Inan, U.S.; Bell, T.F. Sprites as evidence of vertical gravity wave structures above mesoscale thunderstorms. Geophys. Res. Lett. 1997, 24, 1735–1738. [Google Scholar] [CrossRef]
- Yang, J.; Qie, X.S.; Feng, G.L. Characteristics of one sprite-producing summer thunderstorm. Atmos. Res. 2013, 127, 90–115. [Google Scholar] [CrossRef]
- Wen, Y.; Zhang, Q.L.; Xu, J.Y.; Li, Q.Z.; Gao, H.Y. Propagation characteristics of mesospheric concentric gravity waves excited by a thunderstorm. Chin. J. Geophys. 2019, 62, 1218–1229. [Google Scholar] [CrossRef]
- Taranenko, Y.N.; Inan, U.S.; Bell, T.F. Interaction with the lower ionosphere of electromagnetic pulses from lightning: Heating, attachment, and ionization. Geophys. Res. Lett. 1993, 20, 1539–1542. [Google Scholar] [CrossRef]
- Ren, H.; Tian, Y.; Lu, G.P.; Zhang, Y.F.; Fan, Y.F.; Jiang, R.B.; Liu, M.Y.; Li, D.S.; Qie, X.S. Examining the influence of current waveform on the lightning electromagnetic field at the altitude of halo formation. J. Atmos. Terr. Phys. 2019, 189, 114–122. [Google Scholar] [CrossRef]
- Pérez-Invernón, F.J.; Luque, A.; Gordillo-Vázquez, F.J.; Sato, M.; Ushio, T.; Adachi, T.; Chen, A.B. Spectroscopic diagnostic of halos and elves detected from space-based photometers. J. Geophys. Res. Atmos. 2019, 27, 12917–12941. [Google Scholar] [CrossRef]
- Rowland, H.L. Theories and simulations of elves, sprites and blue jets. J. Atmos. Terr. Phys. 1998, 60, 831–844. [Google Scholar] [CrossRef]
- Heavner, M.; Sentman, D.D.; Moudry, D.R.; Wescott, E.; Siefring, C.L.; Morrill, J.; Bucsela, E. Sprites, Blue Jets, and Elves: Optical evidence of energy transport across the stratopause. Am. Geophys. Union 2013. [Google Scholar] [CrossRef]
- Wen, Y.; Zhang, Q.L.; Gao, H.Y.; Xu, J.Y.; Li, Q.Z. A Case Study of the stratospheric and mesospheric concentric gravity waves excited by thunderstorm in northern China. Atmosphere 2018, 9, 489. [Google Scholar] [CrossRef]
- Vadas, S.; Yue, J.; Nakamura, T. Mesospheric concentric gravity waves generated by multiple convective storms over the North American Great Plain. J. Geophys. Res. Atmos. 2012, 117, D7. [Google Scholar] [CrossRef]
- Vargas, F.; Swenson, G.; Liu, A.; Pautet, D. Evidence of the excitation of a ring-like gravity wave in the mesosphere over the Andes Lidar Observatory. J. Geophys. Res. Atmos. 2016, 121, 8896–8912. [Google Scholar] [CrossRef]
- Xu, J.Y.; Li, Q.Z.; Yue, J.; Hoffmann, L.; Straka, W.C.; Wang, C.M.; Liu, M.H.; Yuan, W.; Han, S.; Miller, S.D.; et al. Concentric gravity waves over northern China observed by an airglow imager network and satellites. J. Geophys. Res. Atmos. 2015, 120, 11058–11078. [Google Scholar] [CrossRef]
- Lai, C.; Li, W.; Xu, J.Y.; Liu, X.; Yuan, W.; Yue, J.; Li, Q.Z. Extraction of quasi-monochromatic gravity waves from an airglow imager network. Atmosphere 2020, 11, 615. [Google Scholar] [CrossRef]
- Siefring, C.L.; Morrill, J.S.; Sentman, D.D.; Heavner, M.J. Simultaneous near-infrared and visible observations of sprites and acoustic-gravity waves during the EXL98 campaign. J. Geophys. Res. Space Phys. 2010, 115, A00E57. [Google Scholar] [CrossRef]
- Gong, J.; Yue, J.; Wu, D.L. Global survey of concentric gravity waves in AIRS images and ECMWF analysis. J. Geophys. Res. Atmos. 2015, 120, 2210–2228. [Google Scholar] [CrossRef]
- Yue, J.; Miller, S.D.; Hoffmann, L.; Straka, W.C. Stratospheric and mesospheric concentric gravity waves over tropical cyclone Mahasen: Joint AIRS and VIIRS satellite observations. J. Atmos. Terr. Phys. 2014, 119, 83–90. [Google Scholar] [CrossRef]
- Gong, S.H.; Yang, G.T.; Xu, J.Y.; Liu, X.; Li, Q.Z. Gravity wave propagation from the stratosphere into the mesosphere studied with Lidar, Meteor Radar, and TIMED/SABER. Atmosphere 2019, 10, 81. [Google Scholar] [CrossRef]
- Yue, J.; Lyons, W.A. Structured elves: Modulation by convectively generated gravity waves. Geophys. Res. Lett. 2015, 42, 1004–1011. [Google Scholar] [CrossRef]
- Marshall, R.A.; Yue, J.; Lyons, W.A. Numerical simulation of an elve modulated by a gravity wave. Geophys. Res. Lett. 2015, 42, 6120–6127. [Google Scholar] [CrossRef]
- Yee, K.S. Numerical solution of initial boundary value problems involving maxwell’s equations in isotropic media. IEEE Trans. Antennas Propag. 1966, 14, 302–307. [Google Scholar] [CrossRef]
- Hu, W.Y. A Numerical Model of Lightning-Generated EM Waves and Remote Sensing Applications; Duke University: Durham, NC, USA, 2005. [Google Scholar]
- Pasko, V.P. Dynamic Coupling of Quasi-Electrostatic Thundercloud Fields to the Mesosphere and Lower Ionosphere: Sprites and Jets. Ph.D. Thesis, Stanford University, Stanford, CA, USA, 1996. [Google Scholar]
- Dejnakarintra, M.; Park, C.G. Lightning-induced electric fields in the ionosphere. J. Geophys. Res. Atmos. 1974, 79, 1903–1910. [Google Scholar] [CrossRef]
- Hegerberg, R.; Reid, I.D. Electron drift velocities in air. Aust. J. Phys. 1980, 33, 227–238. Available online: https://www.publish.csiro.au/PH/pdf/PH800227a (accessed on 25 December 1979). [CrossRef]
- Han, F.; Cummer, S.A. Midlatitude nighttime D region ionosphere variability on hourly to monthly time scales. J. Geophys. Res. Atmos. 2010, 115, A09323. [Google Scholar] [CrossRef]
- Papadopoulos, K.; Milikh, G.; Gurevich, A.; Drobot, A.; Shanny, R. Ionization rates for atmospheric and ionospheric breakdown. J. Geophys. Res. Space Phys. 1993, 98, 17593–17596. [Google Scholar] [CrossRef]
- Taranenko, Y.N.; Inan, U.S.; Bell, T.F. The interaction with the lower ionosphere of electromagnetic pulses from lightning: Excitation of optical emissions. Geophys. Res. Lett. 1993, 20, 2675–2678. [Google Scholar] [CrossRef]
- Sipler, D.P.; Biondi, M.A. Measurements of O(1D) quenching rates in the F region. J. Geophys. Res. Space Phys. 1972, 77, 6202–6212. [Google Scholar] [CrossRef]
- Qin, J.Q.; Pasko, V.P.; McHarg, M.G.; Stenbaek-Nielsen, H.C. Plasma irregularities in the D-region ionosphere in association with sprite streamer initiation. Nat. Commun. 2014, 5, 536–538. [Google Scholar] [CrossRef]
- Rowland, H.L.; Fernsler, R.F.; Bernhardt, P.A. Breakdown of the neutral atmosphere in the D region due to lightning driven electromagnetic pulses. J. Geophys. Res. Space Phys. 1996, 101, 7935–7946. [Google Scholar] [CrossRef]
- Liu, N.; Boggs, L.D.; Cummer, S.A. Observation-constrained modeling of the ionospheric impact of negative sprites. Geophys. Res. Lett. 2016, 43, 2365–2373. [Google Scholar] [CrossRef]
- Barrington-Leigh, C.P.; Inan, U.S.; Stanley, M. Identification of sprites and elves with intensified video and broadband array photometry. J. Geophys. Res. Space Phys. 2001, 106, 1741–1750. [Google Scholar] [CrossRef]


Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, C.; Wen, Y.; Zhang, J.; Zhang, Q.; Qiu, J. The Modulation Effect on the ELVEs and Sprite Halos by Concentric Gravity Waves Based on the Electromagnetic Pulse Coupled Model. Atmosphere 2021, 12, 617. https://doi.org/10.3390/atmos12050617
Wang C, Wen Y, Zhang J, Zhang Q, Qiu J. The Modulation Effect on the ELVEs and Sprite Halos by Concentric Gravity Waves Based on the Electromagnetic Pulse Coupled Model. Atmosphere. 2021; 12(5):617. https://doi.org/10.3390/atmos12050617
Chicago/Turabian StyleWang, Chao, Ying Wen, Jinbo Zhang, Qilin Zhang, and Juwei Qiu. 2021. "The Modulation Effect on the ELVEs and Sprite Halos by Concentric Gravity Waves Based on the Electromagnetic Pulse Coupled Model" Atmosphere 12, no. 5: 617. https://doi.org/10.3390/atmos12050617
APA StyleWang, C., Wen, Y., Zhang, J., Zhang, Q., & Qiu, J. (2021). The Modulation Effect on the ELVEs and Sprite Halos by Concentric Gravity Waves Based on the Electromagnetic Pulse Coupled Model. Atmosphere, 12(5), 617. https://doi.org/10.3390/atmos12050617






