Multiscale Spatiotemporal Analysis of Extreme Events in the Gomati River Basin, India
Abstract
1. Introduction
2. Study Area and Data
2.1. Gomati Basin
2.2. Data
3. Methods
3.1. Sen’s Slope Estimator Test
3.2. Rescaled Range Analysis
- (i).
- To define a mean sequence:
- (ii).
- To create a cumulative deviation series:
- (iii).
- To create a range series:
- (iv).
- To create a standard deviation series:
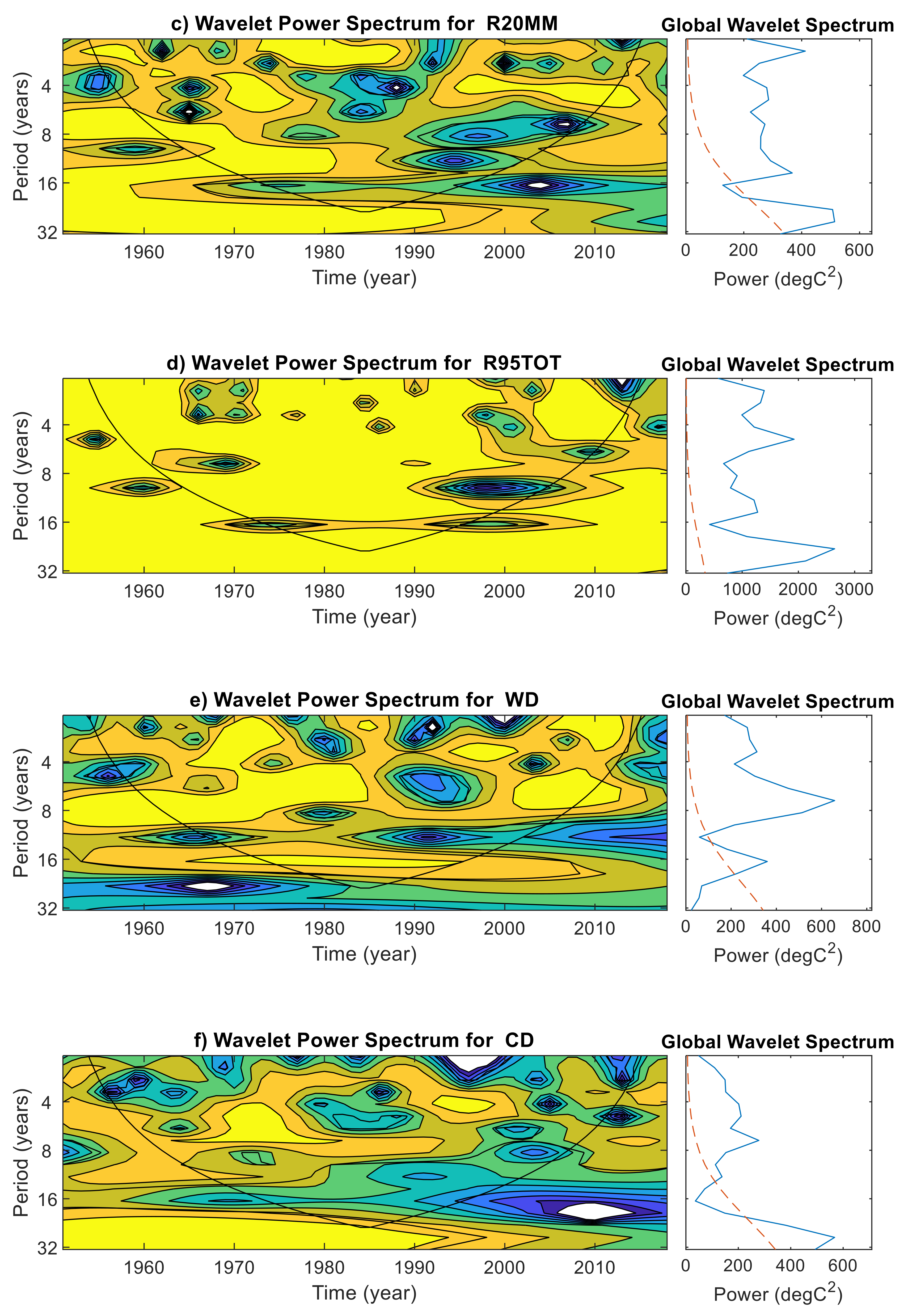
3.3. Wavelet Transform
3.4. Bivariate Copula Functions
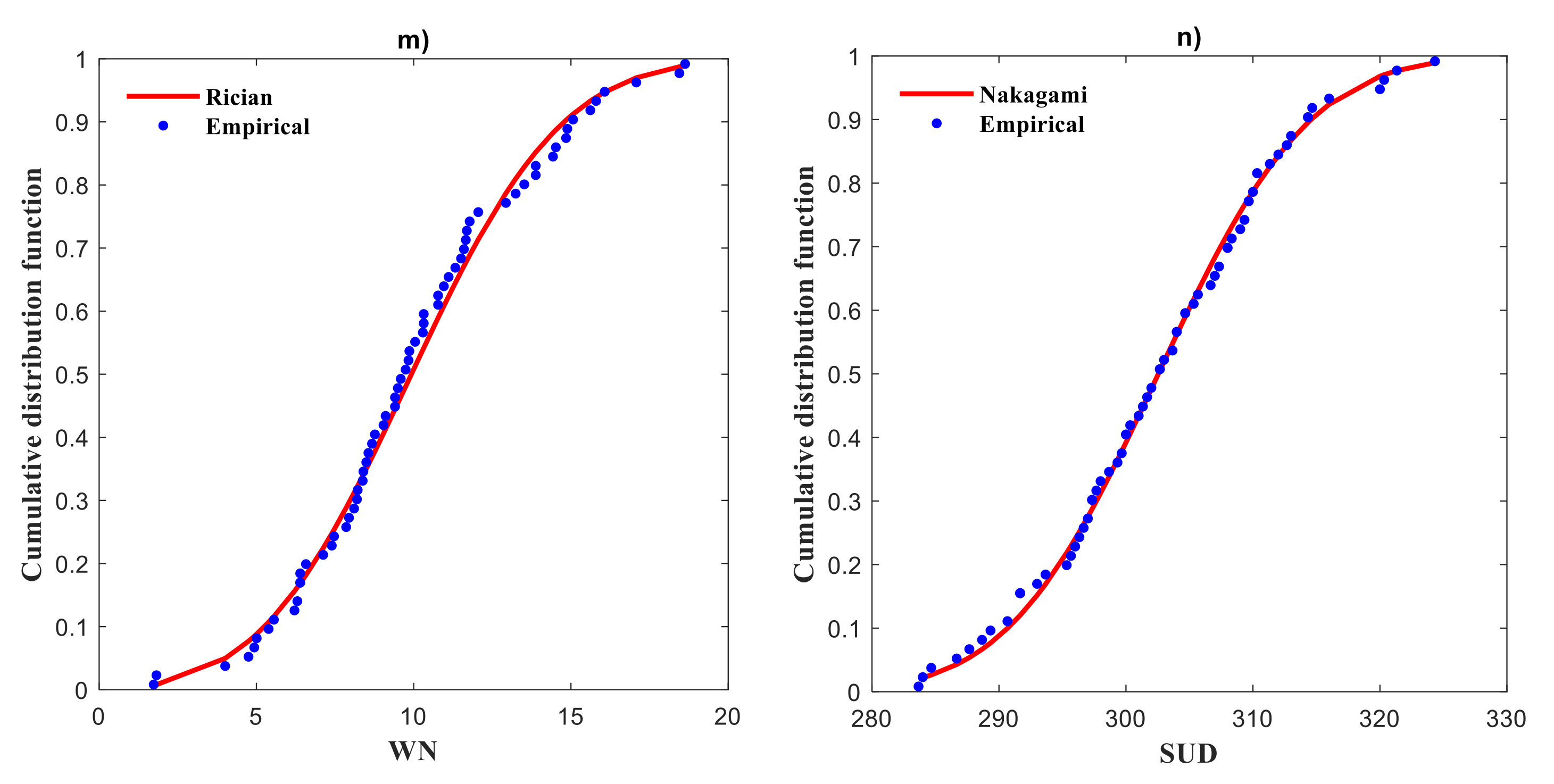
- Fit a marginal distribution for the climate indices (for example, RTWD and R95TOT) and develop their cumulative distribution functions (CDF). We used 15 marginal distributions (1) Weibull distributions, (2) gamma, (3) extreme value, (4) exponential, (5) Birnbaum–Saunders, (6) generalized extreme value, (7) inverse Gaussian, (8) lognormal, (9) Nakagami, (10) logistic, (11) log-logistic, (12) t location-scale, (13) Rayleigh, (14) normal, and (15) Rician,
- The Bayesian Information Criterion (BIC) is applied to identify each climate indices’ best marginal distribution. The marginal distributions parameters are calculated using a maximum likelihood algorithm, and the Χ2 goodness-of-fit is used for statistical significance.
- The correlation was calculated between different climate indices to evaluate the mutual dependence between the two climate indices using Kendall’s Tau Correlation coefficient.
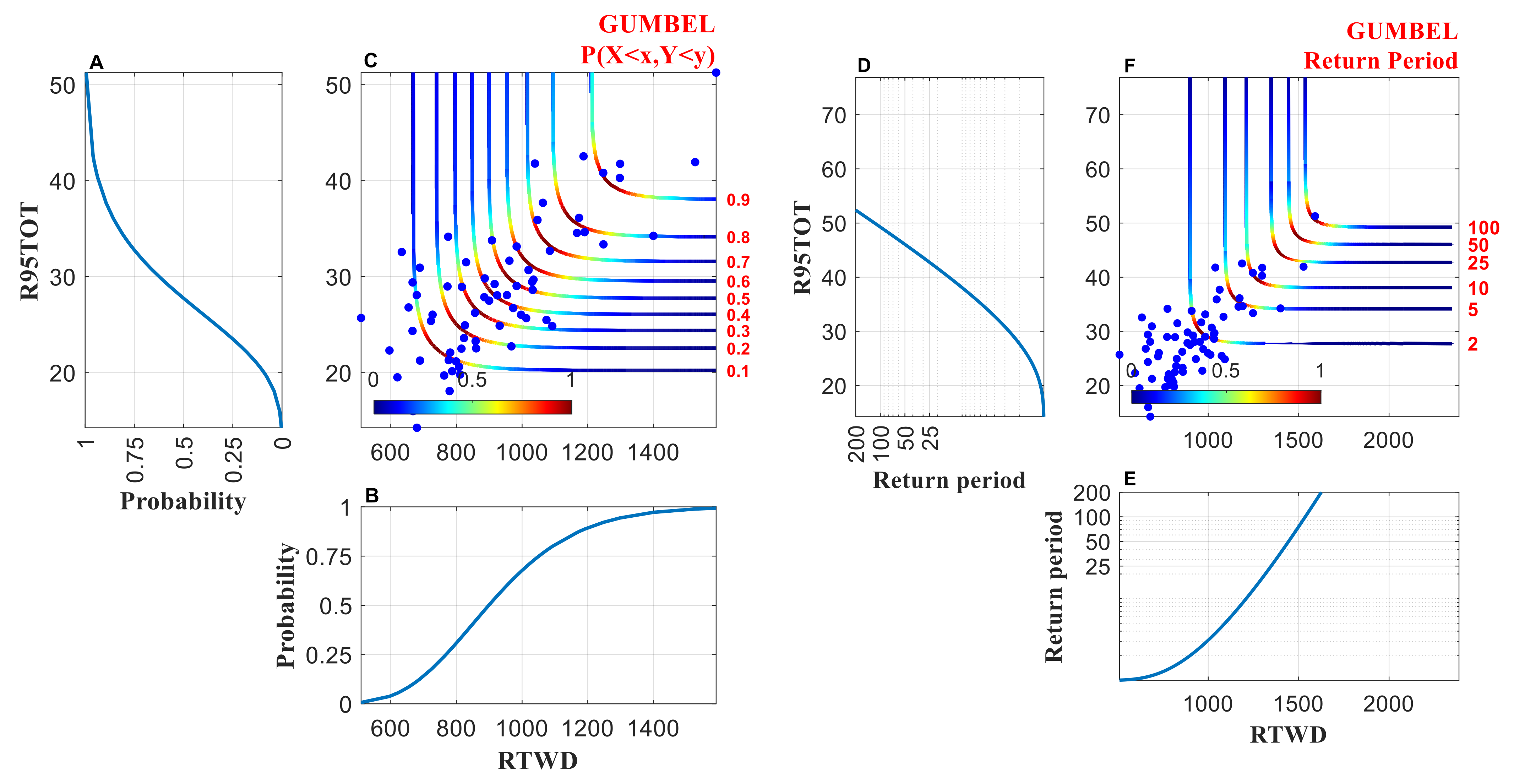
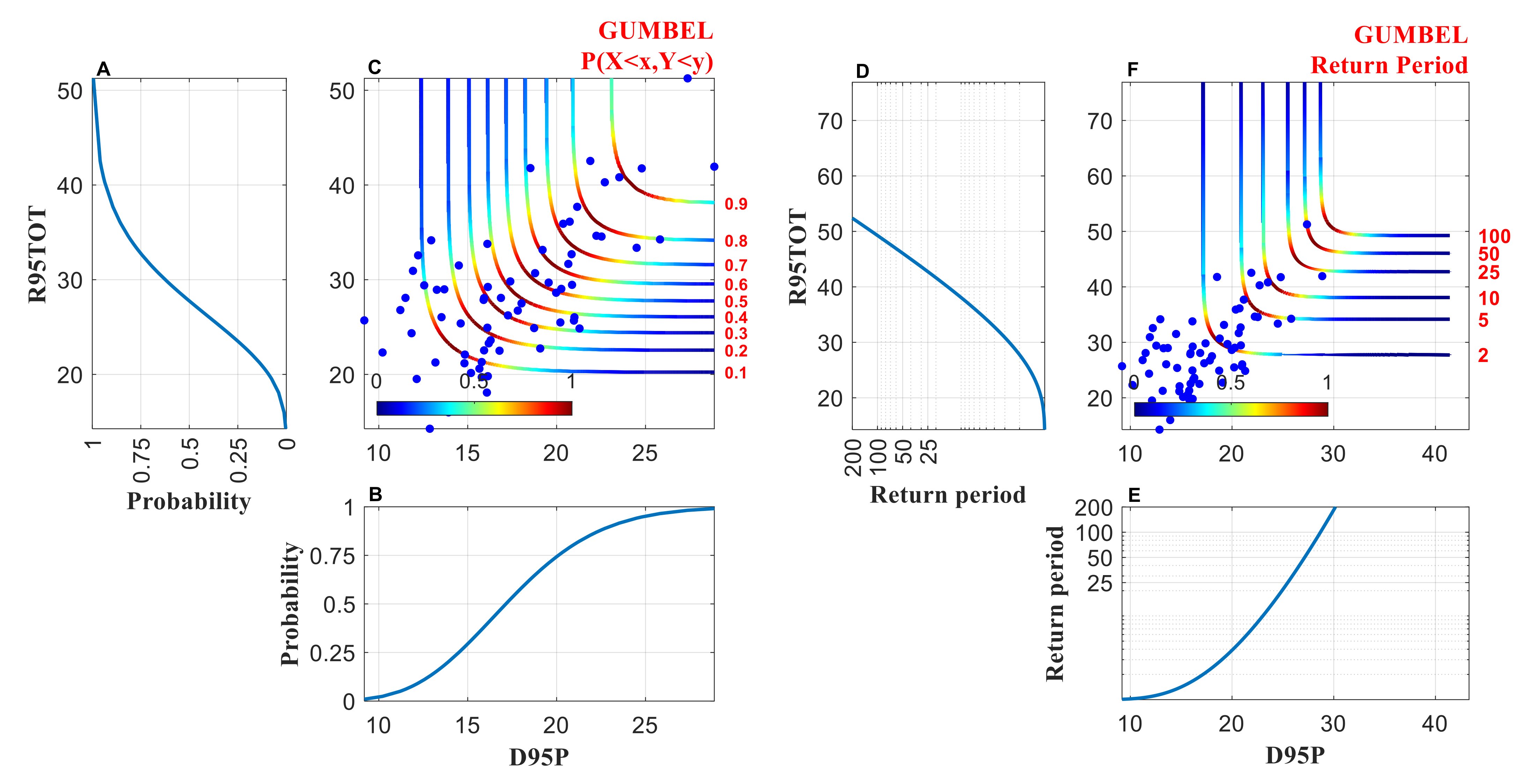
- Obtain the joint CDF of two variables by estimating the copula parameters (see Table 2)
- Finally, a summary report is generated containing copulas from best fitted to the lowest one. The best copula function was selected based on the minimum principle of Akaike Information criterion (AIC) and RMSE following [48].
4. Results and Discussion
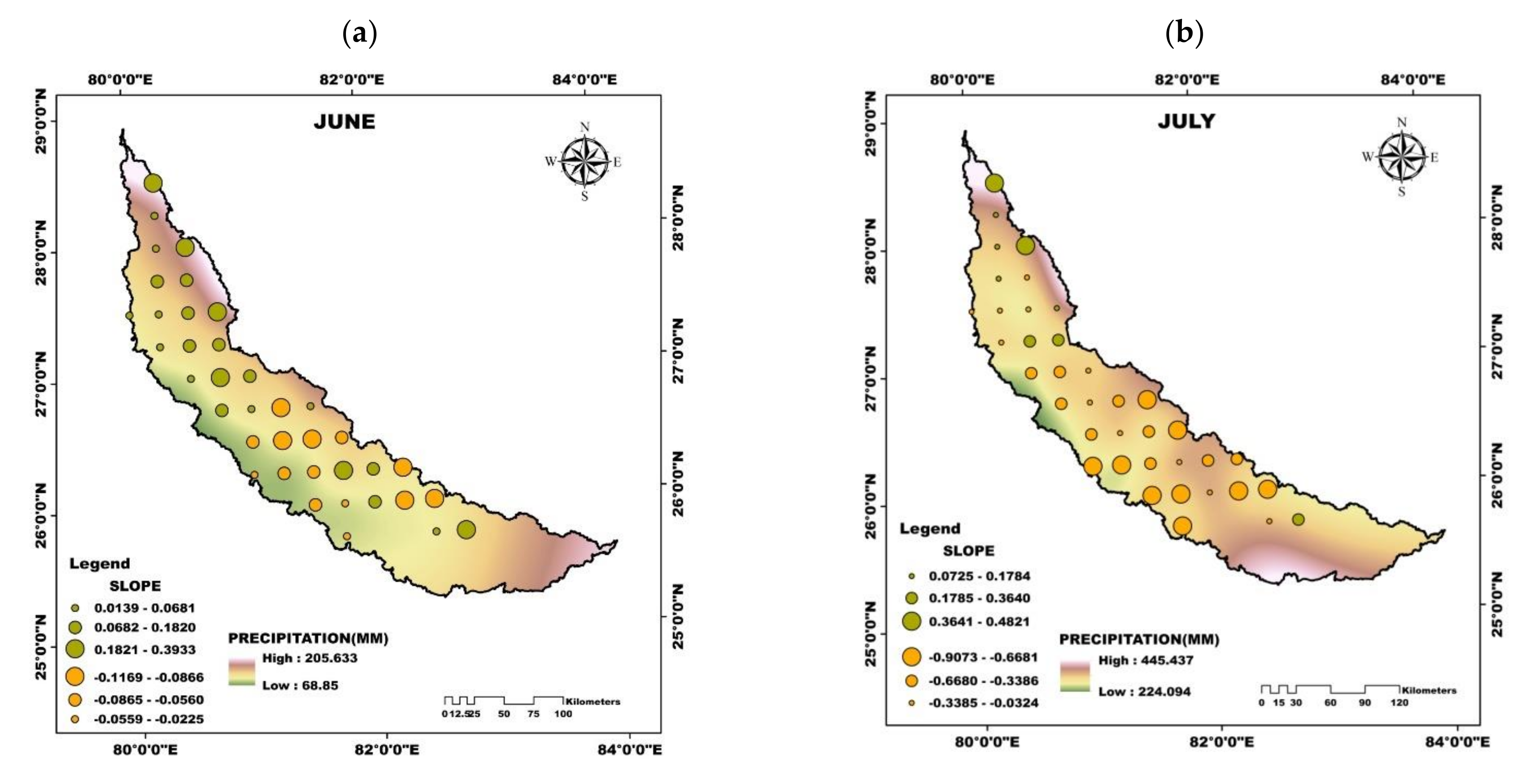
4.1. Spatio-Temporal Variability of Precipitation
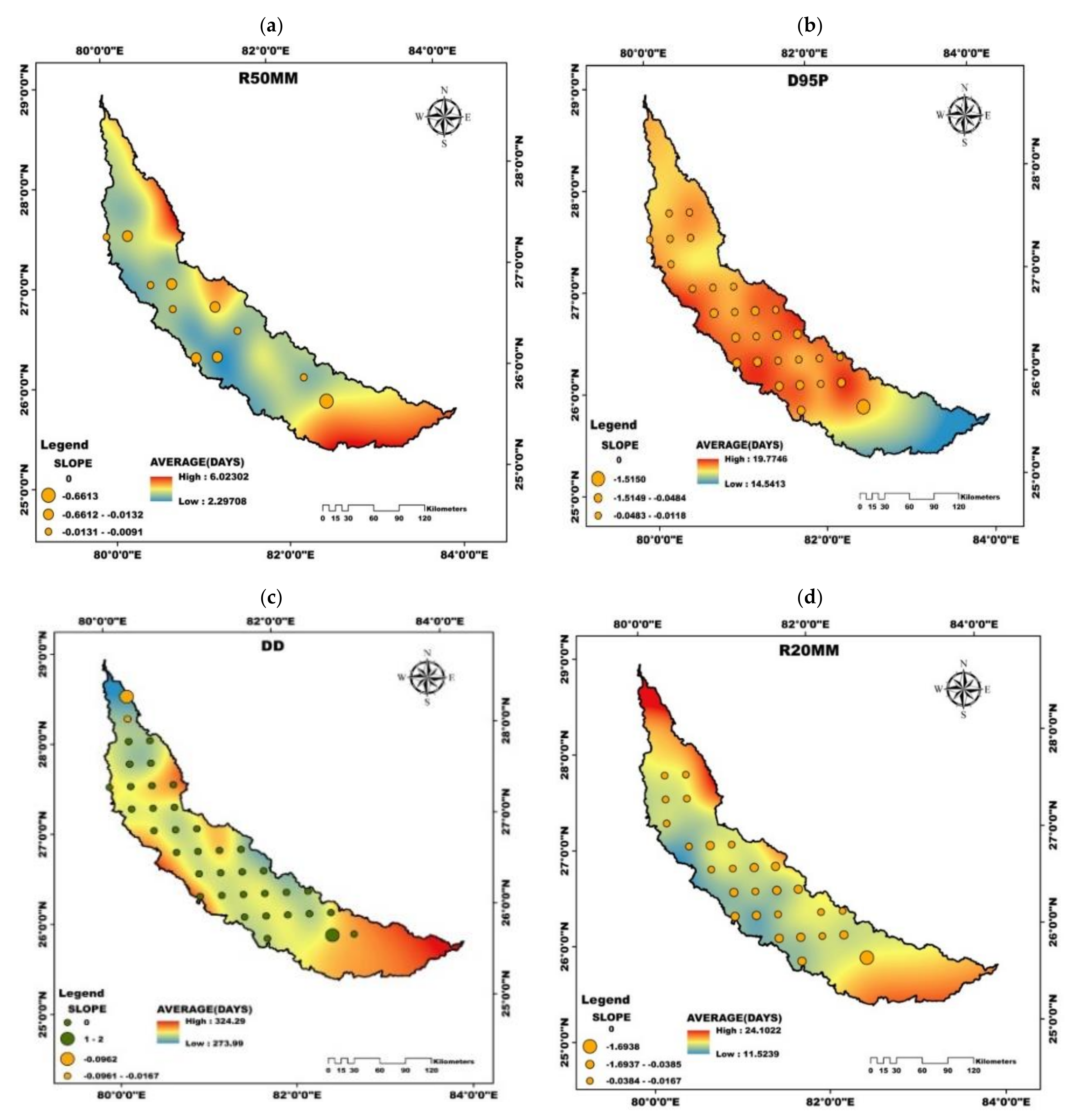
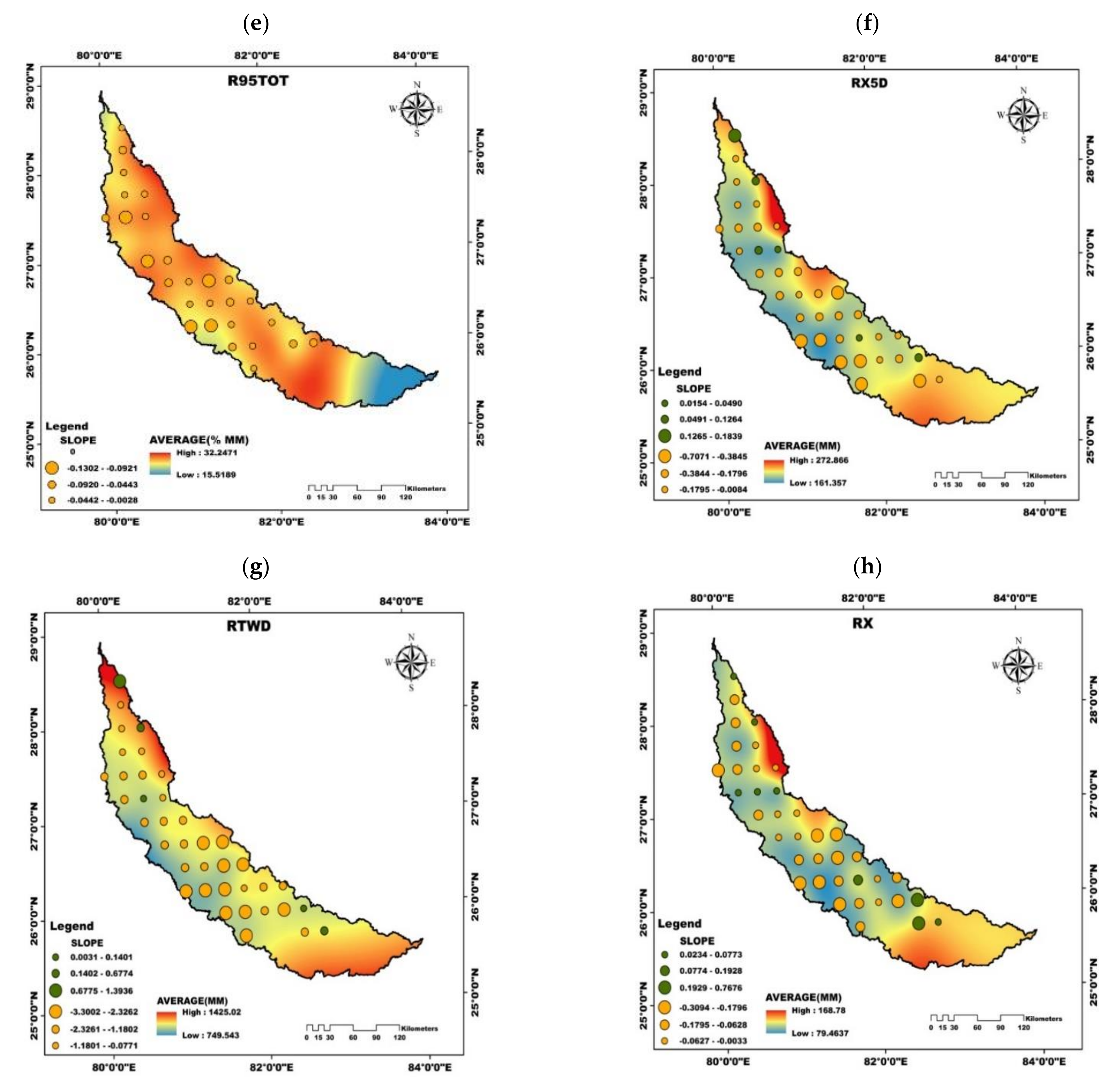
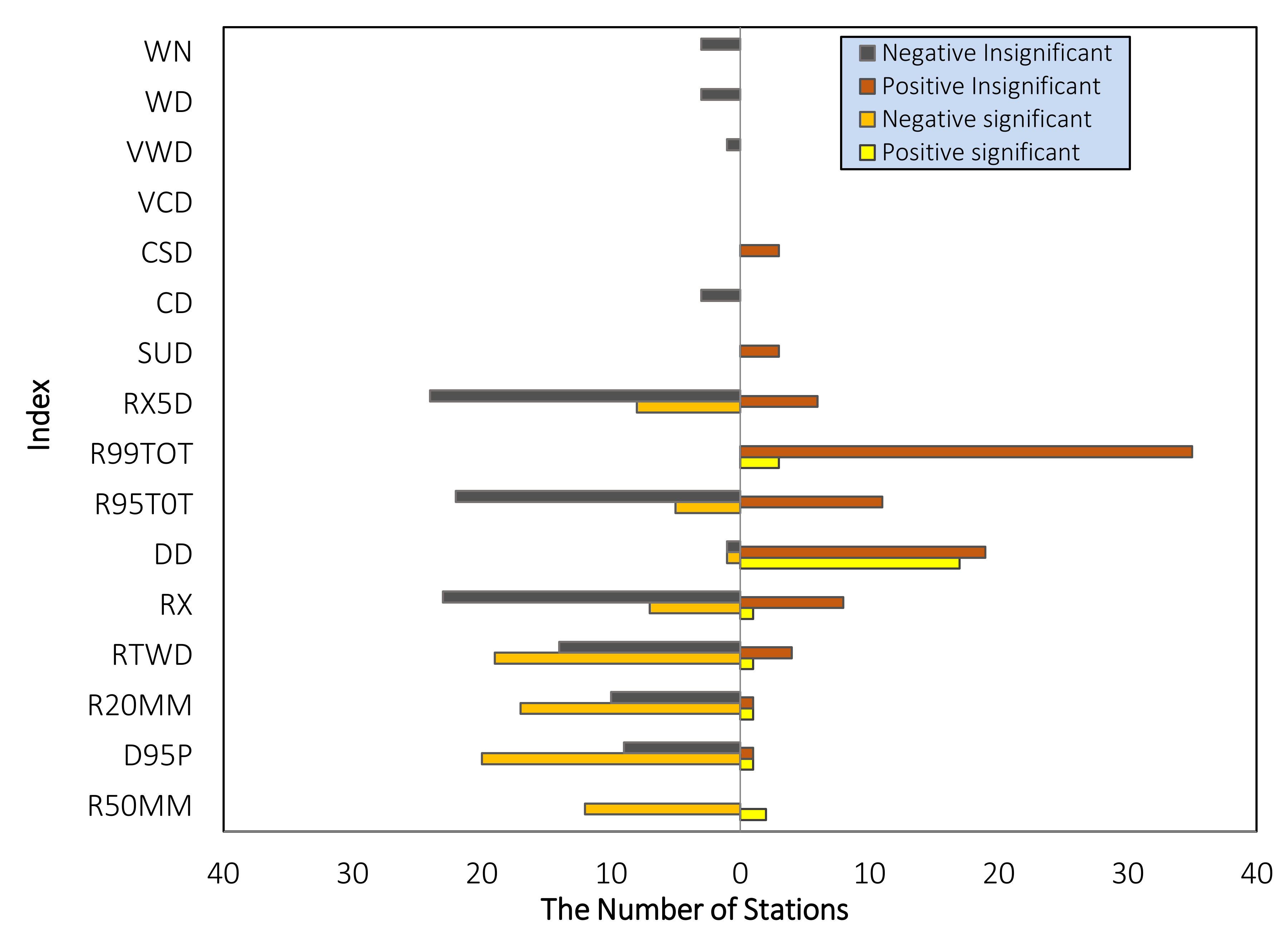
4.2. Spatio-Temporal Variability of Extreme Climate Indices
4.3. Hurst Analysis of Extreme Climate Indices
4.4. Periodic Oscillation Analysis
4.5. Bivariate Joint Probability and Return Period Analysis
5. Conclusions
- (a)
- Different extreme precipitation indices (D95P, R95TOT) show significant decreasing trends whereas the extreme temperature indices show an increasing trend indicating that the basin is experiencing a warm and dry climate compared to the past. Further, the positive persistence present in these variables show that the trend would continue in the future resulting in warmer and drier climate. The results presented in the study is in congruence with the other studies wherein the climate has become warmer
- (b)
- The presence of the significant periodicities (4–8 years and decadal timescale) in the precipitation extreme indicate a possibility of connection with some of the well-known global atmospheric patterns such as ENSO and PDO. However, interestingly, the extreme temperature indices did not exhibit significant periodicities.
- (c)
- Further, the analysis from the CWT of the extreme precipitation indices indicate that there is a significant non-stationarity indicating that the changes in the precipitation patterns after the 1980s.
- (d)
- Further copula modelling is applied to analyze compound extremes. The multivariate framework better represented the risk due to the consideration of mutual dependence. Interestingly, the copula modelling shows that the joint probabilities for higher annual precipitation and precipitation receiving from very wet days are smaller than the marginal exceedance probabilities. Intuitively, consideration of mutual dependence improves the compound risk of climate extremes.
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Stocker, T.F.; Qin, D.; Plattner, G.-K.; Alexander, L.V.; Allen, S.K.; Bindoff, N.L.; Bréon, F.-M.; Church, J.A.; Cubasch, U.; Emori, S.; et al. Technical summary. In Climate Change 2013: The Physical Science Basis. Contribution of Working Group I to the Fifth Assessment Report of the Intergovernmental Panel on Climate Change; Cambridge University Press: Cambridge, UK, 2013; pp. 33–115. [Google Scholar]
- Mutiibwa, D.; Vavrus, S.J.; McAfee, S.A.; Albright, T.P. Recent Spatiotemporal Patterns in Temperature Extremes across Conterminous United States. J. Geophys. Res. Atmos. 2015, 120, 7378–7392. [Google Scholar] [CrossRef]
- Shrestha, U.B.; Shrestha, B.B. Climate Change Amplifies Plant Invasion Hotspots in Nepal. Divers. Distrib. 2019, 25, 1599–1612. [Google Scholar] [CrossRef]
- Van Wilgen, B.W.; Fill, J.M.; Baard, J.; Cheney, C.; Forsyth, A.T.; Kraaij, T. Historical Costs and Projected Future Scenarios for the Management of Invasive Alien Plants in Protected Areas in the Cape Floristic Region. Biol. Conserv. 2016, 200, 168–177. [Google Scholar] [CrossRef]
- Zhou, Y.; Ren, G. Change in Extreme Temperature Event Frequency over Mainland China, 1961–2008. Clim. Res. 2011, 50, 125–139. [Google Scholar] [CrossRef]
- Shukla, R.; Chakraborty, A.; Sachdeva, K.; Joshi, P.K. Agriculture in the Western Himalayas—An Asset Turning into a Liability. Dev. Pract. 2018, 28, 318–324. [Google Scholar] [CrossRef]
- El-Zein, A.; Tonmoy, F.N. Assessment of Vulnerability to Climate Change Using a Multi-Criteria Outranking Approach with Application to Heat Stress in Sydney. Ecol. Indic. 2015, 48, 207–217. [Google Scholar] [CrossRef]
- Bahinipati, C.S.; Venkatachalam, L. Role of Climate Risks and Socio-Economic Factors in Influencing the Impact of Climatic Extremes: A Normalisation Study in the Context of Odisha, India. Reg. Environ. Chang. 2016, 16, 177–188. [Google Scholar] [CrossRef]
- Shukla, R.; Agarwal, A.; Sachdeva, K.; Kurths, J.; Joshi, P.K. Climate Change Perception: An Analysis of Climate Change and Risk Perceptions among Farmer Types of Indian Western Himalayas. Clim. Chang. 2019, 152, 103–119. [Google Scholar] [CrossRef]
- Zhang, J.; Terrones, M.; Park, C.R.; Mukherjee, R.; Monthioux, M.; Koratkar, N.; Kim, Y.S.; Hurt, R.; Frackowiak, E.; Enoki, T.; et al. Carbon Science in 2016: Status, Challenges and Perspectives. Carbon 2016, 98, 708–732. [Google Scholar] [CrossRef]
- Stocker, T.F.; Clarke, G.K.C.; Le Treut, H.; Lindzen, R.S.; Meleshko, V.P.; Mugara, R.K.; Palmer, T.N.; Pierrehumbert, R.T.; Sellers, P.J.; Trenberth, K.E.; et al. Physical Climate Processes and Feedbacks. In IPCC, 2001: Climate Change 2001: The Scientific Basis. Contribution of Working Group I to the Third Assessment Report of the Intergovernmental Panel on Climate Change; Houghton, J.T., Ding, Y., Griggs, D.J., Noguer, M., van der Linden, P.J., Dai, X., Maske, K., Eds.; Cambridge University Press: Cambridge, UK, 2001; pp. 417–470. ISBN 978-0-521-01495-3. [Google Scholar]
- Easterling, D.R. Climate Extremes: Observations, Modeling, and Impacts. Science 2000, 289, 2068–2074. [Google Scholar] [CrossRef]
- Farajzadeh, H.; Matzarakis, A. Quantification of Climate for Tourism in the Northwest of Iran: Quantification of climate for tourism in the northwest of iran. Meteorol. Appl. 2009, 16, 545–555. [Google Scholar] [CrossRef]
- Keggenhoff, I.; Elizbarashvili, M.; Amiri-Farahani, A.; King, L. Trends in Daily Temperature and Precipitation Extremes over Georgia, 1971–2010. Weather Clim. Extrem. 2014, 4, 75–85. [Google Scholar] [CrossRef]
- Ruml, M.; Gregorić, E.; Vujadinović, M.; Radovanović, S.; Matović, G.; Vuković, A.; Počuča, V.; Stojičić, D. Observed Changes of Temperature Extremes in Serbia over the Period 1961–2010. Atmos. Res. 2017, 183, 26–41. [Google Scholar] [CrossRef]
- Guo, E.; Zhang, J.; Wang, Y.; Quan, L.; Zhang, R.; Zhang, F.; Zhou, M. Spatiotemporal Variations of Extreme Climate Events in Northeast China during 1960–2014. Ecol. Indic. 2019, 96, 669–683. [Google Scholar] [CrossRef]
- Zhang, X.; Alexander, L.; Hegerl, G.C.; Jones, P.; Tank, A.K.; Peterson, T.C.; Trewin, B.; Zwiers, F.W. Indices for Monitoring Changes in Extremes Based on Daily Temperature and Precipitation Data: Indices for Monitoring Changes in Extremes. WIREs Clim. Chang. 2011, 2, 851–870. [Google Scholar] [CrossRef]
- García-Cueto, O.R.; Cavazos, M.T.; de Grau, P.; Santillán-Soto, N. Analysis and Modeling of Extreme Temperatures in Several Cities in Northwestern Mexico under Climate Change Conditions. Theor. Appl. Climatol. 2014, 116, 211–225. [Google Scholar] [CrossRef]
- Wang, H.; Zhang, G.; Li, N.; Zhang, B.; Yang, H. Soil Erodibility as Impacted by Vegetation Restoration Strategies on the Loess Plateau of China: Effect of Vegetation Restoration on Soil Erodibility. Earth Surf. Process. Landf. 2019, 44, 796–807. [Google Scholar] [CrossRef]
- Sharma, P.J.; Loliyana, V.D.; Resmi, S.R.; Timbadiya, P.V.; Patel, P.L. Spatiotemporal Trends in Extreme Rainfall and Temperature Indices over Upper Tapi Basin, India. Theor. Appl. Climatol. 2018, 134, 1329–1354. [Google Scholar] [CrossRef]
- Abeysingha, N.; Singh, M.; Sehgal, V.; Khanna, M.; Pathak, H. Analysis of Rainfall and Temperature Trends in Gomti River Basin. J. Agric. Phys. 2014, 14, 56–66. [Google Scholar]
- Dutta, V.; Kumar, R.; Sharma, U. Assessment of Human-Induced Impacts on Hydrological Regime of Gomti River Basin, India. Manag. Environ. Qual. Int. J. 2015, 26, 631–649. [Google Scholar] [CrossRef]
- Pai, D.; Sridhar, L.; Rajeevan, M.; Sreejith, O.; Satbhai, N.; Mukhopadhyay, B. Development of a New High Spatial Resolution (0.25 × 0.25) Long Period (1901–2010) Daily Gridded Rainfall Data Set over India and Its Comparison with Existing Data Sets over the Region. Mausam 2014, 65, 1–18. [Google Scholar]
- Srivastava, A.K.; Rajeevan, M.; Kshirsagar, S.R. Development of a High Resolution Daily Gridded Temperature Data Set (1969–2005) for the Indian Region. Atmos. Sci. Lett. 2009, 10, 249–254. [Google Scholar] [CrossRef]
- Guntu, R.K.; Maheswaran, R.; Agarwal, A.; Singh, V.P. Accounting for Temporal Variability for Improved Precipitation Regionalization Based on Self-Organizing Map Coupled with Information Theory. J. Hydrol. 2020, 590, 125236. [Google Scholar] [CrossRef]
- Guntu, R.K.; Rathinasamy, M.; Agarwal, A.; Sivakumar, B. Spatiotemporal Variability of Indian Rainfall Using Multiscale Entropy. J. Hydrol. 2020, 587, 124916. [Google Scholar] [CrossRef]
- Pingale, S.M.; Khare, D.; Jat, M.K.; Adamowski, J. Spatial and Temporal Trends of Mean and Extreme Rainfall and Temperature for the 33 Urban Centers of the Arid and Semi-Arid State of Rajasthan, India. Atmos. Res. 2014, 138, 73–90. [Google Scholar] [CrossRef]
- Alexander, L.V.; Fowler, H.J.; Bador, M.; Behrangi, A.; Donat, M.G.; Dunn, R.; Funk, C.; Goldie, J.; Lewis, E.; Rogé, M.; et al. On the Use of Indices to Study Extreme Precipitation on Sub-Daily and Daily Timescales. Environ. Res. Lett. 2019, 14, 125008. [Google Scholar] [CrossRef]
- Sen, P.K. Robustness of Some Nonparametric Procedures in Linear Models. Ann. Math. Stat. 1968, 39, 1913–1922. [Google Scholar] [CrossRef]
- Lettenmaier, D.P.; Wood, E.F.; Wallis, J.R. Hydro-Climatological Trends in the Continental United States, 1948–1988. J. Clim. 1994, 7, 586–607. [Google Scholar] [CrossRef]
- Yue, S.; Hashino, M. Long term trends of annual and monthly precipitation in japan1. JAWRA J. Am. Water Resour. Assoc. 2003, 39, 587–596. [Google Scholar] [CrossRef]
- Partal, T.; Kahya, E. Trend Analysis in Turkish Precipitation Data. Hydrol. Process. 2006, 20, 2011–2026. [Google Scholar] [CrossRef]
- Koutsoyiannis, D. Hydrologic Persistence and the Hurst Phenomenon. In Water Encyclopedia; Lehr, J.H., Keeley, J., Eds.; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2005; p. sw434. ISBN 978-0-471-47844-7. [Google Scholar]
- Graves, T.; Gramacy, R.; Watkins, N.; Franzke, C. A Brief History of Long Memory: Hurst, Mandelbrot and the Road to ARFIMA, 1951–1980. Entropy 2017, 19, 437. [Google Scholar] [CrossRef]
- Maheswaran, R.; Khosa, R. Comparative Study of Different Wavelets for Hydrologic Forecasting. Comput. Geosci. 2012, 46, 284–295. [Google Scholar] [CrossRef]
- Agarwal, A.; Maheswaran, R.; Kurths, J.; Khosa, R. Wavelet Spectrum and Self-Organizing Maps-Based Approach for Hydrologic Regionalization—A Case Study in the Western United States. Water Resour. Manag. 2016, 30, 4399–4413. [Google Scholar] [CrossRef]
- Tamaddun, K.A.; Kalra, A.; Ahmad, S. Wavelet Analyses of Western US Streamflow with ENSO and PDO. J. Water Clim. Chang. 2017, 8, 26–39. [Google Scholar] [CrossRef]
- Nicolay, S.; Mabille, G.; Fettweis, X.; Erpicum, M. 30 and 43 Months Period Cycles Found in Air Temperature Time Series Using the Morlet Wavelet Method. Clim. Dyn. 2009, 33, 1117–1129. [Google Scholar] [CrossRef]
- Narasimha, R.; Bhattacharyya, S. A Wavelet Cross-Spectral Analysis of Solar–ENSO–Rainfall Connections in the Indian Monsoons. Appl. Comput. Harmon. Anal. 2010, 28, 285–295. [Google Scholar] [CrossRef]
- Farge, M. Wavelet Transforms and Their Applications to Turbulence. Annu. Rev. Fluid Mech. 1992, 24, 395–458. [Google Scholar] [CrossRef]
- Torrence, C.; Compo, G.P. A Practical Guide to Wavelet Analysis. Bull. Am. Meteorol. Soc. 1998, 79, 61–78. [Google Scholar] [CrossRef]
- Yeditha, P.K.; Venkatesh, K.; Maheswaran, R.; Ankit, A. Forecasting of extreme flood events using different satellite precipitation products and wavelet-based machine learning methods. Chaos Interdiscip. J. Nonlinear Sci. 2020, 30, 063115. [Google Scholar]
- Sklar, M. Fonctions de Repartition an Dimensions et Leurs Marges. Publ. Inst. Statist. Univ. Paris 1959, 8, 229–231. [Google Scholar]
- Ganguli, P.; Merz, B. Extreme Coastal Water Levels Exacerbate Fluvial Flood Hazards in Northwestern Europe. Sci. Rep. 2019, 9, 1–14. [Google Scholar] [CrossRef]
- Anandalekshmi, A.; Panicker, S.T.; Adarsh, S.; Muhammed Siddik, A.; Aloysius, S.; Mehjabin, M. Modeling the Concurrent Impact of Extreme Rainfall and Reservoir Storage on Kerala Floods 2018: A Copula Approach. Model. Earth Syst. Environ. 2019, 5, 1283–1296. [Google Scholar] [CrossRef]
- Nelsen, R.B. An Introduction to Copulas; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2007. [Google Scholar]
- Genest, C.; Rivest, L.-P. Statistical Inference Procedures for Bivariate Archimedean Copulas. J. Am. Stat. Assoc. 1993, 88, 1034–1043. [Google Scholar] [CrossRef]
- Ghosh, S.; Vittal, H.; Sharma, T.; Karmakar, S.; Kasiviswanathan, K.S.; Dhanesh, Y.; Sudheer, K.P.; Gunthe, S.S. Indian Summer Monsoon Rainfall: Implications of Contrasting Trends in the Spatial Variability of Means and Extremes. PLoS ONE 2016, 11, e0158670. [Google Scholar] [CrossRef]
- Singh, D.; Ghosh, S.; Roxy, M.K.; McDermid, S. Indian Summer Monsoon: Extreme Events, Historical Changes, and Role of Anthropogenic Forcings. WIREs Clim. Chang. 2019, 10. [Google Scholar] [CrossRef]
- Rathinasamy, M.; Agarwal, A.; Sivakumar, B.; Marwan, N.; Kurths, J. Wavelet Analysis of Precipitation Extremes over India and Teleconnections to Climate Indices. Stoch. Environ. Res. Risk Assess. 2019, 33, 2053–2069. [Google Scholar] [CrossRef]
- Sadegh, M.; Ragno, E.; AghaKouchak, A. Multivariate C Opula A Nalysis T Oolbox (MvCAT): Describing Dependence and Underlying Uncertainty Using a B Ayesian Framework. Water Resour. Res. 2017, 53, 5166–5183. [Google Scholar] [CrossRef]
- Solomon, S. IPCC (2007): Climate Change the Physical Science Basis. In Proceedings of the Agu Fall Meeting Abstracts, San Francisco, CA, USA, 10–14 December 2007; Volume 2007, p. U43D-01. [Google Scholar]
- Alexander, L.V.; Zhang, X.; Peterson, T.C.; Caesar, J.; Gleason, B.; Klein Tank, A.M.G.; Haylock, M.; Collins, D.; Trewin, B.; Rahimzadeh, F.; et al. Global Observed Changes in Daily Climate Extremes of Temperature and Precipitation. J. Geophys. Res. 2006, 111, D05109. [Google Scholar] [CrossRef]
- Arora, M.; Goel, N.K.; Singh, P. Evaluation of Temperature Trends over India/Evaluation de Tendances de Température En Inde. Hydrol. Sci. J. 2005, 50, 12. [Google Scholar] [CrossRef]
- Kothawale, D.; Munot, A.; Krishna Kumar, K. Surface Air Temperature Variability over India during 1901–2007, and Its Association with ENSO. Clim. Res. 2010, 42, 89–104. [Google Scholar] [CrossRef]
- Guntu, R.K.; Agarwal, A. Investigation of Precipitation Variability and Extremes Using Information Theory. Environ. Sci. Proc. 2021, 4, 14. [Google Scholar] [CrossRef]
- Kurths, J.; Agarwal, A.; Marwan, N.; Rathinasamy, M.; Caesar, L.; Krishnan, R.; Merz, B. Unraveling the spatial diversity of Indian precipitation teleconnections via nonlinear multi-scale approach. Nonlinear Process. Geophys. 2019, 26, 251–266. [Google Scholar] [CrossRef]
- Kumar, S.; Chanda, K.; Pasupuleti, S. Spatiotemporal Analysis of Extreme Indices Derived from Daily Precipitation and Temperature for Climate Change Detection over India. Theor. Appl. Climatol. 2020, 140, 343–357. [Google Scholar] [CrossRef]
- Trenberth, K. Changes in Precipitation with Climate Change. Clim. Res. 2011, 47, 123–138. [Google Scholar] [CrossRef]



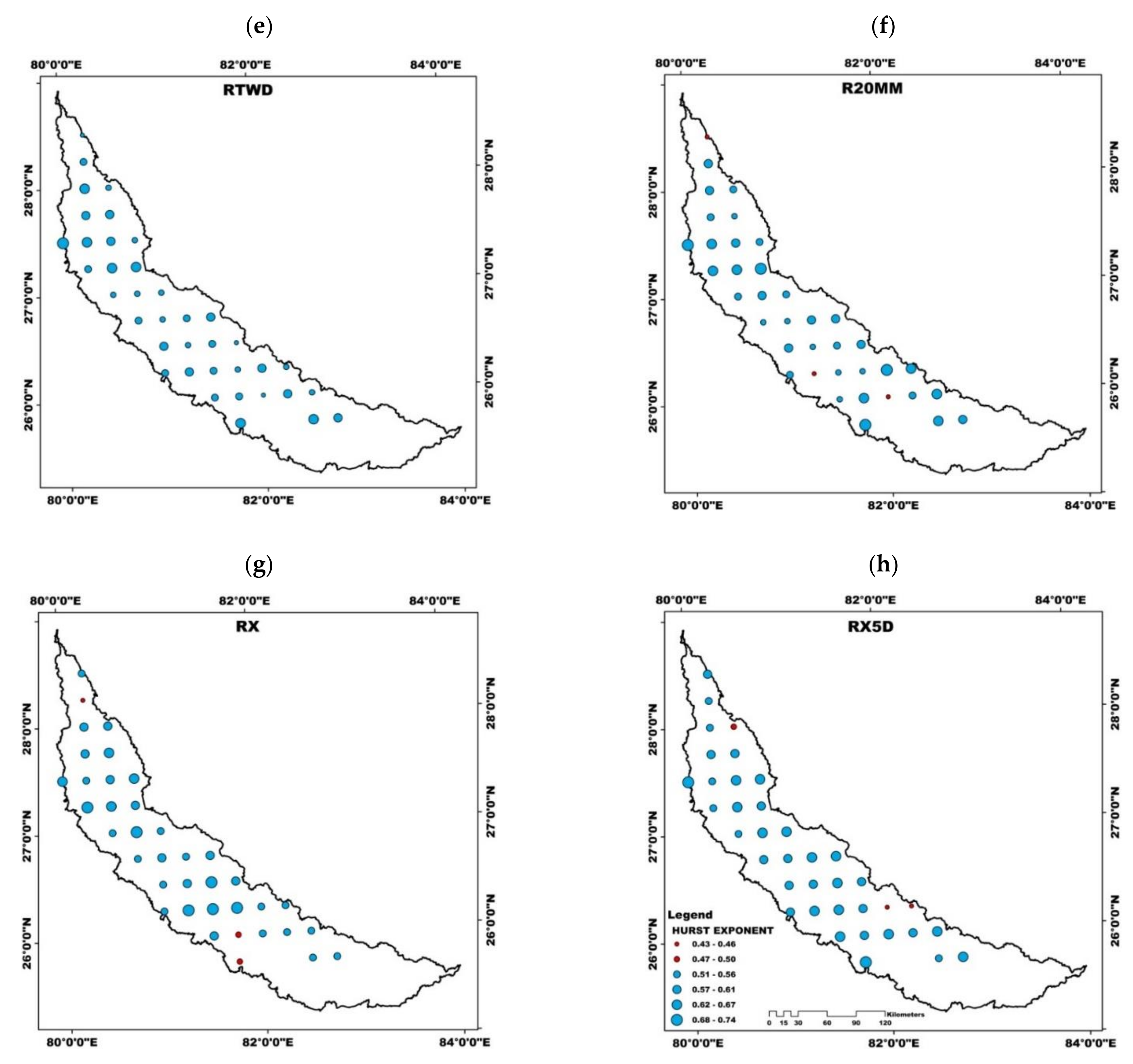


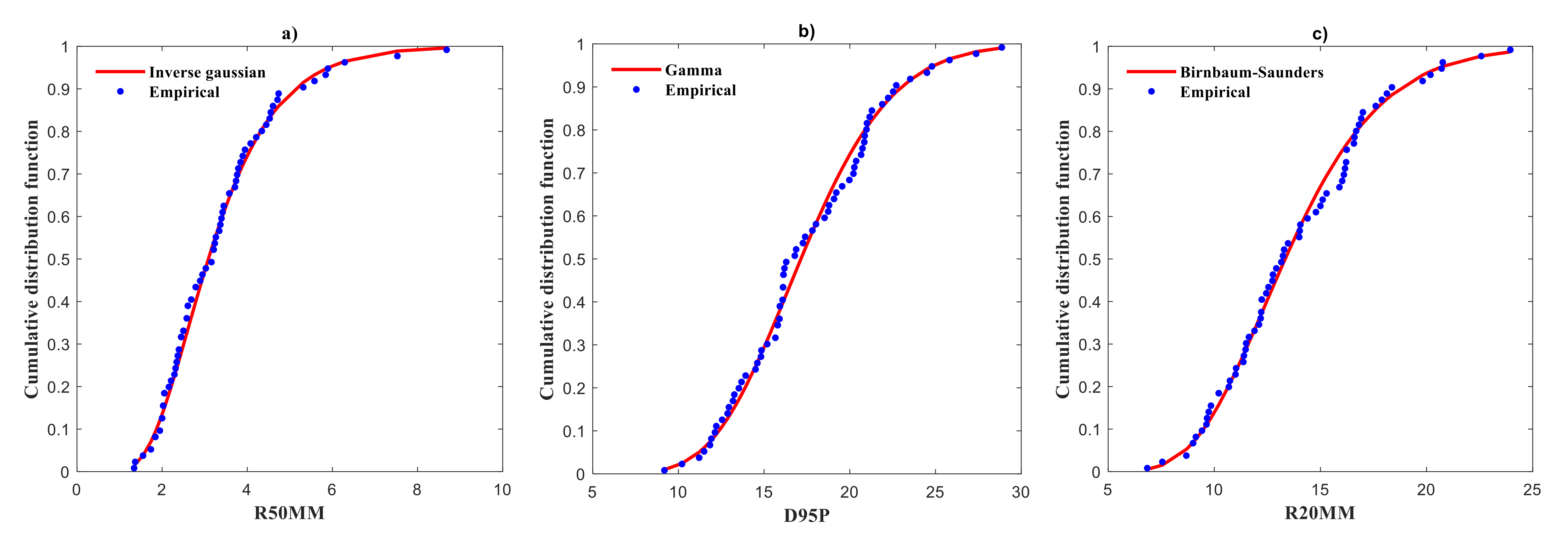
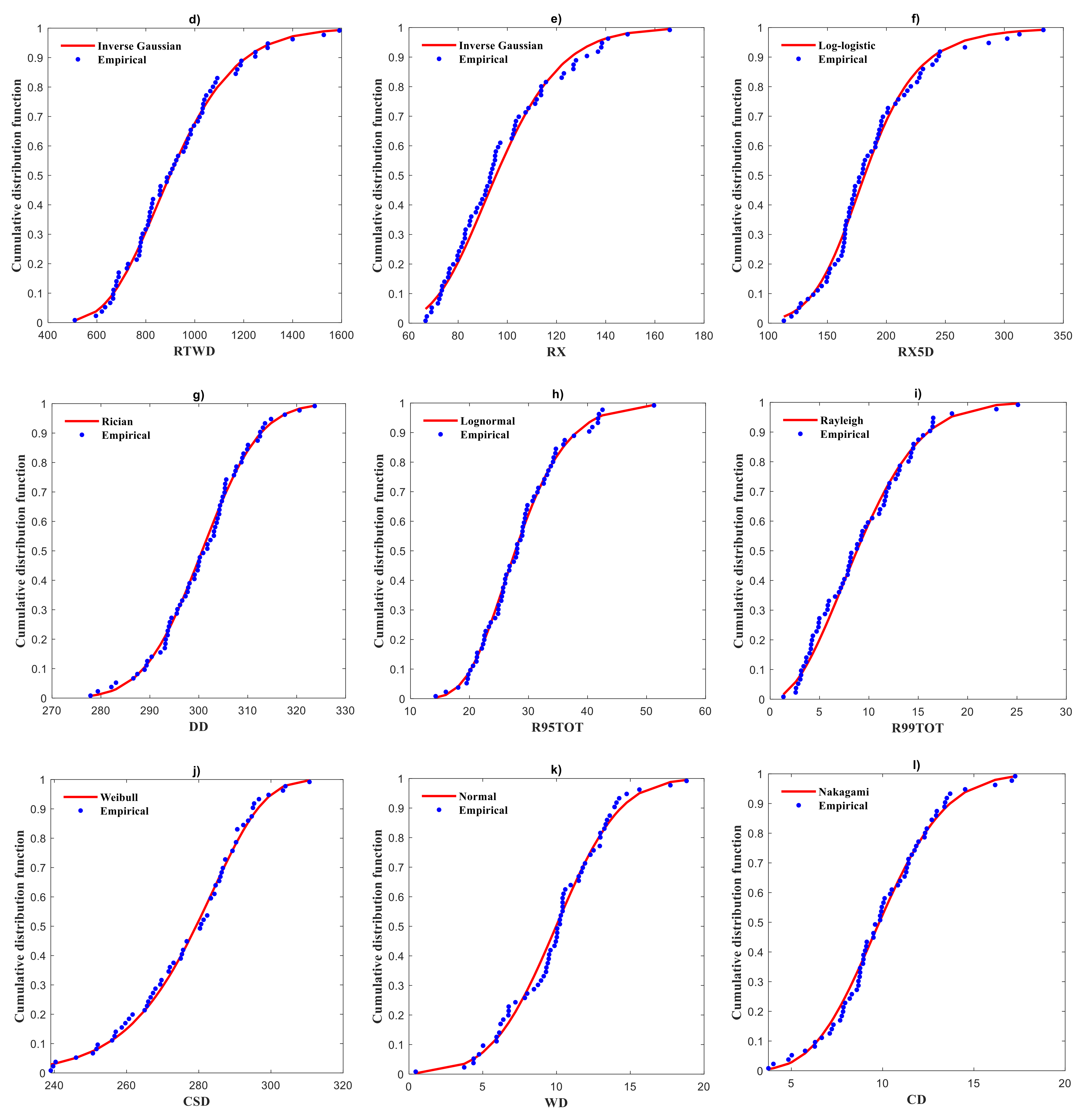
| S.NO. | INDICATOR | DESCRIPTIVE NAME | DEFINITION | UNIT |
|---|---|---|---|---|
| 1 | R50MM | Very heavy precipitation days | Number of days with precipitation above 50 mm. | Days |
| 2 | D95P | Very wet days | Days with precipitation > 95p. | Days |
| 3 | DD | Dry days | Days with precipitation less than 1 mm. | Days |
| 4 | R20MM | Heavy precipitation days | Days with daily precipitation amount ≥ 20 mm. | Days |
| 5 | R95TOT | Percentage precipitation of very wet days | Precipitation at days exceeding the 95th percentile divided by total precipitation expressed in percentage. | Percent |
| 6 | R99TOT | Precipitation fraction extremely wet days | Precipitation at days exceeding the 99th percentile divided by total precipitation expressed in percentage. | Percent |
| 7 | RTWD | Total precipitation wet days | Precipitation amount on days with RR ≥ 1 mm. | mm |
| 8 | RX | Maximum precipitation | The highest amount of daily precipitation. | mm |
| 9 | RX5D | Maximum 5 days R | Maximum consecutive five-days precipitation. | mm |
| 10 | CD | Percentage of cold days | Percentage of days with TX lower than the 10th percentile. | Days |
| 11 | VCD | Very cold days | Days with TN < 1st percentile. | Days |
| 12 | WN | Warm nights | Percentages of days with TN higher than the 90th percentile. | Days |
| 13 | CSD | Maximum consecutive summer days | Maximum number of consecutive summer days (TX > 25 Celsius). | Days |
| 14 | VWD | Very warm days | Days with TX > 99th percentile per year. | Days |
| 15 | WD | Warm days | Total no. of days with TX higher than the 90th percentile. | Days |
| 16 | SUD | Summer days | Number of days with TX > 25 Celsius. | Days |
| Copula Function | C(u,v) | Parameter Range |
|---|---|---|
| Frank | ||
| Clayton | ||
| Gumbel |
| Climate Index | Type of Distribution Function | |||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| Weibull | Gamma | Birbaum-Saunders | Inverse Gaussian | Log Normal | Nakagami | Log-Logistic | Rayleigh | Normal | Rician | |
| R50MM | 241.5 | 230.6 | 226.9 | 226.8 | 227.0 | 237.0 | 228.9 | 242.8 | 247.7 | 243.8 |
| D95P | 399.3 | 393.8 | 394.0 | 394.0 | 394.2 | 394.5 | 397.0 | 446.7 | 396.6 | 396.4 |
| R20MM | 379.4 | 373.0 | 372.8 | 372.8 | 373.0 | 374.2 | 375.7 | 416.9 | 377.1 | 376.8 |
| RTWD | 940.1 | 928.6 | 927.2 | 927.2 | 927.3 | 931.0 | 929.1 | 985.7 | 934.8 | 934.5 |
| RX | 628.4 | 614.7 | 612.1 | 612.0 | 612.2 | 617.9 | 615.4 | 679.0 | 622.1 | 621.9 |
| RX5D | 725.1 | 709.2 | 706.7 | 706.6 | 706.6 | 713.0 | 706.1 | 769.1 | 717.9 | 717.6 |
| DD | 512.3 | 505.8 | 505.9 | 505.9 | 505.9 | 505.7 | 506.7 | 822.2 | 505.6 | 505.6 |
| R95TOT | 470.9 | 462.2 | 462.0 | 462.0 | 462.0 | 463.7 | 462.8 | 514.0 | 466.6 | 466.4 |
| R99TOT | 410.5 | 410.1 | 413.5 | 414.8 | 413.9 | 410.5 | 416.7 | 406.3 | 421.0 | 410.5 |
| CSD | 576.6 | 581.5 | 582.3 | 582.3 | 582.4 | 580.8 | 583.8 | 811.5 | 580.1 | 580.1 |
| WD | 371.8 | 385.9 | 433.2 | 437.5 | 409.7 | 376.1 | 385.9 | 385.7 | 368.0 | 368.9 |
| CD | 342.9 | 343.0 | 347.5 | 347.7 | 347.0 | 341.4 | 344.3 | 375.0 | 341.8 | 341.6 |
| WN | 378.2 | 383.5 | 397.5 | 398.7 | 393.6 | 379.2 | 385.8 | 387.7 | 378.6 | 377.9 |
| SUD | 512.6 | 505.0 | 505.1 | 505.1 | 505.1 | 505.0 | 507.7 | 823.0 | 505.1 | 505.0 |
| Climate Index Combination | Copula Family | RMSE | AIC | Rank |
|---|---|---|---|---|
| RTWD&R95TOT | Clayton | 0.4175 | −403.707 | 3 |
| Frank | 0.2214 | −490.013 | 2 | |
| Gumbell | 0.1956 | −506.819 | 1 | |
| D95P&R95TOT | Clayton | 0.3863 | −414.272 | 3 |
| Frank | 0.2253 | −487.603 | 2 | |
| Gumbell | 0.1995 | −504.131 | 1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kalyan, A.; Ghose, D.K.; Thalagapu, R.; Guntu, R.K.; Agarwal, A.; Kurths, J.; Rathinasamy, M. Multiscale Spatiotemporal Analysis of Extreme Events in the Gomati River Basin, India. Atmosphere 2021, 12, 480. https://doi.org/10.3390/atmos12040480
Kalyan A, Ghose DK, Thalagapu R, Guntu RK, Agarwal A, Kurths J, Rathinasamy M. Multiscale Spatiotemporal Analysis of Extreme Events in the Gomati River Basin, India. Atmosphere. 2021; 12(4):480. https://doi.org/10.3390/atmos12040480
Chicago/Turabian StyleKalyan, AVS, Dillip Kumar Ghose, Rahul Thalagapu, Ravi Kumar Guntu, Ankit Agarwal, Jürgen Kurths, and Maheswaran Rathinasamy. 2021. "Multiscale Spatiotemporal Analysis of Extreme Events in the Gomati River Basin, India" Atmosphere 12, no. 4: 480. https://doi.org/10.3390/atmos12040480
APA StyleKalyan, A., Ghose, D. K., Thalagapu, R., Guntu, R. K., Agarwal, A., Kurths, J., & Rathinasamy, M. (2021). Multiscale Spatiotemporal Analysis of Extreme Events in the Gomati River Basin, India. Atmosphere, 12(4), 480. https://doi.org/10.3390/atmos12040480








