Transport Characteristics of the Electrification and Lightning of the Gas Mixture Representing the Atmospheres of the Solar System Planets
Abstract
1. Introduction
2. Method
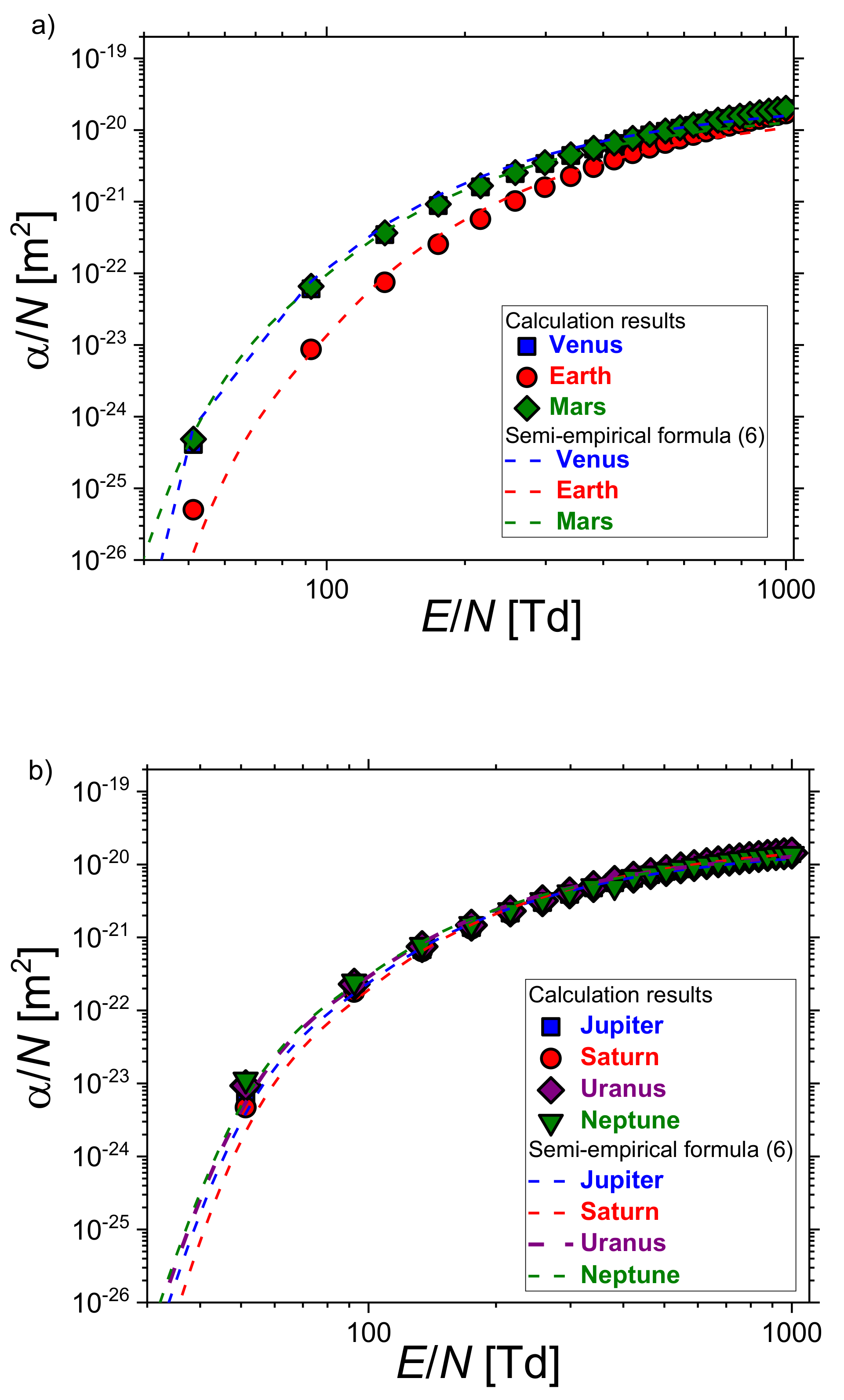
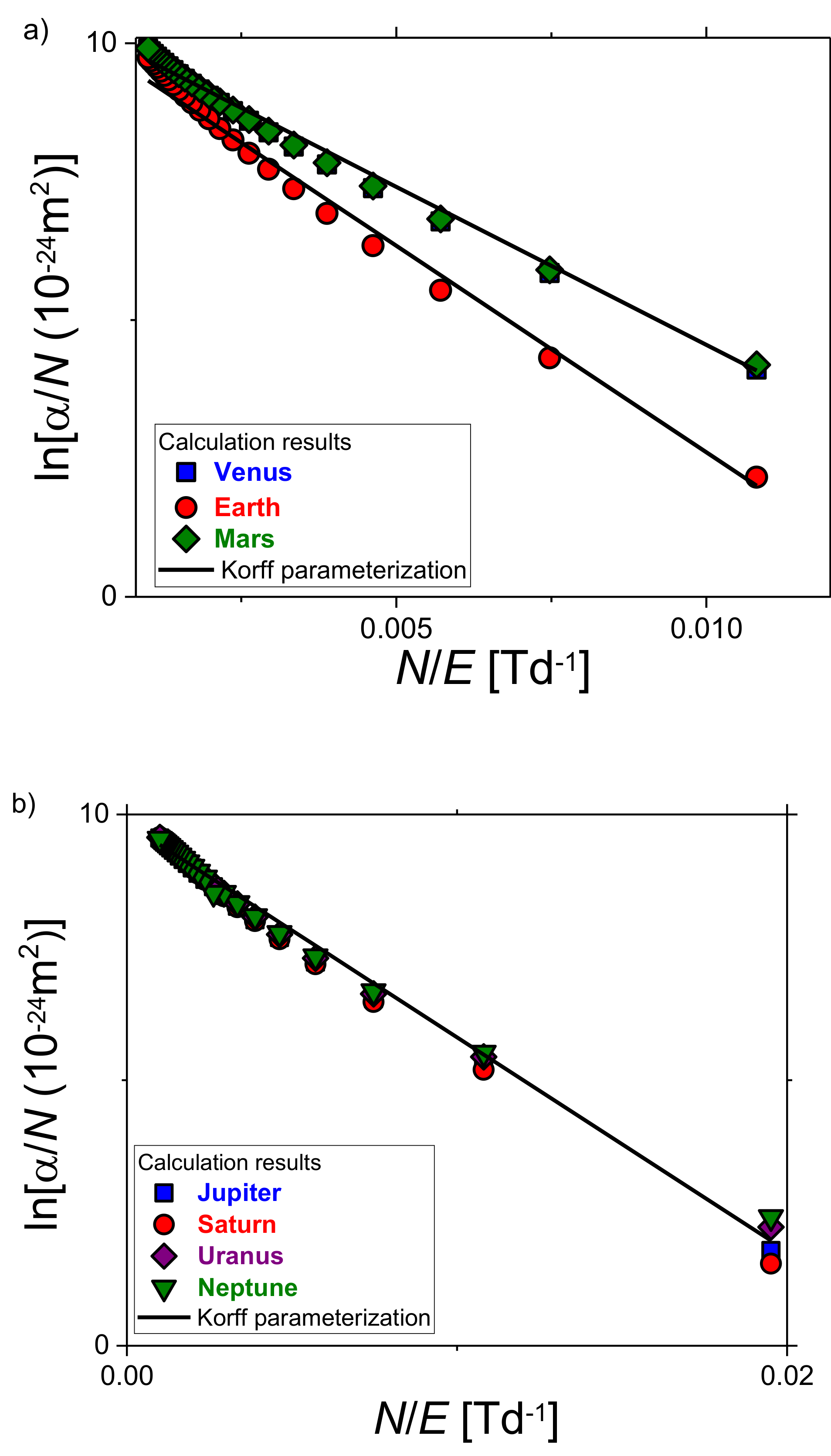
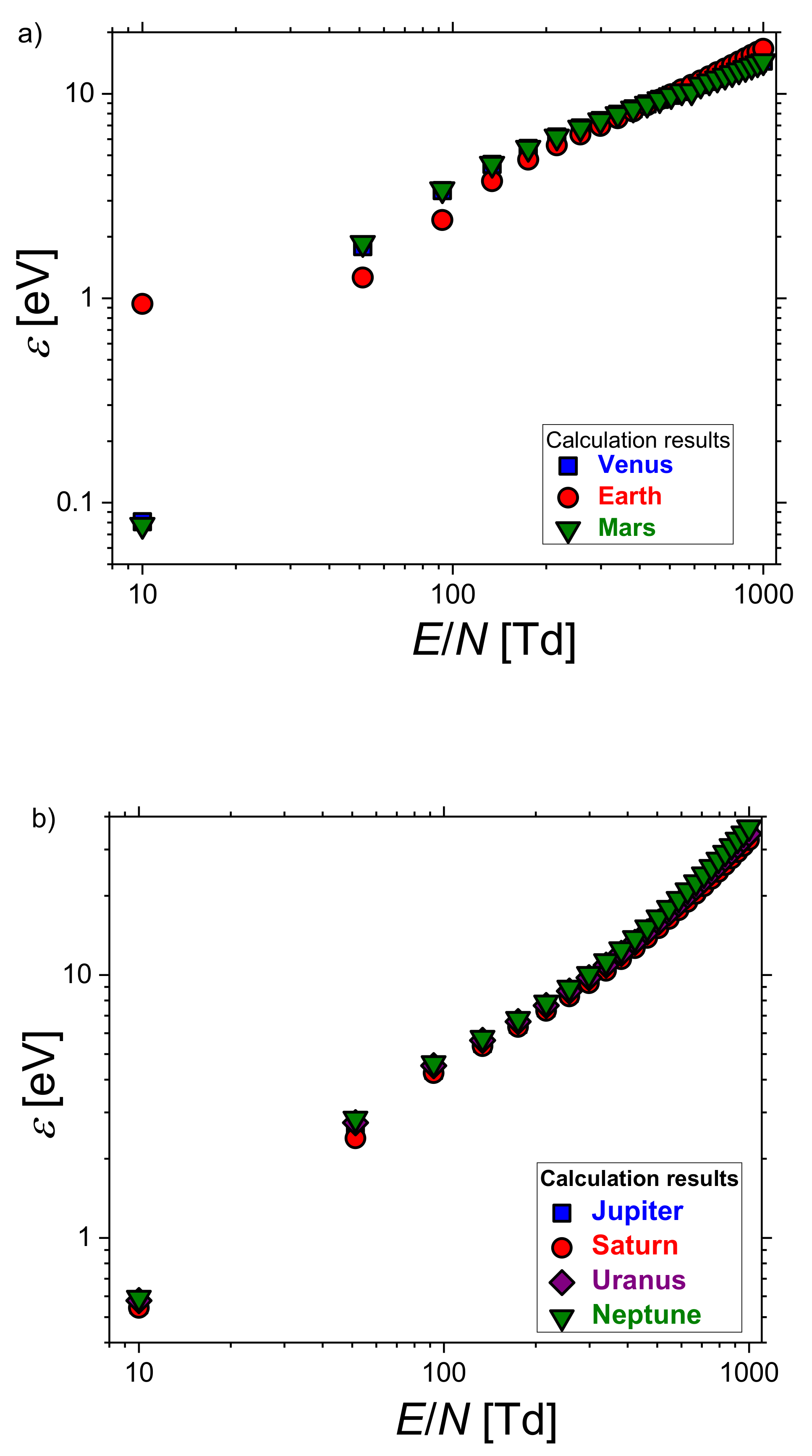
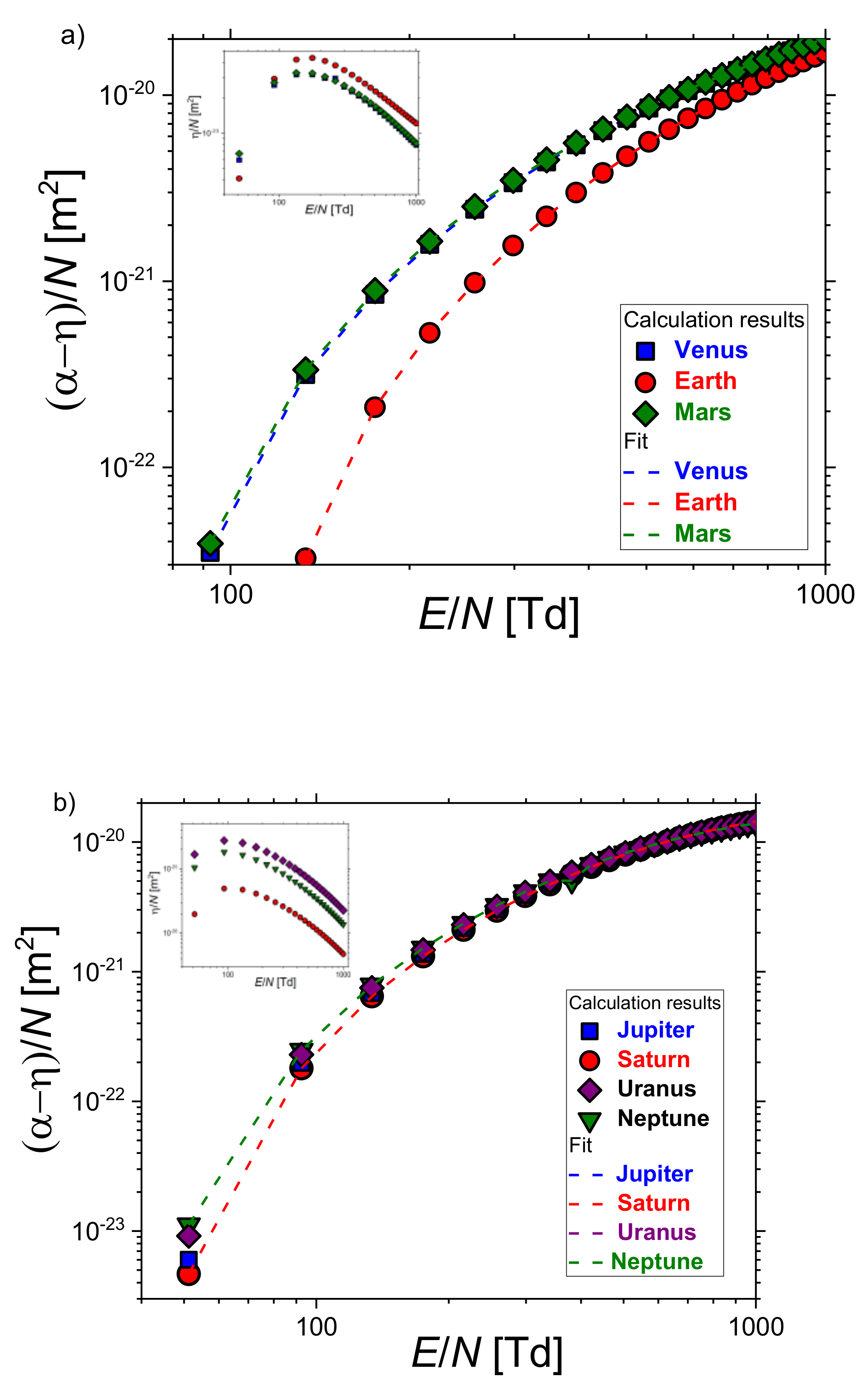
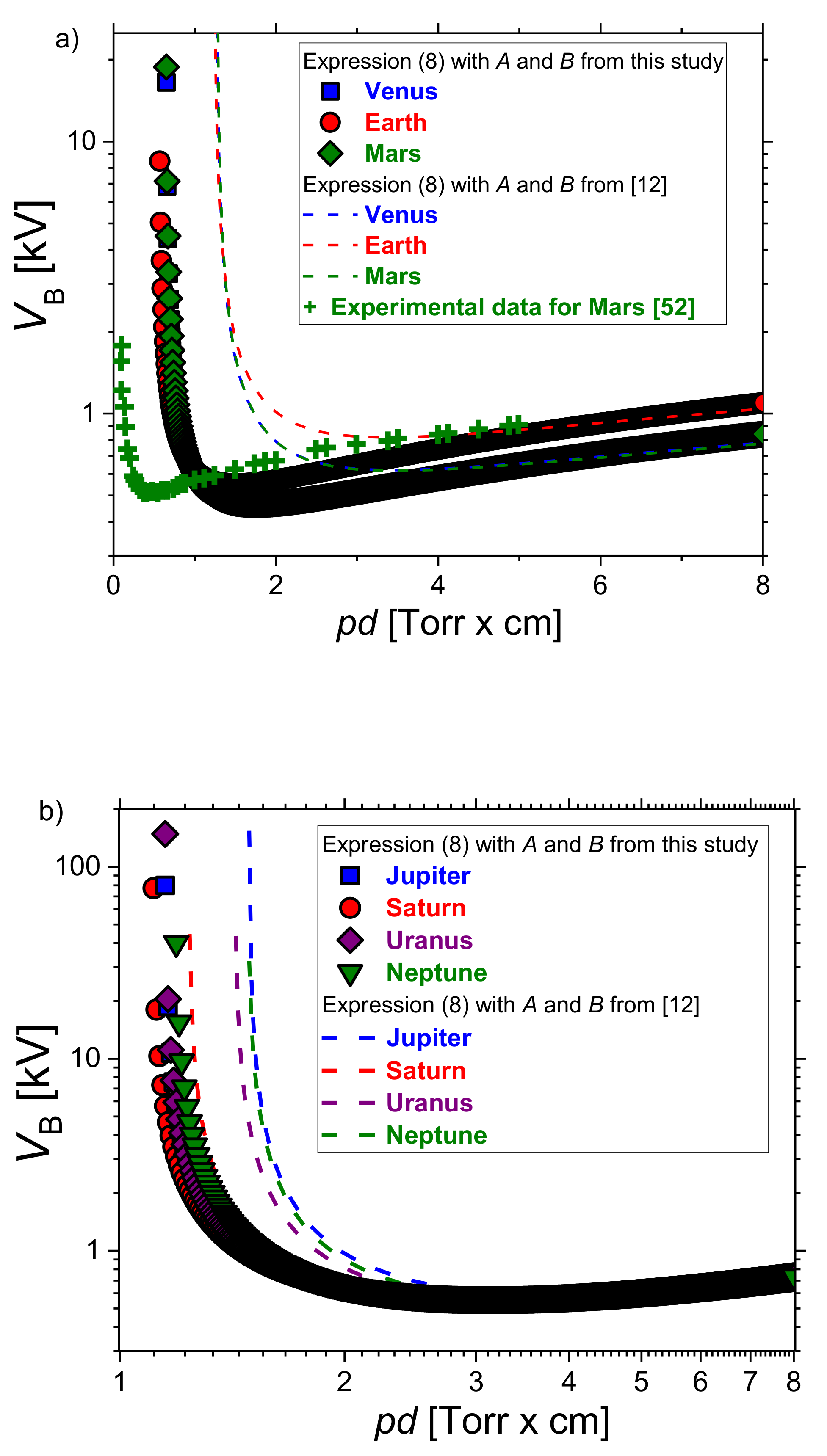
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Golombek, M.P. The Mars Pathfinder Mission. J. Geophys. Res. Planets 1997, 102, 3953–3965. [Google Scholar] [CrossRef]
- Squyres, S.W.; Arvidson, R.E.; Bell, J.F.; Brückner, J.; Cabrol, N.A.; Calvin, W.; Carr, M.H.; Christensen, P.R.; Clark, B.C.; Crumpler, L.; et al. The opportunity Rover’s Athena science investigation at Meridiani Planum, Mars. Science 2004, 306, 1698–1703. [Google Scholar] [CrossRef] [PubMed]
- Krapivin, V.F.; Varotsos, C.A.; Christodoulakis, J. Mission to Mars: Adaptive identifier for the solution of inverse optical metrology tasks. Earth Moon Planets 2016, 118, 1–14. [Google Scholar] [CrossRef]
- Chow, D. NASA’s Mars Rover Successfully Touches Down on the Red Planet. Available online: https://www.nbcnews.com/science/space/nasas-mars-rover-perseverance-touches-red-planet-rcna295 (accessed on 19 February 2021).
- Gibney, E. Rescued Japanese spacecraft delivers first results from Venus. Nature 2016, 532, 157–158. [Google Scholar] [CrossRef][Green Version]
- Barth, E. Planet CARMA: A new framework for studying the microphysics of planetary atmospheres. Atmosphere 2020, 11, 1064. [Google Scholar] [CrossRef]
- Inza, A.G.M.; Lopez-Reyes, G. Mars Exploration-A Step Forward; IntechOpen: London, UK, 2020. [Google Scholar]
- Mazur, V.; Moreau, J. Aircraft-triggered lightning: Processes following strike initiation that affect aircraft. J. Aircr. 1992, 29, 575–580. [Google Scholar] [CrossRef]
- Larsson, A. The interaction between a lightning flash and an aircraft in flight. Comptes Rendus Phys. 2002, 3, 1423–1444. [Google Scholar] [CrossRef]
- SKYbrary. Lightning. Available online: https://www.skybrary.aero/index.php/Lightning (accessed on 5 August 2019).
- Manning, H.; Kate, I.T.; Battel, S.; Mahaffy, P. Electric discharge in the Martian atmosphere, Paschen curves and implications for future missions. Adv. Space Res. 2010, 46, 1334–1340. [Google Scholar] [CrossRef]
- Helling, C.; Jardine, M.; Stark, C.R.; Diver, D.A. Ionization in atmospheres of brown dwarfs and extrasolar planets. III. breakdown conditions for mineral clouds. Astrophys. J. 2013, 767, 136. [Google Scholar] [CrossRef]
- Zahnle, K.; Schaefer, L.; Fegley, B. Earth’s earliest atmosphere. Cold Spring Harb. Perspect. Biol. 2010, 2, a004895. [Google Scholar] [CrossRef] [PubMed]
- Camprubí, E.; De Leeuw, J.W.; House, C.H.; Raulin, F.; Russell, M.J.; Spang, A.; Tirumalai, M.R.; Westall, F. The Emergence of Life. Space Sci. Rev. 2019, 215, 56. [Google Scholar] [CrossRef]
- Hodosán, G.; Helling, C.; Asensio-Torres, R.; Vorgul, I.; Rimmer, P.B. Lightning climatology of exoplanets and brown dwarfs guided by Solar system data. Mon. Not. R. Astron. Soc. 2016, 461, 3927–3947. [Google Scholar] [CrossRef]
- Pasko, V.P.; Yair, Y.; Kuo, C.L. Lighning related transient luminous events at high altitude in the Earth’s atmosphere: Phenomenology, mechanisms and effects. Space Sci. Rev. 2012, 168, 475–516. [Google Scholar] [CrossRef]
- Cooray, V. Interaction of lightning flashes with the Earth’s atmosphere. In An Introduction to Lightning; Springer: Dordrecht, The Netherlands, 2014. [Google Scholar] [CrossRef]
- Gurnett, D.A.; Shaw, R.R.; Anderson, R.R.; Kurth, W.S.; Scarf, F.L. Whistlers observed by Voyager 1: Detection of lightning on Jupiter. Geophys. Res. Lett. 1979, 6, 511–514. [Google Scholar] [CrossRef]
- Cook, A.F.; Duxbury, T.C.; Hunt, G.E. First results on Jovian lightning. Nature 1979, 280, 794. [Google Scholar] [CrossRef]
- Gurnett, D.A.; Kurth, W.S.; Cairns, I.H.; Granroth, L.J. Whistlers in Neptune’s magnetosphere: Evidence of atmospheric lightning. J. Geophys. Res. 1990, 95, 20967. [Google Scholar] [CrossRef]
- Yair, Y. New results on planetary lightning. Adv. Space Res. 2012, 50, 293–310. [Google Scholar] [CrossRef]
- Dubrovin, D.; Luque, A.; Gordillo-Vazquez, F.J.; Yair, Y.; Parra-Rojas, F.C.; Ebert, U.; Price, C. Impact of lightning on the lower ionosphere of Saturn and possible generation of halos and sprites. Icarus 2014, 241, 313–328. [Google Scholar] [CrossRef]
- Luque, A.; Gordillo-Vazquez, F.J.; Pallé, E. Ground-based search for lightning in Jupiter with GTC/OSIRIS fast photometry and tunable filters. Astron. Astrophys. 2015, 577, A94. [Google Scholar] [CrossRef]
- Giles, R.S.; Greathouse, T.K.; Bonfond, B.; Gladstone, G.R.; Kammer, J.A.; Hue, V.; Grodent, D.C.; Gérard, J.C.; Versteeg, M.H.; Wong, M.H.; et al. Possible transient luminous events observed in Jupiter’s upper atmosphere. J. Geophys. Res. Planets 2020, 125, e2020JE006659. [Google Scholar] [CrossRef]
- Scarf, F.L.; Taylor, W.W.L.; Russell, C.T.; Brace, L.H. Lightning on Venus: Orbiter detection of whistler signals. J. Geophys. Res. 1980, 85, 8158–8166. [Google Scholar] [CrossRef]
- Pérez-Invernón, F.J.; Luque, A.; Gordillo-Vázquez, F.J. Mesospheric optical signatures of possible lightning on Venus. J. Geophys. Res. Space Phys. 2016, 121, 7026–7048. [Google Scholar] [CrossRef]
- Brace, L.H.; Kliore, A.J. The structure of the Venus ionosphere. Space Sci. Rev. 1991, 55, 81–163. [Google Scholar] [CrossRef]
- Huba, J.D. Theory of small-scale density and electric field fluctuations in the nightside Venus ionosphere. J. Geophys. Res. Space Phys. 1992, 97, 43. [Google Scholar] [CrossRef]
- Moinelo, A.C.; Abildgaard, S.; Muñoz, A.G.; Piccioni, G.; Grassi, D. No statistical evidence of lightning in Venus night-side atmosphere from VIRTIS-Venus Express Visible observations. Icarus 2016, 277, 395–400. [Google Scholar] [CrossRef]
- Pérez-Invernón, F.J.; Lehtinen, N.G.; Gordillo-Vázquez, F.J.; Luque, A. Whistler wave propagation through the ionosphere of Venus. J. Geophys. Res. Space Phys. 2017, 122, 11633–11644. [Google Scholar] [CrossRef]
- Lorenz, R.D. Lightning detection on Venus: A critical review. Prog. Earth Planet. Sci. 2018, 5, 34. [Google Scholar] [CrossRef]
- Yair, Y.; Takahashi, Y.; Yaniv, R.; Ebert, U.; Goto, Y. A study of the possibility of sprites in the atmospheres of other planets. J. Geophys. Res. Planets 2009, 114, 09002. [Google Scholar] [CrossRef]
- Luque, A.; Dubrovin, D.; Vázquez, F.J.G.; Ebert, U.; Rojas, F.C.P.; Yair, Y.; Price, C. Coupling between atmospheric layers in gaseous giant planets due to lightning-generated electromagnetic pulses. J. Geophys. Res. Space Phys. 2014, 119, 8705–8720. [Google Scholar] [CrossRef]
- Riousset, J.A.; Nag, A.; Palotai, C. Scaling of conventional breakdown threshold: Impact for predictions of lightning and TLEs on Earth, Venus, and Mars. Icarus 2020, 338, 113506. [Google Scholar] [CrossRef]
- Yuan, T.; Remer, L.A.; Pickering, K.E.; Yu, H. Observational evidence of aerosol enhancement of lightning activity and convective invigoration. Geophys. Res. Lett. 2011, 38, 4701. [Google Scholar] [CrossRef]
- Borucki, W.J.; McKay, C.P. Optical efficiencies of lightning in planetary atmospheres. Nature 1987, 328, 509–510. [Google Scholar] [CrossRef] [PubMed]
- Yair, Y.; Levin, Z.; Tzivion, S. Lightning generation in a jovian thundercloud: Results from an axisymmetric numerical cloud model. Icarus 1995, 115, 421–434. [Google Scholar] [CrossRef]
- Fischer, G.; Kurth, W.S.; Dyudina, U.A.; Kaiser, M.L.; Zarka, P.; Lecacheux, A.; Ingersoll, A.P.; Gurnett, D.A. Analysis of a giant lightning storm on Saturn. Icarus 2007, 190, 528–544. [Google Scholar] [CrossRef]
- Fischer, G.; Gurnett, D.A.; Kurth, W.S.; Akalin, F.; Zarka, P.; Dyudina, U.A.; Farrell, W.M.; Kaiser, M.L. Atmospheric electricity at Saturn. Space Sci. Rev. 2008, 137, 271–285. [Google Scholar] [CrossRef]
- Dyudina, U.A.; Ingersoll, A.P.; Ewald, S.P.; Porco, C.C.; Fischer, G.; Kurth, W.S.; West, R.A. Detection of visible lightning on Saturn. Geophys. Res. Lett. 2010, 37, 09205. [Google Scholar] [CrossRef]
- Dyudina, U.A.; Ingersoll, A.P.; Ewald, S.P.; Porco, C.C.; Fischer, G.; Yair, Y. Saturn’s visible lightning, its radio emissions, and the structure of the 2009–2011 lightning storms. Icarus 2013, 226, 1020–1037. [Google Scholar] [CrossRef]
- Becker, H.N.; Alexander, J.W.; Atreya, S.K.; Bolton, S.J.; Brennan, M.J.; Brown, S.T.; Guillaume, A.; Guillot, T.; Ingersoll, A.P.; Levin, S.M.; et al. Small lightning flashes from shallow electrical storms on Jupiter. Nature 2020, 584, 55–58. [Google Scholar] [CrossRef]
- Hagelaar, G.J.M.; Pitchford, L.C. Solving the Boltzmann equation to obtain electron transport coefficients and rate coefficients for fluid models. Plasma Sources Sci. Technol. 2005, 14, 722–733. [Google Scholar] [CrossRef]
- Hagelaar, G.J.M. Coulomb collisions in the Boltzmann equation for electrons in low-temperature gas discharge plasmas. Plasma Sources Sci. Technol. 2016, 25, 015015. [Google Scholar] [CrossRef]
- Bolsig+. Electron Boltzmann Equation Solver Bolsig+. Available online: http://www.bolsig.laplace.univ-tlse.fr/download.html (accessed on 5 January 2021).
- Hagelaar, G.J.M. Brief Documentation of BOLSIG+ Version 03/2016. Available online: http://www.bolsig.laplace.univ-tlse.fr/wp-content/uploads/2016/03/bolsigdoc0316.pdf (accessed on 5 January 2021).
- The Atmospheres of the Solar System. Available online: https://www.compoundchem.com/2014/07/25/planetatmospheres/ (accessed on 25 July 2014).
- The Plasma Data Exchange Project. Available online: https://nl.lxcat.net/home/ (accessed on 5 January 2021).
- Marić, D.; Radmilović-Radjenović, M.; Petrović, Z.L. On parametrization and mixture laws for electron ionization Coefficients. Eur. Phys. J. D 2005, 35, 313–321. [Google Scholar] [CrossRef]
- Petri, A.; Goncalves, J.; Mangiarotti, A.; Botelho, S.; Bueno, C. Measurement of the first Townsend ionization coefficient in a methane-based tissue-equivalent gas. Nucl. Instrum. Methods Phys. Res. Sect. A 2017, 849, 31–40. [Google Scholar] [CrossRef]
- Engle, J.A.; Riousset, J.A. Numerical and analytical studies of critical radius in new geometries for corona discharge in air and CO2-rich environments. In Proceedings of the 2017 CEDAR Workshop, Workshop on Thunderstorm Coupling and Effects, Keystone, CO, USA, 22 June 2017; Available online: http://riousset.com/jeremy/wp-content/uploads/EngleCEDAR2017a.pdf (accessed on 4 February 2021).
- Calle, C.; Mackey, P.; Hogue, M.; Johansen, M.; Kelley, J.; Phillips, J.R., III; Clements, J.S. An electrostatic precipitator system for the Martian environment. J. Electrost. 2013, 71, 254–256. [Google Scholar] [CrossRef][Green Version]
- Rafkin, S.; Michaels, T. The Mars Regional Atmospheric Modeling System (MRAMS): Current status and future directions. Atmosphere 2019, 10, 747. [Google Scholar] [CrossRef]
- Fairén, A.G.; Parro, V.; Schulze-Makuch, D.; Whyte, L. Is Searching for Martian life a priority for the Mars community? Astrobiology 2018, 18, 101–107. [Google Scholar] [CrossRef] [PubMed]


| BOLSIG+ Results | Data Taken from Ref. [12] | |||||||
|---|---|---|---|---|---|---|---|---|
| Planet | A [1/(cm·Torr)] | B [V/(cm·Torr)] | Vmin [V] | (pd)min [cm ×Torr] | A [1/(cm·Torr)] | B [V/(cm·Torr)] | Vmin [V] | (pd)min [cm × Torr] |
| Venus | 14.32 | 268 | 468 | 1.75 | 7.27 | 180 | 465 | 2.58 |
| Earth | 16.56 | 365 | 552 | 1.51 | 7.44 | 243 | 617 | 2.53 |
| Mars | 14.30 | 264 | 463 | 1.75 | 7.23 | 178 | 462 | 2.60 |
| Jupiter | 8.03 | 182 | 567 | 3.12 | 6.19 | 143 | 434 | 3.06 |
| Saturn | 8.32 | 188 | 565 | 3.01 | 7.46 | 156 | 392 | 2.52 |
| Uranus | 8.02 | 177 | 553 | 3.12 | 6.47 | 138 | 401 | 2.90 |
| Neptune | 7.78 | 174 | 562 | 3.22 | 6.22 | 135 | 408 | 3.02 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Radmilović-Radjenović, M.; Sabo, M.; Radjenović, B. Transport Characteristics of the Electrification and Lightning of the Gas Mixture Representing the Atmospheres of the Solar System Planets. Atmosphere 2021, 12, 438. https://doi.org/10.3390/atmos12040438
Radmilović-Radjenović M, Sabo M, Radjenović B. Transport Characteristics of the Electrification and Lightning of the Gas Mixture Representing the Atmospheres of the Solar System Planets. Atmosphere. 2021; 12(4):438. https://doi.org/10.3390/atmos12040438
Chicago/Turabian StyleRadmilović-Radjenović, Marija, Martin Sabo, and Branislav Radjenović. 2021. "Transport Characteristics of the Electrification and Lightning of the Gas Mixture Representing the Atmospheres of the Solar System Planets" Atmosphere 12, no. 4: 438. https://doi.org/10.3390/atmos12040438
APA StyleRadmilović-Radjenović, M., Sabo, M., & Radjenović, B. (2021). Transport Characteristics of the Electrification and Lightning of the Gas Mixture Representing the Atmospheres of the Solar System Planets. Atmosphere, 12(4), 438. https://doi.org/10.3390/atmos12040438





