The Micrometeorology of the Haifa Bay Area and Mount Carmel during the Summer
Abstract
1. Introduction
2. Materials and Methods
2.1. Weather Stations
2.2. Wind Field Interpolation
2.3. Nonparametric Bivariate Density
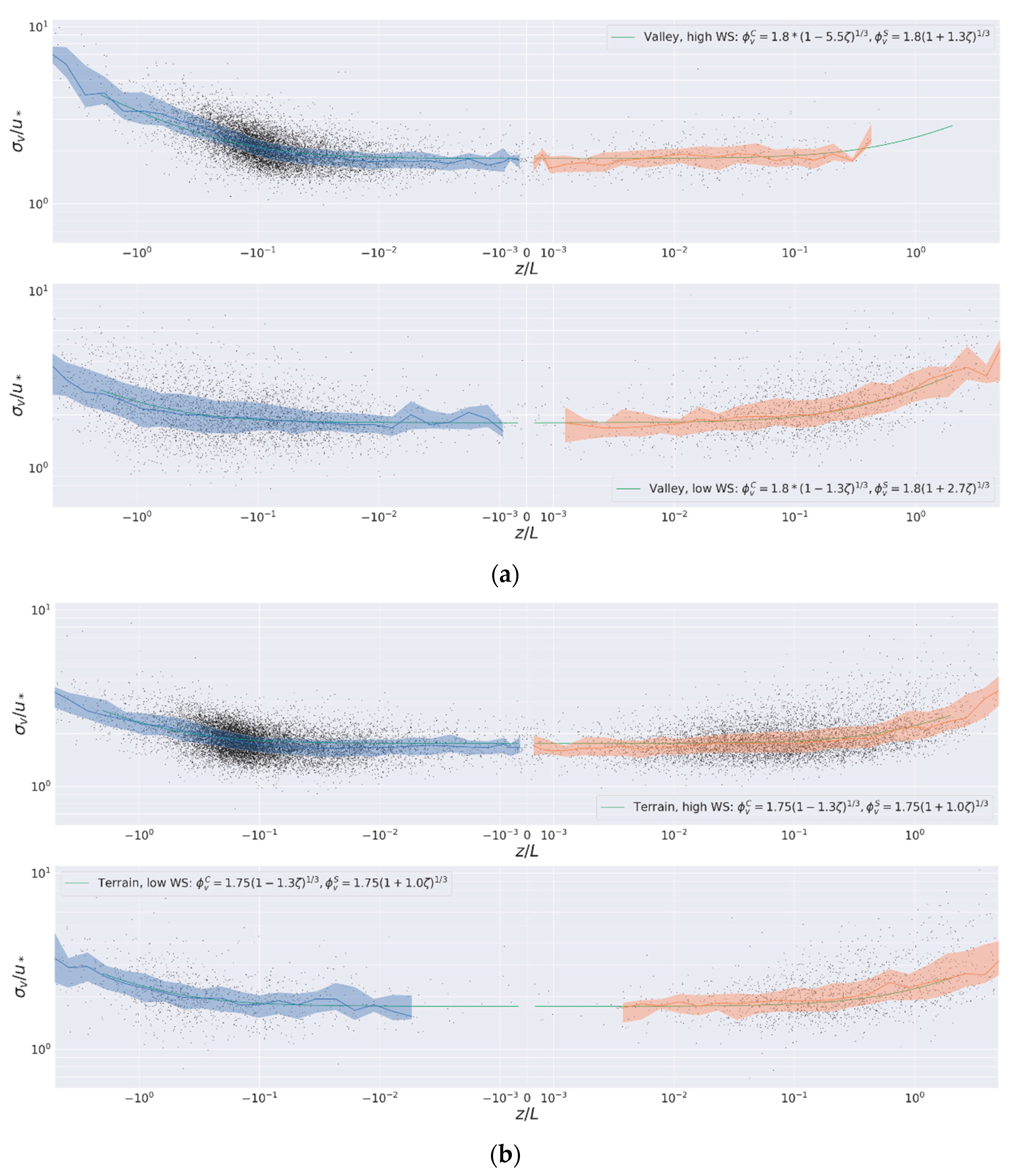
2.4. Surface Layer Turbulence Parameters
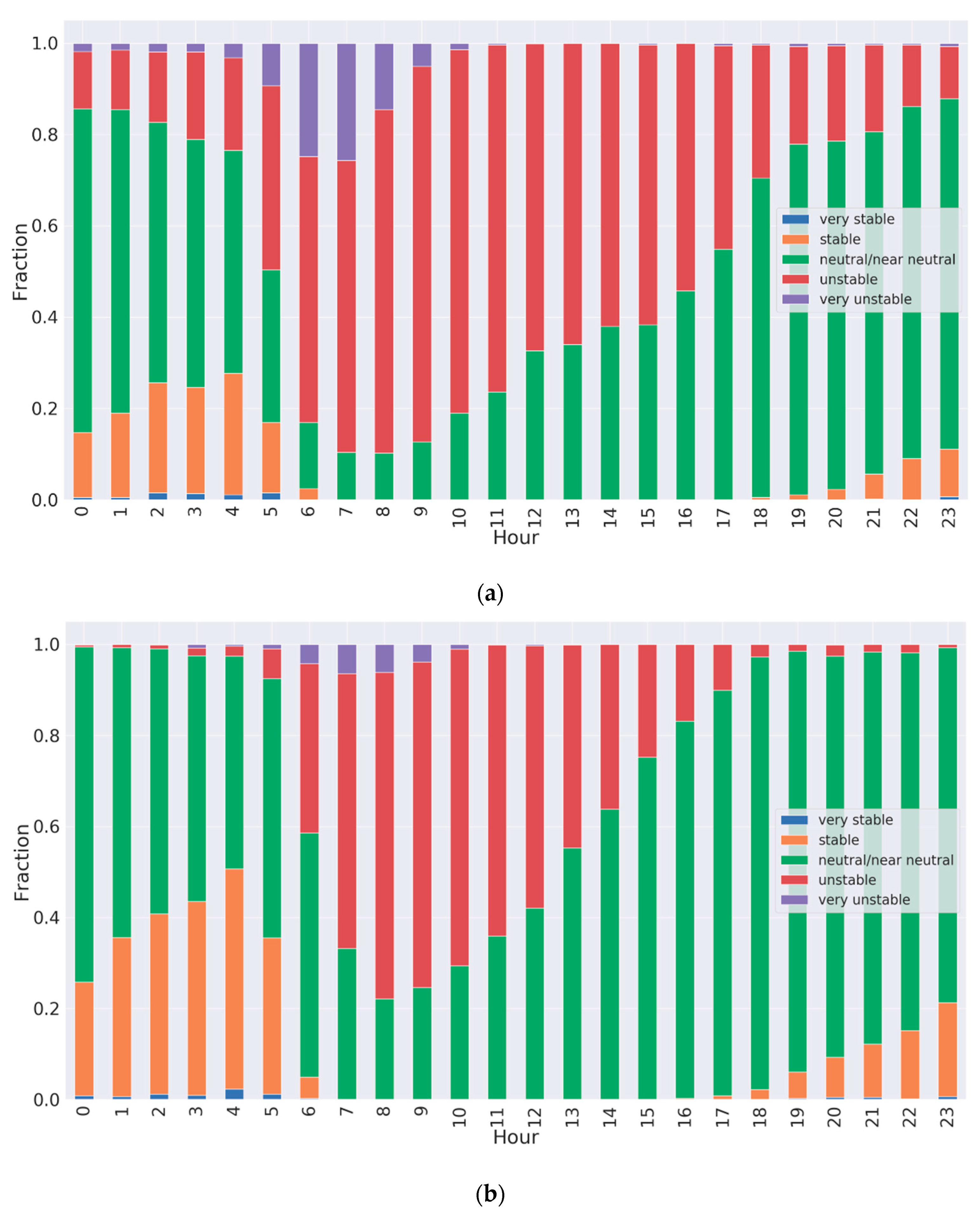
3. Results
4. Discussion and Summary
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- CBS. I.C.B. of S. Statistical Abstract of Israel—No.71. Available online: https://www.cbs.gov.il/he/publications/doclib/2020/2.shnatonpopulation/st02_25.pdf (accessed on 8 November 2020).
- Goldreich, Y.; Freundlich, A.; Alpert, P. Rainfall anomaly over the lee side of Mount Carmel (Israel) and the associated wind field. J. Appl. Meteorol. 1997, 36, 748–762. [Google Scholar] [CrossRef]
- Federman, R.; Mendelson, A.; Romem, E.; Mor-Federman, T.; Lahav, C.; Ron, M.; Ramon, U. Zevulun Survey—Survey, Analysis and Evaluation of Nature, Landscape and Human Heritage; Open Landscape Institute (OLI): Tel-Aviv, Israel, 2015. [Google Scholar]
- Freiman, M.T.; Hirshel, N.; Broday, D.M. Urban-Scale variability of ambient particulate matter attributes. Atmos. Environ. 2006, 40, 5670–5684. [Google Scholar] [CrossRef]
- Yuval; Broday, D.M. High-Resolution spatial patterns of long-term mean concentrations of air pollutants in Haifa Bay area. Atmos. Environ. 2006, 40, 3653–3664. [Google Scholar] [CrossRef]
- Eitan, O.; Yuval; Barchana, M.; Dubnov, J.; Linn, S.; Carmel, Y.; Broday, D.M. Spatial analysis of air pollution and cancer incidence rates in Haifa Bay, Israel. Sci. Total Environ. 2010, 408, 4429–4439. [Google Scholar] [CrossRef] [PubMed]
- Broday, D.; Dayan, U.; Aharonov, E.; Laufer, D.; Adel, M. Emissions from gas processing platforms to the atmosphere-case studies versus benchmarks. Environ. Impact Assess. Rev. 2020, 80, 106313. [Google Scholar] [CrossRef]
- Gavze, E.; Fattal, E. A semi-analytical model for short-range near-ground continuous dispersion. Bound. Layer Meteorol. 2018, 169, 297–326. [Google Scholar] [CrossRef]
- Shnapp, R.; Bohbot-Raviv, Y.; Liberzon, A.; Fattal, E. Turbulence-Obstacle interactions in the Lagrangian framework: Applications for stochastic modeling in canopy flows. Phys. Rev. Fluids 2020, 5, 094601. [Google Scholar] [CrossRef]
- Koch, J.; Dayan, U. A synoptic analysis of the meteorological conditions affecting dispersion of pollutants emitted from tall stacks in the coastal plain of Israel. Atmos. Environ. 1992, 26, 2537–2543. [Google Scholar] [CrossRef]
- Bitan, A.; Rubin, S. Climatic Atlas of Israel for Physical and Environmental Planning and Design; Ramot Publishing—Tel Aviv University: Tel-Aviv, Israel, 1991. [Google Scholar]
- Saaroni, H.; Levi, E.; Ziv, B. Particulate matter in the summer season and its relation to synoptic conditions and regional climatic stress—The case of Haifa, Israel. Water Air Soil Pollut. 2018, 229. [Google Scholar] [CrossRef]
- Alpert, P.; Osetinsky, I.; Ziv, B.; Shafir, H. A new seasons definition based on classified daily synoptic systems: An example for the eastern Mediterranean. Int. J. Climatol. 2004, 24, 1013–1021. [Google Scholar] [CrossRef]
- Finkelstein, P.L.; Kaimal, J.C.; Gaynor, J.E.; Graves, M.E.; Lockhart, T.J. Comparison of wind monitoring systems. Part I: In situ sensors. J. Atmos. Ocean. Technol. 1986, 3, 583–593. [Google Scholar] [CrossRef]
- Bowen, B.M. Improved wind and turbulence measurements using a low-cost 3-D sonic anemometer at a low-wind site. Open Atmos. Sci. J. 2008, 2, 131–138. [Google Scholar] [CrossRef]
- Izumi, Y.; Barad, M.L. Wind speeds as measured by cup and sonic anemometers and influenced by tower structure. J. Appl. Meteorol. 1970, 9, 851–856. [Google Scholar] [CrossRef]
- Busch, N.E.; Kristensen, L. Cup anemometer overspeeding. J. Appl. Meteorol. 1976, 15, 1328–1332. [Google Scholar] [CrossRef]
- Kristensen, L. Cup anemometer behavior in turbulent environments. J. Atmos. Ocean. Technol. 1998, 15, 5–17. [Google Scholar] [CrossRef]
- Louka, P.; Belcher, S.E.; Harrison, R.G. Coupling between air flow in streets and the well-developed boundary layer aloft. Atmos. Environ. 2000, 34, 2613–2621. [Google Scholar] [CrossRef]
- Lu, G.Y.; Wong, D.W. An adaptive inverse-distance weighting spatial interpolation technique. Comput. Geosci. 2008, 34, 1044–1055. [Google Scholar] [CrossRef]
- Yao, X.; Fu, B.; Lü, Y.; Sun, F.; Wang, S.; Liu, M. Comparison of four spatial interpolation methods for estimating soil moisture in a complex terrain catchment. PLoS ONE 2013, 8. [Google Scholar] [CrossRef] [PubMed]
- Palomino, I.; Martín, F. A simple method for spatial interpolation of the wind in complex terrain. J. Appl. Meteorol. 1995, 34, 1678–1693. [Google Scholar] [CrossRef]
- Fattal, E. A non-homogenous non-Gaussian Lagrangian stochastic model for pollutant dispersion in complex topography—Comparison to Haifa 2009 urban tracer campaign (in Hebrew); 2014/56/53/5614; Israel Institute for Biological Research: Ness-Ziona, Israel, 2014. [Google Scholar]
- Goodin, W.R.; McRa, G.J.; Seinfeld, J.H. A comparison of interpolation methods for sparse data: Application to wind and concentration Fields. J. Appl. Meteorol. 1979, 18, 761–771. [Google Scholar] [CrossRef][Green Version]
- Stull, R.B. (Ed.) An Introduction to Boundary Layer Meteorology; Springer Netherlands: Dordrecht, The Netherlands, 1988; ISBN 978-90-277-2769-5. [Google Scholar]
- Wilson, J.D. Monin-Obukhov functions for standard deviations of velocity. Bound. Layer Meteorol. 2008, 129, 353–369. [Google Scholar] [CrossRef]
- Charuchittipan, D.; Wilson, J.D. Turbulent kinetic energy dissipation in the surface layer. Bound. Layer Meteorol. 2009, 132, 193–204. [Google Scholar] [CrossRef]
- Hicks, B.B.; Pendergrass, W.R.; Vogel, C.A.; Keener, R.N.; Leyton, S.M. On the micrometeorology of the southern great plains 1: Legacy relationships revisited. Bound. Layer Meteorol. 2014, 151, 389–405. [Google Scholar] [CrossRef]
- Klausner, Z.; Fattal, E. An objective and automatic method for identification of pattern changes in wind direction time series. Int. J. Climatol. 2011, 31, 783–790. [Google Scholar] [CrossRef]
- Cheng, H.; Castro, I.P. Near wall flow over urban-like roughness. Bound. Layer Meteorol. 2002, 104, 229–259. [Google Scholar] [CrossRef]
- Raupach, M.R.; Antonia, R.A.; Rajagopalan, S. Rough-Wall turbulent boundary layers. Appl. Mech. Rev. 1991, 44, 1–25. [Google Scholar] [CrossRef]
- Trini Castelli, S.; Falabino, S. Analysis of the parameterization for the wind-velocity fluctuation standard deviations in the surface layer in low-wind conditions. Meteorol. Atmos. Phys. 2013, 119, 91–107. [Google Scholar] [CrossRef]
- Hanna, S.R.; Britter, R.E. Wind Flow and Vapor Cloud Dispersion at Industrial and Urban Sites; Center for Chemical Process Safety/AIChE: New York, NY, USA, 2002; ISBN 0-8169-0863-X. [Google Scholar]
- Irwin, J.S. A theoretical variation of the wind profile power-law exponent as a function of surface roughness and stability. Atmos. Environ. 1979, 13, 191–194. [Google Scholar] [CrossRef]
- Goldreich, Y. The Climate of Israel: Observation, Research and Application; Springer: New York, NY, USA, 2003; ISBN 978-1-4613-5200-6. [Google Scholar]
- Berkovic, S.; Feliks, Y. The land breeze characteristics in Israel during the summer by the MM5 model. In Proceedings of the World Weather Research Program Symposium on Nowcasting and Very Short Range Forecasting, Toulouse, France, 5 September 2005; p. 1.05. [Google Scholar]
- Deardorff, J.W. Convective velocity and temperature scales for the unstable planetary boundary layer and for rayleigh convection. J. Atmos. Sci. 1970, 27, 1211–1213. [Google Scholar] [CrossRef]
- Kader, B.A.; Yaglom, A.M. Mean fields and fluctuation moments in unstably stratified turbulent boundary layers. J. Fluid Mech. 1990, 212, 637. [Google Scholar] [CrossRef]
- Al-Jiboori, M.H.; Yumao, X.; Yongfu, Q. Turbulence characteristics over complex terrain in West China. Bound. Layer Meteorol. 2001, 101, 109–126. [Google Scholar] [CrossRef]
- Figueroa-Espinoza, B.; Salles, P. Local monin-obukhov similarity in heterogeneous terrain. Atmos. Sci. Lett. 2014, 15, 299–306. [Google Scholar] [CrossRef]
- Roth, M. Review of atmospheric turbulence over cities. Q. J. R. Meteorol. Soc. 2000, 126, 941–990. [Google Scholar] [CrossRef]
- Panofsky, H.A.; Dutton, J.A. Atmospheric Turbulence: Models and Methods for Engineering Applications, 1st ed.; Wiley-Interscience: New York, NY, USA, 1984; ISBN 0471057142. [Google Scholar]
- de Franceschi, M.; Zardi, D.; Tagliazucca, M.; Tampieri, F. Analysis of second-order moments in surface layer turbulence in an Alpine valley. Q. J. R. Meteorol. Soc. 2009, 135, 1750–1765. [Google Scholar] [CrossRef]
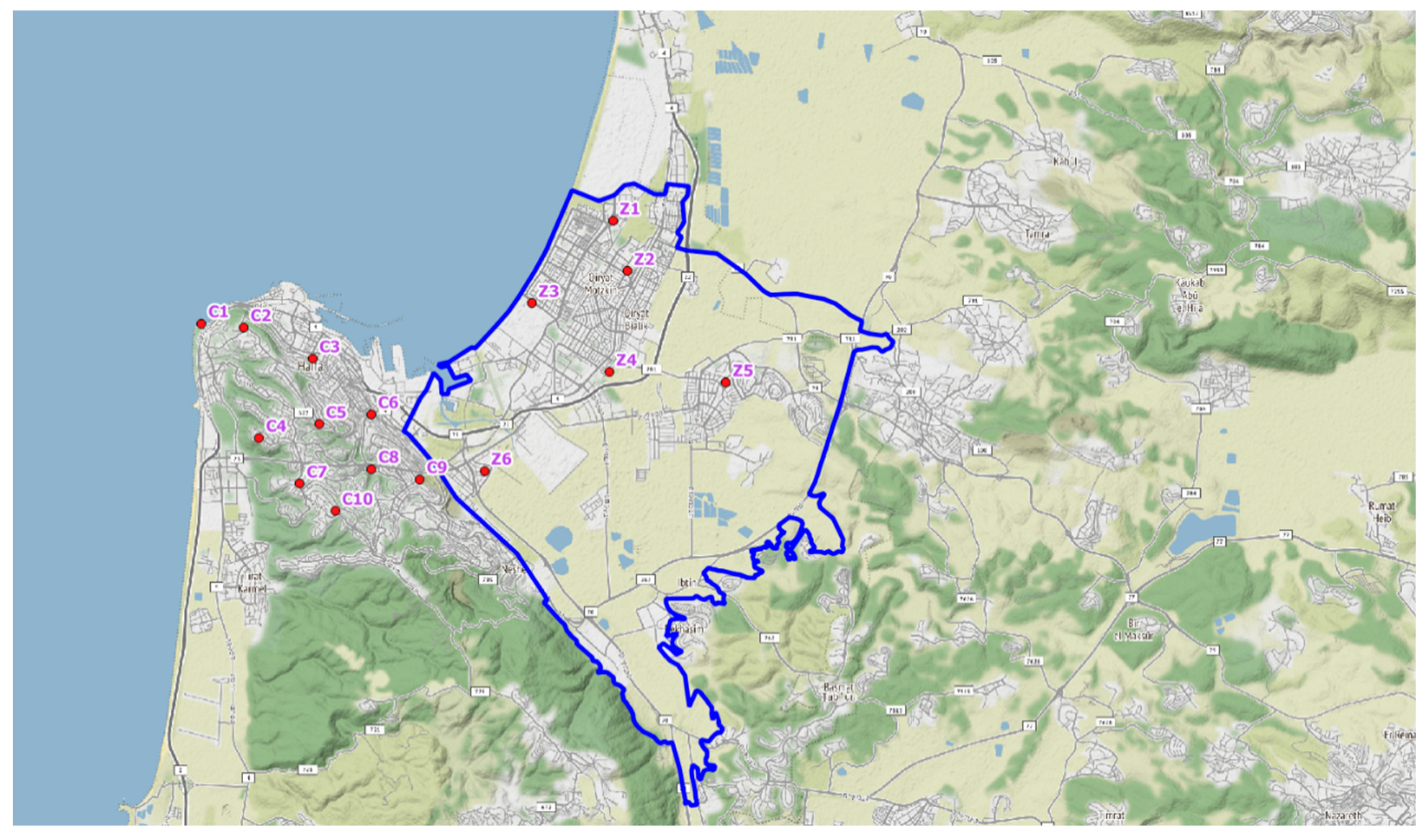
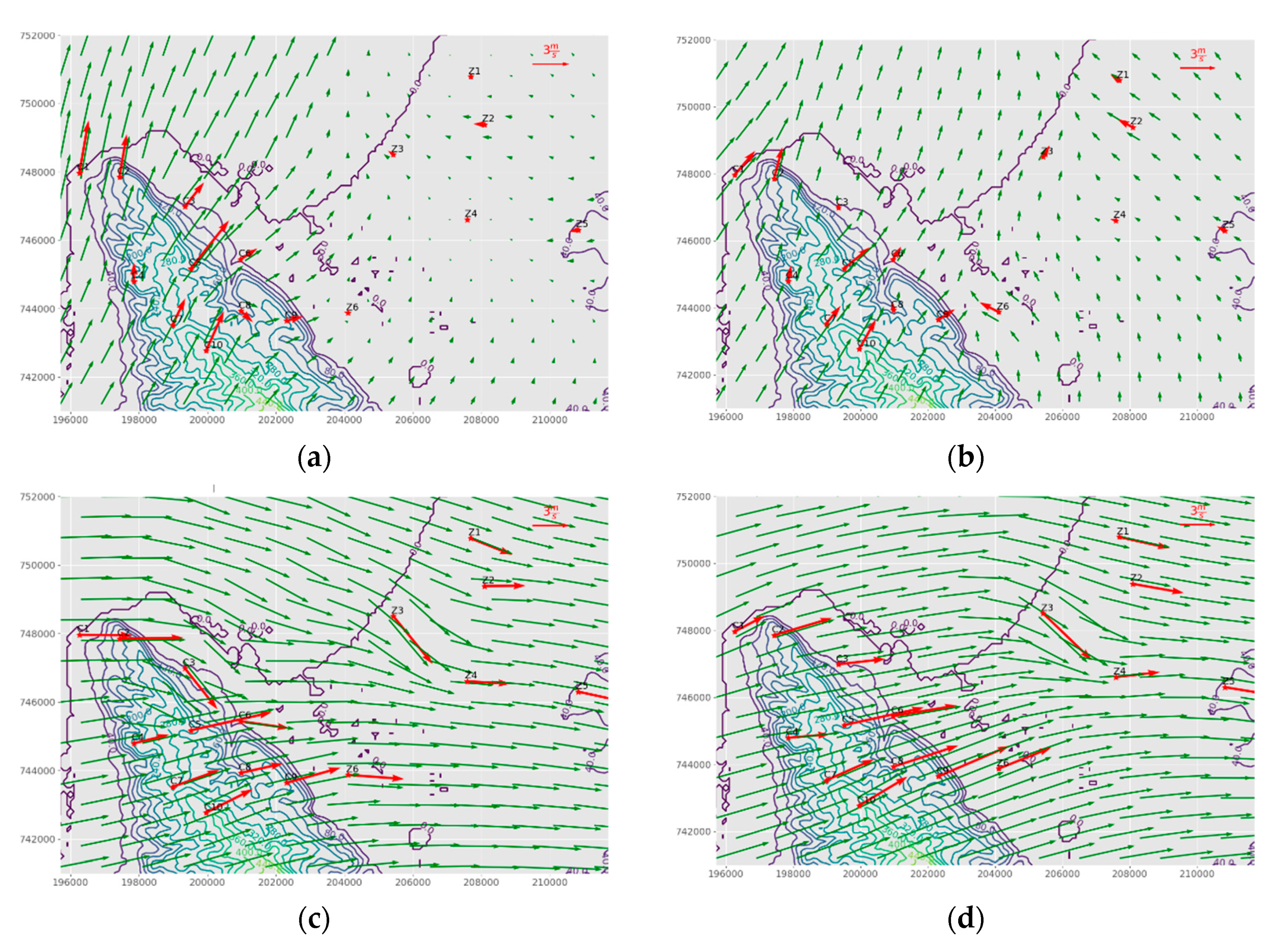
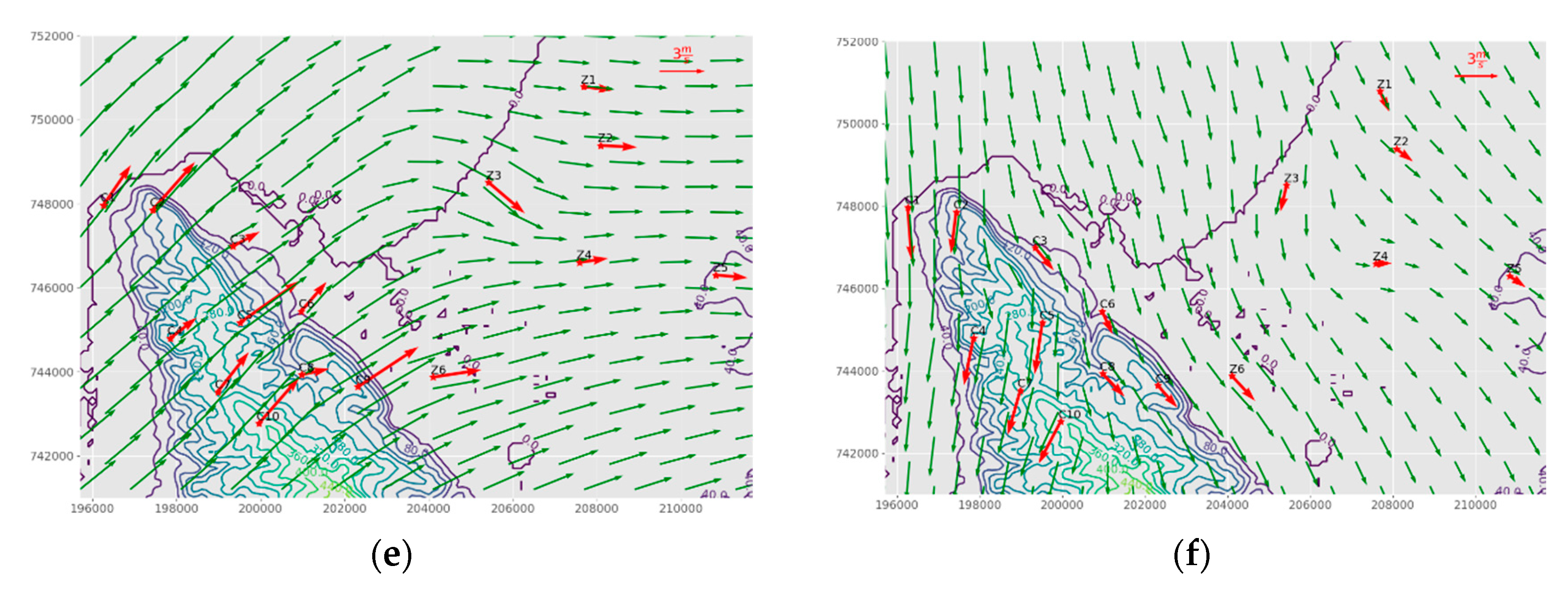
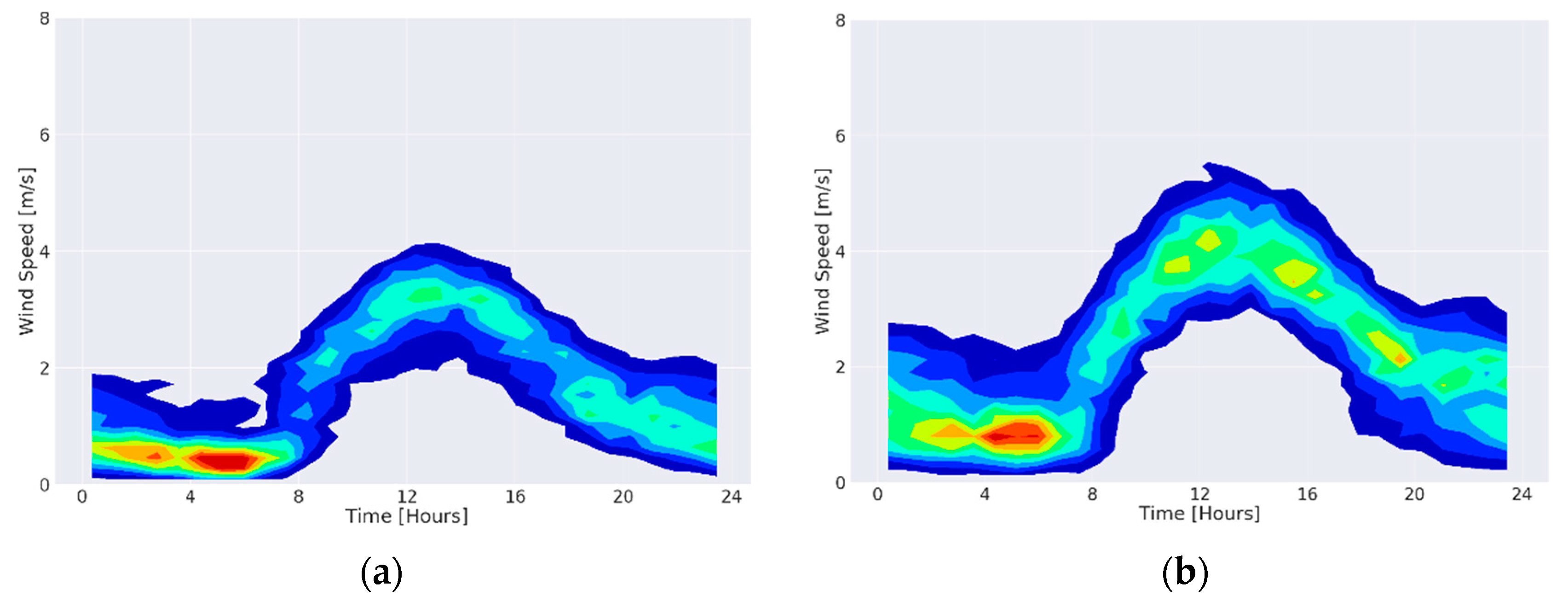

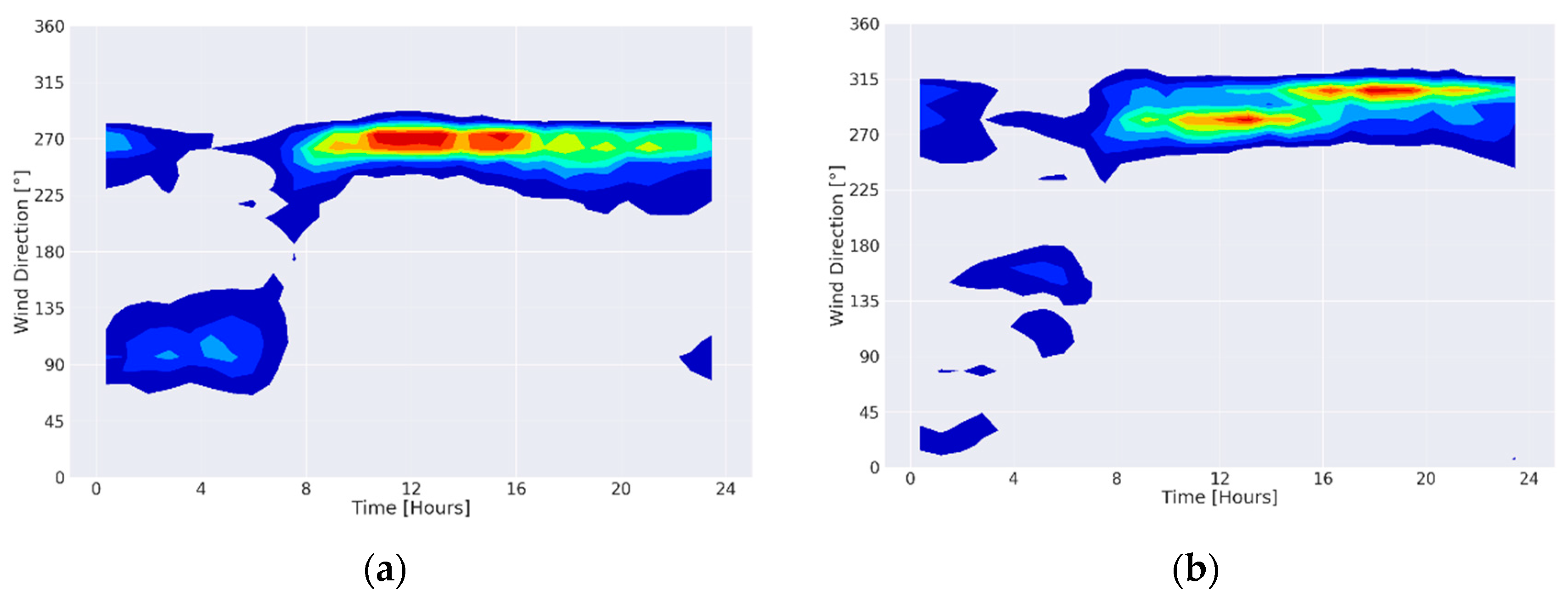
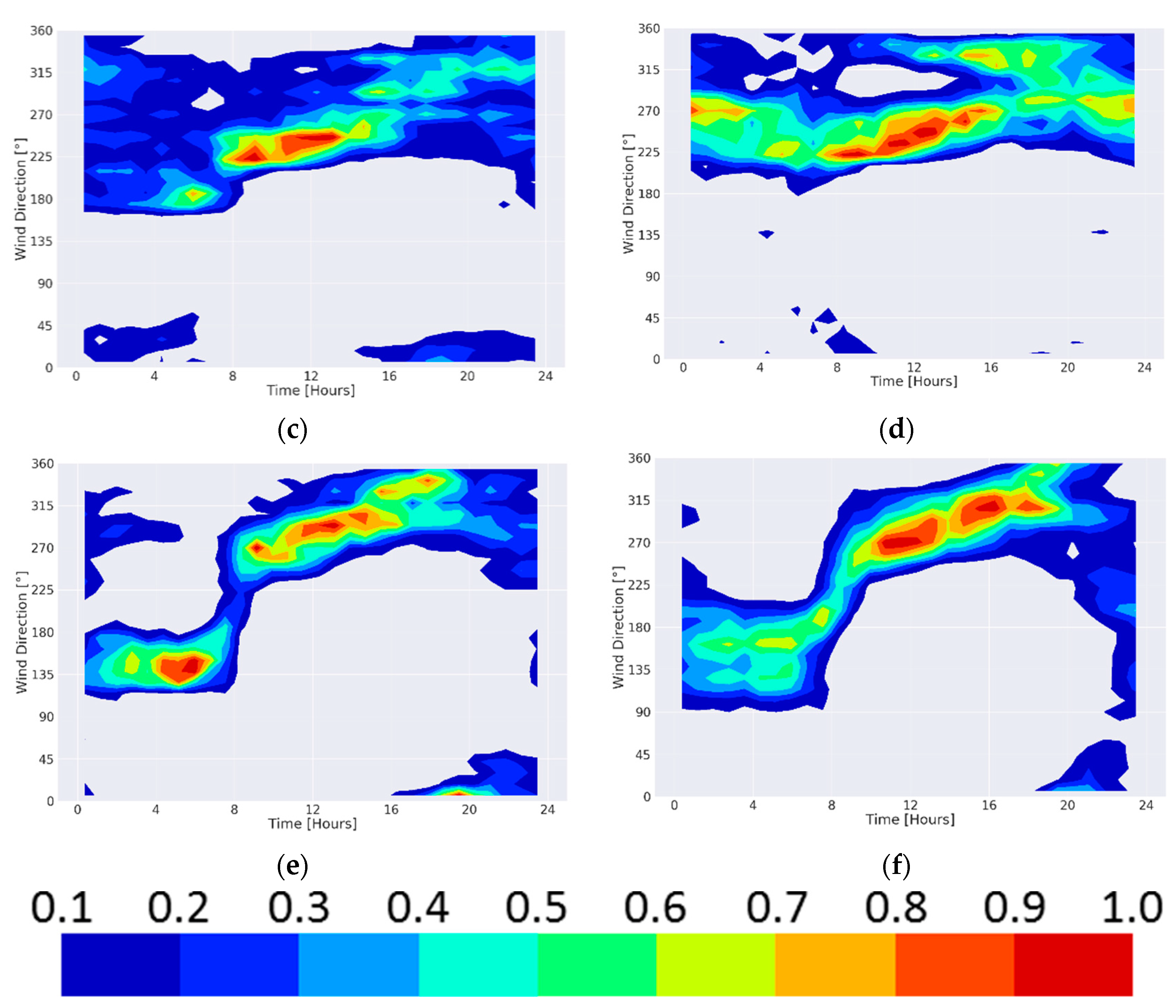
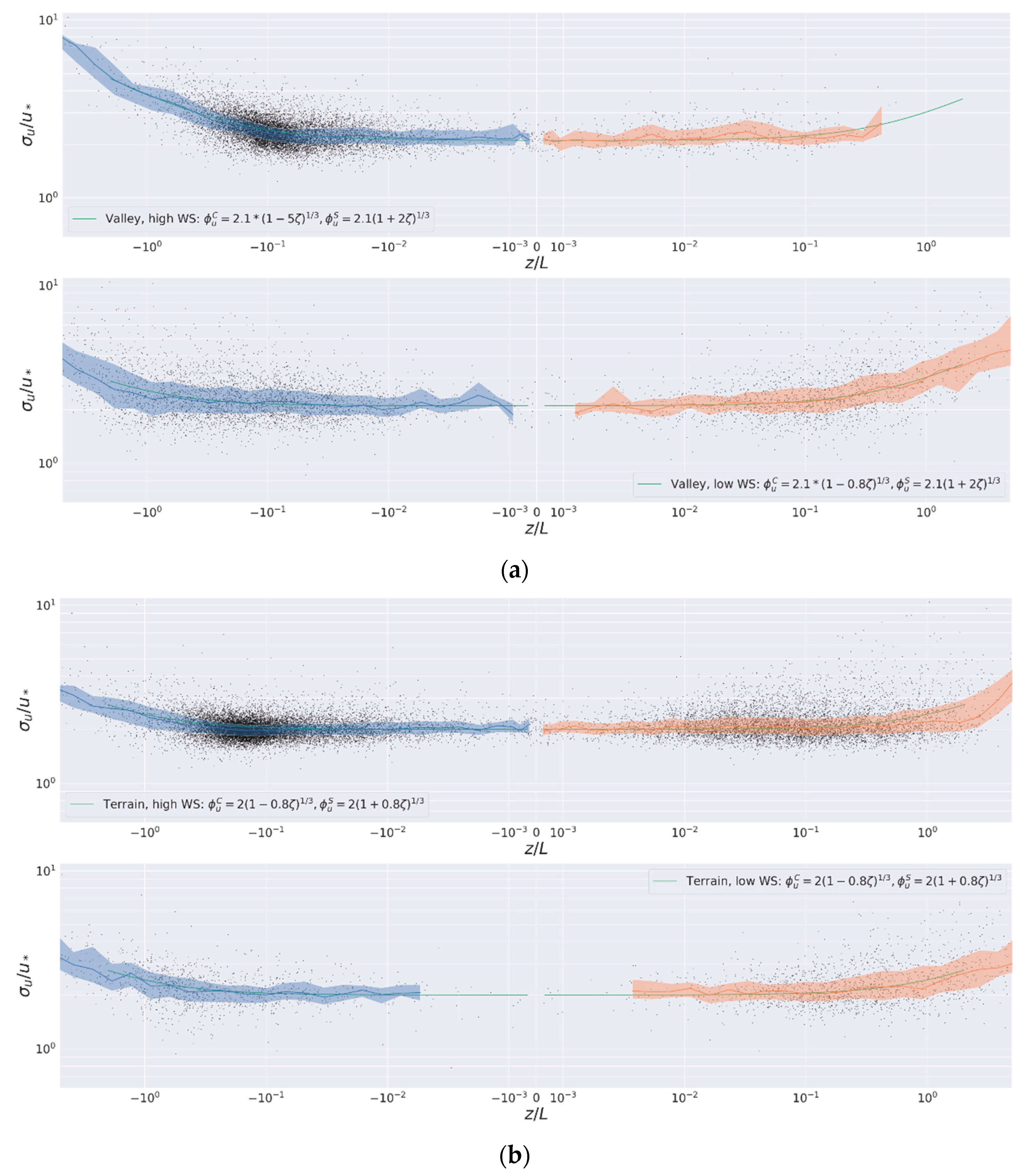
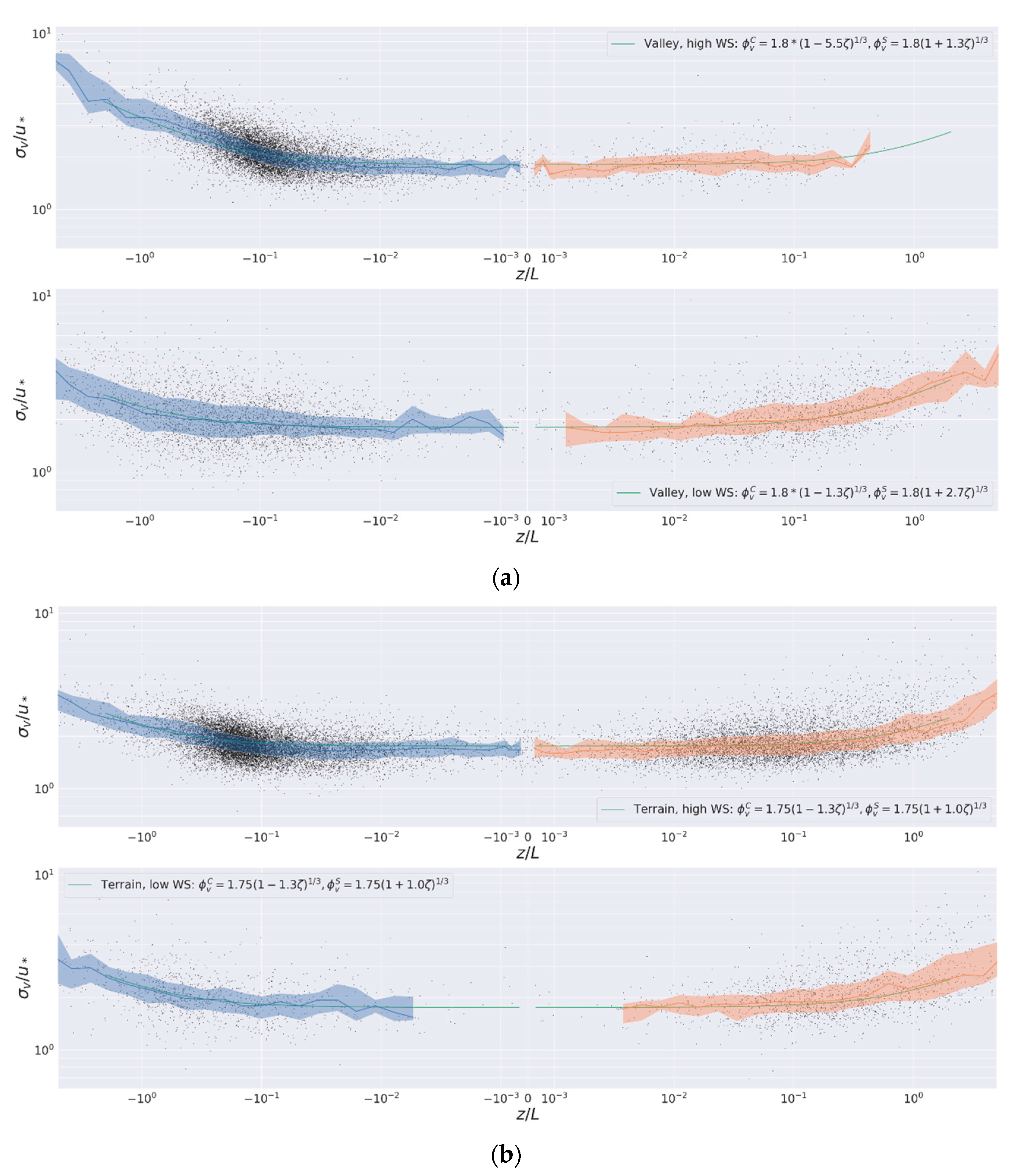
| Station Code | Location | Wind Sensor | Measurement Height AGL [m] | Location Elevation ASL [m] | Owner |
|---|---|---|---|---|---|
| C1 | 32°49′34″ N 34°57′24″ E | Ultrasonic anemometer | 21 | 0 | IIBR |
| C2 | 32°49′31″ N 34°58′9″ E | Ultrasonic anemometer | 30 | 145 | IIBR |
| C3 | 32°49′3″ N 34°59′23″ E | Ultrasonic anemometer | 19 | 270 | IIBR |
| C4 | 32°47′52″ N 34°58′26″ E | Ultrasonic anemometer | 22 | 240 | IIBR |
| C5 | 32°48′4″ N 34°59′30″ E | Ultrasonic anemometer | 28 | 220 | IIBR |
| C6 | 32°48′13″ N 35°0′26″ E | Ultrasonic anemometer | 25 | 19 | IIBR |
| C7 | 32°47′11″ N 34°59′9″ E | Ultrasonic anemometer | 26 | 285 | IIBR |
| C8 | 32°47′24″ N 35°0′26″ E | Ultrasonic anemometer | 19 | 175 | IIBR |
| C9 | 32°47′15″ N 35°1′17” E | Ultrasonic anemometer | 28 | 200 | IIBR |
| C10 | 32°46′46” N 34°59′47″ E | Ultrasonic anemometer | 19 | 350 | IIBR |
| Z1 | 32°51′8″ N 35°4′46″ E | Propeller-vane anemometer | 12 | 27 | HBUA |
| Z2 | 32°50′21″ N 35°4′59″ E | Ultrasonic anemometer | 19 | 10 | IIBR |
| Z3 | 32°49′51° N 35°3′15″ E | Propeller-vane anemometer | 6 | 7 | HBUA |
| Z4 | 32°48′52″ N 35°4′42″ E | Propeller-vane anemometer | 10 | 4 | HBUA |
| Z5 | 32°48′43″ N 35°6′47″ E | Propeller-vane anemometer | 20 | 65 | HBUA |
| Z6 | 32°47′22″ N 35°2′26″ E | Ultrasonic anemometer | 19 | 5 | IIBR |
| Station | Standard Deviation | ||||||
|---|---|---|---|---|---|---|---|
| Convective/Stable | Convective | Stable | |||||
| 2.1 | 2.1 | 5 | 0.8 | 2 | 2 | ||
| Z2 | 1.8 | 1.8 | 5.5 | 1.3 | 1.3 | 2.7 | |
| 1.25 | 1.25 | 4 | 1 | 3.5 | 1.7 | ||
| 2 | 2 | 0.8 | 0.8 | 0.8 | 0.8 | ||
| C9 | 1.75 | 1.75 | 1.3 | 1.3 | 1 | 1 | |
| 1.15 | 1.15 | 1.6 | 0.6 | 1 | 0.6 | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Klausner, Z.; Ben-Efraim, M.; Arav, Y.; Tas, E.; Fattal, E. The Micrometeorology of the Haifa Bay Area and Mount Carmel during the Summer. Atmosphere 2021, 12, 354. https://doi.org/10.3390/atmos12030354
Klausner Z, Ben-Efraim M, Arav Y, Tas E, Fattal E. The Micrometeorology of the Haifa Bay Area and Mount Carmel during the Summer. Atmosphere. 2021; 12(3):354. https://doi.org/10.3390/atmos12030354
Chicago/Turabian StyleKlausner, Ziv, Mattya Ben-Efraim, Yehuda Arav, Eran Tas, and Eyal Fattal. 2021. "The Micrometeorology of the Haifa Bay Area and Mount Carmel during the Summer" Atmosphere 12, no. 3: 354. https://doi.org/10.3390/atmos12030354
APA StyleKlausner, Z., Ben-Efraim, M., Arav, Y., Tas, E., & Fattal, E. (2021). The Micrometeorology of the Haifa Bay Area and Mount Carmel during the Summer. Atmosphere, 12(3), 354. https://doi.org/10.3390/atmos12030354






