Diurnal Dynamics of the Umov Kinetic Energy Density Vector in the Atmospheric Boundary Layer from Minisodar Measurements
Abstract
:1. Introduction
2. Theoretical Background
3. Applied Approach
4. Results and Discussion
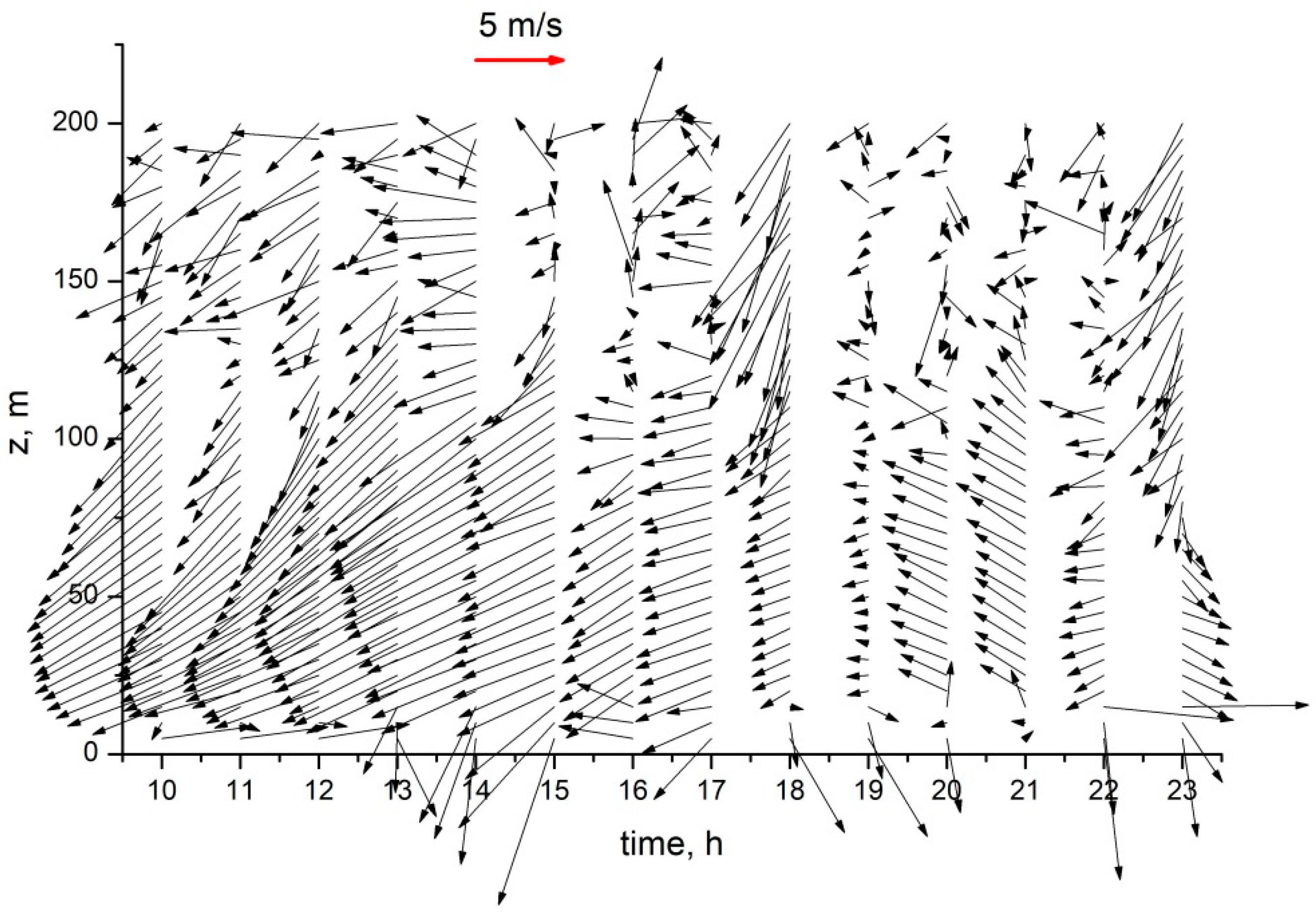
4.1. Diurnal Hourly Dynamics of the Wind Velocity Vector
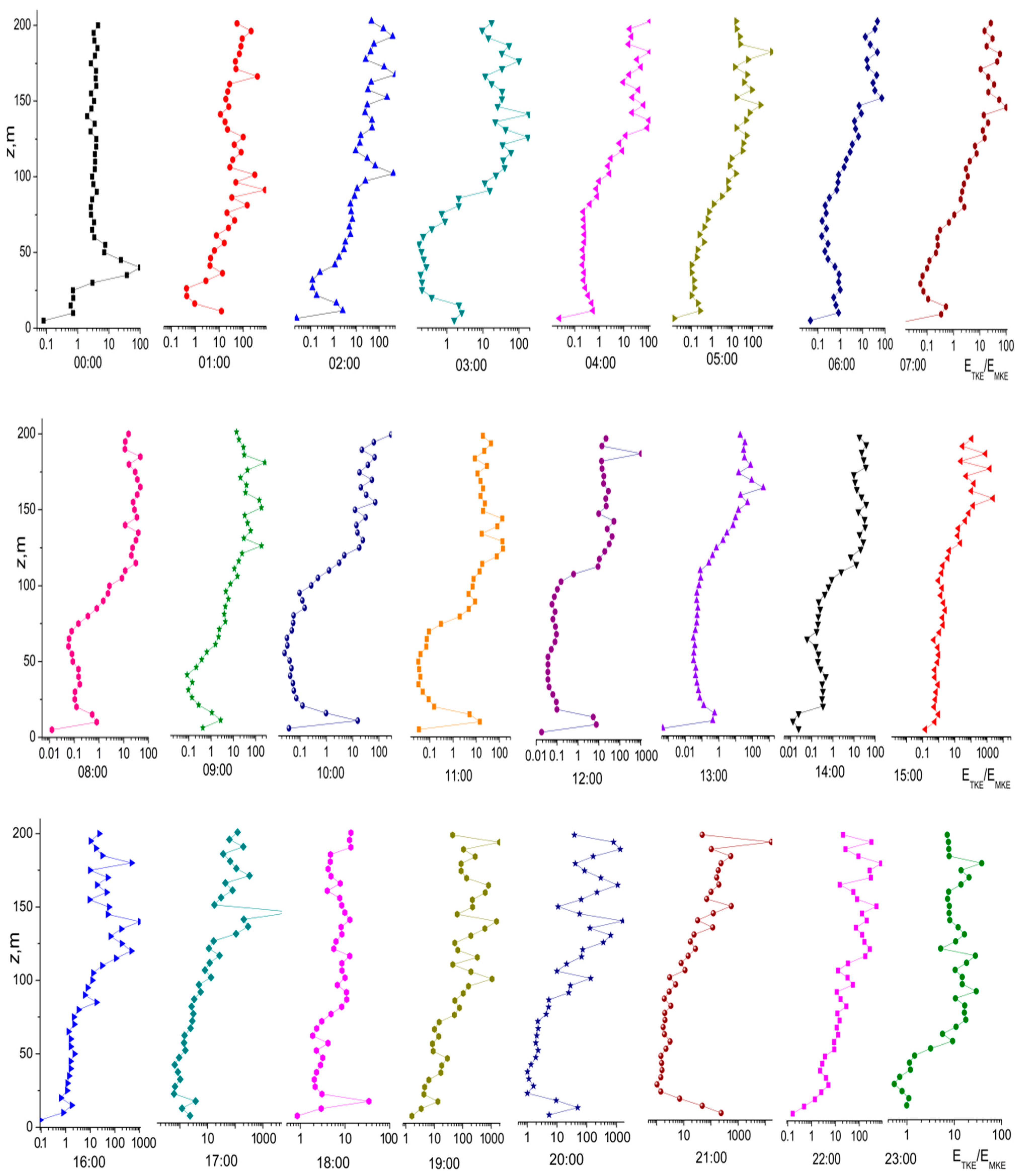
4.2. Diurnal Hourly Dynamics of Relative Contributions of the Mean and Turbulent Kinetic Energy Components
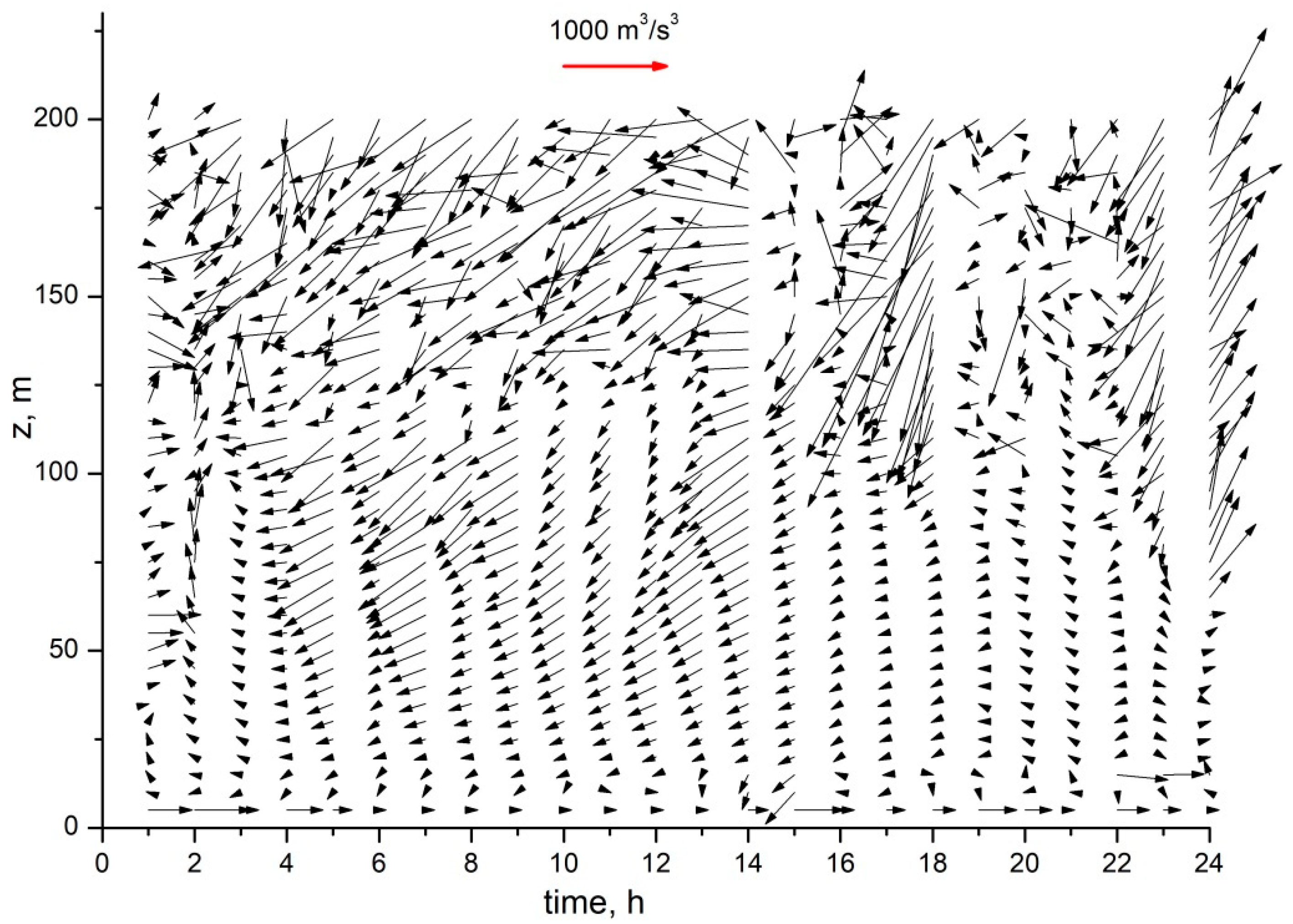
4.3. Diurnal Hourly Dynamics of the Total Kinetic Flux Energy Density Vector (the Umov Vector)
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Umov, N.A. The Equation of Motion for the Energy of a Body. Doctoral Thesis, Odessa University, Odessa, Ukraine, 1874. [Google Scholar]
- Fangwei, H.; Mohammad, J. Investigation approaches to quantify wind-induced load and response of tall buildings: A review. Sustain. Cities Soc. 2020, 62, 102376. [Google Scholar]
- Wang, B.H.; Wang, D.B.; Ali, Z.A.; Bai, T.T.; Wang, H. An overview of various kinds of wind effects on unmanned aerial vehicle. Meas. Control 2019, 52, 731–739. [Google Scholar] [CrossRef]
- Peña, A.; Hasager, C.B.; Badger, M.; Barthelmie, R.J.; Bingöl, F.; Cariou, J.-P.; Emeis, S.; Frandsen, S.T.; Harris, M.; Karagali, I.; et al. Remote Sensing for Wind Energy; DTU Wind Energy: Roskilde, Denmark, 2015; p. 260. [Google Scholar]
- Monzikova, A.K.; Kudryavtsev, V.N.; Larsen, E.S.; Chapron, B. Estimation of wind power potential of the Gulf of Finland. Proc. Russ. State Hydrometeorol. Univ. 2013, 30, 116–133. [Google Scholar]
- Banakh, V.A.; Smalikho, I.N. Coherent Doppler Wind Lidar; Publishing House of the Institute of Atmospheric Optics of the Siberian Branch of the Russian Academy of Sciences: Tomsk, Russia, 2013; p. 304. [Google Scholar]
- Bolbasova, L.A.; Shikhovtsev, A.Y.; Kopylov, E.A.; Selin, A.A.; Lukin, V.P.; Kovadlo, P.G. Daytime optical turbulence and wind speed distributions at the Baikal Astrophysical Observatory. Mon. Not. R. Astron. Soc. 2019, 482, 2619–2626. [Google Scholar] [CrossRef]
- Valldecabres, L.; Nygaard, N.G.; Vera-Tudela, L.; von Bremen, L.; Kühn, M. On the use of dual Doppler radar measurements for very short-term wind power forecasts. Remote Sens. 2018, 10, 1701. [Google Scholar] [CrossRef] [Green Version]
- Haggagy, M.A. A Sodar-Based Investigation of the Atmospheric Boundary Layer; Berichte des Meteorologischen Institutes des Universität Freiburg: Freiburg, Germay, 2003; p. 235. [Google Scholar]
- Potekaev, A.I.; Shamanaeva, L.G.; Kulagina, V.V. Spatiotemporal dynamics of the kinetic energy in the atmospheric boundary layer from minisodar measurements. Atmosphere 2021, 12, 421. [Google Scholar] [CrossRef]
- Shamanaeva, L.G.; Potekaev, A.I.; Krasnenko, N.P.; Kapegesheva, O.F. Dynamics of the kinetic energy in the atmospheric boundary layer from the results of minisodar measurements. Russ. Phys. J. 2018, 61, 2282–2287. [Google Scholar] [CrossRef]
- Tarasenkov, M.V.; Krasnenko, N.P.; Shamanaeva, L.G. Program for Constructing the Altitude-Temporal Distribution of Wind Velocity Components in the Lower Atmosphere from the Data of Acoustic Sounding; RF Certificate of State Registration of Computer Program, No. 2016619428; Rospatent: Moscow, Russia, 2016.
- Krasnenko, N.; Simakhin, V.; Shamanaeva, L.; Cherepanov, O. Robust nonparametric methods of statistical analysis of wind velocity components in acoustic sounding of the lower layer of the atmosphere. Symmetry 2019, 11, 961. [Google Scholar] [CrossRef] [Green Version]
- Doppler MiniSoDAR System: Operation and Maintenance Manual. Available online: https://home.chpc.utah.edu/~{}u0035056/5910_2010/Minisodarmanual.pdf (accessed on 30 December 2020).
- Yang, X. Atmospheric Acoustics; Walter de Gruyter GmbH: Berlin, Germany, 2016; p. 392. [Google Scholar]
- Underwood, K.H.; Shamanaeva, L.G. Turbulence characteristics from minisodar data. Russ. Phys. J. 2010, 53, 526–532. [Google Scholar] [CrossRef]
- Potekaev, A.I.; Shamanaeva, L.G.; Kulagina, V.V. Dynamics of the kinetic energy in the atmospheric boundary layer retrieved from the data of minisodar measurements. In Proceedings of the SPIE, 27th International Symposium on Atmospheric and Ocean Optics: Atmospheric Physics (in press), Moscow, Russia, 5–9 July 2021; p. 5. [Google Scholar]
- Sklyarov, Y.A. Development of Methods for Climatologic Estimation of the Wind Power Potential at Different Altitudes (on the Example of Southeastern Territory of Russia); Publishing House of Saratov State University: Saratov, Russia, 2012; p. 132. [Google Scholar]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Potekaev, A.; Krasnenko, N.; Shamanaeva, L. Diurnal Dynamics of the Umov Kinetic Energy Density Vector in the Atmospheric Boundary Layer from Minisodar Measurements. Atmosphere 2021, 12, 1347. https://doi.org/10.3390/atmos12101347
Potekaev A, Krasnenko N, Shamanaeva L. Diurnal Dynamics of the Umov Kinetic Energy Density Vector in the Atmospheric Boundary Layer from Minisodar Measurements. Atmosphere. 2021; 12(10):1347. https://doi.org/10.3390/atmos12101347
Chicago/Turabian StylePotekaev, Alexander, Nikolay Krasnenko, and Liudmila Shamanaeva. 2021. "Diurnal Dynamics of the Umov Kinetic Energy Density Vector in the Atmospheric Boundary Layer from Minisodar Measurements" Atmosphere 12, no. 10: 1347. https://doi.org/10.3390/atmos12101347
APA StylePotekaev, A., Krasnenko, N., & Shamanaeva, L. (2021). Diurnal Dynamics of the Umov Kinetic Energy Density Vector in the Atmospheric Boundary Layer from Minisodar Measurements. Atmosphere, 12(10), 1347. https://doi.org/10.3390/atmos12101347






