Volcanic Ash Resuspension in Patagonia: Numerical Simulations and Observations
Abstract
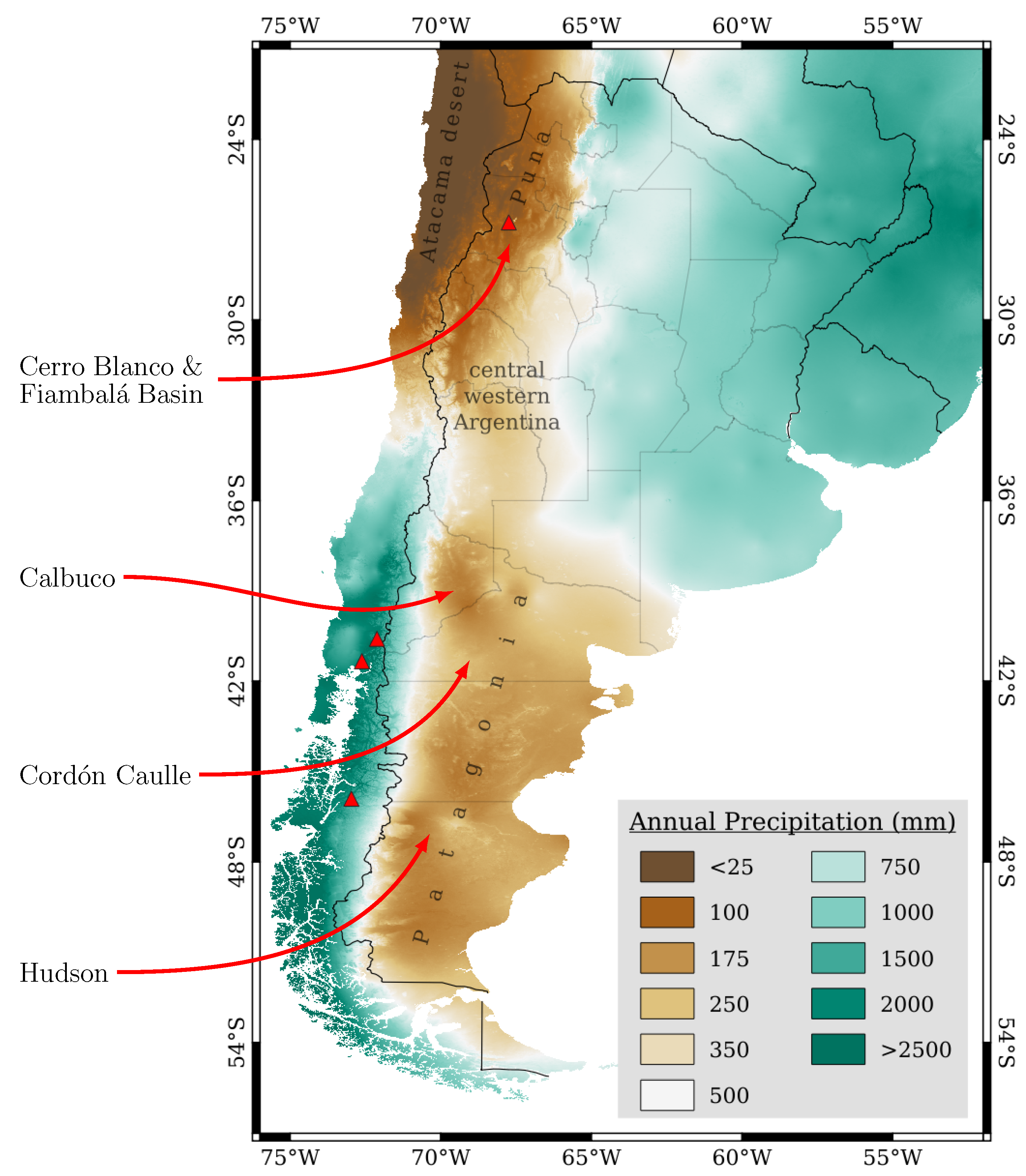
1. Introduction
2. Background
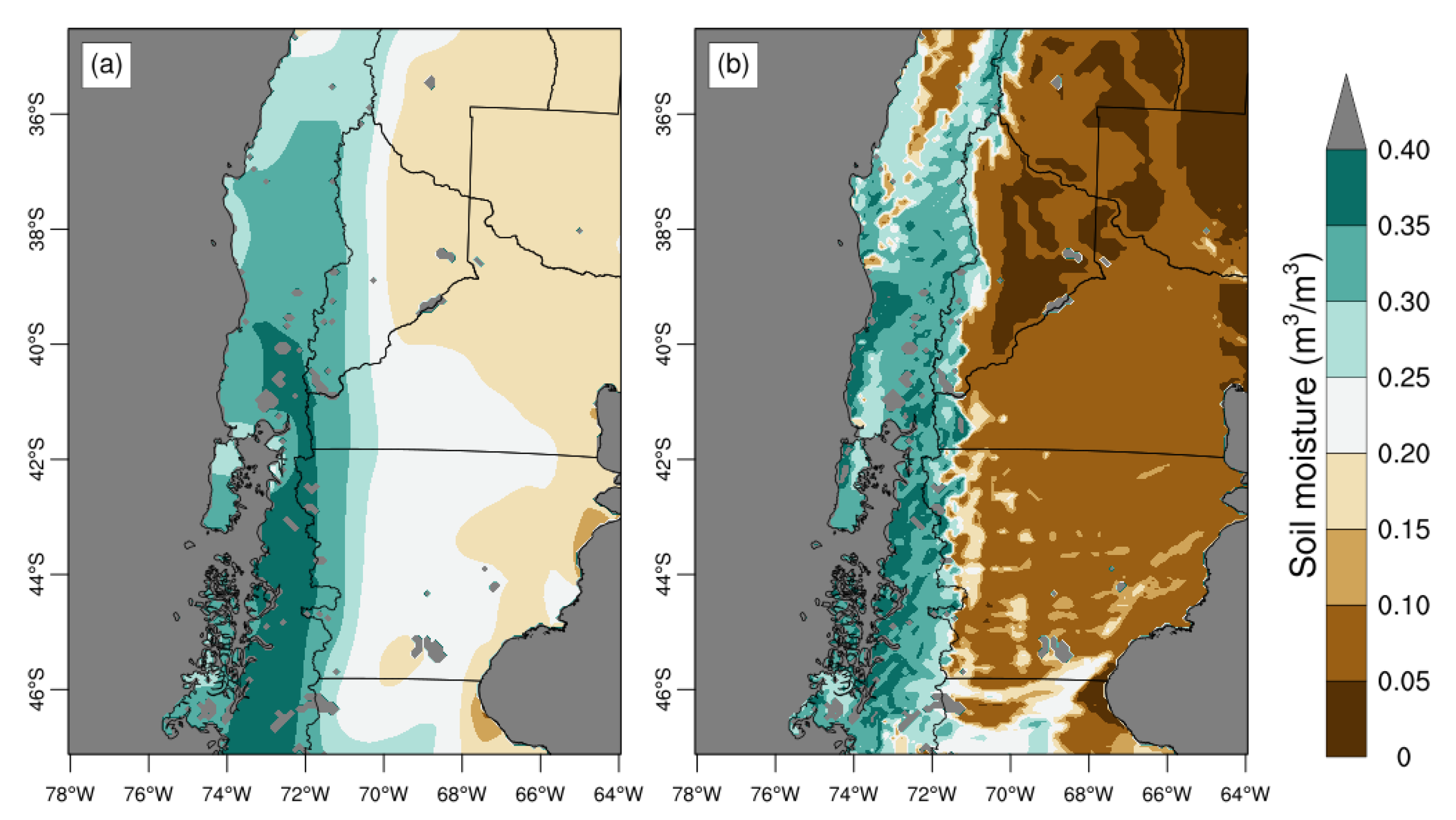
2.1. Primary Tephra-Fallout Deposit
3. Materials and Methods
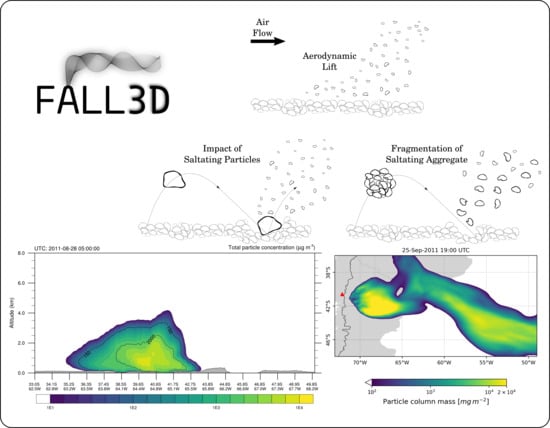
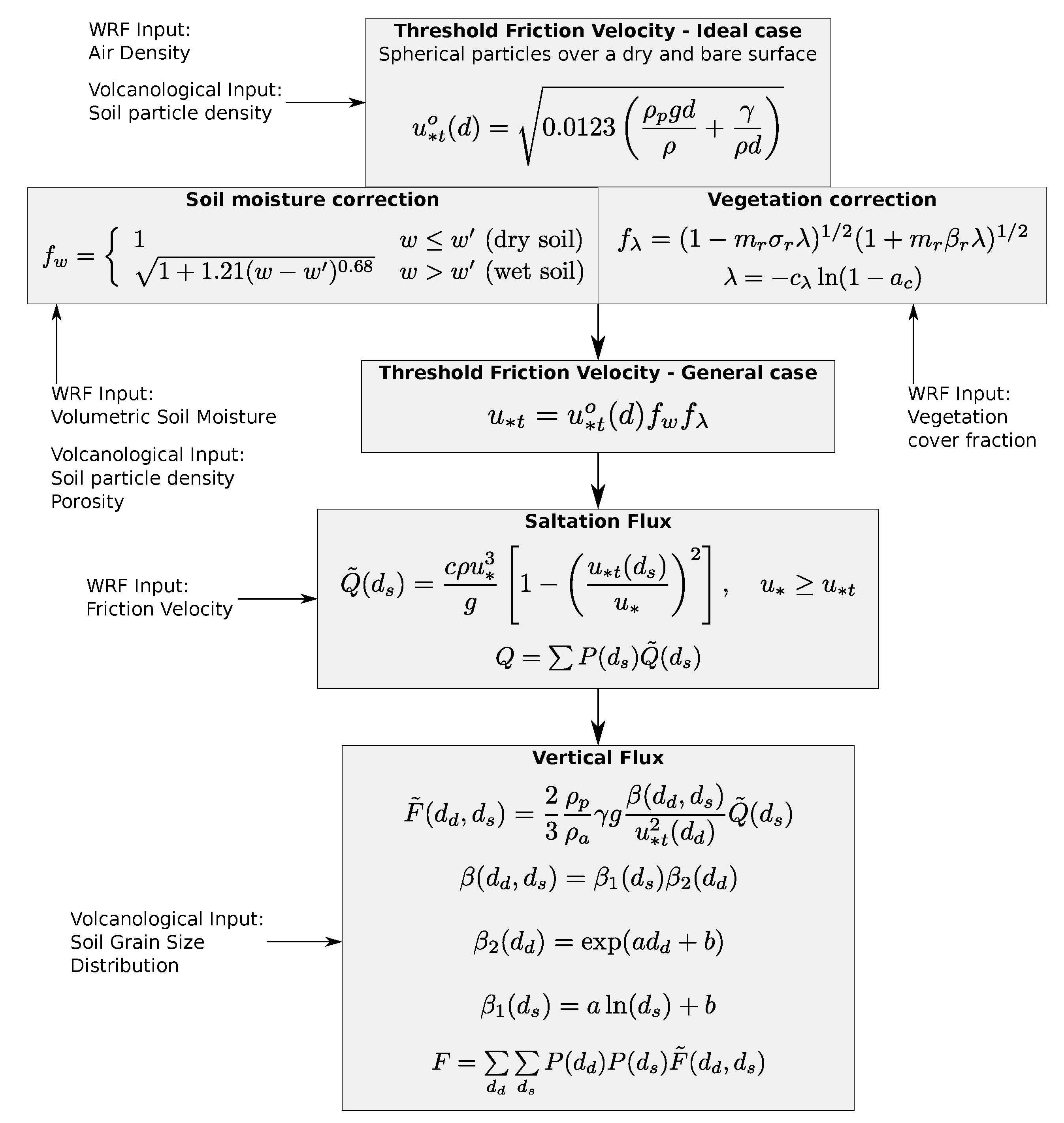
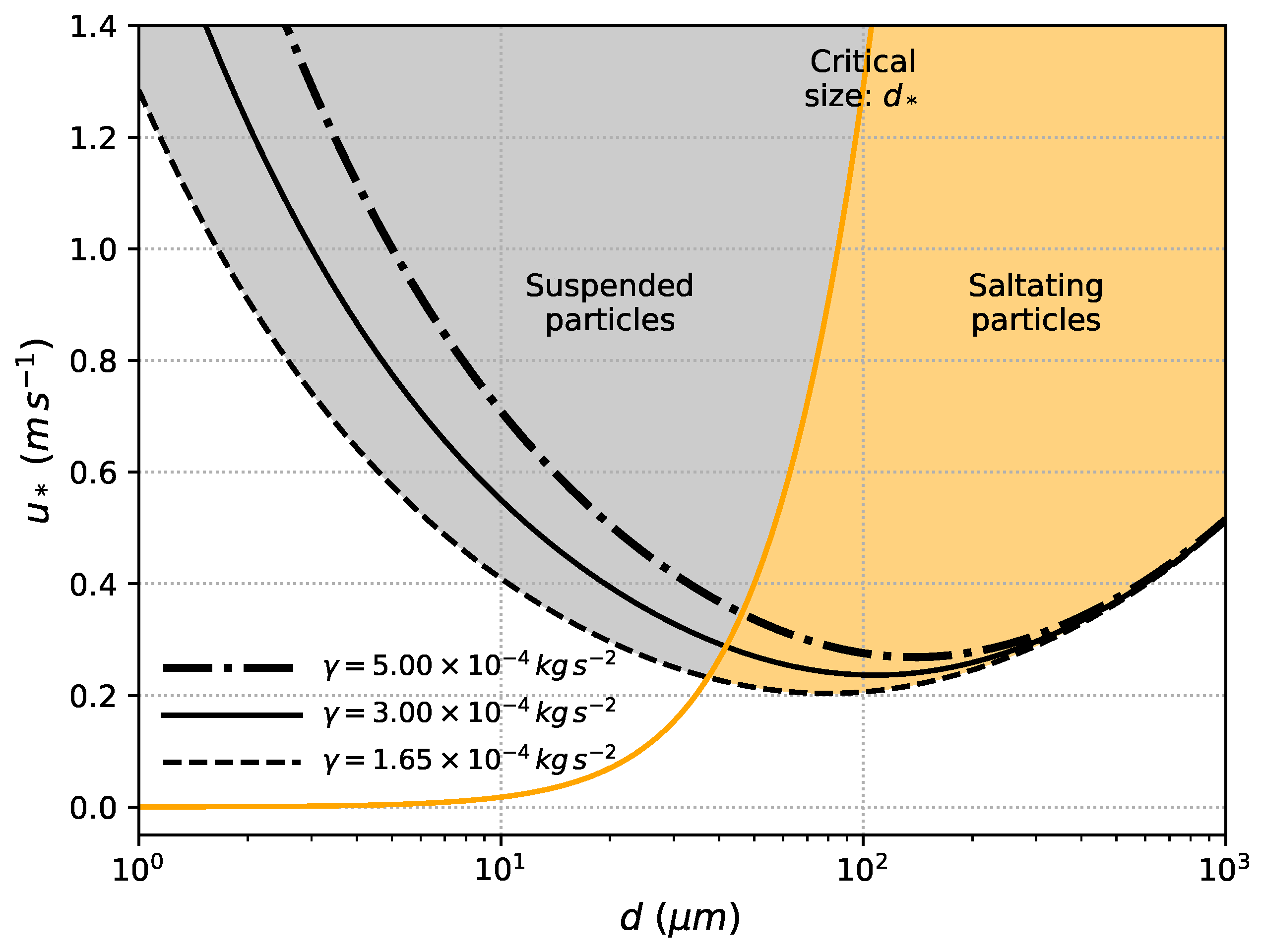
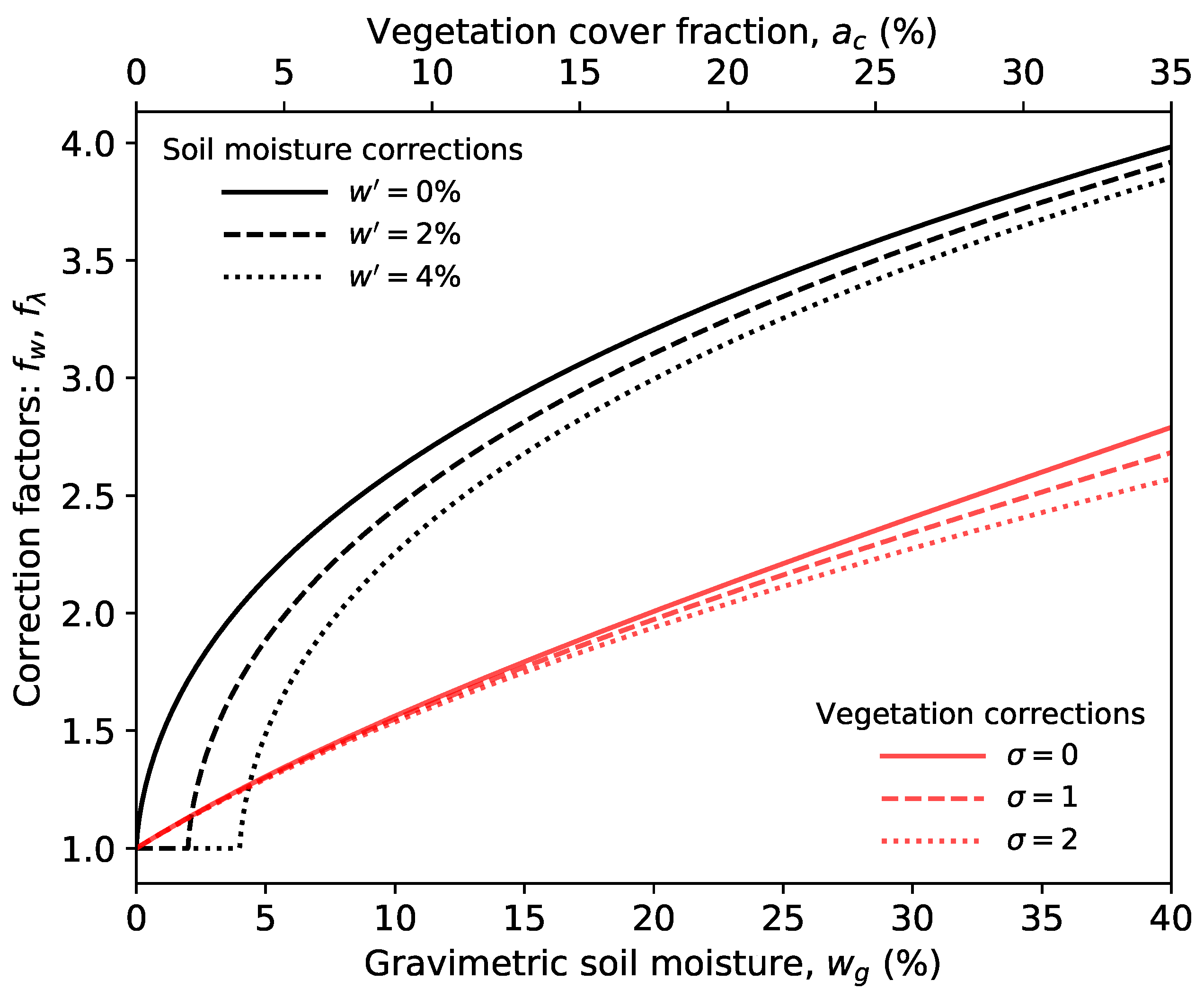
3.1. Volcanic Ash Resuspension Module
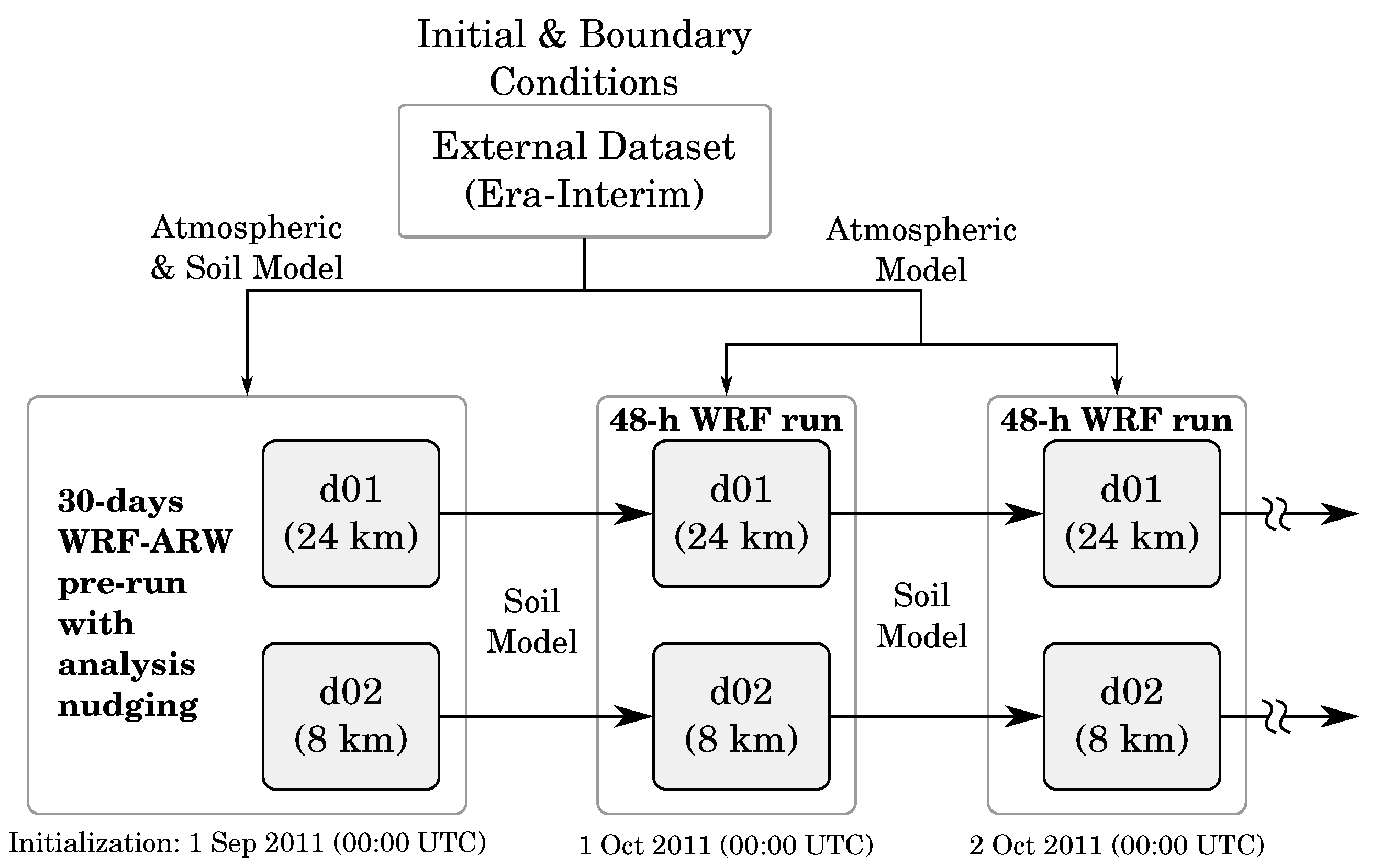
3.2. WRF-ARW Simulations
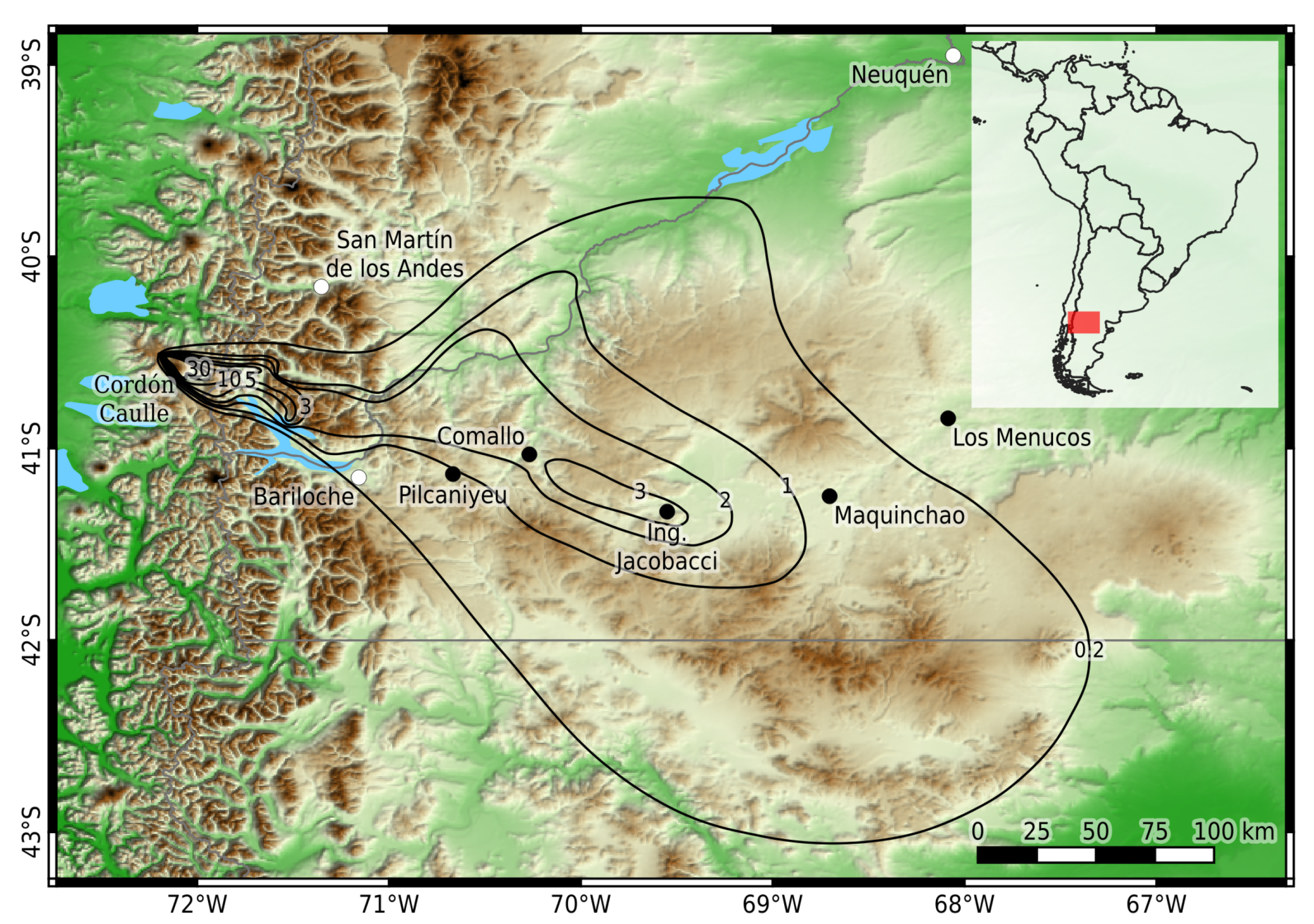
4. Results: The Example of the 2011 Cordón Caulle Eruption
4.1. Seasonal and Diurnal Variability
4.2. Timeseries for October 2011
4.3. FALL3D Ash Dispersal Simulations
4.3.1. Emission Scheme
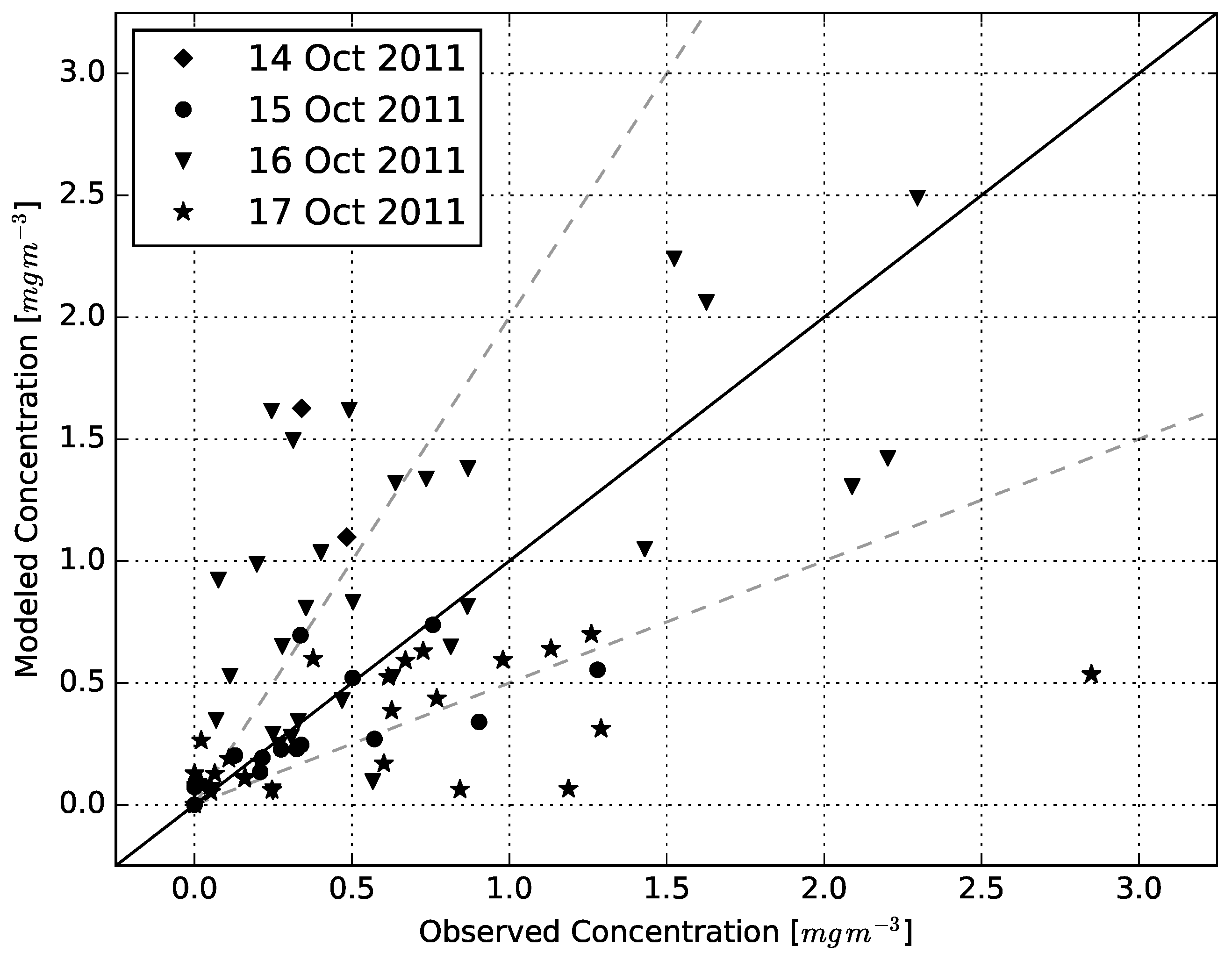
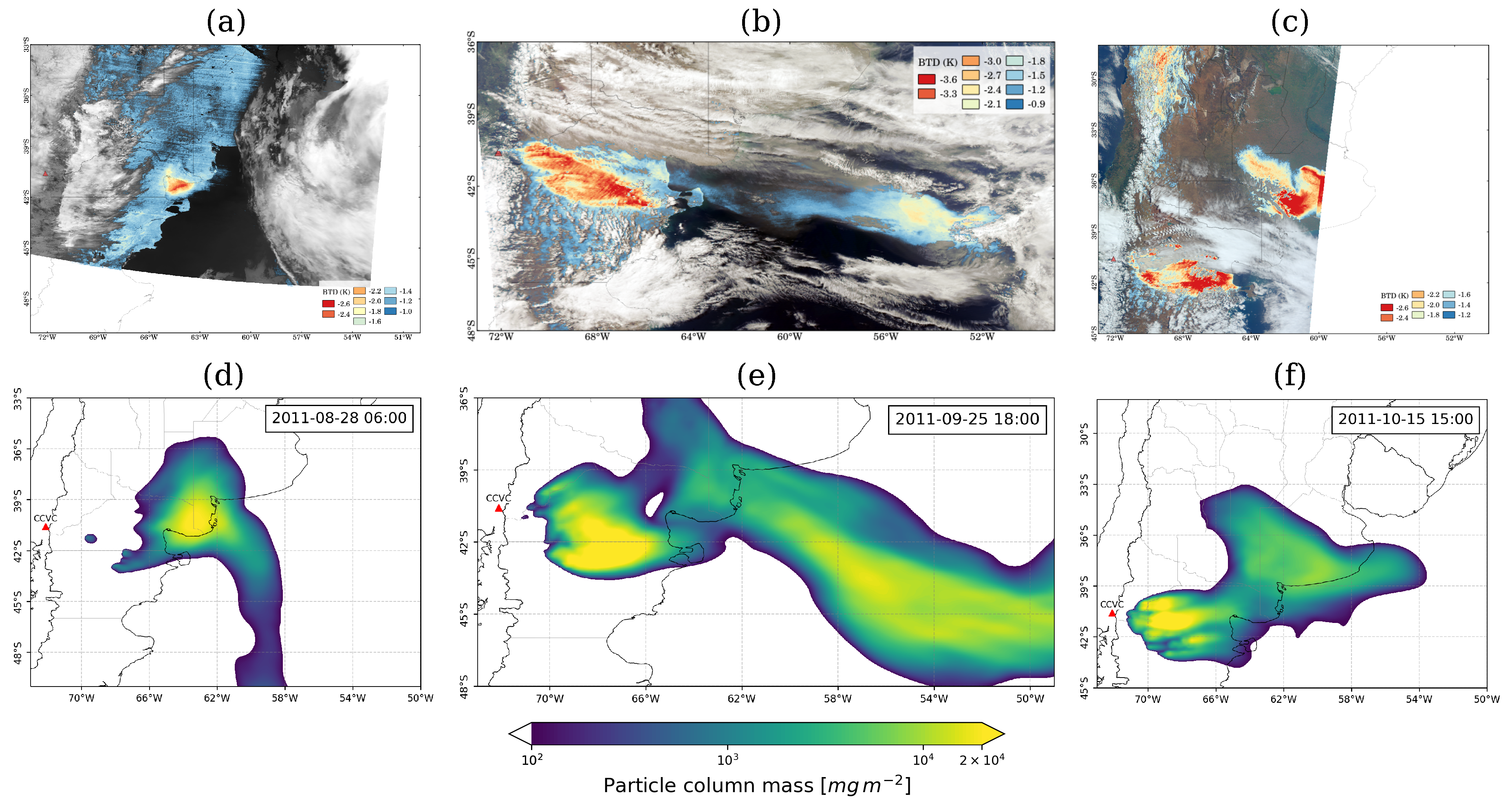
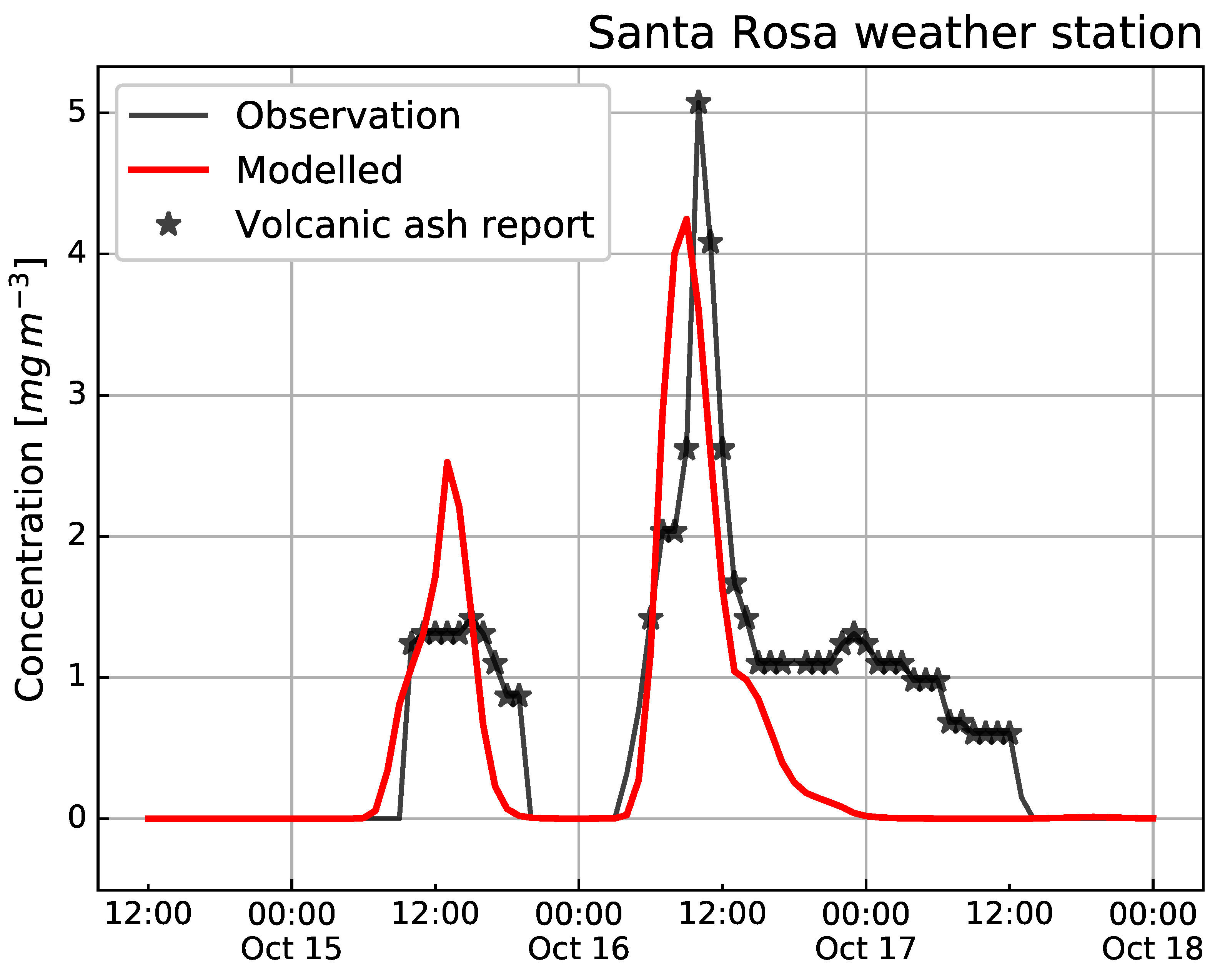
4.3.2. FALL3D Results And Validation
5. Discussion
5.1. Emission Scheme
5.2. Operational Implementation
5.3. Spatial Distribution of Sources
5.4. Diurnal & Seasonal Variability
5.5. Spatio-Temporal Distribution of Airborne Ash
6. Conclusions
- A better integration of the data on primary tephra-fallout deposit (i.e., field-based GSD data from both proximal and distal samples and particle density).
- A new strategy to obtain a more realistic description of the top soil moisture of significant relevance to the ash emission scheme.
- The inclusion of the effects of vegetation cover on wind erosion.
- A new approach to determine the grain size associated with resuspended particles. In this case, the maximum size for resuspended particles depends on atmospheric conditions (i.e., friction velocity) and particle properties (i.e., particle size and density), and no arbitrary restriction was imposed on the grain size of the emitted particles (e.g., even coarse ash could be resuspended under certain conditions).
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Collini, E.; Osores, M.S.; Folch, A.; Viramonte, J.G.; Villarosa, G.; Salmuni, G. Volcanic ash forecast during the June 2011 Cordón Caulle eruption. Nat. Hazards 2013, 66, 389–412. [Google Scholar] [CrossRef]
- Pistolesi, M.; Cioni, R.; Bonadonna, C.; Elissondo, M.; Baumann, V.; Bertagnini, A.; Chiari, L.; Gonzales, R.; Rosi, M.; Francalanci, L. Complex dynamics of small-moderate volcanic events: The example of the 2011 rhyolitic Cordón Caulle eruption, Chile. Bull. Volcanol. 2015, 77, 3. [Google Scholar] [CrossRef]
- Bonadonna, C.; Cioni, R.; Pistolesi, M.; Elissondo, M.; Baumann, V. Sedimentation of long-lasting wind-affected volcanic plumes: The example of the 2011 rhyolitic Cordón Caulle eruption, Chile. Bull. Volcanol. 2015, 77, 13. [Google Scholar] [CrossRef]
- Bonadonna, C.; Pistolesi, M.; Cioni, R.; Degruyter, W.; Elissondo, M.; Baumann, V. Dynamics of wind-affected volcanic plumes: The example of the 2011 Cordón Caulle eruption, Chile. J. Geophys. Res. Solid Earth 2015, 120, 2242–2261. [Google Scholar] [CrossRef]
- Wilson, T.M.; Stewart, C.; Bickerton, H.; Baxter, P.; Outes, V.; Villarosa, G.; Rovere, E. Impacts of the June 2011 Puyehue–Cordón Caulle Volcanic complex Eruption on Urban Infrastructure, Agriculture and Public Health; GNS Science Report 2012/20; Institute of Geology and Nuclear Sciences: Lower Hutt, New Zealand, 2013; p. 88. [Google Scholar]
- Elissondo, M.; Baumann, V.; Bonadonna, C.; Pistolesi, M.; Cioni, R.; Bertagnini, A.; Biasse, S.; Herrero, J.C.; Gonzalez, R. Chronology and impact of the 2011 Cordón Caulle eruption, Chile. Nat. Hazards Earth Syst. Sci. 2016, 16, 675–704. [Google Scholar] [CrossRef]
- Folch, A.; Mingari, L.; Osores, M.S.; Collini, E. Modeling volcanic ash resuspension—Application to the 14–18 October 2011 outbreak episode in central Patagonia, Argentina. Nat. Hazards Earth Syst. Sci. 2014, 14, 119–133. [Google Scholar] [CrossRef]
- Ulke, A.G.; Torres Brizuela, M.M.; Raga, G.B.; Baumgardner, D. Aerosol properties and meteorological conditions in the city of Buenos Aires, Argentina, during the resuspension of volcanic ash from the Puyehue-Cordón Caulle eruption. Nat. Hazards Earth Syst. Sci. 2016, 16, 2159–2175. [Google Scholar] [CrossRef]
- Shao, Y. Physics and Modelling of Wind Erosion, 2nd ed.; Springer Science & Business Media: Dordrecht, The Netherlands, 2008; Volume 37. [Google Scholar]
- Panebianco, J.E.; Mendez, M.J.; Buschiazzo, D.E.; Bran, D.; Gaitán, J.J. Dynamics of volcanic ash remobilisation by wind through the Patagonian steppe after the eruption of Cordón Caulle, 2011. Sci. Rep. 2017, 7, 45529. [Google Scholar] [CrossRef]
- Romero, J.E.; Morgavi, D.; Arzilli, F.; Daga, R.; Caselli, A.; Reckziegel, F.; Viramonte, J.; Díaz-Alvarado, J.; Polacci, M.; Burton, M.; et al. Eruption dynamics of the 22–23 April 2015 Calbuco Volcano (Southern Chile): Analyses of tephra fall deposits. J. Volcanol. Geotherm. Res. 2016, 317, 15–29. [Google Scholar] [CrossRef]
- Reckziegel, F.; Bustos, E.; Mingari, L.; Báez, W.; Villarosa, G.; Folch, A.; Collini, E.; Viramonte, J.; Romero, J.; Osores, M.S. Forecasting volcanic ash dispersal and coeval resuspension during the April–May 2015 Calbuco eruption. J. Volcanol. Geotherm. Res. 2016, 321, 44–57. [Google Scholar] [CrossRef]
- Castruccio, A.; Clavero, J.; Segura, A.; Samaniego, P.; Roche, O.; Le Pennec, J.L.; Droguett, B. Eruptive parameters and dynamics of the April 2015 sub-Plinian eruptions of Calbuco volcano (southern Chile). Bull. Volcanol. 2016, 78, 62. [Google Scholar] [CrossRef]
- Wilson, T.M.; Cole, J.W.; Stewart, C.; Cronin, S.J.; Johnston, D.M. Ash storms: Impacts of wind-remobilised volcanic ash on rural communities and agriculture following the 1991 Hudson eruption, southern Patagonia, Chile. Bull. Volcanol. 2011, 73, 223–239. [Google Scholar] [CrossRef]
- Hadley, D.; Hufford, G.L.; Simpson, J.J. Resuspension of relic volcanic ash and dust from Katmai: Still an aviation hazard. Weather Forecast. 2004, 19, 829–840. [Google Scholar] [CrossRef]
- Thorsteinsson, T.; Jóhannsson, T.; Stohl, A.; Kristiansen, N.I. High levels of particulate matter in Iceland due to direct ash emissions by the Eyjafjallajökull eruption and resuspension of deposited ash. J. Geophys. Res. Solid Earth 2012, 117. [Google Scholar] [CrossRef]
- Leadbetter, S.J.; Hort, M.C.; Löwis, S.; Weber, K.; Witham, C.S. Modeling the resuspension of ash deposited during the eruption of Eyjafjallajökull in spring 2010. J. Geophys. Res. Atmos. 2012, 117. [Google Scholar] [CrossRef]
- Liu, E.J.; Cashman, K.V.; Beckett, F.M.; Witham, C.S.; Leadbetter, S.J.; Hort, M.C.; Guðmundsson, S. Ash mists and brown snow: Remobilization of volcanic ash from recent Icelandic eruptions. J. Geophys. Res. Atmos. 2014, 119, 9463–9480. [Google Scholar] [CrossRef]
- Miwa, T.; Nagai, M.; Kawaguchi, R. Resuspension of ash after the 2014 phreatic eruption at Ontake volcano, Japan. J. Volcanol. Geotherm. Res. 2018, 351, 105–114. [Google Scholar] [CrossRef]
- Flower, V.J.B.; Kahn, R.A. Distinguishing Remobilized Ash From Erupted Volcanic Plumes Using Space-Borne Multiangle Imaging. Geophys. Res. Lett. 2017, 44, 10772–10779. [Google Scholar] [CrossRef]
- Flower, V.J.B.; Kahn, R.A. Karymsky volcano eruptive plume properties based on MISR multi-angle imagery and the volcanological implications. Atmos. Chem. Phys. 2018, 18, 3903–3918. [Google Scholar] [CrossRef]
- Hobbs, P.V.; Hegg, D.A.; Radke, L.F. Resuspension of volcanic ash from Mount St. Helens. J. Geophys. Res. Ocean. 1983, 88, 3919–3921. [Google Scholar] [CrossRef]
- Fowler, W.B.; Lopushinsky, W. Wind-blown volcanic ash in forest and agricultural locations as related to meteorological conditions. Atmos. Environ. 1986, 20, 421–425. [Google Scholar] [CrossRef]
- Del Bello, E.; Taddeucci, J.; Merrison, J.P.; Alois, S.; Iversen, J.J.; Scarlato, P. Experimental simulations of volcanic ash resuspension by wind under the effects of atmospheric humidity. Sci. Rep. 2018, 8, 14509. [Google Scholar] [CrossRef]
- Etyemezian, V.; Gillies, J.A.; Mastin, L.G.; Crawford, A.; Hasson, R.; Van Eaton, A.R.; Nikolich, G. Laboratory Experiments of Volcanic Ash Resuspension by Wind. J. Geophys. Res. Atmos. 2019, 124, 9534–9560. [Google Scholar] [CrossRef]
- Dominguez, L.; Bonadonna, C.; Forte, P.; Jarvis, P.A.; Cioni, R.; Mingari, L.; Bran, D.; Panebianco, J.E. Aeolian Remobilisation of the 2011-Cordón Caulle Tephra-Fallout Deposit: Example of an Important Process in the Life Cycle of Volcanic Ash. Front. Earth Sci. 2020, 7, 343. [Google Scholar] [CrossRef]
- Jones, A.; Thomson, D.; Hort, M.; Devenish, B. The U.K. Met Office’s Next-Generation Atmospheric Dispersion Model, NAME III. In Air Pollution Modeling and its Application XVII; Borrego, C., Norman, A.L., Eds.; Springer Science & Business Media: Boston, MA, USA, 2007; pp. 580–589. [Google Scholar] [CrossRef]
- Mingari, L.; Collini, E.A.; Folch, A.; Báez, W.; Bustos, E.; Osores, M.S.; Reckziegel, F.; Alexander, P.; Viramonte, J.G. Numerical simulations of windblown dust over complex terrain: The Fiambalá Basin episode in June 2015. Atmos. Chem. Phys. 2017, 17, 6759–6778. [Google Scholar] [CrossRef]
- Forte, P.; Domínguez, L.; Bonadonna, C.; Gregg, C.E.; Bran, D.; Bird, D.; Castro, J.M. Ash resuspension related to the 2011–2012 Cordón Caulle eruption, Chile, in a rural community of Patagonia, Argentina. J. Volcanol. Geotherm. Res. 2018, 350, 18–32. [Google Scholar] [CrossRef]
- Gaiero, D.M.; Simonella, L.; Gassó, S.; Gili, S.; Stein, A.F.; Sosa, P.; Becchio, R.; Arce, J.; Marelli, H. Ground/satellite observations and atmospheric modeling of dust storms originating in the high Puna-Altiplano deserts (South America): Implications for the interpretation of paleo-climatic archives. J. Geophys. Res. Atmos. 2013, 118, 3817–3831. [Google Scholar] [CrossRef]
- Prospero, J.M.; Ginoux, P.; Torres, O.; Nicholson, S.E.; Gill, T.E. Environmental characterization of global sources of atmospheric soil dust identified with the Nimbus 7 Total Ozone Mapping Spectrometer (TOMS) absorbing aerosol product. Rev. Geophys. 2002, 40, 2-1–2-31. [Google Scholar] [CrossRef]
- Middleton, N. The Geography of Dust Storms. Ph.D. Thesis, University of Oxford, Oxford, UK, 1986. [Google Scholar]
- Fick, S.E.; Hijmans, R.J. WorldClim 2: New 1-km spatial resolution climate surfaces for global land areas. Int. J. Climatol. 2017, 37, 4302–4315. [Google Scholar] [CrossRef]
- Garreaud, R.D. The Andes climate and weather. Adv. Geosci. 2009, 22, 3–11. [Google Scholar] [CrossRef]
- Paruelo, J.M.; Beltran, A.; Jobbagy, E.; Sala, O.E.; Golluscio, R.A. The climate of Patagonia: General patterns and controls on biotic processes. Ecol. Austral 1998, 8, 85–101. [Google Scholar]
- Van Eaton, A.R.; Amigo, A.; Bertin, D.; Mastin, L.G.; Giacosa, R.E.; González, J.; Valderrama, O.; Fontijn, K.; Behnke, S.A. Volcanic lightning and plume behavior reveal evolving hazards during the April 2015 eruption of Calbuco volcano, Chile. Geophys. Res. Lett. 2016, 43, 3563–3571. [Google Scholar] [CrossRef]
- Craig, H.; Wilson, T.; Stewart, C.; Outes, V.; Villarosa, G.; Baxter, P. Impacts to agriculture and critical infrastructure in Argentina after ashfall from the 2011 eruption of the Cordón Caulle volcanic complex: An assessment of published damage and function thresholds. J. Appl. Volcanol. 2016, 5. [Google Scholar] [CrossRef]
- Gaitán, J.J.; Ayesa, J.A.; Umaña, F.; Raffo, F.; Bran, D.B. Cartografía del área Afectada por Cenizas Volcánicas en las Provincias de Río Negro y Neuquén; Technical Report; Instituto Nacional de Tecnología Agropecuaria: Bariloche, Argentina, 2011. [Google Scholar]
- Costa, A.; Macedonio, G.; Folch, A. A three-dimensional Eulerian model for transport and deposition of volcanic ashes. Earth Planet. Sci. Lett. 2006, 241, 634–647. [Google Scholar] [CrossRef]
- Folch, A.; Costa, A.; Macedonio, G. FALL3D: A computational model for transport and deposition of volcanic ash. Comput. Geosci. 2009, 35, 1334–1342. [Google Scholar] [CrossRef]
- Folch, A.; Mingari, L.; Gutierrez, N.; Hanzich, M.; Macedonio, G.; Costa, A. FALL3D-8.0: A computational model for atmospheric transport and deposition of particles, aerosols and radionuclides—Part 1: Model physics and numerics. Geosci. Model Dev. 2020, 13, 1431–1458. [Google Scholar] [CrossRef]
- Stull, R.B. An Introduction to Boundary Layer Meteorology; Kluwer Academic Publishers: Dordrecht, The Netherland, 1988. [Google Scholar]
- Davidson, P.A. Turbulence: An Introduction for Scientists and Engineers; Oxford University Press: New York, NY, USA, 2015. [Google Scholar]
- Marticorena, B.; Bergametti, G. Modeling the atmospheric dust cycle: 1. Design of a soil-derived dust emission scheme. J. Geophys. Res. Atmos. 1995, 100, 16415–16430. [Google Scholar] [CrossRef]
- Alfaro, S.C.; Gaudichet, A.; Gomes, L.; Maillé, M. Modeling the size distribution of a soil aerosol produced by sandblasting. J. Geophys. Res. Atmos. 1997, 102, 11239–11249. [Google Scholar] [CrossRef]
- Alfaro, S.C.; Gomes, L. Modeling mineral aerosol production by wind erosion: Emission intensities and aerosol size distributions in source areas. J. Geophys. Res. Atmos. 2001, 106, 18075–18084. [Google Scholar] [CrossRef]
- Shao, Y. A model for mineral dust emission. J. Geophys. Res. Atmos. 2001, 106, 20239–20254. [Google Scholar] [CrossRef]
- Shao, Y. Simplification of a dust emission scheme and comparison with data. J. Geophys. Res. Atmos. 2004, 109. [Google Scholar] [CrossRef]
- Gillette, D.A. Production of dust that may be carried great distances. Geol. Soc. Am. Spec. Pap. 1981, 186, 11–26. [Google Scholar]
- Westphal, D.L.; Toon, O.B.; Carlson, T.N. A two-dimensional numerical investigation of the dynamics and microphysics of Saharan dust storms. J. Geophys. Res. Atmos. 1987, 92, 3027–3049. [Google Scholar] [CrossRef]
- Shao, Y.; Raupach, M.R.; Findlater, P.A. Effect of saltation bombardment on the entrainment of dust by wind. J. Geophys. Res. Atmos. 1993, 98, 12719–12726. [Google Scholar] [CrossRef]
- Shao, Y.; Raupach, M.R.; Leys, J.F. A model for predicting aeolian sand drift and dust entrainment on scales from paddock to region. Aust. J. Soil Res. 1996, 34, 309–342. [Google Scholar] [CrossRef]
- Shao, Y.; Lu, H. A simple expression for wind erosion threshold friction velocity. J. Geophys. Res. Atmos. 2000, 105, 22437–22443. [Google Scholar] [CrossRef]
- Dominguez, L.; Rossi, E.; Mingari, L.; Bonadonna, C.; Forte, P.; Panebianco, J.E.; Bran, D. Mass flux decay timescales of volcanic particles due to aeolian processes in the Argentinian Patagonia steppe. Sci. Rep. 2020, 10, 14456. [Google Scholar] [PubMed]
- Fécan, F.; Marticorena, B.; Bergametti, G. Parametrization of the increase of the aeolian erosion threshold wind friction velocity due to soil moisture for arid and semi-arid areas. Ann. Geophys. 1999, 17, 149–157. [Google Scholar] [CrossRef]
- Raupach, M.R. Drag and drag partition on rough surfaces. Bound. Lay. Meteorol. 1992, 60, 375–395. [Google Scholar] [CrossRef]
- Raupach, M.R.; Gillette, D.A.; Leys, J.F. The effect of roughness elements on wind erosion threshold. J. Geophys. Res. Atmos. 1993, 98, 3023–3029. [Google Scholar] [CrossRef]
- Owen, P.R. Saltation of uniform grains in air. J. Fluid Mech. 1964, 20, 225–242. [Google Scholar] [CrossRef]
- Shao, Y.; Leslie, L.M. Wind erosion prediction over the Australian continent. J. Geophys. Res. Atmos. 1997, 102, 30091–30105. [Google Scholar] [CrossRef]
- Scott, W.D.; Hopwood, J.M.; Summers, K.J. A mathematical model of suspension with saltation. Acta Mech. 1995, 108, 1–22. [Google Scholar] [CrossRef]
- Ganser, G.H. A rational approach to drag prediction of spherical and nonspherical particles. Powder Technol. 1993, 77, 143–152. [Google Scholar] [CrossRef]
- Skamarock, W.C.; Klemp, J.B.; Dudhia, J.; Gill, D.O.; Barker, D.M.; Duda, M.G.; Huang, X.Y.; Wang, W.; Powers, J.G. A Description of the Advanced Research WRF Version 3; Technical Report; NCAR Technical Note, NCAR/TN-475+STR; National Center for Atmospheric Research: Boulder, CO, USA, 2008. [Google Scholar]
- Dee, D.P.; Uppala, S.M.; Simmons, A.J.; Berrisford, P.; Poli, P.; Kobayashi, S.; Andrae, U.; Balmaseda, M.A.; Balsamo, G.; Bauer, P.; et al. The ERA-Interim reanalysis: Configuration and performance of the data assimilation system. Quart. J. R. Meteorol. Soc. 2011, 137, 553–597. [Google Scholar] [CrossRef]
- Darmenova, K.; Sokolik, I.N.; Shao, Y.; Marticorena, B.; Bergametti, G. Development of a physically based dust emission module within the Weather Research and Forecasting (WRF) model: Assessment of dust emission parameterizations and input parameters for source regions in Central and East Asia. J. Geophys. Res. Atmos. 2009, 114. [Google Scholar] [CrossRef]
- Smirnova, T.G.; Brown, J.M.; Benjamin, S.G.; Kim, D. Parameterization of cold-season processes in the MAPS land-surface scheme. J. Geophys. Res. Atmos. 2000, 105, 4077–4086. [Google Scholar] [CrossRef]
- Smirnova, T.G.; Brown, J.M.; Benjamin, S.G.; Kenyon, J.S. Modifications to the Rapid Update Cycle Land Surface Model (RUC LSM) Available in the Weather Research and Forecasting (WRF) Model. Mon. Weather Rev. 2016, 144, 1851–1865. [Google Scholar] [CrossRef]
- Stauffer, D.R.; Seaman, N.L. Use of Four-Dimensional Data Assimilation in a Limited-Area Mesoscale Model. Part I: Experiments with Synoptic-Scale Data. Mon. Weather Rev. 1990, 118, 1250–1277. [Google Scholar] [CrossRef]
- Stauffer, D.R.; Seaman, N.L. Multiscale Four-Dimensional Data Assimilation. J. Appl. Meteorol. 1994, 33, 416–434. [Google Scholar] [CrossRef]
- Angevine, W.M.; Bazile, E.; Legain, D.; Pino, D. Land surface spinup for episodic modeling. Atmos. Chem. Phys. 2014, 14, 8165–8172. [Google Scholar] [CrossRef]
- Bonadonna, C.; Jarvis, P.A.; Dominguez, L.; Frischknecht, C.; Forte, P.; Bran, D.; Aguilar, R.; Beckett, F.; Elissondo, M.; Gillies, J.; et al. Workshop on Wind-Remobilisation Processes of Volcanic Ash, Consensual Document. 2020. Available online: https://vhub.org/resources/4602 (accessed on 10 September 2020).
- Dominguez, L.; Bonadonna, C.; Bran, D. Workshop on the Impacts Associated with the Primary Fallout of Volcanic Ash and Subsequent Aeolian Remobilisation, Consensual Document. 2020. Available online: https://vhub.org/resources/4611 (accessed on 10 September 2020).
- Shao, Y.; Wang, J. A climatology of Northeast Asian dust events. Meteorol. Z. 2003, 12, 187–196. [Google Scholar] [CrossRef]
- Heidke, P. Berechnung Des Erfolges Und Der Güte Der Windstärkevorhersagen Im Sturmwarnungsdienst. Geogr. Ann. 1926, 8, 301–349. [Google Scholar] [CrossRef]
- Toyos, G.; Mingari, L.; Pujol, G.; Villarosa, G. Investigating the nature of an ash cloud event in Southern Chile using remote sensing: Volcanic eruption or resuspension? Remote Sens. Lett. 2017, 8, 146–155. [Google Scholar] [CrossRef]
- Gassó, S.; Stein, A.F. Does dust from Patagonia reach the sub-Antarctic Atlantic Ocean? Geophys. Res. Lett. 2007, 34. [Google Scholar] [CrossRef]
- Prata, A.J. Observations of volcanic ash clouds in the 10–12 µm window using AVHRR/2 data. Int. J. Remote Sens. 1989, 10, 751–761. [Google Scholar] [CrossRef]

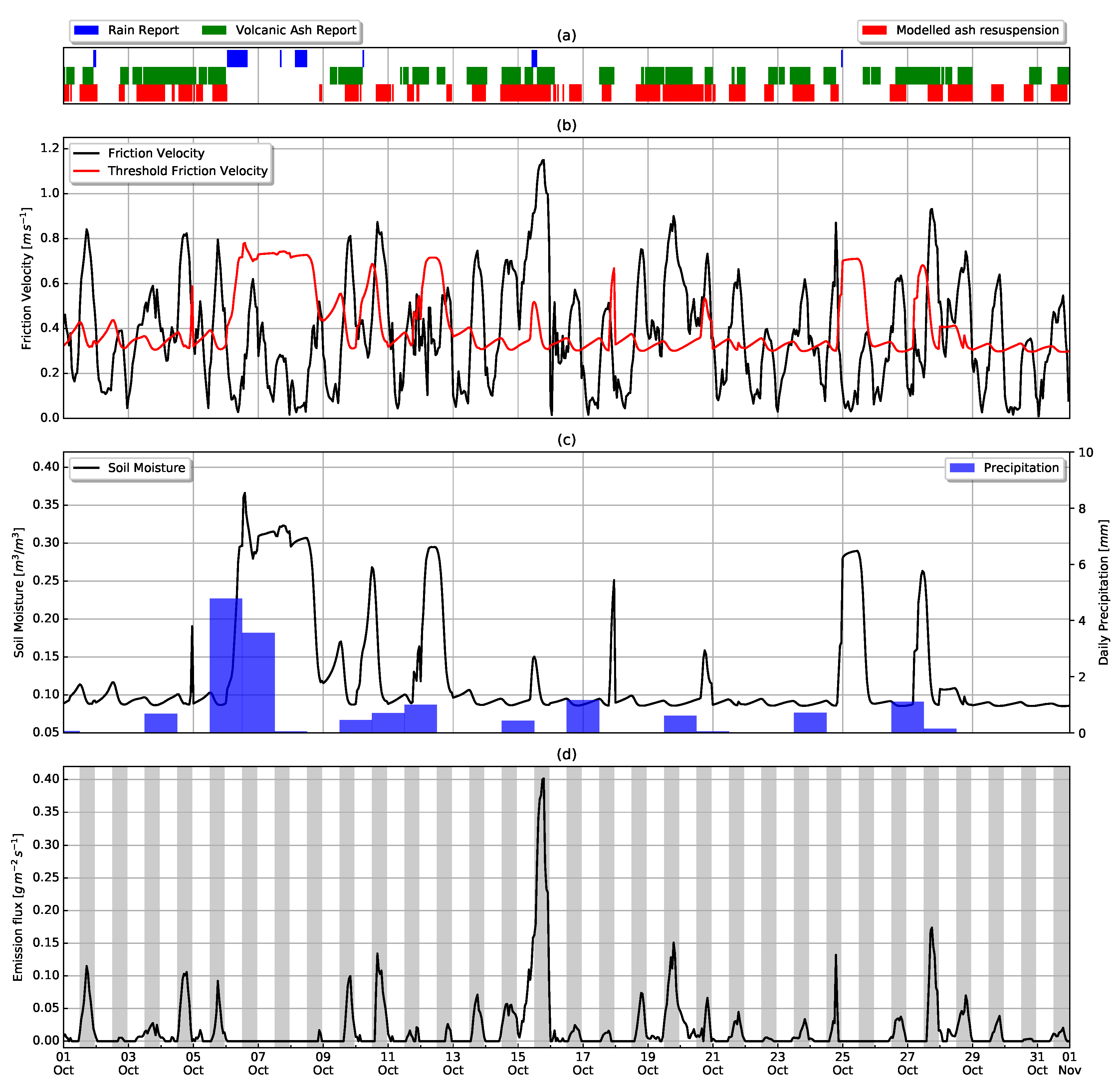
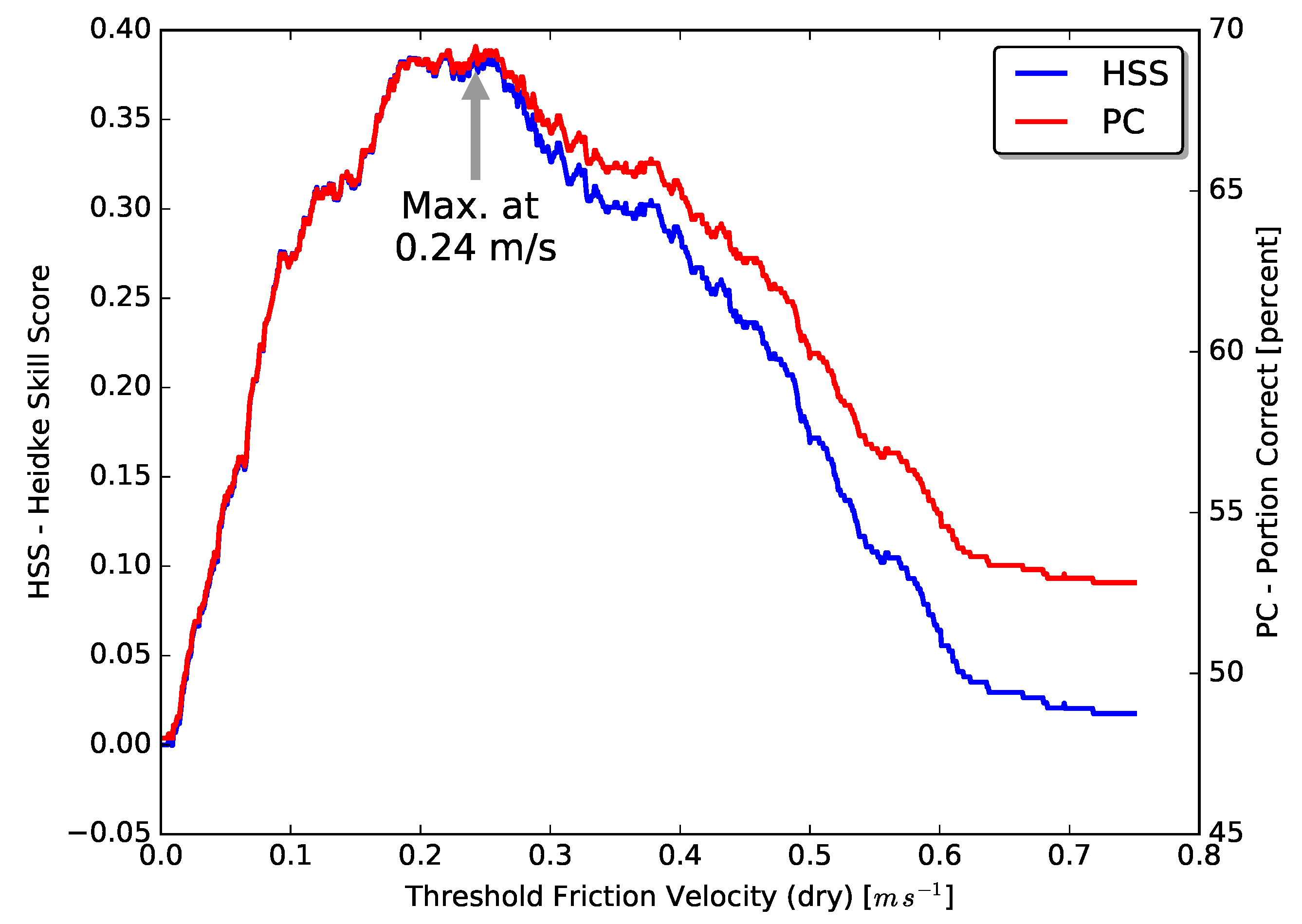

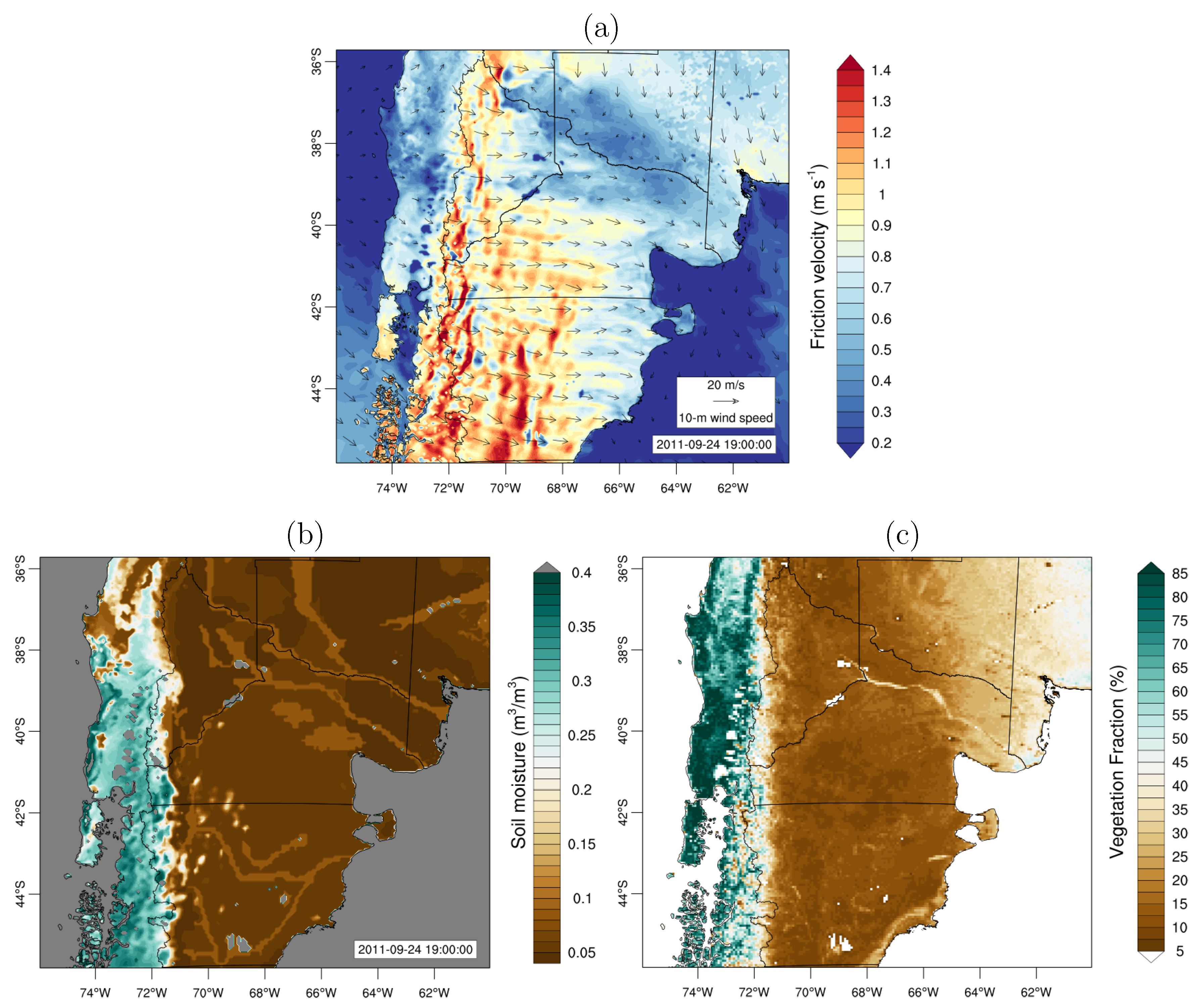

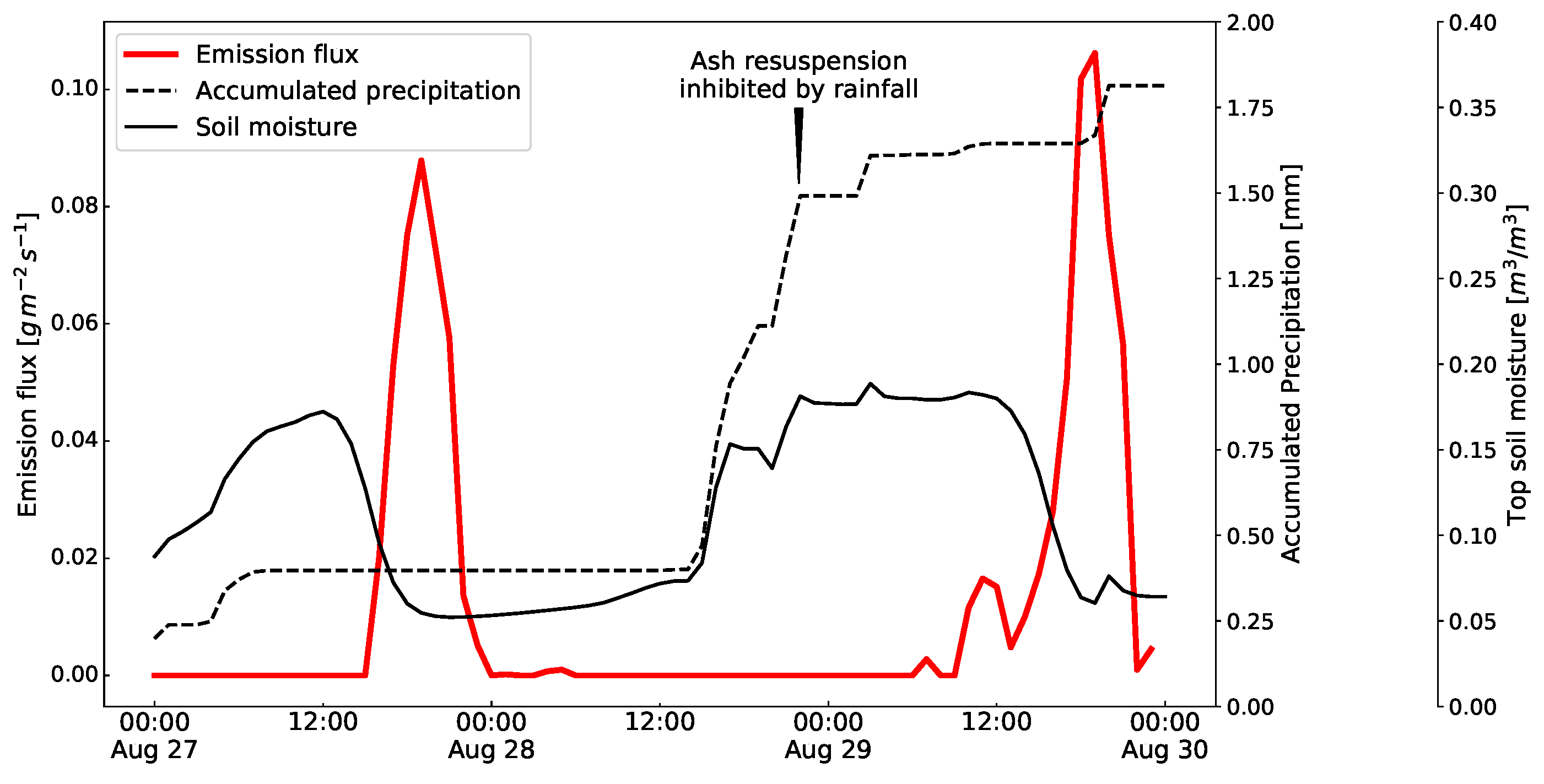

| Start Date | Start Time (UTC) | Duration | Horizontal Resolution | Input Data |
|---|---|---|---|---|
| WRF-ARW | ||||
| 26 August 2011 | 12:00 | 84 h | 18/6 | ERA-Interim † |
| 23 September 2011 | 12:00 | 60 h | 18/6 | ERA-Interim † |
| 14 October 2011 | 00:00 | 78 h | 18/6 | ERA-Interim † |
| 16 October 2011 | 12:00 | 36 h | 18/6 | ERA-Interim † |
| Emission flux | ||||
| ؇ | ؇ | ؇ | 0.01° | WRF (6 ) |
| FALL3D | ||||
| 27 August 2011 | 00:00 | 72 h | 0.1° | WRF (18 ) |
| 24 September 2011 | 00:00 | 48 h | 0.1° | WRF (18 ) |
| 14 October 2011 | 12:00 | 84 h | 0.1° | WRF (18 ) |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mingari, L.; Folch, A.; Dominguez, L.; Bonadonna, C. Volcanic Ash Resuspension in Patagonia: Numerical Simulations and Observations. Atmosphere 2020, 11, 977. https://doi.org/10.3390/atmos11090977
Mingari L, Folch A, Dominguez L, Bonadonna C. Volcanic Ash Resuspension in Patagonia: Numerical Simulations and Observations. Atmosphere. 2020; 11(9):977. https://doi.org/10.3390/atmos11090977
Chicago/Turabian StyleMingari, Leonardo, Arnau Folch, Lucia Dominguez, and Costanza Bonadonna. 2020. "Volcanic Ash Resuspension in Patagonia: Numerical Simulations and Observations" Atmosphere 11, no. 9: 977. https://doi.org/10.3390/atmos11090977
APA StyleMingari, L., Folch, A., Dominguez, L., & Bonadonna, C. (2020). Volcanic Ash Resuspension in Patagonia: Numerical Simulations and Observations. Atmosphere, 11(9), 977. https://doi.org/10.3390/atmos11090977