Vortex Initialization in the NCEP Operational Hurricane Models
Abstract
1. Introduction
2. The HWRF Cycling System
3. Vortex Initialization
3.1. Separation of the Vortex from Its Environmental Fields
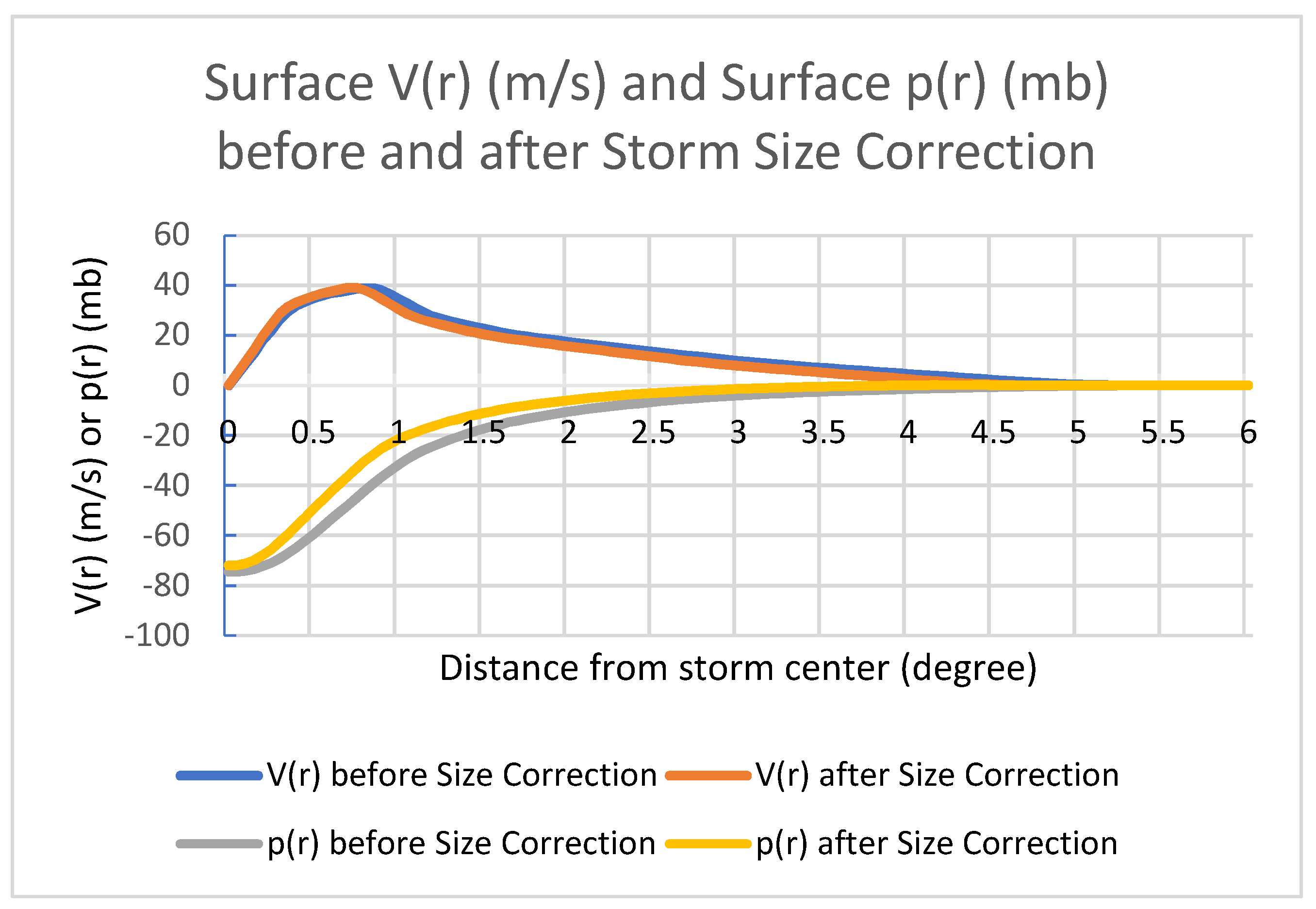
3.2. Storm Size Correction
3.2.1. Surface Pressure Adjustment after the Storm Size Correction
3.2.2. Temperature Adjustment
3.2.3. Water Vapor Adjustment
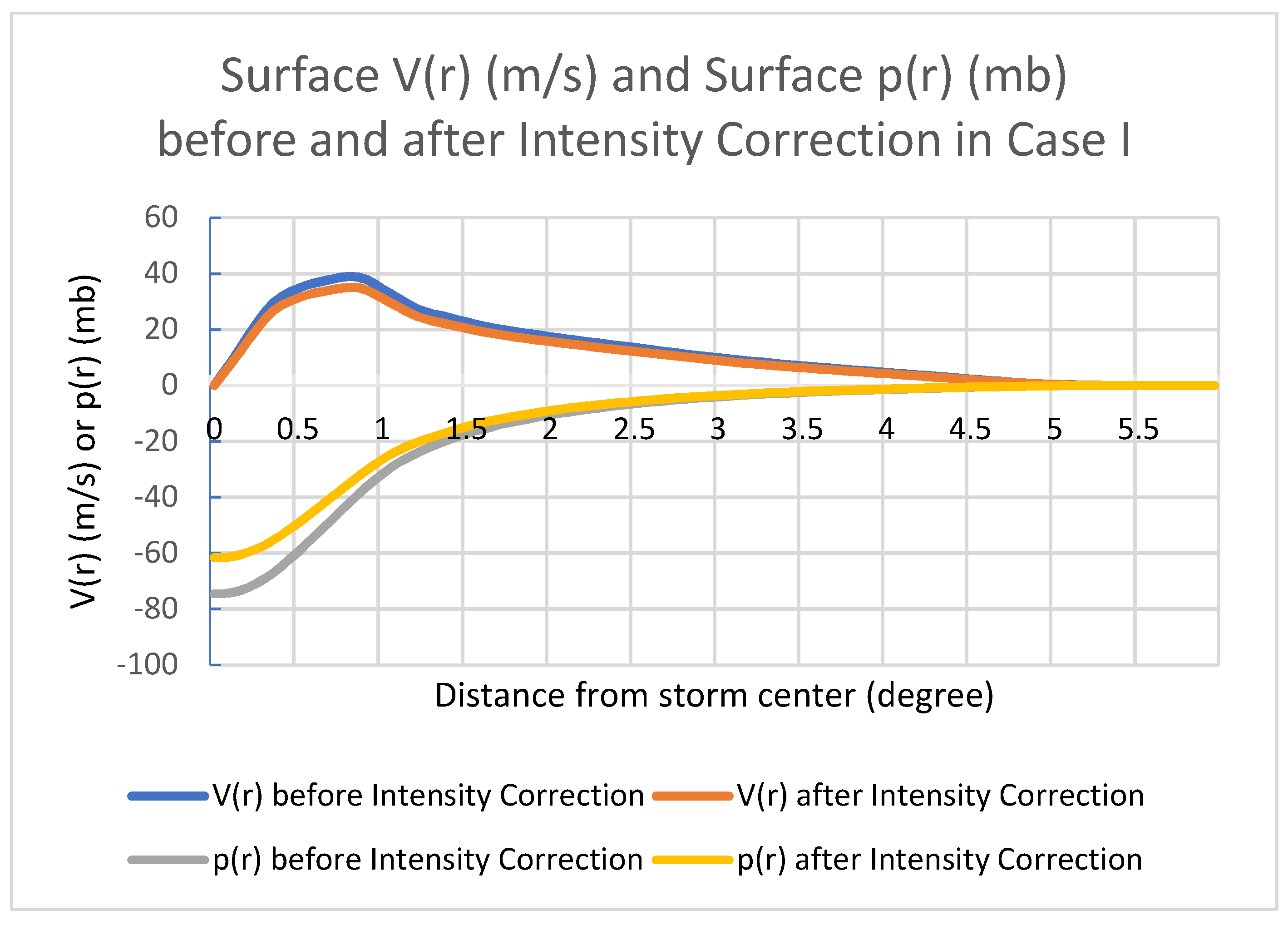
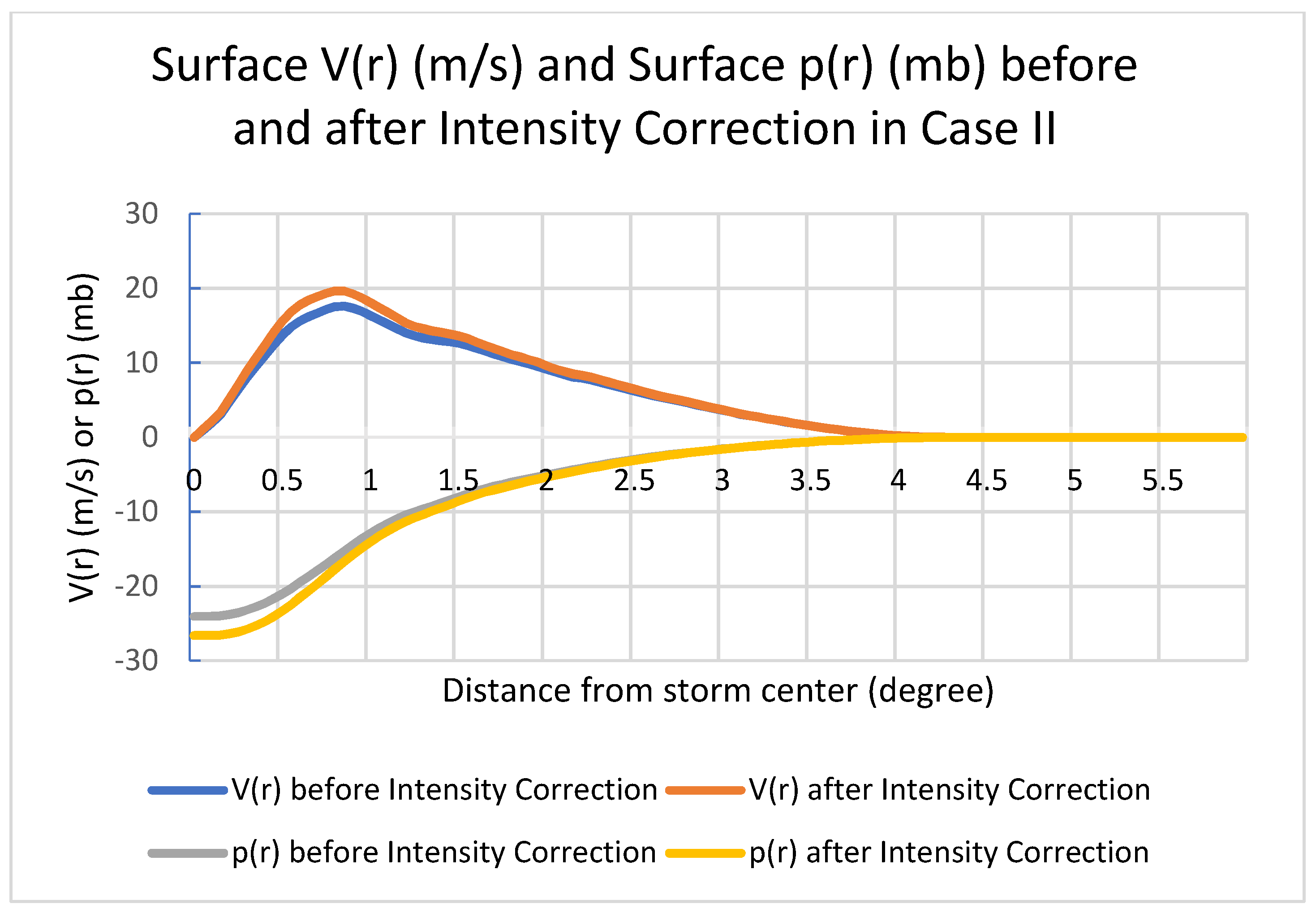
3.3. Storm Intensity Correction
3.3.1. Computation of the Intensity Correction factor β
3.3.2. Surface Pressure, Temperature, and Moisture Adjustments
4. Bogus Vortex Used to Correct Storm Intensity
5. Implementation in the HWRF Model
5.1. Merge Data from Parent and Nests to a Single Domain
- -
- Find the surrounding four grid points 1, 2, 3, and 4 (source points);
- -
- Vertically interpolate data (U, V, T, r) at the four grid points 1, 2, 3, and 4 onto the constant pressure P level (the same pressure level as the target point);
- -
- Horizontally interpolate the new data at level P from the surrounding four points to the target grid point (E-grid to E-grid interpolation).
5.2. Separation of the Vortex from Its Environment
- (1)
- Interpolate parent data onto a 40 × 40 degree domain with 1 degree resolution, and convert the data to constant pressure levels. Then interpolate data from the 3× domain to this 40 × 40 degree domain, and replace the data in the overlapping area with data from the 3× domain.
- (2)
- As in the GFDL model, the filter domain is defined from the original wind components at the model level closest to sigma = 0.85. The radius of the filter domain is limited at 1.2 times ROCI, and is always smaller than 11 degrees.
- (3)
- Separate the vortex from its environment on 1 degree resolution grids using the GFDL method.
- (4)
- Interpolate the environmental field to the original 3 km resolution (3× domain) only for the grids inside the filter domain.
- (5)
- Subtract the new 3× domain data (environmental fields) from its original data to obtain the high resolution vortex. The vortex is stored at (2N + 1) constant pressure levels, and the environmental fields of the 3× domain are still on hybrid vertical grids. After the vortex separation, the surface pressure is changed in the hurricane area, so a new vertical coordinate needs to be defined, and all the fields need to interpolate to the new coordinate.
5.3. Vortex Relocation and Size Correction
5.4. Surface Pressure, Temperature, and Mixing Ratio Correction
5.5. Intensity Correction
6. Test Results and Some Real-Time Runs
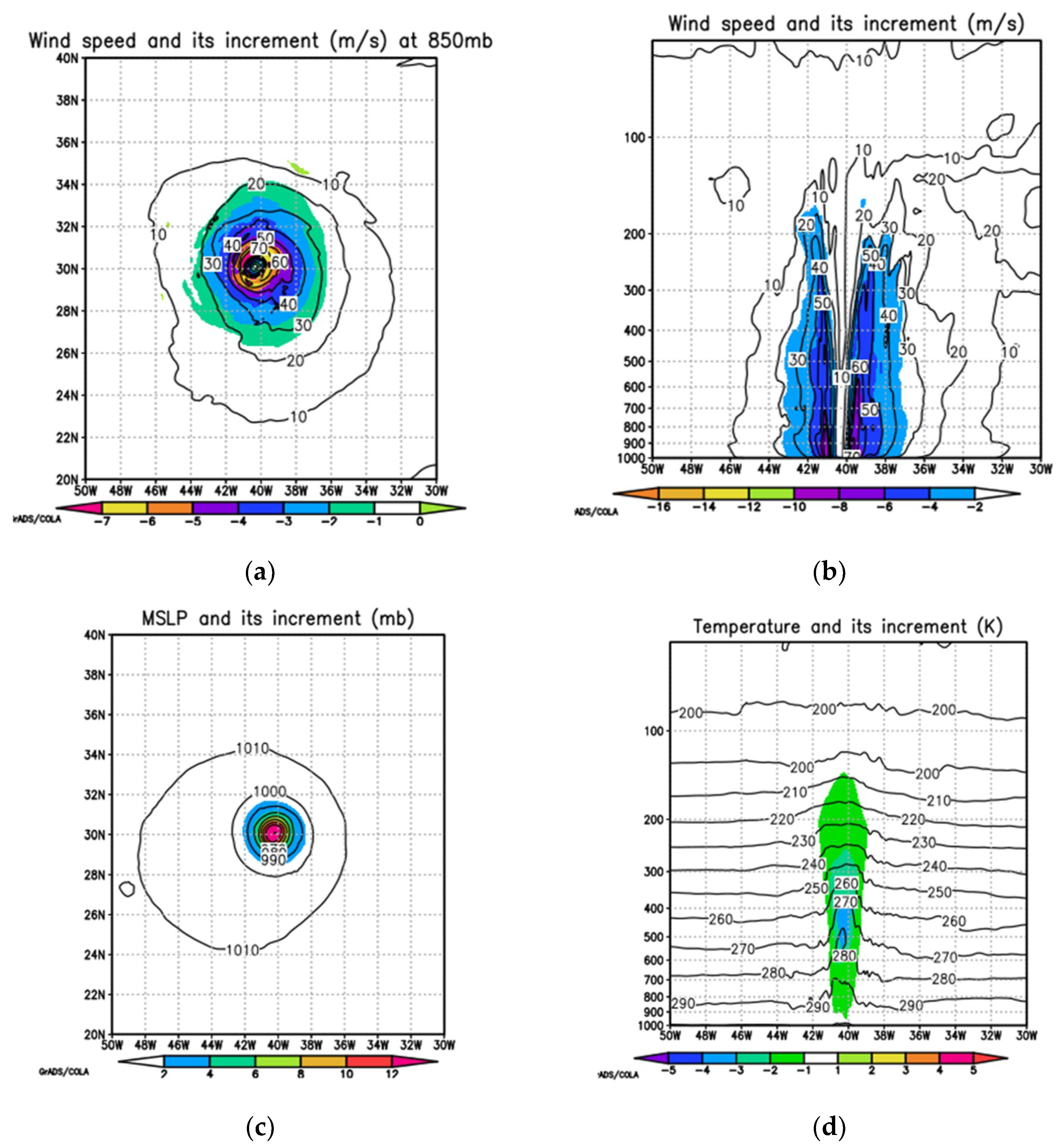
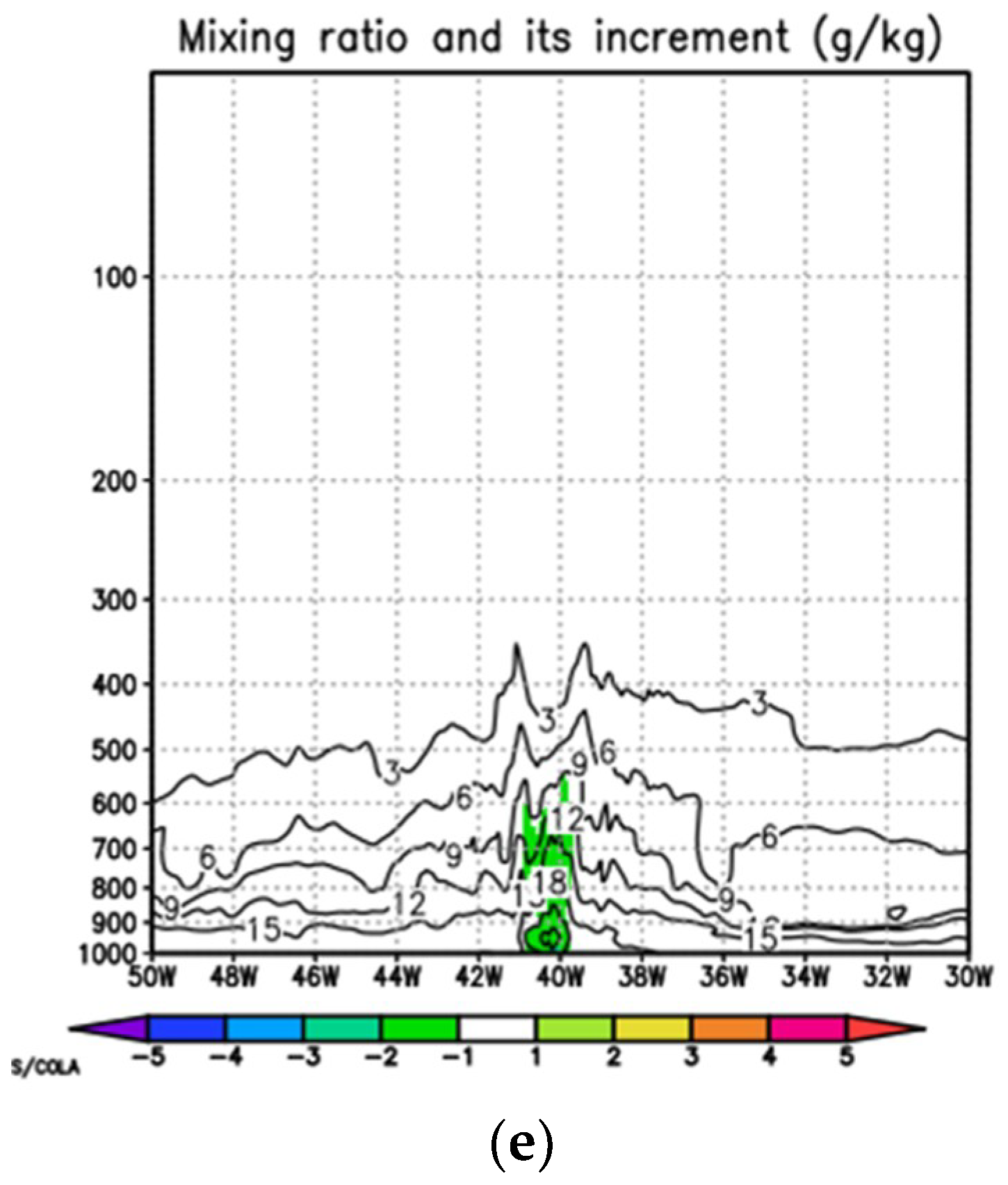
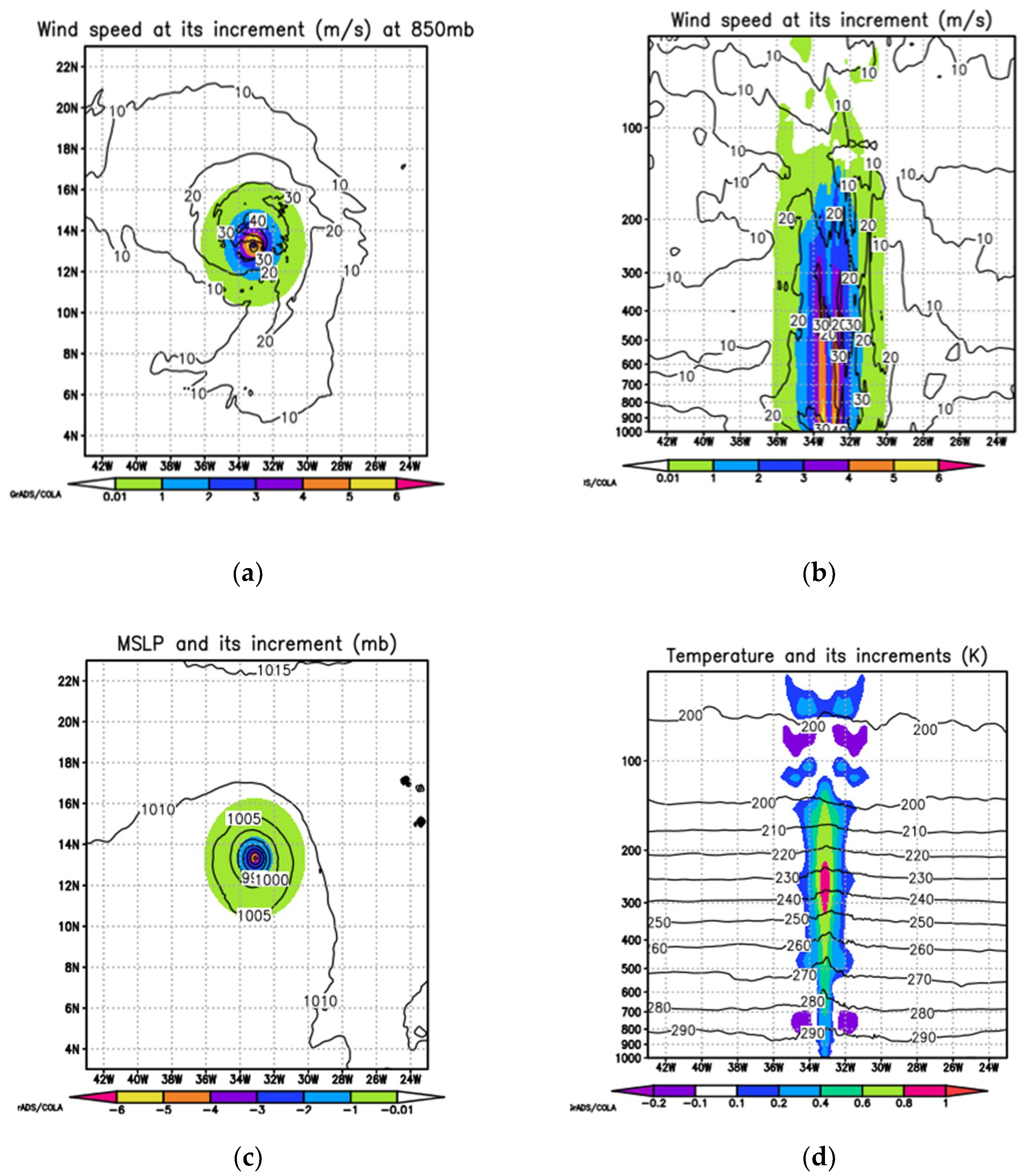
6.1. Impacts of Background Vortex Initialization
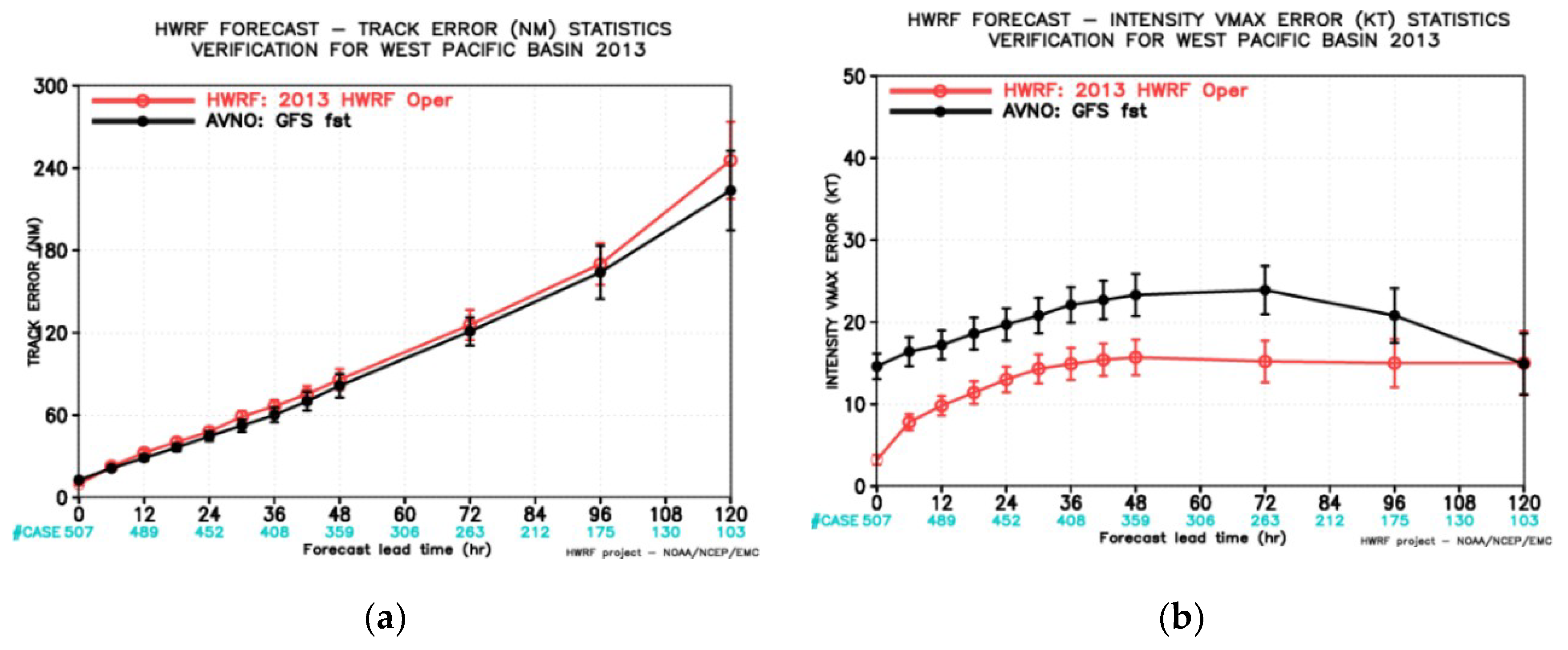
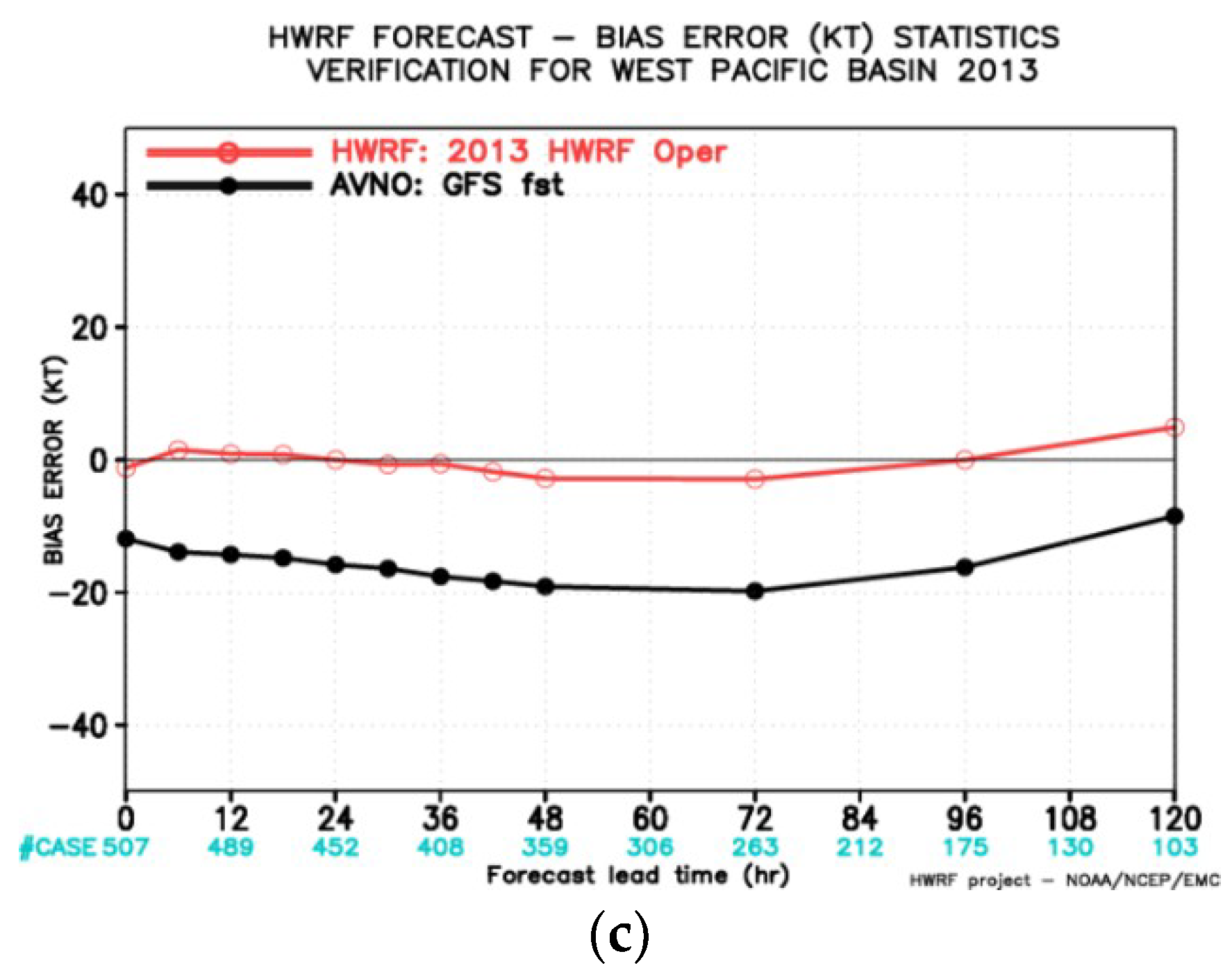
6.2. Some Results from 2013 Real-Time Runs
7. Discussion and Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A. Steps in HWRF Cycling System
- (1)
- Interpolate the 6-h GFS analysis fields onto the HWRF model parent grids, then interpolate the HWRF parent domain onto the inner nests.
- (2)
- Separate the GFS vortex from its GFS environmental field.
- (3)
- Merge the parent and nest data from the 6-h HWRF forecast.
- (4)
- Separate the HWRF vortex from its HWRF environment. For the vortex initialization we need to use GFS vortex, HWRF vortex, and GFS environmental fields.
- (5)
- Determine which vortex will be added to the GFS environmental fields. Check the availability of the HWRF 6-h forecast from the previous run (initialized 6 h before the current run) and observed storm intensity. Based the availability, perform the following steps:
- If the HWRF forecast is not available, then perform the following sub-step:
- If the observed storm maximum wind speed is greater than or equal to 20 ms−1, then use a bogus vortex.
- If the observed maximum wind speed is less than 20 ms−1, then use a corrected GFS vortex.
- If the HWRF forecast is available, then perform the following sub-step:
- If the observed maximum wind speed is equal to or more than 14 ms−1, then extract the vortex from the forecast fields and correct it based on the TCVitals.
- If the observed maximum wind speed is less than 14 ms−1, then use a corrected GFS vortex based on the TCVitals.
- (6)
- Add the vortex obtained in (5) to the environmental fields obtained in (2).
- (7)
- Interpolate the data obtained from (6) onto the outer and ghost domains. If performing an inner core data assimilation (optional in the HWRF v3.5a as detailed in Section 1 and Section 2), assimilate the inner core data on the ghost domain. This domain is created for inner core data assimilation only, has the same resolution as the innermost nest and is about three times larger than the inner nest. Finally, merge the data from the ghost domain onto the outer and inner nest domains.
- (8)
- Run the HWRF forecast model.
- Storm location (data used: storm center position).
- Storm size (data used: radius of maximum surface wind speed, 34-kt wind radius, and radius of the outmost closed isobar).
- Storm intensity (data used: maximum surface wind speed and, secondarily, the minimum sea level pressure).
Appendix B. E-Grid to E-Grid Interpolation
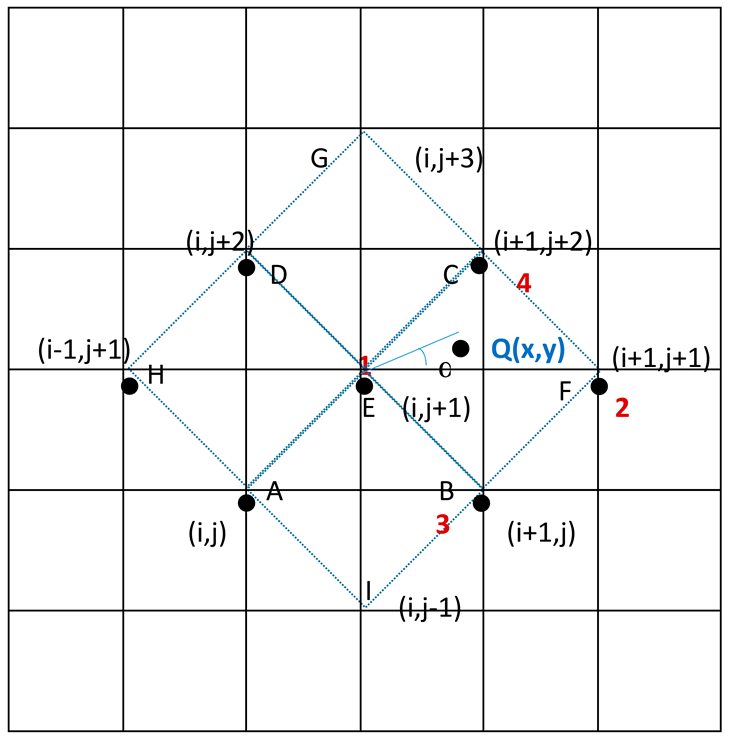
- at point E with index (i, j + 1);
- at point F with index (i + 1, j + 1);
- at point B with index (i + 1, j); and
- At point C with index (i + 1, j + 2).
- at point D with index (i, j + 2);
- at point C with index (i + 1, j + 2);
- at point E with index (i, j + 1); and
- at point G with index (i, j + 3).
- at point H with index (i − 1, j + 1);
- at point E with index (i, j + 1);
- at point A with index (i, j); and
- at point D with index (i, j + 2).
- at point A with index (i, j);
- at point B with index (i + 1, j);
- at point I with index (i, j − 1); and
- at point E with index (i, j + 1).
References
- Leslie, L.M.; Holland, G.J. On the bogussing of tropical cyclones in numerical models: A comparison of vortex profiles. Meteorol. Atmos. Phys. 1995, 56, 101–110. [Google Scholar] [CrossRef]
- Davidson, N.E.; Weber, H.C. The BMRC high-resolution tropical cyclone prediction system: TC-LAPS. Mon. Weather Rev. 2000, 128, 1245–1265. [Google Scholar] [CrossRef]
- Kwon, I.-H.; Cheong, H.-B. Tropical cyclone initialization with a spherical high-order filter and an idealized three-dimensional bogus vortex. Mon. Weather Rev. 2010, 138, 1344–1367. [Google Scholar] [CrossRef]
- Rappin, E.D.; Nolan, D.S.; Majumdar, S.J. A highly configurable vortex initialization method for tropical cyclones. Mon. Weather Rev. 2013, 141, 3556–3575. [Google Scholar] [CrossRef]
- Lord, S.J. A bogussing system for vortex circulations in the National Meteorological Center Global Forecast Model. Preprints. In 19th Conference on Hurricanes and Tropical Meteorology; American Meteor Society: Miami, FL, USA, 1991; pp. 328–330. [Google Scholar]
- Fiorino, M.; Elsberry, R.L. Some aspects of vortex structure related to tropical cyclone motion. J. Atmos. Sci. 1989, 46, 975–990. [Google Scholar] [CrossRef]
- Goerss, J.; Jeffries, R. Assimilation of synthetic tropical cyclone observations into the Navy Operational Global Atmospheric Prediction System. Weather Forecast. 1994, 9, 557–576. [Google Scholar] [CrossRef][Green Version]
- Radford, A.M. Forecasting the movement of tropical cyclones at the Met. Off. Meteorol. Appl. 1994, 1, 355–363. [Google Scholar] [CrossRef]
- Heming, J.T.; Chan, J.C.-L.; Radford, A.M. A new scheme for the initialization of tropical cyclones in the UK Meteorological Office global model. Meteorol. Appl. 1995, 2, 171–184. [Google Scholar] [CrossRef]
- Kurihara, Y.; Bender, M.A.; Tuleya, R.E.; Ross, R.J. Prediction experiments of Hurricane Gloria (1985) using a multiply nested movable mesh model. Mon. Weather Rev. 1990, 118, 2185–2198. [Google Scholar] [CrossRef][Green Version]
- Pu, Z.; Braun, S.A. Evaluation of bogus vortex techniques with four-dimensional variational data assimilation. Mon. Weather Rev. 2001, 129, 2023–2039. [Google Scholar] [CrossRef]
- Cha, D.-H.; Wang, Y. A dynamical initialization scheme for real-time forecasts of tropical cyclones using the WRF model. Mon. Weather Rev. 2013, 141, 964–986. [Google Scholar] [CrossRef]
- Hendricks, E.A.; Peng, M.S.; Li, T.; Ge, X. Performance of a dynamic initialization scheme in the Coupled Ocean– Atmosphere Mesoscale Prediction System for Tropical Cyclones (COAMPS-TC). Weather Forecast. 2011, 26, 650–663. [Google Scholar] [CrossRef]
- Gall, R.; Franklin, J.; Marks, F.D.; Rappaport, E.N.; Toepfer, F. The Hurricane Forecast Improvement Project. Bull. Am. Meteorol. Soc. 2013, 94, 329–343. [Google Scholar] [CrossRef]
- Kurihara, Y.; Bender, M.; Tuleya, R.; Ross, R. Improvements in the GFDL Hurricane Prediction System. Mon. Weather Rev. 1995, 123, 2791–2801. [Google Scholar] [CrossRef]
- Kleist, D.T.; Parrish, D.F.; Derber, J.C.; Treadon, R.; Wu, W.-S.; Lord, S.J. Introduction of the GSI into the NCEP Global Data Assimilation System. Weather Forecast. 2009, 24, 1691–1705. [Google Scholar] [CrossRef]
- Liu, Q.; Surgi, N.; Lord, S.; Wu, W.-S.; Parrish, S.; Gopalakrishnan, S.; Waldrop, J.; Gamache, J. Hurricane Initialization in HWRF Model, Preprints. In Proceedings of the 27th Conference on Hurricanes and Tropical Meteorology, Monterey, CA, USA, 24–28 April 2006. [Google Scholar]
- Liu, Q.; Marchok, T.; Pan, H.-L.; Bender, M.; Lord, S. Improvements in Hurricane Initialization and Forecasting at NCEP with Global and Regional (GFDL) Models; NCEP Office Note 472; NCEP/NOAA/DOC: Silver Spring, MD, USA, 2000.
- Liu, Q.; Lord, S.; Surgi, N.; Pan, H.L.; Marchok, T.; Tuleya, R.; Bender, M. Hurricane Initialization in High Resolution Models. In Proceedings of the 26th AMS Conference on Hurricanes and Tropical Meteorology, Miami, FL, USA, 2–7 May 2004. [Google Scholar]
- Liu, Q.; Lord, S.; Surgi, N.; Zhu., Y.; Wobus, R.; Toth, Z.; Marchok, T. Hurricane relocation in global ensemble forecast system. Preprints. In 27th Conference on Hurricanes and Tropical Meteorology; American Meteor Society: Monterey, CA, USA, 2006; Volume 5, p. 13. [Google Scholar]
- Tong, M.; Sippel, J.A.; Tallapragada, V.; Liu, E.; Kieu, C.; Kwon, I.H.; Wang, W.; Liu, Q.; Ling, Y.; Zhang, B. Impact of assimilating aircraft reconnaissance observations on tropical cyclone initialization and prediction using operational HWRF and GSI ensemble–variational hybrid data assimilation. Mon. Weather Rev. 2018, 146, 4155–4177. [Google Scholar] [CrossRef]
- Gopalakrishnan, S.G.; Goldenberg, S.; Quirino, T.; Marks, F.; Zhang, X.; Yeh, K.-S.; Atlas, R.; Tallapragada, V. Towards improving high-resolution numerical hurricane forecasting: Influence of model horizontal grid resolution, initialization, and physics. Weather Forecast. 2012, 27, 647–666. [Google Scholar] [CrossRef]
- Schubert, W.H.; Hack, J.J. Transformed Eliassen balanced vortex model. J. Atmos. Sci. 1983, 40, 1571–1583. [Google Scholar] [CrossRef]
- Bolton, D. The computation of equivalent potential temperature. Mon. Weather Rev. 1980, 108, 1046–1053. [Google Scholar] [CrossRef]
- Janjic, Z. A nonhydrostatic model based on a new approach. Meteorol. Atmos. Phys. 2003, 82, 271–285. [Google Scholar] [CrossRef]


© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, Q.; Zhang, X.; Tong, M.; Zhang, Z.; Liu, B.; Wang, W.; Zhu, L.; Zhang, B.; Xu, X.; Trahan, S.; et al. Vortex Initialization in the NCEP Operational Hurricane Models. Atmosphere 2020, 11, 968. https://doi.org/10.3390/atmos11090968
Liu Q, Zhang X, Tong M, Zhang Z, Liu B, Wang W, Zhu L, Zhang B, Xu X, Trahan S, et al. Vortex Initialization in the NCEP Operational Hurricane Models. Atmosphere. 2020; 11(9):968. https://doi.org/10.3390/atmos11090968
Chicago/Turabian StyleLiu, Qingfu, Xuejin Zhang, Mingjing Tong, Zhan Zhang, Bin Liu, Weiguo Wang, Lin Zhu, Banglin Zhang, Xiaolin Xu, Samuel Trahan, and et al. 2020. "Vortex Initialization in the NCEP Operational Hurricane Models" Atmosphere 11, no. 9: 968. https://doi.org/10.3390/atmos11090968
APA StyleLiu, Q., Zhang, X., Tong, M., Zhang, Z., Liu, B., Wang, W., Zhu, L., Zhang, B., Xu, X., Trahan, S., Bernardet, L., Mehra, A., & Tallapragada, V. (2020). Vortex Initialization in the NCEP Operational Hurricane Models. Atmosphere, 11(9), 968. https://doi.org/10.3390/atmos11090968





