Calibration and Improved Speckle Statistics of IM-CW Lidar for Atmospheric CO2 Measurements
Abstract
1. Introduction
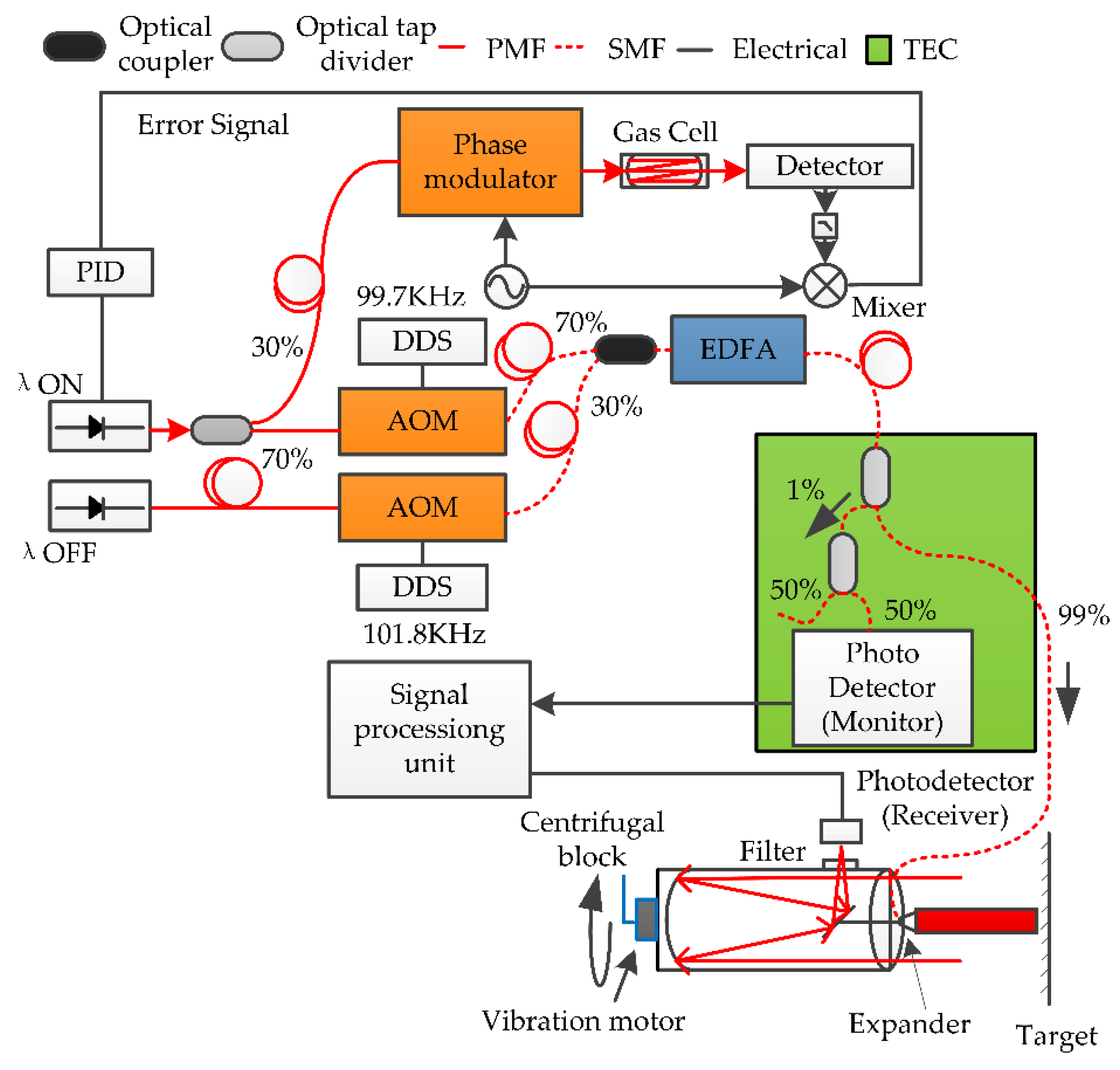
2. Theory and Experimental Instrument
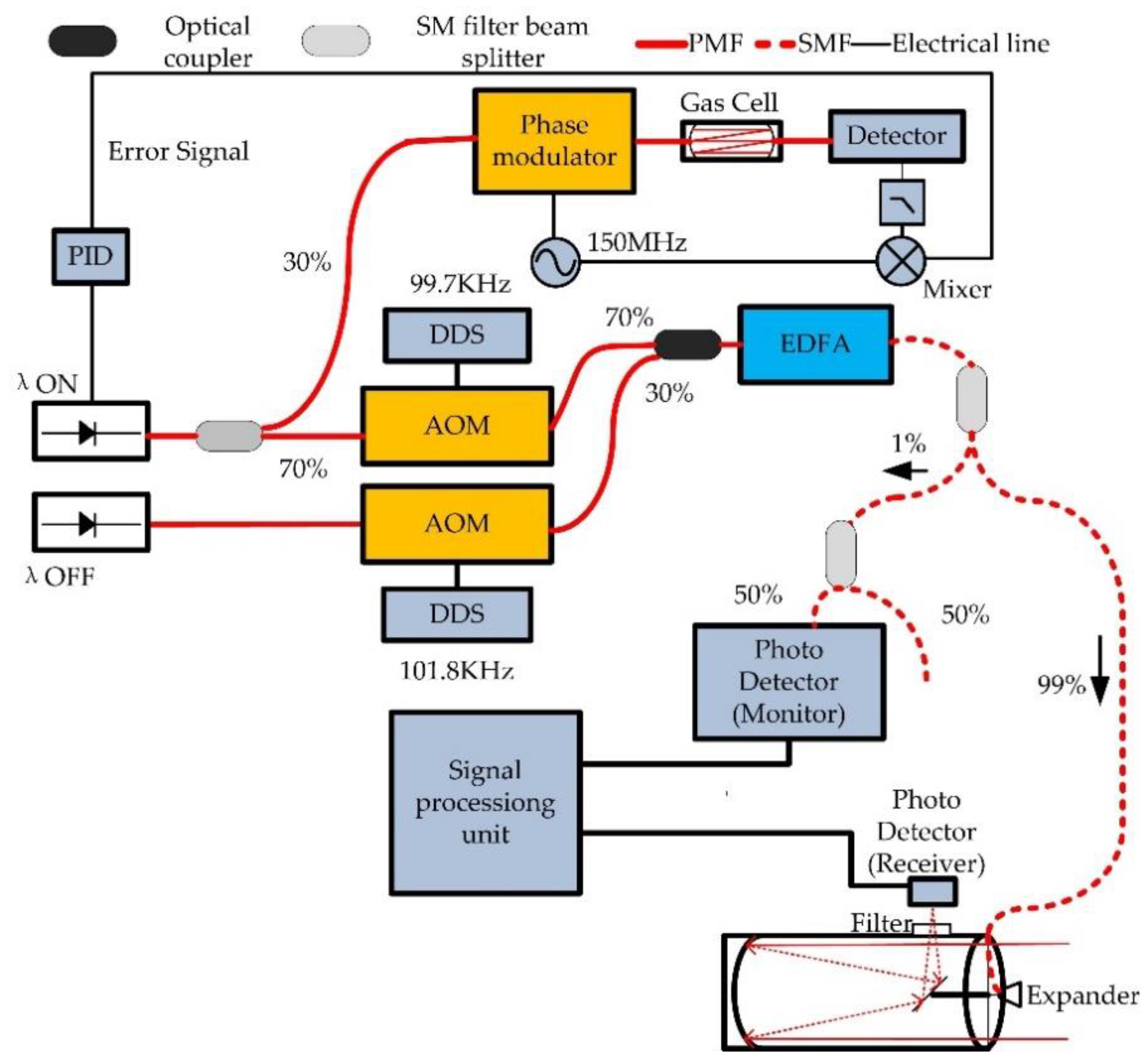
2.1. Measurement Principle
2.2. Preliminary Configuration and Results
3. Calibration System and Improved Speckle Statistics
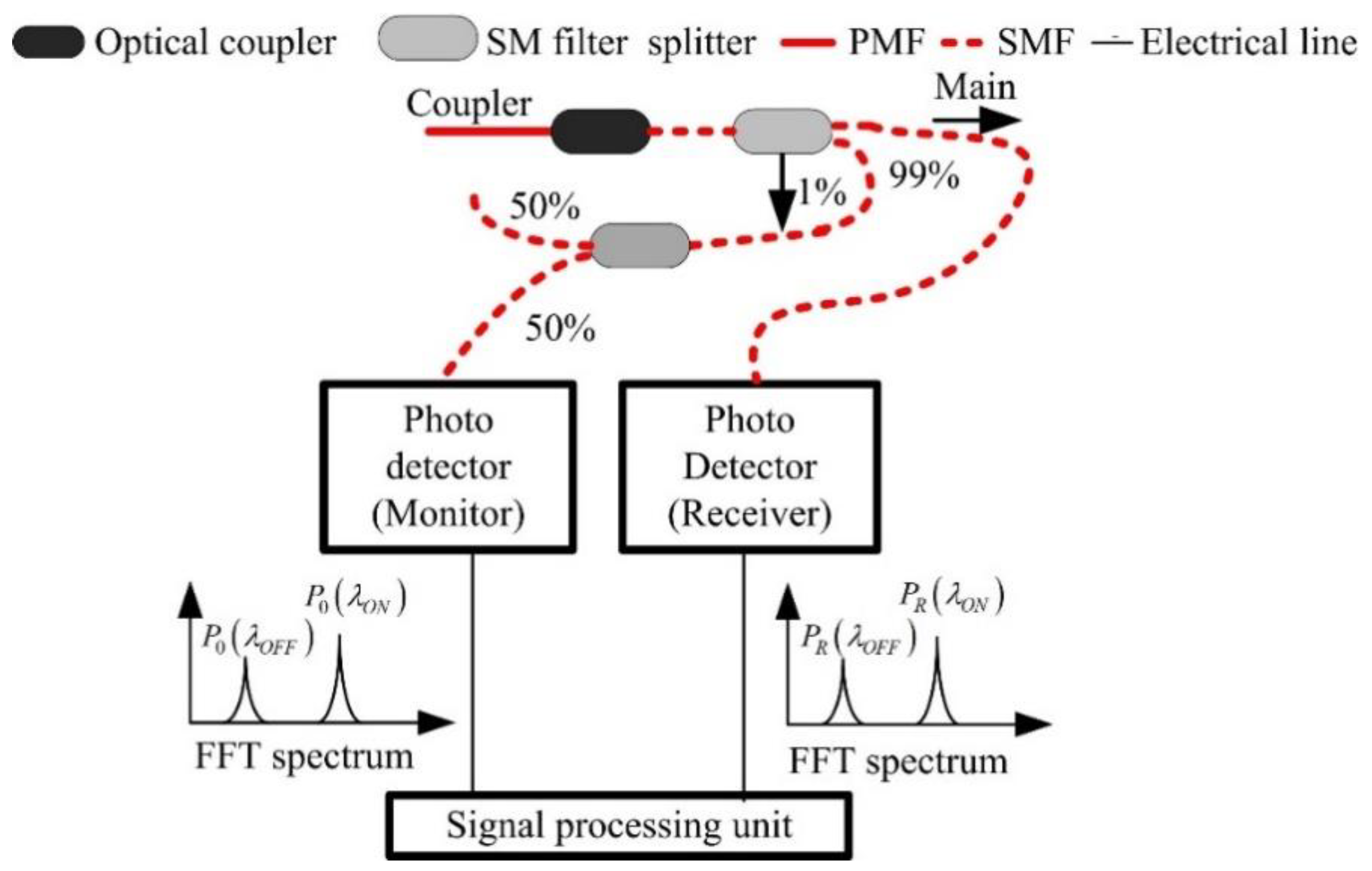
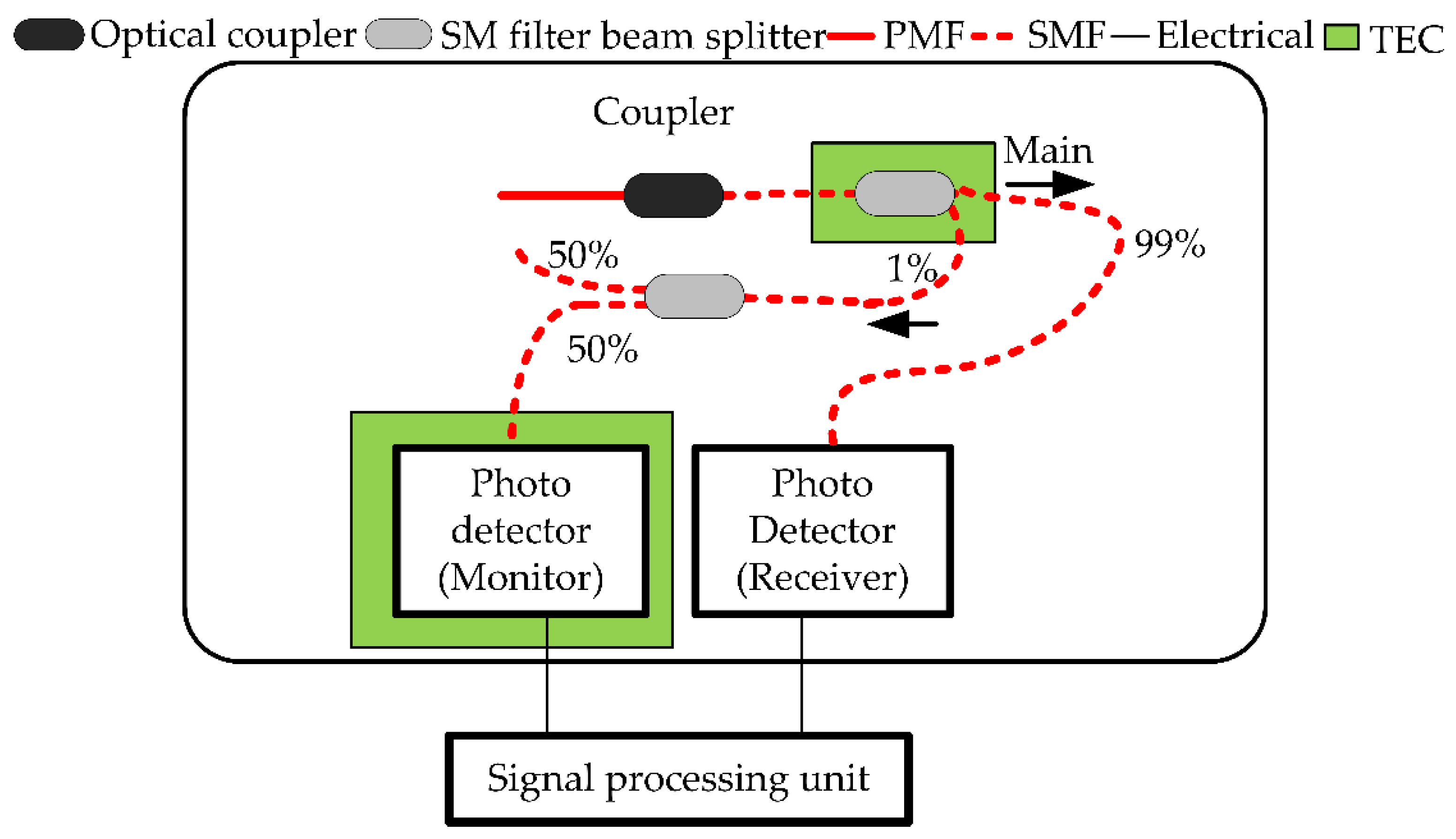
3.1. System Errors Sources
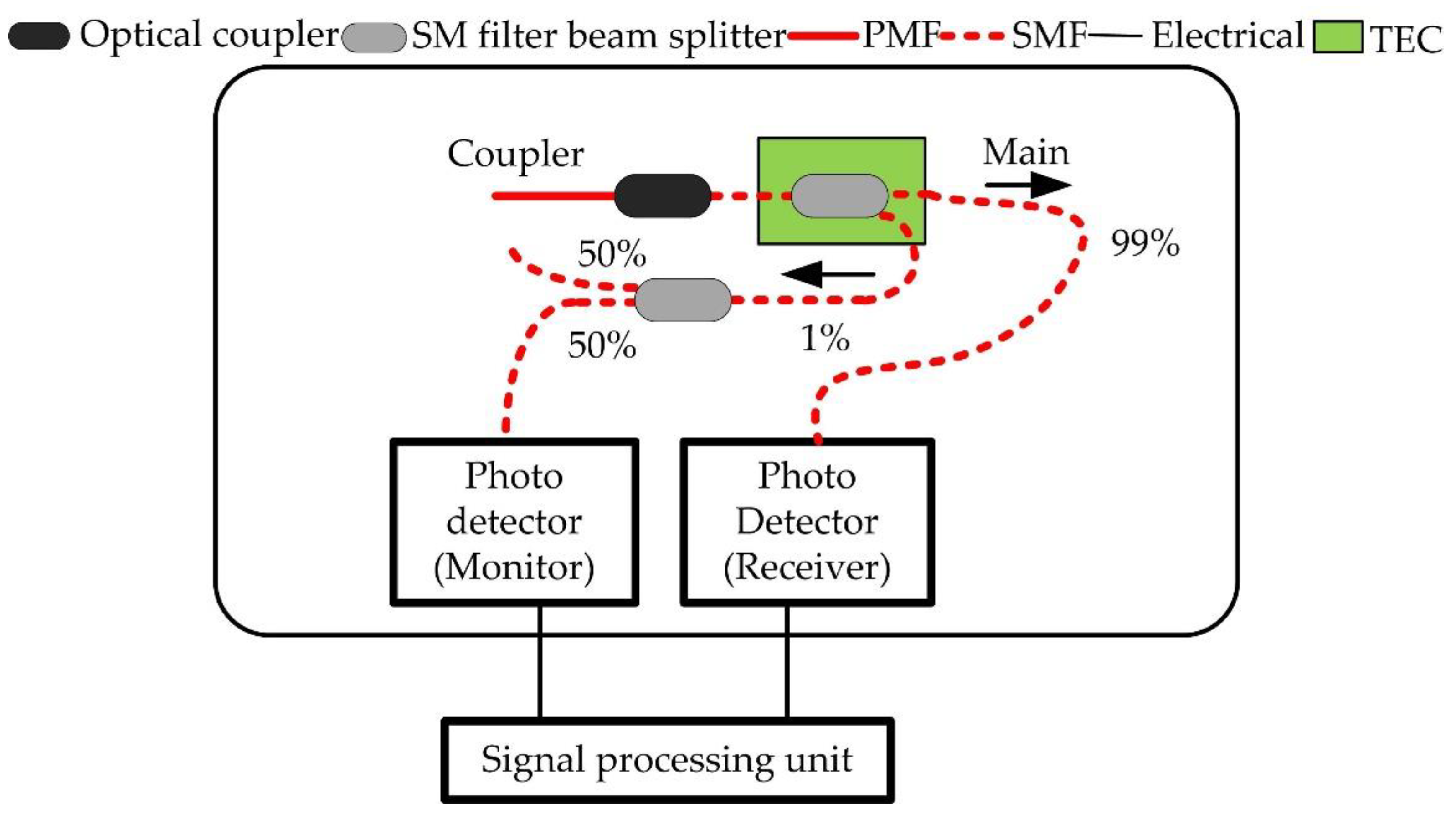
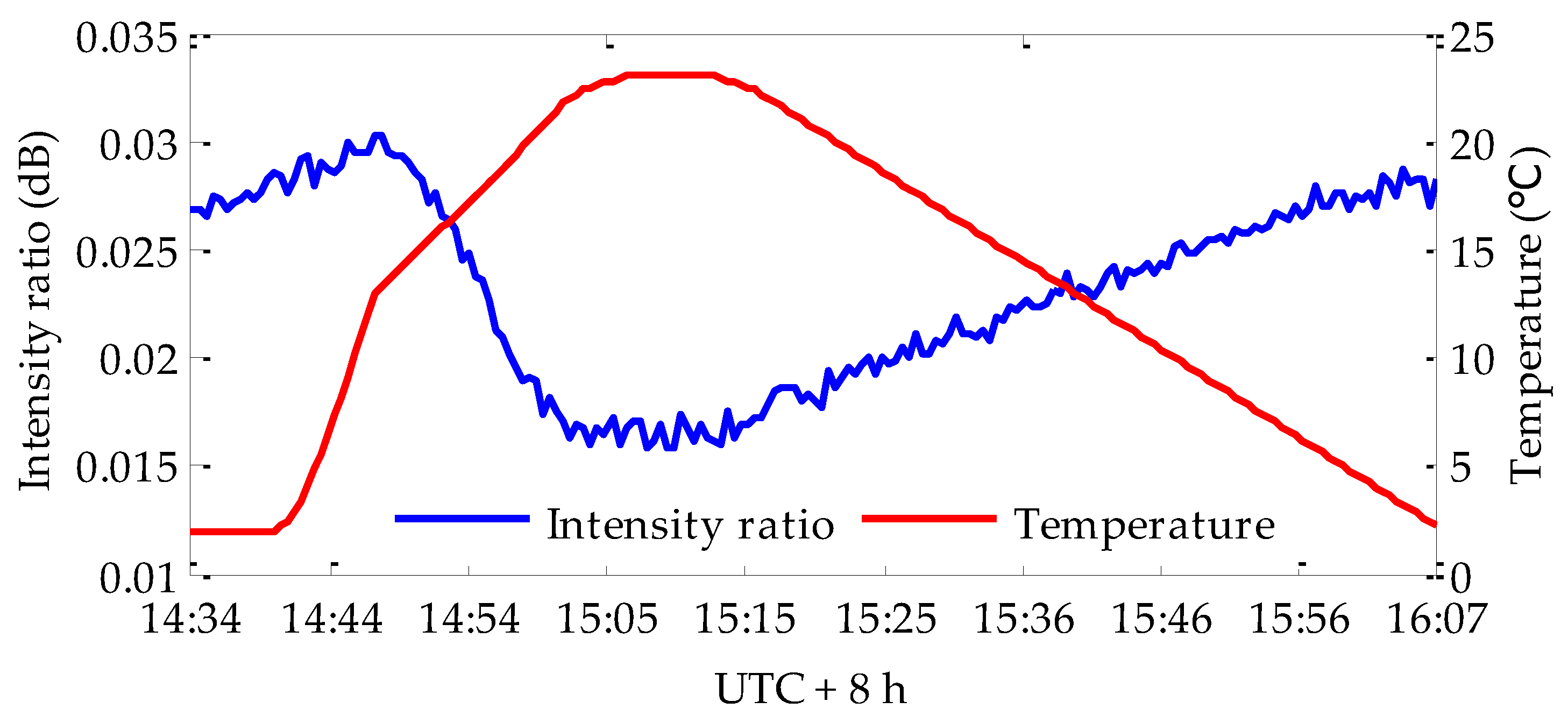
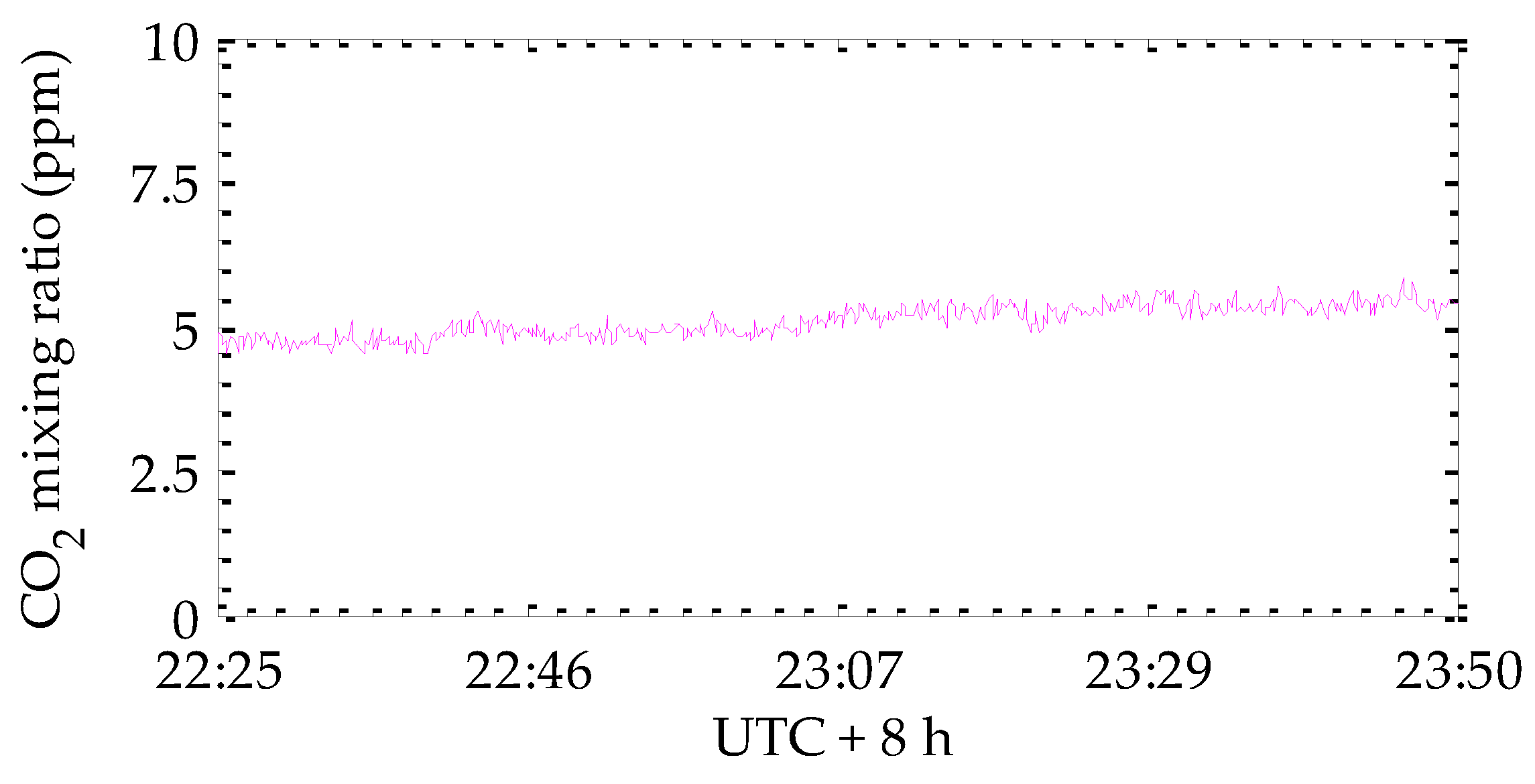
3.2. Target Calibration Precision and Optical Fiber Link Test
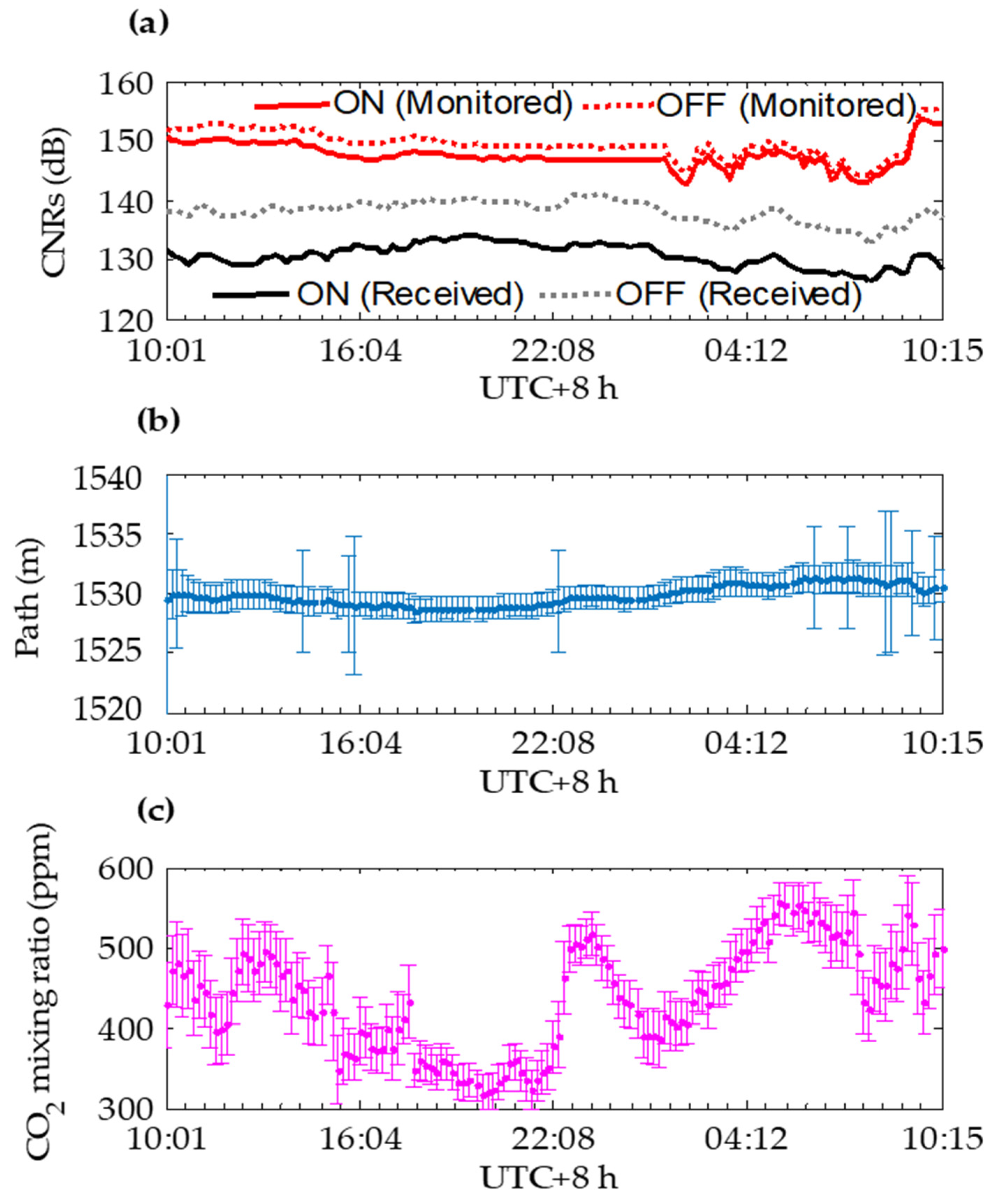
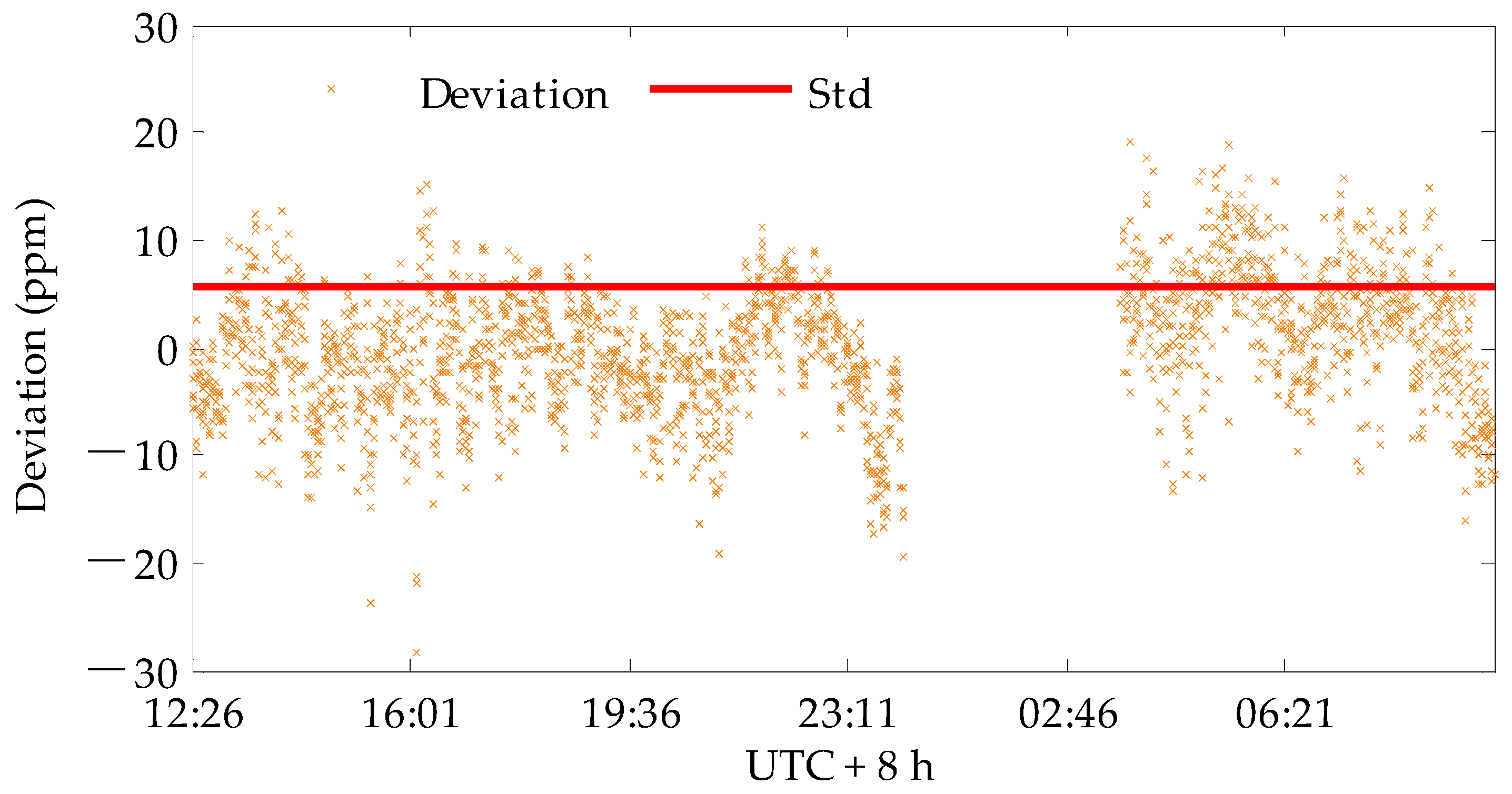
3.3. Verification Experiments of System Calibration
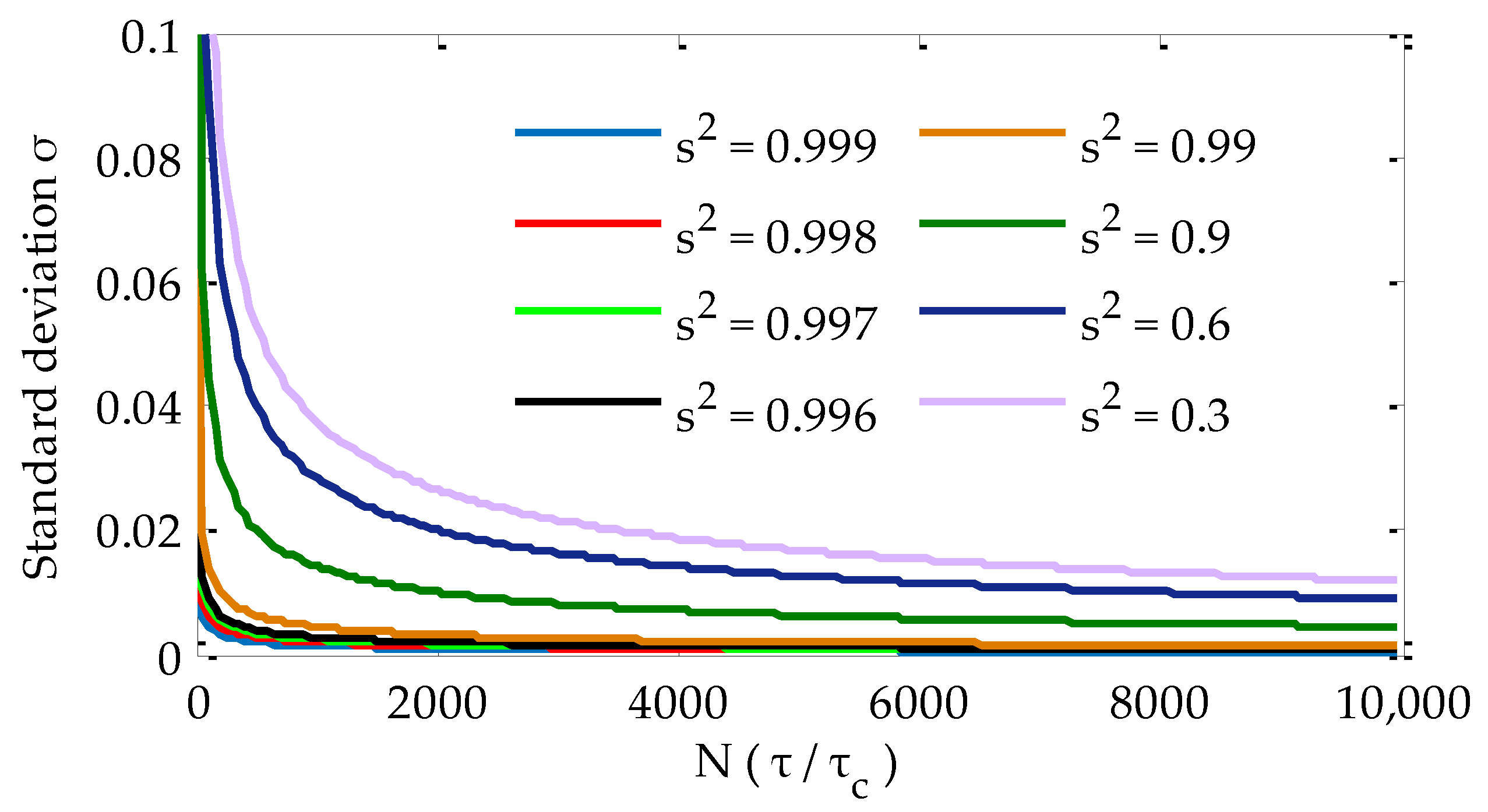
3.4. Improved Speckle Statistics
4. Experiments and Results
4.1. Analysis of Speckle Suppression
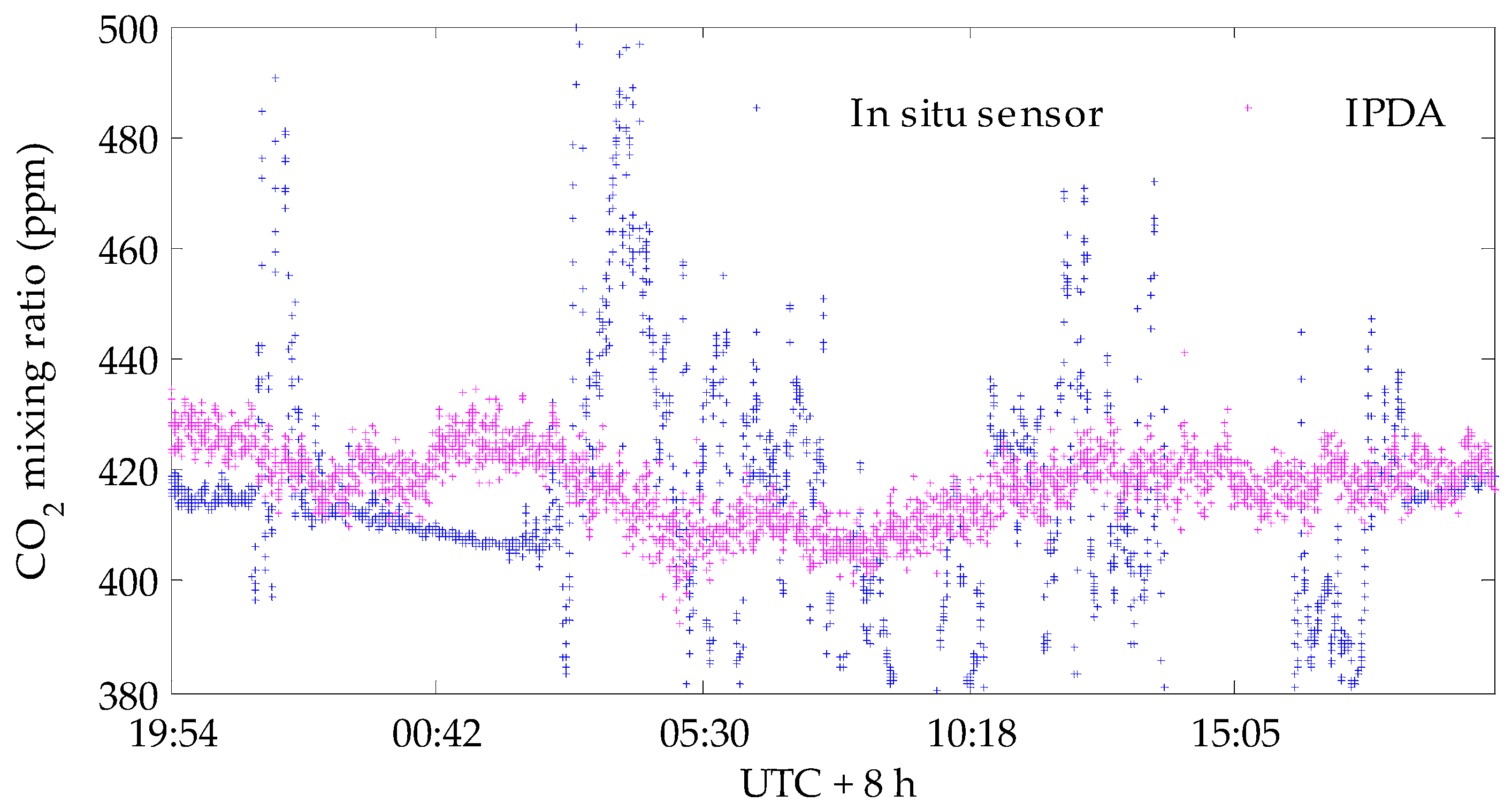
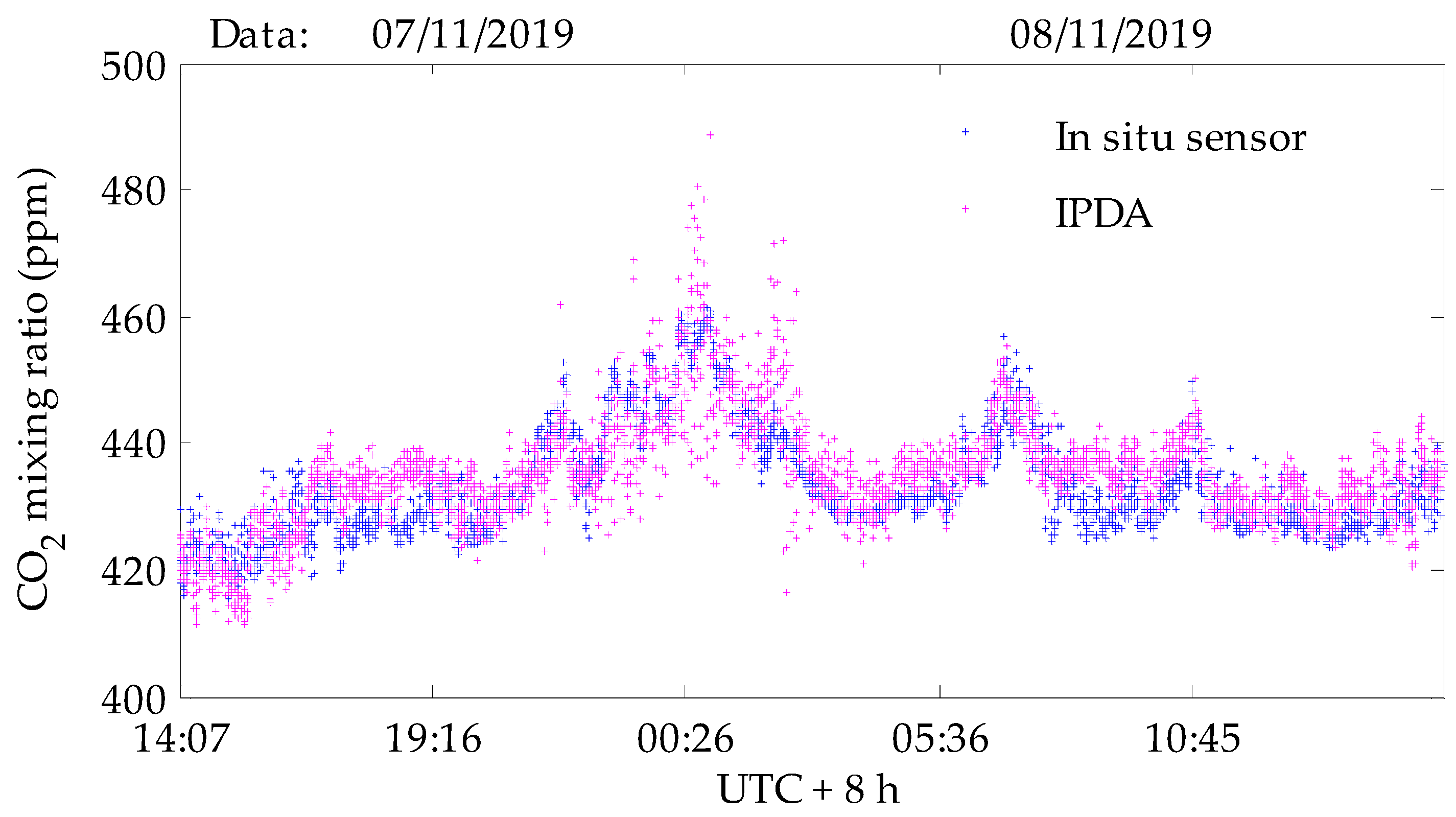
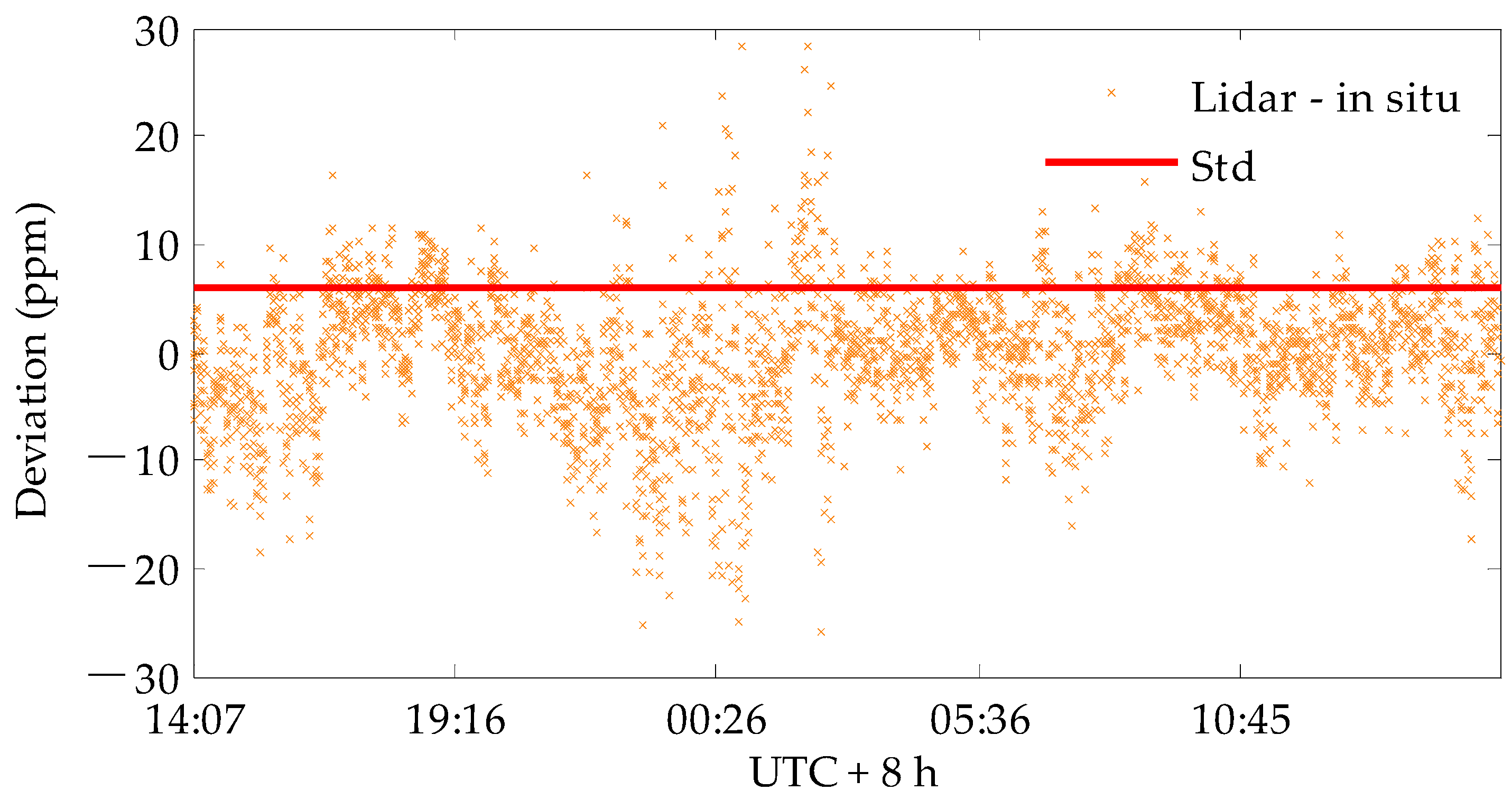
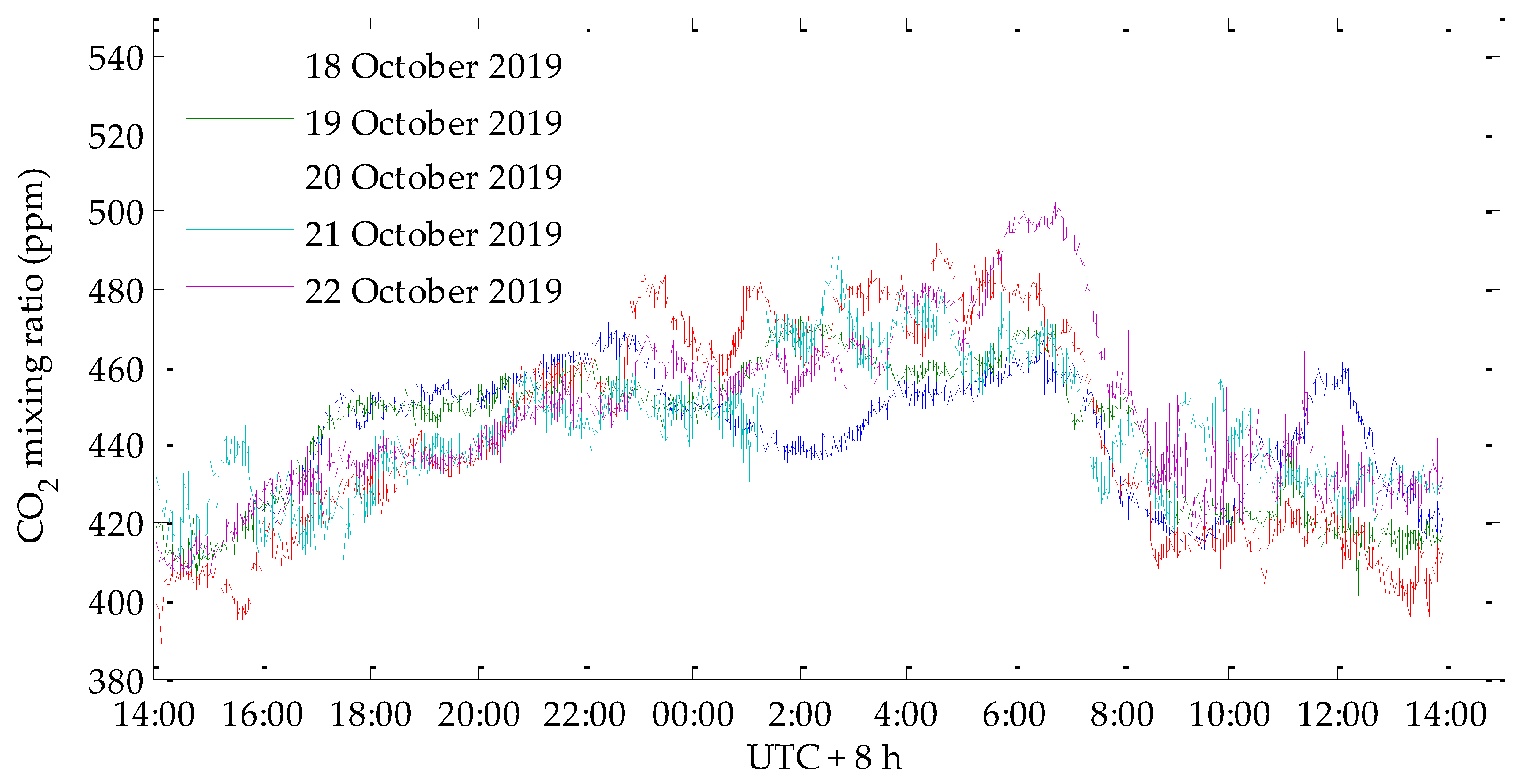
4.2. CO2 Concentration Outcomes
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Houweling, S.; Aben, I.; Breon, F.M.; Chevallier, F.; Deutscher, N.; Engelen, R.; Gerbig, C.; Griffith, D.; Hungershoefer, K.; Macatangay, R.; et al. The importance of transport model uncertainties for the estimation of CO2 sources and sinks using satellite measurements. Atmos. Chem. Phys. 2010, 10, 9981–9992. [Google Scholar] [CrossRef]
- Plattner; GianKasper. IPCC, 2014: Climate Change 2014: Synthesis Report. Contribution of Working Groups I, II and III to the Fifth Assessment Report of the Intergovernmental Panel on Climate Change. J. Roman. Stud. 2014, 4, 85–88. [Google Scholar]
- Miller, C.; Crisp, D.; DeCola, P.; Olsen, S.; Randerson, J.; Michalak, A.; Alkhaled, A.; Rayner, P.; Jacob, D.; Suntharalingam, P.; et al. Precision requirements for space-based XCO2 data. J. Geophys. Res. 2007, 112. [Google Scholar] [CrossRef]
- Refaat, T.; Ismail, S.; Koch, G.; Rubio, M.; Mack, T.; Notari, A.; Collins, J.; Lewis, J.; Young, R.; Choi, Y.; et al. Backscatter 2-μm Lidar Validation for Atmospheric CO2 Differential Absorption Lidar Applications. IEEE Trans. Geosci. Remote Sens. 2011, 49, 572–580. [Google Scholar] [CrossRef]
- Gibert, F.; Flamant, P.H.; Bruneau, D.; Loth, C. Two-micrometer heterodyne differential absorption lidar measurements of the atmospheric CO2 mixing ratio in the boundary layer. Appl. Opt. 2006, 45, 4448–4458. [Google Scholar] [CrossRef]
- Gibert, F.; Pellegrino, J.; Edouart, D.; Cénac, C.; Lombard, L.; Le Gouët, J.; Nuns, T.; Cosentino, A.; Spano, P.; Di Nepi, G. 2-μm double-pulse single-frequency Tm:fiber laser pumped Ho:YLF laser for a space-borne CO2 lidar. Appl. Opt. 2018, 57, 10370–10379. [Google Scholar] [CrossRef]
- Gibert, F.; Edouart, D.; Cénac, C.; Le Mounier, F.; Dumas, A. 2-μm Ho emitter-based coherent DIAL for CO2 profiling in the atmosphere. Opt. Lett. 2015, 40, 3093–3096. [Google Scholar] [CrossRef]
- Kameyama, S.; Imaki, M.; Hirano, Y.; Ueno, S.; Kawakami, S.; Sakaizawa, D.; Kimura, T.; Nakajima, M. Feasibility study on 1.6 μm continuous-wave modulation laser absorption spectrometer system for measurement of global CO2 concentration from a satellite. Appl. Opt. 2011, 50, 2055–2068. [Google Scholar] [CrossRef]
- Amediek, A.; Fix, A.; Wirth, M.; Ehret, G. Development of an OPO system at 1.57 μm for integrated path DIAL measurement of atmospheric carbon dioxide. Appl. Phys. B 2008, 92, 295–302. [Google Scholar] [CrossRef]
- Abshire, J.B.; Ramanathan, A.; Riris, H.; Mao, J.P.; Allan, G.R.; Hasselbrack, W.E.; Weaver, C.J.; Browell, E.V. Airborne Measurements of CO2 Column Concentration and Range Using a Pulsed Direct- Detection IPDA Lidar. Remote Sens. 2014, 6, 443–469. [Google Scholar] [CrossRef]
- Ridley, K.D.; Pearson, G.N.; Harris, M. Improved speckle statistics in coherent differential absorption lidar with in-fiber wavelength multiplexing. Appl. Opt. 2001, 40, 2017–2023. [Google Scholar] [CrossRef] [PubMed]
- Dobler, J.T.; Harrison, F.W.; Browell, E.V.; Lin, B.; McGregor, D.; Kooi, S.; Choi, Y.; Ismail, S. Atmospheric CO2 column measurements with an airborne intensity-modulated continuous wave 1.57 μm fiber laser lidar. Appl. Opt. 2013, 52, 2874–2892. [Google Scholar] [CrossRef] [PubMed]
- Lin, B.; Nehrir, A.R.; Harrison, F.W.; Browell, E.V.; Ismail, S.; Obland, M.D.; Campbell, J.; Dobler, J.; Meadows, B.; Fan, T.F.; et al. Atmospheric CO2 column measurements in cloudy conditions using intensity-modulated continuous-wave lidar at 1.57 micron. Opt. Express 2015, 23, A582–A593. [Google Scholar] [CrossRef] [PubMed]
- Browell, E.V.; Dobler, J.; Kooi, S.A.; Choi, Y.; Harrison, F.W.; Moore, B., III; Zaccheo, T.S. Airborne Validation of Laser Remote Measurements of Atmospheric Carbon Dioxide. In Proceedings of the ILRC25 (25th International Laser Radar Conference), St. Petersburg, Russia, 5–9 July 2010; pp. 779–782. [Google Scholar]
- Browell, E.; Dobler, J.; Kooi, S.; Fenn, M.; Choi, Y.; Vay, S.; Harrison, F.; Moore, B. Airborne laser CO2 column measurements: Evaluation of precision and accuracy under a wide range of surface and atmospheric conditions. In Proceedings of the American Geophysical Union Fall Meeting 2011, San Francisco, CA, USA, 5–9 December 2011. [Google Scholar]
- Browell, E.V.; Dobbs, M.E.; Dobler, J.; Kooi, S.; Moore, B. Airborne demonstration of 1.57-micron laser absorption spectrometer for atmospheric CO2 measurements. In Proceedings of the 24th International Laser Radar Conference, Boulder, CO, USA, 23–27 June 2008. [Google Scholar]
- Kameyama, S.; Imaki, M.; Hirano, Y.; Ueno, S.; Kawakami, S.; Sakaizawa, D.; Nakajima, M. Development of 1.6 μm continuous-wave modulation hard-target differential absorption lidar system for CO2 sensing. Opt. Lett. 2009, 34, 1513–1515. [Google Scholar] [CrossRef]
- Kameyama, S.; Imaki, M.; Hirano, Y.; Ueno, S.; Kawakami, S.; Sakaizawa, D.; Nakajima, M. Performance improvement and analysis of a 1.6 μm continuous-wave modulation laser absorption spectrometer system for CO2 sensing. Appl. Opt. 2011, 50, 1560–1569. [Google Scholar] [CrossRef]
- Gordon, I.E.; Rothman, L.S.; Hill, C.; Kochanov, R.V.; Tan, Y.; Bernath, P.F.; Birk, M.; Boudon, V.; Campargue, A.; Chance, K.V.; et al. The HITRAN2016 molecular spectroscopic database. J. Quant. Spectrosc. Radiat. Transf. 2017, 203, 3–69. [Google Scholar] [CrossRef]
- Liu, H.; Chen, T.; Shu, R.; Hong, G.; Zheng, L.; Ge, Y.; Hu, Y. Wavelength-locking-free 1.57 µm differential absorption lidar for CO2 sensing. Opt. Express 2014, 22, 27675–27680. [Google Scholar] [CrossRef]
- Cassé; Gibert; Edouart; Chomette; Crevoisier. Optical Energy Variability Induced by Speckle: The Cases of MERLIN and CHARM-F IPDA Lidar. Atmosphere 2019, 10, 540. [Google Scholar] [CrossRef]
- Refaat, T.F.; Singh, U.N.; Yu, J.; Petros, M.; Remus, R.; Ismail, S. Double-pulse 2-μm integrated path differential absorption lidar airborne validation for atmospheric carbon dioxide measurement. Appl. Opt. 2016, 55, 4232–4246. [Google Scholar] [CrossRef]
- Du, J.; Zhu, Y.; Li, S.; Zhang, J.; Sun, Y.; Zang, H.; Liu, D.; Ma, X.; Bi, D.; Liu, J.; et al. Double-pulse 1.57 μm integrated path differential absorption lidar ground validation for atmospheric carbon dioxide measurement. Appl. Opt. 2017, 56, 7053–7058. [Google Scholar] [CrossRef] [PubMed]
- Sakaizawa, D.; Kawakami, S.; Nakajima, M.; Tanaka, T.; Morino, I.; Uchino, O. An airborne amplitude-modulated 1.57 μm differential laser absorption spectrometer: Simultaneous measurement of partial column-averaged dry air mixing ratio of CO2 and target range. Atmos. Meas. Tech. 2013, 6, 387–396. [Google Scholar] [CrossRef]
- Freund, I.; Joseph, W. Goodman: Speckle Phenomena in Optics: Theory and Applications. J. Stat. Phys. 2008, 130, 413–414. [Google Scholar] [CrossRef]
- Zhang, G.; Wu, Z. Two-frequency mutual coherence function of scattering from arbitrarily shaped rough objects. Opt. Express 2011, 19, 7007–7019. [Google Scholar] [CrossRef]








| Transmitter | |
| Seed Laser Type | DFB Diode Laser |
| Wavelength | : 1572.335 nm; : 1572.205 nm; :1572.480 nm |
| Laser linewidth (FWHM) | <1 MHz each wavelength |
| Wavelength stability | ON: 0.05 pm (rms); OFF: 0.4 pm (rms) |
| Spectral purity | >99.9% |
| Modulation type | IM-CW |
| Modulation frequency | ON: 99.733 KHz; OFF: 101.833 KHz |
| Optical amplifier | EYDFA-HP-BA-33C-211B |
| Power output (max) | 33 dBm |
| Laser divergence angle | 0.57 mrad (half angle) |
| Receiver | |
| Receiver diameter | 150 mm |
| Field of view | 1mrad |
| Detector | InGaAs-PIN |
| Transmitting and receiving axis | Coaxial |
| Distinguish ON/OFF | FFT |
| Motor Speed | Correlation | 1s-Smoothed Correlation | ||||
|---|---|---|---|---|---|---|
| Stationary | 0.9214 | 0.9361 | 0.9980 | 0.0013 | 0.9980 | 0.00086 |
| 60 rpm | 0.9847 | 0.9963 | 0.9971 | 0.00074 | 0.9971 | 0.00031 |
| 200 rpm | 0.9937 | 0.9982 | 0.9969 | 0.00073 | 0.9969 | 0.00023 |
| 600 rpm | 0.9971 | 0.9997 | 0.9971 | 0.00071 | 0.9971 | 0.00014 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liang, X.; Liu, H.; Chen, T.; Kong, W.; Hong, G. Calibration and Improved Speckle Statistics of IM-CW Lidar for Atmospheric CO2 Measurements. Atmosphere 2020, 11, 737. https://doi.org/10.3390/atmos11070737
Liang X, Liu H, Chen T, Kong W, Hong G. Calibration and Improved Speckle Statistics of IM-CW Lidar for Atmospheric CO2 Measurements. Atmosphere. 2020; 11(7):737. https://doi.org/10.3390/atmos11070737
Chicago/Turabian StyleLiang, Xindong, Hao Liu, Tao Chen, Wei Kong, and Guanglie Hong. 2020. "Calibration and Improved Speckle Statistics of IM-CW Lidar for Atmospheric CO2 Measurements" Atmosphere 11, no. 7: 737. https://doi.org/10.3390/atmos11070737
APA StyleLiang, X., Liu, H., Chen, T., Kong, W., & Hong, G. (2020). Calibration and Improved Speckle Statistics of IM-CW Lidar for Atmospheric CO2 Measurements. Atmosphere, 11(7), 737. https://doi.org/10.3390/atmos11070737





