The Spiderweb Structure of Stratocumulus Clouds
Abstract
:1. Introduction
2. Methodology
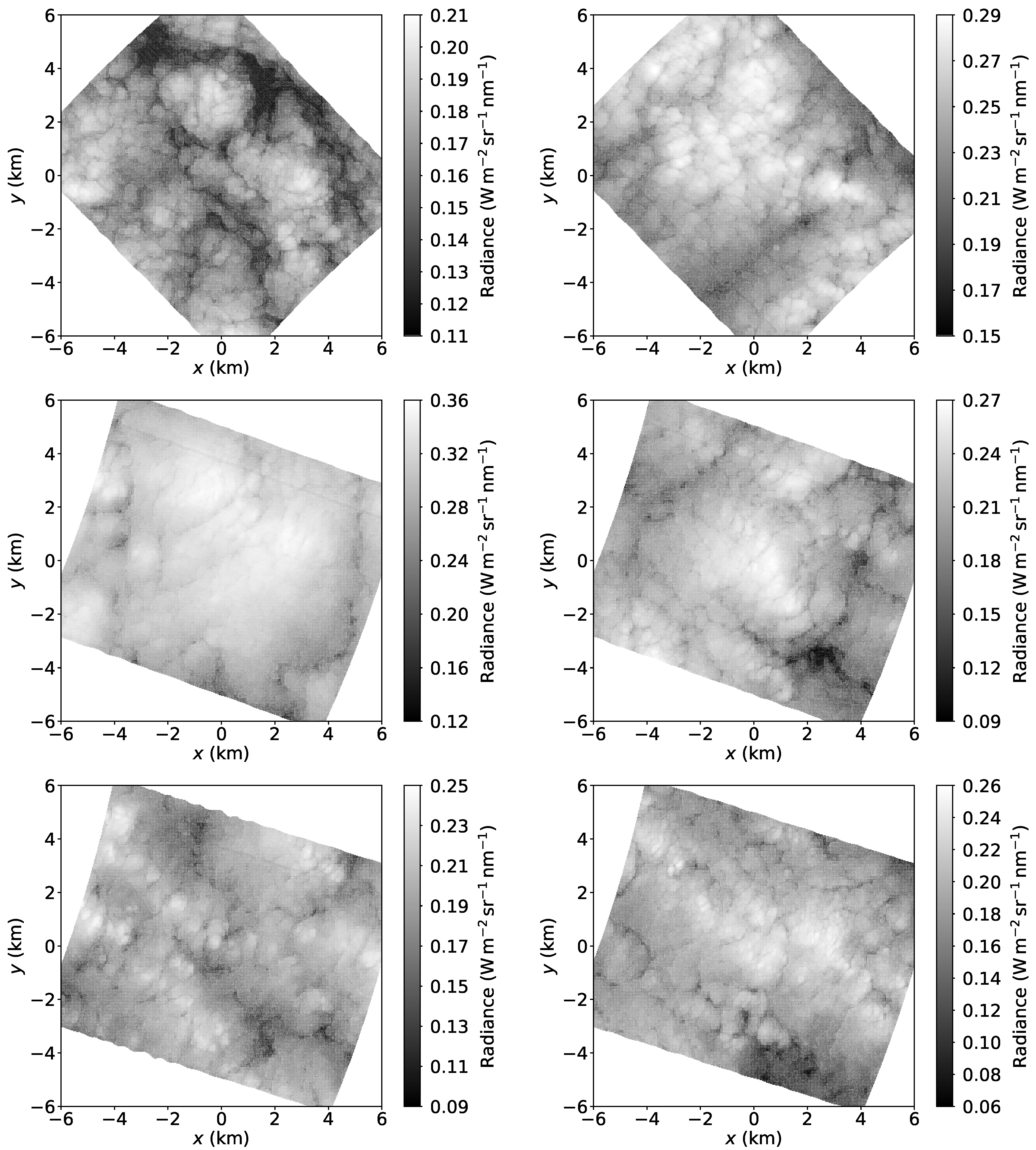
2.1. Observations
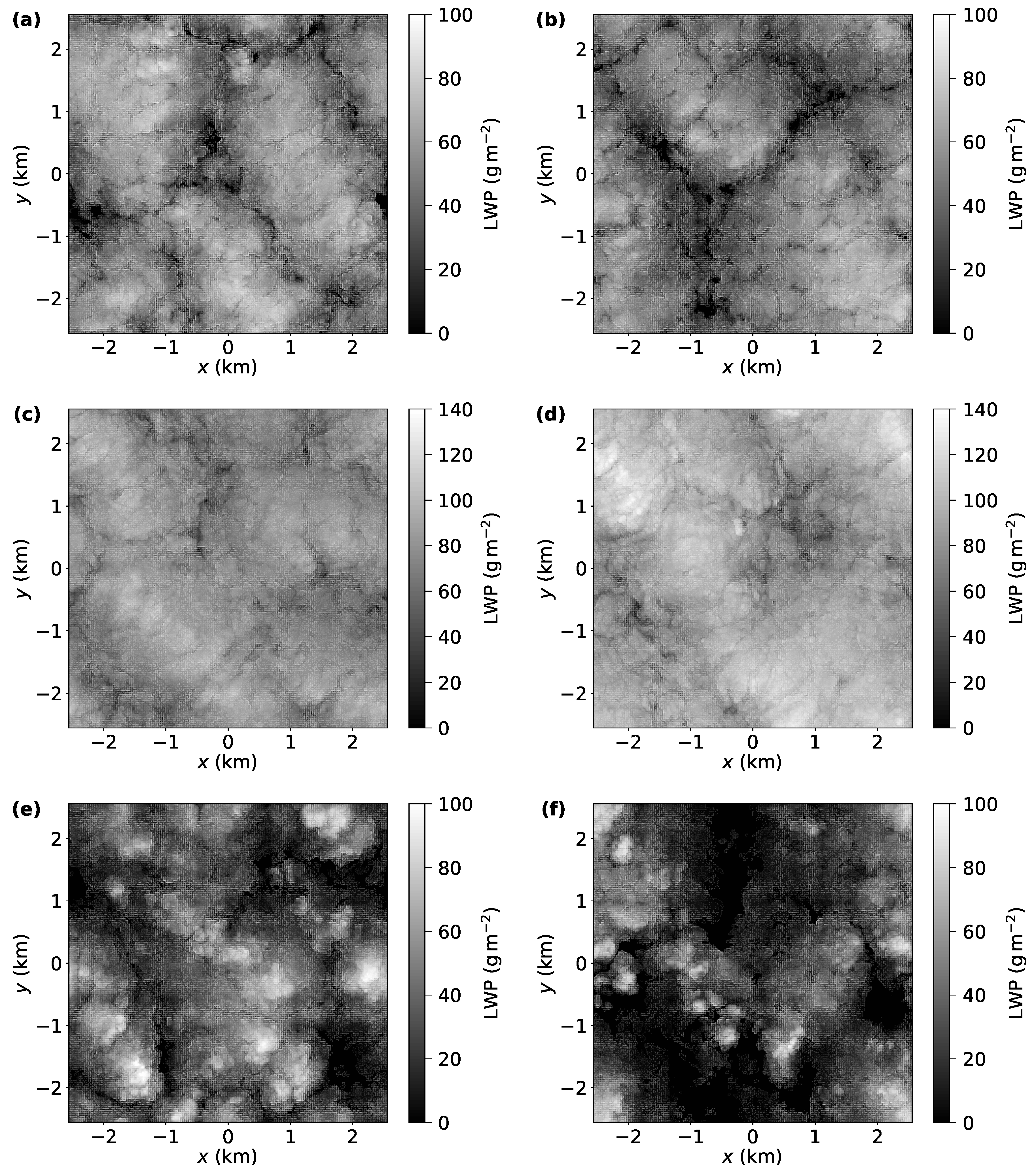
2.2. Model
3. Results
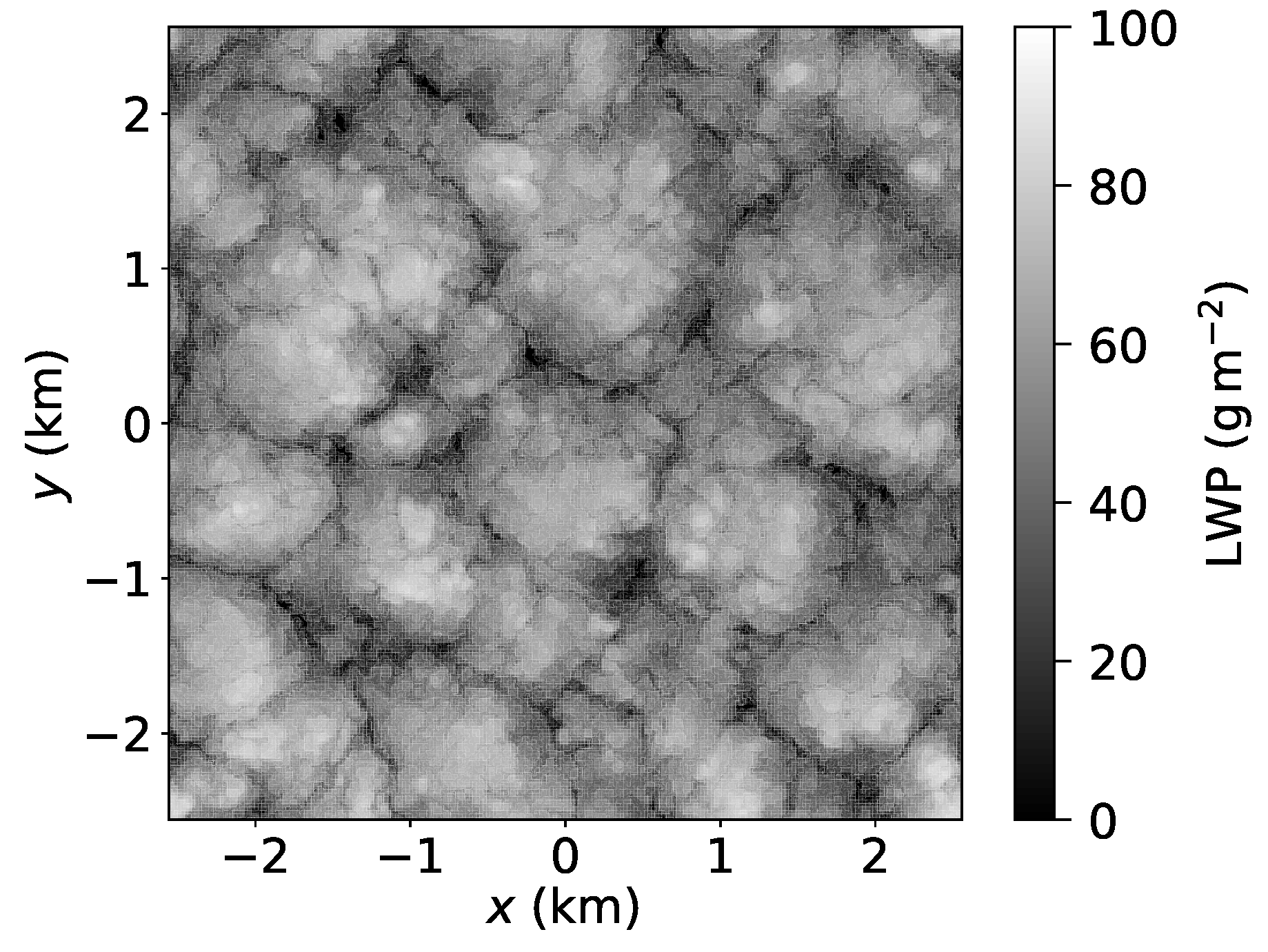
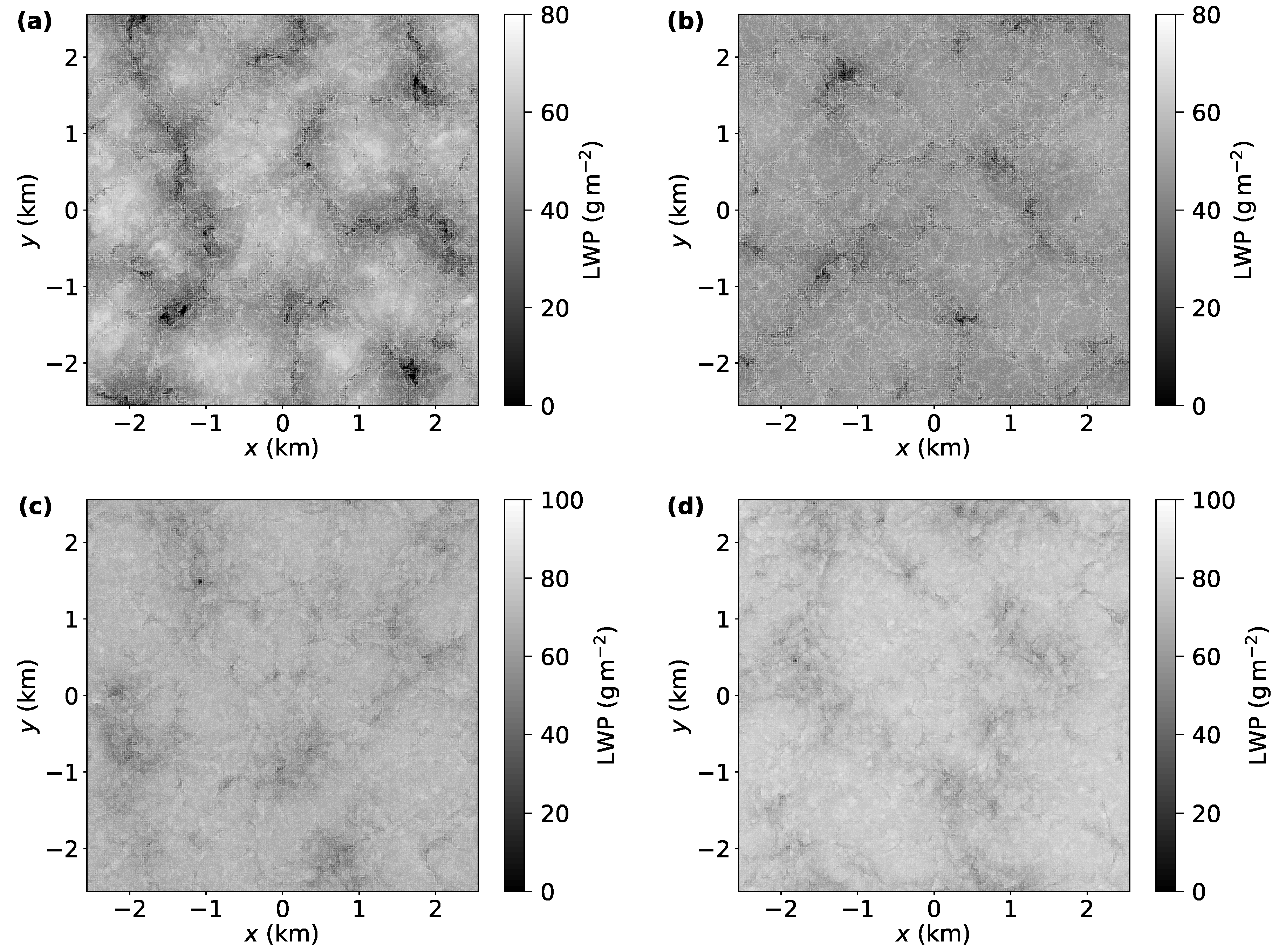
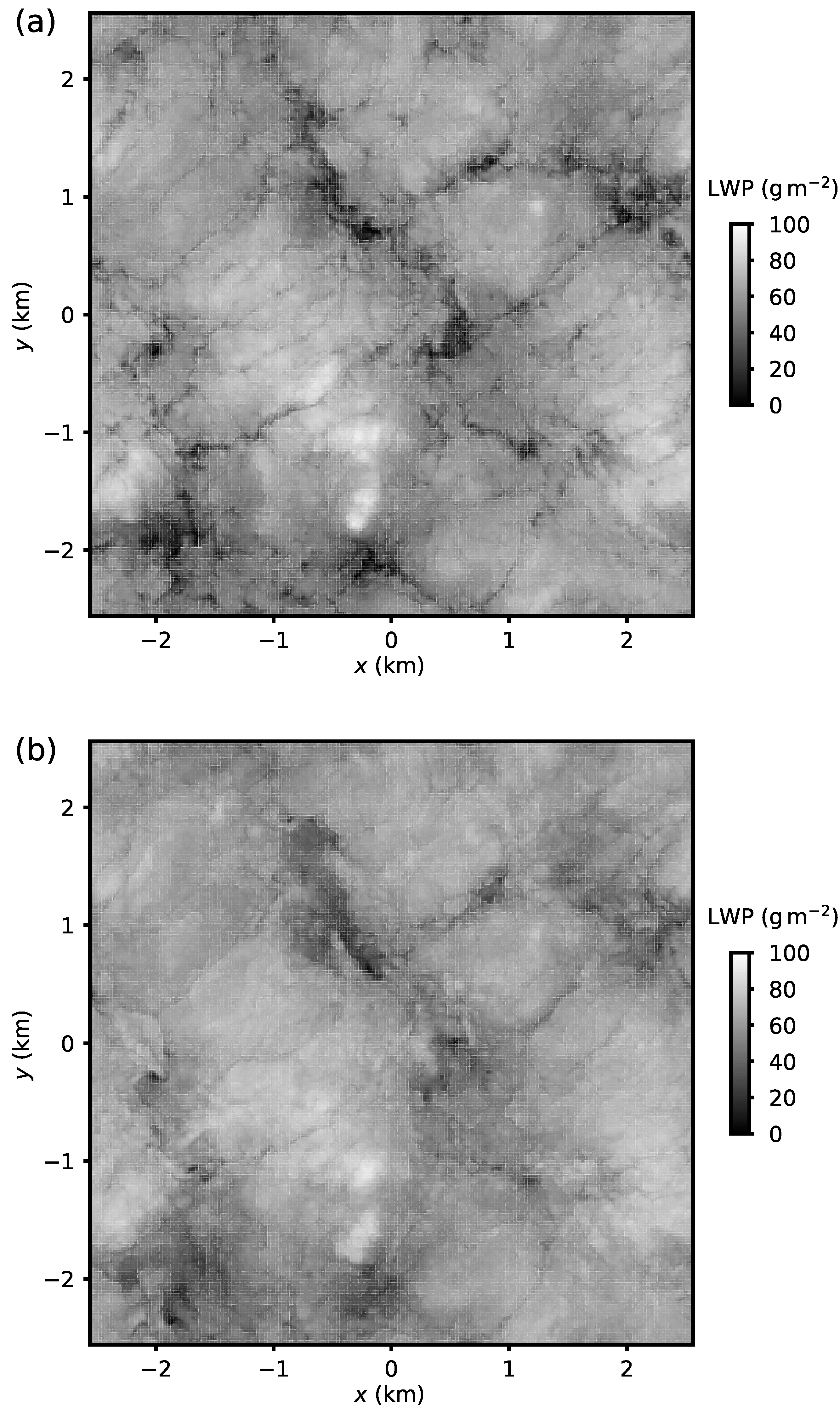
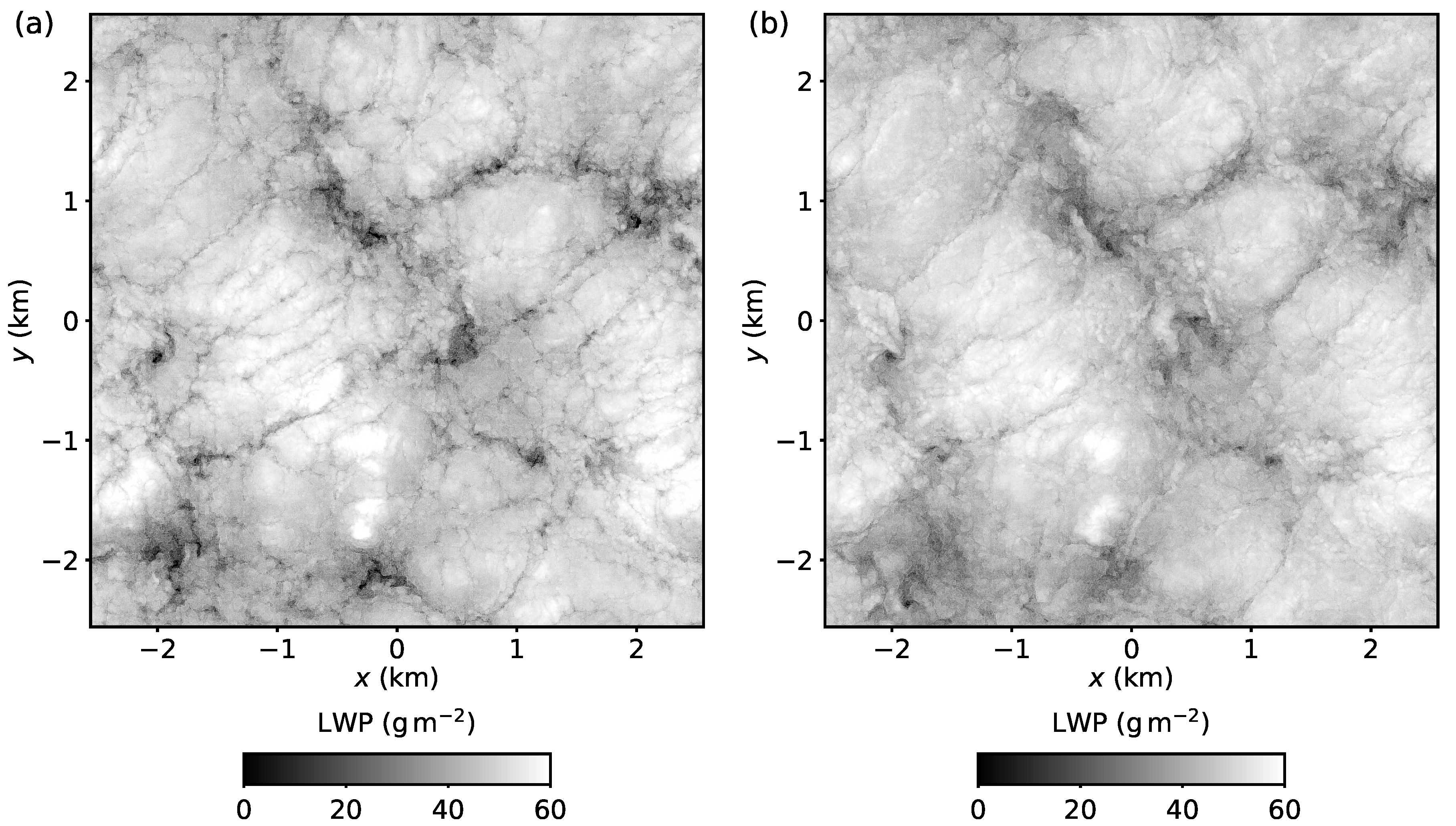
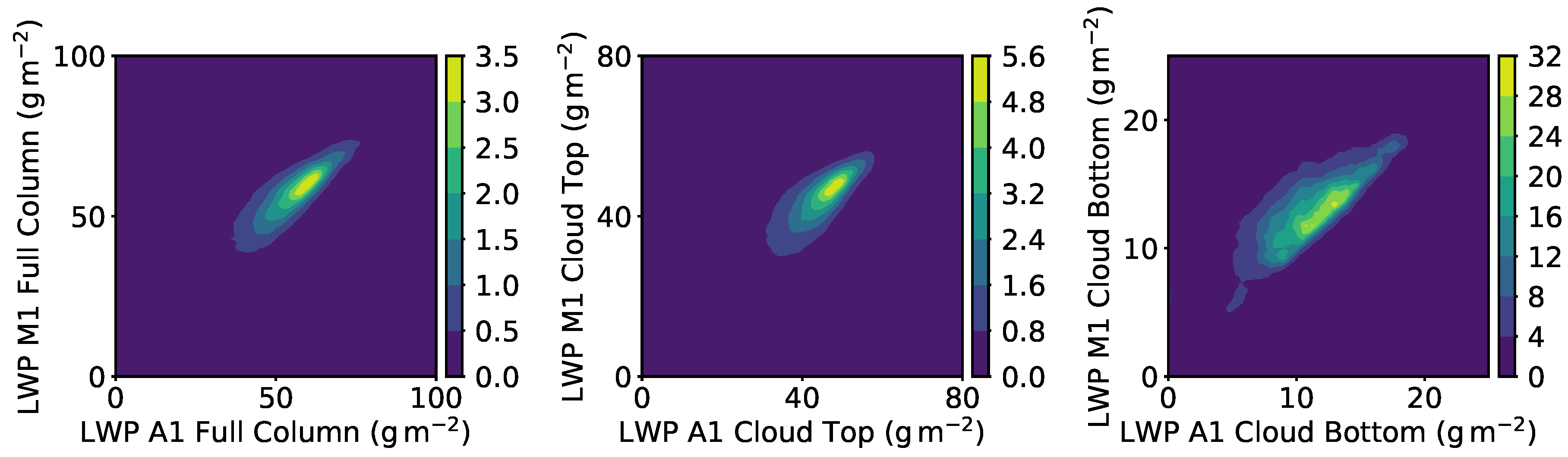
3.1. Liquid Water Path Spatial Structure
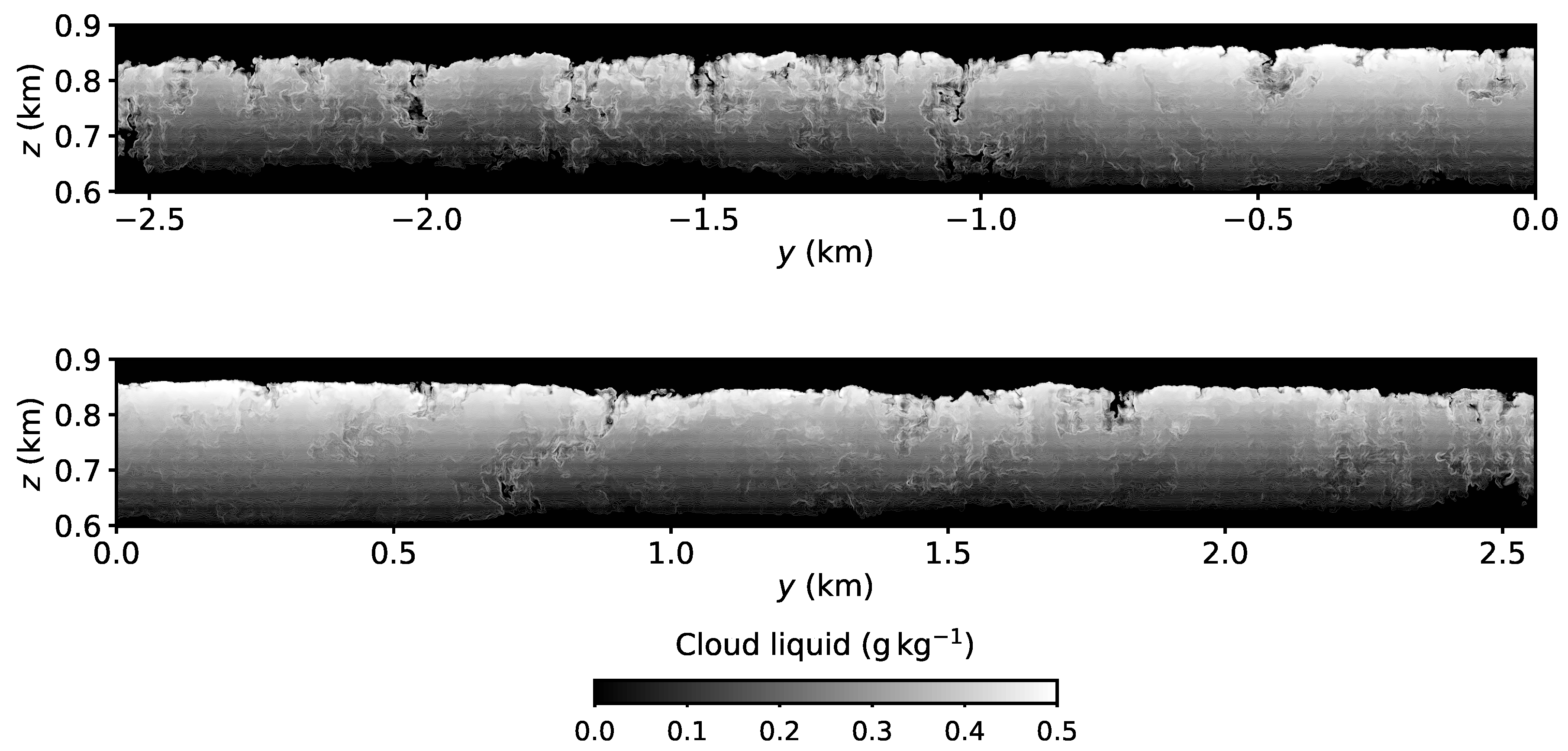
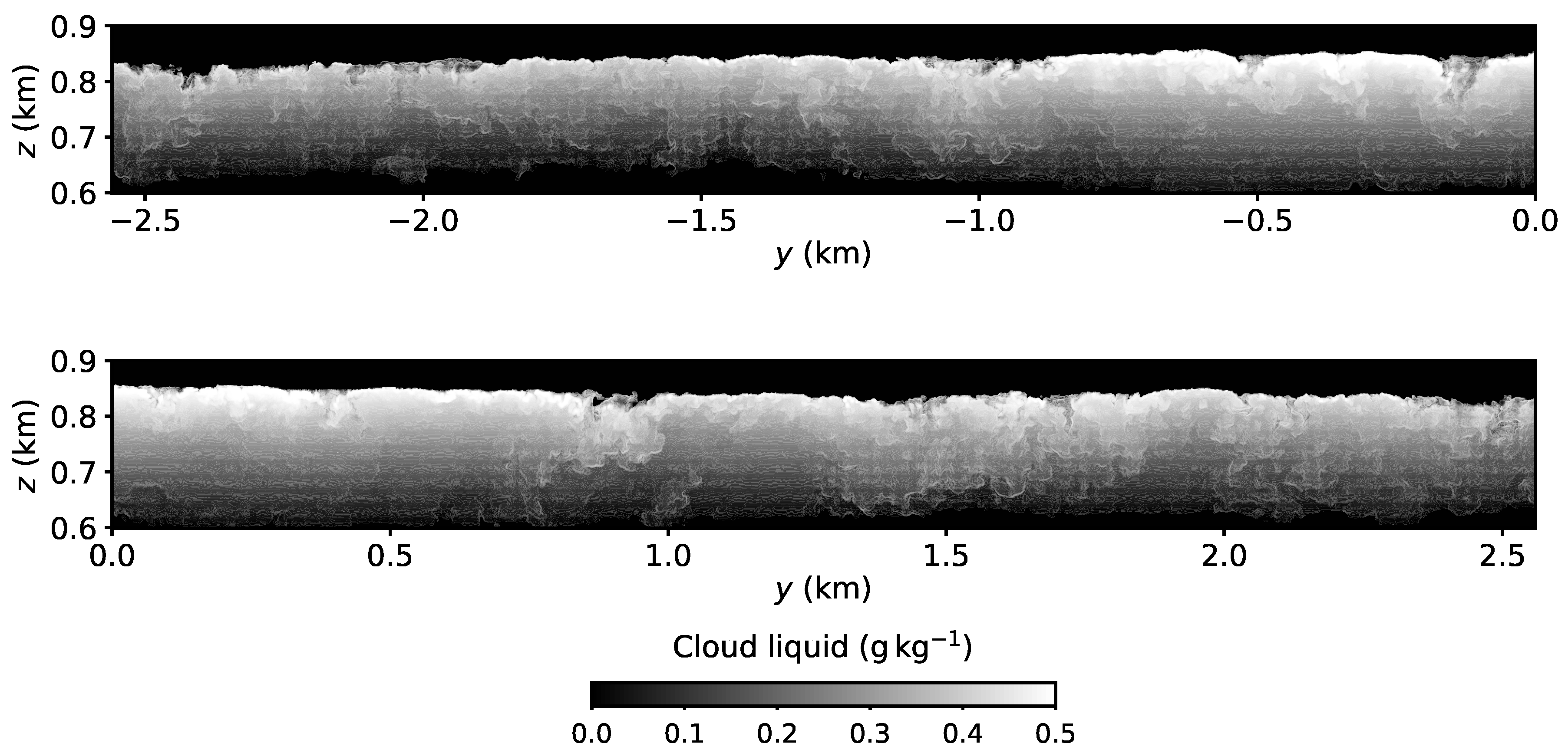
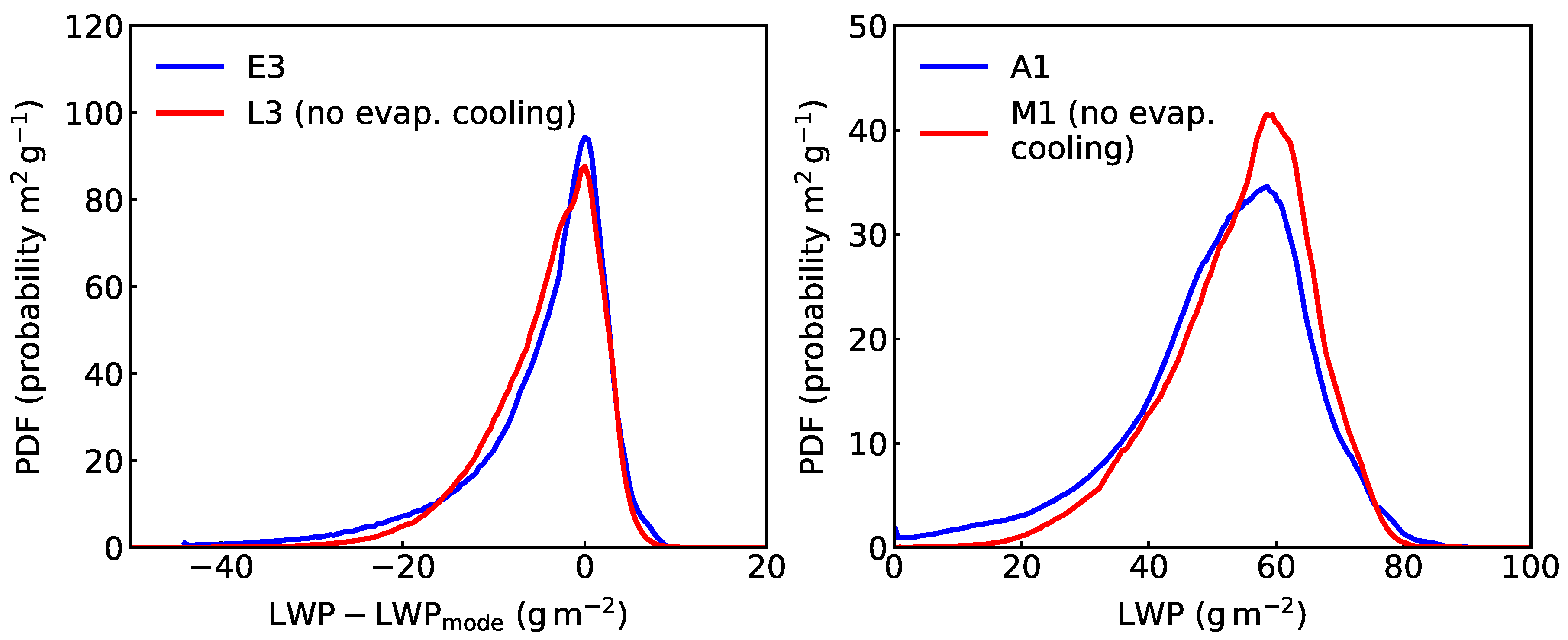
3.2. Cloud Liquid and LWP Distributions
3.3. Entrainment Rate and Turbulence
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Hartmann, D.L.; Ockert-Bell, M.E.; Michelsen, M.L. The effect of cloud type on Earth’s energy balance: Global analysis. J. Clim. 1992, 5, 1281–1304. [Google Scholar] [CrossRef] [Green Version]
- Bretherton, C.S. Convection in stratocumulus-topped atmospheric boundary layers. In The Physics and Parameterization of Moist Atmospheric Convection; Springer: Berlin, Germany, 1997; pp. 127–142. [Google Scholar]
- Stevens, B. Atmospheric moist convection. Annu. Rev. Earth Planet. Sci. 2005, 33, 605–643. [Google Scholar] [CrossRef]
- Wood, R. Stratocumulus clouds. Mon. Weather Rev. 2012, 140, 2373–2423. [Google Scholar] [CrossRef]
- Haman, K.E. Simple approach to dynamics of entrainment interface layers and cloud holes in stratocumulus clouds. Q. J. R. Meteorol. Soc. 2009, 135, 93–100. [Google Scholar] [CrossRef]
- Xu, F.; van Harten, G.; Diner, D.J.; Davis, A.B.; Seidel, F.C.; Rheingans, B.; Tosca, M.; Alexandrov, M.D.; Cairns, B.; Ferrare, R.A.; et al. Coupled retrieval of liquid water cloud and above-cloud aerosol properties using the Airborne Multiangle SpectroPolarimetric Imager (AirMSPI). J. Geophys. Res. 2018, 123, 3175–3204. [Google Scholar] [CrossRef]
- Yamaguchi, T.; Randall, D.A. Cooling of entrained parcels in a large-eddy simulation. J. Atmos. Sci. 2012, 69, 1118–1136. [Google Scholar] [CrossRef]
- Yamaguchi, T.; Feingold, G. On the size distribution of cloud holes in stratocumulus and their relationship to cloud-top entrainment. Geophys. Res. Lett. 2013, 40, 2450–2454. [Google Scholar] [CrossRef]
- Matheou, G. Turbulence structure in a stratocumulus cloud. Atmosphere 2018, 9, 392. [Google Scholar] [CrossRef] [Green Version]
- Nicholls, S. The structure of radiatively driven convection in stratocumulus. Q. J. R. Meteorol. Soc. 1989, 115, 487–511. [Google Scholar] [CrossRef]
- Gerber, H.; Frick, G.; Malinowski, S.P.; Brenguier, J.L.; Burnet, F. Holes and entrainment in stratocumulus. J. Atmos. Sci. 2005, 62, 443–459. [Google Scholar] [CrossRef] [Green Version]
- Haman, K.E.; Malinowski, S.P.; Kurowski, M.J.; Gerber, H.; Brenguier, J.L. Small scale mixing processes at the top of a marine stratocumulus–A case study. Q. J. R. Meteorol. Soc. 2007, 133, 213–226. [Google Scholar] [CrossRef]
- Karpińska, K.; Bodenschatz, J.F.; Malinowski, S.P.; Nowak, J.L.; Risius, S.; Schmeissner, T.; Shaw, R.A.; Siebert, H.; Xi, H.; Xu, H.; et al. Turbulence-induced cloud voids: Observation and interpretation. Atmos. Chem. Phys. 2019, 19, 4991–5003. [Google Scholar] [CrossRef] [Green Version]
- Schmidt, H.; Schumann, U. Coherent structure of the convective boundary layer derived from large-eddy simulations. J. Fluid Mech. 1989, 200, 511–562. [Google Scholar] [CrossRef] [Green Version]
- Sullivan, P.G.; Patton, E.G. The effect of mesh resolution on convective boundary layer statistics and structures generated by large-eddy simulation. J. Atmos. Sci. 2011, 68, 2395–2415. [Google Scholar] [CrossRef]
- Mellado, J.P. Cloud-top entrainment in stratocumulus clouds. Annu. Rev. Fluid Mech. 2017, 49, 145–169. [Google Scholar] [CrossRef]
- Mellado, J.P.; Stevens, B.; Schmidt, H. Wind shear and buoyancy reversal at the top of stratocumulus. J. Atmos. Sci. 2014, 71, 1040–1057. [Google Scholar] [CrossRef]
- Davis, A.B.; Marshak, A.; Gerber, H.; Wiscombe, W.J. Horizontal structure of marine boundary layer clouds from centimeter to kilometer scales. J. Geophys. Res. Atmos. 1999, 104, 6123–6144. [Google Scholar] [CrossRef]
- Ma, Y.F.; Malinowski, S.P.; Karpińska, K.; Gerber, H.E.; Kumala, W. Scaling analysis of temperature and liquid water content in the marine boundary layer clouds during POST. J. Atmos. Sci. 2017, 74, 4075–4092. [Google Scholar] [CrossRef]
- Van Zanten, M.C.; Duynkerke, P.G. Radiative and evaporative cooling in the entrainment zone of stratocumulus – The role of longwave radiative cooling above cloud top. Bound.-Layer Meteorol. 2002, 102, 253–280. [Google Scholar] [CrossRef]
- Malinowski, S.P.; Andrejczuk, M.; Grabowski, W.W.; Korczyk, P.; Kowalewski, T.A.; Smolarkiewicz, P.K. Laboratory and modeling studies of cloud–clear air interfacial mixing: Anisotropy of small-scale turbulence due to evaporative cooling. New J. Phys. 2008, 10, 075020. [Google Scholar] [CrossRef] [Green Version]
- Petters, J.L.; Harrington, J.Y.; Clothiaux, E.E. Radiative–dynamical feedbacks in low liquid water path stratiform clouds. J. Atmos. Sci. 2012, 69, 1498–1512. [Google Scholar] [CrossRef]
- De Lozar, A.; Mellado, J.P. Evaporative cooling amplification of the entrainment velocity in radiatively driven stratocumulus. Geophys. Res. Lett. 2015, 42, 7223–7229. [Google Scholar] [CrossRef] [Green Version]
- Matheou, G.; Teixeira, J. Sensitivity to physical and numerical aspects of large-eddy simulation of stratocumulus. Mon. Weather Rev. 2019, 147, 2621–2639. [Google Scholar] [CrossRef]
- Stevens, B.; Lenschow, D.H.; Vali, G.; Gerber, H.; Bandy, A.; Blomquist, B.; Brenguier, J.; Bretherton, C.; Burnet, F.; Campos, T.; et al. Dynamics and chemistry of marine stratocumulus – DYCOMS-II. Bull. Am. Meteor. Soc. 2003, 84, 579–593. [Google Scholar] [CrossRef]
- Diner, D.J.; Xu, F.; Garay, M.J.; Martonchik, J.V.; Rheingans, B.E.; Geier, S.; Davis, A.; Hancock, B.R.; Jovanovic, V.M.; Bull, M.A.; et al. The Airborne Multiangle SpectroPolarimetric Imager (AirMSPI): A new tool for aerosol and cloud remote sensing. Atmos. Meas. Tech. 2013, 6, 2007. [Google Scholar] [CrossRef] [Green Version]
- Zuidema, P.; Redemann, J.; Haywood, J.; Wood, R.; Piketh, S.; Hipondoka, M.; Formenti, P. Smoke and clouds above the southeast Atlantic: Upcoming field campaigns probe absorbing aerosol’s impact on climate. Bull. Am. Meteor. Soc. 2016, 97, 1131–1135. [Google Scholar] [CrossRef] [Green Version]
- Matheou, G.; Chung, D. Large-eddy simulation of stratified turbulence. Part II: Application of the stretched-vortex model to the atmospheric boundary layer. J. Atmos. Sci. 2014, 71, 4439–4460. [Google Scholar] [CrossRef]
- Ogura, Y.; Phillips, N.A. Scale analysis of deep and shallow convection in the atmosphere. J. Atmos. Sci. 1962, 19, 173–179. [Google Scholar] [CrossRef] [Green Version]
- Morinishi, Y.; Lund, T.S.; Vasilyev, O.V.; Moin, P. Fully conservative higher order finite difference schemes for incompressible flow. J. Comput. Phys. 1998, 143, 90–124. [Google Scholar] [CrossRef] [Green Version]
- Matheou, G.; Dimotakis, P.E. Scalar excursions in large-eddy simulations. J. Comput. Phys. 2016, 327, 97–120. [Google Scholar] [CrossRef] [Green Version]
- Leonard, B.P. A stable and accurate convective modelling procedure based on quadratic upstream interpolation. Comput. Methods Appl. Mech. Eng. 1979, 19, 59–98. [Google Scholar] [CrossRef]
- Lundgren, T.S. Strained spiral vortex model for turbulent fine structure. Phys. Fluids 1982, 25, 2193–2203. [Google Scholar] [CrossRef]
- Misra, A.; Pullin, D.I. A vortex-based subgrid stress model for large-eddy simulation. Phys. Fluids 1997, 9, 2443–2454. [Google Scholar] [CrossRef]
- Pullin, D.I. A vortex-based model for the subgrid flux of a passive scalar. Phys. Fluids 2000, 12, 2311–2316. [Google Scholar] [CrossRef] [Green Version]
- Voelkl, T.; Pullin, D.I.; Chan, D.C. A physical-space version of the stretched-vortex subgrid-stress model for large-eddy simulation. Phys. Fluids 2000, 12, 1810–1825. [Google Scholar] [CrossRef] [Green Version]
- Chung, D.; Matheou, G. Large-eddy simulation of stratified turbulence. Part I: A vortex-based subgrid-scale model. J. Atmos. Sci. 2014, 71, 1863–1879. [Google Scholar] [CrossRef]
- Spalart, P.R.; Moser, R.D.; Rogers, M.M. Spectral methods for the Navier–Stokes equations with one infinite and two periodic directions. J. Comput. Phys. 1991, 96, 297–324. [Google Scholar] [CrossRef]
- Stevens, B.; Moeng, C.H.; Ackerman, A.S.; Bretherton, C.S.; Chlond, A.; De Roode, S.; Edwards, J.; Golaz, J.C.; Jiang, H.L.; Khairoutdinov, M.; et al. Evaluation of large-eddy simulations via observations of nocturnal marine stratocumulus. Mon. Weather Rev. 2005, 133, 1443–1462. [Google Scholar] [CrossRef] [Green Version]
- Mellado, J.P. The evaporatively driven cloud-top mixing layer. J. Fluid. Mech. 2010, 660, 5–36. [Google Scholar] [CrossRef]
- De Lozar, A.; Mellado, J.P. Mixing driven by radiative and evaporative cooling at the stratocumulus top. J. Atmos. Sci. 2015, 72, 4681–4700. [Google Scholar] [CrossRef]


| Run | Wind | Radiation | Buoyancy | Surface | |||||
|---|---|---|---|---|---|---|---|---|---|
| Fluxes | |||||||||
| A1 | 1.25 | 5.12 | 4096 | 1200 | 3.75 | Yes | multi-phase | prescribed | |
| A3 | 5 | 5.12 | 1024 | 300 | 3.75 | Yes | multi-phase | prescribed | |
| B3 | 5 | 5.12 | 1024 | 300 | 3.75 | No | multi-phase | prescribed | |
| C3 | 5 | 5.12 | 1024 | 300 | 3.75 | Yes | modified | prescribed | |
| E3 | 5 | 5.12 | 1024 | 300 | 0 | 0 | Yes | multi-phase | |
| L3 | 5 | 5.12 | 1024 | 300 | 0 | 0 | Yes | modified | |
| M1 | 1.25 | 5.12 | 4096 | 1200 | 3.75 | Yes | modified | prescribed |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Matheou, G.; Davis, A.B.; Teixeira, J. The Spiderweb Structure of Stratocumulus Clouds. Atmosphere 2020, 11, 730. https://doi.org/10.3390/atmos11070730
Matheou G, Davis AB, Teixeira J. The Spiderweb Structure of Stratocumulus Clouds. Atmosphere. 2020; 11(7):730. https://doi.org/10.3390/atmos11070730
Chicago/Turabian StyleMatheou, Georgios, Anthony B. Davis, and João Teixeira. 2020. "The Spiderweb Structure of Stratocumulus Clouds" Atmosphere 11, no. 7: 730. https://doi.org/10.3390/atmos11070730
APA StyleMatheou, G., Davis, A. B., & Teixeira, J. (2020). The Spiderweb Structure of Stratocumulus Clouds. Atmosphere, 11(7), 730. https://doi.org/10.3390/atmos11070730





