Scale-Dependent Turbulent Dynamics and Phase-Space Behavior of the Stable Atmospheric Boundary Layer
Abstract
1. Introduction
2. The CASES-99 Data-Set
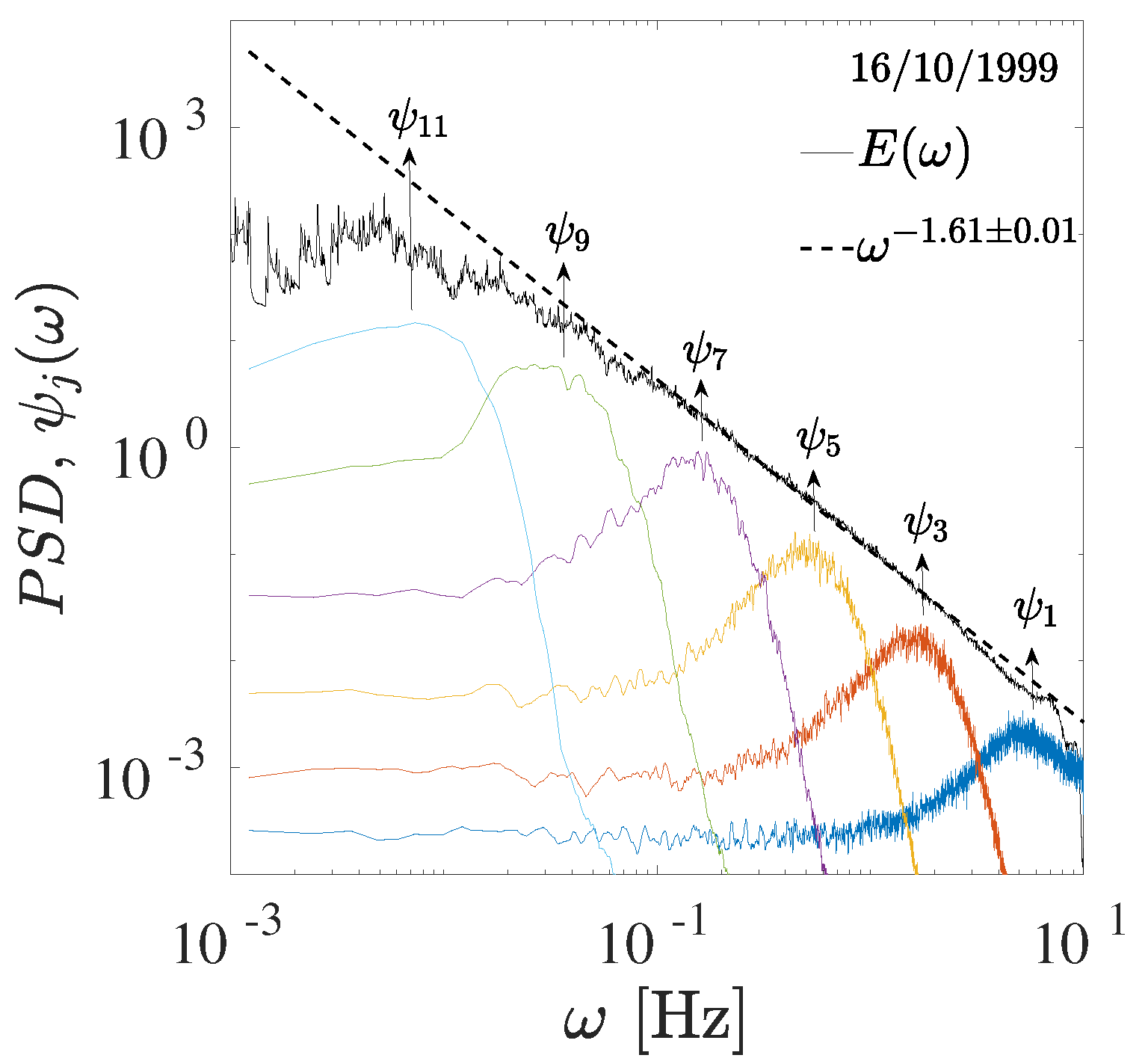
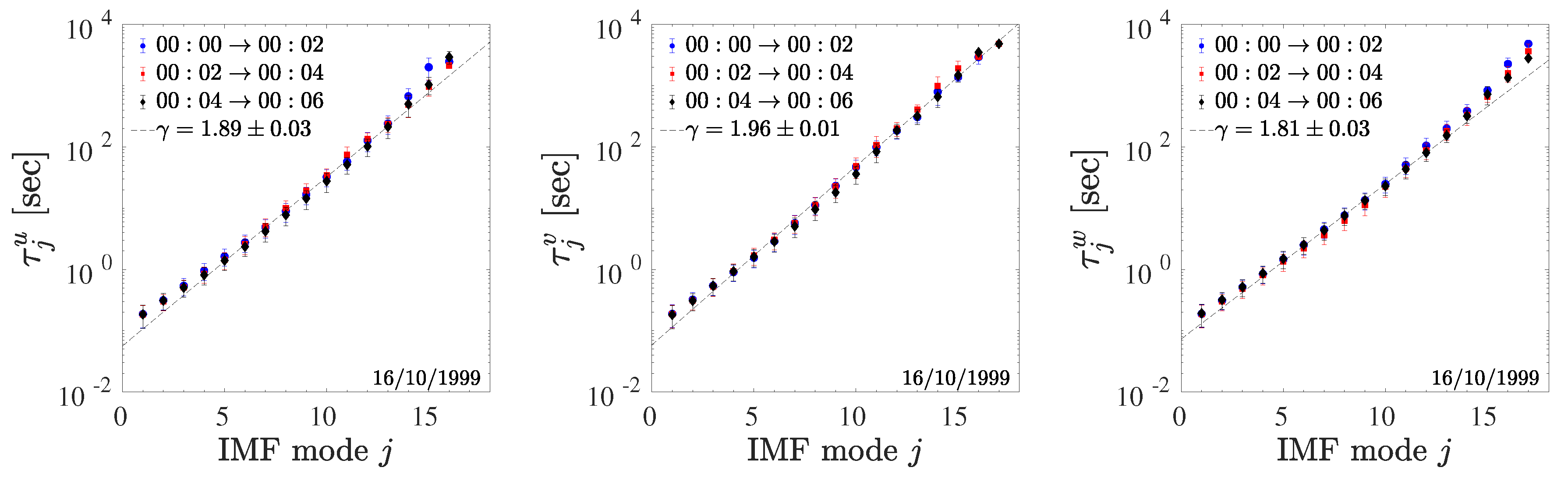
3. Empirical Mode Decomposition of SBL Turbulent Fluctuations
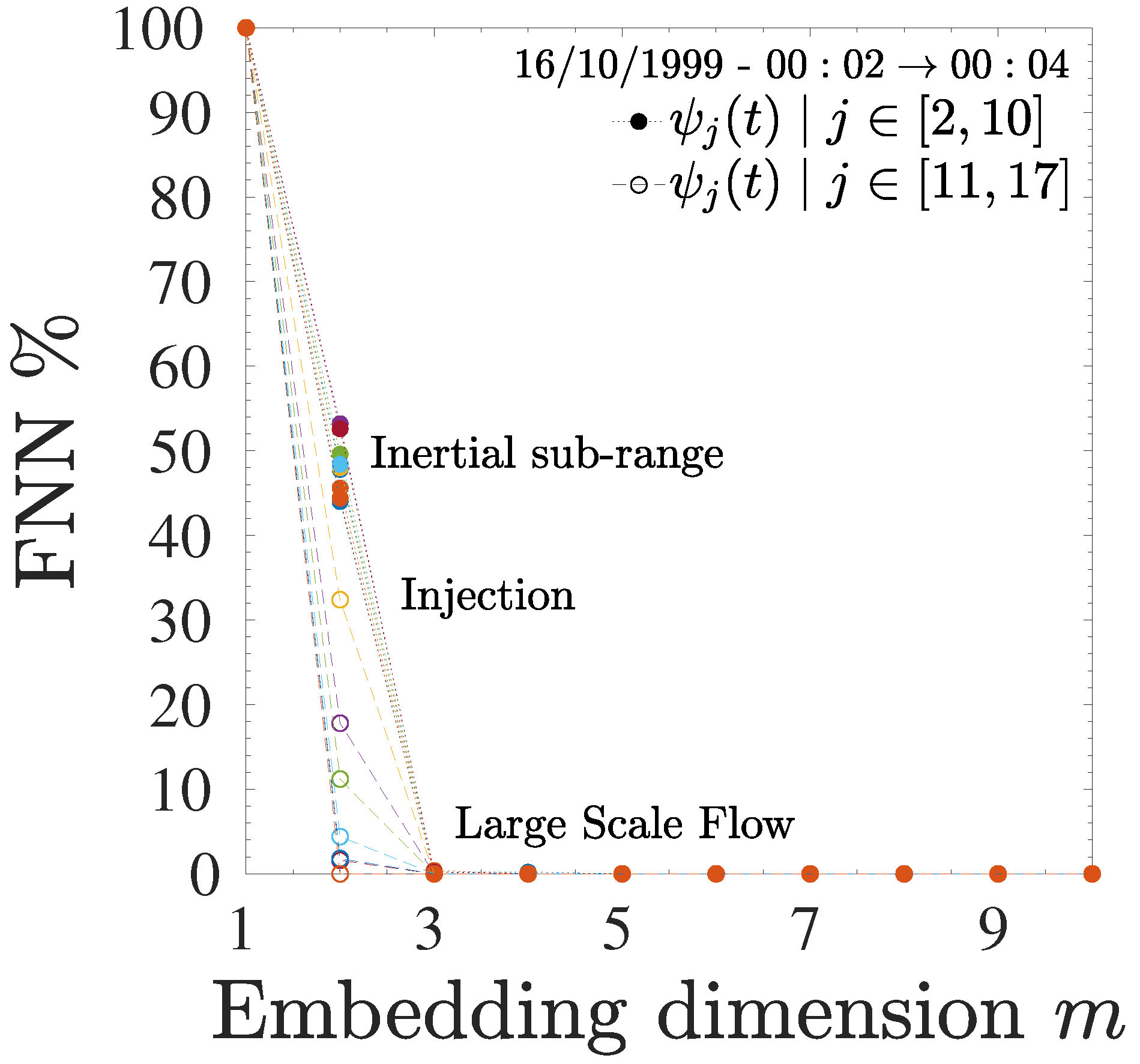
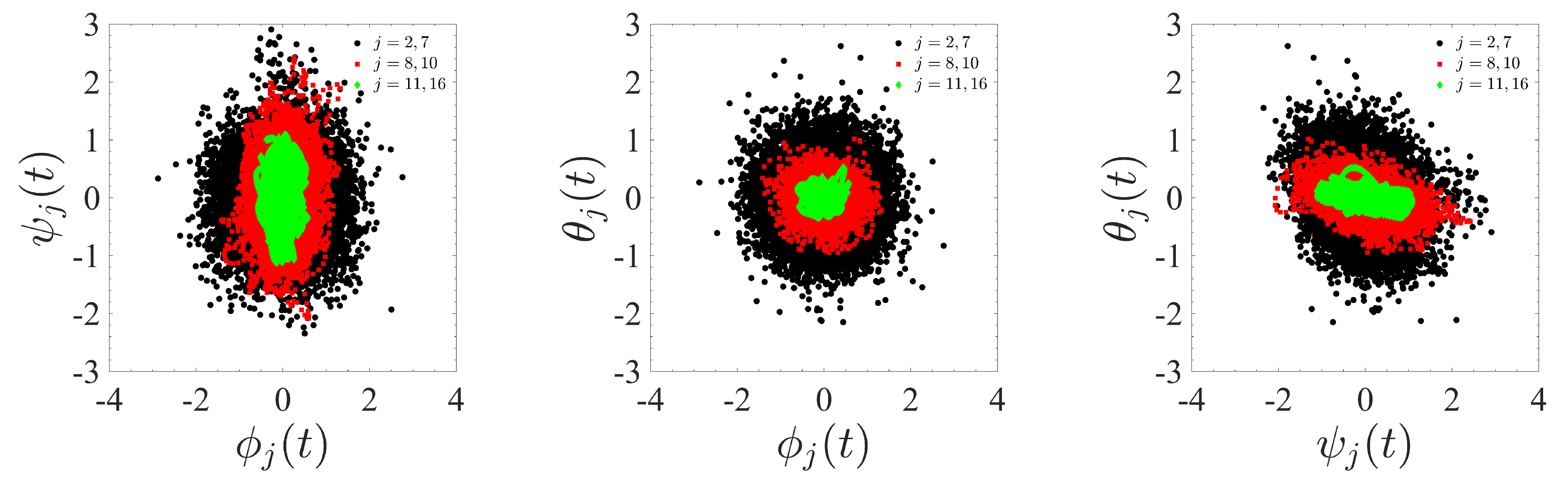
4. Phase-Space Reconstruction and Time Delay of Local SBL Fluctuations
5. Local Correlation Dimension for Turbulent SBL
6. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Stull, R.B. An Introduction to Boundary Layer Meteorology, 1st ed.; Kluwer Academic Publishers: Dordrecht, The Netherlands, 1988. [Google Scholar]
- Garratt, J.R. The Atmospheric Boundary Layer; Cambridge Atmospheric and Space Science Series; Cambridge University Press: Cambridge, UK, 1992. [Google Scholar]
- Rorai, C.; Mininni, P.D.; Pouquet, A. Turbulence comes in bursts in stably stratified flows. Phys. Rev. E 2014, 89, 043002. [Google Scholar] [CrossRef] [PubMed]
- Feraco, F.; Marino, R.; Pumir, A.; Primavera, L.; Mininni, P.D.; Pouquet, A.; Rosenberg, D. Vertical drafts and mixing in stratified turbulence: Sharp transition with Froude number. EPL 2018, 123, 44002. [Google Scholar] [CrossRef]
- Marino, R.; Rosenberg, D.; Herbert, C.; Pouquet, A. Interplay of waves and eddies in rotating stratified turbulence and the link with kinetic-potential energy partition. EPL 2015, 112, 49001. [Google Scholar] [CrossRef][Green Version]
- Smedman, A.S.; Tjernström, M.; Högström, U. Analysis of the turbulence structure of a marine low-level jet. Boundary-Layer Meteorol. 1993, 66, 105–126. [Google Scholar] [CrossRef]
- Mahrt, L. Stably Stratified Atmospheric Boundary Layers. Annu. Rev. Fluid Mech. 2014, 46, 23–45. [Google Scholar] [CrossRef]
- Wyngaard, J.C. Atmospheric Turbulence. Annu. Rev. Fluid Mech. 1992, 24, 205. [Google Scholar] [CrossRef]
- Amati, G.; Benzi, R.; Succi, S. Extended self-similarity in boundary layer turbulence. Phys. Rev. E 1997, 55, 6985–6988. [Google Scholar] [CrossRef]
- Van de Wiel, B.J.H.; Ronda, R.J.; Moene, A.F.; De Bruin, H.A.R.; Holtslag, A.A.M. Intermittent Turbulence and Oscillations in the Stable Boundary Layer over Land. Part I: A Bulk Model. J. Atmos. Sci. 2002, 59, 942–958. [Google Scholar] [CrossRef]
- Wei, W.; Zhang, H.S.; Schmitt, F.G.; Huang, Y.X.; Cai, X.H.; Song, Y.; Huang, X.; Zhang, H. Investigation of Turbulence Behaviour in the Stable Boundary Layer Using Arbitrary-Order Hilbert Spectra. Bound.-Layer Meteorol. 2017, 163. [Google Scholar] [CrossRef]
- Kiliyanpilakkil, V.P.; Basu, S. Extended self-similarity of atmospheric boundary layer wind fields in mesoscale regime: Is it real? EPL (Europhys. Lett.) 2015, 112, 64003. [Google Scholar] [CrossRef][Green Version]
- Kiliyanpilakkil, V.P.; Basu, S.; Ruiz-Columbié, A.; Araya, G.; Castillo, L.; Hirth, B.; Burgett, W. Buoyancy effects on the scaling characteristics of atmospheric boundary-layer wind fields in the mesoscale range. Phys. Rev. E 2015, 92, 033005. [Google Scholar] [CrossRef] [PubMed]
- Carbone, F.; Telloni, D.; Bruno, A.G.; Hedgecock, I.M.; De Simone, F.; Sprovieri, F.; Sorriso-Valvo, L.; Pirrone, N. Scaling Properties of Atmospheric Wind Speed in Mesoscale Range. Atmosphere 2019, 10, 611. [Google Scholar] [CrossRef]
- Mahrt, L. Weak-wind mesoscale meandering in the nocturnal boundary layer. Environ. Fluid Mech. 2007, 7, 331–347. [Google Scholar] [CrossRef]
- Alexander, B.; Branko, G. Atmospheric Boundary Layers: Nature, Theory and Applications to Environmental Modelling and Security, 1st ed.; Springer: New York, NY, USA, 2007. [Google Scholar]
- Mortarini, L.; Cava, D.; Giostra, U.; Costa, F.D.; Degrazia, G.; Anfossi, D.; Acevedo, O. Horizontal Meandering as a Distinctive Feature of the Stable Boundary Layer. J. Atmos. Sci. 2019, 76, 3029–3046. [Google Scholar] [CrossRef]
- Rotta, J. Statistische Theorie nichthomogener Turbulenz. Zeitschrift für Physik 1951, 129, 547–572. [Google Scholar] [CrossRef]
- Biferale, L.; Procaccia, I. Anisotropy in turbulent flows and in turbulent transport. Phys. Rep. 2005, 414, 43–164. [Google Scholar] [CrossRef]
- Mazzitelli, I.; Lanotte, A.S. Active and passive scalar intermittent statistics in turbulent atmospheric convection. Phys. D Nonlinear Phenom. 2012, 241, 251–259. [Google Scholar] [CrossRef]
- Perot, B.; Chartrand, C. Modeling return to isotropy using kinetic equations. Phys. Fluids 2005, 17, 035101. [Google Scholar] [CrossRef]
- Antonelli, M.; Lanotte, A.; Mazzino, A. Anisotropies and Universality of Buoyancy-Dominated Turbulent Fluctuations: A Large-Eddy Simulation Study. J. Atmos. Sci. 2007, 64, 2642–2656. [Google Scholar] [CrossRef][Green Version]
- Frisch, U. (Ed.) Turbulence: The Legacy of A. N. Kolmogorov; Cambridge Univ. Press: Cambridge, UK, 1995. [Google Scholar]
- Tomas, B.; Mogens, H.J.; Giovanni, P.; Vulpiani, A. Dynamical Systems Approach to Turbulence; Cambridge Nonlinear Science Series; Cambridge University Press: Cambridge, UK, 2005. [Google Scholar]
- Narita, Y. Four-dimensional energy spectrum for space–time structure of plasma turbulence. Nonlinear Process. Geophys. 2014, 21, 41–47. [Google Scholar] [CrossRef]
- Carbone, F.; Sorriso-Valvo, L. Experimental analysis of intermittency in electrohydrodynamic instability. Eur. Phys. J. E 2014, 37, 61. [Google Scholar] [CrossRef] [PubMed]
- Politano, H.; Pouquet, A. von Kármán–Howarth equation for magnetohydrodynamics and its consequences on third-order longitudinal structure and correlation functions. Phys. Rev. E 1998, 57, R21–R24. [Google Scholar] [CrossRef]
- Santhanam, M.; Kantz, H. Long-range correlations and rare events in boundary layer wind fields. Phys. A Stat. Mech. Its Appl. 2005, 345, 713–721. [Google Scholar] [CrossRef]
- Carbone, F.; Sorriso-Valvo, L.; Versace, C.; Strangi, G.; Bartolino, R. Anisotropy of Spatiotemporal Decorrelation in Electrohydrodynamic Turbulence. Phys. Rev. Lett. 2011, 106, 114502. [Google Scholar] [CrossRef] [PubMed]
- Servidio, S.; Carbone, V.; Dmitruk, P.; Matthaeus, W.H. Time decorrelation in isotropic magnetohydrodynamic turbulence. EPL (Europhys. Lett.) 2011, 96, 55003. [Google Scholar] [CrossRef]
- Meneveau, C.; Sreenivasan, K.R. The multifractal nature of turbulent energy dissipation. J. Fluid Mech. 1991, 224, 429–484. [Google Scholar] [CrossRef]
- Schmitt, F.G. A causal multifractal stochastic equation and its statistical properties. Eur. Phys. J. B Condens. Matter Complex Syst. 2003, 34, 85–98. [Google Scholar] [CrossRef]
- Carbone, F.; Yoshida, H.; Suzuki, S.; Fujii, A.; Strangi, G.; Versace, C.; Ozaki, M. Clustering of elastic energy due to electrohydrodynamics instabilities in nematic liquid crystals. EPL (Europhys. Lett.) 2010, 89, 46004. [Google Scholar] [CrossRef]
- Tuck, A.F. From molecules to meteorology via turbulent scale invariance. Q. J. R. Meteorol. Soc. 2010, 136, 1125–1144. [Google Scholar] [CrossRef]
- Sorriso-Valvo, L.; Carbone, F.; Leonardis, E.; Chen, C.H.; Šafránková, J.; Němeček, Z. Multifractal analysis of high resolution solar wind proton density measurements. Adv. Space Res. 2017, 59, 1642–1651. [Google Scholar] [CrossRef]
- Liu, L.; Hu, F.; Huang, S. A Multifractal Random-Walk Description of Atmospheric Turbulence: Small-Scale Multiscaling, Long-Tail Distribution, and Intermittency. Bound.-Layer Meteorol. 2019, 172, 351–370. [Google Scholar] [CrossRef]
- Grassberger, P.; Procaccia, I. Characterization of Strange Attractors. Phys. Rev. Lett. 1983, 50, 346–349. [Google Scholar] [CrossRef]
- UCAR/NCAR. E.O.L. CASES-99. 2015. Available online: https://doi.org/10.5065/D6QV3JTK (accessed on 10 April 2020).
- Poulos, G.S.; Blumen, W.; Fritts, D.C.; Lundquist, J.K.; Sun, J.; Burns, S.P.; Nappo, C.; Banta, R.; Newsom, R.; Cuxart, J.; et al. CASES-99: A Comprehensive Investigation of the Stable Nocturnal Boundary Layer. Bull. Am. Meteorol. Soc. 2002, 83, 555–582. [Google Scholar] [CrossRef]
- Van de Wiel, B.J.H.; Moene, A.F.; Ronda, R.J.; De Bruin, H.A.R.; Holtslag, A.A.M. Intermittent Turbulence and Oscillations in the Stable Boundary Layer over Land. Part II: A System Dynamics Approach. J. Atmos. Sci. 2002, 59, 2567–2581. [Google Scholar] [CrossRef]
- Van de Wiel, B.J.H.; Moene, A.F.; Hartogensis, O.K.; De Bruin, H.A.R.; Holtslag, A.A.M. Intermittent Turbulence in the Stable Boundary Layer over Land. Part III: A Classification for Observations during CASES-99. J. Atmos. Sci. 2003, 60, 2509–2522. [Google Scholar] [CrossRef]
- Huang, N.E.; Shen, Z.; Long, S.R.; Wu, M.C.; Shih, H.H.; Zheng, Q.; Yen, N.C.; Tung, C.C.; Liu, H.H. The empirical mode decomposition and the Hilbert spectrum for nonlinear and non-stationary time series analysis. Proc. R. Soc. Lond. A Math. Eng. Sci. 1998, 454, 903–995. [Google Scholar] [CrossRef]
- Rilling, G.; Flandrin, P.; Goncalves, P. On empirical mode decomposition and its algorithms. In Proceedings of the IEEE-EURASIP Workshop on Nonlinear Signal and Image Processing, Trieste, Italy, 8–11 June 2003. [Google Scholar]
- Wu, Z.; Huang, N.E. A study of the characteristics of white noise using the empirical mode decomposition method. Proc. R. Soc. Lond. A Math. Eng. Sci. 2004, 460, 1597–1611. [Google Scholar] [CrossRef]
- Flandrin, P.; Rilling, G.; Goncalves, P. Empirical mode decomposition as a filter bank. IEEE Signal Process. Lett. 2004, 11, 112–114. [Google Scholar] [CrossRef]
- Flandrin, P.; Goncalves, P. Empirical mode decomposition as data-driven wavelet-like expansions. Int. J. Wavelets Multiresolut. Inf. Process. 2004, 1, 477–496. [Google Scholar] [CrossRef]
- Huang, N.E.; Shen, S.S.P. (Eds.) The Hilbert-Huang Transform and Its Applications; World Scientific: Singapore, 2005. [Google Scholar]
- Huang, Y.X.; Schmitt, F.G.; Lu, Z.M.; Liu, Y.L. An amplitude-frequency study of turbulent scaling intermittency using Empirical Mode Decomposition and Hilbert Spectral Analysis. EPL (Europhys. Lett.) 2008, 84, 40010. [Google Scholar] [CrossRef]
- Carbone, F.; Gencarelli, C.N.; Hedgecock, I.M. Lagrangian statistics of mesoscale turbulence in a natural environment: The Agulhas return current. Phys. Rev. E 2016, 94, 063101. [Google Scholar] [CrossRef] [PubMed]
- Carbone, F.; Sorriso-Valvo, L.; Alberti, T.; Lepreti, F.; Chen, C.H.K.; Němeček, Z.; Šafránková, J. Arbitrary-order Hilbert Spectral Analysis and Intermittency in Solar Wind Density Fluctuations. Astrophys. J. 2018, 859, 27. [Google Scholar] [CrossRef]
- Kolmogorov, A.N. The local structure of turbulence in incompressible viscous fluid for very large Reynolds numbers. C. R. Acad. Sci. URSS 1941, 36, 301. [Google Scholar]
- Takens, F. Detecting strange attractors in turbulence. In Dynamical Systems and Turbulence, Warwick 1980; Rand, D., Young, L.S., Eds.; Springer: Berlin/Heidelberg, Germany, 1981; pp. 366–381. [Google Scholar]
- Kantz, H.; Schreiber, T. Nonlinear Time Series Analysis, 2nd ed.; Cambridge University Press: Cambridge, UK, 2003. [Google Scholar]
- Sauer, T.; Yorke, J.A.; Casdagli, M. Embedology. J. Stat. Phys. 1991, 65, 579–616. [Google Scholar] [CrossRef]
- Kennel, M.B.; Brown, R.; Abarbanel, H.D.I. Determining embedding dimension for phase-space reconstruction using a geometrical construction. Phys. Rev. A 1992, 45, 3403–3411. [Google Scholar] [CrossRef] [PubMed]
- Fraser, A.M.; Swinney, H.L. Independent coordinates for strange attractors from mutual information. Phys. Rev. A 1986, 33, 1134–1140. [Google Scholar] [CrossRef] [PubMed]
- Vlachos, I.; Kugiumtzis, D. State Space Reconstruction from Multiple Time Series. In Topics on Chaotic Systems—Selected Papers from CHAOS 2008 International Conference, Chania, Crete, Greece, 3–6 June 2008; Skiadas, C.H., Dimotikalis, I., Skiadas, C., Eds.; World Scientific: Singapore, 2009; pp. 378–387. [Google Scholar] [CrossRef]
- Fredkin, D.R.; Rice, J.A. Method of false nearest neighbors: A cautionary note. Phys. Rev. E 1995, 51, 2950–2954. [Google Scholar] [CrossRef]
- Wallot, S.; Mønster, D. Calculation of Average Mutual Information (AMI) and False-Nearest Neighbors (FNN) for the Estimation of Embedding Parameters of Multidimensional Time Series in Matlab. Front. Psychol. 2018, 9, 1679. [Google Scholar] [CrossRef]
- Consolini, G.; Alberti, T.; De Michelis, P. On the Forecast Horizon of Magnetospheric Dynamics: A Scale-to-Scale Approach. J. Geophys. Res. (Space Phys.) 2018, 123, 9065–9077. [Google Scholar] [CrossRef]
- Alberti, T.; Consolini, G.; Carbone, V.; Yordanova, E.; Marcucci, M.F.; De Michelis, P. Multifractal and Chaotic Properties of Solar Wind at MHD and Kinetic Domains: An Empirical Mode Decomposition Approach. Entropy 2019, 21, 320. [Google Scholar] [CrossRef]
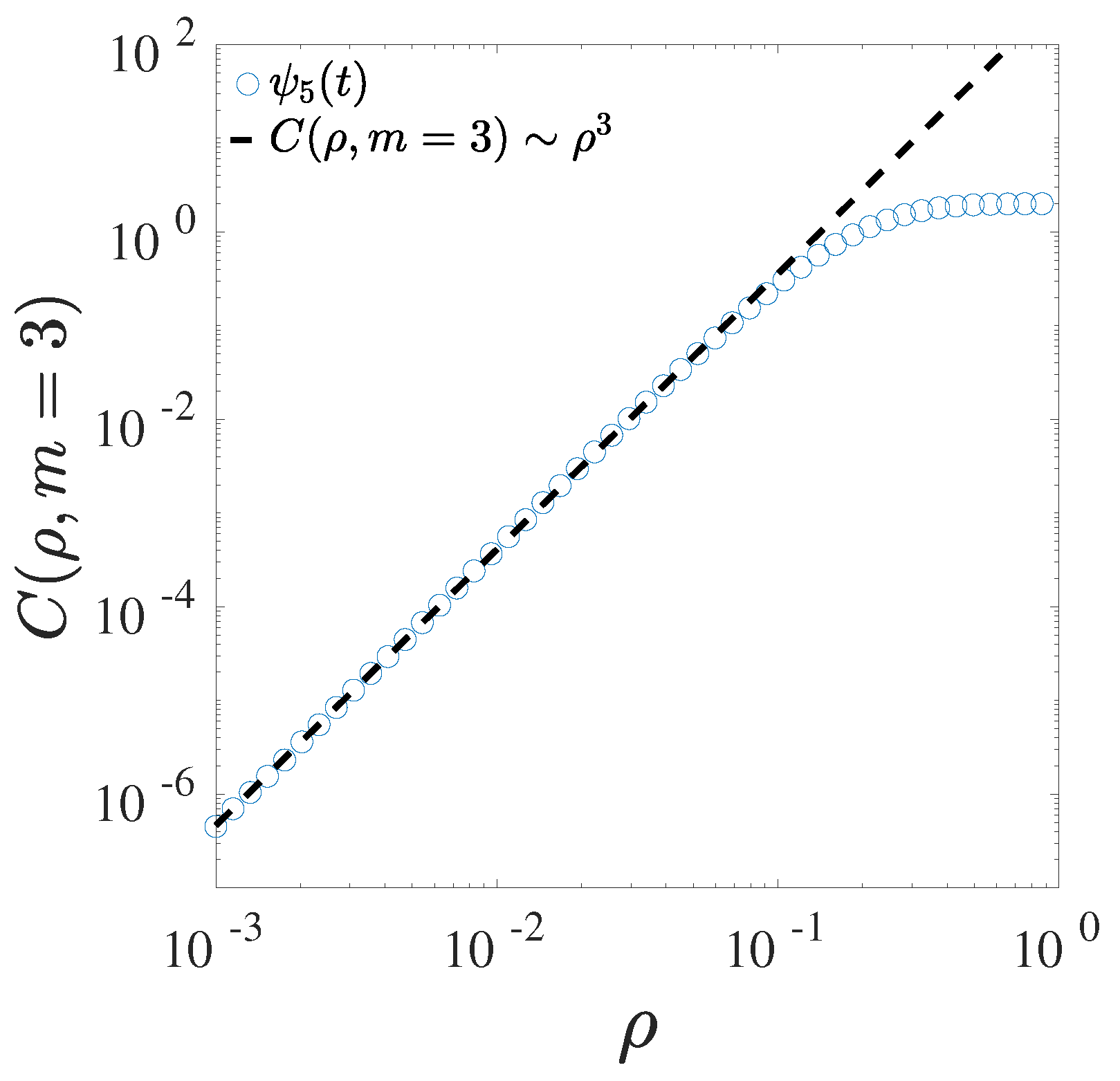
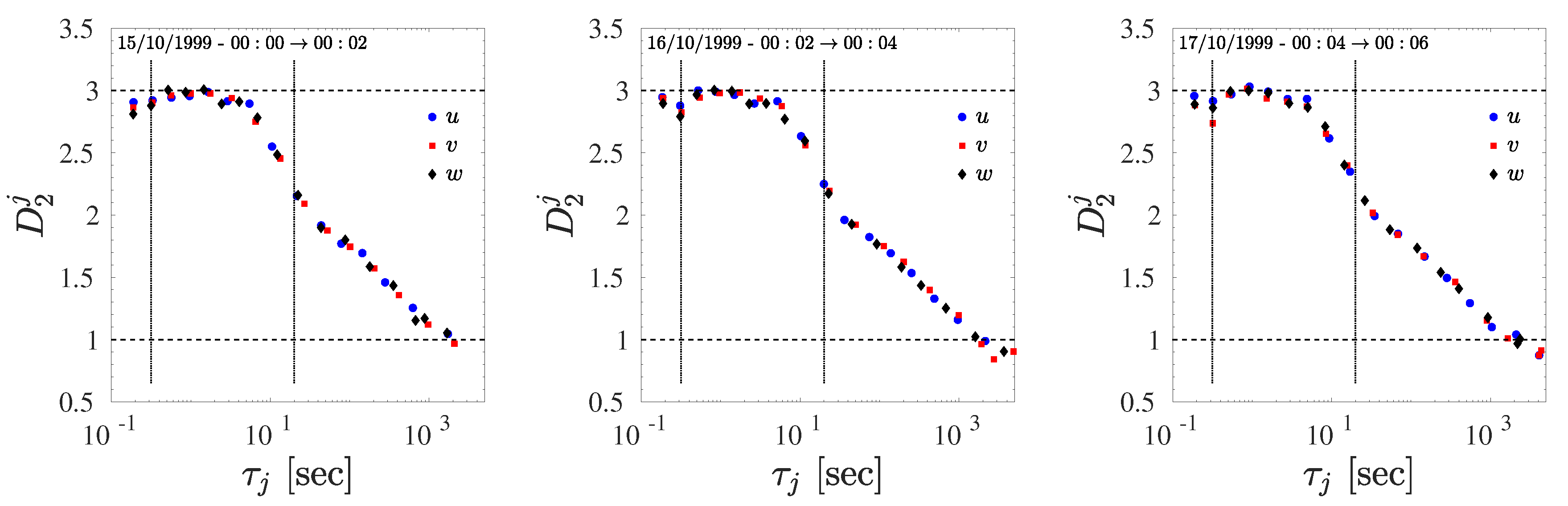
| SBL | d | [s] | m | |
|---|---|---|---|---|
| 1 | 0.35 | 3 | 3 | |
| 1 | 0.40 | 3 | 3 | |
| 1 | 0.35 | 3 | 3 | |
| 2 | 0.38 | 2 | 4 | |
| 2 | 0.35 | 2 | 4 | |
| 2 | 0.38 | 2 | 4 | |
| 3 | 0.37 | 1 | 3 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Carbone, F.; Alberti, T.; Sorriso-Valvo, L.; Telloni, D.; Sprovieri, F.; Pirrone, N. Scale-Dependent Turbulent Dynamics and Phase-Space Behavior of the Stable Atmospheric Boundary Layer. Atmosphere 2020, 11, 428. https://doi.org/10.3390/atmos11040428
Carbone F, Alberti T, Sorriso-Valvo L, Telloni D, Sprovieri F, Pirrone N. Scale-Dependent Turbulent Dynamics and Phase-Space Behavior of the Stable Atmospheric Boundary Layer. Atmosphere. 2020; 11(4):428. https://doi.org/10.3390/atmos11040428
Chicago/Turabian StyleCarbone, Francesco, Tommaso Alberti, Luca Sorriso-Valvo, Daniele Telloni, Francesca Sprovieri, and Nicola Pirrone. 2020. "Scale-Dependent Turbulent Dynamics and Phase-Space Behavior of the Stable Atmospheric Boundary Layer" Atmosphere 11, no. 4: 428. https://doi.org/10.3390/atmos11040428
APA StyleCarbone, F., Alberti, T., Sorriso-Valvo, L., Telloni, D., Sprovieri, F., & Pirrone, N. (2020). Scale-Dependent Turbulent Dynamics and Phase-Space Behavior of the Stable Atmospheric Boundary Layer. Atmosphere, 11(4), 428. https://doi.org/10.3390/atmos11040428







