Geoengineering: Impact of Marine Cloud Brightening Control on the Extreme Temperature Change over East Asia
Abstract
1. Introduction
2. Data and Methods
2.1. Data
2.2. Methods
3. Results
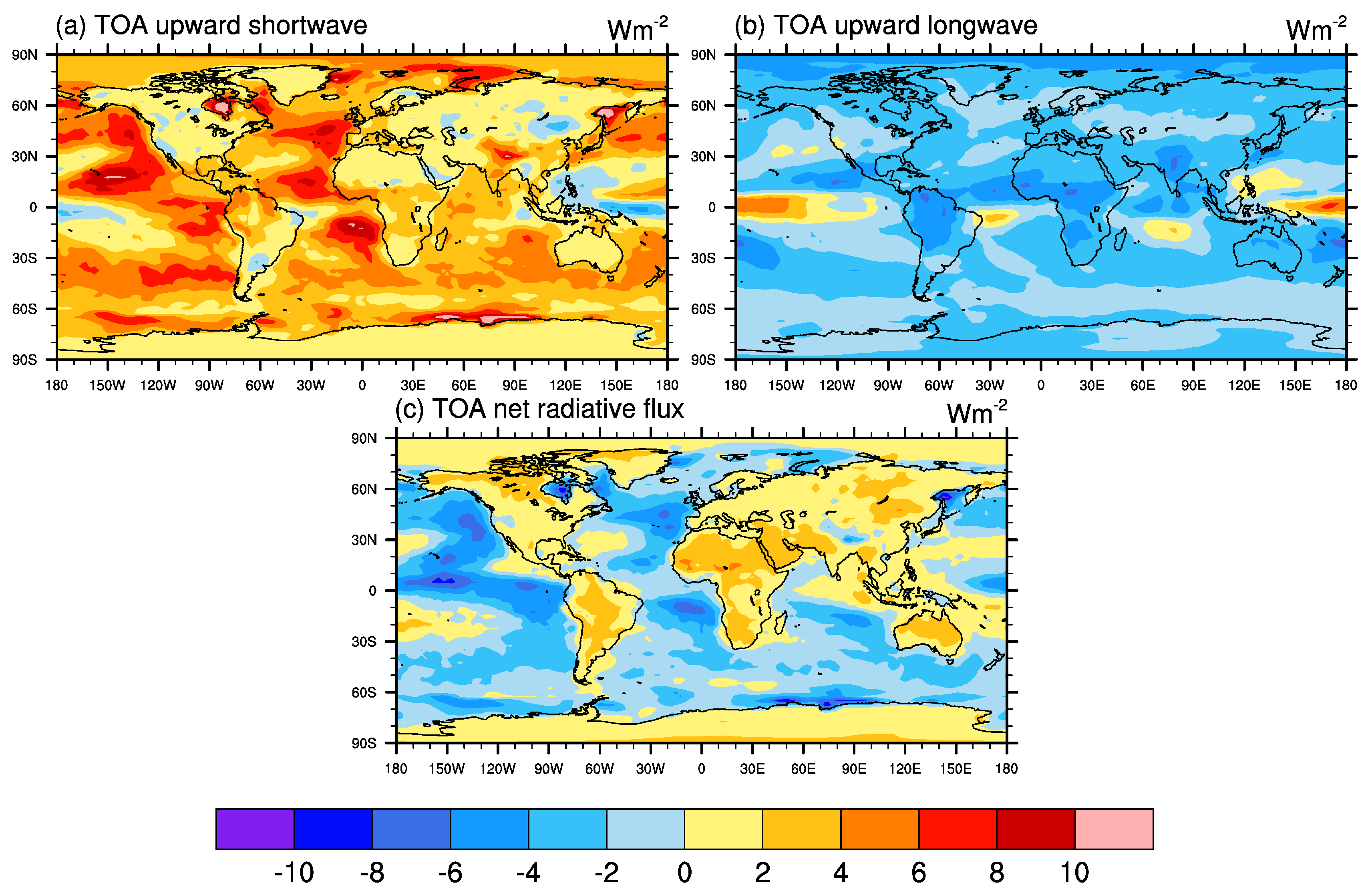
3.1. Radiation Budget at TOA
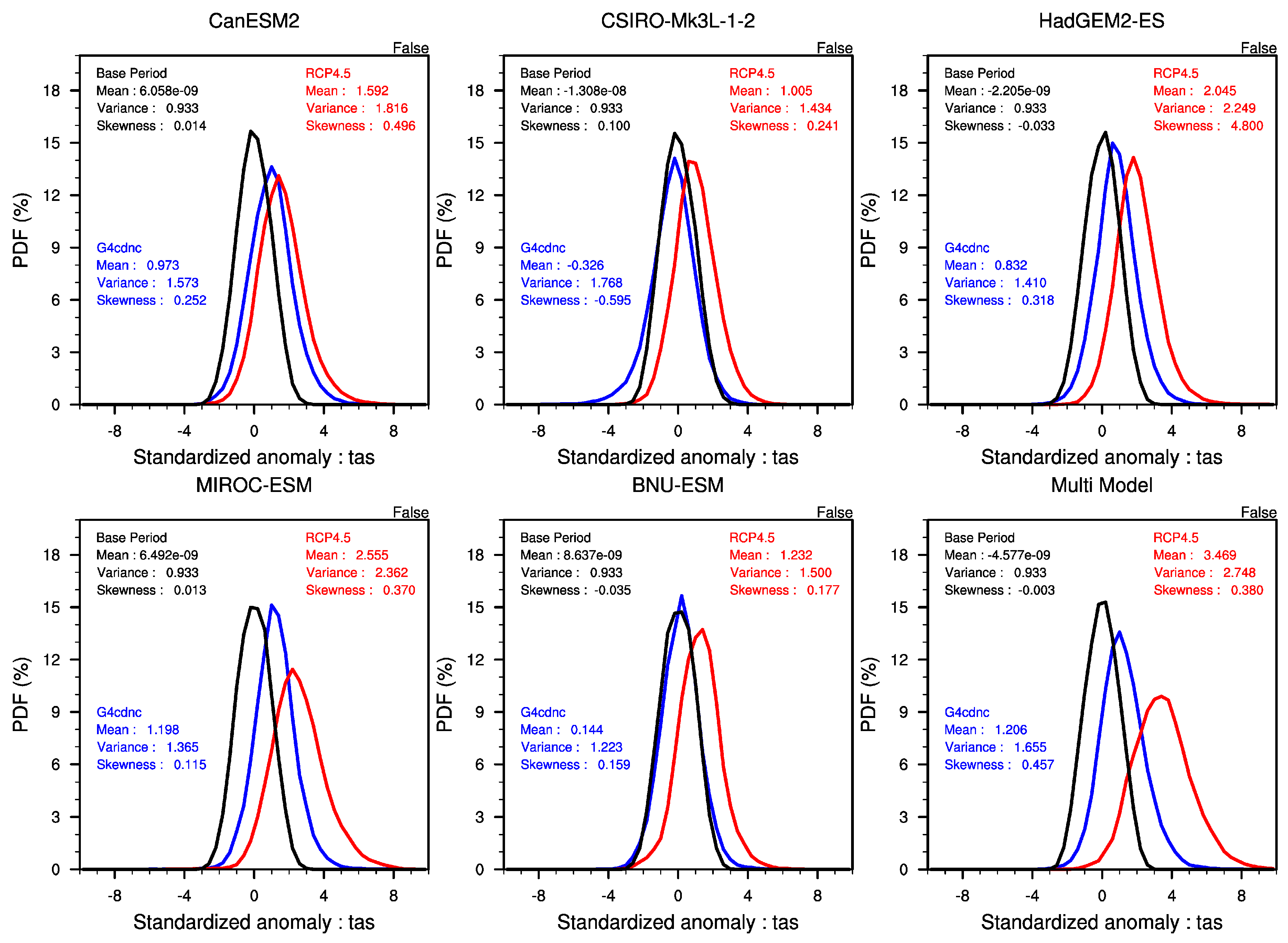
3.2. Mean Temperature
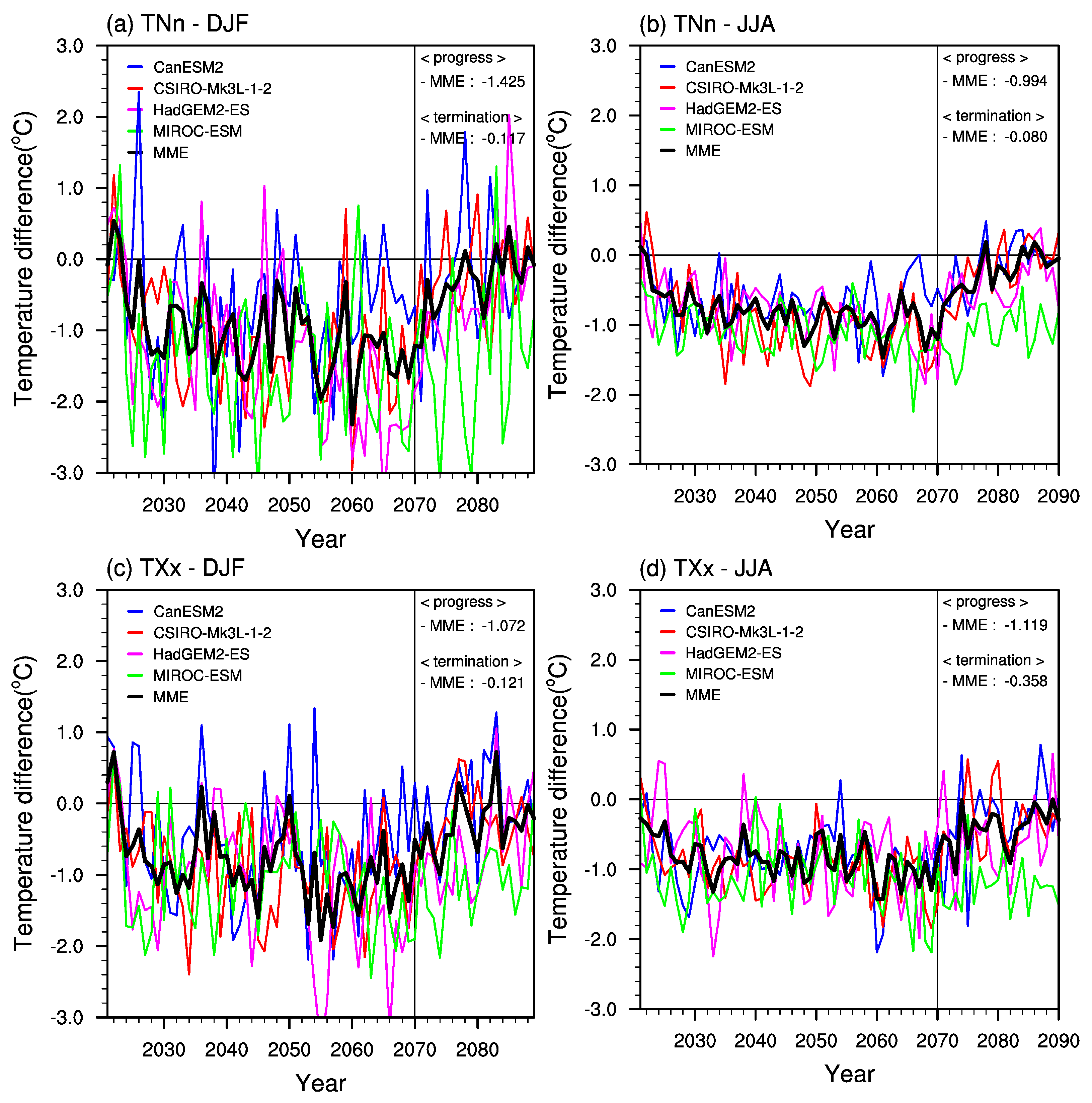
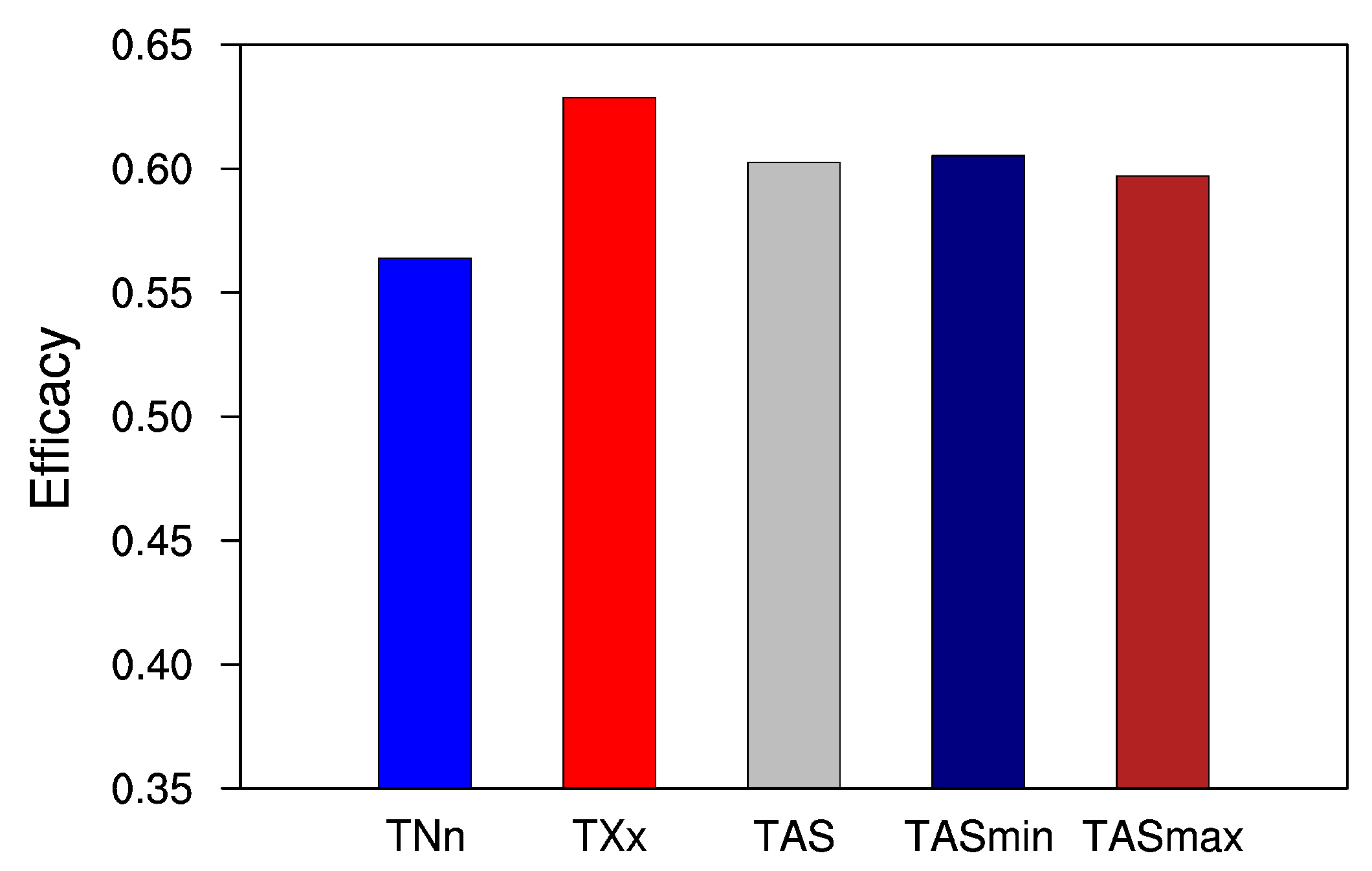
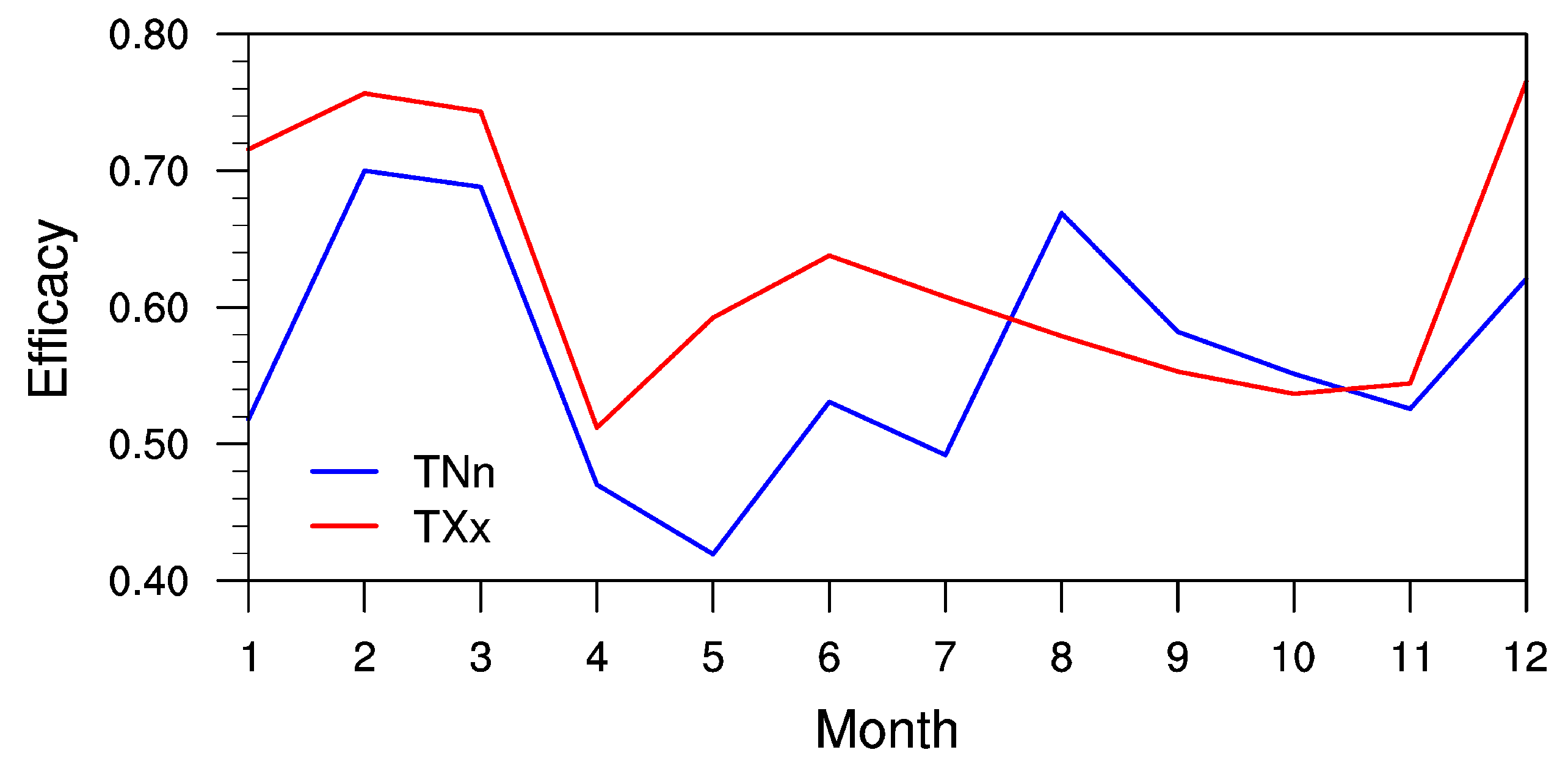
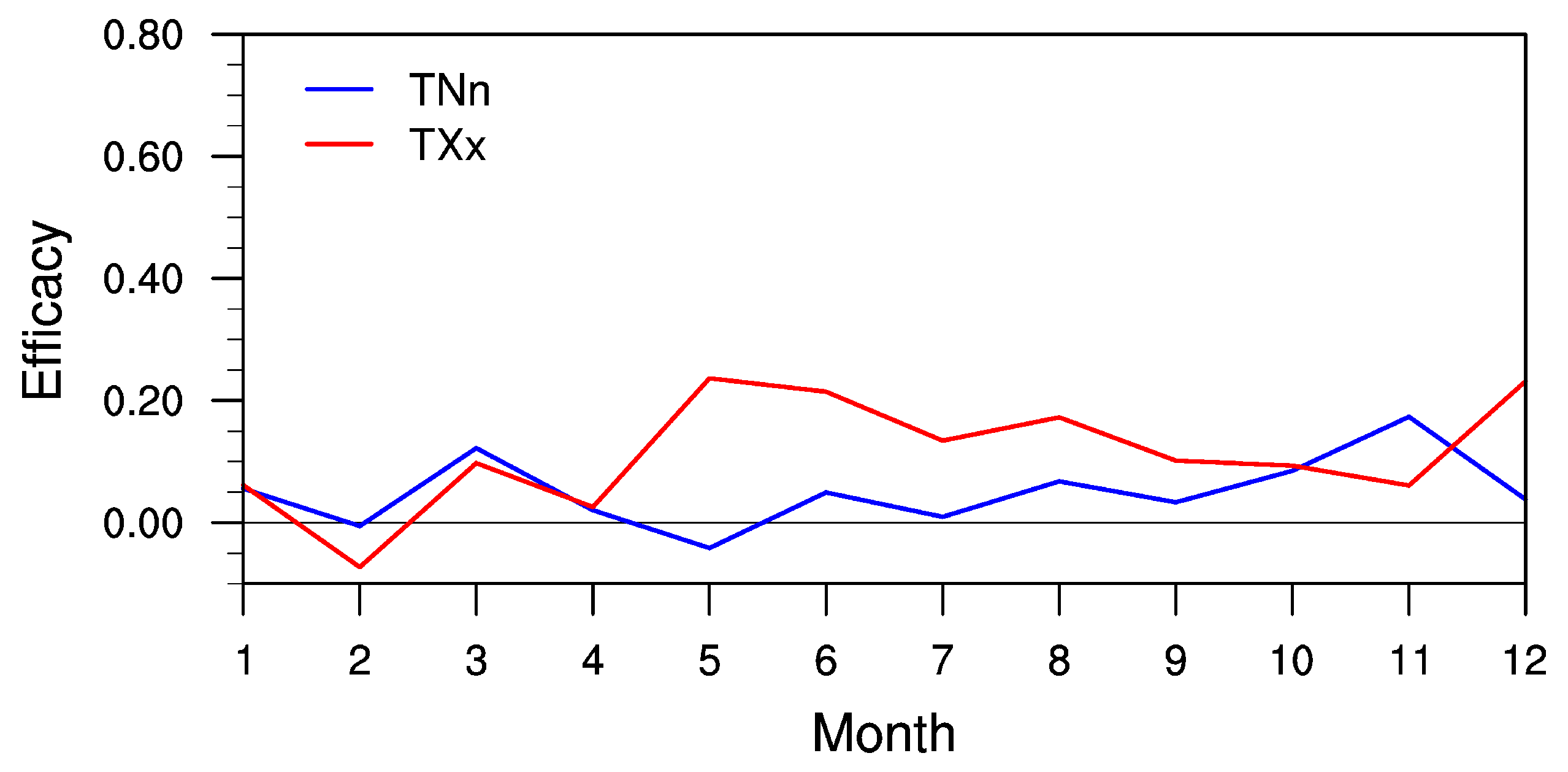
3.3. Temporal and Spatial Response in Extreme Temperatures
3.4. Seasonality of Extreme Temperature Response
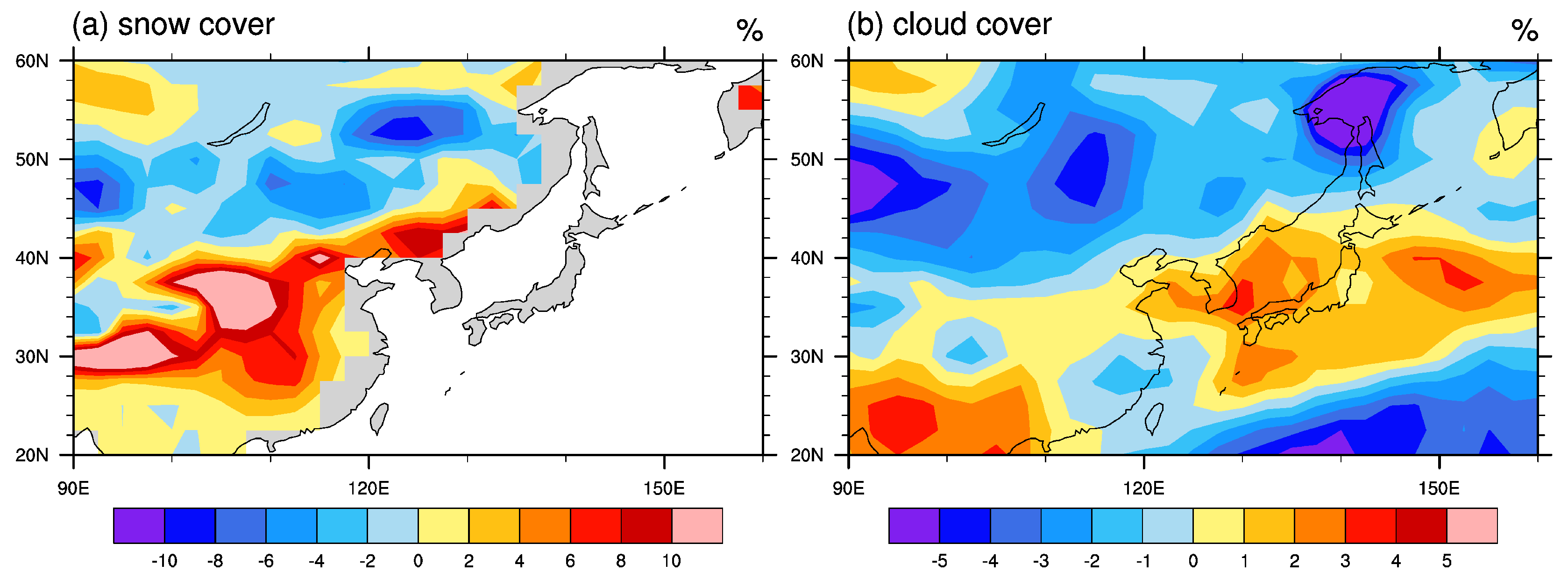
3.5. Relative Effect of Extreme Temperature
3.6. Durability of Effects after the Termination of G4cdnc
4. Summary and Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Data Availability
References
- Sanderson, B.M.; O’Neill, B.C.; Tebaldi, C. What would it take to achieve the Paris temperature targets? Geophys. Res. Lett. 2016, 43, 7133–7142. [Google Scholar] [CrossRef]
- Yang, H.; Dobbie, S.; Ramirez-Villegas, J.; Feng, K.; Challinor, A.J.; Chen, B.; Gao, Y.; Lee, L.; Yin, Y.; Sun, L.; et al. Potential negative consequences of geoengineering on crop production: A study of Indian groundnut. Geophys. Res. Lett. 2016, 43, 11786–11795. [Google Scholar] [CrossRef] [PubMed]
- Bellamy, R.; Lezaun, J.; Palmer, J. Public perceptions of geoengineering research governance: An experimental deliberative approach. Glob. Environ. Chang. 2017, 45, 194–202. [Google Scholar] [CrossRef]
- Trisos, C.H.; Amatulli, G.; Gurevitch, J.; Robock, A.; Xia, L.; Zambri, B. Potentially dangerous consequences for biodiversity of solar geoengineering implementation and termination. Nat. Ecol. Evol. 2018, 2, 475–482. [Google Scholar] [CrossRef] [PubMed]
- Mautner, M. A Space-Based Solar Screen against Climatic Warming. J. Br. Interplanet. Soc. 1991, 44, 135–148. [Google Scholar]
- Budyko, M.I. On present-day climatic changes. Tellus 1977, 29, 193–204. [Google Scholar] [CrossRef]
- Crutzen, P.J. Albedo Enhancement by Stratospheric Sulfur Injections: A Contribution to Resolve a Policy Dilemma? Clim. Chang. 2006, 77, 211. [Google Scholar] [CrossRef]
- Latham, J. Control of global warming? Nature 1990, 347, 339–340. [Google Scholar] [CrossRef]
- Bala, G.; Duffy, P.B.; Taylor, K.E. Impact of geoengineering schemes on the global hydrological cycle. Proc. Natl. Acad. Sci. USA 2008, 105, 7664–7669. [Google Scholar] [CrossRef]
- Lenton, T.M.; Vaughan, N.E. The radiative forcing potential of different climate geoengineering options. Atmos. Chem. Phys. 2009, 9, 5539–5561. [Google Scholar] [CrossRef]
- Irvine, P.J.; Ridgwell, A.; Lunt, D.J. Assessing the regional disparities in geoengineering impacts. Geophys. Res. Lett. 2010, 37, L18702. [Google Scholar] [CrossRef]
- Kravitz, B.; Robock, A.; Boucher, O.; Schmidt, H.; Taylor, K.E.; Stenchikov, G.; Schulz, M. The Geoengineering Model Intercomparison Project (GeoMIP). Atmos. Sci. Lett. 2011, 12, 162–167. [Google Scholar] [CrossRef]
- Kravitz, B.; Forster, P.M.; Jones, A.; Robock, A.; Alterskjær, K.; Boucher, O.; Jenkins, A.K.L.; Korhonen, H.; Kristjánsson, J.E.; Muri, H.; et al. Sea spray geoengineering experiments in the geoengineering model intercomparison project (GeoMIP): Experimental design and preliminary results. J. Geophys. Res. Atmos. 2013, 118, 11175–11186. [Google Scholar] [CrossRef]
- Twomey, S. Pollution and the planetary albedo. Atmos. Environ. 1974, 8, 1251–1256. [Google Scholar] [CrossRef]
- Stjern, C.W.; Muri, H.; Ahlm, L.; Boucher, O.; Cole, J.N.S.; Ji, D.; Jones, A.; Haywood, J.; Kravitz, B.; Lenton, A.; et al. Response to marine cloud brightening in a multi-model ensemble. Atmos. Chem. Phys. 2018, 18, 621–634. [Google Scholar] [CrossRef]
- Sillmann, J.; Kharin, V.V.; Zhang, X.; Zwiers, F.W.; Bronaugh, D. Climate extremes indices in the CMIP5 multimodel ensemble: Part 1. Model evaluation in the present climate. J. Geophys. Res. Atmos. 2013, 118, 1716–1733. [Google Scholar] [CrossRef]
- Kharin, V.V.; Zwiers, F.W. Changes in the Extremes in an Ensemble of Transient Climate Simulations with a Coupled Atmosphere–Ocean GCM. J. Clim. 2000, 13, 3760–3788. [Google Scholar] [CrossRef]
- Kharin, V.V.; Zwiers, F.W. Estimating Extremes in Transient Climate Change Simulations. J. Clim. 2005, 18, 1156–1173. [Google Scholar] [CrossRef]
- Easterling, D.R.; Kunkel, K.E.; Wehner, M.F.; Sun, L. Detection and attribution of climate extremes in the observed record. Weather Clim. Extrem. 2016, 11, 17–27. [Google Scholar] [CrossRef]
- Boo, K.-O.; Kwon, W.-T.; Baek, H.-J. Change of extreme events of temperature and precipitation over Korea using regional projection of future climate change. Geophys. Res. Lett. 2006, 33, L01701. [Google Scholar] [CrossRef]
- Park, C.; Min, S.-K.; Lee, D.; Cha, D.-H.; Suh, M.-S.; Kang, H.-S.; Hong, S.-Y.; Lee, D.-K.; Baek, H.-J.; Boo, K.-O.; et al. Evaluation of multiple regional climate models for summer climate extremes over East Asia. Clim. Dyn. 2016, 46, 2469–2486. [Google Scholar] [CrossRef]
- Li, D.; Zhou, T.; Zou, L.; Zhang, W.; Zhang, L. Extreme High-Temperature Events Over East Asia in 1.5 °C and 2 °C Warmer Futures: Analysis of NCAR CESM Low-Warming Experiments. Geophys. Res. Lett. 2018, 45, 1541–1550. [Google Scholar] [CrossRef]
- Xu, Y.; Gao, X.; Giorgi, F.; Zhou, B.; Shi, Y.; Wu, J.; Zhang, Y. Projected Changes in Temperature and Precipitation Extremes over China as Measured by 50-yr Return Values and Periods Based on a CMIP5 Ensemble. Adv. Atmos. Sci. 2018, 35, 376–388. [Google Scholar] [CrossRef]
- Taylor, K.E.; Stouffer, R.J.; Meehl, G.A. An Overview of CMIP5 and the Experiment Design. Bull. Am. Meteorol. Soc. 2012, 93, 485–498. [Google Scholar] [CrossRef]
- Ji, D.; Wang, L.; Feng, J.; Wu, Q.; Cheng, H.; Zhang, Q.; Yang, J.; Dong, W.; Dai, Y.; Gong, D.; et al. Description and basic evaluation of Beijing Normal University Earth System Model (BNU-ESM) version 1. Geosci. Model Dev. 2014, 7, 2039–2064. [Google Scholar] [CrossRef]
- Arora, V.K.; Scinocca, J.F.; Boer, G.J.; Christian, J.R.; Denman, K.L.; Flato, G.M.; Kharin, V.V.; Lee, W.G.; Merryfield, W.J. Carbon emission limits required to satisfy future representative concentration pathways of greenhouse gases. Geophys. Res. Lett. 2011, 38, L05805. [Google Scholar] [CrossRef]
- Phipps, S.J.; Rotstayn, L.D.; Gordon, H.B.; Roberts, J.L.; Hirst, A.C.; Budd, W.F. The CSIRO Mk3L climate system model version 1.0—Part 1: Description and evaluation. Geosci. Model Dev. 2011, 4, 483–509. [Google Scholar] [CrossRef]
- Collins, W.J.; Bellouin, N.; Doutriaux-Boucher, M.; Gedney, N.; Halloran, P.; Hinton, T.; Hughes, J.; Jones, C.D.; Joshi, M.; Liddicoat, S.; et al. Development and evaluation of an Earth-System model—HadGEM2. Geosci. Model Dev. 2011, 4, 1051–1075. [Google Scholar] [CrossRef]
- Watanabe, S.; Hajima, T.; Sudo, K.; Nagashima, T.; Takemura, T.; Okajima, H.; Nozawa, T.; Kawase, H.; Abe, M.; Yokohata, T.; et al. MIROC-ESM 2010: Model description and basic results of CMIP5-20c3m experiments. Geosci. Model Dev. 2011, 4, 845–872. [Google Scholar] [CrossRef]
- Zhang, X.; Alexander, L.; Hegerl, G.C.; Jones, P.; Tank, A.K.; Peterson, T.C.; Trewin, B.; Zwiers, F.W. Indices for monitoring changes in extremes based on daily temperature and precipitation data. Wires Clim. Chang. 2011, 2, 851–870. [Google Scholar] [CrossRef]
- Donat, M.G.; Alexander, L.V.; Yang, H.; Durre, I.; Vose, R.; Caesar, J. Global Land-Based Datasets for Monitoring Climatic Extremes. Bull. Am. Meteorol. Soc. 2013, 94, 997–1006. [Google Scholar] [CrossRef]
- Orlowsky, B.; Seneviratne, S.I. Global changes in extreme events: Regional and seasonal dimension. Clim. Chang. 2012, 110, 669–696. [Google Scholar] [CrossRef]
- Curry, C.L.; Sillmann, J.; Bronaugh, D.; Alterskjaer, K.; Cole, J.N.S.; Ji, D.; Kravitz, B.; Kristjánsson, J.E.; Moore, J.C.; Muri, H.; et al. A multimodel examination of climate extremes in an idealized geoengineering experiment. J. Geophys. Res. Atmos. 2014, 119, 3900–3923. [Google Scholar] [CrossRef]
- Aswathy, V.N.; Boucher, O.; Quaas, M.; Niemeier, U.; Muri, H.; Mülmenstädt, J.; Quaas, J. Climate extremes in multi-model simulations of stratospheric aerosol and marine cloud brightening climate engineering. Atmos. Chem. Phys. 2015, 15, 9593–9610. [Google Scholar] [CrossRef]
- Ji, D.; Fang, S.; Curry, C.L.; Kashimura, H.; Watanabe, S.; Cole, J.N.S.; Lenton, A.; Muri, H.; Kravitz, B.; Moore, J.C. Extreme temperature and precipitation response to solar dimming and stratospheric aerosol geoengineering. Atmos. Chem. Phys. 2018, 18, 10133–10156. [Google Scholar] [CrossRef]
- Jones, P.W. First- and Second-Order Conservative Remapping Schemes for Grids in Spherical Coordinates. Mon. Weather Rev. 1999, 127, 2204–2210. [Google Scholar] [CrossRef]
- Schmidt, H.; Alterskjær, K.; Bou Karam, D.; Boucher, O.; Jones, A.; Kristjánsson, J.E.; Niemeier, U.; Schulz, M.; Aaheim, A.; Benduhn, F.; et al. Solar irradiance reduction to counteract radiative forcing from a quadrupling of CO2: Climate responses simulated by four earth system models. Earth Syst. Dyn. 2012, 3, 63–78. [Google Scholar] [CrossRef]
- Haigh, J.; Conover, W.J. Practical Nonparametric Statistics. J. R. Stat. Soc. Ser. A (Gen.) 1981, 3, 370–371. [Google Scholar] [CrossRef]
- Kravitz, B.; Caldeira, K.; Boucher, O.; Robock, A.; Rasch, P.J.; Alterskjær, K.; Karam, D.B.; Cole, J.N.S.; Curry, C.L.; Haywood, J.M.; et al. Climate model response from the Geoengineering Model Intercomparison Project (GeoMIP). J. Geophys. Res. Atmos. 2013, 118, 8320–8332. [Google Scholar] [CrossRef]
- Tebaldi, C.; Hayhoe, K.; Arblaster, J.M.; Meehl, G.A. Going to the Extremes. Clim. Chang. 2006, 79, 185–211. [Google Scholar] [CrossRef]
- Sillmann, J.; Kharin, V.V.; Zwiers, F.W.; Zhang, X.; Bronaugh, D. Climate extremes indices in the CMIP5 multimodel ensemble: Part 2. Future climate projections. J. Geophys. Res. Atmos. 2013, 118, 2473–2493. [Google Scholar] [CrossRef]
- Rasch, P.J.; Latham, J.; Chen, C.C.J. Geoengineering by cloud seeding: Influence on sea ice and climate system. Environ. Res. Lett. 2009, 4, 045112. [Google Scholar] [CrossRef]
- Moore, J.C.; Rinke, A.; Yu, X.; Ji, D.; Cui, X.; Li, Y.; Alterskjær, K.; Kristjánsson, J.E.; Muri, H.; Boucher, O.; et al. Arctic sea ice and atmospheric circulation under the GeoMIP G1 scenario. J. Geophys. Res. Atmos. 2014, 119, 567–583. [Google Scholar] [CrossRef]
- Holland, M.M.; Bitz, C.M. Polar amplification of climate change in coupled models. Clim. Dyn. 2003, 21, 221–232. [Google Scholar] [CrossRef]
- Bekryaev, R.V.; Polyakov, I.V.; Alexeev, V.A. Role of Polar Amplification in Long-Term Surface Air Temperature Variations and Modern Arctic Warming. J. Clim. 2010, 23, 3888–3906. [Google Scholar] [CrossRef]
- Pithan, F.; Mauritsen, T. Arctic amplification dominated by temperature feedbacks in contemporary climate models. Nat. Geosci. 2014, 7, 181–184. [Google Scholar] [CrossRef]
- Alterskjær, K.; Kristjánsson, J.E.; Boucher, O.; Muri, H.; Niemeier, U.; Schmidt, H.; Schulz, M.; Timmreck, C. Sea-salt injections into the low-latitude marine boundary layer: The transient response in three Earth system models. J. Geophys. Res. Atmos. 2013, 118, 12195–12206. [Google Scholar] [CrossRef]
- Volodin, E.M.; Kostrykin, S.V.; Ryaboshapko, A.G. Climate response to aerosol injection at different stratospheric locations. Atmos. Sci. Lett. 2011, 12, 381–385. [Google Scholar] [CrossRef]
- Zhao, P.; Zhang, X.; Zhou, X.; Ikeda, M.; Yin, Y. The Sea Ice Extent Anomaly in the North Pacific and Its Impact on the East Asian Summer Monsoon Rainfall. J. Clim. 2004, 17, 3434–3447. [Google Scholar] [CrossRef]
- Stroeve, J.C.; Serreze, M.C.; Holland, M.M.; Kay, J.E.; Malanik, J.; Barrett, A.P. The Arctic’s rapidly shrinking sea ice cover: A research synthesis. Clim. Chang. 2012, 110, 1005–1027. [Google Scholar] [CrossRef]
- Berdahl, M.; Robock, A.; Ji, D.; Moore, J.C.; Jones, A.; Kravitz, B.; Watanabe, S. Arctic cryosphere response in the Geoengineering Model Intercomparison Project G3 and G4 scenarios. J. Geophys. Res. Atmos. 2014, 119, 1308–1321. [Google Scholar] [CrossRef]
- Jones, A.; Haywood, J.M.; Alterskjær, K.; Boucher, O.; Cole, J.N.S.; Curry, C.L.; Irvine, P.J.; Ji, D.; Kravitz, B.; Kristjánsson, J.E.; et al. The impact of abrupt suspension of solar radiation management (termination effect) in experiment G2 of the Geoengineering Model Intercomparison Project (GeoMIP). J. Geophys. Res. Atmos. 2013, 118, 9743–9752. [Google Scholar] [CrossRef]





| Model | Institution | No. of Grid Cells (lon × lat) | Termination Effect Experiment | Reference |
|---|---|---|---|---|
| BNU-ESM | Beijing Normal University, China | 128 × 64 | Yes | [25] |
| CanESM2 | Canadian Centre for Climate Modeling and Analysis, Canada | 128 × 64 | Yes | [26] |
| CSIRO-Mk3L-1-2 | University of New South Wales, Australia | 64 × 56 | Yes | [27] |
| HadGEM2-ES | Met Office Hadley Centre, UK | 192 × 145 | Yes | [28] |
| MIROC-ESM | Japan Agency for Marine-Earth Science and Technology, Japan | 128 × 64 | No | [29] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kim, D.-H.; Shin, H.-J.; Chung, I.-U. Geoengineering: Impact of Marine Cloud Brightening Control on the Extreme Temperature Change over East Asia. Atmosphere 2020, 11, 1345. https://doi.org/10.3390/atmos11121345
Kim D-H, Shin H-J, Chung I-U. Geoengineering: Impact of Marine Cloud Brightening Control on the Extreme Temperature Change over East Asia. Atmosphere. 2020; 11(12):1345. https://doi.org/10.3390/atmos11121345
Chicago/Turabian StyleKim, Do-Hyun, Ho-Jeong Shin, and Il-Ung Chung. 2020. "Geoengineering: Impact of Marine Cloud Brightening Control on the Extreme Temperature Change over East Asia" Atmosphere 11, no. 12: 1345. https://doi.org/10.3390/atmos11121345
APA StyleKim, D.-H., Shin, H.-J., & Chung, I.-U. (2020). Geoengineering: Impact of Marine Cloud Brightening Control on the Extreme Temperature Change over East Asia. Atmosphere, 11(12), 1345. https://doi.org/10.3390/atmos11121345





