Adaption of an Evaporative Desert Cooler into a Liquid Desiccant Air Conditioner: Experimental and Numerical Analysis
Abstract
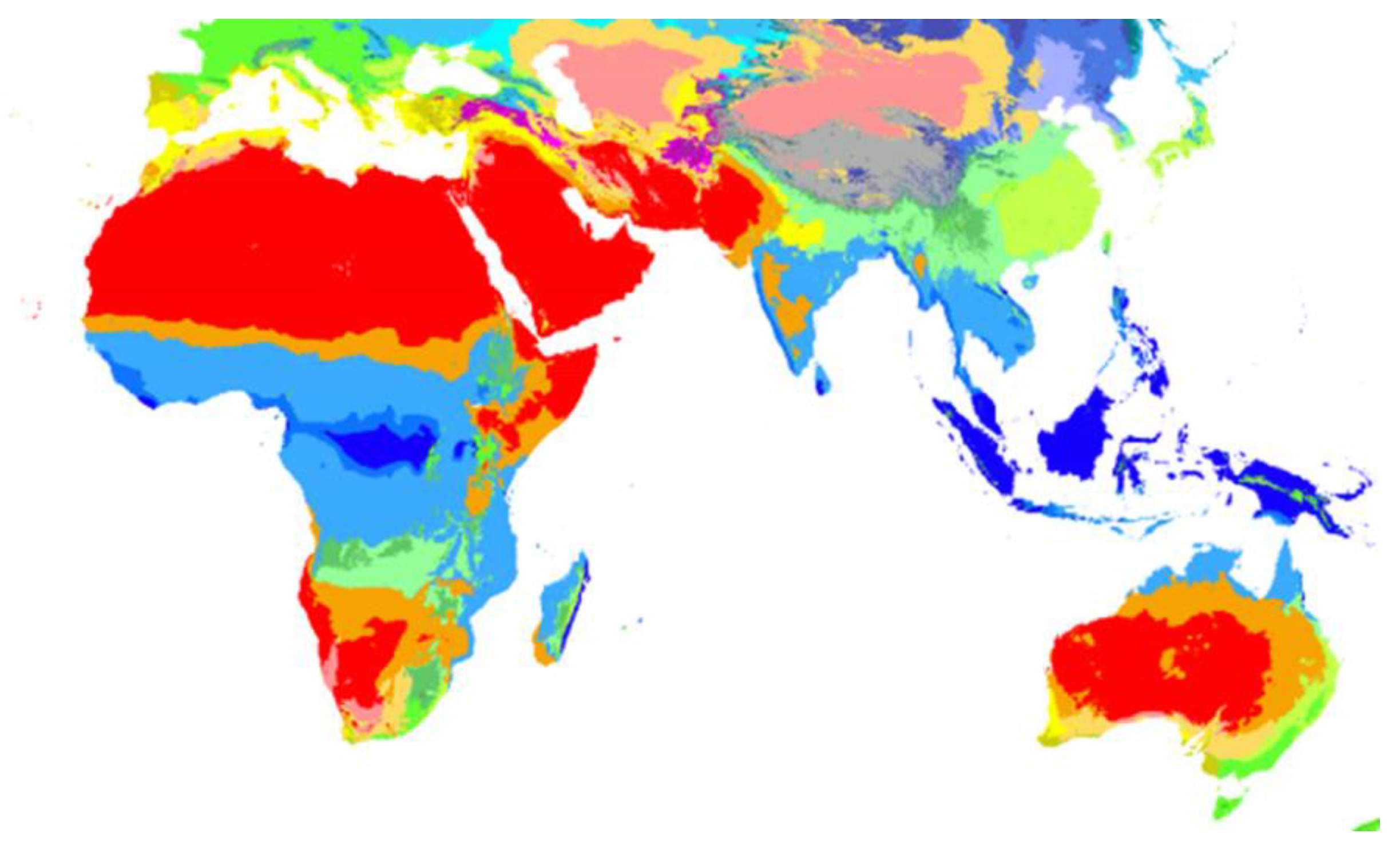
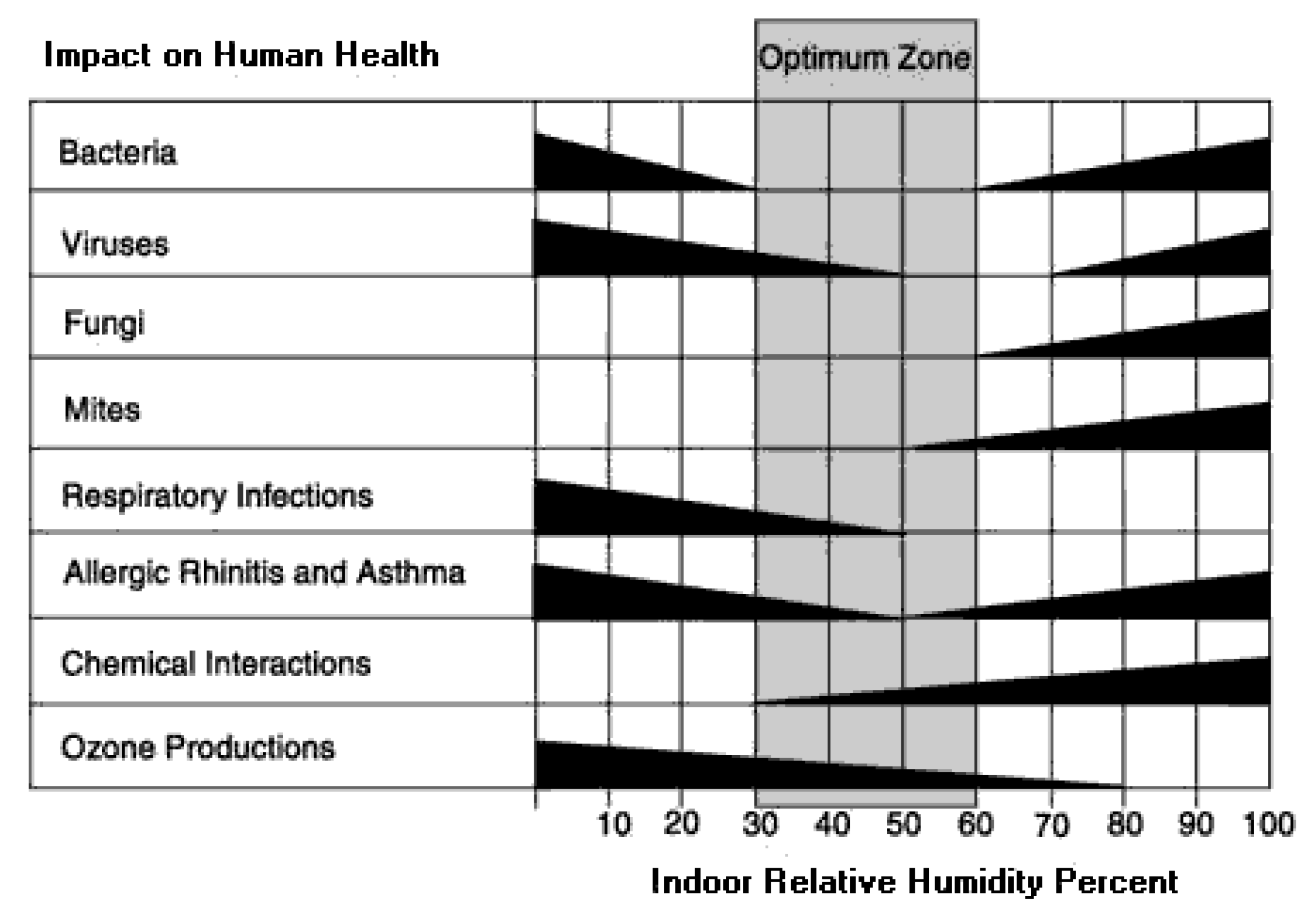
1. Introduction
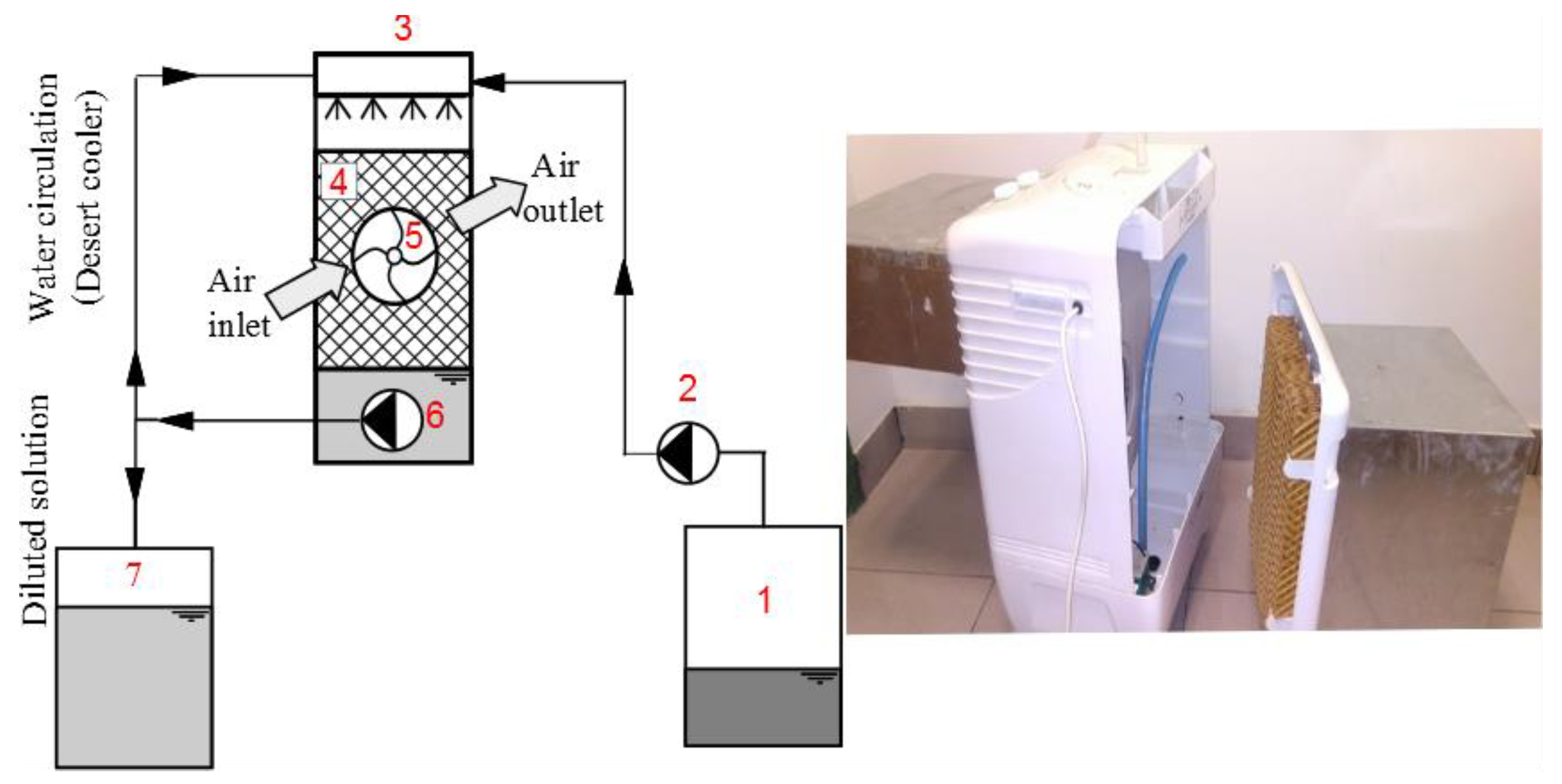
2. Description of the Modified Desert Cooler
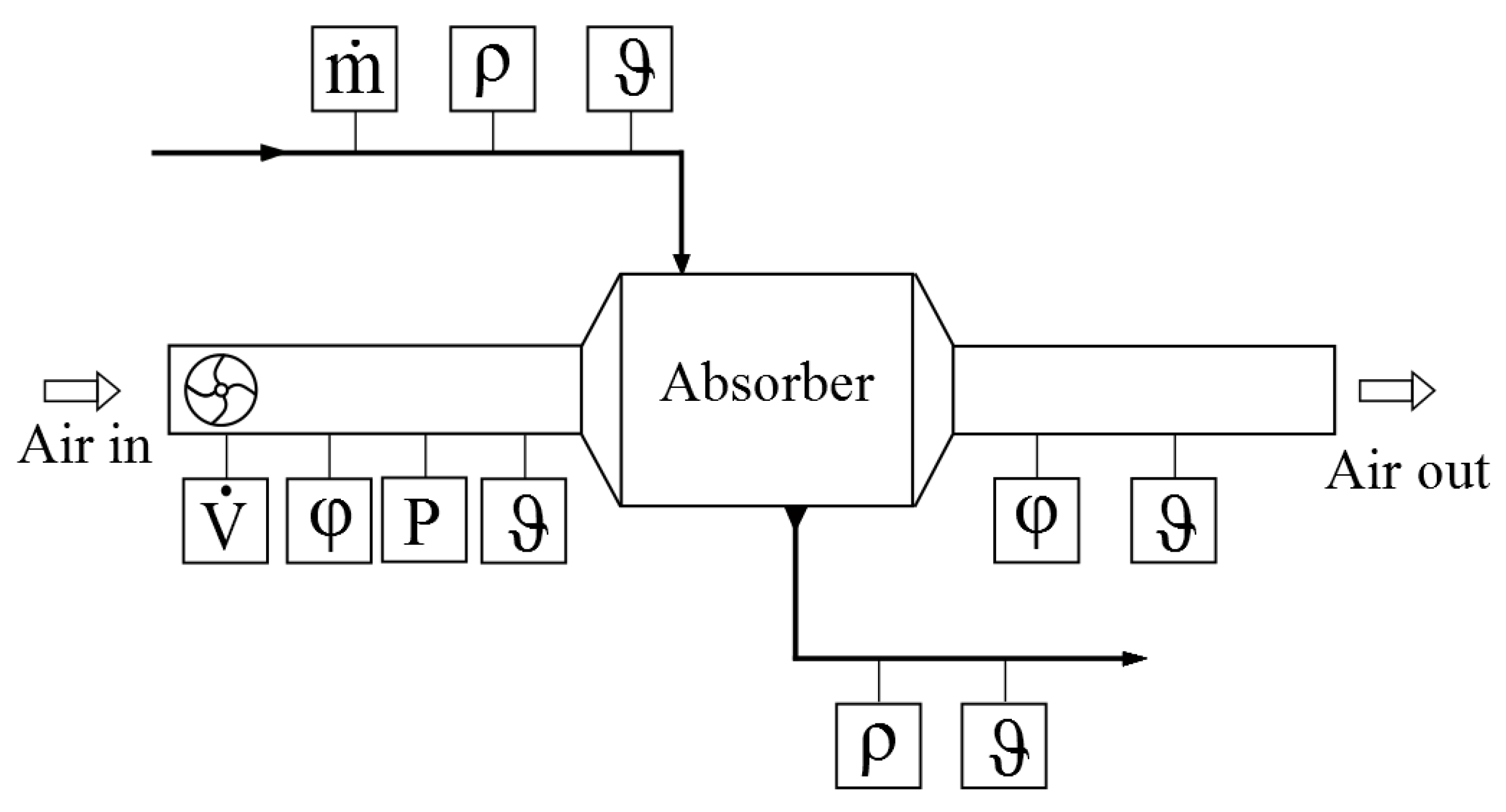
3. Instrumentation and Experimental Setup
4. Numerical Model
5. Results and Discussion
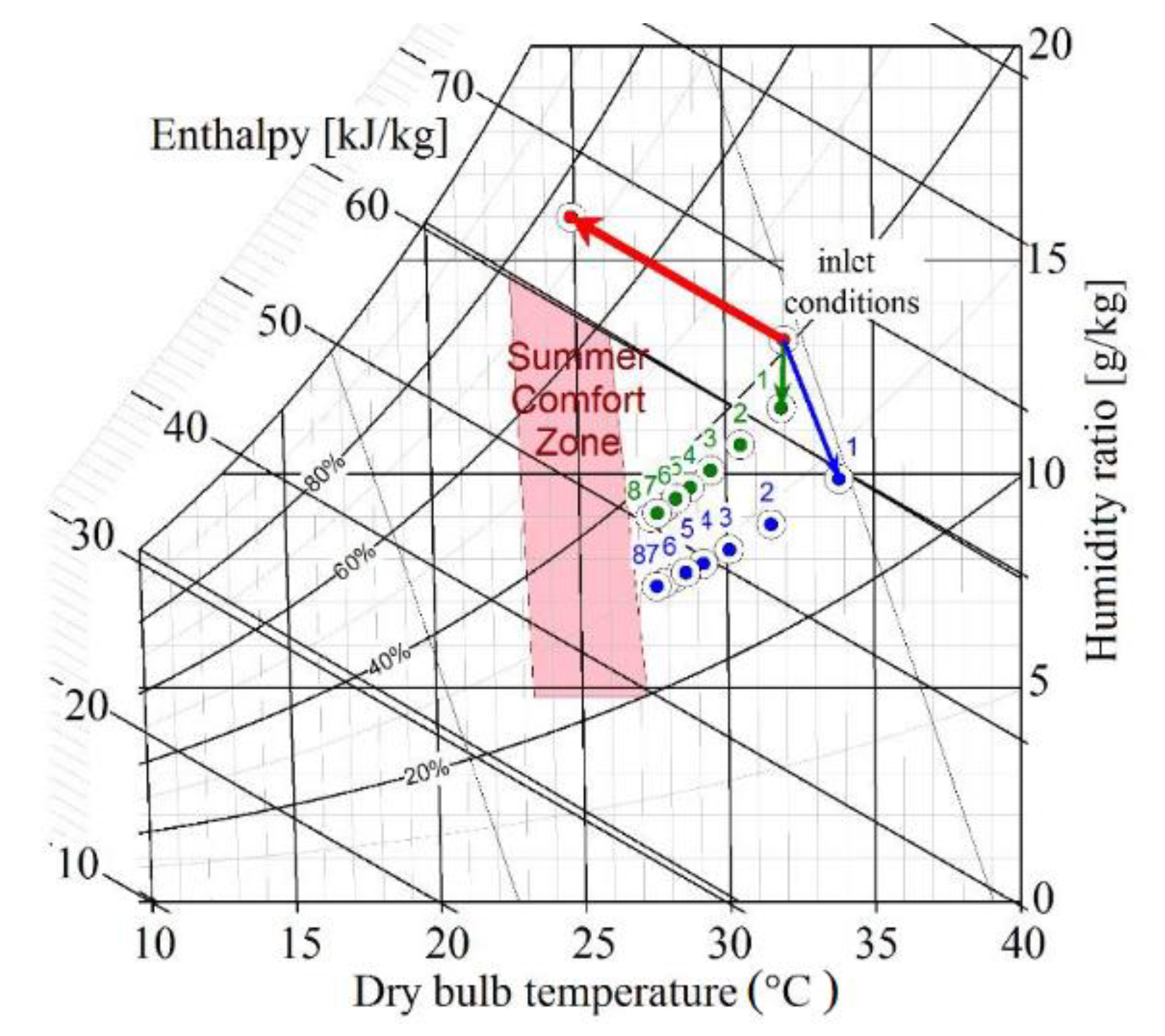
5.1. Conventional Direct Evaporative Cooling
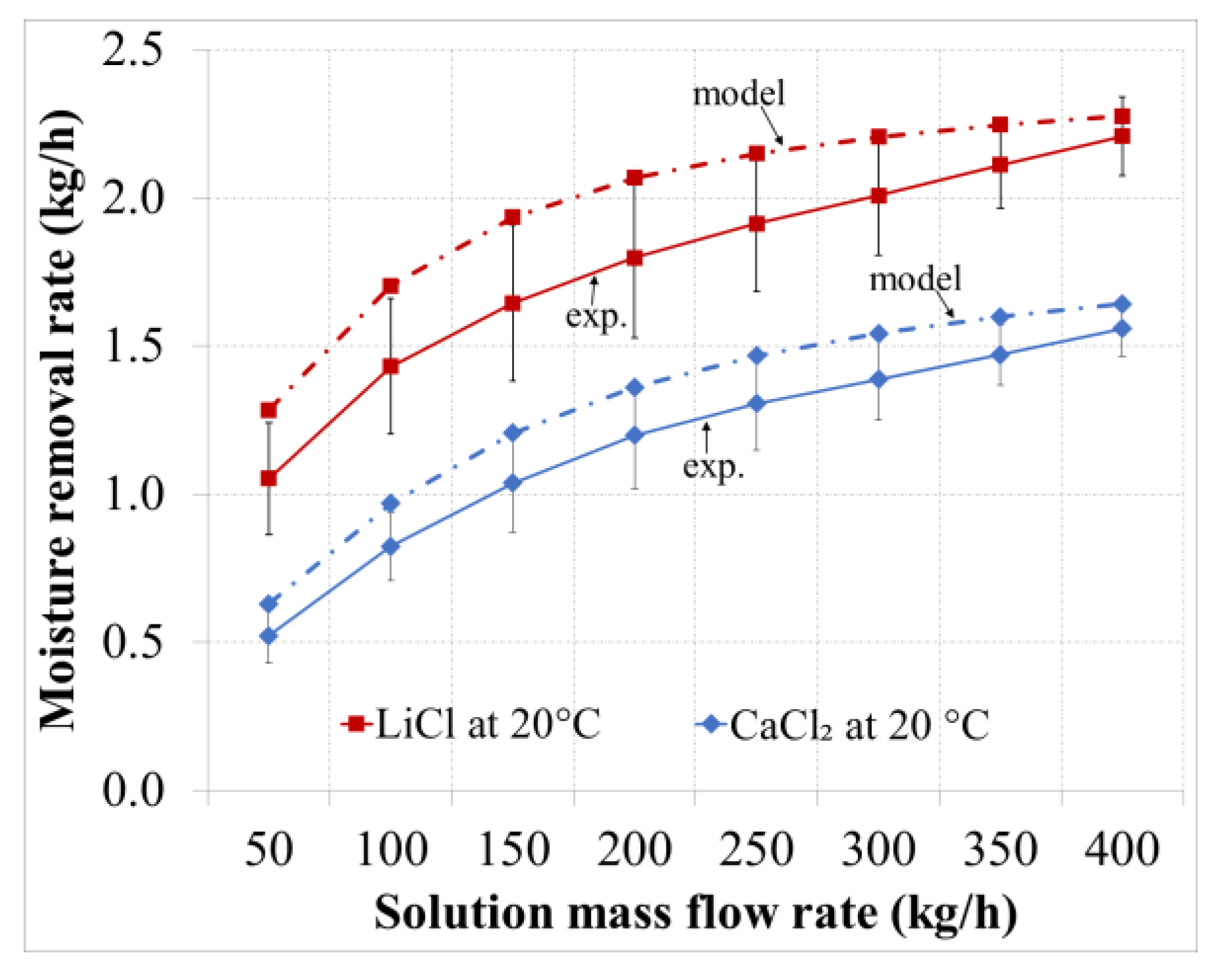
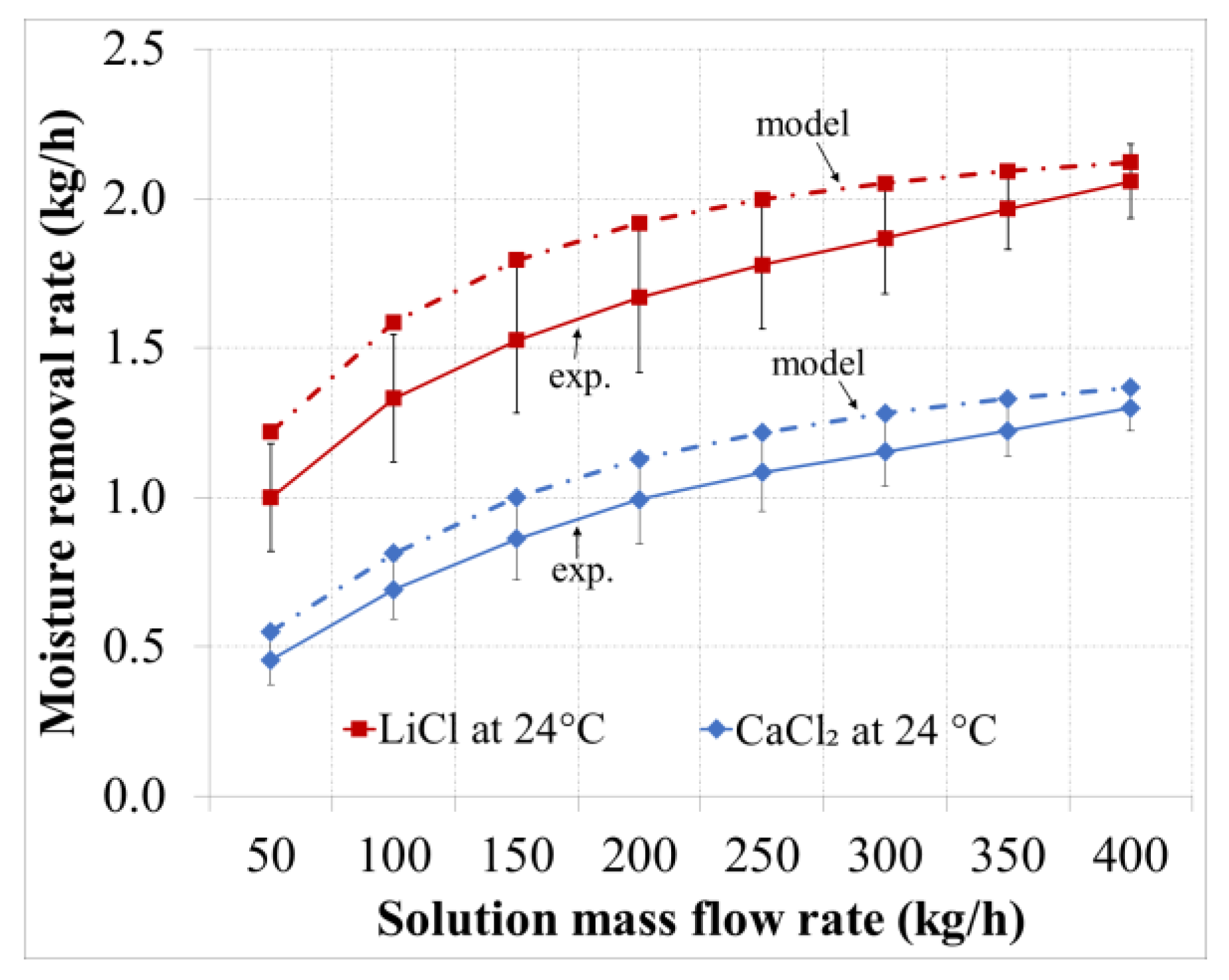
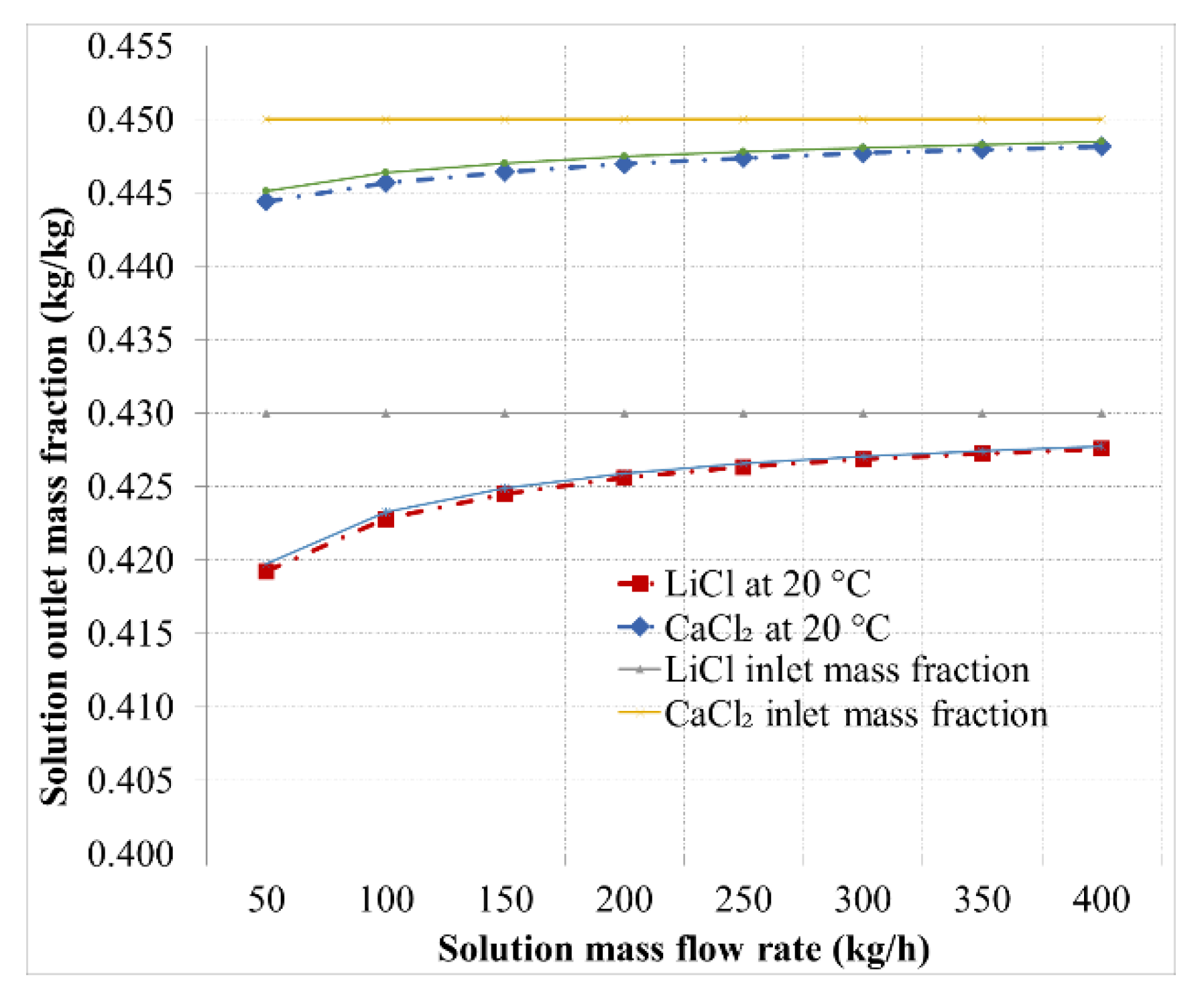
5.2. Modified Desert Cooler: Adiabatic Liquid Desiccant Dehumidification
5.3. Dehumidification Load
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Nomenclature
| Symbol | Meaning | Unit |
| A | Area | |
| heat transfer coefficient | ||
| mass transfer coefficient | ||
| effectiveness | ||
| enthalpy of vaporization | ||
| mass flow rate | ||
| pressure | ||
| air to solution mass flow ratio | ||
| mass of water per mass of salt | ||
| volume | ||
| difference | ||
| thermal conductivity | ||
| humidity ratio | ||
| relative humidity | ||
| density | ||
| temperature | ||
| mass fraction of the desiccant | ||
| Subscripts | ||
| a | air | |
| CaCl2 | calcium chloride | |
| i | inlet conditions | |
| da | dry air | |
| deh | dehumidification | |
| LiCl | lithium chloride as salt | |
| NTU | number of transfer units | |
| o | outlet conditions | |
| s | saturated | |
| sol | solution | |
| v | water vapor | |
| w | water |
References
- Damiano, L.; Dougan, D. ANSI/ASHRAE Standard 62.1-2004. In Encyclopedia of Energy Engineering and Technology—3 Volume Set (Print Version); Capehart, B., Ed.; CRC Press: Boca Raton, FL, USA, 2007; pp. 50–62. [Google Scholar] [CrossRef]
- Abdel-Salam, A.H.; Simonson, C.J. State-of-the-art in liquid desiccant air conditioning equipment and systems. Renew. Sustain. Energy Rev. 2016, 58, 1152–1183. [Google Scholar] [CrossRef]
- International Energy Agency (IEA). World Energy Outlook 2018. ISBN 9789264306776 (PDF). Available online: https://doi.org/10.1787/weo-2018-en (accessed on 27 August 2019).
- Zegenhagen, M.T.; Ricart, C.; Meyer, T.; Kühn, R.; Ziegler, F. Experimental Investigation of A Liquid Desiccant System for Air Dehumidification Working With Ionic Liquids. Energy Procedia 2015, 70, 544–551. [Google Scholar] [CrossRef]
- Wang, S.K. Handbook of Air Conditioning and Refrigeration, 2nd ed.; McGraw-Hill Handbooks; McGraw-Hill: New York, NY, USA, 2001. [Google Scholar]
- Tu, M.; Ren, C.-Q.; Tang, G.-F.; Zhao, Z.-S. Performance comparison between two novel configurations of liquid desiccant air-conditioning system. Build. Environ. 2010, 45, 2808–2816. [Google Scholar] [CrossRef]
- Bishoyi, D.; Sudhakar, K. Experimental performance of a direct evaporative cooler in composite climate of India. Energy Build. 2017, 153, 190–200. [Google Scholar] [CrossRef]
- Lemons, A.R.; Hogan, M.B.; Gault, R.A.; Holland, K.J.; Sobek, E.; Olsen-Wilson, K.A.; Green, B.J. Fungal Metagenomic Analysis of Indoor Evaporative Cooler Environments in the Great Basin Desert Region. J. Allergy Clin. Immunol. 2016, 137, AB181. [Google Scholar] [CrossRef]
- Kim, W.; Dong, H.-W.; Park, J.; Sung, M.; Jeong, J.-W. Impact of an Ultraviolet Reactor on the Improvement of Air Quality Leaving a Direct Evaporative Cooler. Sustainability 2018, 10, 1123. [Google Scholar] [CrossRef]
- Feddema, J.J. A Revised Thornthwaite-Type Global Climate Classification. Phys. Geogr. 2005, 26, 442–466. [Google Scholar] [CrossRef]
- Xuan, Y.M.; Xiao, F.; Niu, X.F.; Huang, X.; Wang, S.W. Research and application of evaporative cooling in China: A Review (I)—Research. Renew. Sustain. Energy Rev. 2012, 16, 3535–3546. [Google Scholar] [CrossRef]
- Kang, D.; Strand, R.K. Analysis of the system response of a spray passive downdraft evaporative cooling system. Build. Environ. 2019, 157, 101–111. [Google Scholar] [CrossRef]
- Yang, Y.; Cui, G.; Lan, C.Q. Developments in evaporative cooling and enhanced evaporative cooling—A review. Renew. Sustain. Energy Rev. 2019, 113, 109230. [Google Scholar] [CrossRef]
- ASHRAE. Ventilation for Acceptable Indoor Air Quality; ASHRAE Standard 62-1999; Thermal Comfort; American Society of Heating, Refrigerating and Air-Conditioning Engineers: Atlanta, GA, USA, 1999. [Google Scholar]
- Arundel, A.; Sterling, E.; Biggin, J.; Sterling, T. Indirect Health Effects of Relative Humidity in Indoor Environments. Environ. Health Perspect. 1986, 65, 351. [Google Scholar] [CrossRef] [PubMed]
- Al-Sulaiman, F. Evaluation of the performance of local fibers in evaporative cooling. Energy Convers. Manag. 2002, 43, 2267–2273. [Google Scholar] [CrossRef]
- Liao, C.-M.; Chiu, K.-H. Wind tunnel modeling the system performance of alternative evaporative cooling pads in Taiwan region. Build. Environ. 2002, 37, 177–187. [Google Scholar] [CrossRef]
- Darwesh, M.; Abouzaher, S.; Fouda, T.; Helmy, M. Effect of Using Pad Manufactured from Agricultural Residues on the Performance of Evaporative Cooling System. Jordan J. Agric. Sci. 2009, 5, 17. [Google Scholar]
- Ahmed, E.M.; Abaas, O.; Ahmed, M.; Ismail, M.R. Performance evaluation of three different types of local evaporative cooling pads in greenhouses in Sudan. Saudi J. Biol. Sci. 2011, 18, 45–51. [Google Scholar] [CrossRef]
- Malli, A.; Seyf, H.R.; Layeghi, M.; Sharifian, S.; Behravesh, H. Investigating the performance of cellulosic evaporative cooling pads. Energy Convers. Manag. 2011, 52, 2598–2603. [Google Scholar] [CrossRef]
- Harby, K.; Al-Amri, F. An investigation on energy savings of a split air-conditioning using different commercial cooling pad thicknesses and climatic conditions. Energy 2019, 182, 321–336. [Google Scholar] [CrossRef]
- Wang, J.; Meng, Q.; Zhang, L.; Zhang, Y.; He, B.-J.; Zheng, S.; Santamouris, M. Impacts of the water absorption capability on the evaporative cooling effect of pervious paving materials. Build. Environ. 2019, 151, 187–197. [Google Scholar] [CrossRef]
- Sharma, A.K.; Bishnoi, P. Development and testing of natural draught desert cooler. Int. J. Sci. Eng. Appl. 2013, 2, 19–22. [Google Scholar] [CrossRef]
- Elhelw, M. Performance evaluation for solar liquid desiccant air dehumidification system. Alex. Eng. J. 2016, 55, 933–940. [Google Scholar] [CrossRef][Green Version]
- Jaradat, M.; Fleig, D.; Vajen, K.; Jordan, U. Investigations of a Dehumidifier in a Solar-Assisted Liquid Desiccant Demonstration Plant. J. Sol. Energy Eng. 2018, 141, 031001. [Google Scholar] [CrossRef]
- Öberg, V.; Goswami, D.Y. Experimental Study of the Heat and Mass Transfer in a Packed Bed Liquid Desiccant Air Dehumidifier. J. Sol. Energy Eng. 1998, 120, 289. [Google Scholar] [CrossRef]
- Lowenstein, A. Review of Liquid Desiccant Technology for HVAC Applications. HVAC&R Res. 2008, 14, 819–839. [Google Scholar] [CrossRef]
- Kessling, W.; Laevemann, E.; Peltzer, M. Energy storage in open cycle liquid desiccant cooling systems. Int. J. Refrig. 1998, 21, 150–156. [Google Scholar] [CrossRef]
- Yu, N.; Wang, R.Z.; Wang, L.W. Sorption thermal storage for solar energy. Prog. Energy Combust. Sci. 2013, 39, 489–514. [Google Scholar] [CrossRef]
- Kabeel, A.E.; Khalil, A.; Elsayed, S.S.; Alatyar, A.M. Theoretical investigation on energy storage characteristics of a solar liquid desiccant air conditioning system in Egypt. Energy 2018, 158, 164–180. [Google Scholar] [CrossRef]
- Jaradat, M. Construction and Analysis of Heat-and Mass Exchangers for Liquid Desiccant Systems. Ph.D. Thesis, Universität Kassel, Kassel, Germany, 2016. [Google Scholar]
- Rafique, M.M.; Gandhidasan, P.; Bahaidarah, H.M.S. Liquid desiccant materials and dehumidifiers—A review. Renew. Sustain. Energy Rev. 2016, 56, 179–195. [Google Scholar] [CrossRef]
- Park, J.-Y.; Yoon, D.-S.; Li, S.; Park, J.; Bang, J.-I.; Sung, M.; Jeong, J.-W. Empirical analysis of indoor air quality enhancement potential in a liquid-desiccant assisted air conditioning system. Build. Environ. 2017, 121, 11–25. [Google Scholar] [CrossRef]
- Al-Farayedhi, A.A.; Gandhidasan, P.; Al-Mutairi, M.A. Evaluation of heat and mass transfer coefficients in a gauze-type structured packing air dehumidifier operating with liquid desiccant. Int. J. Refrig. 2002, 25, 330–339. [Google Scholar] [CrossRef]
- Abdul-Wahab, S.A.; Zurigat, Y.H.; Abu-Arabi, M.K. Predictions of moisture removal rate and dehumidification effectiveness for structured liquid desiccant air dehumidifier. Energy 2004, 29, 19–34. [Google Scholar] [CrossRef]
- Elsarrag, E. Performance study on a structured packed liquid desiccant regenerator. Sol. Energy 2006, 80, 1624–1631. [Google Scholar] [CrossRef]
- ASHRAE. Handbook—Fundamentals; Chapter 6: Psychrometrics; American Society of Heating, Refrigerating and Air-Conditioning Engineers: Atlanta, GA, USA, 1997. [Google Scholar]
- Conde, M.R. Properties of aqueous solutions of lithium and calcium chlorides: Formulations for use in air conditioning equipment design. Int. J. Therm. Sci. 2004, 43, 367–382. [Google Scholar] [CrossRef]
- Stevens, D.I.; Braun, J.E.; Klein, S.A. An effectiveness model of liquid-desiccant system heat/mass exchangers. Sol. Energy 1989, 42, 449–455. [Google Scholar] [CrossRef]
- Baehr, H.D.; Stephan, K. Heat and Mass Transfer, 3rd ed.; Springer-Verlag: Berlin, Germany, 2011. [Google Scholar]
- ASHRAE. Handbook—Fundamentals; Chapter 8: Thermal Comfort; American Society of Heating, Refrigerating and Air-Conditioning Engineers: Atlanta, GA, USA, 1997. [Google Scholar]




| Instrument | Model | Uncertainty | Range of Operation |
|---|---|---|---|
| Air temperature | Hygroflex 420 | 0.5 K | −50–100 °C |
| Relative humidity | Hygroflex 420 | 2% | 0%–100% |
| Air volume flow rate | Optiswirl 4070 DN 150 | 3% | 370–4800 m3/h |
| Solution temperature | Pt100 | 0.5 K | |
| Solution volume flow rate | Optiflux 1050 | 1% | 20–3900 L/h |
| Solution density | L-Dens 323 | 1 g/cm3 | 0.5–2 g/cm3 |
| 50–400 | 400 | 32 | 13.12 | 20–24 | 0.43 | 0.45 |
| ° | |||||
|---|---|---|---|---|---|
| 400 | 32.0 | 13.12 | 24.7 | 16.07 | 0.68 |
| Air Outlet Conditions Using LiCl-H2O as Desiccant | Air Outlet Conditions Using CaCl2-H2O as Desiccant | |||||||
|---|---|---|---|---|---|---|---|---|
| Exp. | ||||||||
| 1 | 33.8 | 9.87 | 59.4 | 0.71 | 31.9 | 11.53 | 61.6 | 0.46 |
| 2 | 31.5 | 8.81 | 54.3 | 1.27 | 30.5 | 10.67 | 58.0 | 0.86 |
| 3 | 30.1 | 8.22 | 51.3 | 1.60 | 29.5 | 10.07 | 55.4 | 1.15 |
| 4 | 29.2 | 7.89 | 49.5 | 1.80 | 28.8 | 9.67 | 53.7 | 1.34 |
| 5 | 28.6 | 7.68 | 48.4 | 1.93 | 28.3 | 9.41 | 52.5 | 1.47 |
| 6 | 28.2 | 7.54 | 47.6 | 2.02 | 27.9 | 9.22 | 51.6 | 1.57 |
| 7 | 27.9 | 7.43 | 47.0 | 2.08 | 27.6 | 9.07 | 51.0 | 1.64 |
| 8 | 27.6 | 7.36 | 46.6 | 2.13 | 27.4 | 8.97 | 50.5 | 1.70 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Jaradat, M.; Al-Addous, M.; Albatayneh, A. Adaption of an Evaporative Desert Cooler into a Liquid Desiccant Air Conditioner: Experimental and Numerical Analysis. Atmosphere 2020, 11, 40. https://doi.org/10.3390/atmos11010040
Jaradat M, Al-Addous M, Albatayneh A. Adaption of an Evaporative Desert Cooler into a Liquid Desiccant Air Conditioner: Experimental and Numerical Analysis. Atmosphere. 2020; 11(1):40. https://doi.org/10.3390/atmos11010040
Chicago/Turabian StyleJaradat, Mustafa, Mohammad Al-Addous, and Aiman Albatayneh. 2020. "Adaption of an Evaporative Desert Cooler into a Liquid Desiccant Air Conditioner: Experimental and Numerical Analysis" Atmosphere 11, no. 1: 40. https://doi.org/10.3390/atmos11010040
APA StyleJaradat, M., Al-Addous, M., & Albatayneh, A. (2020). Adaption of an Evaporative Desert Cooler into a Liquid Desiccant Air Conditioner: Experimental and Numerical Analysis. Atmosphere, 11(1), 40. https://doi.org/10.3390/atmos11010040





