Integrated Correction Algorithm for X Band Dual-Polarization Radar Reflectivity Based on CINRAD/SA Radar
Abstract
1. Introduction
2. Observations and RIC Algorithm
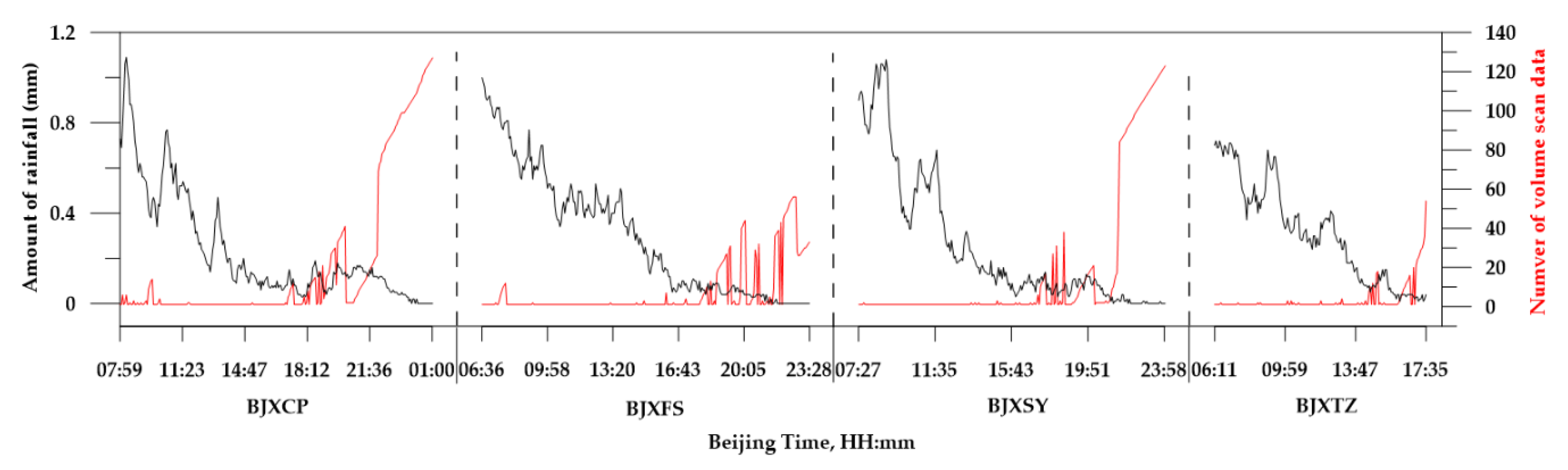
2.1. Observations
2.2. RIC Algorithm
3. Results
3.1. Ratio a
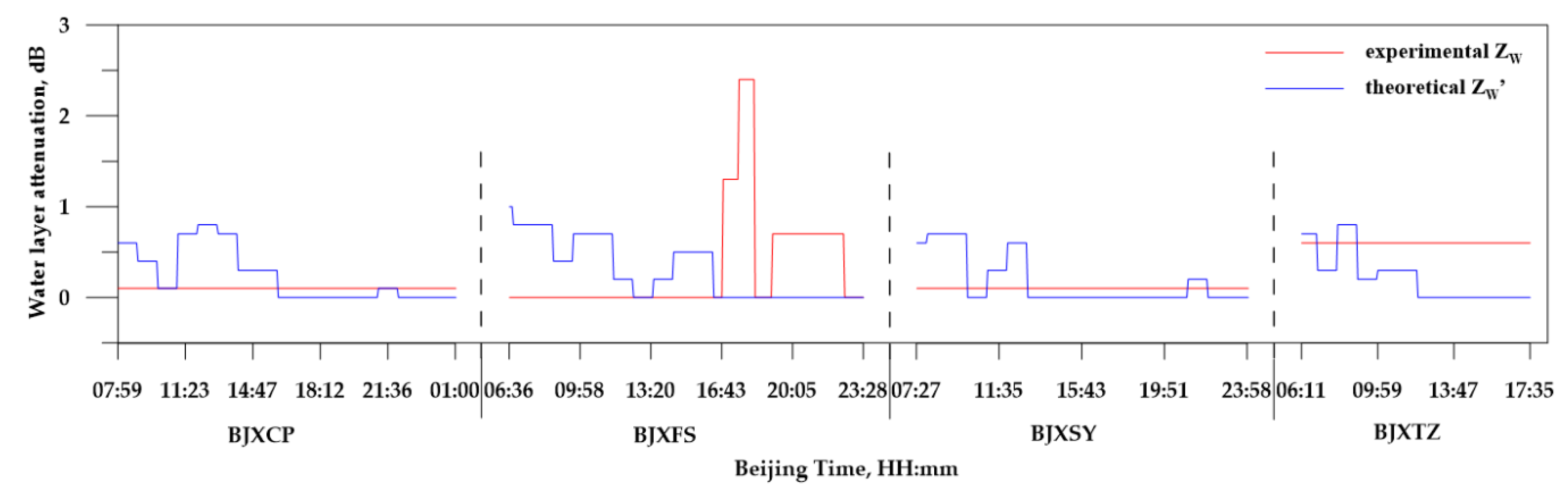
3.2. Reflectivity Attenuation of Water Layer on Antenna Cover
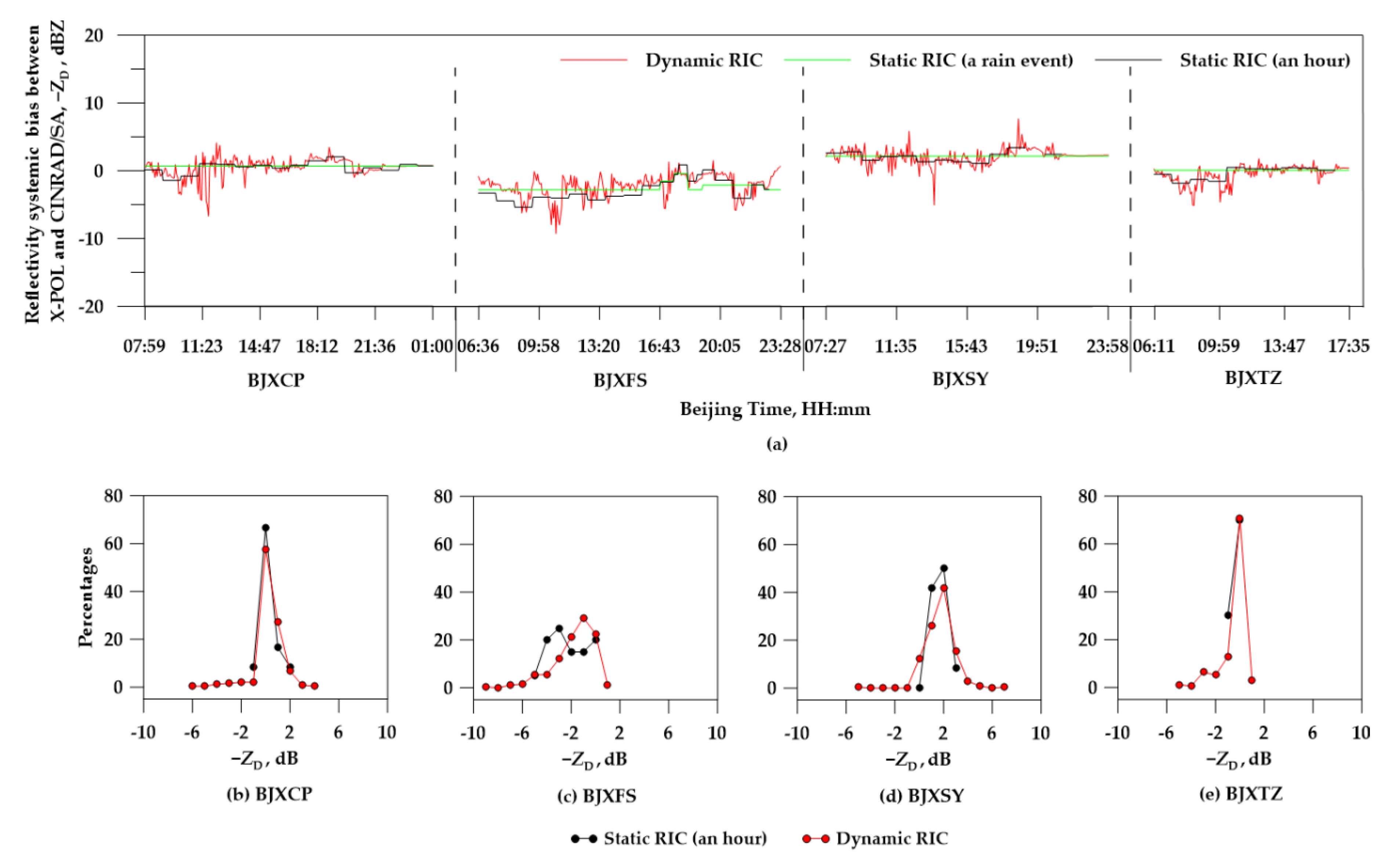
3.3. Systemic Reflectivity Bias between X-POL and CINRAD/SA Radars
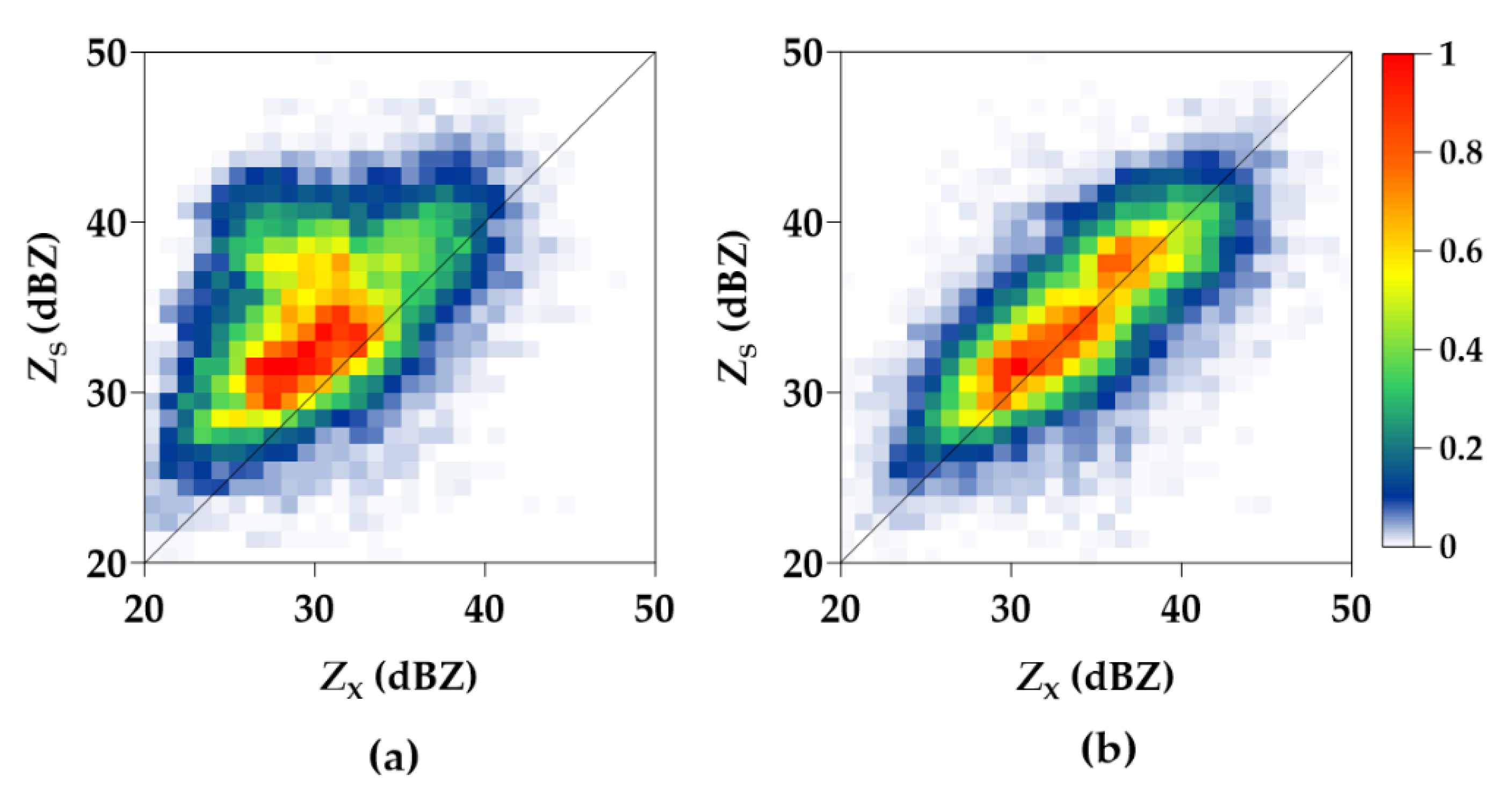
4. Evaluations of Rain Attenuation Correction
5. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Chandrasekar, V.; Lim, S.; Bharadwaj, N.; Li, W.; McLaughlin, D.; Bringi, V.N.; Gorgucci, E. Principles of networked weather radar operation at attenuating frequencies. In Proceedings of the Third European Conference on Radar in Meteorology and Hydrology (ERAD), Gotland, Sweden, 6–10 September 2004; pp. 109–114. [Google Scholar]
- Wang, Y.; Chandrasekar, V. Quantitative Precipitation Estimation in the CASA X-band Dual-Polarization Radar Network. J. Atmos. Ocean. Technol. 2010, 27, 1665–1676. [Google Scholar] [CrossRef]
- Bringi, V.N.; Chandrasekar, V.; Balakrishnan, N.; Zrnić, D.S. An examination of propagation effects in rainfall on radar measurements at microwave frequencies. J. Atmos. Ocean. Technol. 1990, 7, 829–840. [Google Scholar] [CrossRef]
- Hitschfeld, W.; Bordan, J. Errors inherent in the radar measurement of rainfall at attenuating wavelengths. J. Atmos. Ocean. Technol. 1954, 11, 58–67. [Google Scholar] [CrossRef]
- Xiaoping, X.; Zhang, J. Reflectivity Attenuation of Water Layer over Radar Antenna Cover. Meteorol. Sci. Technol. 1981, S2, 57–58. (In Chinese) [Google Scholar]
- Zhao, H.; Chen, Z.; Zhou, F. Real-Time Attenuation Correction for Weather Radar Reflectivity Factor. Plateau Meteorol. 2003, 22, 365–370. (In Chinese) [Google Scholar]
- Jameson, A.R. The Effect of Temperature on Attenuation-Correction Schemes in Rain Using Polarization Propagation Differential Phase Shift. J. Appl. Meteor. 1992, 31, 1106–1120. [Google Scholar] [CrossRef]
- Matrosov, S.Y.; Clark, K.A.; Martner, B.E.; Tokay, A. X-band polarimetric radar measurements of rainfall. J. Appl. Meteor. 2002, 41, 941–952. [Google Scholar] [CrossRef]
- Park, S.G.; Maki, M.; Iwanami, K.; Bringi, V.N.; Chandrasekar, V. Correction of radar reflectivity and differential reflectivity for rain attenuation at X band. Part II: Evaluation and application. J. Atmos. Ocean. Technol. 2005, 22, 1633–1655. [Google Scholar] [CrossRef]
- Testud, J.; Le Bouar, E.; Obligis, E.; Ali-Mehenni, M. The rain profiling algorithm applied to polarimetric weather radar. J. Atmos. Ocean. Technol. 2000, 17, 332–356. [Google Scholar] [CrossRef]
- Matrosov, S.Y.; Kennedy, P.C.; Cifelli, R. Experimentally based estimates of relations between X-band radar signal attenuation characteristics and differential phase in rain. J. Atmos. Ocean. Technol. 2014, 31, 2442–2450. [Google Scholar] [CrossRef][Green Version]
- Gu, J.-Y.; Ryzhkov, A.; Zhang, P.; Neilley, P.; Knight, M.; Wolf, B.; Lee, D.-I. Polarimetric attenuation correction in heavy rain at C band. J. Appl. Meteor. 2011, 50, 39–58. [Google Scholar] [CrossRef]
- Bringi, V.N.; Keenan, T.D.; Chandrasekar, V. Correcting C-band radar reflectivity and differential reflectivity data for rain attenuation: A self-consistent method with constraints. Trans. IEEE Geosci. Remote Sens. 2001, 39, 1906–1915. [Google Scholar] [CrossRef]
- Carey, L.D.; Rutledge, S.A.; Ahijevych, D.A.; Keenan, T.D. Correcting propagation effects in C-band polarimetric radar observations of tropical convection using differential propagation phase. J. Appl. Meteorol. 2000, 39, 1405–1433. [Google Scholar] [CrossRef]
- Ryzhkov, A.V. The Impact of Beam Broadening on the Quality of Radar Polarimetric Data. J. Atmos. Ocean. Technol. 2007, 24, 729–744. [Google Scholar] [CrossRef]
- Vulpiani, G.; Tabary, P.; Parent du Chatelet, J.; Marzano, F.S. Comparison of advanced radar polarimetric techniques for operational attenuation correction at C band. J. Atmos. Ocean. Technol. 2008, 25, 1118–1135. [Google Scholar] [CrossRef]
- Doviak, R.J.; Zrnic, D.S.; Schotland, R.M. Doppler Radar and Weather Observations; Elsevier: Amsterdam, The Netherlands, 1993; Volume 33, 4531p. [Google Scholar]
- Bi, Y.; Liu, J.; Duan, S.; Lv, D.; Su, D.; Chen, Y. Attenuation correction of reflectivity for X-band dual-polarization radar. Chin. J. Atmos. Sci. 2012, 36, 495–506. (In Chinese) [Google Scholar]
- Xiao, Y.J.; Wang, B.; Chen, X.H.; Cao, J.W.; Yang, X.M. Differential Phase Data Quality Control of Mobile X-Band Dual-Polarimetric Doppler Weather Radar. Plateau Meteorol. 2012, 31, 223–230. (In Chinese) [Google Scholar]
- Zrnic, D.S.; Melnikov, V.M.; Ryzhkov, A.V. Correlation Coefficients between Horizontally and Vertically Polarized Returns from Ground Clutter. J. Atmos. Ocean. Technol. 2006, 23, 381–394. [Google Scholar] [CrossRef]
- Matrosov, S.Y. Assessment of radar signal attenuation caused by the melting hydrometeor layer. Trans. IEEE Geosci. Remote Sens. 2008, 46, 1039–1047. [Google Scholar] [CrossRef]
- Green, A.W. An approximation for the shapes of large raindrops. J. Appl. Meteorol. 1975, 14, 1578–1583. [Google Scholar] [CrossRef]
- Pruppacher, H.R.; Beard, K.V. A wind tunnel investigation of the internal circulation and shape of water drops falling at terminal velocity in air. Quart. J. R. Meteorol. Soc. 1970, 96, 247–256. [Google Scholar] [CrossRef]
- Keenan, T.D.; Zrnic, D.S.; Carey, L.; May, P.; Rutledge, S. Sensitivity of C-band polarimetric variables to propagation and backscatter effects in rain. In Proceedings of the 28th Conference on Radar Meteorology, Austin, TX, USA, 7–12 September 1997; pp. 13–14. [Google Scholar]
- Keenan, T.D.; Carey, L.D.; Zrnić, D.S.; May, P.T. Sensitivity of 5-cm wavelength polarimetric radar variables to raindrop axial ratio and drop size distribution. J. Appl. Meteorol. 2001, 40, 526–545. [Google Scholar] [CrossRef]
- Andsager, K.; Beard, K.V.; Laird, N.F. Laboratory measurements of axis ratios for large raindrops. J. Atmos. Sci. 1999, 56, 2673–2683. [Google Scholar] [CrossRef]
- Kim, D.S.; Maki, M.; Lee, D.I. Retrieval of Three-Dimensional Raindrop Size Distribution Using X-Band Polarimetric Radar Data. J. Atmos. Ocean. Technol. 2010, 27, 1265–1285. [Google Scholar] [CrossRef]
- Anagnostou, M.N.; Anagnostou, E.N.; Vivekanandan, J.; Ogden, F.L. Comparison of two raindrop size distribution retrieval algorithms for X-band dual polarization observations. J. Hydrometeorol. 2008, 9, 589–600. [Google Scholar] [CrossRef]
- Matrosov, S.Y.; Kingsmill, D.E.; Martner, B.E.; Ralph, F.M. The utility of X-band polarimetric radar for quantitative estimates of rainfall parameters. J. Hydrometeorol. 2005, 6, 248–262. [Google Scholar] [CrossRef]
- Matrosov, S.Y. Evaluating Polarimetric X-Band Radar Rainfall Estimators during HMT. J. Atmos. Ocean. Technol. 2009, 27, 122–134. [Google Scholar] [CrossRef]
- Beard, K.V.; Chuang, C. A new model for the equilibrium shape of raindrops. J. Atmos. Sci. 1987, 44, 1509–1524. [Google Scholar] [CrossRef]
- Snyder, J.C.; Bluestein, H.B.; Zhang, G.; Frasier, S.J. Attenuation Correction and Hydrometeor Classification of High-Resolution, X-band, Dual-Polarized Mobile Radar Measurements in Severe Convective Storms. J. Atmos. Ocean. Technol. 2010, 27, 1979–2001. [Google Scholar] [CrossRef]
- Brandes, E.A.; Zhang, G.; Vivekanandan, J. Experiments in rainfall estimation with a polarimetric radar in a subtropical environment. J. Appl. Meteorol. 2002, 41, 674–685. [Google Scholar] [CrossRef]
- Zhang, G. Weather Radar Polarimetry; CRC Press: Boca Raton, FL, USA, 2016. [Google Scholar]
- Gourley, J.J.; Kaney, B.; Maddox, R.A. Evaluating the calibrations of radars: A software approach. In Proceedings of the 31st International Conference on Radar Meteorology, Seattle, WA, USA, 6 August 2003; pp. 459–462. [Google Scholar]
- He, Y.; Lv, D.; Xiao, H.; Lei, H.; Liu, D.; Duan, S. Attenuation correction of reflectivity for X-band dual polarization radar. Chin. J. Atmos. Sci. 2009, 33, 1027–1037. (In Chinese) [Google Scholar]
- Ryzhkov, A.; Zrnic, D.S. Precipitation and Attenuation Measurements at a 10-cm Wavelength. J. Appl. Meteorol. 2010, 34, 2120–2134. [Google Scholar] [CrossRef]
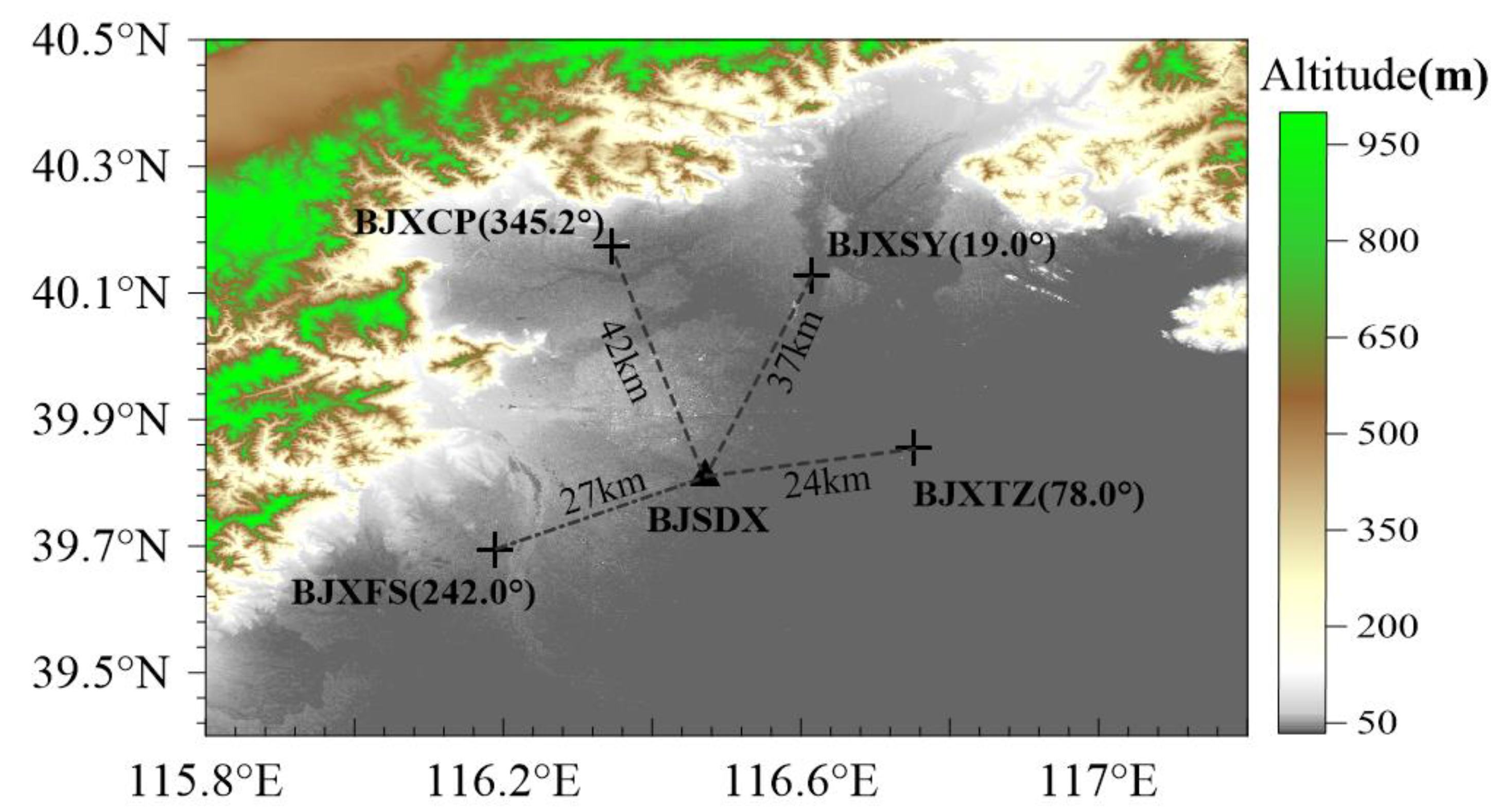
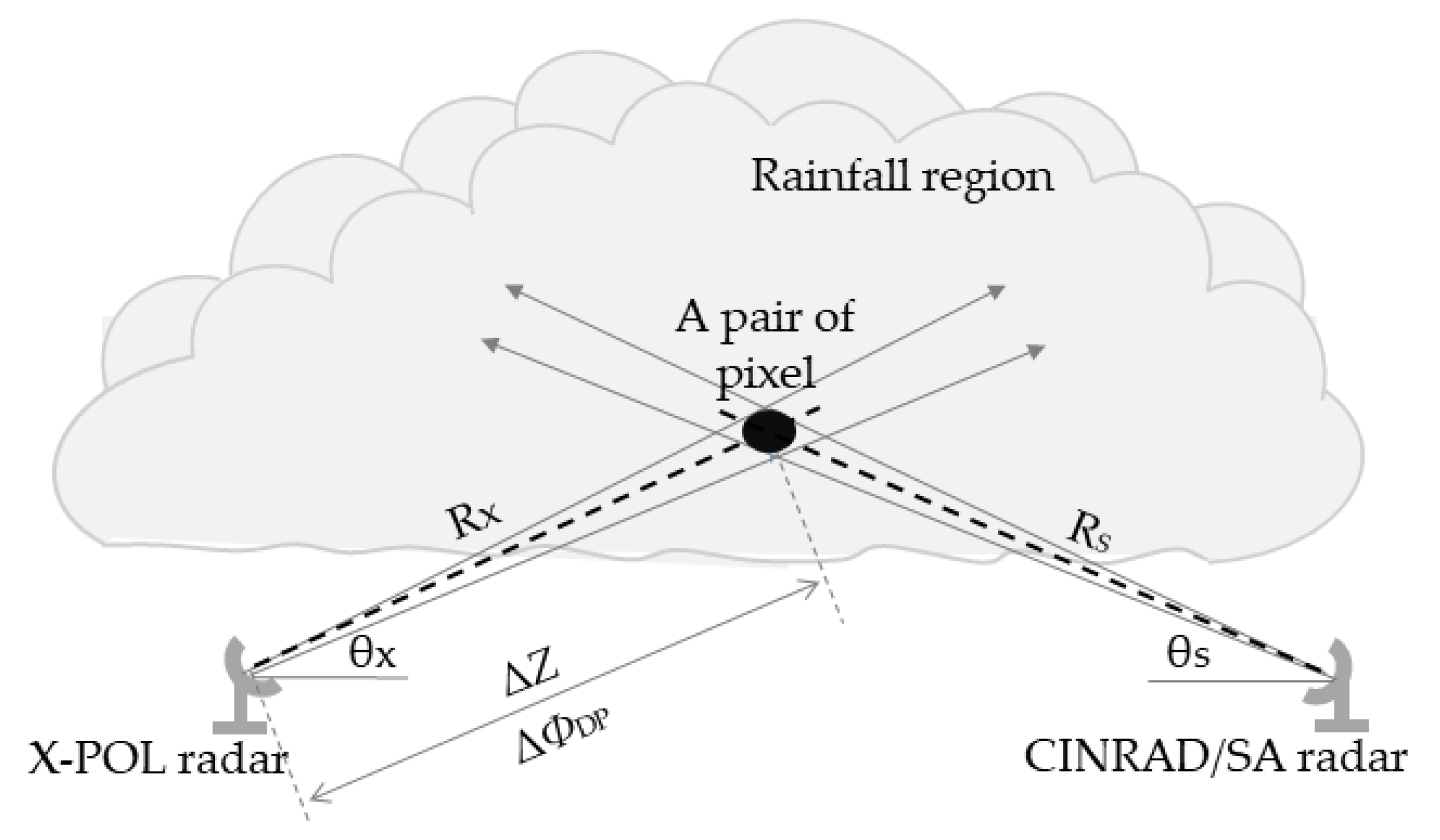
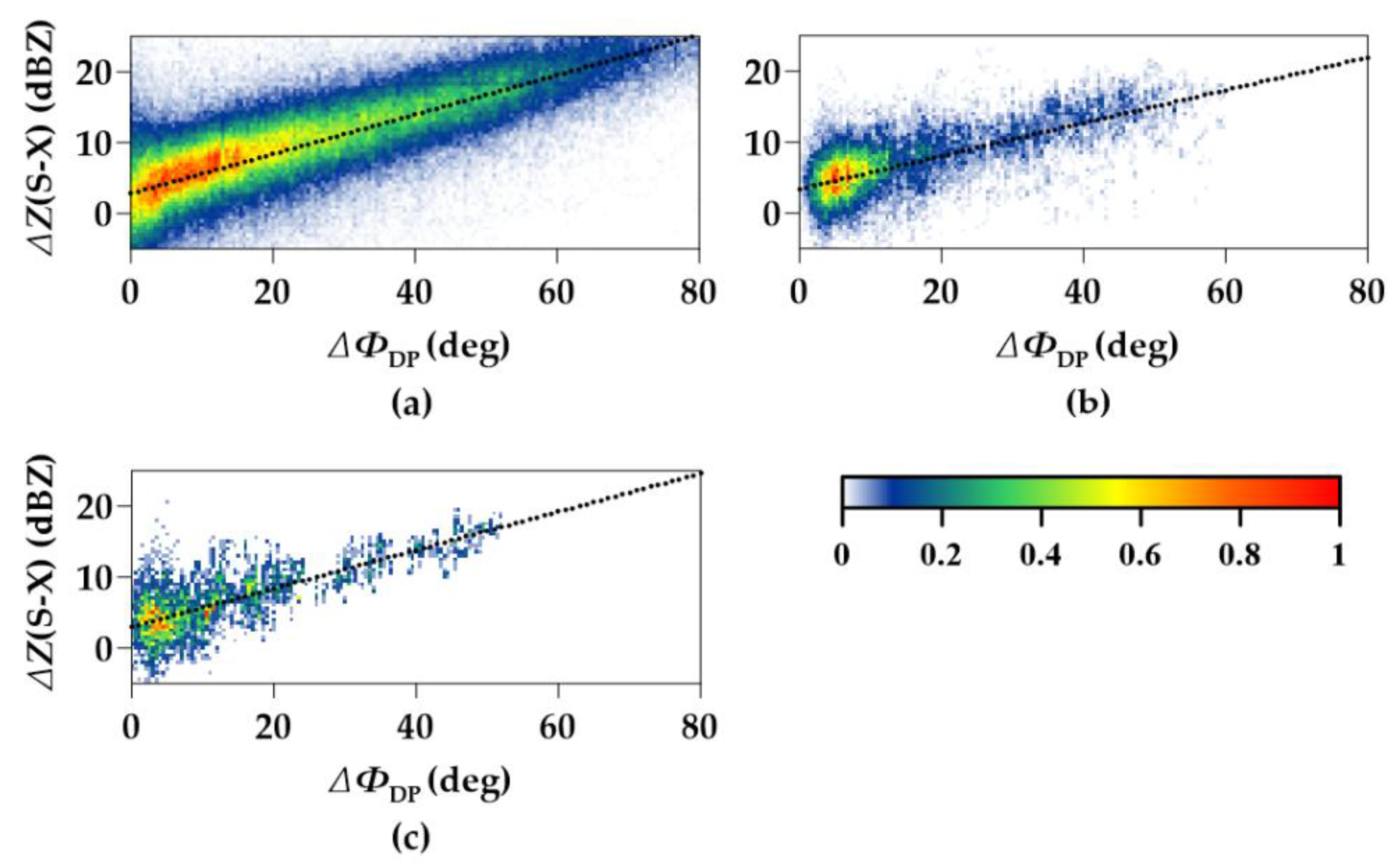
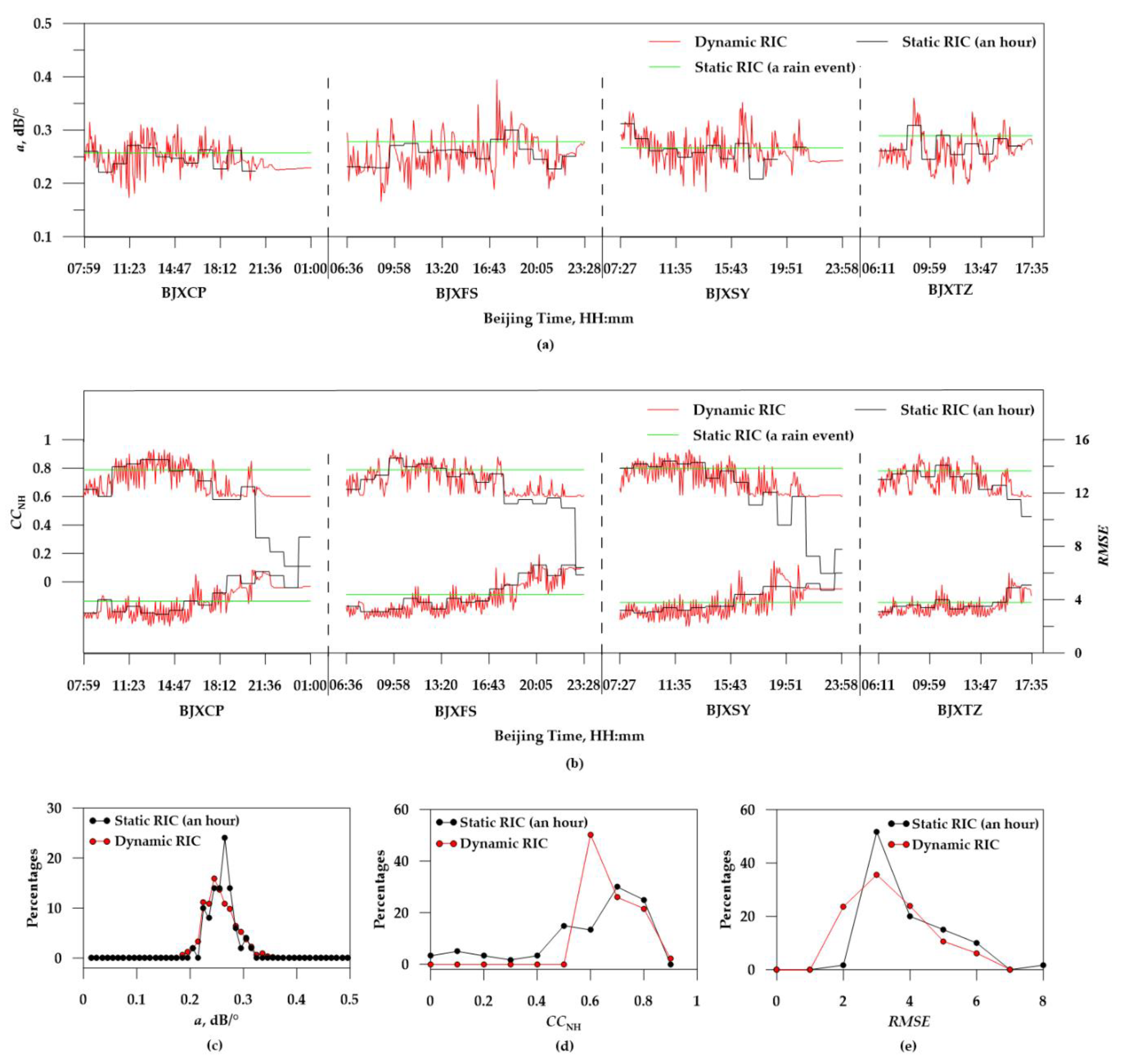

| Parameter | X-POL Radar | CINRAD/SA Radar |
|---|---|---|
| Frequency | 9300–9500 MHz | 2700–3000 MHz |
| Antenna cover diameter | ≥4 m | 11.9 m |
| Polarization | Linear H and V | Linear H |
| Volume coverage patterns | VCP 21 | VCP 21 |
| Time of VCP 21 | 4 min | 6 min |
| Range resolution | 75 m | Z (1000 m), VD and W (250 m) |
| Observation range | 90 km | Z (230 km), VD and W (150 km) |
| Measurement accuracy | Z (≤ 1), VD (≤ 1), W (≤ 1), ρHV(0) (≤ 0.01), ZDR (≤ 0.2), ΦDP (≤ 3), KDP (≤ 0.2) | Z (≤ 1), VD and W (≤ 1) |
| Article | a (dB deg−1) | Temperature (°C) | Mean Drop Aspect Ratio–Size Relations |
|---|---|---|---|
| Bringi et al., (1990) [3] | 0.247 | 15 | Green et al., (1975) [22] |
| Jameson et al., (1992) [7] | 0.248–0.195 | 0–40 | Pruppacher et al., (1970) [23] |
| Testud et al., (2000) [10] | 0.315 | Keenan et al., (1997) [24] | |
| Matrosov et al., (2002) [8] | 0.22 | 5 | Pruppacher et al., (1970) [23,24] |
| Park et al., (2005) [9] | 0.173–0.315 | 15 | Keenan et al., (2001) [25], Andsager et al., (1999) [26], Park et al., (2005) [9] |
| Kim et al., (2010) [27] | 0.1–0.6 | 0–30 | Anagnostou et al., (2008) [28], Matrosov et al., (2005) [29] |
| Matrosov et al., (2009) [30] | 0.23–0.28 | Beard et al., (1987) [31] | |
| Snyder et al., (2010) [32] | 0.313 | 10 | Brandes et al., (2002) [33] |
| Matrosov et al., (2014) [11] | 0.20–0.31 |
| Fitting Method | Fitting Relations | CCNH | RMSE | Data Number |
|---|---|---|---|---|
| Static RIC (a rain event) | ΔZ(S–X) = 0.279 ΔΦDP(X) + 2.8 | 0.80 | 4.4 | 266 |
| Static RIC (an hour) | ΔZ(S–X) = 0.232 ΔΦDP(X) + 3.4 | 0.66 | 3.4 | 8 |
| Dynamic RIC | ΔZ(S–X) = 0.270 ΔΦDP(X) + 3.0 | 0.76 | 2.8 | 1 |
| Parameter Interval | Dynamic RIC (%) | Static RIC (An Hour) (%) | Static RIC (A Rain Event) (%) | Empirical Ratio a = 0.247 dB deg−1 (%) |
|---|---|---|---|---|
| MAD = 0 | 85.0 | 100.0 | 60.0 | 43.3 |
| CC > 0.7 | 46.7 | 52.9 | 48.3 | 44.9 |
| SD ≤ 3 | 49.9 | 56.8 | 48.3 | 48.3 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, C.; Wu, C.; Liu, L.; Liu, X.; Chen, C. Integrated Correction Algorithm for X Band Dual-Polarization Radar Reflectivity Based on CINRAD/SA Radar. Atmosphere 2020, 11, 119. https://doi.org/10.3390/atmos11010119
Wang C, Wu C, Liu L, Liu X, Chen C. Integrated Correction Algorithm for X Band Dual-Polarization Radar Reflectivity Based on CINRAD/SA Radar. Atmosphere. 2020; 11(1):119. https://doi.org/10.3390/atmos11010119
Chicago/Turabian StyleWang, Chao, Chong Wu, Liping Liu, Xi Liu, and Chao Chen. 2020. "Integrated Correction Algorithm for X Band Dual-Polarization Radar Reflectivity Based on CINRAD/SA Radar" Atmosphere 11, no. 1: 119. https://doi.org/10.3390/atmos11010119
APA StyleWang, C., Wu, C., Liu, L., Liu, X., & Chen, C. (2020). Integrated Correction Algorithm for X Band Dual-Polarization Radar Reflectivity Based on CINRAD/SA Radar. Atmosphere, 11(1), 119. https://doi.org/10.3390/atmos11010119





