Anatomy of a Cyclonic Eddy in the Kuroshio Extension Based on High-Resolution Observations
Abstract
1. Introduction
2. Data
2.1. Field Data
2.2. Satellite Data
2.3. Other Data
3. Observational Results
3.1. Satellite Observations
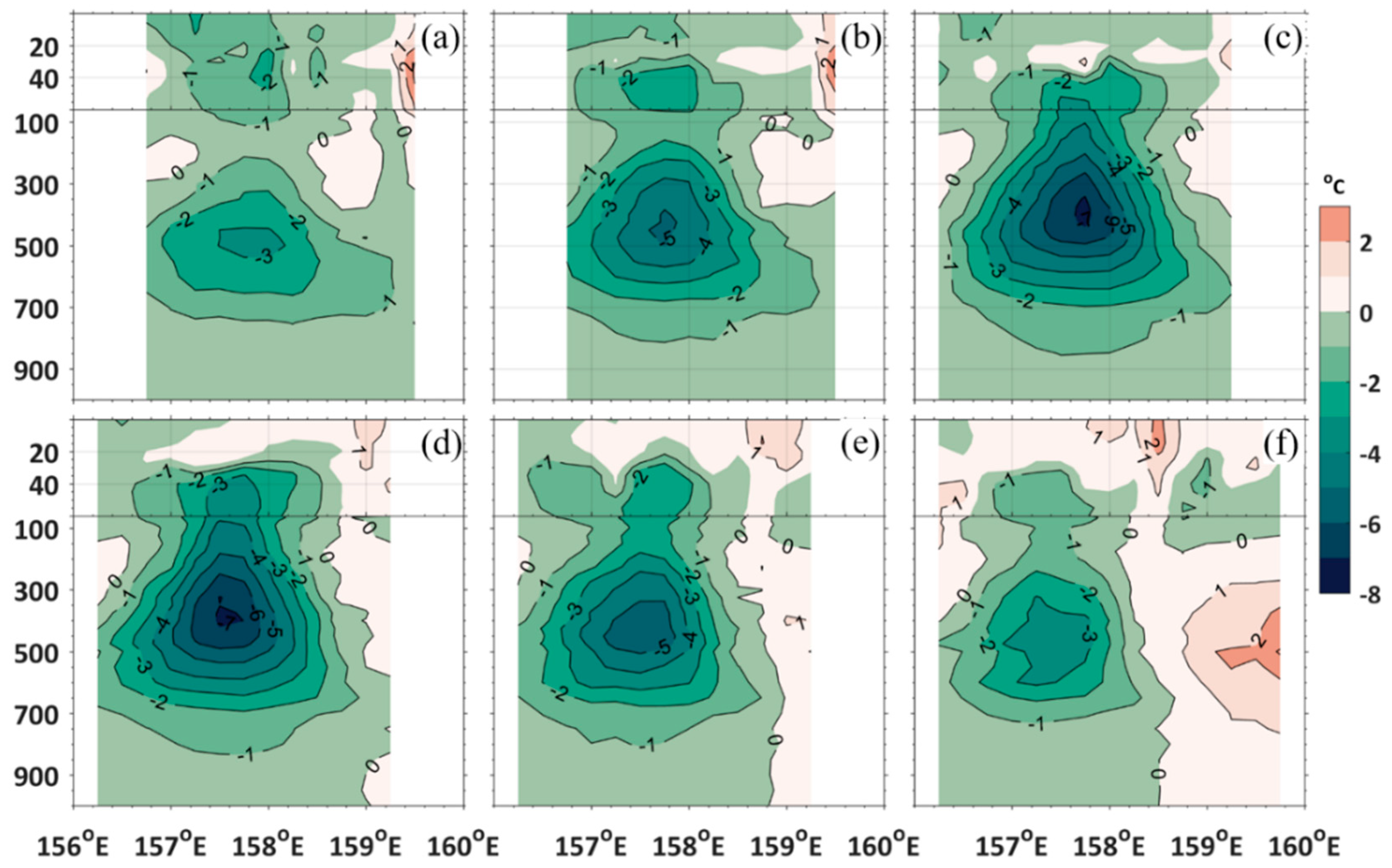
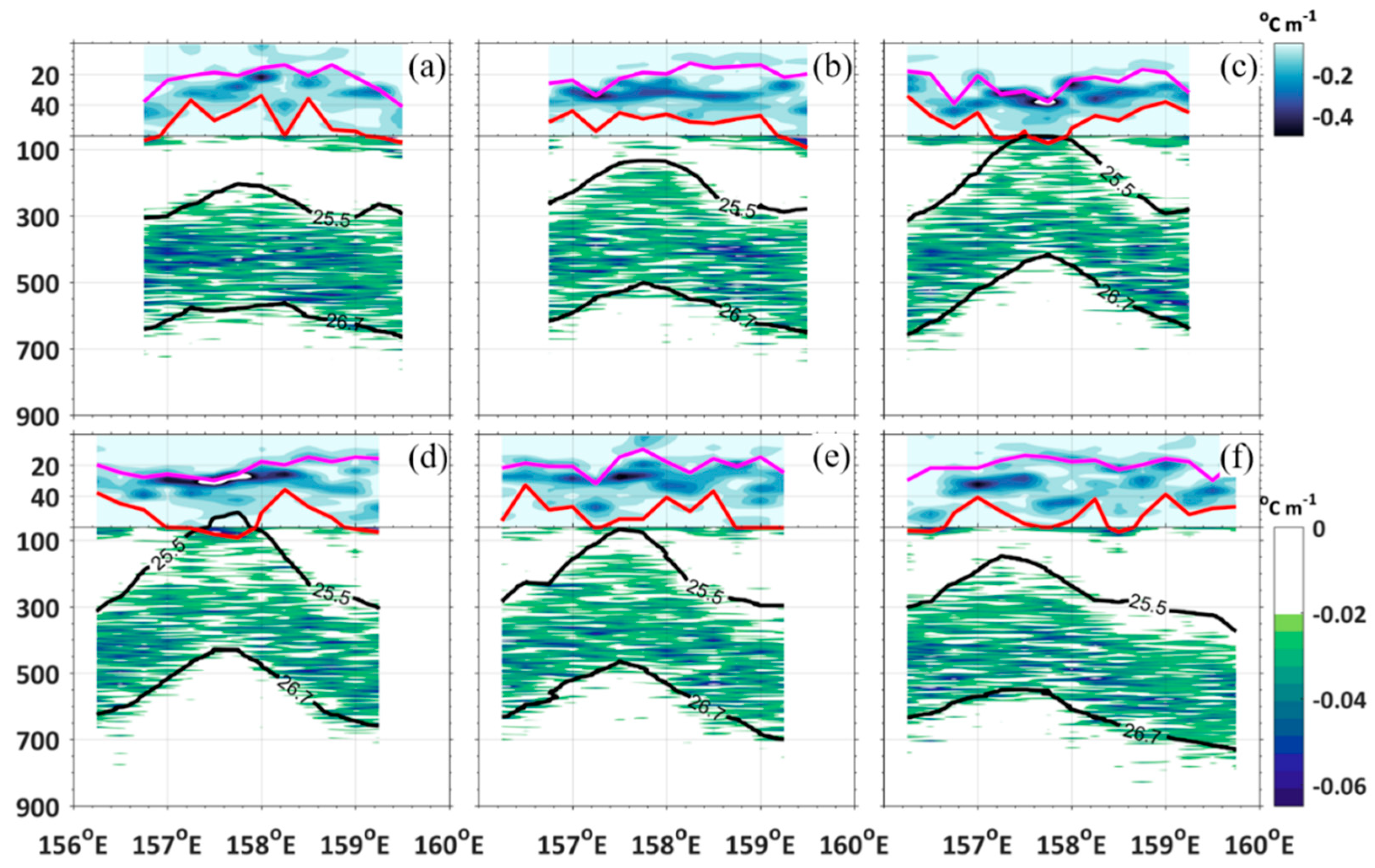
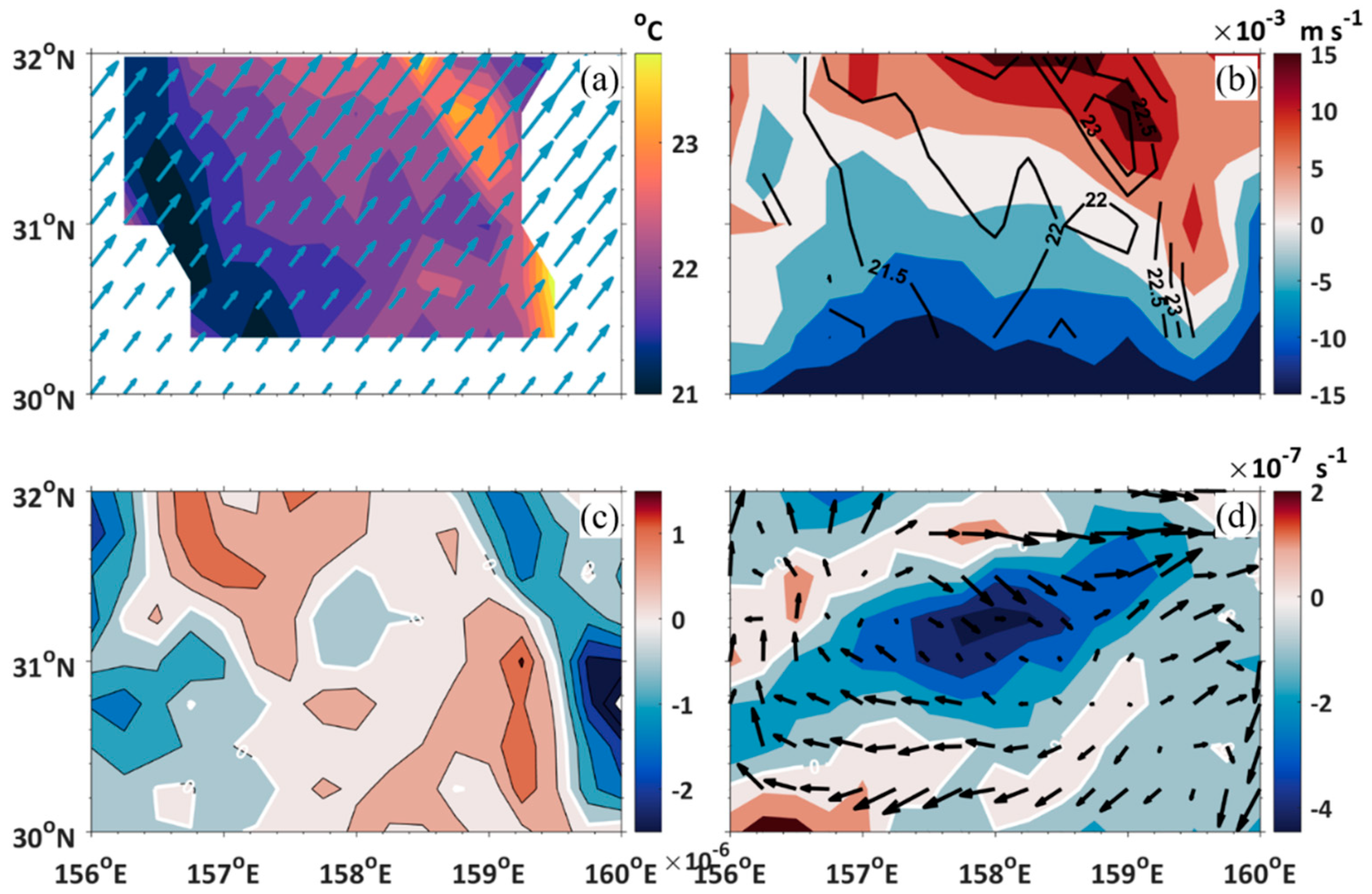
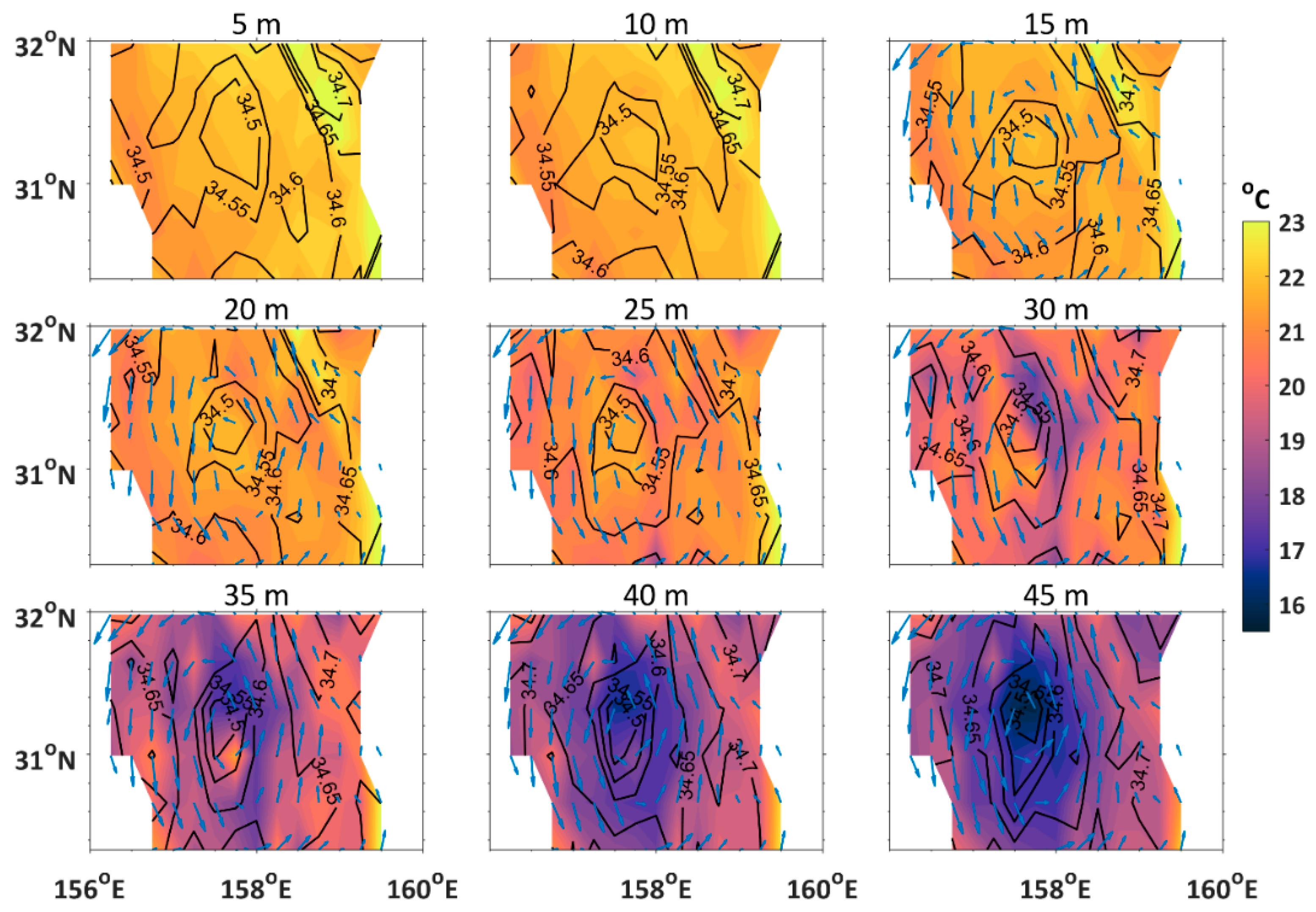
3.2. Temperature
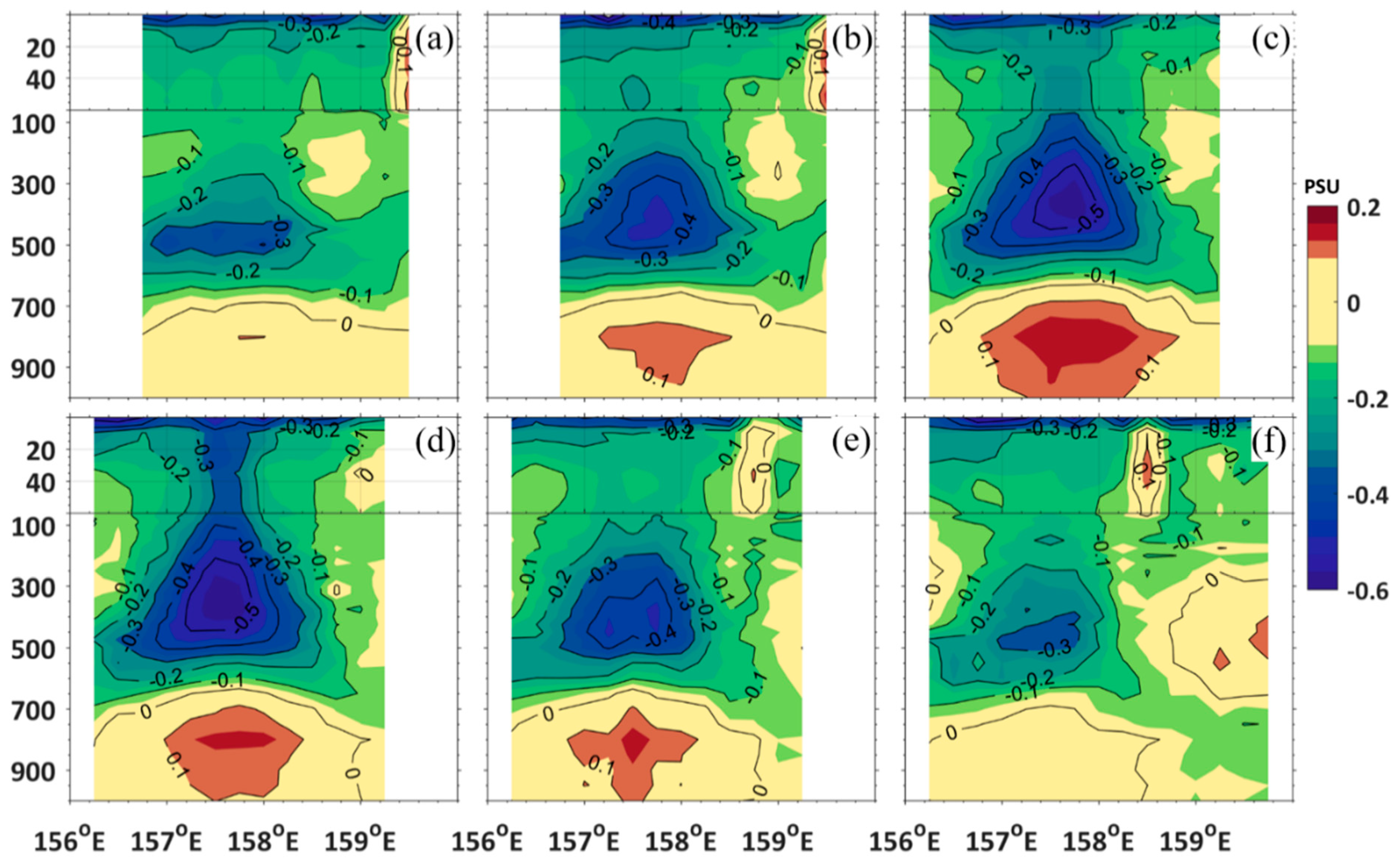
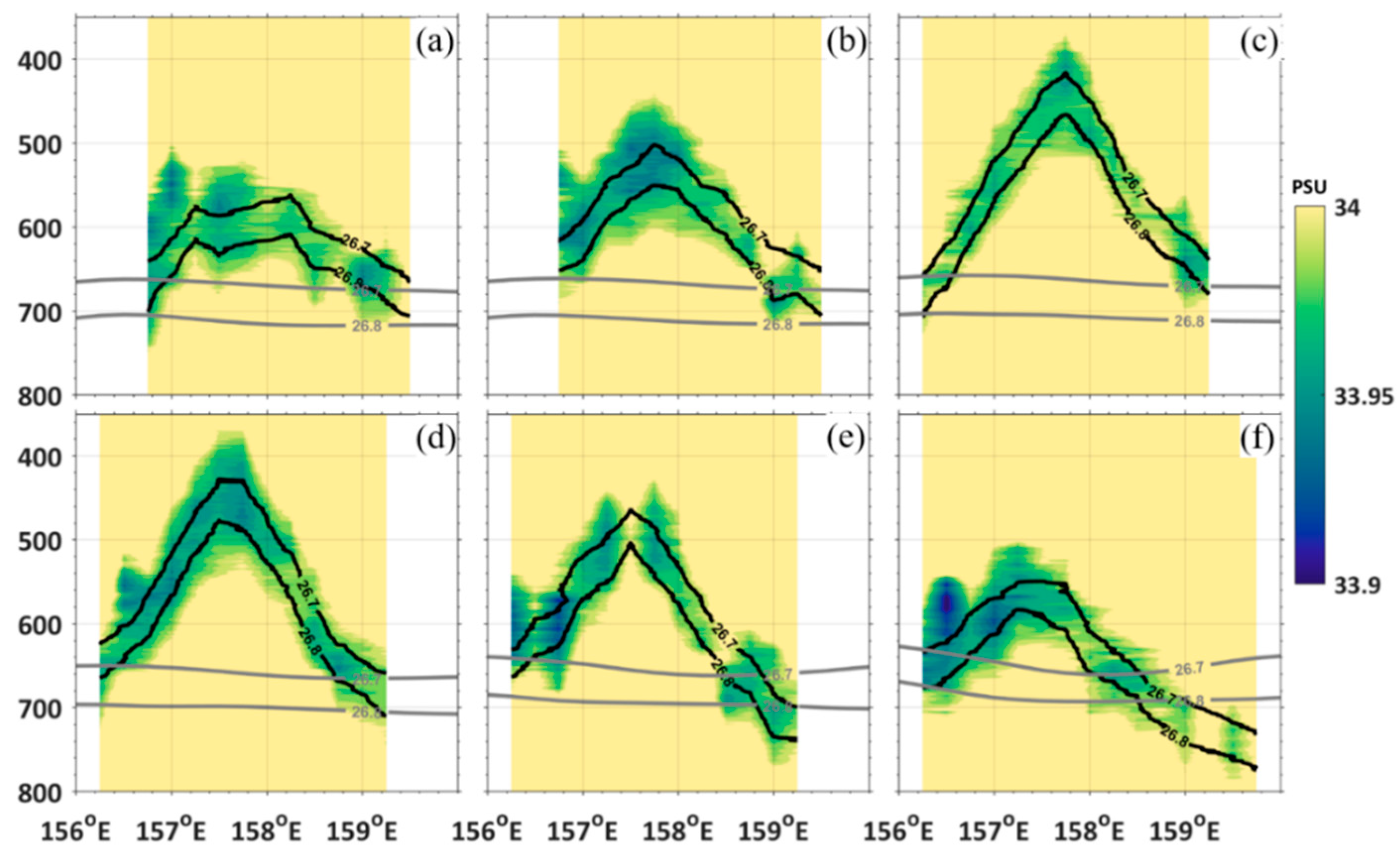
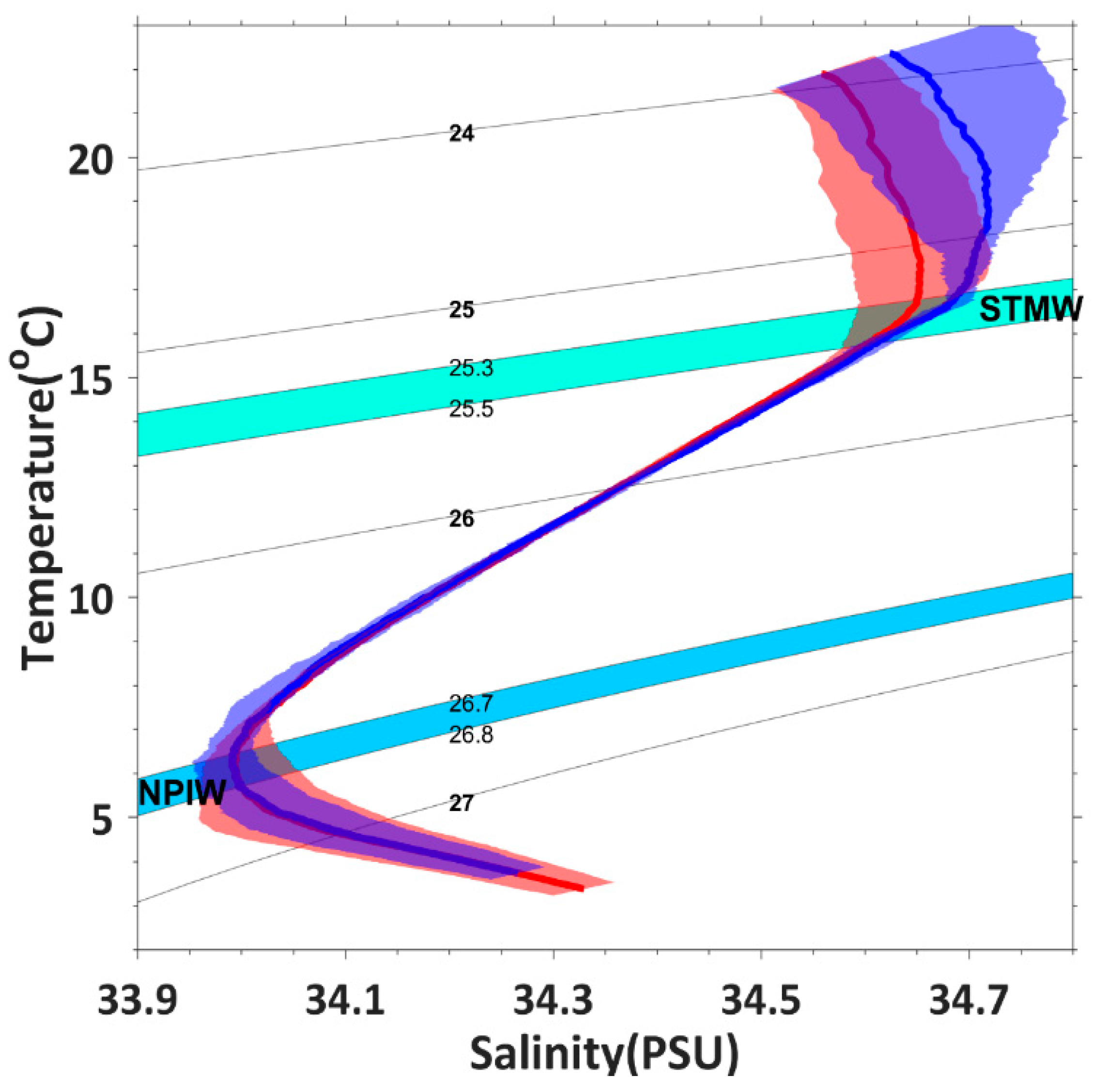
3.3. Salinity
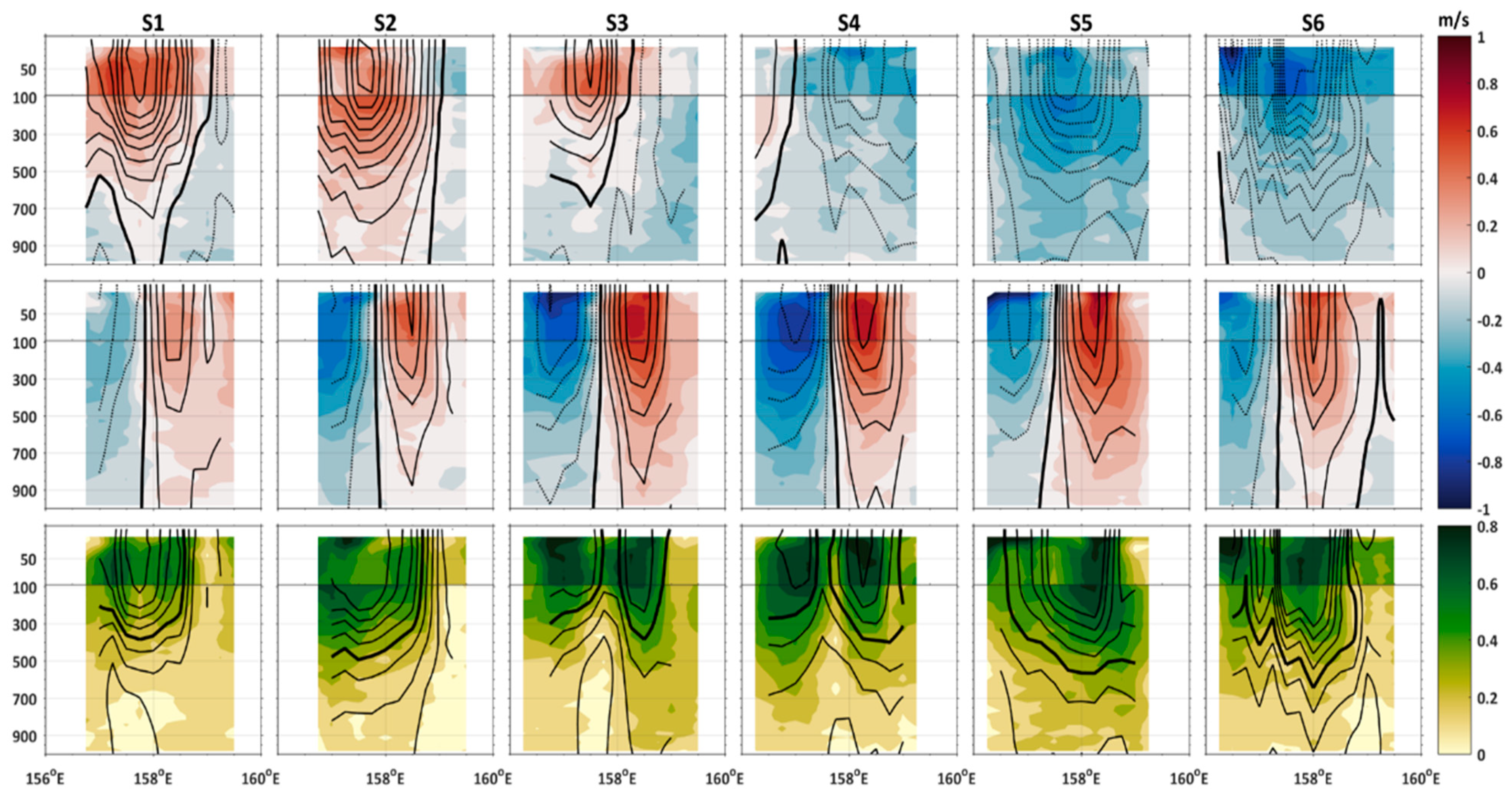
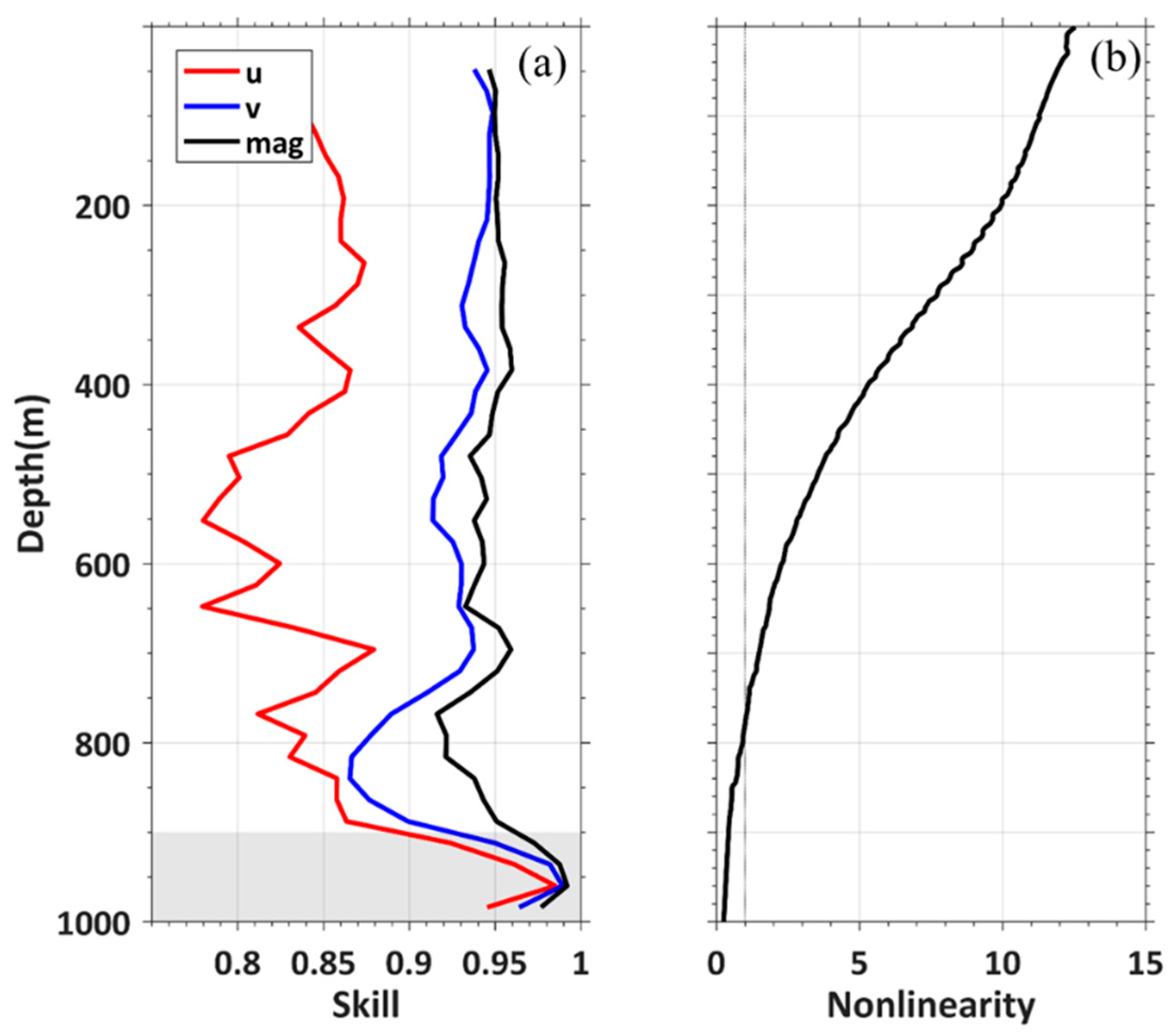
3.4. Currents
4. Thermohaline Impact
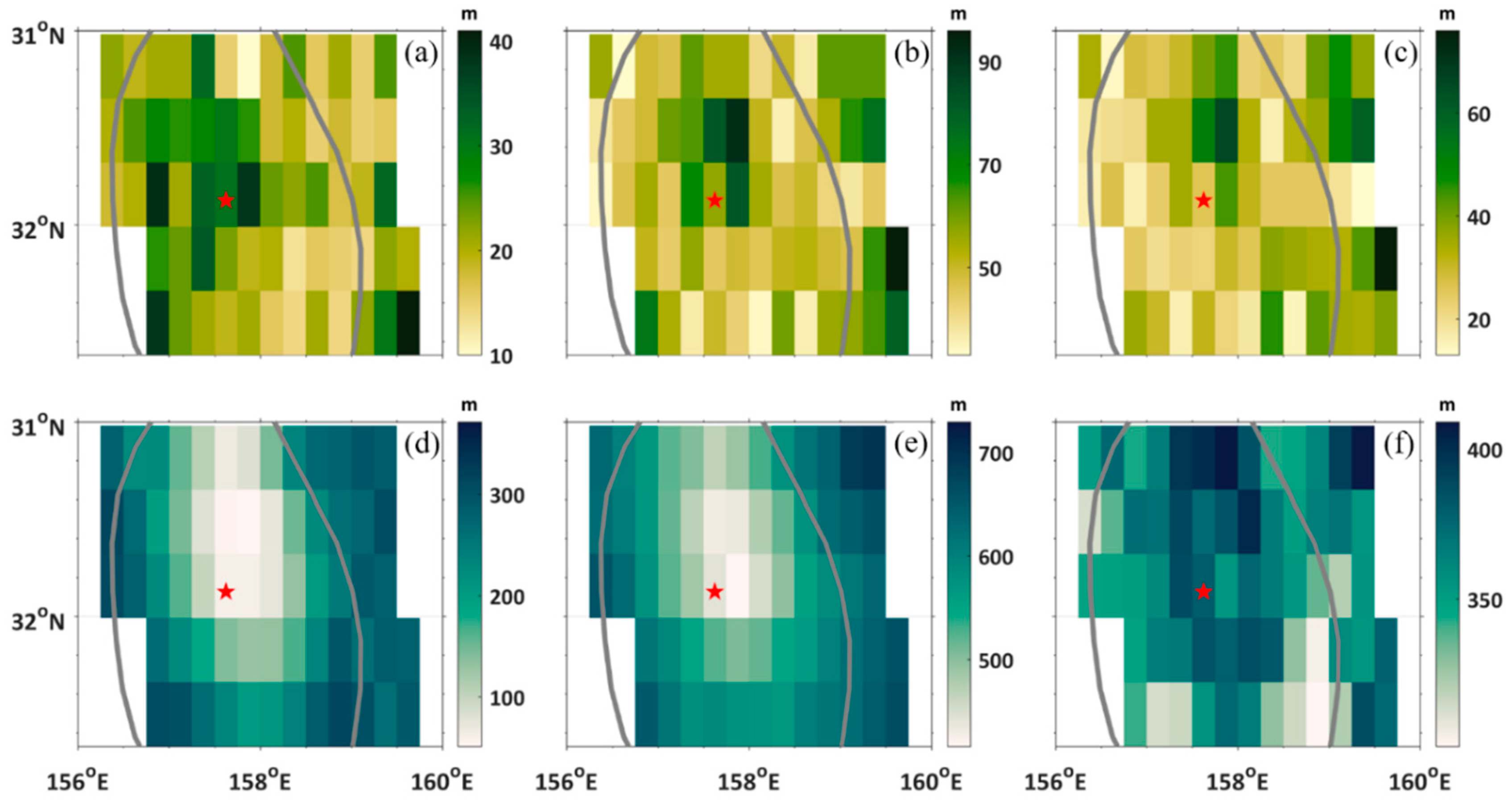
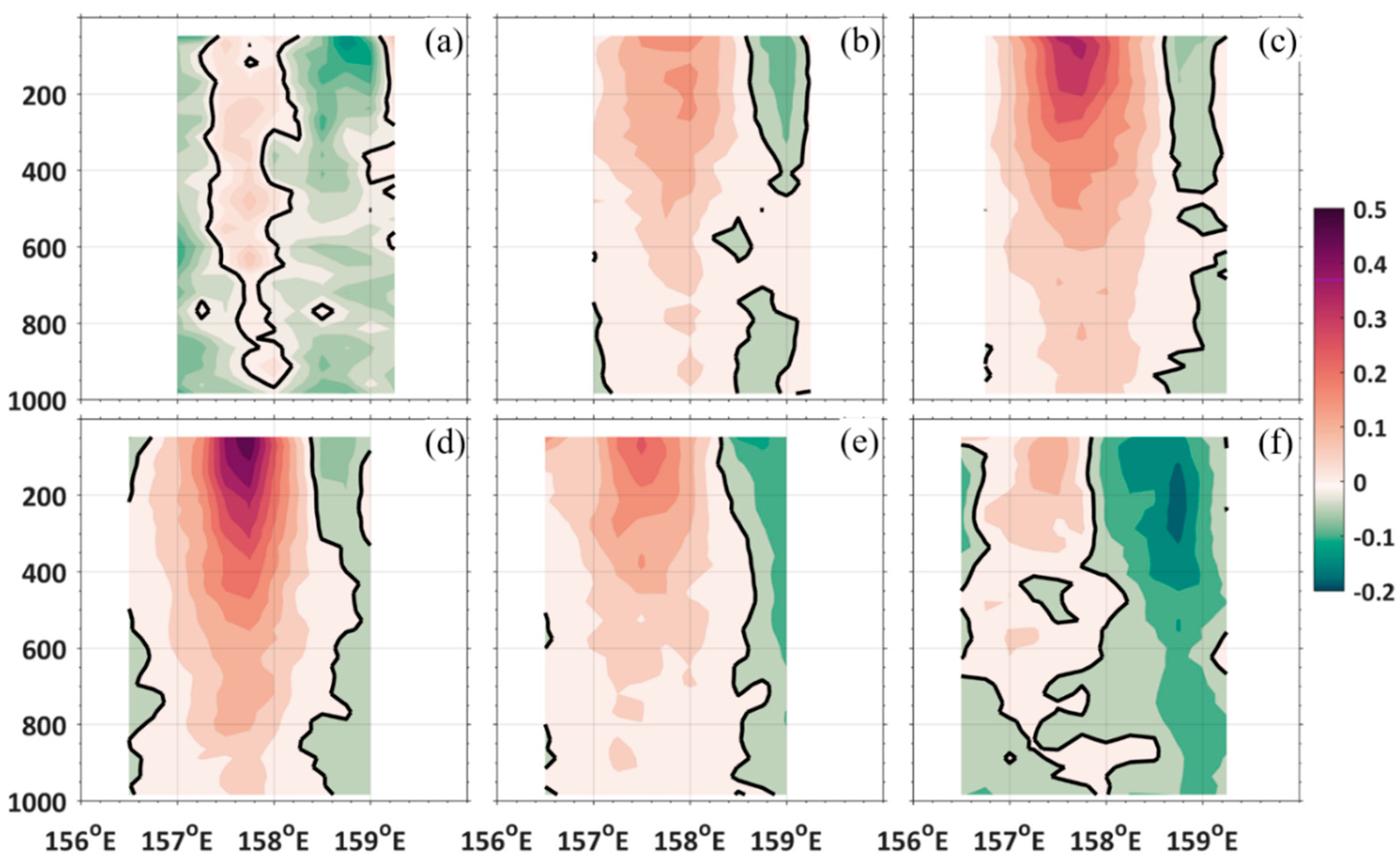
4.1. Mixed Layer
4.2. Thermocline
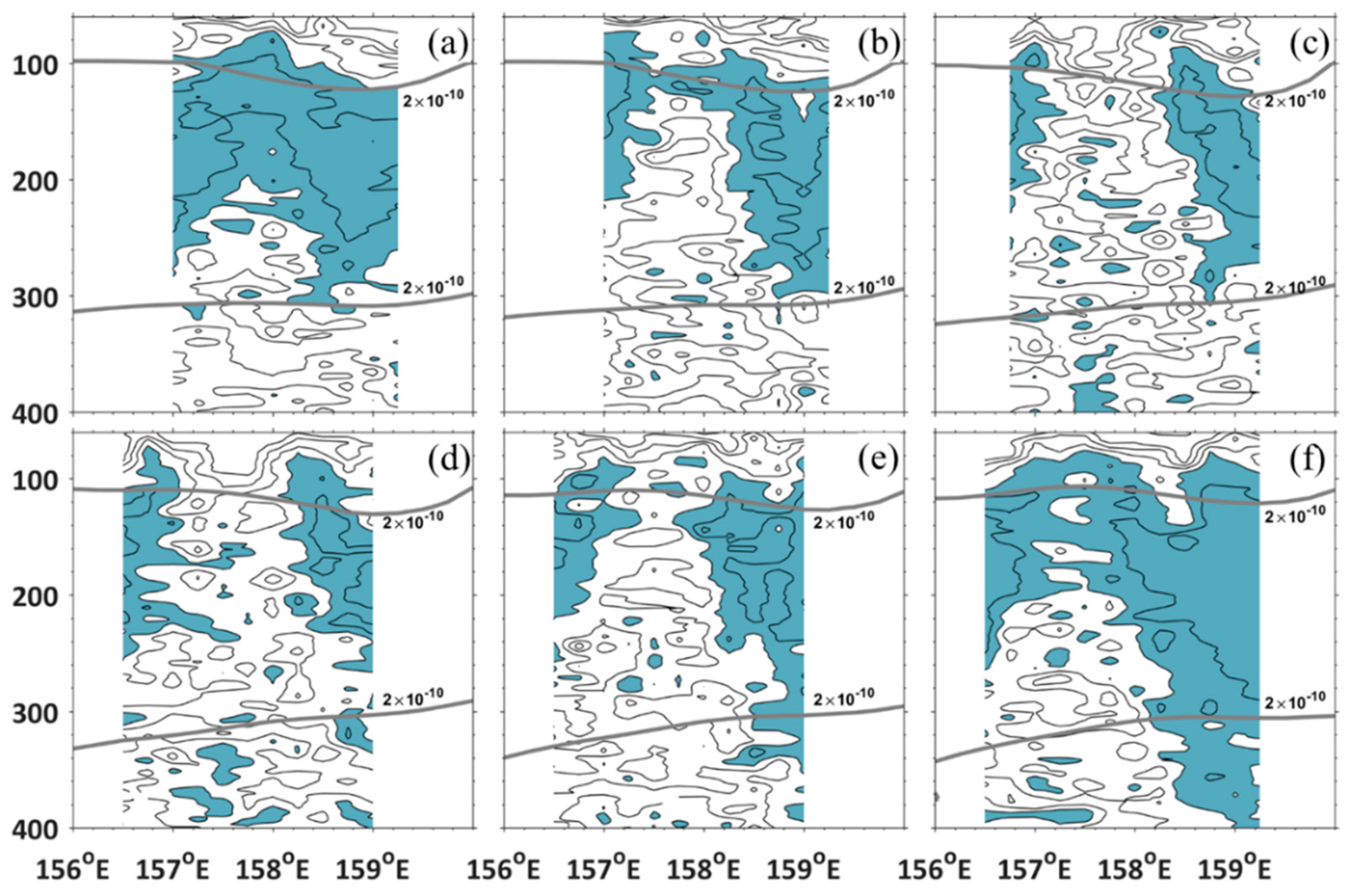
4.3. STMW
4.4. NPIW
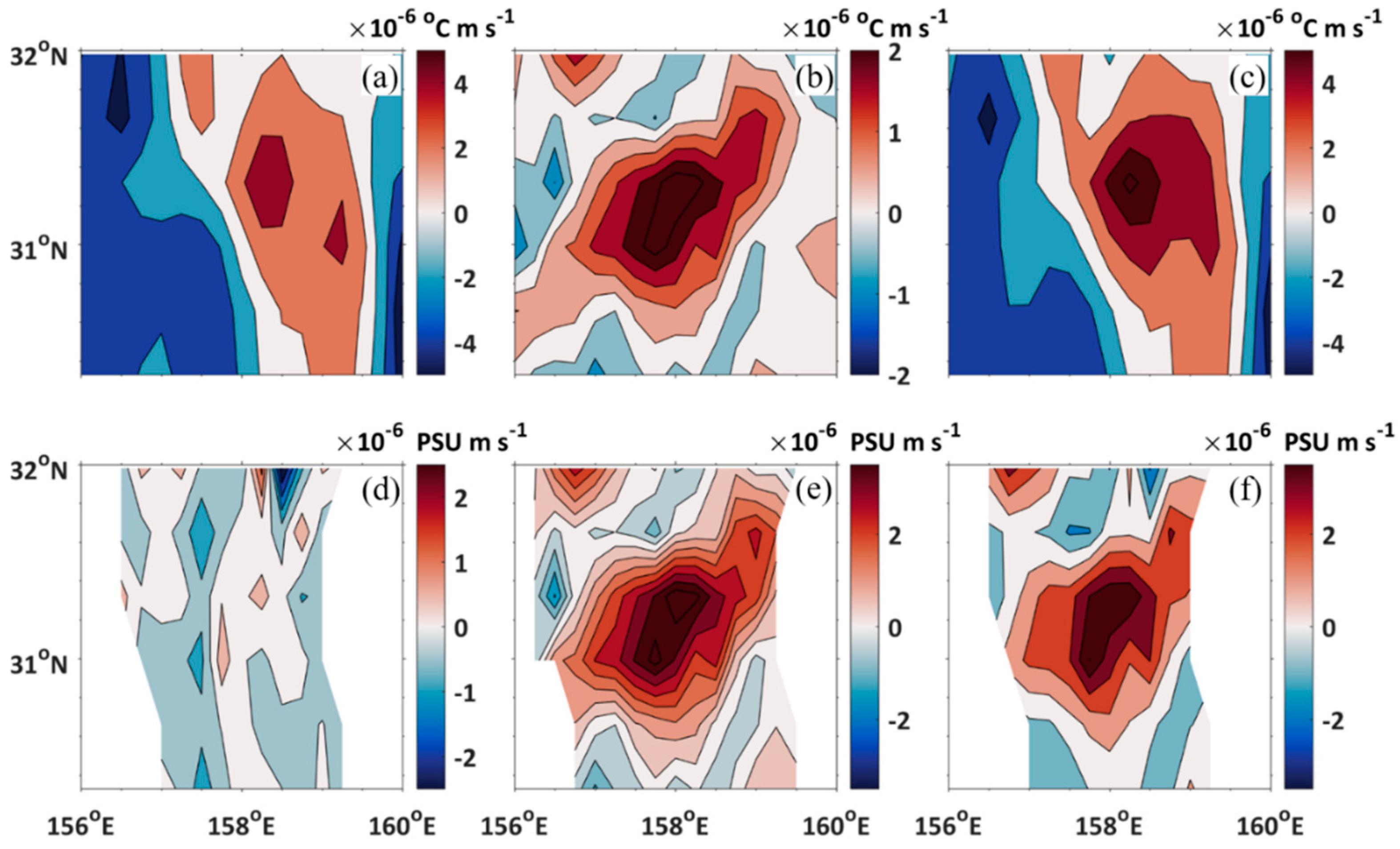
5. Dynamic Impact
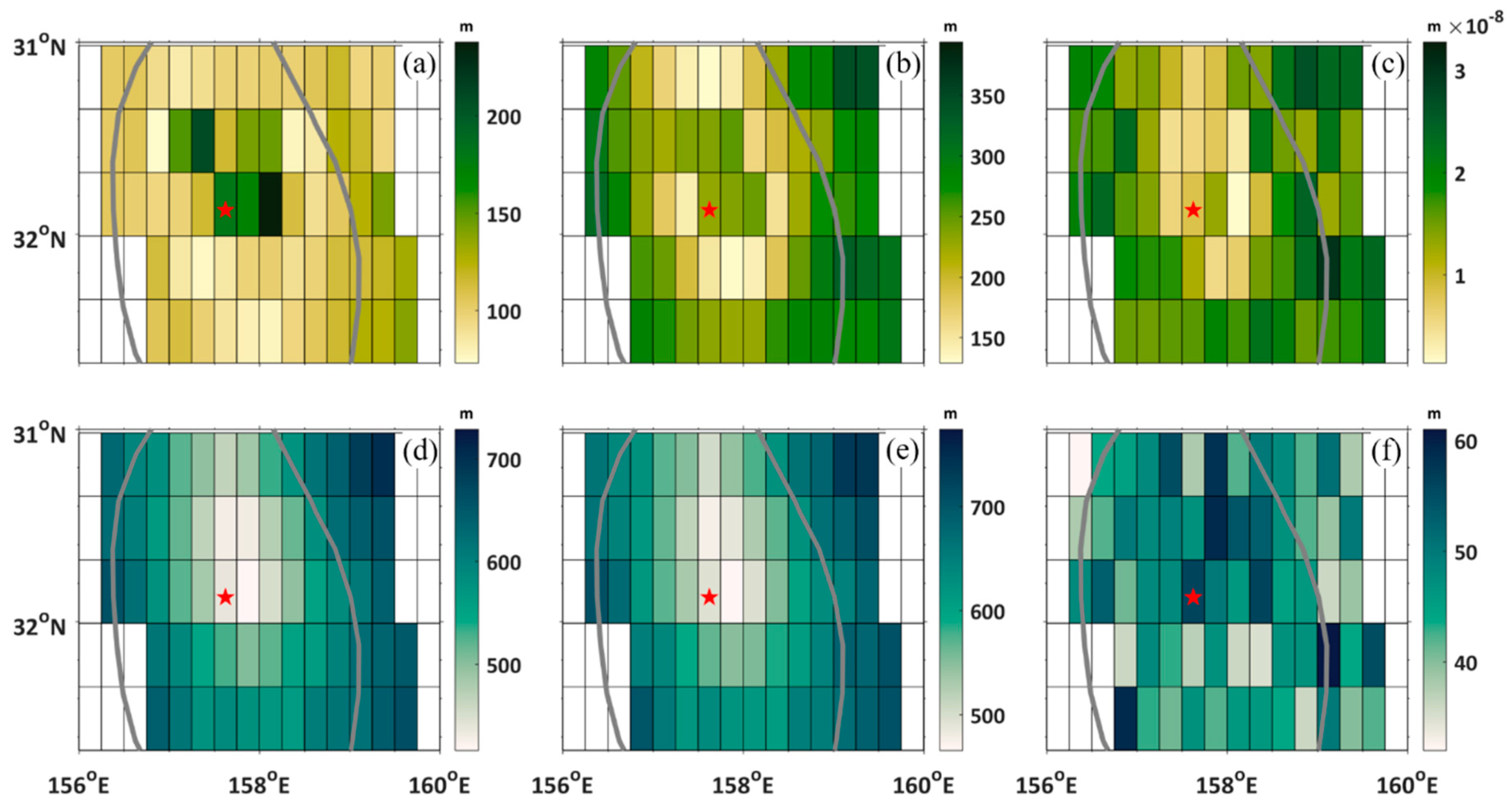
5.1. Geostrophy
5.2. Nonlinearity
6. Discussion
6.1. Eddy-Wind Interaction
6.2. Dipole Temperature vs. Monopole Salinity
7. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Chelton, D.B.; Schlax, M.G.; Samelson, R.M. Global observations of nonlinear mesoscale eddies. Prog. Oceanogr. 2011, 91, 167–216. [Google Scholar] [CrossRef]
- Group, M. The mid-ocean dynamics experiment. Deep Sea Res. 1978, 25, 859–910. [Google Scholar]
- McWilliams, J.; Brown, E.; Bryden, H.; Ebbesmeyer, C.; Elliott, B.; Heinmiller, R.; Hua, B.L.; Leaman, K.; Lindstrom, E.; Luyten, J. The local dynamics of eddies in the Western North Atlantic. Springer 1983, 92–113. [Google Scholar] [CrossRef]
- Benitez-Nelson, C.R.; Bidigare, R.R.; Dickey, T.D.; Landry, M.R.; Leonard, C.L.; Brown, S.L.; Nencioli, F.; Rii, Y.M.; Maiti, K.; Becker, J.W. Mesoscale eddies drive increased silica export in the subtropical Pacific Ocean. Science 2007, 316, 1017–1021. [Google Scholar] [CrossRef]
- McGillicuddy, D.J.; Anderson, L.A.; Bates, N.R.; Bibby, T.; Buesseler, K.O.; Carlson, C.A.; Davis, C.S.; Ewart, C.; Falkowski, P.G.; Goldthwait, S.A. Eddy/wind interactions stimulate extraordinary mid-ocean plankton blooms. Science 2007, 316, 1021–1026. [Google Scholar] [CrossRef]
- Pascual, A.; Ruiz, S.; Olita, A.; Troupin, C.; Claret, M.; Casas, B.; Mourre, B.; Poulain, P.-M.; Tovar-Sanchez, A.; Capet, A. A multiplatform experiment to unravel meso-and submesoscale processes in an intense front (AlborEx). Front. Mar. Sci. 2017, 4, 39. [Google Scholar] [CrossRef]
- Zhang, Z.; Qiu, B.; Tian, J.; Zhao, W.; Huang, X. Latitude-dependent finescale turbulent shear generations in the Pacific tropical-extratropical upper ocean. Nat. Commun. 2018, 9, 4086. [Google Scholar] [CrossRef]
- Roemmich, D.; Johnson, G.C.; Riser, S.; Davis, R.; Gilson, J.; Owens, W.B.; Garzoli, S.L.; Schmid, C.; Ignaszewski, M. The Argo Program: Observing the global ocean with profiling floats. Oceanography 2009, 22, 34–43. [Google Scholar] [CrossRef]
- Castelao, R.M. Mesoscale eddies in the South Atlantic Bight and the Gulf Stream recirculation region: vertical structure. J. Geophys. Res. Ocean. 2014, 119, 2048–2065. [Google Scholar] [CrossRef]
- Chaigneau, A.; Le Texier, M.; Eldin, G.; Grados, C.; Pizarro, O. Vertical structure of mesoscale eddies in the eastern South Pacific Ocean: A composite analysis from altimetry and Argo profiling floats. J. Geophys. Res. Ocean. 2011, 116. [Google Scholar] [CrossRef]
- He, Q.; Zhan, H.; Cai, S.; He, Y.; Huang, G.; Zhan, W. A New Assessment of Mesoscale Eddies in the South China Sea: Surface Features, Three-Dimensional Structures and Thermohaline Transports. J. Geophys. Res. Ocean. 2018, 123, 4906–4929. [Google Scholar] [CrossRef]
- Sun, W.; Dong, C.; Wang, R.; Liu, Y.; Yu, K. Vertical structure anomalies of oceanic eddies in the Kuroshio Extension region. J. Geophys. Res. Ocean. 2017, 122, 1476–1496. [Google Scholar] [CrossRef]
- Yang, G.; Wang, F.; Li, Y.; Lin, P. Mesoscale eddies in the northwestern subtropical Pacific Ocean: Statistical characteristics and three-dimensional structures. J. Geophys. Res. Ocean. 2013, 118, 1906–1925. [Google Scholar] [CrossRef]
- Xu, L.; Li, P.; Xie, S.-P.; Liu, Q.; Liu, C.; Gao, W. Observing mesoscale eddy effects on mode-water subduction and transport in the North Pacific. Nat. Commun. 2016, 7, 10505. [Google Scholar] [CrossRef] [PubMed]
- Zhang, Z.; Tian, J.; Qiu, B.; Zhao, W.; Chang, P.; Wu, D.; Wan, X. Observed 3D structure, generation and dissipation of oceanic mesoscale eddies in the South China Sea. Sci. Rep. 2016, 6. [Google Scholar] [CrossRef] [PubMed]
- Rudnick, D.L. Ocean research enabled by underwater gliders. Annu. Rev. Mar. Sci. 2016, 8, 519–541. [Google Scholar] [CrossRef] [PubMed]
- Kolodziejczyk, N.; Testor, P.; Lazar, A.; Echevin, V.; Krahmann, G.; Chaigneau, A.; Gourcuff, C.; Wade, M.; Faye, S.; Estrade, P. Subsurface Fine-Scale Patterns in an Anticyclonic Eddy Off Cap-Vert Peninsula Observed From Glider Measurements. J. Geophys. Res. Ocean. 2018, 123, 6312–6329. [Google Scholar] [CrossRef]
- Shu, Y.; Chen, J.; Li, S.; Wang, Q.; Yu, J.; Wang, D. Field-observation for an anticyclonic mesoscale eddy consisted of twelve gliders and sixty-two expendable probes in the northern South China Sea during summer 2017. Sci. China Earth Sci. 2018, 62, 451–458. [Google Scholar] [CrossRef]
- Barceló-Llull, B.; Sangrà, P.; Pallàs-Sanz, E.; Barton, E.D.; Estrada-Allis, S.N.; Martínez-Marrero, A.; Aguiar-González, B.; Grisolía, D.; Gordo, C.; Rodríguez-Santana, Á. Anatomy of a subtropical intrathermocline eddy. Deep Sea Research Part I: Oceanographic Research Papers. ScienceDirect 2017, 124, 126–139. [Google Scholar]
- Hu, J.; Gan, J.; Sun, Z.; Zhu, J.; Dai, M. Observed three-dimensional structure of a cold eddy in the southwestern South China Sea. J. Geophys. Res. Ocean. 2011, 116, C05016. [Google Scholar] [CrossRef]
- Kurczyn, J.; Beier, E.; Lavín, M.; Chaigneau, A.; Godínez, V. Anatomy and evolution of a cyclonic mesoscale eddy observed in the northeastern Pacific tropical-subtropical transition zone. J. Geophys. Res. Ocean. 2013, 118, 5931–5950. [Google Scholar] [CrossRef]
- Garreau, P.; Dumas, F.; Louazel, S.; Stegner, A.; Le Vu, B. High-Resolution Observations and Tracking of a Dual-Core Anticyclonic Eddy in the Algerian Basin. J. Geophys. Res. Ocean. 2018, 123, 9320–9339. [Google Scholar] [CrossRef]
- Flierl, G.; McGillicuddy, D.J. Mesoscale and submesoscale physical-biological interactions. The Sea 2002, 12, 113–185. [Google Scholar]
- McGillicuddy, D.J., Jr. Mechanisms of physical-biological-biogeochemical interaction at the oceanic mesoscale. Annu. Rev. Mar. Sci. 2016, 8, 125–159. [Google Scholar] [CrossRef] [PubMed]
- Pegliasco, C.; Chaigneau, A.; Morrow, R. Main eddy vertical structures observed in the four major Eastern Boundary Upwelling Systems. J. Geophys. Res. Ocean. 2015, 120, 6008–6033. [Google Scholar] [CrossRef]
- Zhang, Z.; Li, P.; Xu, L.; Li, C.; Zhao, W.; Tian, J.; Qu, T. Subthermocline eddies observed by rapid-sampling Argo floats in the subtropical northwestern Pacific Ocean in Spring 2014. Geophys. Res. Lett. 2015, 42, 6438–6445. [Google Scholar] [CrossRef]
- Li, C.; Zhang, Z.; Zhao, W.; Tian, J. A statistical study on the subthermocline submesoscale eddies in the northwestern P acific O cean based on A rgo data. J. Geophys. Res. Ocean. 2017, 122, 3586–3598. [Google Scholar] [CrossRef]
- Pelland, N.A.; Eriksen, C.C.; Lee, C.M. Subthermocline eddies over the Washington continental slope as observed by Seagliders, 2003–2009. J. Phys. Oceanogr. 2013, 43, 2025–2053. [Google Scholar] [CrossRef]
- Zhang, Z.; Liu, Z.; Richards, K.; Shang, G.; Zhao, W.; Tian, J.; Huang, X.; Zhou, C. Elevated diapycnal mixing by a sub-thermocline eddy in the western equatorial Pacific. Geophys. Res. Lett. 2019, 46, 2628–2636. [Google Scholar] [CrossRef]
- Dong, C.; Lin, X.; Liu, Y.; Nencioli, F.; Chao, Y.; Guan, Y.; Chen, D.; Dickey, T.; McWilliams, J.C. Three-dimensional oceanic eddy analysis in the Southern California Bight from a numerical product. J. Geophys. Res. Ocean. 2012, 117. [Google Scholar] [CrossRef]
- Qiu, B.; Chen, S.; Hacker, P. Effect of mesoscale eddies on subtropical mode water variability from the Kuroshio Extension System Study (KESS). J. Phys. Oceanogr. 2007, 37, 982–1000. [Google Scholar] [CrossRef]
- Xu, L.; Xie, S.P.; Liu, Q.; Liu, C.; Li, P.; Lin, X. Evolution of the North Pacific subtropical mode water in anticyclonic eddies. J. Geophys. Res. Ocean. 2017, 122, 10118–10130. [Google Scholar] [CrossRef]
- Von Schuckmann, K.; Gaillard, F.; Le Traon, P.Y. Global hydrographic variability patterns during 2003–2008. J. Geophys. Res. Ocean. 2009, 114, C09007. [Google Scholar] [CrossRef]
- Ji, J.; Dong, C.; Zhang, B.; Liu, Y.; Zou, B.; King, G.P.; Xu, G.; Chen, D. Oceanic Eddy Characteristics and Generation Mechanisms in the Kuroshio Extension Region. J. Geophys. Res. Ocean. 2018, 123, 8548–8567. [Google Scholar] [CrossRef]
- Frenger, I.; Münnich, M.; Gruber, N.; Knutti, R. Southern O cean eddy phenomenology. J. Geophys. Res. Ocean. 2015, 120, 7413–7449. [Google Scholar] [CrossRef]
- Gaube, P.; McGillicuddy, D.J.; Moulin, A.J. Mesoscale Eddies Modulate Mixed Layer Depth Globally. Geophys. Res. Lett. 2018, 46. [Google Scholar] [CrossRef]
- Hausmann, U.; McGillicuddy, D.J.; Marshall, J. Observed mesoscale eddy signatures in Southern Ocean surface mixed-layer depth. J. Geophys. Res. Ocean. 2017, 122, 617–635. [Google Scholar] [CrossRef]
- Wunsch, C. The vertical partition of oceanic horizontal kinetic energy. J. Phys. Oceanogr. 1997, 27, 1770–1794. [Google Scholar] [CrossRef]
- McGillicuddy, D.J., Jr.; Robinson, A.; Siegel, D.; Jannasch, H.; Johnson, R.; Dickey, T.; McNeil, J.; Michaels, A.; Knap, A. Influence of mesoscale eddies on new production in the Sargasso Sea. Nature 1998, 394, 263. [Google Scholar] [CrossRef]
- Sugimoto, S.; Hanawa, K.; Watanabe, T.; Suga, T.; Xie, S.-P. Enhanced warming of the subtropical mode water in the North Pacific and North Atlantic. Nat. Clim. Chang. 2017, 7, 656–658. [Google Scholar] [CrossRef]
- Oka, E.; Suga, T.; Sukigara, C.; Toyama, K.; Shimada, K.; Yoshida, J. “Eddy resolving” observation of the North Pacific subtropical mode water. J. Phys. Oceanogr. 2011, 41, 666–681. [Google Scholar] [CrossRef]
- Shi, F.; Luo, Y.; Xu, L. Volume and Transport of Eddy-Trapped Mode Water South of the Kuroshio Extension. J. Geophys. Res. Ocean. 2018, 123. [Google Scholar] [CrossRef]
- Nishikawa, S.; Tsujino, H.; Sakamoto, K.; Nakano, H. Effects of mesoscale eddies on subduction and distribution of subtropical mode water in an eddy-resolving OGCM of the western North Pacific. J. Phys. Oceanogr. 2010, 40, 1748–1765. [Google Scholar] [CrossRef]
- Kouketsu, S.; Tomita, H.; Oka, E.; Hosoda, S.; Kobayashi, T.; Sato, K. The role of meso-scale eddies in mixed layer deepening and mode water formation in the western North Pacific. In New Developments in Mode-Water Research; Springer: New York, NY, USA, 2011; pp. 59–73. [Google Scholar]
- Talley, L.D. Distribution and formation of North Pacific intermediate water. J. Phys. Oceanogr. 1993, 23, 517–537. [Google Scholar] [CrossRef]
- Qiu, B.; Chen, S. Effect of decadal Kuroshio Extension jet and eddy variability on the modification of North Pacific Intermediate Water. J. Phys. Oceanogr. 2011, 41, 503–515. [Google Scholar] [CrossRef]
- Zhang, Z.; Qiu, B. Evolution of Submesoscale Ageostrophic Motions Through the Life Cycle of Oceanic Mesoscale Eddies. Geophys. Res. Lett. 2018, 45, 11847–11855. [Google Scholar] [CrossRef]
- Tomczak, M.; Godfrey, J.S. Regional Oceanography: An Introduction; Pergamon: Oxford, UK, 1994. [Google Scholar]
- Peña-Molino, B.; Rintoul, S.; Mazloff, M. Barotropic and baroclinic contributions to along-stream and across-stream transport in the A ntarctic C ircumpolar C urrent. J. Geophys. Res. Ocean. 2014, 119, 8011–8028. [Google Scholar] [CrossRef]
- Chelton, D.B.; DeSzoeke, R.A.; Schlax, M.G.; El Naggar, K.; Siwertz, N. Geographical variability of the first baroclinic Rossby radius of deformation. J. Phys. Oceanogr. 1998, 28, 433–460. [Google Scholar] [CrossRef]
- Qiu, B.; Chen, S. Decadal variability in the large-scale sea surface height field of the South Pacific Ocean: Observations and causes. J. Phys. Oceanogr. 2006, 36, 1751–1762. [Google Scholar] [CrossRef]
- Small, R.; Xie, S.; O’Neill, L.; Seo, H.; Song, Q.; Cornillon, P.; Spall, M.; Minobe, S. Air–sea interaction over ocean fronts and eddies. Dyn. Atmos. Ocean. 2008, 45, 274–319. [Google Scholar] [CrossRef]
- Chelton, D.B.; Schlax, M.G.; Freilich, M.H.; Milliff, R.F. Satellite measurements reveal persistent small-scale features in ocean winds. science 2004, 303, 978–983. [Google Scholar] [CrossRef]
- Frenger, I.; Gruber, N.; Knutti, R.; Münnich, M. Imprint of Southern Ocean eddies on winds, clouds and rainfall. Nat. Geosci. 2013, 6, 608. [Google Scholar] [CrossRef]
- Chelton, D.B.; Schlax, M.G.; Samelson, R.M. Summertime coupling between sea surface temperature and wind stress in the California Current System. J. Phys. Oceanogr. 2007, 37, 495–517. [Google Scholar] [CrossRef]
- Seo, H.; Miller, A.J.; Norris, J.R. Eddy–wind interaction in the California Current System: Dynamics and impacts. J. Phys. Oceanogr. 2016, 46, 439–459. [Google Scholar] [CrossRef]
- Gaube, P.; McGillicuddy, D.J., Jr.; Chelton, D.B.; Behrenfeld, M.J.; Strutton, P.G. Regional variations in the influence of mesoscale eddies on near-surface chlorophyll. J. Geophys. Res. Ocean. 2014, 119, 8195–8220. [Google Scholar] [CrossRef]
- Frankignoul, C.; Czaja, A.; L’Heveder, B. Air–sea feedback in the North Atlantic and surface boundary conditions for ocean models. J. Clim. 1998, 11, 2310–2324. [Google Scholar] [CrossRef]
- Delcroix, T.; Cravatte, S.; McPhaden, M.J. Decadal variations and trends in tropical Pacific sea surface salinity since 1970. J. Geophys. Res. Ocean. 2007, 112. [Google Scholar] [CrossRef]
- Dong, D.; Brandt, P.; Chang, P.; Schütte, F.; Yang, X.; Yan, J.; Zeng, J. Mesoscale Eddies in the Northwestern Pacific Ocean: Three-Dimensional Eddy Structures and Heat/Salt Transports. J. Geophys. Res. Ocean. 2017, 122, 9795–9813. [Google Scholar] [CrossRef]


© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Y.; Chen, X.; Dong, C. Anatomy of a Cyclonic Eddy in the Kuroshio Extension Based on High-Resolution Observations. Atmosphere 2019, 10, 553. https://doi.org/10.3390/atmos10090553
Zhang Y, Chen X, Dong C. Anatomy of a Cyclonic Eddy in the Kuroshio Extension Based on High-Resolution Observations. Atmosphere. 2019; 10(9):553. https://doi.org/10.3390/atmos10090553
Chicago/Turabian StyleZhang, Yongchui, Xi Chen, and Changming Dong. 2019. "Anatomy of a Cyclonic Eddy in the Kuroshio Extension Based on High-Resolution Observations" Atmosphere 10, no. 9: 553. https://doi.org/10.3390/atmos10090553
APA StyleZhang, Y., Chen, X., & Dong, C. (2019). Anatomy of a Cyclonic Eddy in the Kuroshio Extension Based on High-Resolution Observations. Atmosphere, 10(9), 553. https://doi.org/10.3390/atmos10090553





