Analysis of Mountain Wave Effects on a Hard Landing Incident in Pico Aerodrome Using the AROME Model and Airborne Observations
Abstract
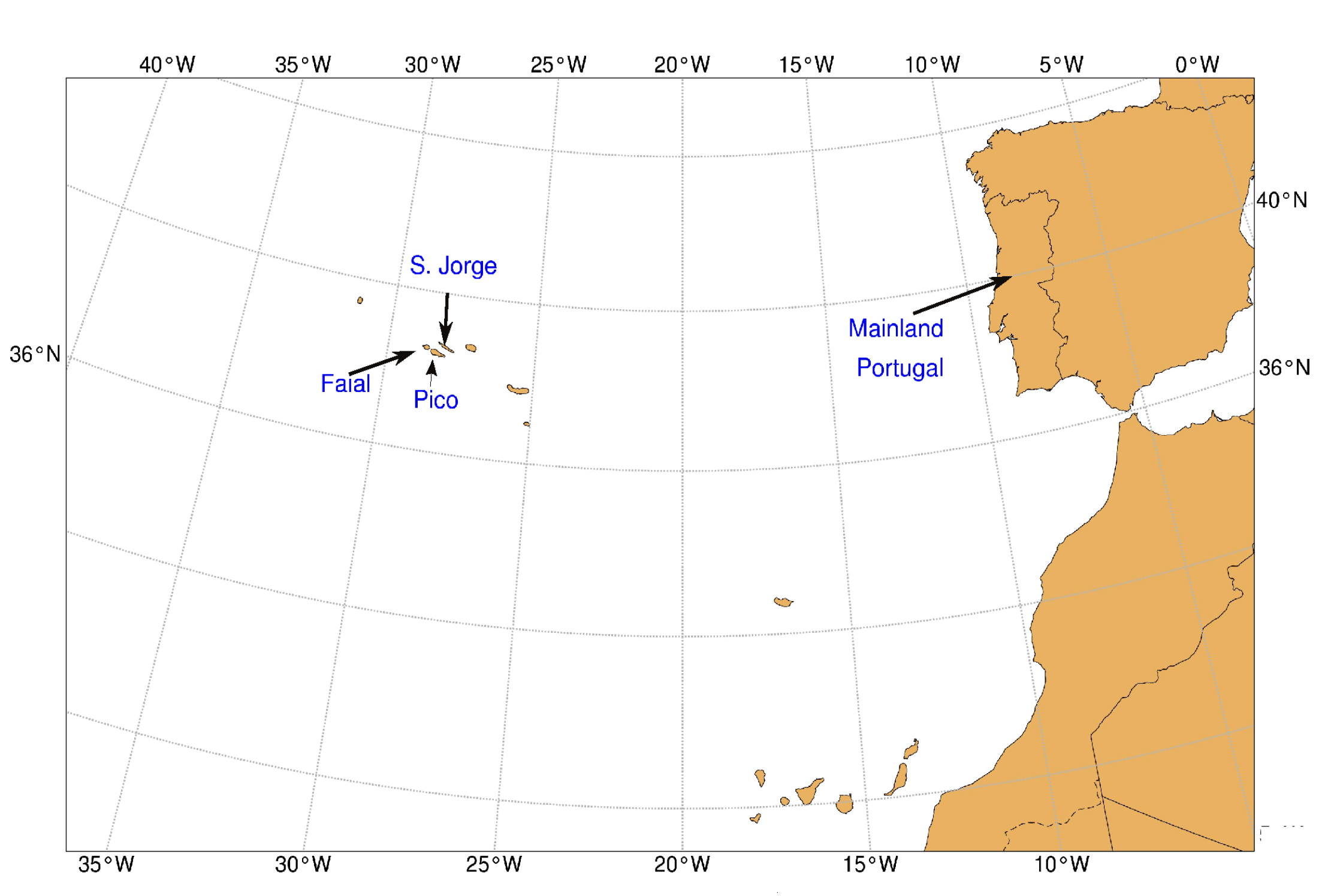
1. Introduction
2. Data and Methodology
2.1. SATA Airborne Measurements
2.2. Wind Shear Intensity Factor “I”
2.3. Description of the AROME Model
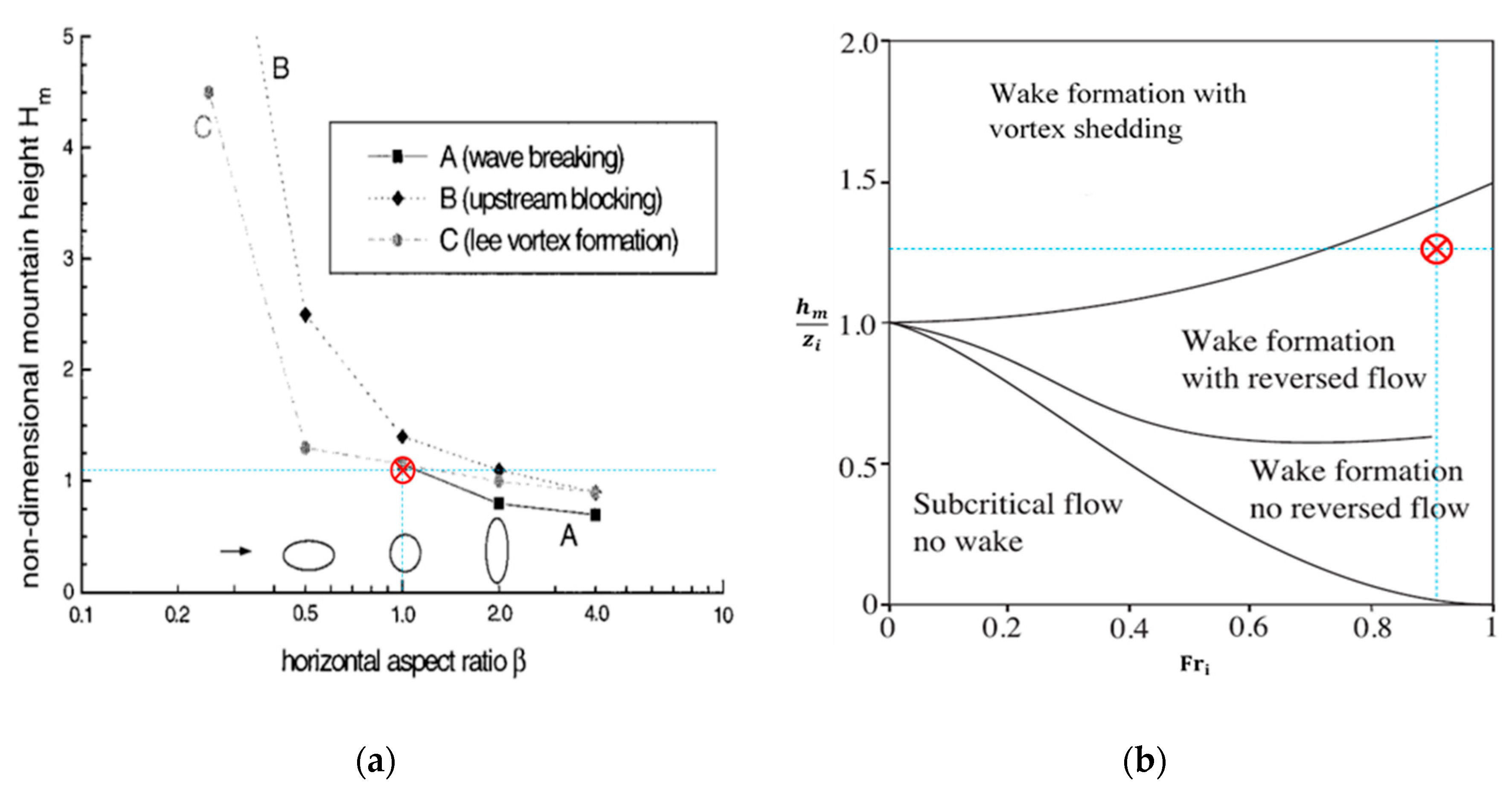
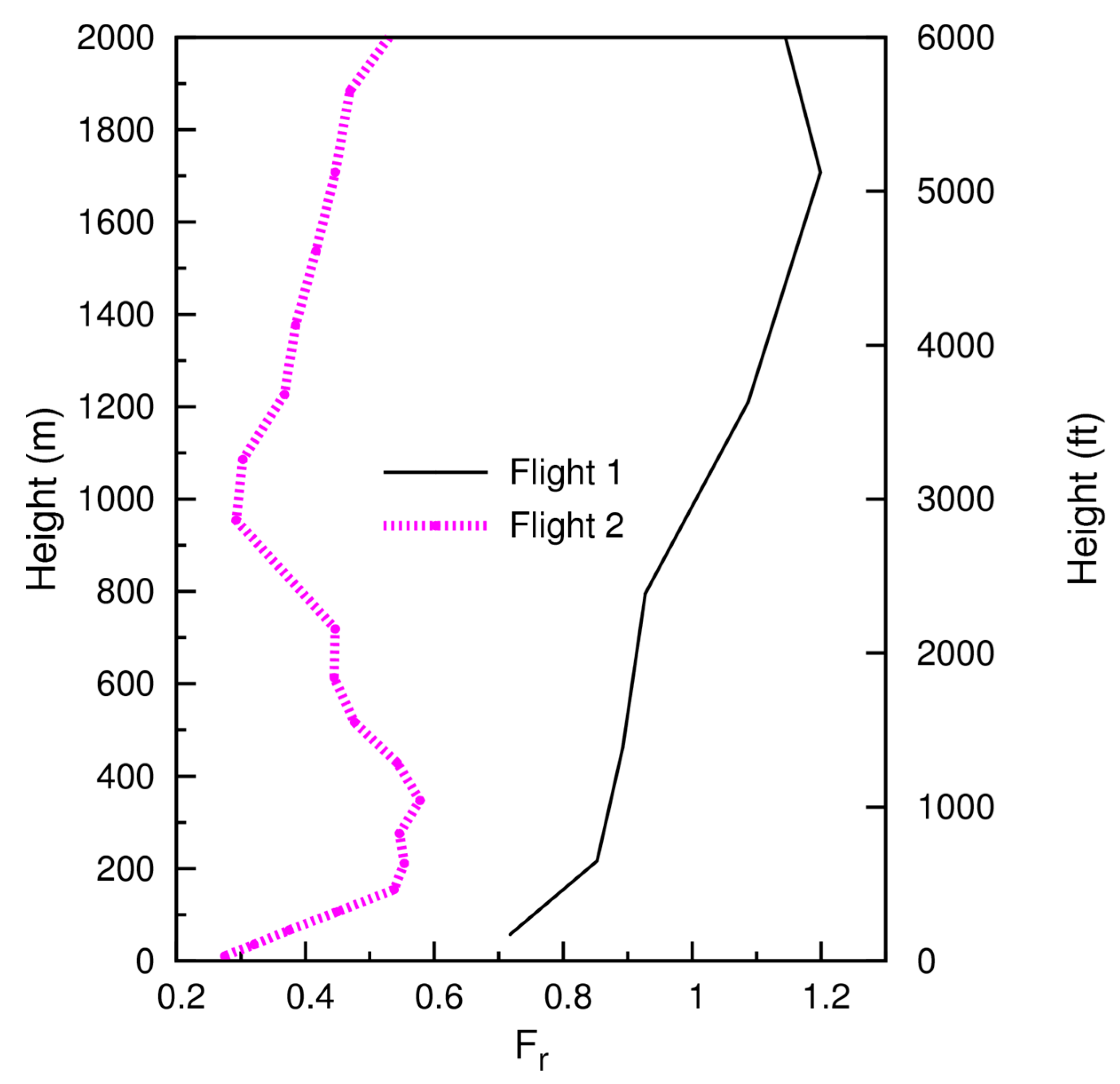
2.4. Froude Number Definitions
2.4.1. Classical Froude Number,
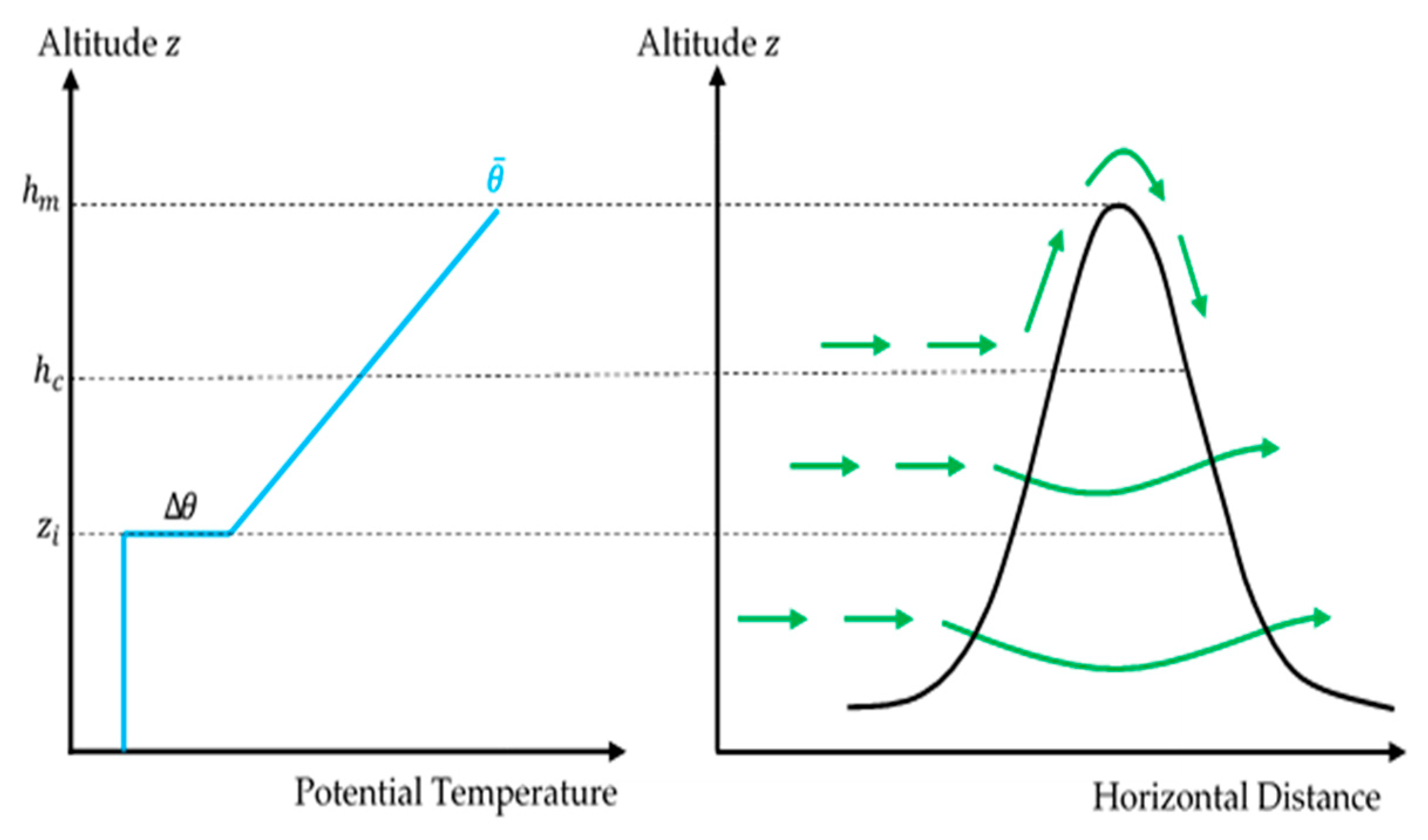
2.4.2. Froude Number () in Terms of the Dividing-Streamline Height
2.4.3. Inversion Froude Number ()
2.5. Turbulence Indicators
2.5.1. Brown Index
2.5.2. Ellrod TI Indexes
2.5.3. CAT1 Indicator
2.5.4. EDR Indicator
2.6. RMSE and MAE for Wind Speed and Direction
- The absolute difference between all AROME levels for each recorded flight altitude, expressed as , is computed and the level with the minimum difference is selected as the best match. This process is repeated for each available flight altitude.
- Once every flight altitude is paired with an AROME level, a latitude and longitude pair must be chosen at each one of these correspondences. Following a similar logic, the best coordinate pair from the model was deemed to minimize the distance relative to the flight coordinates at each altitude.
3. Results and Discussion
3.1. Observations
3.1.1. Aerodrome Wind Data
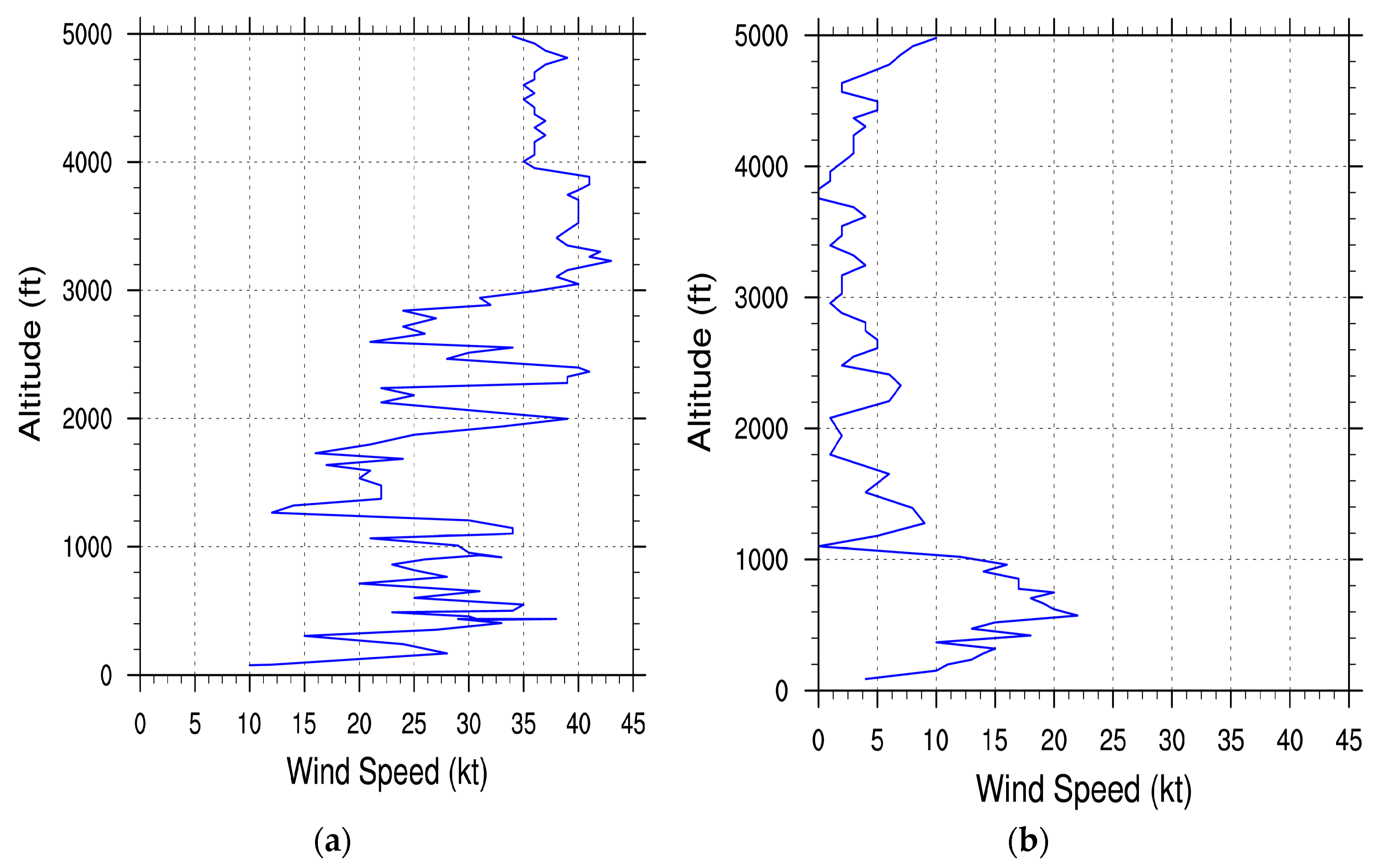
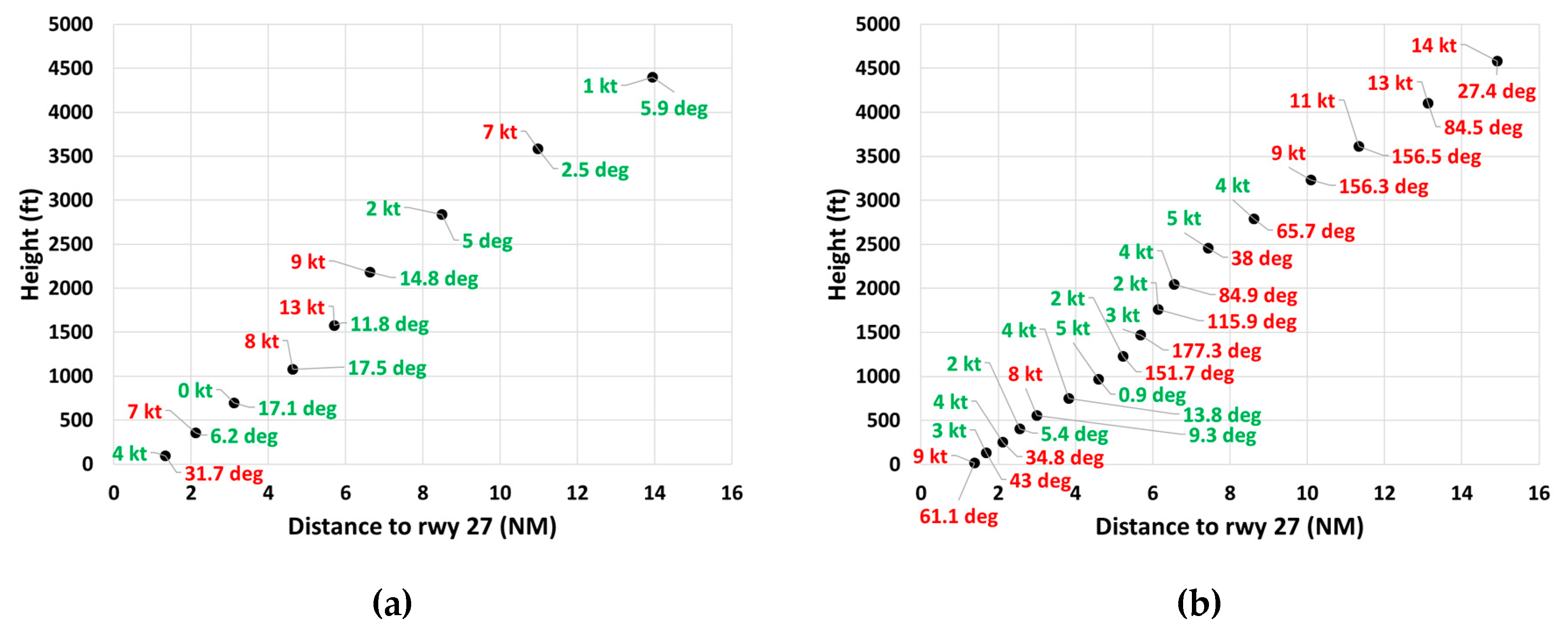
3.1.2. Characterization of Wind Profiles
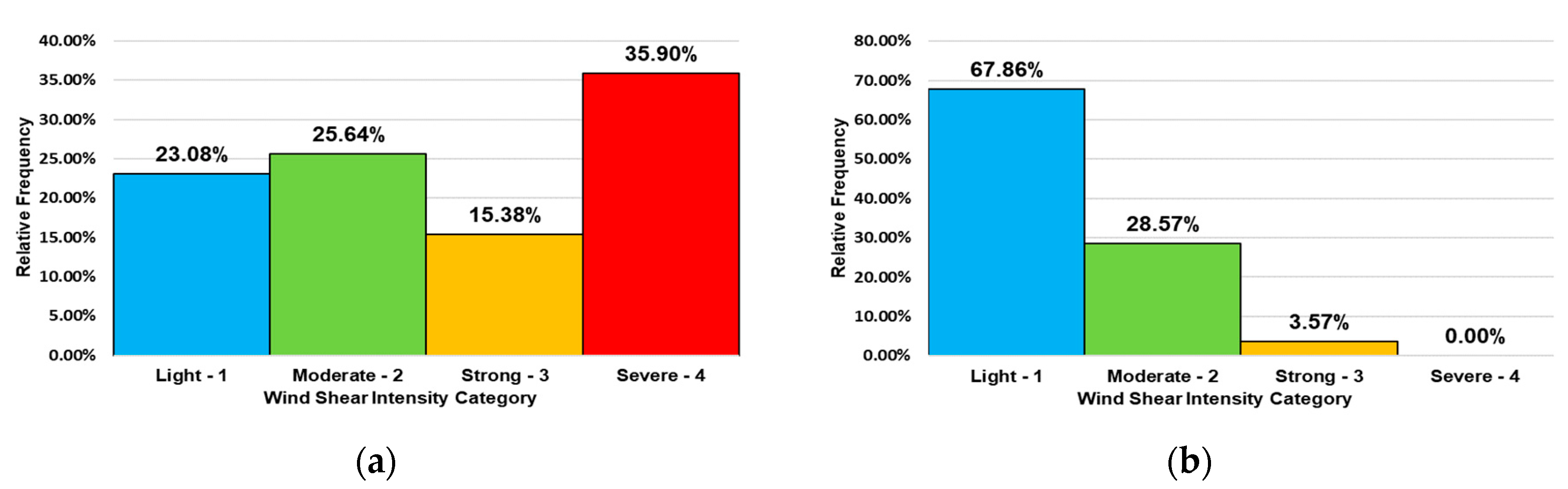
3.1.3. Wind Shear Intensity Factor “I”
3.2. Synoptic Analysis
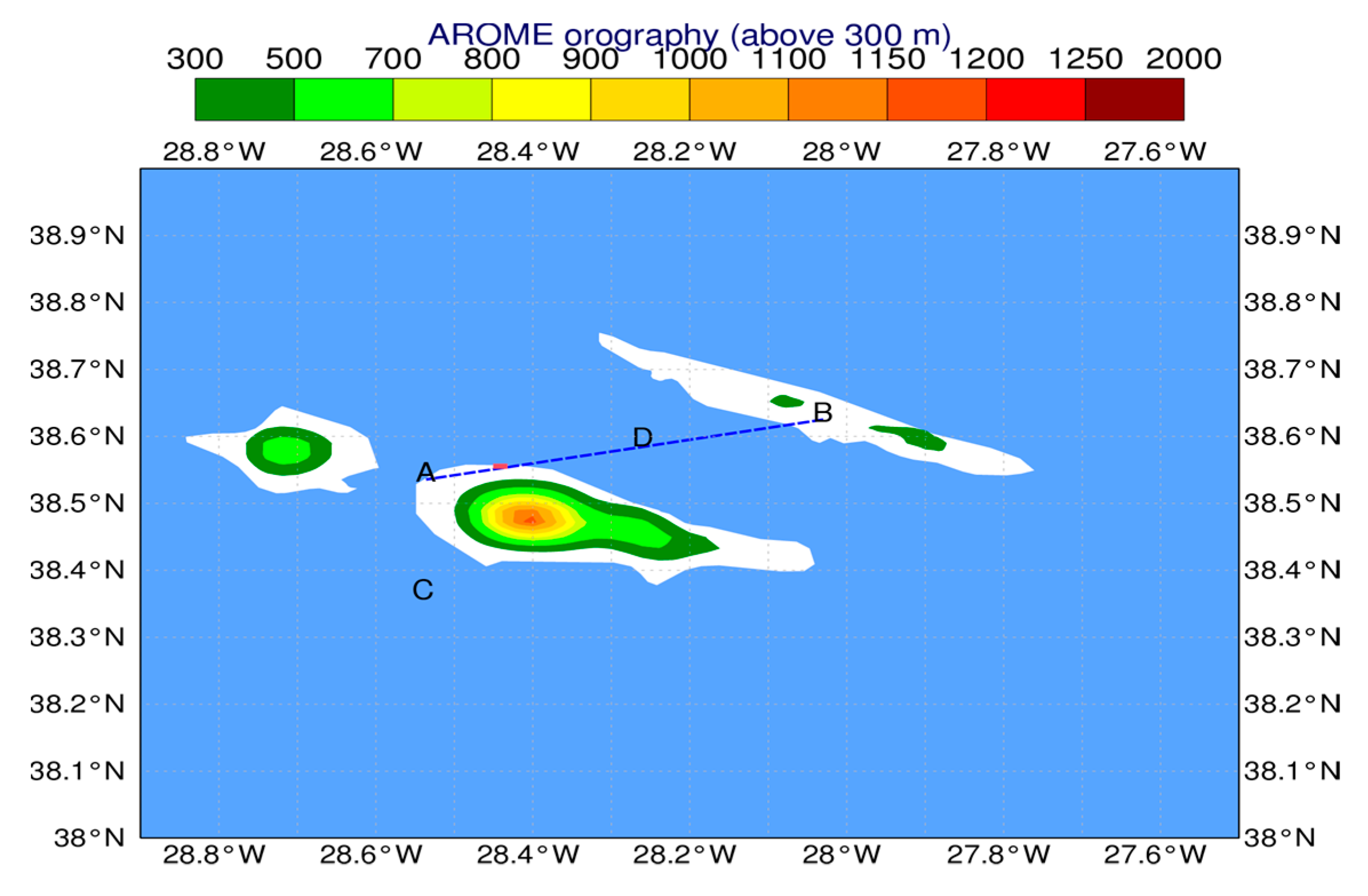
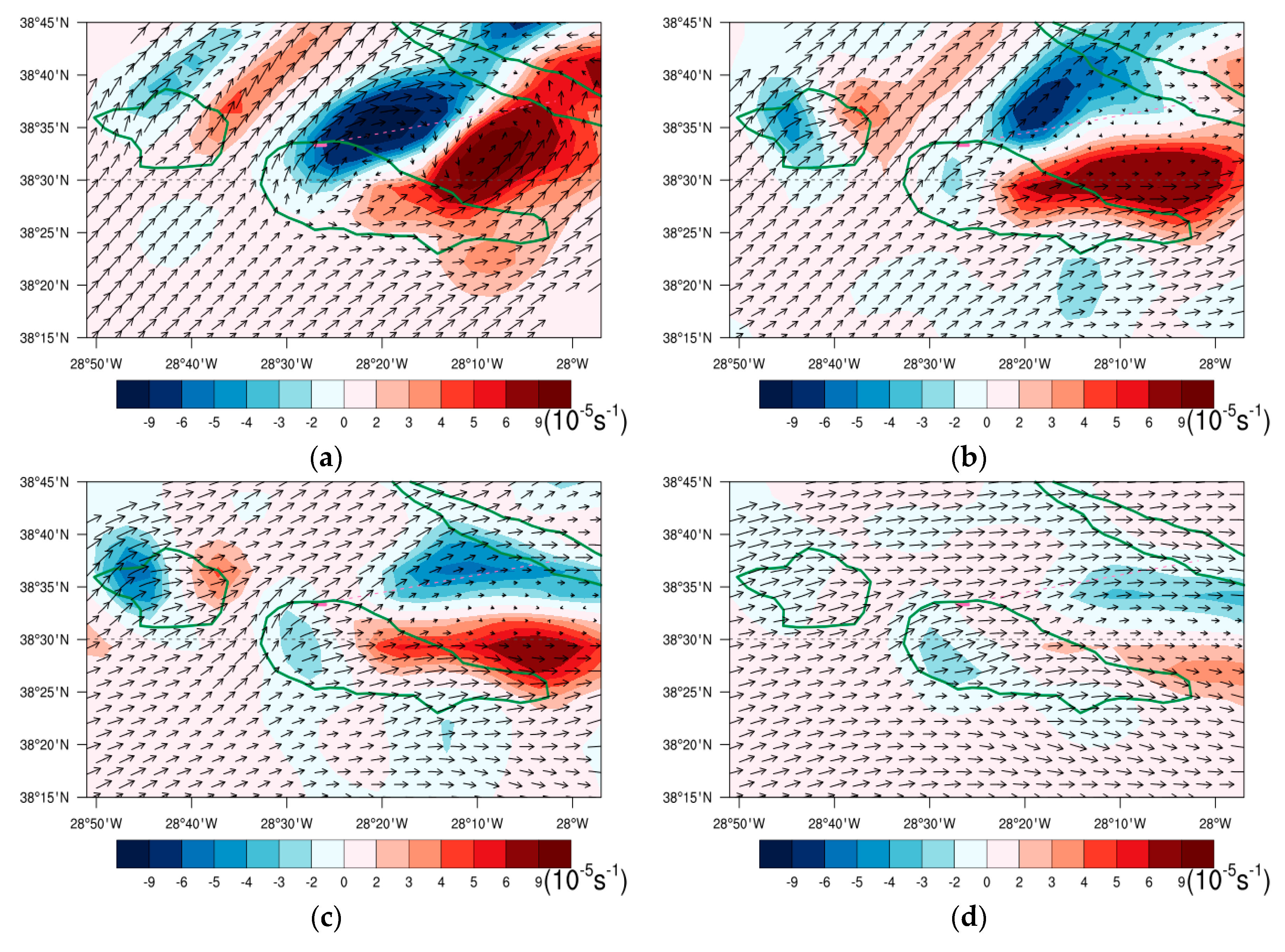
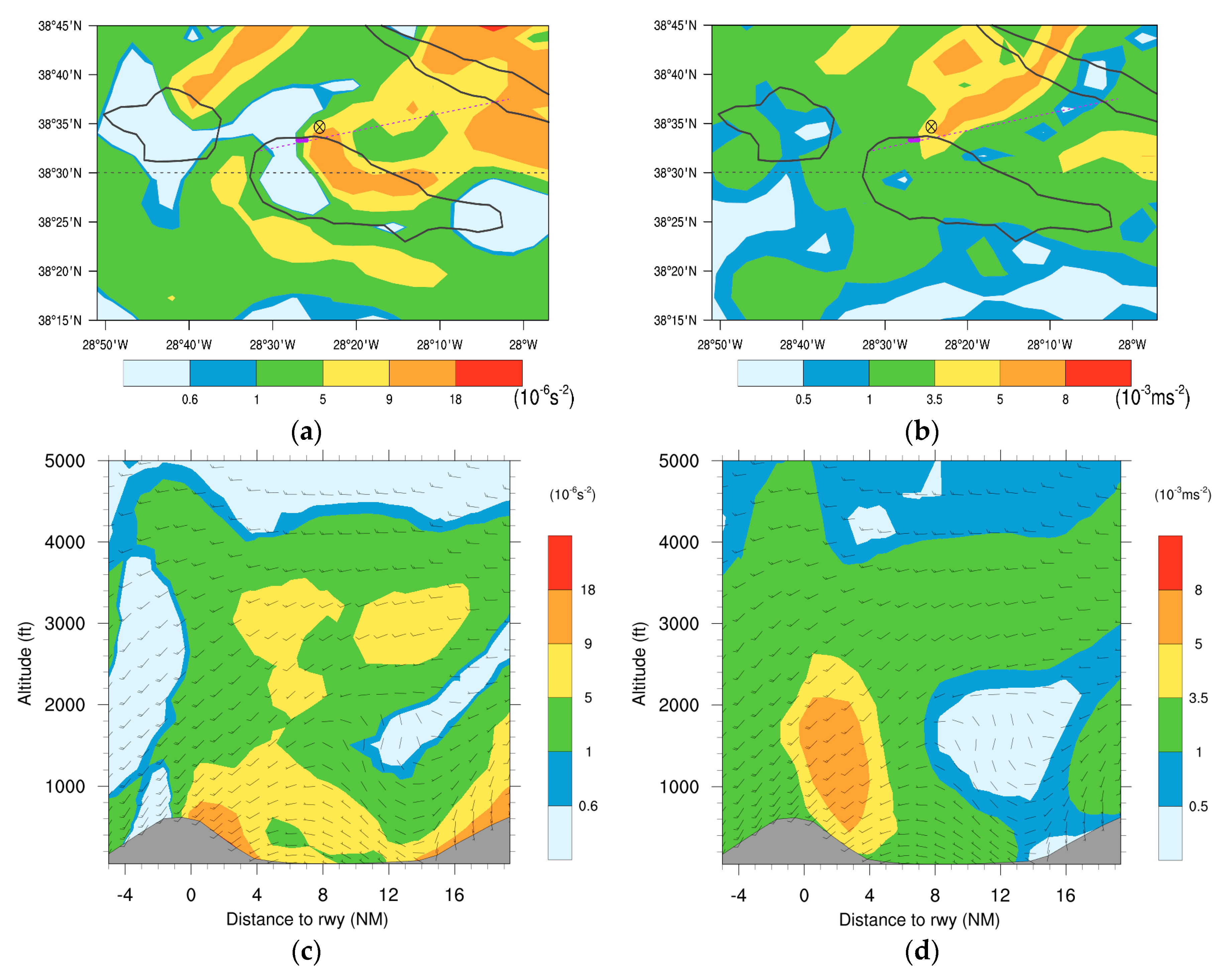
3.3. Mesoscale Analysis
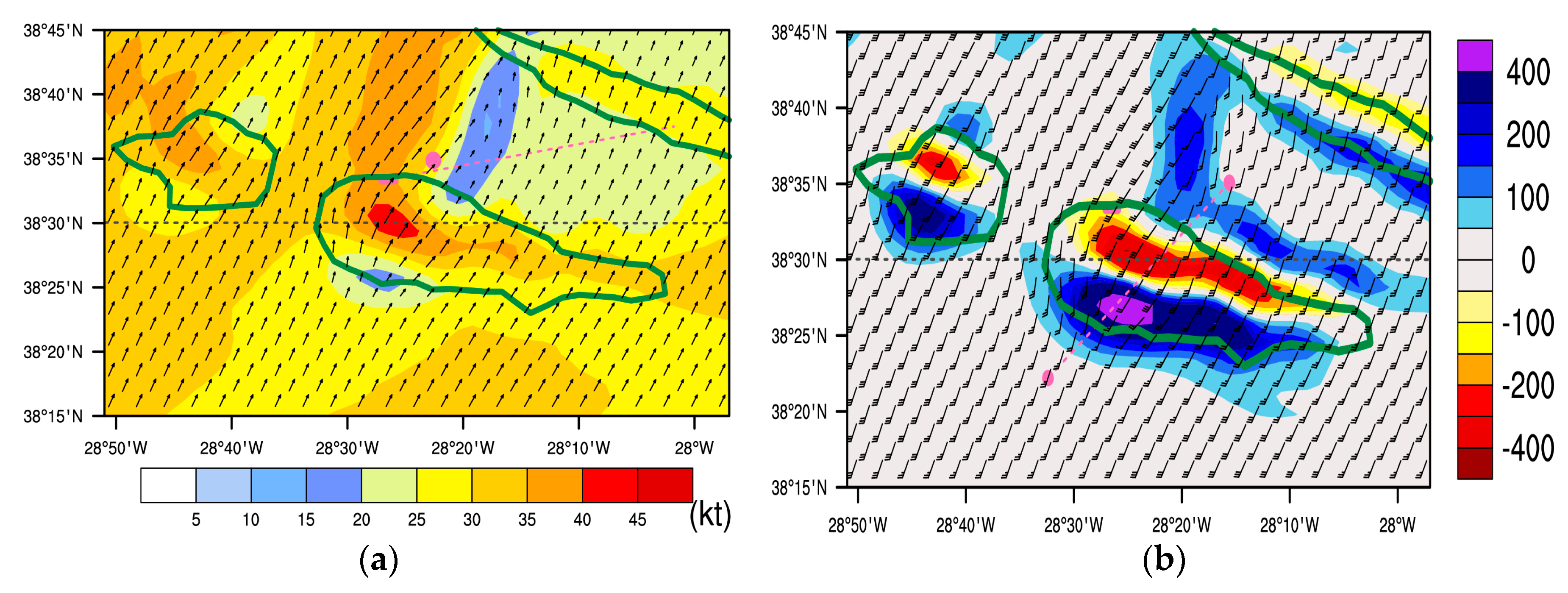
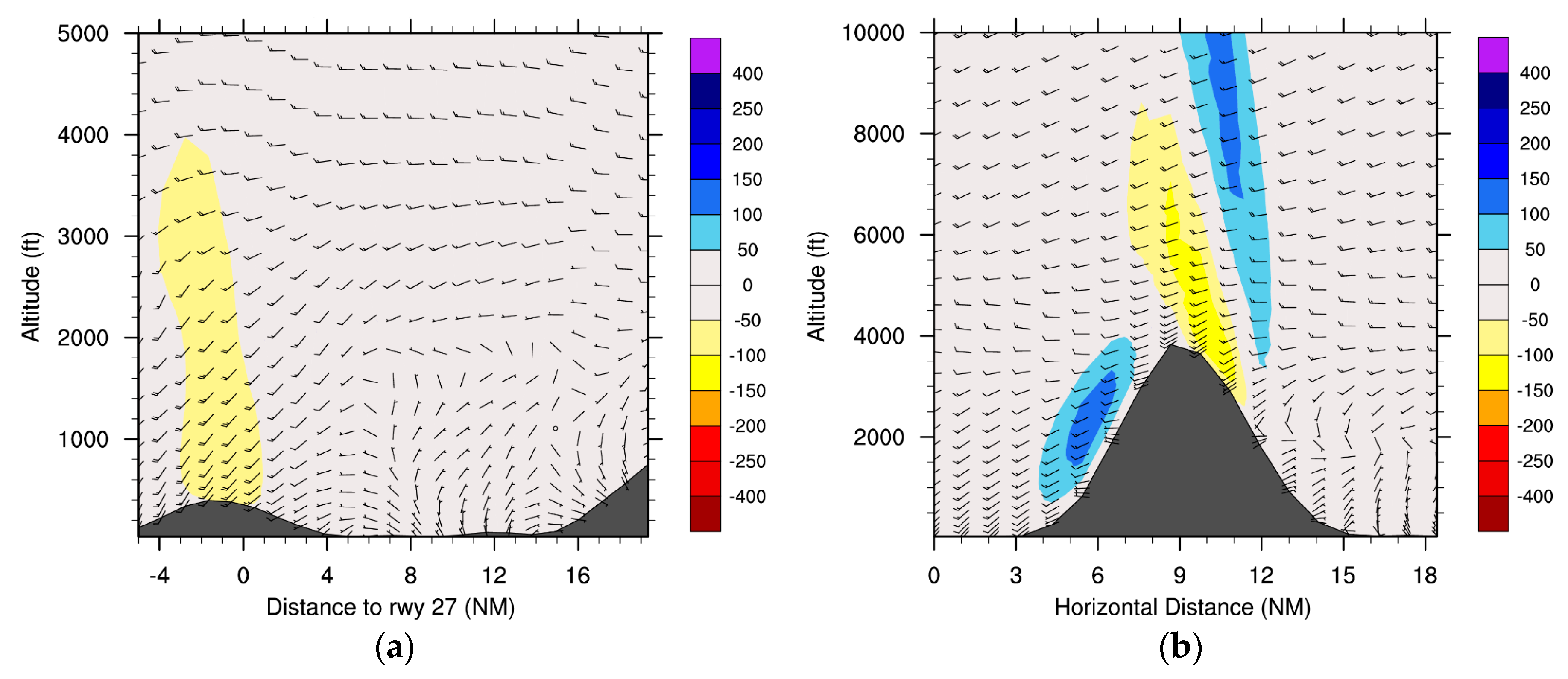
3.3.1. Characterization of Orographic Flow during Flight 1
3.3.2. Characterization of Orographic Flow during Flight 2
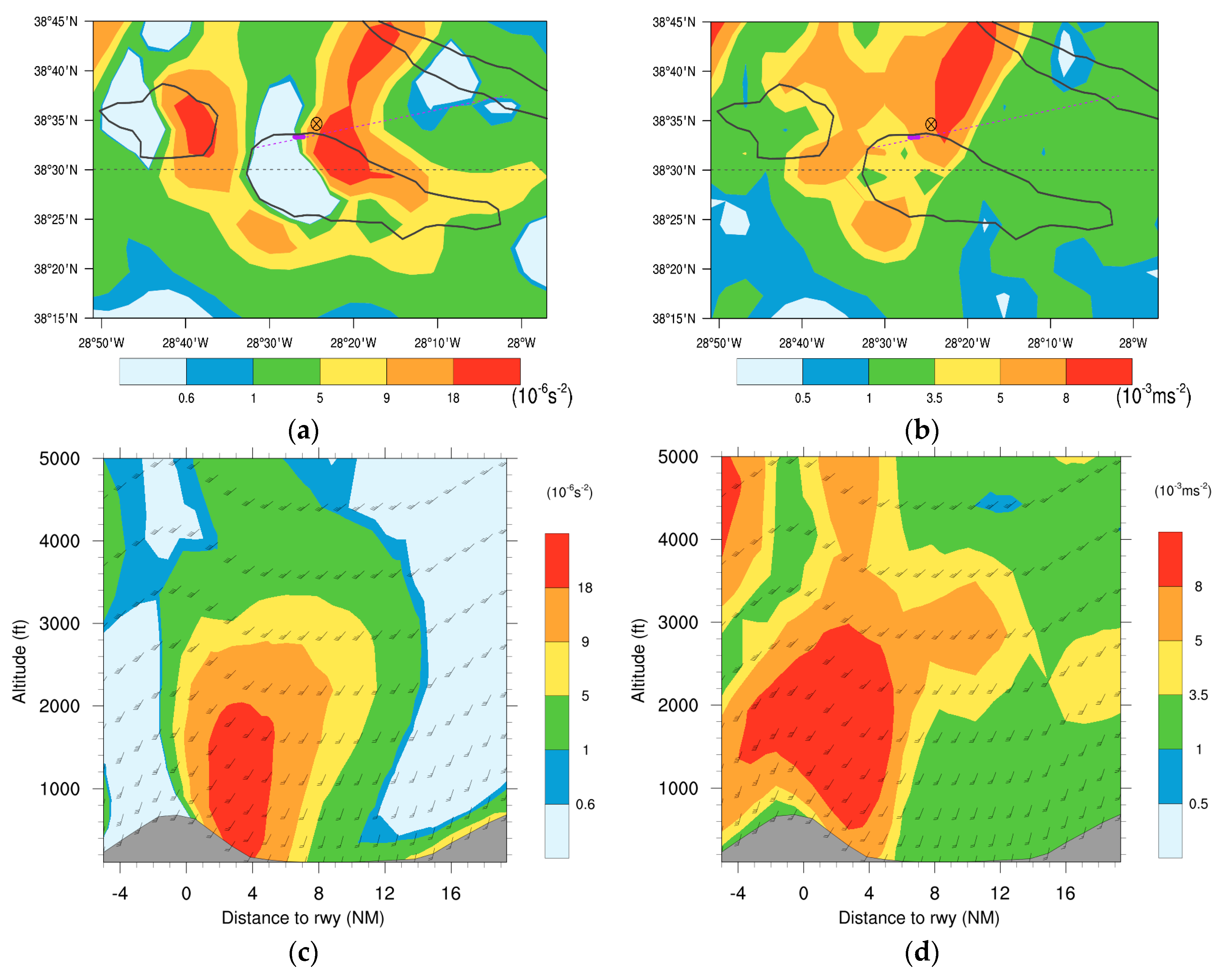
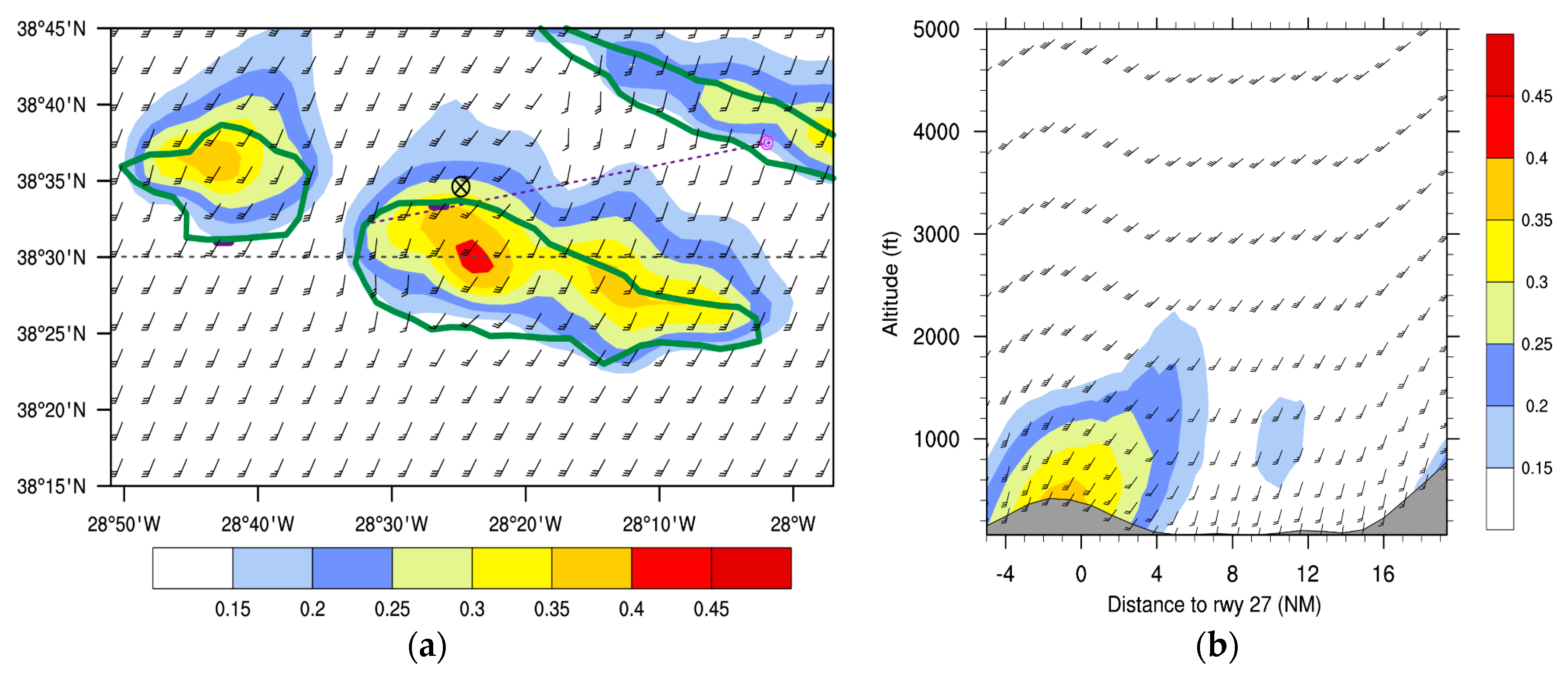
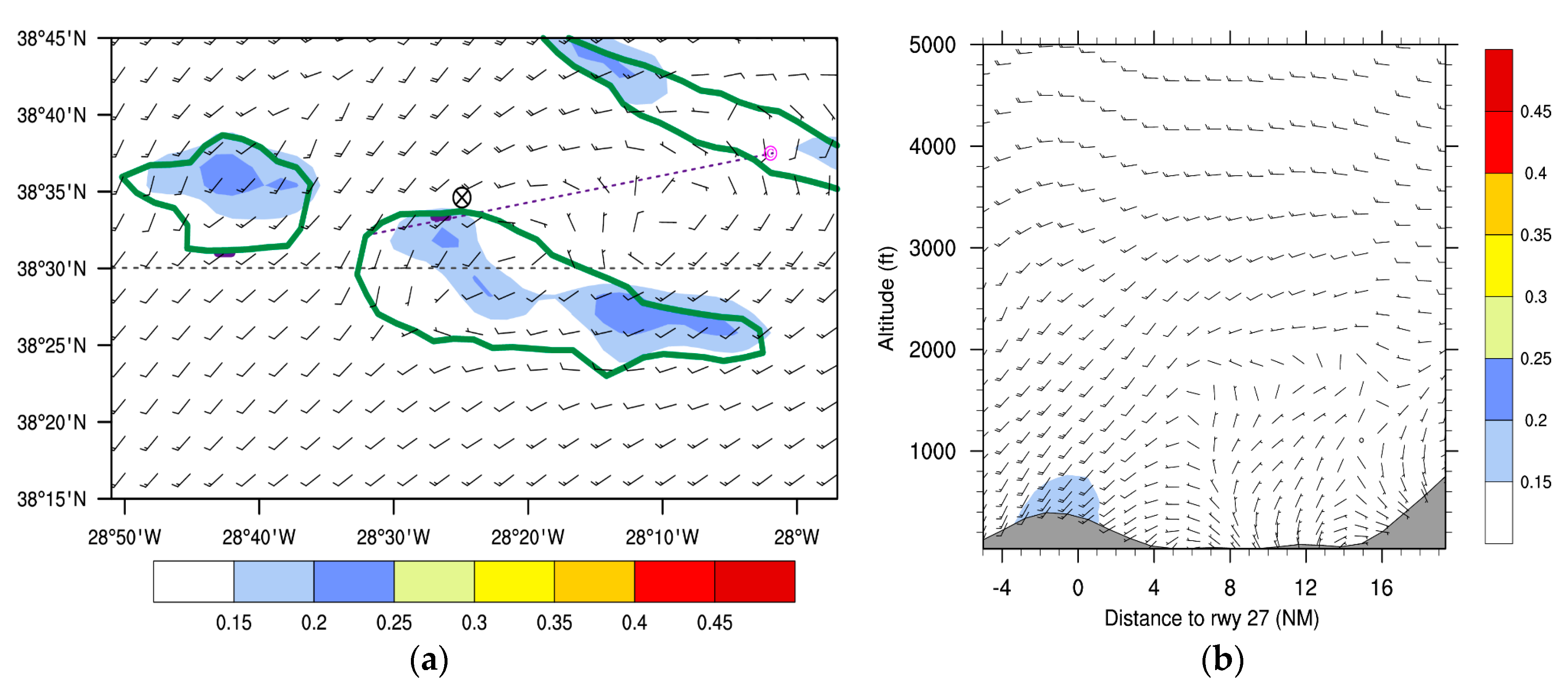
3.4. Turbulence Indicators
3.5. Objective Verification of Wind Forecasts from AROME
4. Summary and Conclusions
Supplementary Materials
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
| Indicator | “Strong” | “Severe” |
|---|---|---|
| Ellrod TI2 [] | ||
| CAT1 [] | ||
| EDR |
References
- Markowski, P.; Richardson, Y. Mesoscale Gravity Waves. In Mesoscale Meteorology in Midlatitudes, 1st ed.; Wiley-Blackwell: Hoboken, NJ, USA, 2010; pp. 161–180. [Google Scholar]
- Bauer, M.H.; Mayr, G.J.; Vergeiner, I.; Pichler, H. Strongly Nonlinear Flow over and around a Three-Dimensional Mountain as a Function of the Horizontal Aspect Ratio. J. Atmos. Sci. 2000, 57, 3971–3991. [Google Scholar] [CrossRef]
- Ólafsson, H.; Bougeault, P. Nonlinear Flow Past an Elliptic Mountain Ridge. J. Atmos. Sci. 1996, 53, 2465–2489. [Google Scholar] [CrossRef]
- Epifanio, C.C. Lee Vortices. In Encyclopedia of the Atmospheric Sciences; Cambridge University Press: Cambridge, UK, 2014; pp. 1150–1160. [Google Scholar]
- Grubišić, V.; Sachsperger, J.; Caldeira, R.M. Atmospheric Wake of Madeira: First Aerial Observations and Numerical Simulations. J. Atmos. Sci. 2015, 72, 4755–4776. [Google Scholar] [CrossRef][Green Version]
- Heinze, R.; Raasch, S.; Etling, D. The Structure of Kármán Vortex Streets in the Atmospheric Boundary Layer derived from Large Eddy Simulation. Meteorol. Z. 2012, 21, 221–237. [Google Scholar] [CrossRef]
- Smolarkiewicz, P.K.; Rotunno, R. Low Froude-Number Flow Past Three-dimensional Obstacles. Part I: Baroclinically Generated Lee Vortices. J. Atmos. Sci. 1989, 46, 1154–1164. [Google Scholar] [CrossRef]
- Li, L.; Chen, Y.-L. Numerical Simulations of Two Trapped Mountain Lee Waves Downstream of Oahu. J. Appl. Meteorol. Climatol. 2017, 56, 1305–1324. [Google Scholar] [CrossRef]
- Keller, T.L.; Trier, S.B.; Hall, W.D.; Sharman, R.D.; Xu, M.; Liu, Y. Lee Waves Associated with a Commercial Jetliner Accident at Denver International Airport. J. Appl. Meteorol. Climatol. 2015, 54, 1373–1392. [Google Scholar] [CrossRef][Green Version]
- Parker, T.J.; Lane, T.P. Trapped Mountain Waves during a Light Aircraft Accident. Aust. Meteorol. Oceanogr. J. 2013, 63, 377–389. [Google Scholar] [CrossRef]
- Caldeira, R.M.A.; Tomé, R. Wake Response to an Ocean-Feedback Mechanism: Madeira Island Case Study. Bound. Layer Meteorol. 2013, 148, 419–436. [Google Scholar] [CrossRef][Green Version]
- Couvelard, X.; Caldeira, R.M.A.; Araújo, I.B.; Tomé, R. Wind Meditated Vorticity-generation and Eddy-confinement, Leeward of the Madeira Island: 2008 Numerical Case Study. Dyn. Atmos. Oceans 2012, 58, 128–149. [Google Scholar] [CrossRef]
- Barata, J.M.M.; Medeiros, R.C.; Silva, A.R.R. An Experimental Study of Terrain-Induced Airflow at the LPPI Airport. In Proceedings of the 10th AIAA Aviation Technology, Integration, and Operations (ATIO) Conference, Fort Worth, TX, USA, 13–15 September 2010; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2010; pp. 1–8. [Google Scholar]
- Sayers, D.; Stewart, M. Background Information. In Azores, 6th ed.; The Globe Pequot Press Inc.: Guilford, CT, USA, 2016; pp. 2–3. [Google Scholar]
- Sharman, R.D.; Pearson, J.M. Prediction of Energy Dissipation Rates for Aviation Turbulence. Part I: Forecasting Nonconvective Turbulence. J. Appl. Meteor. Climatol. 2017, 56, 317–337. [Google Scholar] [CrossRef]
- Stull, R.B. An Introduction to Boundary Layer Meteorology; Springer: Berlin, Germany, 1988; 666p. [Google Scholar]
- Jiménez, P.A.; Dudhia, J. Improving the Representation of Resolved and Unresolved Topographic Effects on Surface Wind in the WRF Model. J. Appl. Meteorol. Climatol. 2012, 51, 300–316. [Google Scholar] [CrossRef]
- Chow, F.K.; Street, R.L. Evaluation of Turbulence Closure Models for Large-Eddy Simulation over Complex Terrain: Flow over Askervein Hill. J. Appl. Meteorol. Climatol. 2009, 48, 1050–1065. [Google Scholar] [CrossRef]
- Svensson, G.; Holtslag, A.A.M. Analysis of Model Results for the Turning of the Wind and Related Momentum Fluxes in the Stable Boundary Layer. Bound. Layer Meteorol. 2009, 132, 261–277. [Google Scholar] [CrossRef]
- Brown, A.R.; Beljaars, A.C.M.; Hersbach, H.; Hollingsworth, A.; Miller, M.; Vasiljevic, D. Wind turning across the marine atmospheric boundary layer. Q. J. R. Meteorol. Soc. 2005, 131, 1233–1250. [Google Scholar] [CrossRef]
- Brown, A.R.; Beare, R.J.; Edwards, J.M.; Lock, A.P.; Keogh, S.J.; Milton, S.F.; Walters, D.N. Upgrades to the boundary-layer scheme in the Met Office numerical weather prediction model. Bound. Layer Meteor. 2008, 128, 117–132. [Google Scholar] [CrossRef]
- Ricard, D.; Lac, C.; Riette, S.; Legrand, R.; Mary, A. Kinetic energy spectra characteristics of two convection-permitting limited-area models AROME and Meso-NH. Q. J. R. Meteorol. Soc. 2013, 139, 1327–1341. [Google Scholar] [CrossRef]
- Woodfield, A.A.; Woods, J.F. Worldwide experience of windshear during 1981–1982. In AGARD Flight Mechanics Panel Conf. on Flight Mechanics and System Design Lessons from Operational Experience; AGARD CP; NATO-STO: Brussel, Belgium, 1983; Volume 347, 28p. [Google Scholar]
- International Civil Aviation Organization (ICAO). Manual on Low-level Wind Shear, 1st ed.; International Civil Aviation Organization: Montreal, QC, Canada, 2005; p. 213. [Google Scholar]
- Guan, W.-L.; Yong, K. Review of Aviation Accidents Caused by Wind Shear and Identification Methods. J. Chin. Soc. Mech. Eng. 2002, 23, 99–110. [Google Scholar]
- Seity, Y.; Brousseau, P.; Malardel, S.; Hello, G.; Bénard, P.; Bouttier, F.; Lac, C.; Masson, V. The AROME-France Convective-Scale Operational Model. Mon. Weather Rev. 2011, 139, 976–991. [Google Scholar] [CrossRef]
- Cuxart, J.; Bougeault, P.; Redelsperger, J.-L. A Turbulence Scheme Allowing for Mesoscale and Large-eddy Simulations. Q. J. R. Meteorol. Soc. 2006, 126, 1–30. [Google Scholar] [CrossRef]
- Sutherland, B.R. Generation Mechanisms. In Internal Gravity Waves; Cambridge University Press: Cambridge, UK, 2018; pp. 284–285. [Google Scholar]
- Lynch, A.H.; Cassano, J.J. Mountain Weather. In Atmospheric Dynamics; Wiley: Hoboken, NJ, USA, 2006; pp. 209–232. [Google Scholar]
- Holton, J. An Introduction to Dynamic Meteorology, 4th ed.; Elsevier Inc.: Amsterdam, The Netherlands, 2004; Volume 88, 535p. [Google Scholar]
- Sheridan, P.F.; Vosper, S.B. A Flow Regime Diagram for Forecasting Lee Waves, Rotors and Downslope Winds. Meteorol. Appl. 2006, 13, 179–195. [Google Scholar] [CrossRef]
- Sheppard, P.A. Airflow over Mountains. Q. J. R. Meteorol. Soc. 1956, 82, 528–529. [Google Scholar] [CrossRef]
- Sha, W.; Nakabayashi, K.; Ueda, H. Numerical Study on Flow Pass of a Three-dimensional Obstacle under a Strong Stratification Condition. J. Appl. Meteorol. 1998, 37, 1047–1054. [Google Scholar] [CrossRef]
- Vosper, S.B.; Gardner, B.A. Measurements of the Near Surface Flow over a Hill. Q. J. R. Meteorol. Soc. 2002, 128, 2257–2280. [Google Scholar] [CrossRef]
- Jiang, Q.; Doyle, J.D.; Smith, R.B. Blocking, Descent and Gravity Waves: Observations and Modelling of a MAP Northerly Föhn Event. Q. J. R. Meteorol. Soc. 2005, 131, 675–701. [Google Scholar] [CrossRef]
- Trombetti, F.; Tampieri, F. An Application of the Dividing-Streamline Concept to the Stable Airflow over Mesoscale Mountains. Mon. Weather Rev. 1987, 115, 1802–1806. [Google Scholar] [CrossRef]
- Wooldridge, G.L.; Fox, D.G.; Furman, R.W. Air-Flow Patterns over and around a Large 3-Dimensional Hill. Meteorol. Atmos. Phys. 1987, 37, 259–270. [Google Scholar] [CrossRef]
- Vosper, S.B. Inversion Effects on Mountain Lee Waves. Q. J. R. Meteorol. Soc. 2004, 130, 1723–1748. [Google Scholar] [CrossRef]
- Schär, C.; Smith, R.B. Shallow-Water Flow past Isolated Topography. Part I: Vorticity Production and Wake Formation. J. Atmos. Sci. 1993, 50, 1373–1400. [Google Scholar]
- Gill, P.G.; Buchanan, P. An Ensemble based Turbulence Forecasting System. Meteorol. Appl. 2014, 21, 12–19. [Google Scholar] [CrossRef]
- Sharman, R.; Tebaldi, C.; Wiener, G.; Wolff, J. An Integrated Approach to Mid- and Upper-Level Turbulence Forecasting. Weather Forecast. 2006, 21, 268–287. [Google Scholar] [CrossRef]
- Frech, M.; Holzäpfel, F.; Tafferner, A.; Gerz, T. High-Resolution Weather Database for the Terminal Area of Frankfurt Airport. Am. Meteorol. Soc. 2007, 46, 1913–1932. [Google Scholar] [CrossRef]
- International Civil Aviation Organization (ICAO). Meteorological Service for International Air Navigation: Annex 3 to the Convention on International Civil Aviation, 20th ed.; International Standards and Recommended Practices Technical Report; International Civil Aviation Organization: Montreal, QC, Canada, 2007; p. 218. [Google Scholar]
- Jiménez, P.A.; Dudhia, J. On the Ability of the WRF Model to Reproduce the Surface Wind Direction over Complex Terrain. J. Appl. Meteorol. Climatol. 2013, 52, 1610–1617. [Google Scholar] [CrossRef]
- Smith, R.B.; Grubišić, V. Aerial Observations of Hawaii’s Wake. J. Atmos. Sci. 1993, 50, 3728–3750. [Google Scholar] [CrossRef]
- International Civil Aviation Organization (ICAO). Meeting of the Meteorology Panel (METP): Proposed Revision to Eddy Dissipation Rate (EDR) Values in Annex 3; International Civil Aviation Organization: Montreal, QC, Canada, 2018; pp. 1–6. [Google Scholar]
- Sharman, R.D.; Cornman, L.B.; Meymaris, G.; Pearson, J.; Farrar, T. Descriptions and Derived Climatologies of Automated in Situ Eddy-Dissipation-Rate Reports of Atmospheric Turbulence. J. Appl. Meteorol. Climatol. 2014, 53, 1416–1432. [Google Scholar] [CrossRef]
- Colle, B.A.; Mass, C.F. High-Resolution Observations and Numerical Simulations of Easterly Gap Flow through the Strait of Juan de Fuca on 9–10 December 1995. Mon. Weather Rev. 2000, 128, 2398–2422. [Google Scholar] [CrossRef]





| Flight | Time | Wind Direction | Wind Speed | Gust | ||||
|---|---|---|---|---|---|---|---|---|
| Flight 1 | T1 | 220° | 18 | 28 | −12 | −14 | −18 | −21 |
| T1+1hr | 220° | 21 | 31 | −13 | −16 | −20 | −24 | |
| Flight 2 | T2 | 200° | 13 | − | −4 | −12 | − | − |
| T2+0.5hr | 210° | 12 | − | −6 | −10 | − | − |
| Flight | Altitude | Classification | Rating | ||
|---|---|---|---|---|---|
| Flight 1 | 228 ft (69 m) | 1.649 | 0.170 | Severe | 4 |
| 164 ft (50 m) | 8.820 | 0.476 | Severe | 4 | |
| 90 ft (27 m) | 2.962 | 0.138 | Severe | 4 | |
| Flight 2 | 214 ft (65 m) | 0.265 | 0.015 | Light | 1 |
| 169 ft (52 m) | 0.264 | 0.015 | Light | 1 | |
| 128 ft (39 m) | 0.729 | 0.042 | Moderate | 2 |
| Parameter | Flight 1 | Flight 2 |
|---|---|---|
| (Equation (3)) | 0.95 | 0.42 |
| (Equation (6)) | 0.90 | 0.31 |
| (Equation (7)) | 0.92 | |
| (Equation (5)) | 107 | 855 |
| (Equations (3),(6)) | 60 | 696 |
| − | 872 |
| Flight | RMSEWSPD [ms−1] | RMSEWDIR [deg] | MAEWSPD [ms−1] | MAEWDIR [deg] |
|---|---|---|---|---|
| Flight 1 | 3.4 | 11.4 | 2.8 | 8.1 |
| Flight 2 | 4.4 | 83.3 | 3.8 | 65.4 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Maruhashi, J.; Serrão, P.; Belo-Pereira, M. Analysis of Mountain Wave Effects on a Hard Landing Incident in Pico Aerodrome Using the AROME Model and Airborne Observations. Atmosphere 2019, 10, 350. https://doi.org/10.3390/atmos10070350
Maruhashi J, Serrão P, Belo-Pereira M. Analysis of Mountain Wave Effects on a Hard Landing Incident in Pico Aerodrome Using the AROME Model and Airborne Observations. Atmosphere. 2019; 10(7):350. https://doi.org/10.3390/atmos10070350
Chicago/Turabian StyleMaruhashi, Jin, Pedro Serrão, and Margarida Belo-Pereira. 2019. "Analysis of Mountain Wave Effects on a Hard Landing Incident in Pico Aerodrome Using the AROME Model and Airborne Observations" Atmosphere 10, no. 7: 350. https://doi.org/10.3390/atmos10070350
APA StyleMaruhashi, J., Serrão, P., & Belo-Pereira, M. (2019). Analysis of Mountain Wave Effects on a Hard Landing Incident in Pico Aerodrome Using the AROME Model and Airborne Observations. Atmosphere, 10(7), 350. https://doi.org/10.3390/atmos10070350






