Macro–Microscale Research on the Single Shear Characteristics of the Root–Loess Interface in Robinia pseudoacacia
Abstract
1. Introduction
2. Materials and Methods
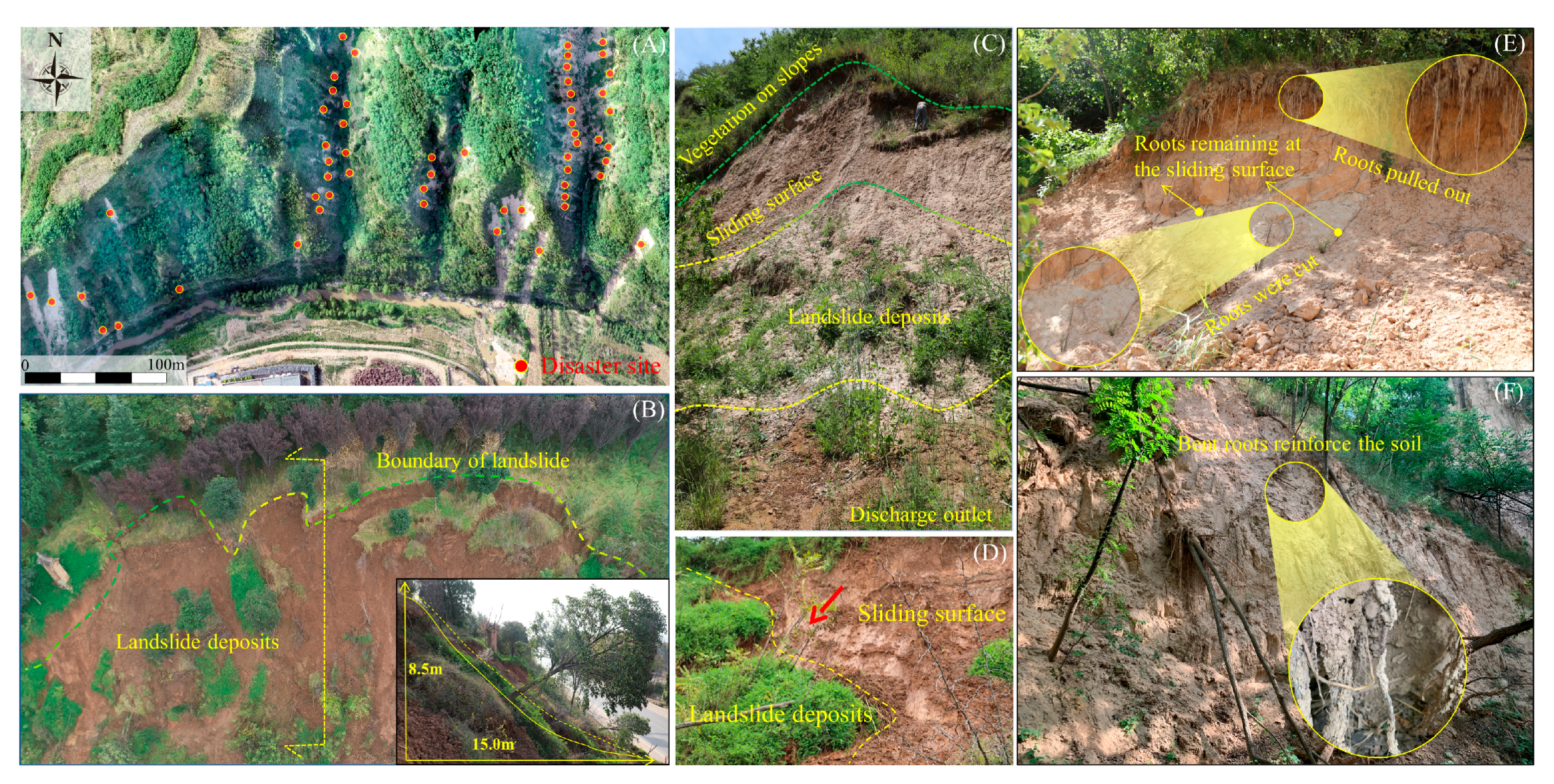
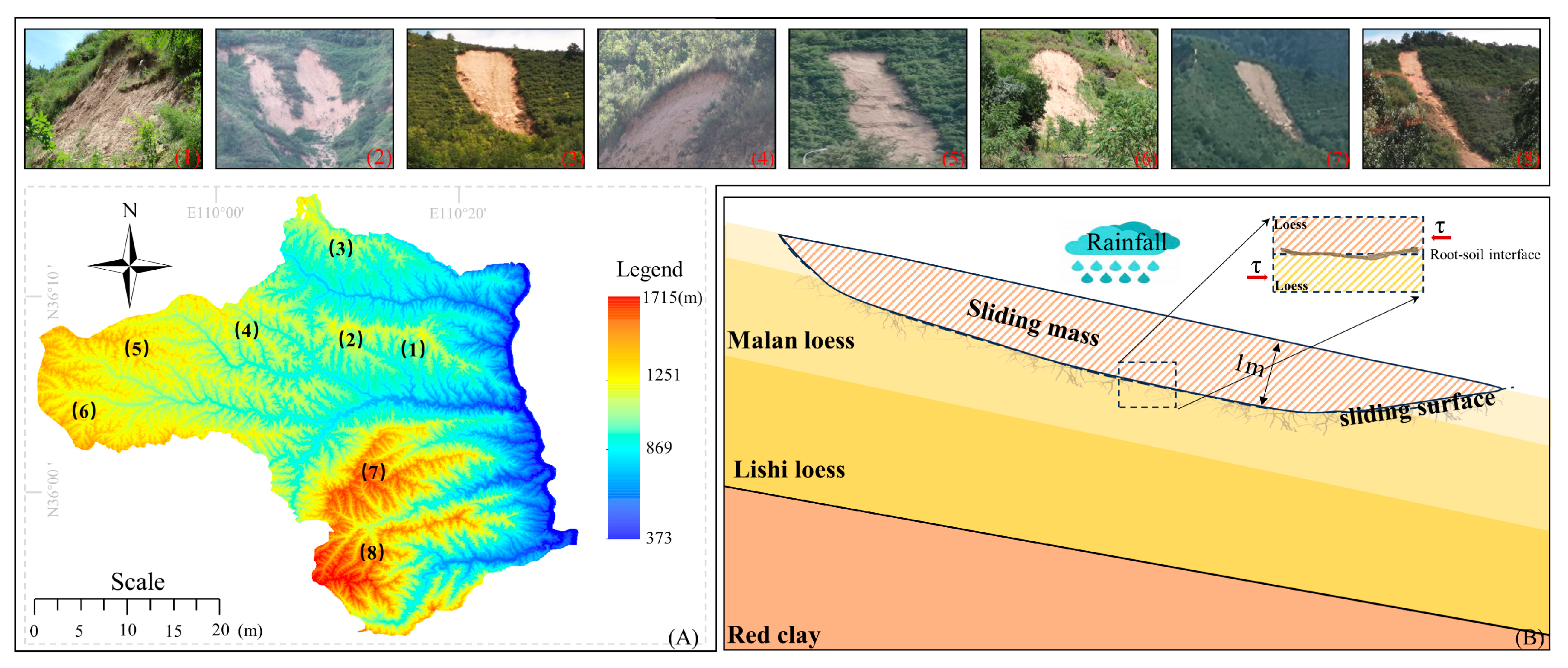
2.1. Geologic Conditions of Typical Landslide Area to Be Engineered
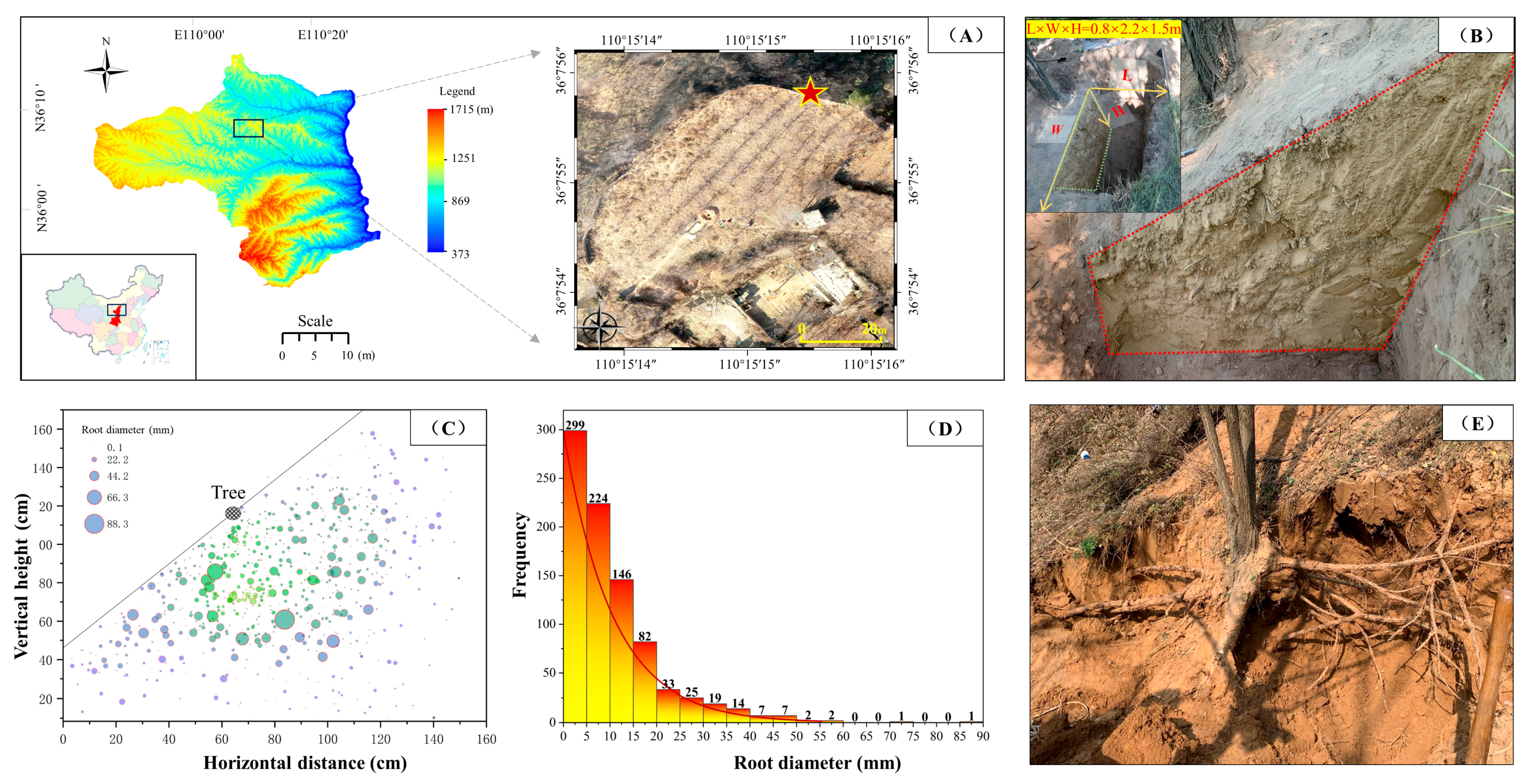
2.2. Sample Collection and Classification
2.3. Test Methods
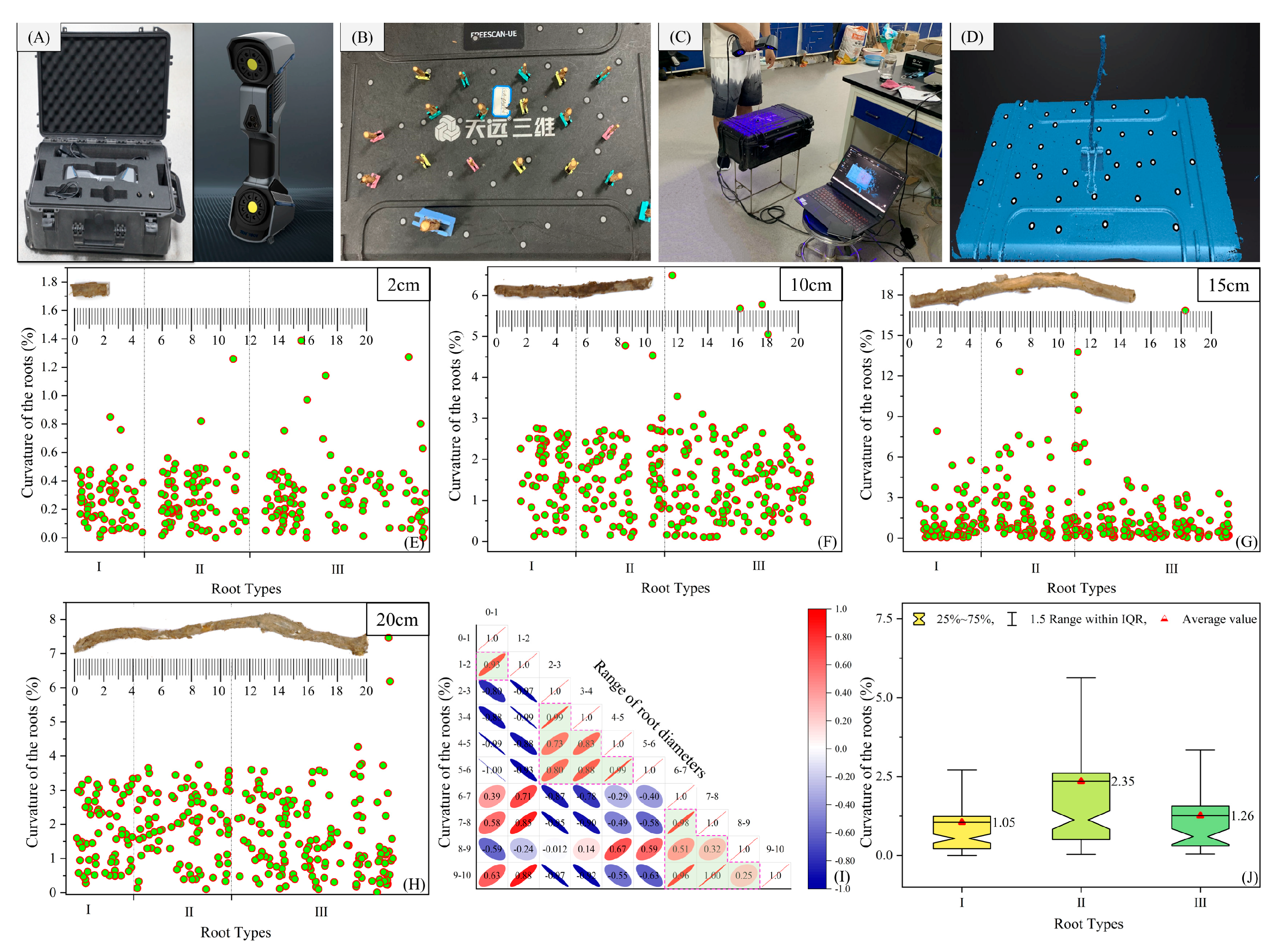
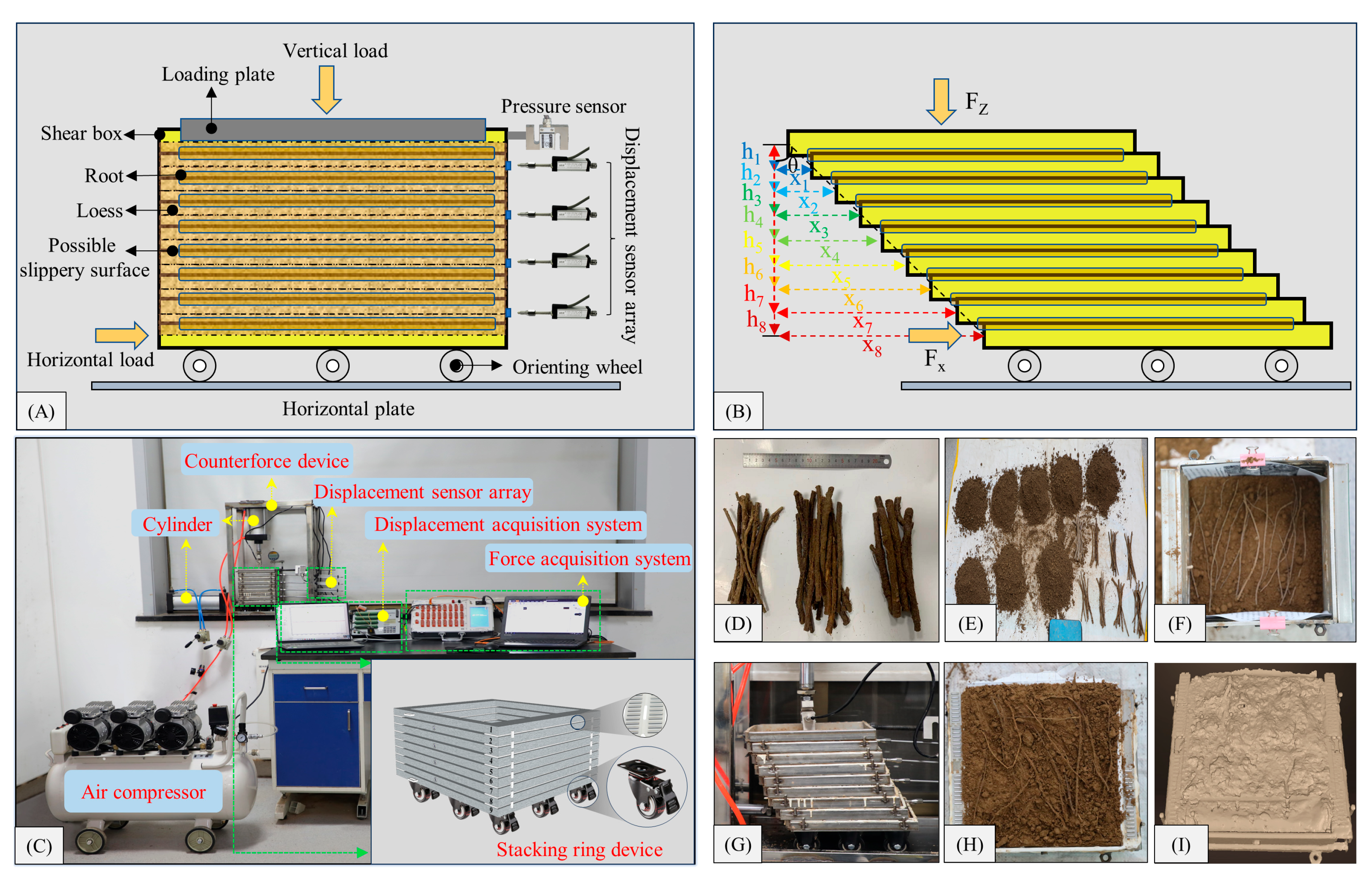
2.4. Test Apparatus and Methods
2.4.1. Single Shear Test at the Root–Loess Interface
2.4.2. Particle Flow Discrete Element Test
3. Results and Discussion
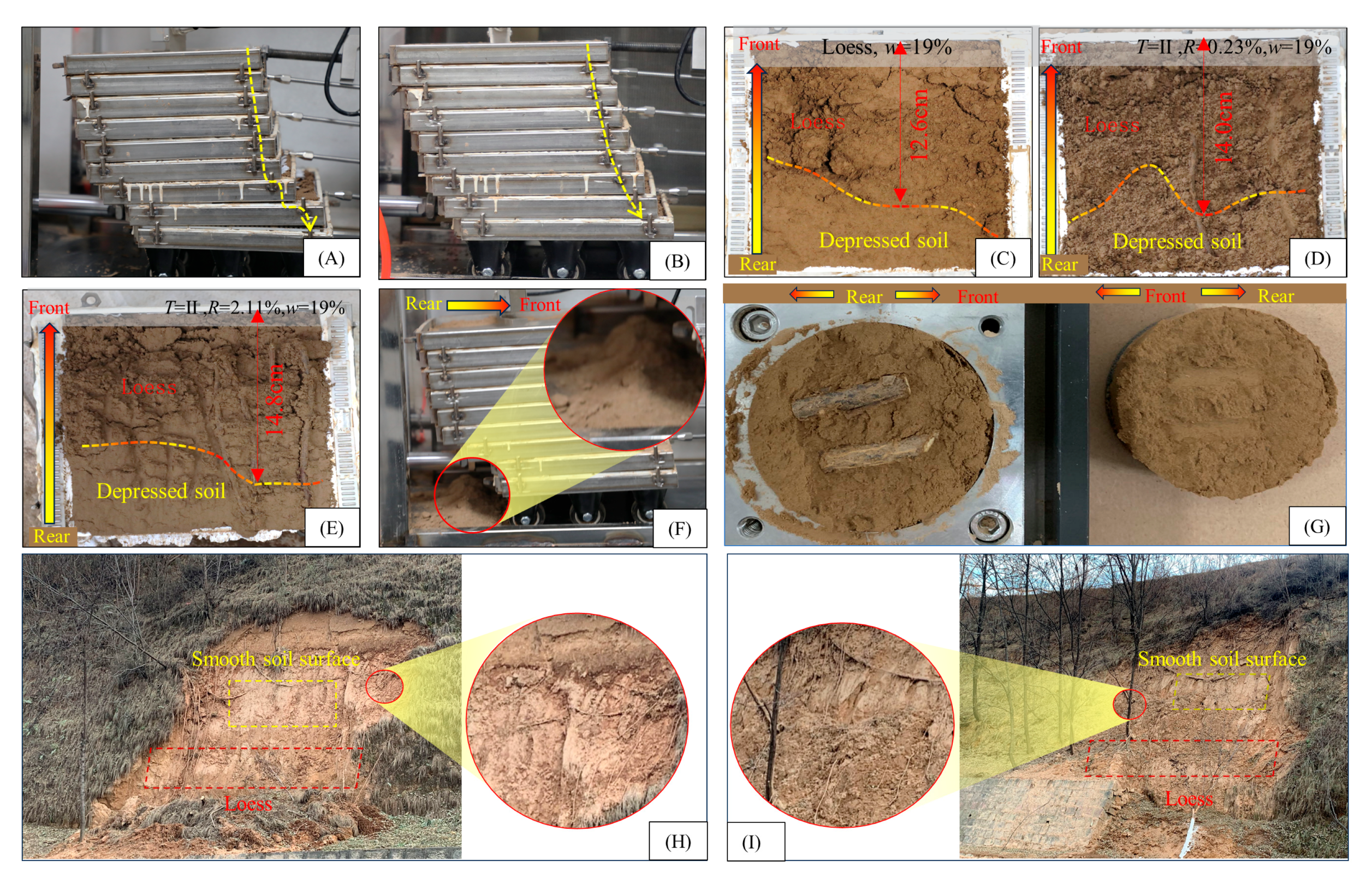
3.1. Characteristics of the Root–Loess Shear Failure Surface
3.1.1. Macroscopic Morphological Characteristics of the Root–Loess Shear Failure Surface
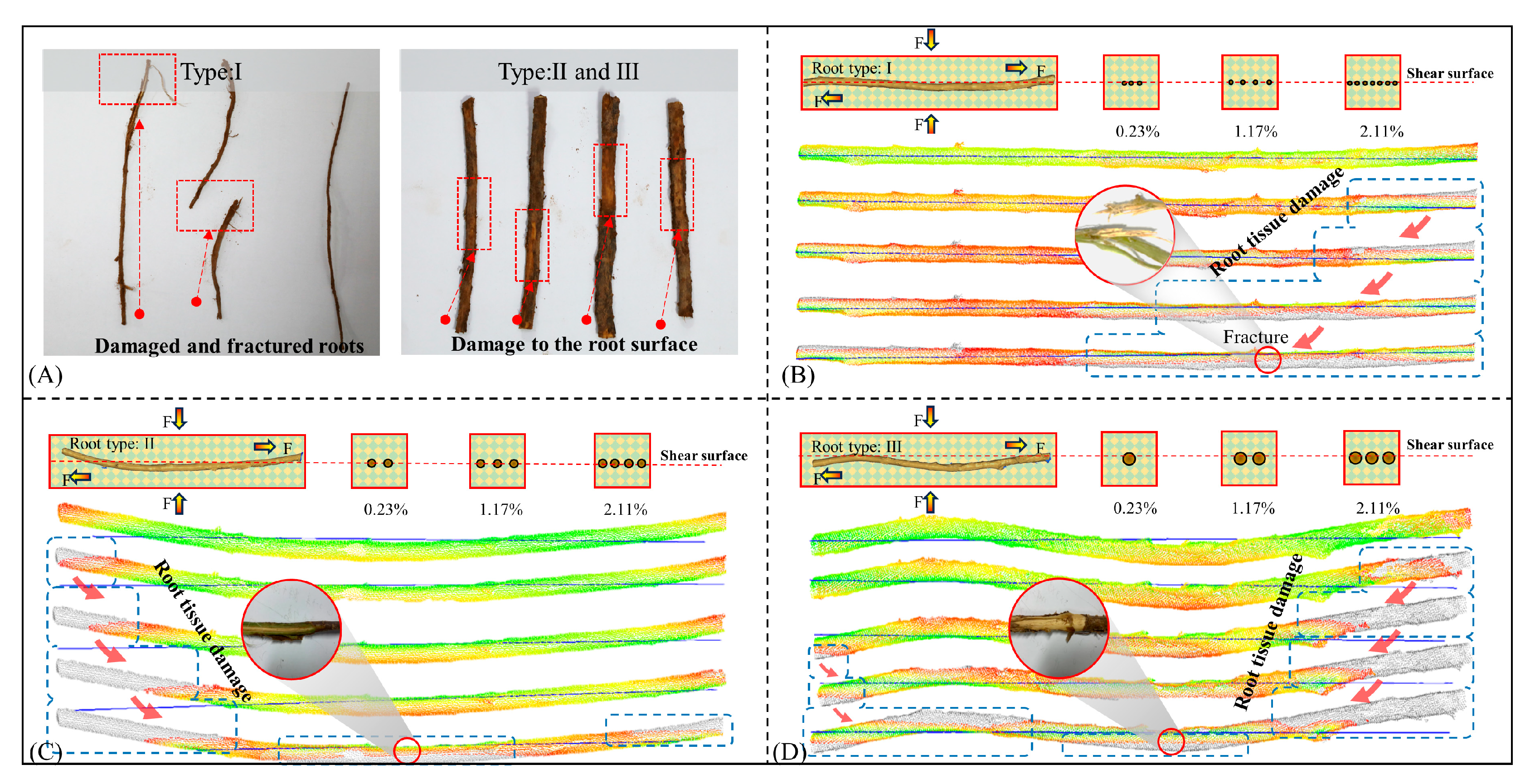
3.1.2. Macroscopic Characterization of Root Damage in Root–Loess Shear Damage Surfaces
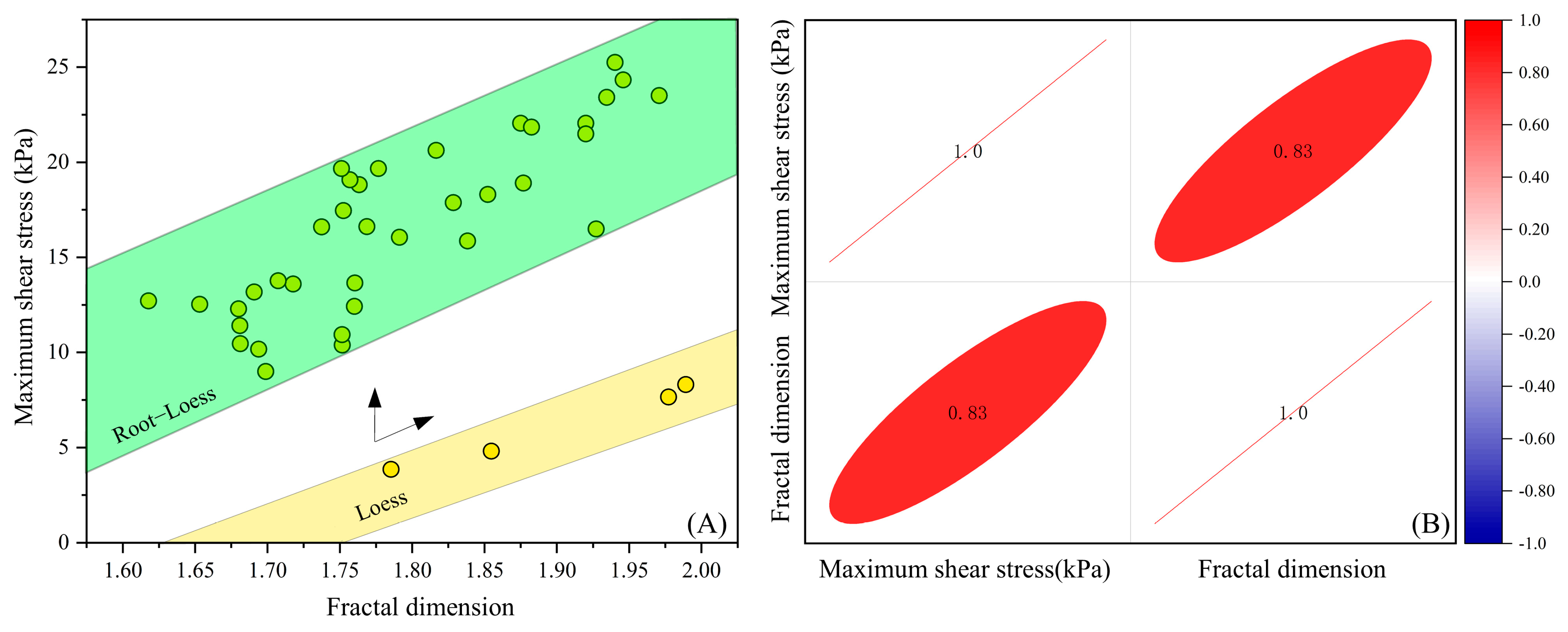
3.1.3. Roughness Characteristics of Root–Loess Shear Damage Surfaces
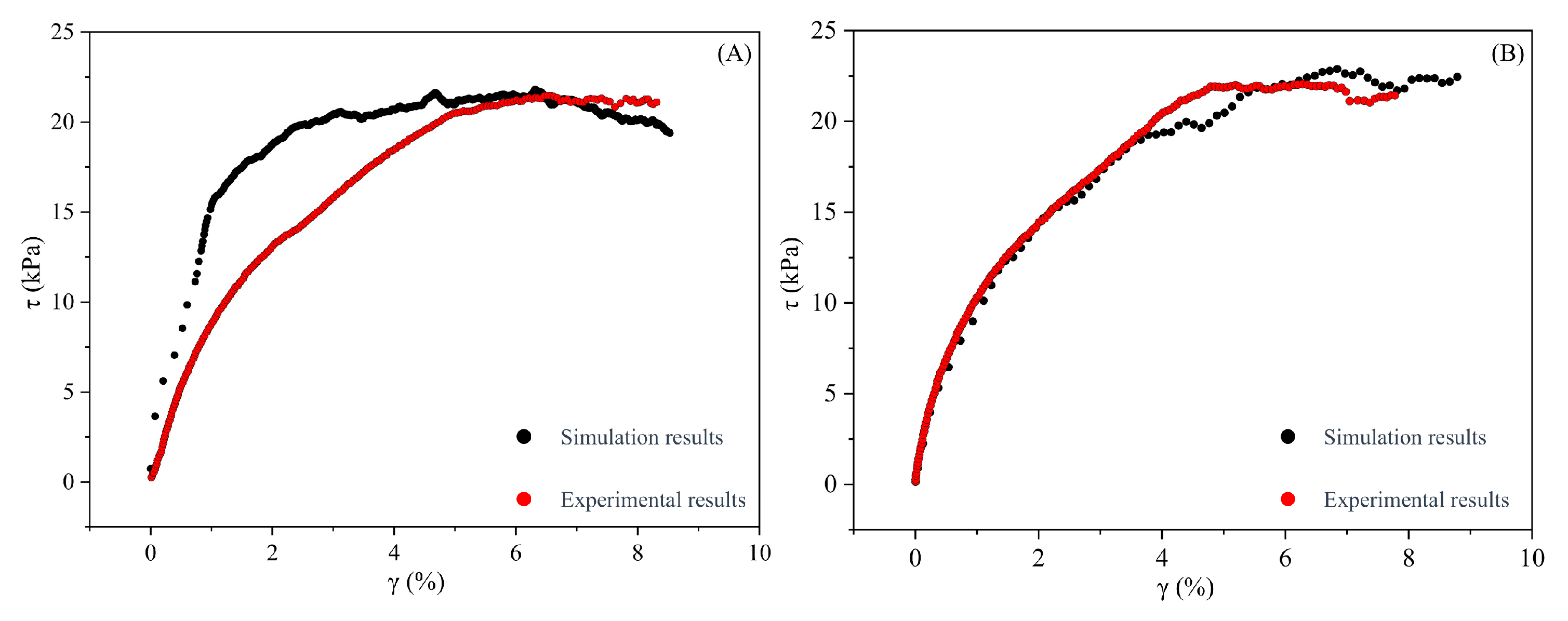
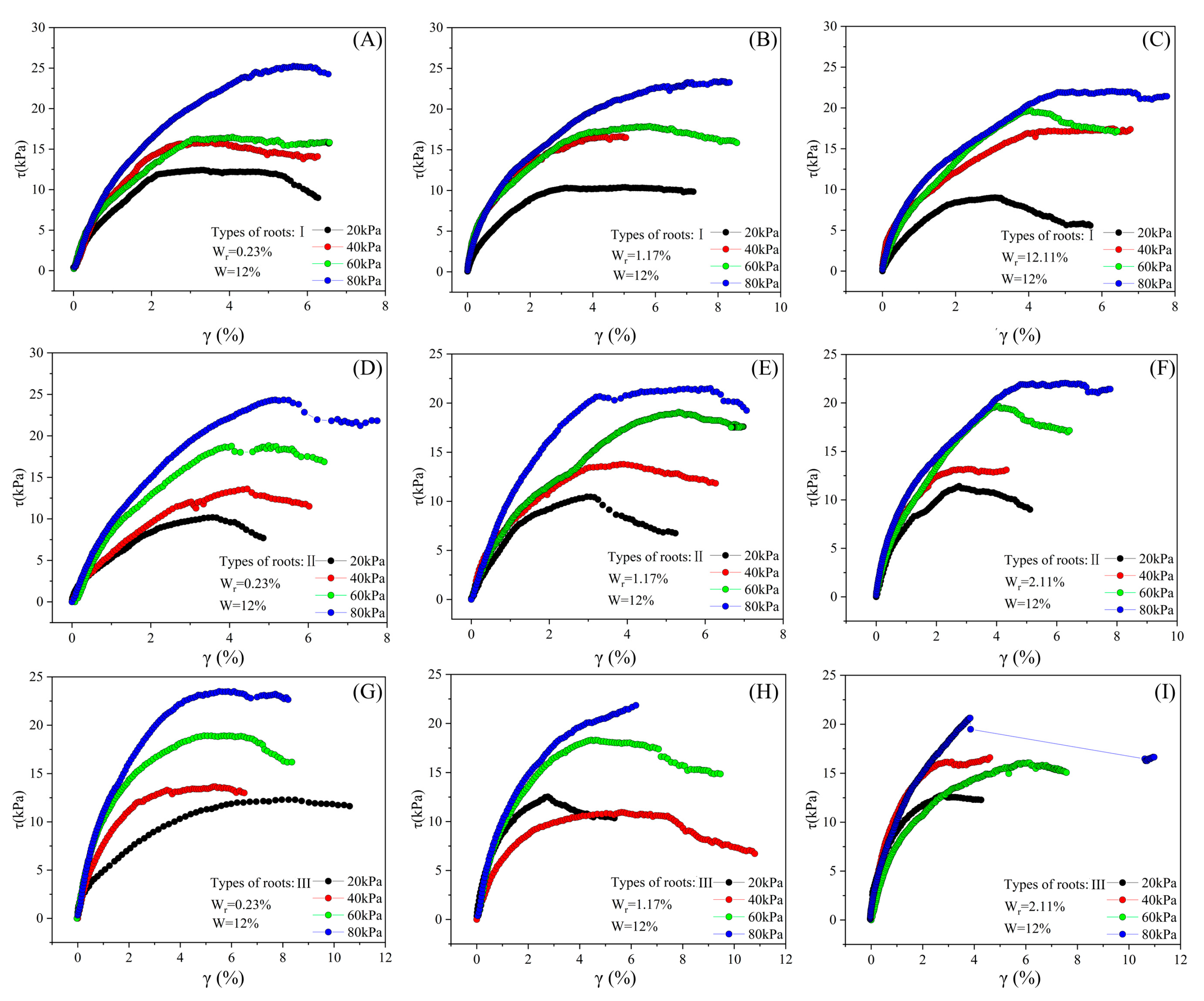
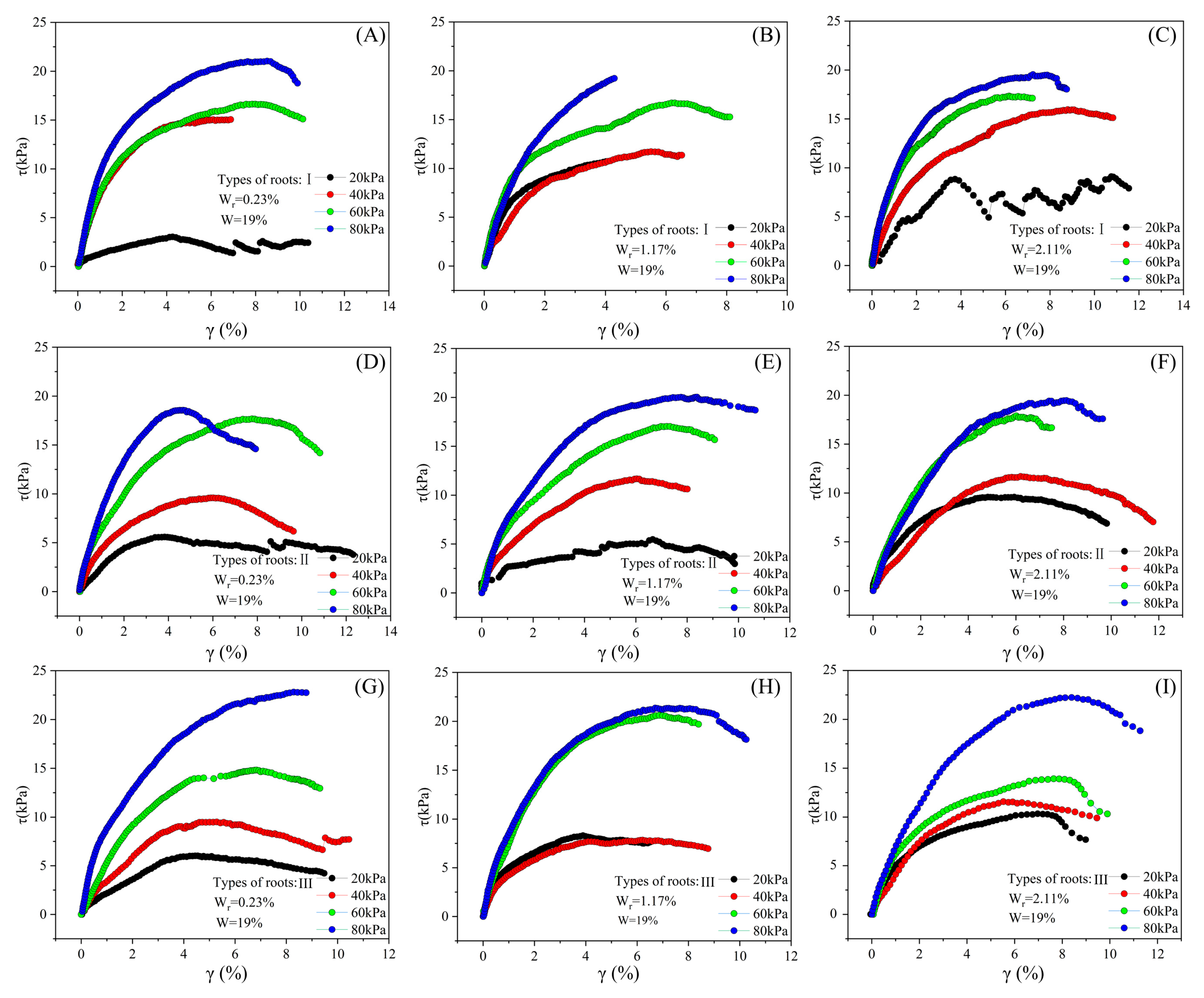
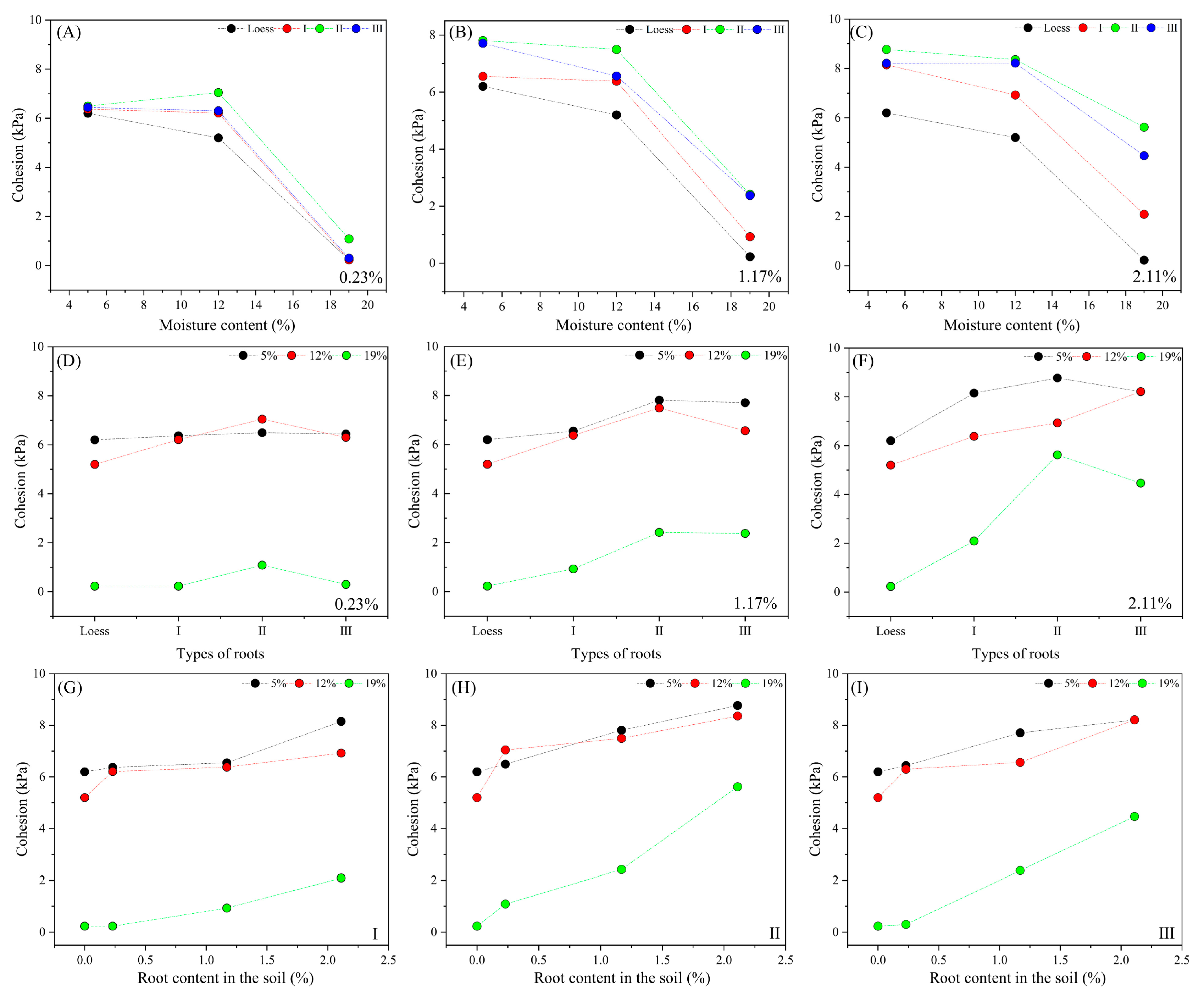
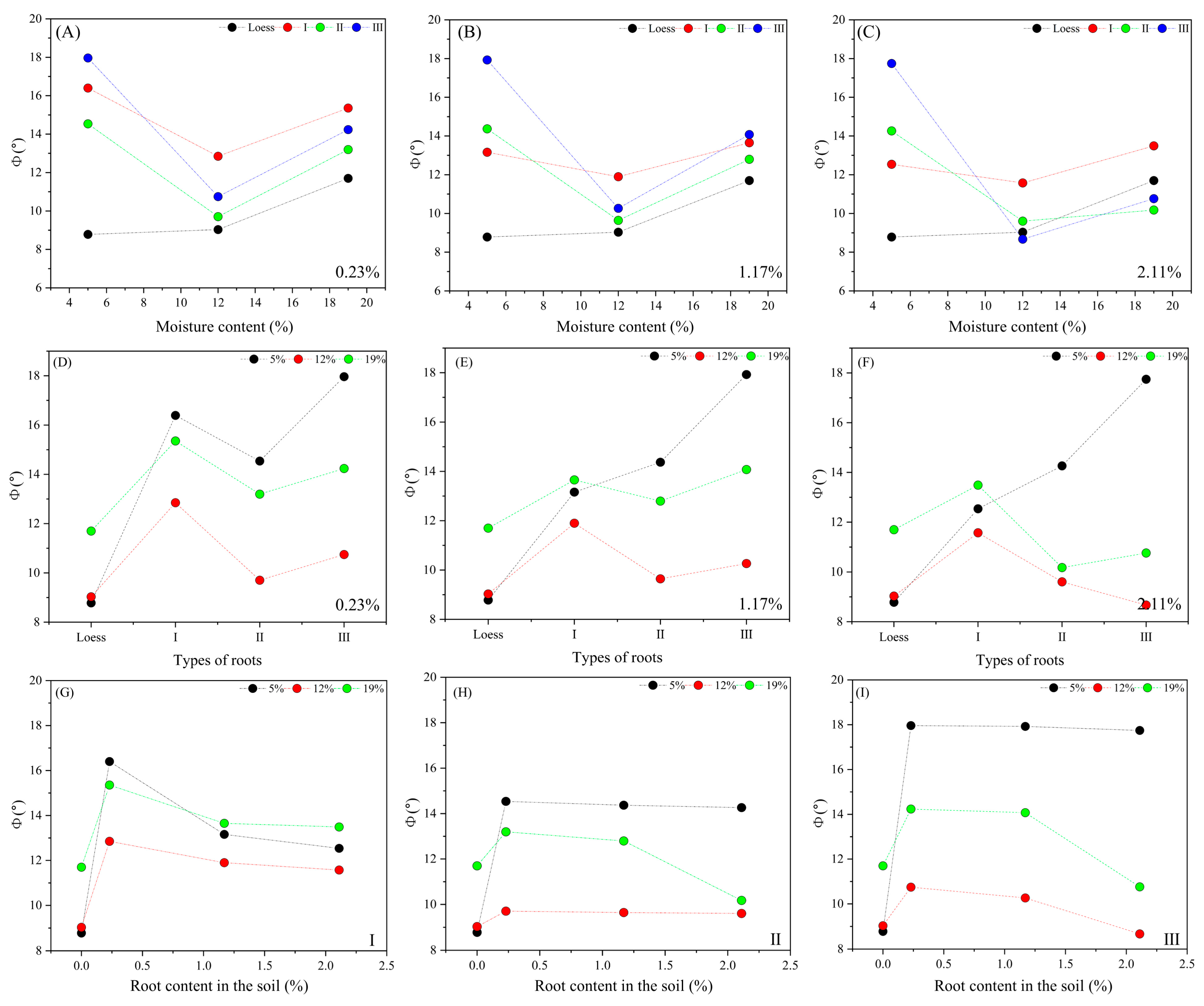
3.2. Macroscopic Mechanical Properties of the Root–Loess Interface
Variation Characteristics of Shear Strength Indicators at the Root–Loess Interface
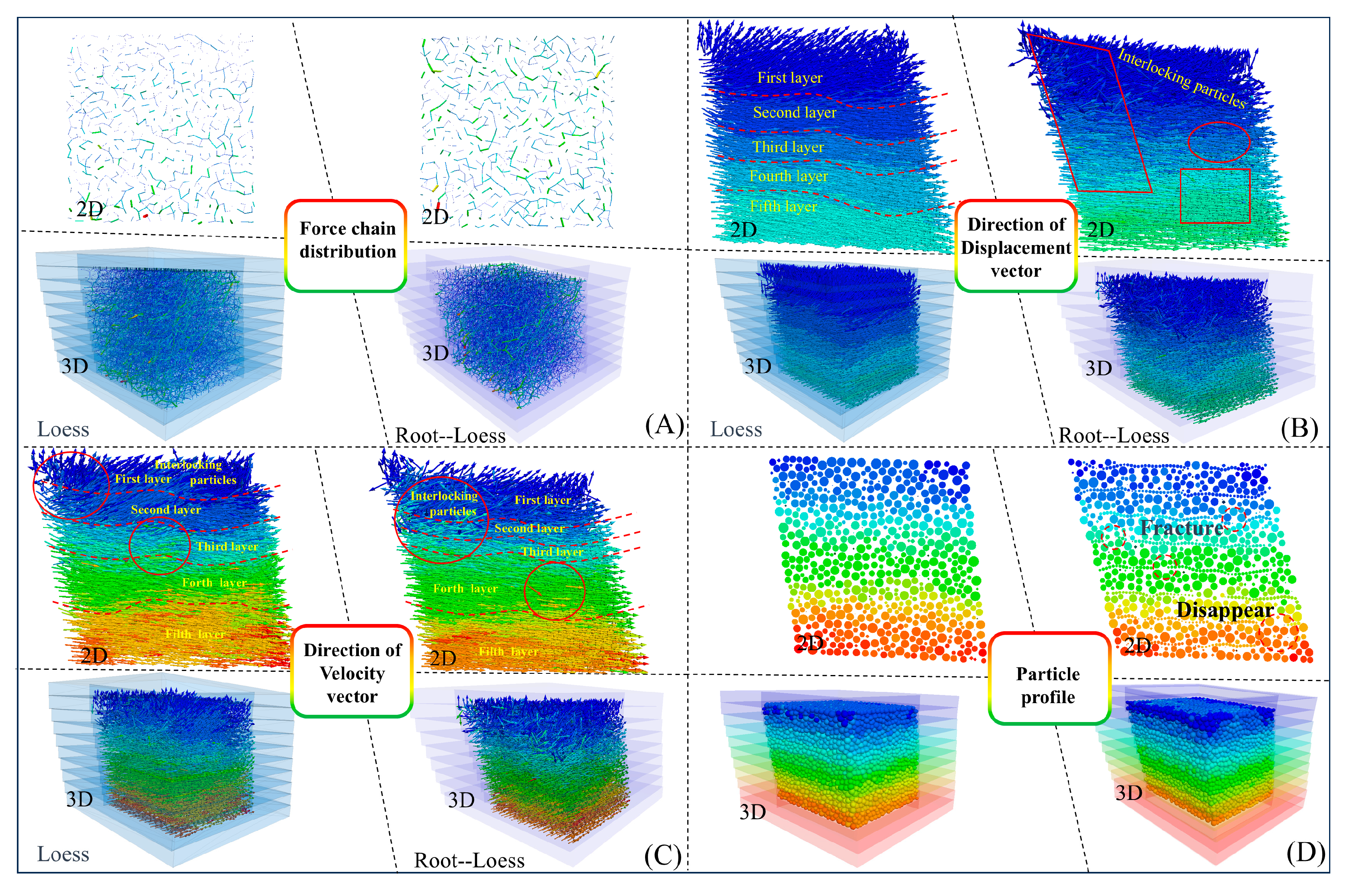
3.3. Micromechanical Properties of the Root–Loess Interface
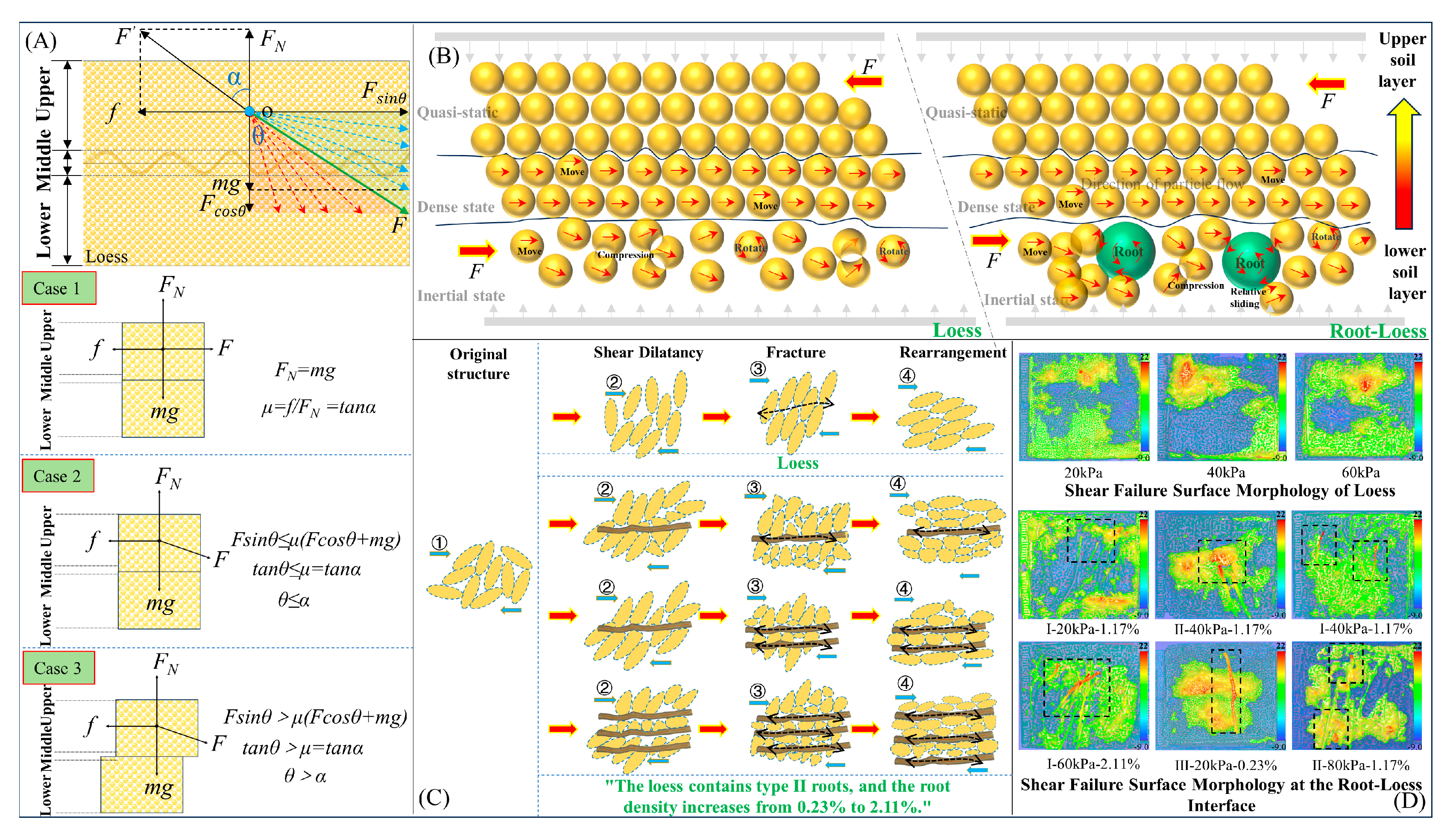
3.4. Physical Mechanism of Shear Behavior at the Root–Loess Interface
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Jin, Z.; Peng, J.; Zhuang, J.; Feng, L.; Huo, A.; Mu, X.; Wang, W. Gully erosion and expansion mechanisms in loess tablelands and the scientific basis of gully consolidation and tableland protection. Sci. China Earth Sci. 2023, 66, 821–839. [Google Scholar] [CrossRef]
- Li, J.; Wang, X.; Jia, H.; Liu, Y.; Zhao, Y.; Shi, C.; Zhang, F. Effect of herbaceous plant root density on slope stability in a shallow landslide-prone area. Nat. Hazards 2022, 112, 2337–2360. [Google Scholar] [CrossRef]
- Xiao, T.; Li, P.; Fei, W.; Wang, J. Effects of vegetation roots on the structure and hydraulic properties of soils: A perspective review. Sci. Total Environ. 2024, 906, 167524. [Google Scholar] [CrossRef] [PubMed]
- Zhang, F.; Shu, H.; Yan, B.; Wu, X.; Lan, H.; Peng, J. Characteristic analysis and potential hazard assessment of reclaimed mountainous areas in Lanzhou, China. Catena 2023, 221, 106771. [Google Scholar] [CrossRef]
- Feng, L.; Zhang, M.; Jin, Z.; Zhang, S.; Sun, P.; Gu, T.; Liu, X.; Lin, H.; An, Z.; Peng, J.; et al. The genesis, development, and evolution of original vertical joints in loess. Earth Sci. Rev. 2021, 214, 103526. [Google Scholar] [CrossRef]
- Xu, L.; Dai, F.; Tu, X.; Tham, L.G.; Zhou, Y.; Iqbal, J. Landslides in a loess platform, North–West China. Landslides 2014, 11, 993–1005. [Google Scholar] [CrossRef]
- Bellugi, D.G.; Milledge, D.G.; Cuffey, K.M.; Dietrich, W.E.; Larsen, L.G. Controls on the size distributions of shallow landslides. Proc. Natl. Acad. Sci. USA 2021, 118, e2021855118. [Google Scholar] [CrossRef]
- Khan, Y.A.; Lateh, H. Failure Mechanism of a Shallow Landslide at Tun–Sardon Road Cut Section of Penang Island, Malaysia. Geotech. Geol. Eng. 2011, 29, 1063–1072. [Google Scholar] [CrossRef]
- Li, L.; Lan, H.; Strom, A.; Macciotta, R. Landslide length, width, and aspect ratio: Path–dependent measurement and a revisit of nomenclature. Landslides 2022, 19, 3009–3029. [Google Scholar] [CrossRef]
- Chen, H.X.; Zhang, L.M.; Gao, L.; Zhu, H.; Zhang, S. Presenting regional shallow landslide movement on three-dimensional digital terrain. Eng. Geol. 2015, 195, 122–134. [Google Scholar] [CrossRef]
- Sun, P.; Wang, H.; Wang, G.; Li, R.; Zhang, Z.; Huo, X. Field model experiments and numerical analysis of rainfall-induced shallow loess landslides. Eng. Geol. 2021, 295, 106411. [Google Scholar] [CrossRef]
- Fan, C.-C. A displacement–based model for estimating the shear resistance of root–permeated soils. Plant Soil 2012, 355, 103–119. [Google Scholar] [CrossRef]
- Forster, M.; Ugarte, C.; Lamandé, M.; Faucon, M.-P. Root traits of crop species contributing to soil shear strength. Geoderma 2022, 409, 115642. [Google Scholar] [CrossRef]
- Ji, X.; Cong, X.; Dai, X.; Zhang, A.; Chen, L. Studying the mechanical properties of the soil–root interface using the pullout test method. J. Mt. Sci. 2018, 15, 882–893. [Google Scholar] [CrossRef]
- Schwarz, M.; Preti, F.; Giadrossich, F.; Lehmann, P.; Or, D. Quantifying the role of vegetation in slope stability: A case study in Tuscany (Italy). Ecol. Eng. 2010, 36, 285–291. [Google Scholar] [CrossRef]
- Jiang, R.; Xie, J.; Zhao, Y.; He, H.; He, G. Spatiotemporal variability of extreme precipitation in shaanxi province under climate change. Theor. Appl. Climatol. 2017, 130, 831–845. [Google Scholar] [CrossRef]
- Genet, M.; Stokes, A.; Salin, F.; Mickovski, S.B.; Fourcaud, T.; Dumail, J.-F.; van Beek, R. The Influence of Cellulose Content on Tensile Strength in Tree Roots. Plant Soil 2005, 278, 1–9. [Google Scholar] [CrossRef]
- Ghestem, M.; Veylon, G.; Bernard, A.; Vanel, Q.; Stokes, A. Influence of plant roots morphology and architectural traits on soil shear resistance. Plant Soil 2014, 377, 43–61. [Google Scholar] [CrossRef]
- Liu, J.; Zhou, Z.; Liu, J.; Su, X. Effects of root density on soil detachment capacity by overland flow during one growing season. J. Soils Sediments 2022, 22, 1500–1510. [Google Scholar] [CrossRef]
- Tanaka, N.; Samarakoon, M.B.; Yagisawa, J. Effects of root architecture, physical tree characteristics, and soil shear strength on maximum resistive bending moment for overturning Salix babylonica and Juglans ailanthifolia. Landsc. Ecol. Eng. 2012, 8, 69–79. [Google Scholar] [CrossRef]
- Mickovski, S.B.; Hallett, P.D.; Bransby, M.F.; Davies, M.C.R.; Sonnenberg, R.; Bengough, A.G. Mechanical reinforcement of soil by willow roots: Impacts of root properties and root failure mechanism. Soil Sci. Soc. Am. J. 2009, 73, 1276–1285. [Google Scholar] [CrossRef]
- Docker, B.B.; Hubble, T.C.T. Quantifying root-reinforcement of river bank soils by four Australian tree species. Geomorphology 2008, 100, 401–418. [Google Scholar] [CrossRef]
- Nan, J.; Peng, J.; Zhu, F.; Ma, P.; Liu, R.; Leng, Y.; Meng, Z. Shear behavior and microstructural variation in loess from the Yan’an area, China. Eng. Geol. 2021, 280, 105964. [Google Scholar] [CrossRef]
- Yang, Q.C.; Hao, Z.; Cheng, W.; Lei, S.Y.; Zhang, Y.; Teng, D.; Zhang, Q.; Wang, X.M. Study on the cohesive shear characteristics and intrinsic modelling of the root-tailing soil interface of Amorpha fruticosa. Sci. Rep. 2022, 12, 11800. [Google Scholar] [CrossRef]
- Comino, E.; Marengo, P.; Rolli, V. Root reinforcement effect of different grass species: A comparison between experimental and models results. Soil Tillage Res. 2010, 110, 60–68. [Google Scholar] [CrossRef]
- Fan, C.-C.; Su, C.-F. Role of roots in the shear strength of root-reinforced soils with high moisture content. Ecol. Eng. 2008, 33, 157–166. [Google Scholar] [CrossRef]
- Thomas, R.E.; Pollen-Bankhead, N. Modeling root-reinforcement with a fiber-bundle model and Monte Carlo simulation. Ecol. Eng. 2010, 36, 47–61. [Google Scholar] [CrossRef]
- Wu, T.H. Root reinforcement of soil: Review of analytical models, test results, and applications to design. Can. Geotech. J. 2013, 50, 259–274. [Google Scholar] [CrossRef]
- Waghmare, K.D.; Tripathi, K.K. Review on Field Direct Shear Test Methodologies. In Recent Trends in Construction Technology and Management; Lecture Notes in Civil Engineering; Ranadive, M.S., Das, B.B., Mehta, Y.A., Gupta, R., Eds.; Springer Nature: Singapore, 2023; pp. 665–672. [Google Scholar] [CrossRef]
- Jiang, Y.; Alam, M.; Li-Jun, S.; Umar, M.; Sadiq, S.; Jia, L.J.; Rahman, M. Effect of root orientation on the strength characteristics of loess in drained and undrained triaxial tests. Eng. Geol. 2022, 296, 106459. [Google Scholar] [CrossRef]
- GB/T50123; Standard for Soil Test Method. Ministry of Construction: Beijing, China, 1999. (In Chinese)
- ASTM D422-63(2007)e1; Standard Test Method for Particle-Size Analysis of Soils. US Department of Defense: Washington, DC, USA, 2007.
- Pollen, N. Temporal and spatial variability in root reinforcement of streambanks: Accounting for soil shear strength and moisture. Catena 2007, 69, 197–205. [Google Scholar] [CrossRef]
- Schwarz, M.; Cohen, D.; Or, D. Root-soil mechanical interactions during pullout and failure of root bundles. J. Geophys. Res. Earth Surf. 2010, 115, F04035. [Google Scholar] [CrossRef]
- Ari, A.; Akbulut, S. Effect of fractal dimension on sand-geosynthetic interface shear strength. Powder Technol. 2022, 401, 117349. [Google Scholar] [CrossRef]
- Chang, R.; Fu, B.; Liu, G.; Yao, X.; Wang, S. Effects of soil physicochemical properties and stand age on fine root biomass and vertical distribution of plantation forests in the Loess Plateau of China. Ecol. Res. 2012, 27, 827–836. [Google Scholar] [CrossRef]
- Lian, B.; Peng, J.; Zhan, H.; Wang, X. Mechanical response of root-reinforced loess with various water contents. Soil Tillage Res. 2019, 193, 85–94. [Google Scholar] [CrossRef]
- Meng, S.; Zhao, G.; Yang, Y. Impact of Plant Root Morphology on Rooted-Soil Shear Resistance Using Triaxial Testing. Adv. Civ. Eng. 2020, 2020, e8825828. [Google Scholar] [CrossRef]
- Abernethy, B.; Rutherfurd, I.D. The distribution and strength of riparian tree roots in relation to riverbank reinforcement. Hydrol. Process 2001, 15, 63–79. [Google Scholar] [CrossRef]
- Stokes, A.; Sotir, R.; Chen, W.; Ghestem, M. Soil bio- and eco-engineering in China: Past experience and future priorities. Ecol. Eng. 2010, 36, 247–257. [Google Scholar] [CrossRef]
- Booth, A.M.; Hurley, R.; Lamb, M.P.; Andrade, J.E. Force chains as the link between particle and bulk friction angles in granular material. Geophys. Res. Lett. 2014, 41, 8862–8869. [Google Scholar] [CrossRef]
- Huang, Y.; Daniels, K.E. Friction and pressure–dependence of force chain communities in granular materials. Granul. Matter 2016, 18, 85. [Google Scholar] [CrossRef]
- Hunt, G.W.; Tordesillas, A.; Green, S.C.; Shi, J. Force-chain buckling in granular media: A structural mechanics perspective. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2010, 368, 249–262. [Google Scholar] [CrossRef]
- Amundson, J.M.; Burton, J.C. Quasi-Static Granular Flow of Ice Mélange. J. Geophys. Res. Earth Surf. 2018, 123, 2243–2257. [Google Scholar] [CrossRef]
- Canakci, H.; Hamed, M.; Celik, F.; Sidik, W.; Eviz, F. Friction characteristics of organic soil with construction materials. Soils Found. 2016, 56, 965–972. [Google Scholar] [CrossRef]
- Zhang, L.; Sun, J.; Shi, C. Numerical simulation on the influence of plant root morphology on shear strength in the sandy soil, Northwest China. J. Arid. Land 2024, 16, 1444–1462. (In Chinese) [Google Scholar]




| Sample | w (%) | ρ (g/cm3) | ρd (g/cm3) | wL (%) | wp (%) | Ip (%) | Classification |
|---|---|---|---|---|---|---|---|
| Malan loess | 12.50 | 1.69 | 1.30 | 28.14 | 16.82 | 12.32 | Silty clay |
| Indicator Name | Radius Range (mm) | Particle Density (kg/m3) | Porosity n | Linear Stiffness Ratio K* |
|---|---|---|---|---|
| Loess | 5–8 | 2.7 × 103 | 0.55 | 2.50 |
| roots | 5.5 | 1.5 × 103 | 0.70 | 1.50 |
| Indicator Name | Effective modulus /MPa | Coefficient of friction μ | radius multiplier | Normal bond strength /MPa |
| Loess | 33.5 | 0.577 | 1.0 | 0.25 |
| roots | 0.4 | 0.577 | 0.25 | 100 |
| Indicator Name | Friction angle | Bonded stiffness ratio | Linear normal stiffness MPa | Tangential bond strength /MPa |
| Loess | 33.5 | 2.50 | / | 0.1 |
| roots | / | 1.50 | 0.5 | 100 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gu, Q.; Hong, B.; Huang, Q.; Kang, X.; Zhang, D.; Guo, X.; Liu, G.; Xiao, T. Macro–Microscale Research on the Single Shear Characteristics of the Root–Loess Interface in Robinia pseudoacacia. Agronomy 2025, 15, 847. https://doi.org/10.3390/agronomy15040847
Gu Q, Hong B, Huang Q, Kang X, Zhang D, Guo X, Liu G, Xiao T. Macro–Microscale Research on the Single Shear Characteristics of the Root–Loess Interface in Robinia pseudoacacia. Agronomy. 2025; 15(4):847. https://doi.org/10.3390/agronomy15040847
Chicago/Turabian StyleGu, Qi, Bo Hong, Qiangbing Huang, Xiaosen Kang, Dengfei Zhang, Xiaopeng Guo, Gang Liu, and Tao Xiao. 2025. "Macro–Microscale Research on the Single Shear Characteristics of the Root–Loess Interface in Robinia pseudoacacia" Agronomy 15, no. 4: 847. https://doi.org/10.3390/agronomy15040847
APA StyleGu, Q., Hong, B., Huang, Q., Kang, X., Zhang, D., Guo, X., Liu, G., & Xiao, T. (2025). Macro–Microscale Research on the Single Shear Characteristics of the Root–Loess Interface in Robinia pseudoacacia. Agronomy, 15(4), 847. https://doi.org/10.3390/agronomy15040847






