Recent Advances in the Hydrogen Gas Barrier Performance of Polymer Liners and Composites for Type IV Hydrogen Storage Tanks: Fabrication, Properties, and Molecular Modeling
Abstract
:1. Introduction
2. Fabrication Processes and Properties
2.1. Common Polymers Used (HDPE, PA6, PA11, EVOH)
- -
- Mechanical properties: The addition of EVOH significantly enhanced the tensile strength of the blends. Particularly, adding 10 wt% EVOH led to a 159% increase in the tensile strength for the optimized composition (70 wt% PA6 and 30 wt% EVOH, referred to as P7E3). However, the elongation at break decreased with increasing EVOH content, particularly beyond 50 wt%, owing to the semicrystalline nature of EVOH. Additionally, incorporating EPDM rubber into the optimized P7E3 blend improved the elongation at break, thereby enhancing processability, which is essential for applications such as hydrogen tank liners.
- -
- Morphological characteristics: Morphological characterization was conducted to examine the miscibility and structural characteristics of the ternary polymer blends, as depicted in Figure 3. To obtain precise insights into the morphology of each polymer within the blends, etching was performed for 4 h using dimethylformamide for the P7E3 blend and xylene for the P7E3R blend. As shown in Figure 3a,d neat PA6 exhibited a distorted surface with noticeable pores resulting from the intrinsic moisture in the material. Conversely, the PA6/EVOH blend exhibited a significant reduction in porosity, suggesting enhanced compatibility between the two polymers (Figure 3b,e). Additionally, the ternary blends revealed a distinct core–shell structure owing to the differing hydrophilic and hydrophobic properties of the components, as illustrated in Figure 3c,f. The hydrophilic EVOH pellets effectively coated the EPDM rubber particles, contributing to the improved miscibility and elongation at break. This configuration minimized the interfacial area between the matrix and rubber, which possess inherently different characteristics, thereby enhancing the mechanical properties of the composite.
- -
- Thermal behavior: DSC analysis indicated that the ternary blends exhibited single melting (Tm) and crystallization (Tc) temperatures, reflecting good miscibility among the polymers. The presence of EPDM affected the crystallization process, as evidenced by the reduction in the melting and crystallization enthalpies with its addition.
- -
- Rheological properties: Rheological tests indicated that the addition of EPDM rubber particles slightly increased the viscosity of the blends, enhancing their solid-like characteristics necessary for maintaining the structure during injection molding.
- -
- Gas barrier performance: The developed ternary polymer blends exhibited superior mechanical and hydrogen gas barrier properties compared to a commercial reference material, indicating their suitability for use as hydrogen tank liners (Figure 3g). This improvement indicates the potential for material thickness and weight reductions in liner applications.
- -
- Mechanism illustration: The enhanced gas barrier properties of the PA6/EVOH/EPDM ternary blends are attributed to several key structural features (Figure 3h). The semi-crystalline arrangement of the PA6/EVOH matrix contributed to a longer tortuous path for gas diffusion, effectively reducing its permeability. Moreover, the strong inter- and intramolecular interactions formed by the hydroxyl groups in EVOH enhanced the gas barrier performance of the material by restricting polymer chain movement. Additionally, the incorporation of EPDM rubber, which is characterized by its cold resistance, benefits the overall integrity and impact strength of the blend. EVOH also played a critical role in coating the EPDM particles, improving its miscibility and overall performance, and making the ternary blend system a promising candidate for hydrogen tank liner applications owing to its superior mechanical and gas barrier characteristics.
2.2. Polymer Composites and Nanocomposites
3. Molecular Insights into Hydrogen Transport and Permeability
3.1. Analysis of Factors Affecting Barrier Properties Using MD Simulations
3.1.1. Temperature and Pressure
- -
- Tg: As shown in Figure 7a, the Tg values of HDPE and EVOH are 226.08 and 319.42 K, respectively.
- -
- Solubility coefficient: Figure 7b illustrates the solubility coefficients of hydrogen in HDPE and EVOH at 2.5 MPa and 30 °C. The hydrogen solubility in HDPE was significantly higher than that in EVOH. As the temperature increased from 30 to 80 °C, both materials exhibited increased solubility coefficients, with that of HDPE rising by 18.7% and EVOH by 15.9%, demonstrating a “reverse dissolution” behavior contrary to typical gas solubility trends. The solubility coefficients also showed different responses to pressure; that of HDPE initially decreased from 6.8 × 10−8 to 5.8 × 10−8 cm3 (STP)/(cm3·Pa), then slightly increased to 6.3 × 10−8 cm3 (STP)/(cm3·Pa). The solubility coefficient of EVOH increased from 4.4 × 10−8 cm3 (STP)/(cm3 Pa) to a peak of 5.0 × 10−8 cm3 (STP)/(cm3·Pa) at 6 MPa before decreasing to 4.1 × 10−8 cm3 (STP)/(cm3·Pa) at 10 MPa. As the temperature increased, the isosteric heat of adsorption increased, resulting in greater molecular motion and enhanced adsorption capacity (Figure 7c). Similarly, as the pressure increased, the isosteric heat in HDPE and EVOH aligned with the changes in the dissolution coefficient. Although the isosteric heat of H2 adsorption was nearly identical at 6 MPa, the solubility coefficients differed significantly because of factors such as entropy changes during solvation and interactions between the polymer and gas molecules. The nonpolar nature of hydrogen limited its interaction with the polymer chain, whereas the hydroxyl groups of EVOH formed hydrogen bonds that increased cohesion, thus restricting the polymer segment mobility and H2 permeation through the matrix.
- -
- Diffusion coefficient: The calculated diffusion coefficients of H2 in HDPE and EVOH increased with increasing temperature and pressure, as illustrated in Figure 7d. When the temperature increased from 30 to 80 °C, the H2 diffusion coefficients in HDPE and EVOH increased by 92.9% and 81.6%, respectively. However, pressure had a negligible effect on the hydrogen diffusion coefficient, which remained consistent beyond a threshold of 4 MPa. The free volume fraction, indicating the unoccupied space within a polymer, increases with increasing temperature owing to polymer expansion, which enhances the diffusion coefficient for hydrogen (Figure 7e). Higher temperatures also improve the thermal motion of gas molecules, facilitating diffusion. Conversely, pressure changes had a negligible effect on the free volume fraction and diffusion coefficient. Notably, the diffusion coefficient of hydrogen in HDPE is approximately twice that in EVOH, primarily because the hydroxyl groups of EVOH lead to stronger hydrogen bonding and a reduced free volume, which hinders diffusion.
- -
- Permeability coefficient: The permeability coefficient of H2 was higher in HDPE than in EVOH, reflecting similar trends in the diffusion and solubility coefficients (Figure 7f). Both materials showed significant increases in permeability upon increasing the temperature from 30 to 80 °C; the permeability of HDPE and EVOH increased by 129.0% and 112.7%, respectively. Pressure had a negligible effect on permeability, with variations from 2.5 to 10 MPa resulting in changes of only 3.7% and 7.5% for HDPE and EVOH, respectively. In low-pressure environments, the gas permeability is primarily dependent on temperature rather than pressure or gas concentration.
- -
- Permeability mechanism: The mechanisms by which H2 permeates through HDPE and EVOH share common features, including an aggregation adsorption process that occurs in the low-potential energy regions and a diffusion process characterized by transitions between holes driven by vibrational motion (Figure 8). During the adsorption phase, H2 molecules predominantly accumulate in low-potential energy areas, with EVOH displaying a particular affinity for regions devoid of hydrogen bonds. In the subsequent diffusion phase, H2 molecules vibrate within a specific hole before moving to another hole, where they continue to vibrate. Higher temperatures result in an enhanced range of motion and increased frequency of transitions for H2 molecules.
- -
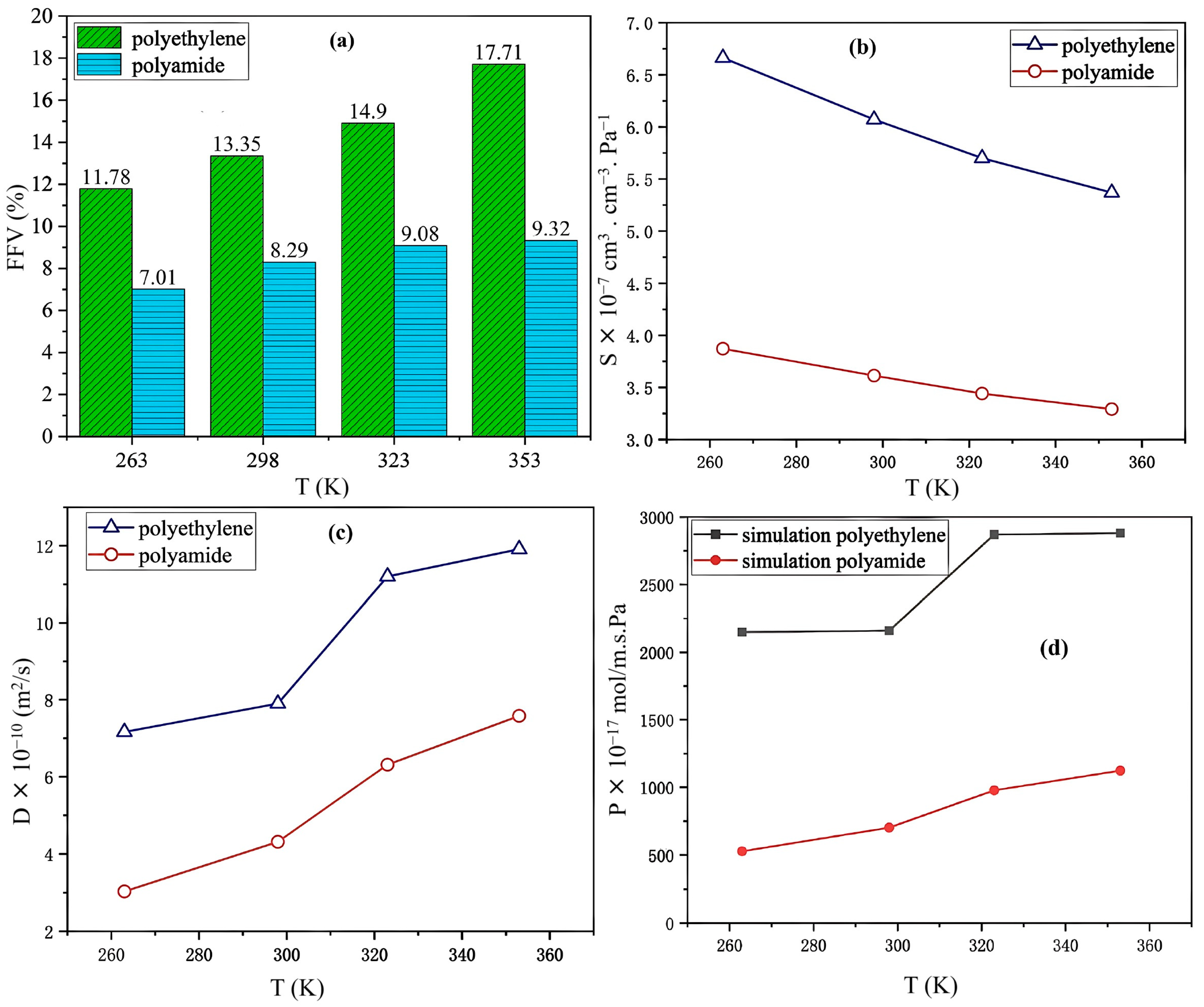
- Free Volume: The histogram shown in Figure 9a illustrates the FFV of PE and PA6 at 263, 298, 323, and 353 K, indicating that the FFV for both materials increased with increasing temperature. Notably, at all temperatures, the FFV of PE consistently exceeded that of PA6. Specifically, the FFV of PE increased from 11.78% at 263 K to 17.71% at 353 K, reflecting an increase of 5.93%. In comparison, the FFV of PA6 increased from 7.07% to 9.322% over the same temperature range, which is a smaller increase of 2.315%. This demonstrated that the FFV of PE was significantly more sensitive to temperature changes than that of PA6.
- -
- Solubility coefficient: The solubility coefficients of hydrogen in PE and PA6 decreased as the temperature increased. Within the temperature range of 263–353 K, PE consistently demonstrated a higher solubility coefficient than PA6 (Figure 9b).
- -
- Diffusion coefficient: The diffusion coefficient showed a positive correlation with FFV, with both parameters increasing as the temperature increased (Figure 9c). As the temperature increased, the free volume of the system expanded, thereby increasing the effective diffusion space available for H2 molecules. This heightened thermal motion of the molecular chains in PE and PA6, along with the H2 molecules, enhanced the likelihood of diffusion, which, in turn, increased the diffusion coefficient.
- -
- Permeability coefficient: Compared to PE, PA6 exhibited superior resistance to hydrogen and better gas permeability, with its ability to prevent hydrogen gas permeation improving by three to four times. Additionally, the permeability coefficient of PE was more significantly influenced by temperature changes than that of PA6 (Figure 9d).
- -
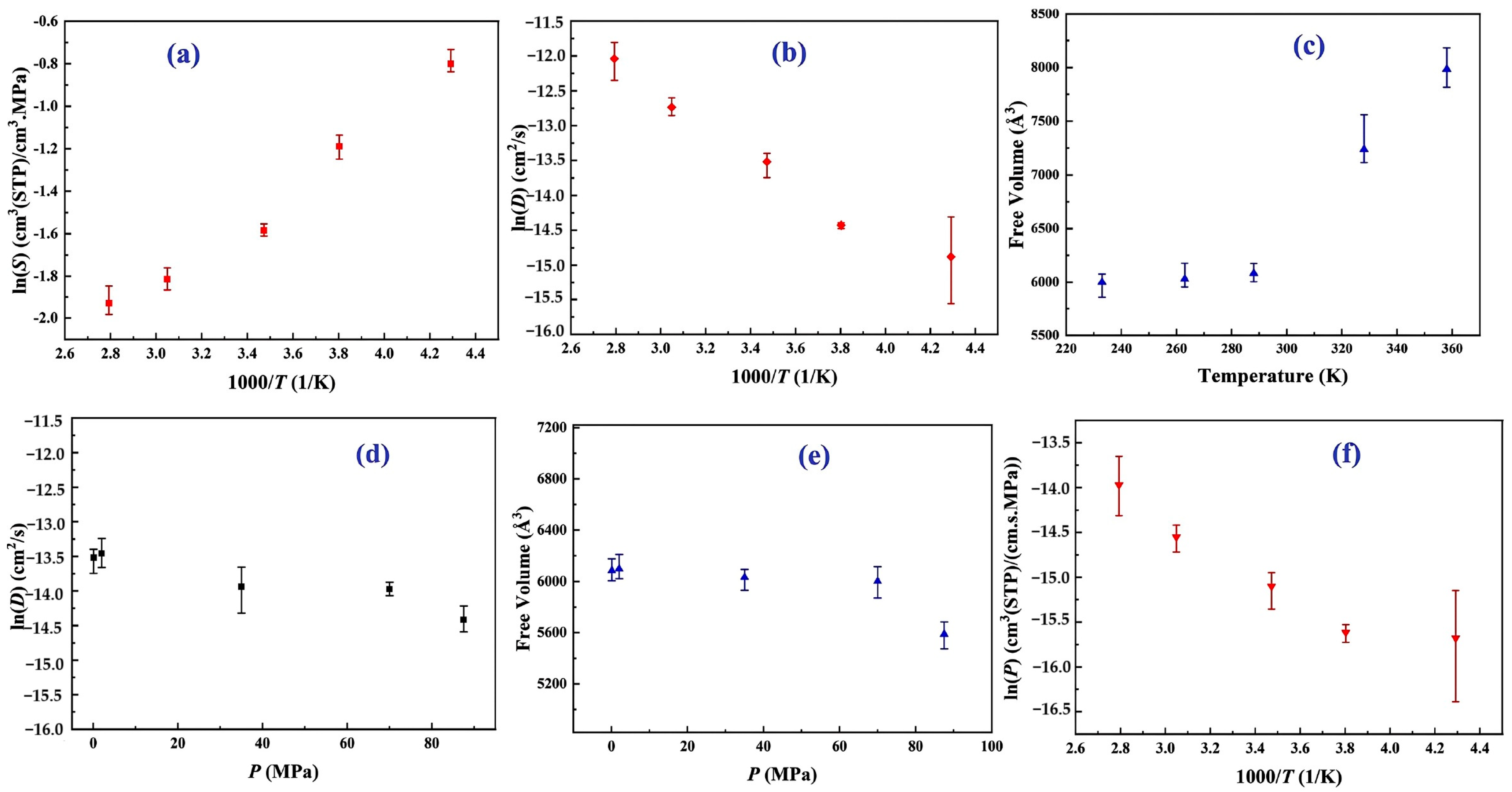
- The solubility coefficients of H2 in PA6, with 30.00% crystallinity, exhibit Henry-type behavior that is distinct from gases with higher solubilities, such as CO2 [33]. Temperature negatively affects the solubility coefficient of H2 in PA6; as temperature increases, the thermal motion of the gas and polymer molecules rises, making gas adsorption more difficult. Consequently, this leads to a gradual increase in the impact of temperature on the solubility coefficient, resulting in a slower rate of change, as illustrated in Figure 10a.
- -
- The results revealed a positive correlation between the temperature and the diffusion coefficient of H2 in PA6, which was attributed to the increased kinetic energy that facilitated diffusion within the polymer (Figure 10b). Multiple Arrhenius regions were observed in the diffusion coefficient relative to the temperature for the type IV hydrogen tanks. Additionally, the study assesses free volume in the polymer using a hard probe approach with a probe radius of 1.445 Å, representing the kinetic size of H2 (Figure 10c). Below the Tg of 323.01 K, the free volume remained nearly constant, whereas above the Tg, it increased significantly, with the free volume at 358 K being 1.33 times higher than that at 233 K. This greater free volume enhanced gas diffusion, which was reflected in the elevated diffusion coefficient.
- -
- The gas diffusion in PA6 is affected by pressure. Five characteristic pressures (0.1, 2.0, 35.0, 70.0, and 87.5 MPa) were analyzed at 288 K (Figure 10d). The findings indicated a slight decrease in the diffusion coefficient as the pressure increased, with the coefficient at 87.5 MPa being only 40.70% of that at 0.1 MPa. This suggests that, although pressure influences gas diffusion, the effect is limited, particularly within a narrow pressure range. Additionally, increased pressure compacted the polymer, resulting in a reduced free volume and more strenuous gas diffusion (Figure 10e), a phenomenon referred to as the hydrostatic effect. The permeability coefficient was slightly reduced at a constant temperature, correlating with the changes in the diffusion and solubility coefficients.
- -
- This study presented the permeability coefficients derived from the solubility and diffusion coefficients at varying temperatures. Although the solubility and diffusion coefficients exhibit opposite trends with increasing temperature, the permeability coefficient increases overall (Figure 10f). This trend aligns with the findings from previous research, indicating that higher temperatures reduce the gas barrier properties of polymers [5]. The effect of temperature on diffusion was notably stronger than that on dissolution, resulting in a permeability coefficient that mirrored the diffusion coefficient trend. The presence of multiple Arrhenius regions suggests challenges in applying Arrhenius’ law to characterize hydrogen gas permeation in type IV tanks.
3.1.2. Polymer Crystallinity and Molecular Chain Structure
3.1.3. Effect of Hydrogen Content
- -
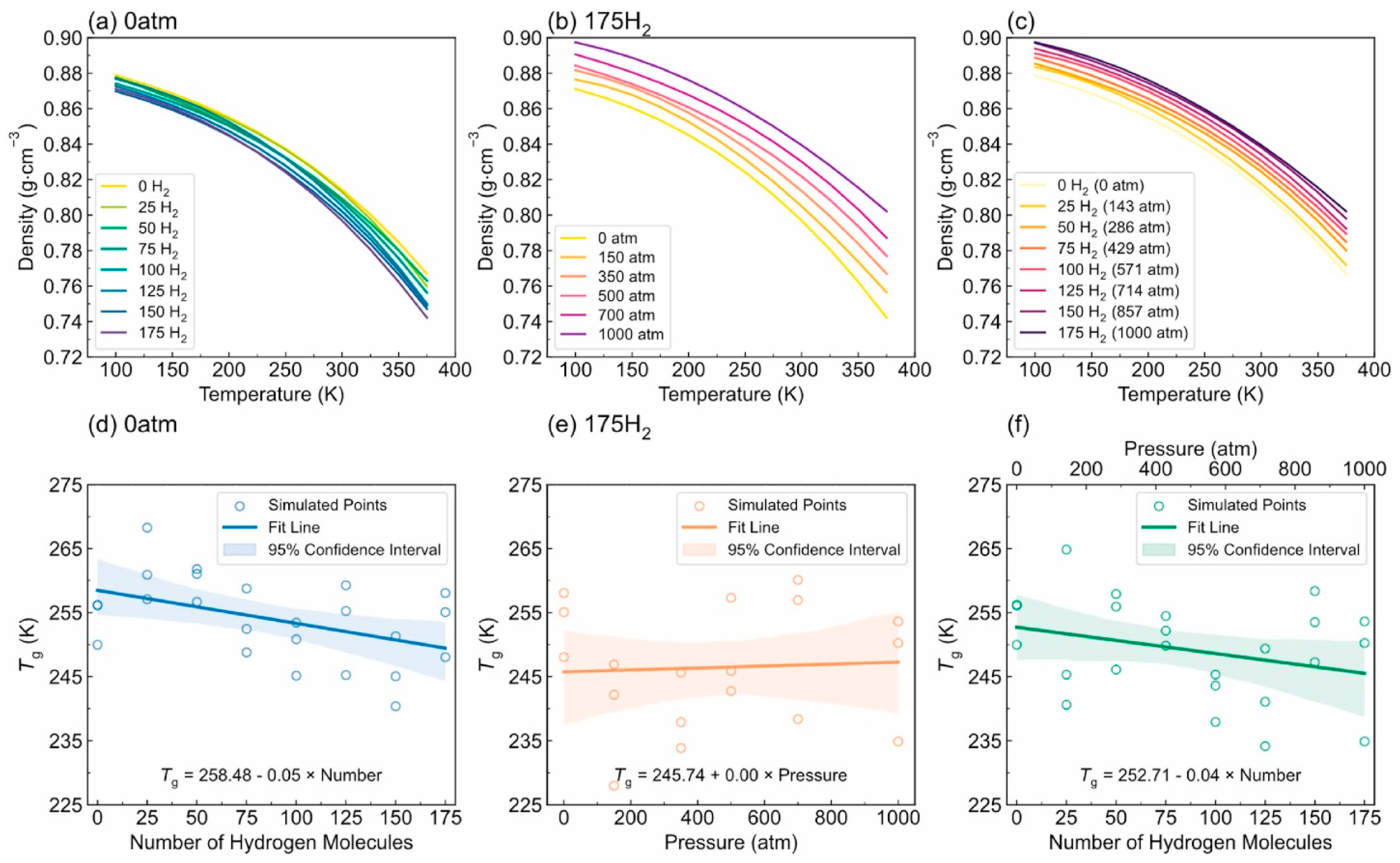
- Tg: Figure 12 illustrates the complex interplay between the hydrogen content, pressure, and the resulting density–temperature characteristics and Tg of the PE systems. As depicted in Figure 12a, increasing the hydrogen content at a constant pressure (0 atm) results in decreased density, contributing to enhanced free volume and segmental mobility of the polymer. Figure 12d shows a corresponding significant reduction in Tg, demonstrating a negative correlation attributed to the plasticizing effect of H2 molecules. Figure 12b reveals that under a constant hydrogen content (175 H2 molecules), increasing the pressure leads to an increase in density but does not significantly affect Tg, as indicated in Figure 12e, where the slope is effectively zero. Furthermore, the coupled effects illustrated in Figure 12c,f show that although the hydrogen content and pressure influence the density and Tg, the primary factor for Tg reduction remains hydrogen, with a slope of 0.04, highlighting that the plasticizing effect of hydrogen outweighs the compressive influence of pressure on Tg, thus underscoring the critical role of hydrogen in modulating the thermal properties of PE in high-pressure environments.
- -
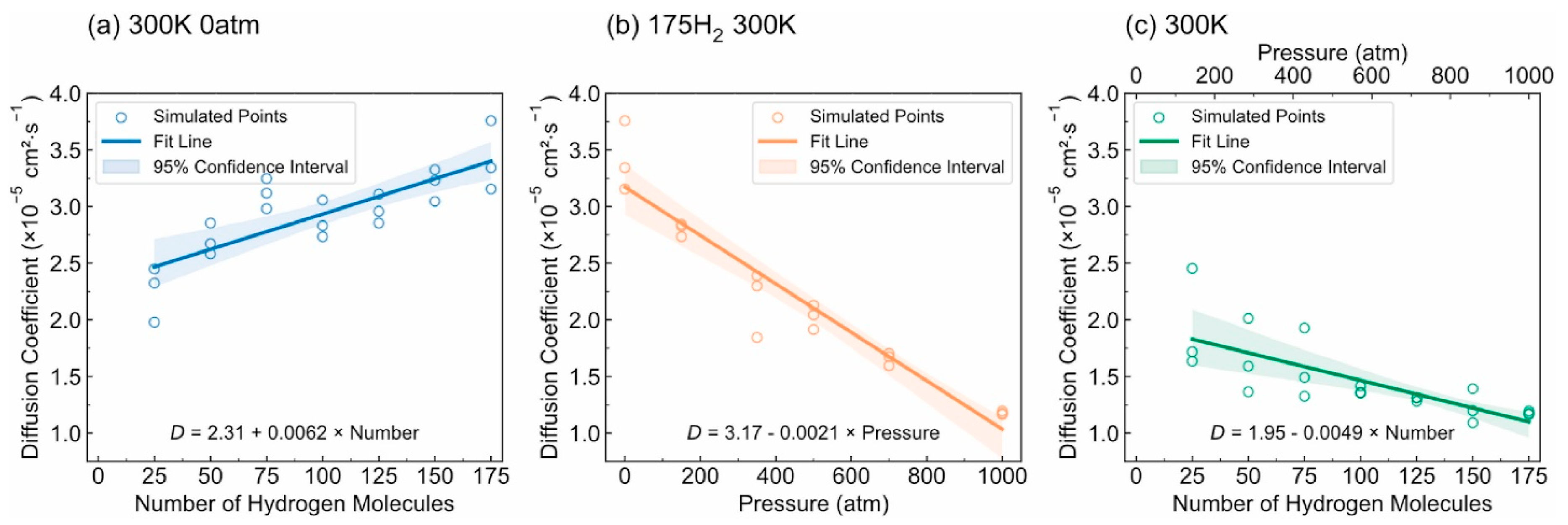
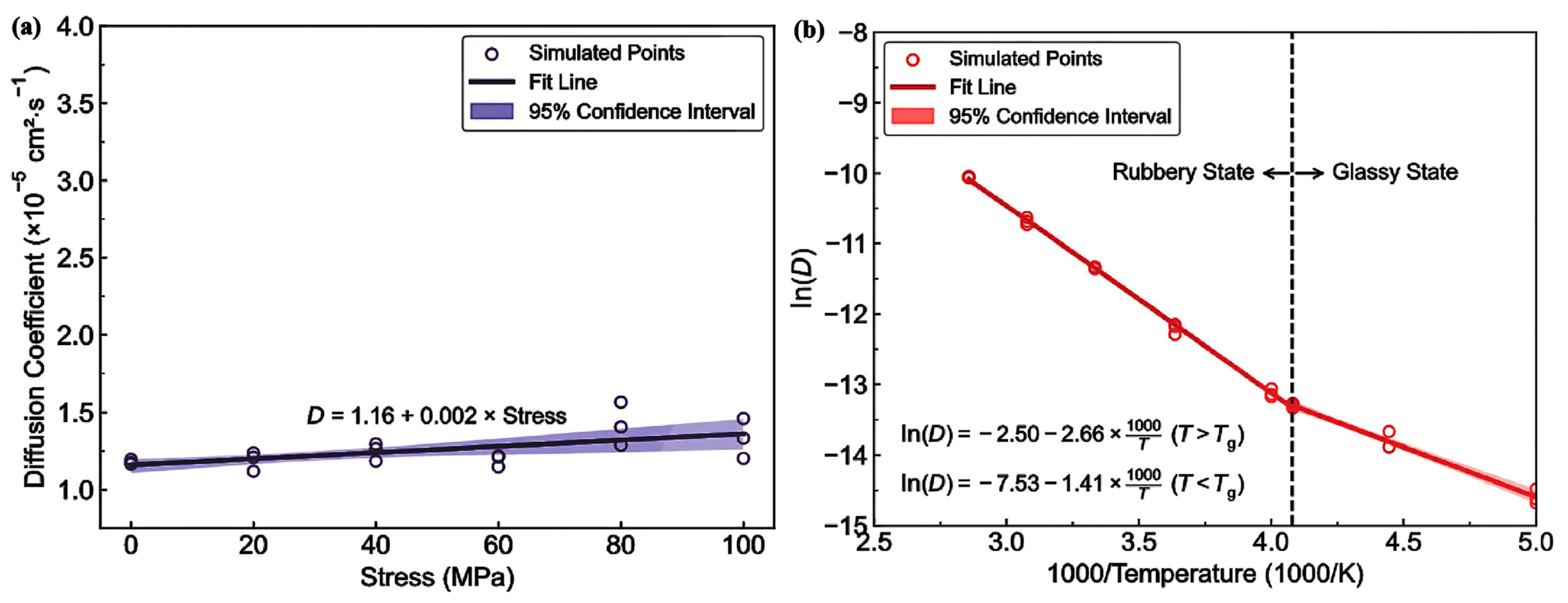
- Diffusivity: The results illustrated in Figure 13 offer important insights into the diffusion characteristics of PE at different hydrogen contents and pressures. Figure 13a reveals a positive relationship between the hydrogen content and diffusion coefficient at 0 atm pressure, suggesting that an increase in the number of H2 molecules enhances the diffusion capacity of PE. This enhancement is attributed to the plasticizing effect, which increases the free volume and improves chain mobility. Conversely, Figure 13b indicates that under a fixed content of 175 H2 molecules, increasing the pressure results in a significant decrease in the diffusion coefficient, with a negative regression coefficient, demonstrating that the applied pressure restricts the free volume, thereby hindering the mobility of H2 molecules within the PE matrix. Furthermore, the coupled effects illustrated in Figure 13c confirm that when the hydrogen content and pressure is increased, the overall diffusion coefficient decreases, indicating that the negative impact of pressure outweighs the positive contribution of the increased hydrogen content, leading to reduced diffusion rates in practical applications. As shown in Figure 14a, under tensile stress conditions at constant temperature (300 K) and pressure (1000 atm), the diffusion coefficients of hydrogen in PE exhibit a nuanced response to tensile stress, suggesting enhanced molecular mobility. Figure 14b reveals that the diffusion coefficients vary with temperature, further emphasizing that higher temperatures facilitate the increased diffusion of H2 molecules within the PE, thus illustrating how tensile stress and thermal conditions significantly affect hydrogen mobility in high-pressure scenarios.
- -
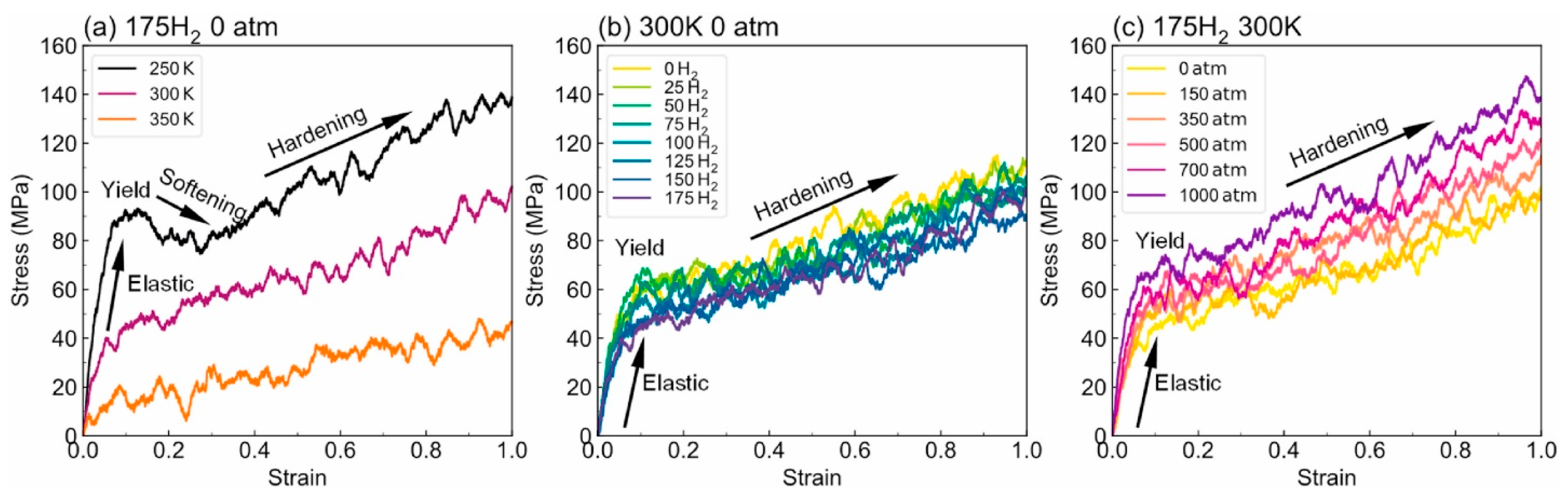
- Tensile properties: The findings from Figure 15 illustrate the complex interplay of temperature, hydrogen content, and pressure on the stress–strain behavior of PE. Figure 15a indicates that as the temperature increases from 250 to 350 K, the stress–strain curve transitions from a more pronounced elastic behavior to a bilinear response, indicating reduced stiffness and strength owing to weakened intermolecular interactions and enhanced chain mobility at elevated temperatures. Figure 15b shows the effects of increasing hydrogen content, where a higher number of H2 molecules correlates with decreased slopes in the elastic region, signifying a reduction in the elastic modulus and yield strength owing to the plasticizing effects of hydrogen that promote chain mobility and reduce intermolecular cohesion. Furthermore, Figure 15c emphasizes that increasing the pressure leads to significant enhancements in the elastic modulus and yield strength, as the tighter packing of the polymer chains and improved intermolecular interactions under high pressure counter the weakening effects of hydrogen. The results presented in Figure 16 further quantify these observations, revealing that the elastic modulus and yield strength of PE decrease linearly with the addition of H2 molecules while increasing markedly with pressure. This indicates a nuanced relationship in which the detrimental effects of hydrogen can be mitigated by the application of pressure, thereby improving the mechanical properties of PE in hydrogen-rich environments.
3.1.4. Effect of High-Pressure Hydrogen Decompression
- -
- The effect of H2 molecules on tensile properties and Tg: The incorporation of H2 into PE significantly affects its tensile properties and Tg. As the hydrogen content increased, density and tensile modulus clearly decreased. The yield stress initially increased with H2 concentrations below 300 molecules but subsequently decreased with higher H2 contents, which was attributed to the poor interaction between H2 molecules and polyolefin chains. H2 molecules do not enhance the mechanical performance of the matrix and act similarly to voids, thereby affecting the tensile properties owing to their mobility and weak interaction with PE. Additionally, the calculated Tg values for PE decreased with increasing H2 content, from 268.0 K for 0 H2 molecules to 263.0 K for 500 H2 molecules. However, obtaining a Tg for PE with 1000 H2 molecules proved challenging owing to abnormal trends, likely resulting from phase separation, emphasizing the complexity of the influence of hydrogen on the properties of the material.
- -
- Influence of polymer structure on the D: Examining degree of polymerization, side chains, and orientation: The diffusion constant of hydrogen in PE does not show a significant correlation with the degree of polymerization (DP), particularly when the chain length is long, which results in fewer end groups that affect the diffusion rates (Figure 17a). Prior studies have suggested a negative correlation between the diffusion rate and DP, but this research infers that beyond a certain DP, other factors such as the molecular structure and processing conditions (including crystallinity and orientation) play more critical roles. Additionally, the diffusion pathways of H2 molecules at different temperatures (200 and 400 K) revealed that at lower temperatures, H2 molecules exhibit limited movement mainly within stable free volume regions (Figure 17b), whereas at higher temperatures, their trajectories become more dispersed and irregular, suggesting that a free volume redistribution occurs as the polymer transitions to a rubbery state, which may deviate from the relationship between the diffusion constant and the mean squared displacement established from the Einstein relation (Figure 17c). Figure 17d illustrates the correlation between the orientation of PE chains and the hydrogen diffusion constant. Increased chain orientation led to a reduction in the diffusion constant for hydrogen within the polymer matrix. This phenomenon can be attributed to the denser and more compact structure created by the oriented chains, which restricts the movement of H2 molecules compared to a system lacking such orientation. As a result, the compact arrangement of the oriented chains presents a barrier that impedes the free diffusion of hydrogen.
- -
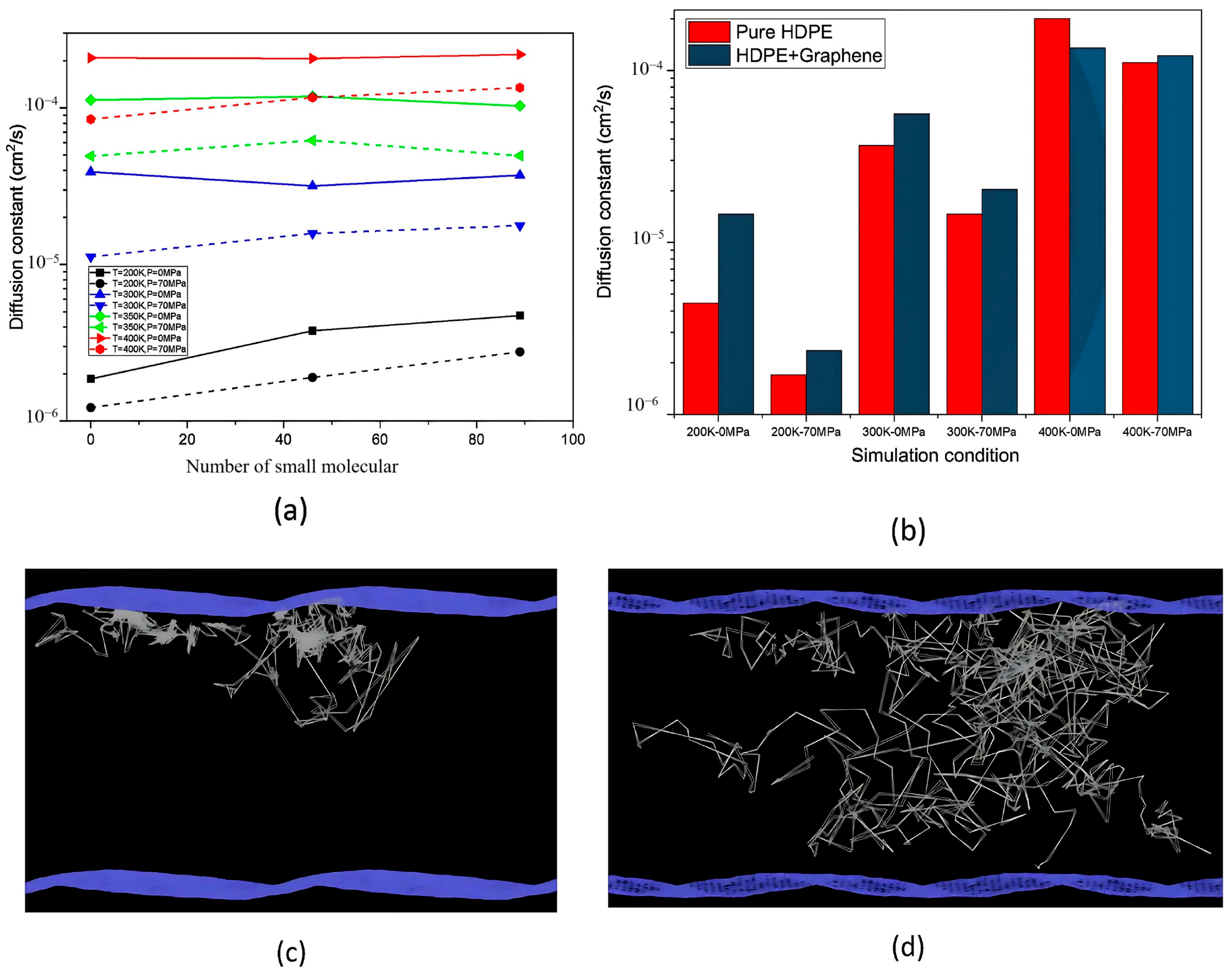
- Influence of additives and reinforcements on hydrogen diffusion in PE: The inclusion of small molecules, specifically C20H42, significantly enhances the H2 diffusion constant in PE systems at various temperatures and pressures (Figure 18a), demonstrating a plasticizing effect that increases chain mobility by creating additional free volume. This trend aligns with previous research conducted on nylon 11 [2]. However, the relationship between diffusion and small molecules becomes less clear in systems operating above Tg, where other factors may also influence the diffusion process. Figure 18b presents a comparison of the hydrogen diffusion constants between the PE system and graphene/PE composite systems, along with the trajectories of the 10 H2 molecules. The presence of the graphene layer leads to an increase in the hydrogen diffusion constant at lower temperatures, whereas at higher temperatures, it decreases. These findings indicate that the diffusion of H2 molecules is enhanced at the interface between graphene and PE, which is attributed to the weak van der Waals interactions between the two materials (Figure 18c). However, the presence of graphene can decrease the diffusion constant, especially at higher temperatures (400 K) (Figure 18d), because it may obstruct H2 molecules and constrain the mobility of nearby polymer chains. Additionally, the redistribution of free volume around graphene is likely limited. The simulations were conducted without a pressure drop; however, H2 is anticipated to diffuse in the direction of the pressure drop, suggesting that to optimize the effectiveness of graphene as a filler, it should be aligned opposite to the pressure drop direction.
- -
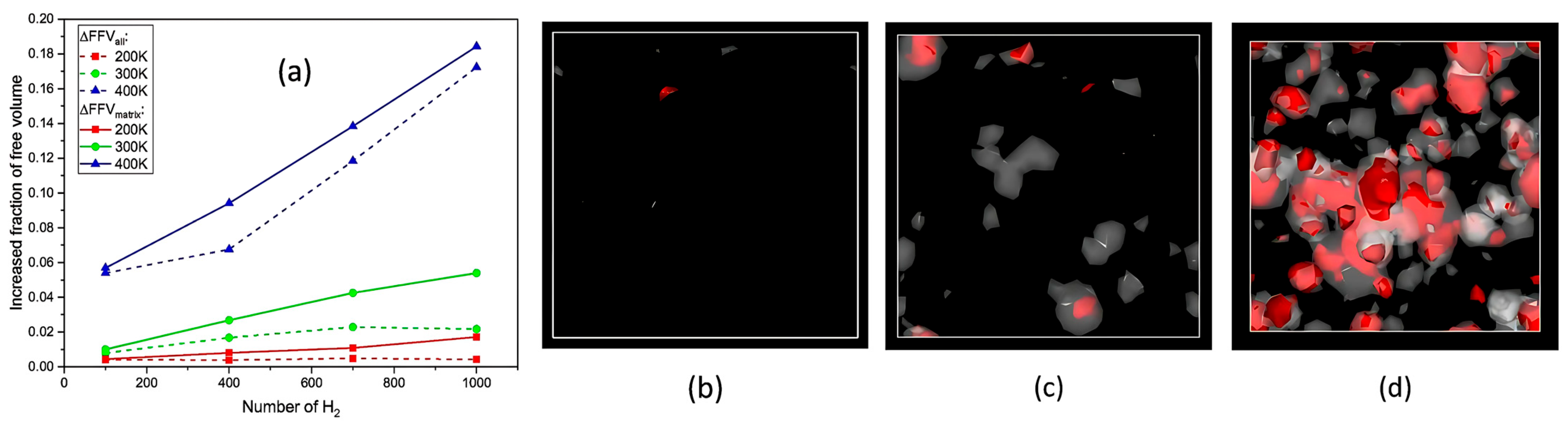
- Formation of bubbles during rapid pressure drops: Figure 19 presents the relationship between the changes in free volume (ΔFFV) for different components in a PE system containing 1000 H2 molecules. The solid line indicates ΔFFVmatrix, whereas the dashed line represents ΔFFVall. ΔFFVmatrix and ΔFFVall increase with the concentration of H2 (Figure 19a), suggesting the formation of voids during depressurization. The free volume distribution across simulations at 200, 300, and 400 K is shown in Figure 19b–d, respectively. As the temperature increases, ΔFFVall increases, with the distribution of free volume indicating that H2 molecules may aggregate, particularly at higher temperatures. This reinforces the trend that the H2 content within the PE matrix significantly impacts the free volume behavior and highlights the dynamic nature of H2 diffusion and aggregation under varying thermal conditions. To gain deeper insights into the behavior of the PE/H2 system during rapid depressurization, a 10 ns simulation was performed on a system comprising four branchless PE chains with a DP of 2000, containing 1000 H2 molecules, at a temperature of 400 K. Gas bubbles were initially detected at approximately 35 MPa but exhibited instability, appearing and disappearing at lower pressures (24, 16, and 8 MPa). At approximately 5 MPa, the bubbles began to expand, with the final sizes reaching 1–2 nm (Figure 20). These findings suggest that high-pressure conditions can lead to the formation of H2 nanobubbles in an oversaturated PE matrix, particularly if depressurization occurs too quickly for adequate diffusion to occur. Methods such as SAXS and XRD are recommended to analyze this phenomenon further.
- -
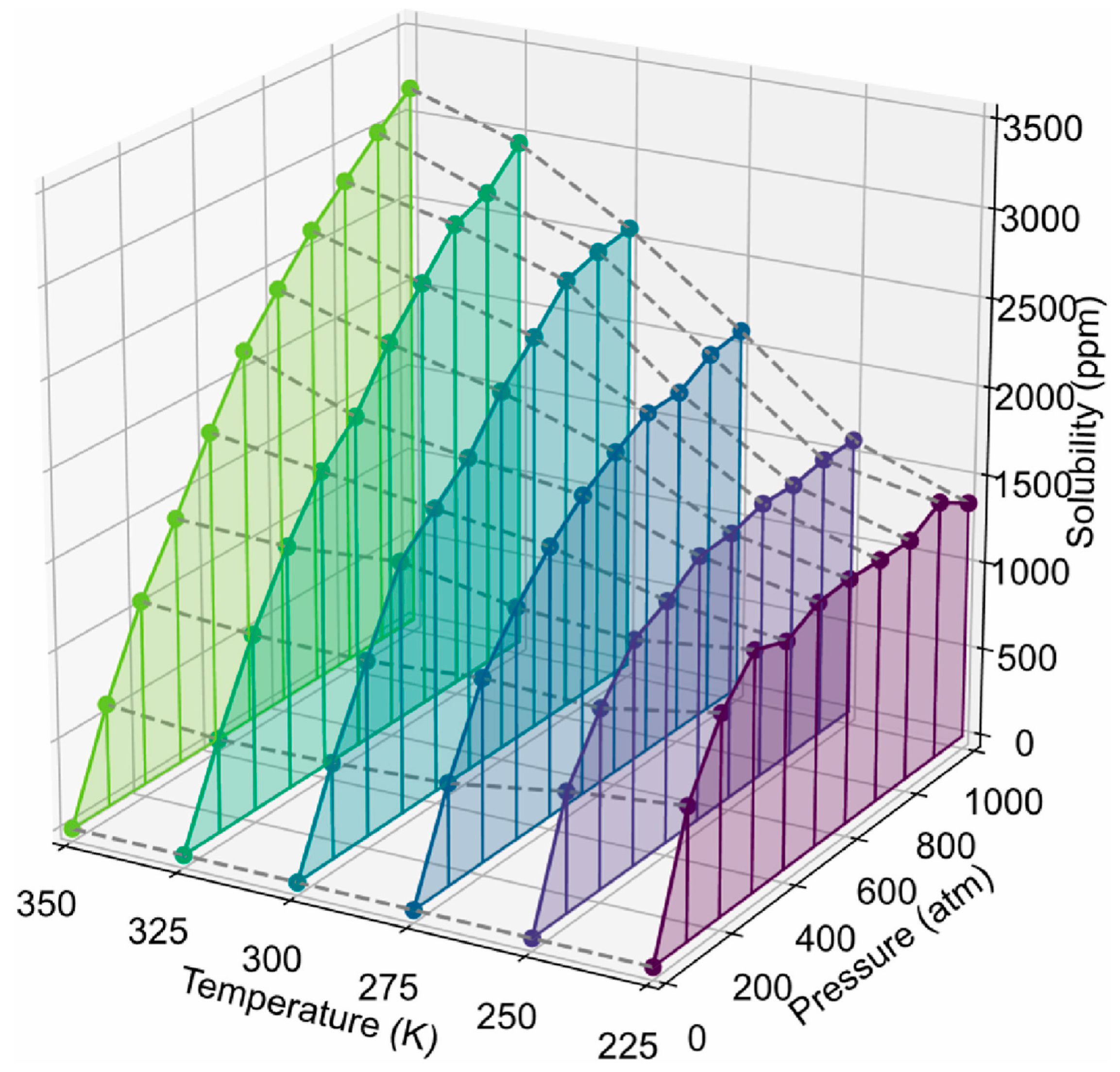
- Solubility of hydrogen: The analysis of hydrogen solubility in amorphous PE, illustrated in Figure 21, revealed that at 700 atm and 300 K, the solubility reached 2245 ppm. This value is in close agreement with a prior simulation result of 2200 ppm [39] but exceeds the experimental measurement of 1768 ppm [50]. This discrepancy may be attributed to crystalline regions that restrict solubility in actual materials. These findings highlight a clear shift in the solubility behavior. Below the Tg of 256 K, the solubility adheres to a Langmuir-type sorption model, where it increases with pressure until saturation is reached. Conversely, above the Tg, a dual-mode sorption mechanism emerges, revealing a more intricate relationship in which solubility continues to increase with pressure, encompassing elements of Henry’s law and Langmuir sorption as the polymer transitions from a glassy to a rubbery state.
- -
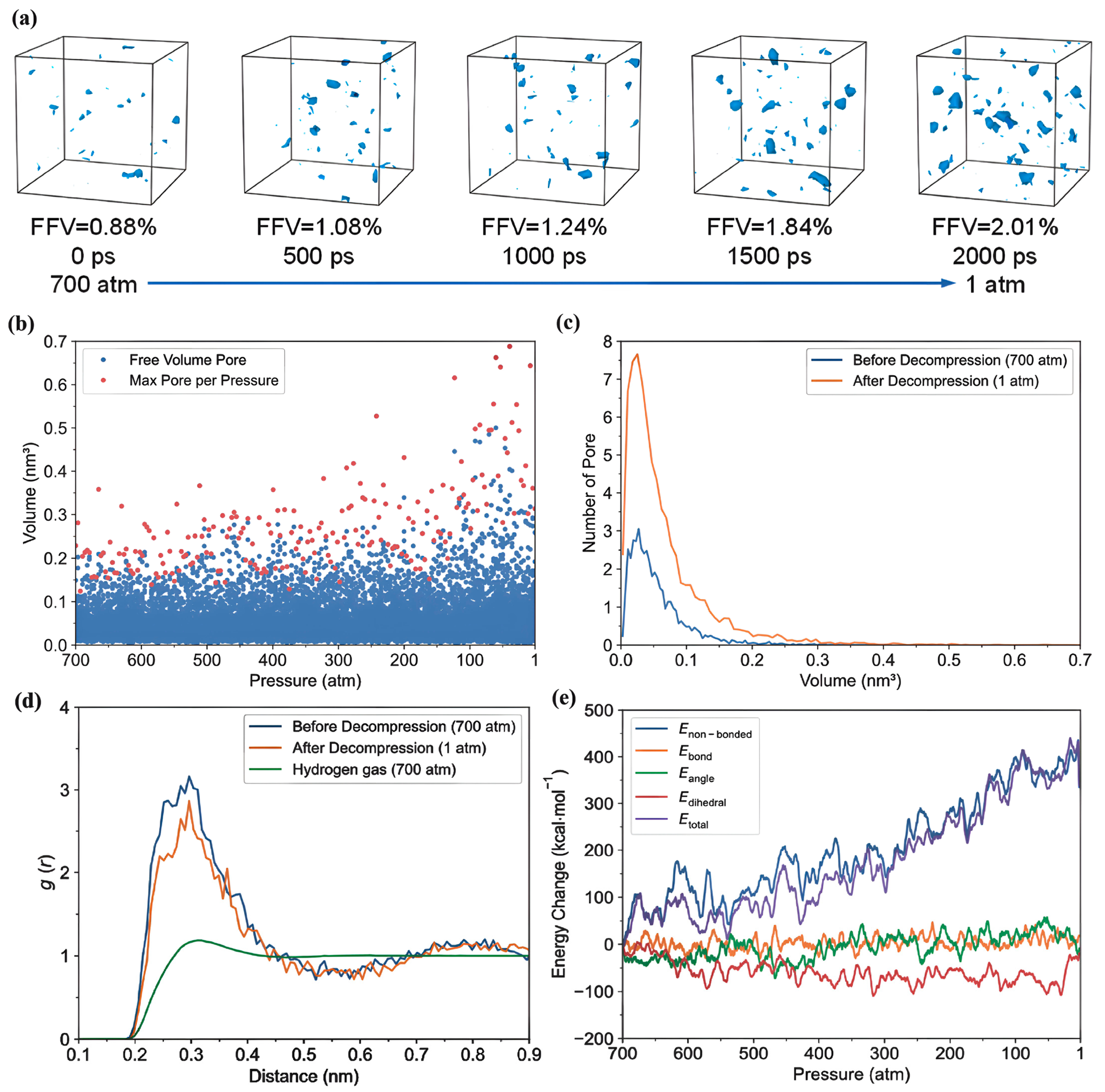
- Energy changes during decompression: Figure 22a depicts the changes in the free volume within PE systems containing 155 H2 molecules following decompression from 700 to 1 atm at 300 K. Data were collected at intervals of 0, 500, 1000, 1500, and 2000 ps. During this process, the FFV rose from 0.88% to 2.01%, accompanied by an increase in the number of cavities from 42 to 80, and the average pore size growing from 0.06 to 0.07 nm3 at the end of decompression. Figure 22b shows a scatter plot illustrating that as the pressure decreased, the maximum pore size increased, with the majority of pore sizes remaining under 0.2 nm3. Additionally, Figure 22c shows a significant increase in the quantity and dimensions of the pores following decompression. In Figure 22d, the RDF reveals that the peak at approximately 0.3 nm for hydrogen clustering remains significant, and the peak height post-decompression is slightly reduced, suggesting an increased dispersion of H2 molecules within the PE matrix. The analysis of internal energy shows that energy changes during decompression are predominantly influenced by non-bonded interactions among the polymer chains, particularly as the intermolecular distances increase (Figure 22e). This expansion in intermolecular spacing weakens the cohesive forces within the polymer matrix, establishing favorable conditions for the generation of free volume and consequently, increasing the risk of cavitation damage.
- -
- Factors increasing cavitation risk: Figure 23 highlights the significant effects of the initial pressure, temperature, and tensile stress on the behavior of a PE system during decompression. As shown in Figure 23a, the relative volume change exhibits a linear increase with higher initial pressures, increasing from 0.48% at 100 atm to 4.80% at 1000 atm. In Figure 23b, the FFV exhibits a slight decline before decompression owing to mechanical compression but shows an increase post-decompression, suggesting an elevated risk of cavitation. Furthermore, Figure 23c and d indicate that at an initial pressure of 700 atm, the relative volume changes and FFV increased significantly with increasing temperature. This underscores the fact that temperatures exceeding the Tg result in a notable expansion of the free volume, thereby increasing the likelihood of cavitation. Finally, Figure 23e,f demonstrates that although the relative volume changes during decompression remained relatively stable across different levels of tensile stress, the FFV significantly increased with higher applied stress. This indicates that tensile tension promotes the expansion of the free volume, consequently increasing the risk of cavitation within the PE material. The influences of the initial pressure, hydrogen content, temperature, and applied tensile stress notably enhanced the increase in free volume during decompression, further increasing the risk of cavitation.
3.1.5. Effect of Nanofillers
- -
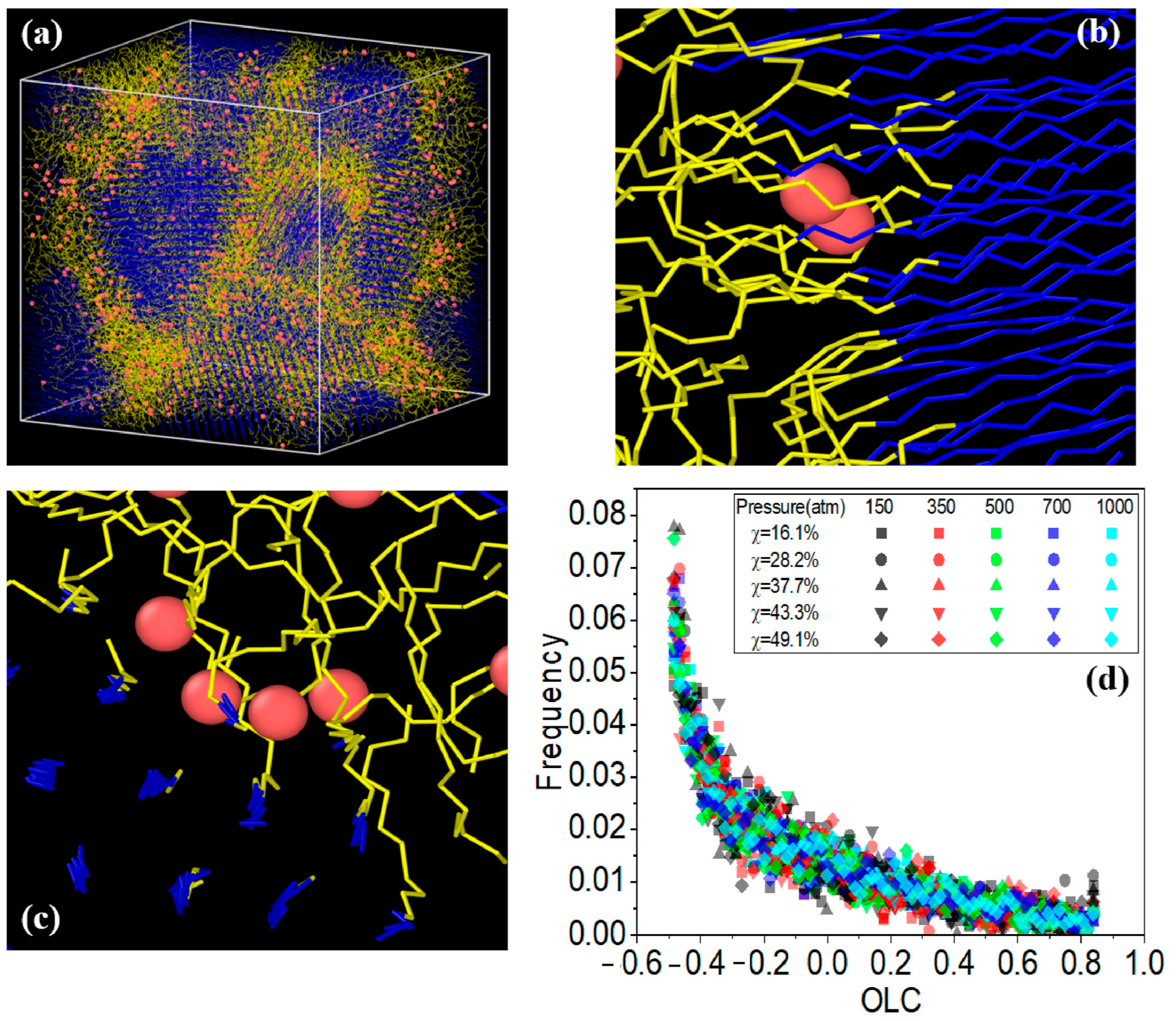
- The diffusion coefficients of six systems at different temperatures (233 K, 298 K, and 358 K) show an increase with temperature, as illustrated in Figure 24a. At 358 K and 70 MPa, pure PA6 has the highest diffusion coefficient of 2.28 × 10−6 cm2/s, attributed to enhanced molecular mobility with rising kinetic energy [26]. A prior study by Dong et al. [26] reported a similar coefficient of 2.36 × 10−6 cm2/s for PA6 at 358 K and 87.5 MPa, with a 10.6% error margin deemed acceptable for gas diffusion measurements. The diffusion coefficients for graphene/PA6 composites also increase with temperature; for instance, the 3 wt% composite at 358 K shows a 48% increase compared to 298 K. Higher graphene concentrations (4 wt% to 7 wt%) lead to increases in diffusion coefficients by approximately 77%, 138%, 115%, and 89%, highlighting the considerable effect of temperature on diffusion properties, aligning with earlier findings [53].
- -
- The diffusion coefficients of hydrogen decrease linearly with increasing pressure for all tested materials, with pure PA6 exhibiting the highest coefficients and the 4 wt% graphene/PA6 composite showing the lowest (Figure 24b). Permeability coefficients also decline as pressure rises. At 0.1 MPa, the 5 wt% graphene/PA6 composite has the lowest permeability at 2.44 × 10−13 cm3·cm/(cm2·s·Pa), while the 4 wt% composite records the lowest permeability values (2.15 × 10−13 to 1.96 × 10−13 cm3·cm/(cm2·s·Pa)) above 35 MPa. This trend aligns with findings that increased pressure compresses the polymer and reduces free volume, hindering H2 diffusion. Additionally, higher pressures lead to increased polymer crystallinity, further restricting hydrogen diffusion.
- -
- The FFV directly affects the diffusion coefficients of materials, with a larger FFV resulting in higher diffusion coefficients (Figure 24c,d). In Gr/PA6 composite systems, hydrogen diffusion occurs through a “vibration + leap” mechanism. The 3 wt% Gr/PA6 composite shows significant vibrational movements of hydrogen, whereas the 7 wt% composite enables both substantial forward and backward leaps of H2 molecules. Furthermore, increased pressure in the 4 wt% Gr/PA6 system leads to longer leap distances and higher leap frequencies for hydrogen.
3.2. Multi-Scale Modeling of Composite Hydrogen Storage Tanks
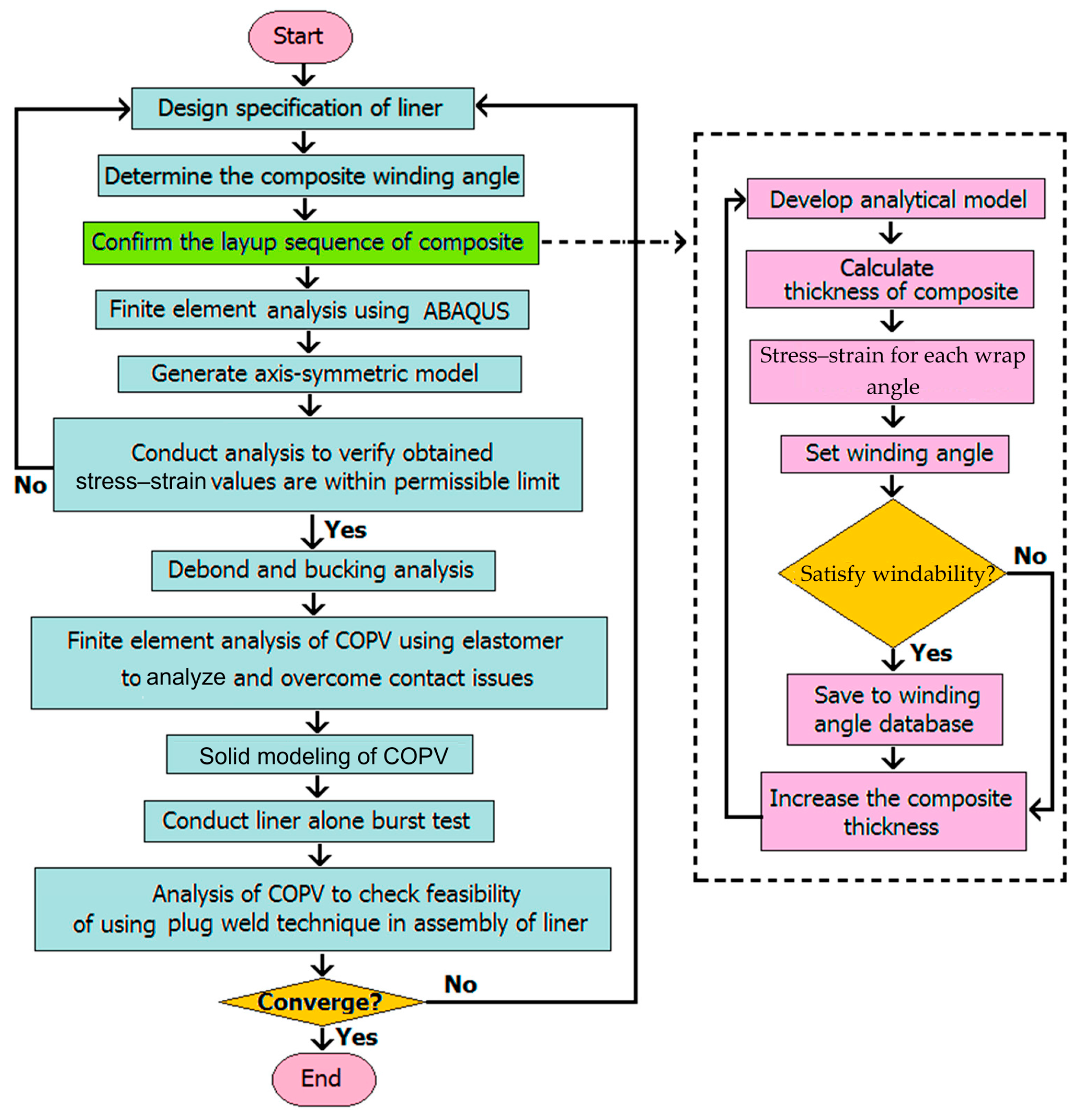
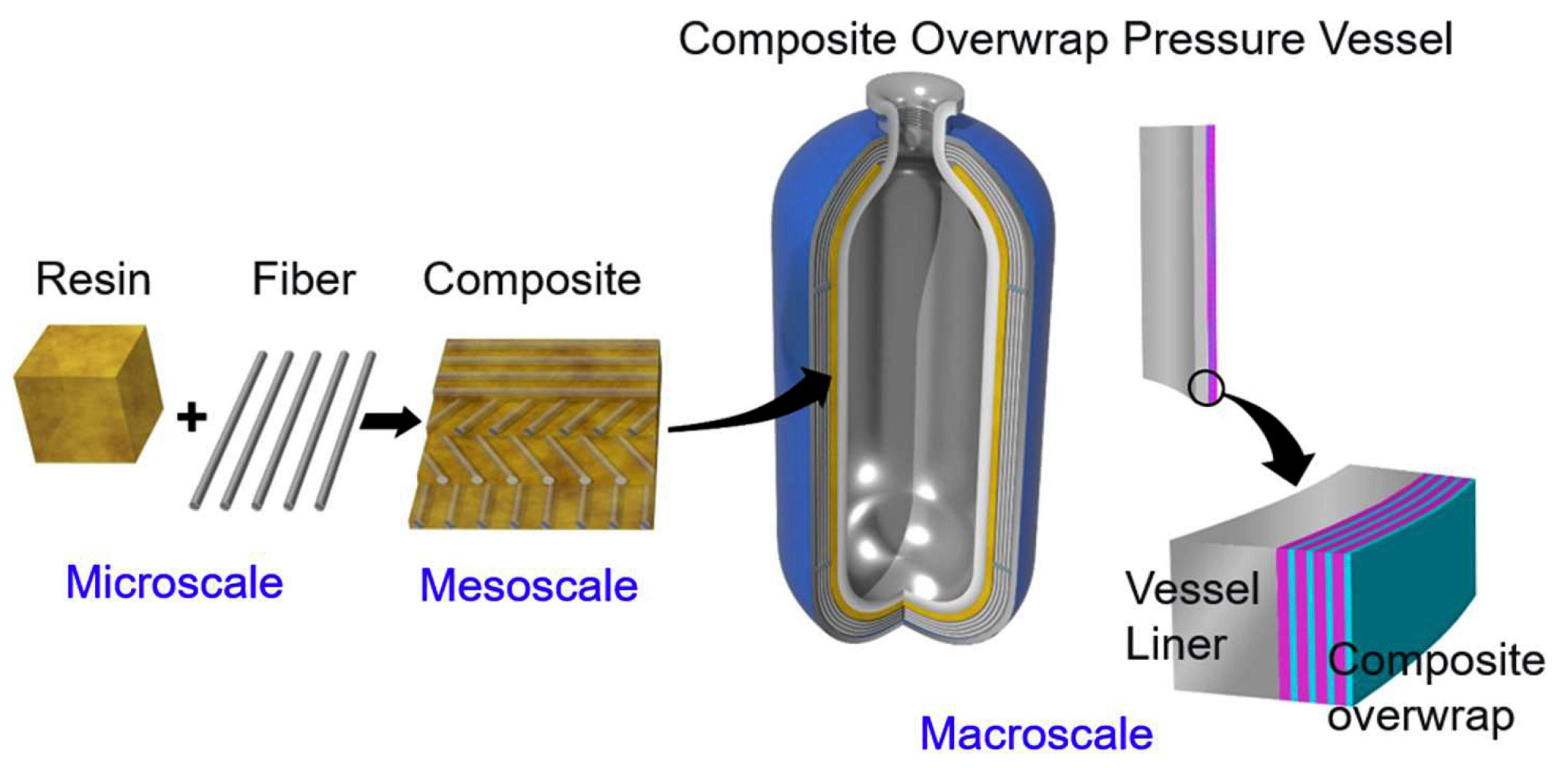
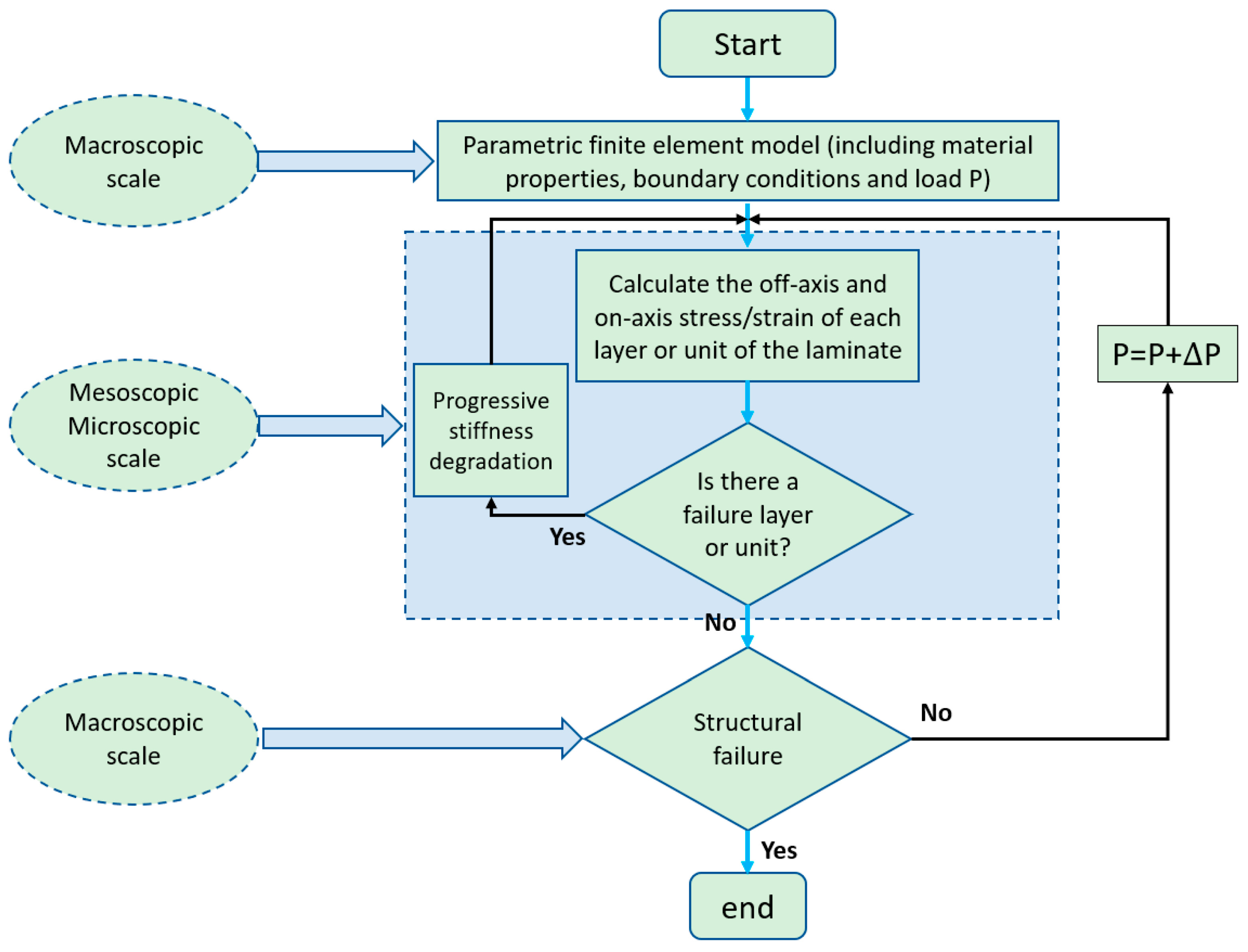
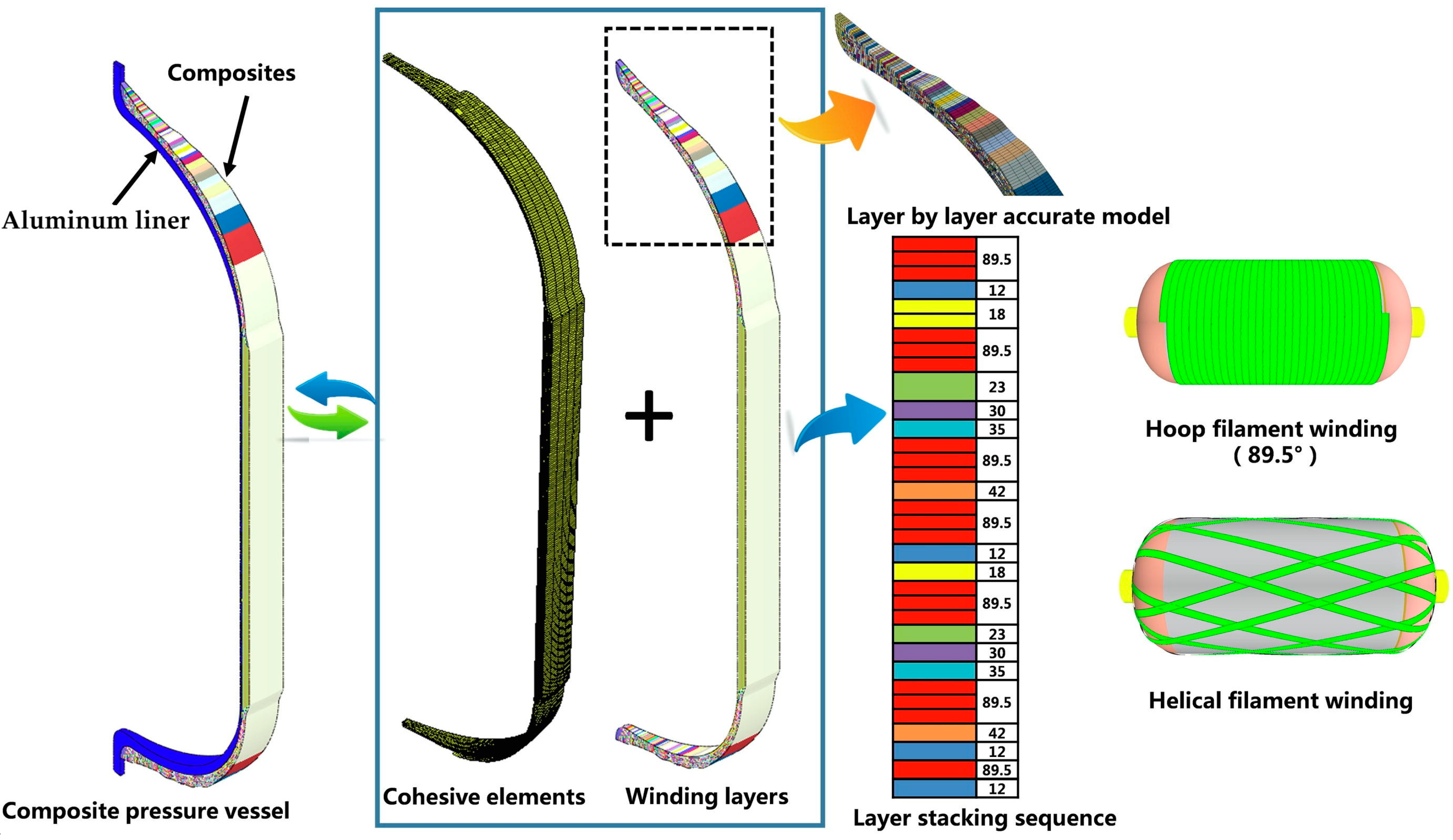


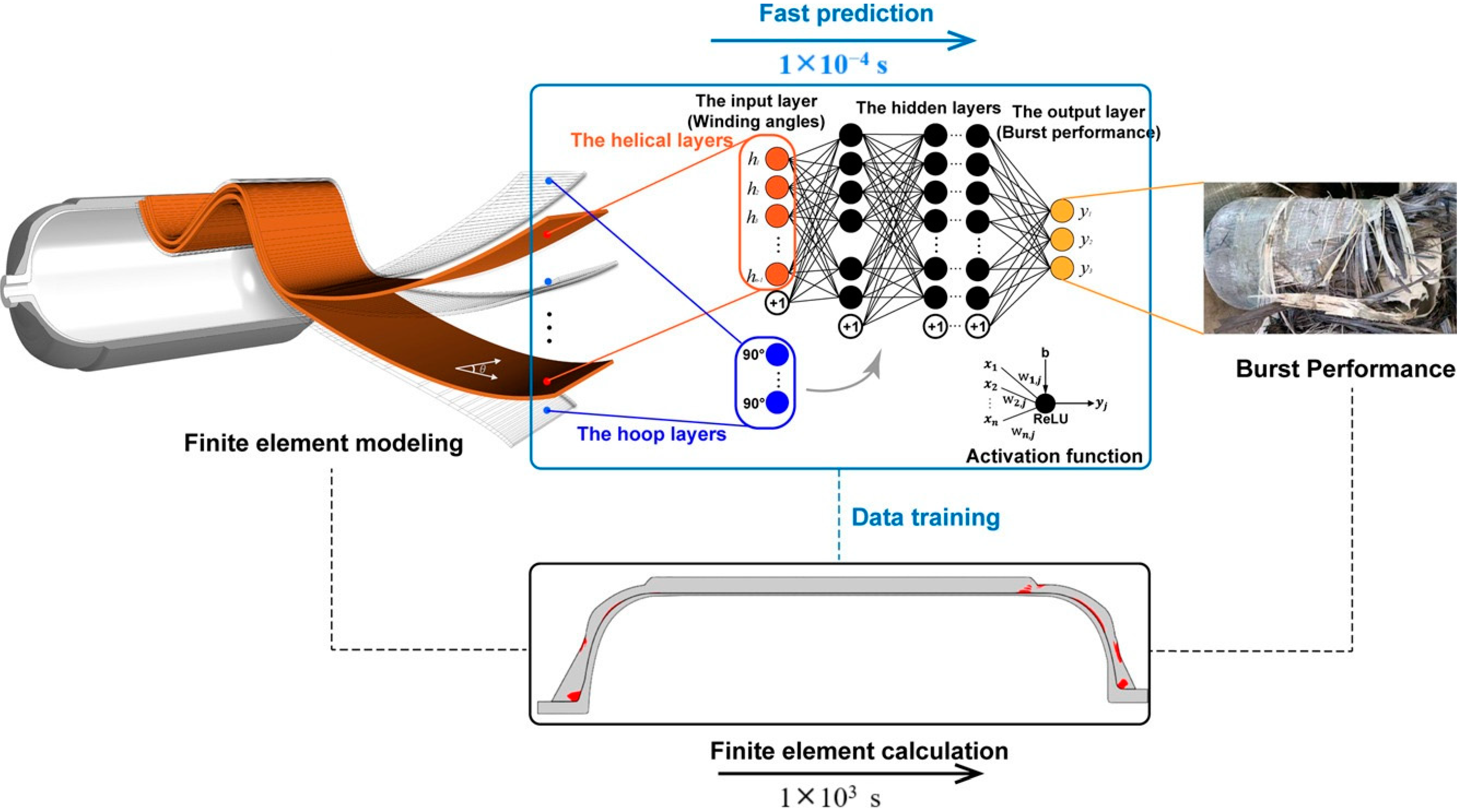
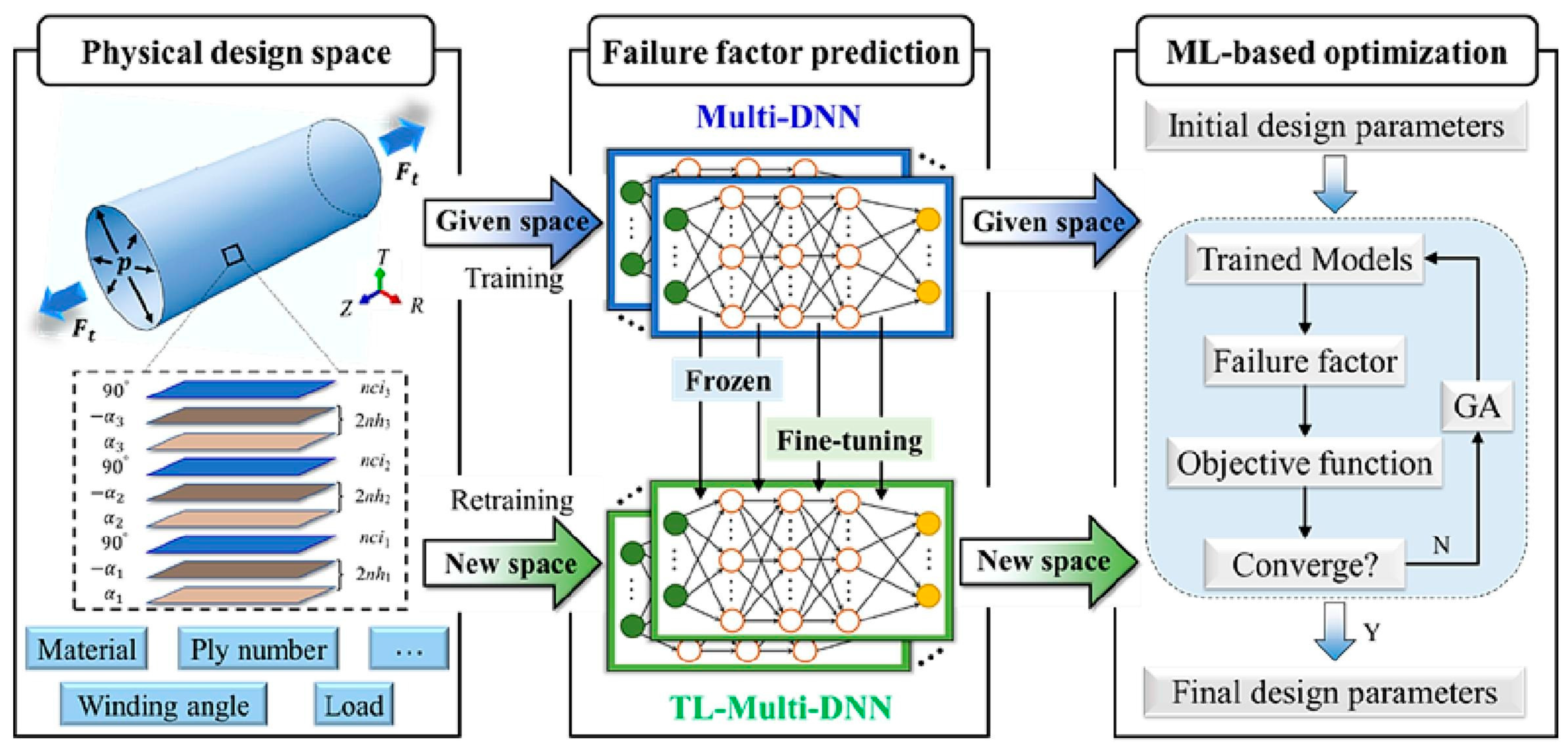
3.3. Artificial Intelligence for Hydrogen Storage Vessels
4. Challenges and Future Perspectives
5. Conclusions and Research Gaps
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
Abbreviations
| PE | Polyethylene |
| FFV | Fractional free volume |
| Tg | Glass transition temperature |
| MD | Molecular dynamics simulation |
| P | Pressure |
| T | Temperature |
| HDPE | High-density polyethylene |
| S | Solubility coefficient |
| MC | Monte Carlo simulation |
| MMT | Montmorillonite |
| MSD | Mean square displacement |
| PA6 | Polyamide 6 |
| D | Diffusion coefficient |
| PEEK | Polyether ether ketone |
| EVOH | Ethylene-vinyl alcohol copolymer |
| PFSA | Perfluorosulfonic acid polymer |
| CFRP | Carbon fiber reinforced plastic |
| RDF | Radial distribution functions |
| P | Permeability coefficient |
| MS | Materials Studio |
| OMMT | Modified montmorillonite |
| GCMC | Grand Canonical Monte Carlo |
| DP | Degree of polymerization |
| RGD | Rapid gas decompression |
| HE | Hydrogen embrittlement |
| LIC | Lamellar inorganic components |
| CNC | Computer numerical control |
| PPS | Polyphenylene sulfide |
| POM | Polyoxymethylene |
| UTM | Universal testing machine |
| Tm | Melting temperature |
| Tc | Crystallization temperature |
| SAXS | Small-angle X-ray scattering |
| CDM | Continuum damage mechanics |
| RVE | Representative volume element |
| ANN | Artificial neural network |
| FEM | Finite element method |
| CPVs | Composite pressure vessels |
| CVFF | Consistent valence forcefield |
References
- Lee, S.; Han, H.S.; Lee, J.H.; Jeong, J.; Seong, D.G. EPDM Rubber-Reinforced PA6/EVOH Composite with Enhanced Gas Barrier Properties and Injection Moldability for Hydrogen Tank Liner. Fibers Polym. 2024, 25, 2327–2335. [Google Scholar] [CrossRef]
- Su, Y.; Lv, H.; Zhou, W.; Zhang, C. Review of the Hydrogen Permeability of the Liner Material of Type IV On-Board Hydrogen Storage Tank. World Electr. Veh. J. 2021, 12, 130. [Google Scholar] [CrossRef]
- Jin, Z.; Su, Y.; Lv, H.; Liu, M.; Li, W.; Zhang, C. Review of Decompression Damage of the Polymer Liner of the Type IV Hydrogen Storage Tank. Polymers 2023, 15, 2258. [Google Scholar] [CrossRef] [PubMed]
- Li, X.; Huang, Q.; Liu, Y.; Zhao, B.; Li, J. Review of the Hydrogen Permeation Test of the Polymer Liner Material of Type IV On-Board Hydrogen Storage Cylinders. Materials 2023, 16, 5366. [Google Scholar] [CrossRef]
- Sun, Y.; Lv, H.; Zhou, W.; Zhang, C. Research on Hydrogen Permeability of Polyamide 6 as the Liner Material for Type IV Hydrogen Storage Tank. Int. J. Hydrogen Energy 2020, 45, 24980–24990. [Google Scholar] [CrossRef]
- Cui, Y.; Kumar, S.; Kona, B.R.; Houcke, D. van Gas Barrier Properties of Polymer/Clay Nanocomposites. RSC Adv. 2015, 5, 63669–63690. [Google Scholar] [CrossRef]
- Cui, Y.; Kundalwal, S.I.; Kumar, S. Gas Barrier Performance of Graphene/Polymer Nanocomposites. Carbon 2016, 98, 313–333. [Google Scholar] [CrossRef]
- Barthelemy, H.; Weber, M.; Barbier, F. Hydrogen Storage: Recent Improvements and Industrial Perspectives. Int. J. Hydrogen Energy 2017, 42, 7254–7262. [Google Scholar] [CrossRef]
- Li, J.; Zhao, X.; Liang, J.; Zhao, C.; Feng, N.; Guo, G.; Zhou, Z. Molecular Dynamics Simulation of Hydrogen Barrier Performance of Modified Polyamide 6 Lining of IV Hydrogen Storage Tank with Graphene. Polymers 2024, 16, 2185. [Google Scholar] [CrossRef]
- Zhang, X.; Zhai, L.; Li, H.; Qi, G.; Gao, X.; Yang, W. Molecular Simulation Study on the Hydrogen Permeation Behavior and Mechanism of Common Polymers. Polymers 2024, 16, 953. [Google Scholar] [CrossRef]
- Wu, P.; Zhang, J.; Yang, Z.; Zhao, J. Molecular Dynamics Simulation of Helium Barrier Performance of Modified Polyamide 6 Lining of IV Hydrogen Storage Tank with Montmorillonite. Molecules 2023, 28, 3333. [Google Scholar] [CrossRef] [PubMed]
- Fang, Q.; Ji, D. Molecular Simulation of Hydrogen Permeation Behavior in Liner Polymer Materials of Type IV Hydrogen Storage Vessels. Mater. Today Commun. 2023, 35, 106302. [Google Scholar] [CrossRef]
- Ding, G.; Tam, L.; Wu, C. Molecular Investigation on Physical and Tensile Properties of Polyethylene (PE) under High-Pressure Hydrogen Environments. Int. J. Hydrogen Energy 2024, 86, 1036–1050. [Google Scholar] [CrossRef]
- Hollingsworth, S.A.; Dror, R.O. Molecular Dynamics Simulation for All. Neuron 2018, 99, 1129–1143. [Google Scholar] [CrossRef]
- Barraco, M.; Neyertz, S.; Benes, N.E.; Brown, D. Comparison of Eight Classical Lennard-Jones-Based H2 Molecular Models in the Gas Phase at Temperatures and Pressures Relevant to Hydrogen On-Board Storage Tanks. J. Phys. Chem. A 2023, 127, 6335–6346. [Google Scholar] [CrossRef]
- Neyertz, S.; Brown, D.; Salimi, S.; Radmanesh, F.; Benes, N.E. Molecular Characterization of Membrane Gas Separation under Very High Temperatures and Pressure: Single- and Mixed-Gas CO2/CH4 and CO2/N2 Permselectivities in Hybrid Networks. Membranes 2022, 12, 526. [Google Scholar] [CrossRef]
- Wang, X.; Tian, M.; Chen, X.; Xie, P.; Yang, J.; Chen, J.; Yang, W. Advances on Materials Design and Manufacture Technology of Plastic Liner of Type IV Hydrogen Storage Vessel. Int. J. Hydrogen Energy 2022, 47, 8382–8408. [Google Scholar] [CrossRef]
- Moradi, R.; Groth, K.M. Hydrogen Storage and Delivery: Review of the State of the Art Technologies and Risk and Reliability Analysis. Int. J. Hydrogen Energy 2019, 44, 12254–12269. [Google Scholar] [CrossRef]
- Liu, J.; Ma, H.; Zheng, S.; Zhang, Z.; Zheng, J.; Zhao, Y. Numerical Investigation on Temperature-Rise of on-Bus Gaseous Hydrogen Storage Cylinder with Different Thickness of Liner and Wrapping Material. Int. J. Hydrogen Energy 2021, 46, 20607–20620. [Google Scholar] [CrossRef]
- Goh, K.; Heising, J.K.; Yuan, Y.; Karahan, H.E.; Wei, L.; Zhai, S.; Koh, J.-X.; Htin, N.M.; Zhang, F.; Wang, R.; et al. Sandwich-Architectured Poly(Lactic Acid)–Graphene Composite Food Packaging Films. ACS Appl. Mater. Interfaces 2016, 8, 9994–10004. [Google Scholar] [CrossRef]
- Vannini, M.; Marchese, P.; Celli, A.; Lorenzetti, C. Strategy To Modify the Crystallization Behavior of EVOH32 through Interactions with Low-Molecular-Weight Molecules. Ind. Eng. Chem. Res. 2016, 55, 3517–3524. [Google Scholar] [CrossRef]
- Pandey, S.; Jana, K.K.; Aswal, V.K.; Rana, D.; Maiti, P. Effect of Nanoparticle on the Mechanical and Gas Barrier Properties of Thermoplastic Polyurethane. Appl. Clay Sci. 2017, 146, 468–474. [Google Scholar] [CrossRef]
- Wang, G.; Zhang, D.; Wan, G.; Li, B.; Zhao, G. Glass Fiber Reinforced PLA Composite with Enhanced Mechanical Properties, Thermal Behavior, and Foaming Ability. Polymer 2019, 181, 121803. [Google Scholar] [CrossRef]
- Li, T.; Chen, S.; Wan, S.; Cai, S.; He, X. The Effect of Functional Zirconium Phosphate on Aging Resistance of Nitrile Butadiene Rubber Composites. Polym. Compos. 2020, 41, 1867–1877. [Google Scholar] [CrossRef]
- Doblhofer, E.; Schmid, J.; Rieß, M.; Daab, M.; Suntinger, M.; Habel, C.; Bargel, H.; Hugenschmidt, C.; Rosenfeldt, S.; Breu, J.; et al. Structural Insights into Water-Based Spider Silk Protein–Nanoclay Composites with Excellent Gas and Water Vapor Barrier Properties. ACS Appl. Mater. Interfaces 2016, 8, 25535–25543. [Google Scholar] [CrossRef]
- Dong, C.; Liu, Y.; Li, J.; Bin, G.; Zhou, C.; Han, W.; Li, X. Hydrogen Permeability of Polyamide 6 Used as Liner Material for Type IV On-Board Hydrogen Storage Cylinders. Polymers 2023, 15, 3715. [Google Scholar] [CrossRef]
- Li, X.; Dong, C.; Liu, Y.; Li, J.; Bin, G.; Zhou, C.; Han, W. Study on the Effect of Hydrogen Cycle Pressure Relief Time on the Hydrogen Permeability and Mechanical Properties of Polyamide Liner Materials for Type IV Hydrogen Storage Cylinders of HFCVs. Int. J. Hydrogen Energy 2024, 95, 993–1003. [Google Scholar] [CrossRef]
- Li, L.; Zhao, J.; Wang, X.; Yang, Q.; Wang, X.; Yin, H. Studies on Modification of Polyamide 6 Plastics for Hydrogen Storage. Polymers 2025, 17, 523. [Google Scholar] [CrossRef]
- Kis, D.I.; Bata, A.; Takács, J.; Kókai, E. Mechanical Properties of Clay-Reinforced Polyamide 6 Nanocomposite Liner Materials of Type IV Hydrogen Storage Vessels. Nanomaterials 2024, 14, 1385. [Google Scholar] [CrossRef]
- Zheng, D.; Li, J.; Liu, B.; Yu, B.; Yang, Y.; Han, D.; Li, J.; Huang, Z. Molecular Dynamics Investigations into the Hydrogen Permeation Mechanism of Polyethylene Pipeline Material. J. Mol. Liq. 2022, 368, 120773. [Google Scholar] [CrossRef]
- Theodorou, D.N.; Suter, U.W. Atomistic Modeling of Mechanical Properties of Polymeric Glasses. Macromolecules 1986, 19, 139–154. [Google Scholar] [CrossRef]
- Su, Y.; Lv, H.; Feng, C.; Zhang, C. Hydrogen Permeability of Polyamide 6 as the Liner Material of Type IV Hydrogen Storage Tanks: A Molecular Dynamics Investigation. Int. J. Hydrogen Energy 2024, 50, 1598–1606. [Google Scholar] [CrossRef]
- Minelli, M.; Oradei, S.; Fiorini, M.; Sarti, G.C. CO2 Plasticization Effect on Glassy Polymeric Membranes. Polymer 2019, 163, 29–35. [Google Scholar] [CrossRef]
- Takeuchi, K.; Kuo, A.-T.; Hirai, T.; Miyajima, T.; Urata, S.; Terazono, S.; Okazaki, S.; Shinoda, W. Hydrogen Permeation in Hydrated Perfluorosulfonic Acid Polymer Membranes: Effect of Polymer Crystallinity and Equivalent Weight. J. Phys. Chem. C 2019, 123, 20628–20638. [Google Scholar] [CrossRef]
- Klopffer, M.H.; Berne, P.; Castagnet, S.; Weber, M.; Hochstetter, G.; Espuche, E. Polymer pipes for distributing mixtures of hydrogen and natural gas. Evolution of their transport and mechanical properties after an ageing under an hydrogen environment. In Proceedings of the 18th World Hydrogen Energy Conference, Essen, Germany, 16–21 May 2010. [Google Scholar]
- Yersak, T.A.; Baker, D.R.; Yanagisawa, Y.; Slavik, S.; Immel, R.; Mack-Gardner, A.; Herrmann, M.; Cai, M. Predictive Model for Depressurization-Induced Blistering of Type IV Tank Liners for Hydrogen Storage. Int. J. Hydrogen Energy 2017, 42, 28910–28917. [Google Scholar] [CrossRef]
- Pepin, J.; Lainé, E.; Grandidier, J.-C.; Castagnet, S.; Blanc-vannet, P.; Papin, P.; Weber, M. Determination of Key Parameters Responsible for Polymeric Liner Collapse in Hyperbaric Type IV Hydrogen Storage Vessels. Int. J. Hydrogen Energy 2018, 43, 16386–16399. [Google Scholar] [CrossRef]
- Zhao, J.; Li, X.; Wang, X.; Zhang, Q.; Yang, Q.; Yin, H.; Zhang, S.; Wu, C. Insights into the Solubility of H2 in Various Polyethylene Matrices at High Pressure: A Coarse-Grained MC/MD Study. Int. J. Hydrogen Energy 2023, 48, 19619–19632. [Google Scholar] [CrossRef]
- Thompson, A.P.; Aktulga, H.M.; Berger, R.; Bolintineanu, D.S.; Brown, W.M.; Crozier, P.S.; In’t Veld, P.J.; Kohlmeyer, A.; Moore, S.G.; Nguyen, T.D.; et al. LAMMPS—A Flexible Simulation Tool for Particle-Based Materials Modeling at the Atomic, Meso, and Continuum Scales. Comput. Phys. Commun. 2022, 271, 108171. [Google Scholar] [CrossRef]
- Bowman, A.L.; Mun, S.; Nouranian, S.; Huddleston, B.D.; Gwaltney, S.R.; Baskes, M.I.; Horstemeyer, M.F. Free Volume and Internal Structural Evolution during Creep in Model Amorphous Polyethylene by Molecular Dynamics Simulations. Polymer 2019, 170, 85–100. [Google Scholar] [CrossRef]
- Briscoe, B.J.; Savvas, T.; Kelly, C.T. “Explosive Decompression Failure” of Rubbers: A Review of the Origins of Pneumatic Stress Induced Rupture in Elastomers. Rubber Chem. Technol. 1994, 67, 384–416. [Google Scholar] [CrossRef]
- Sgambitterra, E.; Pagnotta, L. Permeability: The Driving Force That Influences the Mechanical Behavior of Polymers Used for Hydrogen Storage and Delivery. Energies 2024, 17, 2216. [Google Scholar] [CrossRef]
- Yamabe, J.; Nishimura, S. Nanoscale Fracture Analysis by Atomic Force Microscopy of EPDM Rubber Due to High-Pressure Hydrogen Decompression. J. Mater. Sci. 2011, 46, 2300–2307. [Google Scholar] [CrossRef]
- Jaravel, J.; Castagnet, S.; Grandidier, J.-C.; Gueguen, M. Experimental Real-Time Tracking and Diffusion/Mechanics Numerical Simulation of Cavitation in Gas-Saturated Elastomers. Int. J. Solids Struct. 2013, 50, 1314–1324. [Google Scholar] [CrossRef]
- Kane-Diallo, O.; Castagnet, S.; Nait-Ali, A.; Benoit, G.; Grandidier, J.-C. Time-Resolved Statistics of Cavity Fields Nucleated in a Gas-Exposed Rubber under Variable Decompression Conditions—Support to a Relevant Modeling Framework. Polym. Test. 2016, 51, 122–130. [Google Scholar] [CrossRef]
- Ono, H.; Fujiwara, H.; Onoue, K.; Nishimura, S. Influence of Repetitions of the High-Pressure Hydrogen Gas Exposure on the Internal Damage Quantity of High-Density Polyethylene Evaluated by Transmitted Light Digital Image. Int. J. Hydrogen Energy 2019, 44, 23303–23319. [Google Scholar] [CrossRef]
- Pépin, J.; Lainé, E.; Grandidier, J.-C.; Benoit, G.; Mellier, D.; Weber, M.; Langlois, C. Replication of Liner Collapse Phenomenon Observed in Hyperbaric Type IV Hydrogen Storage Vessel by Explosive Decompression Experiments. Int. J. Hydrogen Energy 2018, 43, 4671–4680. [Google Scholar] [CrossRef]
- Zhao, J.; Wang, X.; Yang, Q.; Yin, H.; Zhao, B.; Zhang, S.; Wu, C. Molecular Dynamics Simulation of H2 in Amorphous Polyethylene System: H2 Diffusion in Various PE Matrices and Bubbling during Rapid Depressurization. Int. J. Hydrogen Energy 2022, 47, 39572–39585. [Google Scholar] [CrossRef]
- Ding, G.; Tighe, C.J.; Tam, L.; Wu, C. Molecular Study of High-Pressure Hydrogen Decompression in Amorphous Polyethylene. Int. J. Hydrogen Energy 2025, 114, 267–278. [Google Scholar] [CrossRef]
- Fujiwara, H.; Ono, H.; Ohyama, K.; Kasai, M.; Kaneko, F.; Nishimura, S. Hydrogen Permeation under High Pressure Conditions and the Destruction of Exposed Polyethylene-Property of Polymeric Materials for High-Pressure Hydrogen Devices (2)-. Int. J. Hydrogen Energy 2021, 46, 11832–11848. [Google Scholar] [CrossRef]
- Melnichuk, M.; Gardavaud, Q.; Thiébaud, F.; Perreux, D. Temperature Effect in Cavitation Risk Assessments of Polymers for Hydrogen Systems. Int. J. Hydrogen Energy 2020, 45, 23020–23026. [Google Scholar] [CrossRef]
- Gerland, M.; Boyer, S.A.E.; Castagnet, S. Early Stages of Cavitation in a Stretched and Decompressed Poly(Vinylidene Fluoride) Exposed to Diffusive Hydrogen, Observed by Transmission Electronic Microscopy at the Nanoscale. Int. J. Hydrogen Energy 2016, 41, 1766–1774. [Google Scholar] [CrossRef]
- Bian, L.; Shu, Y.; Xu, J.; Wang, L. Molecular Dynamics Study on Permeability of Gas Molecules through Amorphous PPX Polymers. Int. Polym. Process. 2013, 28, 24–33. [Google Scholar] [CrossRef]
- Zhang, N.; Gao, S.; Song, M.; Chen, Y.; Zhao, X.; Liang, J.; Feng, J. A Multiscale Study of CFRP Based on Asymptotic Homogenization with Application to Mechanical Analysis of Composite Pressure Vessels. Polymers 2022, 14, 2817. [Google Scholar] [CrossRef] [PubMed]
- Zhang, M.; Lv, H.; Kang, H.; Zhou, W.; Zhang, C. A Literature Review of Failure Prediction and Analysis Methods for Composite High-Pressure Hydrogen Storage Tanks. Int. J. Hydrogen Energy 2019, 44, 25777–25799. [Google Scholar] [CrossRef]
- Lin, S.; Yang, L.; Xu, H.; Jia, X.; Yang, X.; Zu, L. Progressive Damage Analysis for Multiscale Modelling of Composite Pressure Vessels Based on Puck Failure Criterion. Compos. Struct. 2021, 255, 113046. [Google Scholar] [CrossRef]
- Son, D.-S.; Hong, J.-H.; Chang, S.-H. Determination of the Autofrettage Pressure and Estimation of Material Failures of a Type III Hydrogen Pressure Vessel by Using Finite Element Analysis. Int. J. Hydrogen Energy 2012, 37, 12771–12781. [Google Scholar] [CrossRef]
- Wu, Q.G.; Chen, X.D.; Fan, Z.C.; Nie, D.F. Stress and Damage Analyses of Composite Overwrapped Pressure Vessel. Procedia Eng. 2015, 130, 32–40. [Google Scholar] [CrossRef]
- Tsai, S.W.; Wu, E.M. A General Theory of Strength for Anisotropic Materials. J. Compos. Mater. 1971, 5, 58–80. [Google Scholar] [CrossRef]
- Park, W.R.; Fatoni, N.F.; Kwon, O.H. Evaluation of Stress and Crack Behavior Using the Extended Finite Element Method in the Composite Layer of a Type III Hydrogen Storage Vessel. J. Mech. Sci. Technol. 2018, 32, 1995–2002. [Google Scholar] [CrossRef]
- Rafiee, R.; Salehi, A. A Novel Recursive Multi-Scale Modeling for Predicting the Burst Pressure of Filament Wound Composite Pressure Vessels. Appl. Phys. A 2022, 128, 388. [Google Scholar] [CrossRef]
- Nguyen, B.N.; Roh, H.S.; Merkel, D.R.; Simmons, K.L. A Predictive Modeling Tool for Damage Analysis and Design of Hydrogen Storage Composite Pressure Vessels. Int. J. Hydrogen Energy 2021, 46, 20573–20585. [Google Scholar] [CrossRef]
- Nachtane, M.; Tarfaoui, M.; Abichou, M.A.; Vetcher, A.; Rouway, M.; Aâmir, A.; Mouadili, H.; Laaouidi, H.; Naanani, H. An Overview of the Recent Advances in Composite Materials and Artificial Intelligence for Hydrogen Storage Vessels Design. J. Compos. Sci. 2023, 7, 119. [Google Scholar] [CrossRef]
- Bouhala, L.; Karatrantos, A.; Reinhardt, H.; Schramm, N.; Akin, B.; Rauscher, A.; Mauersberger, A.; Taşkıran, S.T.; Ulaşlı, M.E.; Aktaş, E.; et al. Advancement in the Modeling and Design of Composite Pressure Vessels for Hydrogen Storage: A Comprehensive Review. J. Compos. Sci. 2024, 8, 339. [Google Scholar] [CrossRef]
- Suwarno, S.; Dicky, G.; Suyuthi, A.; Effendi, M.; Witantyo, W.; Noerochim, L.; Ismail, M. Machine Learning Analysis of Alloying Element Effects on Hydrogen Storage Properties of AB2 Metal Hydrides. Int. J. Hydrogen Energy 2022, 47, 11938–11947. [Google Scholar] [CrossRef]
- Pan, B.; Song, T.; Yue, M.; Chen, S.; Zhang, L.; Edlmann, K.; Neil, C.W.; Zhu, W.; Iglauer, S. Machine Learning—Based Shale Wettability Prediction: Implications for H2, CH4 and CO2 Geo-Storage. Int. J. Hydrogen Energy 2024, 56, 1384–1390. [Google Scholar] [CrossRef]
- Salehi, K.; Rahmani, M.; Atashrouz, S. Machine Learning Assisted Predictions for Hydrogen Storage in Metal-Organic Frameworks. Int. J. Hydrogen Energy 2023, 48, 33260–33275. [Google Scholar] [CrossRef]
- Sharma, A.; Mukhopadhyay, T.; Rangappa, S.M.; Siengchin, S.; Kushvaha, V. Advances in Computational Intelligence of Polymer Composite Materials: Machine Learning Assisted Modeling, Analysis and Design. Arch. Comput. Methods Eng. 2022, 29, 3341–3385. [Google Scholar] [CrossRef]
- Xu, R.; Yang, J.; Yan, W.; Huang, Q.; Giunta, G.; Belouettar, S.; Zahrouni, H.; Zineb, T.B.; Hu, H. Data-Driven Multiscale Finite Element Method: From Concurrence to Separation. Comput. Methods Appl. Mech. Eng. 2020, 363, 112893. [Google Scholar] [CrossRef]
- Li, F.; Chen, X.; Xu, P.; Fan, Z.; Wang, Q.; Lyu, C.; Zhang, Q.; Yu, H.; Wu, H. Optimal Design of Thin-Layered Composites for Type IV Vessels: Finite Element Analysis Enhanced by ANN. Thin-Walled Struct. 2023, 187, 110752. [Google Scholar] [CrossRef]
- Wang, Q.; Qin, H.; Jia, L.; Li, Z.; Zhang, G.; Li, Y.; Liu, Y. Failure Prediction and Optimization for Composite Pressure Vessel Combining FEM Simulation and Machine Learning Approach. Compos. Struct. 2024, 337, 118099. [Google Scholar] [CrossRef]
- Kadri, K.; Kallel, A.; Guerard, G.; Abdallah, A.B.; Ballut, S.; Fitoussi, J.; Shirinbayan, M. Study of Composite Polymer Degradation for High Pressure Hydrogen Vessel by Machine Learning Approach. Energy Storage 2024, 6, e645. [Google Scholar] [CrossRef]
- Hong, H.; Kim, W.; Kim, S.; Lee, K.; Kim, S.S. Deep Transfer Learning for Efficient and Accurate Prediction of Composite Pressure Vessel Behaviors. Compos. Part A Appl. Sci. Manuf. 2024, 186, 108413. [Google Scholar] [CrossRef]
- Islam, M.; Sohaib, M.; Kim, J.; Kim, J.-M. Crack Classification of a Pressure Vessel Using Feature Selection and Deep Learning Methods. Sensors 2018, 18, 4379. [Google Scholar] [CrossRef] [PubMed]
- Azizian, M.; Almeida, J.H.S. Stochastic, Probabilistic and Reliability Analyses of Internally-Pressurised Filament Wound Composite Tubes Using Artificial Neural Network Metamodels. Mater. Today Commun. 2022, 31, 103627. [Google Scholar] [CrossRef]
- Hopmann, C.; Magura, N.; Müller, R.; Schneider, D.; Fischer, K. Impact of Winding Parameters on the Fiber Bandwidth in the Cylindrical Area of a Hydrogen Pressure Vessel for Generating a Digital Twin. Polym. Compos. 2022, 43, 1577–1589. [Google Scholar] [CrossRef]
- Qarssis, Y.; Nachtane, M.; Karine, A.; Abichou, A.; Faik, A.; Tarfaoui, M. Machine Learning-Based Analytical Approach for Mechanical Analysis of Composite Hydrogen Storage Tanks under Internal Pressure. Int. J. Hydrogen Energy 2024, 89, 1440–1453. [Google Scholar] [CrossRef]
- Alves, M.P.; Gul, W.; Cimini Junior, C.A.; Ha, S.K. A Review on Industrial Perspectives and Challenges on Material, Manufacturing, Design and Development of Compressed Hydrogen Storage Tanks for the Transportation Sector. Energies 2022, 15, 5152. [Google Scholar] [CrossRef]
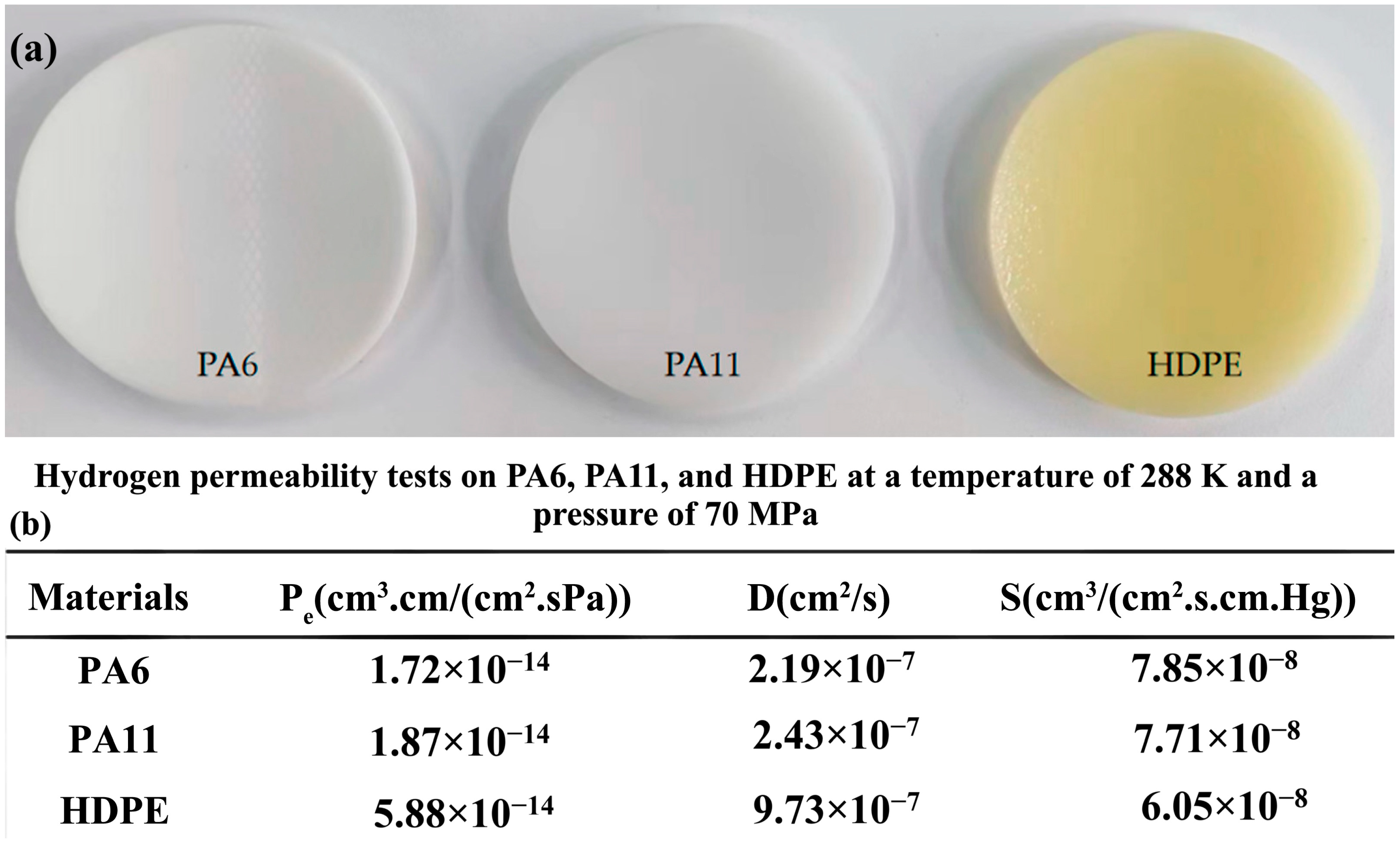


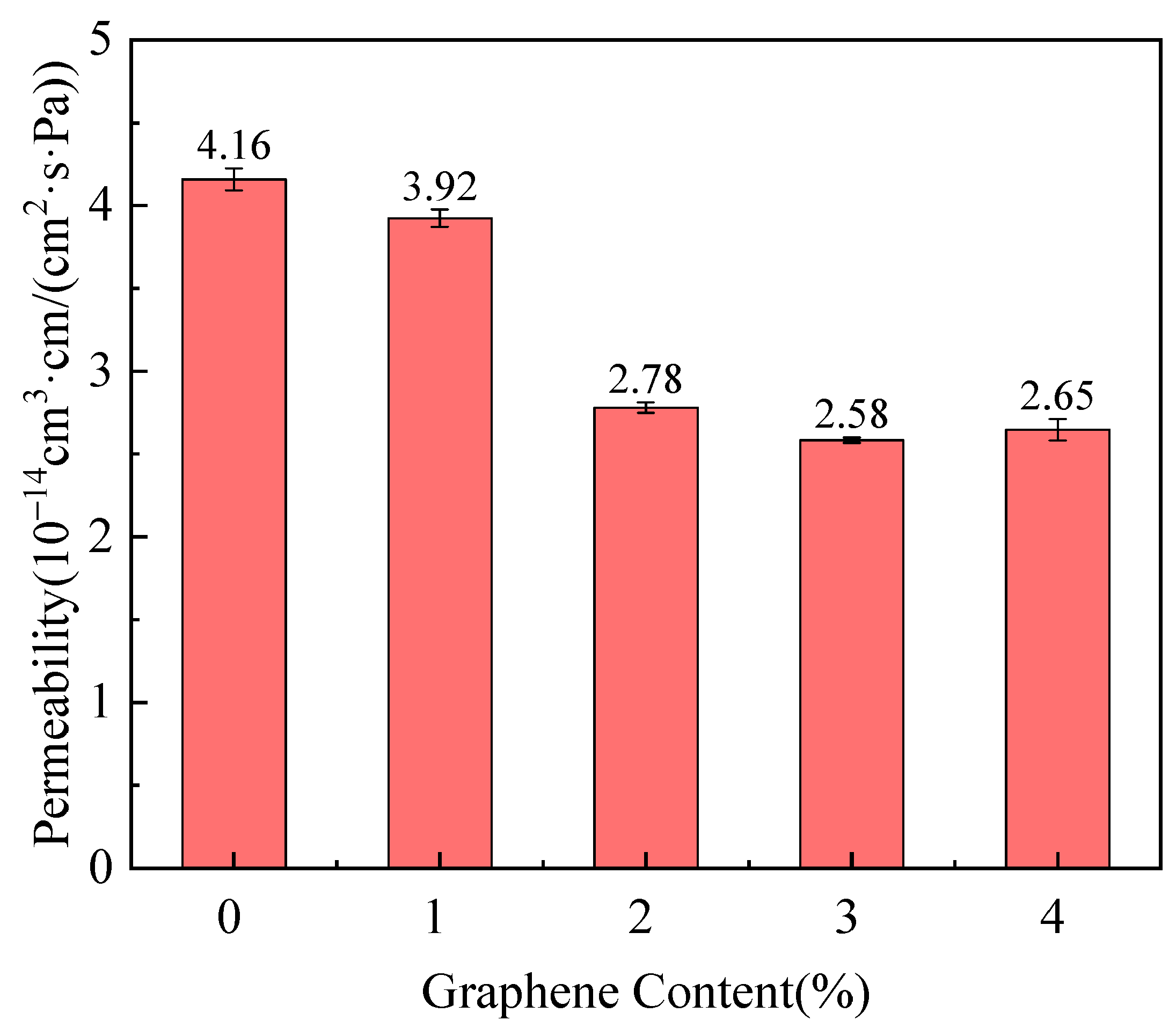
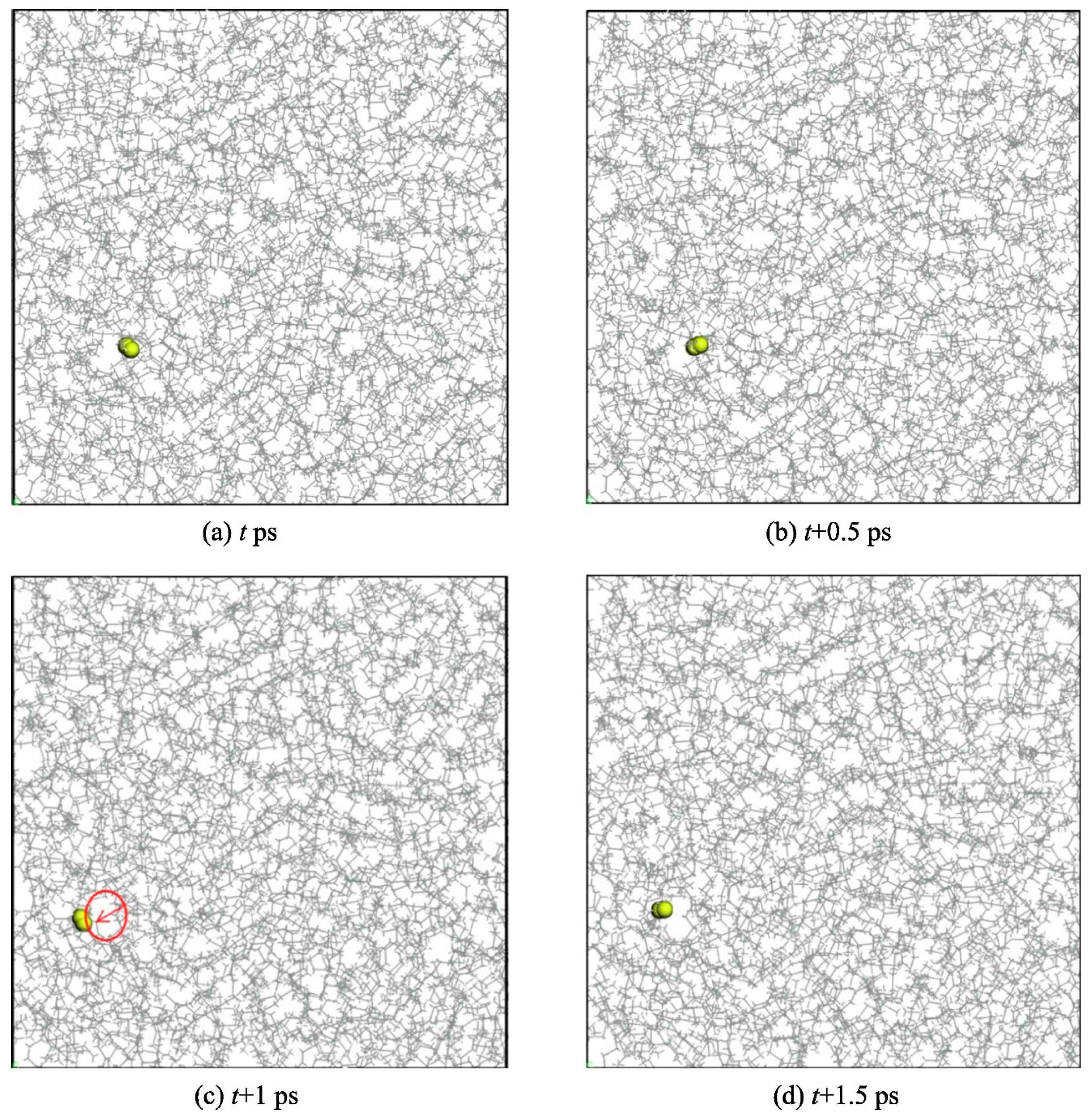

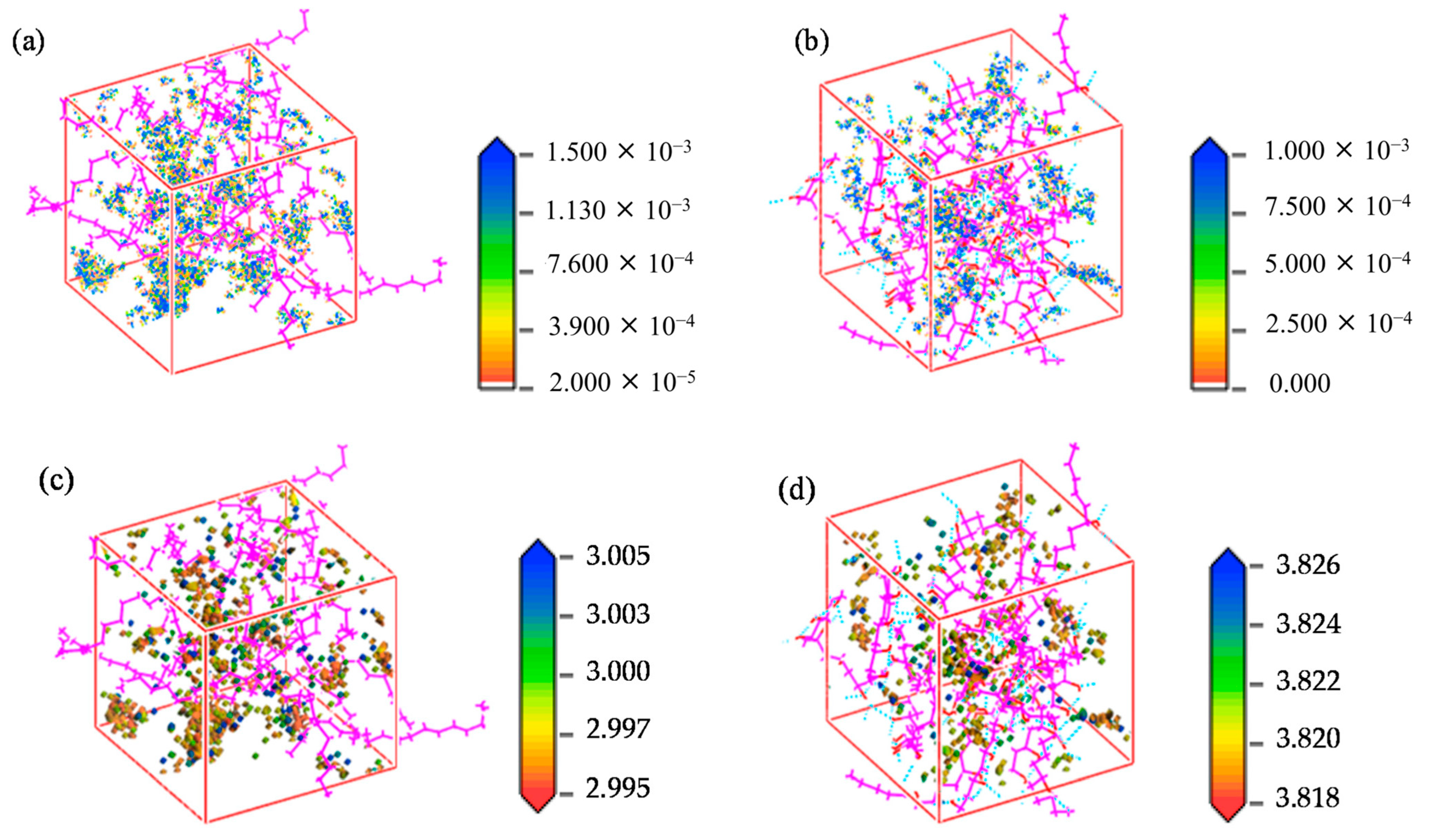



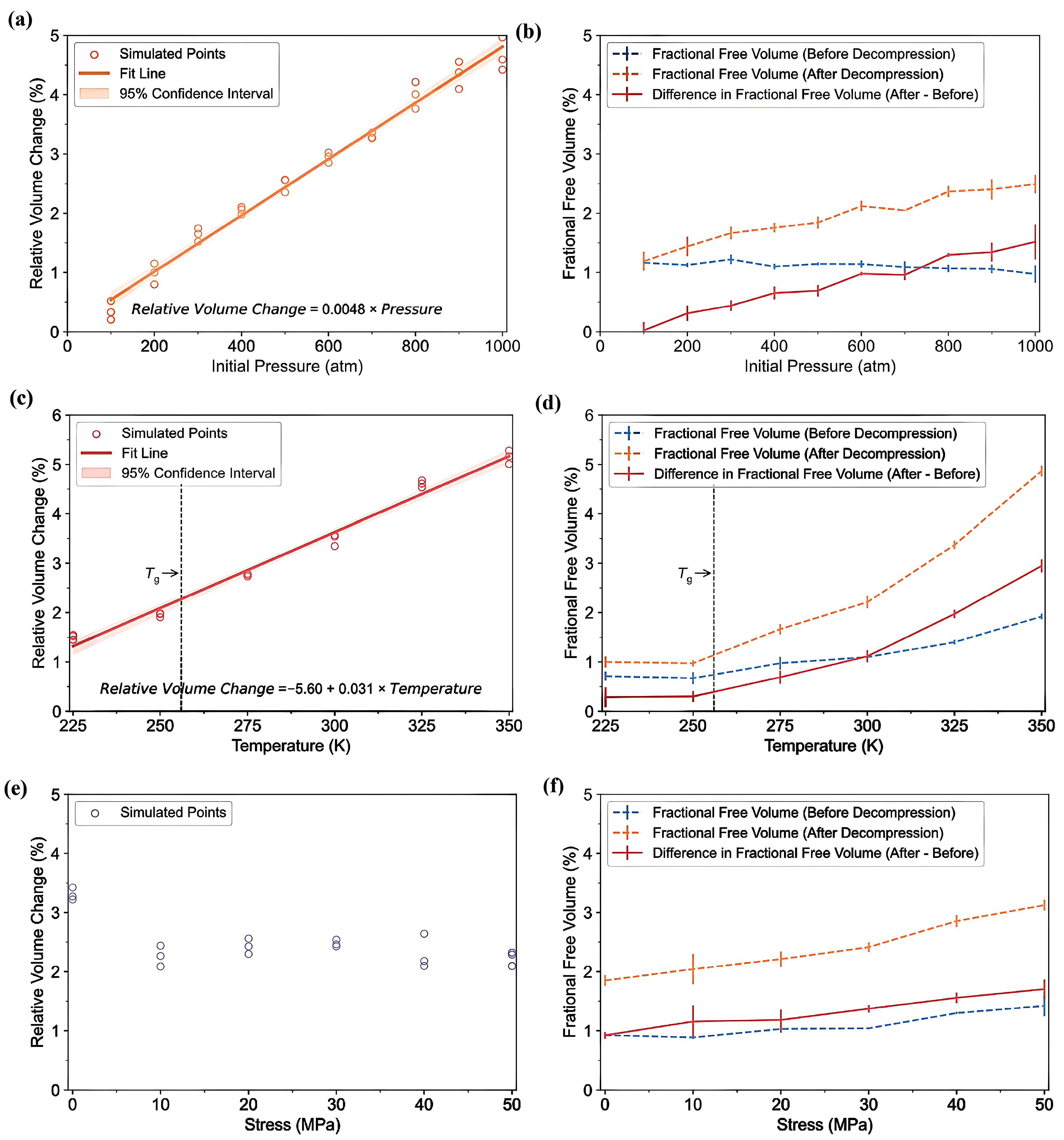

| Matrices | S (cm3/cm3.Pa).10−7 | D (cm2/s).10−6 | P (cm3.cm/cm2.s.Pa).10−13 |
| 288 K and 0.1 MPa | |||
| PA6/3%OMMT | 2.23 | 1.72 | 5.56 |
| PA6/4%OMMT | 1.93 | 1.65 | 3.18 |
| PA6/5%OMMT | 1.26 | 1.48 | 1.85 |
| PA6/6%OMMT | 1.55 | 1.58 | 2.54 |
| PA6/7%OMMT | 1.60 | 2.21 | 3.54 |
| 328 K | |||
| PA6/3%OMMT | 2.58 | 5.38 | 10.39 |
| PA6/4%OMMT | 1.51 | 3.58 | 5.41 |
| PA6/5%OMMT | 1.02 | 2.66 | 2.71 |
| PA6/6%OMMT | 1.17 | 3.15 | 3.63 |
| PA6/7%OMMT | 1.32 | 4.82 | 6.43 |
| 5%PA6/OMMT at 288 K | |||
| 0.1 MPa | 1.26 | 1.48 | 1.86 |
| 41.6 MPa | 1.38 | 1.74 | |
| 52 MPa | 3.41 | 4.30 | |
| 60 MPa | 4.09 | 5.15 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Dagdag, O.; Kim, H. Recent Advances in the Hydrogen Gas Barrier Performance of Polymer Liners and Composites for Type IV Hydrogen Storage Tanks: Fabrication, Properties, and Molecular Modeling. Polymers 2025, 17, 1231. https://doi.org/10.3390/polym17091231
Dagdag O, Kim H. Recent Advances in the Hydrogen Gas Barrier Performance of Polymer Liners and Composites for Type IV Hydrogen Storage Tanks: Fabrication, Properties, and Molecular Modeling. Polymers. 2025; 17(9):1231. https://doi.org/10.3390/polym17091231
Chicago/Turabian StyleDagdag, Omar, and Hansang Kim. 2025. "Recent Advances in the Hydrogen Gas Barrier Performance of Polymer Liners and Composites for Type IV Hydrogen Storage Tanks: Fabrication, Properties, and Molecular Modeling" Polymers 17, no. 9: 1231. https://doi.org/10.3390/polym17091231
APA StyleDagdag, O., & Kim, H. (2025). Recent Advances in the Hydrogen Gas Barrier Performance of Polymer Liners and Composites for Type IV Hydrogen Storage Tanks: Fabrication, Properties, and Molecular Modeling. Polymers, 17(9), 1231. https://doi.org/10.3390/polym17091231






