Elastic Characterization of Acrylate-Based Liquid Crystal Elastomers
Abstract
1. Introduction
2. Materials and Methods
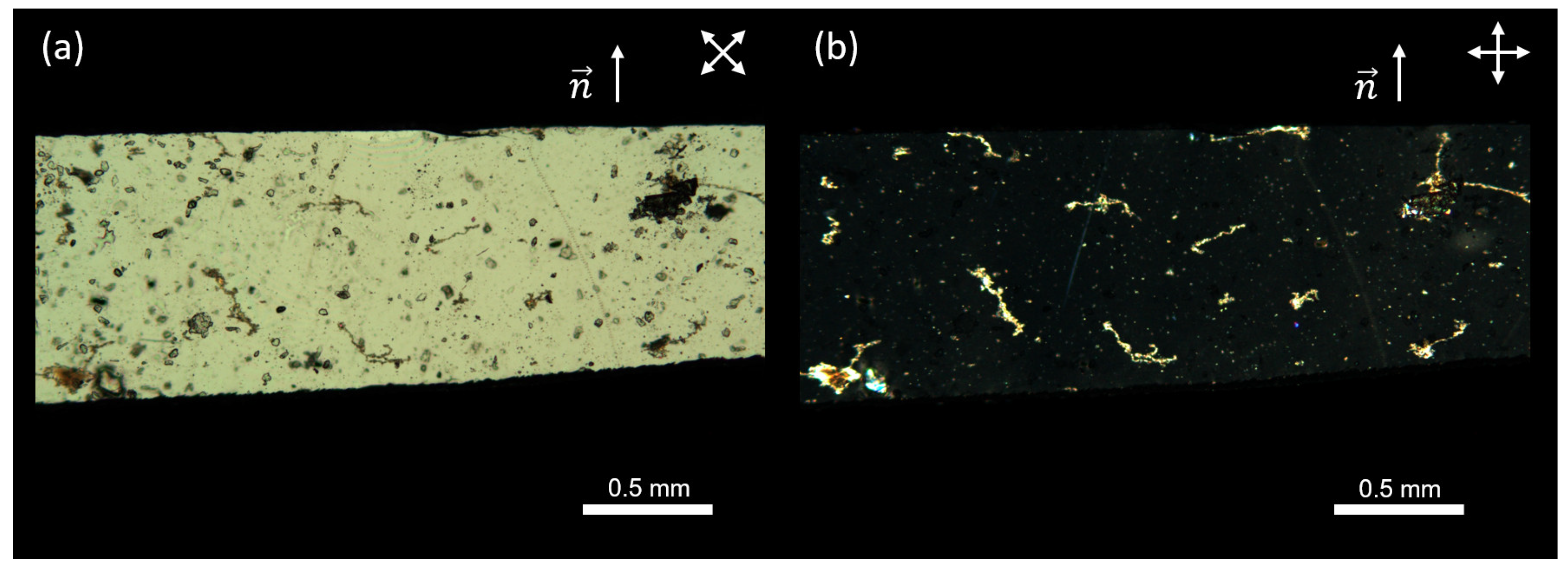
2.1. LCE Sample Fabrication
2.2. Tensile Experiments
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
Determining Elastic Constants for Nematic LCE
References
- de Gennes, P.G. Physique moléculaire—Réflexions sur un type de polymères nématiques. Comptes Rendus L’académie Sci. B 1975, 281, 101–103. [Google Scholar]
- Finkelmann, H.; Koch, H.J.; Rehage, G. Investigations on liquid crystalline polysiloxanes 3, Liquid crystalline elastomers—A new type of liquid crystalline material. Makromol. Chem. Rapid Commun. 1981, 2, 317–322. [Google Scholar] [CrossRef]
- Küpfer, J.; Finkelmann, H. Nematic liquid single crystal elastomers. Die Makromol. Chem. Rapid Commun. 1991, 12, 717–726. [Google Scholar] [CrossRef]
- Yu, Y.; Ikeda, T. Soft actuators based on liquid-crystalline elastomers. Angew. Chem. 2006, 45, 5416–5418. [Google Scholar] [CrossRef]
- He, Q.; Wang, Z.; Wang, Y.; Minori, A.; Tolley, M.T.; Cai, S. Electrically controlled liquid crystal elastomer-based soft tubular actuator with multimodal actuation. Sci. Adv. 2019, 5, eaax5746. [Google Scholar] [CrossRef] [PubMed]
- Ohm, C.; Brehmer, M.; Zentel, R. Liquid crystalline elastomers as actuators and sensors. Adv. Mater. 2010, 22, 3366–3387. [Google Scholar] [CrossRef] [PubMed]
- Mistry, D.; Traugutt, N.A.; Yu, K.; Yakacki, C.M. Processing and reprocessing liquid crystal elastomer actuators. J. Appl. Phys. 2021, 129, 130901. [Google Scholar] [CrossRef]
- Sun, D.; Zhang, J.; Li, H.; Shi, Z.; Meng, Q.; Liu, S.; Chen, J.; Liu, X. Toward Application of Liquid Crystalline Elastomer for Smart Robotics: State of the Art and Challenges. Polymers 2021, 13, 1889. [Google Scholar] [CrossRef] [PubMed]
- Ula, S.W.; Traugutt, N.A.; Volpe, R.H.; Patel, R.R.; Yu, K.; Yakacki, C.M. Liquid crystal elastomers: An introduction and review of emerging technologies. Liq. Cryst. Rev. 2018, 6, 78–107. [Google Scholar] [CrossRef]
- Schuhladen, S.; Preller, F.; Rix, R.; Petsch, S.; Zentel, R.; Zappe, H. Iris-Like Tunable Aperture Employing Liquid-Crystal Elastomers. Adv. Mater. 2014, 26, 7247–7251. [Google Scholar]
- Brannum, M.T.; Steele, A.M.; Venetos, M.C.; Korley, L.T.J.; Wnek, G.E.; White, T.J. Light Control with Liquid Crystalline Elastomers. Adv. Opt. Mater. 2019, 7, 1801683. [Google Scholar] [CrossRef]
- Brommel, F.; Kramer, D.; Finkelmann, H. Preparation of Liquid Crystalline Elastomers. Adv. Polym. Sci. 2012, 250, 1–48. [Google Scholar]
- Herbert, K.M.; Fowler, H.E.; McCracken, J.M.; Schlafmann, K.R.; Koch, J.A.; White, T.J. Synthesis and alignment of liquid crystalline elastomers. Nat. Rev. Mater. 2022, 7, 23–38. [Google Scholar] [CrossRef]
- Urayama, K.; Arai, Y.O.; Takigawa, T. Volume Phase Transition of Monodomain Nematic Polymer Networks in Isotropic Solvents Accompanied by Anisotropic Shape Variation. Macromolecules 2005, 38, 3469–3474. [Google Scholar] [CrossRef]
- Mistry, D.; Morgan, P.B.; Clamp, J.H.; Gleeson, H.F. New insights into the nature of semi-soft elasticity and ‘mechanical-Fréedericksz transitions’ in liquid crystal elastomers. Soft Matter 2018, 14, 1301–1310. [Google Scholar] [CrossRef]
- Mistry, D.; Connell, S.D.; Mickthwaite, S.L.; Morgan, P.B.; Clamp, J.H.; Gleeson, H.F. Coincident molecular auxeticity and negative order parameter in a liquid crystal elastomer. Nat. Commun. 2018, 9, 5095. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.; Raistrick, T.; Street, A.; Reynolds, M.; Liu, Y.; Gleeson, H.F. Direct Observation of Biaxial Nematic Order in Auxetic Liquid Crystal Elastomers. Materials 2023, 16, 393. [Google Scholar] [CrossRef] [PubMed]
- Verwey, G.C.; Warner, M.; Terentjev, E.M. Elastic instability and stripe domains in liquid crystalline elastomers. J. Phys. II 1996, 6, 1273. [Google Scholar] [CrossRef]
- Warner, M.; Terentjev, E.M. Liquid Crystal Elastomers. In International Series of Monographs on Physics; Oxford University Press: Oxford, UK, 2003. [Google Scholar]
- Raistrick, T.; Zhang, Z.; Mistry, D.; Mattson, J.; Gleeson, H.F. Understanding the physics of the auxetic response in a liquid crystal elastomer. Phys. Rev. Res. 2021, 3, 023191. [Google Scholar] [CrossRef]
- Golubovic, L.; Lubensky, T.C. Nonlinear elasticity of amorphous solids. Phys. Rev. Lett. 1989, 63, 1082. [Google Scholar] [CrossRef]
- Lubensky, T.C.; Mukhopadhyay, R.; Radzihovsky, L.; Xing, X. Symmetries and elasticity of nematic gels. Phys. Rev. E 2002, 66, 011702. [Google Scholar] [CrossRef] [PubMed]
- Finkelmann, H.; Greve, A.; Warner, M. The elastic anisotropy of nematic elastomers. Eur. J. Phys. E 2001, 5, 281. [Google Scholar] [CrossRef]
- Mihai, L.A.; Goriely, A. A plate theory for nematic liquid crystalline solids. J. Mech. Phys. Solids 2020, 144, 104101. [Google Scholar] [CrossRef]
- Mistry, D.; Gleeson, H.F. Mechanical deformations of a liquid crystal elastomer at director angles between 0 degrees and 90 degrees: Deducing an empirical model encompassing anisotropic nonlinearity. J. Polym. Sci. Part B-Polym. Phys. 2019, 57, 1367–1377. [Google Scholar] [CrossRef]
- Azoug, A.; Vasconcellos, V.; Dooling, J.; Saed, M.; Yakacki, C.; Nguyen, T. Viscoelasticity of the polydomain-monodomain transition in main-chain liquid crystal elastomers. Polymer 2016, 98, 165–171. [Google Scholar] [CrossRef]
- Saed, M.O.; Elmadih, W.; Terentjev, A.; Chronopoulos, D.; Williamson, D.; Terentjev, E.M. Impact damping and vibration attenuation in nematic liquid crystal elastomers. Nat. Commun. 2021, 12, 6676. [Google Scholar] [CrossRef] [PubMed]
- Oh, S.W.; Guo, T.; Kuenstler, A.S.; Hayward, R.; Palffy-Muhoray, P.; Zheng, X. Measuring the five elastic constants of a nematic liquid crystal elastomer. Liq. Cryst. 2020, 48, 511–520. [Google Scholar] [CrossRef]
- Sargsyan, M.; Gevorgyan, G.; Hakobyan, M.; Hakobyan, R. Determination of the Elasticity Coefficients for Nematic Liquid Crystal Elastomers. Crystals 2022, 12, 1654. [Google Scholar] [CrossRef]
- Berrow, S.R.; Raistrick, T.; Mandle, R.J.; Gleeson, H.F. Structure-Property Relationships in Auxetic Liquid Crystal Elastomers—The Effect of Spacer Length. Polymers 2024, 16, 1957. [Google Scholar] [CrossRef]
- Thomsen, D.L.; Keller, P.; Naciri, J.; Pink, R.; Jeon, H.; Shenoy, D.; Ratna, B.R. Liquid crystal elastomers with mechanical properties of a muscle. Macromolecules 2001, 34, 868–5875. [Google Scholar] [CrossRef]
- Beyer, P.; Terentjev, E.M.; Zentel, R. Monodomain liquid crystal main chain elastomers by photocrosslinking. Macromol. Rapid Commun. 2007, 28, 1485–1490. [Google Scholar] [CrossRef]
- de Gennes, P.G. Liquid Crystals of One and Two-Dimensional Order; Helfrich, W., Heppke, G., Eds.; Springer: New York, NY, USA, 1980. [Google Scholar]



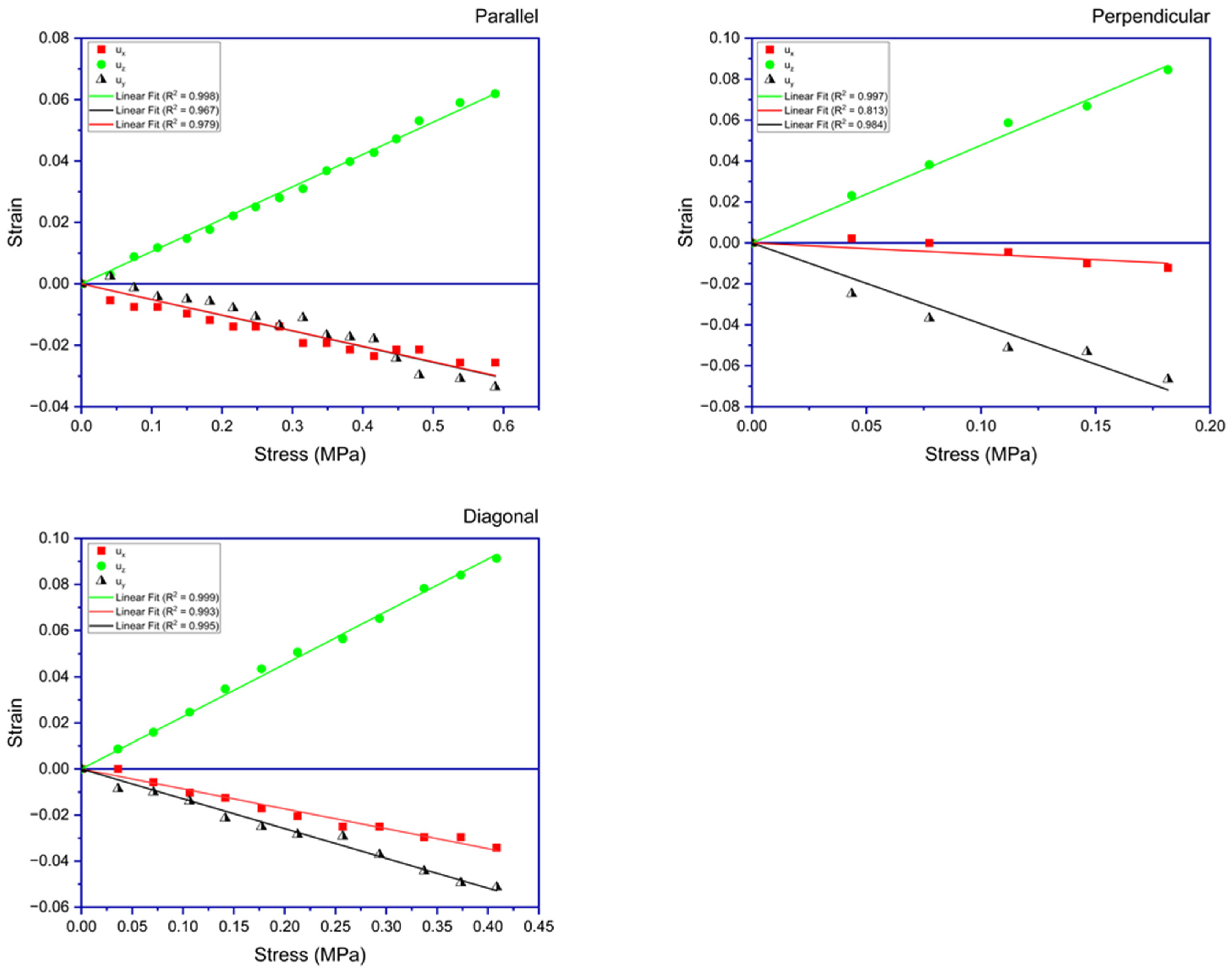

| (MPa) | (MPa) | (MPa) | ||||
|---|---|---|---|---|---|---|
| 9.48 | 4.39 | 2.096 | 0.486 | 0.48 | 0.831 | 0.112 |
| (MPa) | (MPa) | (MPa) | (MPa) | (MPa) |
|---|---|---|---|---|
| 0.572 | 15.042 | −0.11 | −0.052 | 8.23 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gevorgyan, G.S.; Sargsyan, M.L.; Hakobyan, M.R.; Reynolds, M.; Gleeson, H.F.; Hakobyan, R.S. Elastic Characterization of Acrylate-Based Liquid Crystal Elastomers. Polymers 2025, 17, 614. https://doi.org/10.3390/polym17050614
Gevorgyan GS, Sargsyan ML, Hakobyan MR, Reynolds M, Gleeson HF, Hakobyan RS. Elastic Characterization of Acrylate-Based Liquid Crystal Elastomers. Polymers. 2025; 17(5):614. https://doi.org/10.3390/polym17050614
Chicago/Turabian StyleGevorgyan, Gevorg S., Maksim L. Sargsyan, Mariam R. Hakobyan, Matthew Reynolds, Helen F. Gleeson, and Rafik S. Hakobyan. 2025. "Elastic Characterization of Acrylate-Based Liquid Crystal Elastomers" Polymers 17, no. 5: 614. https://doi.org/10.3390/polym17050614
APA StyleGevorgyan, G. S., Sargsyan, M. L., Hakobyan, M. R., Reynolds, M., Gleeson, H. F., & Hakobyan, R. S. (2025). Elastic Characterization of Acrylate-Based Liquid Crystal Elastomers. Polymers, 17(5), 614. https://doi.org/10.3390/polym17050614







