Fabrication of Polycaprolactone-Based Polyurethanes with Enhanced Thermal Stability
Abstract
1. Introduction
2. Materials and Methods
2.1. Materials
2.2. Surface Modification of TiO2 Nanoparticles
2.3. Synthesis of Pure Polyurethane and Composites
2.4. Characterization
3. Results and Discussion
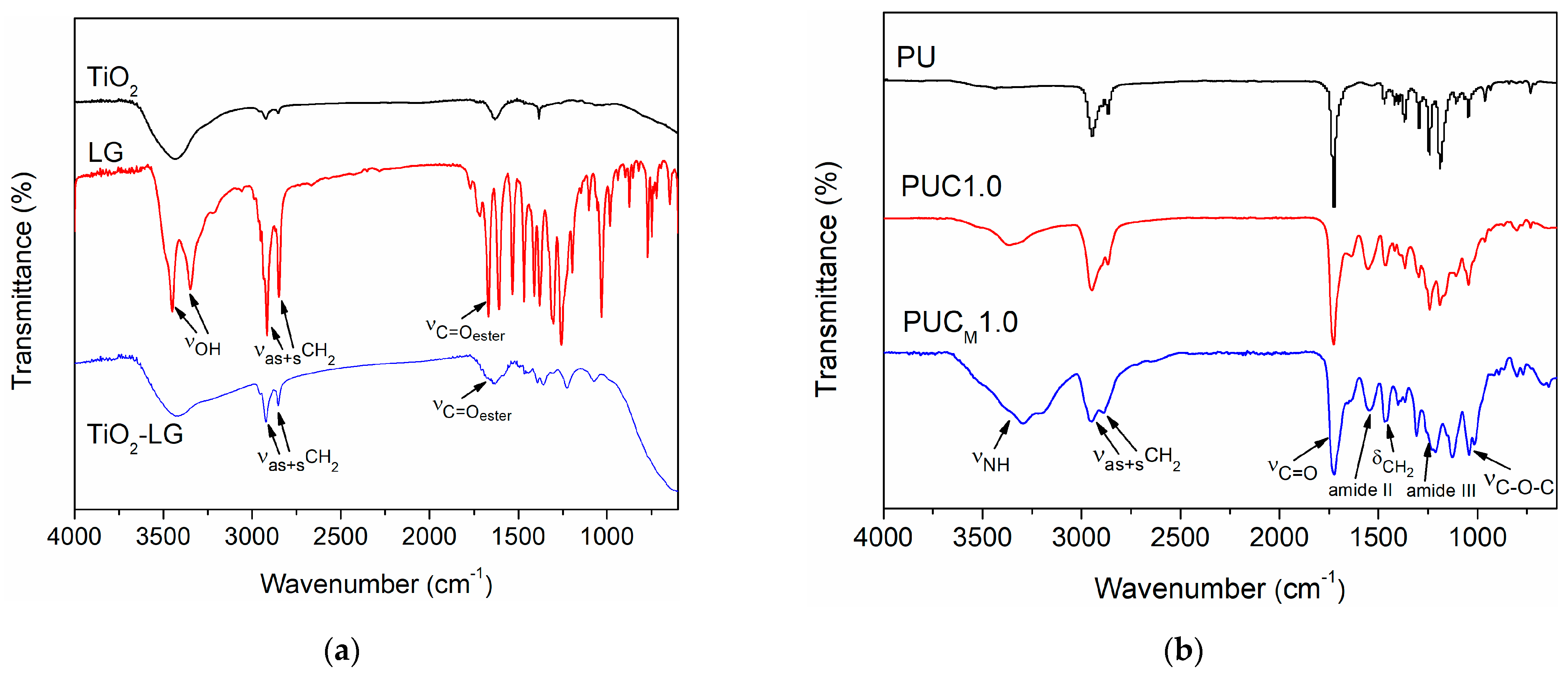
3.1. FTIR Spectroscopy
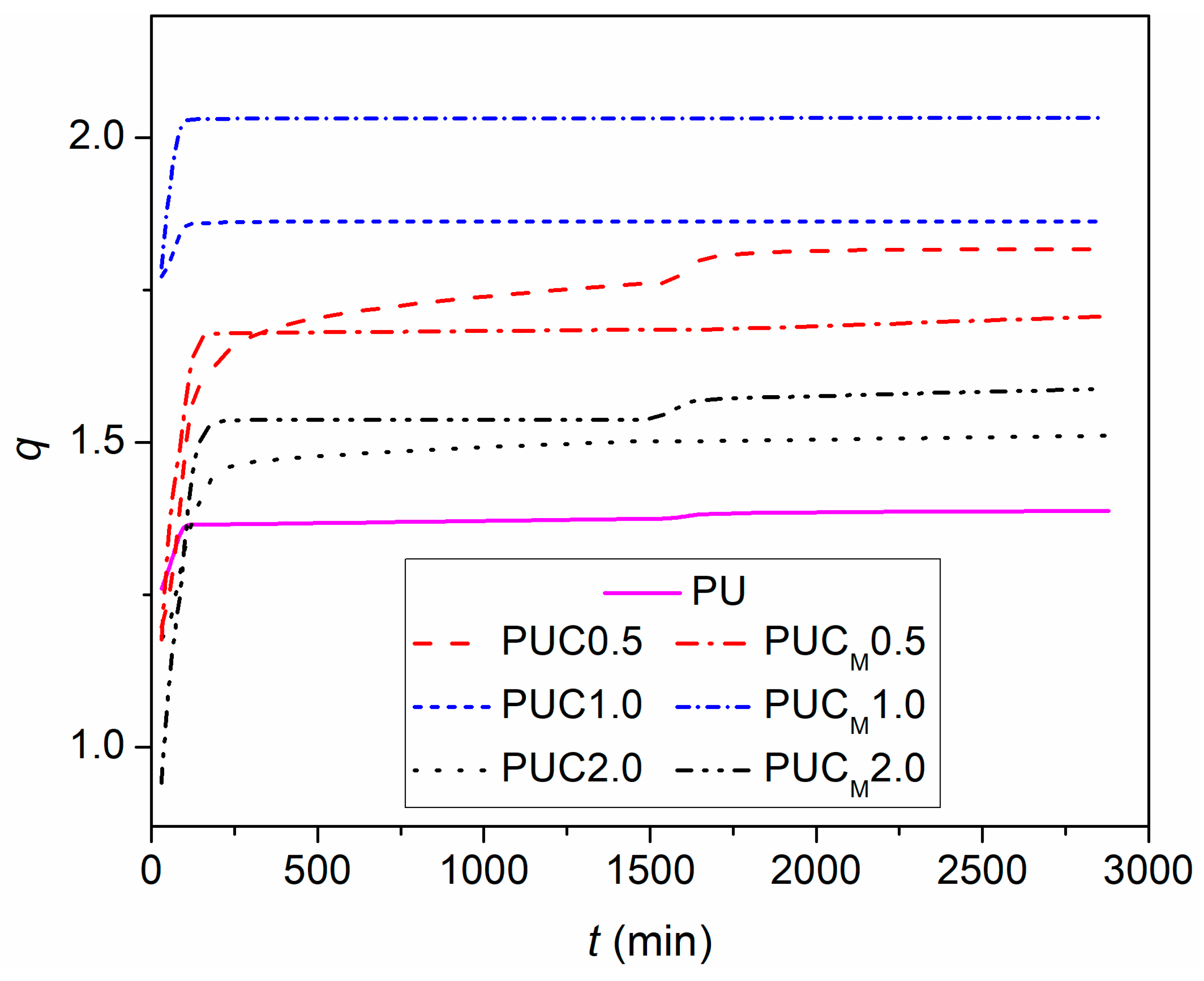
3.2. Swelling Measurements
3.3. SEM Analysis
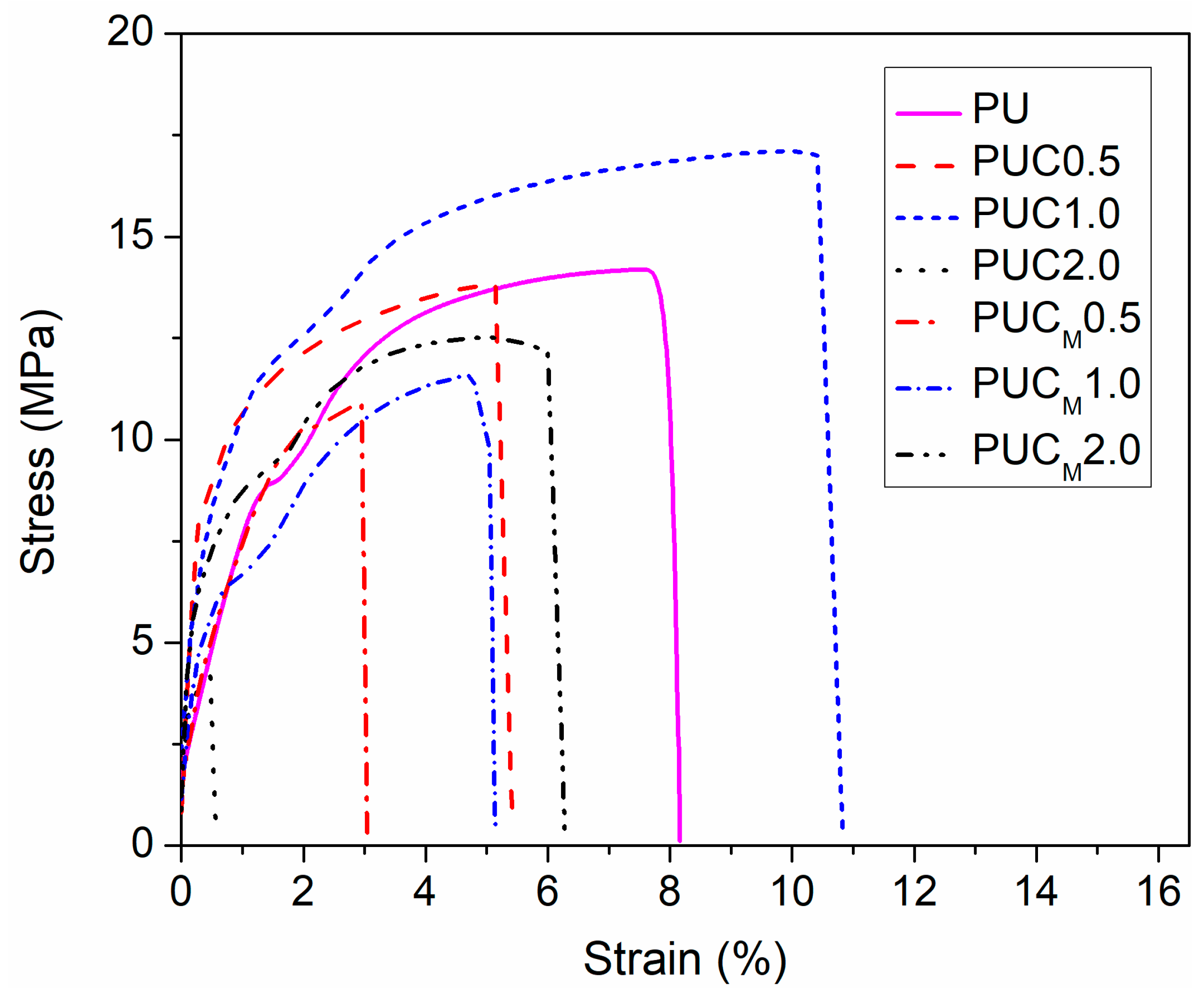
3.4. Mechanical Properties
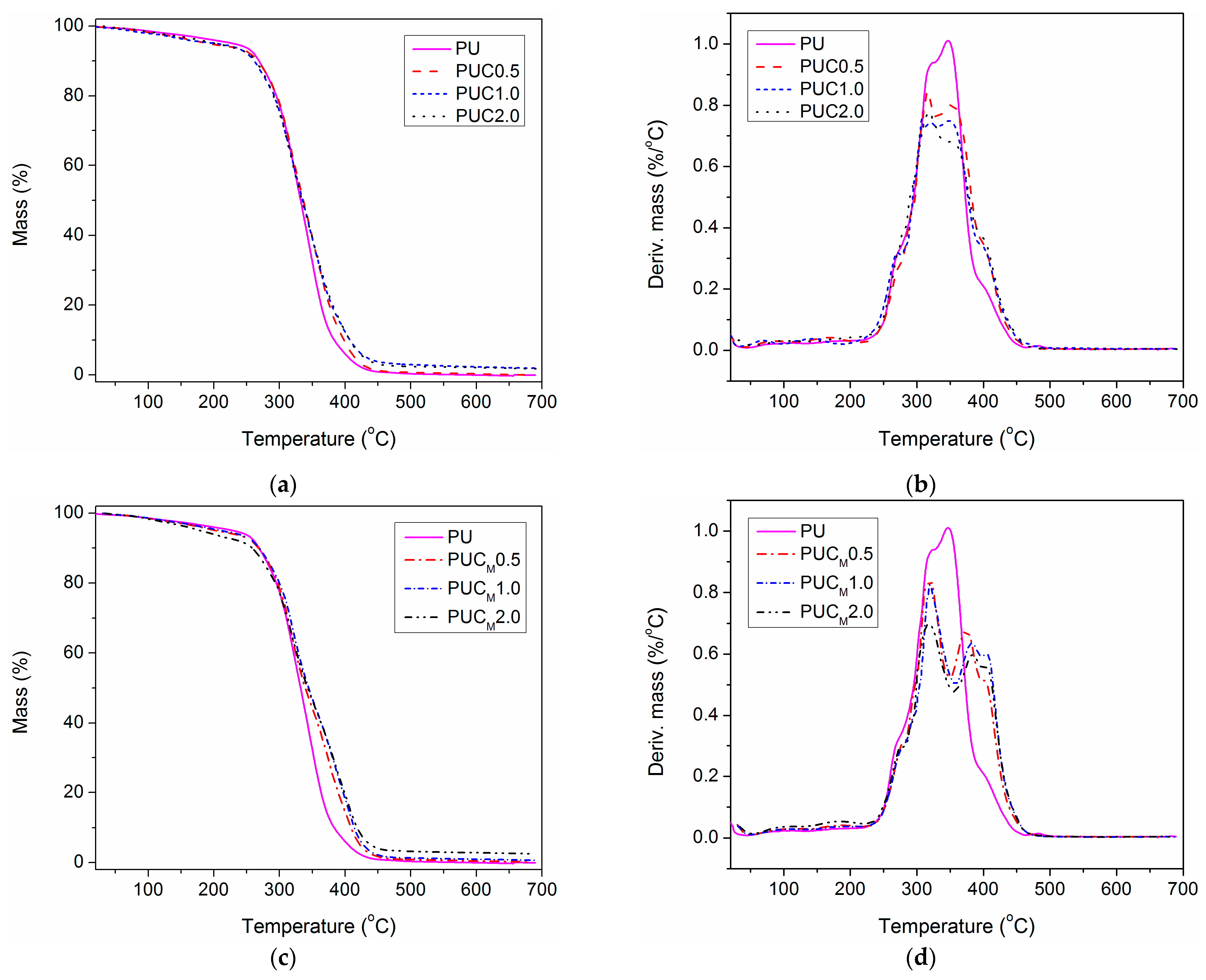
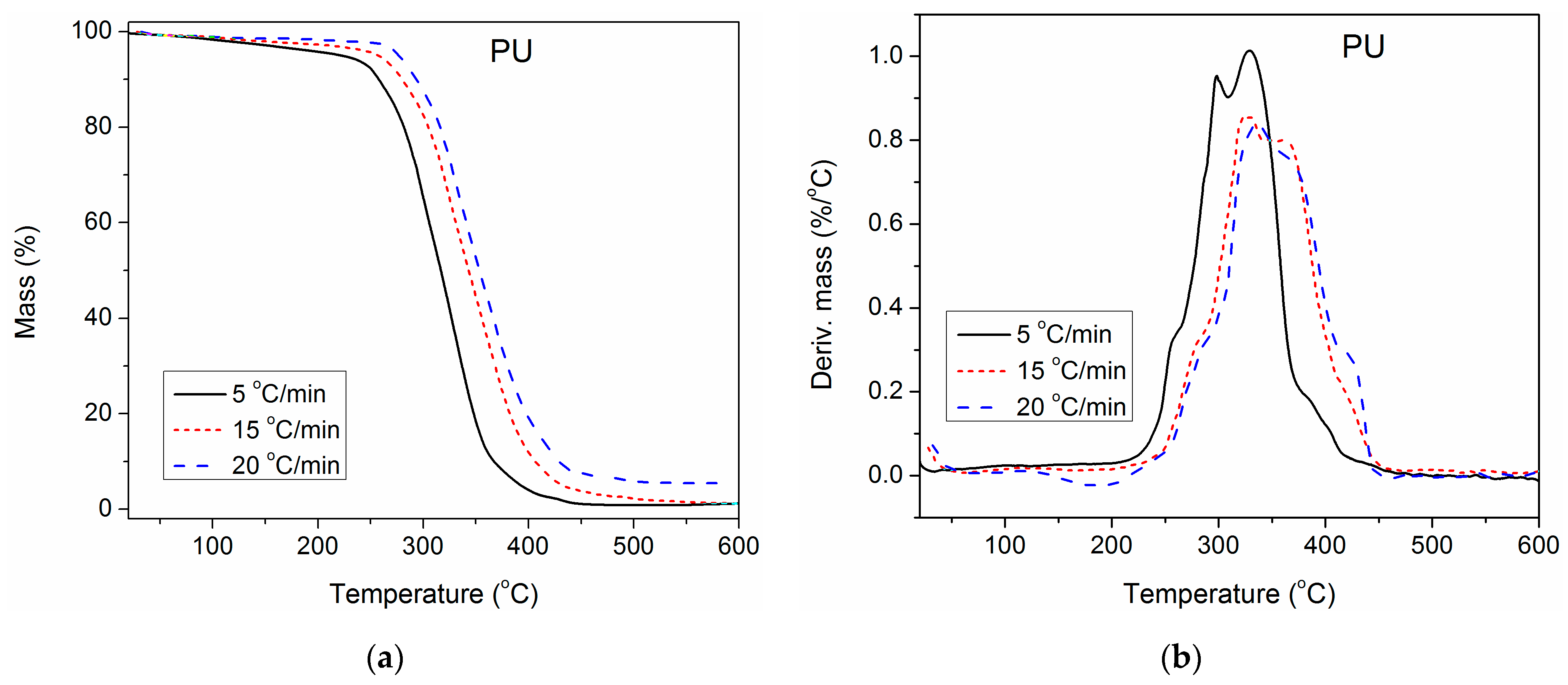
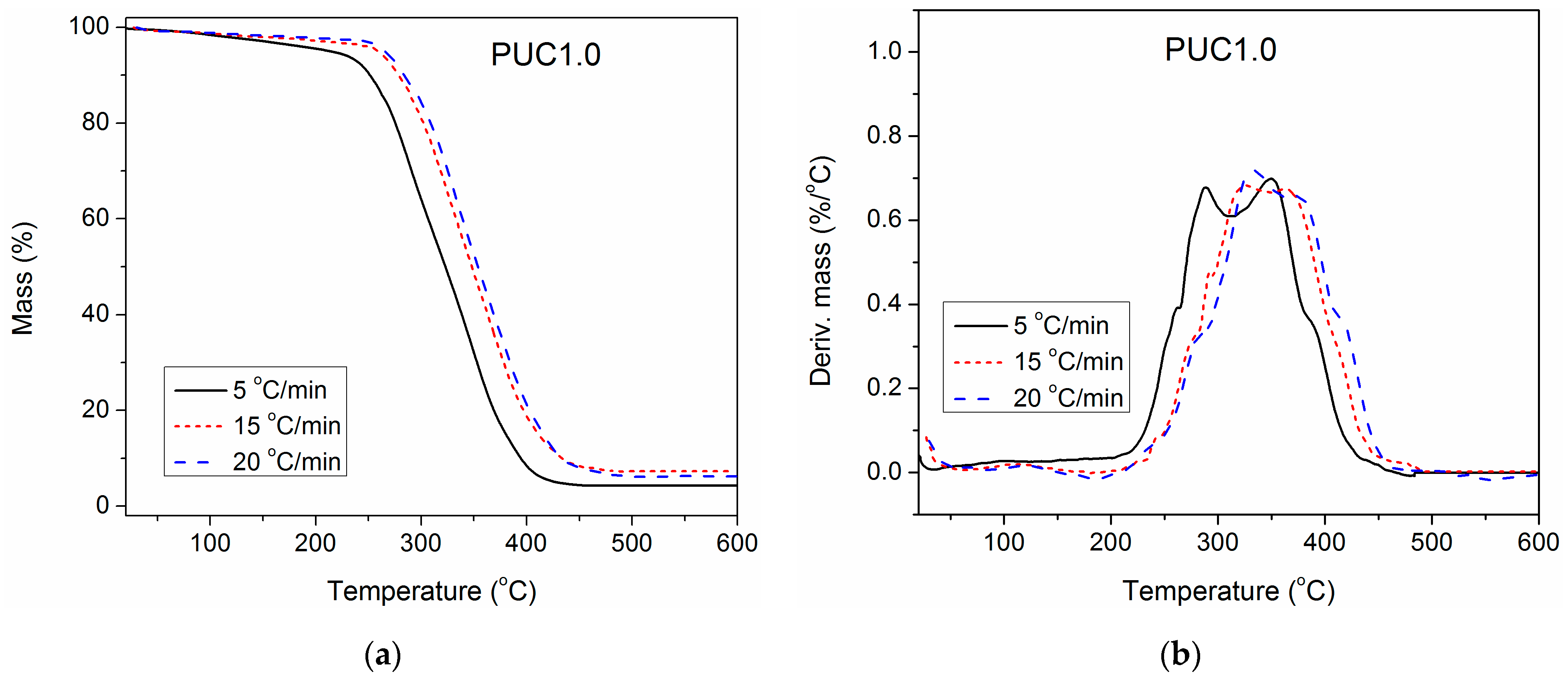
3.5. Thermal Stability
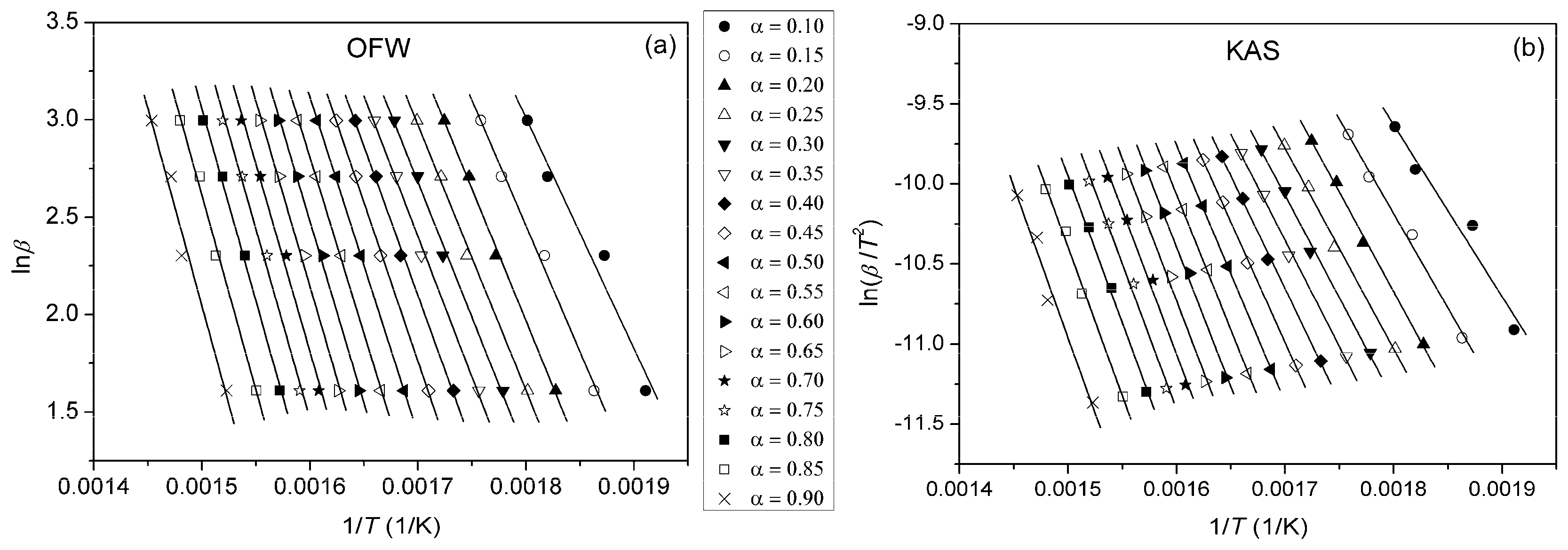
3.6. Estimation of the Apparent Activation Energy of Thermal Degradation
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Mahanwar, D.P. A brief discussion on advances in polyurethane applications. Adv. Ind. Eng. Polym. Res. 2020, 3, 93–101. [Google Scholar] [CrossRef]
- Yilgor, I.; Yilgor, E.; Wilkes, G.L. Critical parameters in designing segmented polyurethanes and their effect on morphology and properties: A comprehensive review. Polymer 2015, 58, A1–A36. [Google Scholar] [CrossRef]
- Chattopadhyay, D.K.; Raju, K.V.S.N. Structural engineering of polyurethane coatings for high performance applications. Prog. Polym. Sci. 2007, 32, 352–418. [Google Scholar] [CrossRef]
- Cheng, B.-X.; Gao, W.-C.; Ren, X.-M.; Ouyang, X.-Y.; Zhao, Y.; Zhao, H.; Wu, W.; Huang, C.-X.; Liu, Y.; Liu, X.-Y.; et al. A review of microphase separation of polyurethane: Characterization and applications. Polym. Test. 2022, 107, 107489. [Google Scholar] [CrossRef]
- Chattopadhyay, D.K.; Webster, D.C. Thermal stability and flame retardancy of polyurethanes. Prog. Polym. Sci. 2009, 34, 1068–1133. [Google Scholar] [CrossRef]
- Petrović, Z.S.; Zavargo, Z.; Flyn, J.H.; Macknight, W.J. Thermal degradation of segmented polyurethanes. J. Appl. Polym. Sci. 1994, 51, 1087–1095. [Google Scholar] [CrossRef]
- Oenema, J.; Liu, H.; De Coensel, N.; Eschenbacher, A.; Van de Vijver, R.; Weng, J.; Li, L.; Wang, C.; Van Geem, K.M. Review on the pyrolysis products and thermal decomposition mechanisms of polyurethanes. J. Anal. Appl. Pyrolysis 2022, 168, 105723. [Google Scholar] [CrossRef]
- Džunuzović, J.V.; Stefanović, I.S.; Džunuzović, E.S.; Dapčević, A.; Šešlija, S.I.; Balanč, B.D.; Lama, G.C. Polyurethane networks based on polycaprolactone and hyperbranched polyester: Structural, thermal and mechanical investigation. Prog. Org. Coat. 2019, 137, 105305. [Google Scholar] [CrossRef]
- Ristić, I.; Krakovsky, I.; Janić, T.; Cakić, S.; Miletić, A.; Jotanović, M.; Radusin, T. The influence of the nanofiller on thermal properties of thermoplastic polyurethane elastomers. J. Therm. Ana. Calorim. 2018, 134, 895–901. [Google Scholar] [CrossRef]
- Kausar, A. Polyurethane nanocomposite coatings: State of the art and perspectives. Polym. Int. 2018, 67, 1470–1477. [Google Scholar] [CrossRef]
- Sun, J.-T.; Li, J.-W.; Tsou, C.-H.; Pang, J.-C.; Chung, R.-J.; Chiu, C.-W. Polyurethane/nanosilver-doped halloysite nanocomposites: Thermal, mechanical properties, and antibacterial properties. Polymers 2020, 12, 2729. [Google Scholar] [CrossRef] [PubMed]
- Meera, K.M.S.; Sankar, R.M.; Paul, J.; Jaisankar, S.N.; Mandal, A.B. The influence of applied silica nanoparticles on a bio-renewable castor oil based polyurethane nanocomposite and its physicochemical properties. Phys. Chem. Chem. Phys. 2014, 16, 9276–9288. [Google Scholar] [CrossRef]
- Jakhmola, S.; Das, S.; Dutta, K. Emerging research trends in the field of polyurethane and its nanocomposites: Chemistry, synthesis, characterization, application in coatings and future perspectives. J. Coat. Technol. Res. 2024, 21, 137–172. [Google Scholar] [CrossRef]
- Ding, G.; Tai, H.; Chen, C.; Sun, C.; Tang, Z.; Cheng, G.; Wan, X.; Wang, Z. The effect of silicon dioxide nanoparticle-covered graphene oxide on mechanical properties, thermal stability and rheological performance of thermoplastic polyurethanes. J. Appl. Polym. Sci. 2022, 139, 51947. [Google Scholar] [CrossRef]
- Alam, M.; Alandis, N.M.; Zafar, F.; Sharmin, E.; Al-Mohammadi, Y.M. Polyurethane-TiO2 nanocomposite coatings from sunflower-oil-based amide diol as soft segment. J. Macromol. Sci.-Pure Appl. Chem. 2018, 55, 698–708. [Google Scholar] [CrossRef]
- Allami, T.; Alamiery, A.; Nassir, M.H.; Kadhum, A.H. Investigating physio-thermo-mechanical properties of polyurethane and thermoplastics nanocomposite in various applications. Polymers 2021, 13, 2467. [Google Scholar] [CrossRef]
- Hornak, J.; Černohous, J.; Prosr, P.; Rous, P.; Trnka, P.; Baran, A.; Hardoň, Š. A Comprehensive study of polyurethane potting compounds doped with magnesium oxide nanoparticles. Polymers 2023, 15, 1532. [Google Scholar] [CrossRef]
- Gurunathan, T.; Chung, J.S. Physicochemical properties of amino−silane-terminated vegetable oil-Based Waterborne polyurethane nanocomposites. ACS Sustain. Chem. Eng. 2016, 4, 4645–4653. [Google Scholar] [CrossRef]
- Shu, B.; Liu, Z.; Liu, Z.; Li, P.; Li, X.; Yang, S.; Chen, H.; Liu, J.; Yang, Z.; Hu, Y. Preparation of SiO2-decorated GO sheets and their influences on the properties of castor oil-based polyurethane coating film. Prog. Org. Coat. 2023, 175, 107382. [Google Scholar] [CrossRef]
- Chen, X.; Mao, S.S. Titanium dioxide nanomaterials: Synthesis, properties, modifications, and applications. Chem. Rev. 2007, 107, 2891–2959. [Google Scholar] [CrossRef]
- Bokhimi, X.; Morales, A.; Aguilar, M.; Toledo-Antonio, J.A.; Pedraza, F. Local order in titania polymorphs. Int. J. Hydrogen Energy 2001, 26, 1279–1287. [Google Scholar] [CrossRef]
- Nam, Y.; Lim, J.H.; Ko, K.C.; Lee, J.Y. Photocatalytic activity of TiO2 nanoparticles: A theoretical aspect. J. Mater. Chem. A 2019, 7, 13833–13859. [Google Scholar] [CrossRef]
- Li, Q.; Mahendra, S.; Lyon, D.Y.; Brunet, L.; Liga, M.V.; Li, D.; Alvarez, P.J.J. Antimicrobial nanomaterials for water disinfection and microbial control: Potential applications and implications. Water Res. 2008, 42, 4591–4602. [Google Scholar] [CrossRef] [PubMed]
- Thakur, N.; Thakur, N.; Kumar, A.; Thakur, V.K.; Kalia, S.; Arya, V.; Kumar, A.; Kumar, S.; Kyzas, G.Z. A critical review on the recent trends of photocatalytic, antibacterial, antioxidant and nanohybrid applications of anatase and rutile TiO2 nanoparticles. Sci. Total Environ. 2024, 914, 169815. [Google Scholar] [CrossRef] [PubMed]
- Chen, X.D.; Wang, Z.; Liao, Z.F.; Mai, Y.L.; Zhang, M.Q. Roles of anatase and rutile TiO2 nanoparticles in photooxidation of polyurethane. Polym. Test. 2007, 26, 202–208. [Google Scholar] [CrossRef]
- Pavlićević, J.; Špirková, M.; Aroguz, A.; Jovičić, M.; Kojić, D.; Govedarica, D.; Ikonić, B. The effect of TiO2 particles on thermal properties of polycarbonate-based polyurethane nanocomposite films. J. Therm. Anal. Calorim. 2019, 138, 2043–2055. [Google Scholar] [CrossRef]
- da Silva, V.; Santos, L.M.; Subda, S.M.; Ligabue, R.; Seferin, M.; Carone, C.L.P.; Einloft, S. Synthesis and characterization of polyurethane/titanium dioxide nanocomposites obtained by in situ polymerization. Polym. Bull. 2013, 70, 1819–1833. [Google Scholar] [CrossRef]
- Yang, B.; Liang, Q.; Wang, L. Investigation of self-adaptive breathable nano-TiO2/PU membrane with water vapor transmission. AATCC J. Res. 2021, 8, 72–77. [Google Scholar] [CrossRef]
- Behniafar, H.; Alimohammadi, M.; Malekshahinezhad, K. Transparent and flexible films of new segmented polyurethane nanocomposites incorporated by NH2-functionalized TiO2 nanoparticles. Prog. Org. Coat. 2015, 88, 150–154. [Google Scholar] [CrossRef]
- Vuković, J. Synthesis and Characterization of Aliphatic Hyperbranched Polyesters. Ph.D. Thesis, University of Osnabrück, Osnabrück, Germany, 2006. [Google Scholar]
- Marand, Å.; Dahlin, J.; Karlsson, D.; Skarping, G.; Dalene, M. Determination of technical grade isocyanates used in the production of polyurethane plastics. J. Environ. Monit. 2004, 6, 606–614. [Google Scholar] [CrossRef]
- Flory, P.J.; Rehner, J. Statistical mechanics of cross-linked polymer networks II. Swelling. J. Chem. Phys. 1943, 11, 521–526. [Google Scholar] [CrossRef]
- Stefanović, I.S.; Džunuzović, J.V.; Džunuzović, E.S.; Dapčević, A.; Šešlija, S.I.; Balanč, B.D.; Dobrzyńska-Mizera, M. Composition-property relationship of polyurethane networks based on polycaprolactone diol. Polym. Bull. 2021, 78, 7103–7128. [Google Scholar] [CrossRef]
- Džunuzović, E.S.; Džunuzović, J.V.; Marinković, A.D.; Marinović-Cincović, M.T.; Jeremić, K.B.; Nedeljković, J.M. Influence of surface modified TiO2 nanoparticles by gallates on the properties of PMMA/TiO2 nanocomposites. Eur. Polym. J. 2012, 48, 1385–1393. [Google Scholar] [CrossRef]
- Džunuzović, E.S.; Džunuzović, J.V.; Radoman, T.S.; Marinović-Cincović, M.T.; Nikolić, L.B.; Jeremić, K.B.; Nedeljković, J.M. Characterization of in situ prepared nanocomposites of PS and TiO2 nanoparticles surface modified with alkyl gallates: Effect of alkyl chain length. Polym. Compos. 2013, 34, 399–407. [Google Scholar] [CrossRef]
- Radoman, T.S.; Džunuzović, J.V.; Jeremić, K.B.; Grgur, B.N.; Miličević, D.S.; Popović, I.G.; Džunuzović, E.S. Improvement of epoxy resin properties by incorporation of TiO2 nanoparticles surface modified with gallic acid esters. Mater. Des. 2014, 62, 158–167. [Google Scholar] [CrossRef]
- Anancharoenwong, E.; Chueangchayaphan, W.; Rakkapao, N.; Marthosa, S.; Chaisrikhwun, B. Thermo-mechanical and antimicrobial properties of natural rubber-based polyurethane nanocomposites for biomedical applications. Polym. Bull. 2021, 78, 833–848. [Google Scholar] [CrossRef]
- Mi, H.-Y.; Jing, X.; Yilmaz, G.; Hagerty, B.S.; Enriquez, E.; Turng, L.-S. In situ synthesisof polyurethane scaffolds with tunable properties by controlled crosslinking of tri-block copolymer and polycaprolactone triol for tissue regeneration. Chem. Eng. J. 2018, 348, 786–798. [Google Scholar] [CrossRef]
- Stroea, L.; Chibac-Scutaru, A.-L.; Melinte, V. Aliphatic polyurethane elastomers quaternized with silane-functionalized TiO2 nanoparticles with UV-shielding features. Polymers 2021, 13, 1318. [Google Scholar] [CrossRef]
- Guo, H.; Zhang, C.; Zhou, X.; Chen, J.; Yu, L.; Li, H.; Wang, X. Boron-based mildew preventive and ultraviolet absorbent modification of waterborne polyurethane coatings on laminated bamboo. Coatings 2023, 13, 687. [Google Scholar] [CrossRef]
- Moussout, H.; Ahlafi, H.; Aazza, M.; Bourakhouadar, M. Kinetics and mechanism of the thermal degradation of biopolymers chitin and chitosan using thermogravimetric analysis. Polym. Degrad. Stab. 2016, 130, 1–9. [Google Scholar] [CrossRef]
- Li, Q.; Wang, Y.; Fan, M.; Zhang, J.; Cheng, J. Thermal degradation kinetics of poly(acrylate/α-methyl styrene) copolymers. Polym. Degrad. Stab. 2016, 128, 158–164. [Google Scholar] [CrossRef]
- Li, M.; Jiang, L.; He, J.-J.; Sun, J.-H. Kinetic triplet determination and modified mechanism function construction for thermo-oxidative degradation of waste polyurethane foam using conventional methods and distributed activation energy model method. Energy 2019, 175, 1–13. [Google Scholar] [CrossRef]
- Agnieszka Gola, A.; Knysak, T.; Mucha, I.; Musiał, W. Synthesis, thermogravimetric analysis, and kinetic study of poly-N-isopropylacrylamide with varied initiator content. Polymers 2023, 15, 2427. [Google Scholar] [CrossRef] [PubMed]
- Džunuzović, J.V.; Stefanović, I.S.; Džunuzović, E.S.; Basagni, A.; Marega, C. Study on thermal stability of water-based polyurethanes derived from hydroxypropyl terminated poly(dimethylsiloxane). J. Polym. Res. 2024, 31, 139. [Google Scholar] [CrossRef]
- Omrani, A.; Hasankola, S.M.M. Kinetic study on solid state thermal degradation of epoxy nanocomposite containing octasilane polyhedral oligomeric silsesquioxane. J. Non-Cryst. Solids 2012, 358, 1656–1666. [Google Scholar] [CrossRef]
- Ozawa, T. A new method of analyzing thermogravimetric data. Bull. Chem. Soc. Jpn. 1965, 38, 1881–1886. [Google Scholar] [CrossRef]
- Flynn, J.H.; Wall, L.A. A quick, direct method for the determination of activation energy from thermogravimetric data. Polym. Lett. 1966, 4, 323–328. [Google Scholar] [CrossRef]
- Kissinger, H.E. Reaction kinetics in differential thermal analysis. Anal. Chem. 1957, 29, 1702–1706. [Google Scholar] [CrossRef]
- Akahira, T.; Sunose, T. Method of determining activation deterioration constant of electrical insulating materials, Joint convention of four electrical institutes. Res. Rep. Chiba. Inst. Technol. (Sci. Technol.) 1971, 16, 22–31. [Google Scholar]
- Friedman, H.L. Kinetics of thermal degradation of char-forming plastics from thermogravimetry. Application to a phenolic plastic. J. Polym. Sci. Part C Polym. Symp. 1964, 6, 183–195. [Google Scholar] [CrossRef]
- Vyazovkin, S.V.; Lesnikovich, A.I. An approach to the solution of the inverse kinetic problem in the case of complex processes. Part1. Methods employing a series of thermoanalytical curves. Thermochim. Acta 1990, 165, 273–280. [Google Scholar] [CrossRef]
- Vyazovkin, S. Isoconversional methods: The many uses of variable activation energy. Thermochim. Acta 2024, 733, 179701. [Google Scholar] [CrossRef]
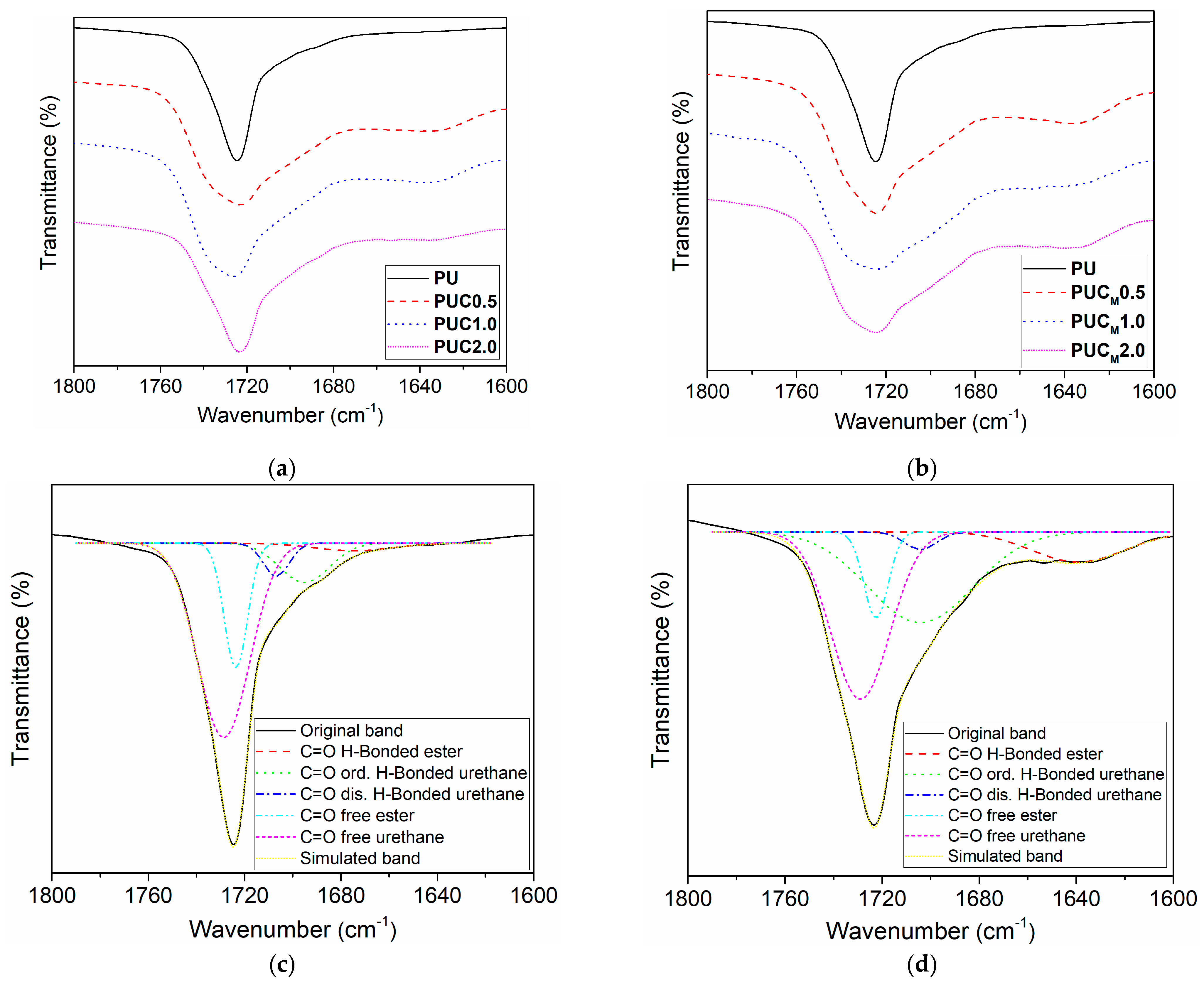




| Sample | Area (%) | ||||
|---|---|---|---|---|---|
| C=O free urethane 1729 cm−1 | C=O free ester 1722 cm−1 | C=O disorder H-bonded urethane 1705 cm−1 | C=O order H-bonded urethane 1695 cm−1 | C=O H-bonded ester 1640 cm−1 | |
| PU | 40.4 | 23.6 | 8.5 | 15.6 | 11.9 |
| PUC0.5 | 36.9 | 11.2 | 4.6 | 31.2 | 16.1 |
| PUC1.0 | 36.7 | 11.3 | 4.2 | 30.8 | 17.0 |
| PUC2.0 | 33.2 | 12.5 | 5.7 | 29.8 | 18.8 |
| PUCM0.5 | 15.4 | 19.6 | 20.1 | 35.2 | 9.7 |
| PUCM1.0 | 9.9 | 27.8 | 15.9 | 33.8 | 12.6 |
| PUCM2.0 | 6.7 | 36.5 | 14.9 | 28.7 | 13.2 |
| Sample | ρ (g cm−3) | V | ν × 104 (mol cm−3) | Mc (g mol−1) | Gel% (wt.%) |
|---|---|---|---|---|---|
| PU | 1.011 ± 0.010 | 0.320 ± 0.025 | 6.25 ± 0.29 | 1620 ± 170 | 79.0 ± 0.2 |
| PUC0.5 | 1.017 ± 0.016 | 0.233 ± 0.012 | 2.93 ± 0.31 | 3470 ± 453 | 70.0 ± 0.1 |
| PUC1.0 | 1.024 ± 0.015 | 0.244 ± 0.031 | 3.38 ± 0.42 | 3030 ± 391 | 67.1 ± 0.1 |
| PUC2.0 | 1.031 ± 0.017 | 0.298 ± 0.020 | 5.66 ± 0.45 | 1820 ± 211 | 77.1 ± 0.2 |
| PUCM0.5 | 1.022 ± 0.016 | 0.213 ± 0.009 | 2.45 ± 0.37 | 4160 ± 550 | 62.2 ± 0.1 |
| PUCM1.0 | 1.030 ±0.018 | 0.259 ± 0.028 | 3.99 ± 0.32 | 2580 ± 198 | 74.5 ± 0.2 |
| PUCM2.0 | 1.037 ± 0.020 | 0.265 ± 0.011 | 4.31 ± 0.45 | 2400 ± 215 | 74.6 ± 0.1 |
| Sample | Tensile Strength (MPa) | Elongation at Break (%) | Young’s Modulus (MPa) |
|---|---|---|---|
| PU | 14.2 ± 1.2 | 8.2 ± 0.6 | 678.2 ± 37.1 |
| PUC0.5 | 13.8 ± 1.0 | 5.4 ± 0.4 | 522.9 ± 29.5 |
| PUC1.0 | 17.1 ± 1.5 | 10.8 ± 0.7 | 663.4 ± 33.4 |
| PUC2.0 | 4.2 ± 0.3 | 0.6 ± 0.1 | 529.9 ± 28.1 |
| PUCM0.5 | 10.9 ± 0.8 | 3.0 ± 0.2 | 574.9 ± 30.0 |
| PUCM1.0 | 11.6 ± 0.9 | 5.1 ± 0.3 | 563.3 ± 26.8 |
| PUCM2.0 | 12.5 ± 1.1 | 6.3 ± 0.5 | 597.0 ± 31.3 |
| Sample | T10 (°C) | T50 (°C) | Tsh1 (°C) | Tmax1 (°C) | Tmax2 (°C) | Tsh2 (°C) |
|---|---|---|---|---|---|---|
| PU | 269 | 332 | 270 | 323 | 347 | 401 |
| PUC0.5 | 265 | 335 | 274 | 316 | 352 | 403 |
| PUC1.0 | 262 | 335 | 270 | 318 | 351 | 400 |
| PUC2.0 | 263 | 335 | 279 | 318 | 352 | 400 |
| PUCM0.5 | 268 | 339 | 278 | 317 | 373 | 402 |
| PUCM1.0 | 269 | 344 | 277 | 319 | 382 | 405 |
| PUCM2.0 | 258 | 344 | 277 | 319 | 383 | 405 |
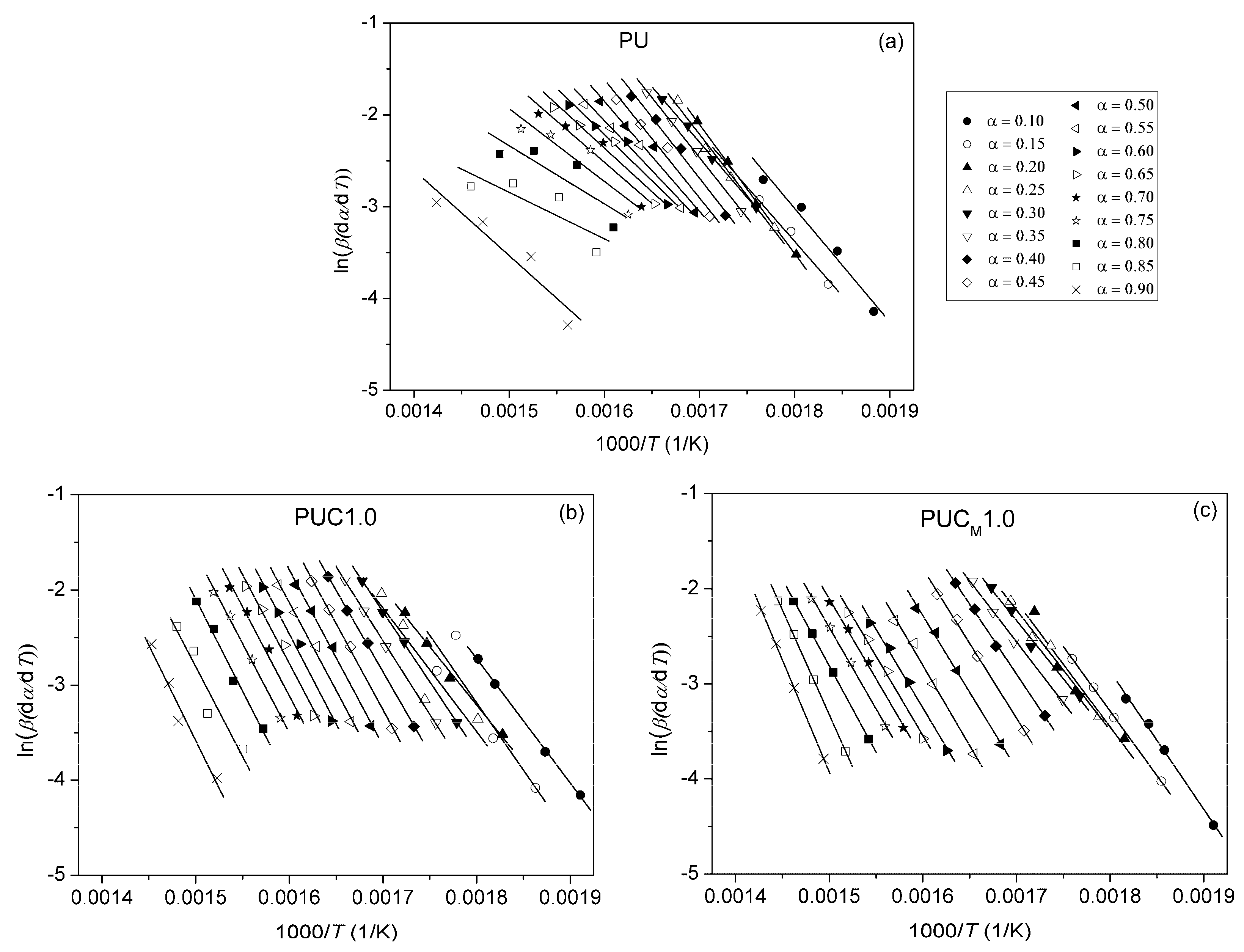
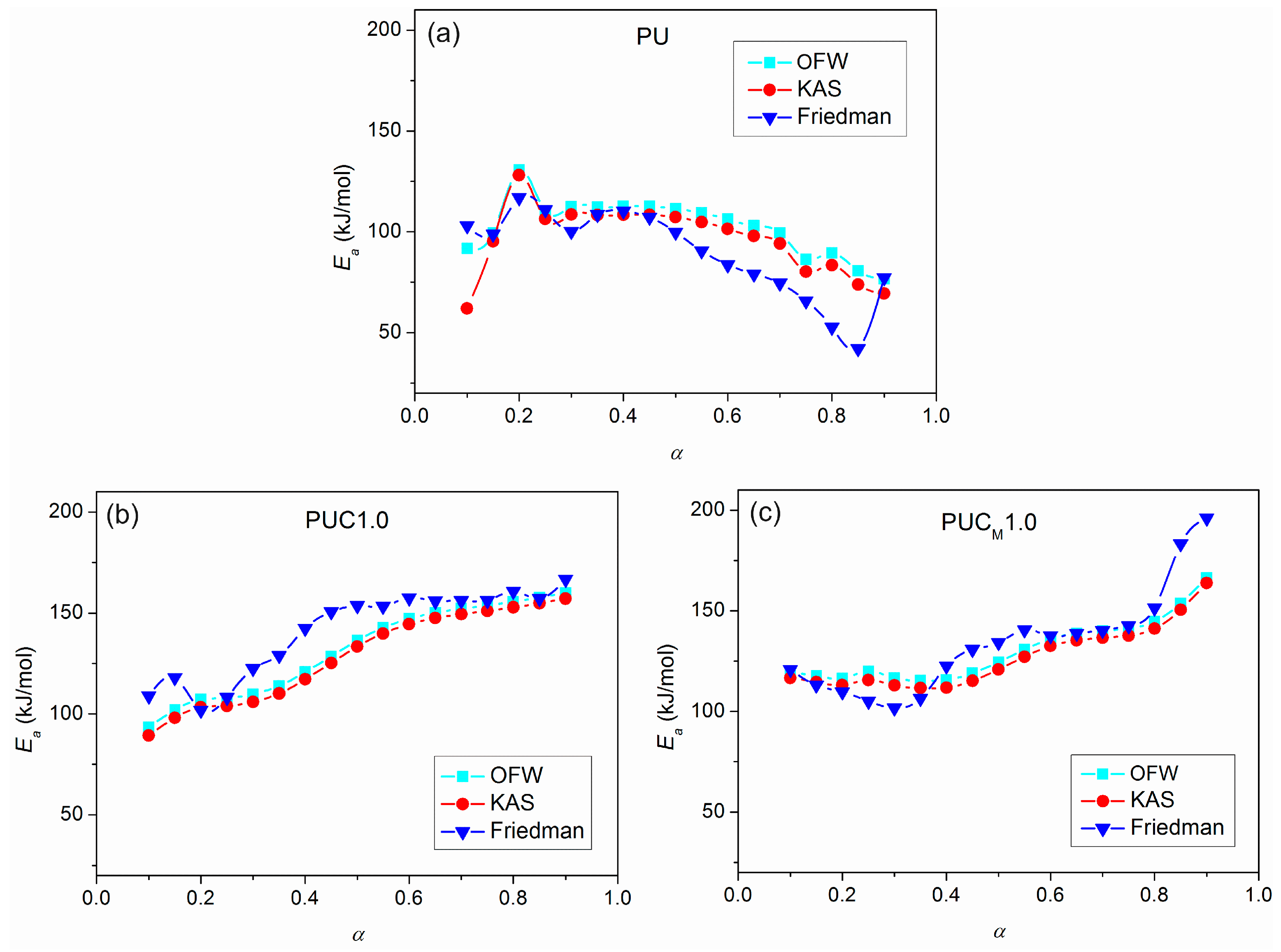
| α | Ea (OFW) (kJ/mol) | R2 (OFW) | Ea (KAS) (kJ/mol) | R2 (KAS) | Ea (Friedman) (kJ/mol) | R2 (Friedman) |
|---|---|---|---|---|---|---|
| PU | ||||||
| 0.10 < α′ < 0.25 | 108 ± 15 | 0.9621 | 98 ± 23 | 0.9521 | 107 ± 7 | 0.9797 |
| 0.30 < α″ < 0.90 | 101 ± 13 | 0.9936 | 96 ± 14 | 0.9841 | 84 ± 21 | 0.9298 |
| PUC1.0 | ||||||
| 0.10 < α′ < 0.30 | 104 ± 6 | 0.9889 | 100 ± 6 | 0.9846 | 112 ± 7 | 0.9516 |
| 0.35 < α″ < 0.90 | 139 ± 14 | 0.9957 | 136 ± 15 | 0.9966 | 149 ± 9 | 0.9548 |
| PUCM1.0 | ||||||
| 0.10 < α′ < 0.40 | 117 ± 1 | 0.9955 | 114 ± 2 | 0.9932 | 111 ± 7 | 0.9831 |
| 0.45 < α″ < 0.90 | 143 ± 13 | 0.9973 | 141 ± 13 | 0.9964 | 153 ± 21 | 0.9957 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Džunuzović, J.V.; Stefanović, I.S.; Džunuzović, E.S.; Kovač, T.S.; Malenov, D.P.; Basagni, A.; Marega, C. Fabrication of Polycaprolactone-Based Polyurethanes with Enhanced Thermal Stability. Polymers 2024, 16, 1812. https://doi.org/10.3390/polym16131812
Džunuzović JV, Stefanović IS, Džunuzović ES, Kovač TS, Malenov DP, Basagni A, Marega C. Fabrication of Polycaprolactone-Based Polyurethanes with Enhanced Thermal Stability. Polymers. 2024; 16(13):1812. https://doi.org/10.3390/polym16131812
Chicago/Turabian StyleDžunuzović, Jasna V., Ivan S. Stefanović, Enis S. Džunuzović, Tijana S. Kovač, Dušan P. Malenov, Andrea Basagni, and Carla Marega. 2024. "Fabrication of Polycaprolactone-Based Polyurethanes with Enhanced Thermal Stability" Polymers 16, no. 13: 1812. https://doi.org/10.3390/polym16131812
APA StyleDžunuzović, J. V., Stefanović, I. S., Džunuzović, E. S., Kovač, T. S., Malenov, D. P., Basagni, A., & Marega, C. (2024). Fabrication of Polycaprolactone-Based Polyurethanes with Enhanced Thermal Stability. Polymers, 16(13), 1812. https://doi.org/10.3390/polym16131812









