Influence of Implant Impression Methods, Polymer Materials, and Implant Angulation on the Accuracy of Dental Models
Abstract
:1. Introduction
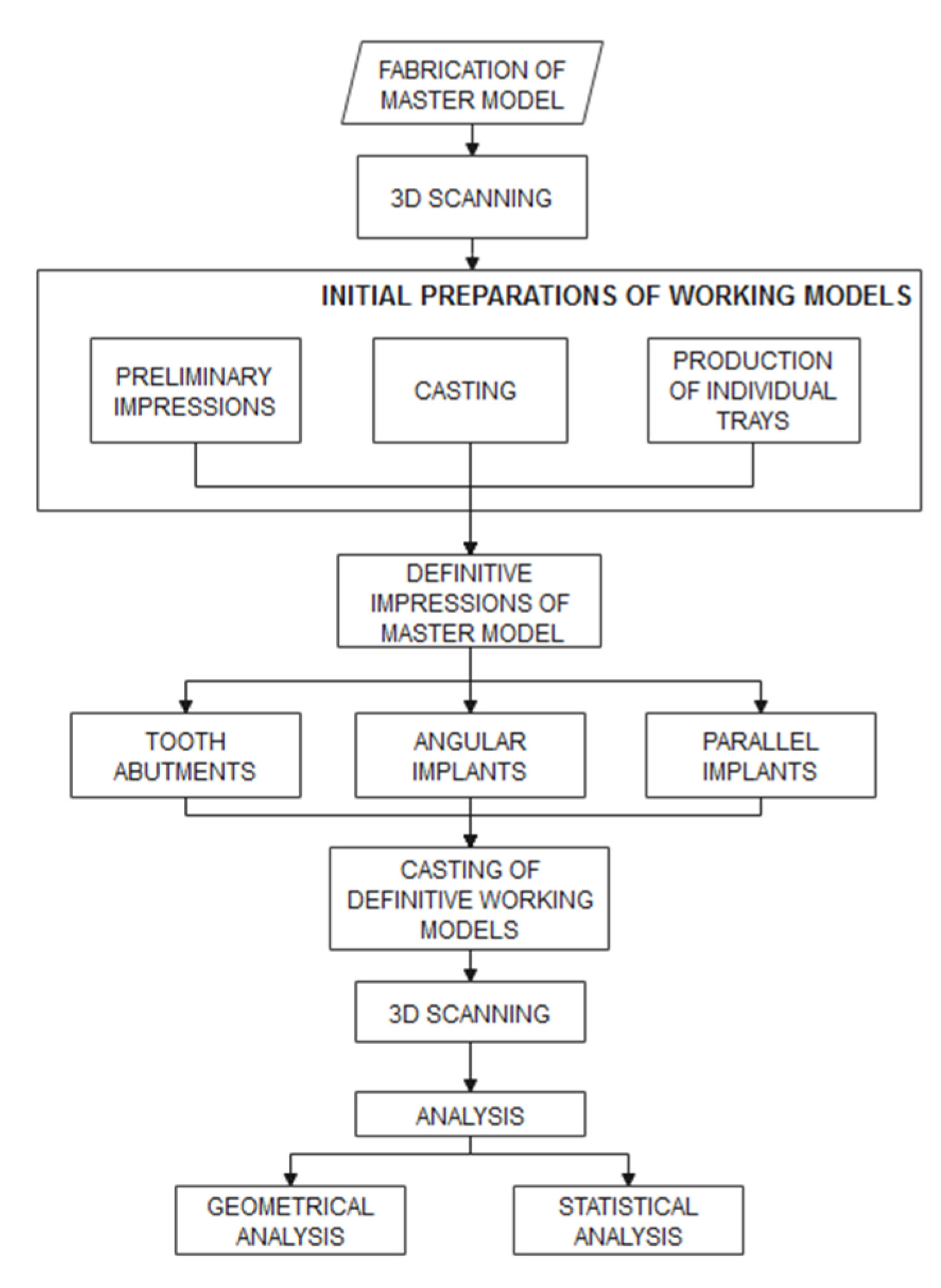
2. Materials and Methods
2.1. Fabrication of Master Model
2.2. 3D Digitization
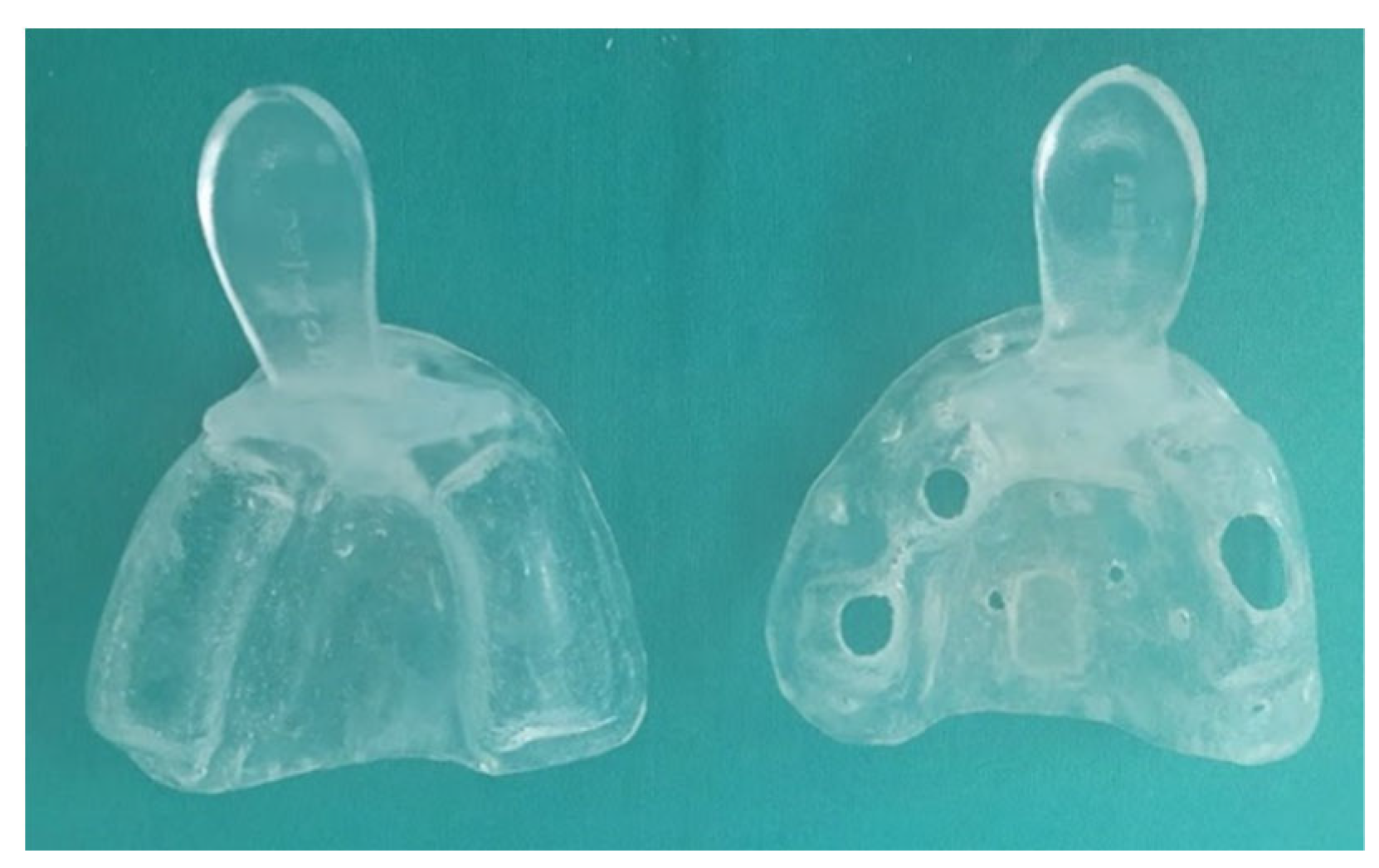
2.3. Preliminary Preparations
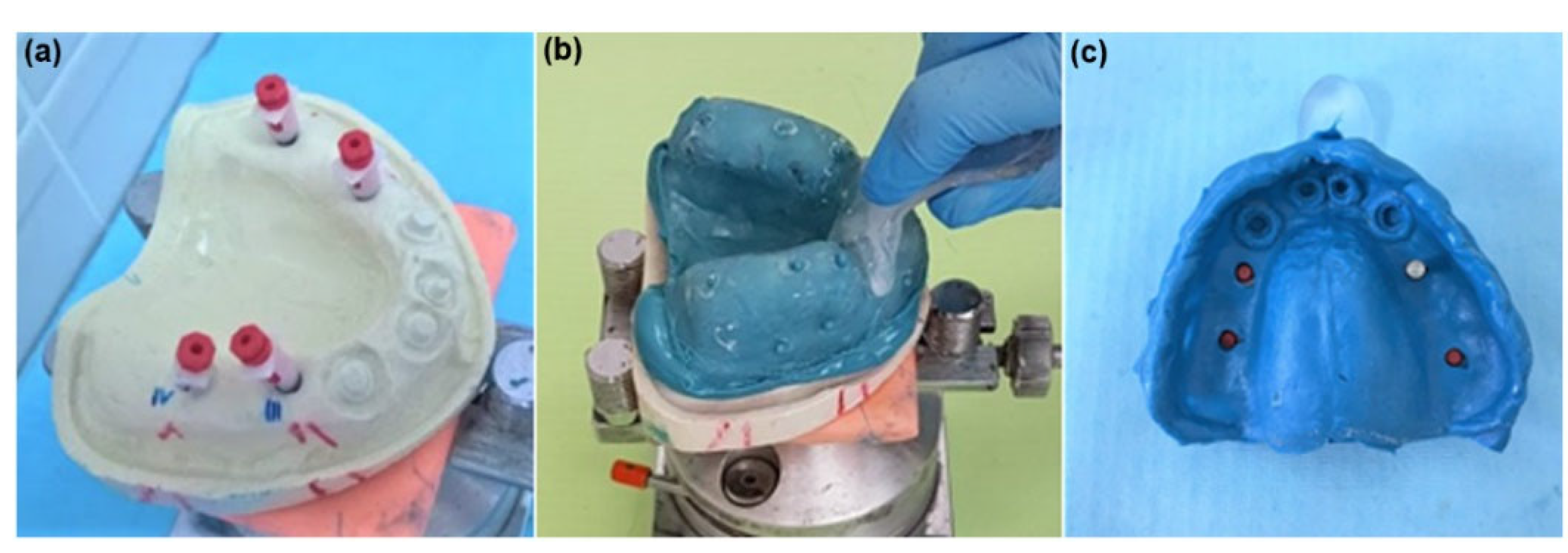
2.4. Definitive Impressions
2.5. Definitive Working Model Casting
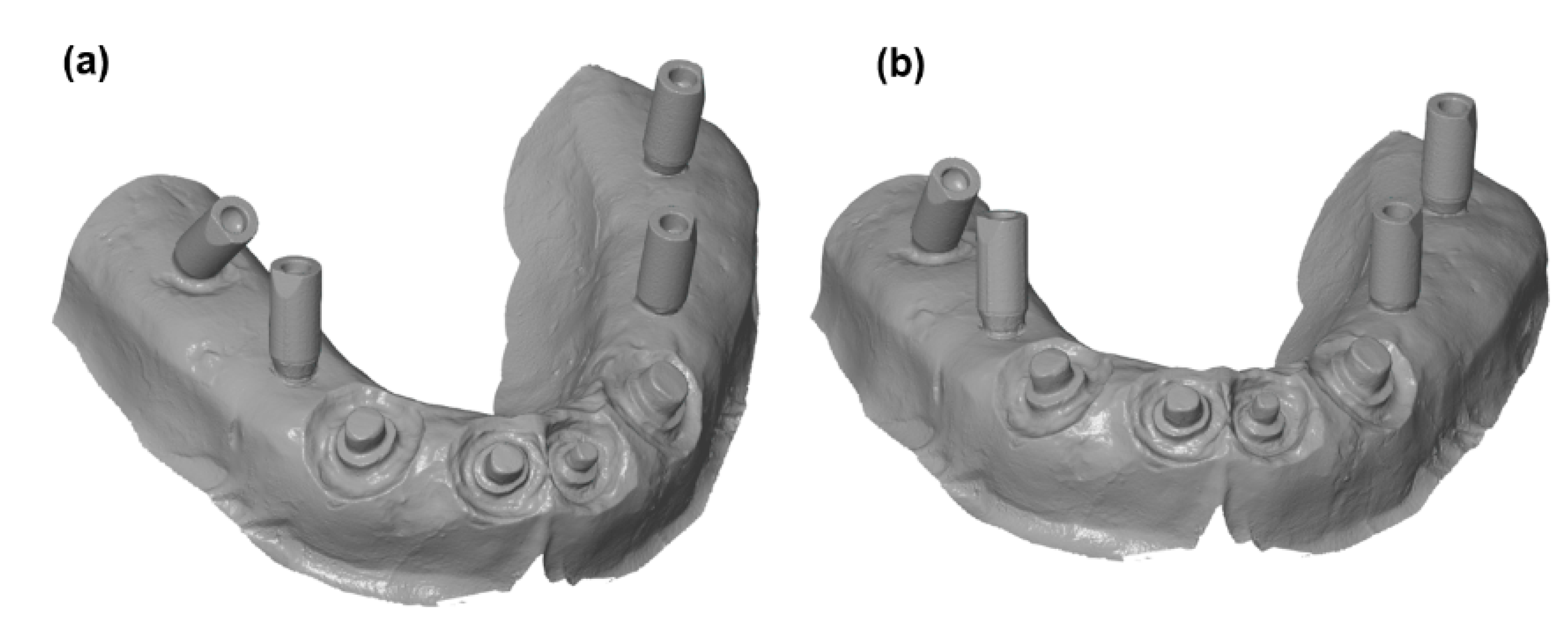
2.6. 3D Digitization of Definitive Working Models
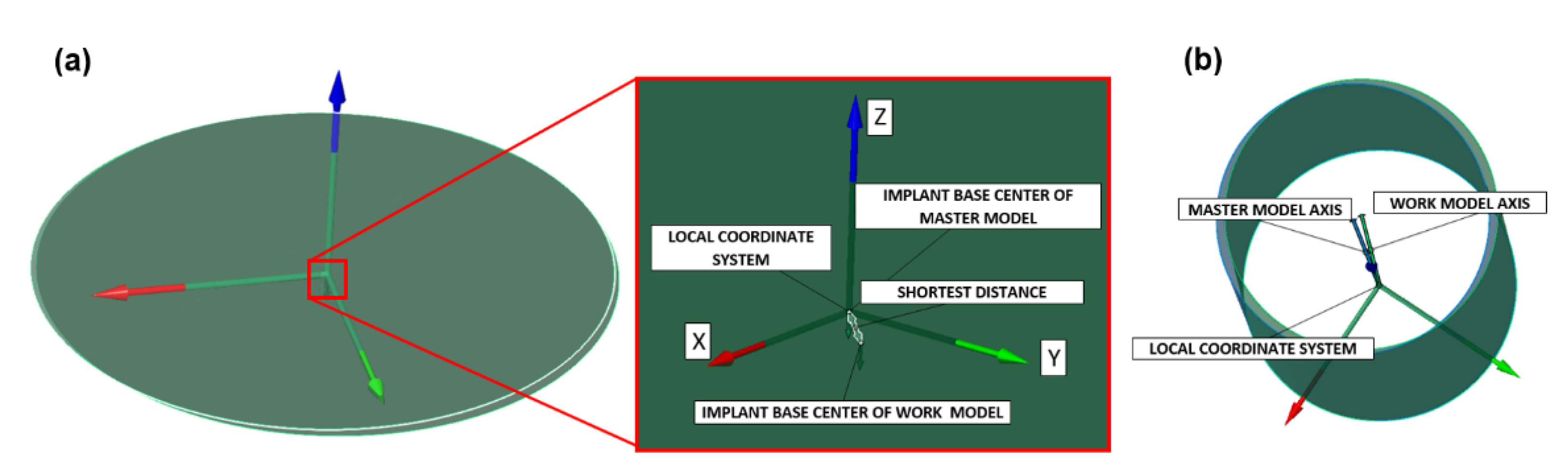
2.7. Geometrical Analysis
2.8. Statistical Analysis
3. Results
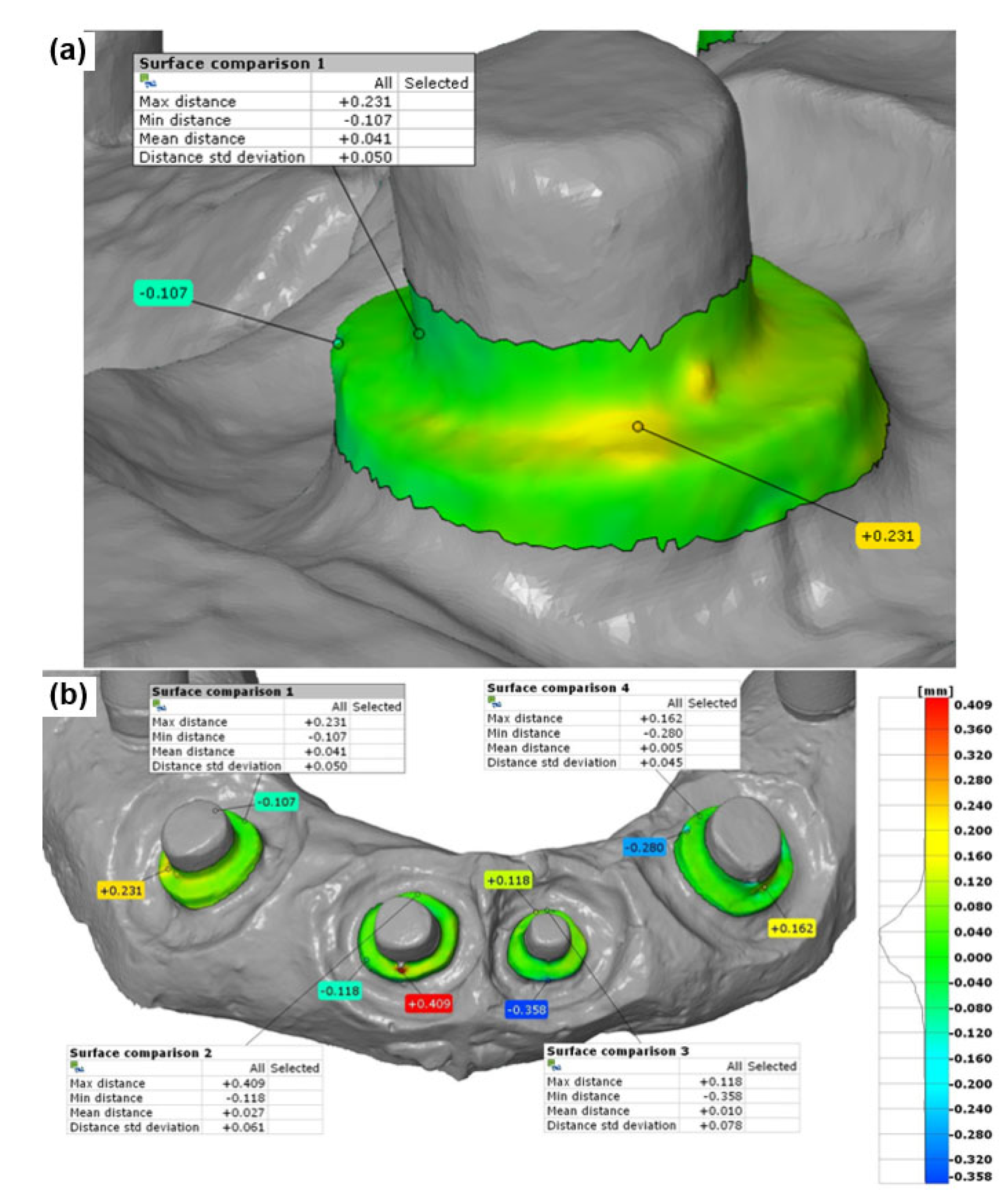
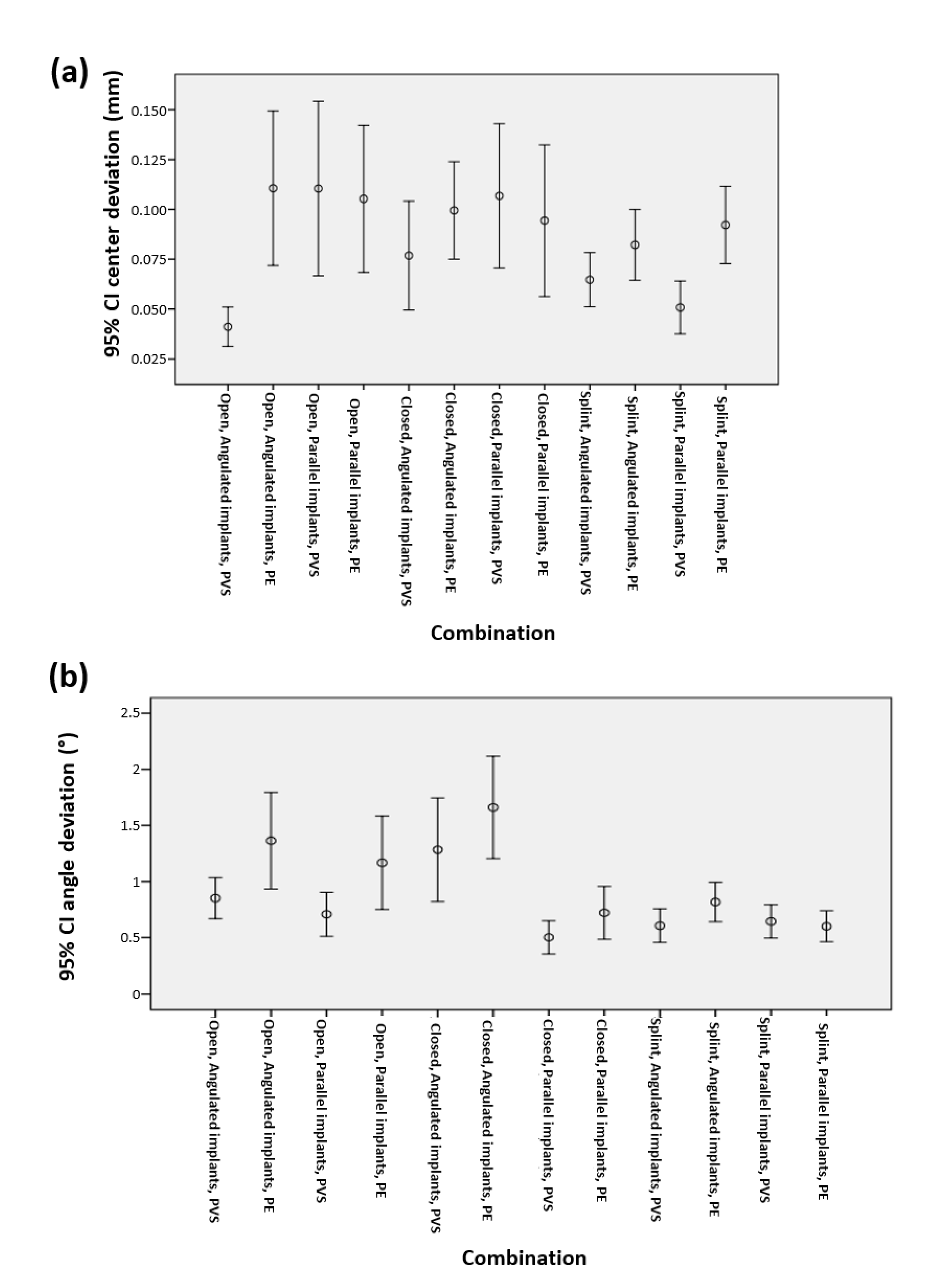
3.1. CAD Inspection and Implant Base and Lateral Axis Deviation Measurements
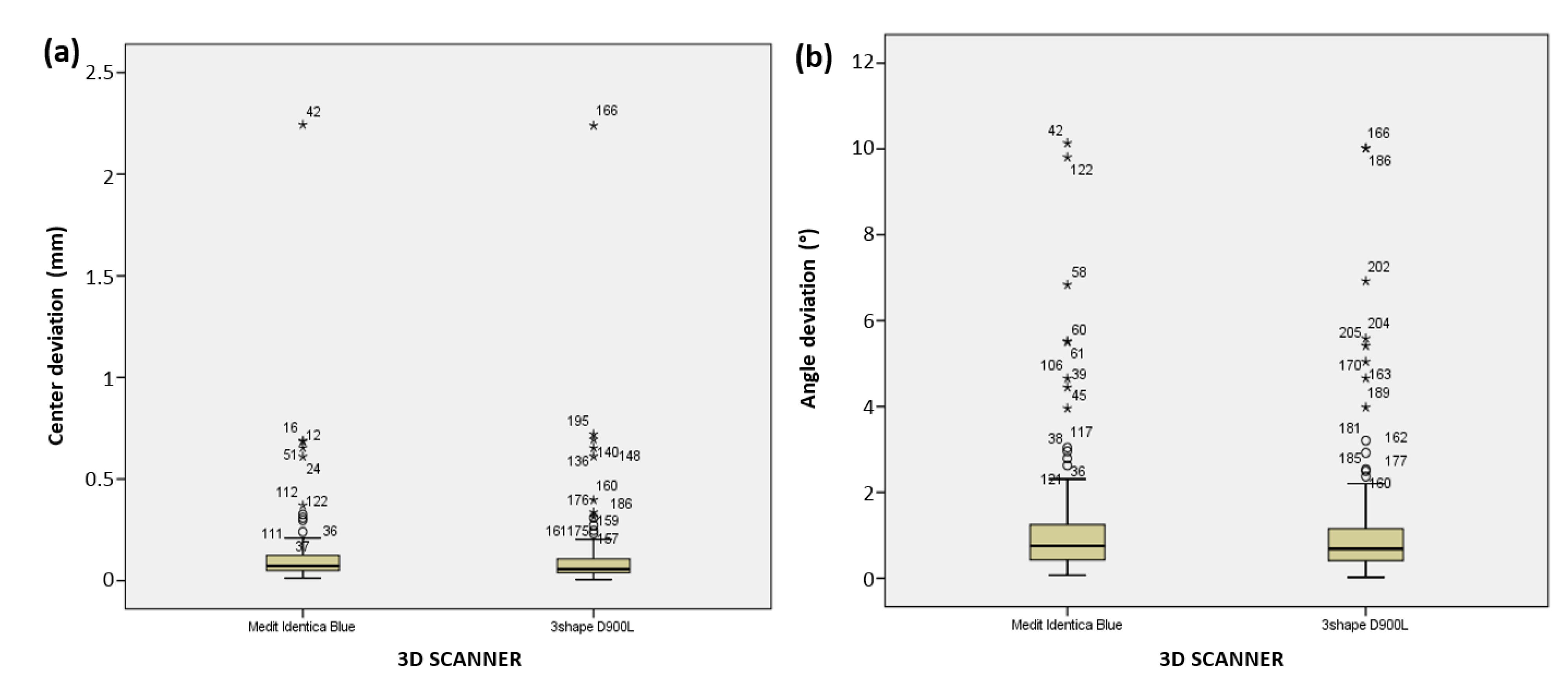
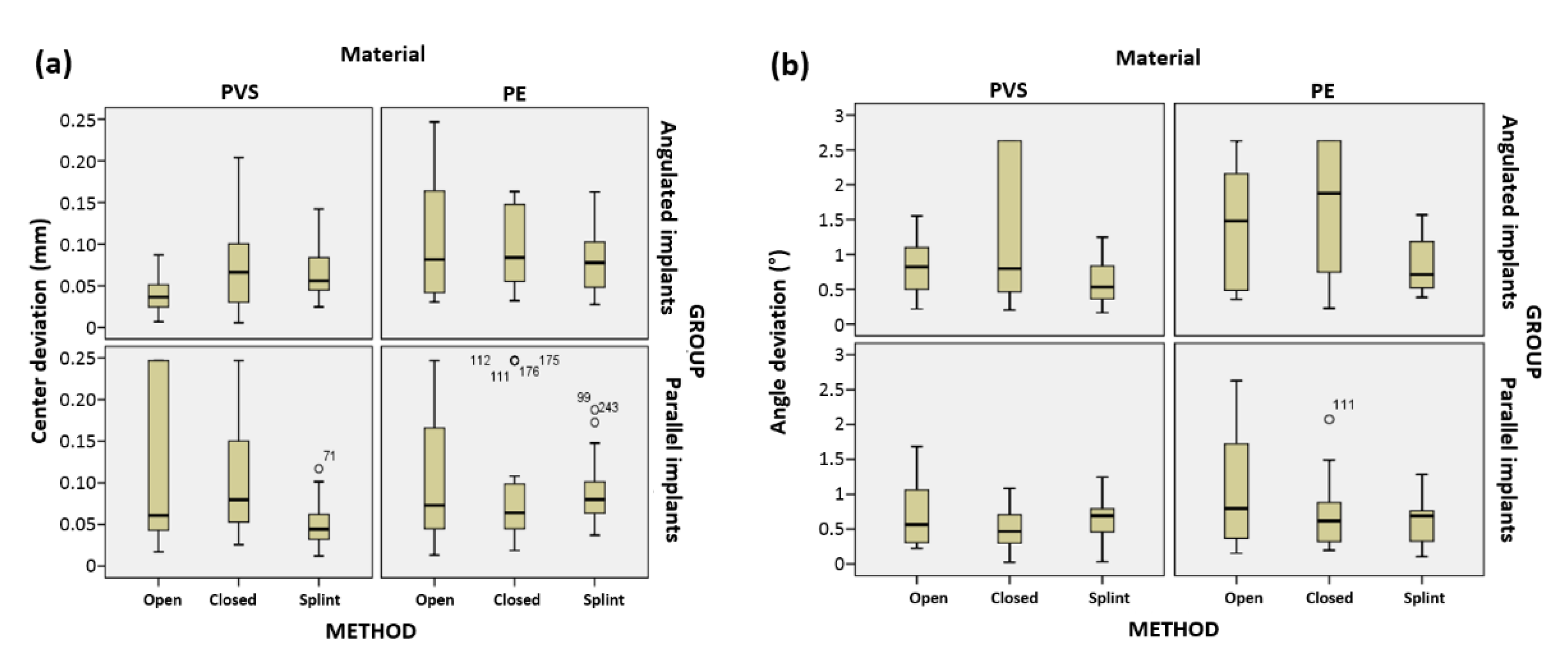
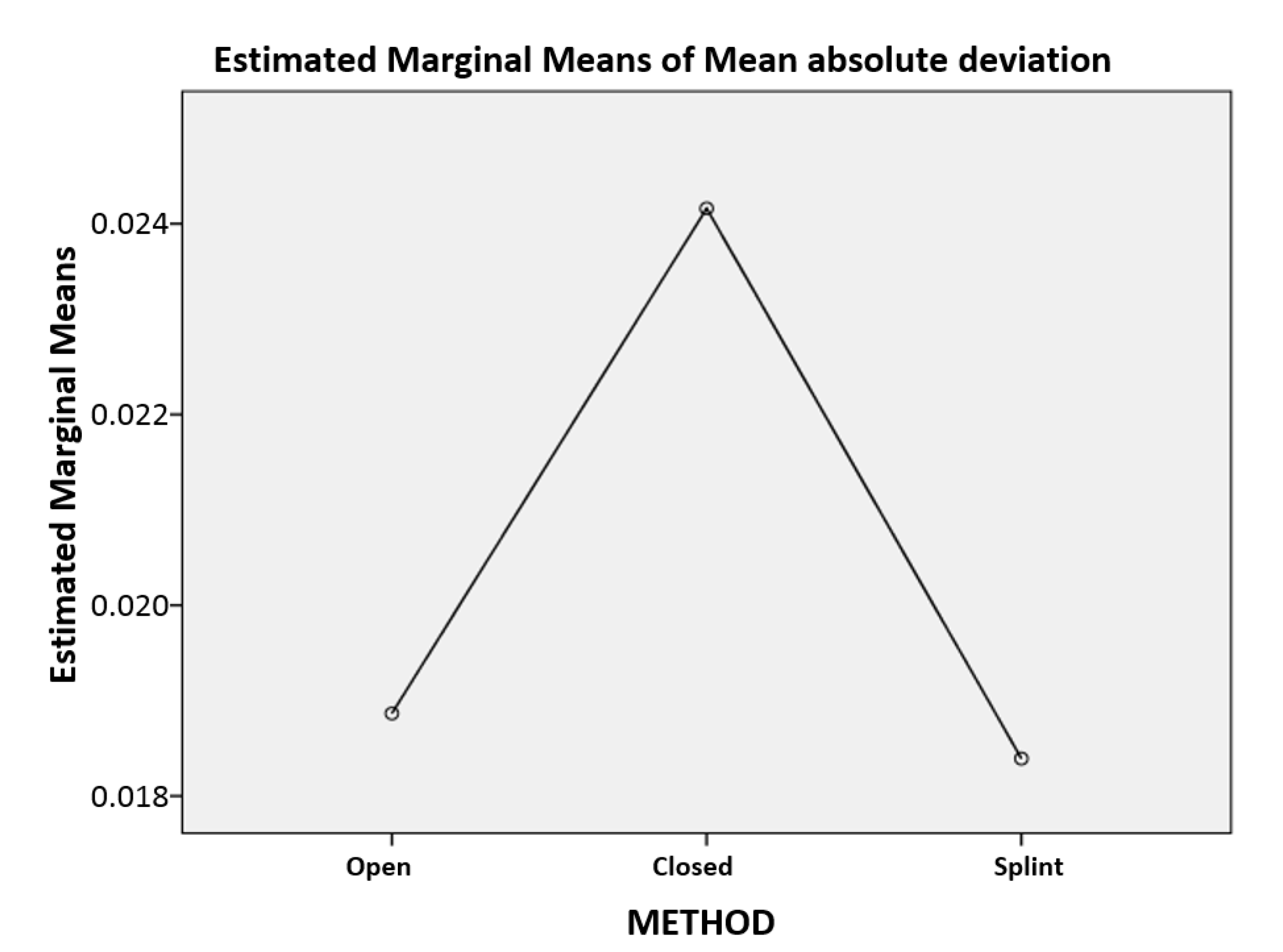
3.2. Implant Spatial Deviation Results
- Three groups based on the applied impression method (open, closed, and splint),
- Two groups based on implant type (angulated and parallel),
- Two groups based on the impression material (additive silicone/PVS and polyether/PE).
3.3. Tooth Abutments Deviation Results
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Misch, C. Dental Implant Prosthetics, 2nd ed.; Elsevier: St. Louis, MO, USA, 2015. [Google Scholar]
- Jung, R.E.; Zembic, A.; Pjetursson, B.E.; Zwahlen, M.; Thoma, D.S. Systematic review of the survival rate and the incidence of biological, technical, and aesthetic complications of single crowns on implants reported in longitudinal studies with a mean follow-up of 5 years. Clin. Oral Implants Res. 2012, 23, 2–21. [Google Scholar] [CrossRef] [PubMed]
- Jensen, C.; Meijer, H.J.A.; Raghoebar, G.M.; Kerdijk, W.; Cune, M.S. Implant-supported removable partial dentures in the mandible: A 3–16 year retrospective study. J. Prosthodont. Res. 2017, 61, 98–105. [Google Scholar] [CrossRef] [PubMed]
- Pjetursson, B.E.; Thoma, D.; Jung, R.; Zwahlen, M.; Zembic, A. A systematic review of the survival and complication rates of implant-supported fixed dental prostheses (FDPs) after a mean observation period of at least 5 years. Clin. Oral Implants Res. 2012, 23, 22–38. [Google Scholar] [CrossRef] [PubMed]
- De Araújo Nobre, M.; Mano Azul, A.; Rocha, E.; Maló, P.; Salvado, F. Attributable fractions, modifiable risk factors and risk stratification using a risk score for peri-implant pathology. J. Prosthodont. Res. 2017, 61, 43–53. [Google Scholar] [CrossRef]
- Kan, J.Y.; Rungcharassaeng, K.; Bohsali, K.; Goodacre, C.J.; Lang, B.R. Clinical methods for evaluating implant framework fit. J. Prosthet. Dent. 1999, 81, 7–13. [Google Scholar] [CrossRef]
- Buzayan, M.M.; Yunus, N.B. Passive fit in screw retained multi-unit implant prosthesis understanding and achieving: A review of the literature. J. Indian Prosthodont. Soc. 2014, 14, 16–23. [Google Scholar] [CrossRef]
- Tsagkalidis, G.; Tortopidis, D.; Mpikos, P.; Kaisarlis, G.; Koidis, P. Accuracy of 3 different impression techniques for internal connection angulated implants. J. Prosthet. Dent. 2015, 114, 517–523. [Google Scholar] [CrossRef]
- Silva, G.C.; Cornacchia, T.M.; De Magalhães, C.S.; Bueno, A.C.; Moreira, A.N. Biomechanical evaluation of screw- and cement-retained implant-supported prostheses: A nonlinear finite element analysis. J. Prosthet. Dent. 2014, 112, 1479–1488. [Google Scholar] [CrossRef]
- Kim, J.H.; Kim, K.R.; Kim, S. Critical appraisal of implant impression accuracies: A systematic review. J. Prosthet. Dent. 2015, 114, 185–192. [Google Scholar] [CrossRef]
- Kurtulmus-Yilmaz, S.; Ozan, O.; Ozcelik, T.B.; Yagiz, A. Digital evaluation of the accuracy of impression techniques and materials in angulated implants. J. Dent. 2014, 42, 1551–1559. [Google Scholar] [CrossRef]
- Kim, S.M.; Son, K.; Kim, D.Y.; Lee, K.B. Digital evaluation of the accuracy of computer-guided dental implant placement: An in vitro study. Appl. Sci. 2019, 9, 1–8. [Google Scholar] [CrossRef] [Green Version]
- Lin, W.S.; Harris, B.T.; Zandinejad, A.; Morton, D. Use of digital data acquisition and CAD/CAM technology for the fabrication of a fixed complete dental prosthesis on dental implants. J. Prosthet. Dent. 2014, 111, 1–5. [Google Scholar] [CrossRef] [PubMed]
- Lin, W.S.; Harris, B.T.; Özdemir, E.; Morton, D. Maxillary rehabilitation with a CAD/CAM-fabricated, long-term interim and anatomic contour definitive prosthesis with a digital workflow: A clinical report. J. Prosthet. Dent. 2013, 110, 1–7. [Google Scholar] [CrossRef]
- Arunyanak, S.P.; Harris, B.T.; Grant, G.T.; Morton, D.; Lin, W.S. Digital approach to planning computer-guided surgery and immediate provisionalization in a partially edentulous patient. J. Prosthet. Dent. 2016, 116, 8–14. [Google Scholar] [CrossRef] [PubMed]
- Ajioka, H.; Kihara, H.; Odaira, C.; Kobayashi, T.; Kondo, H. Examination of the position accuracy of implant abutments reproduced by intra-oral optical impression. PLoS ONE 2016, 11, 1–12. [Google Scholar] [CrossRef]
- Do Jeong, I.; Lee, J.J.; Jeon, J.H.; Kim, J.H.; Kim, H.Y.; Kim, W.C. Accuracy of complete-arch model using an intraoral video scanner: An in vitro study. J. Prosthet. Dent. 2016, 115, 755–759. [Google Scholar] [CrossRef]
- Lin, W.S.; Harris, B.T.; Morton, D. The use of a scannable impression coping and digital impression technique to fabricate a customized anatomic abutment and zirconia restoration in the esthetic zone. J. Prosthet. Dent. 2013, 109, 187–191. [Google Scholar] [CrossRef]
- Franchina, A.; Stefanelli, L.V.; Maltese, F.; Mandelaris, G.A.; Vantaggiato, A.; Pagliarulo, M.; Pranno, N.; Brauner, E.; De Angelis, F.; Di Carlo, S. Validation of an intra-oral scan method versus cone beam computed tomography superimposition to assess the accuracy between planned and achieved dental implants: A randomized in vitro study. Int. J. Environ. Res. Public Health 2020, 17, 1–21. [Google Scholar] [CrossRef]
- Matta, R.E.; Adler, W.; Wichmann, M.; Heckmann, S.M. Accuracy of impression scanning compared with stone casts of implant impressions. J. Prosthet. Dent. 2017, 117, 507–512. [Google Scholar] [CrossRef]
- Pyo, S.W.; Lim, Y.J.; Koo, K.T.; Lee, J. Methods used to assess the 3D accuracy of dental implant positions in computer-guided implant placement: A review. J. Clin. Med. 2019, 8, 54. [Google Scholar] [CrossRef] [Green Version]
- Albanchez-González, M.I.; Brinkmann, J.C.B.; Peláez-Rico, J.; López-Suárez, C.; Rodríguez-Alonso, V.; Suárez-García, M.J. Accuracy of Digital Dental Implants Impression Taking with Intraoral Scanners Compared with Conventional Impression Techniques: A Systematic Review of In Vitro Studies. Int. J. Environ. Res. Public Health 2022, 19, 2026. [Google Scholar] [CrossRef] [PubMed]
- Lim, J.H.; Mangal, U.; Nam, N.E.; Choi, S.H.; Shim, J.S.; Kim, J.E. A comparison of accuracy of different dental restorative materials between intraoral scanning and conventional impression-taking: An in vitro study. Materials 2021, 14, 2060. [Google Scholar] [CrossRef]
- Marcián, P.; Borák, L.; Valášek, J.; Kaiser, J.; Florian, Z.; Wolff, J. Finite element analysis of dental implant loading on atrophic and non-atrophic cancellous and cortical mandibular bone—A feasibility study. J. Biomech. 2014, 47, 3830–3836. [Google Scholar] [CrossRef]
- Djurovic Koprivica, D.; Jeremic Knezevic, M.; Milekic, B.; Maletin, A.; Puskar, T. Conventional impressions in implant prosthetics from the aspect of accuracy. In Proceedings of the International Scientific Conference in Dentistry, Novi Sad, Serbia, 11–13 December 2020. [Google Scholar]
- Stimmelmayr, M.; Güth, J.F.; Erdelt, K.; Happe, A.; Schlee, M.; Beuer, F. Clinical study evaluating the discrepancy of two different impression techniques of four implants in an edentulous jaw. Clin. Oral Investig. 2013, 17, 1929–1935. [Google Scholar] [CrossRef] [PubMed]
- Stimmelmayr, M.; Beuer, F.; Edelhoff, D.; Güth, J.F. Implant Impression Techniques for the Edentulous Jaw: A Summary of Three Studies. J. Prosthodont. 2016, 25, 146–150. [Google Scholar] [CrossRef]
- Stimmelmayr, M.; Erdelt, K.; Güth, J.F.; Happe, A.; Beuer, F. Evaluation of impression accuracy for a four-implant mandibular model-a digital approach. Clin. Oral Investig. 2012, 16, 1137–1142. [Google Scholar] [CrossRef] [PubMed]
- Vigolo, P.; Mutinelli, S.; Fonzi, F.; Stellini, E. An In Vitro Evaluation of Impression Techniques for Multiple Internal- and External-Connection Implant Prostheses. Int. J. Oral Maxillofac. Implants 2014, 29, 807–818. [Google Scholar] [CrossRef] [Green Version]
- Vigolo, P.; Majzoub, Z.; Cordioli, G. Evaluation of the accuracy of three techniques used for multiple implant abutment impressions. J. Prosthet. Dent. 2003, 89, 186–192. [Google Scholar] [CrossRef] [Green Version]
- Papaspyridakos, P.; Benic, G.I.; Hogsett, V.L.; White, G.S.; Lal, K.; Gallucci, G.O. Accuracy of implant casts generated with splinted and non-splinted impression techniques for edentulous patients: An optical scanning study. Clin. Oral Implants Res. 2012, 23, 676–681. [Google Scholar] [CrossRef]
- Papaspyridakos, P.; Hirayama, H.; Chen, C.J.; Ho, C.H.; Chronopoulos, V.; Weber, H.P. Full-arch implant fixed prostheses: A comparative study on the effect of connection type and impression technique on accuracy of fit. Clin. Oral Implants Res. 2016, 27, 1099–1105. [Google Scholar] [CrossRef]
- Papaspyridakos, P.; Chen, C.J.; Gallucci, G.; Doukoudakis, A.; Weber, H.P.; Chronopoulos, V. Accuracy of Implant Impressions for Partially and Completely Edentulous Patients: A Systematic Review. Int. J. Oral Maxillofac. Implants 2014, 29, 836–845. [Google Scholar] [CrossRef] [PubMed]
- Papaspyridakos, P.; Gallucci, G.O.; Chen, C.J.; Hanssen, S.; Naert, I.; Vandenberghe, B. Digital versus conventional implant impressions for edentulous patients: Accuracy outcomes. Clin. Oral Implants Res. 2016, 27, 465–472. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Haghi, H.; Shiehzadeh, M.; Nakhaei, M.; Ahrary, F.; Sabzevari, S. Effect of technique and impression material on the vertical misfit of a screw-retained, three-unit implant bridge: An in vitro study. J. Indian Prosthodont. Soc. 2017, 17, 41–47. [Google Scholar] [PubMed]
- Conrad, H.J.; Pesun, I.J.; DeLong, R.; Hodges, J.S. Accuracy of two impression techniques with angulated implants. J. Prosthet. Dent. 2007, 97, 349–356. [Google Scholar] [CrossRef]
- Alexander Hazboun, G.B.; Masri, R.; Romberg, E.; Kempler, J.; Driscoll, C.F. Effect of implant angulation and impression technique on impressions of Nobel Active implants. J. Prosthet. Dent. 2015, 113, 425–431. [Google Scholar] [CrossRef]
- Nakhaei, M.; Madani, A.S.; Moraditalab, A.; Haghi, H.R. Three-dimensional accuracy of different impression techniques for dental implants. Dent. Res. J. 2015, 12, 431–437. [Google Scholar]
- Alikhasi, M.; Siadat, H.; Rahimian, S. The Effect of Implant Angulation on the Transfer Accuracy of External-Connection Implants. Clin. Implant Dent. Relat. Res. 2015, 17, 822–829. [Google Scholar] [CrossRef]
- Balouch, F.; Jalalian, E.; Nikkheslat, M.; Ghavamian, R.; Toopchi, S.; Jallalian, F.; Jallalian, S. Comparison of Dimensional Accuracy between Open-Tray and Closed-Tray Implant Impression Technique in 15° Angled Implants. J. Dent. 2013, 14, 96–102. [Google Scholar]
- Tabrizi, R.; Pourdanesh, F.; Zare, S.; Daneste, H.; Zeini, N. Do angulated implants increase the amount of bone loss around implants in the anterior maxilla? J. Oral Maxillofac. Surg. 2013, 71, 272–277. [Google Scholar] [CrossRef]
- Geramipanah, F.; Sahebi, M.; Davari, M.; Hajimahmoudi, M.; Rakhshan, V. Effects of impression levels and trays on the accuracy of impressions taken from angulated implants. Clin. Oral Implants Res. 2015, 26, 1098–1105. [Google Scholar] [CrossRef]
- Al-Abdullah, K.; Zandparsa, R.; Finkelman, M.; Hirayama, H. An in vitro comparison of the accuracy of implant impressions with coded healing abutments and different implant angulations. J. Prosthet. Dent. 2013, 110, 90–100. [Google Scholar] [CrossRef]
- Vojdani, M.; Torabi, K.; Ansarifard, E. Accuracy of different impression materials in parallel and nonparallel implants. Dent. Res. J. 2015, 12, 315–322. [Google Scholar]
- Sorrentino, R.; Gherlone, E.F.; Calesini, G.; Zarone, F. Effect of implant angulation, connection length, and impression material on the dimensional accuracy of implant impressions: An in vitro comparative study. Clin. Implant Dent. Relat. Res. 2010, 12, 63–76. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z. Comparison of Dimensional Accuracies Using Two Elastomeric Impression Materials in Casting Three-dimensional Tool Marks. J. Forensic Sci. 2016, 61, 792–797. [Google Scholar] [CrossRef] [PubMed]
- Kumari, N.; Nandeeshwar, D.B. The dimensional accuracy of polyvinyl siloxane impression materials using two different impression techniques: An in vitro study. J. Indian Prosthodont. Soc. 2015, 15, 211–217. [Google Scholar] [CrossRef]








| Tooth Abutments | Angulated Implants | Parallel Implants | |
|---|---|---|---|
| Direct open-tray impressions without transfer splinting using addition silicone | X | X | X |
| Direct open-tray impressions without transfer splinting using polyether | X | X | X |
| Indirect closed-tray impressions using the click method and addition silicone | X | X | X |
| Indirect closed-tray impressions using the click method and polyether | X | X | X |
| Direct open-tray impressions using the splint method and addition silicone | X | X | X |
| Direct open-tray impressions using the splint method and polyether | X | X | X |
| Sample No. | Name | Group | Min. Distance [mm] | Max. Distance [mm] | Standard Deviation [mm] | Mean Value [mm] |
|---|---|---|---|---|---|---|
| 1. | Open (PVS) Identica Blue | T1 | −0.183 | +0.498 | +0.087 | +0.037 |
| T2 | −0.213 | +0.176 | +0.047 | +0.015 | ||
| T3 | −0.222 | +0.202 | +0.028 | +0.013 | ||
| T4 | −0.324 | +0.354 | +0.057 | +0.008 | ||
| Sample No. | Name | Group | Min. Distance [mm] | Max. Distance [mm] | Standard Deviation [mm] | Mean Value [mm] |
| 1. | Open (PVS) D900L | T1 | −0.261 | +0.546 | +0.093 | +0.039 |
| T2 | −0.187 | +0.521 | +0.068 | +0.021 | ||
| T3 | −0.080 | +0.412 | +0.051 | +0.020 | ||
| T4 | −0.326 | +0.267 | +0.050 | +0.010 |
| Sample No. | Name | Group | Distance from the Scanned Abutment Based to the Nominal Abutment Base [mm] | Cylinder Axis Angles Relative to the Base [°] | ||||
|---|---|---|---|---|---|---|---|---|
| x | y | z | xyz | xyz | ||||
| 1. | Open (PVS) Identica Blue | I1 | −0.0015 | −0.0212 | −0.0050 | 0.0219 | 1.5520° | 1°33′7″ |
| I2 | −0.0210 | −0.0421 | 0.0010 | 0.0471 | 0.2817° | 0°16′54″ | ||
| I3 | −0.0524 | 0.0210 | 0.0058 | 0.0568 | 1.1729° | 1°10′22″ | ||
| I4 | 0.0294 | 0.0392 | −0.0030 | 0.0491 | 0.2962° | 0°17′46″ | ||
| Sample No. | Name | Group | Distance from the Scanned Abutment Based to the Nominal Abutment Base [mm] | Cylinder Axis Angles Relative to the Base [°] | ||||
| x | y | z | xyz | xyz | ||||
| 1. | Open (PVS) D900L | I1 | 0.0028 | −0.0061 | 0.0010 | 0.0067 | 1.4504° | 1°27′1″ |
| I2 | −0.0232 | −0.0386 | −0.0119 | 0.0466 | 0.2171° | 0°13′2″ | ||
| I3 | 0.0353 | 0.0280 | −0.0155 | 0.0477 | 0.9010° | 0°54′4″ | ||
| I4 | 0.0178 | 0.0273 | −0.0073 | 0.0334 | 0.3535° | 0°21′13″ | ||
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Djurovic Koprivica, D.; Puskar, T.; Budak, I.; Sokac, M.; Jeremic Knezevic, M.; Maletin, A.; Milekic, B.; Vukelic, D. Influence of Implant Impression Methods, Polymer Materials, and Implant Angulation on the Accuracy of Dental Models. Polymers 2022, 14, 2821. https://doi.org/10.3390/polym14142821
Djurovic Koprivica D, Puskar T, Budak I, Sokac M, Jeremic Knezevic M, Maletin A, Milekic B, Vukelic D. Influence of Implant Impression Methods, Polymer Materials, and Implant Angulation on the Accuracy of Dental Models. Polymers. 2022; 14(14):2821. https://doi.org/10.3390/polym14142821
Chicago/Turabian StyleDjurovic Koprivica, Daniela, Tatjana Puskar, Igor Budak, Mario Sokac, Milica Jeremic Knezevic, Aleksandra Maletin, Bojana Milekic, and Djordje Vukelic. 2022. "Influence of Implant Impression Methods, Polymer Materials, and Implant Angulation on the Accuracy of Dental Models" Polymers 14, no. 14: 2821. https://doi.org/10.3390/polym14142821
APA StyleDjurovic Koprivica, D., Puskar, T., Budak, I., Sokac, M., Jeremic Knezevic, M., Maletin, A., Milekic, B., & Vukelic, D. (2022). Influence of Implant Impression Methods, Polymer Materials, and Implant Angulation on the Accuracy of Dental Models. Polymers, 14(14), 2821. https://doi.org/10.3390/polym14142821






