Characterisation and Modelling of an Artificial Lens Capsule Mimicking Accommodation of Human Eyes
Abstract
1. Introduction
2. Materials and Methods
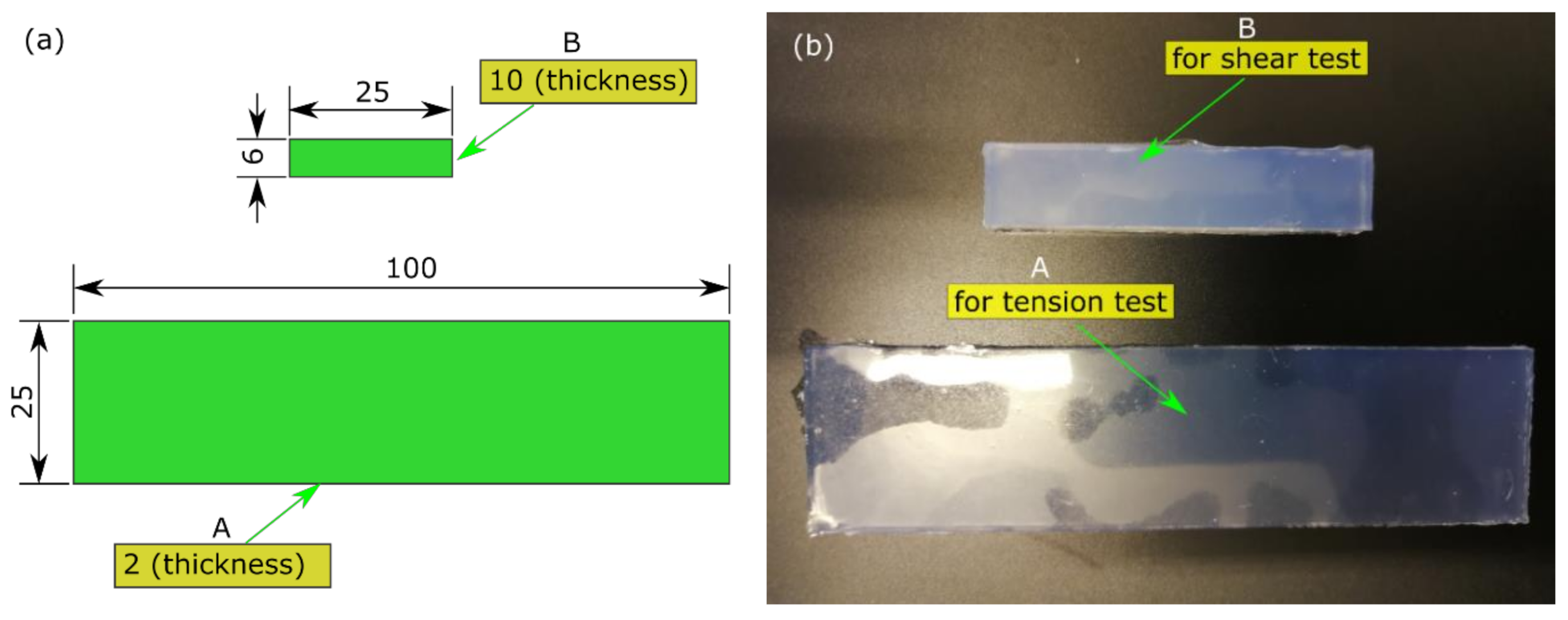
2.1. Material Preparation
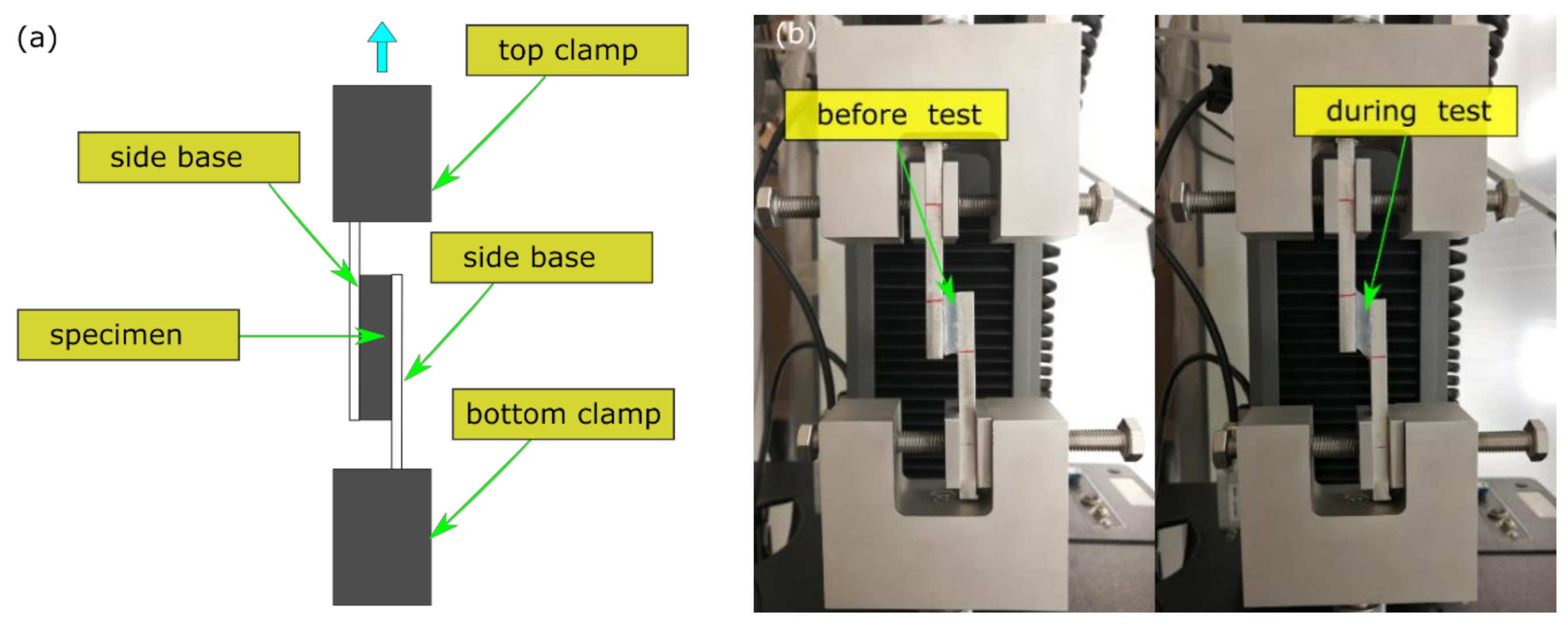
2.2. Mechanical Characterisation
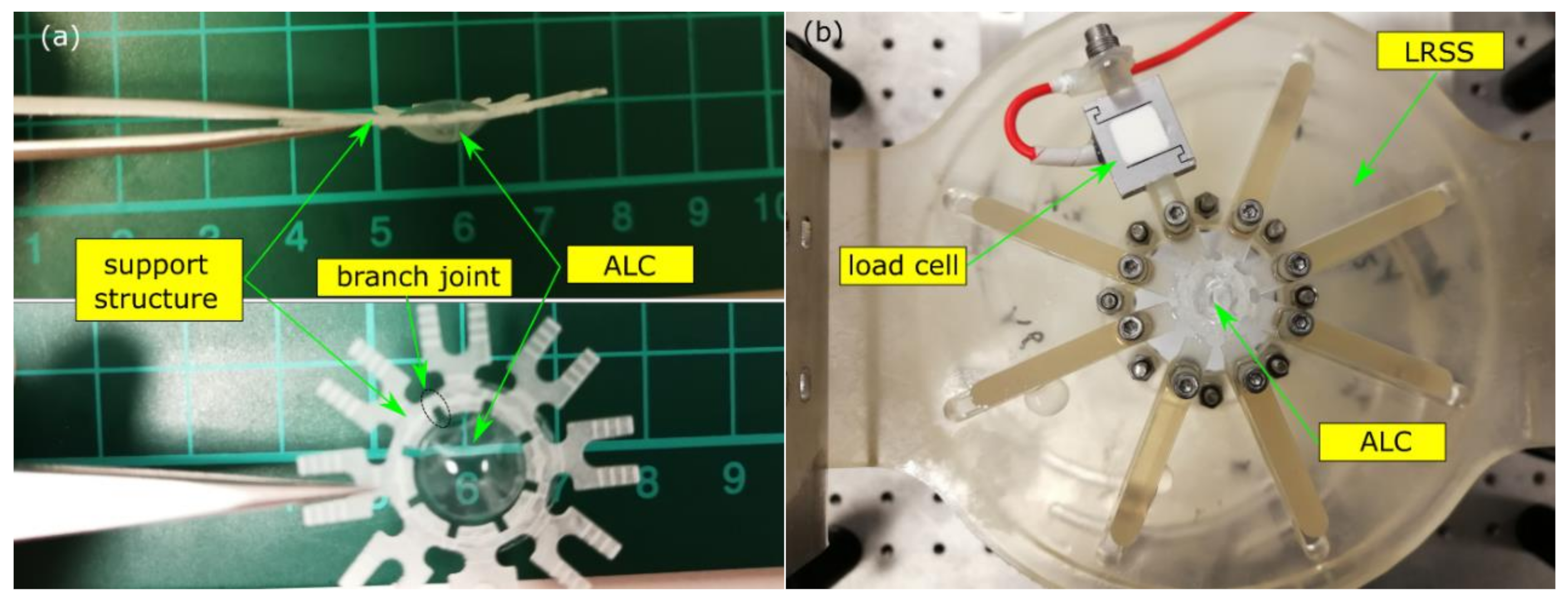
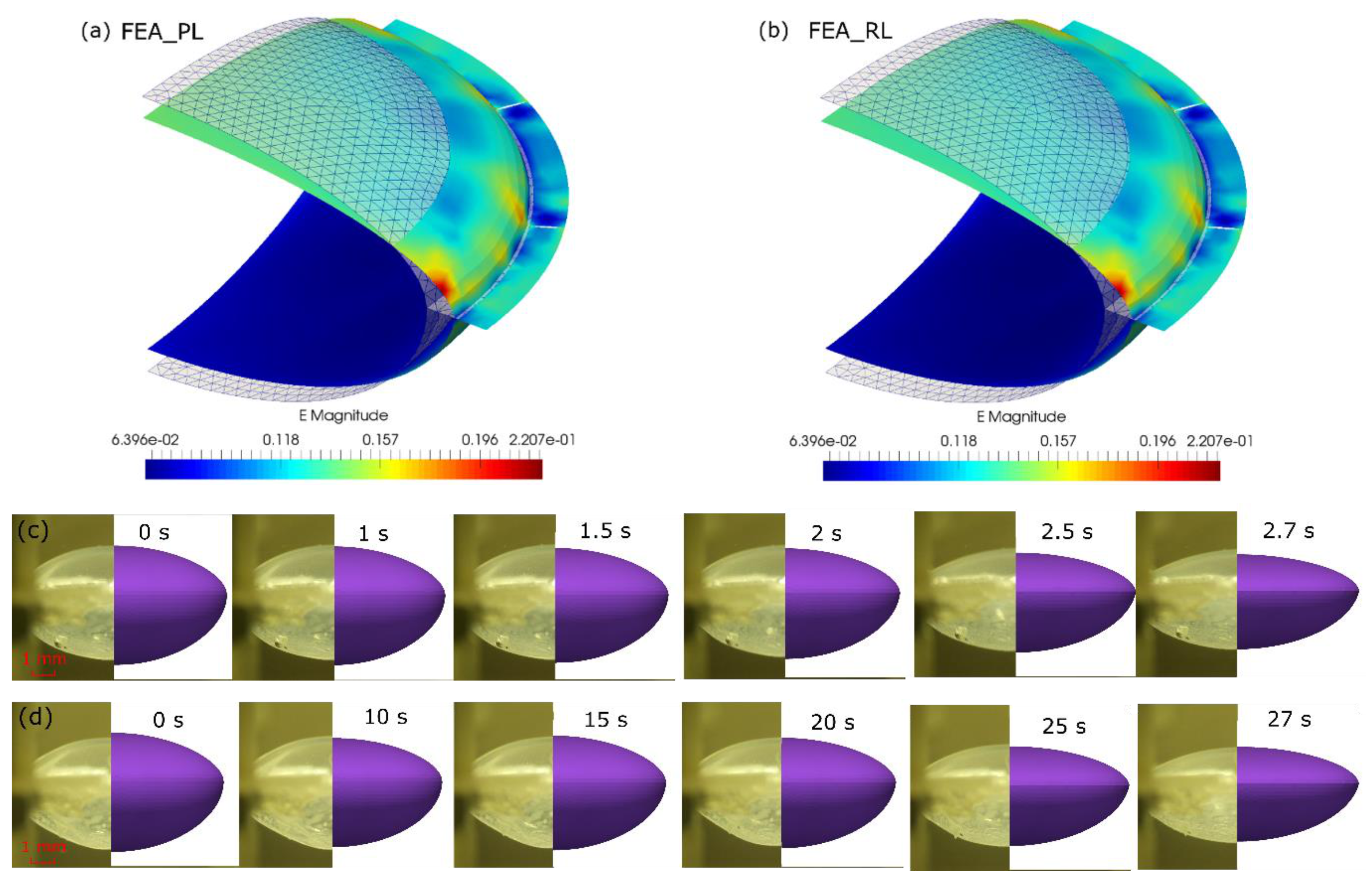
2.3. Accommodating Test
2.4. Constitutive Modelling
3. Results
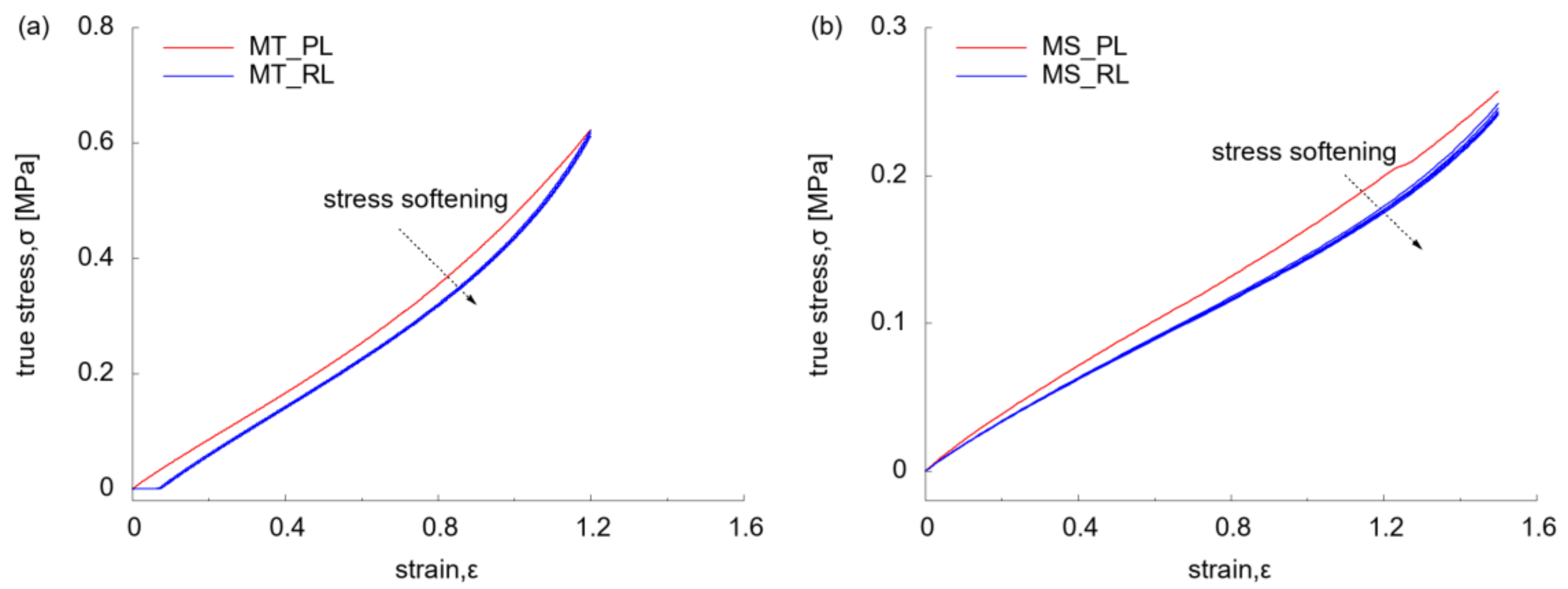
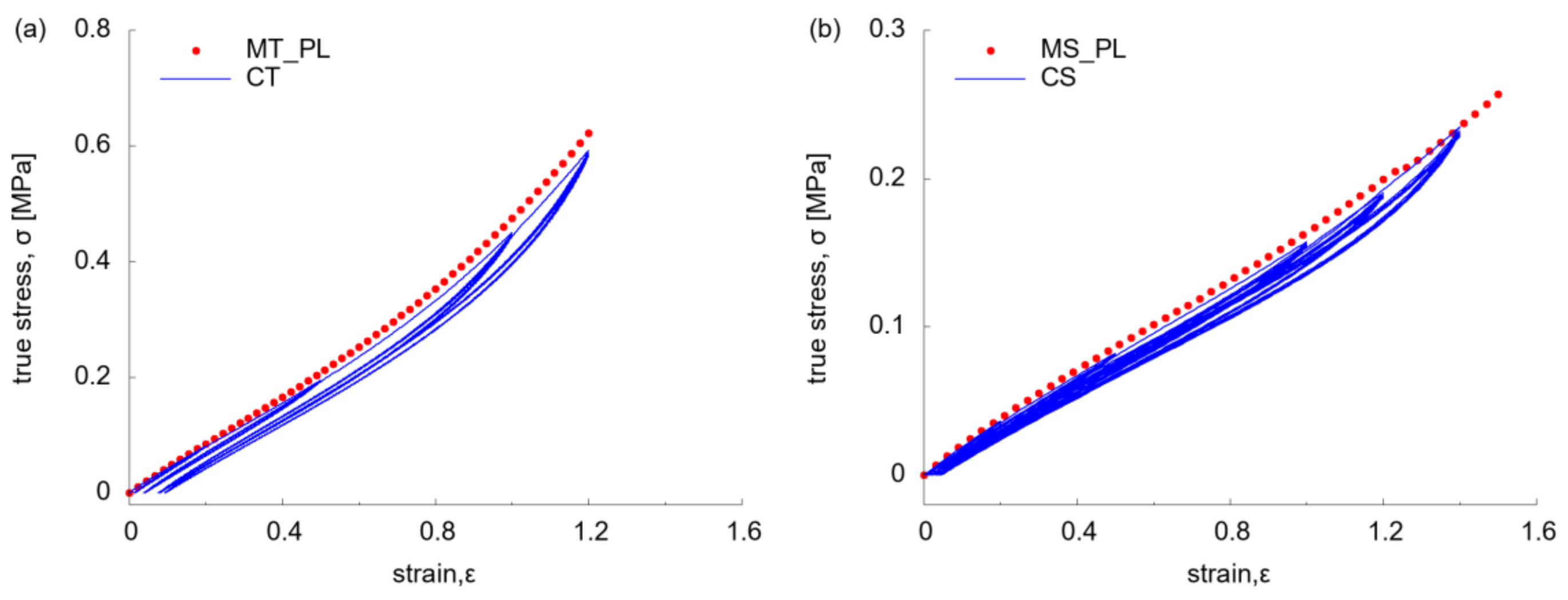
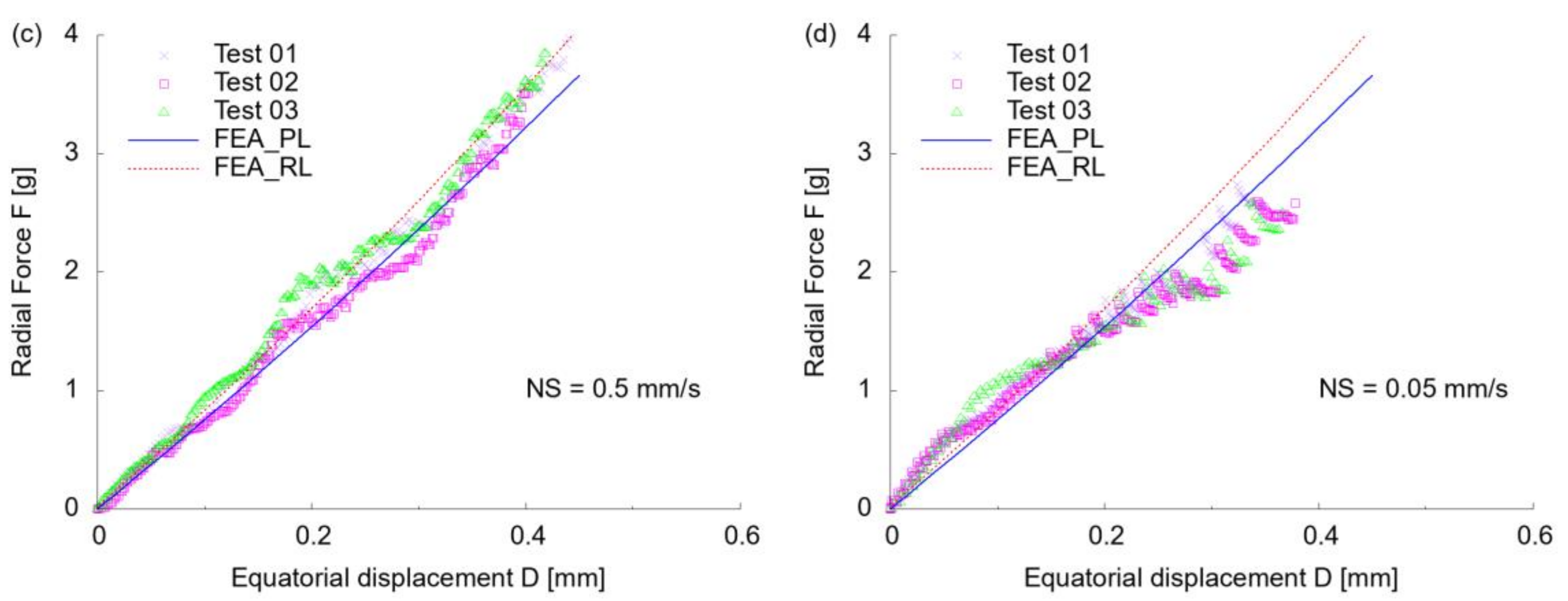
3.1. Monotonic Test
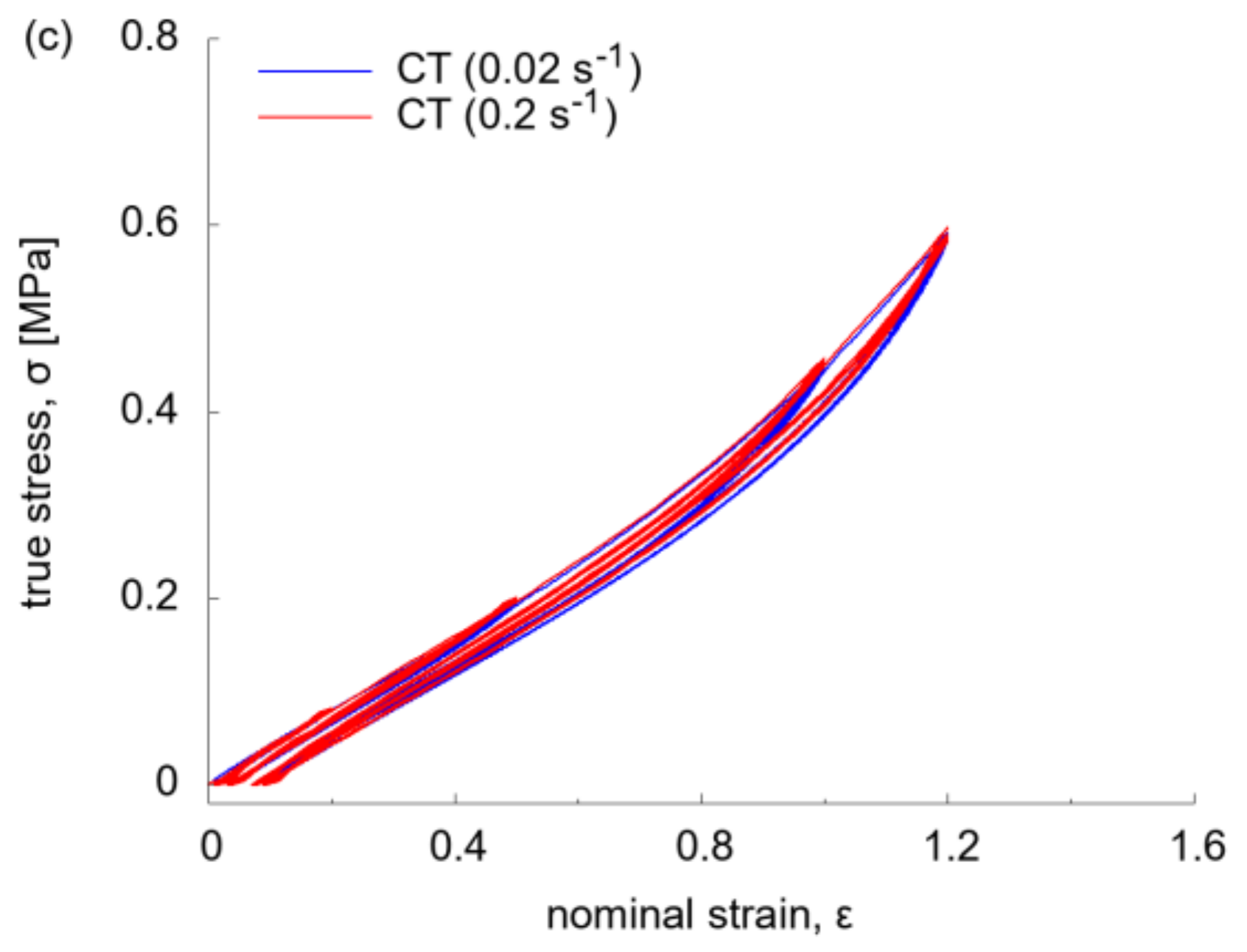
3.2. Cyclic Test
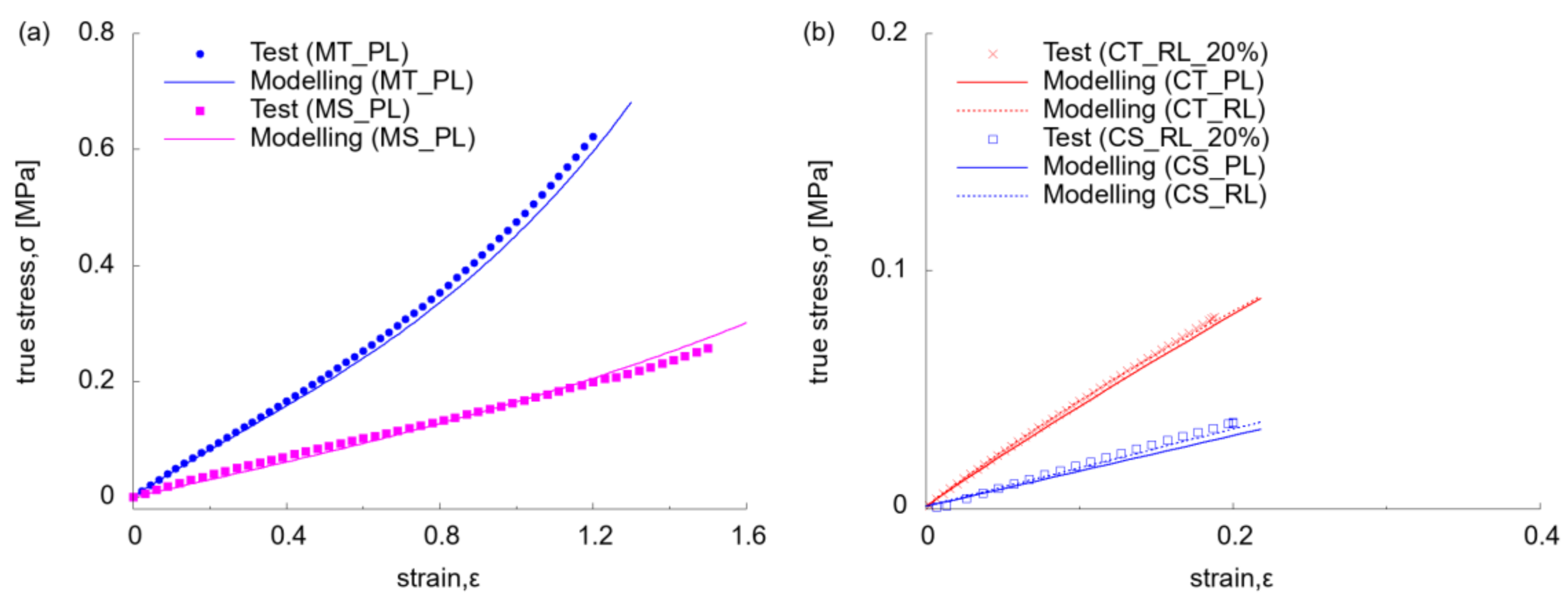
3.3. Modelling and Simulation
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Krag, S.; Andreassen, T.T. Mechanical properties of the human lens capsule. Prog. Retin. Eye Res. 2003, 22, 749–767. [Google Scholar] [CrossRef]
- Fisher, R.F. Elastic constants of the human lens capsule. J. Physiol. 1969, 201, 1–19. [Google Scholar] [CrossRef] [PubMed]
- Krag, S.; Olsen, T.; Andreassen, T.T. Biomechanical characteristics of the human anterior lens capsule in relation to age. Investig. Ophthalmol. Vis. Sci. 1997, 38, 357–363. [Google Scholar]
- Huang, D.; Xu, C.; Guo, R.; Ji, J.; Liu, W. Anterior lens capsule: Biomechanical properties and biomedical engineering perspectives. Acta Ophthalmol. 2021, 99, 1–8. [Google Scholar] [CrossRef] [PubMed]
- Ovenseri-Ogbomo, G.O.; Oduntan, O.A. Mechanism of accommodation: A review of theoretical propositions. Afr. Vis. Eye Health 2015, 74, 1–6. [Google Scholar] [CrossRef]
- Fisher, R.F. The significance of the shape of the lens and capsular energy changes in accommodation. J. Physiol. 1969, 201, 21–47. [Google Scholar] [CrossRef] [PubMed]
- Schachar, R.A.; Bax, A.J. Mechanism of human accommodation as analyzed by nonlinear finite element analysis. Compr. Ther. 2001, 27, 122–132. [Google Scholar] [CrossRef]
- Fisher, R.F.; Pettet, B.E. The postnatal growth of the capsule of the human crystalline lens. J. Anat. 1972, 112, 207–214. [Google Scholar]
- Heys, K.R.; Cram, S.L.; Truscott, R.J.W. Massive increase in the stiffness of the human lens nucleus with age: The basis for presbyopia? Mol. Vis. 2004, 10, 956–963. [Google Scholar] [PubMed]
- Dubbelman, M.; Van der Heijde, G.; Weeber, H. Change in shape of the aging human crystalline lens with accommodation. Vis. Res. 2005, 45, 117–132. [Google Scholar] [CrossRef] [PubMed]
- Weikert, M.P. Update on bimanual microincisional cataract surgery. Curr. Opin. Ophthalmol. 2006, 17, 62–67. [Google Scholar] [CrossRef] [PubMed]
- Wolffsohn, J.S.; Davies, L.N. Presbyopia: Effectiveness of correction strategies. Prog. Retin. Eye Res. 2019, 68, 124–143. [Google Scholar] [CrossRef] [PubMed]
- Pepose, J.S.; Burke, J.S.; Qazi, M.A. Accommodating Intraocular Lenses. Asia-Pac. J. Ophthalmol. 2017, 6, 350–357. [Google Scholar] [CrossRef]
- Ziebarth, N.; Borja, D.; Arrieta, E.; Aly, M.; Manns, F.; Dortonne, I.; Nankivil, D.; Jain, R.; Parel, J.-M. Role of the Lens Capsule on the Mechanical Accommodative Response in a Lens Stretcher. Investig. Opthalmol. Vis. Sci. 2008, 49, 4490–4496. [Google Scholar] [CrossRef] [PubMed]
- Lanchares, E.; Navarro, R.; Calvo, B. Hyperelastic modelling of the crystalline lens: Accommodation and presbyopia. J. Optom. 2012, 5, 110–120. [Google Scholar] [CrossRef]
- Webb, J.N.; Dong, C.; Bernal, A.; Scarcelli, G. Simulating the Mechanics of Lens Accommodation via a Manual Lens Stretcher. J. Vis. Exp. 2018, e57162. [Google Scholar] [CrossRef] [PubMed]
- Ehrmann, K.; Ho, A.; Parel, J. Biomechanical analysis of the accommodative apparatus in primates. Clin. Exp. Optom. 2008, 91, 302–312. [Google Scholar] [CrossRef] [PubMed]
- Cortés, L.P.; Burd, H.J.; Montenegro, G.A.; D’Antin, J.C.; Mikielewicz, M.; Barraquer, R.I.; Michael, R. Experimental Protocols for Ex Vivo Lens Stretching Tests to Investigate the Biomechanics of the Human Accommodation Apparatus. Investig. Ophtalmol. Vis. Sci. 2015, 56, 2926–2932. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Manns, F.; Parel, J.-M.; Denham, D.; Billotte, C.; Ziebarth, N.; Borja, D.; Fernandez, V.; Aly, M.; Arrieta, E.; Ho, A.; et al. Optomechanical Response of Human and Monkey Lenses in a Lens Stretcher. Investig. Ophtalmol. Vis. Sci. 2007, 48, 3260–3268. [Google Scholar] [CrossRef] [PubMed]
- Pierscionek, B.K. Age-related response of human lenses to stretching forces. Exp. Eye Res. 1995, 60, 325–332. [Google Scholar] [CrossRef]
- Doi, T.; Tanabe, S.; Fujita, I. Matching and correlation computations in stereoscopic depth perception. J. Vis. 2011, 11, 1–16. [Google Scholar] [CrossRef]
- Reilly, M.A. A quantitative geometric mechanics lens model: Insights into the mechanisms of accommodation and presbyopia. Vis. Res. 2014, 103, 20–31. [Google Scholar] [CrossRef]
- Ljubimova, D.; Eriksson, A.; Bauer, S. Aspects of eye accommodation evaluated by finite elements. Biomech. Model. Mechanobiol. 2007, 7, 139–150. [Google Scholar] [CrossRef] [PubMed]
- David, G.; Pedrigi, R.M.; Humphrey, J.D. Accommodation of the human lens capsule using a finite element model based on nonlinear regionally anisotropic biomembranes. Comput. Methods Biomech. Biomed. Eng. 2017, 20, 302–307. [Google Scholar] [CrossRef]
- Fisher, R.F.; Wakely, J. The elastic constants and ultrastructural organization of a basement membrane (lens capsule). Proc. R. Soc. London. Ser. B Biol. Sci. 1976, 193, 335–358. [Google Scholar]
- Gent, A.J. On the relation between indentation hardness and Young’s modulus. Inst. Rubber Ind. Trans. 1958, 34, 46–57. [Google Scholar] [CrossRef]
- Meththananda, I.M.; Parker, S.; Patel, M.P.; Braden, M. The relationship between Shore hardness of elastomeric dental materials and Young’s modulus. Dent. Mater. 2009, 25, 956–959. [Google Scholar] [CrossRef]
- Wei, H.; Wolffsohn, J.S.; de Oliveira, O.G.; Davies, L.N. An Artificial Lens Capsule with a Lens Radial Stretching System Mimicking Dynamic Eye Focusing. Polymers 2021, 13, 3552. [Google Scholar] [CrossRef] [PubMed]
- Sheppard, A.L.; Evans, C.J.; Singh, K.D.; Wolffsohn, J.S.; Dunne, M.C.M.; Davies, L.N. Three-Dimensional Magnetic Resonance Imaging of the Phakic Crystalline Lens during Accommodation. Investig. Ophtalmol. Vis. Sci. 2011, 52, 3689–3697. [Google Scholar] [CrossRef] [PubMed]
- Kasthurirangan, S.; Markwell, E.L.; Atchison, D.; Pope, J.M. In Vivo Study of Changes in Refractive Index Distribution in the Human Crystalline Lens with Age and Accommodation. Investig. Ophtalmol. Vis. Sci. 2008, 49, 2531–2540. [Google Scholar] [CrossRef] [PubMed]
- Rashid, B.; Destrade, M.; Gilchrist, M.D. Mechanical characterization of brain tissue in tension at dynamic strain rates. J. Mech. Behav. Biomed. Mater. 2014, 33, 43–54. [Google Scholar] [CrossRef]
- Upadhyay, K.; Subhash, G.; Spearot, D. Visco-hyperelastic constitutive modeling of strain rate sensitive soft materials. J. Mech. Phys. Solids 2020, 135, 103777. [Google Scholar] [CrossRef]
- Wu, Y.; Wang, H.; Li, A. Parameter Identification Methods for Hyperelastic and Hyper-Viscoelastic Models. Appl. Sci. 2016, 6, 386. [Google Scholar] [CrossRef]
- Wilkes, R.P.; Reilly, M.A. A pre-tensioned finite element model of ocular accommodation and presbyopia. Int. J. Adv. Eng. Sci. Appl. Math. 2016, 8, 25–38. [Google Scholar] [CrossRef]
- Dhondt, G. The Finite Element Method for Three-Dimensional Thermomechanical Applications; John Wiley & Sons: Chichester, UK, 2004. [Google Scholar]
- Harwood, J.A.C.; Mullins, L.; Payne, A.R. Stress softening in natural rubber vulcanizates. Part II. Stress softening effects in pure gum and filler loaded rubbers. J. Appl. Polym. Sci. 1965, 9, 3011–3021. [Google Scholar] [CrossRef]
- Burd, H.; Judge, S.; Cross, J. Numerical modelling of the accommodating lens. Vis. Res. 2002, 42, 2235–2251. [Google Scholar] [CrossRef]
- Hermans, E.; Dubbelman, M.; van der Heijde, G.; Heethaar, R. Estimating the external force acting on the human eye lens during accommodation by finite element modelling. Vis. Res. 2006, 46, 3642–3650. [Google Scholar] [CrossRef] [PubMed]
- Krag, S.; Andreassen, T.T. Mechanical Properties of the Human Posterior Lens Capsule. Investig. Ophtalmol. Vis. Sci. 2003, 44, 691–696. [Google Scholar] [CrossRef]
- Efremov, Y.M.; Bakhchieva, N.A.; Shavkuta, B.S.; Frolova, A.A.; Kotova, S.L.; Novikov, I.; Akovantseva, A.A.; Avetisov, K.S.; Avetisov, S.E.; Timashev, P.S. Mechanical properties of anterior lens capsule assessed with AFM and nanoindenter in relation to human aging, pseudoexfoliation syndrome, and trypan blue staining. J. Mech. Behav. Biomed. Mater. 2020, 112, 104081. [Google Scholar] [CrossRef]
- Burd, H.J. A structural constitutive model for the human lens capsule. Biomech. Model. Mechanobiol. 2009, 8, 217–231. [Google Scholar] [CrossRef]
- David, G.; Humphrey, J.D. Finite element model of stresses in the anterior lens capsule of the eye. Comput. Methods Biomech. Biomed. Eng. 2007, 10, 237–243. [Google Scholar] [CrossRef]
- Rebouah, M.; Machado, G.; Chagnon, G.; Favier, D. Anisotropic Mullins stress softening of a deformed silicone holey plate. Mech. Res. Commun. 2013, 49, 36–43. [Google Scholar] [CrossRef]
- Reilly, M.A.; Hamilton, P.D.; Perry, G.; Ravi, N. Comparison of the behavior of natural and refilled porcine lenses in a robotic lens stretcher. Exp. Eye Res. 2009, 88, 483–494. [Google Scholar] [CrossRef] [PubMed]
- Mordi, J.A.; Ciuffreda, K.J. Dynamic aspects of accommodation: Age and presbyopia. Vis. Res. 2004, 44, 591–601. [Google Scholar] [CrossRef]
- Glasser, A.; Campbell, M.C. Biometric, optical and physical changes in the isolated human crystalline lens with age in relation to presbyopia. Vis. Res. 1999, 39, 1991–2015. [Google Scholar] [CrossRef]
- Beers, A.; Van Der Heijde, G. In vivo determination of the biomechanical properties of the component elements of the accommodation mechanism. Vis. Res. 1994, 34, 2897–2905. [Google Scholar] [CrossRef]
- Heistand, M.R.; Pedrigi, R.M.; Delange, S.L.; Dziezyc, J.; Humphrey, J.D. Multiaxial mechanical behavior of the porcine anterior lens capsule. Biomech. Model. Mechanobiol. 2005, 4, 168–177. [Google Scholar] [CrossRef] [PubMed]
- Reilly, M.A.; Cleaver, A. Inverse elastographic method for analyzing the ocular lens compression test. J. Innov. Opt. Health Sci. 2017, 10, 1–12. [Google Scholar] [CrossRef]
- Diani, J.; Fayolle, B.; Gilormini, P.; Diani, J.; Fayolle, B.; Gilormini, P. A review on the Mullins effect. Eur. Polym. J. 2009, 45, 601–612. [Google Scholar] [CrossRef]
- Dorfmann, A.; Ogden, R. A constitutive model for the Mullins effect with permanent set in particle-reinforced rubber. Int. J. Solids Struct. 2004, 41, 1855–1878. [Google Scholar] [CrossRef]
- Liao, Z.; Hossain, M.; Yao, X.; Navaratne, R.; Chagnon, G. A comprehensive thermo-viscoelastic experimental investigation of Ecoflex polymer. Polym. Test. 2020, 86, 106478. [Google Scholar] [CrossRef]
- Guo, L.; Lv, Y.; Deng, Z.; Wang, Y.; Zan, X. Tension testing of silicone rubber at high strain rates. Polym. Test. 2016, 50, 270–275. [Google Scholar] [CrossRef]
- Liao, Z.; Hossain, M.; Yao, X. Ecoflex polymer of different Shore hardnesses: Experimental investigations and constitutive modelling. Mech. Mater. 2020, 144, 103366. [Google Scholar] [CrossRef]
- Pierscionek, B.K. In Vitro Alteration of Human Lens Curvatures by Radial Stretching. Exp. Eye Res. 1993, 57, 629–635. [Google Scholar] [CrossRef] [PubMed]
- Dubbelman, M.; Van der Heijde, G. The shape of the aging human lens: Curvature, equivalent refractive index and the lens paradox. Vis. Res. 2001, 41, 1867–1877. [Google Scholar] [CrossRef]
- Martin, H.; Guthoff, R.; Terwee, T.; Schmitz, K.-P. Comparison of the accommodation theories of Coleman and of Helmholtz by finite element simulations. Vis. Res. 2005, 45, 2910–2915. [Google Scholar] [CrossRef] [PubMed]
- Helmholtz, H. Ueber die Accommodation des Auges. Graefe Arch. Clin. Exp. Ophthalmol. 1855, 2, 1–74. [Google Scholar] [CrossRef]
- Glasser, A.; Kaufman, P.L. The mechanism of accommodation in primates. Ophthalmology 1999, 106, 863–872. [Google Scholar] [CrossRef]
- Farnsworth, P.; Shyne, S. Anterior zonular shifts with age. Exp. Eye Res. 1979, 28, 291–297. [Google Scholar] [CrossRef]
- Wang, K.; Venetsanos, D.T.; Hoshino, M.; Uesugi, K.; Yagi, N.; Pierscionek, B.K. A Modeling Approach for Investigating Opto-Mechanical Relationships in the Human Eye Lens. IEEE Trans. Biomed. Eng. 2020, 67, 999–1006. [Google Scholar] [CrossRef] [PubMed]
- Liu, Z.; Wang, B.; Xu, X.; Wang, C. A study for accommodating the human crystalline lens by finite element simulation. Comput. Med. Imaging Graph. 2006, 30, 371–376. [Google Scholar] [CrossRef] [PubMed]
- Burd, H.J.; Wilde, G.S. Finite element modelling of radial lentotomy cuts to improve the accommodation performance of the human lens. Graefe Arch. Clin. Exp. Ophthalmol. 2016, 254, 727–737. [Google Scholar] [CrossRef]
- Strenk, S.A.; Semmlow, J.L.; Strenk, L.M.; Munoz, P.; Gronlund-Jacob, J.; Demarco, J.K. Age-related changes in human ciliary muscle and lens: A magnetic resonance imaging study. Investig. Ophthalmol. Vis. Sci. 1999, 40, 1162–1169. [Google Scholar]
- Nankivil, D.; Heilman, B.M.; Durkee, H.; Manns, F.; Ehrmann, K.; Kelly, S.; Arrieta-Quintero, E.; Parel, J.-M. The Zonules Selectively Alter the Shape of the Lens During Accommodation Based on the Location of Their Anchorage Points. Investig. Ophtalmol. Vis. Sci. 2015, 56, 1751–1760. [Google Scholar] [CrossRef] [PubMed]
- Fisher, R.F. The force of contraction of the human ciliary muscle during accommodation. J. Physiol. 1977, 270, 51–74. [Google Scholar] [CrossRef]
- Wang, K.; Venetsanos, D.; Wang, J.; Pierscionek, B.K. Gradient moduli lens models: How material properties and application of forces can affect deformation and distributions of stress. Sci. Rep. 2016, 6, 31171. [Google Scholar] [CrossRef] [PubMed]
- Pour, H.M.; Kanapathipillai, S.; Zarrabi, K.; Manns, F.; Ho, A. Stretch-dependent changes in surface profiles of the human crystalline lens during accommodation: A finite element study. Clin. Exp. Optom. 2015, 98, 126–137. [Google Scholar] [CrossRef] [PubMed]
- Michael, R.; Mikielewicz, M.; Gordillo, C.; Montenegro, G.A.; Cortés, L.P.; Barraquer, R.I. Elastic Properties of Human Lens Zonules as a Function of Age in Presbyopes. Investig. Ophtalmol. Vis. Sci. 2012, 53, 6109–6114. [Google Scholar] [CrossRef] [PubMed]



| PL | 2.426 × 10−2 | 5.015 × 10−2 | 3.791 × 10−3 | 1.488 × 10−1 | 4.464 × 10−1 |
| RL | 5.000 × 10−8 | 8.160 × 10−2 | 5.000 × 10−6 | 1.632 × 10−1 | 4.896 × 10−1 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wei, H.; Wolffsohn, J.S.; Gomes de Oliveira, O.; Davies, L.N. Characterisation and Modelling of an Artificial Lens Capsule Mimicking Accommodation of Human Eyes. Polymers 2021, 13, 3916. https://doi.org/10.3390/polym13223916
Wei H, Wolffsohn JS, Gomes de Oliveira O, Davies LN. Characterisation and Modelling of an Artificial Lens Capsule Mimicking Accommodation of Human Eyes. Polymers. 2021; 13(22):3916. https://doi.org/10.3390/polym13223916
Chicago/Turabian StyleWei, Huidong, James S. Wolffsohn, Otavio Gomes de Oliveira, and Leon N. Davies. 2021. "Characterisation and Modelling of an Artificial Lens Capsule Mimicking Accommodation of Human Eyes" Polymers 13, no. 22: 3916. https://doi.org/10.3390/polym13223916
APA StyleWei, H., Wolffsohn, J. S., Gomes de Oliveira, O., & Davies, L. N. (2021). Characterisation and Modelling of an Artificial Lens Capsule Mimicking Accommodation of Human Eyes. Polymers, 13(22), 3916. https://doi.org/10.3390/polym13223916







