A Slow-Release Fertilizer of Urea Prepared via Melt Blending with Degradable Poly(lactic acid): Formulation and Release Mechanisms
Abstract
1. Introduction
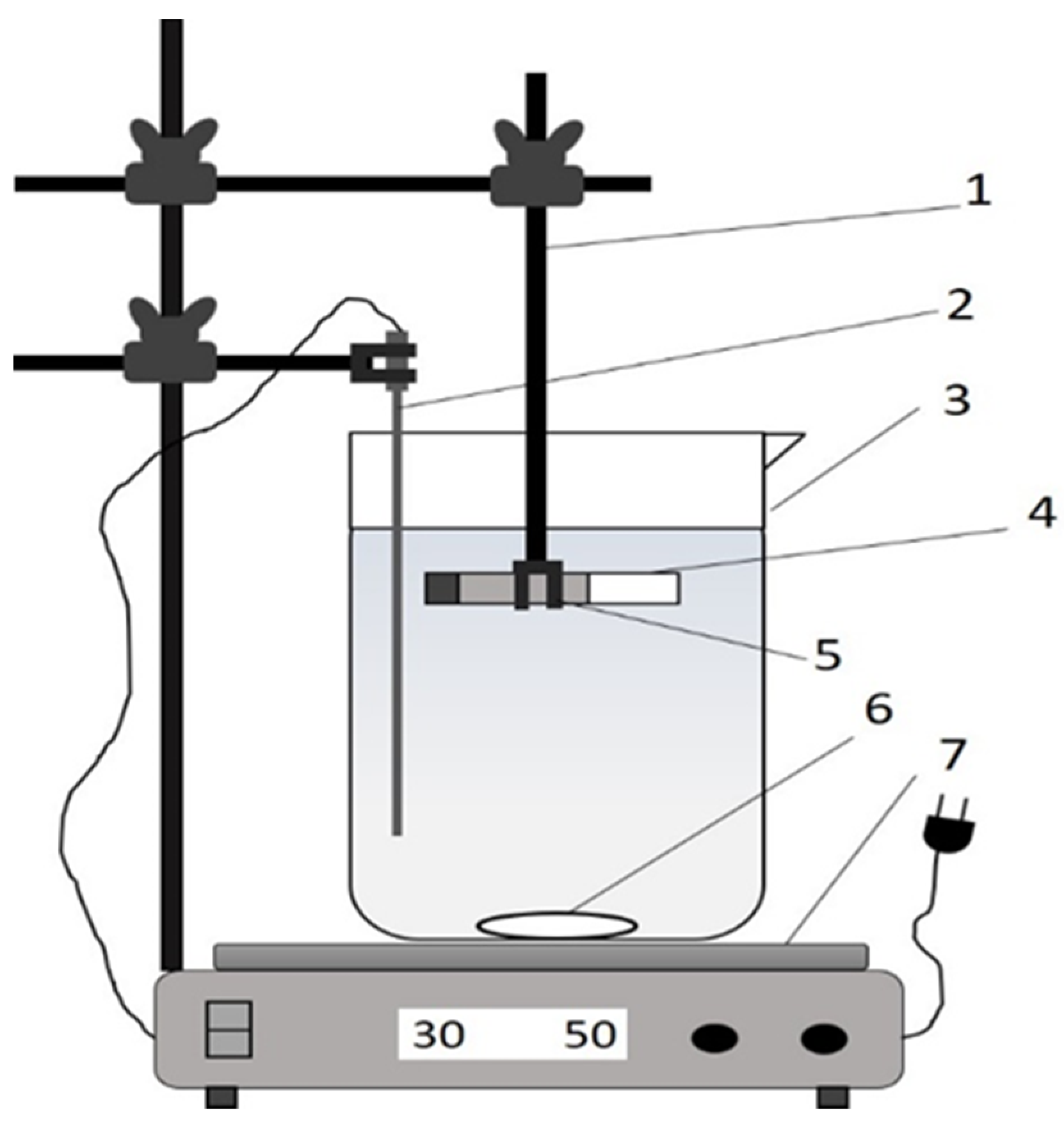
2. Materials and Methods
3. Results and Discussion
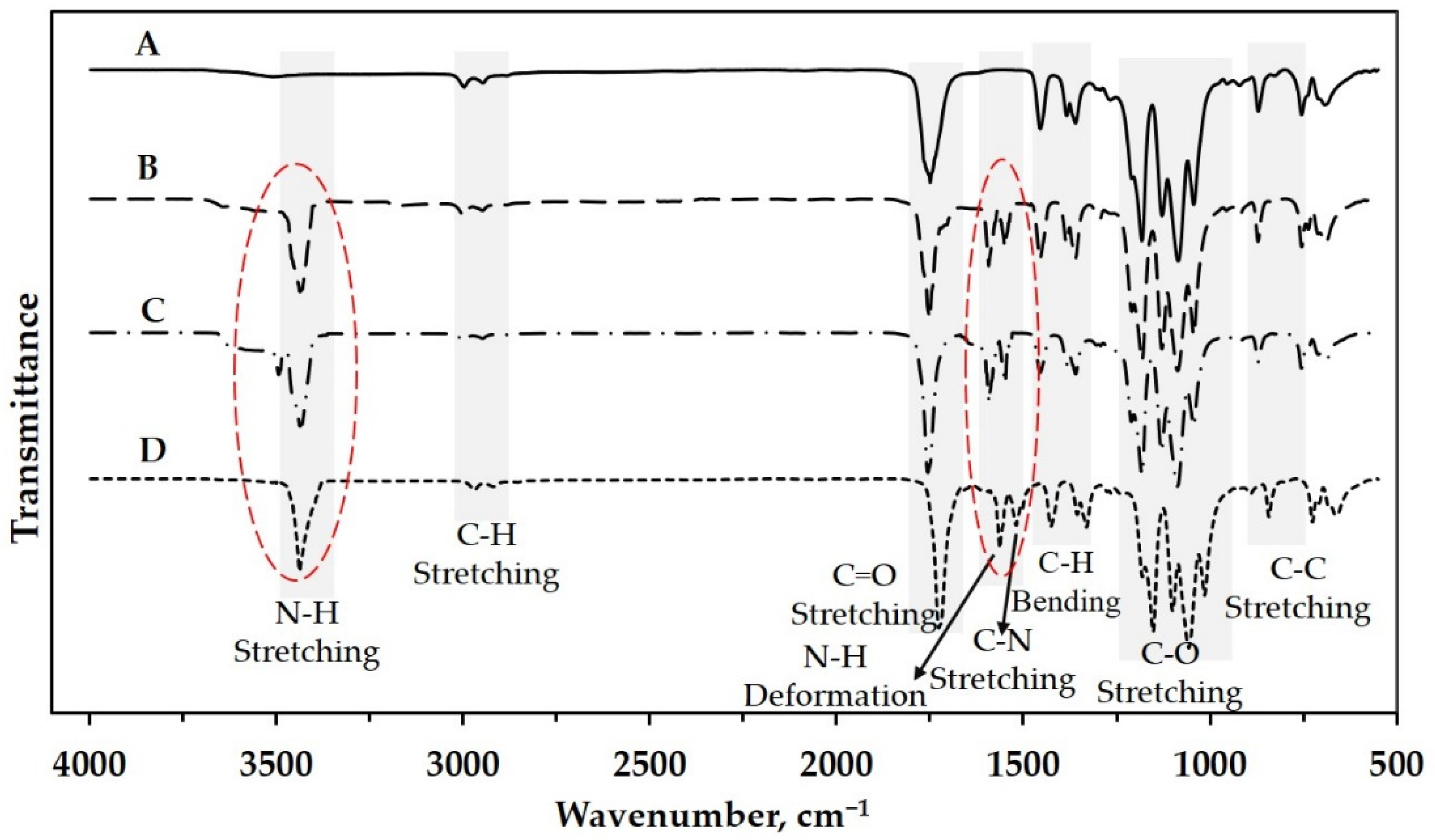
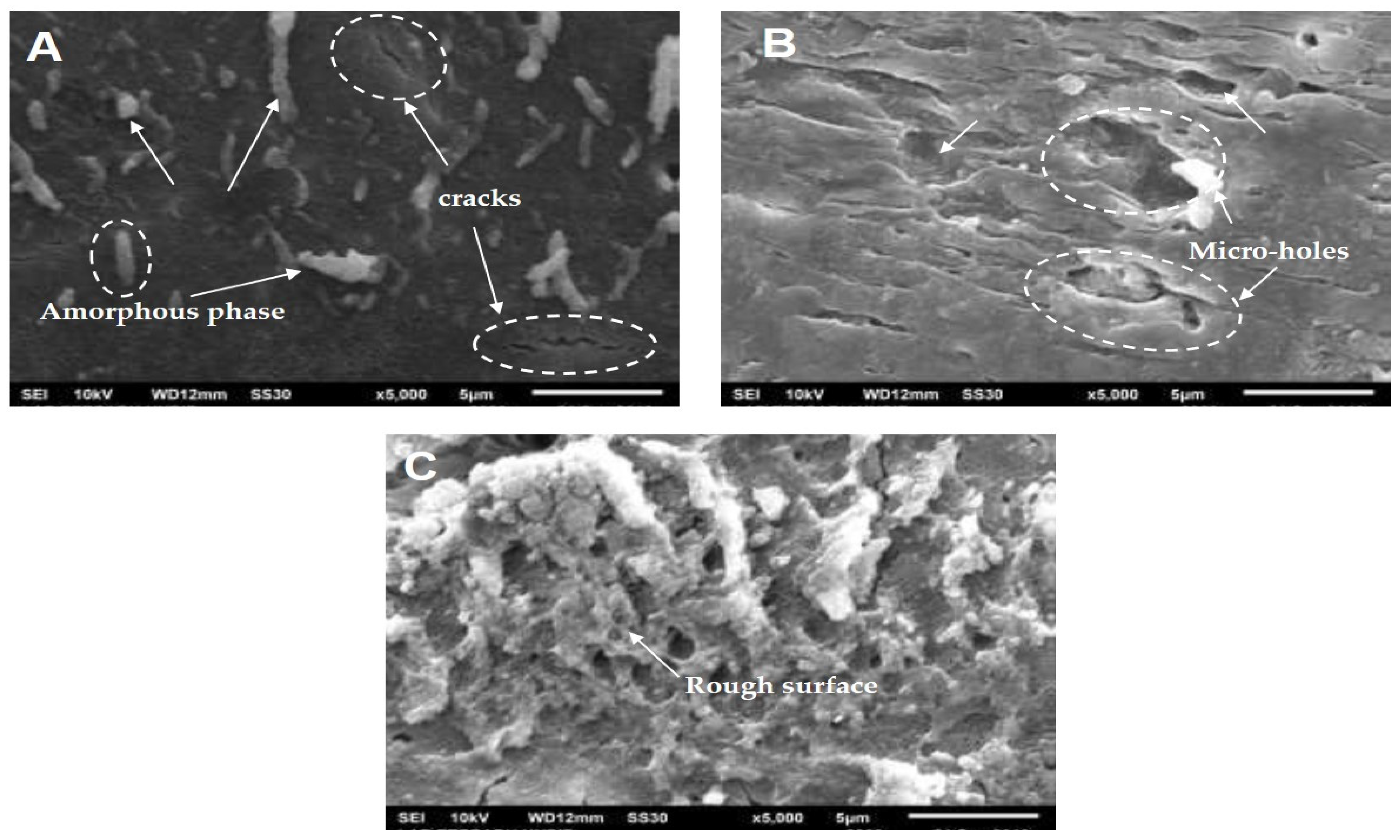
3.1. Molecular Structure of Slow-Release Fertilizer (SRF)
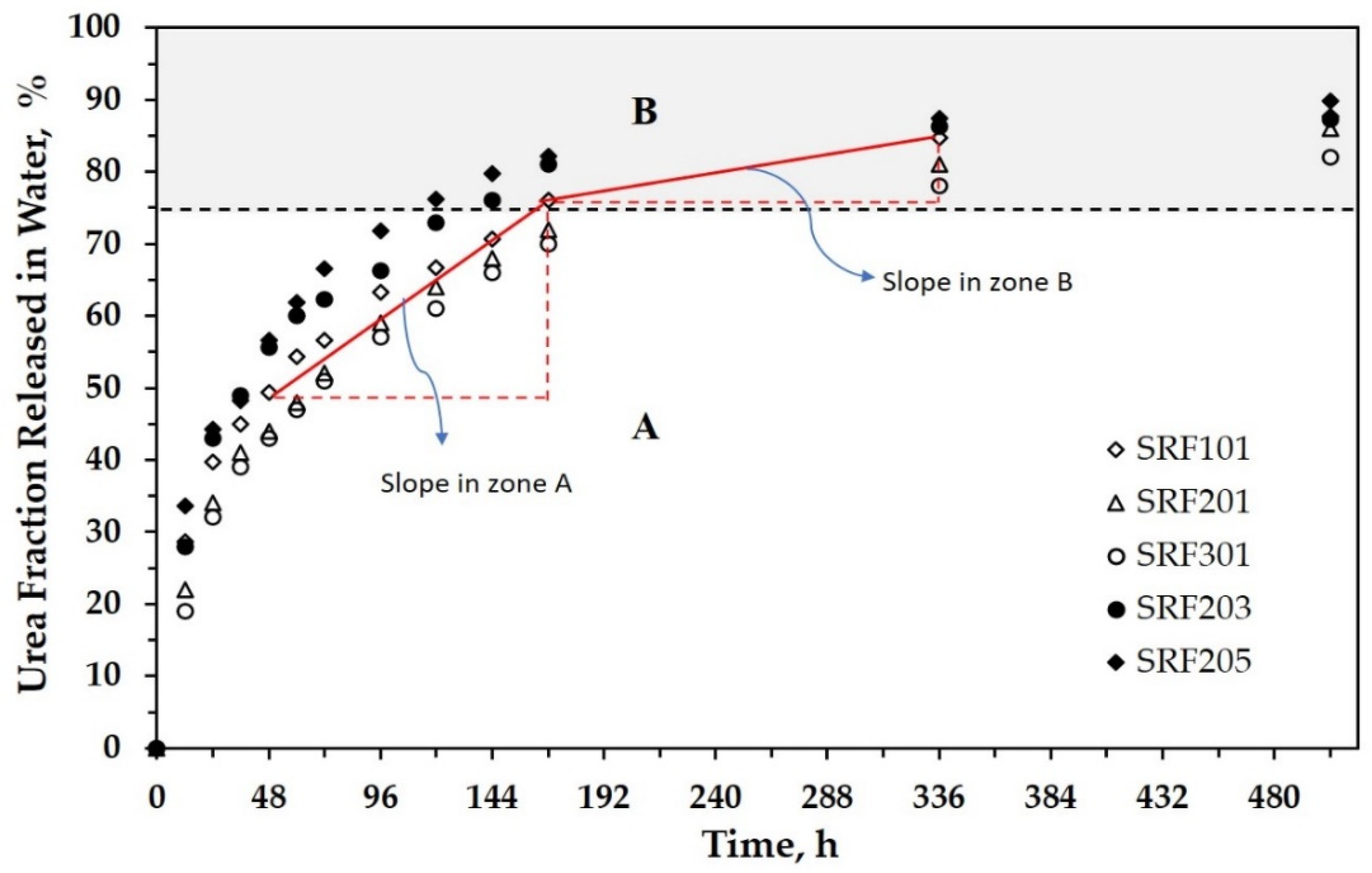
3.2. Urea Release Behavior
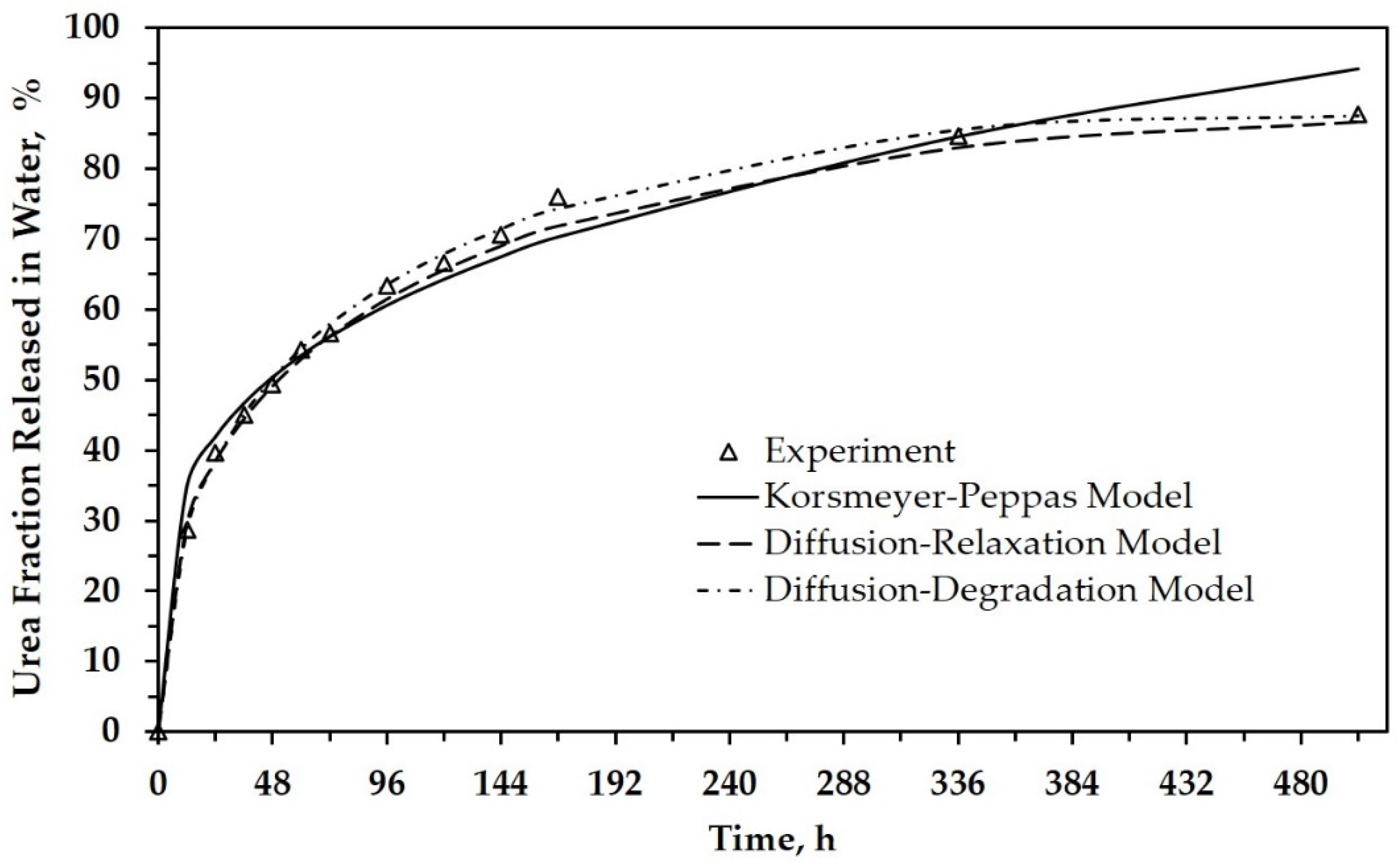
3.3. Modeling of Urea Release Behavior
3.4. Urea Release Duration
| Material + Modifier (Additive) | Preparation Method | Release Test | * Release Duration | Ref. |
|---|---|---|---|---|
| Mineral Natural bentonite + binder: corn starch or hydroxypropyl methyl-cellulose Montmorillonite clay (bentonite) + hydrophobic/hydrophilic polymer: polycaprolactone or polyacrylamide hydrogel | Melt blending Melt blending | Higuchi procedure in water at 30 °C Immersed in an aqueous medium at room temperature | 118 h or 48 h 30 h or 60 h | [9] [6] |
| Sulphur-based Phosphogypsum + paraffin wax + span-80 (as emulsifier) | Coating | Static release test in water at 25 °C | 240 h | [15] |
| Synthetic polymer Polyurethane + mesoporous silica Polystyrene + wax Polystyrene + polyurethane | Coating Coating | Immersed in deionized water at 25 °C Immersed in deionized water at 25 °C | 10–50 d 42 d 70 d | [33] [34] |
| Degradable synthetic polymer Polyesters: poly(hexamethylene succinate)/PHS Polyvinyl alcohol + biochar | Melt blending Melt blending | Immersed in deionized water at 25 °C Buried in soil column experiment at 25 °C | 400 h 25 d | [24] [16] |
| Natural polymer Starch + glycerol Alginate + Κ-carrageenan/celite superabsorbent Chitosan salicylaldehyde Poly(lactic acid) with a low molecular weight | Coating Coating Solvent casting Melt blending | Buried in compost soil at 25 °C Buried in soil at 25 °C Immersed in distilled water at 25 °C Static release test in water at 30 °C | 15–30 d 6 d 200 h 168 h | [32] [35] [36] This study |
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Forleo, M.B.; Palmieri, N.; Suardi, A.; Coaloa, D.; Pari, L. The eco-efficiency of rapeseed and sunflower cultivation in italy. Joining environmental and economic assessment. J. Clean. Prod. 2018, 172, 3138–3153. [Google Scholar] [CrossRef]
- Dwivedi, S.L.; Lammerts van Bueren, E.T.; Ceccarelli, S.; Grando, S.; Upadhyaya, H.D.; Ortiz, R. Diversifying food systems in the pursuit of sustainable food production and healthy diets. Trends Plant Sci. 2017, 22, 842–856. [Google Scholar] [CrossRef]
- Miao, Y.; Stewart, B.A.; Zhang, F. Long-term experiments for sustainable nutrient management in china. A review. Agron. Sustain. Dev. 2011, 31, 397–414. [Google Scholar] [CrossRef]
- Pypers, P.; Sanginga, J.-M.; Kasereka, B.; Walangululu, M.; Vanlauwe, B. Increased productivity through integrated soil fertility management in cassava–legume intercropping systems in the highlands of sud-kivu, dr congo. Field Crop. Res. 2011, 120, 76–85. [Google Scholar] [CrossRef]
- Chien, S.H.; Teixeira, L.A.; Cantarella, H.; Rehm, G.W.; Grant, C.A.; Gearhart, M.M. Agronomic effectiveness of granular nitrogen/phosphorus fertilizers containing elemental sulfur with and without ammonium sulfate: A review. Agron. J. 2016, 108, 1203–1213. [Google Scholar] [CrossRef]
- Pereira, E.I.; da Cruz, C.C.T.; Solomon, A.; Le, A.; Cavigelli, M.A.; Ribeiro, C. Novel slow-release nanocomposite nitrogen fertilizers: The impact of polymers on nanocomposite properties and function. Ind. Eng. Chem. Res. 2015, 54, 3717–3725. [Google Scholar] [CrossRef]
- Dubey, A.; Mailapalli, D.R. Development of control release urea fertilizer model for water and nitrogen movement in flooded rice. Paddy Water Environ. 2018, 16, 1–13. [Google Scholar] [CrossRef]
- Xiaoyu, N.; Yuejin, W.; Zhengyan, W.; Lin, W.; Guannan, Q.; Lixiang, Y. A novel slow-release urea fertiliser: Physical and chemical analysis of its structure and study of its release mechanism. Biosyst. Eng. 2013, 115, 274–282. [Google Scholar] [CrossRef]
- Hermida, L.; Agustian, J. Slow release urea fertilizer synthesized through recrystallization of urea incorporating natural bentonite using various binders. Environ. Technol. Innov. 2019, 13, 113–121. [Google Scholar] [CrossRef]
- Spiertz, J.H.J. Nitrogen, sustainable agriculture and food security. A review. Agron. Sustain. Dev. 2010, 30, 43–55. [Google Scholar] [CrossRef]
- Jat, R.A.; Wani, S.P.; Sahrawat, K.L.; Singh, P.; Dhaka, S.R.; Dhaka, B.L. Recent approaches in nitrogen management for sustainable agricultural production and eco-safety. Arch. Agron. Soil Sci. 2012, 58, 1033–1060. [Google Scholar] [CrossRef]
- Lawrencia, D.; Wong, S.K.; Low, D.Y.S.; Goh, B.H.; Goh, J.K.; Ruktanonchai, U.R.; Soottitantawat, A.; Lee, L.H.; Tang, S.Y. Controlled release fertilizers: A review on coating materials and mechanism of release. Plants 2021, 10, 238. [Google Scholar] [CrossRef] [PubMed]
- Latifah, O.; Ahmed, O.H.; Majid, N.M.A. Enhancing nitrogen availability from urea using clinoptilolite zeolite. Geoderma 2017, 306, 152–159. [Google Scholar] [CrossRef]
- Lateef, A.; Nazir, R.; Jamil, N.; Alam, S.; Shah, R.; Khan, M.N.; Saleem, M. Synthesis and characterization of zeolite based nano–composite: An environment friendly slow release fertilizer. Microporous Mesoporous Mater. 2016, 232, 174–183. [Google Scholar] [CrossRef]
- Yu, X.; Li, B. Release mechanism of a novel slow-release nitrogen fertilizer. Particuology 2019, 45, 124–130. [Google Scholar] [CrossRef]
- Chen, S.; Yang, M.; Ba, C.; Yu, S.; Jiang, Y.; Zou, H.; Zhang, Y. Preparation and characterization of slow-release fertilizer encapsulated by biochar-based waterborne copolymers. Sci. Total Environ. 2018, 615, 431–437. [Google Scholar] [CrossRef]
- Qi, X.; Ren, Y.; Wang, X. New advances in the biodegradation of poly(lactic) acid. Int. Biodeterior. Biodegrad. 2017, 117, 215–223. [Google Scholar] [CrossRef]
- Valentina, I.; Haroutioun, A.; Fabrice, L.; Vincent, V.; Roberto, P. Poly(lactic acid)-based nanobiocomposites with modulated degradation rates. Materials 2018, 11, 1943. [Google Scholar] [CrossRef]
- Kaavessina, M.; Distantina, S.; Chafidz, A.; Fadilah; Al-Zahrani, S. The influences of elastomer toward degradability of poly (lactic acid). Aip Conf. Proc. 2016, 1710, 030031. [Google Scholar]
- Kaavessina, M.; Chafidz, A.; Distantina, S.; Al-Zahrani, S.M. Characterization of poly (lactic acid) synthesized via direct polycondensation with different treatments of sncl2 as a catalyst. Arpn J. Eng. Appl. Sci. 2016, 11, 9992–9998. [Google Scholar]
- Ritger, P.L.; Peppas, N.A. A simple equation for description of solute release ii. Fickian and anomalous release from swellable devices. J. Control. Release 1987, 5, 37–42. [Google Scholar] [CrossRef]
- Dash, S.; Murthy, P.N.; Nath, L.; Chowdhury, P. Kinetic modeling on drug release from controlled drug delivery systems. Acta Pol. Pharm. 2010, 67, 217–223. [Google Scholar]
- Peppas, N.A.; Sahlin, J.J. A simple equation for the description of solute release. Iii. Coupling of diffusion and relaxation. Int. J. Pharm. 1989, 57, 169–172. [Google Scholar] [CrossRef]
- Bi, S.; Barinelli, V.; Sobkowicz, M.J. Degradable controlled release fertilizer composite prepared via extrusion: Fabrication, characterization, and release mechanisms. Polymers 2020, 12, 301. [Google Scholar] [CrossRef]
- Garlotta, D. A literature review of poly(lactic acid). J. Polym. Environ. 2001, 9, 63–84. [Google Scholar] [CrossRef]
- Manivannan, M.; Rajendran, S. Investigation of inhibitive action of urea-zn2+ system in the corrosion control of carbon steel in sea water. Int. J. Eng. Sci. Technol. 2011, 3, 8048–8060. [Google Scholar]
- Bull, H.B.; Breese, K.; Ferguson, G.L.; Swenson, C.A. The ph of urea solutions. Arch. Biochem. Biophys. 1964, 104, 297–304. [Google Scholar] [CrossRef]
- Upadrashta, S.; Katikaneni, P.; Hileman, G.; Keshary, P. Direct compression controlled release tablets using ethylcellulose matrices. Drug Dev. Ind. Pharm. 2008, 19, 449–460. [Google Scholar] [CrossRef]
- Kaavessina, M.; Chafidz, A.; Ali, I.; Al-Zahrani, S.M. Characterization of poly(lactic acid)/hydroxyapatite prepared by a solvent-blending technique: Viscoelasticity and in vitro hydrolytic degradation. J. Elastomers Plast. 2014, 47, 753–768. [Google Scholar] [CrossRef]
- Elsawy, M.; Kim, K.-H.; Park, J.-W.; Deep, A. Hydrolytic degradation of polylactic acid (pla) and its composites. Renew. Sustain. Energy Rev. 2017, 79, 1346–1352. [Google Scholar] [CrossRef]
- Ndazi, B.S.; Karlsson, S. Characterization of hydrolytic degradation of polylactic acid/rice hulls composites in water at different temperatures. Express Polym. Lett. 2011, 5, 119–131. [Google Scholar] [CrossRef]
- Versino, F.; Urriza, M.; García, M.A. Eco-compatible cassava starch films for fertilizer controlled-release. Int. J. Biol. Macromol. 2019, 134, 302–307. [Google Scholar] [CrossRef]
- Li, L.; Sun, Y.; Cao, B.; Song, H.; Xiao, Q.; Yi, W. Preparation and performance of polyurethane/mesoporous silica composites for coated urea. Mater. Des. 2016, 99, 21–25. [Google Scholar] [CrossRef]
- Yang, Y.-c.; Zhang, M.; Li, Y.; Fan, X.-h.; Geng, Y.-q. Improving the quality of polymer-coated urea with recycled plastic, proper additives, and large tablets. J. Agric. Food Chem. 2012, 60, 11229–11237. [Google Scholar] [CrossRef]
- Wang, Y.; Liu, M.; Ni, B.; Xie, L. Κ-carrageenan–sodium alginate beads and superabsorbent coated nitrogen fertilizer with slow-release, water-retention, and anticompaction properties. Ind. Eng. Chem. Res. 2012, 51, 1413–1422. [Google Scholar] [CrossRef]
- Iftime, M.M.; Ailiesei, G.L.; Ungureanu, E.; Marin, L. Designing chitosan based eco-friendly multifunctional soil conditioner systems with urea controlled release and water retention. Carbohydr. Polym. 2019, 223, 115040. [Google Scholar] [CrossRef]

| Sample | Polymerization Time, h | Average MW of PLA, Da | Urea Content in 3 g of SRF, g |
|---|---|---|---|
| Neat PLA | 16 | 6015.2 | 0 |
| SRF101 | 16 | 6015.2 | 0.01 |
| SRF201 | 24 | 10,264.7 | 0.01 |
| SRF301 | 32 | 13,564.2 | 0.01 |
| SRF203 | 24 | 10,264.7 | 0.03 |
| SRF205 | 24 | 10,264.7 | 0.05 |
| Time, h | Acidity (pH) of Solution | |||||
|---|---|---|---|---|---|---|
| Neat PLA | SRF101 | SRF201 | SRF301 | SRF203 | SRF205 | |
| 0 | 6.80 ± 0.01 | 6.80 ± 0.01 | 6.80 ± 0.01 | 6.80 ± 0.01 | 6.80 ± 0.01 | 6.80 ± 0.01 |
| 12 | 6.79 ± 0.02 | 7.27 ± 0.05 | 6.95 ± 0.08 | 6.97 ± 0.05 | 7.19 ± 0.05 | 7.26 ± 0.06 |
| 24 | 6.78 ± 0.02 | 7.39 ± 0.05 | 7.08 ± 0.03 | 7.06 ± 0.05 | 7.33 ± 0.06 | 7.43 ± 0.04 |
| 32 | 6.76 ± 0.02 | 7.52 ± 0.06 | 7.15 ± 0.03 | 7.16 ± 0.06 | 7.41 ± 0.06 | 7.59 ± 0.03 |
| 48 | 6.75 ± 0.01 | 7.55 ± 0.03 | 7.20 ± 0.03 | 7.23 ± 0.07 | 7.54 ± 0.05 | 7.70 ± 0.03 |
| 60 | 6.74 ± 0.01 | 7.38 ± 0.03 | 7.29 ± 0.08 | 7.33 ± 0.05 | 7.67 ± 0.04 | 7.81 ± 0.03 |
| 72 | 6.72 ± 0.01 | 7.22 ± 0.05 | 7.35 ± 0.08 | 7.34 ± 0.03 | 7.71 ± 0.06 | 7.63 ± 0.05 |
| 96 | 6.69 ± 0.02 | 7.19 ± 0.04 | 7.33 ± 0.07 | 7.29 ± 0.04 | 7.57 ± 0.06 | 7.58 ± 0.05 |
| 120 | 6.62 ± 0.04 | 7.16 ± 0.03 | 7.25 ± 0.07 | 7.20 ± 0.06 | 7.51 ± 0.08 | 7.52 ± 0.03 |
| 144 | 6.50 ± 0.04 | 7.08 ± 0.05 | 7.16 ± 0.05 | 7.15 ± 0.08 | 7.50 ± 0.06 | 7.48 ± 0.04 |
| 168 | 6.45 ± 0.03 | 7.01 ± 0.03 | 7.10 ± 0.06 | 7.13 ± 0.05 | 7.44 ± 0.05 | 7.43 ± 0.02 |
| 336 | 6.34 ± 0.04 | 6.96 ± 0.05 | 7.04 ± 0.05 | 7.06 ± 0.04 | 7.38 ± 0.03 | 7.37 ± 0.03 |
| 504 | 6.20 ± 0.03 | 6.92 ± 0.04 | 6.99 ± 0.03 | 7.01 ± 0.09 | 7.31 ± 0.06 | 7.33 ± 0.06 |
| Sample | Diffusion/Korsmeyer–Peppas Model | |||
|---|---|---|---|---|
| k | n | R2 | Type of Diffusion | |
| Neat PLA | - | - | - | - |
| SRF101 | 0.1796 ± 0.0157 | 0.2663 ± 0.0173 | 0.9789 | Fickian |
| SRF201 | 0.1406 ± 0.0155 | 0.3028 ± 0.0216 | 0.9721 | Fickian |
| SRF301 | 0.1343 ± 0.0173 | 0.3043 ± 0.0251 | 0.9629 | Fickian |
| SRF203 | 0.2142 ± 0.0262 | 0.2417 ± 0.0244 | 0.9553 | Fickian |
| SRF205 | 0.2334 ± 0.0274 | 0.2315 ± 0.0235 | 0.9524 | Fickian |
| Sample | Diffusion–Relaxation Model | ||
|---|---|---|---|
| k1 | k2 | R2 | |
| Neat PLA | - | - | - |
| SRF101 | 0.1119 ± 1.98 × 10−3 | −0.0036 ± 1.84 × 10−4 | 0.9949 |
| SRF201 | 0.1015 ± 2.56 × 10−3 | −0.0028 ± 2.39 × 10−4 | 0.9916 |
| SRF301 | 0.0981 ± 3.16 × 10−3 | −0.0028 ± 2.95 × 10−4 | 0.9865 |
| SRF203 | 0.1284 ± 2.27 × 10−3 | −0.0047 ± 2.11 × 10−4 | 0.9972 |
| SRF205 | 0.1352 ± 1.95 × 10−3 | −0.0051 ± 1.81 × 10−4 | 0.9969 |
| Sample | Diffusion–Degradation Model | ||||
|---|---|---|---|---|---|
| a | b | c | d | R2 | |
| Neat PLA | - | - | - | - | - |
| SRF101 | 0.0932 ± 2.67 × 10−3 | −3.11 × 10−3 ± 3.86 × 10−4 | 2.32 × 10−6 ± 1.26 × 10−7 | −1.80 × 10−9 ± 1.51 × 10−7 | 0.9987 |
| SRF201 | 0.0705 ± 2.84 × 10−3 | −7.54 × 10−4 ± 4.12 × 10−5 | −3.33 × 10−6 ± 1.35 × 10−7 | 3.92 × 10−9 ± 1.61 × 10−10 | 0.9985 |
| SRF301 | 0.0656 ± 5.77 × 10−3 | −6.83 × 10−5 ± 8.36 × 10−5 | −5.80 × 10−6 ± 2.74 × 10−7 | 6.91 × 10−9 ± 2.74 × 10−10 | 0.9936 |
| SRF203 | 0.0986 ± 4.19 × 10−3 | −2.91 × 10−3 ± 6.07 × 10−4 | −6.21 × 10−8 ± 1.99 × 10−9 | 1.10 × 10−9 ± 2.37 × 10−10 | 0.9972 |
| SRF205 | 0.1028 ± 3.10 × 10−3 | −2.92 × 10−3 ± 4.48 × 10−4 | −1.23 × 10−6 ± 1.47 × 10−7 | 2.92 × 10−9 ± 1.75 × 10−10 | 0.9984 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kaavessina, M.; Distantina, S.; Shohih, E.N. A Slow-Release Fertilizer of Urea Prepared via Melt Blending with Degradable Poly(lactic acid): Formulation and Release Mechanisms. Polymers 2021, 13, 1856. https://doi.org/10.3390/polym13111856
Kaavessina M, Distantina S, Shohih EN. A Slow-Release Fertilizer of Urea Prepared via Melt Blending with Degradable Poly(lactic acid): Formulation and Release Mechanisms. Polymers. 2021; 13(11):1856. https://doi.org/10.3390/polym13111856
Chicago/Turabian StyleKaavessina, Mujtahid, Sperisa Distantina, and Esa Nur Shohih. 2021. "A Slow-Release Fertilizer of Urea Prepared via Melt Blending with Degradable Poly(lactic acid): Formulation and Release Mechanisms" Polymers 13, no. 11: 1856. https://doi.org/10.3390/polym13111856
APA StyleKaavessina, M., Distantina, S., & Shohih, E. N. (2021). A Slow-Release Fertilizer of Urea Prepared via Melt Blending with Degradable Poly(lactic acid): Formulation and Release Mechanisms. Polymers, 13(11), 1856. https://doi.org/10.3390/polym13111856







