Manufacturing, Characterisation and Mechanical Analysis of Polyacrylonitrile Membranes
Abstract
1. Introduction
2. Materials and Methods
2.1. Preparation of Dope Solutions
2.2. Membrane Forming
2.3. Characterisation of Membranes
3. Numerical Study
3.1. Modelling of Membranes
3.2. Modelling of Representative Elements
4. Results and Discussion
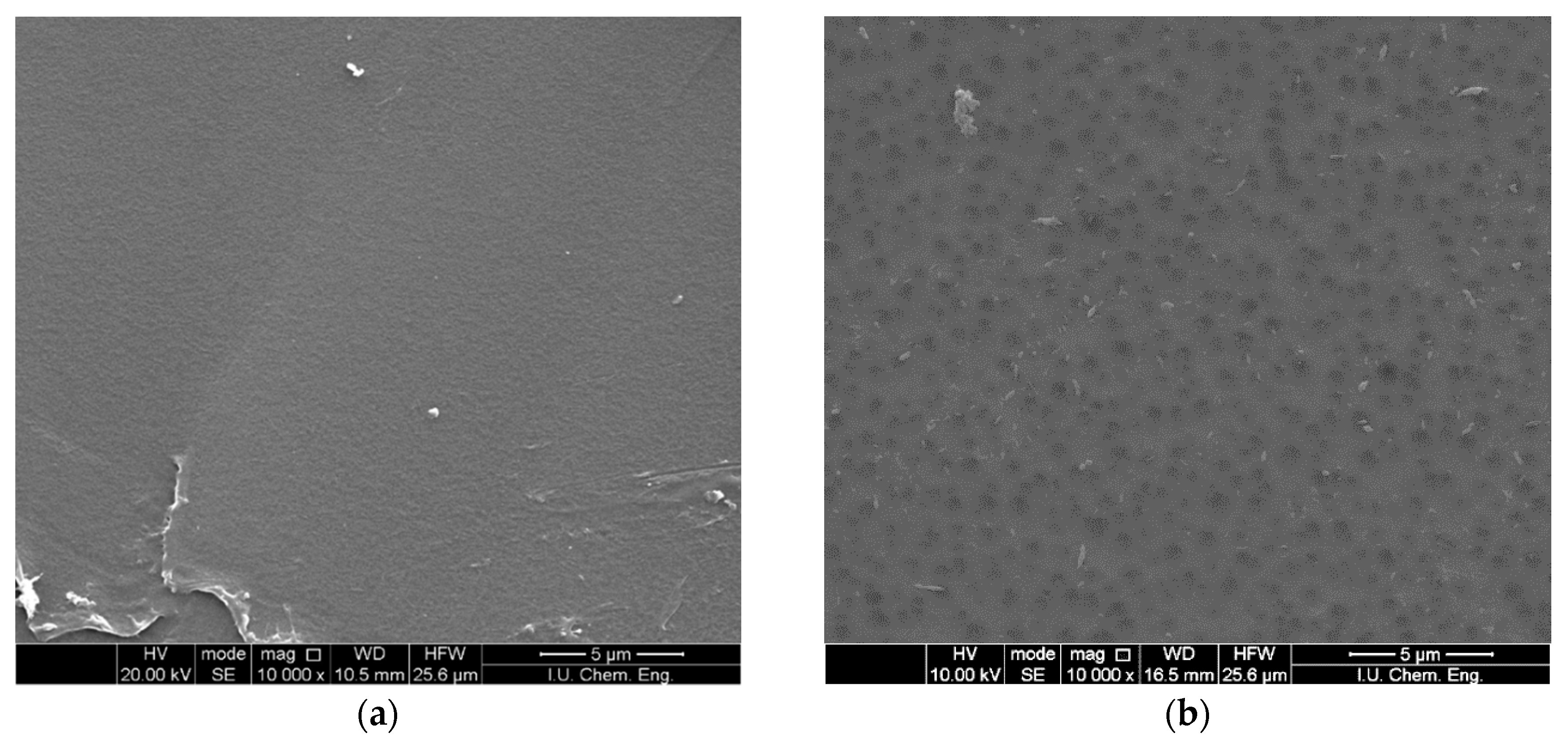
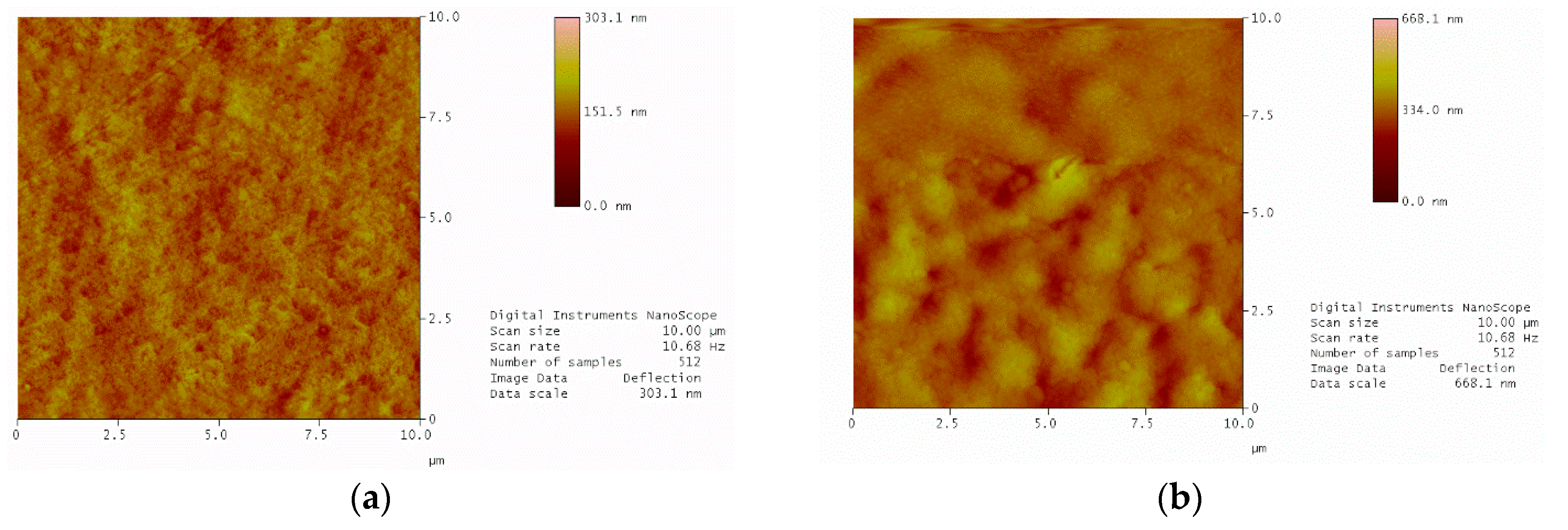
4.1. Material Characterisation
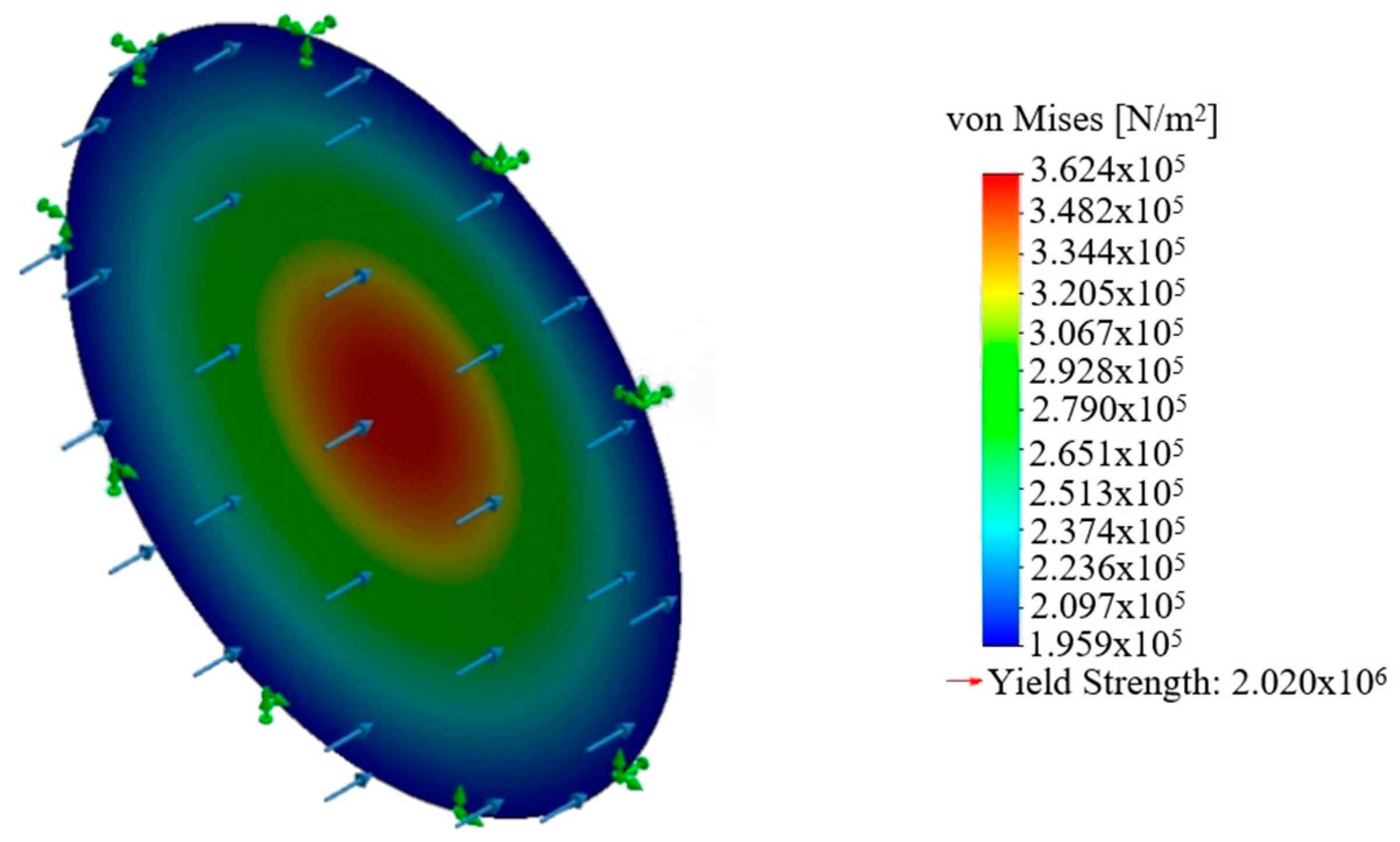
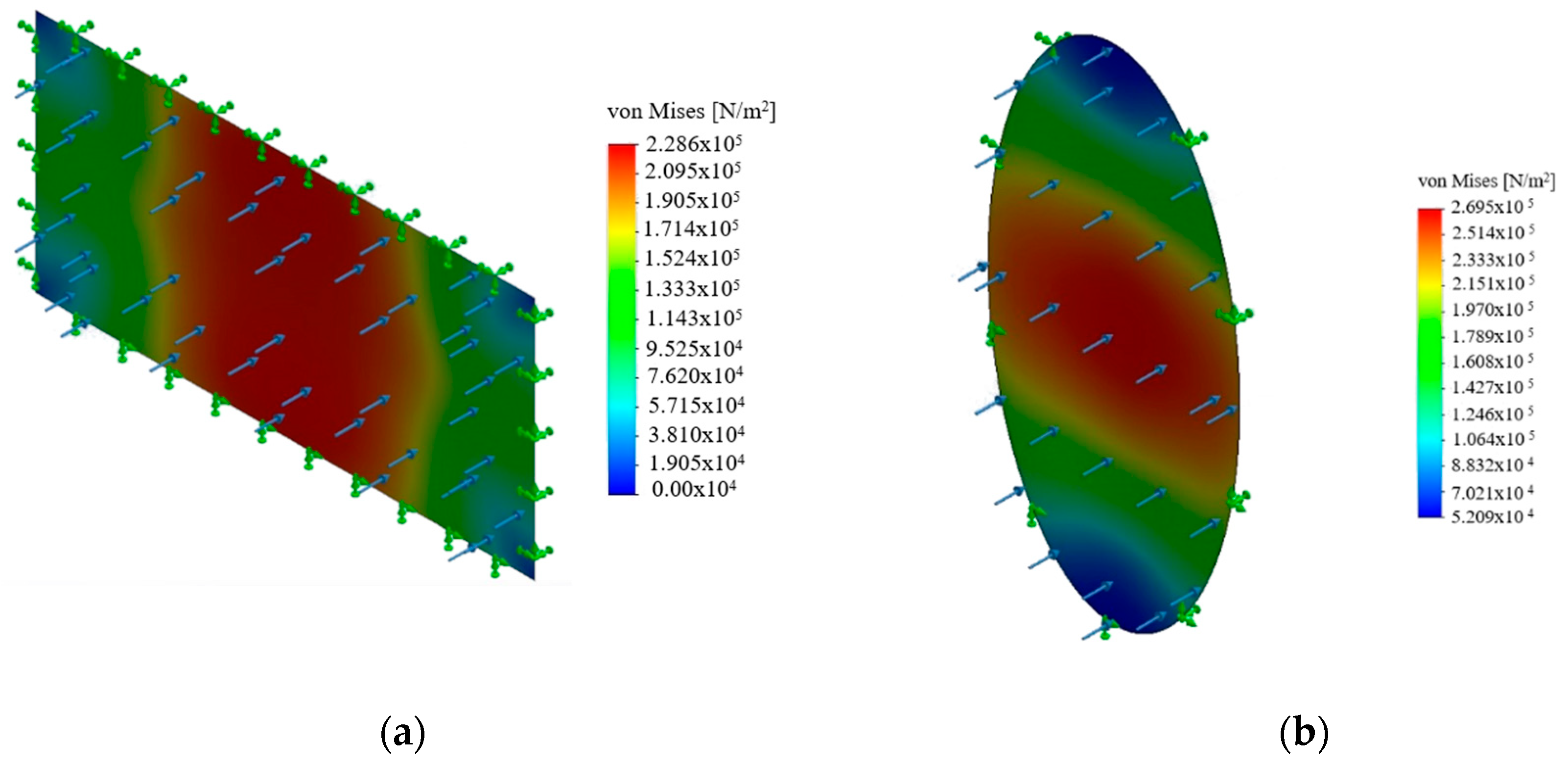
4.2. Numerical Study: Modelling of Membranes
4.2.1. Investigation of Membranes Made of PAN 1
4.2.2. Investigation of Membranes Made of PAN 2
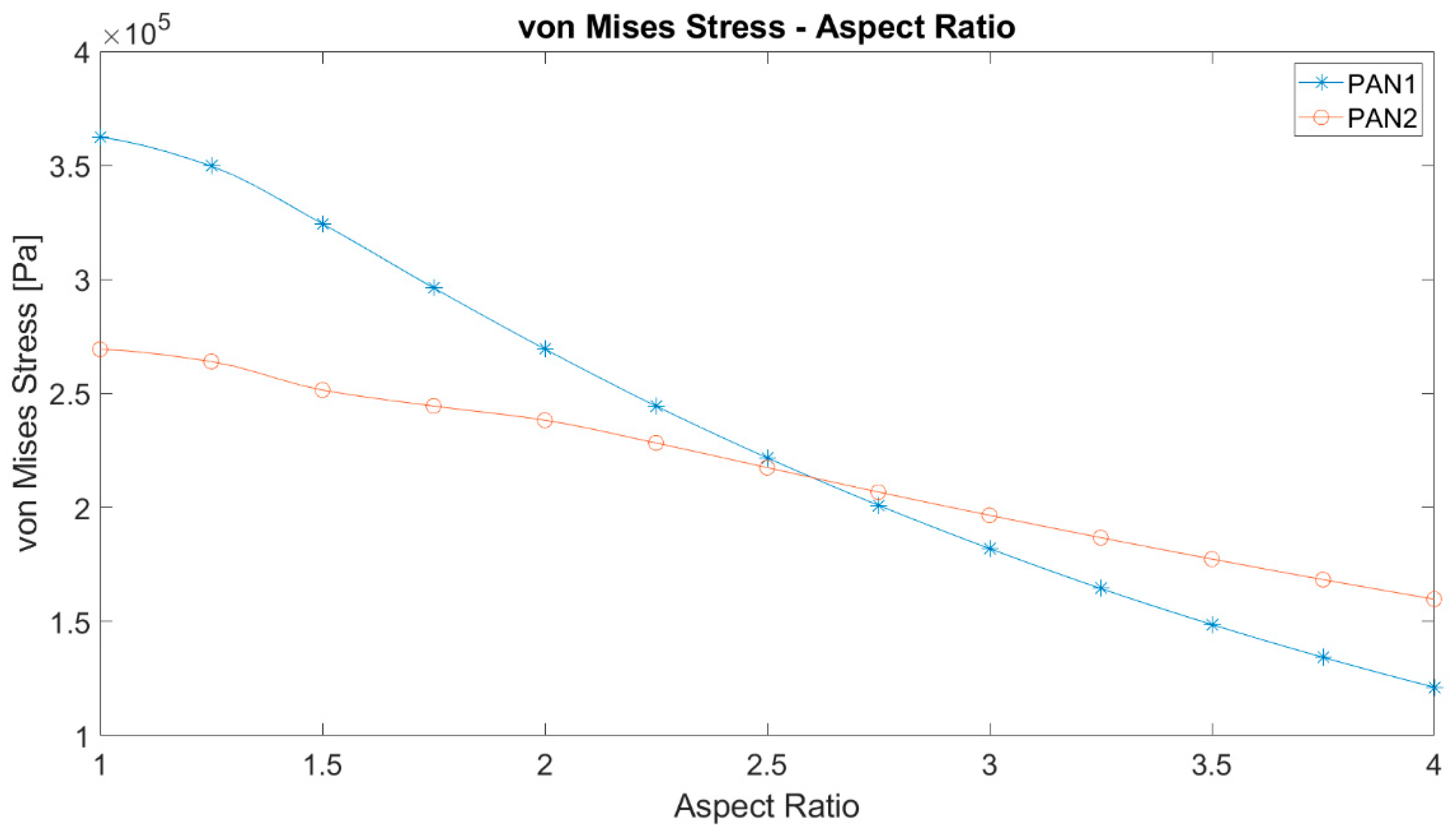
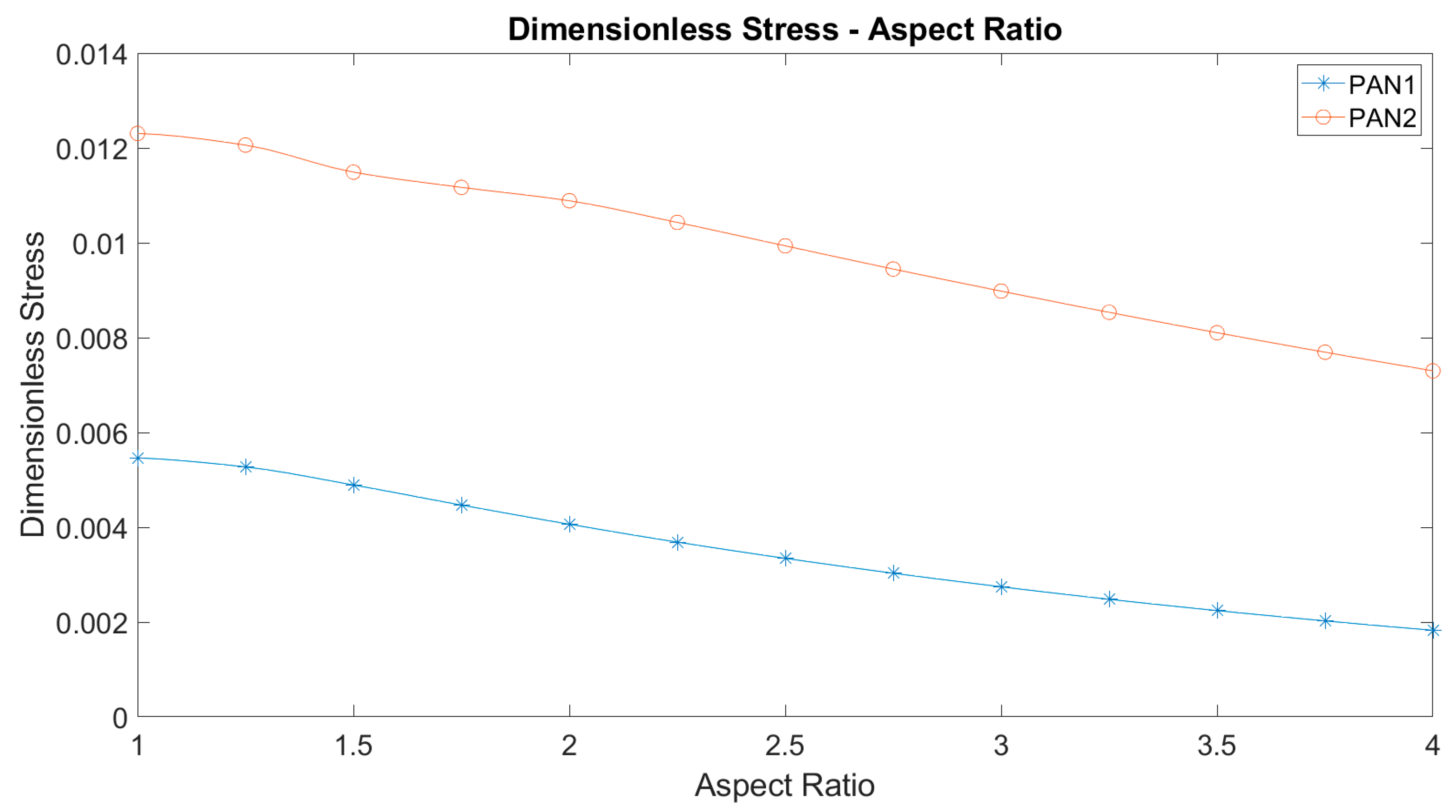
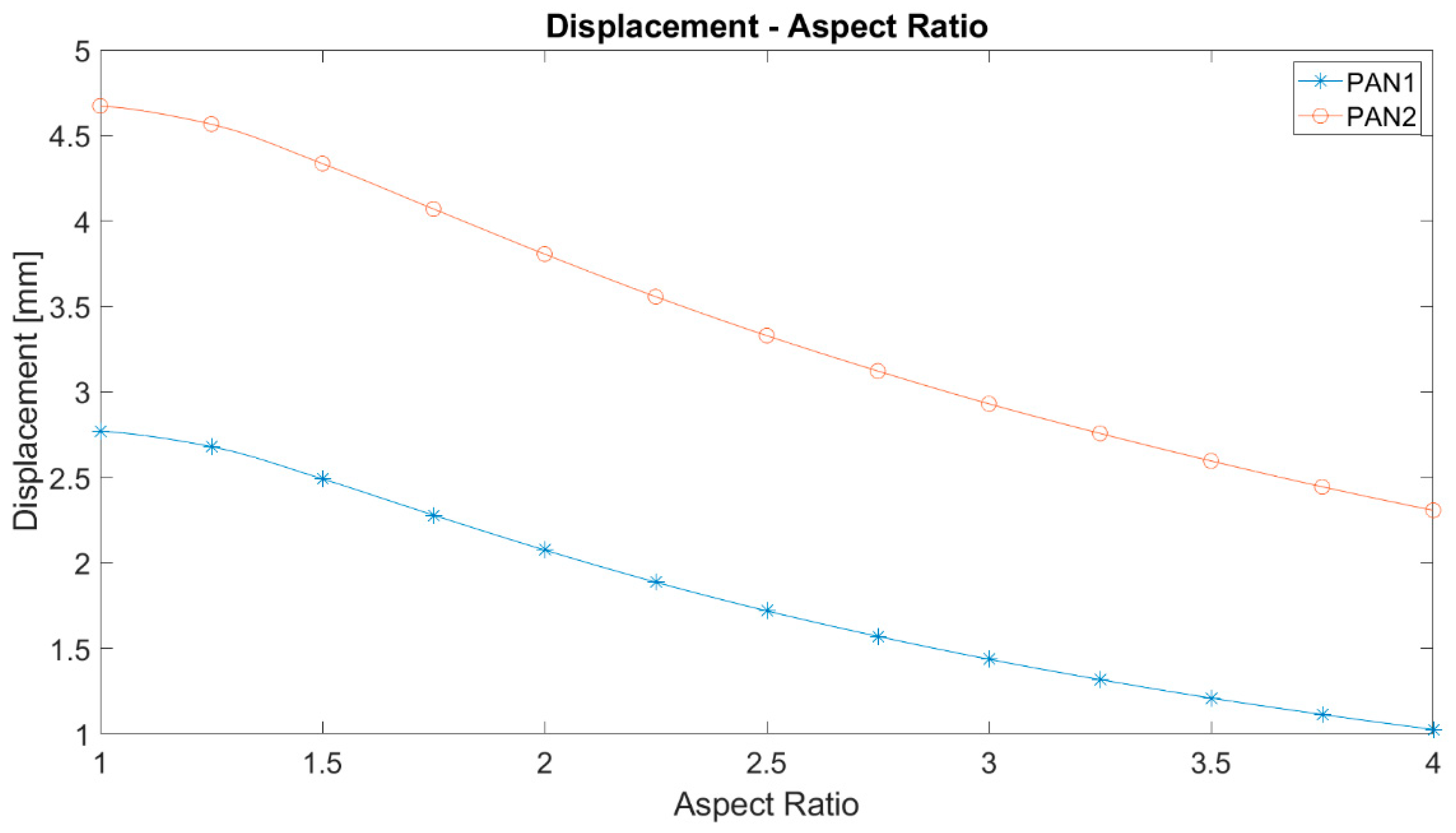
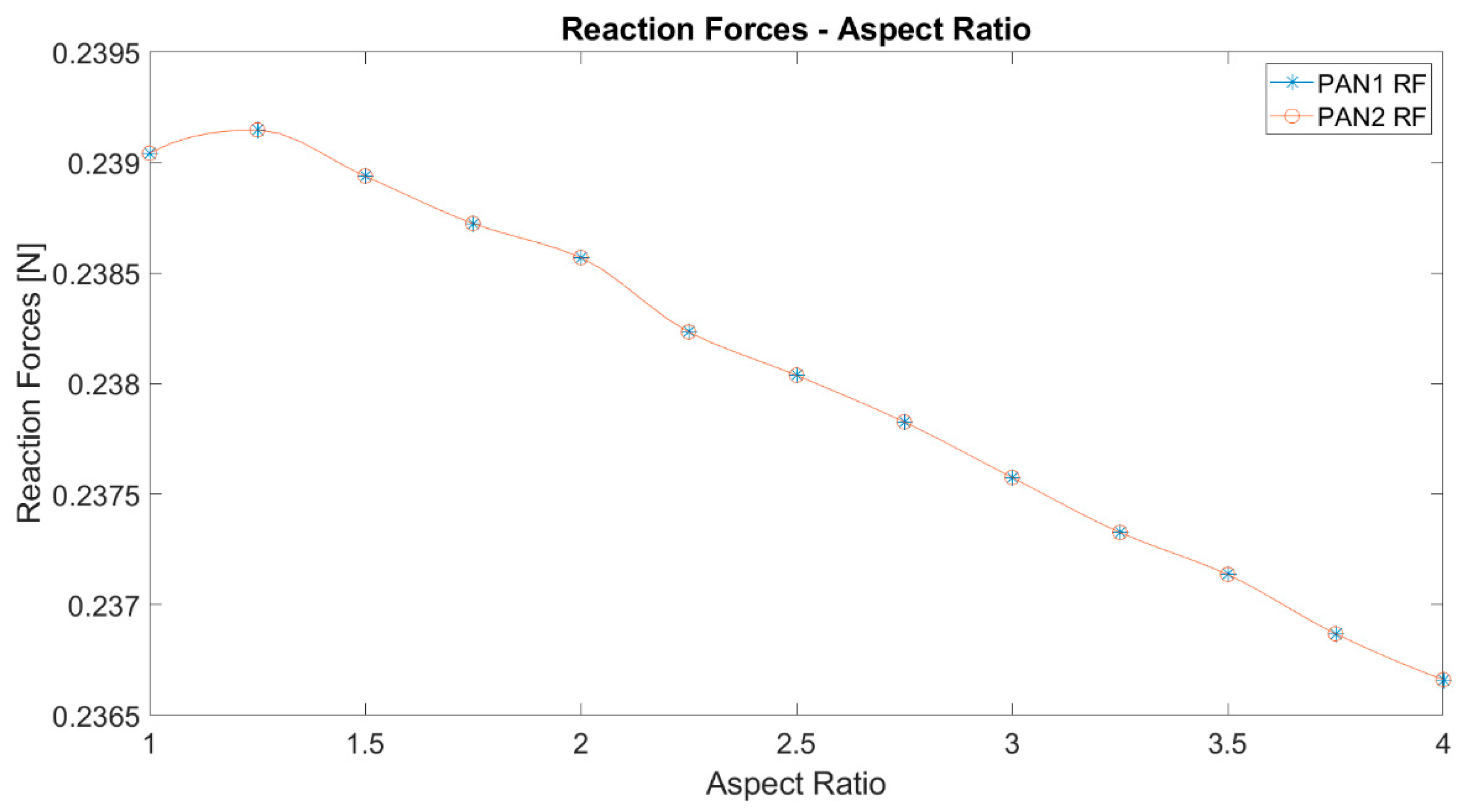
4.2.3. Investigation of Elliptical Membranes with Different Aspect Ratios
4.2.4. Final Remarks
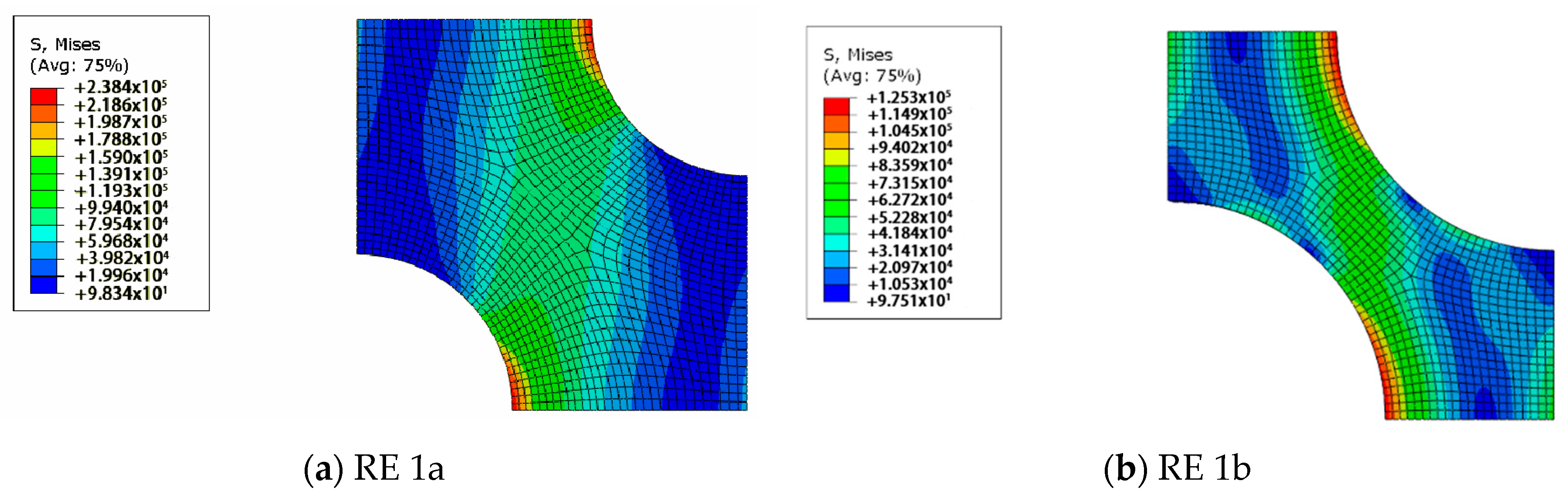
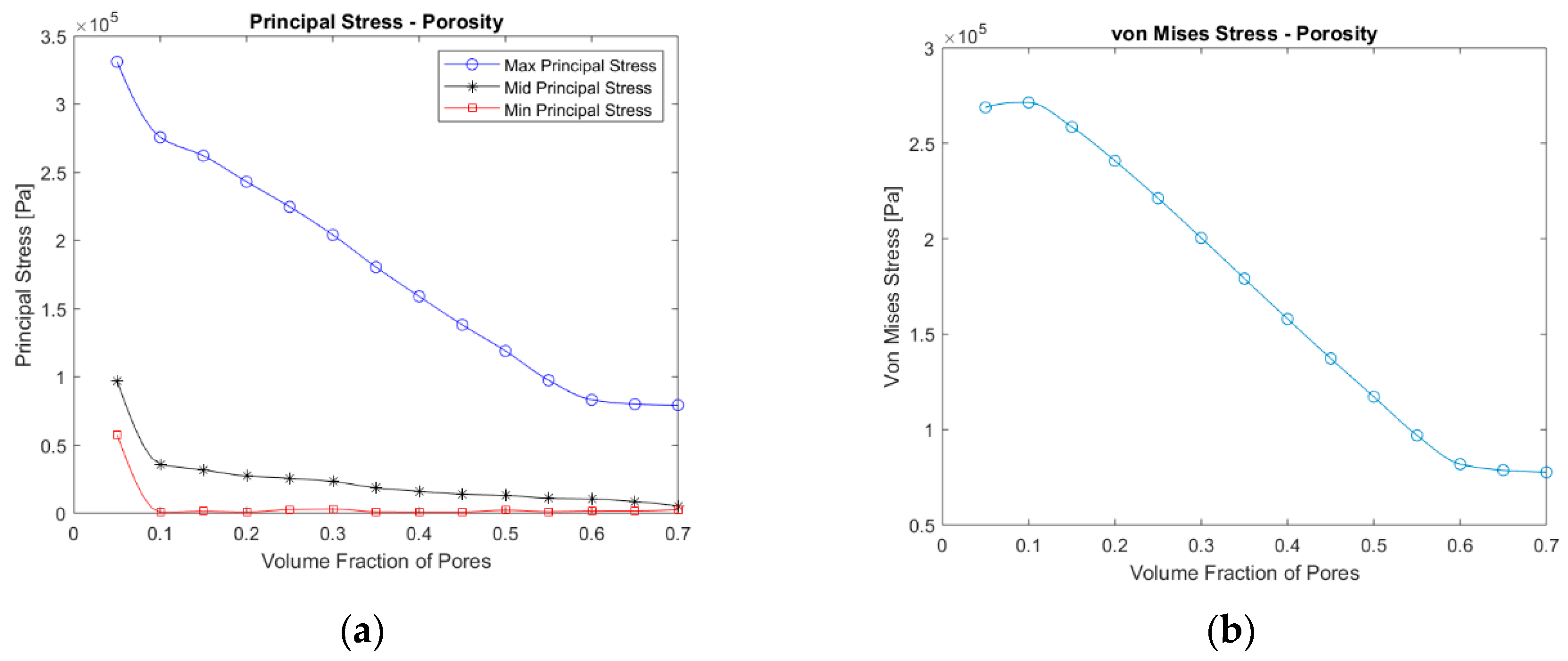
4.3. Numerical Study: Modelling of Representative Elements
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- García Doménech, N.; Purcell-Milton, F.; Gun’ko, Y.K. Recent progress and future prospects in development of advanced materials for nanofiltration. Mater. Today Commun. 2020, 23. [Google Scholar] [CrossRef]
- Dixit, S.; Pal, S. Recent advanced technologies in the processing of hybrid reinforced polymers for applications of membranes. Polym. Polym. Compos. 2016, 24, 289–305. [Google Scholar] [CrossRef]
- Krason, J.; Pietrzak, R. Removal of Iron and Copper Ions from the Liquid Phase by Modified Polymeric Membranes. J. Polym. Environ. 2018, 26, 3237–3242. [Google Scholar] [CrossRef]
- Bandehali, S.; Parvizian, F.; Moghadassi, A.R.; Hosseini, S.M.; Shen, J.N. Fabrication of thin film-PEI nanofiltration membrane with promoted separation performances: Cr, Pb and Cu ions removal from water. J. Polym. Res. 2020, 27. [Google Scholar] [CrossRef]
- Mokhtar, N.M.; Lau, W.J.; Ismail, A.F. Dye wastewater treatment by direct contact membrane distillation using polyvinylidene fluoride hollow fiber membranes. J. Polym. Eng. 2015, 35, 471–479. [Google Scholar] [CrossRef]
- Farjami, M.; Vatanpour, V.; Moghadassi, A. Influence of the various pore former additives on the performance and characteristics of the bare and EPVC/boehmite nanocomposite ultrafiltration membranes. Mater. Today Commun. 2019, 21, 100663. [Google Scholar] [CrossRef]
- Tan, X.M.; Rodrigue, D. A Review on Porous Polymeric Membrane Preparation. Part I: Production Techniques with Polysulfone and Poly (Vinylidene Fluoride). Polymers 2019, 11, 1160. [Google Scholar] [CrossRef]
- Tan, X.M.; Rodrigue, D. A review on porous polymeric membrane preparation. Part II: Production techniques with polyethylene, polydimethylsiloxane, polypropylene, polyimide, and polytetrafluoroethylene. Polymers 2019, 11, 1310. [Google Scholar] [CrossRef]
- Anderson, W.B.; Lakes, R.S. Size effects due to Cosserat elasticity and surface damage in closed-cell polymethacrylimide foam. J. Mater. Sci. 1994, 29, 6413–6419. [Google Scholar] [CrossRef]
- Dai, S.; Cunningham, P.R. Multi-scale damage modelling of 3D woven composites under uni-axial tension. Compos. Struct. 2016, 142, 298–312. [Google Scholar] [CrossRef]
- Yu, H.; Matthew, H.W.; Wooley, P.H.; Yang, S.Y. Effect of porosity and pore size on microstructures and mechanical properties of poly-e-caprolactone-hydroxyapatite composites. J. Biomed. Mater. Res. Part B Appl. Biomater. 2008, 86, 541–547. [Google Scholar] [CrossRef]
- Yasuda, H.; Tsai, J.T. Pore size of microporous polymer membranes. J. Appl. Polym. Sci. 1974, 18, 805–819. [Google Scholar] [CrossRef]
- Vikingsson, L.; Claessens, B.; Gómez-Tejedor, J.A.; Gallego Ferrer, G.; Gómez Ribelles, J.L. Relationship between micro-porosity, water permeability and mechanical behavior in scaffolds for cartilage engineering. J. Mech. Behav. Biomed. Mater. 2015, 48, 60–69. [Google Scholar] [CrossRef]
- Qin, J.J.; Cao, Y.M.; Li, Y.Q.; Li, Y.; Oo, M.H.; Lee, H. Hollow fiber ultrafiltration membranes made from blends of PAN and PVP. Sep. Purif. Technol. 2004, 36, 149–155. [Google Scholar] [CrossRef]
- Dai, J.; Liu, C.M.; Yang, J.H.; Wang, Y.; Zhang, C.L.; Zhou, Z.W. Largely enhanced effective porosity of uniaxial stretched polypropylene membrane achieved by pore-forming agent. J. Polym. Res. 2016, 23, 1–9. [Google Scholar] [CrossRef]
- Salleh, W.N.W.; Ismail, A.F. Carbon hollow fiber membranes derived from PEI/PVP for gas separation. Sep. Purif. Technol. 2011, 80, 541–548. [Google Scholar] [CrossRef]
- Güneş-Durak, S.; Ormancı-Acar, T.; Tüfekci, N. Effect of PVP content and polymer concentration on polyetherimide (PEI) and polyacrylonitrile (PAN) based ultrafiltration membrane fabrication and characterization. Water Sci. Technol. 2018, 2017, 329–339. [Google Scholar] [CrossRef]
- Salmanli, Ö.M.; Durak, S.G.; Demirkol, G.T.; Tüfekci, N. Effect of PVP concentration on prepared PEI membranes for potential use on water treatment: Effect of additive on membranes prepared for water treatment. Water Sci. Technol. Water Supply 2019, 19, 2072–2078. [Google Scholar] [CrossRef]
- Zhang, P.Y.; Wang, Y.L.; Xu, Z.L.; Yang, H. Preparation of poly (vinyl butyral) hollow fiber ultrafiltration membrane via wet-spinning method using PVP as additive. Desalination 2011, 278, 186–193. [Google Scholar] [CrossRef]
- Anvari, A.; Yancheshme, A.A.; Rekaabdar, F.; Hemmati, M.; Tavakolmoghadam, M.; Safekordi, A. PVDF/PAN Blend Membrane: Preparation, Characterization and Fouling Analysis. J. Polym. Environ. 2017, 25, 1348–1358. [Google Scholar] [CrossRef]
- Cui, Z.Y. Preparation of poly(vinylidene fluoride)/P123 blend microporous membranes via thermally induced phase separation process. Polym. Polym. Compos. 2012, 20, 237–252. [Google Scholar] [CrossRef]
- Qiu, F.; Zhang, J.; Wu, D.; Zhang, Q.; Yang, D. Preparation, characterisation and mechanical properties of environmentally friendly waterborne polyurethane and polyurethane-acrylate. Polym. Polym. Compos. 2009, 17, 521–527. [Google Scholar] [CrossRef]
- Ghaffarian, V.; Mousavi, S.M.; Bahreini, M.; Afifi, M. Preparation and Characterization of Biodegradable Blend Membranes of PBS/CA. J. Polym. Environ. 2013, 21, 1150–1157. [Google Scholar] [CrossRef]
- Karunakaran, M.; Kumar, M.; Shevate, R.; Akhtar, F.H.; Peinemann, K.V. CO2-philic thin film composite membranes: Synthesis and characterization of PAN-r-PEGMA copolymer. Polymers 2017, 9, 219. [Google Scholar] [CrossRef]
- Kim, S.Y.; Cho, Y.; Kang, S.W. Preparation and characterization of PEBAX-5513/AgBF4/BMIMBF4 membranes for olefin/paraffin separation. Polymers 2020, 12, 1550. [Google Scholar] [CrossRef]
- Pérez-Álvarez, L.; Ruiz-Rubio, L.; Moreno, I.; Vilas-Vilela, J.L. Characterization and optimization of the alkaline hydrolysis of polyacrylonitrile membranes. Polymers 2019, 11, 1843. [Google Scholar] [CrossRef]
- Shao, H.; Wei, F.; Luo, D.; Zhang, K.; Liang, S.; Tian, Q.; Qin, S.; Yu, J. Improving the antifouling property of polypropylene hollow fiber membranes by in situ ultrasonic wave-assisted polymerization of styrene and maleic anhydride. Polym. Eng. Sci. 2019. [Google Scholar] [CrossRef]
- Chauke, N.M.; Moutloali, R.M.; Ramontja, J. Development of ZSM-22/polyethersulfone membrane for effective salt rejection. Polymers 2020, 12, 1446. [Google Scholar] [CrossRef]
- Kong, F.; Chang, M.; Wang, Z. Analysis of thermal-mechanical properties of silicon dioxide/polyvinylidene fluoride reinforced non-woven fabric (polypropylene) composites. Polymers 2020, 12, 481. [Google Scholar] [CrossRef]
- Otvagina, K.V.; Mochalova, A.E.; Sazanova, T.S.; Petukhov, A.N.; Moskvichev, A.A.; Vorotyntsev, A.V.; Afonso, C.A.M.; Vorotyntsev, I.V. Preparation and characterization of facilitated transport membranes composed of chitosan-styrene and chitosan-acrylonitrile copolymers modified by methylimidazolium based ionic liquids for CO2 separation from CH4 and N2. Membranes 2016, 6, 31. [Google Scholar] [CrossRef]
- Mohamed, N.K.; Kochkodan, V.; Zekri, A.; Ahzi, S. Polysulfone membranes embedded with halloysites nanotubes: Preparation and properties. Membranes 2020, 10, 2. [Google Scholar] [CrossRef]
- Hoy, R.S. Why is understanding glassy polymer mechanics so difficult? J. Polym. Sci. Part B Polym. Phys. 2011, 49, 979–984. [Google Scholar] [CrossRef]
- Silva, M.J.; Hayes, W.C.; Gibson, L.J. The Effects of Non-Periodic Microstructure on the Elastic Properties of Two-Dimensional Cellular Solids. J. Mech. Sci. 1995, 37, 1161–1177. [Google Scholar] [CrossRef]
- Mansourpanah, Y.; Gheshlaghi, A. Effects of adding different ethanol amines during membrane preparation on the performance and morphology of nanoporous PES membranes. J. Polym. Res. 2012, 19. [Google Scholar] [CrossRef][Green Version]
- Hussain, F.; Hojjati, M.; Okamoto, M.; Gorga, R.E. Review article: Polymer-matrix nanocomposites, processing, manufacturing, and application: An overview. J. Compos. Mater. 2006, 40, 1511–1575. [Google Scholar] [CrossRef]
- Sogolova, T.I. Control of the mechanical properties of polymers by modification of their supramolecular structure. Polym. Mech. 1966, 2, 395–404. [Google Scholar] [CrossRef]
- Modi, R.; Mehta, R.; Brahmbhatt, H.; Bhattacharya, A. Tailor Made Thin Film Composite Membranes: Potentiality Towards Removal of Hydroquinone from Water. J. Polym. Environ. 2017, 25, 1140–1146. [Google Scholar] [CrossRef]
- Wu, M.S. Strategies and challenges for the mechanical modeling of biological and bio-inspired materials. Mater. Sci. Eng. C 2011, 31, 1209–1220. [Google Scholar] [CrossRef]
- Schapery, R.A.; Science, E.; Lafayette, W. On the characterization of nonlinear viscoelastic materials. Polym. Eng. Sci. 1969, 9, 295–310. [Google Scholar] [CrossRef]
- Schrodt, M.; Benderoth, G.; Kühhorn, A.; Silber, G. Hyperelastic Description of Polymer Soft Foams at Finite Deformations. Tech. Mech. 2005, 25, 162–173. [Google Scholar]
- Krone, R.; Havenstrite, K.; Shafi, B. Mechanical characterization of thin film, water-based polymer gels through simple tension testing of laminated bilayers. J. Mech. Behav. Biomed. Mater. 2013, 27, 1–9. [Google Scholar] [CrossRef]
- Ferry, J.D. Viscoelastic properties of polymer solutions. J. Res. Natl. Bur. Stand. (1934) 1948, 41, 53–62. [Google Scholar] [CrossRef]
- Carreau, P.; MacDonald, I.; Bird, R.B. A nonlinear viscoelastic model for polymer solutions and melts—II. Chem. Eng. Sci. 1968, 23, 427–434. [Google Scholar] [CrossRef]
- Efimov, A.B. Some Quasi-static Problems for a Viscoelastic Half-space. Mekhanika Polim. 1966, 2, 392–402. [Google Scholar] [CrossRef]
- Shepard, K.B.; Gevgilili, H.; Ocampo, M.; Li, J.; Fisher, F.T.; Kalyon, D.M. Viscoelastic behavior of poly(ether imide) incorporated with multiwalled carbon nanotubes. J. Polym. Sci. Part B Polym. Phys. 2012, 50, 1504–1514. [Google Scholar] [CrossRef]
- Biener, J.; Hodge, A.M.; Hayes, J.R.; Volkert, C.A.; Zepeda-Ruiz, L.A.; Hamza, A.V.; Abraham, F.F. Size effects on the mechanical behavior of nanoporous Au. Nano Lett. 2006, 6, 2379–2382. [Google Scholar] [CrossRef]
- Greer, J.R.; Oliver, W.C.; Nix, W.D. Size dependence of mechanical properties of gold at the micron scale in the absence of strain gradients. Acta Mater. 2005, 53, 1821–1830. [Google Scholar] [CrossRef]
- Huang, Y.; Paul, D.R. Effect of Molecular Weight and Temperature on Physical Aging of ThinGlassy Poly(2,6-dimethyl-1,4-phenylene oxide) Films. J. Polym. Sci. Part B Polym. Phys. 2007, 45, 1390–1398. [Google Scholar] [CrossRef]
- Fetters, L.J.; Lohse, D.J.; Richter, D.; Witten, T.A.; Zirkel, A. Connection between Polymer Molecular Weight, Density, Chain Dimensions, and Melt Viscoelastic Properties. Macromolecules 1994, 27, 4639–4647. [Google Scholar] [CrossRef]
- Graebling, D.; Muller, R.; Palierne, J.F. Linear Viscoelastic Behavior of Some Incompatible Polymer Blends in the Melt. Interpretation of Data with a Model of Emulsion of Viscoelastic Liquids. Macromolecules 1993, 26, 320–329. [Google Scholar] [CrossRef]
- Craig, I.R.; Manolopoulos, D.E. Quantum statistics and classical mechanics: Real time correlation functions from ring polymer molecular dynamics. J. Chem. Phys. 2004, 121, 3368–3373. [Google Scholar] [CrossRef]
- Gulmez, D.E.; Yildiz, Y.O.; Kirca, M. Nanoporous gold reinforced with carbon based nanomaterials: A molecular dynamics study. Compos. Part B Eng. 2018, 151, 62–70. [Google Scholar] [CrossRef]
- Redenbach, C. Microstructure models for cellular materials. Comput. Mater. Sci. 2009, 44, 1397–1407. [Google Scholar] [CrossRef]
- Youssef, S.; Maire, E.; Gaertner, R. Finite element modelling of the actual structure of cellular materials determined by X-ray tomography. Acta Mater. 2005, 53, 719–730. [Google Scholar] [CrossRef]
- Antunes, F.V.; Ferreira, J.A.M.; Capela, C. Numerical modelling of the Young’s modulus of syntactic foams. Finite Elem. Anal. Des. 2011, 47, 78–84. [Google Scholar] [CrossRef]
- Marur, P.R. Numerical estimation of effective elastic moduli of syntactic foams. Finite Elem. Anal. Des. 2010, 46, 1001–1007. [Google Scholar] [CrossRef]
- Schneider, K.; Klusemann, B.; Bargmann, S. Fully periodic RVEs for technological relevant composites: Not worth the effort! J. Mech. Mater. Struct. 2017, 12, 471–484. [Google Scholar] [CrossRef]
- Huang, Y.; Kinloch, A.J. Modelling of the toughening mechanisms in rubber-modified epoxy polymers—Part I Finite element analysis studies. J. Mater. Sci. 1992, 27, 2753–2762. [Google Scholar] [CrossRef]
- Huang, Y.; Kinloch, A.J. Modelling of the toughening mechanisms in rubber-modified epoxy polymers Part II—A quantitative description of the microstructure-fracture property relationships. J. Mater. Sci. 1992, 27, 2763–2769. [Google Scholar] [CrossRef]
- Gitman, I.M.; Askes, H.; Sluys, L.J. Representative volume: Existence and size determination. Eng. Fract. Mech. 2007, 74, 2518–2534. [Google Scholar] [CrossRef]
- Khisaeva, Z.F.; Ostoja-Starzewski, M. On the size of RVE in finite elasticity of random composites. J. Elast. 2006, 85, 153–173. [Google Scholar] [CrossRef]
- Qian, C.; Harper, L.T.; Turner, T.A.; Li, S.; Warrior, N.A. Establishing size effects in discontinuous fibre composites using 2D finite element analysis. Comput. Mater. Sci. 2012, 64, 106–111. [Google Scholar] [CrossRef]
- Gitman, I.M.; Gitman, M.B.; Askes, H. Quantification of stochastically stable representative volumes for random heterogeneous materials. Arch. Appl. Mech. 2006, 75, 79–92. [Google Scholar] [CrossRef]
- Demirkol, G.T.; Dizge, N.; Acar, T.O.; Salmanli, O.M.; Tufekci, N. Influence of nanoparticles on filterability of fruit-juice industry wastewater using submerged membrane bioreactor. Water Sci. Technol. 2017, 76, 705–711. [Google Scholar] [CrossRef]
- Hou, J.; Yun, J.; Byun, H. Fabrication and Characterization of Modified Graphene Oxide/PAN Hybrid Nanofiber Membrane. Membranes 2019, 9, 122. [Google Scholar] [CrossRef]
- Guillen, G.R.; Pan, Y.; Li, M.; Hoek, E.M.V. Preparation and characterization of membranes formed by nonsolvent induced phase separation: A review. Ind. Eng. Chem. Res. 2011, 50, 3798–3817. [Google Scholar] [CrossRef]
- Kupgan, G.; Liyana-Arachchi, T.P.; Colina, C.M. NLDFT Pore Size Distribution in Amorphous Microporous Materials. Langmuir 2017, 33, 11138–11145. [Google Scholar] [CrossRef]
- Cristofari, F.; Piotrowski, B.; Pesci, R. Mechanical properties of a nanoporous membrane used in implantable medical devices. Correlation between experimental characterization and 2D numerical simulation. J. Mech. Behav. Biomed. Mater. 2017, 74, 43–53. [Google Scholar] [CrossRef]
- Tufekci, M.; Gunes-Durak, S.; Ormanci-Acar, T.; Tufekci, N. Effects of geometry and PVP addition on mechanical behavior of PEI membranes for use in wastewater treatment. J. Appl. Polym. Sci. 2018, 47073, 47073. [Google Scholar] [CrossRef]
- Guochang, L.; Huifeng, T.; Xianghong, B. Mechanical Parameters Determination of a Polymeric Membrane by Digital Image Correlation. Polym. Polym. Compos. 2014, 22, 157–162. [Google Scholar] [CrossRef]
- Kerdid, N.; Le Dret, H.; Saïdi, A. Numerical approximation for a non-linear membrane problem. Int. J. Non. Linear. Mech. 2008, 43, 908–914. [Google Scholar] [CrossRef]
- Seide, P. Large deflections of rectangular membranes under uniform pressure. Int. J. Non. Linear. Mech. 1977, 12, 397–406. [Google Scholar] [CrossRef]
- ASTM Standard D638. Standard Test Method for Tensile Properties of Plastics; ASTM International: West Conshohocken, PA, USA, 2014. [Google Scholar]
- Persson, K.M.; Gekas, V.; Trägårdh, G. Study of membrane compaction and its influence on ultrafiltration water permeability. J. Memb. Sci. 1995, 100, 155–162. [Google Scholar] [CrossRef]
- An, A.K.; Guo, J.; Lee, E.J.; Jeong, S.; Zhao, Y.; Wang, Z.; Leiknes, T.O. PDMS/PVDF hybrid electrospun membrane with superhydrophobic property and drop impact dynamics for dyeing wastewater treatment using membrane distillation. J. Memb. Sci. 2017, 525, 57–67. [Google Scholar] [CrossRef]
- Kertész, S.; De Freitas, T.B.; Hodúr, C. Characterization of polymer membranes by contact angle goniometer. Analecta Tech. Szeged. 2014, 8, 18–22. [Google Scholar] [CrossRef]
- Basri, H.; Ismail, A.F.; Aziz, M. Polyethersulfone (PES)-silver composite UF membrane: Effect of silver loading and PVP molecular weight on membrane morphology and antibacterial activity. Desalination 2011, 273, 72–80. [Google Scholar] [CrossRef]
- Han, M.J.; Nam, S.T. Thermodynamic and rheological variation in polysulfone solution by PVP and its effect in the preparation of phase inversion membrane. J. Memb. Sci. 2002, 202, 55–61. [Google Scholar] [CrossRef]
- Liu, Q.; Xu, N.; Fan, L.; Ding, A.; Dong, Q. Polyacrylonitrile (PAN)/TiO2 mixed matrix membrane synthesis by thermally induced self-crosslinking for thermal and organic-solvent resistant filtration. Chem. Eng. Sci. 2020, 228, 115993. [Google Scholar] [CrossRef]
- Chokki, J.; Darracq, G.; Poelt, P.; Baron, J.; Gallard, H.; Joyeux, M.; Teychené, B. Investigation of Poly(ethersulfone)/Polyvinylpyrrolidone ultrafiltration membrane degradation by contact with sodium hypochlorite through FTIR mapping and two-dimensional correlation spectroscopy. Polym. Degrad. Stab. 2019, 161, 131–138. [Google Scholar] [CrossRef]
- Kudryashov, M.; Logunov, A.; Gogova, D.; Mashin, A.; De Filpo, G. Ag/PVP/PAN nanocomposites with triangular nanoprisms of silver synthesized by UV-induced polymerization: Morphology manipulation and optical properties tuning. Opt. Mater. 2020, 101, 109746. [Google Scholar] [CrossRef]
- Beygmohammdi, F.; Nourizadeh Kazerouni, H.; Jafarzadeh, Y.; Hazrati, H.; Yegani, R. Preparation and characterization of PVDF/PVP-GO membranes to be used in MBR system. Chem. Eng. Res. Des. 2020, 154, 232–240. [Google Scholar] [CrossRef]
- Huang, F.; Xu, Y.; Liao, S.; Yang, D.; Hsieh, Y.L.; Wei, Q. Preparation of amidoxime polyacrylonitrile chelating nanofibers and their application for adsorption of metal ions. Materials 2013, 6, 969–980. [Google Scholar] [CrossRef]



| Materials | Polymer Content % | PVP Content % | Solvent Content % |
|---|---|---|---|
| PAN 1 | 15 | - | 85 |
| PAN 2 | 10 | 8 | 82 |
| PAN 1 | PAN 2 | |
|---|---|---|
| Material model type | Elastoplastic Isotropic | Elastoplastic Isotropic |
| Default failure criterion | Max von Mises Stress | Max von Mises Stress |
| Yield strength (MPa) | 2.02 | 0.41 |
| Tensile strength (MPa) | 3.63 | 1.38 |
| Elastic modulus (MPa) | 66.31 | 21.89 |
| Poisson’s ratio | 0.42 | 0.42 |
| Shear modulus (MPa) | 23.35 | 7.77 |
| Porosity (%) | 23 | 51 |
| Water content (%) | 86 | 90 |
| Contact angle (°) | 49 ± 4.1 | 19 ± 4.3 |
| Thickness (mm) | 1.5 | 1.5 |
| Viscosity (Pa.s) | 1.9 | 2.8 |
| Roughness RMS (nm) | 15.4 ± 0.7 | 34.3 ± 2.5 |
| PWF (Lm−2 h−1) | 51.79 ± 2.59 | 91.48 ± 6.08 |
| von Mises Stress (N/m2) | Resultant Displacement (mm) | Equivalent Strain | Resultant Reaction Force (N) | Resultant Moment (N.m) | |
|---|---|---|---|---|---|
| Hexagon | 352,900 | 2.708 | 0.004799 | 0.237998 | 3.82465 × 10−6 |
| Circle | 362,400 | 2.771 | 0.00491 | 0.239043 | 5.59367 × 10−7 |
| Square | 329,300 | 2.551 | 0.004463 | 0.236367 | 7.15237 × 10−6 |
| Rectangle 2 | 228,600 | 1.726 | 0.003099 | 0.237506 | 6.56278 × 10−6 |
| Ellipse 1.25 | 349,700 | 2.68 | 0.004739 | 0.239148 | 2.99675 × 10−6 |
| Ellipse 1.5 | 324,400 | 2.491 | 0.004392 | 0.238939 | 2.04139 × 10−6 |
| Ellipse 1.75 | 296,300 | 2.279 | 0.004012 | 0.238725 | 6.54296 × 10−7 |
| Ellipse 2 | 269,500 | 2.075 | 0.003649 | 0.238571 | 1.16314 × 10−6 |
| Ellipse 2.25 | 244,500 | 1.887 | 0.003312 | 0.238234 | 7.11306 × 10−7 |
| Ellipse 2.5 | 221,800 | 1.719 | 0.003005 | 0.238038 | 2.07609 × 10−7 |
| Ellipse 2.75 | 201,000 | 1.57 | 0.002723 | 0.237827 | 3.75788 × 10−7 |
| Ellipse 3 | 182,000 | 1.436 | 0.002466 | 0.237576 | 7.83426 × 10−6 |
| Ellipse 3.25 | 164,500 | 1.317 | 0.00223 | 0.237327 | 2.73056 × 10−7 |
| Ellipse 3.5 | 148,700 | 1.209 | 0.002015 | 0.237137 | 7.21739 × 10−7 |
| Ellipse 3.75 | 134,300 | 1.113 | 0.00182 | 0.236869 | 5.21311 × 10−7 |
| Ellipse 4 | 121,200 | 1.025 | 0.001642 | 0.236661 | 1.43855 × 10−7 |
| von Mises Stress (N/m2) | Resultant Displacement (mm) | Equivalent Stain | Resultant Reaction Force (N) | Resultant Moment (N.m) | |
|---|---|---|---|---|---|
| Hexagon | 265,100 | 4.572 | 0.05629 | 0.237998 | 3.79577 × 10−6 |
| Circle | 269,400 | 4.673 | 0.05715 | 0.239043 | 5.65258 × 10−7 |
| Square | 254,700 | 4.394 | 0.06405 | 0.236367 | 7.14436 × 10−6 |
| Rectangular 2 | 227,900 | 3.355 | 0.06261 | 0.245 | 6.56139 × 10−6 |
| Ellipse 1.25 | 264,000 | 4.566 | 0.05507 | 0.239148 | 2.97877 × 10−6 |
| Ellipse 1.5 | 251,500 | 4.335 | 0.06064 | 0.238939 | 2.03055 × 10−6 |
| Ellipse 1.75 | 244,500 | 4.07 | 0.06237 | 0.238726 | 6.52721 × 10−7 |
| Ellipse 2 | 238,300 | 3.806 | 0.06048 | 0.238572 | 1.16243 × 10−6 |
| Ellipse 2.25 | 228,300 | 3.557 | 0.05719 | 0.238235 | 7.09091 × 10−7 |
| Ellipse 2.5 | 217,500 | 3.329 | 0.05444 | 0.238039 | 2.05879 × 10−7 |
| Ellipse 2.75 | 206,800 | 3.122 | 0.05114 | 0.237828 | 3.75808 × 10−7 |
| Ellipse 3 | 196,600 | 2.93 | 0.04748 | 0.237576 | 7.83368 × 10−7 |
| Ellipse 3.25 | 186,800 | 2.757 | 0.04373 | 0.237326 | 2.7316 × 10−7 |
| Ellipse 3.5 | 177,400 | 2.596 | 0.03991 | 0.237136 | 7.21877 × 10−7 |
| Ellipse 3.75 | 168,400 | 2.445 | 0.0361 | 0.236868 | 5.20782 × 10−7 |
| Ellipse 4 | 159,800 | 2.307 | 0.03345 | 0.23661 | 1.43446 × 10−7 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tüfekci, M.; Durak, S.G.; Pir, İ.; Acar, T.O.; Demirkol, G.T.; Tüfekci, N. Manufacturing, Characterisation and Mechanical Analysis of Polyacrylonitrile Membranes. Polymers 2020, 12, 2378. https://doi.org/10.3390/polym12102378
Tüfekci M, Durak SG, Pir İ, Acar TO, Demirkol GT, Tüfekci N. Manufacturing, Characterisation and Mechanical Analysis of Polyacrylonitrile Membranes. Polymers. 2020; 12(10):2378. https://doi.org/10.3390/polym12102378
Chicago/Turabian StyleTüfekci, Mertol, Sevgi Güneş Durak, İnci Pir, Türkan Ormancı Acar, Güler Türkoğlu Demirkol, and Neşe Tüfekci. 2020. "Manufacturing, Characterisation and Mechanical Analysis of Polyacrylonitrile Membranes" Polymers 12, no. 10: 2378. https://doi.org/10.3390/polym12102378
APA StyleTüfekci, M., Durak, S. G., Pir, İ., Acar, T. O., Demirkol, G. T., & Tüfekci, N. (2020). Manufacturing, Characterisation and Mechanical Analysis of Polyacrylonitrile Membranes. Polymers, 12(10), 2378. https://doi.org/10.3390/polym12102378








