Elastic Properties of Polychloroprene Rubbers in Tension and Compression during Ageing
Abstract
:1. Introduction
2. Materials and Methods
2.1. DSC/OIT
2.2. Tensile Tests
2.3. Hardness Tests (Shore A)
2.4. Oedometric Compression Test
3. Results
3.1. Chemical Stability: OIT Changes
3.2. Tensile Tests
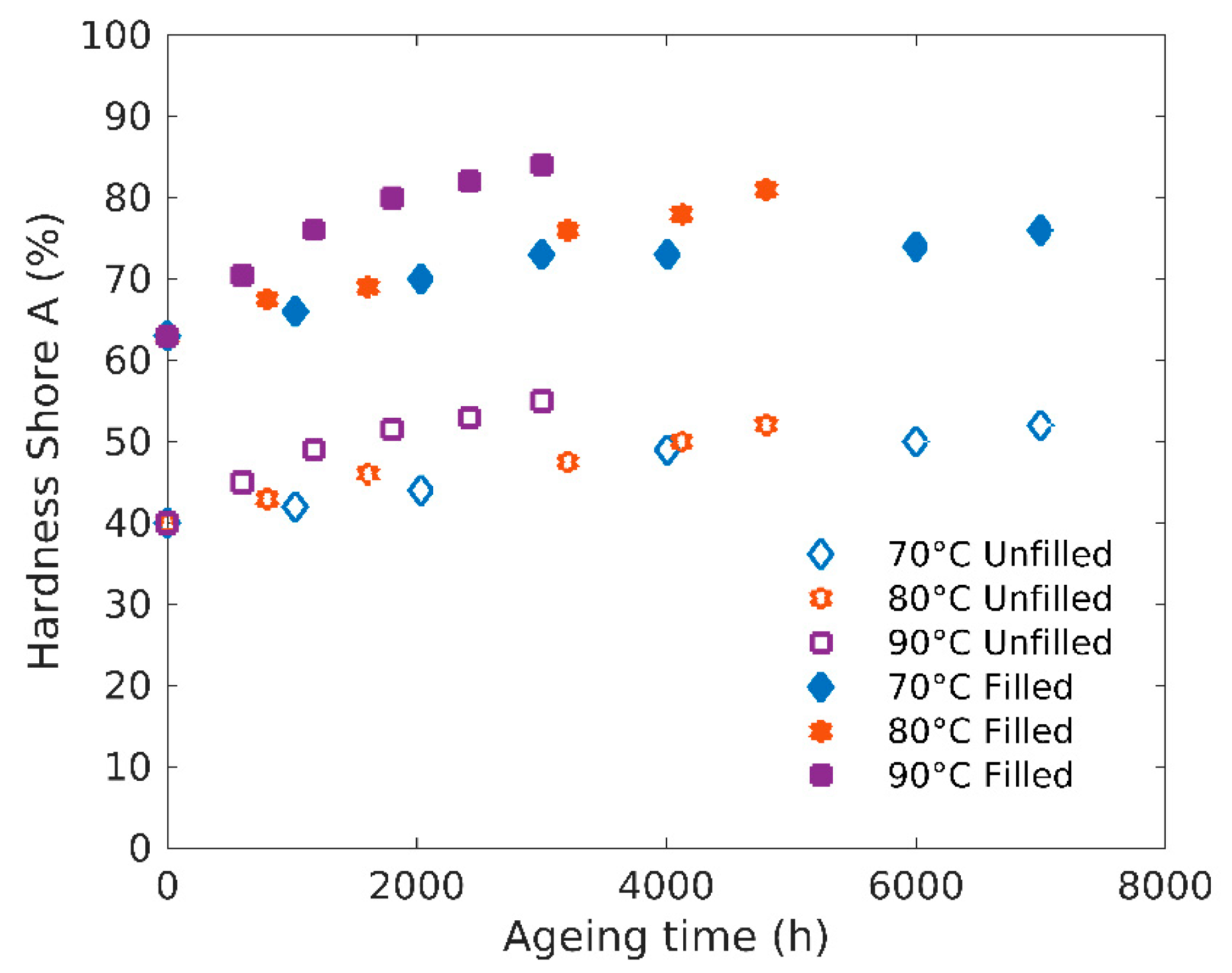
3.3. Hardness Tests (Shore A)
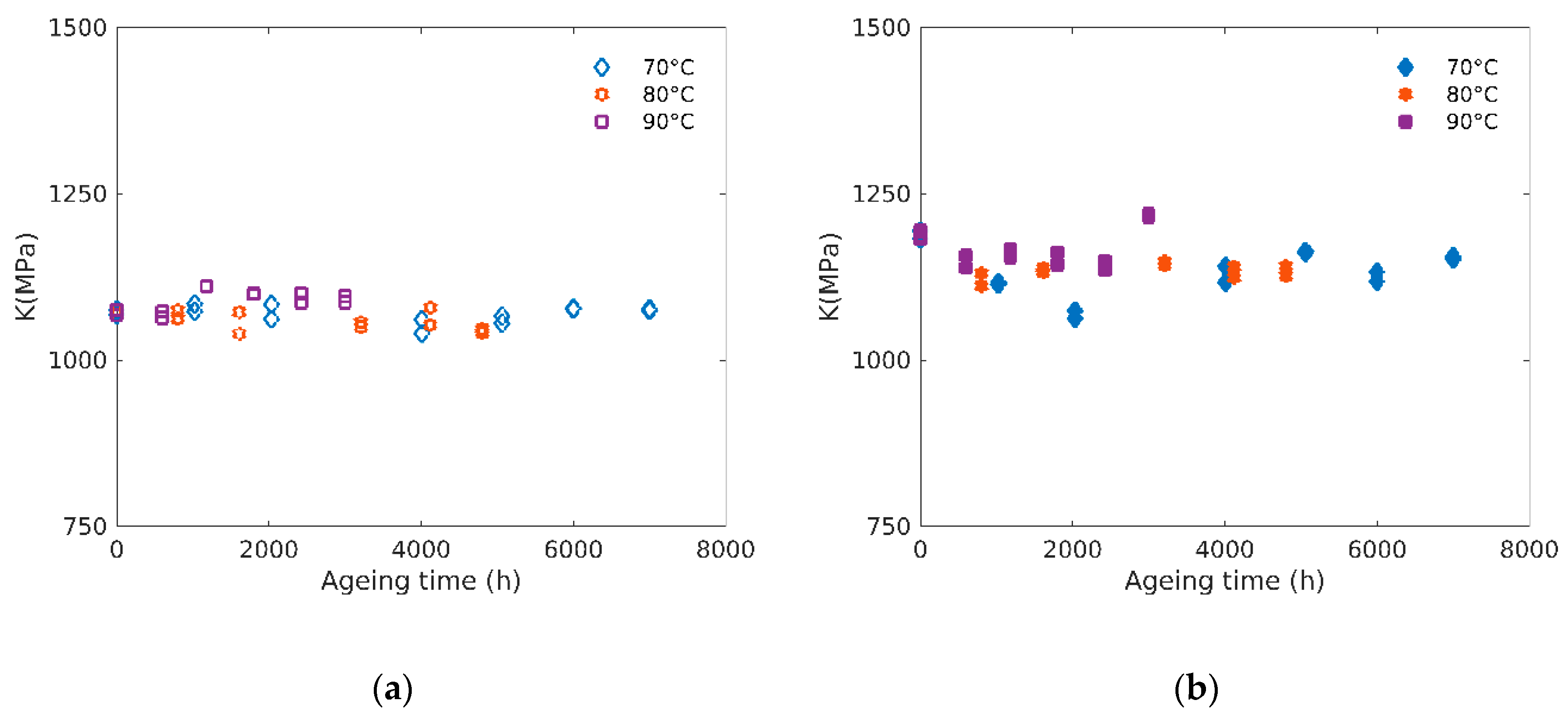
3.4. Bulk Modulus
4. Discussion
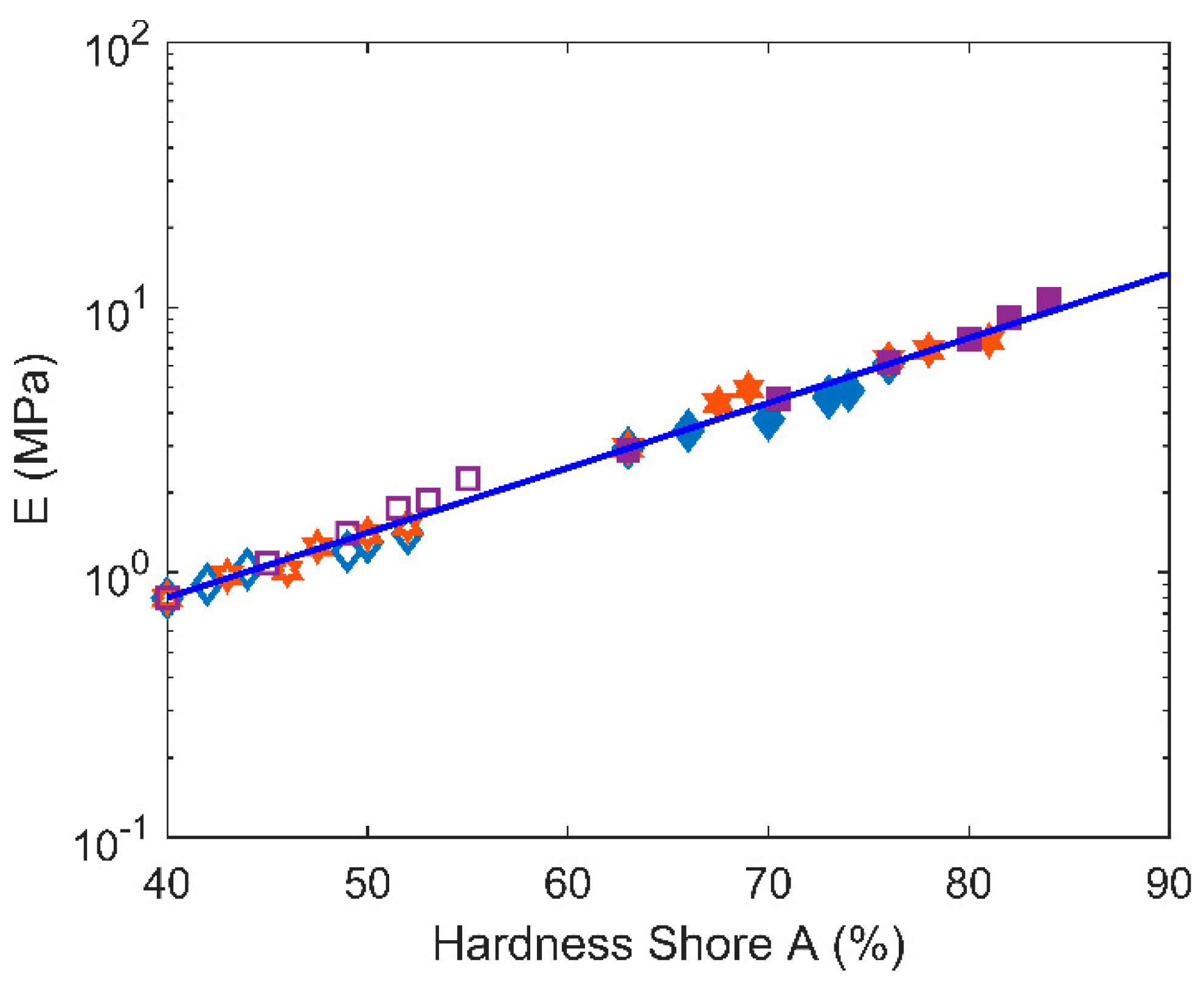
4.1. Structure-Properties Relationships
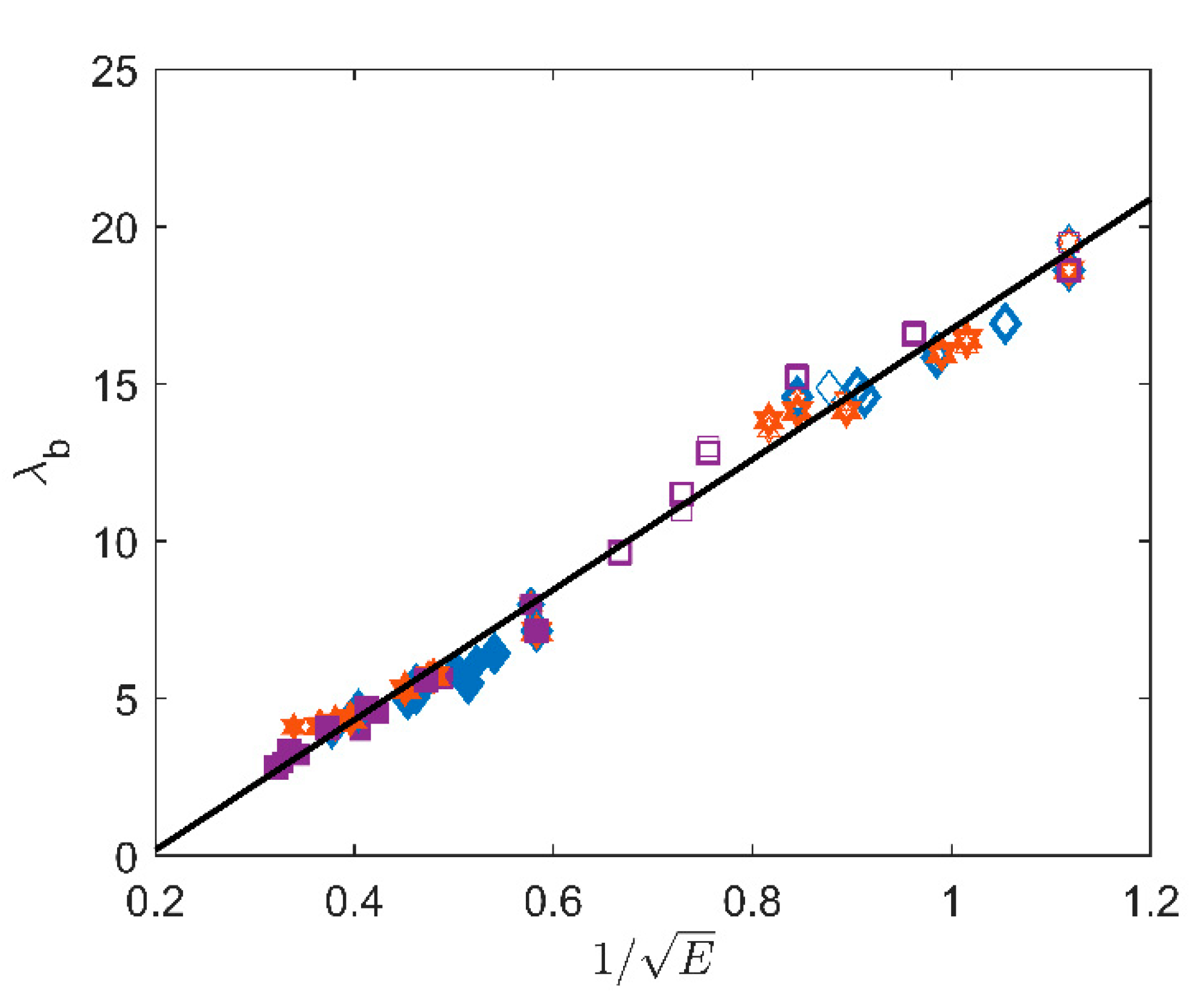
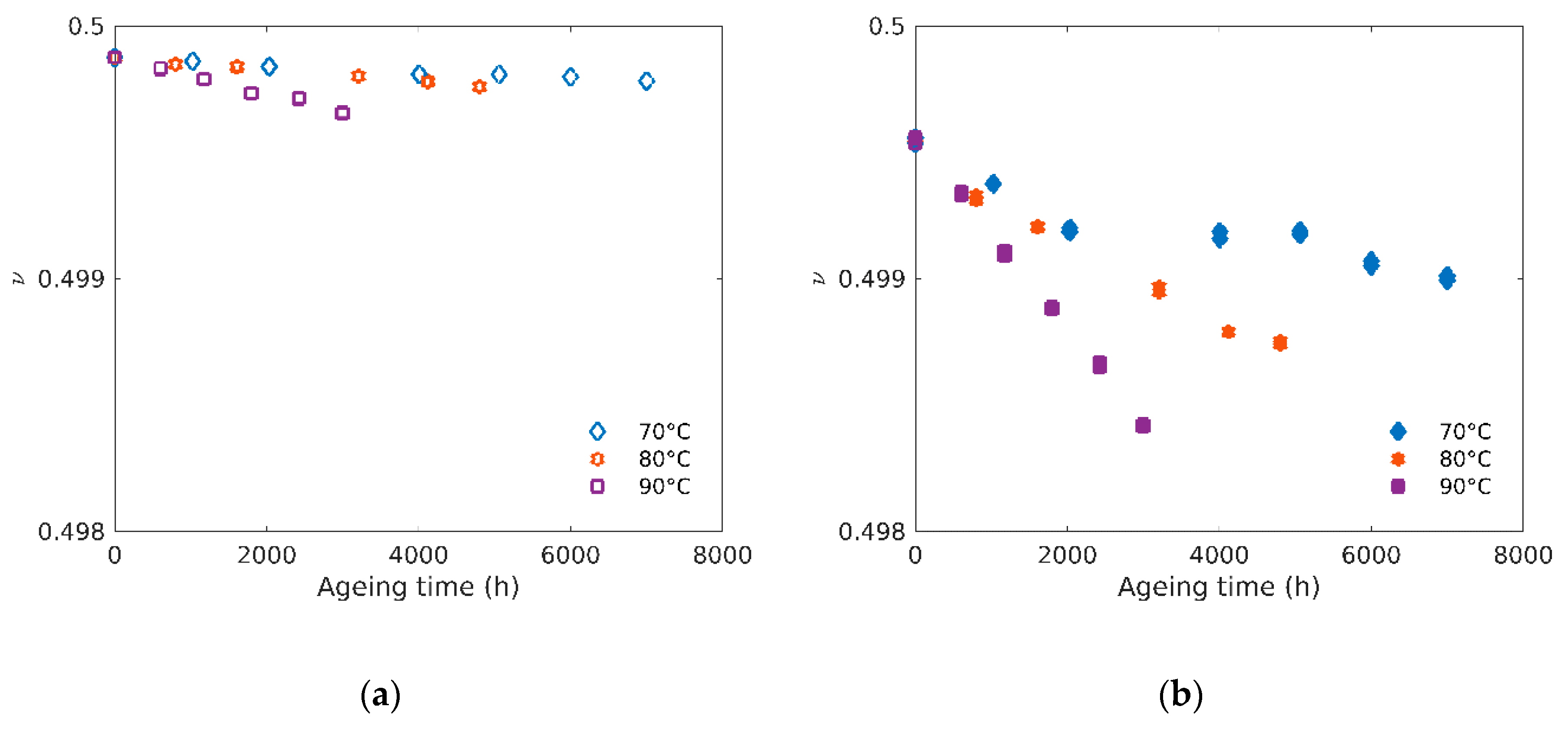
4.2. Poisson’s Ratio
5. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Appendix A
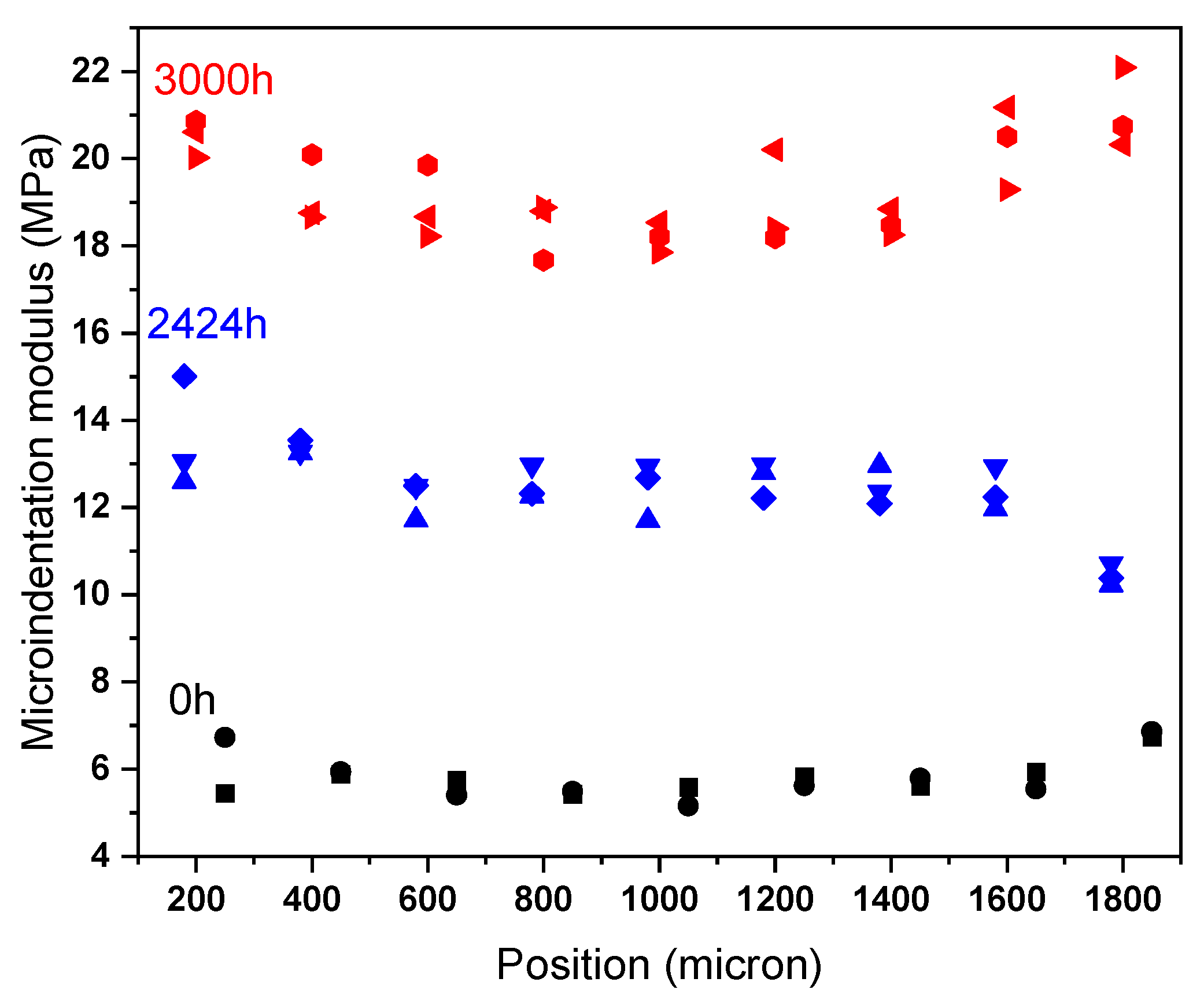
Appendix B
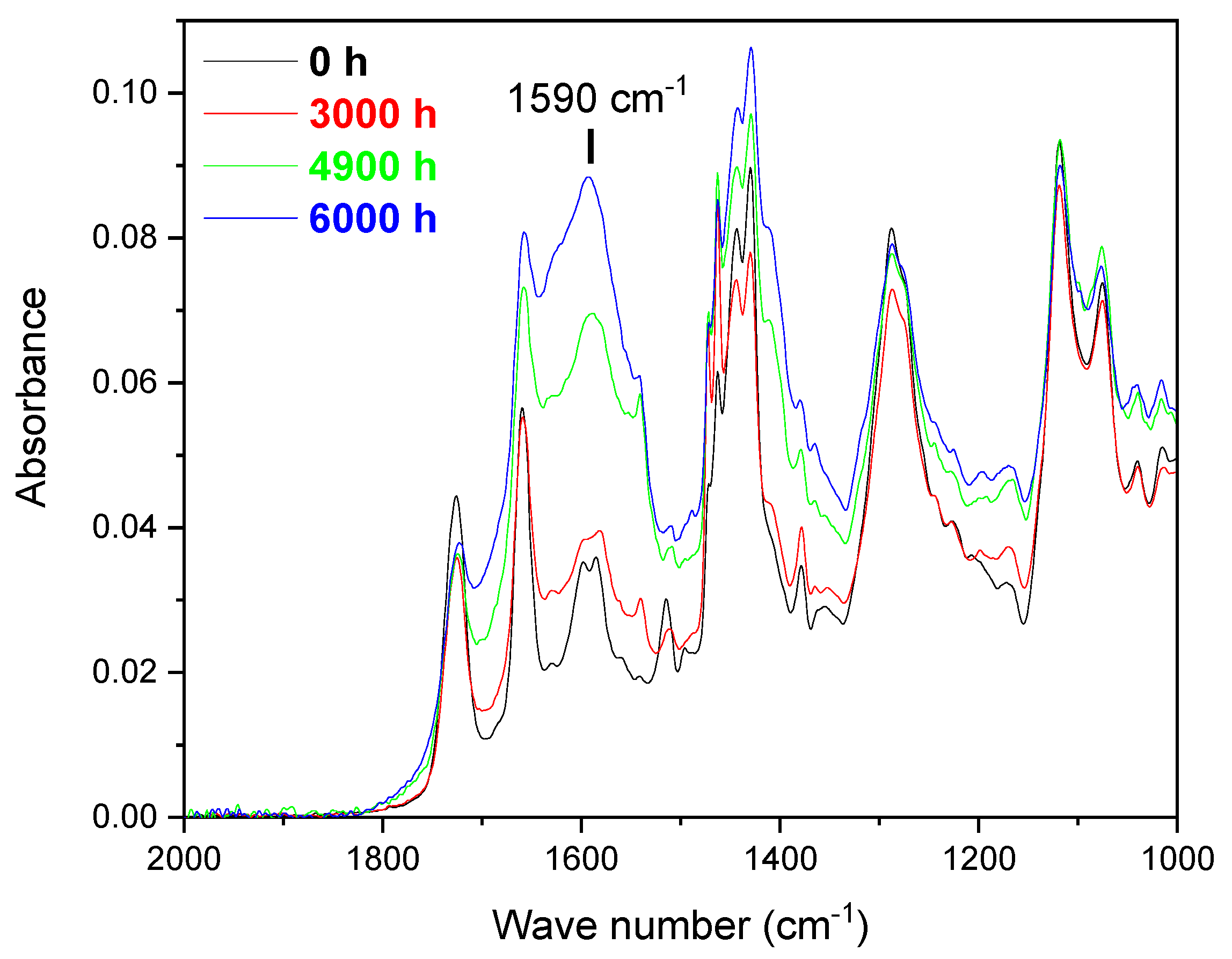
References
- Rubinstein, M.; Colby, R. Polymer Physics; Oxford University Press: Oxford, UK, 2003. [Google Scholar]
- Mark, J. Rubber Elasticity. J. Chem. Educ. 1981, 58, 98. [Google Scholar] [CrossRef] [Green Version]
- Nagdi, K. Rubber as an Engineering Material: Guideline for User; Hanser: Munich, Germany, 1993. [Google Scholar]
- Celina, M.C.; Wise, J.; Ottesen, D.; Gillen, K.; Clough, R. Correlation of chemical and mechanical property changes during oxidative degradation of neoprene. Polym. Degrad. Stabil. 2000, 68, 171–184. [Google Scholar] [CrossRef] [Green Version]
- Shelton, R. Aging and oxidation of elastomers. Rubber Chem. Technol. 1957, 30, 1251–1290. [Google Scholar] [CrossRef]
- Delor, F.; Lacoste, J.; Lemaire, J.; Barrois-Oudin, N.; Cardinet, C. Photo-and thermal ageing of polychloroprene: Effect of carbon black and crosslinking. Polym. Degrad. Stabil. 1996, 53, 361–369. [Google Scholar] [CrossRef]
- Celina, M.C. Review of polymer oxidation and its relationship with materials performance and lifetime prediction. Polym. Degrad. Stabil. 2013, 98, 2419–2429. [Google Scholar] [CrossRef]
- Le Gac, P.-Y.; Roux, G.; Verdu, J.; Davies, P.; Fayolle, B. Oxidation of unvulcanized, unstabilized polychloroprene: A kinetic study. Polym. Degrad. Stabil. 2014, 109, 175–183. [Google Scholar] [CrossRef] [Green Version]
- Bergström, J. Mechanics of Solid Polymers; Elsevier: Amsterdam, The Netherlands, 2015. [Google Scholar]
- Peng, S.; Landel, R. Stored energy function and compressibility of compressible rubberlike materials under large strain. J. Appl. Phys. 1975, 46, 2599. [Google Scholar] [CrossRef]
- Laufer, Z.; Diamant, Y.; Gill, M.; Fortuna, G. A Simple Dilatometric Method for Determining Poisson’s Ratio of Nearly Incompressible Elastomers. Int. J. Polym. Mater. 1978, 6, 159–174. [Google Scholar] [CrossRef]
- Kugler, H.; Stacer, R.; Steimle, C. Direct Measurement of Poisson’s Ratio in Elastomers. Rubber Chem. Technol. 1990, 63, 473–487. [Google Scholar] [CrossRef]
- Elektrova, L.; Melent’ev, P.; Zelenev, Y. Influence of fillers on the poisson ratios of rubber-like polymers. Polym. Mech. 1973, 8, 308–309. [Google Scholar]
- Robertson, C.; Bogoslovov, R.; Roland, C. Effect of structural arrest on Poisson’s ratio in nanoreinforced elastomers. Phys. Rev. E 2007, 75, 051403. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Planes, E.; Chazeau, L.; Vigier, G.; Fournier, J. Evolution of EPDM networks aged by gamma-irradiation—Consequences on the mechanical properties. Polymer 2009, 50, 4028–4038. [Google Scholar] [CrossRef] [Green Version]
- Le Gac, P.-Y.; Broudin, M.; Roux, G.; Verdu, J.; Davies, P.; Fayolle, B. Role of strain induced crystallization and oxidative crosslinking in fracture properties of rubbers. Polymer 2014, 55, 2535–2542. [Google Scholar] [CrossRef] [Green Version]
- Le Gac, P.-Y.; Albouy, P.-A.; Petermann, D. Strain-induced crystallization in an unfilled polychloroprene rubber: Kinetics and mechanical cycling. Polymer 2018, 142, 209–217. [Google Scholar] [CrossRef] [Green Version]
- Le Gac, P.-Y.; Albouy, P.-A.; Sotta, P. Strain-induced crystallization in a carbon-black filled polychloroprene rubber: Kinetics and mechanical cycling. Polymer 2019, 173, 158–165. [Google Scholar] [CrossRef] [Green Version]
- Bouaziz, R.; Ahose, K.; Lejeunes, S.; Eyheramendy, D.; Sosson, F. Characterization and modeling of filled rubber submitted to thermal aging. Int. J. Solids Struct. 2019, 169, 122–140. [Google Scholar] [CrossRef]
- Guth, E. Theory of Filler Reinforcement. J. Appl. Phys. 1945, 16, 596–604. [Google Scholar] [CrossRef]
- Fukahori, Y.; Hon, A.A.; Jha, V.; Busfield, J.J.C. Modified GUTH–GOLD Equation for Carbon Black-Filled Rubbers. Rubber Chem. Technol. 2013, 86, 218–232. [Google Scholar] [CrossRef]
- Mark, J. Polymer Handbook; Oxford University Press: Oxford, UK, 1999. [Google Scholar]
- Mullins, L.; Tobin, N. Stress softening in rubber vulcanizates. Part I. Use of a strain amplification factor to describe the elastic behavior of filler-reinforced vulcanized rubber. J. Appl. Polym. Sci. 1965, 9, 2993–3009. [Google Scholar] [CrossRef]
- Anandakumaran, K.; Seidl, W.; Castaldo, P. Condition Assesment of Cable Insulation Systems in Operating Nuclear Power Plants. IEEE T. Dielect. El. In. 1999, 6, 376–384. [Google Scholar] [CrossRef]
- Burns, J.; Dubbelday, P.; Ting, R. Dynamic Bulk Modulus of Various Elastomers. J. Polym. Sci. B Polym. Phys. 1990, 28, 1187–1205. [Google Scholar] [CrossRef]
- Diani, J.; Fayolle, B.; Gilormini, G. Study on the temperature dependence of the bulk modulus of polyisoprene by molecular dynamics simulations. Mol. Simul. 2008, 34, 1143–1148. [Google Scholar] [CrossRef] [Green Version]
- Seitz, J. The estimation of mechanical properties of polymers from molecular structure. J. Appl. Phys. 1993, 49, 1331–1351. [Google Scholar] [CrossRef]
- Flory, P. Principles of Polymer Chemistry; Cornell University Press: Ithaca, NY, USA; London, UK, 1953. [Google Scholar]
- Gent, A.J. On the relation between indentation hardness and Young’s modulus. Rubber Chem. Technol. 1958, 31, 896–906. [Google Scholar] [CrossRef]
- Qi, H.J.; Joyce, K.; Boyce, M.C. Durometer hardness and the stress-strain behavior of elastomeric materials. Rubber Chem. Technol. 2003, 76, 419–435. [Google Scholar] [CrossRef]
- Wise, J.; Gillen, K.; Clough, R. Quantitative model for the time development of diffusion-limited oxidation profiles. Polymer 1997, 38, 1229–1244. [Google Scholar] [CrossRef]
- Le Gac, P.-Y.; Celina, M.; Roux, G.; Verdu, J.; Davies, P.; Fayolle, B. Predictive ageing of elastomers: Oxidation driven modulus changes for polychloroprene. Polym. Degrad. Stabil. 2016, 130, 348–355. [Google Scholar] [CrossRef] [Green Version]
- Heideman, G.; Datta, R.; Noordermeer, J.; van Baarle, B. Influence of zinc oxide during different stages of sulfur vulcanization. Elucidated by model compound studies. J. Appl. Polym. Sci. 2005, 96, 1388–1404. [Google Scholar] [CrossRef]


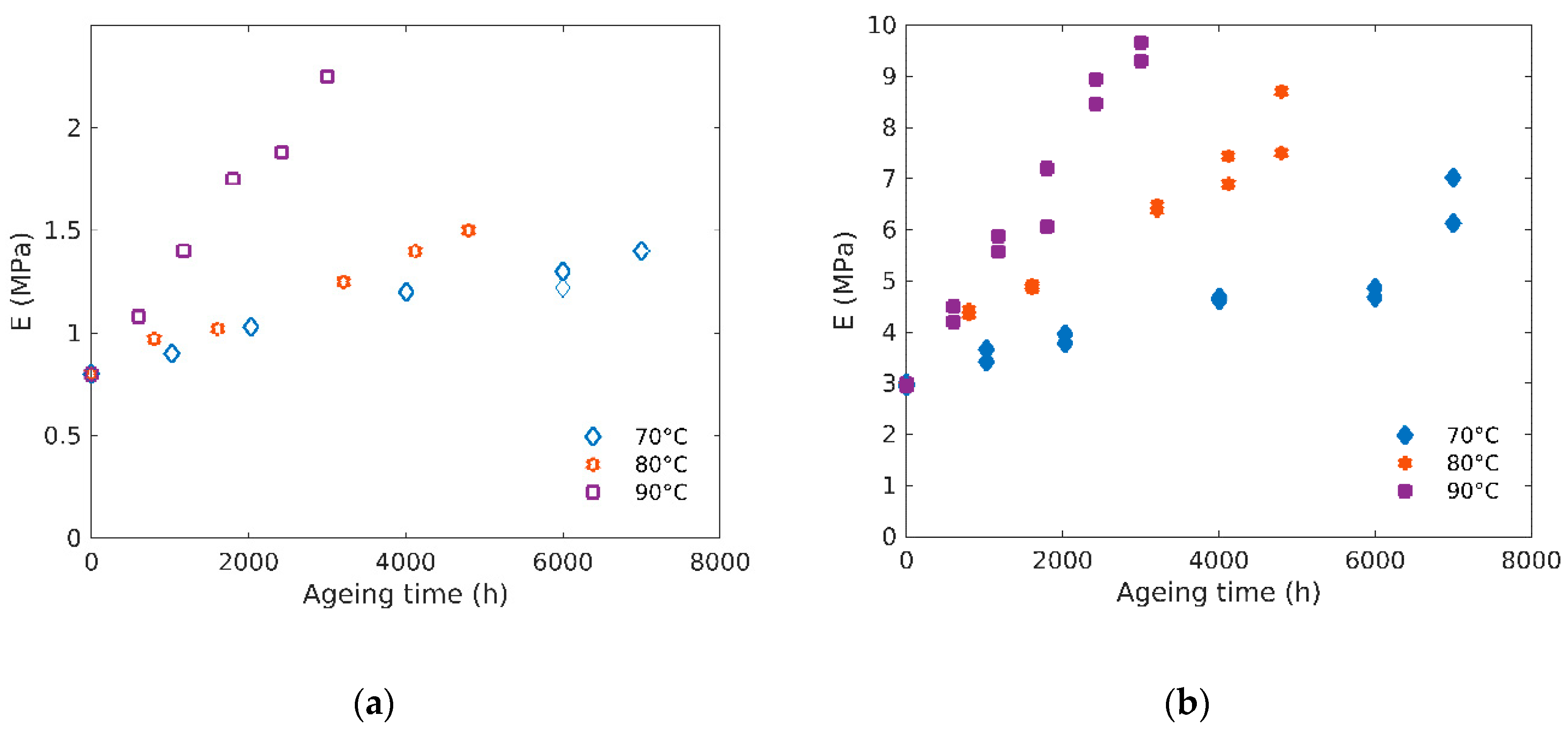
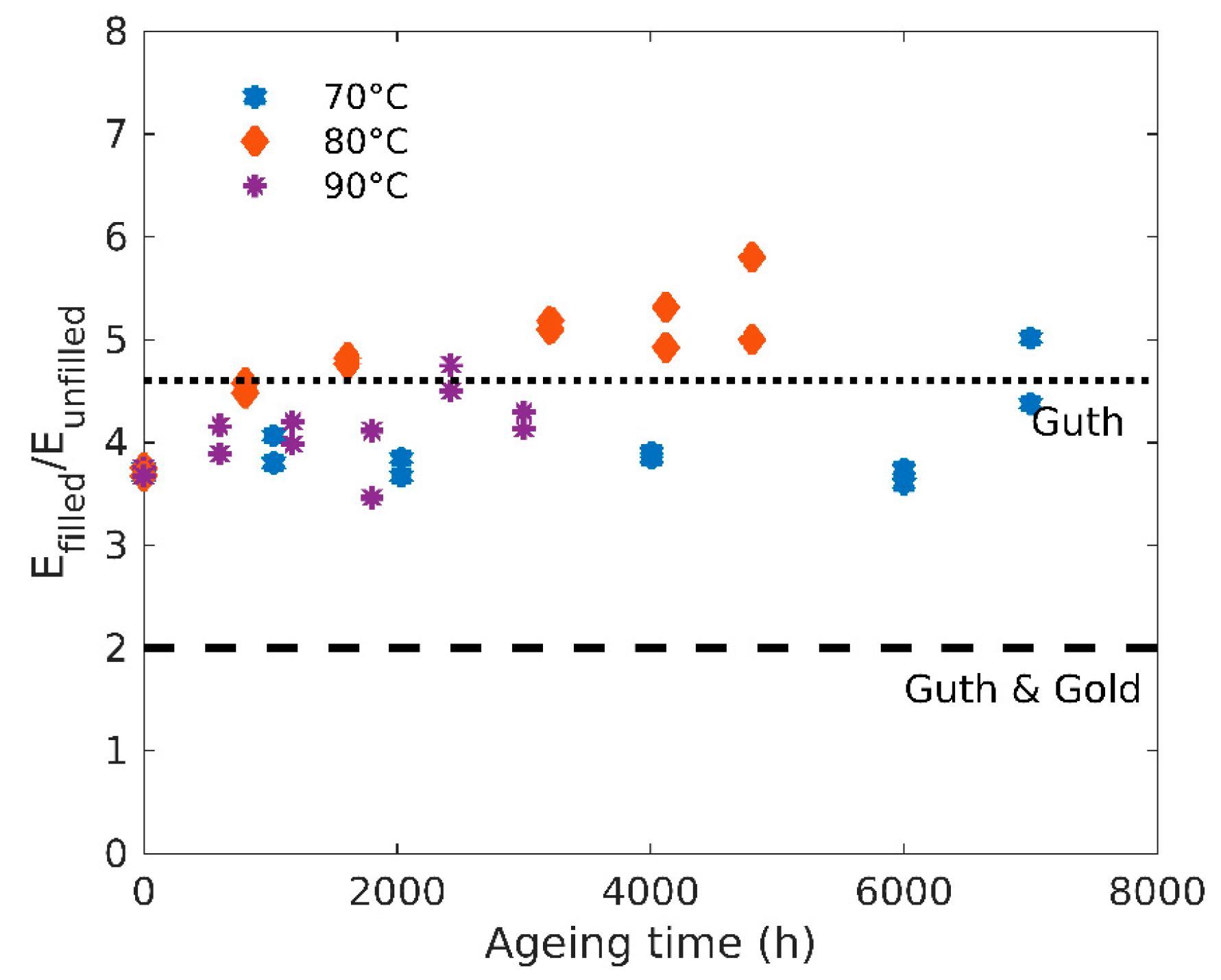


| Ea (kJ/mol) | E | OIT | λb | Hardness |
|---|---|---|---|---|
| Unfilled | 90 | 78 | 86 | 53 |
| Filled | 84 | 78 | 74 | 72 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bouaziz, R.; Truffault, L.; Borisov, R.; Ovalle, C.; Laiarinandrasana, L.; Miquelard-Garnier, G.; Fayolle, B. Elastic Properties of Polychloroprene Rubbers in Tension and Compression during Ageing. Polymers 2020, 12, 2354. https://doi.org/10.3390/polym12102354
Bouaziz R, Truffault L, Borisov R, Ovalle C, Laiarinandrasana L, Miquelard-Garnier G, Fayolle B. Elastic Properties of Polychloroprene Rubbers in Tension and Compression during Ageing. Polymers. 2020; 12(10):2354. https://doi.org/10.3390/polym12102354
Chicago/Turabian StyleBouaziz, Rami, Laurianne Truffault, Rouslan Borisov, Cristian Ovalle, Lucien Laiarinandrasana, Guillaume Miquelard-Garnier, and Bruno Fayolle. 2020. "Elastic Properties of Polychloroprene Rubbers in Tension and Compression during Ageing" Polymers 12, no. 10: 2354. https://doi.org/10.3390/polym12102354
APA StyleBouaziz, R., Truffault, L., Borisov, R., Ovalle, C., Laiarinandrasana, L., Miquelard-Garnier, G., & Fayolle, B. (2020). Elastic Properties of Polychloroprene Rubbers in Tension and Compression during Ageing. Polymers, 12(10), 2354. https://doi.org/10.3390/polym12102354





