Identification of Some New Triply Periodic Mesophases from Molten Block Copolymers
Abstract
1. Introduction
2. Theoretical Methods
3. Results and Discussion
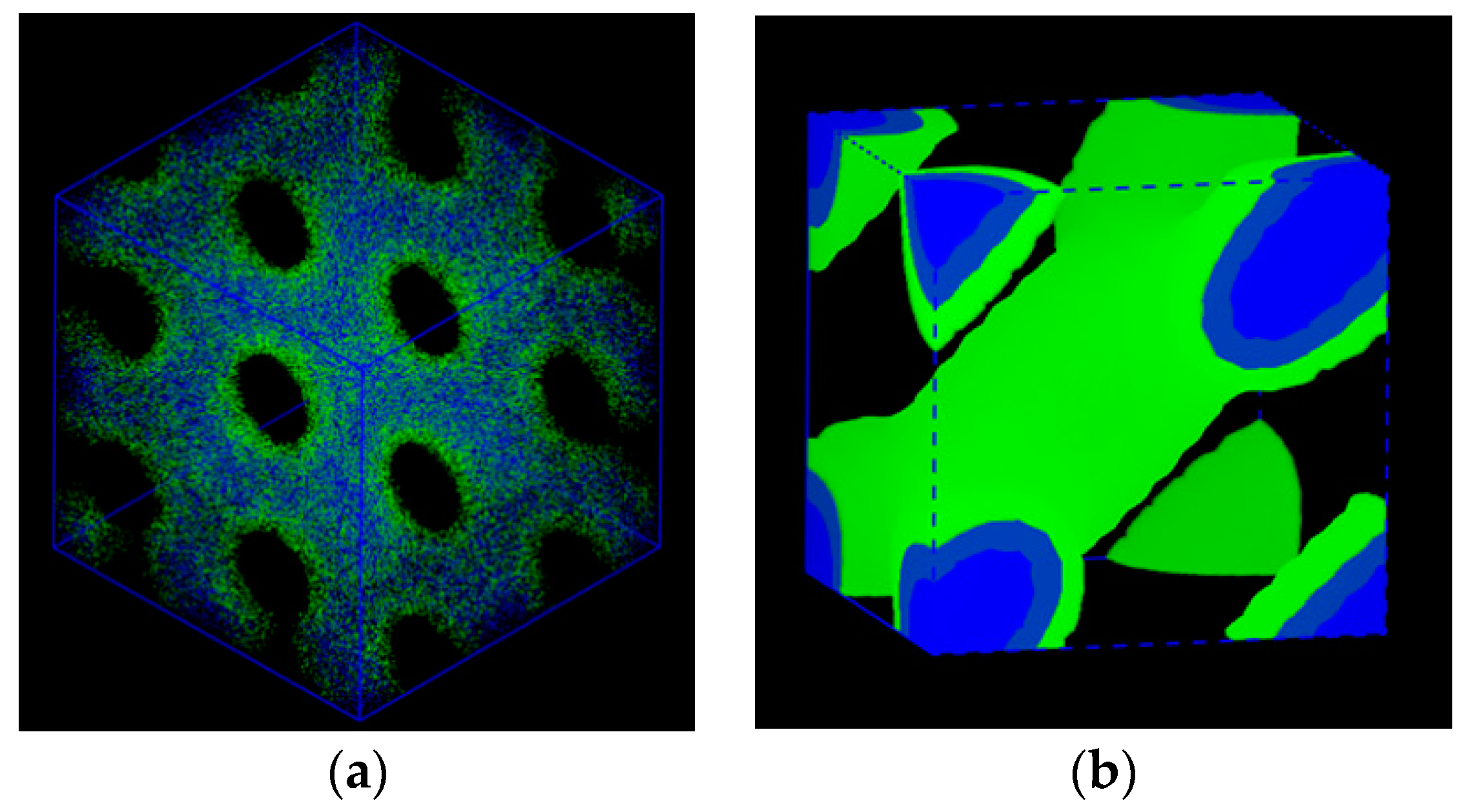
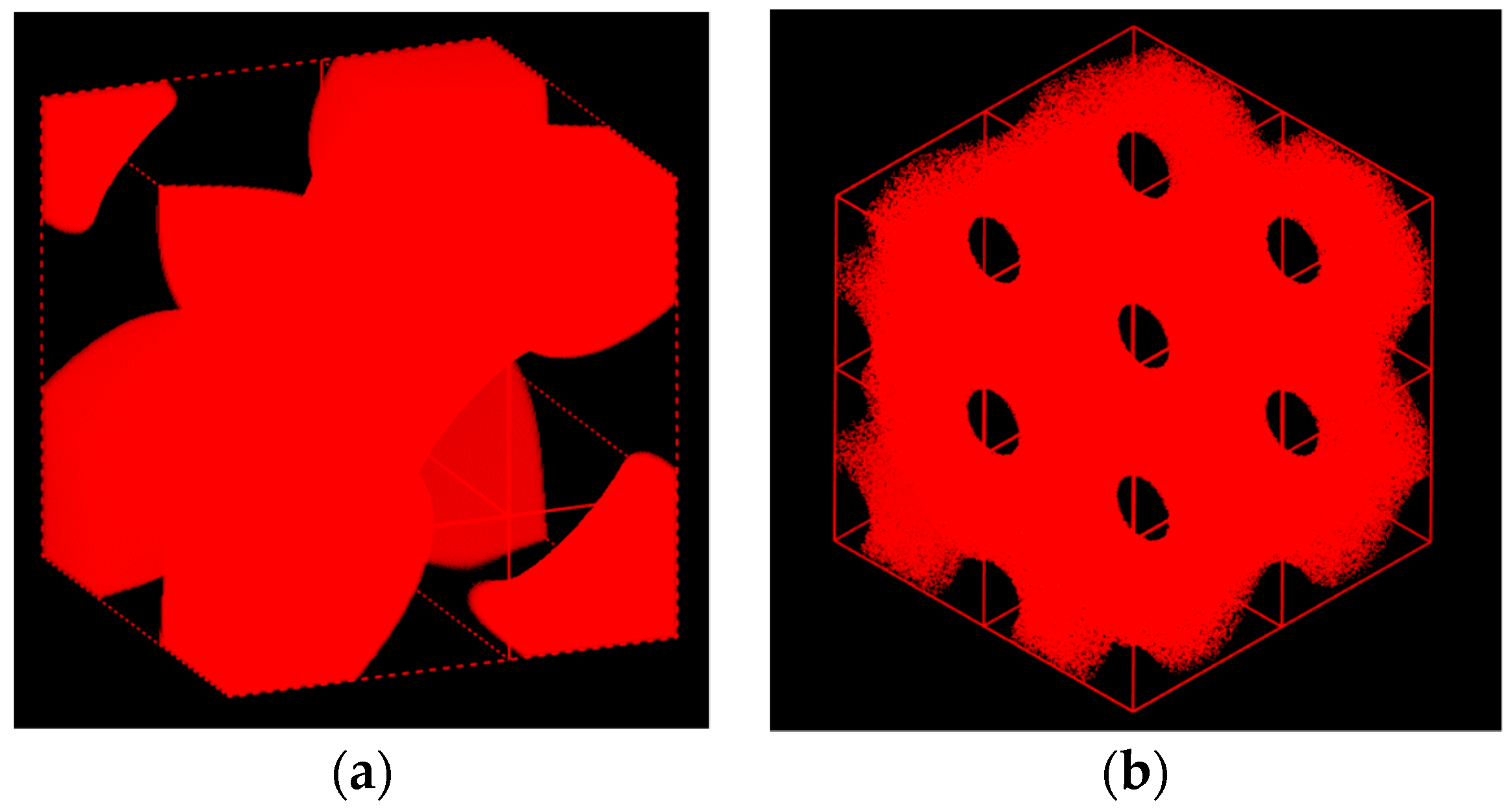
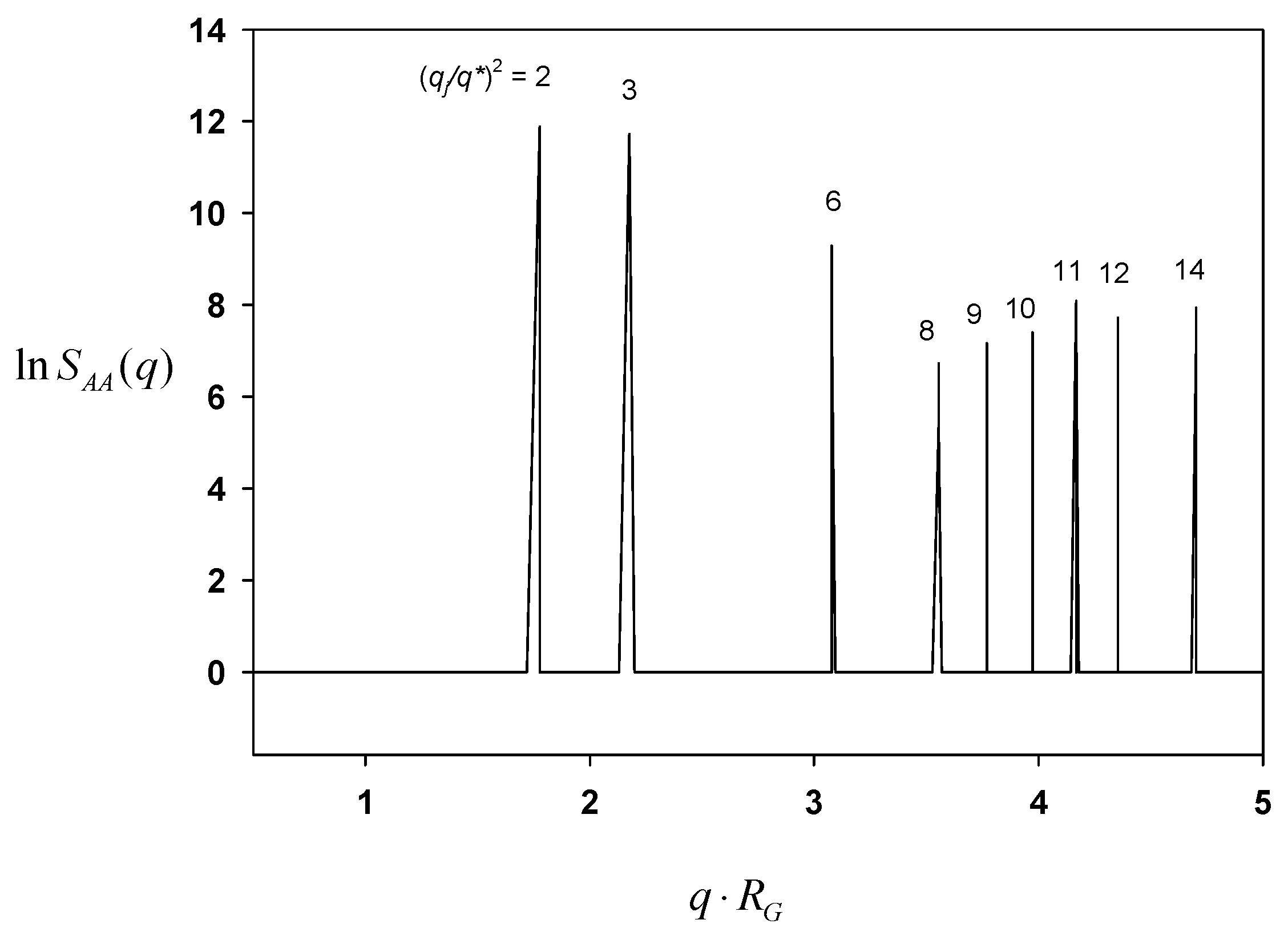
3.1. Im3
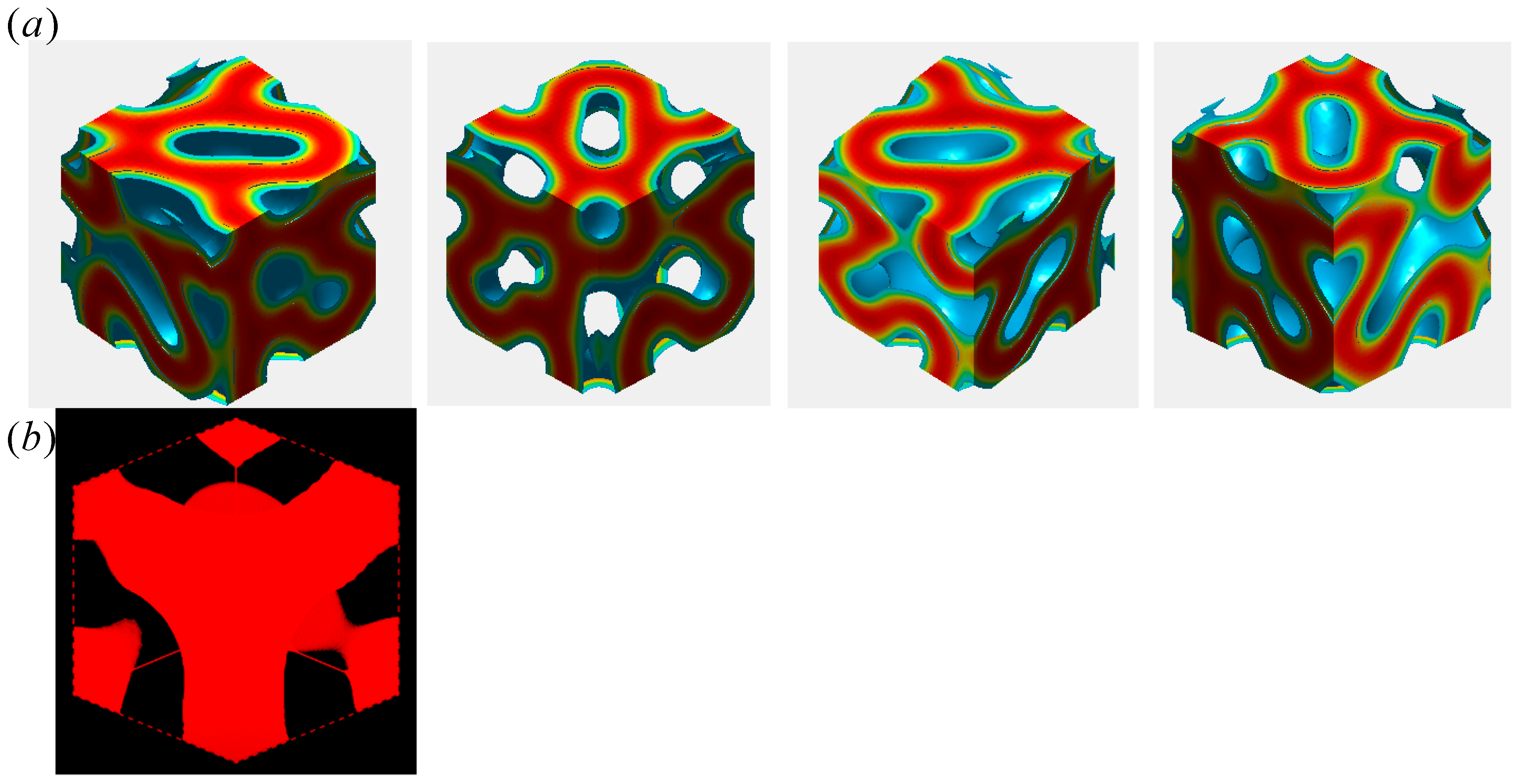
3.2. Metatron’s Cube with Pn3m Symmetry
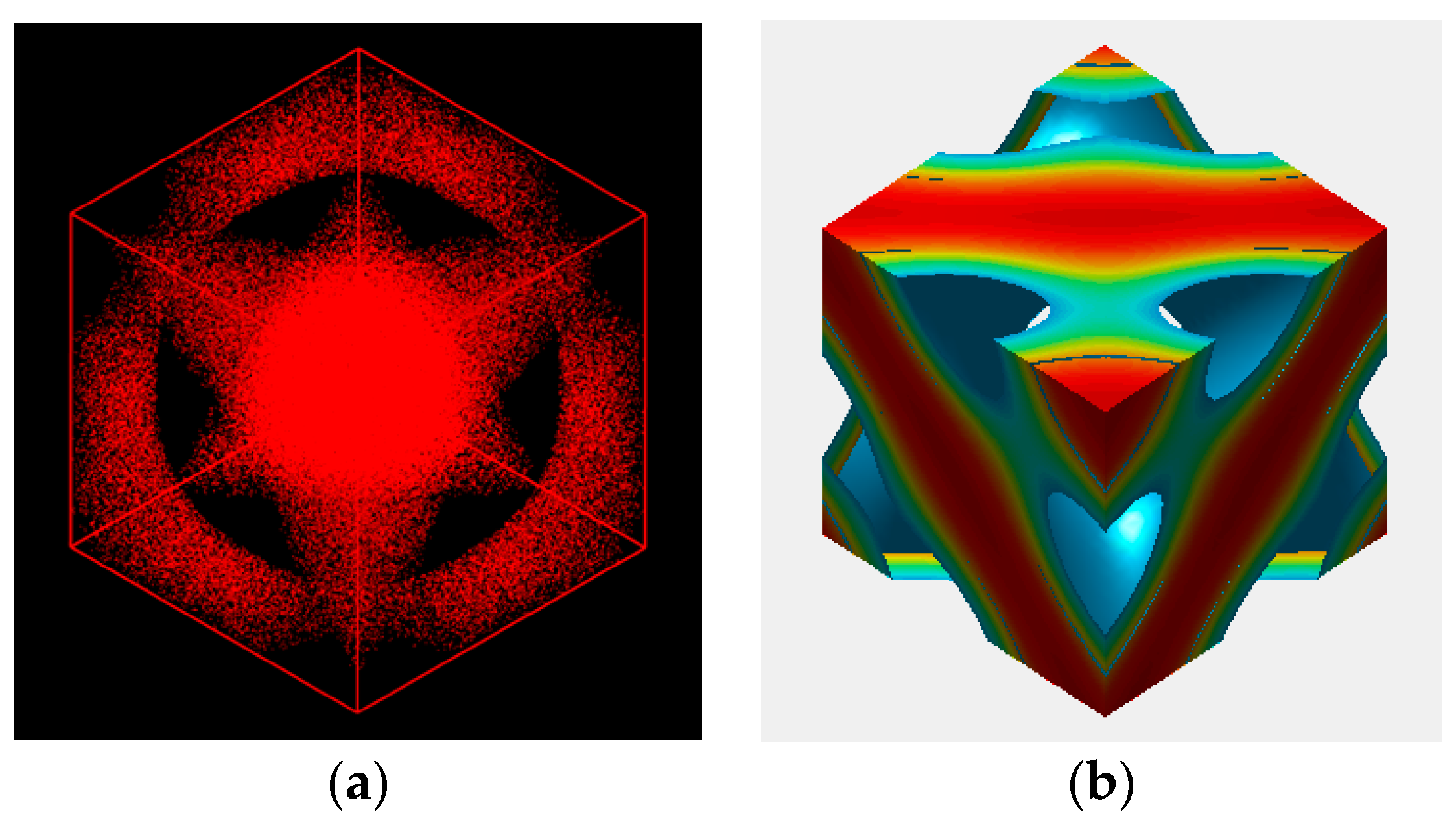
3.3. P432 Symmetry
3.4. Equilibrium Periodicity and Free Energies in the Incompressible Picture
4. Conclusions
Supplementary Materials
Funding
Acknowledgments
Conflicts of Interest
References
- de Gennes, P.-G. Soft matter. Nobel Lecture. College de France: Paris, France, 9 December 1991. [Google Scholar]
- Bates, F.S.; Fredrickson, G.H. Block copolymer thermodynamics: Theory and experiment. Annu. Rev. Phys. Chem. 1990, 41, 525–557. [Google Scholar] [CrossRef] [PubMed]
- Lodge, T.P. Block copolymers: Past successes and future challenges. Macromol. Chem. Phys. 2003, 204, 265–273. [Google Scholar] [CrossRef]
- Hamley, I.W. Developments in Block Copolymer Science and Technology; John Wiley & Sons Ltd.: Chichester, UK, 2004. [Google Scholar]
- Hamley, I.W. Ordering in thin films of block copolymers: Fundamentals to potential applications. Progr. Polym. Sci. 2009, 34, 1161–1210. [Google Scholar] [CrossRef]
- Hadjichristidis, N.; Pispas, S.; Floudas, G.A. Block copolymers: Synthetic Strategies, Physical Properties, and Applications; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2003. [Google Scholar]
- Hamley, I.W. The Physics of Block Copolymers; Oxford University Press, Inc.: New York, NY, USA, 1998. [Google Scholar]
- Leibler, L. Theory of microphase separation in block copolymers. Macromolecules 1980, 13, 1602–1617. [Google Scholar] [CrossRef]
- Epps, T.H.; Cochran, E.W.; Bailey, T.S.; Waletzko, R.S.; Hardy, C.M.; Bates, F.S. Ordered network phases in linear poly (isoprene-b-styrene-b-ethylene oxide) triblock copolymers. Macromolecules 2004, 37, 8325–8341. [Google Scholar] [CrossRef]
- Tyler, C.A.; Morse, D.C. The orthorhombic fddd network in triblock and diblock copolymer melts. Phys. Rev. Lett. 2005, 94, 208302. [Google Scholar] [CrossRef] [PubMed]
- Kim, M.I.; Wakada, T.; Akasaka, S.; Nishitsuji, S.; Saijo, K.; Hasegawa, H.; Ito, K.; Takenaka, M. Stability of the fddd phase in diblock copolymer melts. Macromolecules 2008, 41, 7667–7670. [Google Scholar] [CrossRef]
- Matsen, M.W.; Bates, F.S. Origins of complex self-assembly in block copolymers. Macromolecules 1996, 29, 7641–7644. [Google Scholar] [CrossRef]
- Zheng, W.; Wang, Z.-G. Morphology of abc triblock copolymers. Macromolecules 1995, 28, 7215–7223. [Google Scholar] [CrossRef]
- Bates, F.S.; Fredrickson, G.H. Block copolymers-designer soft materials. Phys. Today 1999, 52, 32–38. [Google Scholar] [CrossRef]
- Bates, F.S. Network phases in block copolymer melts. MRS Bull. 2005, 30, 532. [Google Scholar] [CrossRef]
- Cho, J. Pressure effects on nanostructure development of abc copolymers. Polymer 2016, 97, 589–597. [Google Scholar] [CrossRef]
- Stadler, R.; Auschra, C.; Beckmann, J.; Krappe, U.; Voigt-Martin, I.; Leibler, L. Morphology and thermodynamics of symmetric poly(a-block-b-block-c) triblock copolymers. Macromolecules 1995, 28, 3080–3097. [Google Scholar] [CrossRef]
- Erukhimovich, I.Y. Weak segregation theory and non-conventional morphologies in the ternary abc triblock copolymers. Eur. Phys. J. E 2005, 18, 383–406. [Google Scholar] [CrossRef] [PubMed]
- Lee, S.; Bluemle, M.J.; Bates, F.S. Discovery of a frank-kasper σ phase in sphere-forming block. Science 2010, 330, 349–353. [Google Scholar] [CrossRef] [PubMed]
- Matsen, M.W. Effect of architecture on the phase behavior of ab-type block copolymer melts. Macromolecules 2012, 45, 2161–2165. [Google Scholar] [CrossRef]
- Xie, N.; Li, W.; Qiu, F.; Shi, A.-C. Σ phase formed in conformationally asymmetric ab-type block copolymers. ACS Macro Lett. 2014, 3, 906–910. [Google Scholar] [CrossRef]
- Cho, J. Two new triply periodic bicontinuous network structures for molten block copolymers. Macromol. Res. 2018, 26, 380–387. [Google Scholar] [CrossRef]
- Hahn, T.; Shmueli, U.; Arthur, J.W. International Tables for Crystallography, 5th ed.; Springer: Dordrecht, The Netherlands, 2005; Volume A. [Google Scholar]
- Helfand, E. Theory of inhomogeneous polymers: Fundamentals of the gaussian random-walk model. J. Chem. Phys. 1975, 62, 999–1025. [Google Scholar] [CrossRef]
- Cho, J. Superposition in flory-huggins χ and interfacial tension for compressible polymer blends. ACS Macro Lett. 2013, 2, 544–549. [Google Scholar] [CrossRef]
- Matsen, M.W.; Schick, M. Stable and unstable phases of a diblock copolymer melt. Phys. Rev. Lett. 1994, 72, 2660–2663. [Google Scholar] [CrossRef] [PubMed]
- Drolet, F.; Fredrickson, G.H. Combinatorial screening of complex block copolymer assembly with self-consistent field theory. Phys. Rev. Lett. 1999, 83, 4317–4320. [Google Scholar] [CrossRef]
- Fredrickson, G.H.; Ganesan, V.; Drolet, F. Field-theoretic computer simulation methods for polymers and complex fluids. Macromolecules 2002, 35, 16. [Google Scholar] [CrossRef]
- Edwards, E. The statistical mechanics of polymers with excluded volume. Proc. Phys. Soc. (Lond.) 1965, 85, 613–624. [Google Scholar] [CrossRef]
- Goldenfeld, N. Lectures on Phase Transitions and the Renormalization Group; Addison-Wesley Publishing Company: Reading, UK, 1992. [Google Scholar]
- Cho, J. Analysis of phase separation in compressible polymer blends and block copolymers. Macromolecules 2000, 33, 2228–2241. [Google Scholar] [CrossRef]
- Chiew, Y.C. Percus-yevick integral-equation theory for athermal hard-sphere chains. Mol. Phys. 1990, 70, 129–143. [Google Scholar] [CrossRef]
- Huang, K. Statistical Mechanics, 2nd ed.; Wiley: New York, NY, USA, 1987. [Google Scholar]
- Cho, J. A landau free energy for diblock copolymers with compressibility difference between blocks. J. Chem. Phys. 2003, 119, 5711–5721. [Google Scholar] [CrossRef]
- Cho, J. Effective flory interaction parameter and disparity in equation-of-state properties for block copolymers. Polymer 2007, 48, 429–431. [Google Scholar] [CrossRef]
- Lacombe, R.H.; Sanchez, I.C. Statistical thermodynamics of fluid mixtures. J. Phys. Chem. 1976, 80, 2568–2580. [Google Scholar] [CrossRef]
- Sanchez, I.C.; Lacombe, R.H. Statistical thermodynamics of polymer solutions. Macromolecules 1978, 11, 1145–1156. [Google Scholar] [CrossRef]
- Sanchez, I.C.; Panayiotou, C.G. Equation of state thermodynamics of polymer solutions. In Thermodynamic Modeling; Sandler, S., Ed.; Marcel Dekker: New York, NY, USA, 1992. [Google Scholar]
- Rasmussen, K.; Kalosakas, G. Improved numerical algorithm for exploring block copolymer mesophases. J. Polym. Sci. B. 2002, 40, 1777–1783. [Google Scholar] [CrossRef]
- Rudolf, G. Periodic nodal surfaces and their application in structural chemistry. PhD Thesis, Stuttgart University, Stuttgart, Germany, 1993. [Google Scholar]
- Michielsen, K.; De Raedt, H.; De Hosson, J.T.M. Aspects of mathematical morphology. In Advances in Imaging and Electron Physics; Hawkes, P., Ed.; Elsevier: Amsterdam, The Netherlands, 2003; Volume 125, p. 76. [Google Scholar]
- Jelila. Metatron’s Cube: Remarkable Repository of Sacred Geometry. Kindle, 2014. Available online: https://www.amazon.com/Metatrons-Cube-Remarkable-Repository-Geometry-ebook/dp/B00P26S9G4 (accessed on 23 June 2019).
- Matsen, M.W. Stabilizing new morphologies by blending homopolymer with block copolymer. Phys. Rev. Lett. 1995, 74, 4225–4228. [Google Scholar] [CrossRef] [PubMed]
- Matsen, M.W. Phase behavior of block copolymer/homopolymer blends. Macromolecules 1995, 28, 5765–5773. [Google Scholar] [CrossRef]
- Martinez-Veracoechea, F.; Escobedo, F.A. Bicontinuous phases in diblock copolymer/homopolymer blends: Simulation and self-consistent field theory. Macromolecules 2009, 42, 1775–1784. [Google Scholar] [CrossRef]
- Martinez-Veracoechea, F.; Escobedo, F.A. Plumber’s nightmare in diblok copoly/homopolym blends. A self-consistent field theory study. Macromolecules 2009, 42, 9058–9062. [Google Scholar] [CrossRef]
- Padmanabhan, P.; Martinez-Veracoechea, F.; Escobedo, F.A. Computation of free energies of cubic bicontinuous phases for blends of diblock copolymer and selective homopolymer. Macromolecules 2016, 49, 5232–5243. [Google Scholar] [CrossRef]
- Liu, M.; Qiang, Y.; Li, W.; Qiu, F.; Shi, A.-C. Stabilizing the frank-kasper phases via binary blends of ab diblock copolymers. ACS Macro Lett. 2016, 5, 1167–1171. [Google Scholar] [CrossRef]
- Fredrickson, G.H.; Helfand, E. Fluctuation effects in the theory of microphase separation in block copolymers. J. Chem. Phys. 1987, 87, 697–705. [Google Scholar] [CrossRef]
- Shi, A.-C. Self-consistent field theory of block copolymers. In Developments in Block Copolymer Science and Technology; Hamley, I.W., Ed.; John Wiley & Sons, Ltd.: Chichester, UK, 2004; pp. 265–293. [Google Scholar]
- Grzywacz, P.; Qin, J.; Morse, D.C. Renormalization of the one-loop theory of fluctuations in polymer blends and diblock copolymer melts. Phys. Rev. E. 2007, 76, 061802. [Google Scholar] [CrossRef] [PubMed]



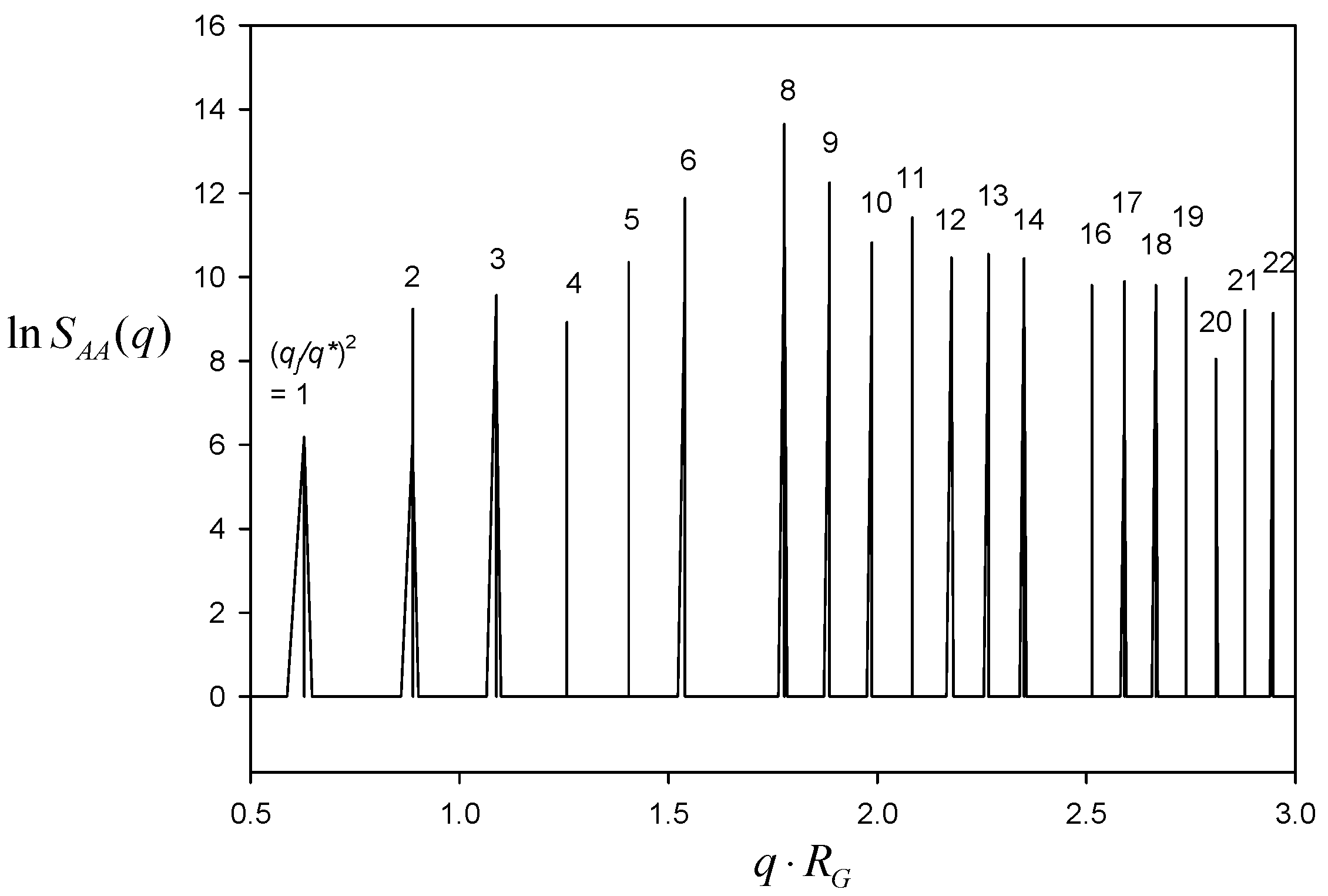
| Parameter | (K) | (Å) | (cm3/g) | |
|---|---|---|---|---|
| Polymer | ||||
| A | 4107 | 4.04 | 0.41857 | |
| B | 3000 | |||
| C | 3000 | |||
| Multiplicity | Position | Discretized Coordinates | |
|---|---|---|---|
| 2a | 0,0,0 | 1,1,1 | 0.012331 |
| 1/2,1/2,1/2 | 9,9,9 | 0.012329 | |
| 6b | 0,1/2,1/2 | 1,9,9 | 0.34353 |
| 1/2,0,1/2 | 9,1,9 | 0.343527 | |
| 1/2,1/2,0 | 9,9,1 | 0.329676 | |
| 1/2,0,0 | 9,1,1 | 0.343811 | |
| 0,1/2,0 | 1,91, | 0.343831 | |
| 0,0,1/2 | 1,1,9 | 0.329386 | |
| 8c | 1/4,1/4,1/4 | 5,5,5 | 0.029919 |
| 1/4,3/4,3/4 | 5,13,13 | 0.344344 | |
| 3/4,1/4,3/4 | 13,5,13 | 0.344256 | |
| 3/4,3/4,1/4 | 13,13,5 | 0.030894 | |
| 3/4,3/4,3/4 | 13,13,13 | 0.029839 | |
| 3/4,1/4,1/4 | 13,5,5 | 0.344491 | |
| 1/4,3/4,1/4 | 5,13,5 | 0.344419 | |
| 1/4,1/4,3/4 | 5,5,13 | 0.030805 |
| Multiplicity | Position | Discretized Coordinates | |
|---|---|---|---|
| 2a | 0,0,0 | 9,9,9 | 0.924754 |
| 1/2,1/2,1/2 | 25,25,25 | 0.912959 | |
| 4b | 1/4,1/4,1/4 | 1,1,1 | 0.184598 |
| 1/4,3/4,3/4 | 17,17,17 | 0.109947 | |
| 3/4,1/4,3/4 | 17,1,17 | 0.109947 | |
| 3/4,3/4,1/4 | 1,17,17 | 0.109947 | |
| 4c | 3/4,3/4,3/4 | 17,17,17 | 0.890423 |
| 3/4,1/4,1/4 | 1,1,17 | 0.819492 | |
| 1/4,3/4,1/4 | 1,17,1 | 0.819492 | |
| 1/4,1/4,3/4 | 17,1,1 | 0.819492 |
| Multiplicity | Position | Discretized Coordinates | |
|---|---|---|---|
| 1a | 0,0,0 | 1,1,1 | 0.905336 |
| 1b | 1/2,1/2,1/2 | 17,17,17 | 0.890529 |
| 3c | 0,1/2,1/2 | 1,17,17 | 0.921184 |
| 1/2,0,1/2 | 17,1,17 | 0.86979 | |
| 1/2,1/2,0 | 17,17,1 | 0.903127 | |
| 3d | 1/2,0,0 | 17,1,1 | 0.882721 |
| 0,1/2,0 | 1,17,1 | 0.911596 | |
| 0,0,1/2 | 1,1,17 | 0.91643 |
| Types of Copolymers | Morphology (Symmetry Group) | ||
|---|---|---|---|
| AB | Double gyroids (Ia3d) | 8.727 | 3.2334 |
| Fddd | 4.055 × 8.136 × 14.404 | 3.2358 | |
| Im3 | 5.007 | 3.2364 | |
| P6/mm (HEX) | 4.064 × 7.040 | 3.2370 | |
| LAM | 3.553 | 3.2377 | |
| P432 | 10.131 | 3.2408 | |
| Metatron’s cube (Pn3m) | 5.304 | 3.2442 | |
| BCC (Im3m) | 5.303 | 3.2442 | |
| Double diamonds (Pn3m) | 5.475 | 3.2451 | |
| Single gyroid (I4132) | 5.012 | 3.2461 | |
| I43d | 8.800 | 3.2470 | |
| Ia3d of g = 25 b | 8.910 | 3.2532 | |
| Disorder | - | 3.3600 |
© 2019 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Cho, J. Identification of Some New Triply Periodic Mesophases from Molten Block Copolymers. Polymers 2019, 11, 1081. https://doi.org/10.3390/polym11061081
Cho J. Identification of Some New Triply Periodic Mesophases from Molten Block Copolymers. Polymers. 2019; 11(6):1081. https://doi.org/10.3390/polym11061081
Chicago/Turabian StyleCho, Junhan. 2019. "Identification of Some New Triply Periodic Mesophases from Molten Block Copolymers" Polymers 11, no. 6: 1081. https://doi.org/10.3390/polym11061081
APA StyleCho, J. (2019). Identification of Some New Triply Periodic Mesophases from Molten Block Copolymers. Polymers, 11(6), 1081. https://doi.org/10.3390/polym11061081





