Theoretical Investigation of Chromium Separation from Chromates Through Photon–Phonon Resonant Absorption

Abstract

Chromium (Cr) is a vital metal utilized in materials physics, healthcare, and various other domains. In this study, we propose an eco-friendly method for separating Cr from potassium chromate (K₂CrO₄) based on photon–phonon resonance absorption theory. Using first-principles density functional theory calculations, we obtained the Raman and infrared spectra of K₂CrO₄ and assigned the vibrational modes to the peaks observed in the experimental spectra. We confirmed that the strongest infrared absorption peak corresponds to the Cr-O bond stretching vibration theoretically located at 931 cm⁻¹. We propose employing a high-power terahertz laser at this resonant frequency for photothermal energy transfer. This approach is expected to enhance the efficiency of separating Cr from K₂CrO₄. Experimental investigations are expected in the future.

1. Introduction

Chromium (Cr) is a hard, lustrous, and corrosion-resistant metal with a body-centered structure [1]. It is widely used in nanotechnology, biomedicine, and electronics [2,3,4,5]. In materials science, Cr can be used to produce luminescent and ferroelectric materials [6,7]. In chemistry, Cr forms the basis of various catalysts [8,9]. Moreover, Cr has industrial uses in lithography and chromized iron manufacturing [10,11]. In recent years, Cr has also been the focus of research on cutting-edge topics in condensed matter physics. In 2023, Chi et al. investigated the anomalous Hall effect in Cr₂Te₃ films [12]. In 2024, Liu et al. reported superconductivity in a Cr-based kagome metal [13].

Cr can be prepared by numerous methods, including electrolysis and hydrogen reduction [14,15,16,17]. In the traditional production process, the preparation of chromium involves various intermediate compounds, including chromates [18]. At present, the primary method of converting chromates to Cr is via reduction to chromium oxide [19,20], followed by further reduction to Cr [17]. The most commonly used reducing agents for the reduction of chromates include carbon, hydrogen, and carbon monoxide. This approach is energy-intensive, owing to the requisite high-temperature conditions. Furthermore, the process of reduction may produce greenhouse gases, which can have an impact on the environment. Alternatively, chromates can be electrolytically converted to chromium oxide, then reduced to Cr [17,21]. However, the electrolytic method for producing elemental Cr is also energy-consuming and not environmentally friendly. Therefore, there is an urgent need for a novel, environmentally friendly technology for the large-scale extraction of Cr from chromates with minimal pollution, high efficiency, and suitability for industrial production.

Potassium chromate (K₂CrO₄) is a familiar and widely studied chromate [22,23]. The Raman and infrared (IR) vibrational spectra of K₂CrO₄ have been extensively investigated in recent decades [24,25,26,27,28,29,30]. In 1992, Etxebarria et al. analyzed its lattice dynamics theoretically [23]. In 2020, Yuriy et al. calculated the interactions between the surfaces of K₂CrO₄ crystals and carbon nanotubes using density functional theory (DFT) [31].

Conventional applications using photothermal effects, such as IR therapy apparatus, emit thermal radiation with a frequency covering a large region of the IR spectrum [32]. In this work, we introduce a laser-targeted photothermal conversion method named photon–phonon resonant absorption (PPRA), which transfers energy from photons to phonons to promote bond breaking [33,34,35]. We simulated the IR and Raman spectra of K₂CrO₄ and compared them with the experimental data. Based on the IR-active peaks related to Cr-O bonds, we selected the ideal terahertz laser frequency for enhancing the separation of Cr from oxides.

2. Methods

A primitive cell of the K₂CrO₄ crystal contains 4 molecules [27]. Using the Cambridge Serial Total Energy Package (CASTEP 6.0), we performed geometric optimization and phonon calculations based on first-principles DFT simulations [36]. Tests of parameter settings showed that generalized gradient approximation (GGA) in the form of the Perdew–Burke–Ernzerhof (PBE, Material Studio 6.0) exchange–correlation functional was the best-matched functional for our purpose [37,38]. The convergence tolerances of both the self-consistent field and energy were set to 1 × 10⁻⁹ eV/atom. The k-point mesh was 2 × 2 × 1, and the energy cutoff was set to 830 eV. The linear response approach was used to calculate IR and Raman intensities using norm-conserving pseudopotentials [39,40].

Based on the IR calculations, we analyzed the dynamic processes of the vibrational modes at the gamma point. Through this analysis, the peaks in the simulated IR and Raman spectra were assigned to special vibrational modes. This enabled us to verify the reported experimental peaks and identify the effective Cr-related IR absorption peaks.

3. Results

The K₂CrO₄ we chose crystallizes in the Pnma space group with a spinel structure, which is same as the crystal structure tested in an experiment on anions on C_s sites [27]. The optimized lattice parameters of the primitive cell were a = 7.771 Å, b = 6.048 Å, c = 10.593 Å, and $\alpha =\beta =\gamma =90°$. Under harmonic approximation, the $4\times 7\times 3-3=81$ optical normal modes of K₂CrO₄ were calculated together with the IR and Raman spectra. As illustrated in Figure 1, the simulated IR and Raman spectra demonstrate the distribution and intensities of the IR and Raman active modes.

Based on the dynamic process of each vibrational mode, we assigned the IR-active and Raman-active modes as listed in

Table 1. Raman- and IR-active vibrational modes. The wavenumbers are displayed in the first column (unit cm⁻¹). The second column indicates whether the mode is Raman- or IR-active, followed by the experimental wavenumber data in the next two columns. The last column presents the assignments of the corresponding vibrational modes.

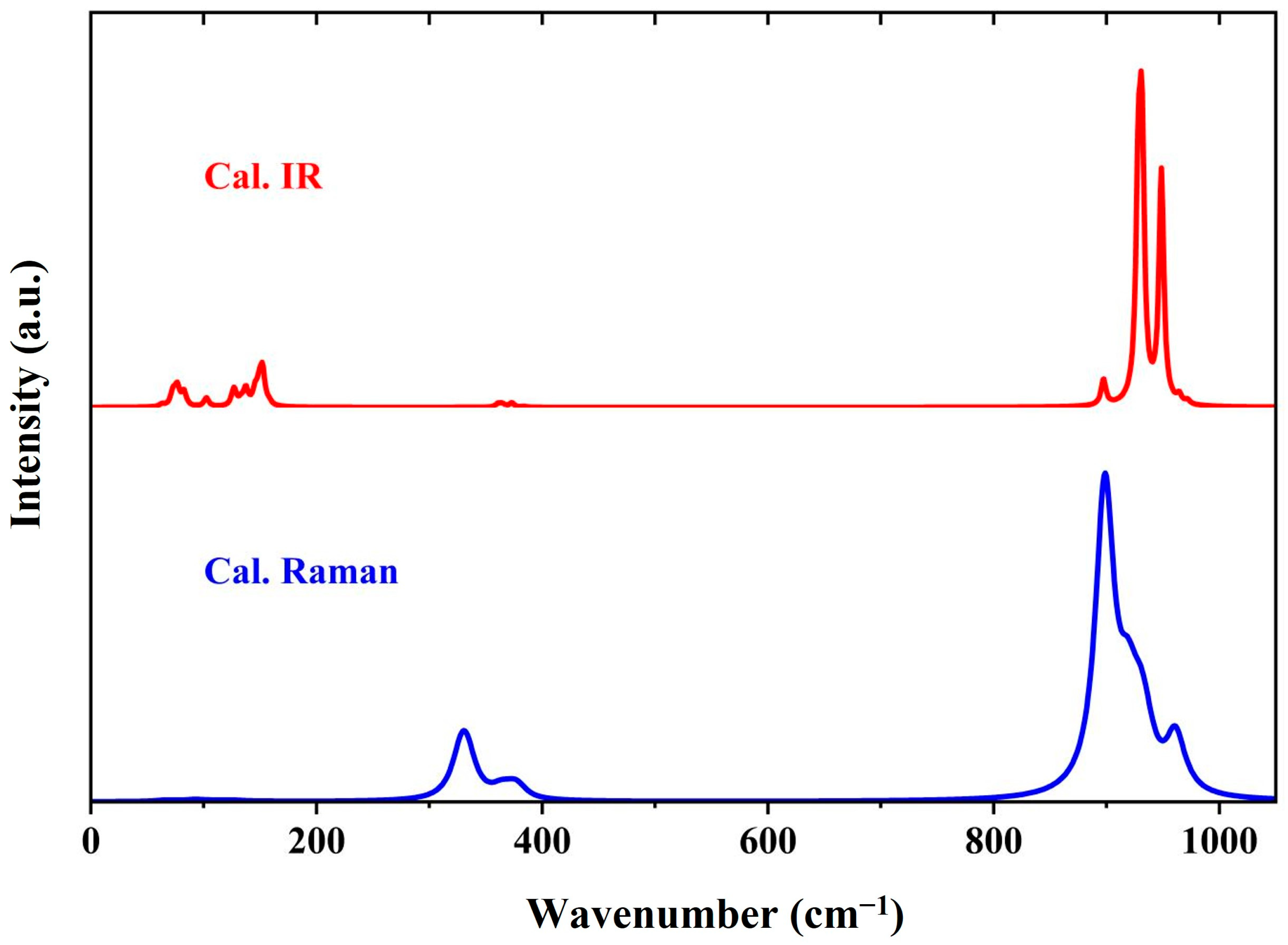
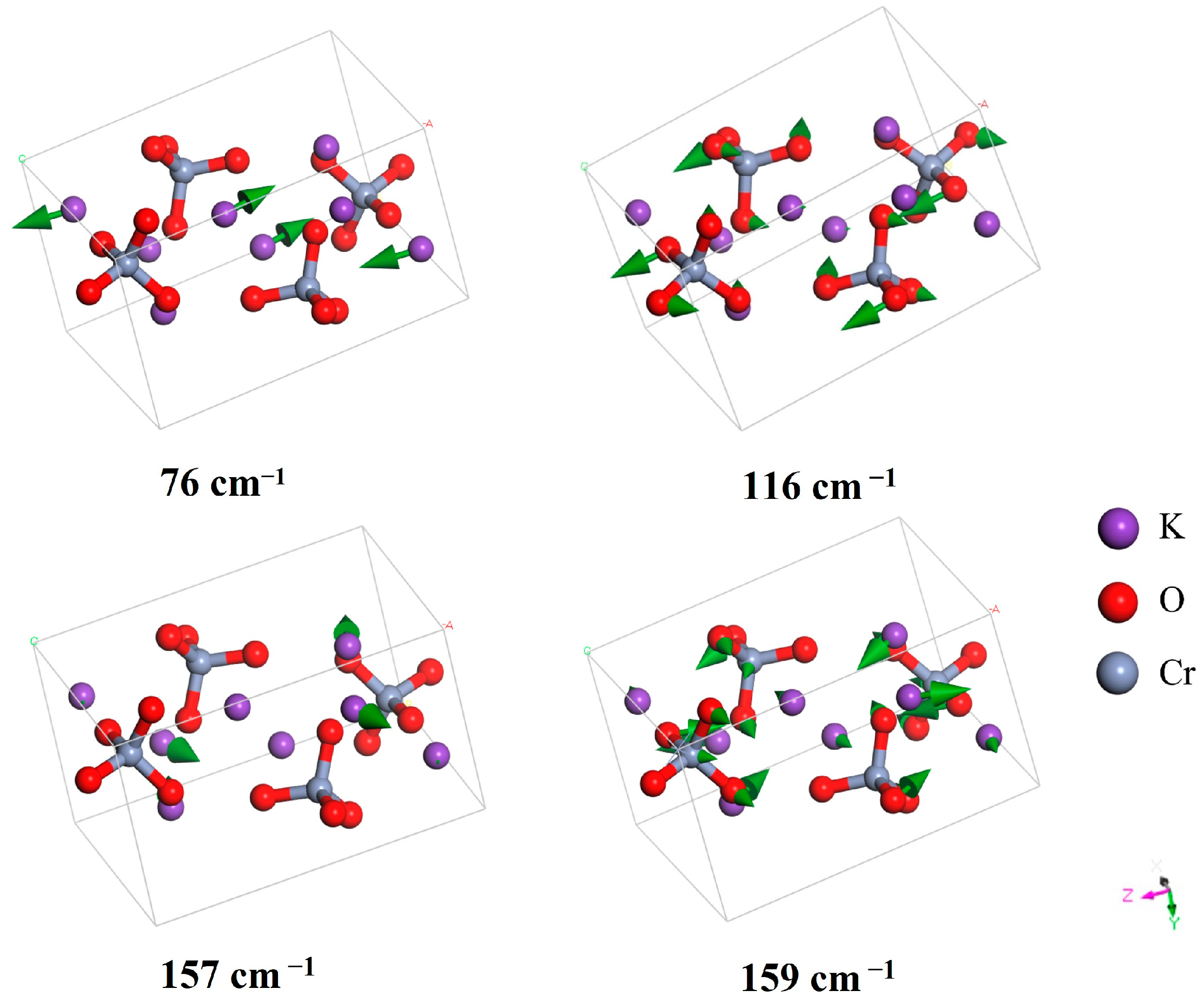
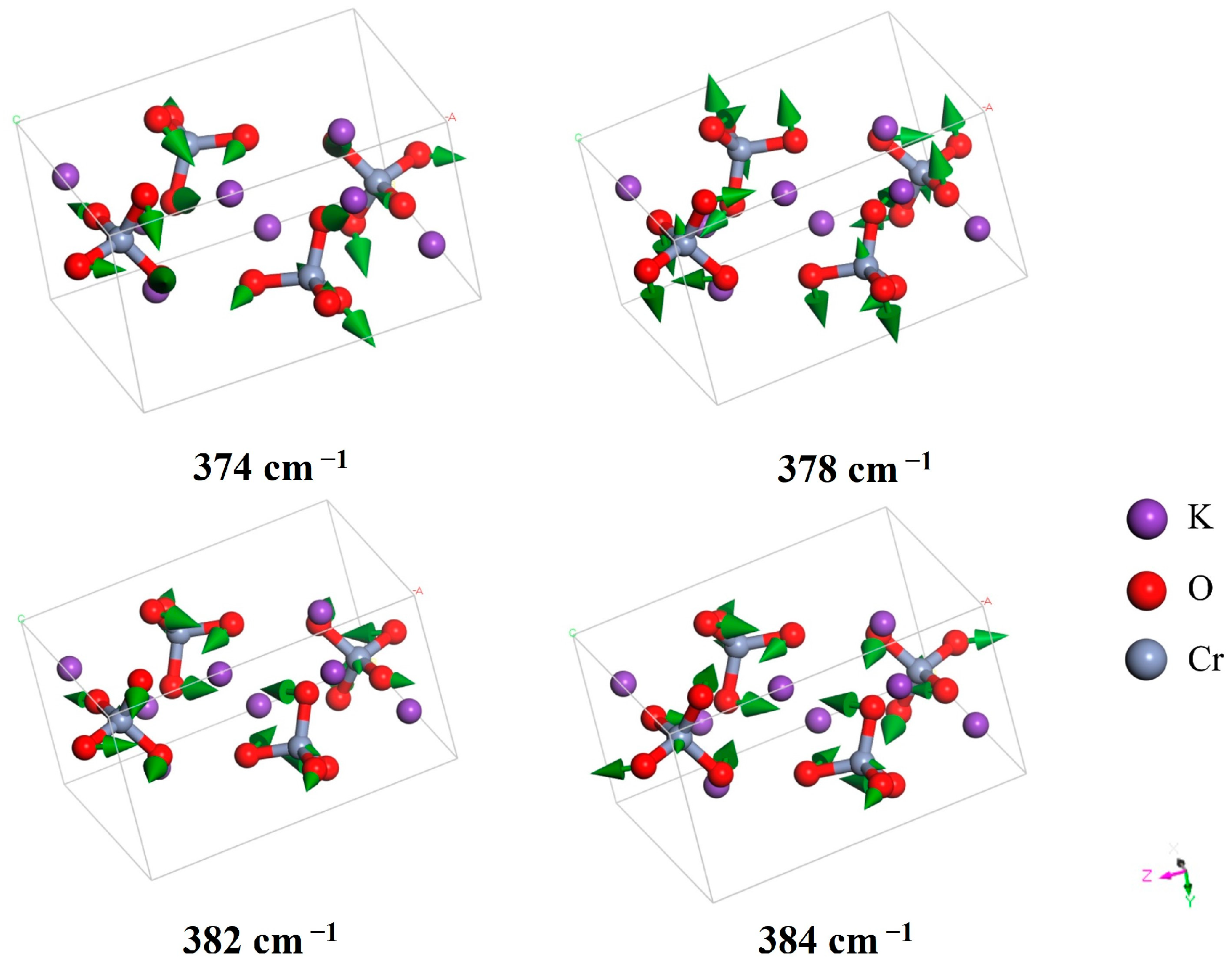
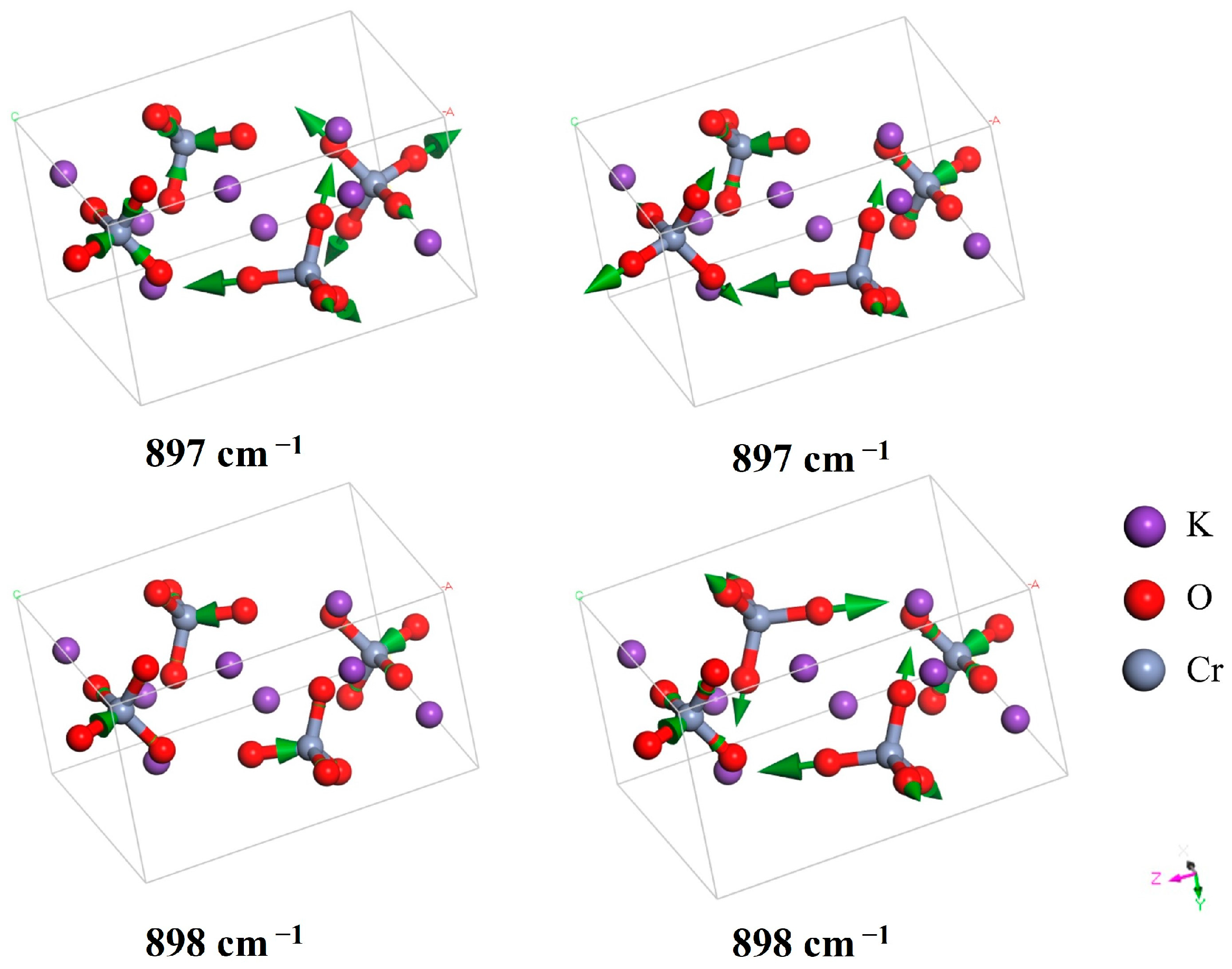
Wavenumber	Activity	IR exp.	Raman exp.	Assignment
23	Raman			Relative motion
39	Raman		37 b	Relative motion
62	Raman		54 b	Relative motion
64	IR			Relative motion
67	Raman		67 b	Relative motion
72	Raman			Relative motion
72	IR			Relative motion
74	Raman			Relative motion
76	IR			K+ translation
76	IR			Relative motion
80	Raman			Relative motion
81	Raman			Relative motion
84	IR			Relative motion
92	Raman		85 b	Relative motion
95	Raman		91 b	Relative motion
100	Raman		93 b	Relative motion
102	IR			Relative motion
103 (2)	Raman		99 b	Relative motion
105	IR			Relative motion
114	Raman		114 b	Relative motion
115	Raman			Relative motion
116	IR	106 d		Relative motion
119	Raman		119 b	Relative motion
125 (2)	IR			Relative motion
126	Raman			Relative motion
127	IR			Relative motion
131	Raman			Relative motion
133	IR			Relative motion
137	IR			Relative motion
138 (2)	Raman		138 b	Relative motion
141	Raman			Relative motion
145	IR			Relative motion
146	Raman			Relative motion
149	IR			Relative motion
152	IR	141 d		Relative motion
157	IR			K+ translation
159	Raman		157 b	Relative motion
165	Raman			Relative motion
324	IR			CrO42− bending
326	Raman			CrO42− bending
326	IR			CrO42− bending
329	Raman			CrO42− bending
332	Raman			CrO42− bending
334	Raman			CrO42− bending
336	IR			CrO42− bending
360	Raman			CrO42− bending
360	IR			CrO42− bending
363	Raman			CrO42− bending
364	IR			CrO42− bending
367	IR			CrO42− bending
369	Raman			CrO42− bending
373	IR	342 b, 343 c,d		CrO42− bending
374	Raman		345 b, 348 c	CrO42− bending
378	Raman		346 b, 351 c, 347 d	CrO42− bending
382	Raman		350 b, 352 c, 351 d	CrO42− bending
384	IR	382 b		CrO42− bending
897 (2)	IR	883 b, 876 c, 850 d		CrO42− stretching
898 (2)	Raman		859 a, 881 b, 884 c, 885 d	CrO42− stretching
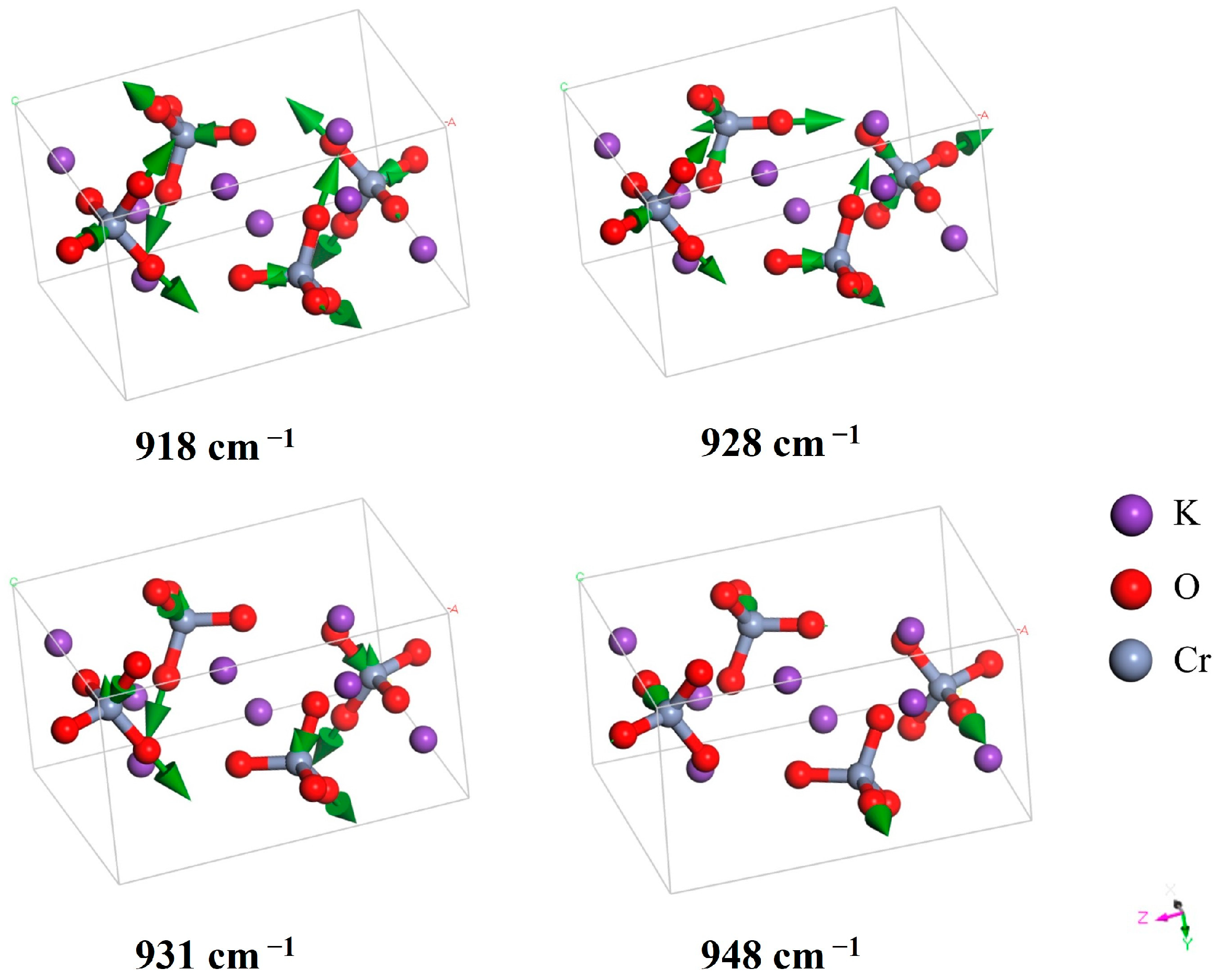
918	Raman		877 a, 918 b, 905 c, 905 d	CrO42− stretching
928	IR			CrO42− stretching
929	Raman			CrO42− stretching
931	IR	910 b, 890 c, 880 d		CrO42− stretching
932	Raman			CrO42− stretching
935	Raman			CrO42− stretching
948	IR	936 b, 920 c, 918 d		CrO42− stretching
961	Raman			CrO42− stretching
964	IR			CrO42− stretching
971	IR			CrO42− stretching
972	Raman			CrO42− stretching

^a, ref. [24]; ^b, ref. [25]; ^c, ref. [27]; ^d, ref. [28].

. We identified 42 Raman-active and 32 IR-active modes. The remaining seven modes are neither IR- nor Raman-active. Among the vibrational modes, 41 modes represent skeletal deformations, which are inter-molecular relative motions and belong to the lowest frequency band. The modes located at 103, 125, and 138 cm⁻¹ exhibit double degeneracy. The intra-molecular bond angle vibrations (referred to as bending vibrations) all exhibit higher vibrational energy. In the band from 324 to 384 cm⁻¹, there are 18 modes representing Cr-O bond bending vibrations. Another 15 vibrations located in the highest frequency band from 897 to 972 cm⁻¹ are intra-molecular stretching vibrations. The stretching vibration of atoms can be simply treated as a harmonic oscillator, where the vibrational angular frequency is given by

$$\omega =\sqrt{{\displaystyle \frac{k}{m}}}$$

(1)

Thus, lower atomic masses and greater bond strengths result in higher frequencies.

Figure 2 illustrate four vibrational modes in the vibrational band below 200 cm⁻¹. In the vibrational band below 200 cm⁻¹, most of the vibrational modes involve relative motion of the anions. Two vibrational modes correspond to the relative motion of K⁺ in the crystal lattice (76 and 157 cm⁻¹). Carter reported experimental Raman and IR spectra and identified Raman peaks located at 37, 54, 67, 85, 91, 93, 99, 114, 119, 138, and 157 cm⁻¹ [25]. As shown in Table 1, these peaks correspond to the simulated wavenumbers at 39, 62, 67, 92, 95, 100, 103, 114, 119, 138, and 159 cm⁻¹. As the intensities of these Raman peaks are much lower than the intensities of the Raman peaks at around 900 cm⁻¹, they cannot be observed in Figure 1.

For the middle band between 300 and 800 cm⁻¹, the vibrational modes are intra-molecular bond angle vibrations. Carter found two peaks in the IR spectrum at 342 and 382 cm⁻¹, corresponding to our simulated modes at 373 and 384 cm⁻¹ [25]. Adams et al. only observed one peak at 343 cm⁻¹ in this band [27]. Davies and Long also confirmed the IR peak at 343 cm⁻¹ [28]. Carter further reported three peaks (345, 346, and 350 cm⁻¹) in the Raman spectrum [25], which correspond to the simulated modes at 374, 378, and 382 cm⁻¹. Adams et al. observed these three peaks at 348, 351, and 352 cm⁻¹, respectively [27]. Davies and Long observed two Raman peaks at 347 and 351 cm⁻¹ in this area [28]. The simulated vibrational modes were consistent with the experimental data, and the dynamic analysis enabled scientific assignment of these peaks. Figure 3 depicts four bending modes located at 373, 378, 382, and 384 cm⁻¹.

The stretching vibrations of CrO₄²⁻ are located in the high-energy band. Venkateswaran identified four Raman peaks at 486, 513, 859, and 877 cm⁻¹ [24]. However, none of our simulated modes match the two peaks at 486 and 513 cm⁻¹. The peaks at 859 and 877 cm⁻¹ correspond to the Raman-active modes at 898 cm⁻¹ (doubly degenerate) and 918 cm⁻¹ obtained from our simulations. These are different stretching vibrational combinations of the four Cr-O bonds. Carter observed these two Raman peaks at 881 and 918 cm⁻¹ [25], Adams et al. reported them at 884 and 905 cm⁻¹, and Davies and Long reported them at 885 and 905 cm⁻¹ [27,28]. As no other experimental studies support the two peaks at 486 and 513 cm⁻¹ reported by Venkateswaran, we suspect that they are artefacts observed in error. In fact, there are eight Raman-active modes (the mode at 898 cm⁻¹ is doubly degenerate) in this band. The peaks missing from previous experiments were overlooked due to their low intensities.

In this band, there are seven IR-active vibrational modes (the mode at 897 cm⁻¹ is doubly degenerate), of which three peaks have been experimentally reported [24,25,27,28]. Carter observed peaks located at 883, 910, and 936 cm⁻¹. These correspond to the simulated vibrational modes at 897, 928/931, and 948 cm⁻¹, respectively [25]. Note that the IR-active mode at 928 cm⁻¹ is strong with high intensity, and hence may have merged with the mode at 931 cm⁻¹ to produce a single large peak in the experimental observations. Adams et al. observed these three peaks at 876, 890, and 920 cm⁻¹, while Davies and Long reported them at 850, 880, and 918 cm⁻¹ [28]. Figure 4 shows the two doubly degenerate modes at 897 cm⁻¹ (IR-active) and 898 cm⁻¹ (Raman-active). Figure 5 shows another four stretching modes.

According to the simulations, the highest-intensity Raman-active mode is located at 898 cm⁻¹, while the highest-intensity IR-active mode is located at 931 cm⁻¹. These represent different stretching vibrational combinations of Cr-O bonds. As the absorption peaks in the IR spectrum result from photon–phonon coupling, the IR-active vibrational mode at 931 cm⁻¹ corresponds to the mode with the strongest photon–phonon coupling intensity, meaning that the Cr-O bond exhibits the highest PPRA efficiency at this frequency (56 THz). Treating this vibrational mode as a harmonic oscillator, the phonon energy is equal to

$$E=\left(n+{\displaystyle \frac{1}{2}}\right)\hslash \omega =h\nu$$

(2)

If a photon with a frequency of $\nu$ is absorbed by a phonon during the PPRA process, the phonon will transition to a higher-energy state. Irradiating crystalline K₂CrO₄ with high-power laser light at this frequency may promote the energy level transition of the Cr-O bond, which in turn may facilitate the breaking of the Cr-O bond; the exact frequency should be accurately measured in real environments. This physical approach to Cr-O bond breaking potentially provides a pollution-free and environmentally friendly method for the separation of Cr.

In chromates, the anionic group CrO₄²⁻ is independent of the cation in terms of the appearance of its vibrational spectra. As a result, the vibrational modes of the Cr-O bond are similar, irrespective of the cation, in both infrared and Raman spectra. Therefore, this study on potassium chromate provides valuable insights for research on other chromates.

Compared with other PPRA works to induce the separation of rare metals, we found that this method shows broad application prospects. Ren et al. studied the vibrational spectra of sodium molybdate and pointed out that a laser at 25 THz can be used for Mo-O bond breaking [34]. Li et al. investigated the potassium heptafluorotantalate mineral and proposed three laser candidates for tantalum separation from the K₂TaF₇ compound [36].

4. Conclusions

Based on first-principles DFT, we simulated the IR and Raman spectra of K₂CrO₄. The experimentally measured peaks were assigned to the simulated vibrational modes. The results indicate that in the low-frequency band, the vibrational modes correspond to the relative motion between the ions in the crystal. In the energy band between 300 and 800 cm⁻¹, the vibrational modes can be attributed to bending vibrations of the Cr-O bonds within the anionic group CrO₄²⁻. In the high-energy band, there are 15 vibrational modes corresponding to stretching vibrations of Cr-O bonds. These represent different combinations of Cr-O bond stretching vibrations. In the experimental data, only three IR-active and two Raman-active peaks can be observed.

We focused on the stretching vibrational modes of the Cr-O bond in the high-frequency band. As shown in Figure 1, the strongest IR absorption peak was located at 931 cm⁻¹, implying that this is the most efficient PPRA frequency (56 THz). Thus, we propose the use of high-power terahertz laser radiation at this frequency for photothermal conversion to facilitate the separation of Cr from chromates. The exact frequency needs to be tested in laboratory experiments. The proposed PPRA method has potential applications in ice melting and natural gas hydrate extraction [34,35,41]. However, realizing its full potential remains highly challenging, as this technology requires high-power, frequency-selectable laser equipment. Nowadays, only free-electron laser facilities can conduct high-power frequency-selective laser experiments. However, conducting in situ tests simultaneously with the chemical preparation of chromium is challenging. Experimental validation will be undertaken in subsequent studies.