London Dispersive and Polar Surface Properties of Styrene–Divinylbenzene Copolymer Modified by 5-Hydroxy-6-Methyluracil Using Inverse Gas Chromatography
Abstract
1. Introduction
2. Materials and Methods
2.1. Adsorbent and Materials
2.2. Inverse Gas Chromatography
2.3. Thermodynamic Methods
2.3.1. Dispersive and Polar Energies and Lewis Acid–Base Parameters
2.3.2. London Dispersive Surface Energy and Lewis Acid–Base Surface Energies
3. Results
3.1. Variations of the Free Energy of Adsorption
3.2. London Dispersive Surface Energy of the System S-DVB-L285 with Different HMU Percentages
3.3. Polar Free Interaction Energy of S-DVB Copolymer Modified by HMU with the Polar Probes
3.4. Polar Enthalpy and Entropy of Adsorption, and Lewis Acid–Base Parameters of Dowex L-285 Modified by HMU
3.5. Polar Acid–Base Surface Energies of S-DVB Copolymer Modified by HMU
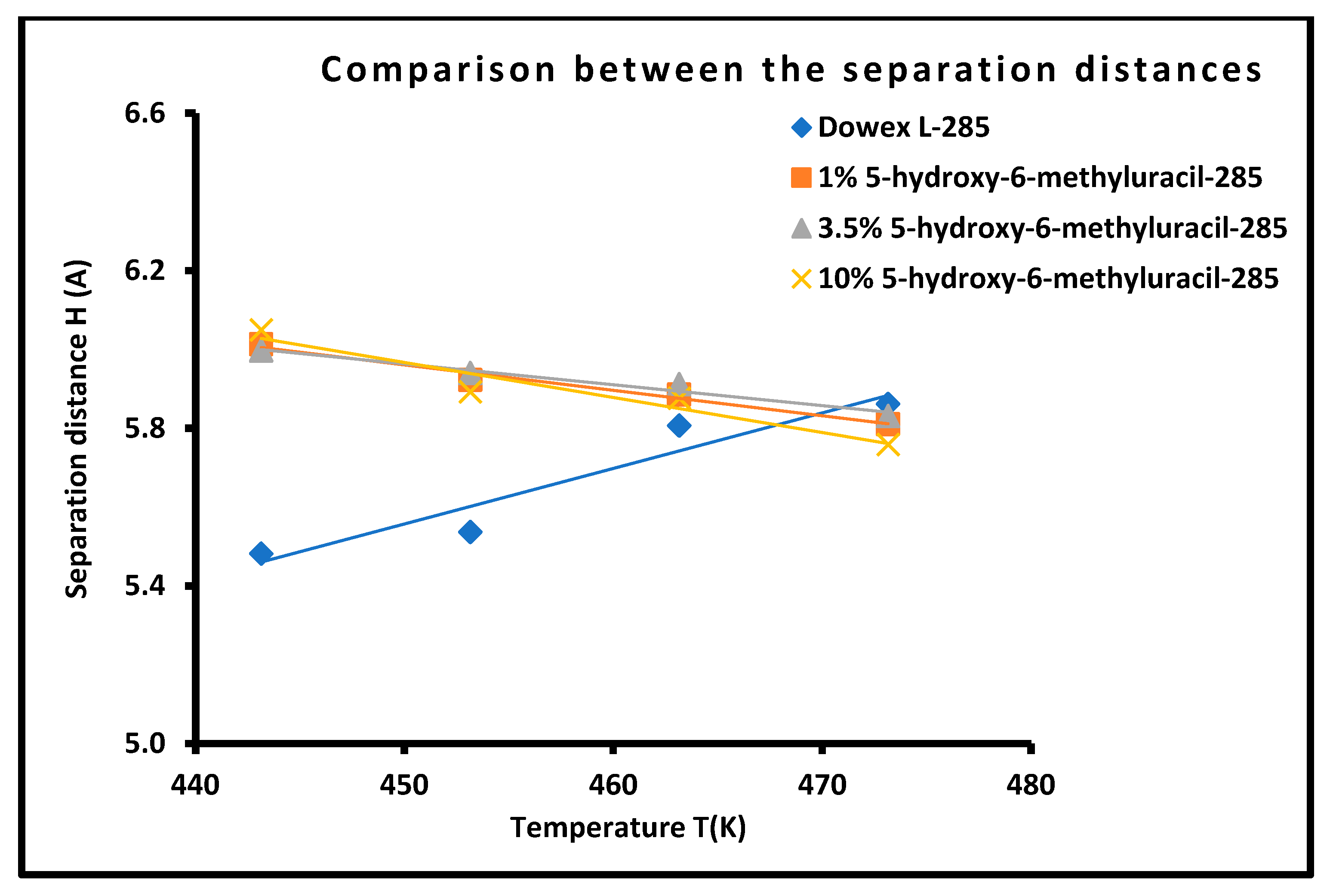
3.6. Determination of the Average Separation Distance H
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Helfferich, F. Ion Exchange; Dover Publications Inc.: New York, NY, USA, 1962; 624p. [Google Scholar]
- Kunin, R. Ion Exchange Resins; Wiley-Interscience: New York, NY, USA, 1972; 504p. [Google Scholar]
- Guo, X.; Zhang, S.; Shan, X. Adsorption of metal ions on lignin. J. Hazard. Mater. 2008, 151, 134–142. [Google Scholar] [CrossRef] [PubMed]
- Dąbrowski, A. Adsorption—From theory to practice. Adv. Colloid Interface Sci. 2001, 93, 135–224. [Google Scholar] [CrossRef]
- Vashurin, A.S.; Barabanov, V.M. Coordination and Sorption Capabilities of 5-Hydroxy-6-Methyluracil-Based Compounds. Russ. J. Gen. Chem. 2015, 85, 2287–2292. [Google Scholar]
- Pałasz, A.; Cież, D. In search of uracil derivatives as bioactive agents. Uracils and fused uracils: Synthesis, biological activity and applications. Eur. J. Med. Chem. 2015, 97, 582–611. [Google Scholar] [CrossRef]
- Samuilov, P. Modified uracils in medicinal chemistry. Russ. Chem. Rev. 2016, 85, 374–390. [Google Scholar]
- Epure, E.L.; Moleavin, I.A.; Taran, E.; Nguyen, A.V.; Nichita, N.; Hurduc, N. Azo-polymers modified with nucleobases and their interactions with DNA molecules. Polym. Bull. 2011, 67, 467–478. [Google Scholar] [CrossRef]
- Lee, K.M.; Kim, K.H.; Yoon, H.; Kim, H. Chemical Design of Functional Polymer Structures for Biosensors: From Nanoscale to Macroscale. Polymers 2018, 10, 551. [Google Scholar] [CrossRef]
- Lehn, J.-M. Supramolecular Chemistry. Concepts and Perspectives; VCH: Weinhiem, Germany, 1995; p. 271. [Google Scholar] [CrossRef]
- Singh, U.P.; Kashyap, S.; Singh, H.J.; Mishra, B.K.; Roy, P.; Chakraborty, A. Effect of adenosine on the supramolecular architecture and activity of 5-fluorouracil. J. Mol. Struct. 2012, 1014, 47–56. [Google Scholar] [CrossRef]
- Zaitsev, S.Y.; Vereschetin, V.P.; Gromov, S.P.; Fedorova, O.A.; Alfimov, M.V.; Huesmann, H.; Möbius, D. Photosensitive supramolecular systems based on amphiphilic crown ethers. Supramol. Sci. 1997, 4, 519–524. [Google Scholar] [CrossRef]
- Gus’kov, V.Y.; Bilalova, R.V.; Kudasheva, F.K. Adsorption of organic molecules on a 5-hydroxy-6-methyluracil -modified porous polymer. Russ. Chem. Bull. 2017, 66, 857–861. [Google Scholar] [CrossRef]
- Sukhareva, D.A.; Gus’kov, V.Y.; Karpov, S.I.; Kudasheva, F.K. Adsorption of organic molecules on the highly ordered MCM-41 sorbent modified by different amounts of 5-hydroxy-6-methyluracil. Russ. Chem. Bull. 2017, 66, 958–962. [Google Scholar] [CrossRef]
- Gus’kov, V.Y.; Gainullina, Y.Y.; Ivanov, S.P.; Kudasheva, F.K. Thermodynamics of organic molecule adsorption on sorbents modified with 5-hydroxy-6-methyluracil by inverse gas chromatography. J. Chromatogr. A 2014, 1356, 230–235. [Google Scholar] [CrossRef] [PubMed]
- Gus’kov, V.Y.; Gainullina, Y.Y.; Ivanov, S.P.; Kudasheva, F.K. Porous polymer adsorbents modified with uracil. Prot. Met. Phys. Chem. Surf. 2014, 50, 55–58. [Google Scholar] [CrossRef]
- Kronberg, B.; Holmberg, K.; Lindman, B. Adsorption of Polymers at Solid Surfaces. In Surface Chemistry of Surfactants and Polymers; Kronberg, B., Holmberg, K., Lindman, B., Eds.; John Wiley & Sons: Hoboken, NJ, USA, 2014. [Google Scholar] [CrossRef]
- Gautam, K.S.; Schwab, A.D.; Dinojwala, A.; Zang, D.; Dougal, S.M.; Yeganeh, M.S. Molecular structure of polystyrene at Air/Polymer and Solid/Polymer interfaces. Phys. Rev. Lett. 2000, 85, 3854. [Google Scholar] [CrossRef] [PubMed]
- Wilson, P.T.; Richter, L.J.; Wallace, W.; Briggman, K.; Stephenson, J.C. Correlation of molecular orientation with adhesion at polystyrene/solid interfaces. Chem. Phys. Lett. 2002, 363, 161. [Google Scholar] [CrossRef]
- Tatek, Y.B.; Tsige, M. Structural properties of atactic polystyrene adsorbed onto solid surfaces. J. Chem. Phys. 2011, 135, 174708. [Google Scholar] [CrossRef] [PubMed]
- Xu, W.; Mihhels, K.; Kotov, N.; Lepikko, S.; Ras, R.H.A.; Johnson, C.M.; Pettersson, T.; Kontturi, E. Solid-state polymer adsorption for surface modification: The role of molecular weight. J. Colloid Interface Sci. 2022, 605, 441–450. [Google Scholar] [CrossRef]
- Fleer, G.J.; Stuart, M.A.C.; Scheutjens, J.M.H.M.; Cosgrove, T.; Vincent, B. General Features of Polymers at Interfaces. In Polymers at Interfaces; Springer: Dordrecht, The Netherlands, 1998. [Google Scholar] [CrossRef]
- Fujisawa, S.; Saito, T.; Kimura, S.; Iwata, T.; Isogai, A. Surface engineering of ultrafine cellulose nanofibrils toward polymer nanocomposite materials. Biomacromolecules 2013, 14, 1541–1546. [Google Scholar] [CrossRef]
- Hasan, N.; Nguyen, T.M.H.; Busse, K.; Kressler, J. Influence of Tacticity on the Structure Formation of Poly(methacrylic acid) in Langmuir/Langmuir–Blodgett and Thin Films. Macromol. Chem. Phys. 2023, 224, 2200428. [Google Scholar] [CrossRef]
- Ohkawa, J.; Kumaki, J. Chain movements of a molecularly flat PMMA substrate surface prepared by thermal imprinting with mica and isolated PMMA chains deposited on the PMMA substrate observed by AFM around the bulk Tg. Polym J. 2022, 54, 281–292. [Google Scholar] [CrossRef]
- Baljon, A.R.C.; Williams, S.; Balabaev, N.K.; Paans, F.; Hudzinskyy, D.; Lyulin, A.V. Simulated glass transition in free-standing thin polystyrene films. J. Polym. Sci, Part B 2010, 48, 1160–1167. [Google Scholar] [CrossRef]
- Wang, Y.; Acton, O.; Ting, G.; Weidner, T.; Shamberge, P.J.; Ma, H.; Ohuchi, F.S.; Castner, D.G.; Jen, A.A.-K. Lowering programmed voltage of organic memory transistors based on polymer gate electrets through heterojunction fabrication. Org. Electron. 2010, 11, 1066. [Google Scholar] [CrossRef]
- Ito, M.; Ishizone, T. Living anionic polymerization of N-methoxymethyl-N-isopropylacrylamide: Synthesis of well-defined poly(N-isopropylacrylamide) having various stereoregularity. J. Polym. Sci. A Polym. Chem. 2006, 44, 4832–4845. [Google Scholar] [CrossRef]
- Bekele, S.; Tsige, M. Interfacial Properties of Oxidized Polystyrene and Its Interaction with Water. Langmuir 2016, 32, 7151. [Google Scholar] [CrossRef]
- Papirer, E.; Perrin, J.-M.; Siffert, B.; Philipponneau, G. Surface characteristics of aluminas in relation with polymer adsorption. J. Colloid Interface Sci. 1991, 144, 263–270. [Google Scholar] [CrossRef]
- Dritsas, G.S.; Karatasos, K.; Panayiotou, C. Investigation of thermodynamic properties of hyperbranched aliphatic polyesters by inverse gas chromatography. J. Chromatogr. A 2009, 1216, 8979–8985. [Google Scholar] [CrossRef]
- Stella, K.P.; Costas, P. Assessment of the thermodynamic properties of poly(2,2,2-trifluoroethyl methacrylate) by inverse gas chromatography. J. Chromatogr. A 2014, 1324, 207–214. [Google Scholar] [CrossRef]
- Das, S.C.; Stewart, P.J. Characterising surface energy of pharmaceutical powders by inverse gas chromatography at finite dilution. J. Pharm. Pharmacol. 2012, 64, 1337–1348. [Google Scholar] [CrossRef] [PubMed]
- Sánchez-Calderón, I.; Bernardo, V.; Martín-de-León, J.; Rodríguez-Pérez, M.A. Thermal conductivity of low-density micro-and nanocellular poly(methyl-methacrylate) (PMMA): Experimental and modeling. Mater. Des. 2022, 221, 110938. [Google Scholar] [CrossRef]
- Hofmeister, A.M. Thermal diffusivity and thermal conductivity of single-crystal MgO and Al2O3 and related compounds as a function of temperature. Phys. Chem Miner. 2014, 41, 361–371. [Google Scholar] [CrossRef]
- Wu, X.; Lee, J.; Varshney, V.; Wohlwend, J.L.; Roy, A.K.; Luo, T. Thermal Conductivity of Wurtzite Zinc-Oxide from First-Principles Lattice Dynamics—A Comparative Study with Gallium Nitride. Sci. Rep. 2016, 6, 22504. [Google Scholar] [CrossRef] [PubMed]
- Schultz, J.; Lavielle, L.; Martin, C. The Role of the Interface in Adhesion. J. Adhes. 1987, 23, 45–60. [Google Scholar] [CrossRef]
- Mittal, K.L. Contact Angle, Wettability and Adhesion; VSP: Leiden, The Netherlands, 2003. [Google Scholar]
- van Oss, C.J. Interfacial Forces in Aqueous Media, 2nd ed.; CRC Press: Boca Raton, FL, USA, 2006. [Google Scholar]
- Hamieh, T. Study of the temperature effect on the surface area of model organic molecules, the dispersive surface energy and the surface properties of solids by inverse gas chromatography. J. Chromatogr. A 2020, 1627, 461372. [Google Scholar] [CrossRef] [PubMed]
- Hamieh, T. New methodology to study the dispersive component of the surface energy and acid–base properties of silica particles by inverse gas chromatography at infinite dilution. J. Chromatogr. Sci. 2022, 60, 126–142. [Google Scholar] [CrossRef]
- Davankov, V.A.; Tsyurupa, M.P. Highly Crosslinked Macroporous Polymers: Synthesis, Properties, Applications. React. Funct. Polym. 2006, 66, 846–856. [Google Scholar]
- Le Vu, H.; Nguyen, S.H.; Dang, K.Q.; Pham, C.V.; Le, H.T. The Effect of Oxidation Temperature on Activating Commercial Viscose Rayon-Based Carbon Fibers to Make the Activated Carbon Fibers (ACFs). Mater. Sci. Forum 2020, 985, 171–176. [Google Scholar] [CrossRef]
- Liu, Y.; Gu, Y.; Wang, S.; Li, M. Optimization for testing conditions of inverse gas chromatography and surface energies of various carbon fiber bundles. Carbon Lett. 2023, 33, 909–920. [Google Scholar] [CrossRef]
- Pal, A.; Kondor, A.; Mitra, S.; Thua, K.; Harish, S.; Saha, B.B. On surface energy and acid–base properties of highly porous parent and surface treated activated carbons using inverse gas chromatography. J. Ind. Eng. Chem. 2019, 69, 432–443. [Google Scholar] [CrossRef]
- Ma, M.; Cui, W.; Guo, Y.; Yu, W. Adsorption-desorption effect on physical aging in PMMA-silica nanocomposites. Polymer 2022, 255, 125124. [Google Scholar] [CrossRef]
- Cui, W.; You, W.; Sun, Z.; Yu, W. Decoupled polymer dynamics in weakly attractive poly(methyl methacrylate)/silica nanocomposites. Macromolecules 2021, 54, 5484–5497. [Google Scholar] [CrossRef]
- Huang, C.-C.; Liu, C.-Y. Peculiar α-β relaxations of Syndiotactic-Poly(methyl methacrylate). Polymer 2021, 225, 123760. [Google Scholar] [CrossRef]
- Kawaguchi, D.; Sasahara, K.; Inutsuka, M.; Abe, T.; Yamamoto, S.; Tanaka, K. Absolute local conformation of poly(methyl methacrylate) chains adsorbed on a quartz surface. J. Chem. Phys. 2023, 159, 244902. [Google Scholar] [CrossRef] [PubMed]
- Hamieh, T.; Schultz, J. New approach to characterise physicochemical properties of solid substrates by inverse gas chromatography at infinite dilution. Some new methods to determine the surface areas of some molecules adsorbed on solid surfaces. J. Chromatogr. A 2002, 969, 17–47. [Google Scholar] [CrossRef] [PubMed]
- Kaur, D.R.; Roychowdhury, T.; Kinarivala, N.; Kaur, K.; Sanduja, M.; Sharma, S. Recent Expansions in the Potential of Uracil Derivatives as Chemotherapeutic, Antimicrobial, and Antiviral Agents: A Review. ChemistrySelect 2025, 11, e202406021. [Google Scholar] [CrossRef]
- Hamieh, T. Inverse Gas Chromatography to Characterize the Surface Properties of Solid Materials. Chem. Mater. 2024, 36, 2231–2244. [Google Scholar] [CrossRef]
- Hamieh, T. Some Irregularities in the Evaluation of Surface Parameters of Solid Materials by Inverse Gas Chromatography. Langmuir 2023, 39, 17059–17070. [Google Scholar] [CrossRef]
- Hamieh, T. New Progress on London Dispersive Energy, Polar Surface Interactions, and Lewis’s Acid–Base Properties of Solid Surfaces. Molecules 2024, 29, 949. [Google Scholar] [CrossRef]
- Hamieh, T. London Dispersive and Lewis Acid-Base Surface Energy of 2D Single-Crystalline and Polycrystalline Covalent Organic Frameworks. Crystals 2024, 14, 148. [Google Scholar] [CrossRef]
- Hamieh, T.; Gus’kov, V.Y. Surface Thermodynamic Properties of Styrene–Divinylbenzene Copolymer Modified by Supramolecular Structure of Melamine Using Inverse Gas Chromatography. Chemistry 2024, 6, 830–851. [Google Scholar] [CrossRef]
- Gutmann, V. The Donor-Acceptor Approach to Molecular Interactions; Springer: New York, NY, USA, 1978; p. 279. [Google Scholar]
- Riddle, F.L.; Fowkes, F.M. Spectral shifts in acid-base chemistry. Van der Waals contributions to acceptor numbers, Spectral shifts in acid-base chemistry. 1. van der Waals contributions to acceptor numbers. J. Am. Chem. Soc. 1990, 112, 3259–3264. [Google Scholar] [CrossRef]
- Fowkes, F.M. Volume 1 Surface Chemistry and Physics. In Surface and Interfacial Aspects of Biomedical Polymers; Andrade, J.D., Ed.; Plenum Press: New York, NY, USA, 1985; pp. 337–372. [Google Scholar]
- Van Oss, C.J.; Good, R.J.; Chaudhury, M.K. Additive and nonadditive surface tension components and the interpretation of contact angles. Langmuir 1988, 4, 884. [Google Scholar] [CrossRef]
- Dorris, G.M.; Gray, D.G. Adsorption of n-alkanes at zero surface coverage on cellulose paper and wood fibers. J. Colloid Interface Sci. 1980, 77, 353–362. [Google Scholar] [CrossRef]
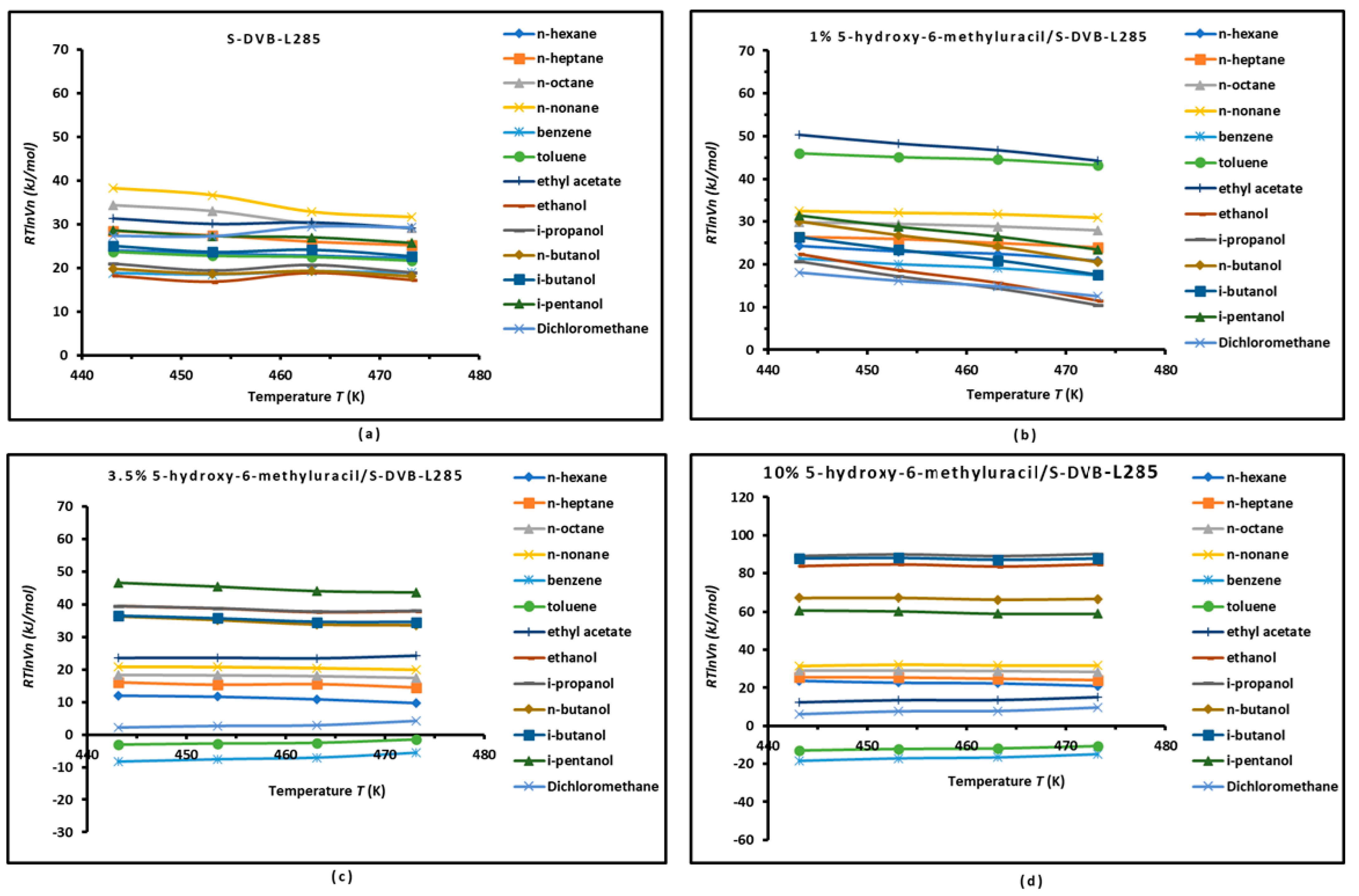
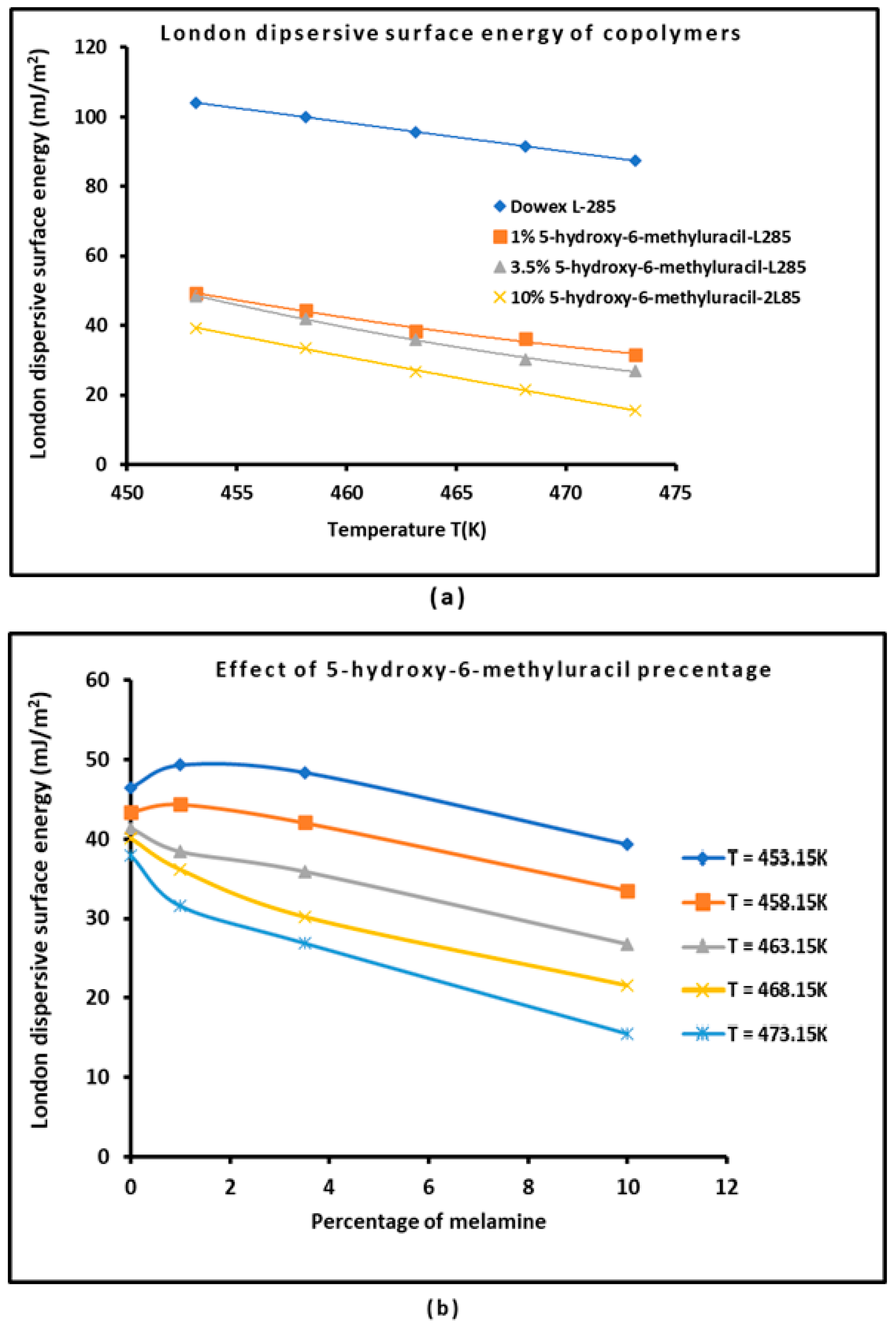
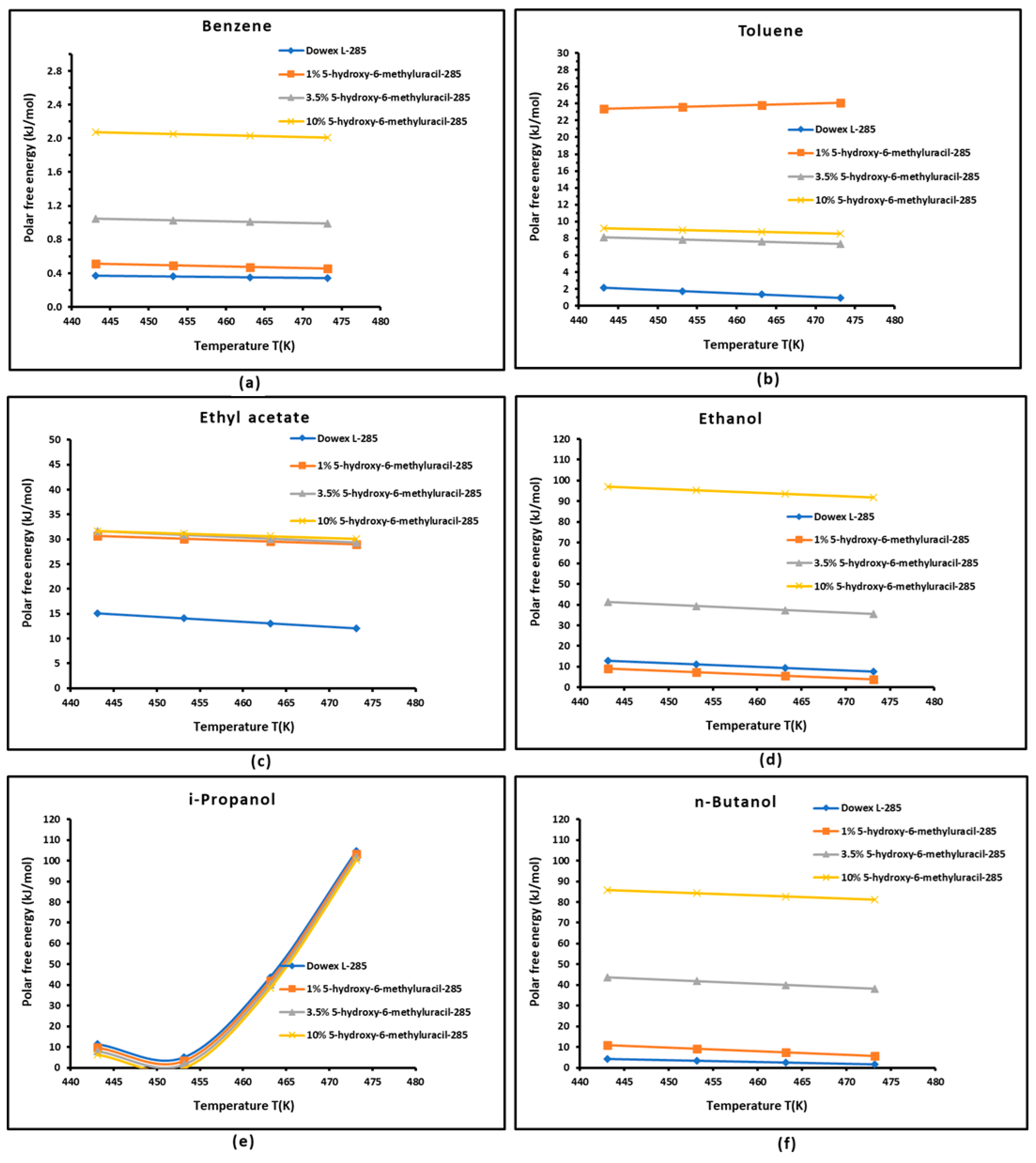
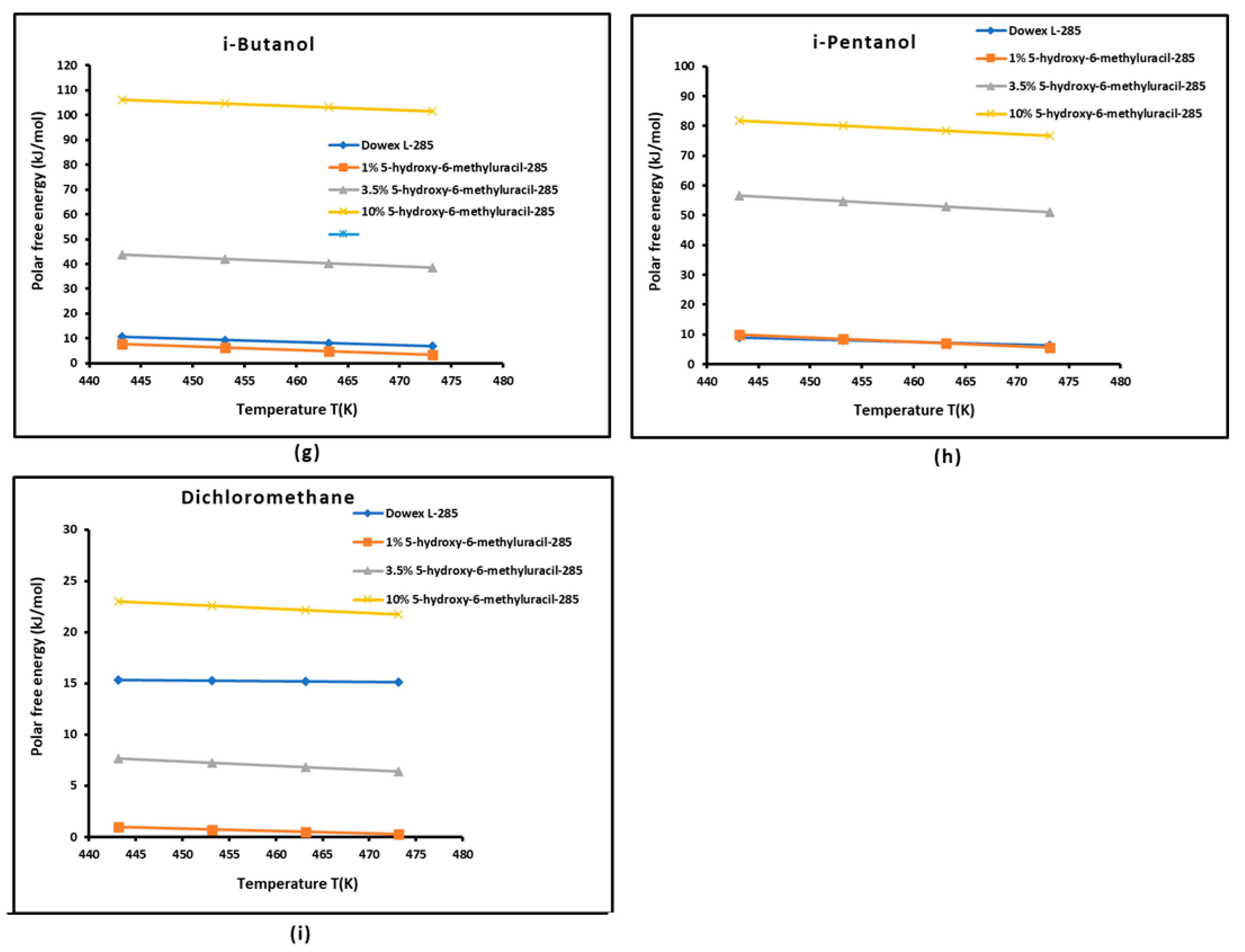
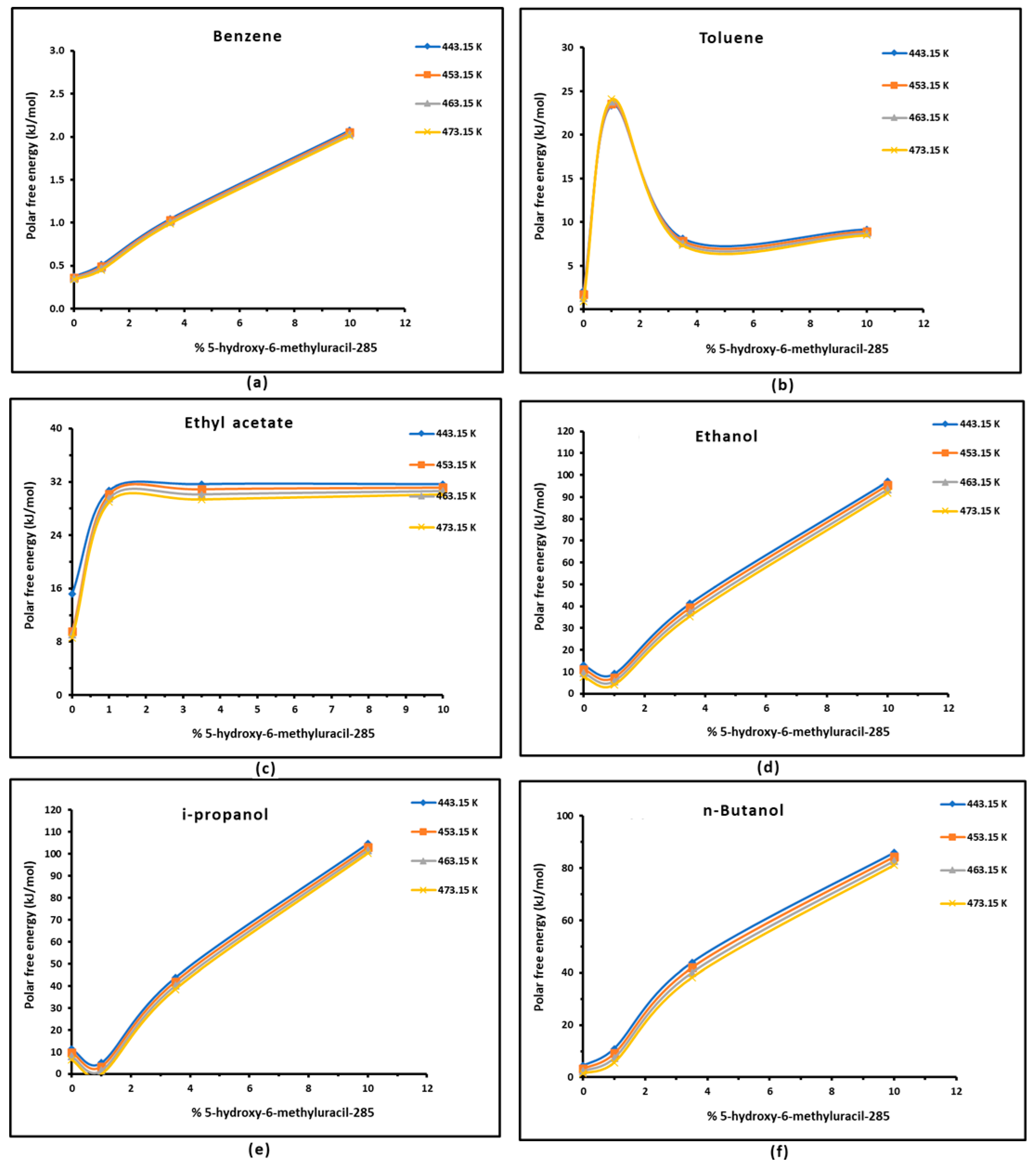
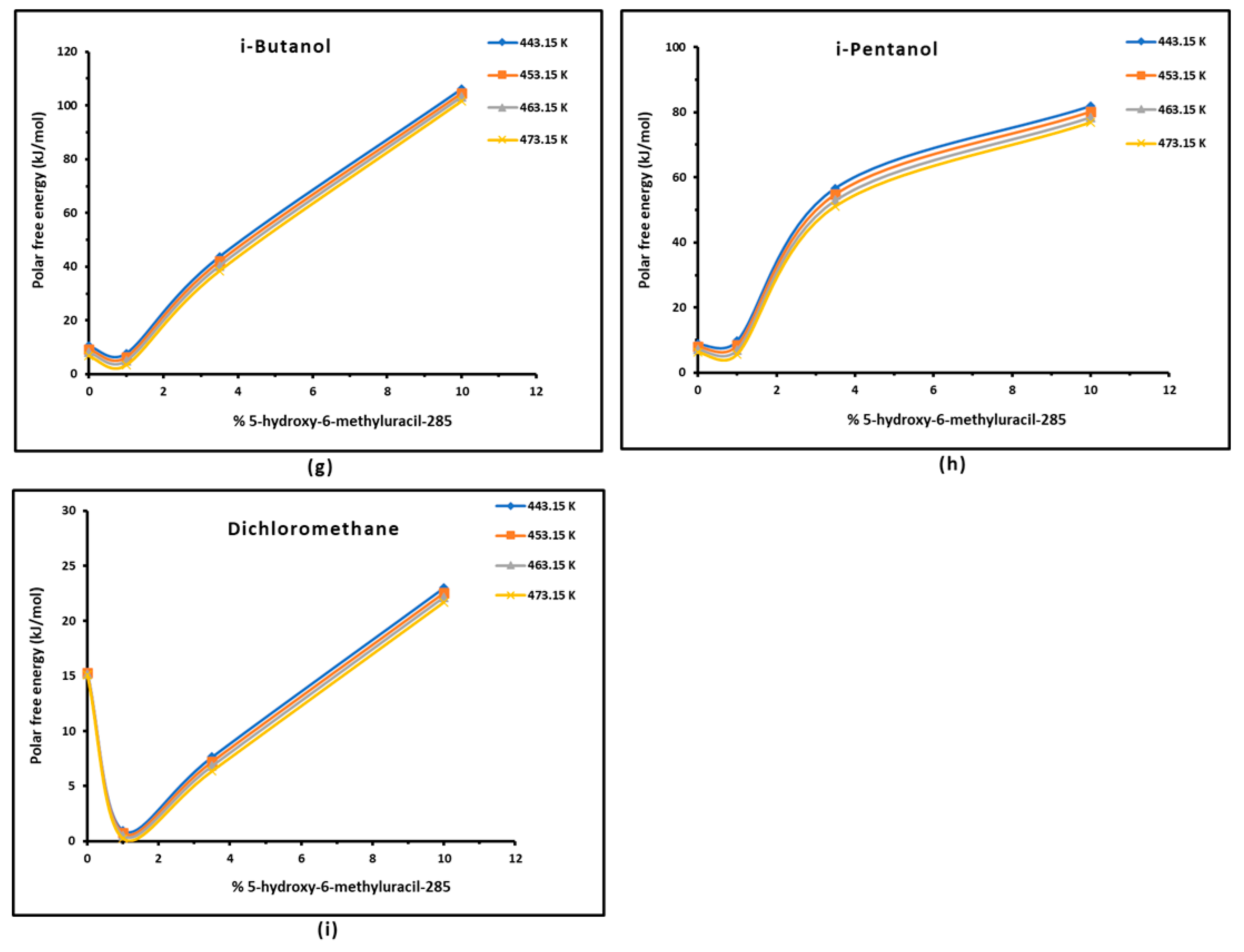

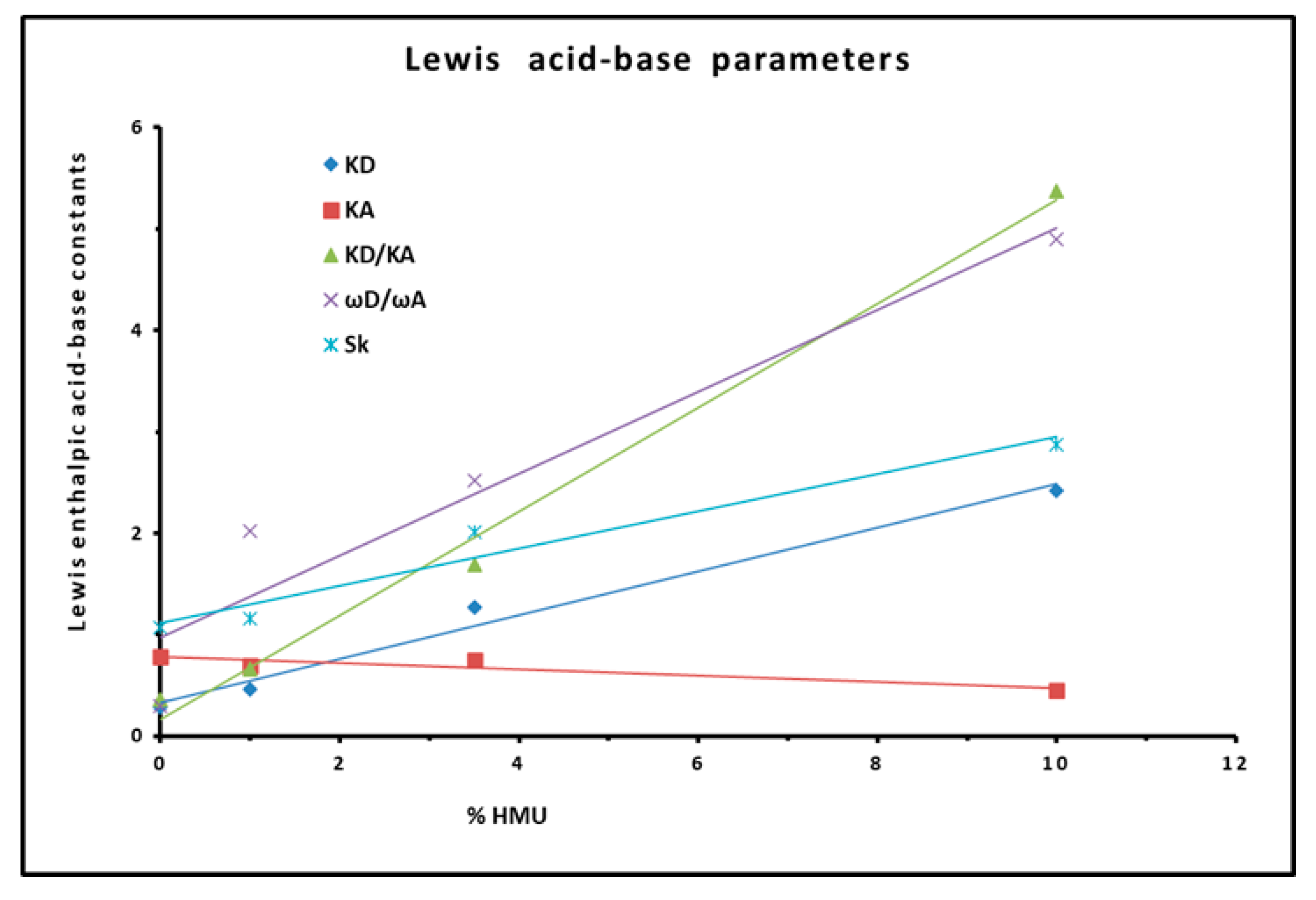

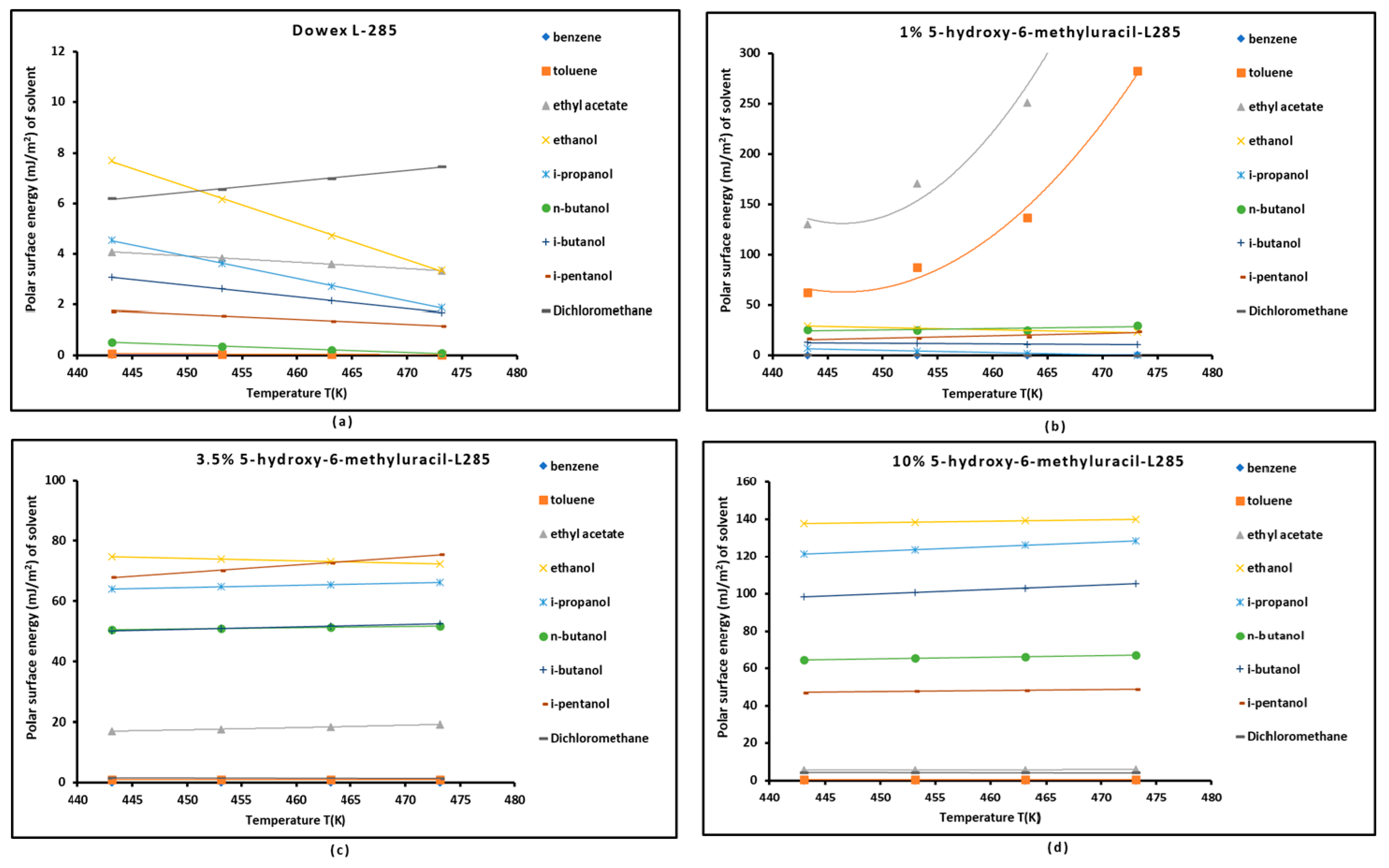
| Copolymer | (mJ/m2) | R2 | (mJ m−2 K−1) | (mJ/m2) | (mJ/m2) | (K) |
|---|---|---|---|---|---|---|
| S-DVB-L-285 | = −0.835 T + 482.43 | 0.9980 | −0.835 | 482.43 | 233.47 | 577.8 |
| 1% HMU/S-DVB-L-285 | = −0.874 T + 444.68 | 0.9837 | −0.874 | 444.68 | 184.16 | 508.9 |
| 3.5% HMU/S-DVB-L-285 | = −1.096 T + 544.23 | 0.9886 | −1.096 | 544.23 | 217.52 | 496.7 |
| 10% HMU/S-DVB-L-285 | = −1.198 T + 582.13 | 0.9973 | −1.198 | 582.13 | 224.95 | 485.9 |
| Copolymer | (mJ/m2) | R2 | (mJ m−2 K−1) | (mJ/m2) | (mJ/m2) | (K) |
|---|---|---|---|---|---|---|
| S-DVB-L-285 | = −3.250 T + 1610.5 | 0.9037 | −3.25 | 1610.5 | 641.51 | 495.54 |
| 1% HMU/S-DVB-L-285 | = 1.065 T − 416.4 | 0.9855 | 1.065 | −416.4 | −98.87 | 390.99 |
| 3.5% HMU/S-DVB-L-285 | = 1.091 T − 421.2 | 0.9493 | 1.091 | −421.2 | −95.92 | 386.07 |
| 10% HMU/S-DVB-L-285 | = 1.474 T − 600.3 | 0.9413 | 1.474 | −600.3 | −160.83 | 407.26 |
| Material | R2 | ) | R2 | |||||||
|---|---|---|---|---|---|---|---|---|---|---|
| S-DVB-L-285 | 0.283 | 0.786 | 0.36 | 1.069 | 0.886 | 0.43 | 1.41 | 0.30 | 1.83 | 0.871 |
| 1% HMU on S-DVB-L-285 | 0.459 | 0.696 | 0.66 | 1.155 | 0.9561 | 1.64 | 0.81 | 2.02 | 2.45 | 0.975 |
| 3.5% HMU on S-DVB-L-285 | 1.265 | 0.75 | 1.69 | 2.015 | 0.994 | 2.07 | 0.82 | 2.52 | 2.89 | 0.9806 |
| 10% HMU on S-DVB-L-285 | 2.425 | 0.451 | 5.38 | 2.876 | 0.9397 | 2.25 | 0.46 | 4.89 | 2.71 | 0.9277 |
| Parameter | Equation | R2 |
|---|---|---|
| Basic constant | = 0.22%HMU + 0.33 | 0.9839 |
| Acid constant | = −0.031%HMU + 0.784 | 0.8638 |
| Ratio | = 0.51%HMU + 0.17 | 0.9926 |
| Ratio / | / = 0.40%HMU + 0.97 | 0.9165 |
| Parameter | = 0.18%HMU + 1.11 | 0.9561 |
| Dichloromethane | ||||
| T(K) | Dowex L-285 | 3.5% HMU/Dowex L-285 | 1% HMU/Dowex L-285 | 10% HMU/Dowex L-285 |
| 453.15 | 15.274 | 15.609 | 24.349 | 9.737 |
| 458.15 | 15.239 | 15.559 | 24.294 | 9.352 |
| 463.15 | 15.204 | 15.509 | 24.239 | 8.967 |
| 468.15 | 15.169 | 15.459 | 24.184 | 8.582 |
| Ethyl Acetate | ||||
| T(K) | Dowex L-285 | 3.5% HMU/Dowex L-285 | 1% HMU/Dowex L-285 | 10% HMU/Dowex L-285 |
| 453.15 | 10.106 | 8.503 | 9.667 | 10.567 |
| 458.15 | 9.593 | 8.378 | 9.507 | 10.314 |
| 463.15 | 9.079 | 8.253 | 9.347 | 10.06 |
| 468.15 | 8.566 | 8.128 | 9.187 | 9.807 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hamieh, T.; Gus’kov, V.Y. London Dispersive and Polar Surface Properties of Styrene–Divinylbenzene Copolymer Modified by 5-Hydroxy-6-Methyluracil Using Inverse Gas Chromatography. Crystals 2025, 15, 438. https://doi.org/10.3390/cryst15050438
Hamieh T, Gus’kov VY. London Dispersive and Polar Surface Properties of Styrene–Divinylbenzene Copolymer Modified by 5-Hydroxy-6-Methyluracil Using Inverse Gas Chromatography. Crystals. 2025; 15(5):438. https://doi.org/10.3390/cryst15050438
Chicago/Turabian StyleHamieh, Tayssir, and Vladimir Yu Gus’kov. 2025. "London Dispersive and Polar Surface Properties of Styrene–Divinylbenzene Copolymer Modified by 5-Hydroxy-6-Methyluracil Using Inverse Gas Chromatography" Crystals 15, no. 5: 438. https://doi.org/10.3390/cryst15050438
APA StyleHamieh, T., & Gus’kov, V. Y. (2025). London Dispersive and Polar Surface Properties of Styrene–Divinylbenzene Copolymer Modified by 5-Hydroxy-6-Methyluracil Using Inverse Gas Chromatography. Crystals, 15(5), 438. https://doi.org/10.3390/cryst15050438







