Research on the Mechanical and Photoelectric Properties Regulation of the New-Type Ceramic Material Ta2AlC
Abstract
1. Introduction
2. Model and Method
3. Results and Discussion
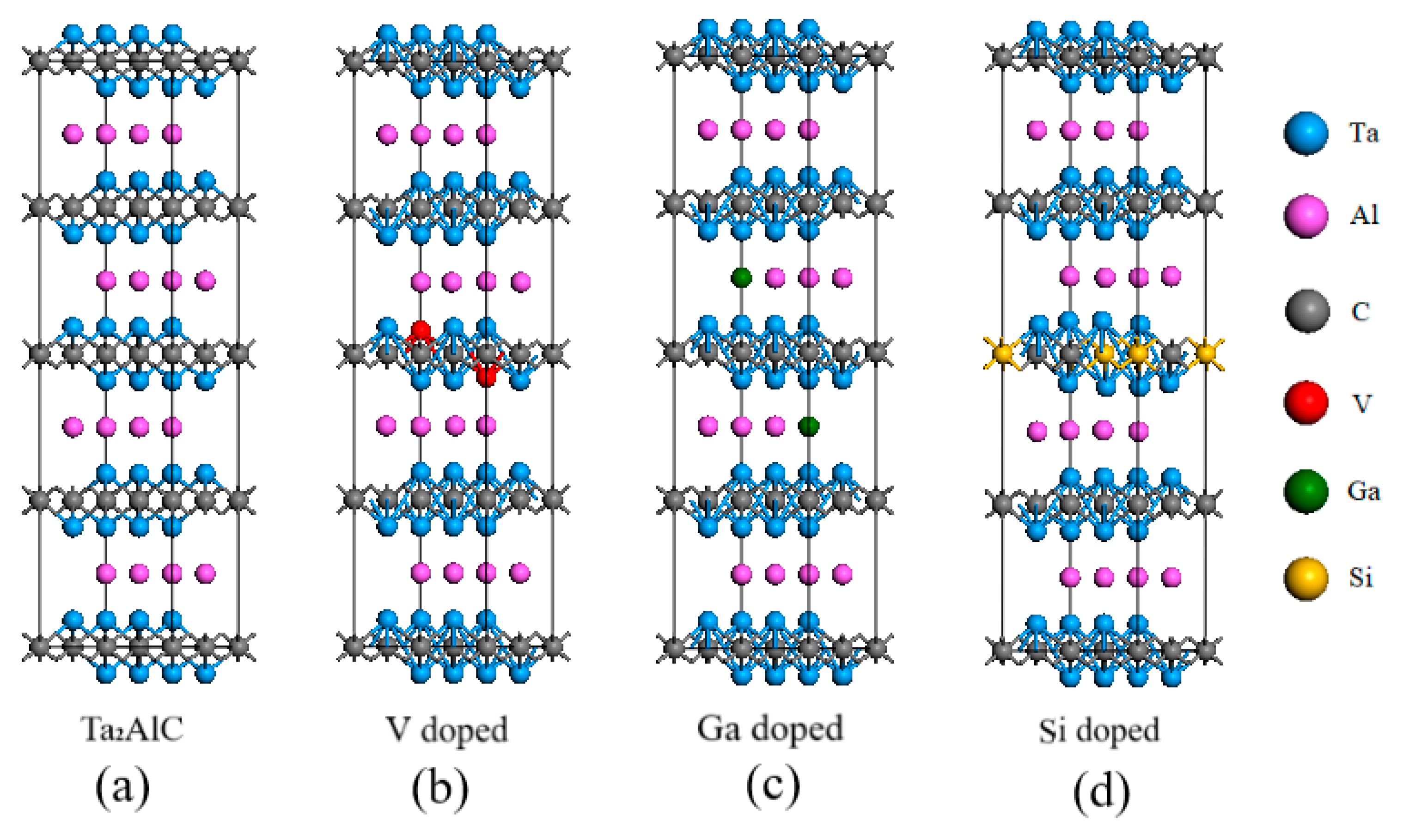
3.1. Geometric Structure
3.2. Mechanical Properties
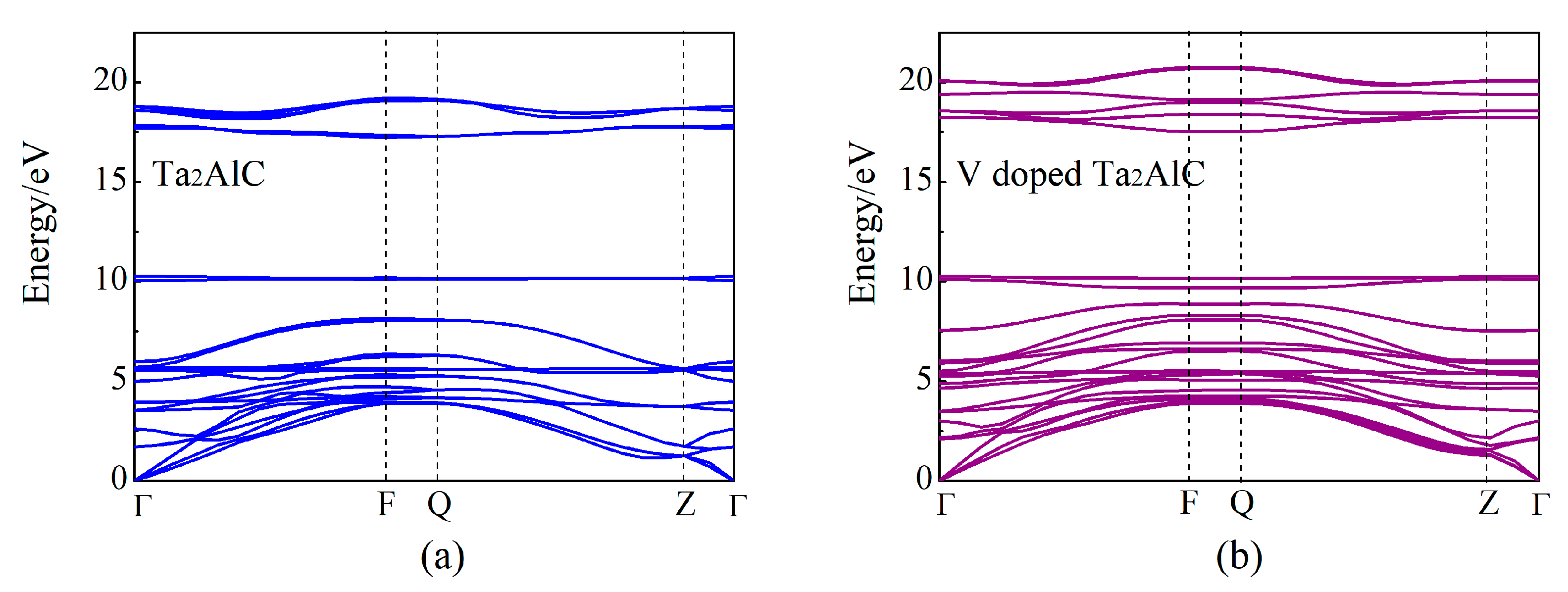
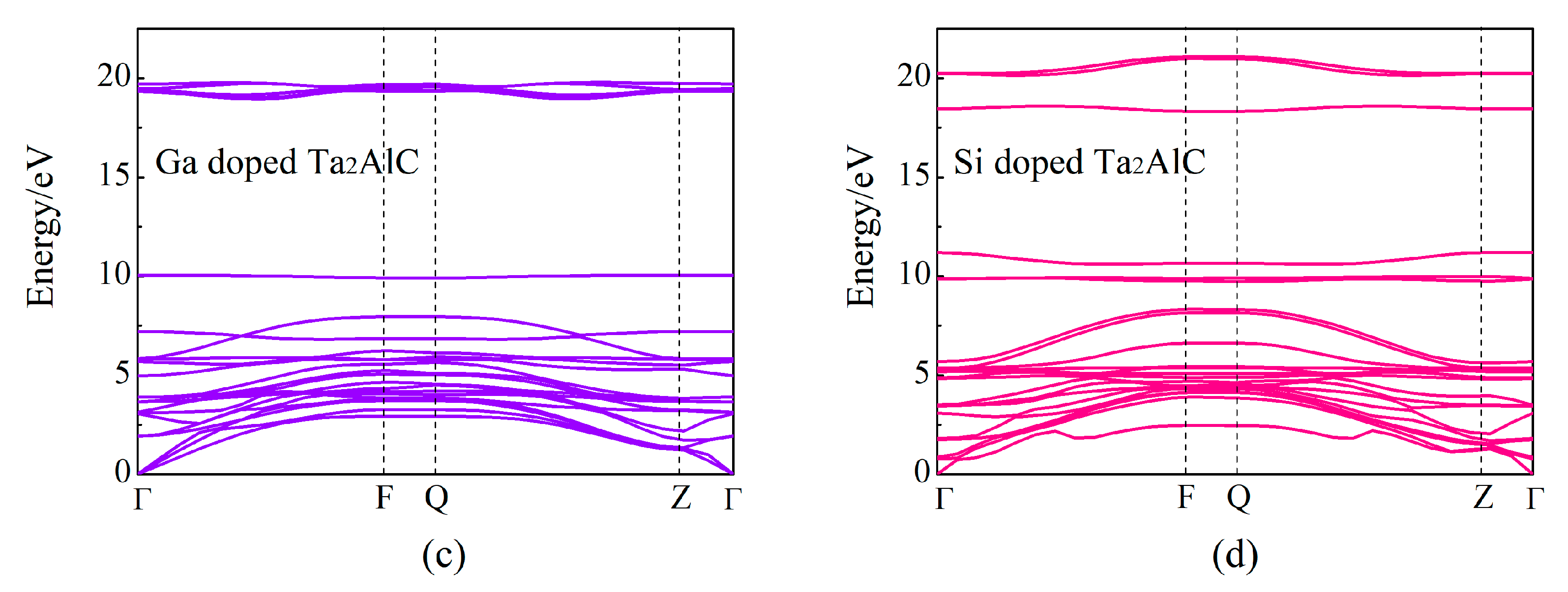
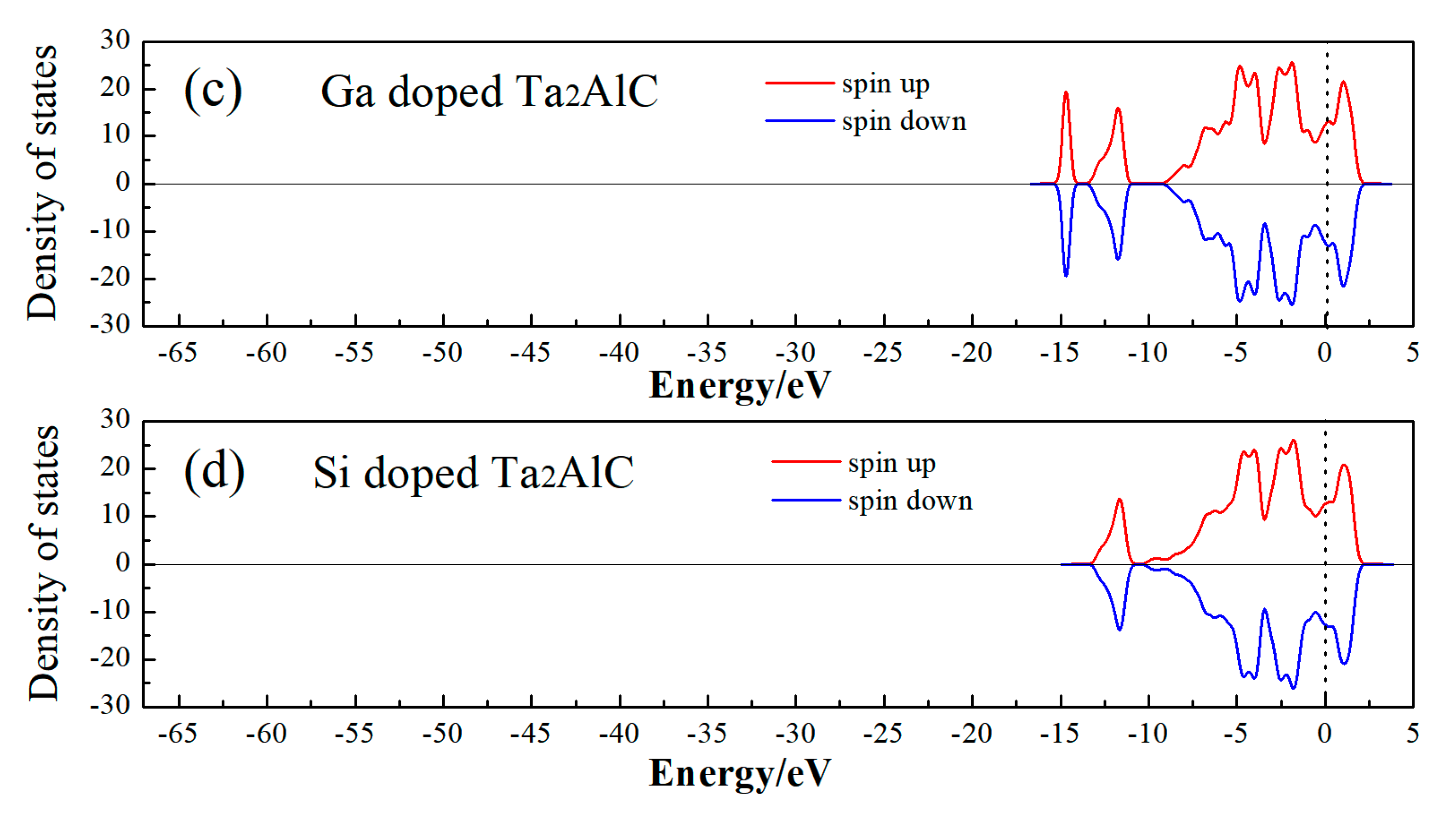
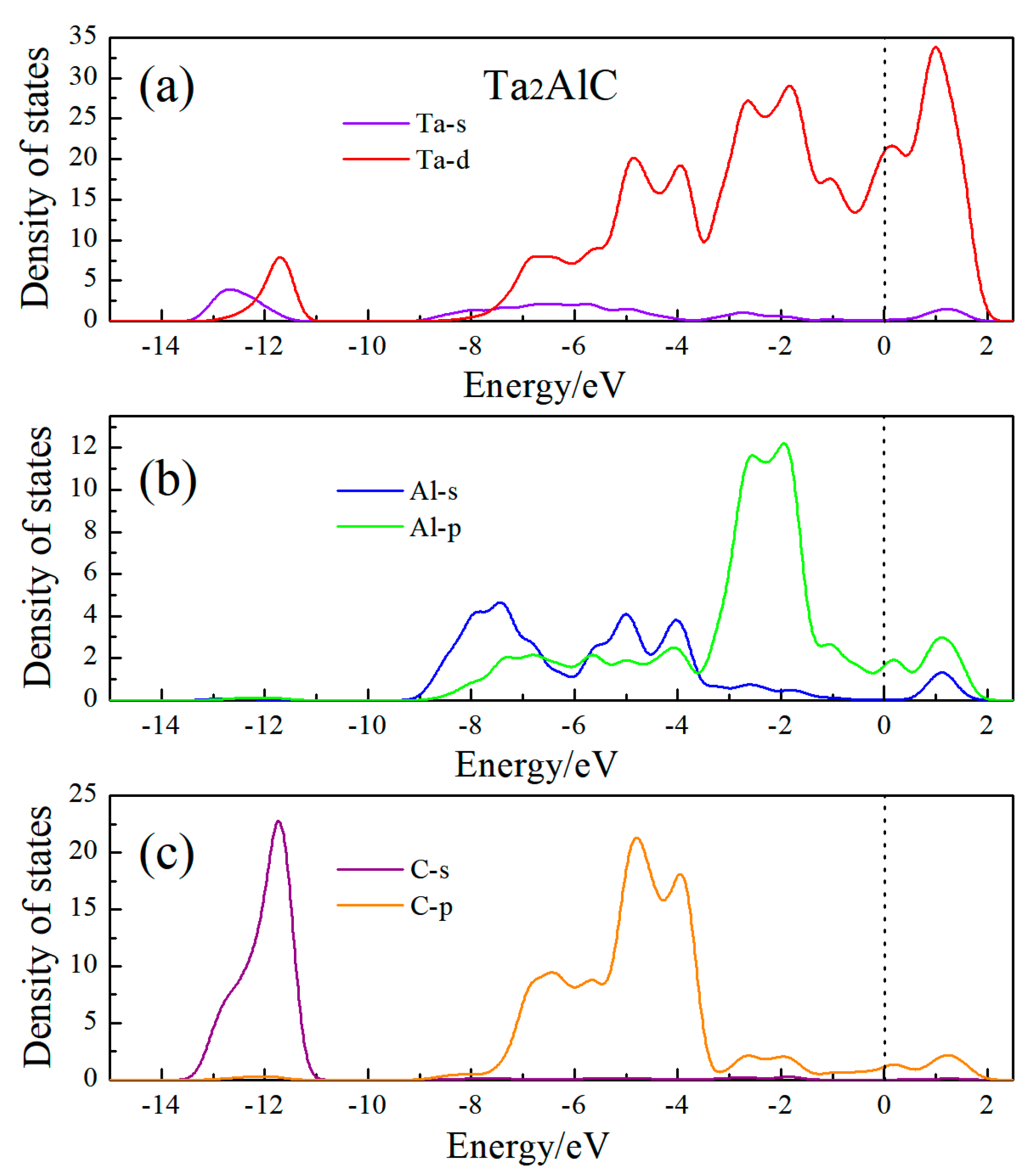
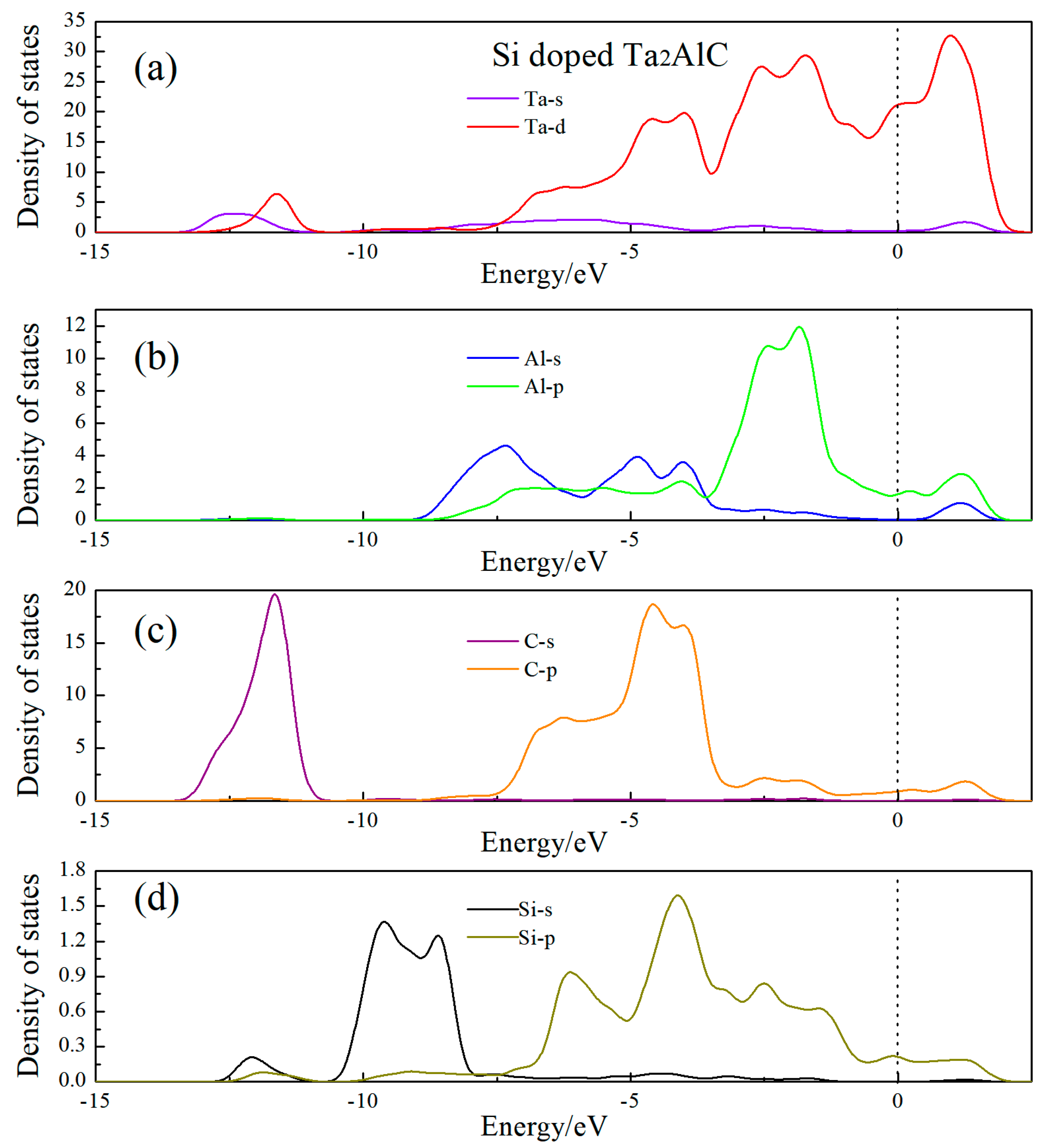
3.3. Electronic Structure
3.4. Mulliken Population Analysis
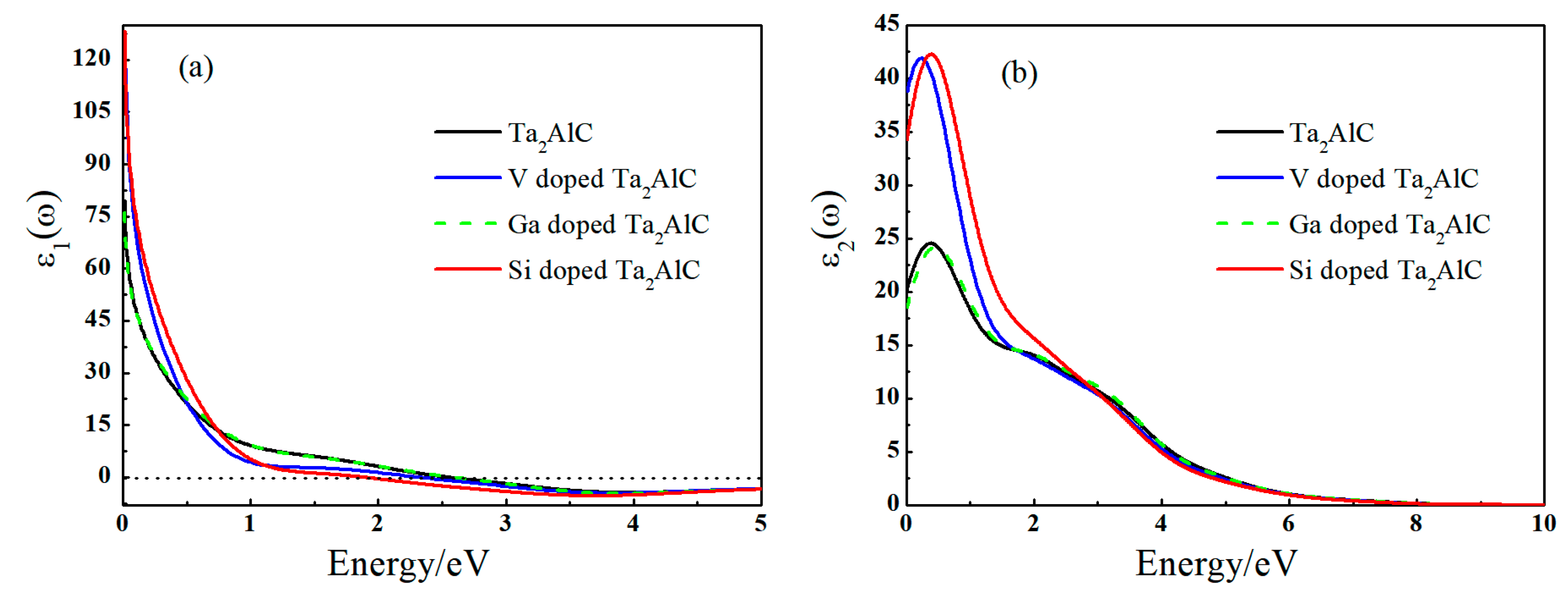
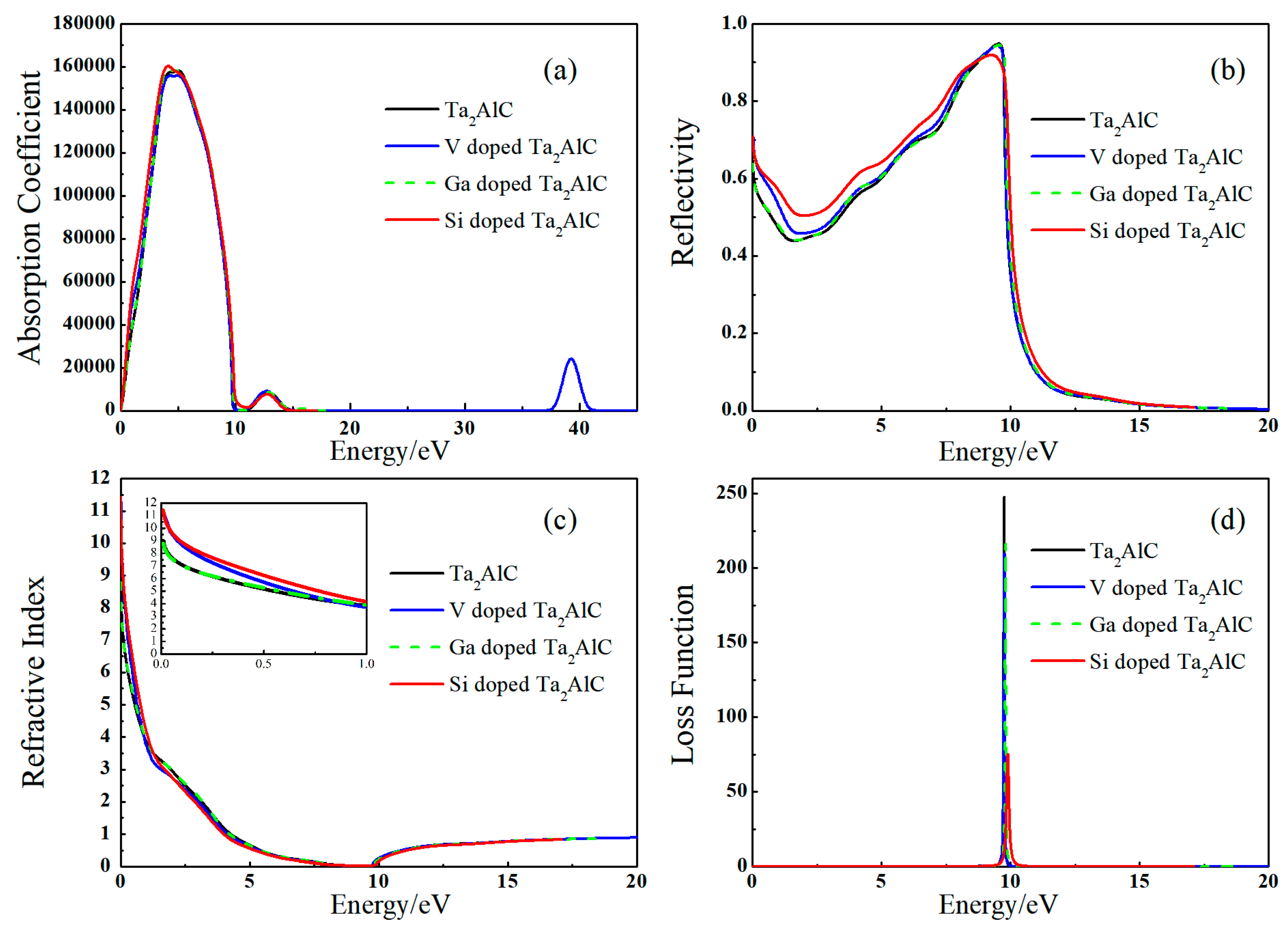
3.5. Optical Properties
4. Conclusions
- (1)
- The structural parameters of Ta2AlC undergo significant changes after doping with V, Ga, and Si. The variations in lattice parameters are attributed to the differences in covalent radii of the substituted atoms. The mechanical stability of bulk Ta2AlC and Ta2AlC doped with V, Ga, and Si were confirmed using Born’s criterion.
- (2)
- The mechanical properties analysis of doped Ta2AlC reveal that V and Si doping weaken the material’s resistance to deformation while enhancing its plasticity. In contrast, Ga doping increases the material’s resistance to lateral deformation and brittleness. The elastic anisotropy indices (AU, Ashear, A1, A2, and A3) of Ta2AlC increase after V, Ga, and Si doping, indicating an enhanced degree of anisotropy.
- (3)
- The electronic structure calculations demonstrate that Ta2AlC is a conductor with excellent electrical conductivity, which is not diminished by V, Ga, or Si doping. The symmetric distribution of spin-up and spin-down electronic states density confirms that the Ta2AlC system remains non-magnetic after doping. The partial density of states diagrams successfully elucidate the mechanisms by which dopant atoms influence the band structure and electronic states density, providing results consistent with the analysis of the material’s lattice parameters and mechanical properties.
- (4)
- The Mulliken population analysis indicates that V and Ga doping enhance the covalent interactions between C-Ta and Al-Ta atoms, while Si doping weakens these interactions.
- (5)
- The optical properties calculations reveal that V and Si doping significantly enhance the electromagnetic energy storage capacity and dielectric loss of Ta2AlC, whereas Ga doping has almost no effect. The reflectivity of Ta2AlC in the ultraviolet region peaks at 95% for the undoped material and decreases to 94%, 94%, and 92% after V, Ga, and Si doping, respectively, indicating that both doped and undoped Ta2AlC can serve as potential anti-ultraviolet coating materials. In the visible light region, doped and undoped Ta2AlC exhibit a similar metallic gray appearance, suggesting their potential as temperature control coating materials in the visible light region. The light loss of Ta2AlC is confined to a narrow energy range, unaffected by doping, indicating its suitability as a light storage material.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Barsoum, M.W. The Mn+1AXn Phases: A New Class of Solids; Thermodynamically Stable Nanolaminates. Prog. Solid State Chem. 2000, 28, 201–281. [Google Scholar] [CrossRef]
- Barsoum, M.W.; El-Raghy, T. The MAX Phases: Unique New Carbide and Nitride Materials. Am. Sci. 2001, 89, 334–343. [Google Scholar] [CrossRef]
- Ganguly, A.; Zhen, T.; Barsoum, M.W. Synthesis and Mechanical Properties of Ti3GeC2 and Ti3(SixGe1−x)C2 (x = 0.5, 0.75) Solid Solutions. J. Alloys Compd. 2004, 376, 287–295. [Google Scholar] [CrossRef]
- Hwang, S.S.; Lee, S.C.; Han, J.H.; Lee, D.; Park, S.-W. Machinability of Ti3SiC2 with Layered Structure Synthesized by Hot Pressing Mixture of TiCx and Si Powder. J. Eur. Ceram. Soc. 2012, 32, 3493–3500. [Google Scholar] [CrossRef]
- Barsoum, M.W.; Salama, I.; El-Raghy, T.; Golczewski, J.; Seifert, H.J.; Aldinger, F.; Porter, W.D.; Wang, H. Thermal and Electrical Properties of Nb2AlC, (Ti,Nb)2AlC and Ti2AlC. Metall. Mater. Trans. A 2002, 33, 2775–2779. [Google Scholar] [CrossRef]
- Wang, X.H.; Zhou, Y.C. Solid-Liquid Reaction Synthesis and Simultaneous Densification of Polycrystalline Ti2AlC. Z. Met. 2002, 93, 66–71. [Google Scholar] [CrossRef]
- Wang, X.H.; Zhou, Y.C. Intermediate-Temperature Oxidation Behavior of Ti2AlC in Air. J. Mater. Res. 2002, 17, 2974–2981. [Google Scholar] [CrossRef]
- Wang, X.H.; Zhou, Y.C. High-Temperature Oxidation Behavior of Ti2AlC in Air. Oxid. Met. 2003, 59, 303–320. [Google Scholar] [CrossRef]
- Sundberg, M.; Malmqvist, G.; Magnusson, A.; El-Raghy, T. Alumina Forming High Temperature Silicides and Carbides. Ceram. Int. 2004, 30, 1899–1904. [Google Scholar] [CrossRef]
- Bouhemadou, A.; Khenata, R. Prediction Study of Structural and Elastic Properties under the Pressure Effect of M2GaC (M = Ti, V, Nb, Ta). J. Appl. Phys. 2007, 102, 043528. [Google Scholar]
- Ying, G.B.; He, X.D.; Li, M.W.; Han, W.; He, F.; Du, S. Synthesis and Mechanical Properties of High-Purity Cr2AlC Ceramic. Mater. Sci. Eng. A 2011, 528, 2635–2640. [Google Scholar] [CrossRef]
- Barsoum, M.W.; El-Raghy, T.; Ogbuji, L.U.J.T. Oxidation of Ti3SiC2 in Air. J. Electrochem. Soc. 1997, 144, 2508–2516. [Google Scholar] [CrossRef]
- Su, X.J.; Liu, G.X.; Dai, B.; Wan, D.; Bao, Y.; Feng, Q.; Grasso, S.; Hu, C. Ablation Mechanisms of Ti3SiC2 Ceramic at 1600 °C in Nitrogen Plasma Flame. Ceram. Int. 2022, 48, 14004–14013. [Google Scholar]
- Ding, W.; Hu, B.T.; Fu, S.; Wan, D.; Bao, Y.; Feng, Q.; Grasso, S.; Hu, C. Ultra-Fast Thermal Shock Evaluation of Ti2AlC Ceramic. Materials 2022, 15, 6877. [Google Scholar] [CrossRef]
- Su, X.J.; Hu, B.T.; Quan, Y.; Qin, Y.; Feng, Q.; Hu, C. Ablation Behavior and Mechanism of Bulk MoAlB Ceramic at 1670–2550 °C in Air Plasma Flame. J. Eur. Ceram. Soc. 2021, 41, 5474–5483. [Google Scholar]
- Gonzalez-Julian, J. Processing of MAX Phases: From Synthesis to Applications. J. Am. Ceram. Soc. 2021, 104, 659–690. [Google Scholar]
- Zhang, Q.Q.; Zhou, Y.C.; San, X.Y.; Li, W.; Bao, Y.; Feng, Q.; Grasso, S.; Hu, C. Zr2SeB and Hf2SeB: Two New MAB Phase Compounds with the Cr2AlC-Type MAX Phase (211 Phase) Crystal Structures. J. Adv. Ceram. 2022, 11, 1764–1776. [Google Scholar] [CrossRef]
- Jeitschko, W.; Nowotny, H.; Benesovsky, F. Carbon-Containing Ternary Compounds (H-Phase). Monatsh. Chem. 1963, 94, 672–676. [Google Scholar]
- Yeh, C.L.; Shen, Y.G. Effects of Al Content on Formation of Ta2AlC by Self-Propagating High-Temperature Synthesis. J. Alloys Compd. 2009, 482, 219–223. [Google Scholar] [CrossRef]
- Tian, B.N.; Ying, G.B.; Wang, P.J.; Wang, C.; Wu, Y.P. In-Situ Synthesis Mechanism of Nano-Layered Ternary Ta2AlC. J. Synth. Cryst. 2015, 44, 1773–1777. [Google Scholar]
- Manoun, B.; Gulve, R.P.; Saxena, S.K.; Gupta, S.; Barsoum, M.W.; Zha, C.S. Compression Behavior of M2AlC (M = Ti, V, Cr, Nb, and Ta) Phases to above 50 GPa. Phys. Rev. B 2006, 73, 024110. [Google Scholar] [CrossRef]
- Hu, C.F.; Zhang, J.; Bao, Y.W.; Wang, J.; Li, M.; Zhou, Y. In-Situ Reaction Synthesis and Decomposition of Ta2AlC. Int. J. Mater. Res. 2008, 99, 8–13. [Google Scholar] [CrossRef]
- Lin, Z.J.; Zhuo, M.J.; Zhou, Y.C.; Li, M.; Wang, J. Microstructures and Theoretical Bulk Modulus of Layered Ternary Tantalum Aluminum Carbides. J. Am. Ceram. Soc. 2006, 89, 3765–3769. [Google Scholar]
- Qian, X.K.; Li, Y.B.; He, X.D.; Fan, H.; Yun, S. First-Principles Study of Structural and Electronic Properties of Ternary Layered Ta2AlC. J. Phys. Chem. Solids 2011, 72, 954–956. [Google Scholar] [CrossRef]
- Sun, Z.M.; Li, S.; Ahuja, R.; Schneider, J.M. Calculated Elastic Properties of M2AlC (M = Ti, V, Cr, Nb and Ta). Solid State Commun. 2004, 129, 589–592. [Google Scholar] [CrossRef]
- Music, D.; Sun, Z.M.; Voevodin, A.A.; Schneider, J.M. Electronic Structure and Shearing in Nanolaminated Ternary Carbides. Solid State Commun. 2006, 139, 139–143. [Google Scholar] [CrossRef]
- Guo, Z.Z. First-Principles Studies of the Effects of Vacancy Defects on the Properties of Ta2AlC. Phys. B Condens. Matter 2023, 668, 415160. [Google Scholar] [CrossRef]
- Lv, L.X.; Xu, W.W.; Zhu, C.C. First-Principles Study on the Electronic Structure and Optical Properties of Ta2AlC. J. Synth. Cryst. 2016, 45, 520–524. [Google Scholar]
- Hu, C.F.; He, L.F.; Zhang, J.; Bao, Y.; Wang, J.; Li, M.; Zhou, Y. Microstructure and Properties of Bulk Ta2AlC Ceramic Synthesized by an In Situ Reaction/Hot Pressing Method. J. Eur. Ceram. Soc. 2008, 28, 1679–1685. [Google Scholar] [CrossRef]
- Segall, M.D.; Lindan, P.J.D.; Probert, M.J.; Pickard, C.J.; Hasnip, P.J.; Clark, S.J.; Payne, M.C. First-Principles Simulation: Ideas, Illustrations and the CASTEP Code. J. Phys. Condens. Matter 2002, 14, 2717–2744. [Google Scholar] [CrossRef]
- Vanderbilt, D. Soft Self-Consistent Pseudopotentials in a Generalized Eigenvalue Formalism. Phys. Rev. B 1990, 41, 7892–7895. [Google Scholar] [CrossRef]
- Perdew, J.P.; Burke, K.; Ernzerhof, M. Generalized Gradient Approximation Made Simple. Phys. Rev. Lett. 1996, 77, 3865–3868. [Google Scholar] [CrossRef] [PubMed]
- Monkhorst, H.J.; Pack, J.D. Special Points for Brillouin-Zone Integrations. Phys. Rev. B 1976, 13, 5188–5192. [Google Scholar] [CrossRef]
- Xiao, B.; Feng, J.; Zhou, C.T.; Xing, J.; Xie, X.; Chen, Y. First Principles Study on the Electronic Structures and Stability of Cr7C3 Type Multi-Component Carbides. Chem. Phys. Lett. 2008, 459, 129–132. [Google Scholar] [CrossRef]
- Watt, J.P.; Peselnick, L. Clarification of the Hashin-Shtrikman Bounds on the Effective Elastic Moduli of Polycrystals with Hexagonal, Trigonal, and Tetragonal Symmetries. J. Appl. Phys. 1980, 51, 1525–1531. [Google Scholar] [CrossRef]
- Reuss, A. Berechnung der Fließgrenze von Mischkristallen auf Grund der Plastizitätsbedingung für Einkristalle. ZAMM 1929, 9, 49–58. [Google Scholar] [CrossRef]
- Hill, R. The Elastic Behaviour of a Crystalline Aggregate. Proc. Phys. Soc. A 1952, 65, 349–354. [Google Scholar] [CrossRef]
- Hadi, M.A.; Kelaidis, N.; Naqib, S.H. Mechanical Behaviors, Lattice Thermal Conductivity and Vibrational Properties of a New MAX Phase Lu2SnC. J. Phys. Chem. Solids 2019, 129, 162–171. [Google Scholar] [CrossRef]
- Chung, D.H.; Buessem, W.R.; Vahldiek, F.W.; Mersol, S.A. Anisotropy in Single Crystal Refractory Compounds; Plenum Press: New York, NY, USA, 1968. [Google Scholar]
- Huang, B.; Duan, Y.H.; Hu, W.C.; Sun, Y.; Chen, S. Structural, Anisotropic Elastic and Thermal Properties of MB (M = Ti, Zr and Hf) Monoborides. Ceram. Int. 2015, 41, 6831–6843. [Google Scholar] [CrossRef]
- Zou, M.T.; Bao, L.K.; Yang, A.C.; Duan, Y.; Peng, M.; Cao, Y.; Li, M. Structural, Elastic, Mechanical, Electronic, Damage Tolerance, Fracture Toughness, and Optical Properties of Cr-Al-B MAB Phases Studied by First-Principles Calculations. J. Mater. Res. 2023, 38, 1396–1409. [Google Scholar] [CrossRef]
- Li, P.; Ma, L.; Peng, M.; Shu, B.; Duan, Y. Elastic Anisotropies and Thermal Conductivities of WB2 Diborides in Different Crystal Structures: A First-Principles Calculation. J. Alloys Compd. 2018, 747, 905–915. [Google Scholar] [CrossRef]
- Maier, S.A. Plasmonics: Fundamentals and Applications; Springer: New York, NY, USA, 2007. [Google Scholar]




| Sample | a (nm) | b (nm) | c (nm) | V (nm3) | E (eV) | Eb (eV) |
|---|---|---|---|---|---|---|
| Ta2A1C | 0.6309 | 0.6309 | 2.7941 | 0.9633 | −7797.3365 | −8.3016 |
| V doped Ta2A1C | 0.6287 | 0.6287 | 2.7849 | 0.9534 | −11,475.9034 | −8.2683 |
| Ga doped Ta2A1C | 0.6315 | 0.6315 | 2.7883 | 0.9631 | −11,789.8840 | −8.2742 |
| Si doped Ta2A1C | 0.6377 | 0.6377 | 2.8050 | 0.9858 | −7696.9951 | −8.1058 |
| Sample | C11 | C12 | C13 | C33 | C44 | C66 |
|---|---|---|---|---|---|---|
| Ta2A1C | 348.7114 | 114.0028 | 128.6486 | 312.5064 | 164.5408 | 118.6462 |
| V doped Ta2A1C | 341.0590 | 114.4546 | 126.3630 | 299.7501 | 163.4924 | 115.7912 |
| Ga doped Ta2A1C | 335.5614 | 94.7569 | 118.5429 | 281.2559 | 164.4988 | 121.4161 |
| Si doped Ta2A1C | 299.7089 | 90.5178 | 121.9969 | 263.7427 | 152.3105 | 102.3365 |
| Sample | B | G | E | υ | B/G |
|---|---|---|---|---|---|
| Ta2A1C | 194.6353 | 129.2186 | 317.4124 | 0.2282 | 1.5062 |
| V doped Ta2A1C | 190.5174 | 126.2935 | 310.3121 | 0.2285 | 1.5085 |
| Ga doped Ta2A1C | 179.3631 | 127.7701 | 309.7579 | 0.2122 | 1.4038 |
| Si doped Ta2A1C | 170.2374 | 112.1657 | 275.9019 | 0.2299 | 1.5177 |
| Sample | AU | Acomp | Ashear | A1 | A2 | A3 |
|---|---|---|---|---|---|---|
| Ta2A1C | 0.2307 | 0.07% | 2.25% | 1.6294 | 1.6294 | 1.1749 |
| V doped Ta2A1C | 0.2652 | 0.14% | 2.57% | 1.6851 | 1.6851 | 1.1935 |
| Ga doped Ta2A1C | 0.2989 | 0.16% | 2.88% | 1.7328 | 1.7328 | 1.2790 |
| Si doped Ta2A1C | 0.4150 | 0.00% | 3.98% | 1.9071 | 1.9071 | 1.2814 |
| Sample | Bond | Population | Length (nm) |
|---|---|---|---|
| Ta2A1C | C-Ta | 0.44 | 0.22 |
| Al-Ta | 0.36 | 0.29 | |
| V doped Ta2A1C | C-Ta | 0.52 | 0.22 |
| Al-Ta | 0.39 | 0.28 | |
| V-C | 0.23 | 0.21 | |
| V-Al | 0.22 | 0.29 | |
| Ga doped Ta2A1C | C-Ta | 0.46 | 0.22 |
| Al-Ta | 0.39 | 0.29 | |
| Ga-Ta | −0.64 | 0.27 | |
| Si doped Ta2A1C | C-Ta | 0.43 | 0.23 |
| Al-Ta | 0.33 | 0.28 | |
| Si-Ta | 0.50 | 0.24 | |
| Ta-Ta | 0.12 | 0.30 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, Z.; Zhang, C.; Qin, X.; Yan, W. Research on the Mechanical and Photoelectric Properties Regulation of the New-Type Ceramic Material Ta2AlC. Crystals 2025, 15, 309. https://doi.org/10.3390/cryst15040309
Zhang Z, Zhang C, Qin X, Yan W. Research on the Mechanical and Photoelectric Properties Regulation of the New-Type Ceramic Material Ta2AlC. Crystals. 2025; 15(4):309. https://doi.org/10.3390/cryst15040309
Chicago/Turabian StyleZhang, Zhongzheng, Chunhong Zhang, Xinmao Qin, and Wanjun Yan. 2025. "Research on the Mechanical and Photoelectric Properties Regulation of the New-Type Ceramic Material Ta2AlC" Crystals 15, no. 4: 309. https://doi.org/10.3390/cryst15040309
APA StyleZhang, Z., Zhang, C., Qin, X., & Yan, W. (2025). Research on the Mechanical and Photoelectric Properties Regulation of the New-Type Ceramic Material Ta2AlC. Crystals, 15(4), 309. https://doi.org/10.3390/cryst15040309






