Liquid-Crystalline Supermolecules Inducing Layer Fluctuations: From Hierarchical to Dissipative Structures
Abstract
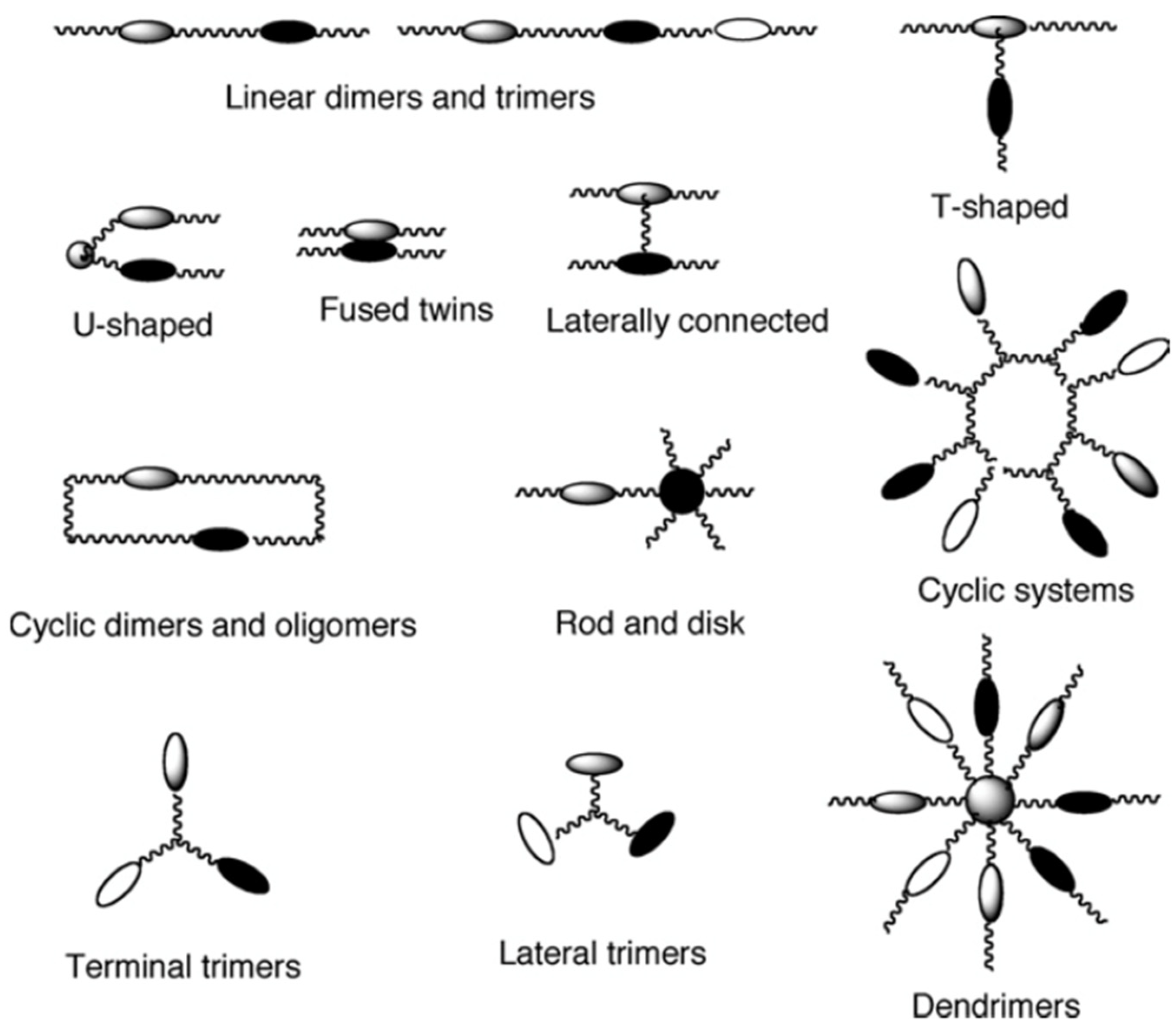
1. Introduction
2. Equilibrium Hierarchical Structures
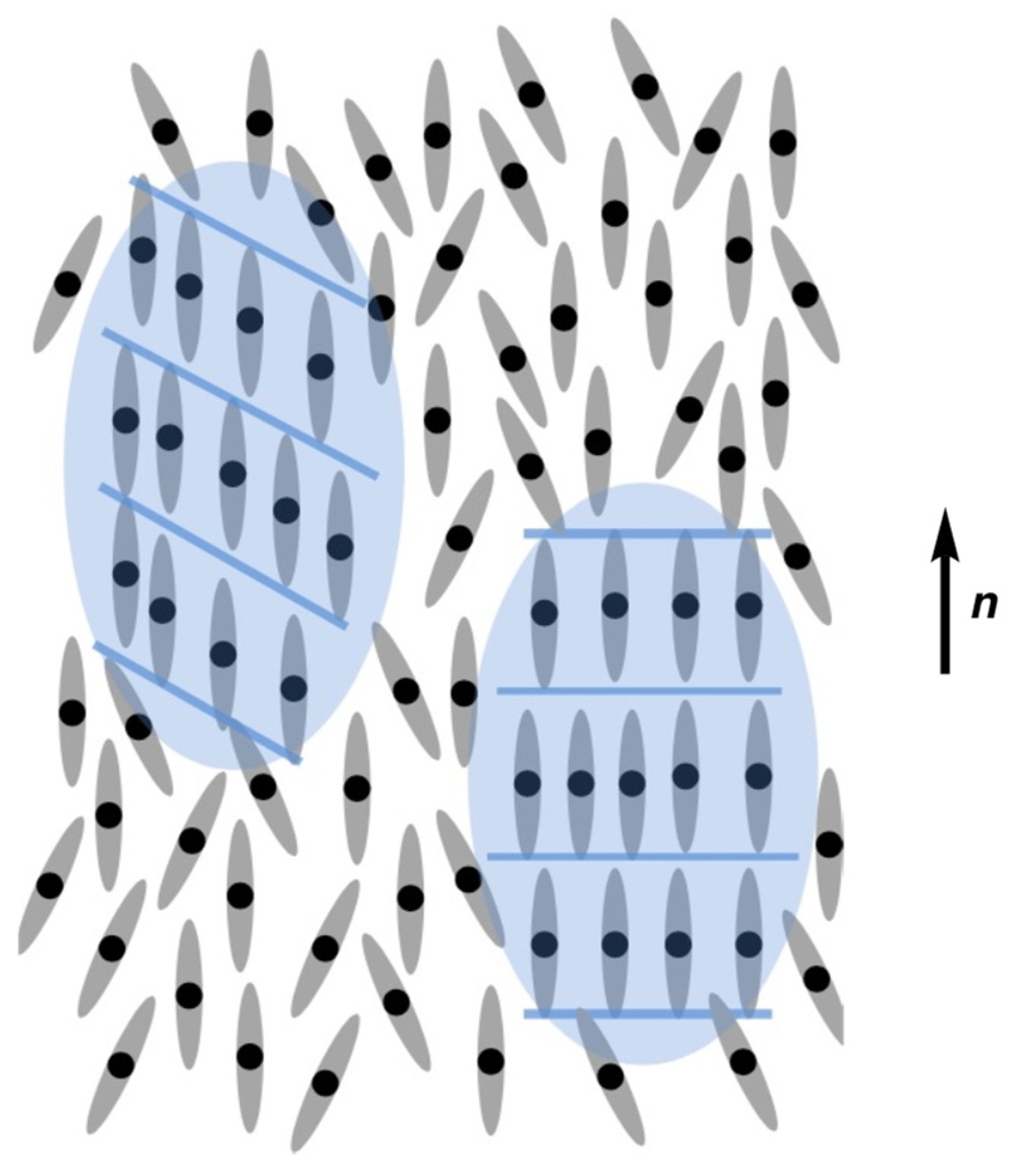
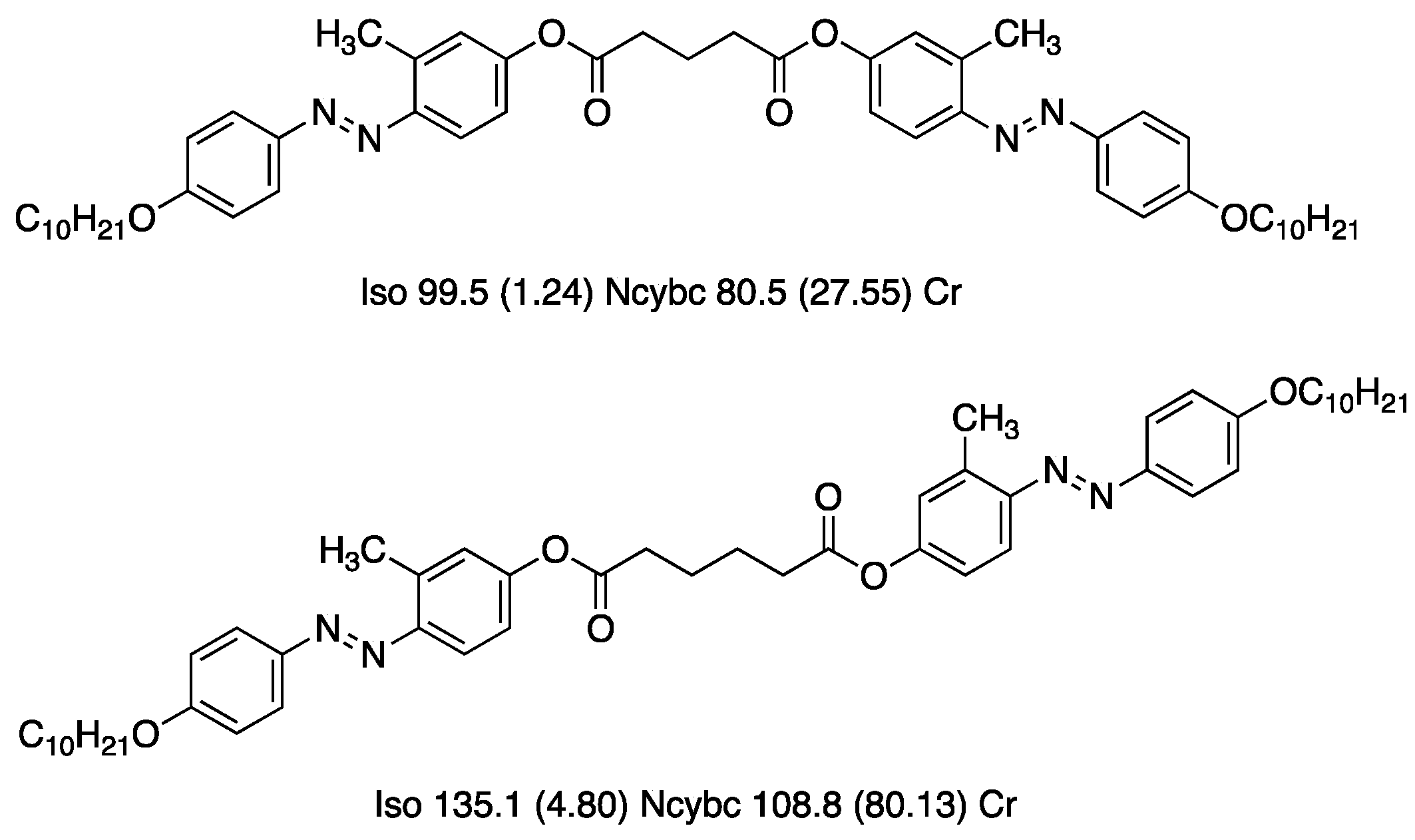
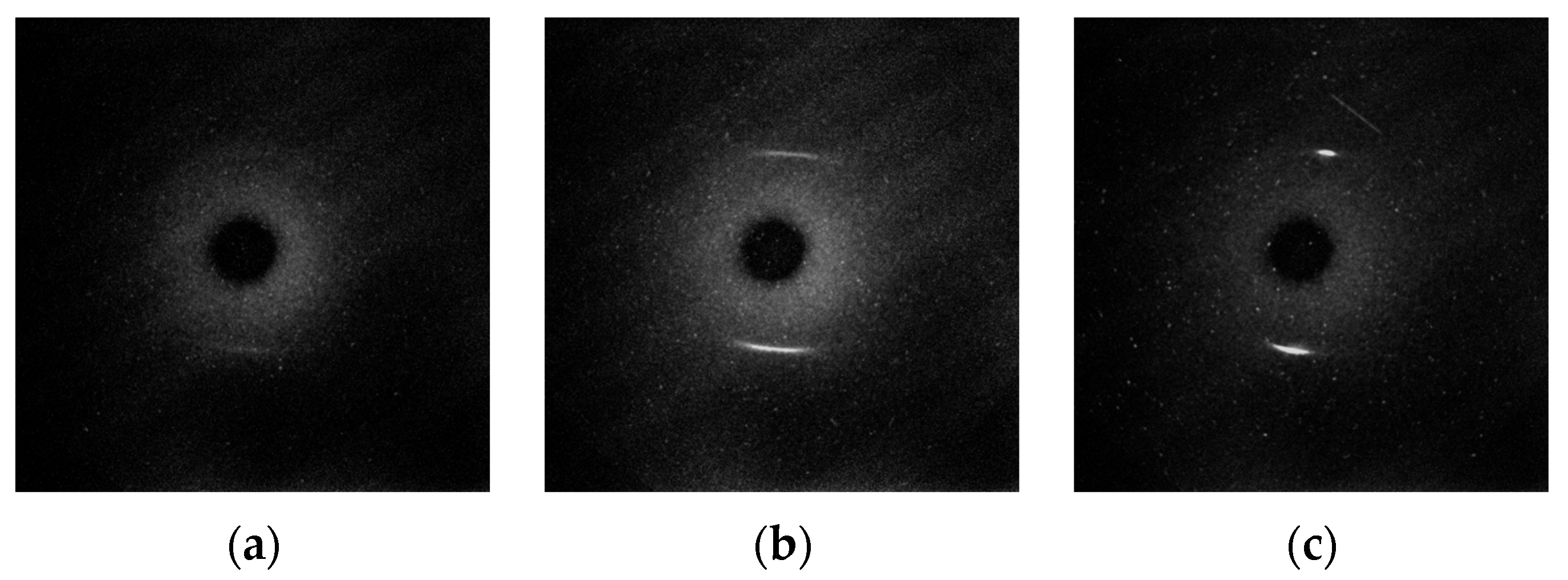
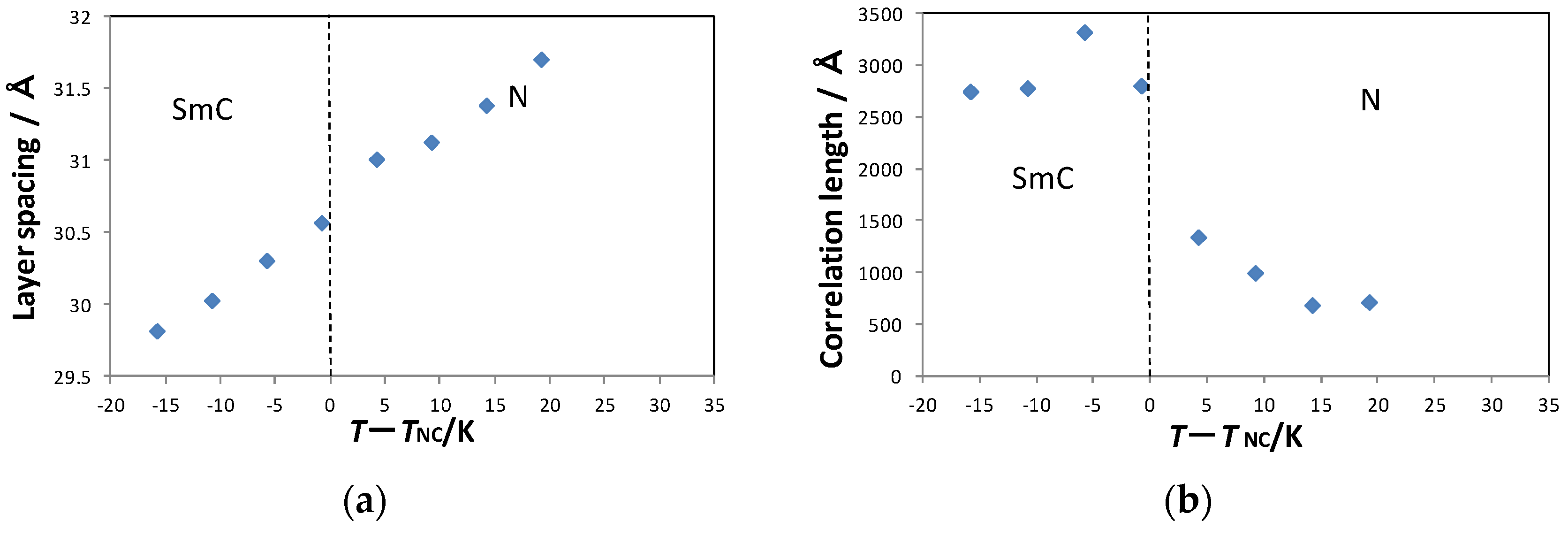
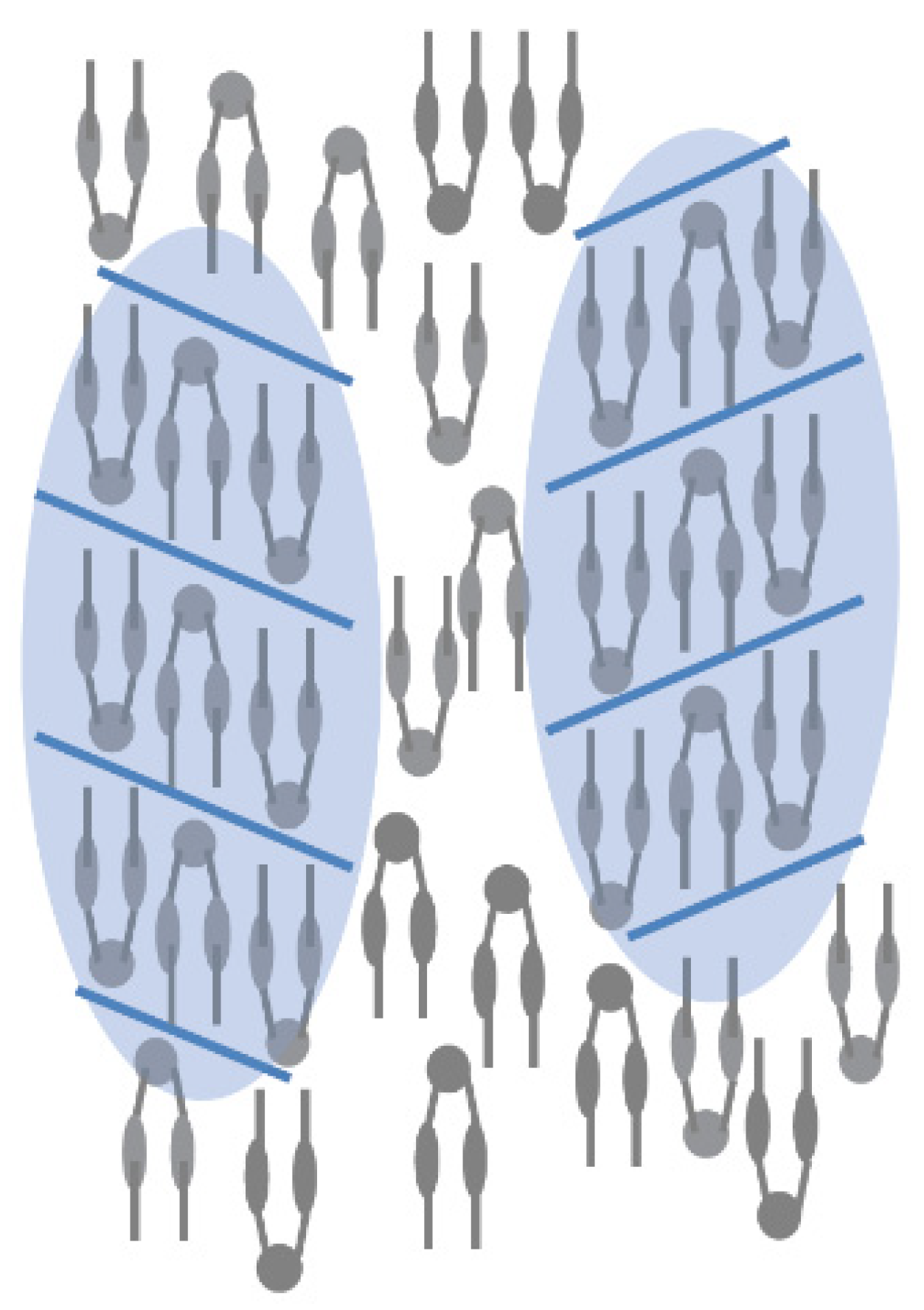
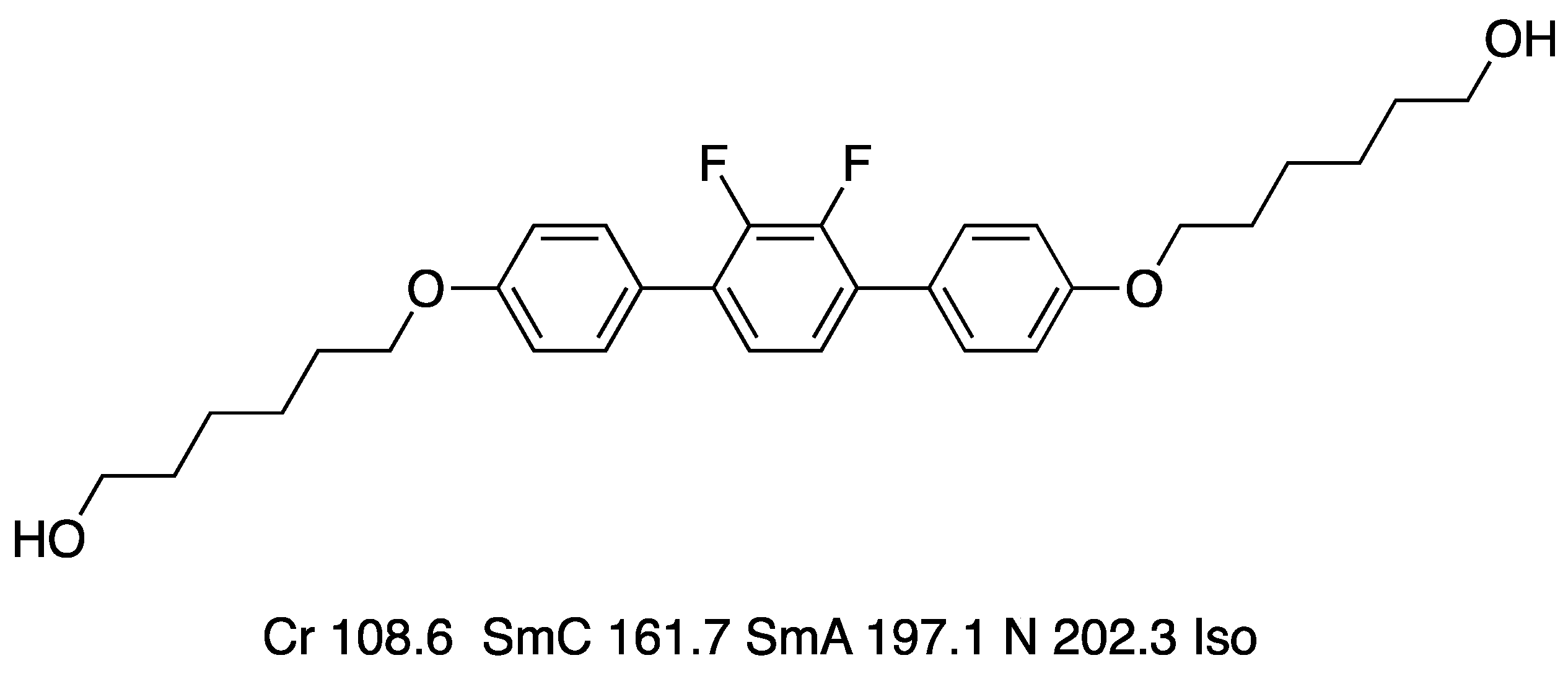
2.1. Cybotactic Nematic Phases
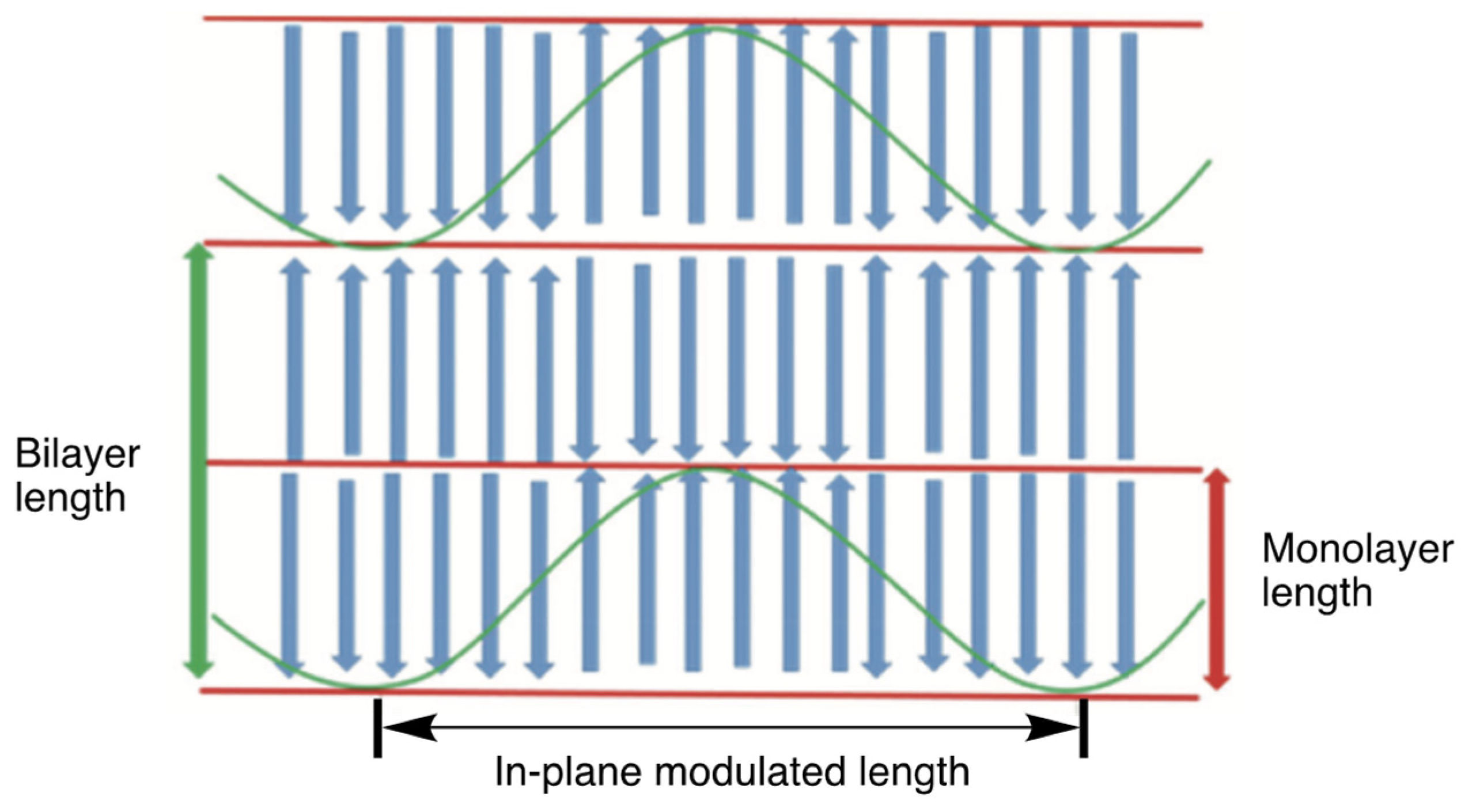
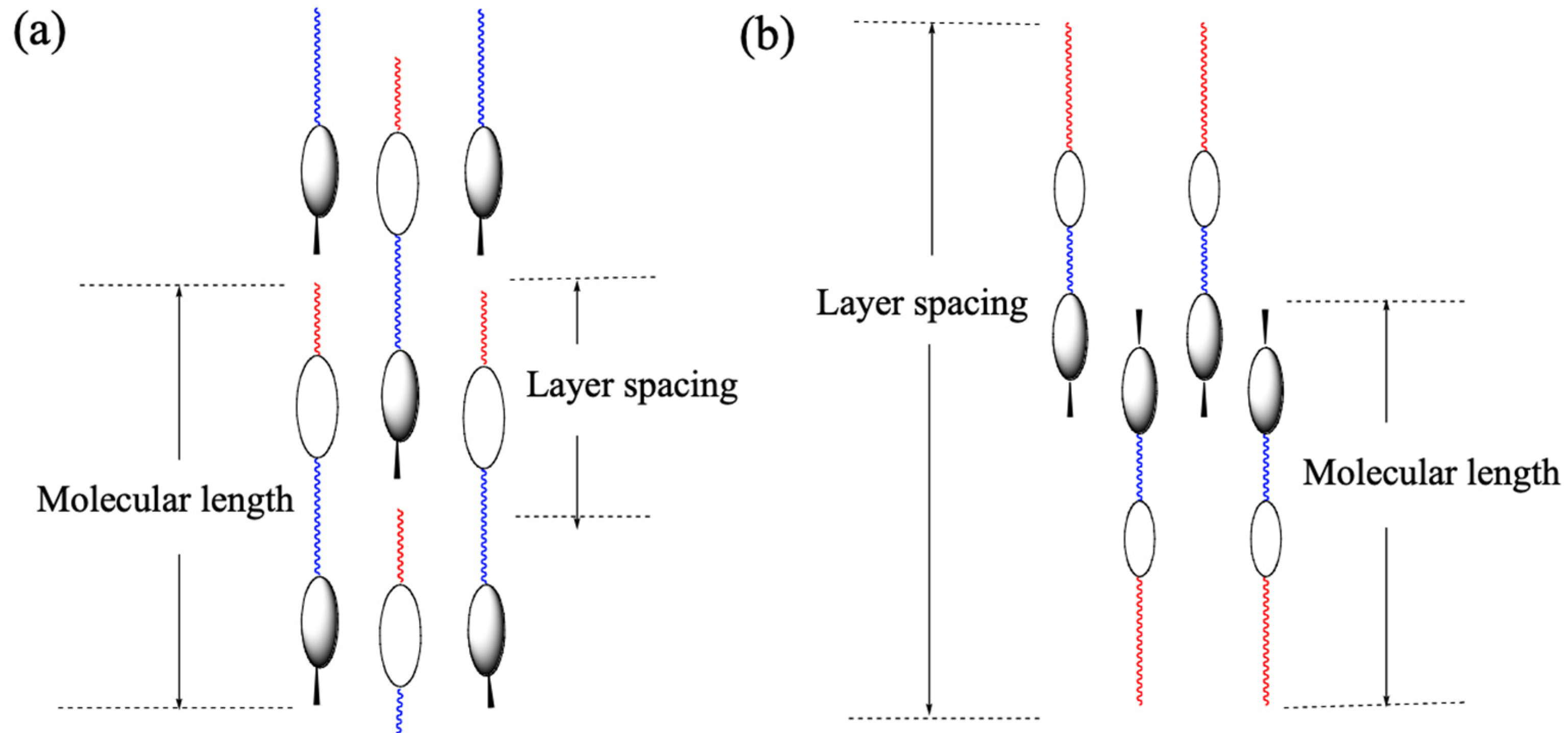
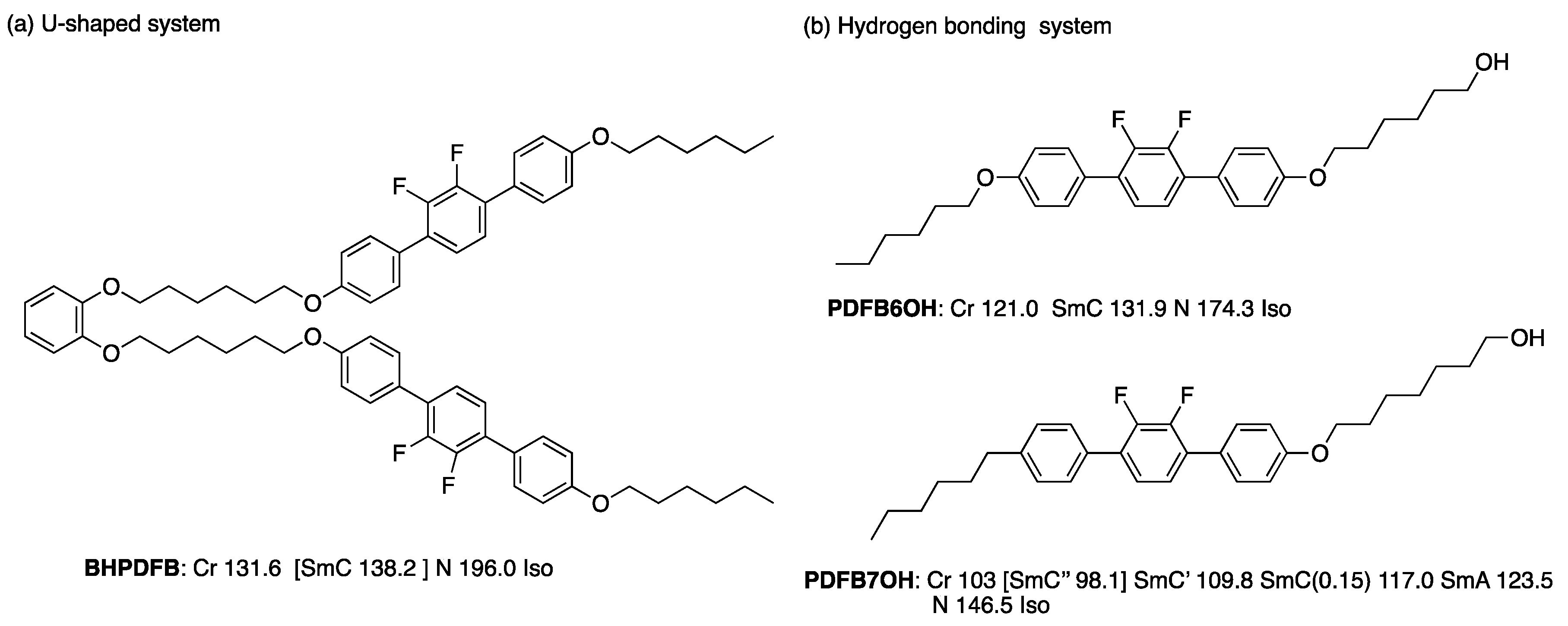
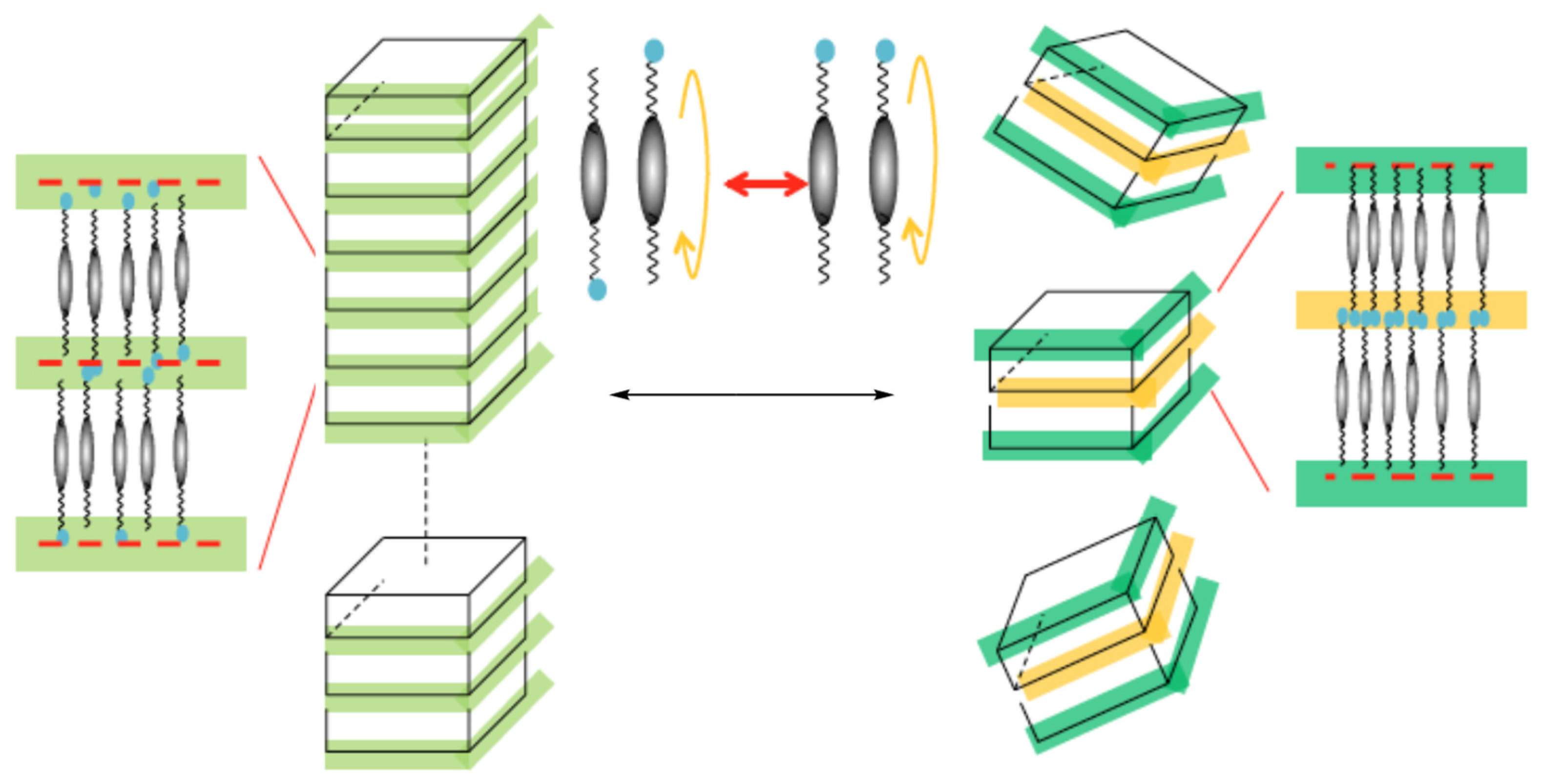
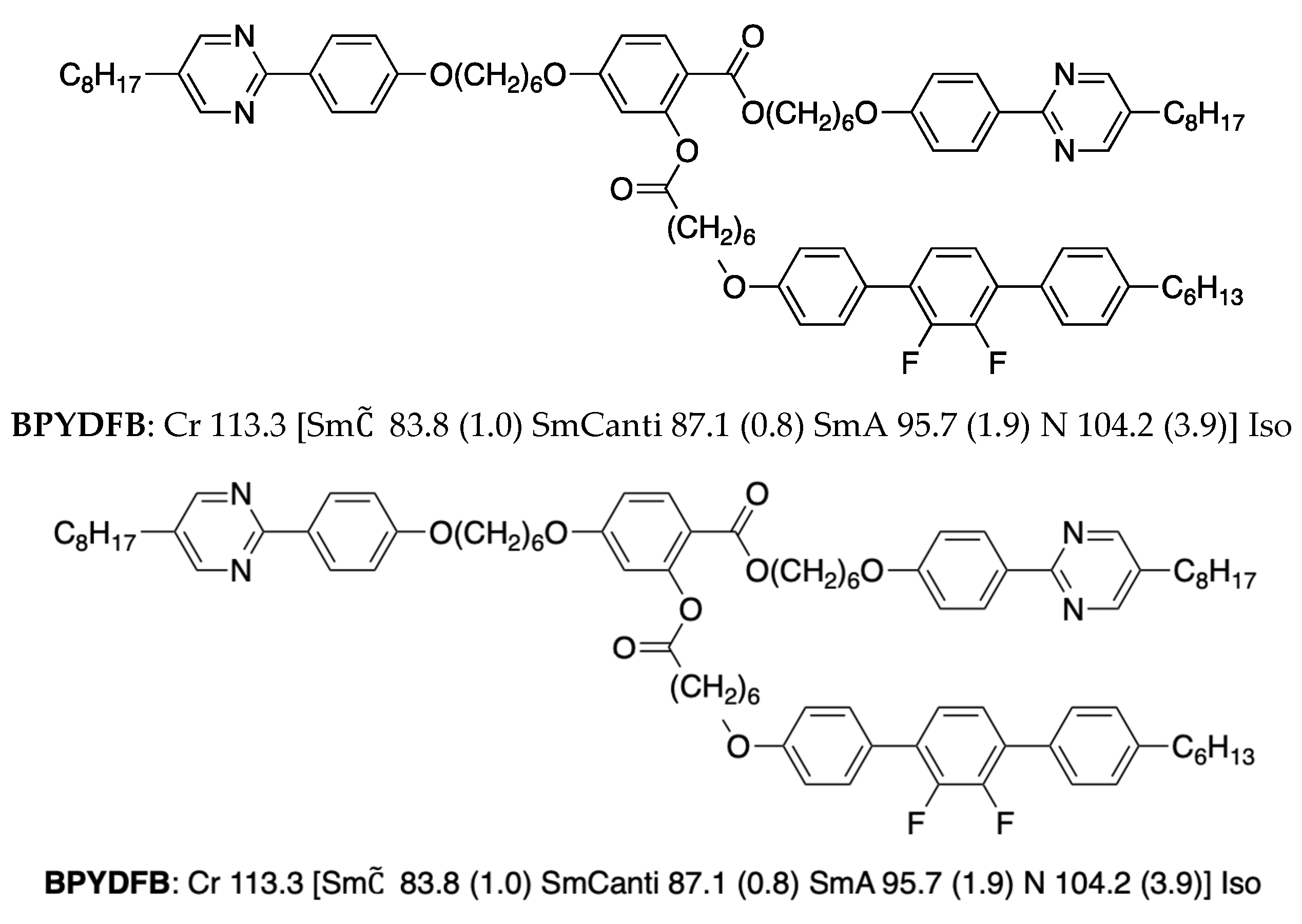
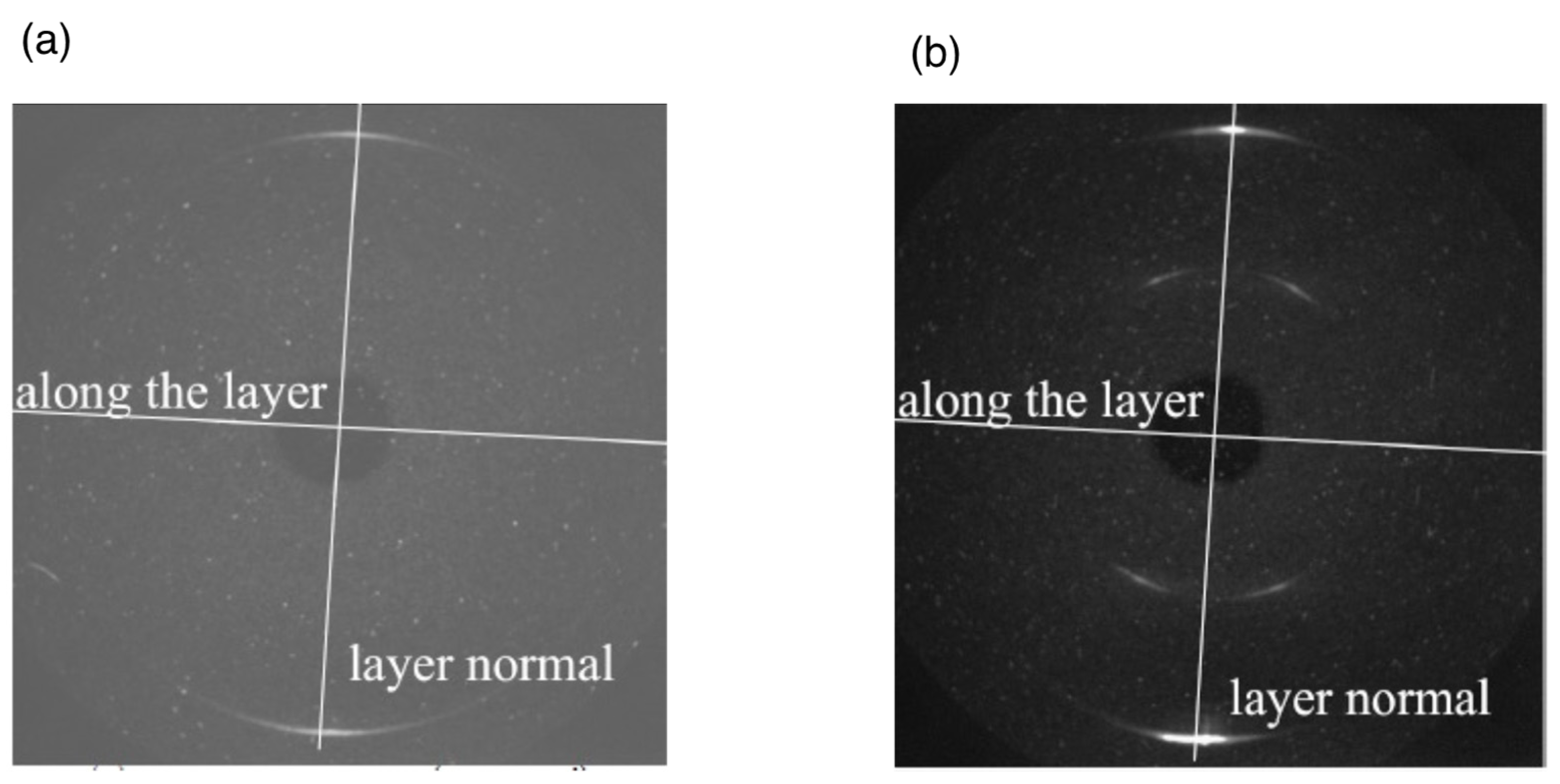
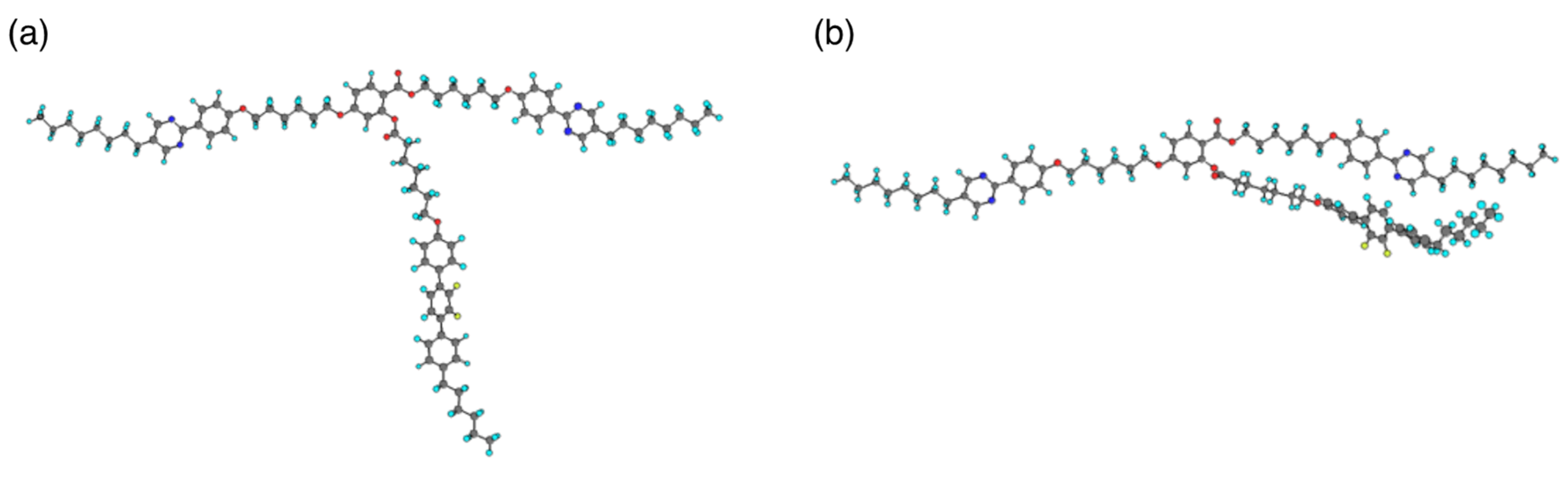
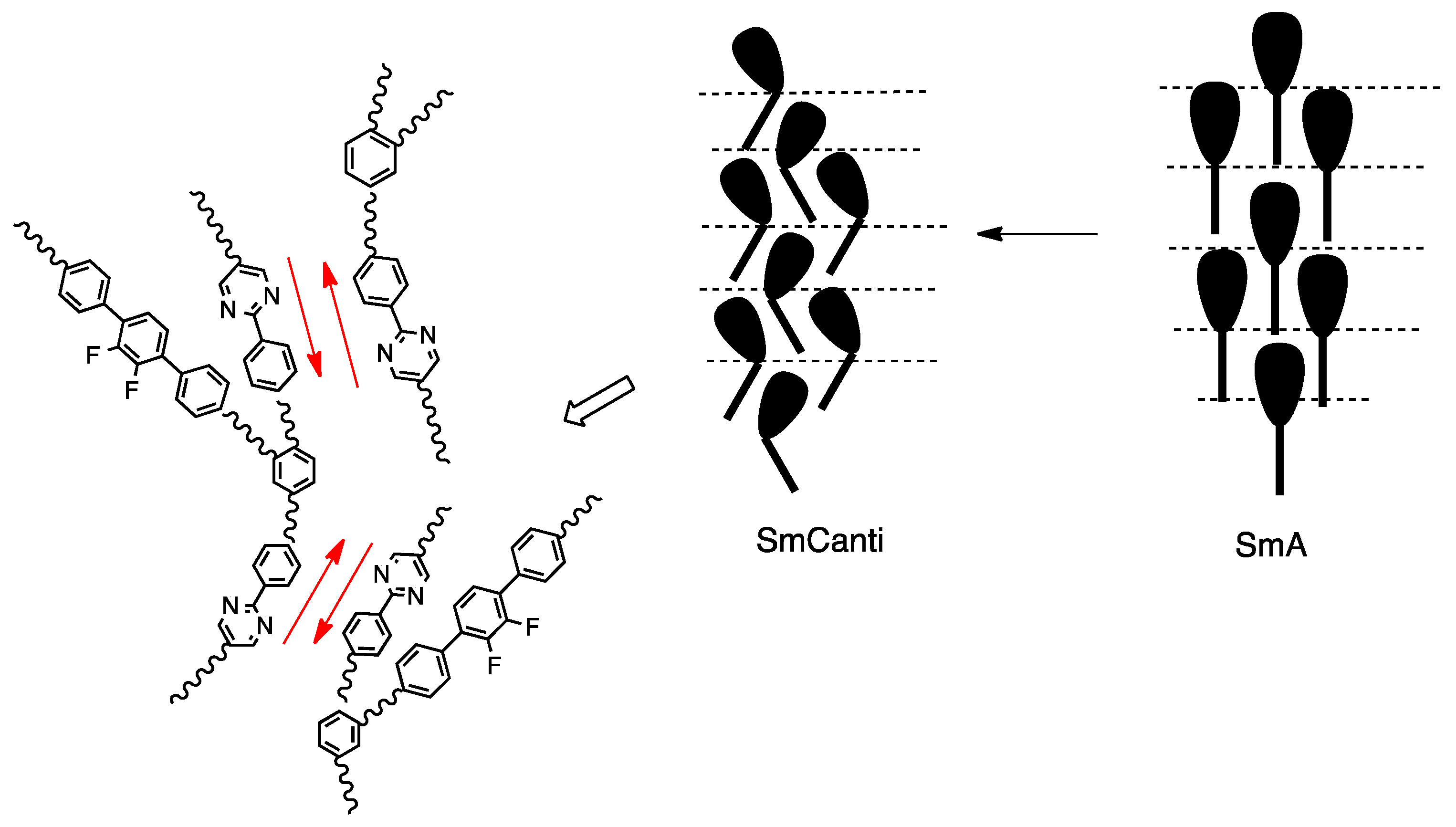
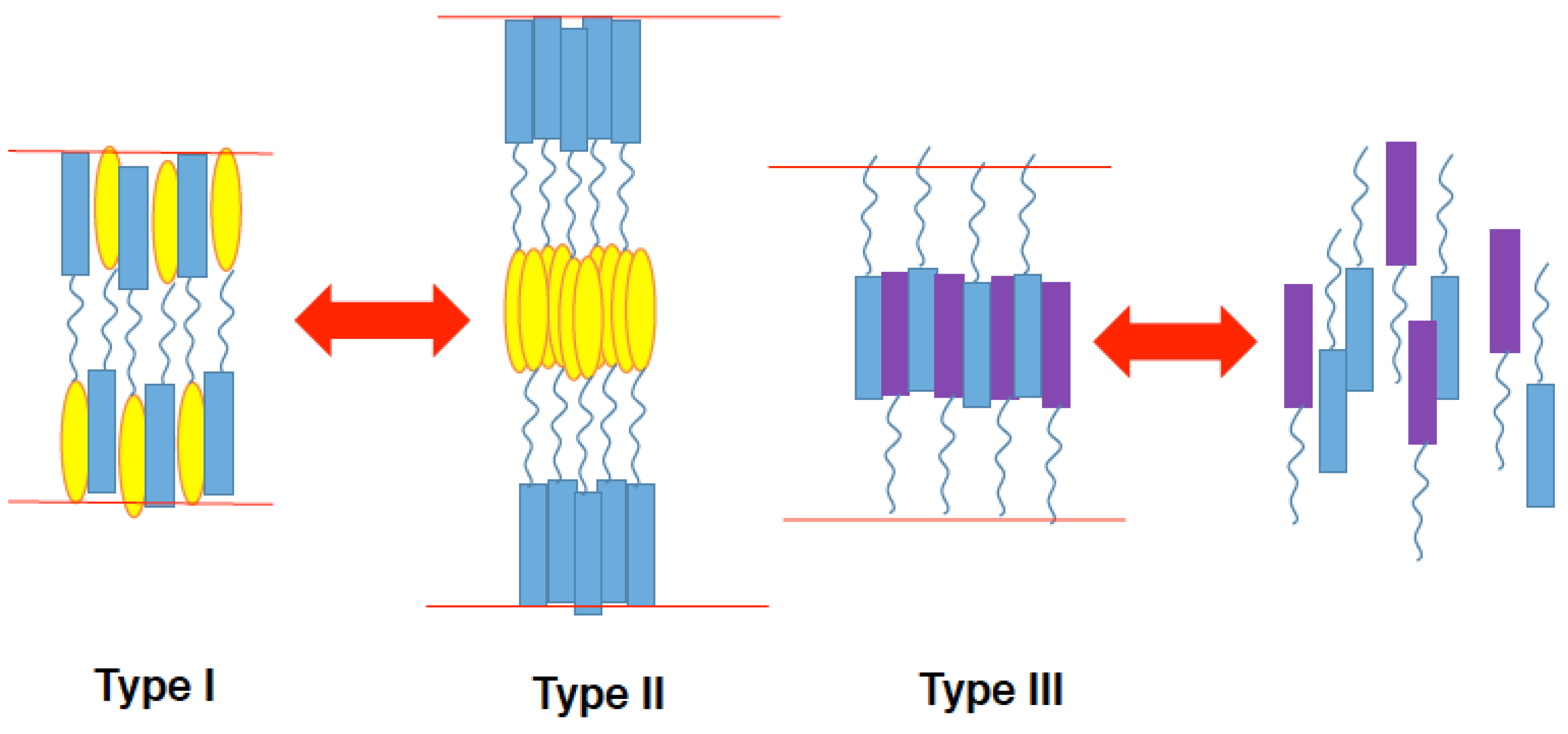
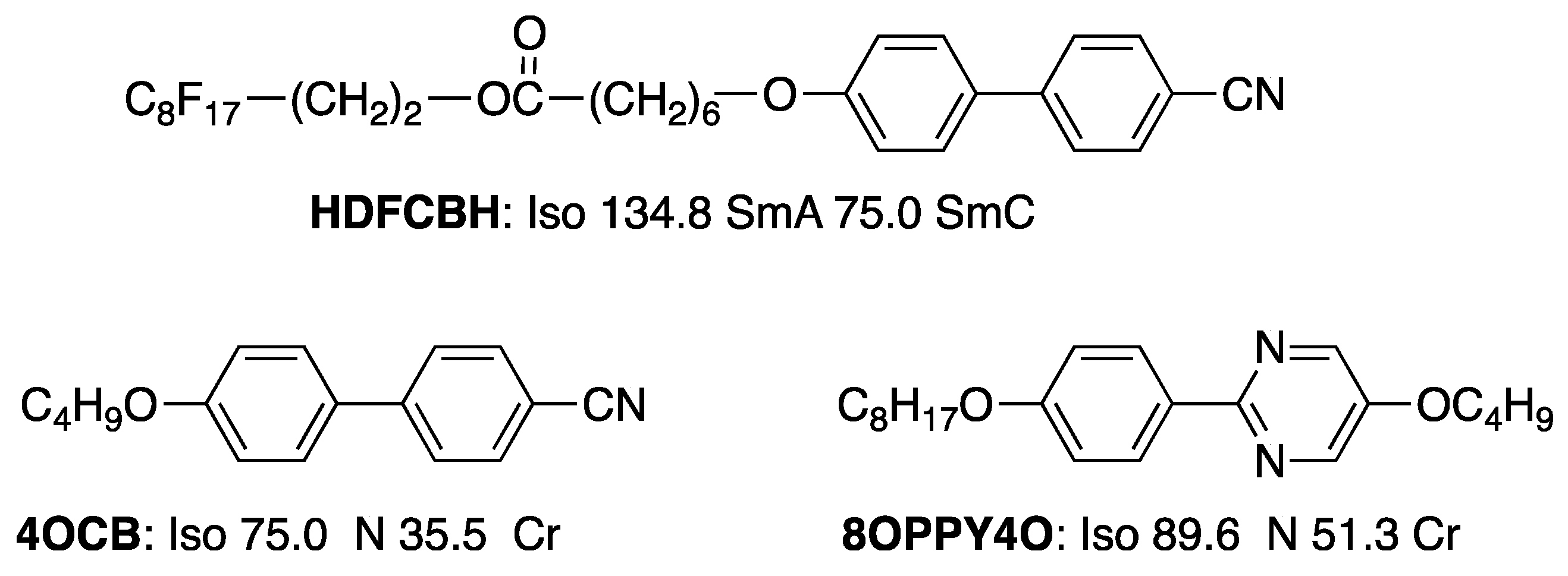
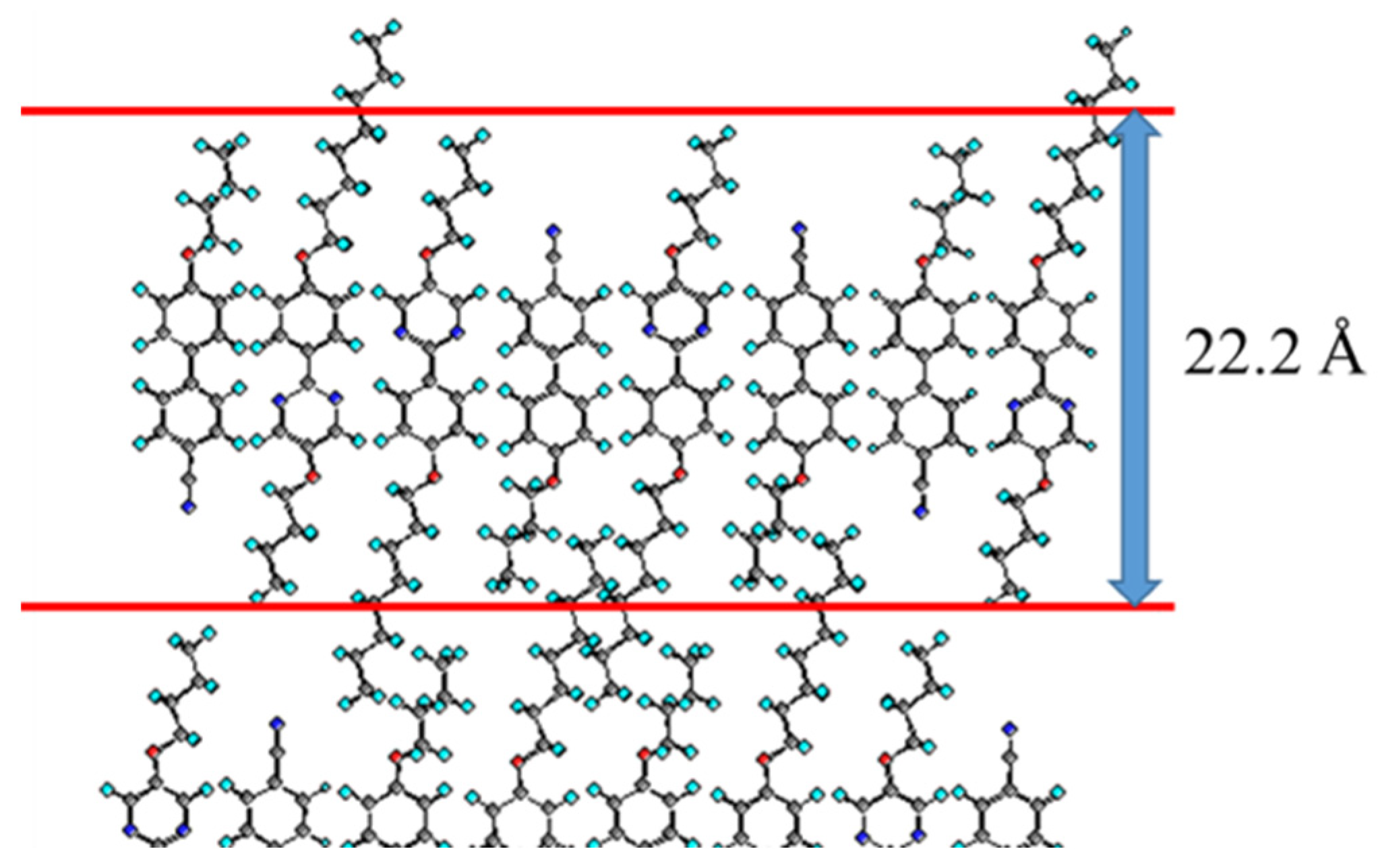
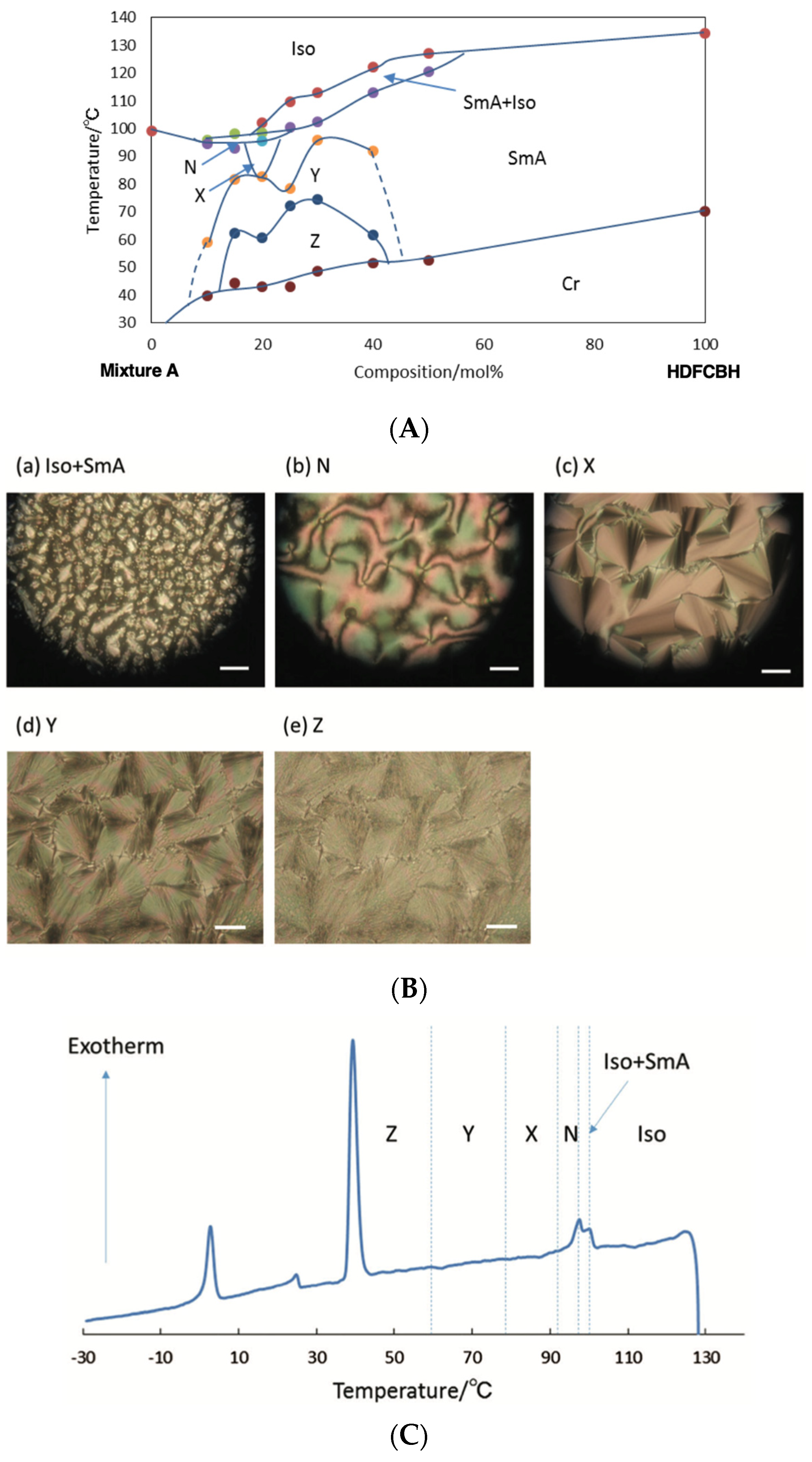
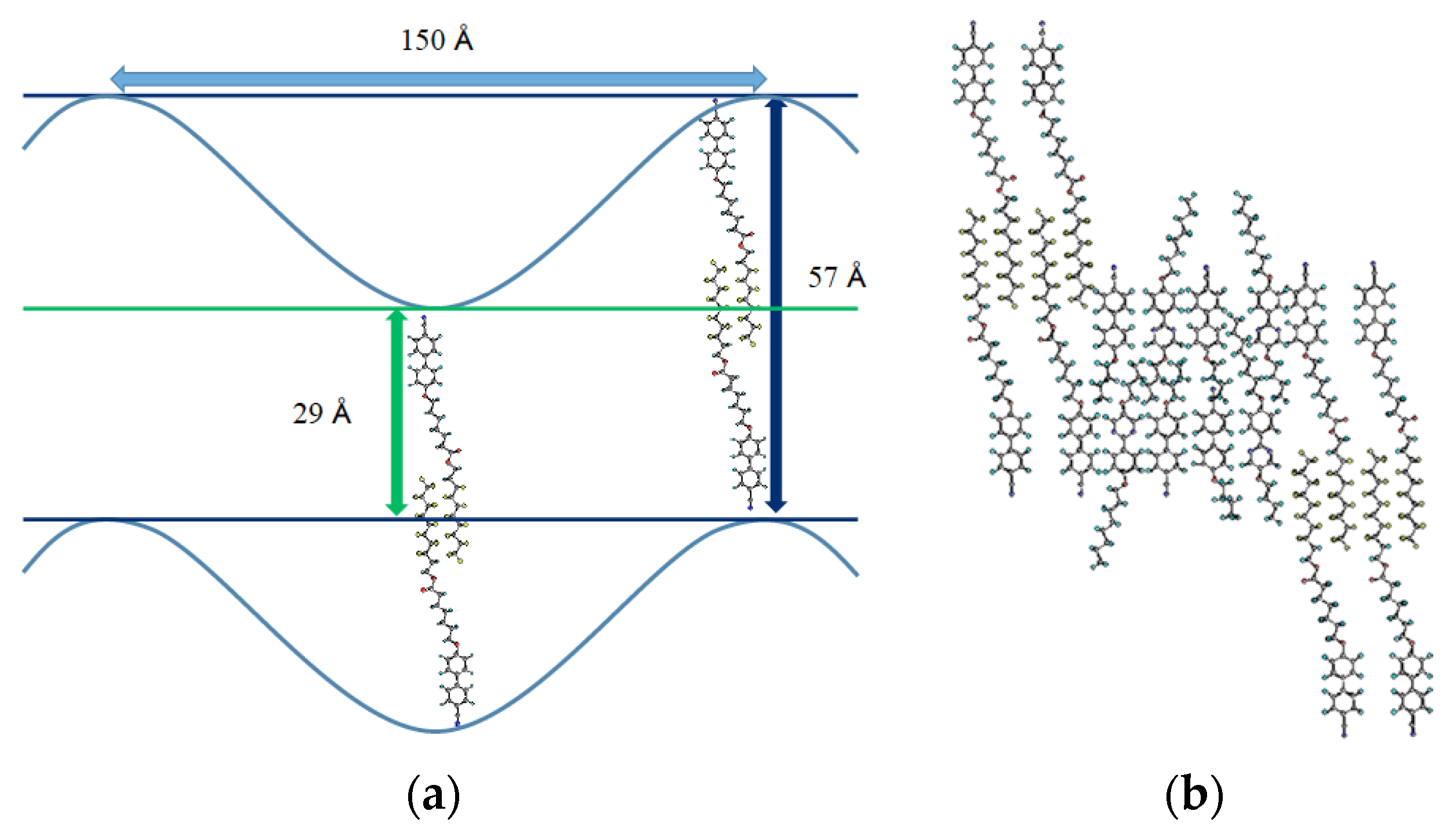
2.2. Modulated Smectic Phases
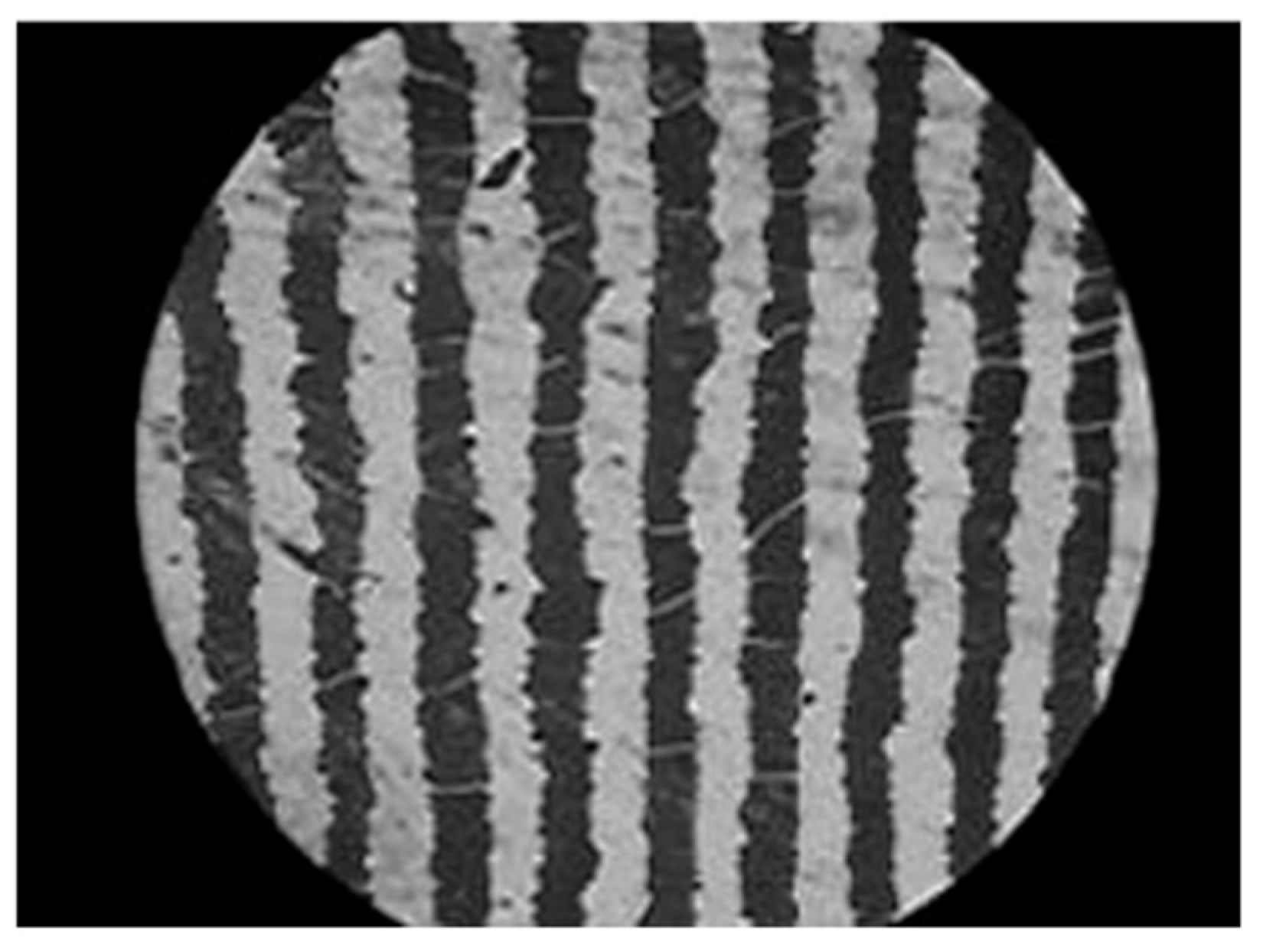
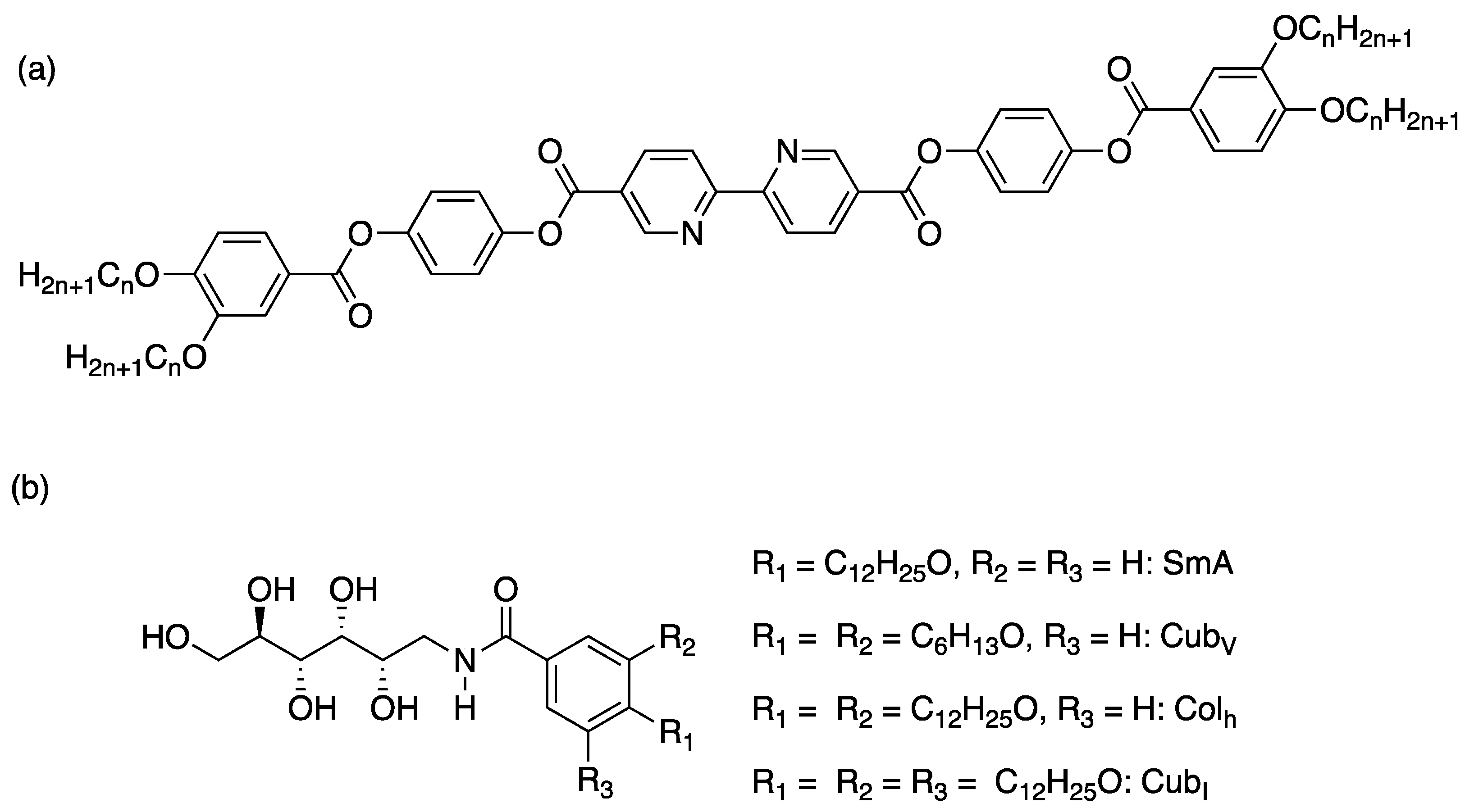
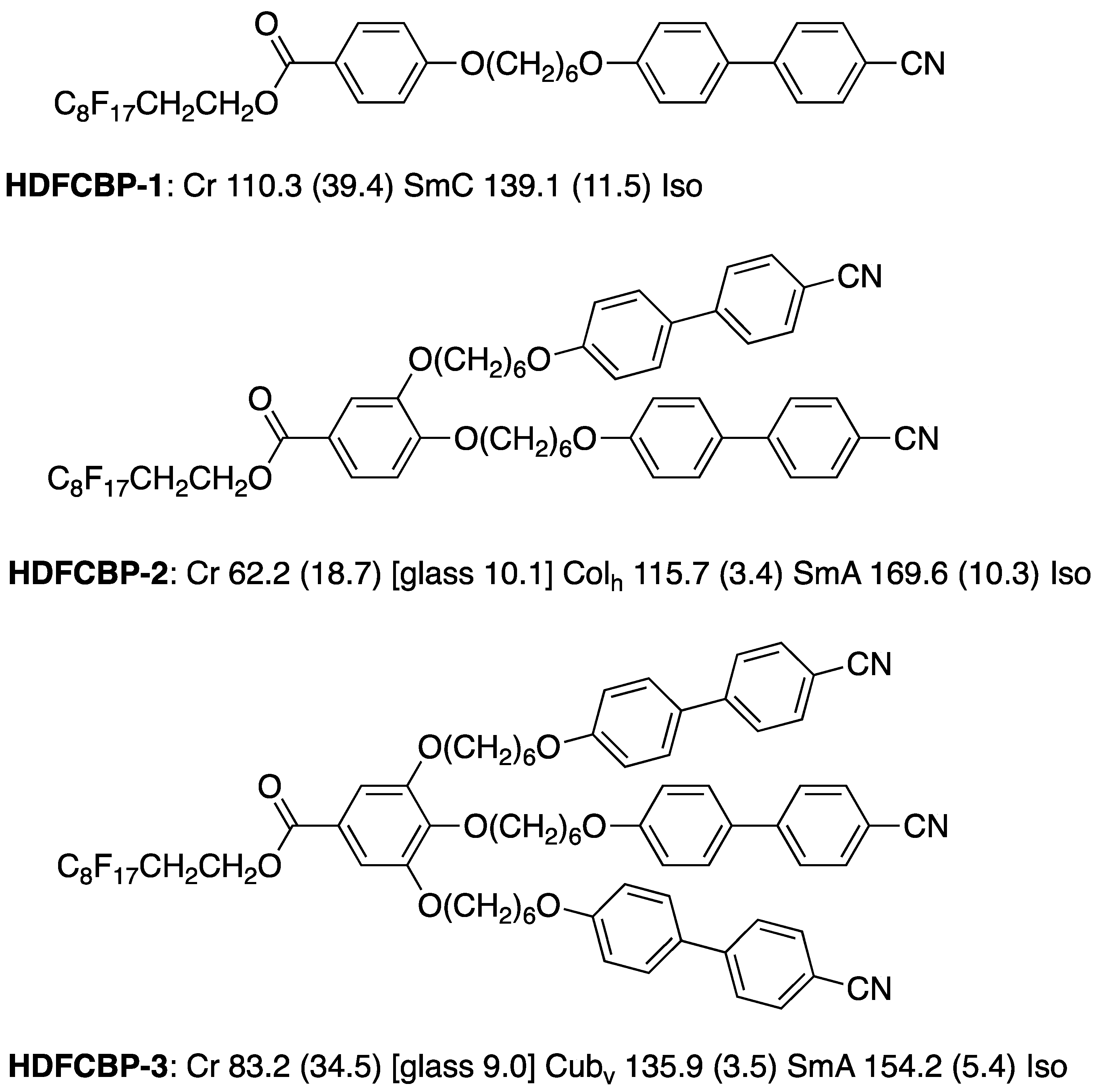
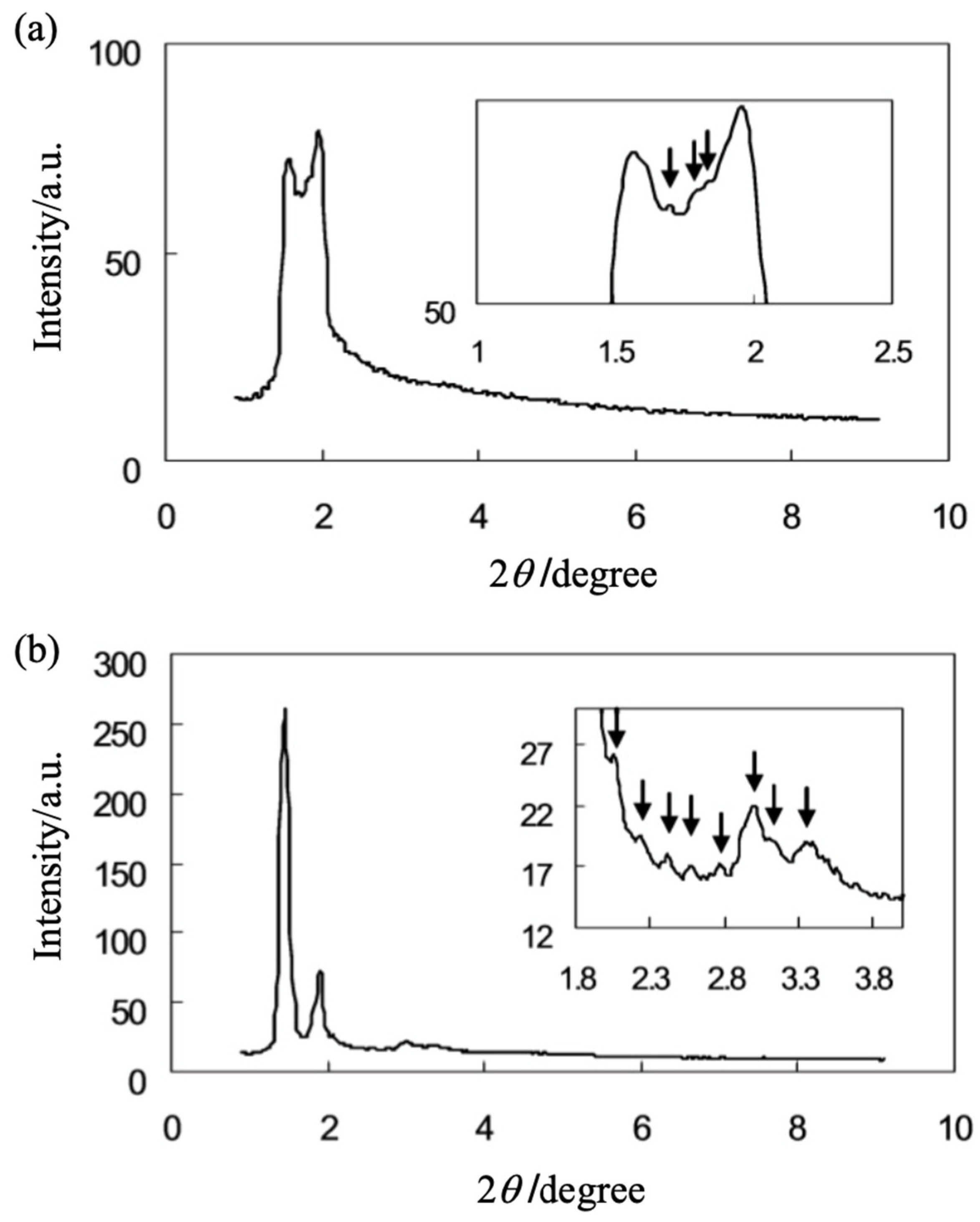
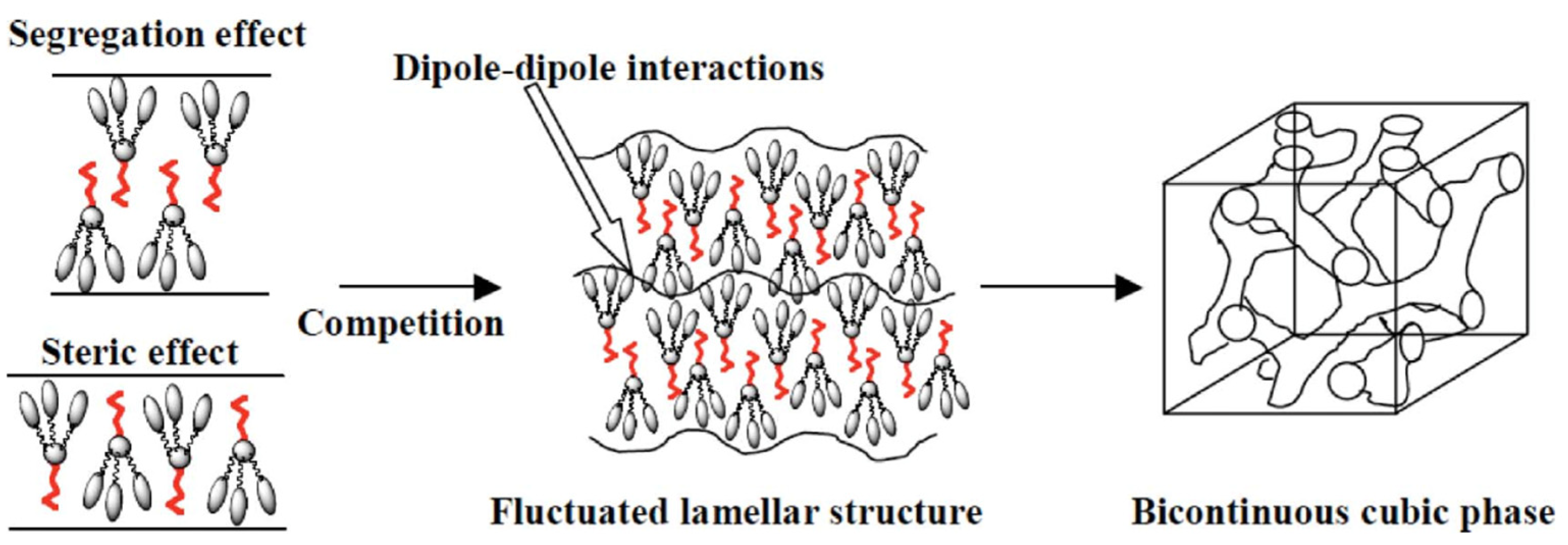
2.3. Bicontinuous Cubic Phases
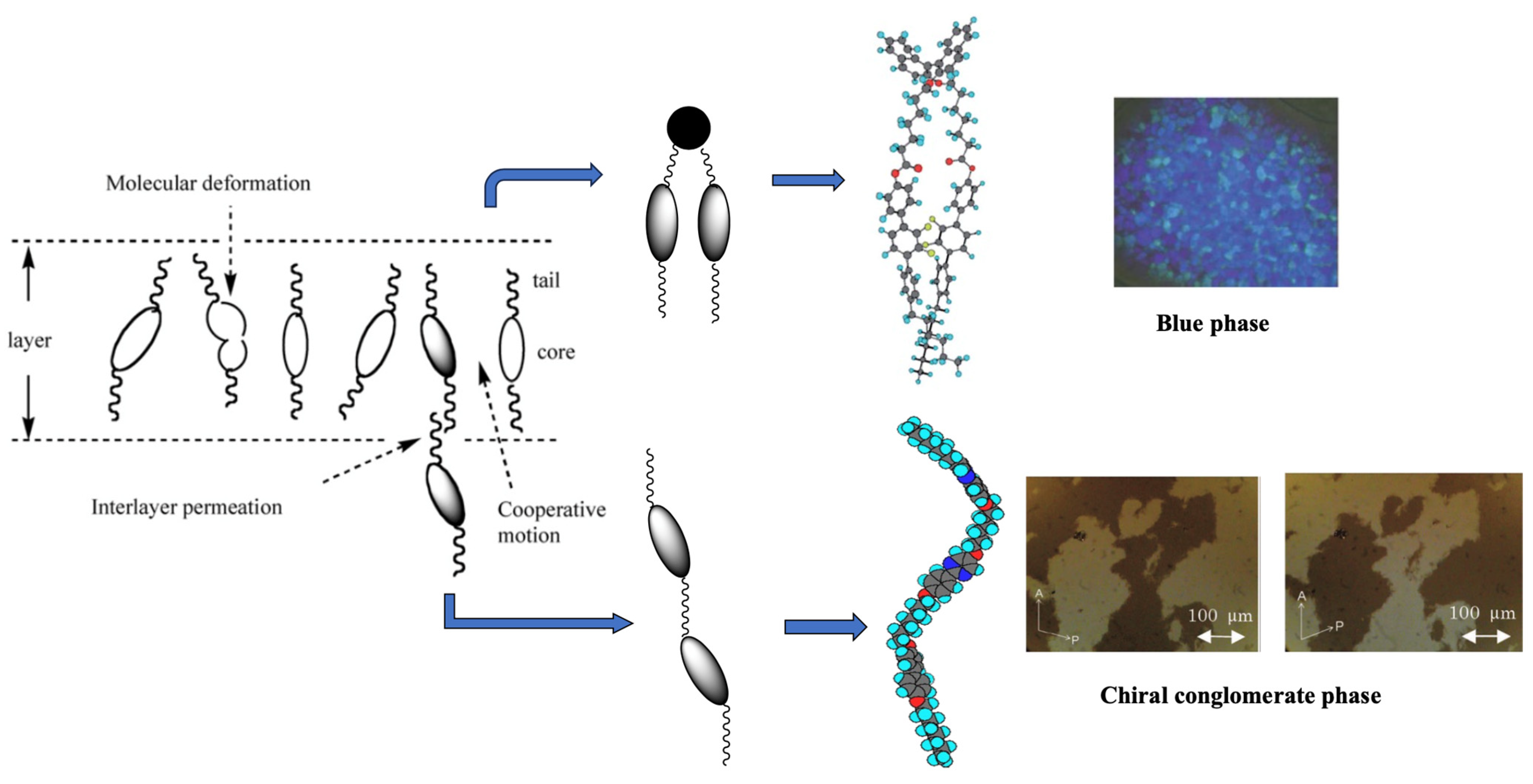
3. Dissipative Structures: Order through Fluctuation
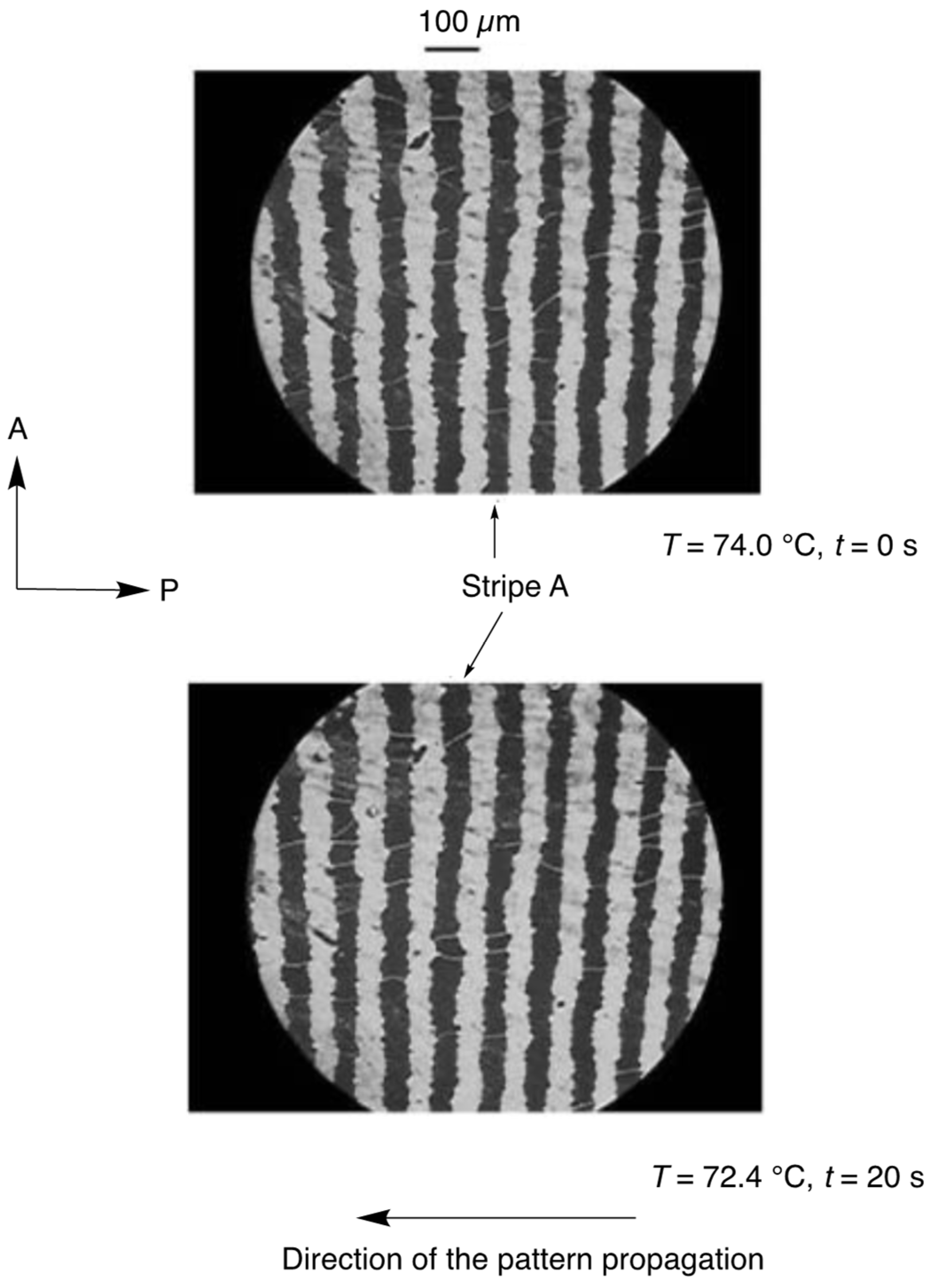
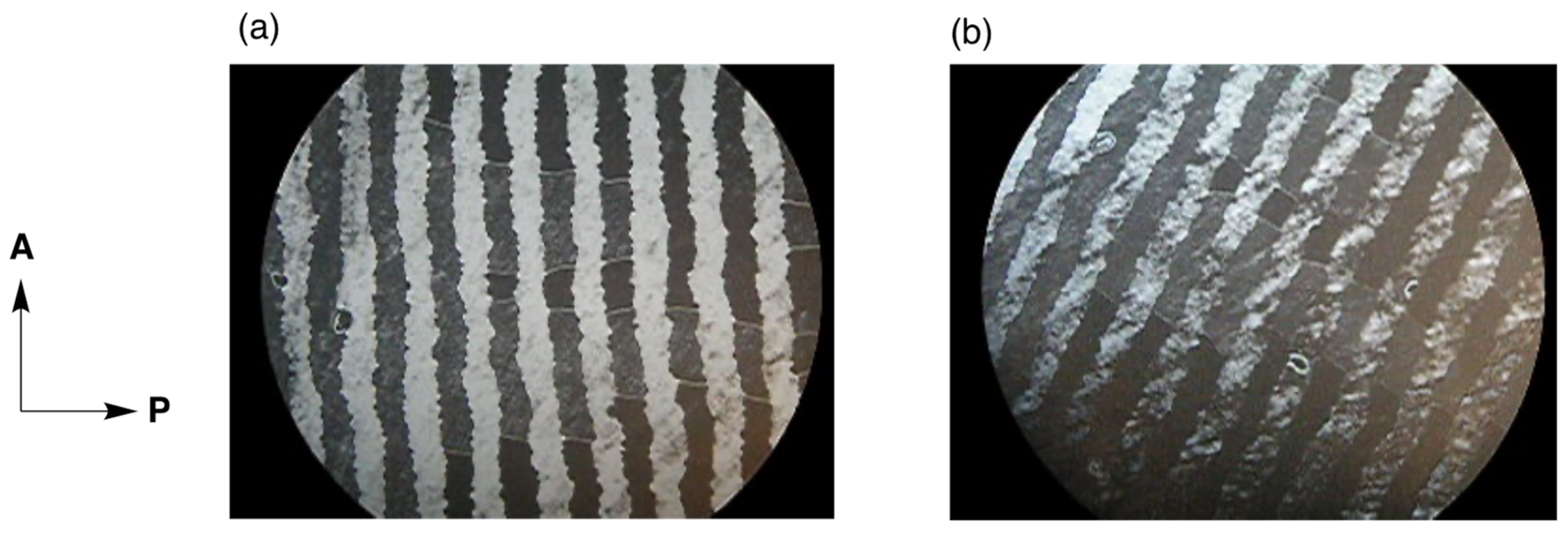
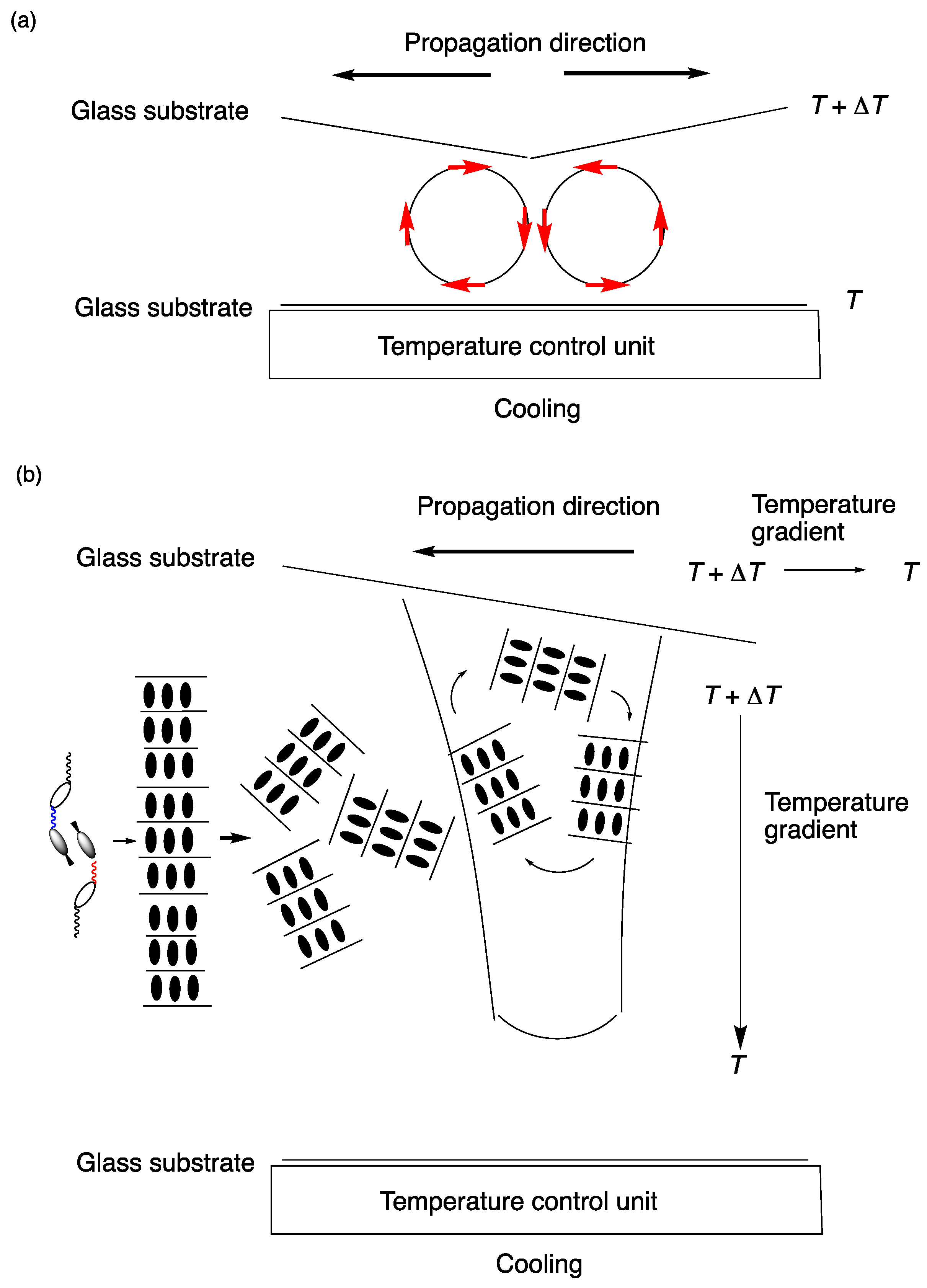
3.1. Pattern Formation and Propagation
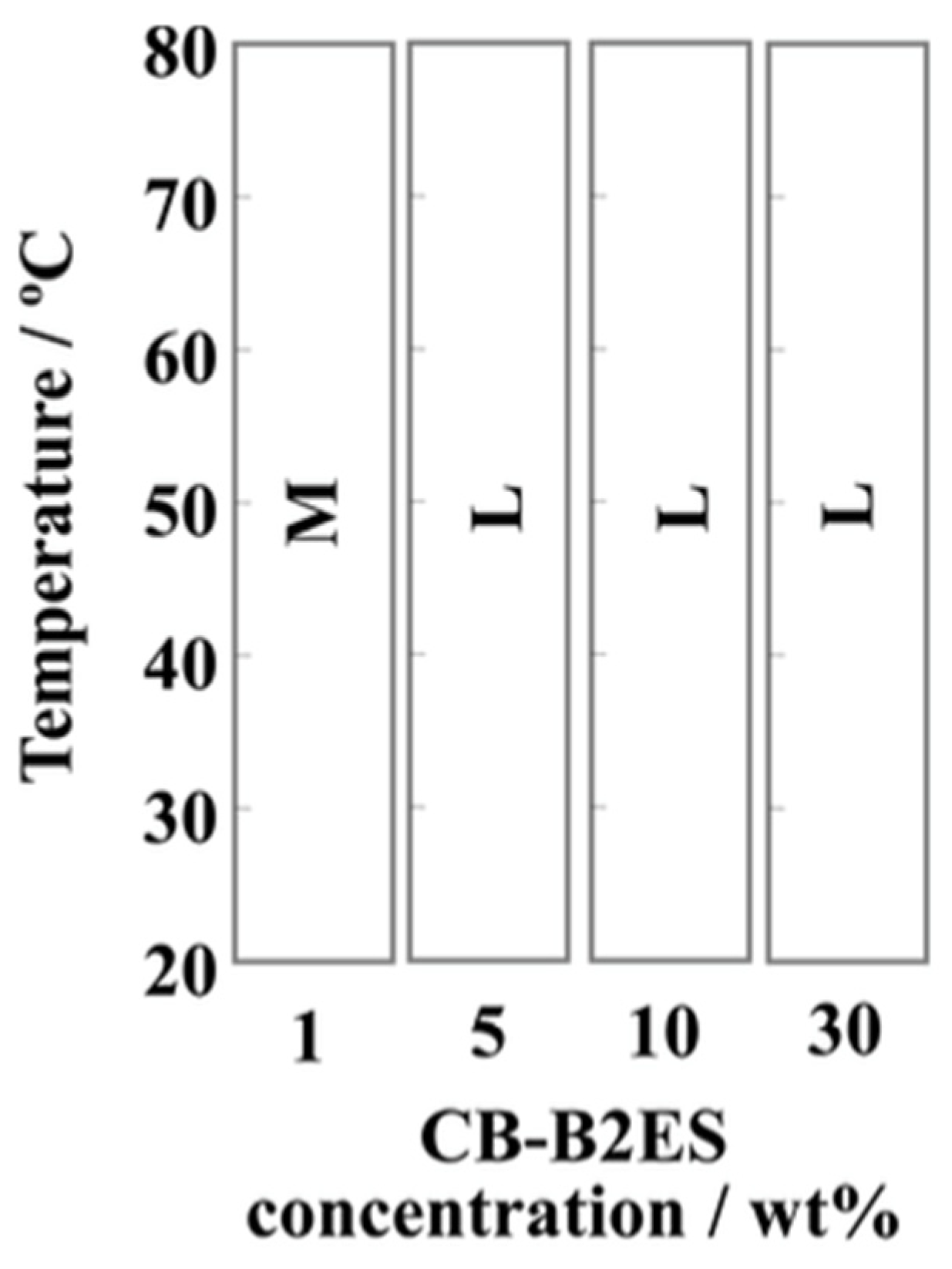
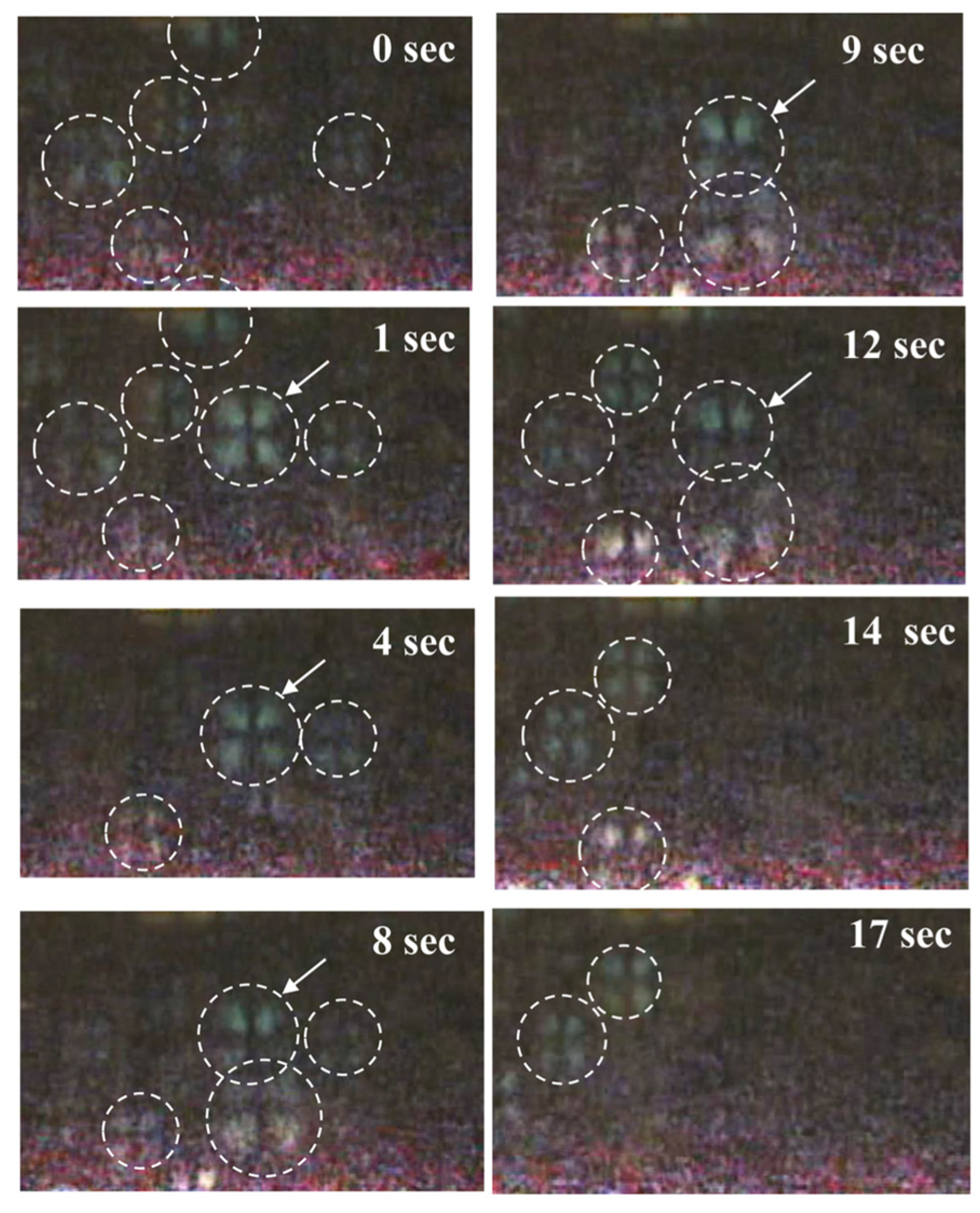
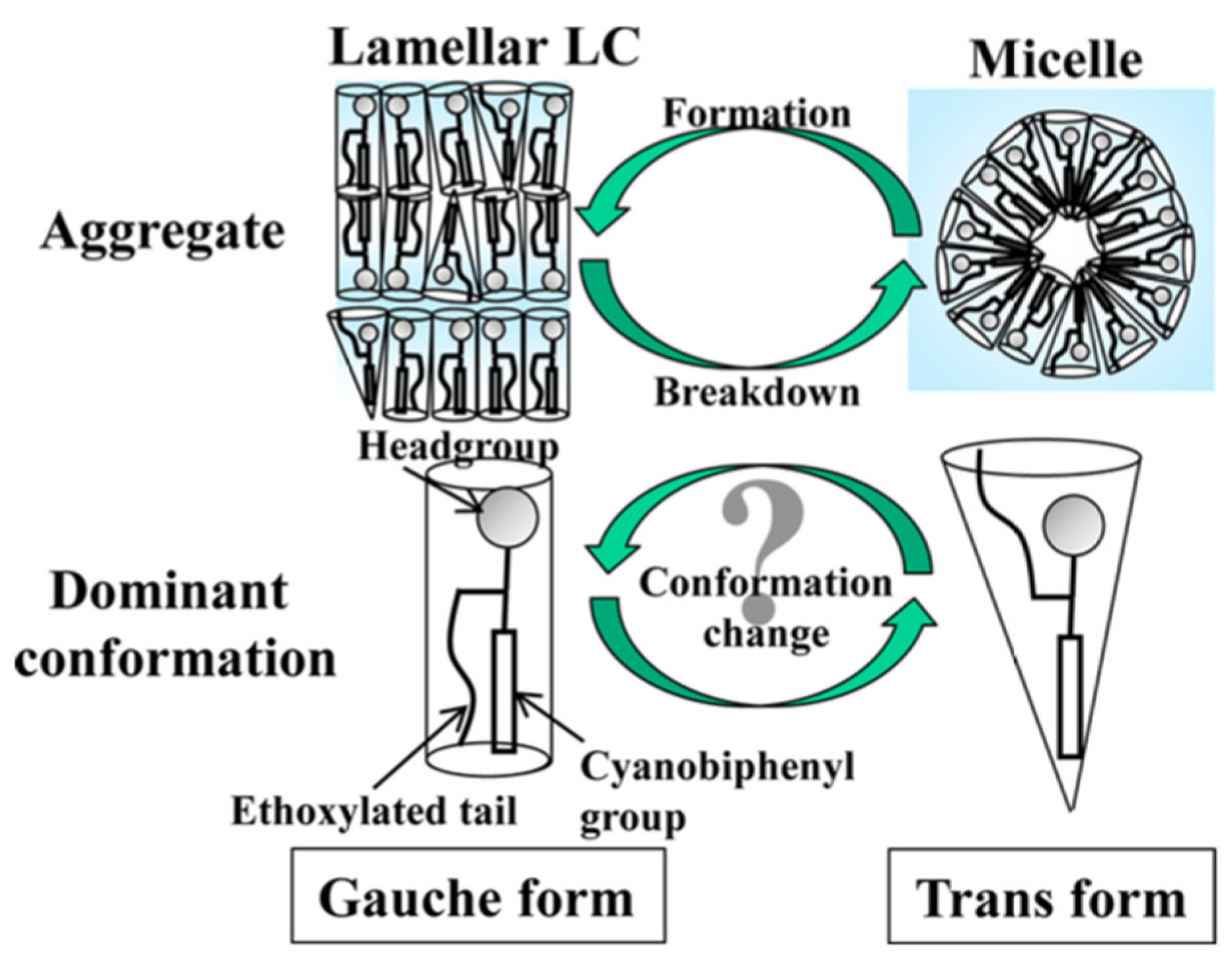
3.2. Periodic Formation/Breakdown of Lamellar Aggregates in Water
4. Concluding Remarks
Supplementary Materials
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Cladis, P.E. Fluctuations and liquid crystal phase transitions. In Handbook of Liquid Crystals, 1st ed.; Demus, D., Goodby, J.W., Gray, G.W., Spiess, H.W., Vill, V., Eds.; Wiley-VCH Verlag GmbH: Weinheim, Germany, 1998; Volume 1, pp. 379–390. [Google Scholar]
- Moses, T.; Reeves, J.; Pirondi, P. Fluctuations near a phase transition in liquid crystals. Am. J. Phys. 2007, 75, 220–225. [Google Scholar] [CrossRef]
- De Vries, A. Evidence for the existence of more than one type of nematic phase. Mol. Cryst. Liq. Cryst. 1970, 10, 31–35. [Google Scholar] [CrossRef]
- De Vries, A. X-ray photographic studies of liquid crystals I. A cybotactic nematic phase. Mol. Cryst. Liq. Cryst. 1970, 10, 219–236. [Google Scholar] [CrossRef]
- Chistyakov, I.G.; Chaikowsky, W.M. The structure of p-azoxybenzenes in magnetic fields. Mol. Cryst. Liq. Cryst. 1969, 7, 269–277. [Google Scholar] [CrossRef]
- Samulski, E.T. meta-Cybotaxis and nematic biaxiality. Liq. Cryst. 2010, 37, 669–678. [Google Scholar] [CrossRef]
- Leadbetter, A.J. Structural classification of liquid crystals. In Thermotropic Liquid Crystals; Critical Reports on Applied Chemistry; Gray, G.W., Ed.; John Wiley & Sons: Chichester, UK, 1987; Volume 22, pp. 1–27. [Google Scholar]
- Nishiya, W.; Takanishi, Y.; Yamamoto, J.; Yoshizawa, A. Molecular design foe a cybotactic nematic phase. J. Mater. Chem. C 2014, 2, 3677–3685. [Google Scholar] [CrossRef]
- Sigaud, G.; Hardoin, F.; Achard, M.F.; Levelut, A.M. A new type of smectic A phase with long range modulation in the layers. J. Phys. 1981, 42, 107–111. [Google Scholar] [CrossRef]
- Prost, J.; Barois, P. Polymorphism in polar mesogens. II—Theoretical aspects. J. Chim. Phys. 1983, 80, 65–80. [Google Scholar] [CrossRef]
- Seddon, J.M. Structural studies of liquid crystals by X-ray diffraction. In Handbook of Liquid Crystals, 1st ed.; Demus, D., Goodby, J.W., Gray, G.W., Spiess, H.W., Vill, V., Eds.; Wiley-VCH Verlag GmbH: Weinheim, Germany, 1998; Volume 1, pp. 635–679. [Google Scholar]
- Imrie, C.T.; Luckhurst, G.R. Liquid crystal dimers and oligomers. In Handbook of Liquid Crystals, 2nd ed.; Goodby, J.W., Collings, P.J., Kato, T., Tschierske, C., Gleeson, H.F., Raynes, P., Eds.; Wiley-VCH Verlag GmbH: Weinheim, Germany, 2014; Volume 7, pp. 137–210. [Google Scholar]
- Sasaki, T.; Takanishi, Y.; Yamamoto, J.; Yoshizawa, A. A frustrated phase driven by competition among layer structures. Soft Matter 2017, 13, 5194–5203. [Google Scholar] [CrossRef]
- Pociecha, D.; Kardas, D.; Gorecka, E.; Szydlowska, J.; Mieczkowski, J.; Guillon, D. Modulated and intercalated smectic phases formed by dimeric molecules. J. Mater. Chem. 2003, 13, 34–37. [Google Scholar] [CrossRef]
- Ungar, G.; Liu, F.; Zeng, X. Cubic and other 3D thermotropic liquid crystal phases and quasicrystals. In Handbook of Liquid Crystals, 2nd ed.; Goodby, J.W., Collings, P.J., Kato, T., Tschierske, C., Gleeson, H.F., Raynes, P., Eds.; Wiley-VCH Verlag GmbH: Weinheim, Germany, 2014; Volume 5, pp. 363–436. [Google Scholar]
- Kutsumizu, S. Recent progress in the synthesis and structural clarification of thermotropic cubic phases. Isr. J. Chem. 2012, 52, 844–853. [Google Scholar] [CrossRef]
- Levelut, A.-M.; Clerc, M. Structural investigation on ‘smectic D’ and related mesophases. Liq. Cryst. 1998, 24, 105–115. [Google Scholar] [CrossRef]
- Dressel, C.; Liu, F.; Prehm, M.; Zeng, X.; Ungar, G.; Tschierske, C. Dynamic mirror-symmetry breaking in bicontinuous cubic phases. Angew. Chem. Int. Ed. 2014, 53, 13115–13120. [Google Scholar] [CrossRef] [PubMed]
- Wang, Y.; Yang, S.-G.; Li, Y.-X.; Cao, Y.; Zeng, X.-B.; Cseh, L.; Ungar, G. Supertwisted chiral gyroid mesophase in chiral rod-like compounds. Angew. Chem. Int. Ed. 2024, 63, 202403156. [Google Scholar] [CrossRef] [PubMed]
- Ichikawa, T.; Yoshio, M.; Hamasaki, A.; Taguchi, S.; Liu, F.; Zeng, X.-B.; Ungar, G.; Ohno, H.; Kato, T. Induction of thermotropic bicontinuous cubic phases in liquid-crystalline ammonium and phosphonium salts. J. Am. Chem. Soc. 2012, 134, 2634–2643. [Google Scholar] [CrossRef]
- Kato, T.; Yoshio, M.; Ichikawa, T.; Soberats, B.; Ohno, H.; Funahashi, M. Transport of ions and electrons in nanostructured liquid crystals. Nat. Rev. Mater. 2017, 2, 17001. [Google Scholar] [CrossRef]
- Prigogine, I. Time, structure, and fluctuations. Science 1978, 201, 777–785. [Google Scholar] [CrossRef] [PubMed]
- Prigogine, I. Evolution and Consciousness: Human Systems in Transition; Jantsch, E., Ed.; Addison-Wesley: Boulder, CO, USA, 1976; pp. 93–130. [Google Scholar]
- Yoshizawa, A. Uncoventional liquid crystal oligomers with a hierarchical structure. J. Mater. Chem. 2008, 18, 2877–2889. [Google Scholar] [CrossRef]
- Saez, I.M.; Goodby, J.W. Supermolecular liquid crystals. Struct. Bond. 2008, 128, 1–62. [Google Scholar]
- Imrie, C.; Henderson, P.A. Liquid crystal dimers and higher oligomers: Between monomers and polymers. Chem. Soc. Rev. 2007, 36, 2096–2124. [Google Scholar] [CrossRef]
- Saez, I.M. Supermolecular liquid crystals. In Handbook of Liquid Crystals, 2nd ed.; Goodby, J.W., Collings, P.J., Kato, T., Tschierske, C., Gleeson, H.F., Raynes, P., Eds.; Wiley-VCH Verlag GmbH: Weinheim, Germany, 2014; Volume 7, pp. 211–258. [Google Scholar]
- Cestari, M.; Diez-Berart, S.; Dunmur, D.A.; Ferrarini, M.R.; Jackson, D.J.B.; Lopez, D.O.; Luckhurst, G.R.; Perez-Jubindo, M.A.; Richardson, R.M.; Salud, J. Phase behavior and properties of the liquid-crystal dimer 1″,7″-bis(4-cyanobiphenyl-4′-yl) heptane: A twist-bend nematic liquid crystal. Phys. Rev. E 2011, 84, 031704. [Google Scholar] [CrossRef]
- Paterson, D.A.; Abberley, J.P.; Harrison, W.T.A.; Storey, J.M.D.; Imrie, C.T. Cyanobiphenyl-based liquid crystal dimers and the twist-bend nematic phase. Liq. Cryst. 2017, 44, 127–146. [Google Scholar] [CrossRef]
- Yoshizawa, A. Nanostructured assemblies of liquid-crystalline supermolecules: From display to medicine. Liq. Cryst. 2019, 46, 1950–1972. [Google Scholar] [CrossRef]
- Yoshizawa, A.; Kikuzaki, H.; Fukumasa, M. Microscopic organization of the molecules in smectic A and chiral (racemic) smectic C phases: Dynamic molecular deformation effect on the SA to SC* (SC) transition. Liq. Cryst. 1995, 18, 351–366. [Google Scholar] [CrossRef]
- Yoshizawa, A.; Kogawa, Y.; Kobayashi, K.; Takanishi, Y.; Yamamoto, J. A binaphthyl derivative with a wide temperature range of a blue phase. J. Mater. Chem. 2009, 19, 5759–5764. [Google Scholar] [CrossRef]
- Sasaki, H.; Takanishi, Y.; Yamamoto, J.; Yoshizawa, A. Achiral flexible liquid crystal trimers exhibiting chiral conglomerates. Soft Matter 2016, 12, 3331–3339. [Google Scholar] [CrossRef] [PubMed]
- Mandle, R.J.; Bevis, E.; Goodby, J.W. Phase structures of nematic liquid crystals. In Handbook of Liquid Crystals, 2nd ed.; Goodby, J.W., Collings, P.J., Kato, T., Tschierske, C., Gleeson, H.F., Raynes, P., Eds.; Wiley-VCH Verlag GmbH: Weinheim, Germany, 2014; Volume 3, pp. 3–29. [Google Scholar]
- Kumar, M.P.; Sai, D.V.; Dhara, S. Effect of Sm-A short-range order on the activation energies of translational and rotational viscosities of nematic liquid crystals with highly polar molecules. Phys. Rev. E 2018, 98, 062701. [Google Scholar] [CrossRef]
- Freiser, M.J. Ordered states of a nematic liquid. Phys. Rev. Lett. 1970, 24, 1041–1043. [Google Scholar] [CrossRef]
- Lehmann, M.; Görtz, V. Design of biaxial nematic mesogens. In Handbook of Liquid Crystals, 2nd ed.; Goodby, J.W., Collings, P.J., Kato, T., Tschierske, C., Gleeson, H.F., Raynes, P., Eds.; Wiley-VCH Verlag GmbH: Weinheim, Germany, 2014; Volume 3, pp. 335–373. [Google Scholar]
- Tschierske, C.; Photinos, D.J. Biaxial nematic phases. J. Mater. Chem. 2010, 20, 4263–4294. [Google Scholar] [CrossRef]
- Bruce, D.W. Towards the biaxial nematic phase through molecular design. Chem. Rec. 2004, 4, 10–22. [Google Scholar] [CrossRef]
- Mandle, R.J. A ten-year perspective on twist-bend nematic materials. Molecules 2022, 27, 2689. [Google Scholar] [CrossRef] [PubMed]
- Mandle, R.J.; Cowling, S.J.; Goodby, J.W. A nematic to nematic transformation exhibited by a rod-like liquid crystal. Phys. Chem. Chem. Phys. 2017, 19, 11429–11435. [Google Scholar] [CrossRef]
- Mandle, R.J.; Cowling, S.J.; Goodby, J.W. Rational design of rod-like liquid crystals exhibiting two nematic phases. Chem.—A Eur. J. 2017, 23, 14554–14562. [Google Scholar] [CrossRef] [PubMed]
- Nishikawa, H.; Shiroshita, K.; Higuchi, H.; Okumura, Y.; Haseba, Y.; Yamamoto, S.; Sago, K.; Kikuchi, H. A fluid liquid-crystal material with highly polar order. Adv. Mater. 2017, 29, 1702354. [Google Scholar] [CrossRef] [PubMed]
- Chen, X.; Korblova, E.; Glaser, M.A.; Maclennan, J.E.; Walba, D.M.; Clark, N.A. Polar in-plane surface orientation of a ferroelectric nematic liquid crystal: Polar monodomains and twisted state electro-optics. Proc. Natl. Acad. Sci. USA 2021, 118, e2104092118. [Google Scholar] [CrossRef] [PubMed]
- Karcz, J.; Herman, J.; Rychłowicz, N.; Kula, P.; Górecka, E.; Szydlowska, J.; Majewski, P.; Pociecha, D. Spontaneous chiral symmetry breaking in polar fluid-heliconical ferroelectric nematic phase. Science 2024, 384, 1096–1099. [Google Scholar] [CrossRef]
- Nasir, A.K.; Rahman, M. Cybotactic nematic liquid crystal—An overview. Liq. Cryst. 2024, 51, 503–535. [Google Scholar] [CrossRef]
- Keith, C.; Lehmann, A.; Baumeister, U.; Prehm, M.; Tschierske, C. Nematic phases of bent-core mesogens. Soft Matter 2010, 6, 1703–1721. [Google Scholar] [CrossRef]
- Deb, R.; Nath, R.K.; Sarkar, D.D.; Araoka, F.; Ishikawa, K.; Takezoe, H.; Takanishi, Y. Synthesis of four-ring unsymmetrical bent-core mesogens and cybotactic cluster formation in their nematic phase. J. Mol. Struct. 2024, 1317, 139055. [Google Scholar] [CrossRef]
- Tadapartri, P.; Hiremath, U.S.; Yelamaggad, C.V.; Krishnamurthy, K.S. Permittivity, conductivity, elasticity, and viscosity measurements in the nematic phase of a bent-core liquid crystal. J. Phys. Chem. B 2010, 114, 1745–1750. [Google Scholar] [CrossRef]
- Panarin, Y.P.; Sreenilayam, S.P.; Vij, J.K.; Lehnmann, A.; Tschierske, C. Formation and development of nanometer-sized cybotactic clusters in bent-core nematic liquid crystalline compounds. Beilstein J. Nanotechnol. 2018, 9, 1288–1296. [Google Scholar] [CrossRef] [PubMed]
- Monika, M.; Roy, A.; Prasad, V. Smectic nanoclusters in the nematic mesophases of dimeric compounds composed of rod-like azo moieties with lateral substituents. New J. Chem. 2017, 41, 11576–11583. [Google Scholar]
- Vita, F.; Sparnacci, K.; Panzarasa, G.; Placentino, I.F.; Marino, S.; Scaramuzza, N.; Portale, G.; Cola, E.D.; Ferrero, C.; Torgova, S.I.; et al. Evidence of cybotactic order in the nematic phase of a main-chain liquid crystal polymer with bent-core repeat unit. ACS Macro Lett. 2014, 3, 91–95. [Google Scholar] [CrossRef]
- Yoshizawa, A.; Yamaguchi, A. Kinetically induced intermolecular association: Unusual enthalpy changes in the nematic phase of a novel dimeric liquid-crystalline molecule. Chem. Commun. 2002, 2060–2061. [Google Scholar] [CrossRef] [PubMed]
- Vanakaras, A.G.; Photinos, D.J. Thermotropic biaxial nematic liquid crystals: Spontaneous or field stabilized? J. Chem. Phys. 2008, 128, 154512. [Google Scholar] [CrossRef] [PubMed]
- Lehmann, M. Biaxial nematics from their prediction to the materials and the vicious circle of molecular design. Liq. Cryst. 2011, 38, 1389–1405. [Google Scholar] [CrossRef]
- Taushanoff, S.; Le, K.V.; Williams, J.; Twieg, R.J.; Sadashiva, B.K.; Takezoe, H.; Jakli, A. Stable amorphous blue phase of bent-core nematic liquid crystals doped with a chiral material. J. Mater. Chem. 2010, 20, 5893–5898. [Google Scholar] [CrossRef]
- Francescangeli, O.; Stanic, V.; Torgova, S.I.; Strigazzi, A.; Scaramuzza, N.; Ferrero, C.; Dolbnya, I.P.; Weiss, T.M.; Beradi, R.; Muccioli, L.; et al. ferroelectric response and induced biaxiality in the nematic phase of bent-core mesogens. Adv. Funct. Mater. 2009, 19, 2592–2600. [Google Scholar] [CrossRef]
- Vita, F.; Adamo, F.C.; Francescangeli, O. Polar order in bent-core nematics: An overview. J. Mol. Liq. 2018, 267, 564–573. [Google Scholar] [CrossRef]
- Nishikawa, H.; Sano, K.; Kurihara, S.; Watanabe, G.; Nisjonyanagi, A.; Dhara, B.; Araoka, F. Nano-clustering mediates phase transitions in a diastereomerically-stabilized ferroelectric nematic system. Commun. Mater. 2022, 3, 89. [Google Scholar] [CrossRef]
- Yoshizawa, A.; Nishizawa, A.; Takeuchi, K.; Takanishi, Y.; Yamamoto, J. Interlayer interactions induced by amphiphilicities of a rod-like molecule produce frustrated structures in conventional calamitic phases. J. Phys. Chem. B 2010, 114, 13304–13311. [Google Scholar] [CrossRef] [PubMed]
- Kimoto, Y.; Nishizawa, A.; Takanishi, Y.; Yoshizawa, A.; Yamamoto, J. Layer modulated smectic-C phase in liquid crystals. Phys. Rev. E 2014, 89, 042503. [Google Scholar] [CrossRef] [PubMed]
- Barois, P. Phase transition theories. In Handbook of Liquid Crystals, 1st ed.; Demus, D., Goodby, J.W., Gray, G.W., Spiess, H.W., Vill, V., Eds.; Wiley-VCH Verlag GmbH: Weinheim, Germany, 1998; Volume 1, pp. 281–309. [Google Scholar]
- Lobko, T.A.; Ostrovskii, B.I.; Hasse, W. X-ray studies of the smectic phase and transitions between reentrant nematic, smectic A1, Ad and phases in binary mixtures of polar mesogens. J. Phys. II 1992, 2, 1195–1213. [Google Scholar] [CrossRef]
- Tinh, N.H.; Destrade, A.M.; Levelut, A.M.; Malthete, J. Biforked mesognes: A new type of thermotropic liquid crystals. J. Phys. 1986, 47, 553–557. [Google Scholar] [CrossRef]
- Date, R.W.; Luckhurst, G.R.; Shuman, M.; Seddon, J.M. Novel modulated hexatic phases in symmetric liquid crystal dimers. J. Phys. II 1995, 5, 587–605. [Google Scholar] [CrossRef]
- Faye, V.; Babeau, A.; Placin, F.; Nguyen, H.T.; Barois, P.; Laux, V.; Isaert, N. SC*A and S[Ctilde]* phases in chiral non-symmetric dimesogens. Liq. Cryst. 1996, 21, 485–503. [Google Scholar] [CrossRef]
- Walker, R.; Pociecha, D.; Storey, J.M.; Gorecka, E.; Imrie, C.T. Remarkable smectic phase behaviour in odd-membered liquid crystal dimers: The CT6O.m series. J. Mater. Chem. C 2021, 9, 5167–5173. [Google Scholar] [CrossRef]
- Pociecha, D.; Vaupotic, N.; Gorecka, E.; Mieczkowski, J.; Gomola, K. 2-D Density-modulated structures in asymmetric bent-core liquid crystals. J. Mater. Chem. 2008, 18, 881–885. [Google Scholar] [CrossRef]
- Nguyen, H.T.; Sigaud, G.; Achard, M.F.; Hardouin, F. Rod-like mesogens with antipathetic fluorocarbon and hydrocarbon tails. Liq. Cryst. 1991, 10, 389–396. [Google Scholar] [CrossRef]
- Davidson, P.; Keller, P.; Levelut, A.M. Molecular organization in side chain liquid crystalline polymers. J. Phys. 1985, 46, 939–946. [Google Scholar] [CrossRef]
- Watanabe, J.; Nakata, Y.; Shimizu, K. Frustrated bilayer smectic phase in main-chain polymers with two different spacers. J. Phys. II 1994, 4, 581–588. [Google Scholar] [CrossRef]
- Endres, B.W.; Ebert, M.; Wendorff, J.H.; Reck, B.; Ringsdorf, H. Combined main chain/side chain polymers: A new class of liquid crystalline polymers with unusual structural thermotropic and dynamic properties. Liq. Cryst. 1990, 7, 217–239. [Google Scholar] [CrossRef]
- Kashima, S.; Takanishi, Y.; Yamamoto, J.; Yoshizawa, A. Flexible taper-shaped liquid crystal trimer exhibiting a modulated smectic phase. Liq. Cryst. 2014, 41, 1752–1761. [Google Scholar] [CrossRef]
- Kashima, S. Supermolecular liquid crystals exhibiting frustrated phases. Master’s Thesis, Hirosaki University, Hirosaki, Japan, 18 February 2014. [Google Scholar]
- Rybalova, T.V.; Bagryanskaya, I.Y. C–F…π, F…H, and F…F intermolecular interactions and F-aggregation: Role in crystal engineering of fluoroorganic compounds. J. Struct. Chem. 2009, 50, 741–753. [Google Scholar] [CrossRef]
- Collings, P.J. The structures of the blue phases. In Handbook of Liquid Crystals, 2nd ed.; Goodby, J.W., Collings, P.J., Kato, T., Tschierske, C., Gleeson, H.F., Raynes, P., Eds.; Wiley-VCH Verlag GmbH: Weinheim, Germany, 2014; Volume 3, pp. 587–609. [Google Scholar]
- Alfutimie, A.; Curtis, R.; Tiddy, G.J.T. Lyotropic surfactant liquid crystals: Micellar systems. In Handbook of Liquid Crystals, 2nd ed.; Goodby, J.W., Collings, P.J., Kato, T., Tschierske, C., Gleeson, H.F., Raynes, P., Eds.; Wiley-VCH Verlag GmbH: Weinheim, Germany, 2014; Volume 6, pp. 377–420. [Google Scholar]
- Gray, G.W.; Jones, B.; Marson, F. 71. Mesomorphism and chemical constitution. Part VIII. The effect of 3′-substituents on the mesomorphism of the 4′-n-alkoxydiphenyl-4-carboxylic acid and their alkyl esters. J. Chem. Soc. 1957, 393–401. [Google Scholar] [CrossRef]
- Diel, S.; Göring, P. Thermotropic cubic phases. In Handbook of Liquid Crystals, 1st ed.; Demus, D., Goodby, J.W., Gray, G.W., Spiess, H.W., Vill, V., Eds.; Wiley-VCH Verlag GmbH: Weinheim, Germany, 1998; Volume 2B, pp. 887–900. [Google Scholar]
- Rowe, K.E.; Bruce, D.W. The synthesis and mesomorphism of di-, tetra- and hexa-catenar liquid crystals based on 2,2′-bipyridine. J. Mater. Chem. 1998, 8, 331–341. [Google Scholar] [CrossRef]
- Borisch, K.; Diel, S.; Göring, P.; Müller, H.; Tschierske, C. Amphiphilic N-benzoyl-1-amino-1-deoxy-D-glucitol derivatives forming thermotropic lamellar, columnar and different types of cubic mesophases. Liq. Cryst. 1997, 22, 427–443. [Google Scholar] [CrossRef]
- Tschierske, C.; Ungar, G. Mirror symmetry braking by chirality synchronization in liquids and liquid crystals of achiral molecules. ChemPhysChem 2016, 17, 9–26. [Google Scholar] [CrossRef]
- Vaupotič, N.; Salamończyk, M.; Matraszek, J.; Vogrin, M.; Pociecha, D.; Górecka, E. New structural model of a chiral cubic liquid crystalline phase. Phys. Chem. Chem. Phys. 2020, 22, 12814–12820. [Google Scholar] [CrossRef]
- Reppe, T.; Poppe, S.; Tschierske, C. Controlling mirror symmetry breaking and network formation in liquid crystalline cubic, isotropic liquid and crystalline phases of benzil-based polycatenars. Chem. Eur. J. 2020, 26, 16066–16079. [Google Scholar] [CrossRef] [PubMed]
- Oka, T.; Yamamura, Y.; Kutsumizu, S.; Saito, K. Aggregation structure of chiral cubic liquid crystals revealed by X-ray diffraction utilizing a new algorithm. Soft Matter 2023, 19, 1194–1201. [Google Scholar] [CrossRef] [PubMed]
- Rancon, Y.; Charvolin, J. Fluctuations and phase transformations in a lyotropic liquid crystal. J. Phys. Chem. 1988, 92, 6339–6344. [Google Scholar] [CrossRef]
- Yamaguchi, A.; Maeda, Y.; Yokoyama, H.; Yoshizawa, A. Self-assembly of amphiphilic liquid-crystalline oligomers possessing a semiperfluorinated alkyl chain. Chem. Mater. 2006, 18, 5704–5710. [Google Scholar] [CrossRef]
- Safinya, C.R.; Roux, D.; Smith, G.S.; Sinha, S.K.; Dimon, P.; Clark, N.A.; Bellocq, A.M. Steric interactions in a model multimembrane system: A synchrotron X-ray study. Phys. Rev. Lett. 1986, 57, 2718–2721. [Google Scholar] [CrossRef]
- Hjduk, D.A.; Takenouchi, H.; Hilmyer, M.A.; Bates, F.S.; Vigild, M.E.; Almdal, K. Stability of the perforated layer (PL) phase in deblock copolymer melts. Macromolecules 1997, 30, 3788–3795. [Google Scholar] [CrossRef]
- Qi, S.; Wang, Z.-G. Kinetics of phase transitions in weakly segregated block copolymers: Pseudostable and transient states. Phys. Rev. E 1997, 55, 1682–1697. [Google Scholar] [CrossRef]
- Laradji, M.; Shi, A.-C.; Noolandi, J.; Desai, R.C. Stability of ordered phases in deblock copolymer melts. Macromolecules 1997, 30, 3242–3255. [Google Scholar] [CrossRef]
- Imai, M.; Kawaguchi, A.; Saeki, A.; Nakaya, K.; Kato, T.; Ito, K.; Amemiya, Y. Fluctuations of a lamella r gyroid transition in a nonionic surfactant system. Phys. Rev. E 2000, 62, 6865–6874. [Google Scholar] [CrossRef]
- Takeuchi, K.; Takanishi, Y.; Yamamoto, J.; Yoshizawa, A. Amplification taper-shaped oligomer exhibiting a monolayer smectic A to columnar phase transition. Liq. Cryst. 2010, 37, 507–515. [Google Scholar] [CrossRef]
- Boussinesq, M.J. Theorie de I’intumescene liquid appellee onde solitaire ou de translation, se propagent dans un canal rectangulaire. Comptes Rendus Acad. Sci. 1871, 72, 755–759. [Google Scholar]
- Oberbeck, A. Über die wärmeletiung der flüssigkeiten bei berücksichtigung der strömungen infolge von temperaturdifferenzen. Ann. Phys. Chem. 1879, 7, 271–292. [Google Scholar] [CrossRef]
- Getling, A.V. Rayleigh-Bénard Convection: Structures and Dynamics; World Scientific: Singapore, 1998. [Google Scholar] [CrossRef]
- Belousov, B.P. A periodic reaction and its mechanism. In Collection of Short Papers on Radiation Medicine for 1958; Meditsina Publishers: Moscow, Russia, 1959. [Google Scholar]
- Zhabotinsky, A.M. Periodical oxidation of malonic acid in solution (a study of the Belousov reaction kinetics). Biofizika 1964, 9, 306–311. [Google Scholar]
- Cassani, A.; Monteverde, A.; Piumetti, M. Belousov-Zhabotinsky type reactions: The non-linear behavior of chemical systems. J. Math. Chem. 2021, 59, 792–826. [Google Scholar] [CrossRef]
- Buka, A.; Kramer, L. (Eds.) Pattern Formation in Liquid Crystals; Springer: New York, NY, USA, 1996. [Google Scholar]
- Wang, Z.; Servio, P.; Rey, A.D. Structure and pattern formation in biological liquid crystals: Insights from theory and simulation of self-assembly and self-organization. Front. Soft Matter 2022, 2, 904069. [Google Scholar] [CrossRef]
- Morris, S.W.; De Bruyn, J.R.; May, A.D. Electroconvection and pattern formation in a suspended smectic film. Phys. Rev. Lett. 1990, 65, 2378–2381. [Google Scholar] [CrossRef] [PubMed]
- Migler, K.B.; Meyer, R.B. Solitons and pattern formation in liquid crystals in a rotating magnetic field. Phys. Rev. Lett. 1991, 66, 1485–1488. [Google Scholar] [CrossRef] [PubMed]
- Andrade-Sliva, I.; Bortolozzo, U.; Clerc, M.G.; González-Cortés, G.; Residori, S.; Wilson, M. Spontaneous light-induced turning patterns in a dye-doped twisted nematic layer. Sci. Rep. 2018, 8, 12867. [Google Scholar] [CrossRef] [PubMed]
- Tang, X.; Atzin, N.; Mozaffari, A.; Das, S.; Abbott, N.L.; De Pablo, J.J. Generation and propagation of flexoelectricity-induced solitons in nematic liquid crystals. ACS Nano 2024, 18, 10768–10775. [Google Scholar] [CrossRef] [PubMed]
- Clerc, M.G.; Gonzalez-Cortes, G.; Morel, M.J.; Hidalgo, P.I.; Vergara, J. Finger front propagation in smectic-A Fréedericksz transition. Phys. Rev. E 2022, 105, 054701. [Google Scholar] [CrossRef]
- Yoshizawa, A.; Kurauchi, M.; Kohama, Y.; Dewa, H.; Yamamoto, K.; Nishiyama, I.; Yamamoto, T.; Yamamoto, J.; Yokoyama, H. Synthesis and physical properties of α-(4-cyanobiphenyl-4′-yloxy)-ω-[4-(5-alkylpyrimidine-2-yl)phenyloxy]alkanes. Liq. Cryst. 2006, 33, 611–619. [Google Scholar] [CrossRef]
- Hegmann, T.; Kain, J.; Diel, S.; Pelzl, G.; Tschierske, C. Evidence for the existence of the McMillan phase in a binary system of a metallomesogen and 2,4,7-trinitrofluorenone. Angew. Chem. Int. Ed. 2001, 40, 887–890. [Google Scholar] [CrossRef]
- Sadashiva, B.K.; Reddy, R.A.; Pratibha, R.; Madhusudana, N.V. Biaxial smectic A phase in homologous series of compounds composed of highly polar unsymmetric substituted core molecules. J. Mater. Chem. 2002, 12, 943–950. [Google Scholar] [CrossRef]
- Yelamaggad, C.V.; Shashikala, I.S.; Rao, D.S.S.; Nair, G.G.; Prasad, S.K. The biaxial smectic (SmAb) phase in nonsymmetric liquid crystal dimers comprising two rodlike anisometric segments: An unusual behavior. J. Mater. Chem. 2006, 16, 4099–4102. [Google Scholar] [CrossRef]
- Guo, V.; Li, Z.; Fung, B.M.; O’Rear, E.A.; Harwell, J.H. Hybrid surfactants containing separate hydrocarbon and fluorocarbon chains. J. Phys. Chem. 1992, 96, 6738–6742. [Google Scholar] [CrossRef]
- Aydogan, N.; Aldis, N.; Guvenir, O. Interfacial and bulk properties of the new fluorocarbon−hydrocarbon hybrid unsymmetrical bolaform surfactant. Langmuir 2003, 19, 10726–10731. [Google Scholar] [CrossRef]
- Ito, A.; Sakai, H.; Kondo, Y.; Yoshino, N.; Abe, M. Micellar solution properties of fluorocarbon−hydrocarbon surfactants. Langmuir 1996, 12, 5768–5772. [Google Scholar] [CrossRef]
- Kondo, Y.; Yoshino, N. Hybrid fluorocarbon/hydrocarbon surfactants. Curr. Opin. Colloid Interface Sci. 2005, 10, 88–93. [Google Scholar] [CrossRef]
- Miyazawa, H.; Igawa, K.; Kondo, Y.; Yoshino, N. Synthesis and solution properties of sulfate-type hybrid surfactants with a benzene ring. J. Fluor. Chem. 2003, 124, 189–196. [Google Scholar] [CrossRef]
- Kondo, Y.; Miyazawa, H.; Sakai, H.; Abe, M.; Yoshino, N. First anionic micelle with unusually long lifetime: Self-assembly of fluorocarbon−hydrocarbon hybrid surfactant. J. Am. Chem. Soc. 2002, 124, 6516–6517. [Google Scholar] [CrossRef]
- Sagisaka, M.; Fujita, Y.; Nakanishi, Y.; Takahashi, H.; Tsuyoshi, N.; James, C.; Yoshizawa, A.; Mohamed, A.; Guittard, F.; Eastoe, J. Periodic formation/breakdown of lamellar aggregates with anionic cyanobiphenyl surfactants. Langmuir 2015, 31, 13040–13047. [Google Scholar] [CrossRef]
- Kunitake, T.; Okahata, Y. A Totally synthetic bilayer membrane. J. Am. Chem. Soc. 1977, 99, 3860–3861. [Google Scholar] [CrossRef]
- Okahata, Y.; Kunitake, T. Formation of stable monolayer membranes and related structures in dilute aqueous solution from two-headed ammonium amphiphiles. J. Am. Chem. Soc. 1979, 101, 5231–5234. [Google Scholar] [CrossRef]
- Fendler, J.H. Surfactant vesicles as membrane mimetic agents: Characterization and utilization. Acc. Chem. Res. 1980, 13, 7–13. [Google Scholar] [CrossRef]
- Zana, R. Dynamics of Surfactant Self-Assemblies: Micelles, Microemulsions, Vesicles and Lyotropic Phases; CRC Press: Boca Raton, FL, USA, 2005. [Google Scholar]










Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Yoshizawa, A. Liquid-Crystalline Supermolecules Inducing Layer Fluctuations: From Hierarchical to Dissipative Structures. Crystals 2024, 14, 681. https://doi.org/10.3390/cryst14080681
Yoshizawa A. Liquid-Crystalline Supermolecules Inducing Layer Fluctuations: From Hierarchical to Dissipative Structures. Crystals. 2024; 14(8):681. https://doi.org/10.3390/cryst14080681
Chicago/Turabian StyleYoshizawa, Atsushi. 2024. "Liquid-Crystalline Supermolecules Inducing Layer Fluctuations: From Hierarchical to Dissipative Structures" Crystals 14, no. 8: 681. https://doi.org/10.3390/cryst14080681
APA StyleYoshizawa, A. (2024). Liquid-Crystalline Supermolecules Inducing Layer Fluctuations: From Hierarchical to Dissipative Structures. Crystals, 14(8), 681. https://doi.org/10.3390/cryst14080681




