Impact of Schwoebel Barriers on the Step-Flow Growth of a Multicomponent Crystal
Abstract
:1. Introduction
2. Formulation of the Problem
3. Results and Discussion
3.1. Adatom Distribution on the Surface
3.2. Rate of Advancement of a Group of Equidistant Steps and Crystal Growth Rate
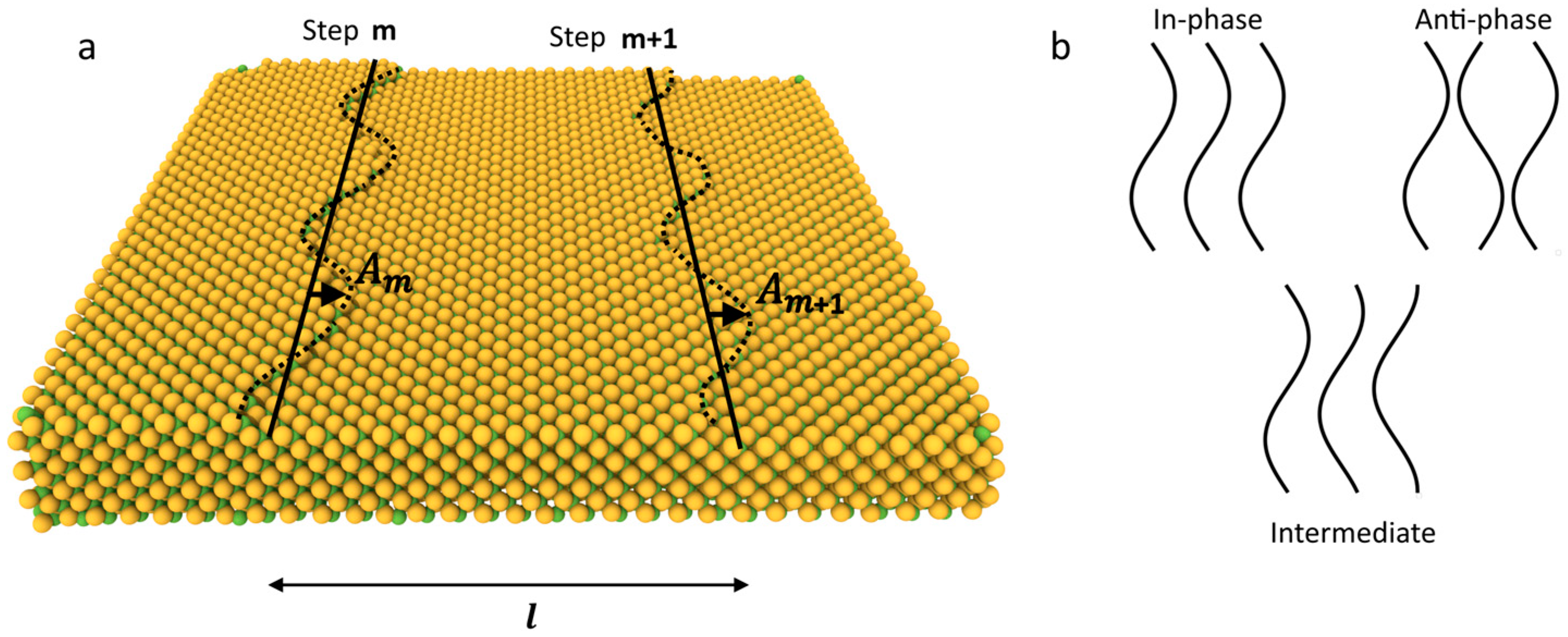
3.3. Instability Analysis
3.3.1. Step System Instability
3.3.2. Nucleation of Pure Components behind the Moving Step
4. Conclusions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
References
- Burton, W.K.; Cabrera, N.; Frank, F.C. The growth of crystals and the equilibrium structure of their surfaces. Philos. Trans. R. Soc. A 1951, 243, 299–358. [Google Scholar]
- Murata, K.I.; Sato, M.; Uwaha, M.; Saito, F.; Nagashima, K.; Sazaki, G. Step-bunching instability of growing interfaces between ice and supercooled water. Proc. Natl. Acad. Sci. USA 2022, 119, e2115955119. [Google Scholar] [CrossRef] [PubMed]
- Bales, G.S.; Zangwill, A. Morphological instability of a terrace edge during step-flow growth. Phys. Rev. B 1990, 41, 5500. [Google Scholar] [CrossRef] [PubMed]
- Kallunki, J.; Krug, J.; Kotrla, M. Competing mechanisms for step meandering in unstable growth. Phys. Rev. B 2002, 65, 205411. [Google Scholar] [CrossRef]
- Toktarbaiuly, O.; Usov, V.; Coileáin, C.Ó.; Siewierska, K.; Krasnikov, S.; Norton, E.; Bozhko, S.I.; Semenov, V.N.; Chaika, A.N.; Murphy, B.E.; et al. Step bunching with both directions of the current: Vicinal W (110) surfaces versus atomistic-scale model. Phys. Rev. B 2018, 97, 035436. [Google Scholar] [CrossRef]
- Latyshev, A.V.; Litvin, L.V.; Aseev, A.L. Peculiarities of step bunching on Si (001) surface induced by DC heating. Appl. Surf. Sci. 1998, 130, 139–145. [Google Scholar] [CrossRef]
- Guin, L.; Jabbour, M.E.; Triantafyllidis, N. Revisiting step instabilities on crystal surfaces. Part I: The quasistatic approximation. J. Mech. Phys. Solids 2021, 156, 104574. [Google Scholar] [CrossRef]
- Hong, W.; Suo, Z.; Zhang, Z. Dynamics of terraces on a silicon surface due to the combined action of strain and electric current. J. Mech. Phys. Solids 2008, 56, 267–278. [Google Scholar] [CrossRef]
- Van der Hoek, B.; Van Der Eerden, J.P.; Bennema, P.; Sunagawa, I. The influence of stress on spiral growth. J. Cryst. Growth 1982, 58, 365–380. [Google Scholar] [CrossRef]
- Cabrera, N.; Levine, M.M. On the dislocation theory of evaporation of crystals. Philos. Mag. 1956, 1, 450–458. [Google Scholar] [CrossRef]
- Sato, M. Effect of the Surface Diffusion and Evaporation of Impurities on Step Bunching Induced by Impurities. J. Phys. Soc. Jpn. 2019, 88, 114801. [Google Scholar] [CrossRef]
- Kosolobov, S. Subsurface diffusion in crystals and effect of surface permeability on the atomic step motion. Sci. Rep. 2019, 9, 13428. [Google Scholar] [CrossRef] [PubMed]
- Sitnikov, S.V.; Latyshev, A.V.; Kosolobov, S.S. Advacancy-mediated atomic steps kinetics and two-dimensional negative island nucleation on ultra-flat Si (111) surface. J. Cryst. Growth 2017, 457, 196–201. [Google Scholar] [CrossRef]
- Meca, E.; Lowengrub, J.; Kim, H.; Mattevi, C.; Shenoy, V.B. Epitaxial graphene growth and shape dynamics on copper: Phase-field modeling and experiments. Nano Lett. 2013, 13, 5692–5697. [Google Scholar] [CrossRef] [PubMed]
- Ju, G.; Xu, D.; Thompson, C.; Highland, M.J.; Eastman, J.A.; Walkosz, W.; Zapol, P.; Stephenson, G.B. Burton-Cabrera-Frank theory for surfaces with alternating step types. Phys. Rev. B 2022, 105, 054312. [Google Scholar] [CrossRef]
- Makoveeva, E.V.; Koroznikova, I.E.; Glebova, A.E.; Alexandrov, D.V. Morphological/dynamic instability of directional crystallization in a finite domain with intense convection. Crystals 2023, 13, 1276. [Google Scholar] [CrossRef]
- Guin, L.; Jabbour, M.E.; Shaabani-Ardali, L.; Triantafyllidis, N. Revisiting step instabilities on crystal surfaces. Part II: General theory. J. Mech. Phys. Solids 2021, 156, 104582. [Google Scholar] [CrossRef]
- Politi, P.; Grenet, G.; Marty, A.; Ponchet, A.; Villain, J. Instabilities in crystal growth by atomic or molecular beams. Phys. Rep. 2000, 324, 271–404. [Google Scholar] [CrossRef]
- Uwaha, M. Introduction to the BCF theory. Prog. Cryst. Growth Charact. Mater. 2016, 62, 58–68. [Google Scholar] [CrossRef]
- Markov, I.V. Crystal Growth for Beginners: Fundamentals of Nucleation, Crystal Growth and Epitaxy; World Scientific: Singapore, 2016; 564p. [Google Scholar]
- Sangwal, K. Effects of impurities on crystal growth processes. Prog. Cryst. Growth Charact. Mater. 1996, 32, 3–43. [Google Scholar] [CrossRef]
- Vekilov, P.G. What Determines the Rate of Growth of Crystals from Solution? Cryst. Growth Des. 2007, 7, 2796–2810. [Google Scholar] [CrossRef]
- Dubrovskii, V.G. Nucleation Theory and Growth of Nanostructures; Springer: Berlin, Germany, 2014; 601p. [Google Scholar]
- Libbrecht, K.G. The physics of snow crystals. Rep. Prog. Phys. 2005, 68, 855. [Google Scholar] [CrossRef]
- Kossel, W. Zur Theorie des Kristallwachstums. Nachrichten Ges. Wiss. Göttingen Math. Phys. Kl. 1927, 1927, 135–143. [Google Scholar]
- Kour, R.; Arya, S.; Verma, S.; Singh, A.; Mahajan, P.; Khosla, A. Recent advances and challenges in indium gallium nitride (InxGa1-xN) materials for solid state lighting. ECS J. Solid State Sci. Technol. 2019, 9, 015011. [Google Scholar] [CrossRef]
- Langpoklakpam, C.; Liu, A.-C.; Chu, K.-H.; Hsu, L.-H.; Lee, W.-C.; Chen, S.-C.; Sun, C.-W.; Shih, M.-H.; Lee, K.-Y.; Kuo, H.-C. Review of Silicon Carbide Processing for Power MOSFET. Crystals 2022, 12, 245. [Google Scholar] [CrossRef]
- Wu, P.; Funato, M.; Kawakami, Y. Environmentally friendly method to grow wide-bandgap semiconductor aluminum nitride crystals: Elementary source vapor phase epitaxy. Sci. Rep. 2015, 5, 17405. [Google Scholar] [CrossRef]
- Kim, M.; Seo, J.H.; Singisetti, U.; Ma, Z. Recent advances in free-standing single crystalline wide band-gap semiconductors and their applications: GaN, SiC, ZnO, β-Ga2O3, and diamond. J. Mater. Chem. C 2017, 5, 8338–8354. [Google Scholar] [CrossRef]
- Wang, Q.; Astruc, D. State of the art and prospects in metal-organic framework (MOF)-based and MOF-derived nanocatalysis. Chem. Rev. 2019, 120, 1438–1511. [Google Scholar] [CrossRef]
- Emam, H.E.; Abdelhameed, R.M.; Ahmed, H.B. Adsorptive performance of MOFs and MOF containing composites for clean energy and safe environment. J. Environ. Chem. Eng. 2020, 8, 104386. [Google Scholar] [CrossRef]
- Voloshin, A.; Rudneva, E.; Manomenova, V.; Vasilyeva, N.; Kovalev, S.; Emelchenko, G.; Masalov, V.; Zhokhov, A. The problem of formation of mixed crystals and high-efficiency K2 (co, ni)(SO4)2• 6H2O optical filters. Crystals 2019, 9, 390. [Google Scholar] [CrossRef]
- Ansari, M.I.H.; Qurashi, A.; Nazeeruddin, M.K. Frontiers, opportunities, and challenges in perovskite solar cells: A critical review. J. Photochem. Photobiol. C Photochem. Rev. 2018, 35, 1–24. [Google Scholar] [CrossRef]
- Karimi-Jafari, M.; Padrela, L.; Walker, G.M.; Croker, D.M. Creating cocrystals: A review of pharmaceutical cocrystal preparation routes and applications. Cryst. Growth Des. 2018, 18, 6370–6387. [Google Scholar] [CrossRef]
- Redkov, A.V.; Kukushkin, S.A. Dynamic interaction of steps and nanoislands during growth of a multicomponent crystal. Cryst. Growth Des. 2021, 21, 4914–4926. [Google Scholar] [CrossRef]
- Tilbury, C.J.; Joswiak, M.N.; Peters, B.; Doherty, M.F. Modeling Step Velocities and Edge Surface Structures during Growth of Non-Centrosymmetric Crystals. Cryst. Growth Des. 2017, 17, 2066–2080. [Google Scholar] [CrossRef]
- Cermelli, P.; Jabbour, M. Multispecies epitaxial growth on vicinal surfaces with chemical reactions and diffusion. Proc. R. Soc. A 2005, 461, 3483–3504. [Google Scholar] [CrossRef]
- Redkov, A. Spiral growth of multicomponent crystals: Theoretical aspects. Front. Chem. 2023, 11, 1189729. [Google Scholar] [CrossRef] [PubMed]
- Cermelli, P.; Jabbour, M.E. Step bunching during the epitaxial growth of a generic binary-compound thin film. J. Mech. Phys. Solids 2010, 58, 810–827. [Google Scholar] [CrossRef]
- Redkov, A.V.; Kukushkin, S.A. Development of Burton–Cabrera–Frank theory for the growth of a non-Kossel crystal via chemical reaction. Cryst. Growth Des. 2020, 20, 2590–2601. [Google Scholar] [CrossRef]
- Redkov, A.; Kukushkin, S. Theoretical aspects of the growth of a non-Kossel crystal from vapours: The role of advacancies. Faraday Discuss. 2022, 235, 362–382. [Google Scholar] [CrossRef]
- Redkov, A.; Kukushkin, S. Influence of the kinetics of atomic steps on the growth of multicomponent crystals under conditions of increased supersaturation. Tech. Phys. Lett. 2023, 13, 35. (In Russian) [Google Scholar] [CrossRef]
- Schwoebel, R.L.; Shipsey, E.J. Step motion on crystal surfaces. J. Appl. Phys. 1966, 37, 3682–3686. [Google Scholar] [CrossRef]
- Schwoebel, R.L. Step Motion on Crystal Surfaces. II. J. Appl. Phys. 1969, 40, 614–618. [Google Scholar] [CrossRef]
- Paulin, S.; Gillet, F.; Pierre-Louis, O.; Misbah, C. Unstable step meandering with elastic interactions. Phys. Rev. Lett. 2001, 86, 5538. [Google Scholar] [CrossRef] [PubMed]
- Pimpinelli, A.; Elkinani, I.; Karma, A.; Misbah, C.; Villain, J. Step motions on high-temperature vicinal surfaces. J. Phys. Condens. Matter 1994, 6, 2661. [Google Scholar] [CrossRef]
- Tonchev, V.; Ranguelov, B.; Omi, H.; Pimpinelli, A. Scaling and universality in models of step bunching: The “C+–C−” model. Eur. Phys. J. B 2010, 73, 539–546. [Google Scholar] [CrossRef]
- Saúl, A.; Métois, J.J.; Ranguis, A. Experimental evidence for an Ehrlich-Schwoebel effect on Si (111). Phys. Rev. B 2002, 65, 075409. [Google Scholar] [CrossRef]
- Rabbering, F.; Wormeester, H.; Everts, F.; Poelsema, B. Quantitative understanding of the growth of Cu/Cu (001) including the determination of the Ehrlich-Schwoebel barrier at straight steps and kinks. Phys. Rev. B 2009, 79, 075402. [Google Scholar] [CrossRef]
- ZaŁuska–Kotur, M.A.; Krzyżewski, F.; Krukowski, S. Emergence of regular meandered step structure in simulated growth of GaN (0001) surface. J. Cryst. Growth 2012, 343, 138–144. [Google Scholar] [CrossRef]
- Bellmann, K.; Pohl, U.W.; Kuhn, C.; Wernicke, T.; Kneissl, M. Controlling the morphology transition between step-flow growth and step-bunching growth. J. Cryst. Growth 2017, 478, 187–192. [Google Scholar] [CrossRef]
- Redkov, A.V.; Kukushkin, S.A.; Osipov, A.V. Growth of faceted pores in a multi-component crystal by applying mechanical stress. CrystEngComm 2020, 22, 5280–5288. [Google Scholar] [CrossRef]
- Redkov, A.V.; Kukushkin, S.A.; Osipov, A.V. Spiral growth of a multicomponent crystal from vapor of its components. J. Cryst. Growth 2020, 548, 125845. [Google Scholar] [CrossRef]
- Kukushkin, S.A.; Osipov, A.V.; Redkov, A.V. Morphological stability criterion for a spherical crystallization front in a multicomponent system with chemical reactions. Phys. Solid State 2014, 56, 2530–2536. [Google Scholar] [CrossRef]
- Chernov, A.A. The spiral growth of crystals. Sov. Phys. Uspekhi 1961, 4, 116. [Google Scholar] [CrossRef]
- Gilmer, G.H.; Ghez, R.; Cabrera, N. An analysis of combined surface and volume diffusion processes in crystal growth. J. Cryst. Growth 1971, 15, 123–128. [Google Scholar] [CrossRef]
- Van Der Eerden, J.P. The advance velocity of steps under the influence of volume and surface diffusion, by direct and indirect incorporation of growth units. J. Cryst. Growth 1982, 56, 174–188. [Google Scholar] [CrossRef]
- Sato, M.; Uwaha, M.; Saito, Y. Instabilities of steps induced by the drift of adatoms and effect of the step permeability. Phys. Rev. B 2000, 62, 8452. [Google Scholar] [CrossRef]
- Kukushkin, S.A. Evolution processes in multicomponent and multiphase films. Thin Solid Film. 1992, 207, 302–312. [Google Scholar] [CrossRef]
- Heying, B.; Smorchkova, I.; Poblenz, C.; Elsass, C.; Fini, P.; Den Baars, S.; Mishra, U.; Speck, J.S. Optimization of the surface morphologies and electron mobilities in GaN grown by plasma-assisted molecular beam epitaxy. Appl. Phys. Lett. 2000, 77, 2885–2887. [Google Scholar] [CrossRef]
- Chen, Y.S.; Liao, C.H.; Kuo, C.T.; Tsiang, R.C.C.; Wang, H.C. Indium droplet formation in InGaN thin films with single and double heterojunctions prepared by MOCVD. Nanoscale Res. Lett. 2014, 9, 334. [Google Scholar] [CrossRef]
- Chugh, M.; Ranganathan, M. Adsorbate interactions on the GaN (0001) surface and their effect on diffusion barriers and growth morphology. Phys. Chem. Chem. Phys. 2017, 19, 2111–2123. [Google Scholar] [CrossRef]



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Redkov, A. Impact of Schwoebel Barriers on the Step-Flow Growth of a Multicomponent Crystal. Crystals 2024, 14, 25. https://doi.org/10.3390/cryst14010025
Redkov A. Impact of Schwoebel Barriers on the Step-Flow Growth of a Multicomponent Crystal. Crystals. 2024; 14(1):25. https://doi.org/10.3390/cryst14010025
Chicago/Turabian StyleRedkov, Alexey. 2024. "Impact of Schwoebel Barriers on the Step-Flow Growth of a Multicomponent Crystal" Crystals 14, no. 1: 25. https://doi.org/10.3390/cryst14010025
APA StyleRedkov, A. (2024). Impact of Schwoebel Barriers on the Step-Flow Growth of a Multicomponent Crystal. Crystals, 14(1), 25. https://doi.org/10.3390/cryst14010025





