Customized Design of Aperiodic Liquid Crystal Grating for Generation of Multiple Optical Patterns
Abstract
:1. Introduction
2. Theory and Methodology
2.1. Design Principle of LC Gratings Based on PB Phase
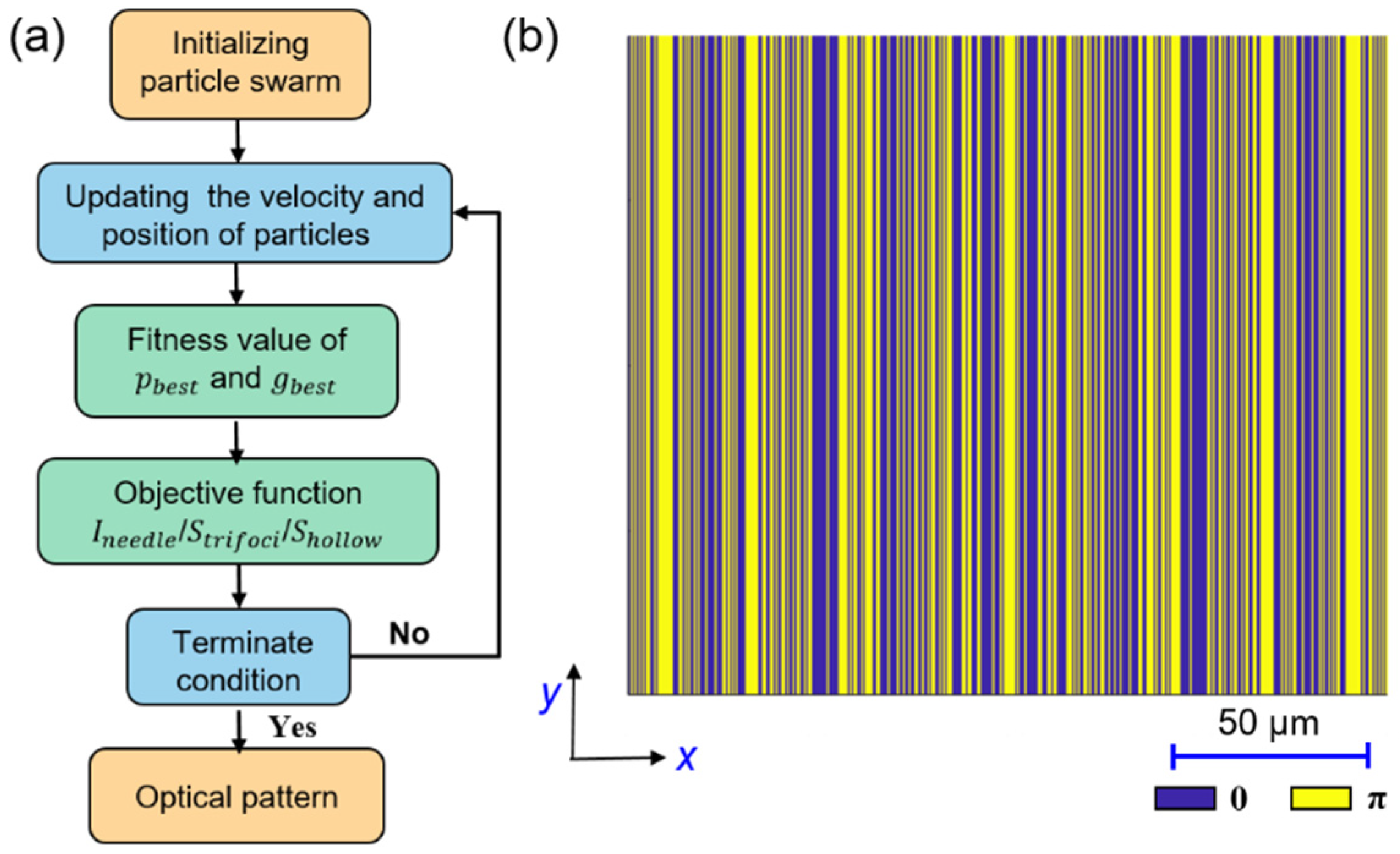
2.2. The Binary Particle Swarm Optimization Algorithm
3. Results and Discussion
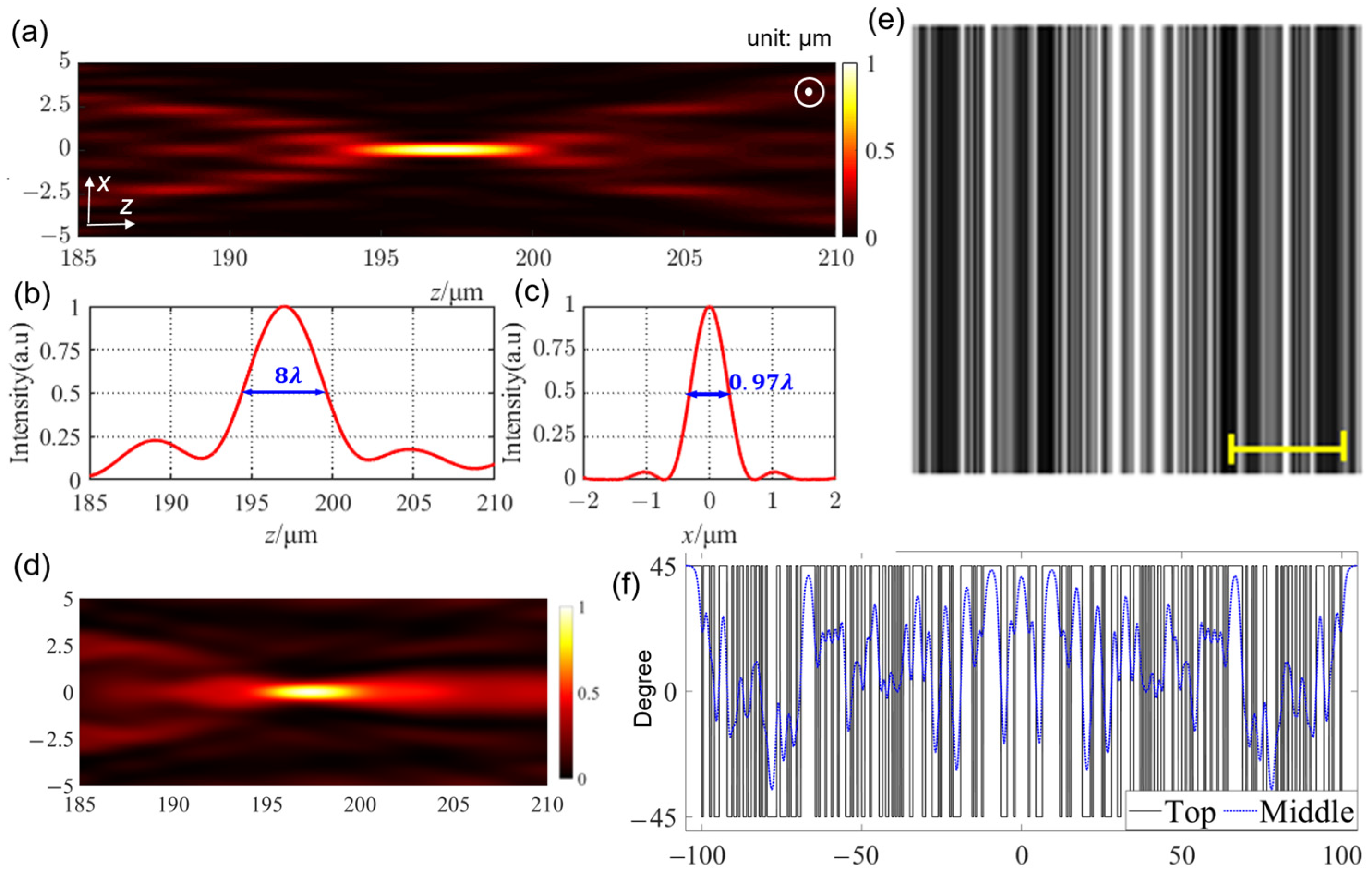
3.1. LC Grating for Needle Beam
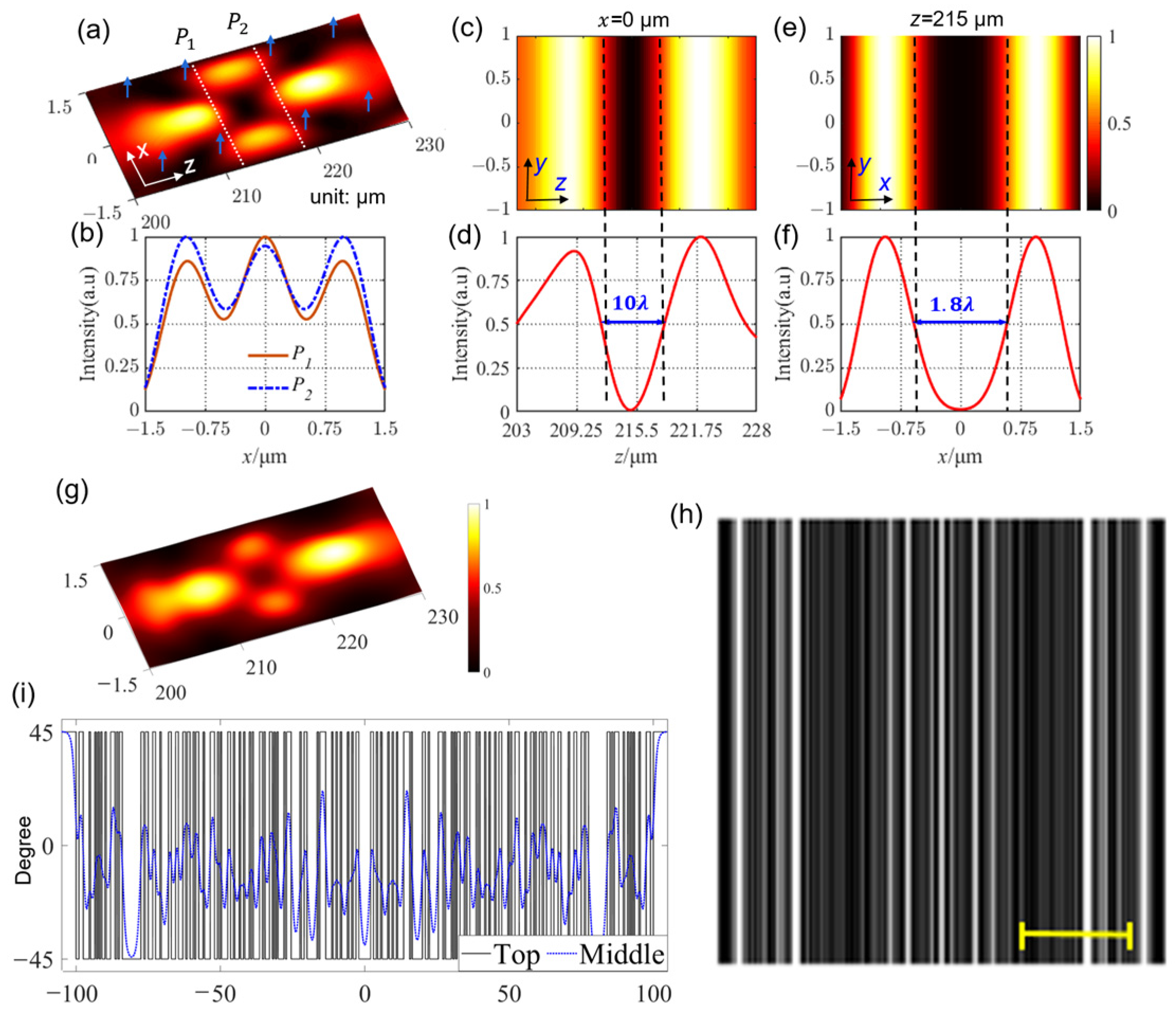
3.2. LC Grating for Linear Multi-Foci
3.3. LC Grating for Hollow Beam
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Yuan, G.; Zhelude, N.I. Detecting nanometric displacements with optical ruler metrology. Science 2019, 364, 771–775. [Google Scholar] [CrossRef]
- Yang, Z.; Albrow-Owen, T.; Cai, W.; Hasan, T. Miniaturization of optical spectrometers. Science 2021, 371, 480. [Google Scholar] [CrossRef]
- Field, J.W.; Berry, S.A.; Bannerman, R.H.S.; Smith, D.H.; Gawith, C.B.E.; Smith, P.G.R.; Gates, J.C. Highly-chirped bragg gratings for integrated silica spectrometers. Opt. Express 2020, 28, 21247. [Google Scholar] [CrossRef]
- Hua, J.; Hua, E.; Zhou, F.; Shi, J.; Wang, C.; Duan, H.; Hu, Y.; Qiao, W.; Chen, L. Foveated glasses-free 3D display with ultrawide field of view via a large-scale 2D-metagrating complex. Light Sci. Appl. 2021, 10, 213. [Google Scholar] [CrossRef]
- Wan, W.; Qiao, W.; Pu, D.; Li, R.; Wang, C.; Hu, Y.; Duan, H.; Guo, L.; Chen, L. Holographic sampling display based on metagratings. iScience 2020, 23, 100773. [Google Scholar] [CrossRef]
- Doneus, M.; Doneus, N.; Briese, C.; Pregesbauer, M.; Mandlburger, G.; Verhoeven, G. Airborne laser bathymetry—Detecting and recording submerged archaeological sites from the air. J. Archaeol. Sci. 2013, 40, 2136–2151. [Google Scholar] [CrossRef]
- Mizuno, K.; Ono, S.; Shimoe, O. Interaction between coherent light waves and free electrons with a reflection grating. Nature 1975, 253, 184–185. [Google Scholar] [CrossRef]
- Wang, Y.; Wang, X.; Flueckiger, J.; Yun, H.; Shi, W.; Bojko, R.; Jaeger, N.A.; Chrostowski, L. Focusing sub-wavelength grating couplers with low back reflections for rapid prototyping of silicon photonic circuits. Opt. Express 2014, 22, 20652–20662. [Google Scholar] [CrossRef]
- Chen, G.; Zhang, K.; Yu, A.; Wang, X.; Zhang, Z.; Li, Y.; Wen, Z.; Li, C.; Dai, L.; Jiang, S.; et al. Far-field sub-diffraction focusing lens based on binary amplitude-phase mask for linearly polarized light. Opt. Express 2016, 24, 11002–11008. [Google Scholar] [CrossRef]
- Rochon, P.; Batalla, E.; Natansohn, A. Optically induced surface gratings on azoaromatic polymer films. Appl. Phys. Lett. 1995, 66, 136–138. [Google Scholar] [CrossRef]
- Kumar, J.; Li, L.; Jiang, X.L.; Kim, D.; Lee, T.S.; Tripathy, S. Gradient force: The mechanism for surface relief grating formation in azo benzene functionalized polymers. Appl. Phys. Lett. 1998, 77, 2096–2098. [Google Scholar] [CrossRef]
- Heitmann, D.; Pole, R.V. Two-dimensional focusing holographic grating coupler. Appl. Phys. Lett. 1980, 37, 585–587. [Google Scholar] [CrossRef]
- Piazzolla, S.; Jenkins, B.K. Holographic grating formation in photopolymers. Opt. Lett. 1996, 21, 1075–1077. [Google Scholar] [CrossRef]
- Zhou, Q.; Zhang, J.; Ren, X.; Xu, Z.; Liu, X. Multi-bottle beam generation using acoustic holographic lens. Appl. Phys. Lett. 2020, 116, 133502. [Google Scholar] [CrossRef]
- Oscurato, S.L.; Reda, F.; Salvatore, M.; Borbone, F.; Maddalena, P.; Ambrosio, A. Large-scale multiplexed azopolymer gratings with engineered diffraction behavior. Adv. Mater. Interfaces 2021, 8, 2101375. [Google Scholar] [CrossRef]
- Carroll, T.O. Liquid-crystal diffraction grating. Appl. Phys. Lett. 1972, 43, 767–770. [Google Scholar] [CrossRef]
- Bryan-Brown, G.P.; Brown, C.V.; Sage, I.C.; Hui, V.C. Voltage-dependent anchoring of a nematic LC on a grating surface. Nature 1998, 392, 365–367. [Google Scholar] [CrossRef]
- Chen, H.; Tan, G.; Huang, Y.; Weng, Y.; Choi, T.H.; Yoon, T.H.; Wu, S.T. A low voltage LC phase grating with switchable diffraction angles. Sci. Rep. 2017, 7, 39923. [Google Scholar] [CrossRef]
- Katayama, K.; Kato, D.; Nagasaka, K.I.; Miyagawa, M.; Sohn, W.Y.; Lee, K.W. Origin of optical nonlinearity of photo-responsive liquid crystals revealed by transient grating imaging. Sci. Rep. 2019, 9, 5754. [Google Scholar] [CrossRef]
- Shin, Y.; Jiang, Y.; Wang, Q.; Zhou, Z.; Qin, G.; Yang, D.K. Flexoelectric-effect-based light waveguide liquid crystal display for transparent display. Photon. Res. 2022, 10, 407–414. [Google Scholar] [CrossRef]
- Sung, G.F.; Wu, P.C.; Zyryanov, V.Y.; Lee, W. Electrically active and thermally passive liquid-crystal device toward smart glass. Photon. Res. 2021, 9, 2288–2295. [Google Scholar] [CrossRef]
- Lu, X.; Li, X.; Guo, Y.; Pu, M.; Wang, J.; Zhang, Y.; Li, X.; Ma, X.; Luo, X. Broadband high-efficiency polymerized liquid crystal metasurfaces with spin-multiplexed functionalities in the visible. Photon. Res. 2022, 10, 1380–1393. [Google Scholar] [CrossRef]
- Chiang, W.; Silalahi, H.; Chiang, Y.C.; Hsu, M.C.; Zhang, Y.S.; Liu, J.H.; Yu, Y.; Lee, C.R.; Huang, C.Y. Continuously tunable intensity modulators with large switching contrasts using liquid crystal elastomer films that are deposited with terahertz metamaterials. Opt. Express 2020, 28, 27676–27687. [Google Scholar] [CrossRef] [PubMed]
- Chiang, W.F.; Lu, Y.Y.; Chen, Y.P.; Lin, X.Y.; Lim, T.S.; Liu, J.H.; Lee, C.R.; Huang, C.Y. Passively tunable terahertz filters using liquid crystal cells coated with metamaterials. Coatings 2021, 11, 381. [Google Scholar] [CrossRef]
- Culbreath, C.; Glazar, N.; Yokoyama, H. Note: Automated maskless micro-multidomain photoalignment. Rev. Sci. Instrum. 2011, 82, 126107. [Google Scholar] [CrossRef]
- Wei, B.; Hu, W.; Ming, Y.; Xu, F.; Rubin, S.; Wang, J.; Chigrinov, V.; Lu, Y. Generating switchable and reconfigurable optical vortices via photopatterning of liquid crystals. Adv. Mater. 2014, 26, 1590–1595. [Google Scholar] [CrossRef] [PubMed]
- Guo, Y.; Jiang, M.; Peng, C.; Sun, K.; Yaroshchuk, O.; Lavrentovich, O.; Wei, Q. High-resolution and high-throughput plasmonic photopatterning of complex molecular orientations in liquid crystals. Adv. Mater. 2016, 28, 2353–2358. [Google Scholar] [CrossRef]
- Jiang, M.; Yu, H.; Feng, X.; Guo, Y.; Chaganava, I.; Turiv, T.; Lavrentovich, O.D.; Wei, Q. Liquid crystal Pancharatnam—Berry micro-optical elements for laser beam shaping. Adv. Opt. Mater. 2018, 6, 1800961. [Google Scholar] [CrossRef]
- Chen, K.; Hou, Y.; Chen, S.; Yuan, D.; Ye, H.; Zhou, G. Design, fabrication, and applications of liquid crystal microlenses. Adv. Opt. Mater. 2021, 9, 2100370. [Google Scholar] [CrossRef]
- Kobashi, J.; Yoshida, H.; Ozaki, M. Planar optics with patterned chiral liquid crystals. Nat. Photon. 2016, 10, 389. [Google Scholar] [CrossRef]
- Murray, B.S.; Pelcovits, R.A.; Rosenblatt, C. Creating arbitrary arrays of two-dimensional topological defects. Phys. Rev. E 2014, 90, 52501. [Google Scholar] [CrossRef]
- Wu, H.; Hu, W.; Hu, H.; Lin, X.; Zhu, G.; Choi, J.; Chigrinov, V.; Lu, Y. Arbitrary photo-patterning in liquid crystal alignments using DMD based lithography system. Opt. Express 2012, 20, 3038. [Google Scholar] [CrossRef]
- McConney, M.E.; Martinez, A.; Tondiglia, V.P.; Lee, K.M.; Langley, D.; Smalyukh, I.I.; White, T.J. Topography from topology: Photoinduced surface features generated in liquid crystal polymer networks. Adv. Mater. 2013, 25, 5880. [Google Scholar] [CrossRef] [PubMed]
- Ye, H.; Sun, Q.; Guo, Z.; Hou, Y.; Wen, F.; Yuan, D.; Qin, F.; Zhou, G. Theoretical realization of single-mode fiber integrated metalens for beam collimating. Opt. Express 2021, 29, 27521. [Google Scholar] [CrossRef] [PubMed]
- Xie, Y.; Quan, J.; Shi, Q.; Cao, Y.; Sun, B.; Xu, Y. Multi-functional high-efficiency light beam splitter based on metagrating. Opt. Express 2022, 30, 4125. [Google Scholar] [CrossRef] [PubMed]
- Khanesar, M.A.; Teshnehlab, M.; Shoorehdeli, M.A. A Novel Binary Particle Swarm Optimization. In Proceedings of the 2007 Mediterranean Conference on Control & Automation, Athens, Greece, 27–29 June 2007. [Google Scholar]
- Su, M.; Yang, B.; Liu, J.; Ye, H.; Zhou, X.; Xiao, J.; Li, Y.; Chen, S.; Fan, D. Broadband graphene-on-silicon modulator with orthogonal hybrid plasmonic waveguides. Nanophotonics 2020, 9, 1529. [Google Scholar] [CrossRef]
- Ma, W.; Liu, Z.; Kudyshev, Z.A.; Boltasseva, A.; Cai, W.; Liu, Y. Deep learning for the design of photonic structures. Nat. Photon. 2021, 15, 77. [Google Scholar] [CrossRef]
- Younes, R.; Dimitrios, L.S.; Andrea, A. Metagratings: Beyond the limits of Graded Metasurfaces for Wave Front Control. Phys. Rev. Lett. 2017, 119, 067404. [Google Scholar]
- Wang, H.; Hao, C.; Lin, H.; Wang, Y.; Lan, T.; Qiu, C.; Jia, B. Generation of super-resolved optical needle and multifocal array using graphene oxide metalenses. Opto-Electron. Adv. 2021, 2, 4. [Google Scholar] [CrossRef]
- Ye, H.; Qiu, C.-W.; Huang, K.; Teng, J.; Luk’yanchuk, B.; Yeo, S.P. Creation of longitudinally polarized subwavelength hotspot with ultra-thin planar lens: Vectorial Rayleigh Sommerfeld method. Laser Phys. Lett. 2013, 10, 065004. [Google Scholar] [CrossRef]
- Rogers, E.T.F.; Lindberg, J.; Roy, T.; Savo, S.; Chad, J.E.; Dennis, M.R.; Zheludev, N.I. A super-oscillatory lens optical microscope for subwavelength imaging. Nat. Mater. 2012, 11, 432–435. [Google Scholar] [CrossRef]
- Xiong, J.; Chen, R.; Wu, S.T. Device simulation of liquid crystal polarization gratings. Opt. Express 2019, 27, 18102. [Google Scholar] [CrossRef]
- Ye, H.; Huang, K.; Liu, H.; Wen, F.; Jin, Z.; Teng, J.; Qiu, C. Intrinsically shaping the focal behavior with multi-ring Bessel-Gaussian beam. Appl. Phys. Lett. 2017, 111, 031103. [Google Scholar] [CrossRef]
- Shvedov, V.G.; Rode, A.V.; Izdebskaya, Y.V.; Desyatnikov, A.S.; Krolikowski, W.; Kivshar, Y.S. Giant optical manipulation. Phys. Rev. Lett. 2010, 105, 118103. [Google Scholar] [CrossRef]


| Number | Position (μm) | Number | Position (μm) | Number | Position (μm) | Number | Position (μm) | Number | Position (μm) | |||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Inner | Outer | Inner | Outer | Inner | Outer | Inner | Outer | Inner | Outer | |||||
| 1 | 0 | 2 | 19 | 28 | 30 | 37 | 47.5 | 49 | 55 | 64.5 | 69 | 73 | 85.5 | 86 |
| 2 | 2 | 2.5 | 20 | 30 | 31 | 38 | 49 | 50 | 56 | 69 | 70 | 74 | 86 | 87 |
| 3 | 2.5 | 4.5 | 21 | 31 | 34 | 39 | 50 | 51 | 57 | 70 | 70.5 | 75 | 87 | 88 |
| 4 | 4.5 | 6.5 | 22 | 34 | 35 | 40 | 51 | 51.5 | 58 | 70.5 | 72 | 76 | 88 | 88.5 |
| 5 | 6.5 | 12 | 23 | 35 | 37 | 41 | 51.5 | 52.5 | 59 | 72 | 72.5 | 77 | 88.5 | 89 |
| 6 | 12 | 12.5 | 24 | 37 | 37.5 | 42 | 52.5 | 53 | 60 | 72.5 | 73 | 78 | 89 | 90 |
| 7 | 12.5 | 14 | 25 | 37.5 | 38 | 43 | 53 | 53.5 | 61 | 73 | 73.5 | 79 | 90 | 90.5 |
| 8 | 14 | 14.5 | 26 | 38 | 38.5 | 44 | 53.5 | 55 | 62 | 73.5 | 75.5 | 80 | 90.5 | 92 |
| 9 | 14.5 | 15 | 27 | 38.5 | 39 | 45 | 55 | 57 | 63 | 75.5 | 76.5 | 81 | 92 | 94.5 |
| 10 | 15 | 15.5 | 28 | 39 | 39.5 | 46 | 57 | 57.5 | 64 | 76.5 | 79.5 | 82 | 94.5 | 96 |
| 11 | 15.5 | 19 | 29 | 39.5 | 40 | 47 | 57.5 | 59 | 65 | 79.5 | 80 | 83 | 96 | 97 |
| 12 | 19 | 21.5 | 30 | 40 | 40.5 | 48 | 59 | 59.5 | 66 | 80 | 81 | 84 | 97 | 97.5 |
| 13 | 21.5 | 22 | 31 | 40.5 | 41.5 | 49 | 59.5 | 61 | 67 | 81 | 81.5 | 85 | 97.5 | 99.5 |
| 14 | 22 | 22.5 | 32 | 41.5 | 42.5 | 50 | 61 | 61.5 | 68 | 81.5 | 82 | 86 | 99.5 | 100 |
| 15 | 22.5 | 25 | 33 | 42.5 | 43.5 | 51 | 61.5 | 63 | 69 | 82 | 83 | |||
| 16 | 25 | 25.5 | 34 | 43.5 | 44.5 | 52 | 63 | 63.5 | 70 | 83 | 83.5 | |||
| 17 | 25.5 | 26 | 35 | 44.5 | 47 | 53 | 63.5 | 64 | 71 | 83.5 | 84.5 | |||
| 18 | 26 | 28 | 36 | 47 | 47.5 | 54 | 64 | 64.5 | 72 | 84.5 | 85.5 | |||
| Number | Position (μm) | Number | Position (μm) | Number | Position (μm) | Number | Position (μm) | Number | Position (μm) | |||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Inner | Outer | Inner | Outer | Inner | Outer | Inner | Outer | Inner | Outer | |||||
| 1 | 0 | 1.5 | 23 | 20 | 20.5 | 45 | 37.5 | 38.5 | 67 | 58.5 | 59 | 89 | 78 | 78.5 |
| 2 | 1.5 | 2.5 | 24 | 20.5 | 21.5 | 46 | 38.5 | 39.5 | 68 | 59 | 59.5 | 90 | 78.5 | 80.5 |
| 3 | 2.5 | 3.5 | 25 | 21.5 | 22 | 47 | 39.5 | 40 | 69 | 59.5 | 61 | 91 | 80.5 | 82 |
| 4 | 3.5 | 5.5 | 26 | 22 | 24 | 48 | 40 | 40.5 | 70 | 61 | 61.5 | 92 | 82 | 83.5 |
| 5 | 5.5 | 6 | 27 | 24 | 24.5 | 49 | 40.5 | 41 | 71 | 61.5 | 62 | 93 | 83.5 | 84.5 |
| 6 | 6 | 6.5 | 28 | 24.5 | 25.5 | 50 | 41 | 41.5 | 72 | 62 | 62.5 | 94 | 84.5 | 86 |
| 7 | 6.5 | 7 | 29 | 25.5 | 26 | 51 | 41.5 | 42.5 | 73 | 62.5 | 63 | 95 | 86 | 87 |
| 8 | 7 | 8 | 30 | 26 | 26.5 | 52 | 42.5 | 43.5 | 74 | 63 | 67.5 | 96 | 87 | 88.5 |
| 9 | 8 | 8.5 | 31 | 26.5 | 28 | 53 | 43.5 | 45 | 75 | 67.5 | 68 | 97 | 88.5 | 89 |
| 10 | 8.5 | 9.5 | 32 | 28 | 29 | 54 | 45 | 46.5 | 76 | 68 | 68.5 | 98 | 89 | 92.5 |
| 11 | 9.5 | 10 | 33 | 29 | 30.5 | 55 | 46.5 | 48 | 77 | 68.5 | 69 | 99 | 92.5 | 93 |
| 12 | 10 | 11 | 34 | 30.5 | 31 | 56 | 48 | 49 | 78 | 69 | 70 | 100 | 93 | 94.5 |
| 13 | 11 | 12 | 35 | 31 | 32 | 57 | 49 | 49.5 | 79 | 70 | 70.5 | 101 | 94.5 | 95.5 |
| 14 | 12 | 12.5 | 36 | 32 | 32.5 | 58 | 49.5 | 50.5 | 80 | 70.5 | 72 | 102 | 95.5 | 96 |
| 15 | 12.5 | 13.5 | 37 | 32.5 | 33 | 59 | 50.5 | 52 | 81 | 72 | 72.5 | 103 | 96 | 96.5 |
| 16 | 13.5 | 16.5 | 38 | 33 | 33.5 | 60 | 52 | 53.5 | 82 | 72.5 | 74.5 | 104 | 96.5 | 97 |
| 17 | 16.5 | 17 | 39 | 33.5 | 34 | 61 | 53.5 | 55 | 83 | 74.5 | 75 | 105 | 97 | 97.5 |
| 18 | 17 | 17.5 | 40 | 34 | 34.5 | 62 | 55 | 55.5 | 84 | 75 | 75.5 | 106 | 97.5 | 98 |
| 19 | 17.5 | 18 | 41 | 34.5 | 35 | 63 | 55.5 | 56 | 85 | 75.5 | 76.5 | 107 | 98 | 98.5 |
| 20 | 18 | 18.5 | 42 | 35 | 36.5 | 64 | 56 | 57 | 86 | 76.5 | 77 | 108 | 98.5 | 99 |
| 21 | 18.5 | 19 | 43 | 36.5 | 37 | 65 | 57 | 57.5 | 87 | 77 | 77.5 | 109 | 99 | 100 |
| 22 | 19 | 20 | 44 | 37 | 37.5 | 66 | 57.5 | 58.5 | 88 | 77.5 | 78 | |||
| Number | Position (μm) | Number | Position (μm) | Number | Position (μm) | Number | Position (μm) | Number | Position (μm) | |||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Inner | Outer | Inner | Outer | Inner | Outer | Inner | Outer | Inner | Outer | |||||
| 1 | 0 | 2 | 20 | 22 | 22.5 | 39 | 40 | 41.5 | 58 | 59 | 59.5 | 77 | 85 | 85.5 |
| 2 | 2 | 3 | 21 | 22.5 | 25.5 | 40 | 41.5 | 42 | 59 | 59.5 | 60.5 | 78 | 85.5 | 86 |
| 3 | 3 | 4 | 22 | 25.5 | 27 | 41 | 42 | 43 | 60 | 60.5 | 61.5 | 79 | 86 | 86.5 |
| 4 | 4 | 4.5 | 23 | 27 | 27.5 | 42 | 43 | 43.5 | 61 | 61.5 | 62 | 80 | 86.5 | 88 |
| 5 | 4.5 | 5.5 | 24 | 27.5 | 28 | 43 | 43.5 | 45 | 62 | 62 | 63 | 81 | 88 | 89.5 |
| 6 | 5.5 | 6 | 25 | 28 | 30 | 44 | 45 | 45.5 | 63 | 63 | 64.5 | 82 | 89.5 | 90 |
| 7 | 6 | 8 | 26 | 30 | 30.5 | 45 | 45.5 | 46.5 | 64 | 64.5 | 65.5 | 83 | 90 | 91 |
| 8 | 8 | 8.5 | 27 | 30.5 | 31 | 46 | 46.5 | 47.5 | 65 | 65.5 | 67 | 84 | 91 | 91.5 |
| 9 | 8.5 | 9.5 | 28 | 31 | 31.5 | 47 | 47.5 | 49.5 | 66 | 67 | 68 | 85 | 91.5 | 92 |
| 10 | 9.5 | 10 | 29 | 31.5 | 32 | 48 | 49.5 | 50 | 67 | 68 | 70.5 | 86 | 92 | 92.5 |
| 11 | 10 | 11 | 30 | 32 | 33 | 49 | 50 | 50.5 | 68 | 70.5 | 71 | 87 | 92.5 | 93 |
| 12 | 11 | 11.5 | 31 | 33 | 34.5 | 50 | 50.5 | 51 | 69 | 71 | 72.5 | 88 | 93 | 93.5 |
| 13 | 11.5 | 13.5 | 32 | 34.5 | 35 | 51 | 51 | 52 | 70 | 72.5 | 73.5 | 89 | 93.5 | 95 |
| 14 | 13.5 | 15.5 | 33 | 35 | 35.5 | 52 | 52 | 53.5 | 71 | 73.5 | 75 | 90 | 95 | 95.5 |
| 15 | 15.5 | 16 | 34 | 35.5 | 36.5 | 53 | 53.5 | 55.5 | 72 | 75 | 76 | 91 | 95.5 | 97.5 |
| 16 | 16 | 16.5 | 35 | 36.5 | 38.5 | 54 | 55.5 | 56 | 73 | 76 | 76.5 | 92 | 97.5 | 99 |
| 17 | 16.5 | 20 | 36 | 38.5 | 39 | 55 | 56 | 57.5 | 74 | 76.5 | 77.5 | 93 | 99 | 100 |
| 18 | 20 | 21 | 37 | 39 | 39.5 | 56 | 57.5 | 58.5 | 75 | 77.5 | 84 | |||
| 19 | 21 | 22 | 38 | 39.5 | 40 | 57 | 58.5 | 59 | 76 | 84 | 85 | |||
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Liu, C.; Guo, Z.; Wu, J.; Yuan, D.; Zhou, G.; Tang, B.; Ye, H. Customized Design of Aperiodic Liquid Crystal Grating for Generation of Multiple Optical Patterns. Crystals 2023, 13, 300. https://doi.org/10.3390/cryst13020300
Liu C, Guo Z, Wu J, Yuan D, Zhou G, Tang B, Ye H. Customized Design of Aperiodic Liquid Crystal Grating for Generation of Multiple Optical Patterns. Crystals. 2023; 13(2):300. https://doi.org/10.3390/cryst13020300
Chicago/Turabian StyleLiu, Chen, Zhenghao Guo, Jun Wu, Dong Yuan, Guofu Zhou, Biao Tang, and Huapeng Ye. 2023. "Customized Design of Aperiodic Liquid Crystal Grating for Generation of Multiple Optical Patterns" Crystals 13, no. 2: 300. https://doi.org/10.3390/cryst13020300
APA StyleLiu, C., Guo, Z., Wu, J., Yuan, D., Zhou, G., Tang, B., & Ye, H. (2023). Customized Design of Aperiodic Liquid Crystal Grating for Generation of Multiple Optical Patterns. Crystals, 13(2), 300. https://doi.org/10.3390/cryst13020300









