Microtubules as One-Dimensional Crystals: Is Crystal-Like Structure the Key to the Information Processing of Living Systems?
Abstract
1. Introduction
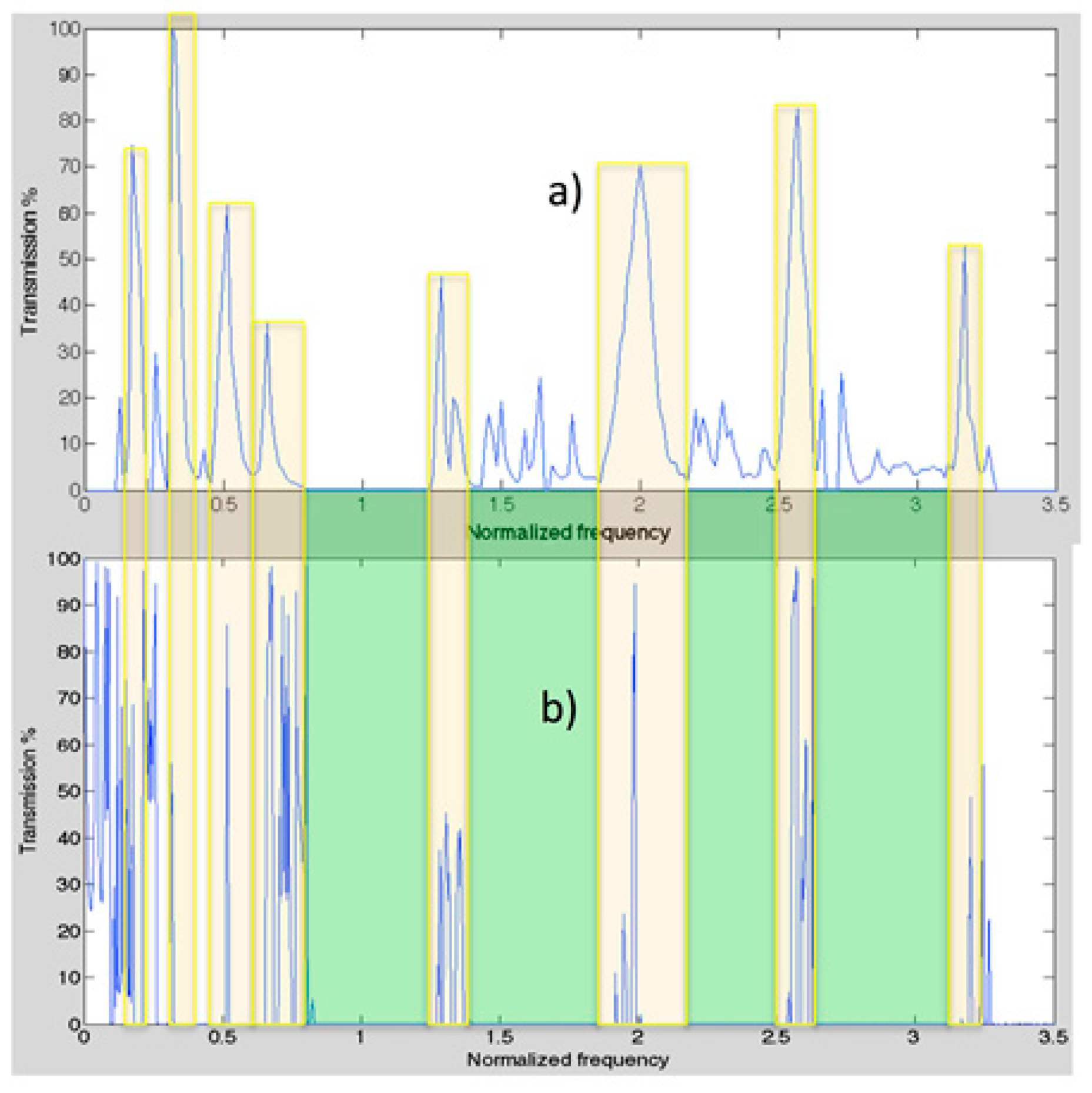
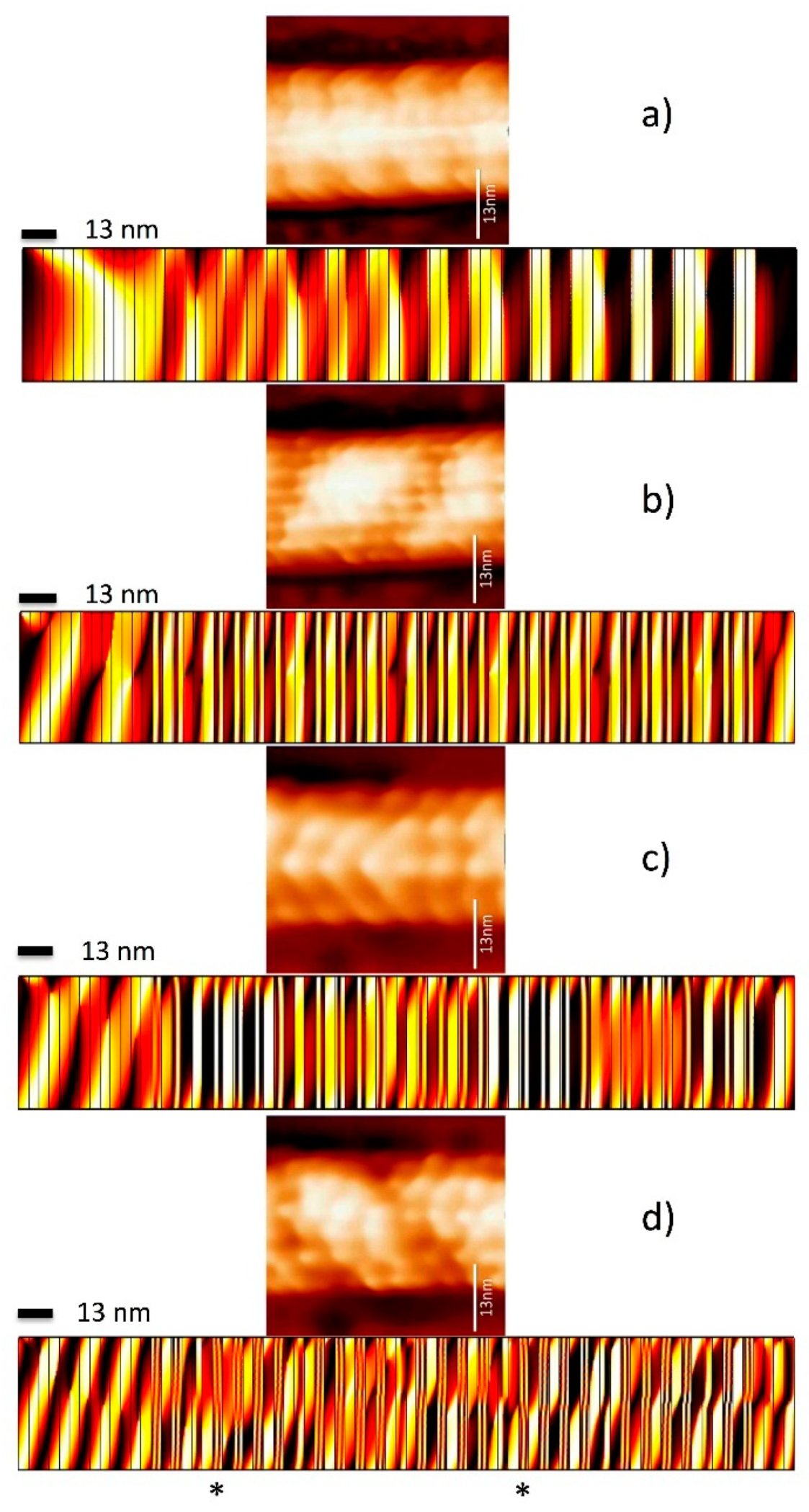
2. Results
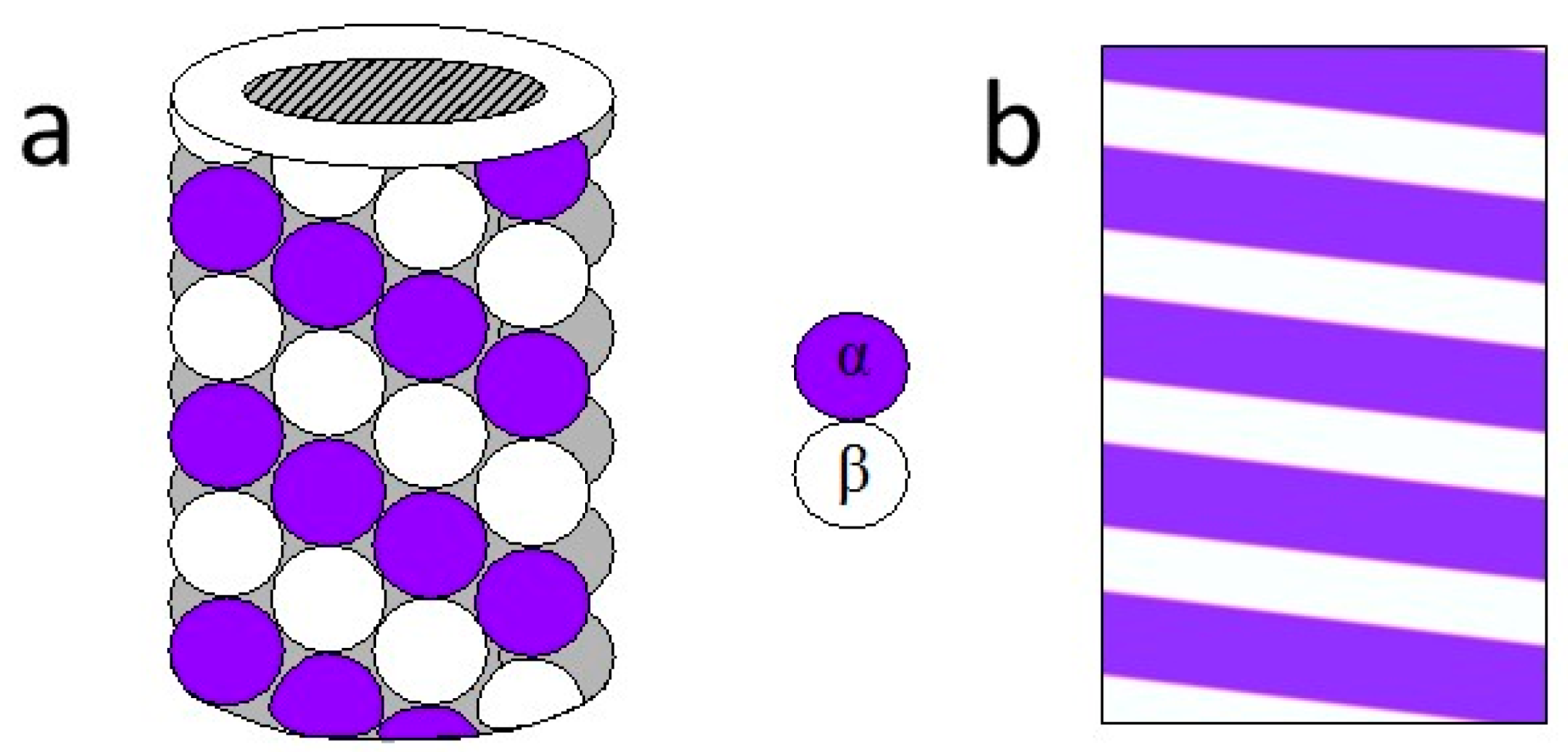
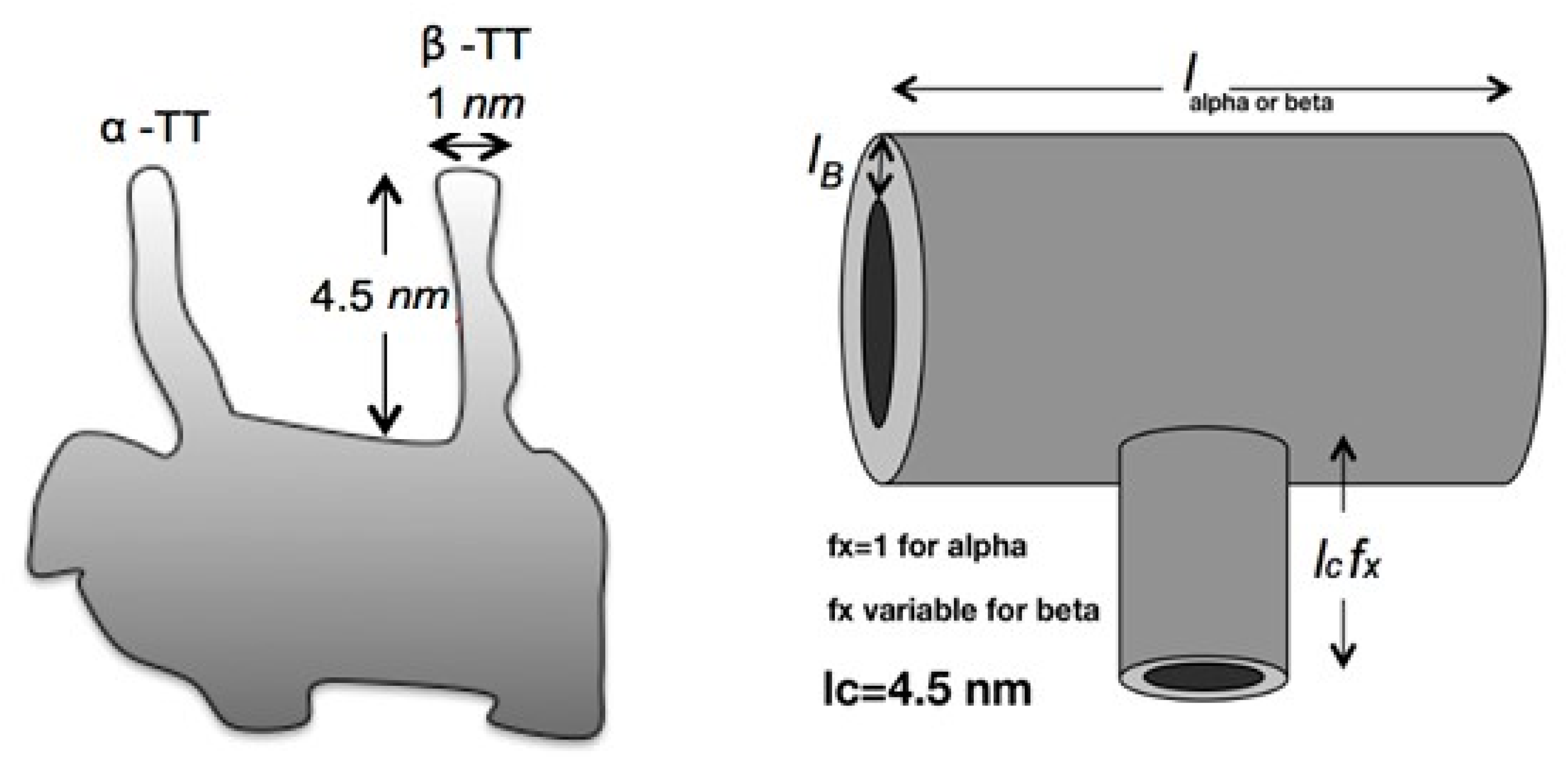
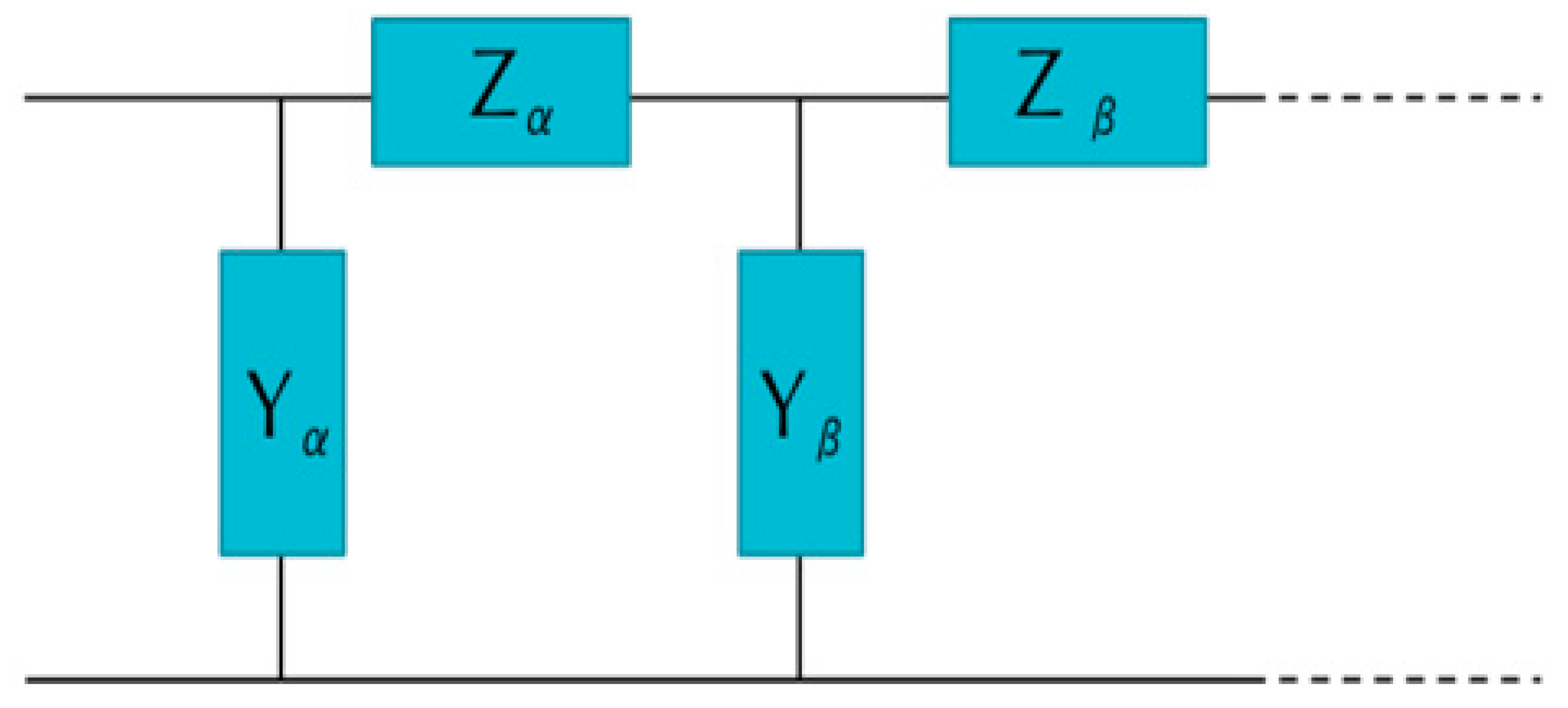
2.1. Crystal Model
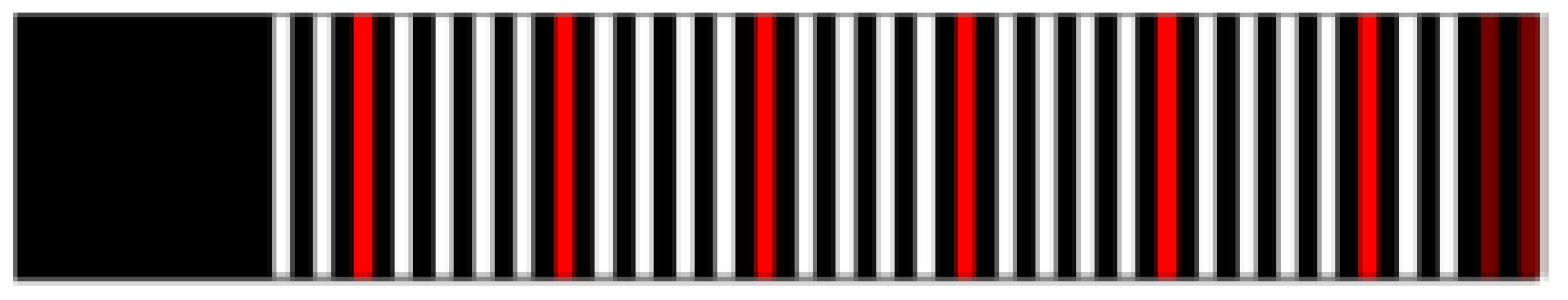
2.2. Finite Element Analysis
3. Discussion
4. Conclusions
5. Materials and Methods
5.1. Microtubules Preparation
5.2. Protein Preparation
5.3. Electrical Transport Measurements
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Byrne, J.H.; Schultz, S.G. Introduction to Membrane Transport and Bioelectricity; Raven Press, University of Delaware: Newark, DE, USA, 1988. [Google Scholar]
- Gilbert, S.F.; Sarkar, S. Embracing complexity: Organicism for the 21st century. Dev. Dyn. 2000, 219, 1–9. [Google Scholar] [CrossRef]
- Lugo, J.E.; Doti, R.; Agarwal, R.; Ray, K.; Faubert, J. Physical Analogies in Biology: From Photons, Phonons, Bloch Waves to Non Linear Oscillators. Adv. Sci. Eng. Med. 2015, 7, 62–75. [Google Scholar] [CrossRef]
- Kittel, C. Crystal Structure. Introduction to Solid State Physics, 8th ed.; John Wiley & Sons: Hoboken, NJ, USA, 2005; pp. 3–6. [Google Scholar]
- Prather, D.W. Photonic Crystals, Theory, Applications and Fabrication; John Wiley & Sons: Hoboken, NJ, USA, 2005. [Google Scholar]
- Hussein, M.I. Reduced Bloch mode expansion for periodic media band structure calculations. In Proceedings of the Royal Society of London A: Mathematical, Physical and Engineering Sciences; The Royal Society: London, UK, 2009; pp. 2825–2848. [Google Scholar]
- Joannopoulos, J.D.; Johnson, S.G.; Winn, J.N.; Meade, R.D. Photonic Crystals Molding the Flow of Light; Princeton University press: Princeton, NJ, USA, 2011. [Google Scholar]
- Vigneron, J.P.; Simonis, P. Natural photonic crystals. Phys. B Condens. Matter 2012, 407, 4032–4036. [Google Scholar] [CrossRef]
- Onslow, H. The Iridescent Colours of Insects. Nature 1920, 106, 181–183. [Google Scholar] [CrossRef][Green Version]
- Zi, J.; Yu, X.; Li, Y.; Hu, X.; Wang, X.; Liu, X.; Fu, R. Coloration strategies in peacock feathers. Proc. Natl. Acad. Sci. USA 2003, 100, 12576–12578. [Google Scholar] [CrossRef]
- Bressloff, P.C.; Cowan, J.D. Thevisualcortexasacrystal. Phys. D Nonlinear Phenom. 2002, 173, 226–258. [Google Scholar] [CrossRef][Green Version]
- Li, D.; Zhao, J. Exact solitary wave and periodic solitary wave solutions for the shallow water wave equation. Chin. Phys. Lett. 2009, 26, 5–8. [Google Scholar]
- Mozziconacci, J.; Sandblad, L.; Wachsmuth, M.; Brunner, D.; Karsenti, E. Tubulin dimers oligomerize before their incorporation into microtubules. PLoS ONE 2008, 3, e3821. [Google Scholar] [CrossRef]
- Chrétien, D.; Fuller, S.D.; Karsenti, E. Structure of growing microtubule ends:Two-dimensional sheets close in to tubes at variable rates. J. Cell Biol. 1995, 129, 1311–1328. [Google Scholar] [CrossRef]
- Georgiev, D.; Papaioanou, S.; Glazebrook, J. Neuronic system inside neurons: Molecular biology and biophysics of neuronal microtubules. Biomed. Rev. 2004, 15, 67–75. [Google Scholar] [CrossRef][Green Version]
- Luchko, T.; Huzil, J.T.; Stepanova, M.; Tuszynski, J. Conformational analysis of the carboxy-terminaltails of human β-tubulin isotypes. Biophys. J. 2008, 94, 1971–1982. [Google Scholar] [CrossRef]
- Feinerman, O.; Segal, M.; Moses, E. Signal propagation along unidimensional neuronal networks. J. Neurophysiol. 2005, 94, 3406–3416. [Google Scholar] [CrossRef] [PubMed]
- Huang, X.; Troy, W.C.; Yang, Q.; Ma, H.; Laing, C.R.; Schiff, S.J.; Wu, J.-Y. Spiral waves in disinhibited mammalian neocortex. J. Neurosci. 2004, 24, 9897–9902. [Google Scholar] [CrossRef]
- Minoura, I.; Muto, E. Dielectric measurement to find individual microtubules using the electro orientation method. Biophys. J. 2006, 90, 3739–3748. [Google Scholar] [CrossRef] [PubMed]
- Ray, K.; Roy, M.K. A theoretical basis for surges of electroencephalogram activity and vivid mental sensation during neardeath experience. Int. J. Eng. Sci. Technol. 2010, 2, 5484. [Google Scholar]
- Sahu, S.; Ghosh, S.; Ghosh, B.; Aswani, K.; Hirata, K.; Fujita, D.; Bandyopadhyay, A. Atomic water channel controlling remarkable properties of a single brain microtubule:correlating single protein to its supramolecular assembly. Biosens. Bioelectron. 2013, 47, 141–148. [Google Scholar] [CrossRef]
- Sahu, S.; Ghosh, S.; Hirata, K.; Fujita, D. A Bandyopadhyay Multi-level memory-switching properties of a single brain microtubule. Appl. Phys. Lett. 2013, 102, 123701. [Google Scholar] [CrossRef]
- Satarić, M.V.; Ilić, D.I.; Ralević, N.; Tuszynski, J.A. A nonlinear model of ionic wave propagation along microtubules. Eur. Biophys. J. 2009, 38, 637–647. [Google Scholar] [CrossRef]
- Ray, K.; Roy, M.K. A theoretical basis for brain waves with implications for a large scale integration required for cognitiveprocesses. In Proceedings of the 9th IEEE International Conference on Cognitive Informatics, Beijing, China, 7–9 July 2010; pp. 436–440. [Google Scholar]
- Llinás, R. The intrinsic electrophysiological properties of mammalian neurons:insights into central nervous system function. Science 1988, 242, 1654–1664. [Google Scholar] [CrossRef]
- Başar, E. Brain oscillations in neuro psychiatric disease. Dialogues. Clin. Neurosci. 2013, 15, 291–300. [Google Scholar]
- Bódizs, R.; Kis, T.; Lázár, A.S.; Havrán, L.; Rigó, P.; Clemens, Z.; Halász, P. Prediction of general mental ability based on neural oscillation measures of sleep. J. Sleep Res. 2005, 14, 285–292. [Google Scholar] [CrossRef]
- Moran, L.V.; Hong, L.E. High vs low frequency neural oscillations in schizophrenia. Schizophr. Bull. 2011, 37, sbr056. [Google Scholar] [CrossRef]
- Komal, S.; Pushpendra, S.; Pathik, S.; Satyajit, S.; Subrata, G.; Kanad, R.; Daisuke, F. DaisukeFujita and Anirban Bandyopadhyay Fractal, scale free electromagnetic resonance of a single brain extracted microtubule nanowire, a single tubulin protein and a single neuron. Fractal Fract. 2020, 4, 11. [Google Scholar] [CrossRef]
- Pushpendra, S.; Subrata, G.; Pathik, S.; Kanad, R.; Fujita, D.; Bandyopadhyay, A. Reducing the Dimension of a Patch-Clamp to the Smallest Physical Limit Using a Coaxial Atom Probe. Prog. Electromagn. Res. B 2020, 89, 29–44. [Google Scholar] [CrossRef]
- Ray, K.; Agarwal, R.; Cacha, L.A.; Poznanski, R.R. Protein Polarization Induced Bloch Waves in Axonal Fibres. In Proceedings of the 11th International Conference on VibrationProblems, Lisbon, Portugal, 9–12 September 2013. [Google Scholar]
- Wang, K.; Rappel, W.-J.; Levine, H. Cooperativity can reduce stochasticity in intracellular calcium dynamics. Phys. Biol. 2004, 1, 27. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Alberts, B.; Bray, D.; Hopkin, K.; Johnson, A.D.; Lewis, J.; Raff, M. Essential Cell Biology: An Introduction to the Molecular Biology of the Cell; Garland Science Publishing: NewYork, NY, USA, 1998. [Google Scholar]
- Yeh, P. Optical Waves in Layered Media; Wiley-Interscience: Hoboken, NJ, USA, 2005. [Google Scholar]
- Dobrowolski, J.A.; Lowe, D. Optical thin film synthesis program based on the use of Fourier transforms. Appl. Opt. 1978, 17, 3039–3050. [Google Scholar] [CrossRef]
- Jackson, D.R. Plane Wave Propagation and Reflection. In The Electrical Engineering Handbook; Chen, W.-K., Ed.; Elsevier Academic Press: Amsterdam, The Netherlands, 2004; pp. 513–524. [Google Scholar]
- Sahu, S.; Ghosh, S.; Fujita, D.; Bandyopadhyay, A. Live visualizations of single isolated tubulin protein self-assembly via tunneling current: Effect of electromagnetic pumping during spontaneous growth of microtubule. Sci. Rep. 2014, 4, 1–9. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sanchez-Castro, N.; Palomino-Ovando, M.A.; Singh, P.; Sahu, S.; Toledo-Solano, M.; Faubert, J.; Lugo, J.E.; Bandyopadhyay, A.; Ray, K. Microtubules as One-Dimensional Crystals: Is Crystal-Like Structure the Key to the Information Processing of Living Systems? Crystals 2021, 11, 318. https://doi.org/10.3390/cryst11030318
Sanchez-Castro N, Palomino-Ovando MA, Singh P, Sahu S, Toledo-Solano M, Faubert J, Lugo JE, Bandyopadhyay A, Ray K. Microtubules as One-Dimensional Crystals: Is Crystal-Like Structure the Key to the Information Processing of Living Systems? Crystals. 2021; 11(3):318. https://doi.org/10.3390/cryst11030318
Chicago/Turabian StyleSanchez-Castro, Noemí, Martha Alicia Palomino-Ovando, Pushpendra Singh, Satyajit Sahu, Miller Toledo-Solano, Jocelyn Faubert, J. Eduardo Lugo, Anirban Bandyopadhyay, and Kanad Ray. 2021. "Microtubules as One-Dimensional Crystals: Is Crystal-Like Structure the Key to the Information Processing of Living Systems?" Crystals 11, no. 3: 318. https://doi.org/10.3390/cryst11030318
APA StyleSanchez-Castro, N., Palomino-Ovando, M. A., Singh, P., Sahu, S., Toledo-Solano, M., Faubert, J., Lugo, J. E., Bandyopadhyay, A., & Ray, K. (2021). Microtubules as One-Dimensional Crystals: Is Crystal-Like Structure the Key to the Information Processing of Living Systems? Crystals, 11(3), 318. https://doi.org/10.3390/cryst11030318







